Artificial selection reveals sex differences in the genetic basis of sexual attractiveness

Significance

Using artificial selection on fruit fly pheromones, we tested how mate choice by both sexes can cause evolutionary change. Results show that although both sexes choose mates, the evolutionary outcomes are fundamentally different for each sex. Specifically, experimental results show that the evolution of pheromones can only be driven through female choice of males because genetic relationships exist between pheromones, mating success, and fitness. Male choice of females, however, cannot drive evolutionary change by itself because the correlation between pheromones and mating success is generated by environmental rather than genetic variation. Therefore, the traits targeted in females by male choice are likely to evolve through correlated responses to selection on males.

Abstract

Mutual mate choice occurs when males and females base mating decisions on shared traits. Despite increased awareness, the extent to which mutual choice drives phenotypic change remains poorly understood. When preferences in both sexes target the same traits, it is unclear how evolution will proceed and whether responses to sexual selection from male choice will match or oppose responses to female choice. Answering this question is challenging, as it requires understanding, genetic relationships between the traits targeted by choice, mating success, and, ultimately, fitness for both sexes. Addressing this, we applied artificial selection to the cuticular hydrocarbons of the fly Drosophila serrata that are targeted by mutual choice and tracked evolutionary changes in males and females alongside changes in mating success. After 10 generations, significant trait evolution occurred in both sexes, but intriguingly there were major sex differences in the associated fitness consequences. Sexually selected trait evolution in males led to a genetically based increase in male mating success. By contrast, although trait evolution also occurred in females, there was no change in mating success. Our results suggest that phenotypic sexual selection on females from male choice is environmentally, rather than genetically, generated. Thus, compared with female choice, male choice is at best a weak driver of signal trait evolution in this species. Instead, the evolution of apparent female ornamentation seems more likely due to a correlated response to sexual selection on males and possibly other forms of natural selection.

Sexual selection through mate choice has long been recognized as an important driver of phenotypic diversity (1, 2). To date the overwhelming majority of studies on mate choice have focused on female preferences for male display traits (2). However, increasing awareness of mate choice by males has stimulated interest in how male choice might also shape phenotypic evolution (3–8). In some species, mutual mate choice occurs, where the mating preferences of males and females target the same phenotypic traits (9). Theory has begun to highlight how mutual mate choice could generate coevolutionary processes that either enhance or constrain diversification depending on the mating system and the sex-specific fitness benefits of choice (7, 9–11). However, from an empirical perspective, we know very little of the potentially complex evolutionary dynamics for traits associated with attractiveness in both sexes (5).

Key to understanding the evolutionary dynamics of mutual mate choice and the evolution of male and female attractiveness is uncovering the genetic relationships between the traits targeted by preferences, mating success, and fitness (12). Work on female preferences has highlighted how choice can improve female fitness either directly, through, for example, signals that indicate a higher likelihood of increased paternal care, or indirectly, via signals that indicate genetic quality, and therefore the likelihood of higher-quality offspring. However, due to the predicted low mating investment costs associated with males, male preferences are thought to target mostly direct benefits, such as mating with more fecund females, since the strength of selection maintaining indirect benefits is generally considered too weak to overcome a “mate with all they meet” strategy (5, 10, 13). In light of these expected differences, even in mutual mate choice systems, the information content of signals targeted by male mate choice might systematically differ from those targeted by female choice (5, 7, 14–16). While there has been increased recognition of female sexual ornaments (3–5, 8), these are rarely as prevalent or exaggerated as those found in males (2, 9). Whether this is due to differences in the strength of selection that results from male and female mate preferences or the underlying genetic architecture of the traits themselves remains unknown (5).

For empirical studies to decipher the complex dynamics that arise in mutual mate choice systems, they must begin to link the within-sex fitness benefits of choice to the between-sex interaction that occurs as a result of the sexes sharing a genome. Broadly, relationships between phenotype, genotype, and fitness can be estimated using classic quantitative genetic approaches by either estimating the phenotypic covariance between trait and fitness (17) and predicting the evolutionary response by pairing it with estimates of genetic variance in the trait (18) or directly estimating the genetic covariance between trait and fitness (19). However, a key limitation of such approaches is the choice of a suitable measure of fitness (20). For studies of sexual selection, mating success is the obvious fitness component of choice, but how mating success covaries with other unmeasured fitness traits and, most importantly, total fitness is notoriously difficult to measure and almost always unknown (20). Many of these hindrances can be overcome using artificial selection, which is a powerful tool for understanding how populations respond to selection (21, 22), and if followed with a period of relaxed selection, can give an insight into the shape of the fitness landscape for the population (23, 24). In studies of sexual attractiveness, the relaxed selection approach can detect opposing natural selection on sexual trait exaggeration in a way that integrates across all fitness-affecting traits while avoiding the pitfall of having to identify the specific traits themselves. These approaches may have much to offer studies of mutual mate choice in the same manner as they have served the study of single-sex attractiveness (25).

Here we use multigenerational artificial selection in a species well-characterized for its mutual mate choice to uncover the relationships between sexually selected traits, mating success, and fitness. Both males and females of the Australian vinegar fly, Drosophila serrata, use blends of contact pheromones, composed of cuticular hydrocarbons (CHCs), as a basis for mate choice. Mutual mate choice generates multivariate sexual selection on the CHCs of males and females which can often differ in its strength and direction (14, 26–31). We tested the evolutionary responses of male and female attractiveness as mediated through these traits by selecting for multiple generations on the multivariate CHC profiles that maximize male and female attractiveness in the founding population (25). The multivariate CHC profiles upon which selection was applied were classified through well-established binomial mate choice trials on the same population from which the selection lines were subsequently taken. The binomial choice trials primarily capture information about mate choice in this system (Methods). Selection was applied on a multivariate index value across three different selection regimes: male-limited (ML), female-limited (FL), and mutual selection (MS). Further, by equalizing the strength of selection for trait combinations that males and females find most attractive for multiple generations, and then following the population-level responses to a period of relaxed selection, we ascertained the presence of other, unmeasured aspects of fitness to infer the relationship between total fitness and mating success for male and female attractiveness traits (23, 24). We then investigated the genetic details of the male and female traits using a biometric approach (32) reliant on trait responses to inbreeding, which point to sex differences in the contributions of rare recessive alleles to genetic variation in the targets of mutual mate choice.

Results

Mutual Mate Choice Generates Sexually Antagonistic Selection.

We first confirmed the presence of mutual mate choice in a long-term laboratory-adapted base population of D. serrata using binomial mate choice trials where the mating success of the focal individual is scored and its CHCs phenotyped. Using ordinary least-squares multiple regression separately for each sex, we estimated the vector of directional selection gradients (17) (β_m and β_f, which are sexual selection on males and females, respectively) and found significant selection driven by mate choice in both sexes (Table 1; males: F_7,395 = 5.20, r² adjusted = 0.069, P < 0.001; females: F_7,389 = 2.87, r² adjusted = 0.033, P = 0.006). Consistent with previous work (30), sexual selection on CHCs differed in strength and direction between sexes (likelihood ratio test: −2 diff lnL = 31.6, df = 7, P < 0.001; see Methods for further details). Selection was much stronger on males than on females (||β_m|| = 0.89; females, ||β_f|| = 0.30) and the direction of sexual selection on individual traits sometimes differed between sexes. For example, the diene 5,9-C_25:2 was disfavored in males but favored in females, whereas the diene 5,9-C_29:2 was favored in males but disfavored in females (Table 1). In a multivariate context, across all eight traits sexual selection was in a net-opposing direction between the sexes (vector correlation between β_m and β_f = −0.43 and angle 115.4°).

Table 1.

Vectors of directional selection gradients along which the artificial selection was applied to male and female CHCs

CHC	βm	βf
5,9-C25:2	−0.11629	0.04863
9-C25:1	−0.06906	−0.16681*
9-C26:1	0.04877	0.12406+
2-Me-C26	0.08924	0.00456
5,9-C27:2	−0.27763*	−0.09912
2-Me-C28	0.01378	0.03046
5,9-C29:2	0.82706***	−0.18864

***P < 0.001, *P < 0.05, ⁺P < 0.1.

Male and Female Signal Traits Respond to Sex-Limited Artificial Selection.

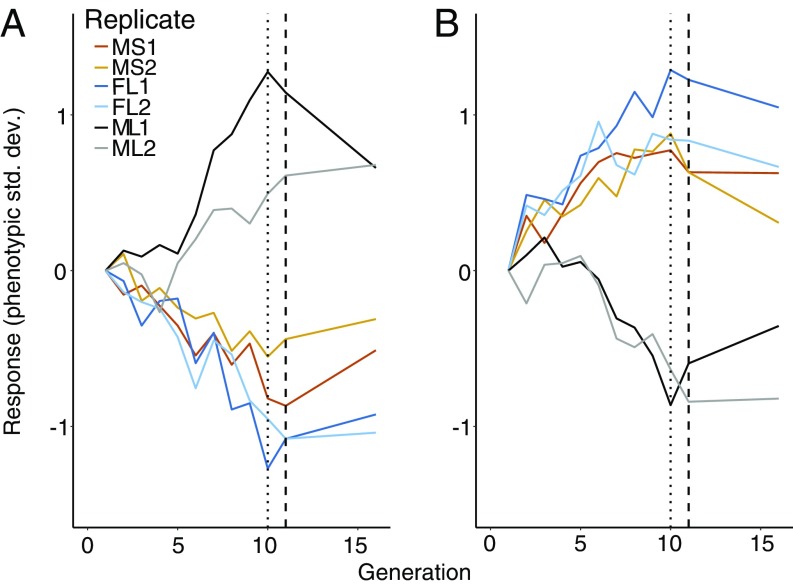
We mimicked the application of sex-limited sexual selection using our estimated mutual sexual selection vectors (β_m or β_f), applying artificial selection to these CHC trait combinations for 10 generations using a family-based design (Methods). In both sex-limited artificial selection treatments, all replicates responded to selection with a significant change in mean trait values between generation 1 and 10 for both sexes (ML treatment β_m Rep 1: F_1,118 = 35.35, P < 0.001, Rep 2: F_1,118 = 6.66, P = 0.011; FL treatment β_f Rep 1: F_1,118 = 66.60, P < 0.001, Rep 2: F_1,118 = 30.22, P < 0.001; Fig. 1). Realized heritability of β_m estimated from the ML treatment was significant in both replicates (Rep 1, t₁ = 12.19, P < 0.001; Rep 2, t₁ = 5.14, P < 0.001), with a mean of 0.212 (Rep 1 h² = 0.294; Rep 2 h² = 0.129). The realized heritability of the selection trait β_f in the FL treatment was also significant for both replicates (Rep 1, t₁ = 17.72, P < 0.001; Rep 2, t₁ = 11.37, P < 0.001), with a mean of 0.448 (Rep 1 h² = 0.440; Rep 2 h² = 0.455).

Fig. 1.

Responses to artificial selection applied along vectors for (A) males, β_m, and (B) females, β_f, for all replicates in each of the three different selection treatments (FL, ML, and MS) with two replicates (1 and 2), corrected by the C treatment means and scaled by the phenotypic SD of the starting population. The dotted line indicates the end of artificial selection at generation 10 and the dashed line indicates the start of relaxed selection.

In both ML and FL selection treatments we also observed a significant and negative correlated response in the sex not under selection (FL treatments β_m, F_1,118 = 56.63, P < 0.001, Rep 2, R_c = −0.131, F_1,117 = 32.05, P < 0.001; ML treatment β_f, Rep 1, F_1,117 = 19.74, P < 0.001, Rep 2, F_1,118 = 12.27, P < 0.001; Fig. 1). Consequently, the realized genetic correlation between β_m and β_f was moderate and negative (male–female comparison: −0.641 female–male −0.849), with the negative estimate expected due to the opposing orientation of β_m and β_f.

Sexually Asymmetric Responses to Mutual Artificial Selection.

For the mutual selection treatment, we found significant changes for both male and female CHCs (β_m Rep 1, F_1,116 = 27.14, P < 0.001, Rep 2, R_c = −0.077, F_1,116 = 12.24, P < 0.001; β_f, Rep 1, F_1,117 = 23.97, P < 0.001, Rep 2, F_1,116 = 229.35, P < 0.001; Fig. 1). However, while the response in β_f was in the same direction in which selection was applied (Fig. 1B), the response in β_m was negative and similar to that seen the FL treatment (Fig. 1A). Therefore, while both male and female attractiveness traits responded to mutual selection, females showed an increase in β_f for both replicates, while for males β_m decreased (Fig. 1). As a result, despite mutual selection on these replicates, the responses to selection were quite similar for the individual traits as seen in the female selection treatment (Fig. 1).

Artificial Selection Boosts Male, but Not Female, Mating Success.

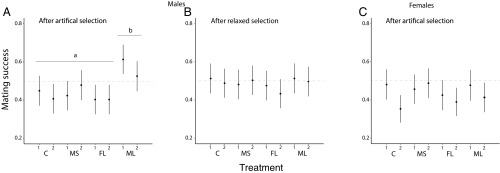
After observing significant CHC trait divergence in both sexes following artificial selection, the next step was to assess whether these trait shifts were accompanied by an increase in mating success, the component of sexual selection that is associated with CHC variation in D. serrata. We found a significant effect of selection treatment on the mating success of males (F_3,4 = 6.75, P = 0.048; Fig. 2). Further investigation revealed this was driven by males from the ML treatment having higher mating success than males from the control (C), FL, and MS treatments (planned contrast F_1,4 = 18.83, P = 0.012).

Fig. 2.

Mating success of males and females. A and B show male mating success after artificial selection was applied (A) and then measured again after five generations of relaxed selection (B). C shows female mating success across the treatments after artificial selection. In A, different letters distinguish significant group differences in post hoc comparisons at P < 0.05. Full results are reported in the text.

By contrast, for female mating success, we found no significant difference between the selection treatments (F_3,4 = 0.61, P = 0.641; Fig. 2). Female flies from the C and FL treatments were rejected in fewer than half the trials (two-sided binomial tests for ≠0.5; C 134 chosen vs. 190 rejected, exact test P = 0.002; FL 130 chosen vs. 190 rejected, exact test P < 0.001), indicating that increasing the combination of traits linked to female attractiveness at the phenotypic level had no detectable effect on female mating success.

Trait Exaggeration Has Fitness Consequences Beyond Mating Success.

After 10 generations of selection, we relaxed selection to determine experimentally whether sexually selected trait exaggeration came at a cost to other components to fitness. Here results were consistent across all treatments; 11 of the 12 comparisons β_m and β_f regressed toward the starting population mean after five generations of relaxed selection (Fig. 1). For females, all six replicates across the three treatments, β_f regressed toward the mean (one-sided binomial test for regression of response, exact test, P = 0.017). For males, β_m shifted toward the starting mean across the three treatments in five of the six replicates (one-sided binomial test for regression of response, exact test, P = 0.1094). Together, this reversal in male and female trait values suggests the possible action of other, unmeasured components of fitness that are antagonistic to β_m and β_f.

Following relaxed selection, we retested mating success of males and found that there was no longer a significant effect of treatment (F_3,4 = 0.69, P = 0.604) and males from the ML treatment were no longer being chosen more often than males from the C, FL, and MS treatment (planned contrast F_1,4 = 0.49, P = 0.522); none of the treatments differed from a mean mating success of 0.5 (two-sided binomial tests for ≠0.5; C 162 chosen vs. 162 rejected, exact test P = 1; FL 148 chosen vs. 178 rejected, exact test P = 0.108; MS 160 chosen vs. 165 rejected, exact test P = 0.824; ML 159 chosen vs. 156 rejected, exact test P = 0.910). This change in male mating success across all treatments further confirms the genetic relationship between β_m and mating success, suggesting that the resulting increase in mating success does not negate the reduction in other unmeasured components of fitness.

Sex Differences in Signal Trait Genetic Architecture.

Despite realized genetic correlations for the CHC traits themselves indicating an appreciable proportion of shared genetic variance, observed sex differences in the heritability of CHC-mediated attractiveness, responses to mutual selection, and changes in fitness suggest that there are also some important differences between the sexes. We tested this using a biometric approach that considers the contribution of rare recessive alleles to genetic variation to be high when the ratio between the change in directional dominance (∆B) of a trait and changes in mean between selected and starting population (∆M) is greater than 1 (32). We estimated ∆B by inbreeding selection lines, via full-sib mating, from the ML, FL, and C treatments at the end of the artificial selection experiment for two generations (Methods). For the two female replicates the ratio ∆B/∆M was negative and significantly smaller than 1, suggesting that the genetic variance in β_f is enriched for intermediate frequency alleles (Rep 1: −0.937, 95% CI −1.585, −0.297; Rep 2: −1.018, 95% CI −2.115, −0.075). For males, the estimate of ∆B/∆M for the two replicates, in keeping with the selection responses, were slightly different for β_m; replicate one ∆B/∆M was greater than 0, although the 95% CIs did not overlap 1 (Rep 1: −0.433, 95% CI −1.435, 0.887), but for replicate two ∆B/∆M was greater than 1 (Rep 2: 1.545, 95% CI −1.013, 12.804). These results, combined with our finding in the MS treatment where β_f responded positively at a cost to β_m, suggest that rare, partially recessive alleles contribute a larger fraction of the genetic variance for β_m than for β_f. This pattern hints at different evolutionary processes maintaining standing variation in males and females.

Discussion

Our experimental results demonstrate convincingly that homologous traits apparently targeted by mutual mate choice in this species have very different genetic relationships with mating success in males and females. For males, the significant selection response increasing β_m values was accompanied by an increase in male mating success in both replicates, and in the replicate with the strongest response both regressed toward their original values once selection was relaxed. This not only suggests there is a significant genetic covariance between β_m and male mating success but also that there is likely to be some form of opposing natural selection affecting these traits (25). By contrast, the significant selection response increasing traits targeted by males in females, β_f, did not increase female mating success, indicating that the significant phenotypic covariance between female mating success and this trait combination in the base population is unlikely to have a genetic basis.

Trait evolution by sexual selection through mate choice requires genetic covariance between the preferred traits and fitness (19). Our artificial selection approach managed to shift the linear combination of female CHCs that experience strongest directional sexual selection in D. serrata, β_f, by ∼1 SD in the female selection treatment (FL), which suggests abundant genetic variance for this trait. However, despite significant heritability and evidence of phenotypic selection there is no genetic covariance between β_f and mating success, meaning that any CHC evolution in females is unlikely to be driven by mate choice, at least as we have measured it here. Thus, selection gradient analysis on CHCs may give the impression that males and females are evaluating mates via what are, in broad terms, very similar traits, but in fact these trait combinations provide quite different information to each sex. To females, a male’s β_m value provides information about his genome, conferring indirect benefits to the female via signals that indicate the genetic quality of the male (33) resulting in a genetic correlation between trait and fitness. However, in females, β_f does not appear be under direct selection by male choice. Regardless of whether the phenotypic correlation between female mating success and CHCs is genetic or environmental, if the CHCs were the primary target of male choice we would expect to see an increase in mating success after experimentally increasing the value of the trait. Intriguingly, we did not see such an effect. One possible reason why this may have occurred is that male choice of CHCs is simply apparent and the true targets of male choice are different traits that elicit environmental associations with CHCs. It has been experimentally established that male D. serrata actively discriminate among female mating partners on the basis of their condition (34). It may be the case that CHCs are environmentally, rather than genetically, correlated with female condition. Thus, the application of artificial selection increased CHC trait values but perhaps not female condition, which could be the true target of male mating preferences. There have been other empirical studies that have demonstrated a phenotypic relationship between a heritable trait and fitness need not always lead to evolutionary change when an unmeasured environmentally correlated variable is the true target of selection (34, 35). Thus, selection on female CHCs in the base population may simply reflect the process of males targeting a different trait such as condition of the female (27, 36) that itself is environmentally correlated with β_f.

Relaxing selection revealed the likely presence of opposing natural selection on CHCs as, in the majority of replicates, β_m regressed back toward the mean of the ancestral population (Fig. 1), with a reduction in male mating success for males from the ML treatment (Fig. 2). Although there is a disagreement in the relaxed selection response between the replicates for β_m in ML (Fig. 1), the strong reversion response in replicate 1 is consistent with a previous study on males of this species that showed a similar pattern of reversion after artificial selection for a similar trait combination (25). A reversion of β_m is, on one hand, easy to understand, since preferred male CHC blends have been shown to have pleiotropic costs with other components of fitness in D. serrata (25, 37) and our experimental results are consistent with this observation. The reversion of β_f after selection was relaxed is a little more difficult to explain in light of the trait’s having no causal genetic relationship with mating success. It may be the case that the reversion of β_f values under relaxed selection is due to indirect opposing natural selection on β_m. There were relatively strong realized genetic correlations between the sexes for these traits and so we would have expected a decrease in β_f for females even if opposing natural selection was only occurring on males. We note that our experimental results suggest it is highly unlikely elevated β_f values regressed due to pleiotropic relationships with other components of fitness, such as condition or fecundity, as these are likely to be environmentally, rather than genetically, correlated with β_f. Thus, the most parsimonious explanation is that the response of β_f under relaxed selection resulted from males’ being pulled back toward their sex-specific fitness optimum, with females showing a correlated response.

The different relationships β_m and β_f have with mating success matches the quantitative genetic architecture estimates for β_m and β_f from our biometric approach. For β_f, a trait that is likely under either weak or no direct sexual selection through male choice, the ratio between ∆B/∆M indicated a greater contribution of intermediate-frequency alleles to the genetic variance, and therefore was able to respond quickly when selection was applied (21). In contrast, our results suggested that the genetic variance in β_m was dominated by more lower-frequency alleles. We observed a slower response at the beginning of selection in the ML replicates (Fig. 1), and the estimated ratio between ∆B/∆M indicated a greater contribution of rare recessive alleles to the genetic variation. This difference also helps to explain the response observed in the MS treatment, where β_f increased while β_m decreased, despite equal selection strength for increased trait expression in both sexes. As β_f is composed of more intermediate-frequency alleles, it is able to respond faster to the artificial selection imposed, with the β_m response being slower due to a greater contribution of rare alleles.

The sex differences we have found in the relationships between sexually selected phenotypes, genotype, and fitness resulting from male and female mate preferences may help to explain why secondary sexual characters in females may not be as prevalent as those found in males. We see clear evidence of causal relationships between male signals, mating success, and nonsexual components to fitness that have likely led to a signal trait with low levels of genetic variation dominated by rare alleles. By contrast, in females, direct genetic sexual selection is weak or nonexistent because the relationship between mating success and trait is likely environmental in origin. Therefore, CHC evolution from female preferences in this population seems to be constrained by opposing natural selection, most likely on males, more so than sexual antagonism driven by sexual selection on both sexes. While our experimental manipulations have made some necessary simplifications of the mating system of this species, our results nonetheless point to important sex differences in the evolutionary consequences of mutual mating decisions. The application of sex-specific artificial selection in other species will hopefully lead to further insights into their generality.

Methods

Mate Choice Assays.

Throughout this experiment we used flies from a laboratory-adapted population founded from 102 inbred lines from the D. serrata Genomic Reference Panel that were established from a single natural population in Brisbane, Australia (38). When the experiment began, the population had been maintained at The University of Queensland for ∼45 generations at a large population size (∼3,000), on a 12-h:12-h light:dark cycle at 25 °C. To determine the linear combinations of CHCs that maximize male and female attractiveness, to which we would subsequently apply artificial selection, we used a standard two-stimulus binomial mating trial (39) that has been used extensively in the D. serrata system for the measurement of mate choice and estimation of sexual selection on males and females (40–42). In each trial we paired two randomly selected 4-d-old virgin competitor individuals of the same sex (one from an orange-eyed inbred stock and the other from the laboratory-adapted stock) with a single 4-d-old virgin individual of the opposite sex, also from the laboratory-adapted stock. Vials were observed continuously until mating occurred. Once mating was observed, from each trial the competing laboratory-adapted stock fly was removed with an aspirator and scored as either chosen (scored as 1) or rejected (scored as 0) and their CHCs were immediately extracted with hexane washes for subsequent phenotyping using established gas chromatography techniques (43). Up to 450 mating trials were performed for each sex and all were performed without the use of CO₂ anesthesia.

Although in this assay mating success can potentially include information about both mate choice and also within-sex competition, D. serrata females have an ability to actively remove unwanted mounted males before intromission (44) and thus there is a component to female behavior that is consistent with choice. There is strong evidence to suggest that the associations between CHCs and mating success measured in our binomial trials are driven by mate choice. Quantitative genetic studies have shown genetic variation can be detected among focal females when analyses are performed using the CHC phenotypes of unrelated successful and unsuccessful males—a pattern that can only be generated by the focal females’ preference genotype and not competition between the unrelated males (45). Further, in a direct experimental demonstration of CHC’s role in mate choice, Chung et al. (46) knocked out a key gene in the D. serrata CHC biosynthetic pathway using a transgenic approach. Transgenic males had altered CHC blends and, in binomial mating trials, had significantly reduced mating success. Critically, mating success of transgenic males was restored to that of the wild-type state through simple CHC perfuming.

For female D. serrata, behavioral observations are not consistent with the existence of any mate competition (44). However, there is evidence of competitive male–male interactions for territory and access to females (47). A recent study found that while it was possible for both male–male competition and female preferences to influence male mating success, they found no evidence for a relationship between male competitive ability and CHCs (47). These studies, taken together, suggest that the binomial choice trials testing for a phenotypic relationship between mating success and CHCs conducted in this species are capturing information primarily about mate choice. Notwithstanding, it remains possible that a low level of within-sex competition exists, and thus our result should be interpreted with this caveat in mind (48).

Estimating Sexual Selection on Male and Female CHCs.

The areas under eight chromatograph peaks of interest (5,9-C_24:2; 5,9-C_25:2; 9-C_25:1; Z-9-C_26:1; 2-Me-C₂₆; 5,9-C_27:2; 2-Me-C₂₈; and 5,9-C_29:2) were integrated and transformed into seven log-contrast values to permit multivariate statistical analyses following Aitchison (49). Following past studies (43, 50), 5,9- C_24:2 was used as the common divisor. After removing multivariate outliers [determined using Mahalanobis distance technique described in Sall et al. (51) and implemented in R] and failed mating trials, we were left with 786 individual CHC phenotypes (396 male and 390 female). We estimated the population-level multivariate selection gradients with traits standardized to have a mean of zero and unitary variance across sexes for mating success using ordinary least-squares multiple regression (17) separately for each sex, giving a vector of selection coefficients (β_m or β_f) along which selection was applied.

Similar to a previous study examining male mate choice (14), the methylalkane 2-Me-C₃₀ was uniformly higher in mated females from the male choice trials, resulting in a disproportionate effect on the variance explained in these choice trials. As in Gosden et al. (14), this is consistent with physical transfer during copulation or physiological changes induced by mating, so this trait was excluded from our analyses for both sexes. To test for differences between the sexes in directional selection, we employed a sequential model-building approach using the same methods outlined in Chenoweth and Blows (52), constructing a model containing only the linear terms of both the quantitative (CHC) and qualitative (sex) variables, compared with the full model which includes the addition of interaction terms between selection gradients and sex for all CHCs, with both models fitted using maximum likelihood (SAS version 9.2; SAS Institute). Testing whether the full model was a better fit than the reduced model is done by comparing the differences between −2 log-likelihood of the two models using a likelihood ratio test (df = 7).

Artificial Selection on Favored Male and Female Trait Combinations.

We applied artificial selection on CHCs in four distinct treatments: (i) antagonistic (MS), males and females were selected along their respective estimated sexual selection vectors; (ii) male-limited (ML), males were selected along the male sexual selection vector and allocated random females from within the treatment; (iii) female-limited (FL), females were selected along the female sexual selection vector, and allocated random males from within the treatment; and (iv) control (C), males and females were randomly allocated into mating pairs.

Each selection treatment was set up using 60 mating pairs randomly taken from the starting population, creating two replicates within each of the four selection treatments (ML, FL, MS, and C). Mating pairs were left for 2 d, before being collected for CHC analysis using techniques described above, and all pupation vials were kept for the next generation. During larval development of the offspring, the parents were phenotyped for their CHCs, integrated and transformed into seven log-contrast values. Pupation vials were then ranked by the scored index value β_m or β_f and truncation selection was applied at 50%, with either two sons or two daughters collected from each of the top-ranked vials to contribute to the next generation. For the C treatment and the unselected sex in the ML and FL selection treatment, two sons or two daughters from a random sample of the pupation vials were used to form 60 mating pairs. In cases where any of the top-ranked males/females produced no offspring, we used the next-highest ranked male/female, ensuring there were 60 mating pairs contributing to each generation. The replicated C treatment was used to correct for the effects of temporal fluctuations, caused by genetic drift, of replicate means of the traits that can occur during selection experiments and allows for a comparison of the behavior of male and female traits under the same experimental conditions but without the application of directional selection. For each generation the average of the control means is subtracted from the generation means of the selection treatment for all traits (53).

Realized Heritability and Genetic Correlations.

Using data from the ML and FL selection treatments we used regression-based approaches for the estimation of the realized heritability, ${\widehat{h}}_r^2$, and the realized genetic correlation between our two index traits. For both the ML and FL selection treatments we used the cumulative selection response, $R_r\left(t\right)={\displaystyle {\sum }_{i=1}^t{R}_i}$ and cumulative selection differential, $S_r\left(t\right)={\displaystyle {\sum }_{i=1}^t{S}_i}$, where $R_i={\overline{z}}_{i+1}-{\overline{z}}_i$ and $S_i={\overline{z}}_i^{\ast }-{\overline{z}}_i$ are the selection differential and single-generation response (respectively) for generation i (54). By estimating the slope of the regression of cumulative response on cumulative selection differential,

$$R_r\left(t\right)=b_r{S}_r\left(t\right)+{\epsilon }_t,$$ [1]

for t = 1,…,10 generations we can estimate the realized heritability as ${\widehat{h}}_r^2=2b_r$, with the multiplier of 2 used as selection was conducted on only one sex. We used the same approach to test for differences between the slopes of males and females in their own selective treatment, modifying Eq. 1 to include the fixed effects of sex and the interaction between sex and the cumulative selection differential, with replicate nested within sex.

We also applied the regression-based approach for the realized genetic correlation, replacing the cumulative response in the index trait under selection, $R_r\left(t\right)$, with the cumulative correlated response in the index trait not under selection, $R_c\left(t\right)$, so that Eq. 1 becomes

$$R_c\left(t\right)=b_c{S}_r\left(t\right)+{\epsilon }_t.$$ [2]

As before, the realized correlated regression coefficient $b_c$ is multiplied by 2 as selection was only applied to one sex. From this we estimated the realized genetic correlation by rearranging from the expectation that

$$b_c=r_G{h}_1{h}_2{\sigma }_2/{\sigma }_1,$$ [3]

where $r_G$ is the realized genetic correlation, $h_1$ and $h_2$ are the square root of the heritabilities of the direct and correlated trait, respectively, with ${\sigma }_1$ and ${\sigma }_2$ their phenotypic SDs (55).

Male and Female Mating Success.

We estimated changes in mating success for all selection treatments. Mating success was measured by competing focal flies from the selection treatment against the same orange-eyed inbred stock used in the estimation of the selection vectors, with a member of the opposite sex from the ancestral line choosing between the two.

Relaxed Selection and Changes to Mating Success.

After 10 generations of artificial selection we relaxed selection for a further five generations and then measured changes in trait means. This approach allows other unmeasured components of fitness to act on the selected traits and can be useful in determining whether there are opposing fitness consequences to trait exaggeration. After selection was stopped, within each replicate in each selection treatment we allowed flies to interact for 2 d before randomly selecting 60 females left singly in a vial for 2 d and repeated this for a further five generations. In the final generation, we retested male mating success and collected the CHCs for 60 males and females set up randomly as mating pairs for 2 d within each treatment using the same protocols described above. Although we measured CHCs for both sexes, we assayed only male mating success due to logistical constraints. We reasoned that the lack of an initial selection response for female mating success made a follow-up analysis of female mating success less informative than an assay of male mating success.

Estimating the Contribution of Rare Recessive Mutations to the Genetic Variance of Male and Female Traits.

The ratio of the covariance of additive effects with homozygous dominance effects (C_AD) to the additive genetic variance (V_A) provides an indication of the contribution of rare recessive alleles to quantitative trait variation (32). In situations where alleles of intermediate frequency dominate, V_A should be greater than C_AD, and when the additive genetic variance is predominately rare recessives C_AD should be greater than V_A. Kelly (32) showed that selection experiments provide a robust means of estimating the relative magnitudes of C_AD and V_A, from the rate of evolution observed through changes in mean. Using the ratio between change in “directional dominance” (∆B) of a trait from inbreeding the selection treatment (difference between the selected and C treatments in directional dominance) and changes in mean between selected and starting population (∆M) gives a robust estimate of C_ad/V_a. Directional dominance is the difference between inbred and outbred means, divided by the inbreeding coefficient, f. To get the estimates of directional dominance, B, we inbred the ML, FL, and C treatment at the end of the artificial selection experiment for two generations of full-sib matings, giving an inbreeding coefficient of f = 0.375 (53). We can then get our estimates from $\mathrm{\Delta }B=({\mu }_I^{\text{'}}-{\mu }_{10}^{\text{'}})/0.375-({\mu }_I-{\mu }_{10})/0.375$ and $\mathrm{\Delta }M={\mu }_{10}^{\text{'}}-{\mu }_1^{\text{'}}$, where µ′ is the population for which selection was applied and µ is the average of the two means from the C treatment. We bootstrapped the selection line data to estimate the 95% CIs.