Ion-triggered selectivity in bacterial sodium channels

Significance

Voltage-gated Na⁺ channels are essential components of the cell membrane in any realm of life. To perform their biological functions, Na⁺ channels need to conduct Na⁺ at high rates, while blocking K⁺. Molecular dynamics simulations showed that the selectivity filter of bacterial Na⁺ channels is a highly flexible structure. This feature favors fast Na⁺ conduction. However, it is still unknown how such highly flexible structure is able to select Na⁺ over K⁺. In this contribution, we show that in the presence of K⁺, the selectivity filter switches to a nonconductive state. The effect of K⁺ on the dynamics of Na⁺ channels explains how these proteins can be contemporarily highly permeable to some ion species and highly selective for similar ones.

Abstract

Since the availability of the first crystal structure of a bacterial Na⁺ channel in 2011, understanding selectivity across this family of membrane proteins has been the subject of intense research efforts. Initially, free energy calculations based on molecular dynamics simulations revealed that although sodium ions can easily permeate the channel with their first hydration shell almost intact, the selectivity filter is too narrow for efficient conduction of hydrated potassium ions. This steric view of selectivity was subsequently questioned by microsecond atomic trajectories, which proved that the selectivity filter appears to the permeating ions as a highly degenerate, liquid-like environment. Although this liquid-like environment looks optimal for rapid conduction of Na⁺, it seems incompatible with efficient discrimination between similar ion species, such as Na⁺ and K⁺, through steric effects. Here extensive molecular dynamics simulations, combined with Markov state model analyses, reveal that at positive membrane potentials, potassium ions trigger a conformational change of the selectivity toward a nonconductive metastable state. It is this transition of the selectivity filter, and not steric effects, that prevents the outward flux of K⁺ at positive membrane potentials. This description of selectivity, triggered by the nature of the permeating ions, might have implications on the current understanding of how ion channels, and in particular bacterial Na⁺ channels, operate at the atomic scale.

Voltage-gated sodium channels are membrane proteins that open in response to depolarization of the cell membrane, and, when open, they preferentially conduct Na⁺ over other monovalent and divalent cations. The bacterial channel NaVAb was the first experimental atomic structure of a voltage-gated Na⁺ channel solved by X-ray crystallography (1). The structures of two other bacterial channels, NaVRh (2) and NaVMs (3), were subsequently obtained, and more recently, in 2017, the structure of the first eukaryotic Na⁺ channel was solved by cryoelectron microscopy (4). Thanks to the availability of these experimental structures, at present, it is possible to investigate the mechanisms of conduction and selectivity in voltage-gated sodium channels at atomistic detail.

Sodium channels share the same fourfold architecture common to voltage-gated K⁺ and Ca²⁺ channels (5, 6), with the region responsible for the selective conduction, the so-called selectivity filter, at the extracellular entrance of the pore (SI Appendix, Fig. S1). Remarkably, the selectivity filter differs between eukaryotic and prokaryotic channels. In eukaryotic channels, selectivity to Na⁺ over K⁺ requires the presence of the conserved signature sequence DEKA, whereas in the prokaryotic homologous the DEKA signature is substituted by a conserved ring of glutamate residues (EEEE). Consequently, also the mechanisms of conduction and selectivity are likely to be different between prokaryotic and eukaryotic sodium channels. The high-density of negative charge facing the lumen of the pore of bacterial Na⁺ channels, in combination with the width of the pore, suggest that more than one cation is likely to bind simultaneously inside the selectivity filter. In agreement with this hypothesis, free energy profiles estimated from molecular dynamics (MD) simulations confirmed that the selectivity filter corresponds to a deep free energy minimum for Na⁺ and that conduction events with only one ion are hampered by high free energy barriers (7, 8). In contrast, when two sodium ions are considered, a low-energy pathway emerges across the selectivity filter. Free energy calculations considering permeation of two K⁺ or mixtures of K⁺ and Na⁺ revealed a remarkable difference between the two ion species: the region of the selectivity filter at the intracellular side of the EEEE ring (between Leu52 and Glu53 in NaVMs), which is a free energy minimum for sodium ions, is a free energy barrier for potassium ions. This small difference in free energy, which is related to the bigger size of K⁺ compared with Na⁺, might easily explain the selectivity for sodium ions. This description of conduction and selectivity was confirmed by extensive MD simulations in the presence of negative membrane potentials (9). The conductance values of NaVAb (10) and NaVMs (9, 11) estimated by MD simulations with negative membrane potentials are in satisfactory agreement with experimental data. The average number of ions occupying the selectivity filter in these trajectories ranges between 1.7 and 2.0, confirming that conduction is likely to involve the presence of two Na⁺ inside the selectivity filter. Moreover, in simulations with K⁺, the conductance of the channel was lower than in simulations with Na⁺, in agreement with the experimental behavior of the channel. The main difference between the two ionic species was ascribed to a region in the middle of the selectivity filter, with much lower density for K⁺ than for Na⁺ (9). This low-density region corresponds to the free energy barrier observed for K⁺ but not Na⁺, at the intracellular side of the EEEE ring in free energy calculations, confirming the hypothesis that selectivity for Na⁺ over K⁺ results from the exclusion of (bigger) potassium ions from the core of the selectivity filter.

The mechanism of Na⁺/K⁺ selectivity described in the previous paragraph, and based on steric effects, was questioned by equilibrium MD simulations of the NaVAb channel in the microsecond timescale (12), where it was shown that residues of the EEEE motif might switch between two states (SI Appendix, Fig. S1), with the side chains directed respectively to the extracellular entrance of the channel (out-facing) or the pore lumen (dunked). The relative probability of these two configurations is strongly correlated with the number of Na⁺ inside the selectivity filter, with higher ion occupancies favoring the dunked state. The same degeneracy of states, and correlation between ion occupancy and structure of the filter, was later observed in free energy calculations where more than two permeating ions and longer MD trajectories were considered (13, 14). Not surprisingly, transitions of the glutamate residues between the out-facing and the dunked states have an effect on the free energy barriers of conduction events and consequently on selectivity. When the degeneracy of states is considered, the difference in free energy between Na⁺ and K⁺/Na⁺ mixtures largely disappears (13). As a consequence of the high mobility of the side chains lining the pore, the selectivity filter appears to the permeating ions as a disordered region. This liquid-like environment looks optimal for efficient conduction of Na⁺. However, it is less obvious how a highly flexible selectivity filter might discriminate between sodium and similar potassium ions.

The ring of glutamate residues of the EEEE motif is located in the region where most of the voltage drop between the extracellular and the intracellular compartment is focused (15–17). Thus, the membrane potential is likely to affect the transitions between the out-facing and the dunked state and, as a result, conduction and selectivity. In agreement with this hypothesis, MD simulations revealed differences between inward (negative membrane potential) and outward (positive membrane potential) conduction. The number of sodium ions inside the selectivity filter is higher in simulations of outward conduction, as well as the probability of the dunked state of residues in the EEEE motif (11). These simulations of outward conduction were performed with membrane potentials close to 500 mV and only in the presence of sodium ions. In contrast, the behavior of the EEEE motif at positive membrane potentials in a physiological range, and in the presence of potassium ions, is still unexplored by MD simulations.

The role of the EEEE motif on conduction and selectivity is investigated in the present study by an extensive set of MD simulations with membrane potentials at ±100 mV in the presence of sodium or potassium ions. The mechanisms of ion selectivity at positive and negative membrane potentials are found to be different. At negative membrane potentials, selectivity to Na⁺ over K⁺ arises from the exclusion of K⁺ from the central region of the selectivity filter, as previously suggested. Instead, at positive membrane potentials, the presence of K⁺ gives rise to a nonconductive metastable state of the selectivity filter, which is absent in analogous simulations with Na⁺. This mechanism of selectivity—where transitions among metastable states are triggered by the nature of the permeating ion—is exploited to discriminate similar ion species, with possible implications on the current understanding of ion channels at the atomic level.

Results

Inward Conduction of Sodium Ions.

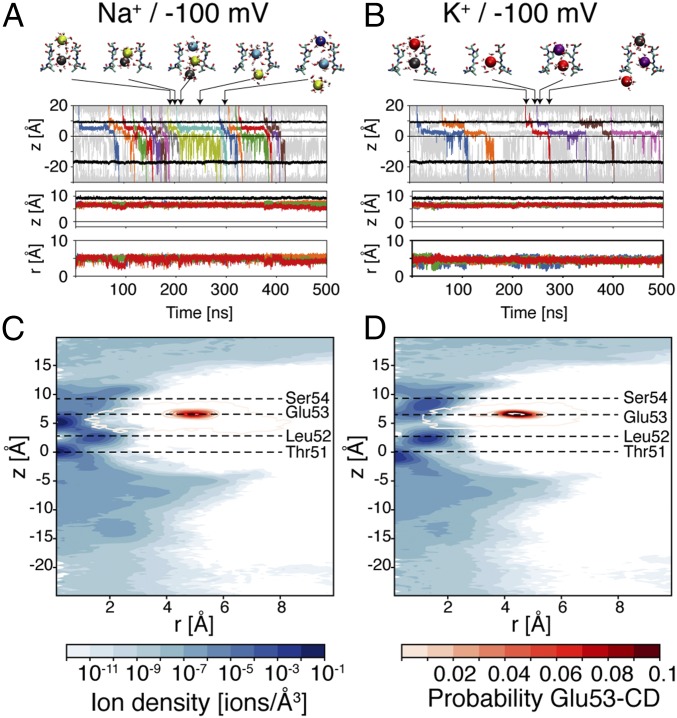
The estimated conductance for the inward flux of Na⁺ in MD simulations with membrane potential at −100 mV is 32 ± 14 pS, in qualitative agreement with experimental data and previous MD simulations (Table 1 and SI Appendix, Table S1) (9). The selectivity filter is most likely occupied by two (57.7%) or one sodium (39.8%) ion (SI Appendix, Table S2). However, complete conduction events might involve the temporary binding of a third ion (Fig. 1 and SI Appendix, Fig. S2). These triple occupancy states are short-lived, and they represent less than 3% of the simulated time. On average, the number of ions interacting with atoms of the selectivity filter is 1.6, distributed among three main binding sites (Fig. 1), in agreement with previous calculations (7, 9). The side chains of Glu53 residues do not deviate significantly from the experimental structure for the entire simulated time (1.5 μs). Rapid movements of Glu53 toward the dunked configuration were observed, but these states were never stable for more than a few nanoseconds. In accordance with this description, the position of the carbon atom of the carboxyl group of Glu53 residues (atom CD; SI Appendix, Fig. S1) exhibits a single density peak, corresponding to the out-facing configuration (Fig. 1).

Table 1.

Channel conductance

Ion	Membrane potential, mV	Length, μs	No. of conduction events	Conductance, pS
Na+	−100	1.5	30	32 ± 14
K+	−100	1.5	16	17 ± 5
Na+	+100	1.8	39	35 ± 6
K+	+100	3.4	50	24 ± 20
	EOUT	2.5	49	31
	EIN	0.9	1	<2

Total length of simulations and number of conduction events are reported separately for simulations with different ion species and membrane potentials. For simulations with K⁺ at +100 mV, the most likely metastable state was calculated for each time step, and the total simulation time and conduction events are reported separately for the two metastable states of the MSM.

Fig. 1.

Conduction with membrane potential at −100 mV. (A and B) Trajectory of ions and Glu53 residues in simulations with Na⁺ and K⁺, respectively. (Top) Ion positions along the axis of the channel (z axis). The trajectories of ions that cross the channel from side to side are shown using lines in different colors. Thin gray lines are used for any other ion in the system that is closer than 12 Å from the channel axis. Other ions are not shown. The black lines show the position of the center of mass of Ala90 residues (intracellular entrance of the channel) and of the side chain oxygens of Ser54 residues (extracellular entrance of the selectivity filter). The zero of the z axis corresponds to the center of the carbonyl oxygen atoms of Thr51 residues. Representative snapshots of the selectivity filter (residues 51–54 of only two opposing subunits in licorice representation), with the permeating ions (VDW representation), and surrounding water molecules (licorice representation) are shown in Top. The colors used for the ions correspond to the ones adopted in the plot. (Middle and Bottom) Trajectories of the carbon atom of the carboxyl group of Glu53 residues respectively along the z axis and in the radial direction. The radial coordinate was defined as the distance on the x–y plane from the center of mass of the carbonyl oxygen atoms of Thr51 residues. (C and D) Distribution of ions and Glu53 residues in simulations with Na⁺ and K⁺ respectively. MD trajectories were sampled with a period of 10 ps. The average positions of the carbonyl oxygen atoms of Thr51 and Leu52, the carbon atoms of the carboxyl group of Glu53, and the side-chain oxygen atoms of Ser54 are highlighted by horizontal dashed lines. The z and the r axes were discretized in bins with side equal to 0.25 Å, and the average number of ions and carbon atom of the carboxyl group of Glu53 (CD atom) residues were computed for each bin. This average number of particles per bin was divided by the volume of the bin to get the densities of ions (blue maps) and of CD atoms (red contour plots).

Inward Conduction of Potassium Ions.

In MD simulations with K⁺ and membrane potential at −100 mV, the conductance is roughly half of the value estimated for sodium ions under the same conditions, being 17 pS with K⁺ versus 32 pS with Na⁺ (Table 1). The ion occupancy of the selectivity filter also differs between K⁺ and Na⁺ (SI Appendix, Table S2). For both ions, the selectivity filter hosts two ions most likely. However, the probability of three-ion states is higher for K⁺ than for Na⁺ (11.9 versus 2.5), as well as the average number of ions inside the selectivity filter (1.9 versus 1.6). The difference between the two ion species emerges clearly at the extracellular side of the selectivity filter. The high-density peak between Leu52 and Glu53 in simulations with Na⁺ disappears in simulations with K⁺ (Fig. 1). The extracellular entrance of the selectivity filter is still an attractive region for K⁺, but ions preferentially bind between Glu53 and Ser54. Despite these differences in ion binding, the dynamics of the selectivity filter is similar in simulations involving both ion species, with side chains of Glu53 residues stable in the out-facing configuration (Fig. 1 and SI Appendix, Fig. S2). This outward facing configuration of Glu53 is critical for the selectivity mechanism that was proposed on the basis of free energy calculations because it creates an energy barrier between Leu52 and Glu53 for K⁺ but not for Na⁺. In agreement with this selectivity mechanism, the ion density has a deep minimum at the intracellular side of the EEEE ring for K⁺ but not for Na⁺ (SI Appendix, Fig. S2). Exclusion of K⁺ from the core of the selectivity filter impairs ion movements across the channel, rendering natural selectivity for Na⁺ over K⁺ for inward fluxes.

Outward Conduction of Sodium Ions.

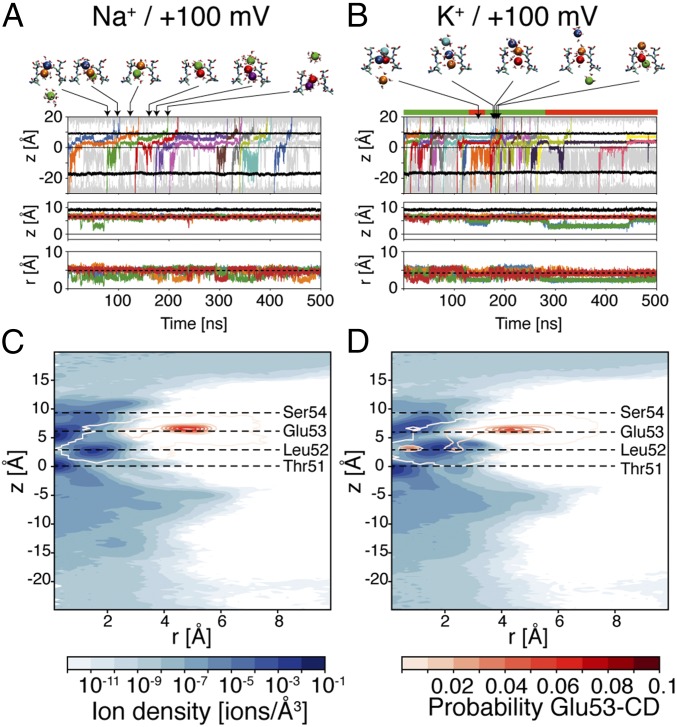
Outward conduction of sodium ions is slightly different from inward conduction, as evidenced by the different number of ions inside the selectivity filter and the dynamic behavior of Glu53 residues. The presence of three ions inside the selectivity filter, which was a rare event in simulations with negative membrane potential, has a probability of 16.7% in simulations with membrane potential at +100 mV (SI Appendix, Table S2). As a consequence, the average number of ions in the selectivity filter increases from 1.6 to 2.0. This increase in ion occupancy is associated with a higher mobility of Glu53 residues. The side chains of Glu53 switch between out-facing and dunked configurations on the same time scale of conduction events (Fig. 2 and SI Appendix, Fig. S3), with the dunked state more likely associated with the binding of three ions inside the selectivity filter. The position of the carbon atom of the carboxyl group of Glu53 residues exhibits a wider distribution compared with simulations with negative membrane potential (compare Fig. 1C with Fig. 2C), which is representative of the degeneracy of states of the selectivity filter. Despite this different behavior of the selectivity filter between positive and negative membrane potentials, the position of the binding sites for sodium ions is almost unaffected, and the estimated conductance of the channel is also close to the value calculated at −100 mV (Table 1). Therefore, although the mechanisms of conduction exhibit some minor differences, the NaVMs channel conducts Na⁺ at similar rates in inward and outward directions.

Fig. 2.

Conduction with membrane potential at +100 mV. Same legend as Fig. 1. The dashed lines in Middle and Bottom (A and B) show the average position of the carbon atom of the carboxyl group of Glu53 residues along the axial and radial directions, respectively, in simulations with the same ion species and membrane potential at −100 mV. The color bar above B shows the most likely metastable state of the MSM; green corresponds to E_OUT, and red corresponds to E_IN.

Outward Conduction of Potassium Ions.

In simulations with K⁺ and membrane potential at +100 mV, the rate of permeation showed high variability among different trajectories (SI Appendix, Table S1). Therefore, to better explore the causes responsible for this variability, a higher number of independent trajectories was simulated for outward conduction of K⁺ than for the other tested conditions. The average conductance, as estimated from eight independent MD simulations and a cumulative simulation time of 3.4 μs, is 24 pS (Table 1). This value is ∼30% lower than the conductance estimated for Na⁺ under the same conditions. However, whereas in simulations with sodium ions the value of the conductance is relatively stable among independent simulations (24–40 pS), in simulations with potassium ions the conductance ranges from 3 pS (1 conduction event in a 500-ns trajectory) to 61 pS (19 conduction events in a 500-ns trajectory). The average ion occupancy of the selectivity filter is greater in simulations with K⁺ than in simulations with Na⁺ (SI Appendix, Table S2). In the most likely configuration, the selectivity filter is occupied by three ions (46.9%), but states with four ions are also observed with significant probability (6.9%). Moreover, the behavior of Glu53 residues is also different from the one observed in any other tested condition. The dunked state of Glu53 has higher probability than in simulations with Na⁺, and these states are stable for hundreds of nanoseconds (Fig. 2 and SI Appendix, Fig. S4). The differences between K⁺ and Na⁺ observed in simulations with a nonpolarizable force field (all-atom additive force field CHARMM36) were confirmed by two independent set of simulations with the Drude polarizable force field (SI Appendix, Fig. S5).

Visual inspection of the trajectories in simulations with K⁺ and positive membrane potential immediately suggests a link between the rate of ion conduction and the configuration of Glu53 residues. For instance, in the trajectory shown in Fig. 2B, all of the conduction events take place when the carbon atoms of the carboxyl group of Glu53 residues are above z = 5 Å. In contrast, long-lived states with these atoms below this threshold correspond to nonconducting periods of the channel. A similar behavior is observed in the other trajectories (SI Appendix, Fig. S4). Therefore, a possible explanation for the high variability of the estimated conductance in this set of MD simulations is that the selectivity filter exists in separate metastable states with different functional properties. This hypothesis is investigated in the next section.

Metastable States of the Selectivity Filter.

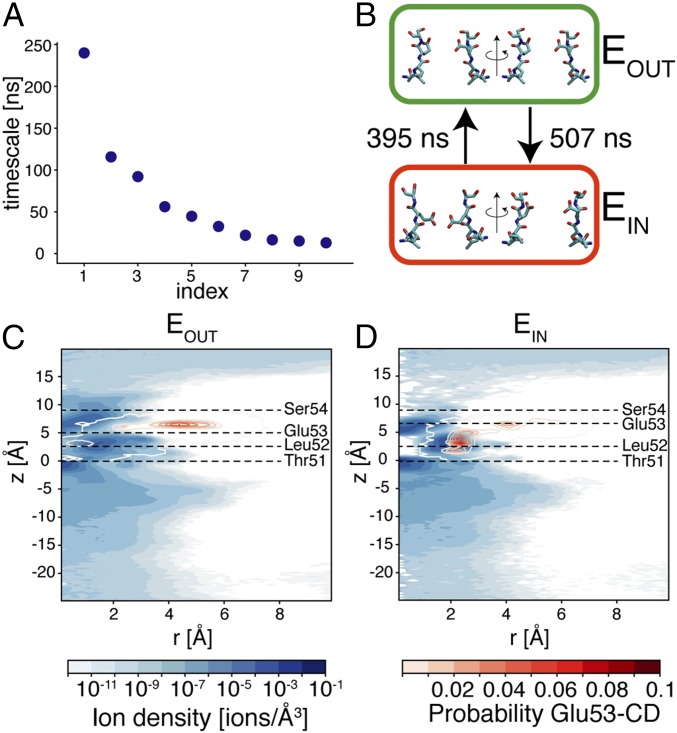
Use of Markov state models (MSM) is a possible strategy to identify metastable states in MD simulations (18, 19). The MD trajectories with K⁺ and positive membrane potential were converted into sequences of discrete microstates using the configuration of the Glu53 residues. The absence of significant memory effects, necessary for an accurate estimate of the MSM, was tested by comparing the relaxation times calculated with increasing sampling periods (20). The relaxation times were marginally affected by the sampling period for time intervals above 10 ns (SI Appendix, Fig. S6A), which was consequently used as lag time for estimating the MSM. The ordered sequence of relaxation times computed from the transition matrix of the MSM exhibits a clear gap between the first and the second relaxation time (Fig. 3A). Therefore, a hidden Markov state model with two states was estimated using the discretized MD trajectories (21).

Fig. 3.

Markov state model of the selectivity filter. (A) Estimated relaxation times of the MSM. (B) Representative configurations of the selectivity filter in metastable states E_IN (red box) and E_OUT (green box). Two alternative views of residues 51–54 (rotated by 90° around the axis of the channel) are shown for two opposite subunits. Mean first passage times between the two metastable states are provided. (C and D) Distribution of ions (blue maps) and of carbon atom of the carboxyl group of Glu53 residues (red contour plots) in metastable states E_OUT and E_IN, respectively, calculated as described in the legend of Fig. 1.

The hidden states of the MSM correspond to different metastable states of the selectivity filter, which were named E_OUT and E_IN, respectively, in agreement with the most likely configuration of the glutamate residues of the EEEE ring. In state E_OUT, Glu53 residues are preferentially directed toward the extracellular entrance of the channel (Fig. 3 B and C), but excursions of one glutamate residue to the dunked state are frequently observed (SI Appendix, Fig. S6B). This configuration of the selectivity filter is most likely occupied by two or three ions, with probability 54.9% and 38.1%, respectively (SI Appendix, Table S2), and the binding sites are remarkably similar to the ones observed at negative membrane potential (compare Fig. 3C with Fig. 1D). Instead, in metastable state E_IN, the distribution of ions inside the selectivity filter differs from the one observed at negative membrane potential, or with sodium ions (Fig. 3D). The selectivity filter is mainly occupied by three ions (68.4%), and the probability of four ions interacting with the filter is 26.1%. The major difference between ion distributions in the two metastable states emerges at the center of the selectivity filter, which in state E_IN is completely depleted from K⁺. This noncanonical distribution of ions is related to a structural change of Glu53 residues. In metastable state E_IN, the most likely configuration of the selectivity filter has two glutamate residues protruding toward the axis of the channel (SI Appendix, Fig. S6B). These configurations of Glu53 residues prevent the binding of ions at the center of the selectivity filter. Ions, instead, accumulate in an off-axis position, where they directly interact with the carboxyl oxygen atoms of the glutamate residues (SI Appendix, Fig. S6C).

The different structures and ion occupancies of the selectivity filter in the two metastable states suggest an effect on the conduction properties. Indeed, the vast majority of the outward conduction events of K⁺ occur when E_OUT is the most likely metastable state of the channel. In detail, E_OUT is the most likely metastable state for ∼2.5 μs, and during this period of time, 49 conduction events are observed, whereas a single conduction event is observed during the 0.9 μs when E_IN is the most likely metastable state (Table 1). Therefore, an approximate estimate of the channel conductance in two metastable states is 31 pS for E_OUT and less than 2 pS for E_IN. The conductance of state E_OUT is remarkably similar to the value estimated in analogous simulations with Na⁺ (35 ps). This lack of selectivity is the natural consequence of the high mobility of Glu53 residues in simulations with positive membrane potential. Indeed, it is important to remark that in state E_OUT, the out-facing configuration of residues Glu53 is more likely than in state E_IN, but Glu53 residues are not confined to this out-facing configuration; instead, they are free to sample several different microscopic states (SI Appendix, Fig. S6B). In agreement, the distribution of the carbon atom of the carboxyl group of Glu53 residues in state E_OUT closely resembles the analogous distribution in simulations with Na⁺ and positive membrane potential (Fig. 2C). The side chains of Glu53 residues rapidly interconvert among different states, and this liquid-like environment is not suited for selecting between two ionic species with identical charge and similar radius, as K⁺ and Na⁺ are. Therefore, the lack of selectivity of this degenerate state is not surprising. The apparent contradiction between a liquid-like environment and the ability of the selectivity filter to discriminate similar ionic species is resolved by conformational transitions to the metastable state E_IN. In state E_IN, the mobility of Glu53 residues is much lower than in state E_OUT. The side chains of Glu53 residues in two opposing subunits are blocked in an inward configuration, where they prevent the passage of ions. This locked state is stable for hundreds of nanoseconds. Similar transitions of the selectivity filter were not observed in simulations with sodium ions. Although it is not possible to exclude that similar states could emerge also in the presence of sodium ions, the consistent behavior observed among Na⁺ simulations and the different ion occupancy of the selectivity filter between the two ion species, also confirmed by simulations with an alternative force field, seem to suggest that Na⁺ and K⁺ can actually interfere with the dynamics of the selectivity filter. In the presence of sodium ions, a unique—conductive—stable state of the selectivity filter is observed. In contrast, in the presence of potassium ions, an alternative—nonconductive—metastable state of the selectivity filter emerges. Switching between the conductive (E_OUT) and the nonconductive (E_IN) metastable states (Fig. 3B) could reduce the permeability of K⁺, making the channel selective for Na⁺.

Discussion

At present, the overall consensus about conduction and selectivity in bacterial Na⁺ channels extracted from MD simulations is primarily focused on two atomic models that are, at least apparently, at conflict with each other. The first atomic model entails a stable selectivity filter, where the glutamate residues of the EEEE motif do not deviate significantly from the experimental structure. In this model, selectivity for Na⁺ over K⁺ arises from steric effects (7, 8). In the second atomic model, the glutamate residues of the EEEE motif rapidly switch among different configurations (12). As a result of this degeneracy of states, the selectivity filter mimics a liquid-like environment for the permeating sodium ions. This liquid-like environment guarantees high sodium permeability. However, it is currently unknown how such a flexible selectivity filter might discriminate Na⁺ over K⁺. In this study, permeation in bacterial Na⁺ channels was investigated by a set of MD simulations with different ion species and physiological membrane potentials (±100 mV). The MD trajectories confirmed that both the hypothesized behaviors of the selectivity filter, either rigid or flexible, are possible. At negative membrane potentials, the selectivity filter does not deviate considerably from the experimental structure, and the lower permeability to K⁺ over Na⁺ might easily be explained by steric effects. Instead, at positive membrane potentials, the glutamate residues of the EEEE motif move between different configurations. The high mobility of the selectivity filter has marginal effects on the permeability of sodium ions, which is similar for negative and positive membrane potentials. In contrast, the presence of potassium ions stabilizes alternative structures of the selectivity filter with remarkably low conduction rates. These transitions between different metastable states of the selectivity filter, rather than steric effects, are the main determinants of selectivity for Na⁺ over K⁺ at positive membrane potentials.

The presence of alternative mechanisms of selectivity for inward and outward ion fluxes is in qualitative agreement with electrophysiological experiments, which demonstrated that the selectivity of bacterial Na⁺ channels depends on the direction of the concentration gradients (22). Despite this qualitative agreement, the quantitative comparison between MD simulations and experimental data are hampered by several issues. First, in electrophysiological experiments, the selectivity for Na⁺ over K⁺ is usually estimated by measuring the reversal potential with the channel exposed to different concentration gradients for the two ion species. Instead, in MD simulations, selectivity was analyzed by comparing Na⁺ and K⁺ trajectories at the same membrane potential and without concentration gradients. The transitions between metastable states observed in trajectories with K⁺ might be the result of a direct effect of the positive membrane potential on the glutamate residues or of ion movements in the outward direction. The second hypothesis is supported by steered MD simulations, which showed that the selectivity filter of bacterial Na⁺ channels behaves asymmetrically for inward and outward fluxes, even at null membrane potential (23). The quantitative comparison with experimental data requires a throughout understanding of the individual contributions of the direction of ion fluxes and of the membrane potential to selectivity, and this is not possible with the simulation protocol employed in the present study. Algorithms to mimic concentration gradients in MD simulations have been proposed (24, 25). However, to estimate reversal potentials, which could then be directly compared with experimental data, simulations of ion fluxes under different electrochemical gradients, some of them close to equilibrium, are required. The high computational cost of MD simulations makes the estimate of reversal potentials in voltage-gated channels a daunting task. Consequently, MD simulations at constant ion concentrations were performed.

The second issue that might hamper a quantitative comparison between simulations and experiments is the accuracy of the force fields adopted for MD simulations of biomolecules. The Na⁺/K⁺ permeability ratio critically depends on two factors: (i) the relative permeability of the two ion species when the glutamate residues of the EEEE motif are in the out-facing configuration and (ii) the probabilities of the different metastable states of the selectivity filter. At present, it is uncertain if classical force fields can render accurate estimates of these relative probabilities. The variability among simulations of bacterial Na⁺ channels with different force fields well represents the current situation. For instance, the Na⁺/K⁺ permeability ratio at −100 mV is close to 10 when ion parameters by Joung et al. are adopted (9), whereas a lower ratio was estimated here, using the ion parameters by Beglov and Roux (26). Despite these quantitative differences, it is important to remark that the picture emerging from independent MD simulations is coherent. Analogous binding sites have been identified, regardless of the simulation method and the adopted force field, and these binding sites are in good agreement with experimental data (7, 8). The number of ions inside the selectivity filter and the differences between inward and outward permeation pathways for Na⁺ coincide in several computational studies (10, 14). Moreover, the sodium conductance estimated from MD simulations is conserved in different force fields, and it is in agreement with experimental data (9–11). Although current simulation protocols and the classical force fields might not be good enough for a quantitative comparison with experimental data about selectivity, the agreement with experiments for sodium ions, together with the coherent picture emerging from simulations using different force fields, strongly supports the hypothesis that the mechanisms of conduction and selectivity extracted from these computational studies are indeed representative of how ion channels operate at atomic resolution.

The mechanism of selectivity observed in simulations with positive membrane potential is intrinsically different from the ones previously proposed for bacterial Na⁺ channels or even other ion channels. Previous mechanisms of selectivity proposed on the bases of MD simulations can be classified into two main categories, here referred to as local selectivity and multiion selectivity for clarity. The term local selectivity is used when selectivity can be accounted for by the presence of a specific region along the pore that favors some particular ionic species. The selectivity for Na⁺ over K⁺ for inward fluxes at negative membrane potentials in bacterial Na⁺ channels is an example of local selectivity. Sodium ions are favored over potassium ions because they better fit in the region at the intracellular side of the EEEE ring. In other ion channels, it is not possible to explain selectivity by the presence of (local) selective binding sites. For instance, the selectivity filter of K⁺ channels exhibits a sequence of binding sites selective for K⁺, alternated by a sequence of binding sites selective for Na⁺ (27). Selectivity depends on the multiion conduction mechanism: single files of K⁺ are energetically favored over single files of ion mixtures (Na⁺/K⁺) (28, 29). Therefore, in K⁺ channels, selectivity cannot be ascribed to any specific position along the pore, because it emerges as a global property of the channel due to the multiion conduction mechanism. The mechanism of selectivity proposed here for outward ion fluxes in bacterial Na⁺ channels is neither a local property nor due to multiion conduction. Instead, it is caused by the effect of the permeating ions on the dynamics of the selectivity filter, and consequently, it will be referred to as ion-triggered selectivity. This concept of ion-triggered selectivity has been proposed before to describe how the selectivity filter of ion channels reacts to the permeating ion species. For instance, the selectivity filter of some K⁺ channels collapses in the presence of sodium ions, and this might add a second layer of selectivity over the one created by the multiion conduction mechanism described above (30). In addition, an effect of the permeating ion species on the dynamics of ion channels has been observed in previous MD simulations of other ion channels and also related to selectivity (31–33). However, here, selectivity is directly related to a transition of the selectivity filter to a different metastable state induced by a particular permeating ion species. In this respect, ion-triggered selectivity could easily explain why an ion channel might appear as a liquid-like, highly conductive environment to some ion species, while at the same time blocking other ion species with high efficiency. Because selectivity is the fingerprint of ion channels, ion-triggered selectivity might be a common mechanism, playing an important role in the function of this class of membrane proteins.

Methods

MD simulations were performed using the molecular dynamics code NAMD, version 2.11 (34). The simulation systems consisted of the pore domain of NaVMs (Protein Data Bank code: 3ZJZ) that was aligned along the z axis and embedded in a POPC lipid membrane. The CHARMM36 force field for protein and lipids was used (26, 35–39), and water molecules were described with the TIP3P model (40). The set of simulations included several independent trajectories in the NvT ensemble with different ion species, NaCl or KCl, and membrane potentials, ±100 mV, as listed in SI Appendix, Table S1. To check for the dependency of the results on the force field, a set of simulations with the Drude polarizable force field was also performed (41–43). The membrane potential was mimicked by a constant electric field acting along the z axis. The python library, PyEMMA, was used to estimate the MSM (44), using as discrete features the coordinates of the carbon atoms of the carboxyl group of Glu53 residues. For additional details about simulation and analyses protocols, see SI Appendix.