Photobiomodulation Optimization for Spinal Cord Injury Rat Phantom Model

Abstract

Spinal Cord Injury (SCI) causes interruption along the severed axonal tract(s) resulting in complete or partial loss of sensation and motor function. SCI can cause tetraplegia or paraplegia. Both these conditions can have lifelong excessive medical costs, as well as can reduce life expectancy. Preclinical research showed that Photobiomodulation therapy (PBMT), also known as Low-level laser (light) therapy (LLLT), possesses reparative and regenerative capabilities that have the potential to be used as a complimentary or supplementary SCI therapy. Despite the promising effects of PBMT, there are still no standardized irradiation parameters (i.e. different wavelengths, power, fluence, irradiance, beam type, beam diameters, and irradiation time) and there is also a lack of standardized experimental protocol(s), which makes it difficult to compare different studies. It is, nonetheless, essential to standardize such irradiation parameters in order to provide better PBMTs. The aim of this study, therefore, is to evaluate the delivery of light in a 3D voxelated SCI rat model for PBMT using different irradiation parameters (wavelengths: 660, 810, and 980 nm; beam types: Gaussian and Flat beam; and beam diameters: 0.04-1.2 cm) using Monte Carlo simulation. This study also aids in providing standardization for preclinical research for PBMT, which will eventually translate into clinical standardization upon clinical research studies and results.

Introduction

Spinal cord injury (SCI) can result in complete or partial loss of sensation and motor function due to interruption along the severed axonal tract(s). SCI can result in tetraplegia or paraplegia, which can have prohibitive lifetime medical costs and can result in shorter life expectancy. Several studies have investigated the treatment of SCI in animal models [1,2,3,4,5,6,7]; however, there still remains no consensus on the most effective treatment parameters for animals and humans.

Photobiomodulation therapy (PBMT), also known as Low-level laser (light) therapy (LLLT), has been clinically applied for treatment of rheumatoid arthritis, pain management, wound healing, and various neurological diseases such as stroke, neurodegenerative diseases and brain injury [8]. Preclinical studies have also shown that PBMT has reparative and regenerative capabilities on transected spinal cords and that PBMT can enhance axonal sprouting in animal models [9,10,11,12,13,14,15]. Recently, several researchers used a contusion SCI rat model and applied laser or LED transcutaneously with different irradiation parameters and treatment periods to investigate the efficacy of LLLT. Table 1 summarizes the LLLT studies [12,13,14,15] on a contusion SCI rat model irradiation parameters and the associated treatment outcomes. Despite PBMT showing promising results as a treatment for SCI, it remains difficult to compare published results due to the use of a wide range of irradiation parameters (i.e., different wavelengths, irradiances, beam types, beam diameters, and irradiation times), and due to the lack of a standardized experimental protocol(s) [16]. More recently, emphasis has been placed on the requirement to report irradiation parameters used in any study. Moreover, it has been proposed that the following beam parameters be reported in any PBMT study: wavelength, power, irradiance, irradiation time, beam area, pulse parameters, anatomical location (i.e., depth below skin), number of treatment, and interval between treatments. As proposed by Hadis et al. [16], these parameters are a minimum requirement for repeatable scientific studies. In many other publications, the significance of describing light parameters and treatment protocols has also been stressed upon [17,18,19,20]. A new approach that can accurately and reliably optimize the irradiation parameters for PBMT therapy for both research and clinical practice is, therefore, needed.

Table 1.

LLLT studies on a contusion SCI rat model irradiation parameters and the associated treatment outcomes [12,13,14,15].

		Source	Power (mW)	Beam Area (cm2)	Irradiation time (s/day)	Fluence (J/ cm2)	Treatment period (day)	Treatment outcome
Anders et al. [12]		810 nm Laser	150	0.295	2997	1589	14	Enhanced axonal regeneration and functional recovery.
Paula et al. [13]		780 nm Laser	44	0.196	28 s ×5 points = 140	6 ×5points = 30	14	Promoted motor recovery, preservation of the nerve tissue in the lesion area, and increased the number of cells and connections.
Veronez et al. [14]		808 nm Laser	30	0.028	141-282	150-300	7	Higher fluence of PBMT improved functional performance and tactile sensitivity and resulted in a reduction in the lesion volume and markers of inflammation.
Giacci et al. [15]	A	670 nm LED	3750	70	530	28.4	14	No significant improvement in function, performance and tactile sensitivity, and reduction in the size of the lesion. They attributed these results to the low fluence used.
	B	830 nm LED	3750	70	430	22.6	14

Monte Carlo (MC) simulation is commonly employed to simulate light propagation in tissues and has improved significantly since the field of laser-tissue interactions was first introduced [21]. MC simulation offers a reliable, precise and flexible method to compare and optimize instrument design and experimental design [22,23,24,25]. More recently, Segars et al. [26] developed a whole-body rat model called ROBY, which can provide an extremely accurate anatomical structure of a whole-rat body that can be employed with MC simulation.

Using MC simulation and ROBY, we evaluated the light fluence distribution in the SCI rat model for different wavelengths, beam types, and beam diameters. The selection of wavelengths, beam types and beam diameters was based on a frequently used experimental setup (i.e., wavelengths: 660, 810, 980 nm; beam types: Gaussian and Flat beam; beam diameters: 0.04-1.2 cm). To our knowledge, this research study is the first to utilize an MC simulation along with a ROBY SCI rat model to help quantitatively optimize the irradiation parameters for PBMT.

Methods

Spinal Cord Injury (SCI) rat model

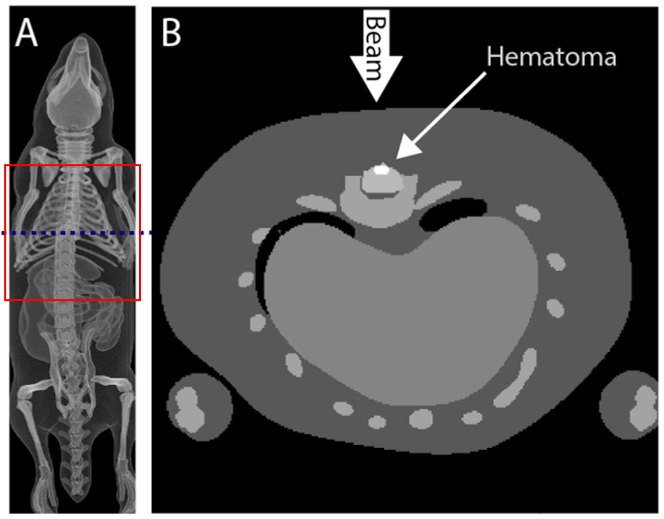
The study uses ROBY [26], a 3D anatomical model of a rat, which was developed by segmentation and 3D reconstruction of rat MRI images obtained on non-uniform rational B-spline. The rat model comprises of a realistic description of the organs and skeletal system of the rat and allows for flexible manipulation of the animal organ(s) using a set of control points. The ROBY model could scale one or more selected organ(s) as well as the entire rat. In this study, the generated dimension of the rat model was 58 mm (laterally)×43 mm (anteroposterior)×225 mm (length). The resulting 3D rat model was 300×300×1500 voxels with a resolution of 0.2×0.2×0.2 mm³ as shown in Figure 1A. The modification of the model was done manually by removing T10 spinous process and implanting a hematoma in the spinal cord using MATLAB (The MathWorks, Inc., Natick, Massachusetts, USA). The implanted hematoma was presumed as an ellipsoid-shaped object that had a volume of 2 mm³ [27]. In order to balance the computational time and precision, only the region of interest was used in the simulation. Therefore, the 3D rat model employed in the simulation has a dimension of 300×300×300 voxels, which comprises the indices of the different rat tissue types, such as skin, muscle, soft tissue, spinal cord, bone and blood. The indices were used to assign tissue-specific optical properties for every voxel.

Figure 1.

(A) 3D visualization of the representative spinal cord injury (SCI) rat model. Only the torso of the rat (red box) was used in the MC simulation. (B) Cross-section of the SCI rat model (from blue dash line in A). The T10 spinous process was removed and an ellipsoid-shaped hematoma, with a volume of 2 mm³, was embedded in the spinal cord.

Monte Carlo Simulation

A Graphics Processing Unit (GPU) based Monte Carlo (MC) light propagation simulation was performed using Monte Carlo eXtreme (MCX) developed by Fang [23]. The optical properties of the skin, muscle, bone, soft tissue, spinal cord and blood (SpO₂>98%) at 660, 810, and 980 nm were used in this study (Table 2). These properties were derived from previous studies [28,29,30,31]. The refractive index (n) for all the tissues was assumed to be 1.37. The anisotropy (g) for the tissues was assumed to be 0.90, except for the blood. In total, 60 irradiation parameters (3 wavelengths: 660, 810, and 980 nm) × 2 beam types (Gaussian and Flat beams) × 10 beam diameters (0.04, 0.08, 0.1, 0.2, 0.3, 0.4, 0.6, 0.8, 1.0, and 1.2 cm) were simulated. For each simulation, 10⁷ photons were launched and repeated ten times, and then averaged to increase the signal-to-noise ratio. As shown in Figure 1B, the beam incident normally on the rat and was centred on the SCI site. Each simulation took ~120 seconds to complete on a GPU Nvidia GTX 1070. The simulated fluence distributions were analyzed to address the optimization of choice of PBMT wavelengths, beam types and beam diameters for the SCI rat model.

Table 2.

Optical properties of SCI rat’s tissue for 660, 810, and 980 nm light [28,29,30,31]. The refractive indices (n) for the tissues were assumed to be 1.37.

Tissue type	660 nm			810 nm			980 nm
	μa	μs	g	μa	μs	g	μa	μs	g
Skin	0.0340	25.80	0.900	0.0195	19.20	0.900	0.045	14	0.900
Muscle	0.0870	8.61	0.900	0.0270	6.87	0.900	0.051	5.56	0.900
Soft tissue	0.0890	13.23	0.900	0.0212	10.16	0.900	0.164	7.955	0.900
Bone	0.0100	15.23	0.900	0.0070	13.28	0.900	0.021	11.93	0.900
Spinal cord	0.0216	15.47	0.900	0.0134	11.13	0.900	0.0405	8.184	0.900
Blood (SpO2>98%)	0.1500	87.61	0.983	0.4000	76.10	0.983	0.68	62.68	0.9798

^∗The unit of both absorption coefficient (μa) and scattering coefficient (μs) are in mm-1

Results

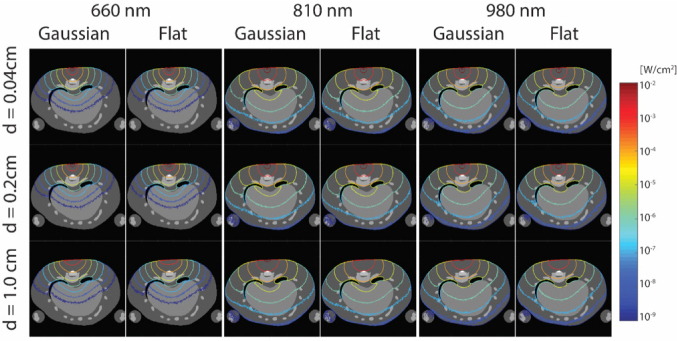
The effects of different wavelengths, beam types and beam diameters on the fluence distribution in the SCI rat model are shown Figure 2. The iso-fluence (iso-F) contour was demonstrated for 10^-2-10^-9 W/cm². The penetration depth of the 810 nm beam was longer than both the 980 nm and 610 nm beams. The 810 nm beam (diameter: 0.2 cm, iso-F contour:10^-2 W/cm²) did penetrate the SCI site, while the 980 nm beam with the same specifications barely touched the surface of the SCI site. The 660 nm beam did not reach the SCI. It was noticed that for the 660 nm beam to penetrate the SCI site, it needed iso-F of 10^-3 W/cm². The iso-F contour was affected strongly by the wavelength. For the beam size effect, the iso-F contour spreads out as the beam diameter increases. It is more noticeable especially for the higher iso-F contours (10^-2-10^-4 W/cm²). Additionally, the penetration depth at the normal central direction decreased as the beam diameter increased. The effect on iso-F contours of the beam size was consistent with the different beam types (e.g., Gaussian or Flat beam). Lastly, the beam type had the weakest effect on the PBMT iso-F contour. The noticeable effect of beam type was that the Gaussian beam formed a more focused iso-F contour (10^-2-10^-4 W/cm²) than did the Flat beam.

Figure 2.

PBM iso-fluence (iso-F) contour in a SCI rat model across different wavelengths (660, 810, and 980 nm), beam types (Gaussian and Flat beam), and beam diameters (0.04, 0.2, and 1.0 cm). The notation “d” represents diameter. The iso-F contour demonstrated here is for 10^-2-10^-9 W/cm².

Figure 3 shows the accumulated fluence of light penetration within the SCI site for different wavelengths, beam types and beam diameters. Wavelength had the most definite effect on the accumulated fluence within the SCI site; where 810 nm was ~1.7× higher than that of 980 nm and ~5.5× higher than that of 660 nm. It is also noticeable that the accumulated fluence decreased with increasing beam diameter. Whereas, the beam type (Gaussian or Flat beam) had a minimal effect on the accumulated fluence within the SCI site. Only at larger beam diameters did the accumulated fluence within the SCI site for Gaussian become higher than that of the flat beam.

Figure 3.

Accumulated fluence of light-penetration within the spinal cord injury (SCI) site of the model for 660 nm Gaussian beam (blue circle) and Flat beam (red circle), 810 nm Gaussian (red triangle) and Flat beam (blue triangle), and 980 nm Gaussian (red square) and Flat beam (blue square) as function of beam diameter.

Discussion and Conclusion

Controversy regarding the efficacy of PBMT is promoted by the presence of articles claiming nil or negative effects, even though positive beneficial effects have been reported in many in vitro studies, in vivo studies and clinical trials [32,33,34,35]. Different irradiation parameters concerning fluence, irradiance and irradiance time alongside pulse structure and insufficient irradiation areas are most probably the reasons for such non-significant effects in certain studies. Whether the results have positive, nil or negative effects may be dependent on the irradiation parameters [36; 37]. There is a plethora of PBMT literature with missing information due to measurement of beam parameters not being performed frequently, not performing the verification calibration of the measuring instrument, important data being frequently unreported, and elementary dose calculation errors[16].

Moreover, the use of fluence as an expression of dosage is unreliable due to the assumption of the inverse relationship between power, beam area, irradiance and irradiation time. Using higher power to reduce the treatments’ irradiance time is not necessarily a reliable approach. Applying the same or similar irradiation parameters, therefore, ensures success in replicating the treatment.

In this study, we quantitatively investigated the PBMT irradiation parameter effects (i.e. wavelengths, beam types and beam diameters) on fluence distribution in a SCI rat model. This was done by utilizing Monte Carlo simulation (MCX) and ROBY (complex 3D rat model).

The findings of this study suggest that wavelength has the most definite effect on the PBMT fluence distribution, followed by beam diameter and then beam type. These findings were verified by calculating accumulated fluence within the SCI site. In conclusion, a new methodology was introduced in this study that can aid in optimizing the irradiation parameters for PBMT in SCI rat models, which may improve the performance of future studies, and may have the potential to be translated to aid in PBMT clinical settings.