Gate-controlled quantum dots and superconductivity in planar germanium

Abstract

Superconductors and semiconductors are crucial platforms in the field of quantum computing. They can be combined to hybrids, bringing together physical properties that enable the discovery of new emergent phenomena and provide novel strategies for quantum control. The involved semiconductor materials, however, suffer from disorder, hyperfine interactions or lack of planar technology. Here we realise an approach that overcomes these issues altogether and integrate gate-defined quantum dots and superconductivity into germanium heterostructures. In our system, heavy holes with mobilities exceeding 500,000 cm² (Vs)⁻¹ are confined in shallow quantum wells that are directly contacted by annealed aluminium leads. We observe proximity-induced superconductivity in the quantum well and demonstrate electric gate-control of the supercurrent. Germanium therefore has great promise for fast and coherent quantum hardware and, being compatible with standard manufacturing, could become a leading material for quantum information processing.

Superconductor–semiconductor hybrid systems can bring together physical properties that are promising for fast and coherent quantum technology. Here, Hendrickx et al. realize such a system in planar germanium heterostructures demonstrating excellent quantum dots and tunable Josephson supercurrents.

Introduction

Solid state quantum computing is actively pursued using superconducting and semiconducting materials^1–3. The group-IV semiconductors Si and Ge come with central advantages for the realisation of spin quantum bits (qubits). Not only has their purity and technology been refined to a formidable level, they also possess an abundant isotope with zero nuclear spin^4,5, enabling spin qubits to reach extremely long coherence times^6,7 and high fidelity⁸. These powerful properties have led to demonstrations of two-qubit logic gates^9,10 and quantum algorithms¹¹. The exchange interaction that is central in these demonstrations is local and cannot directly be used to couple qubits at a distance. Instead, long-range coupling of spin qubits is being explored by incorporating superconductivity and in a first step strong spin–photon coupling has been achieved^12,13.

Hole quantum dots in Ge are particularly promising in this context. Ge has the highest hole mobility of all known semiconductors¹⁴, reaching values up to 1,500,000 cm² (Vs)⁻¹ in doped heterostructures¹⁵ and is expected to host strong spin–orbit coupling^16,17, which facilitates electrical driving for fast qubit operations¹⁸. Furthermore, the valence band in Ge has no valley degeneracy, so, compared to electrons¹⁹, hole qubits do not have the complication of these close quantum levels¹⁶. Experiments have shown readout of holes in Ge/Si nanowires^20,21, self-assembled quantum dots²² and hut wires²³, and promising spin lifetimes have been found^23,24. In addition, the strong Fermi-level pinning at the valence band edge leads to ohmic behaviour for all metal–(p-type) Ge contacts²⁵. The resulting strong coupling between metal and semiconductor enables the fabrication of hybrid devices of quantum dot and superconducting structures^26,27.

Now, the crucial next step is the development of a planar platform that can bring together low disorder, potential for fast driving and avenue for scaling. Here we address this challenge and present the formation of a quantum dot in a planar Ge quantum well. Furthermore, we implement direct Al-based ohmic contacts that eliminate the need for dopant implantation. In addition, the Al leads can proximity-induce superconductivity in the quantum well and we can control the associated supercurrent by tuning electrical gates.

Results

Ge/SiGe heterostructure

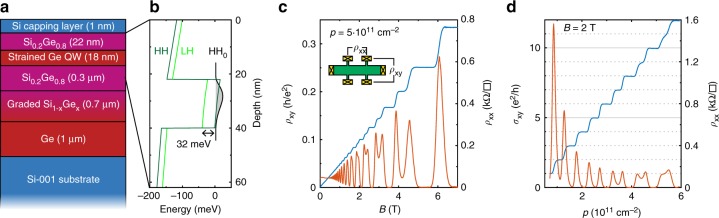
Si and Ge are completely miscible and the lattice constant of their alloy, SiGe, varies continuously between its constituents. This is exploited by using strain-relaxed compositionally graded SiGe layers as virtual substrates to define Ge/SiGe heterostructures as shown schematically in Fig. 1a. We use a high-throughput reduced-pressure chemical vapour deposition (RP-CVD) reactor to grow the complete heterostructure in one deposition cycle on a 100 mm Si(001) substrate. The Ge quantum well is deposited pseudomorphically on a strain-relaxed Si_0.2Ge_0.8/Ge/Si virtual substrate obtained by reverse grading²⁸ (see Methods). The resulting in-plane compressive strain in the quantum well splits the valence band states, which further increases the hole mobility²⁹. The quantum well layer is separated from the surface by a Si_0.2Ge_0.8 spacer and a Si cap.

Fig. 1.

Ge/SiGe heterostructure and magnetotransport measurements. a Schematic representation of the full heterostructure (dimensions not to scale). b Simulated band structure within the SiGe–Ge–SiGe heterostructure, showing strain-induced splitting of the heavy and light hole band edges and strong confinement of heavy holes in the Ge. c, d Magnetotransport measurements in a perpendicular magnetic field B, showing clear Shubnikov–de Haas oscillations and quantised Hall conductance as a function of B (c) and the carrier sheet density p (d) as converted from the top gate potential using low-field Hall effect data

Because of the type-I band alignment in Ge-rich Ge/SiGe heterostructures, the valence band maximum is energetically higher in Ge than in SiGe, such that holes accumulate in the Ge quantum well³⁰. Heavy and light hole (HH and LH, respectively) electronic states and the related band profiles have been calculated by solving the Schrödinger–Poisson equation for low temperatures as a function of vertical electric field. In our simulations, both a multi-valley effective mass approach³¹ and an atomistic tight-binding model³² have been used, obtaining consistent data. The calculated valence band edge profile is shown in Fig. 1b, where the wave function of the fundamental state HH₀, which has HH symmetry and is well confined in the Ge layer, is also sketched. The strain-induced splitting of the HH and LH band edges in the Ge region increases the energy of the fundamental LH state LH₀ (not shown), which is found at about 46 meV above HH₀. Furthermore, we estimate a separation of 14 meV for the first excited HH level HH₁ (not shown). Notice that these energy splittings are significantly larger than those resulting from the valley interaction in Si/SiO₂ and Si/SiGe devices, and thus excellent conditions for the operation of spin qubits are expected in this material.

The hole mobility is measured in magnetotransport experiments at 50 mK using a heterostructure field effect transistor as shown in the inset of Fig. 1c. Here the yellow boxes indicate metallic ohmic contacts to the quantum well created by diffusion into the top SiGe layer and the green structure represents an isolated Hall-bar-shaped top gate, which is used to control the hole density in the quantum well. Clear Shubnikov–de Haas oscillations with zero-resistivity minima and Zeeman splitting of the Landau levels at higher fields are observed in the longitudinal resistance ρ_xx as a function of the magnetic field B (Fig. 1c). Furthermore, we observe flat quantum Hall effect (QHE) plateaus at values 1/ν for integer ν in the transverse resistance ρ_xy in units of h/e², where h is Planck’s constant and e the elementary charge. The investigated heterostructures support a density of up to 6 × 10¹¹ cm⁻² and have a maximum mobility of more than 500,000 cm² (Vs)⁻¹, corresponding to a mean free path of L_m = 6.4 μm and providing new benchmarks for holes in undoped structures. Figure 1d shows the Hall conductivity σ_xy in units of e²/h as a function of the hole density p, controlled by the top gate potential. Again, zero-resistivity minima in ρ_xx and clear linear quantisation steps in σ_xy are observed. This demonstrates the control of the hole density over a large range using the top gate, which is a central prerequisite for the formation of electrostatically defined quantum dots.

Quantum dot

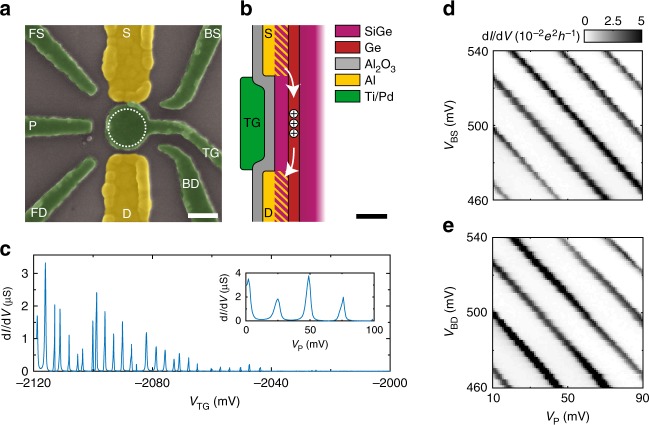
A scanning electron microscope (SEM) image of the quantum dot nanostructure is shown in Fig. 2a. Here the ohmic Al contacts are coloured in yellow and the isolated Ti/Pd gates are shown in green. In a first step, the Al contacts to the Ge quantum well are defined by electron beam lithography, local etching of the Si capping layer and thermal evaporation of Al. Subsequently, an Al₂O₃ gate dielectric is grown by atomic layer deposition at 300 °C, which also serves as an annealing step to enable the diffusion of Al into the SiGe spacer. In the Ti/Pd gate layer, we design a circular top gate between the two Al leads under which a single quantum dot will be formed. In addition, a central plunger gate P is included to control the dot occupation, as well as barrier gates (BS and BD) and additional finger gates (FS and FD) in the corners of the device. These allow for additional control of the dot size and the tunnelling rates between the quantum dot and the source and drain leads.

Fig. 2.

Fabrication and operation of a gate-defined quantum dot. a False-coloured SEM image of the quantum dot device. The quantum dot is defined under the top gate TG (dotted circle) and its occupancy can be controlled by the central plunger gate P. BS and BD correspond to source and drain barriers, respectively; FS and FD are finger gates for additional control. Scale bar is 100 nm. b Schematic of the device gate layers, showing the top gate and the ohmic contacts achieved by in-diffusion of Al. Scale bar is 50 nm. c Transport measurements showing Coulomb oscillations as a function of the top gate with V_P = 0 mV and as a function of the plunger gate with V_TG = −2120 mV (inset). d, e Influence of the barrier gates BS with V_BD = 500 mV (d) and BD with V_BS = 500 mV (e) on the observed conductivity. The coupling of the two individual gates to the quantum dot is nearly identical, emphasising the excellent homogeneity reached in this system

A conceptual drawing of the device cross-section is shown in Fig. 2b, where the diffused Al leads that contact the Ge quantum well are indicated by the stripe-patterned regions. Because of the ohmic nature of the contact, the transport through the quantum dot can be measured without the need for additional reservoir gates and dopant implantation. As a result, no annealing step at temperatures higher than the quantum well deposition temperature (500 °C) is needed during the fabrication, avoiding harmful Ge/Si intermixing at the interface²⁹.

When measuring the source drain conductance dI/dV as a function of the top gate voltage, conductance peaks are expected when the dot potential aligns between the source and drain potentials, which are the so-called Coulomb oscillations. This is shown in Fig. 2c, where dI/dV was measured as a function of the top gate voltage V_TG. The expected oscillations are observed, which is a clear sign of the formation of a quantum dot. The spacing between the peaks is quite regular, indicating that the quantum dot is operated in the many-hole regime. From the period of the conductance peaks, we can extract a top gate capacitance of ~56 aF, which is in very good agreement with the expected capacitance of 52 aF of a parallel plate capacitor using the lithographic dimensions of the top gate. When the top gate voltage is increased and the quantum dot is depleted, the amplitude of the observed peaks is reduced and eventually vanishes, as the tunnelling rates to source and drain reservoirs drop as an effect of the reduced size of the dot. As shown in the supplementary material, we are able to circumvent this effect using an additional accumulation gate in a different device, which allows us to reach the few-hole regime (Supplementary Fig. 1). When TG is tuned to the quantum dot regime, similar oscillations are observed as a function of the plunger gate voltage V_P. Here a larger spacing of the Coulomb peaks is observed, corresponding to a gate capacitance of ~6.4 aF, in agreement with the expected weaker coupling of P to the quantum dot. Note that because of the structure of the source and drain leads no additional tuning of the device is necessary. Equivalent to a classical transistor, the quantum dot can be defined using a single gate (TG), which bodes well for the scaling up of qubits in this system.

As shown in Fig. 2d, e, the dot occupancy can also be controlled using the barrier gates BS and BD. The observed conductance lines are diagonal and very equivalent for each of the two barriers and the plunger, indicating that the coupling to the quantum dot is nearly identical. This confirms that the quantum dot is formed in a central position under TG and that, as an effect of low disorder in the heterostructure, a very high level of control is achieved. Furthermore, by implementing a second layer of Ti/Pd gates we can also independently control the tunnelling rate between the quantum dot and the reservoir (Supplementary Fig. 2).

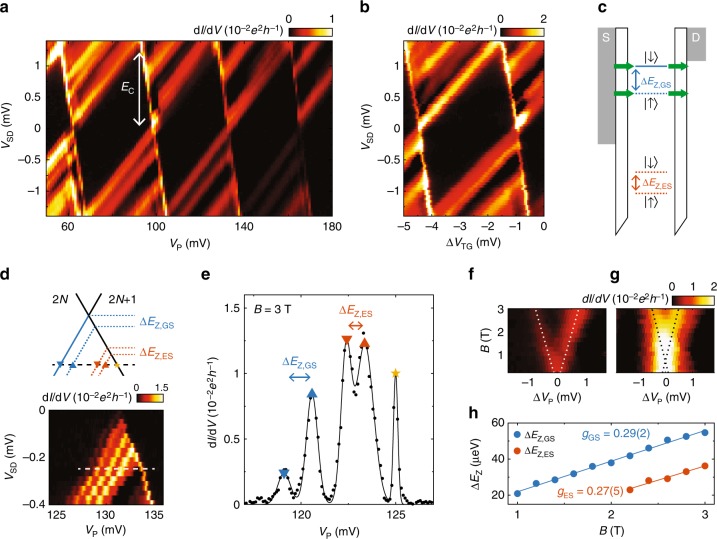
To further characterise the quantum dot, we measure dI/dV as a function of V_P and the DC bias voltage V_SD. As shown in Fig. 3a, Coulomb diamonds are observed³³. From the height and width of these diamonds, the charging energy E_C and the lever arm of the corresponding gate α can be extracted. In the regime shown here (V_BS = V_BD = 550 mV and V_FD = 600 mV), we find α_P = 0.037(3) and E_C = 1.3(1) meV.

Fig. 3.

Bias spectroscopy and g-factor measurements. a, b Colour plots of bias spectroscopy as a function of V_P (a) and ΔV_TG = V_TG + 2084 mV (b), showing Coulomb diamonds with E_C = 1.3(1) meV. c Schematic drawing of the Zeeman splitting ΔE_Z = gμ_BB of the quantum dot levels, illustrating the hole transport via the Zeeman-split energy levels. d Bias spectroscopy showing the line splitting in a 2.9 T in-plane field. Dashed line indicates the cut plotted in e. e Differential conductance as a function of ΔV_P for B = 3 T, at V_SD = −0.26 mV. Solid line corresponds to a fit to the data using the sum of five Gaussian profiles. f, g Differential conductance for both the ground (f) and the excited state (g) as a function of B and ΔV_P, with $\mathrm{\Delta }{V}_{\mathrm{P}}=V_{\mathrm{P}}-{\overline{V}}_{\mathrm{peak}}$ and ${\overline{V}}_{\mathrm{peak}}$ being the average voltage of the two transition peaks corresponding to either the ground or the excited state (for raw data, see Supplementary Fig. 3). Dotted lines represent linear fits to the peak positions (same as in g). h Energy splittings for the ground and excited state as a function of B. Solid lines are linear fits to the data yielding the corresponding g-factors

Similar diamonds are observed as a function of V_TG (Fig. 3b). Here the lever arm is found to be significantly larger (α_TG = 0.41(3)), as is expected because the dot is formed directly under TG. A substructure is clearly visible in the conducting areas. These additional lines could correspond to either charge transport via excited states of the dot or to a modulation of the density of states within the source and drain reservoirs³⁴.

When an external magnetic field B is applied, the energy levels of spin degenerate states are expected to split as a result of the Zeeman effect³³ (cf. Fig. 3c). This becomes apparent as a splitting of the conductance lines related to odd hole occupations 2N + 1 of the quantum dot, as shown in Fig. 3d in an in-plane magnetic field B = 2.9 T. Both the ground state and the excited state are subject to this splitting, which we extract by fitting the observed conductance for V_SD = −0.26 mV using Gaussian profiles and multiplying the splitting in voltage with the measured lever arm α_P (Fig. 3e).

A linear trend is observed as a function of the applied magnetic field as shown in Fig. 3f, g. Note that small splittings ΔE_Z < 20 μeV could not be resolved because of the finite width of the conductance peaks. We find the effective g-factors g_GS = 0.29(2) and g_ES = 0.27(5) for the ground state and excited state, respectively, from the linear fits in Fig. 3h. For the excited state, our data point to either a non-linearity at lower fields or a significant zero-field spin splitting ΔE_Z,0 ≈ −11 μeV. The g-factor of the pure HH state is expected to vanish completely for an in-plane field. However, the additional confinement of the holes in the x,y-plane leads to a significant admixture of LH states and a non-zero in-plane Zeeman splitting³⁵.

The observed spin splitting of the first line parallel to the diamond edge ground state identifies it as belonging to the first excited state rather than being connected to the reservoir. The measured energy splitting with respect to the ground state ΔE ≈ 100 μeV remains unchanged as a function of magnetic field strength. It can be compared to the expected level splitting for a two-dimensional (2D) quantum dot with area A and effective hole mass m^*, which is given by ΔE = πℏ²/m^*A. From the temperature dependence of the Shubnikov–de Haas oscillations measured for the Hall bar structures, we find m^* = 0.08 m_e, with the electron mass m_e, and with our device geometry (A =  0.019 μm²) we obtain ΔE ≈ 150 μeV, in good agreement with the measured value.

The creation of ohmic leads by the diffusion of Al in the direct vicinity of the quantum dot could be suspected of creating additional charge traps that have a negative influence on the coherence of a potential qubit. To quantify this effect, we measure the charge noise acting on our quantum dot by recording the transport current I_dot in a sensitive region, i.e. on the slope of a Coulomb peak. A 100-s-long time trace of I_dot is acquired at a sampling rate of 30.5 kHz and is decomposed into 15 traces of equal lengths. The discrete Fourier spectra obtained from these traces are averaged, yielding the noise spectral density S_E presented in Fig. 4 in comparison to the corresponding spectrum measured in a low sensitivity region (Coulomb blockade). The difference between the two traces confirms that the measured low-frequency noise spectrum is indeed dominated by charge noise acting on the quantum dot. The noise spectrum follows a typical 1/f trend for low frequencies^36,37 (solid line in Fig. 4). We find the equivalent detuning noise at 1 Hz to be 1.4 μeV/$\sqrt{\mathrm{Hz}}$. This compares well to noise figures at 1 Hz in other materials, such as 7.5, 0.5, or 2.0 μeV/$\sqrt{\mathrm{Hz}}$ in GaAs³⁶, Si/SiO₂³⁷ and Si/SiGe³⁷, respectively, showing the suitability of our approach for the creation of low-noise qubits.

Fig. 4.

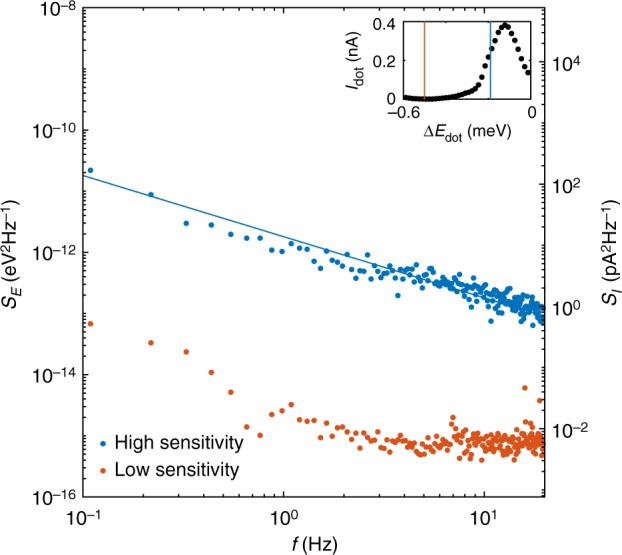
Quantum dot charge noise spectrum. Current noise power spectrum for the quantum dot in transport (blue dots) and in Coulomb blockade (orange dots) as indicated in the inset. Solid line corresponds to an apparent linear fit to the data, yielding a slope of −1.00(4)

Josephson field effect transistor (JoFET)

The direct ohmic contact of the Al leads to the Ge quantum well can proximity-induce superconductivity in the semiconductor system. To demonstrate this effect, we fabricate a JoFET device with two thicker (d = 30 nm) and broader (w = 1 μm) aluminium leads. These are positioned with a separation of 100 nm and are overlaid with a single top gate; a SEM image of the device is shown together with a schematic drawing of the layer structure in Fig. 5a, b. When the source-drain voltage is measured as a function of the sourced current I_SD at negative top gate potential, a zero-resistance plateau is observed as a clear sign of a supercurrent. This is shown in Fig. 5c, where the blue and orange traces represent measurements in different sweep directions, respectively. Sweeping towards higher absolute values of I_SD, the critical current I_c is reached at ~12 nA. Beyond these values, linear (ohmic) behaviour is observed. A hysteresis can be observed when sweeping back, which can be expected for proximity induced SNS-junctions and is usually caused by self-heating of the junction when it exits the superconducting state³⁸. To demonstrate that this supercurrent is induced in the quantum well, we measure the observed bias current dependence of the source-drain resistance dV/dI as a function of the top gate potential V_TG, shown in Fig. 5d. The critical current, which defines the borders of the zero resistance range observed as a black region in the colour plot, is reduced towards increasing V_TG. This is an effect of the decrease in the carrier density and the resulting increase of the normal state resistance. For voltages above V_TG ≈ −1.27 V, I_c cannot be resolved at the limited instrumental resolution in our measurements. For decreasing gate potential, we find that the increase in I_c is more significant than the reduction in the normal resistance R_n. The characteristic voltage I_cR_n hence increases and reaches values higher than ~10 μV. Additionally, a modulation of the critical current can be observed as a function of magnetic field, which is a clear hallmark of the Josephson effect (Supplementary Fig. 4). These proof-of-principle experiments should be followed by a detailed characterisation of the proximity-induced superconductivity in this platform. Interesting future paths of research include the study of ballistic transport and the investigation and optimisation of the sharpness of the Al–Ge interface, which could significantly increase the I_cR_n product.

Fig. 5.

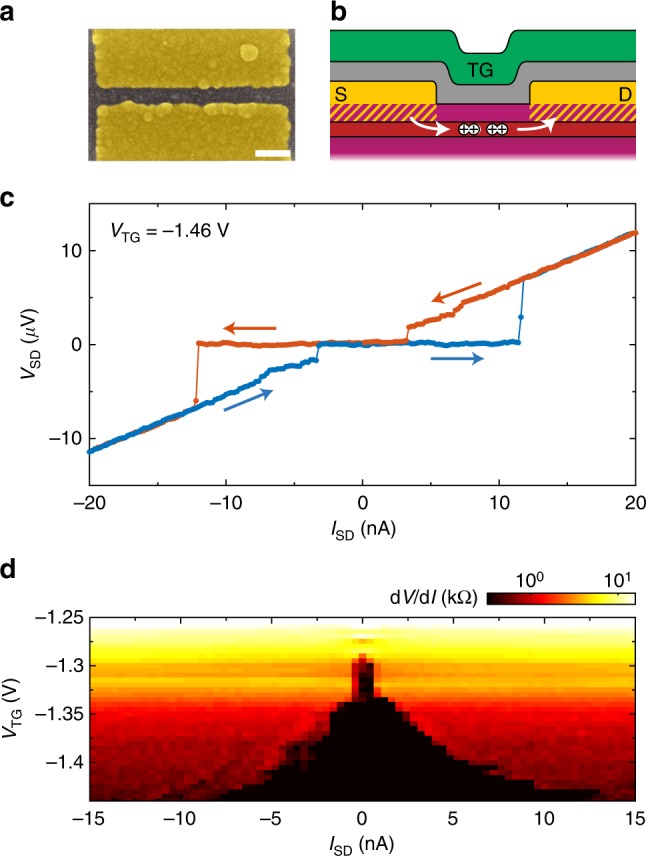
Tunable induced superconductivity in the Ge quantum well. a SEM image of the JoFET device with a gap of 100 nm. Scale bar is 200 nm. b Device schematic using the colour scheme of Fig. 2b. Superconducting transport through the quantum well is illustrated. c Source-drain voltage as a function of the applied current at a top gate voltage of V_TG = −1.46 V. A flat plateau with zero resistance is observed up to a critical current of I_c ≈ 12 nA as a clear signature of a supercurrent through the device. d The critical current can be modulated by tuning the top gate voltage, as shown in the colour plot of the current dependence of the device resistance as a function of V_TG, where I_SD was swept from negative to positive values

Discussion

In conclusion, we have shown the operation of a hole quantum dot in a planar, undoped and buried Ge quantum well with a record hole mobility. The Al ohmic leads to the quantum dot significantly simplify the fabrication and tuning processes without an increase of the measured charge noise in comparison to other systems. The strong capacitive coupling between the superconductor and the quantum dot makes this system ideal for reaching strong spin–photon coupling, while the strong spin–orbit coupling present in HHs could be exploited for fast electrical qubit driving. Furthermore, the demonstration of gate-tunable superconductivity opens up new research directions, including Majorana modes³⁹ and gatemons²⁷. Hole quantum dots in planar Ge constitute thereby a versatile platform that can leverage semiconductor manufacturing to advance and broaden the field of quantum computing.

Methods

Heterostructure growth

The Ge/SiGe heterostructures were grown in one deposition cycle in an Epsilon 2000 (ASMI) RP-CVD reactor on a 100 mm n-type Si(001) substrate (resistivity 5 Ω cm). The growth starts with a 1-μm-thick layer of Ge, using a dual-step process with initial low-temperature (400 °C) growth of a Ge seed layer followed by a higher-temperature (625 °C) overgrowth of a thick relaxed Ge buffer layer. A cycle anneal at 800 °C is performed to promote full relaxation of the Ge. Subsequently, a 700 nm reverse-graded Si_1−xGe_x layer is grown at 800 °C with x changing linearly from 1 to 0.8. A relaxed 300 nm buffer Si_0.2Ge_0.8 is then grown in two steps at an initial high temperature of 800 °C followed by a low-temperature growth at 500 °C. The growth continues with a 18-nm-thick strained Ge quantum well, a 22-nm-thick Si_0.2Ge_0.8 barrier and a 1-nm-thick Si cap. The final layers are all deposited at a temperature of 500 °C.

Device fabrication

The photolithography process for fabricating the Hall bar field effect transistors includes the following steps: mesa etching, deposition of 60-nm-thick Pt layer ohmic contacts, and atomic layer deposition (ALD) at 300 °C of a 30-nm-thick Al₂O₃ dielectric to isolate a Ti/Au top gate (thicknesses 10/150 nm).

For the quantum dot, the contact and gate structures are created by electron beam lithography, electron beam evaporation of Al and Ti/Pd and lift-off. Following the Al contact layer (20 nm), ALD is used to grow 17 nm of Al₂O₃ at 300 °C as a gate dielectric, followed by the Ti/Pd (5/35 nm) gate structures. For the JoFET device, the same process is done with layer thicknesses 30 nm (Al), 25 nm (Al₂O₃) and 5/35 nm (Ti/Pd).

Measurement system

Magnetotransport data has been obtained in a ³He dilution refrigerator with a base temperature of 50 mK, equipped with a 9 T magnet. The carrier density $p={\left(∣e∣\frac{\mathrm{d}{\rho }_{yx}}{\mathrm{d}B}\right)}^{-1}$ in the quantum well is derived from the measured Hall resistivity for fields B < 0.3 T, where no QHE steps are observed. This density can also be derived from the Fourier transform of the longitudinal resistance data as a function of 1/B. From the observed peak width of 1.5 T, we estimate an upper bound for the zero-field spin splitting in our two-dimensional hole gas of 1.5 meV. All quantum dot and JoFET measurements were performed in a ³He dilution refrigerator with a base temperature of <10 mK, equipped with a 3 T magnet. All quantum dot measurements of dI/dV are performed using lock-in amplification with typical modulation amplitude and frequency of δV_SD = 10–100 μV and f_mod = 73.5 Hz, respectively, and a small offset in bias voltage due to the measurement electronics is subtracted. The JoFET device was measured in a four-point configuration, sourcing a current and measuring the potential across the superconducting junctions. The plotted voltage is corrected by a small offset of the measurement electronics. The differential resistance is measured using lock-in amplification with typical a modulation amplitude of δI_SD = 0.3 nA.

Data availability

All data underlying this study are available from the 4TU ResearchData repository at 10.4121/uuid:6a4c90c9-1fd7-442f-bc21-8fbe6876e1ea.

Author contributions

N.W.H. fabricated the quantum dot devices, M.K. fabricated the JoFET devices and N.W.H. and D.P.F. performed the experiments, supported by M.L.V.T. and R.L. A.S. prepared the heterostructures, D.S. fabricated the Hall bar transistors measured at low temperature by D.S., L.Y. and G.S. G.S. supervised the material development. N.W.H., D.P.F., G.S. and M.V. analysed the data; M.Vi. and G.C. carried out additional analysis. N.W.H. and D.P.F. wrote the manuscript with input from all authors. M.V. conceived and supervised the project.

Competing interests

The authors declare no competing interests.