Skip to main content
PMC home page
Light, Science & Applications logoLink to Light, Science & Applications
. 2018 Mar 9;7:17156. doi: 10.1038/lsa.2017.156

Spiniform phase-encoded metagratings entangling arbitrary rational-order orbital angular momentum

Kun Huang 1,2,3,✝, Hong Liu 2,✝, Sara Restuccia 4,✝, Muhammad Q Mehmood 5, Sheng-Tao Mei 1,6, Daniel Giovannini 7, Aaron Danner 1, Miles J Padgett 4, Jing-Hua Teng 2,*, Cheng-Wei Qiu 1,6,8,9,*
PMCID: PMC6060047  PMID: 30839520

Abstract

Quantum entanglements between integer-order and fractional-order orbital angular momentums (OAMs) have been previously discussed. However, the entangled nature of arbitrary rational-order OAM has long been considered a myth due to the absence of an effective strategy for generating arbitrary rational-order OAM beams. Therefore, we report a single metadevice comprising a bilaterally symmetric grating with an aperture, creating optical beams with dynamically controllable OAM values that are continuously varying over a rational range. Due to its encoded spiniform phase, this novel metagrating enables the production of an average OAM that can be increased without a theoretical limit by embracing distributed singularities, which differs significantly from the classic method of stacking phase singularities using fork gratings. This new method makes it possible to probe the unexplored niche of quantum entanglement between arbitrarily defined OAMs in light, which could lead to the complex manipulation of microparticles, high-dimensional quantum entanglement and optical communication. We show that quantum coincidence based on rational-order OAM-superposition states could give rise to low cross-talks between two different states that have no significant overlap in their spiral spectra. Additionally, future applications in quantum communication and optical micromanipulation may be found.

Keywords: metasurface, nanophotonics, orbital angular momentum, superposition state

Introduction

Light has many different properties that are described by its electromagnetic field. One of the most interesting properties of light is its ability to carry orbital angular momentum (OAM), which manifests itself as a helical wavefront with a phase singularity on the beam axis. Since its discovery in 19921, the OAM of light has excited interest because it allows a new degree of freedom and a potentially unbounded number of quantum states for a light beam. The current commonly used technology has resulted in investigations using discrete integer OAMs for applications such as optical trapping and manipulation2, 3, 4, 5, 6, 7, 8, photon entanglement9, 10, 11, 12, astronomy13, microscopy14, 15, remote sensing and detection16, 17, optical communications18, 19, 20 and even integrated photonics21, 22, 23, 24, 25, 26, 27, 28, 29, 30. The rapidly developing exploitation of such diverse areas requires further development of OAM generation technology.

Hitherto, the devices for OAM generation have been primarily concerned with producing integer values of OAM states, even though one can theoretically continuously tune the OAM by changing the topological charges (TCs) of LG and Bessel beams31, 32, 33 or tailoring the ellipticity of Ince–Gaussian modes34. An OAM carrying beam has a helical phase eiℓφ (where ℓ and φ are the winding numbers of the helical phase and angular coordinate, respectively)1, giving rise to an intensity annulus (i.e., doughnut) that is uniform for the integer ℓ, while for fractional ℓ, the intensity annulus is discontinuous with a phase step along φ=0. This smoothness leads to a similar influence on the design of the kinoform for generating the diffractive optical component, for example, fork gratings have smoothly varying fringes for integer ℓ and cutoff fringes with a discontinuity along φ=0 for fractional ℓ35, 36. This distinction makes it fundamentally difficult to transition between integer OAM and fractional OAM in a static device, resulting in poor reconfigurability since different OAM states must be individually addressed by separate devices or phase profiles21, 22, 23, 37, 38, 39, 40. Digital devices such as spatial light modulators (SLMs)41 and digital micromirror devices (DMDs)42 have been used to generate different OAM values. However, their pixel resolution limits lead to spatial phase jumps and account for inaccuracies of fractional OAM (see Section 1 in Supplementary Materials). Therefore, the community has to explore the applications of such digital devices (such as those for quantum entanglement) on the basis of the integer or fractional-order OAM9, 10, 11, 12, 43, 44.

Furthermore, tunable or continuous OAMs have recently received increasing attention for applications like path-OAM-interfaced quantum entanglement45 and optical successive micromanipulation46. Attempts have been made to generate tunable OAMs using indirect methods such, as the weighted superposition of two cross-polarized beams46, the interference of two vortices47, internal conical diffraction48 and optical geometric transformations45, 49, 50, 51. Although these methods offer a new degree of control for the OAM of light, they are intrinsically accompanied by either poor beam quality, very limited tunable ranges or complicated transformations that require optical correction after long-distance propagation. Novel approaches are highly desired for exploring and extending the applications of OAMs in a rational-order manner.

Here, we report a continuous OAM transmitter including bilaterally symmetric gratings with an aperture that produces arbitrary rational-order vortex beams carrying OAMs without any theoretical limit. Distinguished from other vortex beams (e.g., LG and Bessel beams) that change their OAMs by changing TCs, our rational-order OAM beam has a spiniform wavefront with phase singularities located equidistant along a line and tunes its average OAM by changing the number of singularities that the beam accommodates. This approach realizes both non-integer and arbitrary rational-order generation of OAM across the full range by transmitting these phase singularities through the aperture and enables the exploration of quantum entanglement based on such continuous OAMs for communication purposes.

Materials and methods

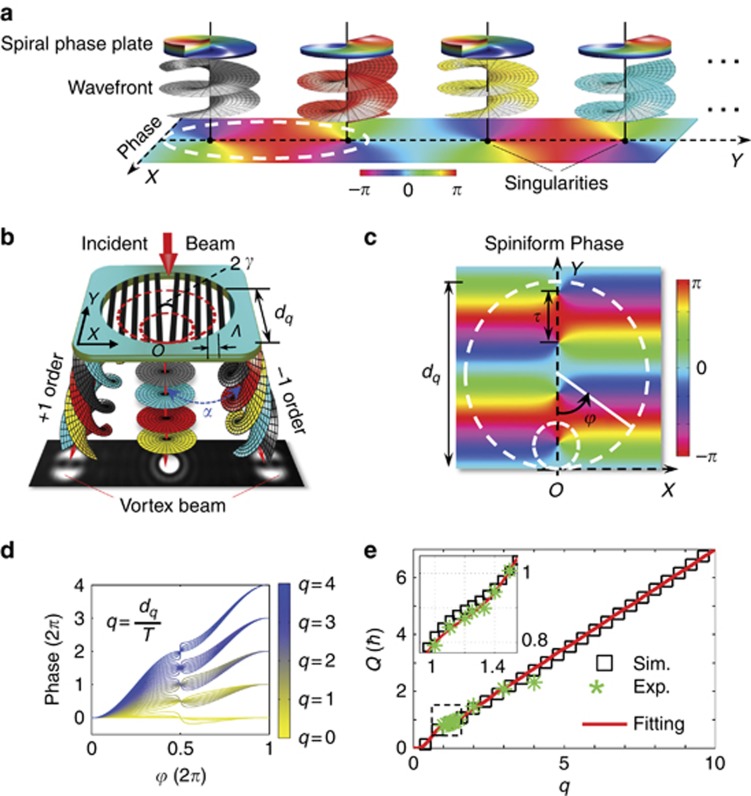
Traditionally, light with a planar wavefront can increase its OAM by successively passing through ℓ concentric and vertically located spiral phase plates (SPPs), each of which has a TC of 135. Similarly, light could also obtain an OAM by passing through a series of transversely located SPPs (Figure 1a), which have wavefronts with spatially separated singularities. One can increase the OAM of light by including more SPPs, leading to more phase singularities in the wavefront of the light. Hence, when a phase profile with regularly distributed (e.g., periodic) singularities is encoded into a beam generator, we infer that the optical vortices will be smoothly emitted, making it possible to generate a continuous OAM by employing a gradually varying aperture.

Figure 1.

Figure 1

Open in a new tab

Mechanism of the analog vortex transmitter. (a) Light obtains a helical wavefront with spatially separated phase singularities (black dots) by passing through four transversely located SPPs. (b) Sketch of the transmitter composed of two inclined (inclination angle of γ) gratings with a period of Λ at both sides and a circular aperture of varying diameter (dq), which geometrically acts as an excircle (red dashed circles) tangent to the x-axis at a reference point O. (c) Phase profile encoded into the vortex transmitter. τ denotes the spatial distance between two neighboring phase singularities. φ is the angle coordinate of the circular aperture and increases anticlockwise from φ=0 (negative y-axis) to 2π. (d) Phase along the circumference (dashed circle in (d)) of the circular aperture for its corresponding q. The phase at φ>π is unwrapped by adding 2π. The curves denote the phase values for q (distinguished by the curve colors). (e) The average OAM (Qћ) of a photon as a function of q. The fitting curve (solid red line) of the simulated results (black square boxes) exhibits a root mean square error of 0.04, while the experimental results are denoted by greenish asterisks. Inset: Zoom-in of the data between q=1 and q=1.5.

To realize this, we propose using a bilaterally symmetric metagrating with an aperture as a vortex transmitter, whose working principle is sketched in Figure 1b. With its y-axis at the line of symmetry, this transmitter consists of two gratings with a tilting angle γ. A circular aperture is placed above the metagratings, and its diameter dq can vary along the y-axis. For a normally incident plane wave, the transmission function of this transmitter can be expressed as

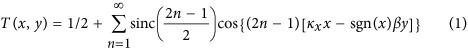graphic file with name lsa2017156e1.jpg

where sinc(x)=sin(πx)/(πx), the diffraction order n is a positive integer, κx is a constant determining the diffraction angle, β stands for a constant phase gradient along y direction, and sgn(x) refers to the sign function of the variable x (and is mathematically responsible for the bilateral symmetry of the structure). The metagrating parameters, such as the period Λ=2π/(κx2+β2)1/2 and the inclination angle γ=tan−1(β/κx), are derived in the Supplementary Materials.

Light from the first-order diffraction (i.e., n=1) possesses a linearly y-dependent phase function:

graphic file with name lsa2017156e2.jpg

where sgn(x) accounts for the opposite phase variation tendency, such that χ increases for positive values of x and decreases for negative values of x. To determine the phase singularities, we show the phase profile after a low-pass filter (see Section 2 in the Supplementary Materials) in Figure 1c, removing the phase jump along the y-axis. Due to its linear y dependence, a phase difference between both sides occurs periodically along the interface, leading to phase singularities at equal spacings of the spatial interval τ. Within one cycle of the 2π phase, the number of phase jumps reaches its maximum of π at a phase singularity twice, which means that the phase difference spanning a distance of τ along y is βτ=π.

Acting as a regulator, the aperture smoothly changes its diameter along the y-axis of symmetry to precisely control the linear output of phase. To quantify this output, we introduce a dimensionless parameter: the singularity strength q≡dq/τ. Because the aperture size dq can be smoothly tuned, q smoothly varies its integral and fractional values to realize the continuous generation of optical vortices by a single transmitter. We plot the phase along the circumference of the aperture for different q values in Figure 1d, showing a phase change of 2π[q], where[q] denotes the round of q and is equal to the number of encircled phase singularities. As expected, our results in Figure 1e reveal that this vortex beam has an average OAM of Qћ (ћ is the reduced Planck constant) for a photon with

graphic file with name lsa2017156e3.jpg

which will be discussed in detail later.

Results and discussion

Considering the operating wavelength (λ<Λ) and fabrication issues, we experimentally applied the following specifications to the sample fabrication: Λ=1 μm, γ=tan−1 (1/240) and, correspondingly, τ=120 μm. This transmitter was patterned on a 100-nm thick chromium film deposited on a quartz substrate via electron beam lithography and a dry etching process. To achieve high-fidelity experimental results, the apertures were directly fabricated on transmitters, leaving the individual samples with different q values. Two groups of specimens with integer q=1–4 and fraction q=1.1–1.5 values were fabricated to exemplify the analog generation concept of rational OAMs. The scanning electron microscopy (SEM) images of the fabricated samples are provided in Section 3 of the Supplementary Materials.

Figure 2 shows the simulated and experimental results of the integer group (q=1–4) at a wavelength of 532 nm. Under the assumption of uniform illumination, the simulated intensity and phase profiles of the light from the first-order diffraction in the Fraunhofer region are shown in Figure 2a. At q=1, an elliptical transverse profile is formed with a single phase singularity, which splits into a two-lobed shape from q=2 onwards due to the spatial mismatch of the singularities. As q increases, the central darkness expands to accommodate more phase singularities, moving the two lobes farther apart. Meanwhile, these two lobes shrink due to gradually weakening diffractions when the aperture continues to increase52. The simulated intensity profiles are well validated by the measurements in Figure 2b. Such an intensity profile originates from the interactions between the spiniform phase and operating circular aperture during its paraxial propagation, which act as low-pass filters in the Fraunhofer region52; see Section 4 in the Supplementary Materials.

Figure 2.

Figure 2

Open in a new tab

Optical vortices with integer q values. (a) Simulated intensity and phase profiles for q=1, 2, 3 and 4, whose Q=0.798, 1.4, 2.126 and 2.8, respectively. (b) Measured intensity profiles of the far field. (c) Experimental interference patterns with plane (left) and spherical (right) waves. (d) Phase profiles retrieved from experimental interference patterns with planar waves. Φ denotes the angular coordinate. (e) Quantitative comparison of the azimuthal phase shift (ΔP≡P(Φ)−P(Φ=0), where P is the phase of this vortex beam) between the experiment (curves) and simulation (square boxes). Data are obtained along the black dashed circles as shown in d.

The optical wavefronts were experimentally revealed in Figure 2c by the interference with a reference Gaussian beam via a Mach–Zehnder interferometer. The dislocated fringes of the plane-wave case and the spiral arms of the spherical-wave case have been revealed in the interferograms. The respective TC is quantifiable through the number of dislocated fringes for the plane-wave case or through the number of arms for the spherical-wave case. Note that the simulated interference patterns (see Sections 5 and 6 in the Supplementary Materials) agree very well with the measured results. Figure 2d plots the corresponding phase profiles retrieved from the experimental results of the plane-wave interference using the Fourier transformation53. The retrieval was validated by the simulation, exhibiting nearly identical phase distributions in Figure 2e. The phase shift accumulated along a closed circle is 2πq, which quantifies the integer TC of q.

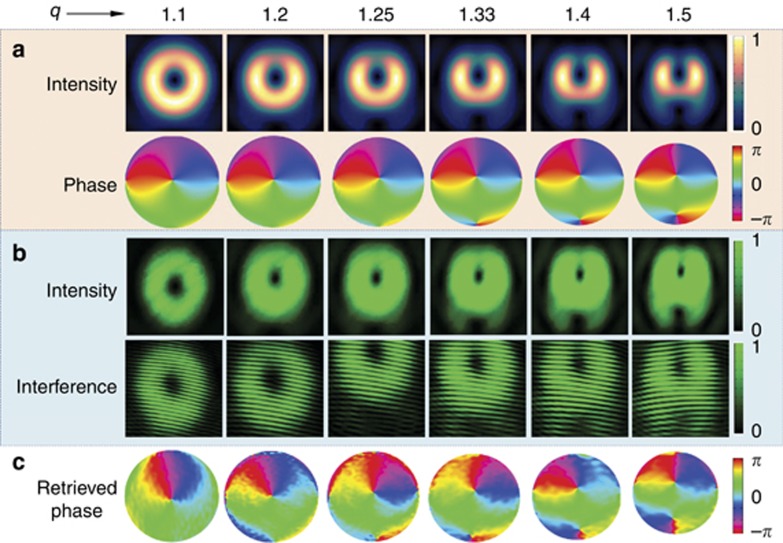
The fractional group (q=1.1–1.5) has been examined under the same conditions, and their results are shown in Figure 3. As q increases, the predicted transverse intensity profile in Figure 3a evolves from an ellipse to an H-shape, and its bottom half tends to enclose a dark core of phase singularity. This result agrees with the measured intensities in Figure 3b, including the experimental interference patterns. The dislocated fringes are enhanced with increasing q, which is attributed to the fact that the neighboring singularity is gradually dominated. The retrieved phase profiles (Figure 3c) agree well with the simulated results (Figure 3a). Additionally, an animation of the continuous generation of these optical vortices with varying q can be found in the Supplementary Movie.

Figure 3.

Figure 3

Open in a new tab

Optical vortices with fraction q values. (a) Simulated intensity and phase profiles for q=1.1, 1.2, 1.25, 1.33, 1.4 and 1.5, whose Q=0.839, 0.868, 0.884, 0.913, 0.946 and 1.007, respectively. (b) Measured intensity profiles (upper) for the different q values and their corresponding interference patterns (lower) with planar waves. (c) Phase profiles reconstructed from experimental interferences with planar waves.

To show the connection between our vortex beam and LG beams, we decompose the spiniform phase in Equation (2) in terms of the angular-dependent helical phase

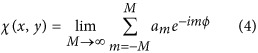graphic file with name lsa2017156e4.jpg

where the coefficient

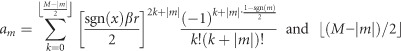graphic file with name lsa2017156e5.jpg

denotes the integer part of (M-|m|)/2. Equation (4) implies that our vortex beam can be written as a weighted superposition of spiral modes and possesses the average OAM per photon, which is similar to the Ince–Gaussian modes33 and fractional-order LG modes36.

To quantify its analog effect, the average OAM carried by this vortex beam has been investigated theoretically (see Section 7 in Supplementary Materials) and experimentally in Figure 1e. The experimental amplitude and phase profiles of our vortex beam in the far field could be obtained with the phase retrieval method, as sketched in Supplementary Fig. S7 of the Supplementary Materials. The average OAM (Q in units of ћ) per photon of the vortex beam is evaluated by using Supplementary Equation (S17) of the Supplementary Materials and is finally correlated as a function of q via Equation (3), which is a fit of the simulated results. Due to the coupling of the sine and sinc functions, this result shows nonlinearity within the interval of [0, +2], beyond which quasilinearity governs the relation between Q and q in the rational range. This is distinct from the pure nonlinear relationship of the LG and Bessel beams31, 32, 33. Thus, the average OAM of such a novel vortex beam has been validated as continuously addressable in rational states without any theoretical limit.

Quantum spiral spectrum

In spontaneous parametric downconversion, OAM-entangled photon pairs have the quantum state54, 55

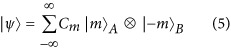graphic file with name lsa2017156e6.jpg

where Cm is the probability amplitude of finding one photon in the signal mode 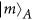Inline graphic and one photon in the idler mode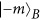 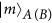Inline graphicInline graphic indicates the optical mode that has one photon with a quantized OAM of mħ in the signal (idler) arm and Inline graphic.

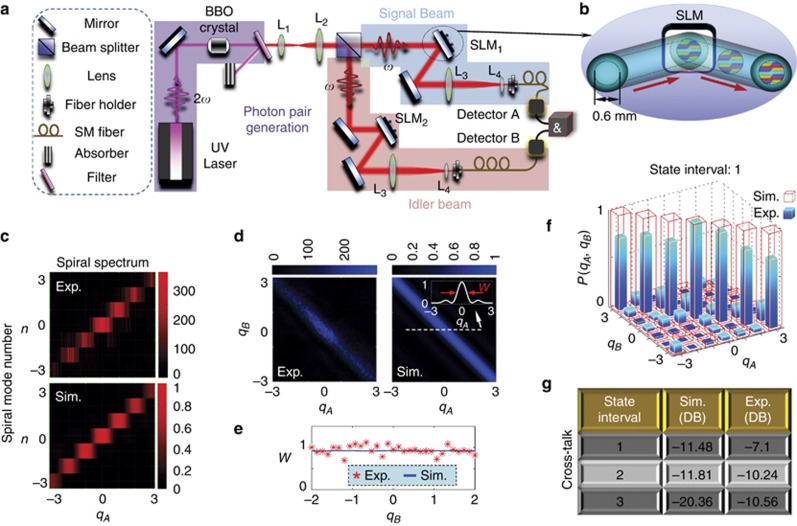
Since our fabricated vortex transmitter has a largest diameter of 480 μm, it is quite challenging to select our vortex beam by using an additional aperture. Thus, the signal beam in the experimental setup given in Figure 4a is imaged on SLM1 is imparted with the spiniform phase (see Figure 4b) to facilitate achieving our fractional OAMs. Note that the vortex beams generated by the spiniform phase-encoded SLM (see Section 8 and Supplementary Fig. S8 of Supplementary Materials) is completely identical to those created by the above vortex transmitters. The only difference is that the SLM cannot, in principle, generate a rigorously continuous OAM. However, this difference will not change the intensity and phase profiles of the proposed vortex beams and, therefore, is still valid for verifying the feasibilities of the use of our vortex beams for quantum operation.

Figure 4.

Figure 4

Open in a new tab

Quantum operation. (a) The experimental setup based on spontaneous parametric downconversion (SPDC). (b) Modulating light with the required phase profiles in a reflective SLM. (c) Quantum spiral spectrum of the generated vortex beam. (d) Quantum coincidences between a vortex beam with qA in the signal beam and that with qB in the idler beam. Inset: Line-scan simulated coincidence as a function of qA when qB=0. (e) The experimental and simulated width w as a function of qB. (f) Quantum coincidences between our vortex beams with discrete qA and qB (=0, ±1, ±2, ±3). (g) The cross-talk of the quantum coincidences for the different state intervals of 1, 2 and 3.

The spiniform phase-encoded SLM will enable the selection of an OAM-superposition state 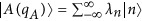Inline graphic, where 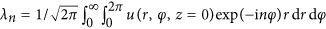Inline graphic; the spiral spectrum is γn=|λn|2/T; and T is a normalization factor, such that 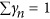Inline graphic (Ref. 56). Similarly, the idler beam modulated by SLM2 is imparted with a helical phase for generating an OAM eigenstate 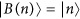Inline graphic. Both resulting beams are separately imaged at the facets of single-mode fibers and are then coupled to avalanche photodiodes for detection. The photodiodes are connected to a coincidence circuit that will allow the recording of the coincidence rate as a function of the states specified by the SLM, thus, by scanning the OAM eigenstate in the idler beam. Thus, one can obtain the coincidence probability

graphic file with name lsa2017156e15.jpg

where the superscript ‘*’ indicates the complex conjugate. Equation (6) can also be taken as the quantum spiral spectrum due to the existence of Cn(Ref. 57). For a maximum entanglement55, Cn is taken as a constant for all the simulations in this paper.

Figure 4c shows the measured and simulated quantum spiral spectra with good agreements. To decrease the experimental error caused by the limited photon flux58, 59, 60, the measured spiral spectrum is evaluated by calculating the quantum contrast for each coincidence measurement, which allows us to express our results as a function of the strength of the quantum correlation. The quantum contrast is defined as the ratio of the recorded coincidence rate to the expected accidental coincidence rate, where the accidental coincidences are calculated by multiplying the time resolution (refer to Ref. 59) of our coincidence counting electronics with the count rates detected by detectors A and B (see Figure 4a)59, 60. In Figure 4c, the experimental quantum contrast gets smaller at larger |qA| values, which is mainly attributed to the limited quantum spiral bandwidth of the system58 and the increasing noise. As qA changes in our experiment, the smooth spiral spectrum confirms that the proposed mechanism is valid for manipulating the OAM at the single-photon level.

Quantum coincidence

Quantum coincidence is carried out by generating two vortex beams with qA and qB in the signal and idler arms. The vortex beam in the idler arm has an OAM-superposition state Inline graphic. The coincidence rate, as a function of qA and qB, can be obtained by

graphic file with name lsa2017156e17.jpg

The experimental coincidence per 4 s is provided in Figure 4d, which is consistent with the simulation results. The diagonal elements with qA=−qB are nearly uniform for the maximum values from both the simulations and experiments. These results indicate that the total angular momentum is also conserved in the spontaneous parametric downconversion process for the OAM-superposition states, which behaves like the case of the OAM eigenstates9.

The coincidence rates decrease gradually when both the qA and qB parameters deviate from qA=−qB. To incorporate this effect, a line-scan simulated coincidence at qB=0 is shown with a width of w (which is evaluated by the full-width at its half-maximum) in the inset of Figure 4d. For a given qB, the width w determines the range of qA where the coincidence is high. The simulated and experimental widths as functions of qB are located at ∼0.925, see Figure 4e. The significance of this result is twofold. First, the vortex beams with discrete q values are preferred to avoid the strong cross-talks between two neighboring states. Second, the state interval (i.e., the minimum difference in OAMs between two states) should be larger than 0.925 to decrease the cross-talk.

Figure 4f shows the simulated and experimental coincidences between these discrete states (qA,B=0, ±1, ±2, ±3) with intervals of 1. Similarly, the maximum coincidence occurs when the diagonal elements obey qA=−qB, as confirmed in both the simulated and experimental results. The coincidence rate of the non-diagonal case stands for the noise and should be suppressed to achieve a low cross-talk. The maximum probability among these non-diagonal cases is 0.0711 (the cross-talk is 10log10(0.0711)=−11.48 dB) in the simulations and 0.1952 (indicating a cross-talk of −7.1 dB) in the experiments. This discrepancy mainly originates from the imperfect generation of our vortex beam caused by SLM pixilation (i.e., the pixel pitch of 15 μm in our SLMs) and the small aperture (0.6 mm in diameter) of the efficient phase of the SLM, which leads to increased noise due to the decreased photon flux used for detection (see Section 8 in the Supplementary Materials). When the state interval is greater, the cross-talk could be further suppressed due to the overlapping of the spiral spectra between two neighboring states becoming smaller. Figure 4g shows that the experimental cross-talks are −10.24 dB for the interval 2 (with qA,B=±1, ±3) and −10.56 dB for the interval 3 (with qA,B=0, ±3), which are comparable to the pure-OAM-based communication requirements18, 19, 61. The experimental and simulated results for intervals 2 and 3 are provided in Section 8 and Supplementary Fig. S9 of the Supplementary Materials.

From the simulated and experimental results, one can find that our vortex beam is able to select the superposition states of OAMs for quantum operations, although this selection is realized by using a phase-type SLM. We have to emphasize that a rigorously continuous generation of rational OAM must refer to the proposed mechanism of our metagratings combined with a smoothly tunable aperture. We also note that two issues should be addressed when carrying out quantum operations using continuous OAMs. First, the total size of the metagratings should be large so that a tunable aperture is available in practice. In this work, the largest diameter of our metagratings is ~480 μm, which is too small for a commonly used aperture. The fabrication of large-scale metagratings can be achieved by using laser direct-writing techniques. Second, the pump laser in the spontaneous parametric downconversion process should be strong enough to enhance the signal-to-noise ratio of the quantum coincidence because the total efficiency of our binary-amplitude gratings has a theoretical value of ~10%.

Conclusions

We have rigorously demonstrated the concept of continuous OAM. The generating optical element is based on periodic gratings of bilateral symmetry with tunable apertures. In addition, the mechanism tailoring the OAM of light via the number of involved phase singularities provides unique insights for investigating the superposition states of OAMs in quantum physics and singular optics. We have demonstrated the feasibility of realizing quantum coincidence by using the OAM-superposition state, which might benefit quantum physics and technology62, 63, 64. Arbitrarily maneuvering OAM across rational states makes is an attractive method for enriching electron vortex beams65, spiral imaging techniques56, 57 and optical continuous manipulation for the effective sorting or selection of microparticles66.

Acknowledgments

We thank Prof Michael V Berry, Prof Etienne Brasselet and Prof Lixiang Chen for their valuable discussions and Dr Yuxuan Ren for his instructive suggestions in the experimental measurements. This research is supported by the National Research Foundation, Prime Minister’s Office, Singapore, under its Competitive Research Programme (CRP Award No. NRF-CRP15-2015-03). This work is also supported by the National Research Foundation, Prime Minister’s Office, Singapore, under its Competitive Research Programme (CRP Award No. NRF-CRP15-2015-01). The work is partially supported by the Institute of Materials Research and Engineering (IMRE) and the Agency for Science, Technology and Research (A*STAR) under Grants 1521480031 and 1527000014. SR and MJP acknowledge support from ERC Advance grant (TWISTS). KH thanks the One-hundred-person Project of the Chinese Academy of Sciences for its support.

Footnotes

Note: Supplementary Information for this article can be found on the Light: Science & Applications website(http://www.nature.com/lsa).

The authors declare no conflict of interest.

Supplementary Material

Supplementary Materials
Click here for additional data file. (1.9MB, pdf)
Supplementary Movie
Click here for additional data file. (7.2MB, avi)

References

  1. Allen L, Beijersbergen MW, Spreeuw RJC, Woerdman JP. Orbital angular-momentum of light and the transformation of Laguerre–Gaussian laser modes. Phys Rev A 1992; 45: 8185–8189. [DOI] [PubMed] [Google Scholar]
  2. Santamato E, Daino B, Romagnoli M, Settembre M, Shen YR. Collective rotation of molecules driven by the angular momentum of light in a nematic film. Phys Rev Lett 1986; 57: 2423–2426. [DOI] [PubMed] [Google Scholar]
  3. He H, Friese MEJ, Heckenberg NR, Rubinsztein-Dunlop H. Direct observation of transfer of angular momentum to absorptive particles from a laser beam with a phase singularity. Phys Rev Lett 1995; 75: 826–829. [DOI] [PubMed] [Google Scholar]
  4. Friese MEJ, Enger J, Rubinsztein-Dunlop H, Heckenberg NR. Optical angular-momentum transfer to trapped absorbing particles. Phys Rev A 1996; 54: 1593–1596. [DOI] [PubMed] [Google Scholar]
  5. Simpson NB, Dholakia K, Allen L, Padgett MJ. Mechanical equivalence of spin and orbital angular momentum of light: an optical spanner. Opt Lett 1997; 22: 52–54. [DOI] [PubMed] [Google Scholar]
  6. Galajda P, Ormos P. Complex micromachines produced and driven by light. Appl Phys Lett 2001; 78: 249–251. [Google Scholar]
  7. Garcés-Chávez V, McGloin D, Padgett MJ, Dultz W, Schmitzer H et al. Observation of the transfer of the local angular momentum density of a multiringed light beam to an optically trapped particle. Phys Rev Lett 2003; 91: 09302. [DOI] [PubMed] [Google Scholar]
  8. Andersen MF, Ryu C, Cladé P, Natarajan V, Vaziri A et al. Quantized rotation of atoms from photons with orbital angular momentum. Phys Rev Lett 2006; 97: 170406. [DOI] [PubMed] [Google Scholar]
  9. Mair A, Vaziri A, Weihs G, Zeilinger A. Entanglement of the orbital angular momentum states of phtotoons. Nature 2001; 412: 313–316. [DOI] [PubMed] [Google Scholar]
  10. Oemrawsingh S, Ma X, Voigt D, Aiello A, Eliel ER et al. Experimental demonstration of fractional orbital angular momentum entanglement of two photons. Phys Rev Lett 2005; 95: 240501. [DOI] [PubMed] [Google Scholar]
  11. Dada AC, Leach J, Buller GS, Padegtt MJ, Anderson E. Experimental high-dimensional two-photon entanglement and violations of generalized Bell inequalities. Nat Phys 2011; 7: 677–680. [Google Scholar]
  12. Fickler R, Lapkiewicz R, Plick WN, Krenn M, Schaeff C et al. Quantum entanglement of high angular momenta. Science 2012; 338: 640–643. [DOI] [PubMed] [Google Scholar]
  13. Foo G, Palacios DM, Swartzlander GA. Optical vortex coronagraph. Opt Lett 2005; 30: 3308–3310. [DOI] [PubMed] [Google Scholar]
  14. Fürhapter S, Jesacher A, Bernet S, Ritsch-Marte M. Spiral phase contrast imaging in microscopy. Opt Express 2005; 13: 689–694. [DOI] [PubMed] [Google Scholar]
  15. Huang K, Li YP. Realization of a subwavelength focused spot without a longitudinal field component in a solid immersion lens-based system. Opt Lett 2011; 36: 3536–3538. [DOI] [PubMed] [Google Scholar]
  16. Lavery M, Speirits F, Barnett S, Padgett MJ. Detection of a spinning object using light’s orbital angular momentum. Science 2013; 341: 537–540. [DOI] [PubMed] [Google Scholar]
  17. Lavery MPJ, Barnett SM, Speirits FC, Padgett MJ. Observation of the rotational Doppler shift of a white-light, orbital-angular-momentum-carrying beam backscattered from a rotating body. Optica 2014; 1: 1–4. [Google Scholar]
  18. Wang J, Yang J-Y, Fazal IM, Ahmed N, Yan Y et al. Terabit free-space data transmission employing orbital angular momentum multiplexing. Nat Photonics 2012; 6: 488–496. [Google Scholar]
  19. Bozinovic N, Yue Y, Ren YX, Tur M, Kristensen P et al. Terabit-scale orbital angular momentum mode division multiplexing in fibers. Science 2013; 340: 1545–1548. [DOI] [PubMed] [Google Scholar]
  20. Lei T, Zhang M, Li YR, Jia P, Liu GN et al. Massive individual orbital angular momentum channels for multiplexing enabled by Dammann gratings. Light Sci Appl 2014; 4: e257, doi:10.1038/lsa.2015.30. [Google Scholar]
  21. Yu N, Genevet P, Kats MA, Aieta F, Tetienne J-P et al. Light propagation with phase discontinuities: generalized laws of reflection and refraction. Science 2011; 334: 333–337. [DOI] [PubMed] [Google Scholar]
  22. Genevet P, Yu NF, Aieta F, Lin J, Kats MA et al. Ultra-thin plasmonic optical vortex plate based on phase discontinuities. Appl Phys Lett 2012; 100: 013101. [Google Scholar]
  23. Chong KE, Staude I, James A, Dominguez D, Liu S et al. Polarization-independent silicon metadevices for efficient optical wavefront control. Nano Lett 2015; 15: 5369–5374. [DOI] [PubMed] [Google Scholar]
  24. Sun JB, Wang X, Xu TY, Kudyshev ZA, Cartwright AN et al. Spinning light on the nanoscale. Nano Lett 2014; 14: 2726–2729. [DOI] [PubMed] [Google Scholar]
  25. Gorodetski Y, Niv A, Kleiner V, Hasman E. Observation of the spin-based plasmonic effect in nanoscale structures. Phys Rev Lett 2008; 101: 043903. [DOI] [PubMed] [Google Scholar]
  26. Gorodetski Y, Drezet A, Genet C, Ebbesen TW. Generating far-field orbital angular momenta from near-field optical chirality. Phys Rev Lett 2013; 110: 203906. [DOI] [PubMed] [Google Scholar]
  27. Liu H, Mehmood MQ, Huang K, Ke L, Ye HP et al. Twisted focusing of optical vortices with broadband flat spiral zone plates. Adv Opt Mat 2014; 2: 1193–1198. [Google Scholar]
  28. Kang M, Chen J, Wang X-L, Wang H-T. Twisted vector field from an inhomogeneous and anisotropic metamaterial. J Opt Soc Am B 2012; 29: 572–576. [Google Scholar]
  29. Cai XL, Wang JW, Strain MJ, Johnson-Morris B, Zhu JB et al. Integrated compact optical vortex beam emitters. Science 2012; 338: 363. [DOI] [PubMed] [Google Scholar]
  30. Brasselet E, Gervinskas G, Seniutinas G, Juodkazis S. Topological shaping of light by closed-path nanoslits. Phys Rev Lett 2013; 111: 193901. [DOI] [PubMed] [Google Scholar]
  31. Leach J, Yao E, Padgett MJ. Observation of the vortex structure of a non-integer vortex beam. N J Phys 2004; 6: 71. [Google Scholar]
  32. Gutiérrez-Vega JC, López-Mariscal C. Nondiffracting vortex beams with continuous orbital angular momentum order dependence. J Opt A 2008; 10: 015009. [Google Scholar]
  33. Götte JB, Franke-Arnold S, Zambrini B, Barnett SM. Quantum formulation of fractional orbital angular momentum. J Mod Opt 2007; 54: 1723–1738. [Google Scholar]
  34. Plick WN, Krenn M, Fickler R, Ramelow S, Zeilinger A. Quantum orbital angular momentum of elliptically symmetric light. Phys Rev A 2013; 87: 033806. [Google Scholar]
  35. Basistiy IV, Soskin MS, Vasnetsov MV. Optical wavefront dislocations and their properties. Opt Commun 1995; 119: 604–612. [Google Scholar]
  36. Basistiy IV, Pas’Ko VA, Slyusar VV, Soskin MS, Vasnetsov MV. Synthesis and analysis of optical vortices with fractional topological charges. J Opt A 2004; 6: S166. [Google Scholar]
  37. Heckenberg NR, McDuff R, Smith CP, White AG. Generation of optical phase singularities by computer-generated holograms. Opt Lett 1992; 17: 221–223. [DOI] [PubMed] [Google Scholar]
  38. Arlt J, Dholakia K, Allen L, Padgett MJ. The production of multiringed Laguerre–Gaussian modes by computer-generated holograms. J Mod Opt 1998; 45: 1231–1237. [Google Scholar]
  39. Beijersbergen MW, Coerwinkel RPC, Kristensen M, Woerdman JP. Helical-wavefront laser beams produced with a spiral phaseplate. Opt Commun 1994; 112: 321–327. [Google Scholar]
  40. Marrucci L, Manzo C, Paparo D. Optical spin-to-orbital angular momentum conversion in inhomogeneous anisotropic media. Phys Rev Lett 2006; 96: 163905. [DOI] [PubMed] [Google Scholar]
  41. Lazarev G, Hermerschmidt A, Krüger S, Osten S. LCOS spatial light modulators: trends and applications In: Osten W, Reingand N. editors. Optical Imaging and Metrology: Advanced Technologies. New York: Wiley-VCH; 2012. [Google Scholar]
  42. Ren Y-X, Li M, Huang K, Wu J-G, Gao H-F et al. Experimental generation of Laguerre–Gaussian beam using digital micromirror device. Appl Opt 2010; 49: 1838–1844. [DOI] [PubMed] [Google Scholar]
  43. Ding DS, Zhang W, Zhou ZY, Shi S, Xiang GY et al. Quantum storage of orbital angular momentum entanglement in an atomic ensemble. Phys Rev Lett 2015; 114: 050502. [DOI] [PubMed] [Google Scholar]
  44. Wang X-L, Cai X-D, Su Z-E, Chen M-C, Wu D et al. Quantum teleportation of multiple degrees of freedom of a single photon. Nature 2015; 518: 516–519. [DOI] [PubMed] [Google Scholar]
  45. Fickler R, Lapkiewicz R, Huber M, Lavery MPJ, Padgett MJ et al. Interface between path and orbital angular momentum entanglement for high-dimensional photonic quantum information. Nat Commun 2014; 5: 4502. [DOI] [PubMed] [Google Scholar]
  46. Gecevičius M, Drevinskas R, Beresna M, Kazansky PG. Single beam optical vortex tweezers with tunable orbital angular momentum. Appl Phys Lett 2014; 104: 231110. [Google Scholar]
  47. Schmitz CHJ, Uhrig K, Spatz JP, Curtis JE. Tuning the orbital angular momentum in optical vortex beams. Opt Express 2006; 14: 6604–6612. [DOI] [PubMed] [Google Scholar]
  48. O’Dwyer DP, Phelan CF, Rakovich YP, Eastham PR, Lunney JG et al. Generation of continuously tunable fractional optical orbital angular momentum using internal conical diffraction. Opt Express 2010; 18: 16480–16485. [DOI] [PubMed] [Google Scholar]
  49. Berkhout GCG, Lavery MPJ, Courtial J, Beijersbergen MW, Padgett MJ. Efficient sorting of orbital angular momentum states of light. Phys Rev Lett 2010; 105: 153601. [DOI] [PubMed] [Google Scholar]
  50. Berkhout GCG, Lavery MPJ, Padgett MJ, Beijersbergen MW. Measuring orbital angular momentum superpositions of light by mode transformation. Opt Lett 2011; 36: 1863–1865. [DOI] [PubMed] [Google Scholar]
  51. Mirhosseini M, Malik M, Shi ZM, Boyd RW. Efficient separation of the orbital angular momentum eigenstates of light. Nat Commun 2013; 4: 2781. [DOI] [PubMed] [Google Scholar]
  52. Goodman JW. Introduction to Fourier Optics. Roberts & Company Publishers; 2005. [Google Scholar]
  53. Takeda M, Ina H, Kobayashi S. Fourier-transform method of fringe-pattern analysis for computer-based topography and interferometry. J Opt Soc Am 1982; 72: 156–160. [Google Scholar]
  54. Franke-Arnold S, Barnett SM, Padgett MJ, Allen L. Two-photon entanglement of orbital angular momentum states. Phys Rev A 2002; 65: 033823. [DOI] [PubMed] [Google Scholar]
  55. Torres JP, Deyanova Y, Torner L, Molina-Terriza G. Preparation of engineered two-photon entangled states for multidimensional quantum information. Phys Rev A 2003; 67: 052313. [Google Scholar]
  56. Torner L, Torres JP, Carrasco S. Digital spiral imaging. Opt Express 2005; 13: 873–881. [DOI] [PubMed] [Google Scholar]
  57. Chen LX, Lei JJ, Romero J. Quantum digital spiral imaging. Light Sci Appl 2014; 3: e153, doi:10.1038/lsa.2014.34. [Google Scholar]
  58. Torres JP, Alexandrescu A, Torner L. Quantum spiral bandwidth of entangled two-photon states. Phys Rev A 2013; 68: 050301. [Google Scholar]
  59. Restuccia S, Giovannini D, Gibson G, Padgett M. Comparing the information capacity of Laguerre–Gaussian and Hermit–Gaussian modal sets in a finite-aperture system. Opt Express 2016; 24: 27127–27136. [DOI] [PubMed] [Google Scholar]
  60. Jack B, Yao AM, Leach J, Romero J, Franke-Arnold S et al. Entanglement of arbitrary superpositions of modes within two-dimensional orbital angular momentum state spaces. Phys Rev A 2010; 81: 043844. [Google Scholar]
  61. Yan Y, Xie GD, Lavery MPJ, Huang H, Ahmd N et al. High-capacity millimetre-wave communications with orbital angular momentum multiplexing. Nat Commun 2014; 5: 4876. [DOI] [PMC free article] [PubMed] [Google Scholar]
  62. Pors B-J, Miatto F, Hooft GW, Eliel ER, Woerdman JP. High-dimensional entanglement with orbital-angular-momentum states of light. J Opt 2011; 13: 064008. [Google Scholar]
  63. Krenn M, Fickler R, Huber M, Lapkiewicz R, Plick W et al. Entangled singularity patterns of photons in Ince–Gauss modes. Phys Rev A 2013; 87: 012326. [Google Scholar]
  64. Mirhosseini M, Magaña-Loaiza OS, O’Sullivan MN, Rodenburg B, Malik M et al. High-dimensional quantum cryptography with twisted light. N J Phys 2015; 17: 033033. [Google Scholar]
  65. Bliokh KY, Bliokh YP, Sevel’ev S, Nori F. Semiclassical dynamics of electron wave packet states with phase vortices. Phys Rev Lett 2007; 99: 190404. [DOI] [PubMed] [Google Scholar]
  66. Padgett M, Bowman R. Tweezers with a twist. Nat Photonics 2011; 5: 343–348. [Google Scholar]

Associated Data

This section collects any data citations, data availability statements, or supplementary materials included in this article.

Supplementary Materials

Supplementary Materials
Click here for additional data file. (1.9MB, pdf)
Supplementary Movie
Click here for additional data file. (7.2MB, avi)

Articles from Light, Science & Applications are provided here courtesy of Nature Publishing Group

RESOURCES