Plasmonic nano-printing: large-area nanoscale energy deposition for efficient surface texturing

Abstract

The lossy nature of plasmonic wave due to absorption is shown to become an advantage for scaling-up a large area surface nanotexturing of transparent dielectrics and semiconductors by a self-organized sub-wavelength energy deposition leading to an ablation pattern—ripples—using this plasmonic nano-printing. Irreversible nanoscale modifications are delivered by surface plasmon polariton (SPP) using: (i) fast scan and (ii) cylindrical focusing of femtosecond laser pulses for a high patterning throughput. The mechanism of ripple formation on ZnS dielectric is experimentally proven to occur via surface wave at the substrate–plasma interface. The line focusing increase the ordering quality of ripples and facilitates fabrication over wafer-sized areas within a practical time span. Nanoprinting using SPP is expected to open new applications in photo-catalysis, tribology, and solar light harvesting via localized energy deposition rather scattering used in photonic and sensing applications based on re-scattering of SPP modes into far-field modes.

Introduction

Light-driven excitation of surface charge waves—plasmons¹—achieves light localization on surfaces and nanoparticles of metals and dielectrics² down to the deep-subwavelength nanoscale. This opens a new toolbox of instruments to engineer and tailor properties of light, its intensity and phase as the plasmons are back re-scattered into propagating light fields^{3, 4, 5}. In all those plasmon applications of metamaterials a reversible nature of surface plasmon-polariton (SPP) is at work, that is, a surface-bound charge-light wave is eventually scattered into a propagating far-field. Remarkable properties of plasmons⁶: their localization at nanoscale and coherency with the driving light field have not yet been explored in the field of nano-technology/fabrication, where there are no tools to deliver permanent modifications by direct writing using light. The lossy nature of plasmons which hampers range of applications^{7, 8} makes here an advantage for the nanoscale patterning. In all practical application, a large surface area coverage by uniform nanostructures is required and are expected to advance wide range of new applications.

Self-organized surface structures—ripples^{9, 10, 11, 12, 13, 14, 15}—first observed 50 years ago under laser irradiation of surfaces—have revealed a strong potential for applications^{15, 16, 17}. Sub-wavelength ripples^{18, 19} formed by exposure to ultra-short laser pulses expanded phenomenology of ripples into an interior of transparent materials²⁰. Several theoretical models have been proposed for subwavelength ripples on the surface and inner volume structuring capturing the important aspects of their formation^{20, 21, 22, 23, 24, 25} including prediction of surface plasmon polariton wave^{21, 23, 24}, which is here verified for the first time experimentally and by modeling.

Here, we show a plasmonic nanoprinting to scale up fabrication of ripples over areas of centimeters in cross section, which are required for most of applications achieved in a practical time span of ~1 h, as shown in Figure 1. In the case of transparent materials, the debated ripple formation mechanisms are resolved showing that surface wave model of an optically excited plasma on the inner interface between the substrate and plasma, accounts quantitatively for the experimentally observed ripple period. The smallest ripple period is observed at the smallest plasma reflection and is nanoimprinted on the interface between plasma and substrate by ablation. At higher irradiance, period is decreasing due to reflectivity of top layer plasma. It is shown that previously considered conditions at the plasma–air interface Re(ε*)<−1 and the optical breakdown Re(ε*)<0 cannot explain the experimental observations. Only when Re(ε*) ≈−n², where n is the refractive index of substrate a surface wave on the inner plasma–substrate interface accounts for observed ablated patterns and provides further insights into control of ripple formation by tailoring sub-surface electron density (hence the permittivity) to support a plasma wave for the required wavelength/period.

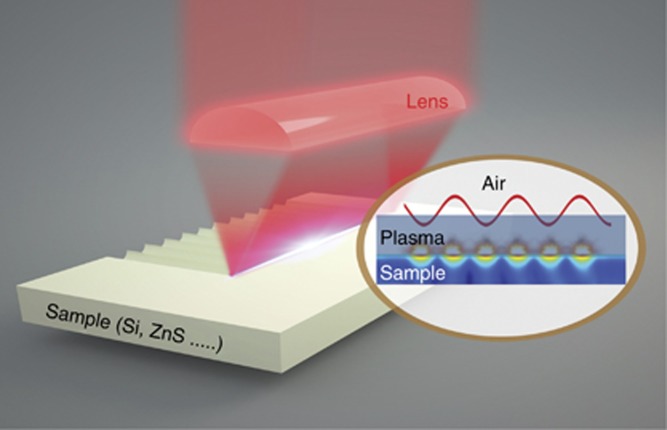
Figure 1.

Plasmonic nano-printing using an energy delivery by absorption of sub-surface sub-wavelength plasmons. Cylindrical lens and fast scanning improves of nano-pattern formation up to 2 × 10⁷ times as compared with the low-kHz repetition femtosecond lasers; typical aspect ratio length-to-width of the cylindrical focusing was 200.

Materials and methods

Sample fabrication

500-μm-thick p-type boron-doped single-side polished silicon wafers of <100> surface orientation (Atecom Technology Co., Ltd.) were used for large period ripple recording with 1030 nm/230 fs pulses. For sub-wavelength ripples, the β-ZnS was used. It has the complex refractive index n*=n−iκ≡(2.313−i10⁻⁴) (Ref. 26), which is related to the permittivity via 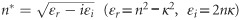. The number density of valence electrons is N_Zn=1 × 10²³ cm⁻³ considering Zn²⁺ valence state and 4 Zn atoms per unit cell volume a³=(0.5413 nm)³.

Optical properties

The permittivity of the optically excited plasma was calculated by²⁷:

where τ_D is the Drude damping time or the electron-phonon scattering time taken equal to the optical cycle of ~3 fs at the wavelength of irradiation. This assumption is used in plasmonics²⁸. The last term is reflecting the Drude contribution of free electrons with N₀ being valance band density of electrons with N₀=N_Zn for the results of ZnS discussed here. Details about calculations of plasma formation are presented in the Supplementary Information.

Laser fabrication

Ripples were recorded on surfaces of an absorbing Si and dielectric ZnS with 1030 nm/230 fs and 800 nm/150 fs laser pulses using cylindrical focusing (focal length<10 cm), as shown in Figure 1. Cylindrical lens was used in conjunction with standard microscope objectives for the final beam delivery onto the surface where an elongated elliptical focal spot with two major cross sections of large/small ratio larger than 10 was created. In the case of 1030 nm/230 fs pulses (Pharos, Light Conversion Ltd.), scanning at υ_s=(10–50) mm s⁻¹ was used at f=(0.1–0.5) MHz laser repetition rate keeping ~15% pulse-to-pulse spot overlay (or 10 pulses per micrometer) and a typical pulse energy of E_p=8 μJ on the sample. A cylindrical lens with focal length of 80 mm was used in front of the objective lens of numerical aperture NA=0.26. Cylindrical focusing formed a d_l=1.25 mm long and d_s=5 μm wide line (at 1/e² intensity level) on the surface of sample, that is, large/small≡d_l/d_s≈250. The ablation length was ~0.4 mm corresponding to the average 0.08 J cm⁻² fluence or 3.5 W cm⁻² intensity per pulse. For fabrication of patterns of ripples with continuous changes in angular orientation a λ/2 plate was synchronously rotated during linear scan (software solution by Altechna Ltd, Lithuanian). A strong air suction of ablation products was implemented; experiments were carried out in a class 1000 (ISO 6) cleanroom.

Results and discussion

Rapid fabrication of large-area structure surface by plasmonic printing

For ripples with periods close to the irradiation wavelength on the absorbing surfaces, the light interference between the incoming and the light scattered from a selvage region of surface is responsible for the ripples formation^{11, 12, 13}. The Fourier component of the surface scattered field close to the wavelength of irradiation form the strongest interference which leads towards the ripples by ablation. Ripples are formed in a multi-shot laser exposure with surface roughness evolving as laser beam is scanned over the surface. An effective refractive index of the surface is slightly smaller compared with that of the substrate due to roughness causing a correspondingly smaller ripple periods by the mechanism presented earlier^{11, 12}. The reduced reflectivity of ripple coated surface facilitates a stronger absorption and makes a positive feedback for ripple imprinting onto surface by ablation. An example of ripples with a close-to-the-wavelength period is shown in Figure 2 with a new record high speed of surface nanotexturing. A 4-inch wafer of Si was coated by ripples made by scanning a femtosecond laser beam with a high aspect ratio ~200 elliptical-focus. Fast linear scan of υ_s=5 cm s⁻¹ was matched with a high laser repetition rate of ƒ=0.5 MHz to fulfill the required condition of a strong overlap between adjacent pulses. In comparison with 1 kHz amplified fs-laser productivity, the throughput of surface texturing is increased by ~500 × 200²=2 × 10⁷ times. For absorbing materials ripples are induced by the interference of incoming and scattered light and this method can be adapted to any absorbing surface. By utilizing cylindrical focusing and rotation of linear polarization during scanning, a complex pattern of arbitrary ripples’ orientation can be obtained, as shown in Figure 2c for the case of a circular grating.

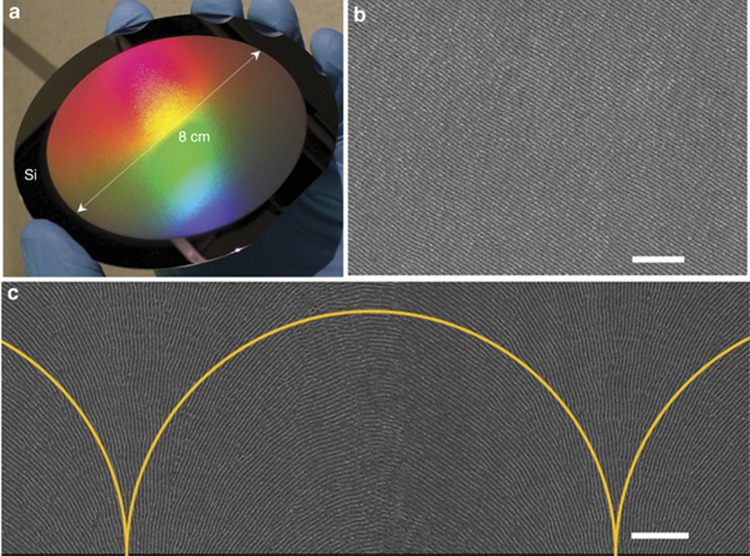
Figure 2.

Large period ripples on an absorbing Si surface made by 1030 nm/230 fs pulses. (a) Large (indefinite) area nanotexturing of Si surface by ripples using cylindrical focusing at 0.5 MHz repetition rate and 50 mm s⁻¹ scan speed; line focus had aspect ratio length/width≈200. (b) Closeup SEM view of the ripples; scale bar=10 μm. (c) Circular grating made by cylindircal focusing with linear polarization rotated at 2° μm⁻¹ using a υ=0.1 mm s⁻¹ linear scan speed; arcs mark tangential line to the ripples orientation. Scale bar=10 μm.

When the longer axis of an elliptical-focus is aligned to the E-field polarization of light and is perpendicular to the scan direction, ripples become more orderly. At such geometrical arrangement the ripple pattern seeded by initial pulses is self-replicated along the scan. The ordering has occurred on the absorbing Si and transparent ZnS substrates shown in Figures 2 and 3, respectively. This ordering is facilitated by simultaneous fabrication of large number of ripple periods fitting the long focus d_l/Λ≥100, which could extend over tens-of-micrometers and even to centimeters by optimized focusing of laser beam, as shown in Figure 3a and 3b and Supplementary Fig. S1. Ripples are formed in a multi-pulse irradiation mode and the scanning speed, υ_s, controls the number of pulses per spot N=ƒ (d_l/υ_s). As scan becomes slower, N increases and more ordered ripples are formed as revealed by Fourier analysis of the scanning electron microscopy (SEM) images (Figure 3c and 3d). This observation is consistent with recently demonstrated self-ordering due to anisotropic light scattering of defects in the sub-surface regions²⁵. The spatial overlap of pulses at the focus defines quality of the pattern and the wider the focal region, the faster scanning speed can be used to further increase the fabrication throughput. Laser polarization (linear) determined the direction of the deep-subwavelength structures (Supplementary Fig. S2a–S2g) and can be changed during scanning to obtain rotation patterns of ripples, as shown in Supplementary Fig. S2h. The depth of ripples can be controlled by wet etching, as shown in Figure 3e. Also, two-dimensional pattern of ripples can be obtained by criss-cross scanning (Figure 3f). It is worth noting that the fabrication area is determined by the length of the linear focus and the scan length capability of the used stage rather than by other fabrication conditions. Hence, such structuring has an indefinite area texturing capability highly sought after for industrial applications.

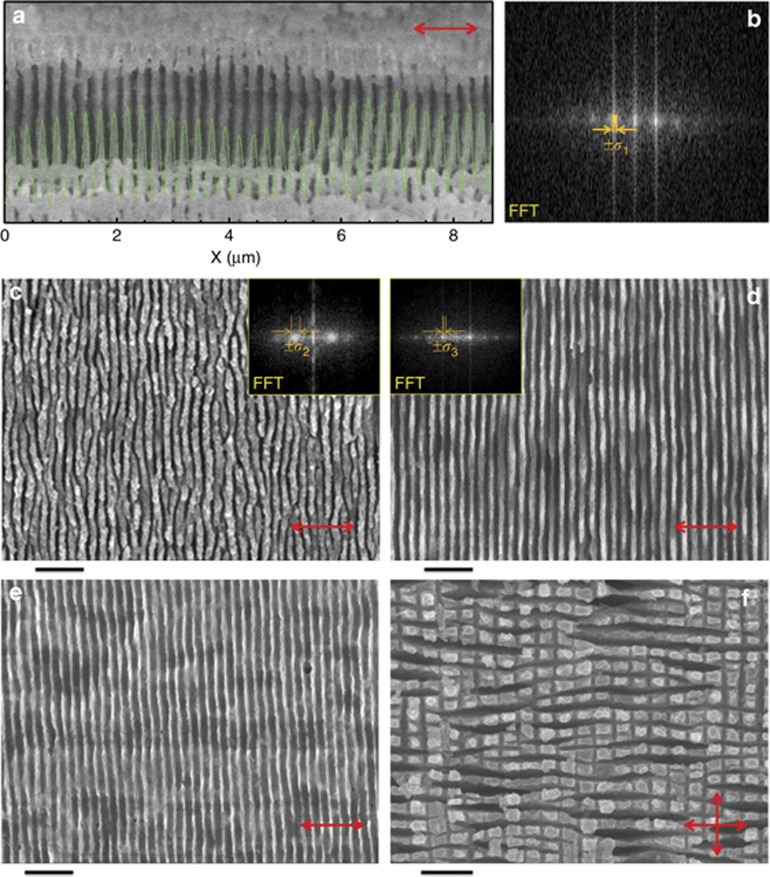
Figure 3.

The deep sub-wavelength period ripples on a dielectric surface. SEM images of ripples on ZnS made by 500 pulse irradiation using the cylindrical lens focusing without scanning. (a) The profile line shows ripples’ continuity; (b) the FFT image (right) shows the period and the standard deviation, ±σ. Ripples recorded at the lower scan velocities had better defined period: υ_s=5 μm s⁻¹ (c) and υ_s=0.2 μm s⁻¹ (d). Insets c and d show FFT images corresponding to Λ=207±10 nm c and 212±25 nm d; the error ±σ margins are shown. (e) Effect of mild etching in H₂SO₄ (pH≈1) solution for 10 min of the pattern shown in d. (f) Two-dimensional pattern of ripples obtained by criss-cross scanning. Irradiation conditions were: pulse intensity on sample I_p=6.67 TW cm⁻², wavelength of 800 nm, pulse duration of 150 fs, a cylindrical focusing, E-field was polarized perpendicular to the scan. A slight change of period for two directions is caused by an effective decrease of refractive index of the surface after the first ripples’ pattern was recorded. Scale bars c–f denote 1 μm; linear polarization is marker by arrow.

Mechanism of plasmonic printing inside volume

Formation of the sub-wavelength ripples on surfaces of dielectrics cannot be explained by the scattering theory^{11, 12} which accounts for the large wavelength-sized ripples on absorbing surfaces (see, Figure 2b and 2c). Coherent light scattering and resonant absorption are apparently responsible for formation of sub-surface (inner volume) ripples^{21, 23, 25}.

Analysis of sub-wavelength ripples on transparent β-ZnS is given next based on the surface plasmon polariton (SPP) surface wave excitation at λ=800 nm wavelength for the complex refractive index n*=n−iκ≡(2.313−i10⁻⁴)²⁶, which is related to the permittivity via n*=ε_r−iε_i (details in Materials and Methods section and Supplementary Information).

The surface wave can be excited on a metal or plasma interface with dielectric when¹: (i) the dielectric permittivity of plasma Re(ε_p*)<−n² (n=1 for the interface with air) and (ii) a phase matching between the surface wave (SPP) and the wavevector |k|=2π/λ of photons is fulfilled. It is noteworthy, that the phase matching defines the polarization sensitivity of the ripple orientation. The first condition is satisfied when plasma density becomes larger than the critical density for the wavelength of excitation of N_cr=1.745 × 10²¹ cm⁻³ at λ=800 nm. At the N_cr, a solid state plasma of free electrons in conduction band drives the dielectric breakdown within few optical cycles ∼6–10 fs and creates breakdown defined by Re(ε*)≡0. The cyclic plasma frequency of electrons oscillating collectively in the driving laser light field is related to the plasma density N_e by:

where ε₀ is the permittivity of vacuum, e is the electron charge, m*=m × m_e is the electron mass m_e factored by the dimensionless constant m<1, which accounts for the optical mass of electron in solid state material (m=0.4 for ZnS)²⁹; for the breakdown plasma m*=m_e. Since the SPP is coherent with light and is launched during the ultra-short laser pulse before the electron-ion thermalization which occurs in several picoseconds, an unperturbed value of m=0.4 is used for simulations. The optical electron mass is defined by the electron scattering mechanisms and their concentration and temperature dependencies³⁰.

The wave vector of the plasmon surface wave riding a conductive-dielectric interface along the linear laser polarization used in our experiments is given by³¹:

where k=2π/λ is the wavevector of the incident light, ε_d is the permittivity of the dielectric and ε* is that of plasma which was calculated for strongly excited ZnS²⁷ (see Materials and Methods section).

The phase matching between the plasmon surface wave k_s and incident light k can be achieved by either of scattering, diffraction from randomly occurring surface roughness, self-diffraction form the optically induced plasma, or via parametric scattering^{9, 10, 11, 12, 14, 22, 32}. The parametric phase matching was demonstrated for metals²² and shows an increasing period of ripples Λ with irradiance. This proves that coupling of a normally incident focused laser beam into a surface wave takes place.

Sub-wavelength ripples are formed by accumulation of consecutive pulses and control of the absorbed energy deposition and plasma formation on the surface. A standing surface wave can be formed when k_s matches the half of the Bragg wave vector of the surface structure period Λ^{1, 23}:

Hence, the period is Λ=λ_s/2 for ripples due to the localized electric field enhancement and absorption occurring at the maximums of the SPP standing wave. The local irradiance is exceeding the dielectric breakdown condition Re(ε*)<0 (Ref. 23). Equation (4) describes a generic condition of wave (phonons, electrons or photons) reflection at the edge of the first Brillouin zone in a periodic structure such as a crystal for phonons and electrons or a photonic crystal for photons¹. The wave reflection corresponds to the smallest wavelength of a propagating wave of the corresponding quasi-particle (phonon, electron, photon). The zone edge at the wave vector k=π/a along the direction of propagation for the pattern with a period a is equal to the wave vector, k_s=2π/λ_s (in the considered here case of plasmon wave) yielding in the period a=λ_s/2. From all the other possible propagating plasmons existing on the plasma surface for which the wave vector matching can be satisfied, the smallest period exists for the standing wave which is finally imprinted on the surface. The polarization of light defines the orientation of the standing wave and determines the ripple orientation as shown in Supplementary Fig. S2.

Imprint of surface wave starts from a coherent surface excitation occurring simultaneously with the light pulse absorption followed by ablation well after the end of the laser pulse. Surface has to be excited to form plasma with Re(ε*)<−n² to support the SPP wave on the plasma substrate surface. Any spatially small perturbation of the surface plasma will cause scattering (diffraction) with a Fourier component matching the standing SPP wave, hence, prevailing among other moving surface waves with different periods. The free carrier absorption pattern at the crests of the SPP wave makes deposition of energy, which, after the light pulse, drives the ablation of the above laying skin layer of plasma (see schematic illustration in Figure 1). When subsequent laser pulses are moving to the fresh sample surface by scanning, the existing ripples are translated over the newly excited surface which supports SPP wave of the already established period λ_s/2. This constitutes a self-replication and can be driven over indefinite areas similarly as shown for the large period ripples (Figure 2). Surface plasma layer has a skin depth for light intensity changing within 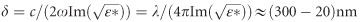at plasma densities ranging from N_cr to 10²² cm³ and only 30–10 nm for the conditions of sub-wavelength ripple recording (Supplementary Fig. S4).

The presented above mechanism was validated experimentally. Ripples were recorded on ZnS at different pulse energies, number of overlapping pulses and compared with period of the standing surface SPP wave prediction Re(ε*)=−n² for the (i) air–plasma (−n²=−1) and (ii) plasma–ZnS (−n²=−5.35) interfaces. Figure 4 shows the model predictions for the ripples’ period at the plasma–substrate interface. If ripples were formed via ablation on the air–plasma interface the periods around 300 nm would be expected but were never observed in experiments.

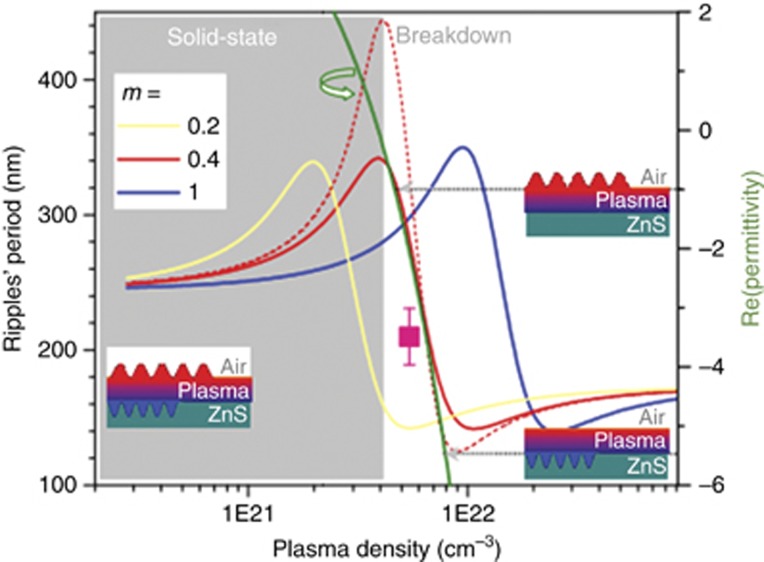
Figure 4.

Plasmonic excitation on the sub-surface plasma substrate boundary. The period of standing plasmon wave is equal to the half of the ripples’ period, Λ=λ_s/2 plotted for different plasma densities N_e, effective mass of electrons m*=m × m_e, and relaxation time of τ_D=3 fs close to an optical cycle (see Materials and Methods section and Supplementary Information for details). The right-side axis shows Re(ε*) dependence on N_e with horizontal arrows indicating surface wave condition at different interfaces Re(ε*)<−n² (pictorial markers); breakdown plasma at Re(ε*)=0. The experimental point corresponding to the excitation conditions shown in Figure 3 are is presented by square marker. Note, the plasma density is calculated by Equation (1) with electron mass m=1 and vacuum wavelength λ. The period (left axis) is calculated for the plasma–substrate interface; periods on the air–plasma interface; periods on the air–plasma interface are considerably larger and are not shown.

Figure 4 shows dependence of ripple period on the plasma density calculated by Equations (3) and (4) for different optical effective mass of electron in ZnS at λ=800 nm. The experimentally observed ripples (Figure 3d and 3e) had periods Λ_ex≈210±15 nm with a±σ span around the average estimated from FFT image analysis of SEM images. The experimentally observed ripples with periods from 120 to 220 nm fall into conditions when surface wave is formed on the inner interface between plasma and the substrate (Figure 4).

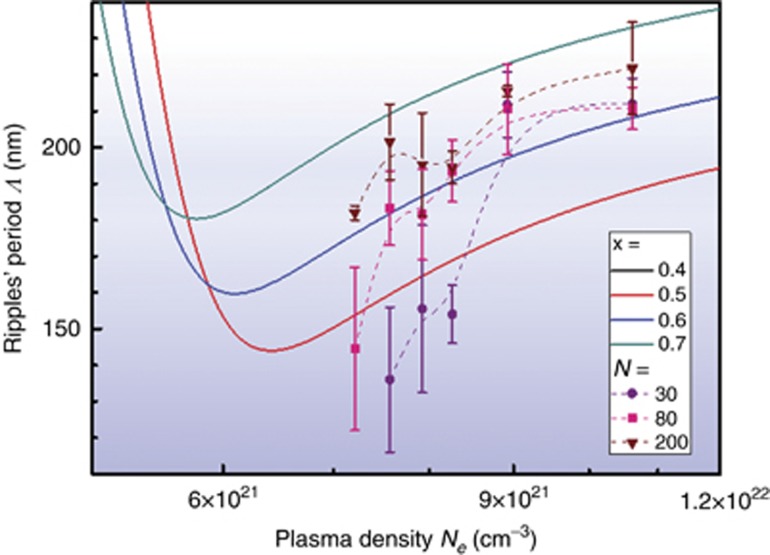
Experimentally determined ripple periods for the different number of pulses and pulse energies are plotted in Figure 5. The plasma density, N_e, was calculated from the known pulse intensity iteratively taking into account the actual time evolution of the Gaussian intensity envelop, I_p(t). The corresponding avalanche (impact), w_imp(t) and multiphoton, w_mpi(t), ionization rates at the instantaneous intensity were calculated together with temporal evolution of plasma screening due to the changing reflectivity, R(t), according to formulae given in Supplementary Information. This procedure allows to compare the experimental data with theoretical predictions earlier not attempted. Interestingly, the smallest periods Λ≈120 nm corresponds to the smallest reflectivity R or the lowest screening by plasma (Supplementary Figs. S3 and S4). As plasma density N_e grows (a larger pulse energy or pulse overlap), the period of ripples is evolving by prediction of the standing surface plasmon wave outlined above (Figure 4). Theoretical curves plotted for the different volume fraction, x, of air accounts for the actual reflectivity of the surface as ripples already were formed by the previous pulses, where effective permittivity is defined by that of material and air as ε_ef (x)=(1−x)ε+xε_air. The actual cross section of ripples is consistent with x=0.4–0.7 estimated by the air volume fraction of the actual ripples¹⁶. For the smaller number of pulses, N, per focal spot, smaller Λ were observed and corresponded to ripples with x=(0.4–0.5) judging from SEM images. For the larger N, a larger portion of surface was ablated and ripples had an air fraction x=(0.6–0.7). For the larger x values, surface becomes more anti-reflective and larger ripple periods are observed for the larger pulse energy due to lower electronic excitation reaching the plasma–substrate interface (a stronger plasma screening at the top air–plasma interface occurred).

Figure 5.

The ripple period, Λ, vs plasma density N_e calculated for different pulse energies and number of the overlapping pulses per spot, N. Theoretical curves are plotted for different ZnS fractions, (1−x), of an effective surface permittivity to account for surface ripple formation and the corresponding change the surface reflectivity; x is the fraction of air.

The tendency Λ∝N was confirmed and can be rationalized by a defect accumulation effect which is reflected in an increasing absorption Im(ε) of the substrate (Supplementary Figs. S3 and S4). This augmented absorption eventuates in higher values of N_e and Λ since the higher plasma densities at the air–plasma region are screening excitation at the plasma–substrate interface causing the larger Λ values as observed in experiment (Figure 5).

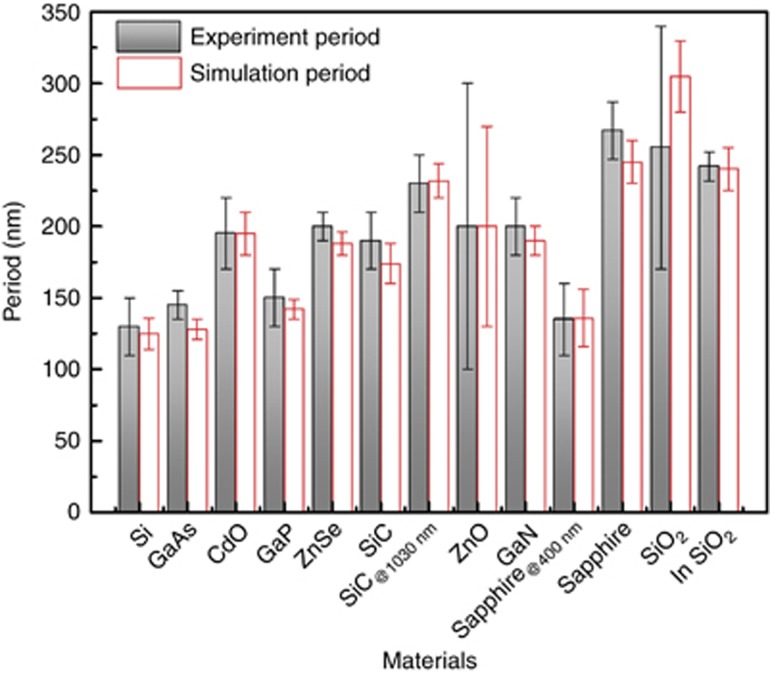
The nanoplasmonic imprinting model was also checked on other materials and compared with the reported experimental results. Shown in Figure 6 and Supplementary Table S1, deep-subwavelength structures were simulated according to the experimentally reported conditions on silicon³³, GaAs³⁴, CdO³⁵, GaP³⁴, ZnSe³⁶, SiC³⁷, ZnO³⁸, GaN²³, sapphire^{39, 40} and silica^{41, 42}, and predictions of herein outlined model had a good correspondence with the experimental observations (SiC and sapphire were structured at different wavelengths). The period calculated for silica (x=0) coincided with experimental results and validated the plamonic nano-imprinting model as universal to explain the origin of deep-subwavelength ripples.

Figure 6.

Deep-subwavelength Period simulation for different materials. Λ denotes the structure period in experiment while Λ_S is the simulation results.(Experimental data of silicon³³, GaAs³⁴, CdO³⁵, GaP³⁴, ZnSe³⁶, SiC³⁷, ZnO³⁸, GaN²³, sapphire^{39, 40} and silica^{41, 42}).

Conclusions

It is demonstrated that indefinite areas can be nanotextured with ripples with limitation defined only by surface processing speed proportional to the laser repetition rate. The use of a cylindrical focusing facilitates large area fabrication of ripples as shown for Si. Surface texturing at ~10 cm s⁻¹ speed required by industry⁴³ is already a feasible technology as shown here by ~10⁷ times improvement in surface texturing speed as compared with a low-kHz repetition laser fabrication. Augmented fabrication throughput in surface nanotexturing will impact applications in sensing, solar energy, fuel cells^{44, 45}.

It is determined that sub-wavelength ripples are formed via plasmonic nano-imprinting of the standing wave with period defined by the plasma density at the interface with the dielectric substrate. The model accounting for the actual electron generation rate simultaneously with evolution of plasma reflectivity is validated for ZnS and confirmed by experimental results on other materials. This shows how an absorptive nature of surface plasmonics waves is utilized for energy deposition with nanoscale precision and becomes an advantage in plasmonic nano-printing.

The presented analysis of ripple formation can be used to refine intricate electron scattering constants and their temperature and concentration dependencies in a strongly nonuniform and periodic density plasma which are currently known only approximately.