Measurements of Local Instantaneous Convective Heat Transfer in a Pipe - Single and Two-phase Flow

Abstract

This manuscript provides step by step description of the manufacturing process of a test section designed to measure the local instantaneous heat transfer coefficient as a function of the liquid flow rate in a transparent pipe. With certain amendments, the approach is extended to gas-liquid flows, with a particular emphasis on the effect of a single elongated (Taylor) air bubble on heat transfer enhancement. A non-invasive thermography technique is applied to measure the instantaneous temperature of a thin metal foil heated electrically. The foil is glued to cover a narrow slot cut in the pipe. The thermal inertia of the foil is small enough to detect the variation in the instantaneous foil temperature. The test section can be moved along the pipe and is long enough to cover a considerable part of the growing thermal boundary layer.

At the beginning of each experimental run, a steady state with a constant water flow rate and heat flux to the foil is attained and serves as the reference. The Taylor bubble is then injected into the pipe. The heat transfer coefficient variations due to the passage of a Taylor bubble propagating in a vertical pipe is measured as function of the distance of the measuring point from the bottom of the moving Taylor bubble. Thus, the results represent the local heat transfer coefficients. Multiple independent runs preformed under identical conditions allow accumulating sufficient data to calculate reliable ensemble-averaged results on the transient convective heat transfer. In order to perform this in a frame of reference moving with the bubble, the location of the bubble along the pipe has to be known at all times. Detailed description of measurements of the length and of the translational velocity of the Taylor bubbles by optical probes is presented.

Download video file ^{(60.3MB, mp4)}

Introduction

Numerous experimental studies of convective heat transfer, using different techniques to measure the wall and/or fluid temperature in a variety of flow configurations, have been performed during the last decades. One of the factors that limits the accuracy of temperature measurements in unsteady processes is the slow response of the sensors. To record local instantaneous wall temperature, the measuring equipment has to respond fast enough, while the surface at which the temperature is recorded has to be in thermal equilibrium with the time-dependent flow. Thus, the thermal inertia of the surface has to be sufficiently small. The relevant time scales are determined by the hydrodynamic phenomena that cause the change in the convective heat transfer. Fast time response is thus crucial for recording the time-dependent temperature in transient flow.

To meet these requirements, an IR camera is used to record a special self-manufactured test section that allows a fast temperature response to any change in the flow. A part of the pipe wall is cut off and replaced with a thin stainless-steel foil. A similar approach was used by Hetsroni et al.1, however, the foil they used was too thick to accurately measure changes of instantaneous temperatures and only time-averaged temperatures were presented. Decreasing the foil thickness improved the time response considerably.2 This method was applied in the lab to measure convective heat transfer coefficients in two-phase flow3,4 and transient phenomena in single phase pipe flow5.

A schematic layout of the two-phase flow facility is given in Figure 1, additional information on the unique air inlet device can be found in Babin et al.3

Investigation of convective heat transfer in two-phase flow is very complex due to the transient flow behavior and the effect of void fraction in the pipe cross-section. Therefore, many studies have only presented an average convective heat transfer coefficient for a given flow regime as a function of specific flow conditions6,7,8,9,10,11. However, the papers by Donnelly et al.12 and Liu et al.13 represent examples of two-phase local convective heat transfer studies.

The present study deals with heat transfer measurements around a single elongated (Taylor) bubble injected into stagnant or flowing liquid in a pipe. The Taylor bubble propagates in a constant translational velocity14,15,16. The bubble propagation velocity is determined using optical probes method consisting of a laser light source and photodiode3,4.

The combination of the IR camera and of the optical probes allows measurements of the local instantaneous convective heat transfer as a function of the distance from either the Taylor bubble top or bottom.

The instantaneous wall temperature can be used to calculate the convective heat transfer coefficient, h, and the Nusselt number:

,          (1)

where q is the heat flux to the foil, T_w and T_∞ are the wall temperature and the inlet water temperature respectively, k is the liquid conductivity and D is the pipe diameter. The bulk temperature which is commonly used to determine the heat transfer coefficients was not measured in order to avoid introducing any interference to the flow.

Protocol

1. Test Section for Measurements of Instantaneous Temperature

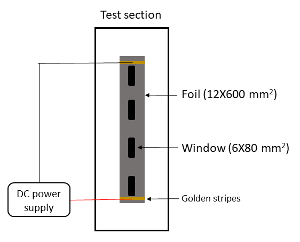
1. Manufacturing process of test section ( Figure 2 )

   1. Cut a segment of a pipe at least 70 cm long. Note: The diameter and wall thickness of the test section should be identical to that of the pipe used in the experimental facility.
   2. Use a milling machine to cut 4 adjacent narrow windows along the pipe in the test section, each window is 6 mm wide and 80 mm long with a 25-mm gap between consecutive windows.
   3. From a 12 µm thin stainless-steel foil, cut 40-60 cmlong and 12 mm wide strips. Note: The foil will be used to seal the windows in the test pipe. The stainless steel should be coated with two gold stripes that mark a small distance from the ends of the foil and are to be used to weld the power supply wires to heat the foil, see Figure 2.
   4. Measure the foil's electrical resistance using an ohmmeter.
   5. Place the foil within the pipe following the steps described below.

      1. Create a basis for inserting the foil into the test section made of a rigid tube with an outer diameter equal to that of the test pipe inner diameter. To this end, cut a 20 mm wide and about 80 cm long part of the pipe wall
      2. Coat the basis with grease before placing the foil on it, to allow disconnecting the basis from the foil at a later stage.
      3. Place the foil on the basis and flatten it. Take a cloth with alcohol and clean the foil from any excess grease.
      4. Apply the adhesive on the foil periphery and on the three segments of the foil corresponding to the location of the bridges between the consecutive windows. Note: The adhesive has to be strong with an initial hardening period of at least 20 minutes in order to ensure sufficient time for the foil attachment process; epoxy DP 460 is used.
      5. Carefully insert the basis with the foil into the test section with the foil facing upward toward the windows. Note: This process should be done by two people.
      6. Ensure that the foil is aligned with the windows, the gold stripes should be at the edge of the two external windows. Once the foil is properly placed and covers the windows, link the basis to the test pipe at each end by using a clamp.
      7. Carefully insert a folded bicycle inner tire into the pipe under the basis, grease the tire if needed. Inflate the tire. During the inflation observe the adhesive spreading across the foil and reaching the basis. Note: The pressure applied by the inflated tire should guarantee that the adhesive will tie the pipe inner wall and the foil. This will also shape the foil to the curvature of the pipe and reduce any possible interference to the flow due to the foil or the adhesive.
      8. With a cloth, clear any excess adhesive that has reached the window openings.
      9. Let the adhesive dry for 24 h. Deflate the tire, extract it and open the clamps.
      10. Disconnect the basis from the foil and pipe in the following steps:

          1. Gently touch each end of the basis to feel which end is easier to disconnect and begin disconnecting from that end.
          2. Use a long roller or a similar object to disconnect the original basis gradually, move slowly into the pipe until the entire basis is disconnected and the foil remains safely undamaged on the pipe. Note: This process should be done carefully in order to protect the seal; if the seal is ruined a new heat transfer test pipe unit should be made.
          3. Check that the pipe is sealed by closing one end of the pipe and filling the pipe with water.
   6. Clean the excess grease from the inner side of the pipe with water and soap.
   7. Connect the electrical heating wires by welding to the golden stripes on the foil. Note: To protect the foil at these spots, it is suggested to first link a small cooper chip to the foil and then weld the wire to it.
   8. With a thermal adhesive connect a T-type thermocouple to the bottom of each window. These thermocouples will be later used in the IR camera calibration process. Note: The temperature readings from the thermocouples are recorded and recorded by a PC using an A/D converter.
   9. Spray paint the outer side of the foil with a black mat spray in order to maximize the emissivity.
2. Applying heat flux to foil

   1. Connect the electrical heating wires from the edge of the foil to a DC power supply.
   2. Set the electrical current so the foil will reach a desired temperature. Note: Do not reach a temperature that could impair the pipe. For Plexiglass the limit is about 45 °C. However, be sure to heat the foil enough to ensure that the foil's temperature remains at least a few degrees over the inlet water temperature, even during the cooling state due to the transient changes expected in the flow.
   3. Calculate the heat flux applied Q = I²R where I is the current applied and R is the foil's electrical resistance. Note: The heat transfer from the foil's outer side that is open to air is negligible compared to the heat transfer to water inside the pipe2.
   4. Connect a PC controllable electrical switch to one of the heat flux wires in order to control the heat flux impulsive initiation and shutdown.
3. IR camera Note: Detailed specifics of the IR camera used in the lab can be seen in Ferhstman3,4. The camera is connected to a PC and controlled by a computer.

   1. If possible, connect the IR camera to a set of rails enabling three-dimensional movement of the camera to easily place it at different positions along the test section.
   2. Turn on the IR camera a few minutes before conducting any measurements, the internal sensors take time to cool down to the required temperature.
   3. Place the IR camera a few centimeters from the surface within the focal distance of the camera to enable focusing. Note: Depending on the camera resolution be sure that the area measured is not smaller than a single pixel. It is preferable to have the surface area measured consisting of a number of pixels.
   4. Set focus of the IR camera.
   5. IR camera calibration process:

      1. Apply heat flux as stated in 1.2 and wait until a thermal steady state is reached, i.e. once the thermocouples placed on the foil record constant temperature. Measure the ambient temperature in the vicinity of the experimental facility with a thermocouple. Note: This temperature will be used as the reflected temperature of the surface. For a high value of surface emissivity, this parameter is almost negligible.
      2. Enter the ambient temperature as the IR camera's parameter of reflected temperature.
      3. For each window in the foil, compare the temperature recorded by the IR camera to the recording of the thermocouple of that window. Adjust the emissivity property of the IR camera until the temperature recording of the IR camera is equal to the temperature recorded by the thermocouple. Note: This can be done for several heat flux values; however, the emissivity is not sensitive at this relatively low-temperature range. In the experimental recordings, the average value of emissivity was 0.98.

2. Measurements of Taylor Bubble Translational Velocity and its Length

1. Optical probe Note: The optical probe includes a laser light source and a photodiode. When the entire cross-section of the pipe contains water, the laser beam is pointed at the photodiode causing the circuit to be closed. When the laser beam hits the air bubble, it is nudged from the photodiode and opens the circuit. Thus, a binary signal is obtained, indicating whether the optical probe is in front of an air bubble or of a liquid slug.

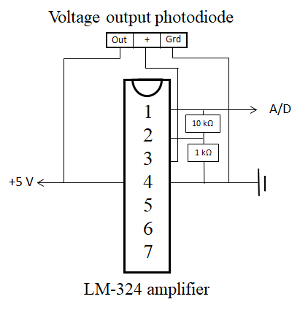
   1. In order to connect the sensor to the A/D card, build the following circuit (Figure 3).
   2. Turn on the laser, point it at the diode. Check the digital input of the circuit A/D software. If the laser hits the diode, then the circuit is closed and a positive signal should appear. Note: Make sure that the diode is sensitive to the wavelength output of the laser. The optical sensors' data is recorded at the rate of 1 kHz.

3. Experimental Procedure

1. Connect the test section to the experimental facility, by flanges or pipe records.

2. Connect the heating electrical wires to the power source and the thermocouples to any computerized temperature recorder.

3. Place the IR camera in front of the test section at the desired location.

4. Make sure that the camera is focused on the foil; this is easier to do with the heat flux on.

5. Apply heat flux (1.2) and perform the IR camera calibration process (1.3.5).

6. Place the two optical probes along the test pipe Note: If possible, place one optical probe at the location of the IR camera; this will allow synchronization between the temperature measurement and the location of the bubble, as the bubble is not visible to the IR camera. If not possible, be sure to shift the results in time accordingly to the bubble velocity and the distance between the IR camera and the optical probe.

   1. Check that the laser beam indeed hits the diode and yields a positive reading.

7. Turn on the water pump and set the desired flow rate. Apply heat flux by adjusting the current of the power supply.

8. Wait until a steady state temperature is attained. Execute the computer program to record the wall temperature of a single-phase pipe flow. Note: In order to isolate the effect of the Taylor bubble on the convective heat transfer coefficient, it is essential to measure first the convective heat transfer coefficient in single-phase flow at an identical flow rate and heat flux as in the two-phase experiments. It is recommended to perform this before each individual event.

9. Run the computer program that governs the bubble injection. Note: This program should first inject a single Taylor bubble and then simultaneously measure the wall temperature using the IR camera and record the binary signals of the two optical probes. These two measurements should be synchronized in order to avoid additional time shifts between the recordings.

10. Between the runs, set sufficient time delay to ensure that the system is back to the initial conditions, i.e. the wall temperature returns to the initial single-phase steady-state value. Note: It is possible that the wall temperature of the single-phase steady state flow will increase in cases where a high heat flux is on continuously. This should be avoided by closing the heat flux and taking a break in the experiments.

4. Data Processing

1. Calculate the heat transfer coefficient for single-phase steady state conditions based on eq. 1. Note: This coefficient is independent of time. It will be used as a normalizing factor in the sequel.

   1. For each individual event recorded under given conditions, calculate the Taylor bubbles' length and translational velocity by dividing the distance between the optical probes, L, by the time period interval of the tip arrival time to each optical sensor: 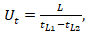 , where t_L1 and t_L2 are the times of the bubble tip arrival to the bottom and upper optical sensors, respectively.
   2. Use the Taylor bubble's translational velocity to calculate the bubble length by multiplying it by the duration of the open circuit of one of the optical probes.
2. Calculating the instantaneous local heat transfer coefficient for two phase flow should be done as following: Note: Although the entire experimental process is computerized, the length and the translational velocity of the Taylor bubbles do not remain exactly constant at each experimental run. Therefore, the instant at which the bubble reaches the measuring point varies. The optical probes and the IR camera have different frame rates, 1000 and 30 Hz respectively.

   1. Using the optical probe located in the vicinity of the IR camera, record the instant of the bubble tip arrival.
   2. This trigger signal provides the time reference for the IR camera recording. Note: Ensemble average process is required in order to obtain the convective heat transfer coefficient as a function of the distance from the bubble bottom to the measuring point, z, where z=0 corresponds to the bubble bottom. This parameter should be normalized by the pipe diameter D.
   3. Set a resolution for z/D. All recorded data values for each individual event (corresponding to identical conditions) fall within the prescribed spatial resolution range and should be averaged to obtain a single representative value of the wall temperature at that distance from the bubble bottom. Note: This spatial resolution should be decided based on the recording frequency of the IR camera and on the translational velocity of the Taylor bubble. In this study, it was taken between 0.15-0.3.
   4. Calculate the heat transfer coefficient as a function of the distance from the bubble tip/bottom using eq. 1.
   5. Normalize the two-phase local instantaneous heat transfer coefficient by the single-phase constant coefficient. Note: This ratio represents an improvement in convective heat transfer rate due to the bubble passage compared to single-phase flow.

Representative Results

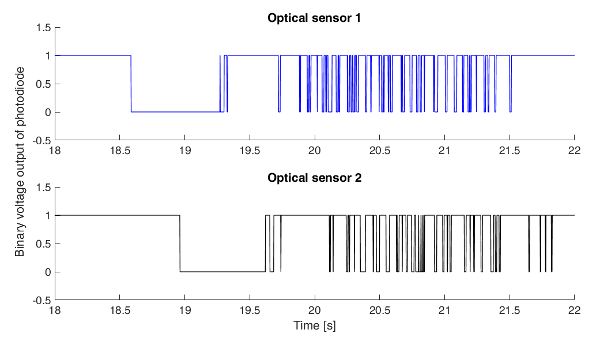
An example of the optical sensors output records is presented in Figure 4 for a single Taylor bubble rising in a vertical pipe filled with stagnant water. The initial large drop represents the opening of the circuit due to the Taylor bubble tip, while the later much shorter drops following the rise to the initial value due to the passage of the elongated bubbles tail, represent the dispersed bubbles in the liquid wake behind the Taylor bubble. The time shift between the outputs of the two optical probes is evident and is due to the distance between the two probes along the pipe. In this experiment, the probes are space by 0.09 m. Calculating the translational velocity by eq. 3 results in U_t=0.23 m/s; in agreement with Dumitrescu13 for a Taylor bubble propagating in a vertical pipe with stagnant water: The Taylor bubble length is measured by multiplying the translational velocity by the duration of the passage of the elongated bubble: which corresponds to L_B = 3.54D.

The representative ensemble-averaged results of the local convective heat transfer coefficient due to the passage of a 3.5D long single Taylor bubble rising in stagnant water in a vertical pipe are plotted in Figure 5. The results are presented in a frame of reference moving with the bubble bottom, therefore the negative values up to z/D=-3.5 correspond to the bubble region where a thin film separates between the bubble and pipe wall. The two-phase flow convective coefficients results are normalized by the single-phase flow coefficient value. It is evident that the maximum increment in the heat transfer convective coefficient is attained a few diameters behind the bubble bottom and can be as much as two times higher compared to the single-phase flow at the same flow rate. Furthermore, the effect of the Taylor bubble on the wall temperature has a prolonged effect, remaining essential up to hundreds of diameters behind the Taylor bubble bottom. This is attributed to the wake behind the bubble. These results serve as a clear demonstration of the growing interest in two-phase flow as a cooling mechanism.

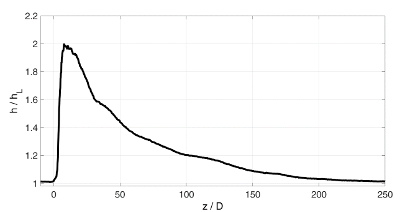
Figure 1.Schematic layout of the experimental facility with heat transfer measurements test section. Details of the air and water entrance section are presented in the insert.Please click here to view a larger version of this figure.

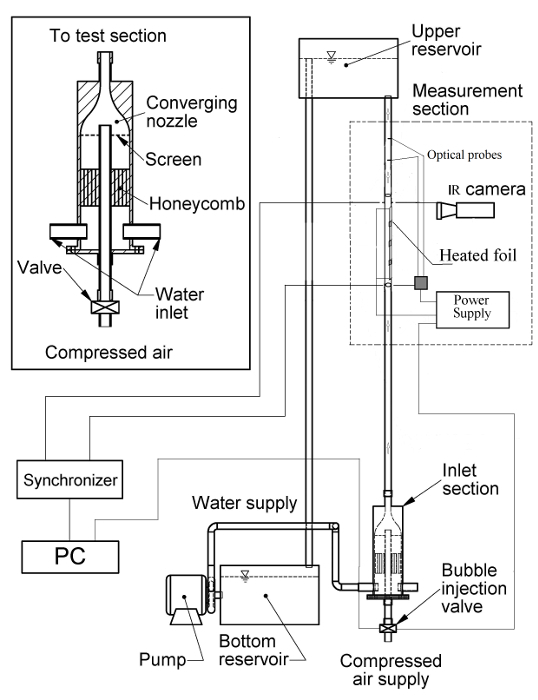
Figure 2. A schematic layout of the test section. Please click here to view a larger version of this figure.

Figure 3 . The optical sensor electrical circuit connecting between the diode and the A/D card linked to the PC. Please click here to view a larger version of this figure.

Figure 4 . The optical sensor recording for a Taylor bubble rising in stagnant water. Please click here to view a larger version of this figure.

Figure 5. Normalized local heat transfer coefficients along a single slug unit for stagnant liquid (q=2100 W/m²). Please click here to view a larger version of this figure.

Discussion

Experimental investigation of local heat transfer in transient pipe flow is a complicated task that requires high-end measuring instruments and methods, as well as a custom-built experimental facility, in particular, a specially designed test section. The present protocol displays a thermography technique that is capable of faithfully measuring fast temporal changes in wall temperature and in heat transfer rate due to variations in flow hydrodynamics.

A detailed description of the manufacturing process of the test section is presented. The critical step in the preparation of the facility is replacing a part of the pipe wall by a thin stainless-steel foil. The foil is heated by an electrical current; its inner side is open to the time-dependent flow field, while the outer side is filmed by an IR camera thus detecting any change in the instantaneous foil temperature. The temporal response of the foil constitutes the only limitation of this technique. The material and the thickness of the foil should be selected to ensure sufficiently fast time response as compared to the characteristic times of the phenomena considered.

The method applied allows instantaneous IR camera-based heat transfer measurements relative to the moving Taylor bubble as determined by optical means. An ensemble averaging procedure over numerous realizations of the experiment for any given operational conditions applied in the present study ensures obtaining reliable results. The proposed technique can be used for characterization of local transient heat transfer in single and multiphase flows.