Hybrid T2- and T1-Weighted Radial Acquisition for Free-Breathing Abdominal Examination

Abstract

Purpose

Most clinical MR examinations require acquisition of different image contrasts. For abdominal exams, the scans are conventionally performed as separate acquisitions using respiratory gating or repeated breath holding, which can be time-inefficient and challenging for patients. Here, a hybrid imaging approach is described that creates T2- and T1-weighted images from a single scan and allows for free-breathing acquisition.

Theory and Methods

T2-weighted data is collected using 3D fast spin-echo (FSE) acquisition with motion-robust radial stack-of-stars sampling. The wait time between the FSE trains is used to acquire T1-weighted gradient-echo (GRE) data. Improved robustness is achieved by extracting a respiratory signal from the GRE data and using it for motion-weighted reconstruction.

Results

As validated in simulations and phantom scans, GRE acquisition in the wait time has minor effect on the signal strength and contrast. Volunteer scans at 1.5 Tesla showed that T2- and T1-weighted hybrid imaging is feasible during free-breathing. Furthermore, it has been demonstrated in a patient that hybrid imaging with T1-weighted Dixon acquisition is possible.

Conclusion

The described hybrid sequence enables comprehensive T2- and T1-weighted imaging in a single scan. In addition to free-breathing abdominal examination, it promises value for clinical applications that are frequently affected by motion artifacts.

INTRODUCTION

Most clinical MR examination protocols require acquisition of multiple sequences with different image-contrast weighting. For instance, abdominal MR examinations typically include separate T₂- and T₁-weighted scans to allow for detection and proper classification of liver lesions (1–4). T₁-weighted contrast is, in most cases, obtained using phase-encoded 3D gradient-echo (GRE) sequences. Because the acquisition time is on the order of 10-20 seconds, these scans are best performed during suspended respiration, which can be a challenge when imaging pediatric, elderly, or sick patients.

T₂-weighted contrast in liver MRI at our institution is routinely obtained with single-shot 2D fast spin-echo (FSE) sequences (e.g., HASTE) without fat suppression (5,6) in combination with fat-suppressed 2D FSE sequences. Because acquisition time is long for the latter, these scans need to be performed either using multiple breath-hold maneuvers, multiple averages, or navigator techniques that monitor the respiration and acquire data only in a certain respiratory state (7). However, navigator-triggered acquisition prolongs the examination significantly and fails quite often in clinical practice, particularly when patients suddenly change their breathing pattern. This can either result in residual motion artifacts or completely non-diagnostic images.

It has been shown that radial k-space sampling provides inherent robustness to motion and allows for free-breathing acquisition during shallow respiration (8). Clinical application has been demonstrated for free-breathing T₁-weighted acquisition (9) as well for dynamic contrast-enhanced acquisition (10,11). It has also been applied for T₁-weighted fat/water separation (12). The combination with T₂ weighting, however, is not straightforward. When using a conventional 2D FSE acquisition scheme (13,14), the overlap of the radial projections in the k-space center leads to an undefined image contrast because all projections acquired along the FSE echo train contribute equally to the contrast. Therefore, the images show a mixture of proton-density (PD) weighting and T₂ weighting. This problem can only be circumvented by using k-space filtering techniques (14,15) or model-based reconstruction (16,17).

An alternative for applying radial sampling to T₂-weighted imaging is to perform a 3D FSE acquisition with a radial stack-of-stars trajectory (18), which uses radial sampling in the k_x-k_y plane and standard Cartesian sampling along the k_z direction. This geometry allows placing the echo train along the Cartesian direction so that the k-space center is acquired at a defined echo time to create consistent T₂ weighting of the images. A limitation, however, is the long acquisition time of several minutes (18), which is necessary because of the volumetric excitation and the need to wait for sufficient recovery of the longitudinal magnetization. To reduce this inefficiency, it has been suggested to use part of the waiting time to acquire an additional image contrast (19).

In this work, we build upon this idea and describe an approach for time-efficient hybrid T₂- and T₁-weighted free-breathing MR examination of the abdomen. The T₂-weighted data is obtained using a radial stack-of-stars 3D FSE acquisition while T₁-weighted data is acquired by inserting a radial stack-of-stars 3D GRE module into the waiting time of each echo train of the FSE sequence. Moreover, it is shown that alternatively a Dixon-type module can be inserted to generate fat/water-separated T₁-weighted images (12). To further improve the robustness of the approach, it is demonstrated that respiratory-motion information can be extracted from the GRE module. The breathing signal can be used to reduce associated blurring and streaking artifacts in both the T₂-weighted and T₁-weighted images. The influence of the hybrid acquisition on the signal strength is investigated in simulations using the extended phase graph (EPG) framework (20–22). Phantom measurements are shown for validation purposes, and in-vivo results are shown for free-breathing abdominal volunteer and patient scans at 1.5T.

THEORY

T₂-Weighted Radial 3D FSE Sequence

The basic sequence timing diagram and k-space sampling scheme of the radial 3D FSE sequence are shown in Figure 1 and Supporting Figure 1. All partitions in the k_z plane for one projection angle are acquired with linear ordering during a single echo train. Therefore, all projections on each k_z partition level have consistent T₂ weighting, resulting in an effective T₂-weighted contrast with echo time TE given by the time between the excitation pulse and sampling of the central k-space partition. This scheme is repeated with repetition time TR, each time acquiring a different projection angle. The acquisition of projections in the k_x-k_y plane follows the golden-angle scheme, which rotates consecutive projections by 111.246° to achieve approximately uniform k-space coverage over time (23).

Fig. 1.

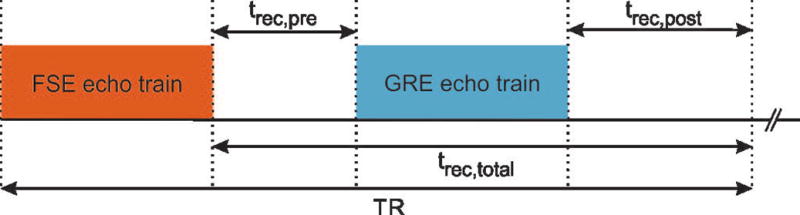
Sequence timing diagram of the hybrid approach, consisting of an FSE and a GRE module.

To avoid high-intensity streaking artifacts from fat, each echo train starts with spectral fat saturation using an inversion recovery (SPIR) RF pulse and subsequent gradient spoiling. Use of a SPIR pulse was necessary because a conventional chemical-shift selective (CHESS) pulse did not provide sufficient fat suppression across the image. A slab-selective 90° RF pulse is used for excitation, followed by a rapid refocusing train (24). Non-selective RF pulses are used to achieve short echo spacing. The flip angle of the initial refocusing pulse is 180°. The remaining RF pulses use a fixed flip angle of 120° to reduce energy deposition. Each refocusing RF pulse is surrounded by crusher gradients to suppress spurious free induction decay (FID) signal contributions. At the end of each echo train, spoiler gradients are applied to dephase residual transversal magnetization. The first echo train of the 3D FSE acquisition is discarded to avoid transient effects.

To improve conspicuity of lesions, blood signal in hepatic vessels can be suppressed by inserting small motion-sensitizing diffusion gradients around the initial refocusing pulse, as indicated in Supporting Figure 1. (14,25). Respective b-values are calculated with the Stejskal and Tanner equation (26) assuming rectangular gradient shapes and neglecting the influence of all imaging gradients. While leading to clearly better lesion conspicuity, the diffusion gradients can also result in some loss of signal strength due to the free-breathing acquisition. Therefore, the strength of the diffusion gradients can be adjusted to match the preference of the reader.

Hybrid T₂-/T₁-Weighted Acquisition

FSE sequences conventionally require a wait time (t_rec,total) after each echo train to allow for recovery of the longitudinal magnetization. For 2D FSE sequences, this can be achieved without significant dead time using interleaved multi-slice acquisition. However, for single-slab 3D FSE sequences, such as the one used here, interleaved acquisition is not possible, which makes the acquisition inefficient.

To improve efficiency, it has recently been suggested to use the wait time for acquisition of complementary image information (19). Utilizing this idea, a GRE module with both RF and gradient spoiling has been inserted between the FSE trains to acquire T₁-weighted data. Because the GRE imaging is performed with small flip angles (usually ≈ 5-15°) (27), the impact on the magnetization recovery and consequently the signal strength of the T₂-weighted FSE data is minor. Analogous to the FSE module, radial stack-of-stars sampling is used such that all partitions for one projection angle are acquired in each repetition (9). Projections are acquired according to the golden-angle ordering scheme.

Two different variations of the GRE module were implemented: First, a fat-suppressed acquisition using a frequency-selective saturation (CHESS) pulse at the beginning of each GRE block (shown in Supporting Figure 1), and second, the previously described Dixon radial volumetric encoding (Dixon-RAVE) method (12), which acquires three echoes within each TR_gre. To improve k-space coverage for the latter, subsequent echoes are rotated by 2° using gradient blips, which are applied between the bipolar readout gradients. A CHESS pulse was used for the fat-suppressed GRE module because it provided good suppression in shorter time but use of a SPIR pulse would be possible as well.

Detection of Respiratory Motion

As shown previously (9), radial stack-of-stars sampling is inherently more robust to motion than phase encoding, which translates motion into aliasing or “ghosting” artifacts that spread along the phase-encoding direction. Due to the non-Cartesian sampling geometry, ghosting artifacts cannot appear with radial acquisition, which makes free-breathing acquisition possible. However, in the case of deep respiration, artifacts such as blurring or streaking remain visible. Several strategies exist to address these artifacts, including self gating, extra-dimensional reconstruction (28), or soft gating (29–31). All these methods require a respiratory signal, which can be extracted from the k-space data.

For the hybrid acquisition, both the FSE and GRE modules can provide motion information. However, the FSE signal tends to be more sensitive to high-intensity streak artifacts originating from the arms. Therefore, the GRE data is used instead to extract a respiratory signal, which is then utilized for reconstruction of both the FSE and GRE images.

The calculation of the respiratory signal is illustrated in Supporting Figure 2a. First, the real and imaginary parts of the center sample of each projection are concatenated for all acquired partitions and coils, resulting in a matrix with N_proj columns and N_par × N_coil × 2 rows. Second, a principal component analysis (PCA) is performed along the concatenated dimension. When the signals are ordered by increasing projection angle, rather than temporally ordered, it can be seen that all components show a dependency on the angle, caused by eddy-current and gradient-delay effects (Supporting Figure 2b). To remove this contamination (32), the first 10 principal components are processed with the second order blind identification (SOBI) algorithm (33), which is an independent component analysis (ICA) method to separate signals from different sources (Supporting Figure 2c).

The respiratory signal is then found as one of the components, while the other components can be attributed to experimental factors such as gradient delays. The frequency spectrum of the components allows for identification of the respiratory signal. When calculating the entropy of the power spectra (Supporting Figure 2d), a jump occurs at the respiratory component. Therefore, the respiratory signal can be detected as the first component where the entropy gradient exceeds its mean value.

Image Reconstruction

For scans without significant respiratory motion, the T₂- and T₁-weighted images can be reconstructed by performing a standard non-uniform fast Fourier transform (NUFFT). If respiratory information should be included or if Dixon-RAVE fat/water separation is desired, the reconstruction can be formulated as the following inverse problem:

$$\underset{x}{\mathrm{argmin}}{\displaystyle \sum _{c,t}{\Vert \mathrm{\Lambda }(\mathrm{A}{x}_{\mathrm{c},\mathrm{t}}-y_{\mathrm{c},\mathrm{t}})\Vert }_2^2}$$ [1]

where y_c,t are the acquired k-space samples and x_c,t the image(s) to calculate. C and t denote the channels and the time. Selection of the forward operator A depends on the acquisition type. For the FSE module and the GRE module with fat suppression, A is just the NUFFT operator, implemented as convolution with a Kaiser-Bessel window. For the Dixon-RAVE module (12), A is an operator that transforms the fat/water maps to k-space based on a six-peak model for the fat spectrum (34) and accounts for off-resonance blurring of fat (35). A B₀ field map is pre-calculated using an unwrapping algorithm (36) and kept constant during the optimization.

To compensate for respiratory-motion artifacts, a diagonal matrix Λ is included that weights the contributions of the projections according to the estimated respiratory signal. Projections in the end-expiratory state are assigned a weight of 1, and with increasing distance on the respiratory curve, weights are decreased exponentially (30,31). Parallel imaging is incorporated by including a multiplication with the coil-sensitivity maps into the forward operator, which are estimated using the adaptive combination technique (37).

All image reconstructions were performed in Matlab (The MathWorks, Natick, MA) using the NUFFT toolbox by Fessler and Sutton (38). The optimization problem (Eq. [1]) was solved using the limited-memory Broyden-Fletcher-Goldfarb-Shanno (lBFGS) algorithm (39) with 3 iterations. A PCA-based channel compression was applied to reduce the computational complexity (40), compressing the multi-channel data into only 8 eigenmodes. To enable parallel processing of multiple slices, an inverse FFT was applied along the k_z partition direction, which disentangles the problem along k_z. On a server with two 16-core Intel Xeon CPUs and 256 GB RAM, all iterative reconstructions finished in less than 15 min.

METHODS

Simulations

To investigate how the proposed hybrid acquisition influences the signal behavior compared to separate 3D FSE and 3D GRE acquisitions, simulations were performed with the EPG concept (20,21). Five different tissue types were simulated using T₁/T₂ values from literature for 1.5 T (41,42): liver (586/46 ms), spleen (1057/79 ms), kidney cortex (966/87 ms), kidney medulla (1412/85 ms), and hepatic cyst (691/371 ms).

The following parameters were used for simulation of the FSE acquisition: echo train length (ETL) = 64, echo spacing (ESP) = 4.42 ms, TE of first echo TE_first = 12.50 ms, TR = 1200 ms, flip angle (FA) of the excitation pulse = 90°, FA of the first refocusing pulse = 180°, FA of the remaining refocusing pulses = 120°. The available wait time between the last refocusing pulse at the start of the next FSE train was t_rec,total = TR − ETL ∙ ESP - TE_first − t_spir = 769.62 ms, where t_spir = 135 ms is the time between the SPIR pulse for fat suppression and the excitation pulse.

Different parameter settings were simulated for the GRE module. First, TR_gre = 3.91 ms and FA_gre = 12° were used. For the hybrid approach, the GRE module was placed in the center of the wait time t_rec,total and 64 echoes were simulated to match the ETL of the FSE acquisition and therefore the actual sequence implementation. For simulation of separate GRE, 1000 echoes were chosen to ensure that a steady-state has been reached. To investigate the influence of FA_gre, TR_gre, and the placement of the GRE module, the simulations for liver tissue were repeated while one of the parameters was varied. Parameter ranges were FA_gre = {4;8;12;16;20}°, TR_gre = {2;4;6;8;10} ms, and t_rec,pre = {50;200;350;500} ms where t_rec,pre denotes the time between the last refocusing pulse of the FSE module and the first RF pulse of the GRE module. When changing TR_gre and FA_gre, the GRE module was centered in the available wait time.

Imaging Experiments

All imaging experiments were performed using 1.5 T scanners (Magnetom Aera, Siemens Healthineers, Erlangen, Germany).

Phantom Scans

To analyze the signal and contrast behavior of the hybrid approach, a NIST phantom (High Precision Devices, Boulder, CO) was scanned with FSE only, GRE only, and the hybrid FSE/GRE acquisition. All measurements were performed with a 20-channel head coil using a field-of-view (FOV) of 350×350×224 mm³ and a matrix size of 256×256 pixels. Sixty-four slices in the axial orientation and 400 radial projections were collected. The following parameters were used for the FSE acquisition: TR/TE_eff = 1200/151 ms, TE_first = 12.50 ms, ETL = 64, ESP = 4.42 ms, FA of refocusing train = 120°, readout bandwidth = 390 Hz/px. The GRE acquisition used: TR_gre/TE_gre = 3.91/1.81 ms, FA_gre = 12°, readout bandwidth = 480 Hz/px. Parameters of the hybrid FSE/GRE scan were identical to the separate acquisitions. The GRE module was placed in the center of the available wait time by choosing t_rec,pre = t_rec,post = 270 ms. Total scan time of the separate GRE scan was 1:59 min. Scan time of the separate FSE and the hybrid FSE/GRE acquisition were 8:03 min each.

SNR maps were calculated using the multiple pseudo replica approach (43). Separately acquired noise samples were used to generate 100 synthetic noise maps. These noise maps were added to the measurements and NUFFT reconstructions were performed for each of the 100 resulting datasets. Pixel-wise SNR values were obtained by dividing the original image by the standard deviation over the 100 reconstructed datasets. Additionally, average SNR was calculated in several regions of interest containing 36 pixels each.

In Vivo Experiments

All in vivo studies were HIPAA compliant and approved by the Institutional Review Board. Written informed consent was obtained from each subject.

Hybrid Liver Scan

A free-breathing liver scan of a healthy volunteer was performed using a 32-channel spine coil array in combination with an 18-channel body coil array for signal reception. Acquisitions were done in FSE, GRE, and hybrid FSE/GRE mode using a FOV of 350×350×224 mm³, matrix size of 256×256 pixels, 64 axial slices, and 400 radial projections. Other imaging parameters were identical to the phantom scans, resulting in a scan time of 1:59 for separate GRE acquisition and 8:03 min for FSE and hybrid FSE/GRE acquisitions. To demonstrate the option for blood-signal suppression, the separate FSE and hybrid FSE/GRE acquisitions were repeated with additional diffusion gradients with an amplitude of 19 mT/m and a duration of 2 ms, which applied an empirically chosen b-value of 0.67 s/mm² in three orthogonal directions.

Images and SNR maps were reconstructed with conventional NUFFT. The hybrid FSE/GRE dataset was additionally reconstructed with the iterative approach after setting the weights of the matrix Λ to 1 (no motion weighting) and again after setting the weights based on the respiratory curve (motion weighting).

For comparison, a clinical T₂-weighted navigator-triggered 2D FSE “prospective acquisition correction” (PACE) scan was performed with the following parameters: FOV = 350×350 mm², matrix size = 256×256 pixels, 32 axial slices, slice thickness = 5 mm, slice distance factor = 20%, TR/TE_eff = 1200/99 ms, FA of refocusing train = 120°, ESP = 5.22 ms, turbo factor = 19, readout bandwidth = 391 Hz/px, SPIR fat suppression, GRAPPA acceleration factor = 2. These images were reconstructed using the manufacturer’s algorithm on the MR system.

Hybrid Dixon Liver Scan

A free-breathing hybrid FSE/Dixon-RAVE acquisition was performed in the same volunteer. Three echoes were acquired with blipped bipolar multi-echo readout and the following parameters: TR_gre = 6.16 ms, TE_gre,1/TE_gre,2/TE_gre,3 = 1.38/2.93/4.48 ms, and readout bandwidth = 810 Hz/px. Additional diffusion weighting (b = 0.67 s/mm² per axis) was applied in the FSE module. All other parameters were kept identical, maintaining an acquisition time of 8:03 min.

To generate water and fat maps, Eq. 1 was solved using the previously described model-based approach (12). The respiratory signal for motion weighting was extracted from the first Dixon-RAVE echo, which was also used for motion-weighted reconstruction of the T₂-weighted FSE data by solving Eq. 1.

To investigate the motion robustness of the proposed technique, additional volunteer scans were performed for different degrees of respiration. For each volunteer, three FSE/Dixon-RAVE datasets were acquired during which the volunteers were asked to perform regular, irregular, and deep breathing for the duration of the scan. Here, no additional diffusion weighting was applied. All other imaging parameters were identical to the scan described above. Image reconstructions were performed both without and with motion weighting.

Patient Hybrid Liver Scans

Hybrid T₂-weighted/Dixon-RAVE acquisition during free-breathing was performed in a clinical patient (female, 63 years). The FOV was adjusted to 390 × 390 mm² and the slice thickness to 3 mm. All other parameters were identical to the volunteer scan. As part of the clinical procedure, 1 mL of Gadobutrol (Gadavist; Bayer Healthcare LLC, Whippany, NJ) was dissolved in water and administered intraorally. No additional contrast agent was injected before the hybrid scan. Motion-weighted reconstruction was performed using Eq 1.

RESULTS

Simulations

Figure 2 shows the simulated signal-time curves for different tissue types. As expected, the signal levels for both the FSE and the GRE modules are affected by the hybrid acquisition. When using linear reordering and comparing the signal levels at the center of the FSE module, the attenuation caused by the hybrid acquisition is 22.3% for liver, 28.8% for spleen, 28.2% for kidney cortex, 30.9% for kidney medulla, and 25.6% for the cyst. For the GRE module, the signal is reduced significantly when looking at the beginning of the echo train. However, GRE imaging is conventionally performed in a steady-state and not during the initial transient state. Therefore, a later time point should be considered for making the comparison. When using linear partition reordering and comparing the center of the hybrid GRE module to the steady-state signal of the separate GRE module, the signal of the hybrid acquisition is increased by 30.6% for liver, 36.9% for spleen, 37.0% for kidney cortex, 38.0% for kidney medulla, and 34.1% for the cyst. The dotted line in the hybrid panel indicates the signal time curve for separate FSE and GRE acquisition of liver tissue.

Fig. 2.

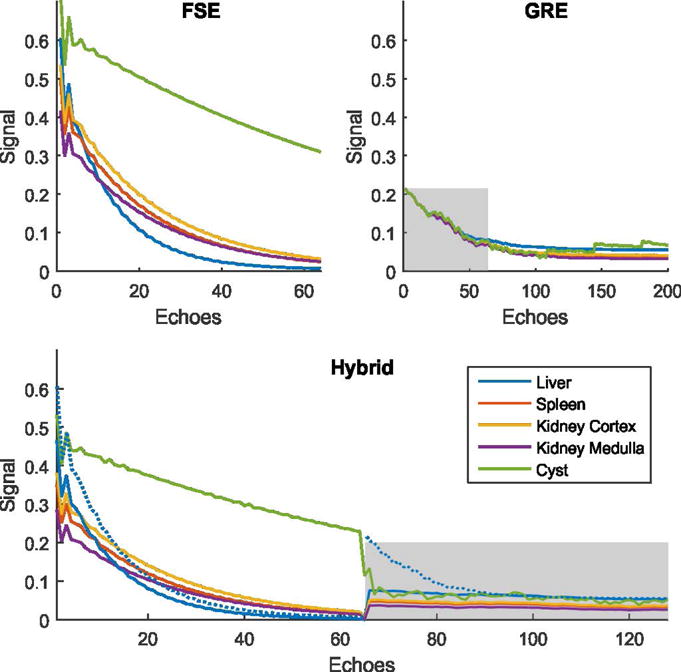
EPG simulations of signal time courses. The gray boxes indicate the GRE module in the hybrid panel and the first 64 echoes, which is the length of this module, in the separate GRE panel. The FSE signal in the hybrid approach is attenuated because the time for recovery of the longitudinal magnetization is reduced by the GRE acquisition. The degree of attenuation depends on the underlying tissue type and varies from 22.3% to 30.9%. Because, with the hybrid approach, GRE data is acquired in the transient state, a signal increase can be seen ranging from 30.6% to 38.0% when comparing the center of the hybrid GRE module with the steady-state signal of the separate GRE acquisition. To facilitate comparison, the signal time courses of separate FSE and GRE acquisitions of liver tissue are additionally shown as dotted line in the hybrid panel.

The influence of different parameter options for the GRE module is summarized in Figure 3. With increasing FA_gre, the attenuation of the FSE signal increases, while the effect on the GRE signal varies depending on the tissue relaxation time. Varying the repetition time of the GRE module has almost no effect on the FSE signal, while the effect on the GRE signal depends again on the tissue type. When varying the position of the GRE module, the smallest attenuation of the FSE signal is obtained when the GRE module is placed right after the FSE module, i.e. with minimum t_rec,pre. In contrast, the attenuation of the GRE signal is lowest when placing the GRE module at the end of the wait time, i.e. with maximum t_rec,pre.

Fig. 3.

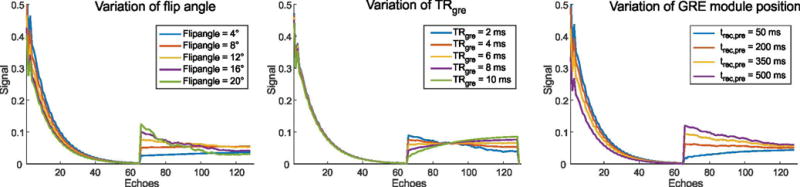
Simulation of the influence of different parameters on the GRE signal of the hybrid imaging technique, shown for liver tissue (T₁/T₂ = 586ms/46ms).

Phantom Scans

Figure 4 shows the calculated SNR maps from the phantom measurements. When comparing the signal strength between separate and hybrid acquisitions, an overall attenuation of the FSE signal can be seen, as expected, which ranges from 3.6 % to 36.2 % for different vials. In contrast, the overall level of the GRE signal is higher for the hybrid measurement. Here, the relative increase ranges from 28.4 % to 46.4 %. This indicates that the degree of SNR change varies for the different vials and, thus, depends on the underlying relaxation times, which is consistent with the findings from the simulations.

Fig. 4.

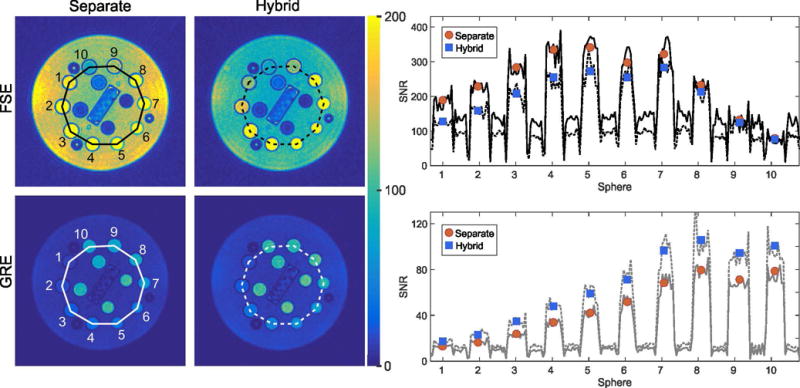
Calculated SNR maps of the phantom measurements. The circles and squares in the line profiles show the average SNR in different vials. While the hybrid approach leads to decrease of the SNR for the T₂-weighted FSE image, the SNR of the T₁-weighted GRE image is increased.

Free-Breathing Hybrid Liver Scan

Figure 5 shows NUFFT reconstructions of the separate FSE and GRE scans as well as the proposed hybrid FSE/GRE acquisition. Overall image appearance and contrast behavior are comparable. Regions of interest drawn in the SNR maps (Supporting Figure 3) confirm the expected signal changes for the hybrid approach. In particular, a signal decrease for the hybrid FSE image of 24.7%, 24.2%, 27.1% and 25.8%, was found for liver, spleen, kidney cortex, and kidney medulla, while the signal increase for the hybrid GRE image for these tissues was 29.6%, 39.9%, 26.9%, and 31.4%, which is in line with both the simulations and the phantom scans.

Fig. 5.

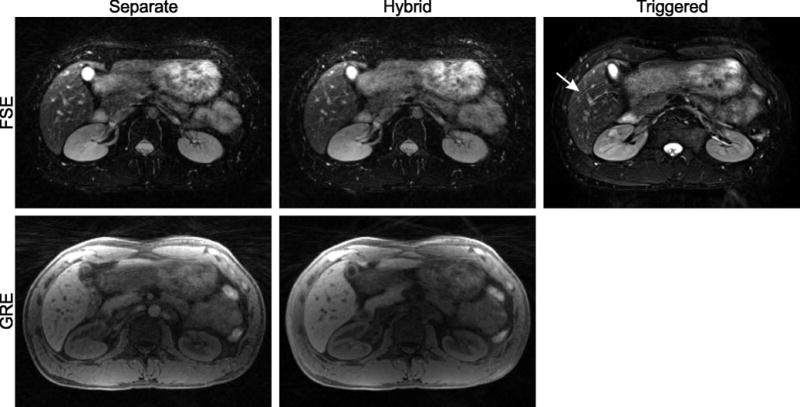
Free-breathing abdominal scan of a volunteer using separate FSE and GRE acquisitions (left column) and hybrid FSE/GRE acquisition (center column). A navigator-triggered 2D FSE acquisition is shown as reference (right column). Diagnostic image quality is achieved with all approaches. Residual respiration leads to slight motion artifacts in the navigator-triggered 2D FSE scan (arrow). Only minor differences are visible between the separate and hybrid free-breathing acquisition.

A further difference in the GRE contrast is that the aorta appears bright in the separate acquisition. This effect is caused by inflow of unsaturated spins into the imaging slab, which appears commonly with slab-selective steady-state acquisition. Because the hybrid scheme uses non-selective refocusing RF pulses together with gradient spoiling, also the areas outside of the imaging slab get saturated, which suppresses most of the inflow effects.

All radial images show a slight drop of the signal intensity towards the center of the subject (Figures 5–9), caused by B₁ inhomogeneity in the RF receiver profiles. When calculating images directly on the scanner, these intensity modulations get automatically corrected (“prescan normalize”). However, the correction has not been implemented in the Matlab offline reconstruction. As reference, a clinical T₂-weighted navigator-triggered 2D FSE scan is shown, which is affected by typical motion artifacts for phase-encoded sequences (arrow) while the overall image quality is quite comparable.

Fig. 9.

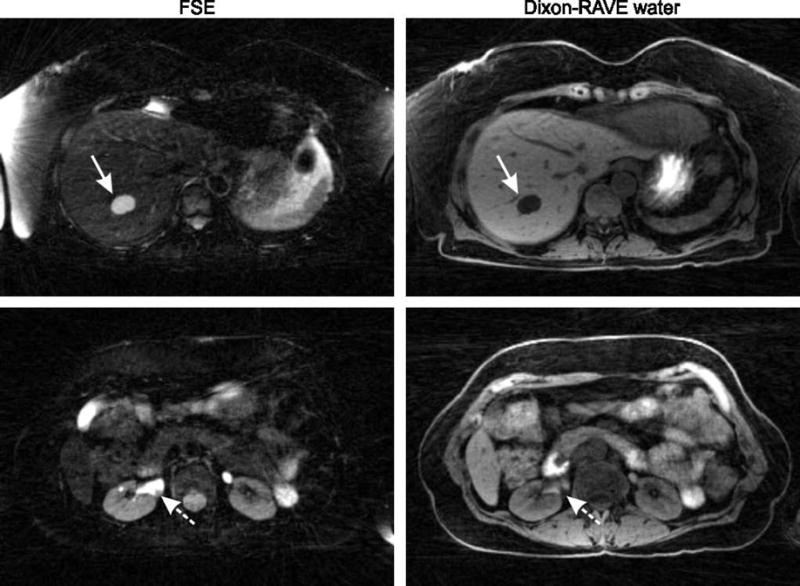
Two sections from a free-breathing hybrid FSE/Dixon-RAVE scan of a patient, revealing a large hepatic cyst (arrows, first row) and a septated hemorrhagic cyst in the right kidney (dashed arrows, second row).

Figure 6 demonstrates the effect of adding stronger diffusion weighting to suppress blood vessels, which clearly improves conspicuity of the cyst in the liver of the volunteer. The suppression works equally well for the separate and hybrid FSE acquisition. It is accompanied by a slight loss of signal strength in the liver parenchyma, likely caused by occurrence of respiratory motion during the diffusion weighting.

Fig. 6.

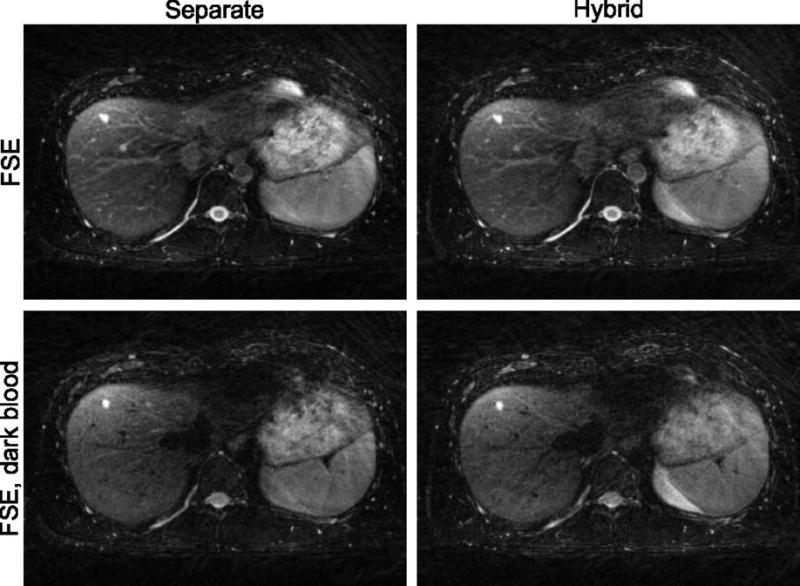
Blood signal in vessels can be suppressed by adding small diffusion gradients (b = 0.67 s/mm²) to the FSE acquisition (second row). This results in improved conspicuity of liver cysts and is applicable to both the separate FSE (left column) and the hybrid acquisition (right column).

The respiratory signal extracted from the GRE module of the hybrid acquisition is shown in Figure 7. The color of the curve indicates the weight used for motion-corrected reconstruction. Although acceptable image quality is already achieved without motion correction (no motion weighting, left column), the cyst (arrow) is blurred along the superior-inferior direction. When motion weighting is added to the reconstruction (right column), blurring is reduced and improved delineation of the cyst is achieved.

Fig. 7.

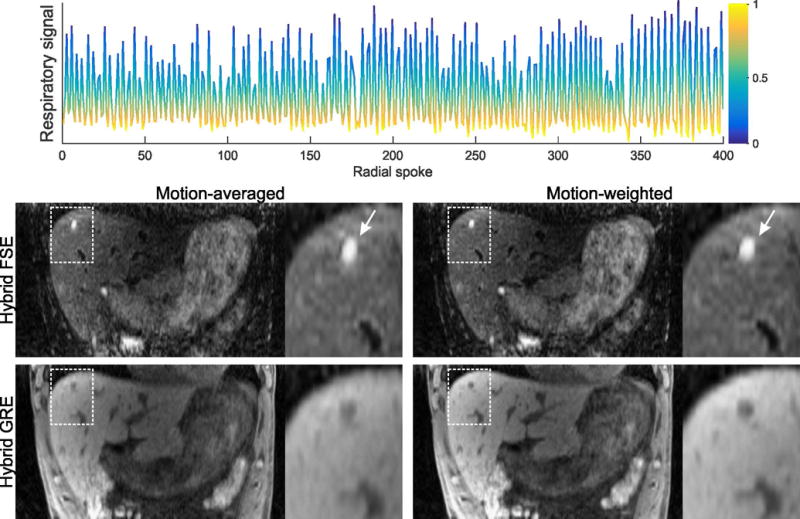
Respiratory curve extracted from the GRE k-space data with the algorithm described in the theory section. This signal was used to assign weights between 0 (end-inspiration) and 1 (end-expiration), as indicated with different colors. When motion-averaged reconstruction is performed (left column), blurring occurs due to respiratory motion and results in smudging of the cyst in superior-inferior direction. By using motion-weighted reconstruction, the blurring is reduced and the cyst appears with improved sharpness.

Hybrid Dixon Liver Scans

Figure 8 shows volunteer results from the hybrid FSE/Dixon-RAVE scan, generating T₂-weighted images, water-only T₁-weighted images (“fat-suppressed”), fat-only images (“water-suppressed”), and synthetically generated T₁-weighted images in the in-phase and opposed-phase condition from a single 8:03 min free-breathing acquisition.

Fig. 8.

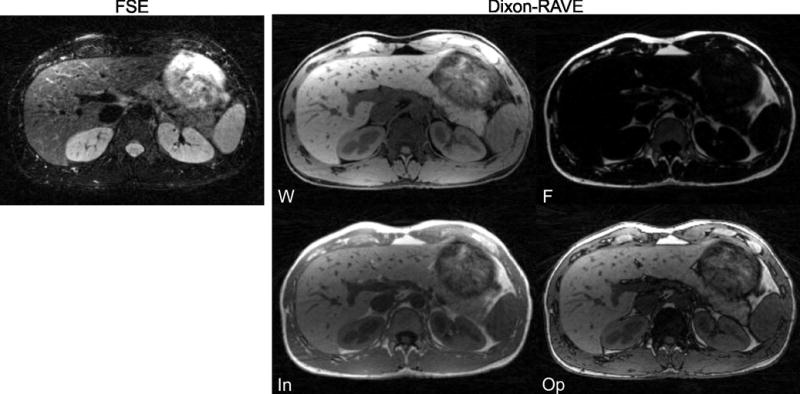
Hybrid FSE/Dixon-RAVE acquisition of a healthy volunteer during free breathing. Shown are the T₂-weighted FSE contrast and T₁-weighted water (W), fat (F), and synthetically generated in-phase (In) and opposed-phase (Op) contrast, all obtained from a single 8:03 min scan.

Results from two volunteer scans with different breathing instructions are shown in Supporting Figure 4. With the motion-averaged reconstruction, image quality for irregular and deep breathing is degraded due to blurring and streaking artifacts. With motion-weighting, these artifacts are reduced and the image quality is comparable to the scan acquired during regular breathing.

Patient Hybrid Liver Scans

Results from the patient examination can be seen in Figure 9, revealing a benign hepatic cyst on the upper slice and a septated hemorrhagic cyst in the right kidney on the lower slice.

DISCUSSION

Conventional abdominal MR examination involves acquisition of a series of differently weighted imaging sequences during repeated breath-hold maneuvers, with multiple averages, or navigator triggering. This makes the examination time-inefficient, complex, and sensitive to artifacts related to incomplete suspension of respiration. To address these limitations, a hybrid imaging technique has been described that provides T₂- and T₁-weighted images from a single free-breathing scan by synergistically combining multiple previously proposed concepts, such as combined 3D FSE and GRE acquisition, radial stack-of-stars sampling, and self-navigated motion-weighted reconstruction.

As shown in simulations (Figure 2) and phantom measurements (Figure 4), the hybrid scheme leads to somewhat modified signal levels for both contrast types. In a conventional FSE sequence, recovery of the longitudinal magnetization occurs during the wait time between two consecutive echo trains. When a GRE train is inserted, the magnetization recovers with modified relaxation time during the influence of the GRE train and towards a lower steady-state value (44). Consequently, the magnetization available at the start of the next TR is reduced, resulting in decreased signal strength of the hybrid FSE acquisition. This signal decrease did not influence the diagnostic utility of the hybrid FSE contrast. Nevertheless, it could be more problematic when acquiring data with much higher spatial resolution, which, however, is rather uncommon in clinical abdominal imaging. When comparing the signal reduction in the hybrid FSE module to the signal reduction of a separate FSE acquisition with reduced TR, simulations have indicated that similar signal strength can be obtained by reducing the TR by 30%. While this value is not insignificant, the proposed hybrid technique additionally offers the advantages of perfectly registered contrasts and improved workflow.

In contrast to FSE data, the signal strength of the GRE data is increased compared to separate acquisition. In conventional GRE scans, k-space lines are acquired during steady-state after driving the magnetization into equilibrium with a train of preparation excitations. In the proposed hybrid approach, GRE data are primarily acquired in the transient state, starting from the magnetization available after the FSE echo train and subsequent waiting time t_rec,pre. This results in an overall stronger signal (Figure 2), particularly for tissues with longer T₁ values because the difference between the steady state and the transient state is higher, but also in a signal modulation along the GRE train that translates into blurring. However, the effective impact on the image contrast is minor and was not noticeable in the in-vivo experiments. One explanation is that the trajectory acquires all partitions for one radial projection with a single GRE train, thus placing the signal modulation in the through-plane direction. Therefore, the magnetization state along k_z is identical for all projections, which avoids visible in-plane artifacts that would arise when mixing differently weighted data from the transient phase (45). Nevertheless, if artifacts became noticeable, steady-state preparation methods could be applied to mitigate the effect (46).

Signal changes between the hybrid technique and the separate acquisitions were consistent in simulations, phantom measurements, and in-vivo experiments. Noteworthy, the signal change for different relaxation times such as liver, spleen, and kidney is similar and therefore, contrast differences are only subtle.

The strength of the signal change caused by hybrid acquisition depends on several sequence parameters and can be influenced by optimizing the values. With increasing flip angle FA_gre, both the apparent relaxation time T₁* and steady-state signal of the GRE module are reduced (44), and the available magnetization at the beginning of the FSE module is decreased. The choice of TR_gre has only minimal influence on the FSE signal, which is consistent with previous findings (19). Extending TR_gre comes at almost no cost and, therefore, low readout bandwidths can be used in the GRE module to maximize SNR. Alternatively, multiple echoes can be acquired within each TR, as done in the Dixon-RAVE module. As the number of acquired echoes is only limited by the available wait time t_rec,total, acquisition of multiple echoes is possible with no increase in total scan time. This could be exploited for quantitative fat/water imaging applications, such as T₂* quantification and accurate proton-density fat-fraction estimation (47,48).

The position of the GRE module was investigated as a third parameter. Here, an opposite effect exists for the FSE and GRE signal: With shorter t_rec,pre, the recovery of magnetization after the FSE train is reduced, leading to signal decrease in the transient phase of the GRE module. During the GRE module, the magnetization approaches a steady-state independent from t_rec,pre. Therefore, a longer t_rec,post leads to increased signal of the subsequent FSE train. Even when the steady-state is not reached at the end of the GRE module (as in Figure 3), the remaining signal difference is small and compensated for by the longer recovery period. All of these effects are inverted by selecting higher t_rec,pre, leading to reduced FSE and increased GRE amplitude.

All measurements shown in this work were acquired using a fixed refocusing angle for the FSE train. Alternatively, variable flip-angle schemes can be employed, which allow lengthening the echo train while maintaining high signal (49–51). In addition, flip-angle modulation can be used for lowering RF power deposition and the specific absorption rate (SAR) (52). However, the use of variable flip angles comes at expense of increased T₁ contribution to the image contrast, which results in modified image appearance relative to standard T₂-weighted FSE images (49). For example, muscle tissue appears hyperintense. While the effect can be reduced by using a T₂ preparation module (53), our radiologists prefer the contrast obtained with fixed flip angle for abdominal examination. At a field strength of 1.5 T, this is possible without SAR constraints for up to relatively high flip angles, while the use at higher field strengths might require implementation of flip-angle modulation. However, such variable flip-angle scheme leads to decreased T₁-relaxation during the FSE module, therefore reducing T₁-contrast in the GRE image of the hybrid acquisition.

In the current implementation, the ETL of the FSE and GRE acquisitions is linked to the number of k_z partitions N_par. Therefore, the total scan time depends only on the repetition time TR and the number of projections N_proj. However, for a small number of slices, this leads to reduced scan efficiency whereas for a large number of slices, the signal amplitude becomes weak due to the long echo train. Furthermore, changing the number of slices results in modified image contrast as the echo time of the center partition changes. Therefore, we usually keep the number of slices at a fixed value to maintain the T₂ weighting and instead modify the slice thickness when adjusting the acquisition volume to the region of interest. This limitation can be resolved by decoupling the ETL from the number of partitions N_par, which, however, requires implementation of an advanced ordering scheme to avoid sharp transitions in T₂ weighting (51). Moreover, it would also be possible to select the ETL (and other parameters) of the FSE and GRE modules independently, which would create additional flexibility for the acquisition. However, in view of the clinical usability of the technique, it is preferable if both modules acquire the contrasts with identical scan settings.

One common artifact with radial k-space acquisition is streaking. Due to the circular point spread function of radial sampling (54), high-intensity regions in the periphery of the object can extend over the entire FOV and impair the overall image quality. In particular, if the fat suppression fails in the arms where the B₀ homogeneity is reduced, spurious streaks can propagate across the entire image. In many cases, the streaks predominantly affect individual receive coil elements located in proximity of the artifact source. Because these coil elements often do not provide relevant image information, one solution is to discard these elements before image reconstruction or to weight them according to the streak level estimated for each element (55–58).

While the use of radial sampling provides inherent robustness to motion and allows for examination during shallow breathing, additional motion correction is needed in patients exhibiting deep breathing. For this purpose, a respiratory signal is required. Various approaches have been described that extract a breathing signal from k-space data, including the commonly used center-of-mass calculation (59). This method, however, did not achieve sufficient reliability for the hybrid sequence, which may be explained by the fact that the respiratory motion is only sampled every TR and therefore with a relatively low frequency compared to typical respiratory rates. This results in a non-continuous curve for which applying additional smoothing to filter out residual biasing components from other sources may not work. To overcome this, the combination of a PCA with SOBI has been introduced for estimation of the signal, which worked well in all acquired datasets and might be promising also for related applications, such as XD-GRASP (28) or XD-Dixon-RAVE (12). However, the robustness of the approach still needs to be evaluated in a larger cohort of subjects. A limitation is reached when the respiratory motion becomes so strong and fast that the motion state changes significantly during one repetition. In this case, it might be possible to incorporate external signals, e.g. changes in the coil load or a camera, for assigning data from a single echo train to different motion states.

CONCLUSION

The described hybrid MRI technique enables volumetric T₂- and T₁-weighted abdominal imaging during free-breathing by collecting both FSE and GRE data in a single scan. Due to the use of radial k-space sampling, the method is inherently robust to ghosting artifacts. Residual motion-related blurring can be reduced by extracting a breathing curve from the k-space data and incorporating it into the reconstruction. Besides free-breathing abdominal imaging, the presented approach could be of value for other clinical applications that require acquisition of both T₂- and T₁-weighted images and that are typically affected by motion artifacts.

Supplementary Material

Supp figS1-4

Supp. Fig. 1: Sequence timing diagram of the hybrid approach. Each FSE train (red box) uses a SPIR pulse for fat suppression, followed by slab-selective 90° excitation and a non-selective refocusing train, consisting of one 180° pulse and a series of 120° pulses. To suppress blood signal, small diffusion gradients (gray) are inserted before and after the 180° pulse. For T1-weighted contrast, a GRE module is used (blue box), which starts with a chemically selective fat suppression pulse, followed by a RF- and gradient-spoiled readout. Alternatively, a blipped bipolar multi-echo readout can be used for Dixon fat/water separation (not shown). Radial stack-of-stars sampling is used for both the FSE and GRE module to achieve motion robustness (gray box). The decreasing intensity of the radial trajectories from top to bottom reflects signal decay during the FSE train.

Supp. Fig. 2a): Workflow for extraction of the respiratory signal. After selecting the center sample of each radial projection, the real and imaginary parts are concatenated for all acquired partitions and coils. A principal component analysis (PCA) is performed along the dimension of length N_par × N_coil × 2. The first 10 principal components are then processed using second order blind identification (SOBI). To automatically select the SOBI component representing breathing motion, the spectrum of the different components is computed with a fast Fourier transform (FFT), followed by calculation of the entropy. In the following figures, the different components are color-coded (PCA = gray, SOBI = green, SOBI spectra = dark yellow, Entropy = blue).

Supp. Fig. 2b): First five principal components. The PCA successfully disentangles signal contributions from B₀ eddy currents (components 1–2), linear eddy currents (resulting in a k-space shift, components 3–4), and respiration (component 5). However, the respiratory component still shows an angular dependency, which makes further post-processing necessary.

Supp. Fig. 2c): When processing the first 10 principal components with SOBI, the components are further separated (only the first 5 SOBI components are shown) and the angular dependency of the respiratory signal is successfully removed (component 5). While the spectra of the eddy-current related components (1–4) show a sharp peak at a single frequency, the spectrum of the respiration signal is broader due to variability of the respiration frequency during data acquisition, which can be used for automatically determining the correct component.

Supp. Fig. 2d): Calculating the entropy E of the power spectra q_i of the different SOBI components s reveals a jump at the respiration component (component 5). Therefore, the respiration component can be identified by searching for the first component for which the gradient of the entropy exceeds the mean gradient of the entropy. This worked reliably in all datasets shown.

Supp. Fig. 3: Calculated SNR maps of the FSE/GRE volunteer scan. Compared to the separate acquisitions, the hybrid approach leads to decreased SNR for the T₂-weighted FSE image, while SNR is increased for the T₁-weighted GRE image. This is consistent with both simulations and phantom scans.

Supp. Fig. 4a): Hybrid FSE/Dixon-RAVE acquisition of a volunteer scan for different respiratory instructions. When a motion-averaged reconstruction is performed, blurring and streaking artifacts degrade image quality for irregular and deep breathing. With motion-weighting, these artifacts are reduced.

Supp. Fig. 4b): The results from the second volunteer scan which was performed with different respiratory instructions are in-line with the finding from Supp. Figure 4a. Again, blurring and streaking artifacts are reduced when motion-weighting is applied.