Ocular changes over 60 min in supine and prone postures

We show that gravity has pronounced transient and sustained effects on the eye by making detailed ocular measurements over 60 min in the supine and prone postures. These data inform our understanding of how gravitational forces can affect ocular structures, which is essential for hypothesizing how ocular changes could occur with microgravity exposure.

Abstract

Some astronauts are returning from long-duration spaceflight with structural ocular and visual changes. We investigated both the transient and sustained effects of changes in the direction of the gravity vector acting on the eye using changes in body posture. Intraocular pressure (IOP; measured by Perkins tonometer), ocular geometry (axial length, corneal thickness, and aqueous depth-noncontact biometer), and the choroid (volume and subfoveal thickness optical coherence tomography) were measured in 10 subjects (5 males and 5 females). Measures were taken over the course of 60 min and analyzed with repeated-measures analysis of covariance to assess the effects of posture and time. In the supine position, choroidal volume increased significantly with time (average value at <5 min = 8.8 ± 2.3 mm³, 60 min = 9.0 ± 2.4 mm³, P = 0.03). In the prone position, IOP and axial length increased with time (IOP at <5 min 15 ± 2.7 mmHg, 60 min = 19.8 ± 4.1 mmHg, P < 0.0001; axial length at <5 min = 24.29 ± 0.77 mm, 60 min = 24.31 ± 0.76 mm, P = 0.002). Each increased exponentially, with time constants of 5.3 and 14 min, respectively. Prone corneal thickness also increased with time (<5 min = 528 ± 35 μm, 60 min = 537 ± 35 μm³, P < 0.001). Aqueous depth was shortened in the prone position (baseline = 3.22 ± 0.31 mm, 60 min = 3.18 ± 0.32 mm, P < 0.0001) but did not change with time. The data show that changes in the gravity vector have pronounced transient and sustained effects on the geometry and physiology of the eye.

NEW & NOTEWORTHY We show that gravity has pronounced transient and sustained effects on the eye by making detailed ocular measurements over 60 min in the supine and prone postures. These data inform our understanding of how gravitational forces can affect ocular structures, which is essential for hypothesizing how ocular changes could occur with microgravity exposure.

as of february 2016, 22 US astronauts have returned from long-duration spaceflight with ocular structural changes such as globe flattening, choroidal folds, or optic disk edema (25, 29). These structural changes are linked to a hyperopic shift in vision (25, 39). The pathophysiological etiology of these changes is unclear but is elicited by the microgravity environment (8, 25, 39, 48). Elevated intracranial pressure (ICP) has been cited as a potential mechanism (25), but recent data indicate pathologically elevated ICP may not occur in microgravity (21). If ICP is not elevated to clinically relevant levels (<16 mmHg; Ref. 19), then other mechanisms must play a role in microgravity-induced vision changes. Mader et al. (25) suggested changes might also be caused by local elevations in ICP at the optic nerve head, anatomic disposition to the microgravity ocular syndrome, or hypotony. This suggests that these microgravity-induced vision changes may result from the interaction of several physiological factors (i.e., intraocular pressure, changes in ocular geometry, and cardiovascular changes) that are all influenced by microgravity exposure.

Two important effects of removing gravitational forces are a loss of all hydrostatic gradients in the body and a headward fluid shift (1). On Earth, hydrostatic gradients in the eye and head cannot be removed, but they can be reversed by moving from the supine to prone position. Moving from the upright to supine or prone position produces a fluid shift, as does microgravity exposure. However, moving from the supine to prone position changes the direction of the gravitational vector but does not alter cardiovascular variables to the same extent as moving from seated to either recumbent posture (28, 34, 43). Therefore, differences in eye measures between the supine and prone positions isolate changes primarily due to a change in the direction of the gravitational vector acting on the body. Ground-based results detailing ocular changes in supine and prone can be used to develop hypotheses about alterations in microgravity.

Timescales are a critical factor when considering microgravity-induced vision changes. Changing gravitational forces can have immediate effects. In an earlier study, we examined the acute (1–5 min) effects of postural changes on the eye. In that study, intraocular pressure, ocular geometry, and choroidal area were altered. IOP in parabolic flight microgravity (16.3 ± 2.7 mmHg) was elevated significantly above seated baseline values (11.5 ± 2.0 mmHg). It was also elevated above supine values (13.7 ± 3.0 mmHg) and was significantly less than in the prone position (20.3 ± 2.6 mmHg). For the choroidal area, the average increase from seated baseline was 0.03 ± 0.06 mm² in the supine position, 0.05 ± 0.07 mm² in the prone position, and 0.09 ± 0.1 mm² in microgravity. Axial length increased an average of 0.01 ± 0.03 mm in the prone position, and aqueous depth decreased by 0.03 ± 0.04 mm. In the supine position, aqueous depth also increased by 0.01 ± 0.04 mm (1). These changes represent the transient influence gravity has on the eye, which indicates which ocular structures may be sensitive to alterations in the force of gravity. Transient effects, however, are unlikely to provide a stimulus for long-term remodeling of the eye. Rather, the long-lasting, steady-state changes to the eye after the transient effects have subsided are essential in understanding what stimuli might exist to promote the ocular changes seen in astronauts. The timescales for ocular geometry and choroidal volume to reach steady-state with alterations in posture are not currently known, although measures of diurnal variation for choroid thickness have been recorded. Choroid thickness changes an average of 33.7 μm (40). The timescale of IOP changes with posture is known. Moving from the seated position to the prone position, Walick et al. (44) reported an average increase in IOP of ~12 mmHg after 60 min, with values stabilizing after ~10 min. Going from seated to supine causes an increase in IOP of ~2–4 mmHg (23, 30), with values remaining constant after 30 min (33). Xu et al. (46) reported a nearly constant with time change in IOP of ~3 mmHg from 1 to 21 min, even though subjects were in 15° head-down tilt. These measures of IOP, however, have not been done in conjunction with other ocular variables to help us understand the response of the eye to changes in the direction of gravity. Data separating the eye’s transient (1–5 min) and longer duration responses over the course of an hour may contribute to understanding the permanent structural changes that occur over weeks and months during microgravity exposure. Both the transient and sustained effects are important to develop hypotheses about the mechanisms that might contribute to microgravity-induced vision changes.

The objective of the present study was to evaluate the ocular effects of posture over a period (60 min), which allows the immediate postural effects to be separated from sustained changes. Intraocular pressure, ocular geometry, and choroid volume were recorded every 15 min in the supine and prone positions, as well as in an upright, seated baseline. We hypothesized a sustained increase in IOP in the prone and supine positions, with the greatest changes in the prone position (30). We hypothesized that posture would alter aqueous depth, axial length, and choroidal volume based on our previous studies (1). These basic data on gravitational effects with time may be used to extrapolate how the eye would be affected in microgravity.

METHODS

Subjects.

All human use protocols were approved by the Committee for the Protection of Human Subjects at Dartmouth College. Informed written consent was obtained from all subjects. Ten subjects (5 male, 5 female) participated in the study. The average subject age was 31 ± 7 yr, with a range of 23 to 43 yr. Subjects were in overall good health and lacked ocular and systemic disease before data collection.

Protocol.

A seated baseline was used for all measures. Subjects were randomly assigned to the supine and or prone position first, counterbalanced by gender. Measures were taken while the subjects were lying on a tilt table (Model 6020–709; Manual Crank Tilt Table; Haussman Industries). In the prone posture, subjects’ heads were supported by an open-face mount, minimizing tissue contact pressure at the eyes. Ocular and cardiovascular measurements were taken immediately upon entering the posture. Additional measures after this first time point were taken after 15, 30, 45, and 60 min. After 60 min, subjects returned to upright for at least 30 min, when seated baseline measurements were repeated. The subject then entered the remaining posture (either supine or prone), and measures were collected in the same manner over 60 min.

Intraocular pressure.

All measures were made on the left eye using a Perkins tonometer (Mk2; Haag-Streit, Essex, UK) by a certified ophthalmologic technician. The Perkins tonometer is a handheld applanation tonometer with a spring-counterweight to compensate for gravity, so IOP can be measured in any posture (9, 31, 36). The root mean square difference between the Perkins and the gold standard Goldmann applanation tonometer is 1.4 mmHg in the seated position (13) with a potential negative bias of 1 mmHg in the seated position (45) and 1.8 mmHg in the supine position (2). Eyes were anaesthetized using fluorescein sodium and benoxinate hydrochloride (0.25/0.4%).

Optical biometry.

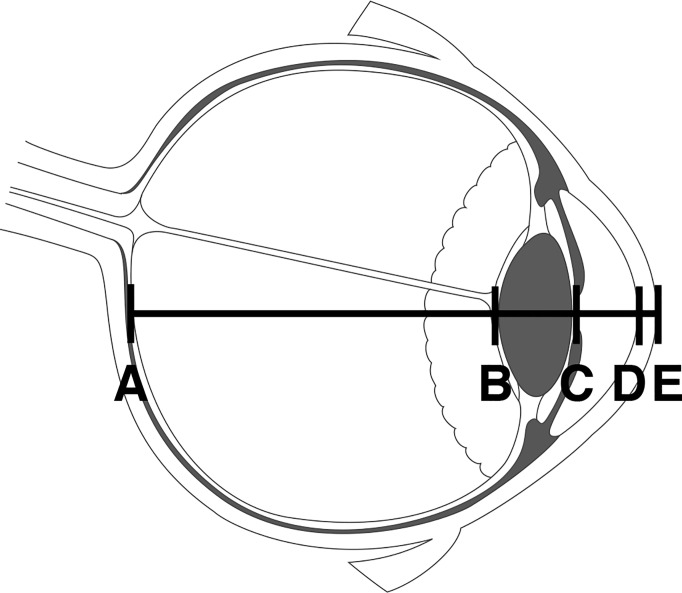
An optical biometer (Lenstar 900; Haag-Streit) was used to measure axial length, lens thickness, aqueous depth, and corneal thickness (Fig. 1) on the right eye. Five trials were averaged to obtain accurate geometric measures. To make the measurement in the supine and prone postures, the device was mounted on a custom-built boom (1) allowing the device to be properly positioned over the subjects’ eyes without them shifting position.

Fig. 1.

The geometry of the ocular globe was measured with a laser optical biometer to determine axial length (distance from A to E), lens thickness (B to C), aqueous depth (C to D), and cornea thickness (D to E). [Eye image credit Montfolio, used with permission.]

Optical coherence tomography.

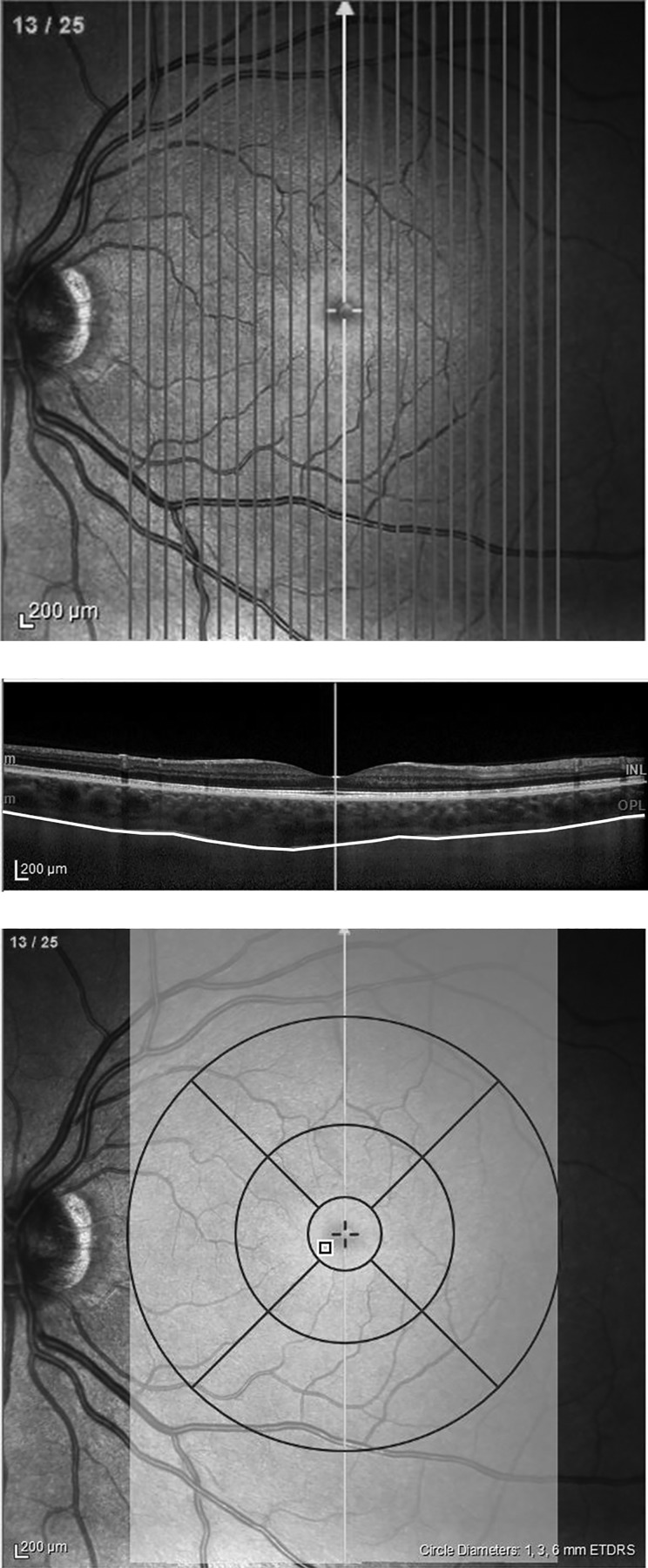
Choroidal volume was measured using optical coherence tomography (OCT) (Spectralis OCT; Heidelberg Engineering, Heidelberg, Germany) on the right eye. Images of the retina and choroid were taken using 25 vertical line scans covering a 20 × 30° field of view over the back of the eye. The set of scans was centered on the fovea. The scan taken at the fovea was also used to measure subfoveal choroid thickness (SFCT). As with the biometer, the OCT was mounted on a boom to allow measurements in the supine and prone position without the subjects shifting position. Enhanced depth imaging allowed the lower boundary of the choroid to be seen (Fig. 2). Images were taken at an Automatic Real-time Tracking (ART) setting of 30 frames but for some subjects was reduced to 20. Choroid boundaries were manually segmented by three independent observers using the Spectralis OCT software v.6.0. Segmenters were blinded to posture and time point, and no autosegmentation was used. The suprachoroidal layer was visible in one subject, and was included in analysis. Volume calculations were restricted to a 6-mm diameter circle centered on the fovea. The average interobserver variability was low for both choroidal volume and SFCT, between 1 and 7% depending on the subject. Therefore, values were averaged to obtain a single measurement at each posture for each subject.

Fig. 2.

Choroidal volume scan using optical coherence tomography. A total of 25 single line scans were taken centered on the fovea (top). Each line scan was manually segmented to determine the top and bottom of the choroid layer (middle). The choroidal volume calculation was restricted to a 6-mm diameter circular region centered on the fovea (bottom).

Cardiovascular parameters.

Heart rate and mean arterial pressure were taken at the brachial artery to monitor subject health for each measurement time point during the experiment (Critikon Dynamap).

Statistics.

The Shapiro-Wilk test was used to test each variable for normality at each time point. The two-seated baseline measures were compared with a paired t-test to evaluate whether the subjects returned to their nominal state between postures. If the baselines were not different, they were averaged. A two factor repeated-measures analysis of covariance was used to measure the main effect of time and posture. The averaged baseline of each variable was used as a covariate in the model to account for individual differences across subjects. Posture was included in the model to assess the difference between supine and prone measures. The other factor included in the model was time. When a main effect of posture was found, pairwise comparisons between the supine and prone posture at each time point were calculated using Bonferroni correction to control for family-wise error (5 comparisons, α = 0.01). Variables with a significant main effect of time were evaluated to determine if the measure had a logarithmic response (logarithmic curve fit R² > 0.8), reaching a plateaued steady state. If so, the time constant was calculated from a logarithmic curve fit to the average change from baseline with time. The time constant is when 63% of the peak value was achieved.

RESULTS

Cardiovascular parameters are shown in Table 1. There was no significant interaction for heart rate between posture and time (F = 1.5, P = 0.2) nor an effect of time (F = 1.1, P = 0.37). There was a significant difference between heart rate in different postures (F = 55, P < 0.0001). The baseline before the prone posture, however, was also significantly higher than the baseline before the supine posture (P = 0.02). Mean arterial pressure did not change with time (F = 0.74, P = 0.57) or posture (F = 2.49, P = 0.12), and there was no significant interaction (F = 1.3, P = 0.27).

Table 1.

Changes from baseline at each time point for heart rate and mean arterial pressure in each posture

	HR, beats/min		MAP, beats/min
	Supine	Prone	Supine	Prone
Baseline	68 ± 8	77 ± 11	91 ± 9	91 ± 11
1–5 min	63 ± 10*	79 ± 10*	86 ± 13	86 ± 7
15 min	66 ± 10	73 ± 9	84 ± 15	84 ± 11
30 min	65 ± 10*	75 ± 11*	86 ± 16	82 ± 10
45 min	64 ± 10	72 ± 10	84 ± 11	83 ± 9
60 min	63 ± 9*	71 ± 12*	89 ± 9*	83 ± 10*
P value posture	<0.0001†		0.12
P value time	0.37		0.57

Data are means ± SD. HR, heart rate; MAP, mean arterial pressure. The P values of the repeated-measures analysis of covariance with (ANCOVA) with baseline as the covariate are given.

^*Supine and prone measures were significant at the α = 0.01 level with Bonferroni pairwise comparisons at a given time point.

^†Significant difference. The factors evaluated in the model were change with time and differences between postures.

All ocular measures were normally distributed. Table 2 shows the average value ± SD at each baseline and time point in the supine and prone postures for ocular geometry and IOP, while Table 3 shows choroidal area and SFCT. There were no significant differences between the two seated baseline values for any variable, indicating subjects returned to their nominal state before beginning the second half of the experiment. Therefore, the baselines were averaged and used as a covariate in the model. Figure 3 shows the means ± SD change from baseline for all subjects’ IOP, aqueous depth, axial length, corneal thickness, choroidal volume, and SFCT.

Table 2.

Changes from baseline at each time point for intraocular pressure, aqueous depth, axial length, and corneal thickness in each posture

	IOP, mmHg		Aqueous Depth, mm		Axial Length, mm		Corneal Thickness, μm
	Supine	Prone	Supine	Prone	Supine	Prone	Supine	Prone
Baseline	10.7 ± 1.6	11.4 ± 1.8	3.22 ± 0.30	3.22 ± 0.31	24.29 ± 0.76	24.29 ± 0.76	530 ± 34	529 ± 35
1–5 min	12.2 ± 1.7	15.0 ± 2.7	3.23 ± 0.31*	3.18 ± 0.33*	24.28 ± 0.76*	24.29 ± 0.77*	530 ± 34	528 ± 35
15 min	12.7 ± 2.2*	18.0 ± 3.9*	3.22 ± 0.31	3.16 ± 0.33	24.28 ± 0.76*	24.30 ± 0.76*	531 ± 35	530 ± 35
30 min	12.6 ± 3.1*	19.8 ± 4.0*	3.22 ± 0.32*	3.18 ± 0.31*	24.28 ± 0.76*	24.31 ± 0.76*	531 ± 36	533 ± 35
45 min	12.3 ± 3.3*	20.3 ± 4.8*	3.22 ± 0.31*	3.15 ± 0.33*	24.28 ± 0.76*	24.31 ± 0.76*	532 ± 35	533 ± 35
60 min	12.2 ± 3.0*	19.8 ± 4.1*	3.24 ± 0.30	3.18 ± 0.32	24.28 ± 0.76*	24.31 ± 0.76*	531 ± 35*	537 ± 35*
P value posture	<0.0001†		<0.0001†		<0.0001†		0.03†
P value time	0.003†		0.29		0.05†		<0.0001†

Data are means ± SD. IOP, intraocular pressure. The P values of the repeated-measures ANCOVA with baseline as the covariate are given.

^*Supine and prone measures were significant at the α = 0.01 level with Bonferroni pairwise comparisons within a given time point.

^†Significant difference. The factors evaluated in the model were change with time and differences between postures.

Table 3.

Changes from baseline at each time point for subfoveal choroid thickness and choroidal volume in each posture

	Subfoveal Choroid Thickness, μm		Choroidal Volume, mm3
	Supine	Prone	Supine	Prone
Baseline	320 ± 101	320 ± 92	8.7 ± 2.3	8.8 ± 2.4
1–5 min	320 ± 93	323 ± 95	8.8 ± 2.3	8.8 ± 2.4
15 min	328 ± 90	322 ± 93	8.8 ± 2.3	8.8 ± 2.4
30 min	322 ± 100	322 ± 100	8.9 ± 2.5	8.8 ± 2.3
45 min	325 ± 94	329 ± 99	8.9 ± 2.4	8.8 ± 2.4
60 min	334 ± 98	335 ± 102	9.0 ± 2.4	8.9 ± 2.4
P value posture	0.75		0.05*
P value time	0.01*		0.18

Data are means ± SD. The P values of the repeated-measures ANCOVA with baseline as the covariate are given. None of the individual time points were different between supine and prone positions for either measure at the α = 0.01 level with Bonferroni pairwise comparisons.

^*Significant difference. The factors evaluated in the model were change with time and differences between postures.

Fig. 3.

The change of intraocular pressure (IOP), aqueous depth (AD), axial length (AL), corneal thickness (CO), choroidal volume (CHV), and subfoveal choroid thickness (SFCT) from seated baseline over an hour in the supine and prone postures. The mean and standard deviation of the difference from baseline are shown. *Measure changed significantly with time in the posture. †Measurements in the postures were significantly different in supine and prone positions. A repeated-measures analysis of covariance with baseline as the covariate was used to assess changes (n = 10 subjects for all tests). [Eye image credit Montfolio, used with permission.]

IOP showed a significant interaction term (F = 4.0, P = 0.005) and a significant main effect of time (F = 4.3, P = 0.003) and posture (F = 164, P < 0.0001). In the supine position, there was no change with time (F = 0.2, P = 0.9), but there was in the prone position (F = 10.6, P < 0.0001). IOP was higher in prone than in supine for every time point, reaching statistical significance at 15 min (P < 0.01) and remaining significant thereafter. At the 1- to 5-min measurement, the difference was not significant when correcting for multiple comparisons (P = 0.026).

Aqueous depth did not show a significant interaction term (F = 0.28, P = 0.89) nor a significant main effect of time (F = 1.3, P = 0.28). There was a significant main effect of posture (F = 60.71, P < 0.0001). Aqueous depth was shorter in prone than supine, reaching significance at the 1- to 5-min, 30-min, and 45-min points (P < 0.01) but not at the 15-min (P = 0.018) and 60-min measurements (P = 0.013) when corrected for multiple comparisons.

Axial length showed a significant interaction term (F = 4.0, P = 0.003) and a significant main effect of time (F = 3, P = 0.05) and posture (F = 157, P < 0.0001). The supine position showed no main effect of time (F = 2, P = 0.22), but the prone position did (F = 5, P = 0.002). Axial length was shorter in supine than in prone for every time point (P < 0.01).

Corneal thickness showed a significant interaction term (F = 6.9, P = 0.0001) and a significant main effect of time (F = 10.4, P < 0.0001) and posture (F = 4.9, P = 0.03). In the supine position, there was not a significant main effect of time (F = 1.08, P = 0.48), but there was in the prone position (F = 19.3, P < 0.0001). Corneal thickness reached statistical significance at the 60-min measure (P < 0.01).

Choroidal volume did not show a significant interaction term (F = 1.22, P = 0.3) nor a significant change with time (F = 1.62, P = 0.18). It did show a change with posture (F = 3.83, P = 0.05). In the supine position, there was a significant main effect of time (F = 3.1, P = 0.03) as choroidal volume increased over time. In the prone position, there was no main effect of time (F = 0.53, P = 0.71). None of the individual time points were different between supine and prone at the α = 0.01 level.

SFCT did not show a significant interaction term (F = 0.38, P = 0.83) nor a significant change with posture (F = 0.1, P = 0.75). It did show a change with time (F = 3.5, P = 0.01). This is in contrast with choroidal volume, where posture showed an effect but not time. When separated by posture, neither condition showed a significant change with time (supine F = 2.2, P = 0.09; prone F = 1.8, P = 0.15). Similarly, at each time point, there was no difference in posture (P > 0.25).

IOP and axial length exhibited a logarithmic response in the prone position. IOP in the prone posture had a time constant of τ = 5.3 min (logarithmic curve fit R² = 0.96). Axial length in the prone posture had a time constant of τ = 14 min (logarithmic curve fit R² = 0.97). A logarithmic curve fit to corneal thickness achieved good accuracy (logarithmic curve fit R² = 0.91), but the data did not appear to reach steady state during our observations. A linear fit to the data showed improved accuracy (linear curve fit R² = 0.95). Choroidal volume in the supine position did not exhibit a logarithmic response (logarithmic curve fit R² = 0.6) and continued to increase with time during our observations. Therefore, no time constant was calculated for corneal thickness and choroidal volume.

DISCUSSION

These results suggest that both time and changes in the direction of the gravity vector acting on the body can produce immediate and relevant physiological changes in ocular measures. These data also give a detailed description of how the eye changes over 60 min in the supine and prone postures. In the prone posture, IOP was higher, aqueous depth was shallower, axial length was longer, and the cornea was thicker than in the supine position. Choroidal volume was greater in the supine position than in the prone position. SFCT increased in both postures, but supine and prone values were not different from each other. The effect of time was also profound. IOP, axial length, corneal thickness, and choroidal volume all increased with time in a single posture, indicating that the initial changes seen in these positions on short timescales do not reflect the sustained effects as the posture is maintained. Alternatively, aqueous depth showed a change with posture but was not affected by time. Taken together, the data show that the direction of gravity has pronounced effects on the eye that progress over time. It is likely that removing gravitational gradients in microgravity would also have significant effects.

Transient changes.

Identifying the acute changes caused by a change in posture allows for determination of the transient influence gravity has on the eye, indicating which ocular structures may be sensitive to alterations in the force of gravity. The first postural measurements taken at 1–2 min are equivalent to our previous published results on the acute (<5 min) effects of changing posture on the eye (1). In this study, IOP was different between the prone and supine positions after 5 min (P = 0.026), as was reported in the previous study. In both studies, aqueous depth was statistically different between supine and prone, with aqueous depth decreased in the prone position from seated baseline. Axial length approached but did not achieve statistical significance between supine and prone in the previous study (P = 0.053) but was significantly in the current study (P = 0.01). No immediate change was found in corneal thickness in either set of data.

In the current study, we found no significant difference between choroidal volumes in the supine and prone postures at the first time point (P = 0.8). Similarly, choroidal area from our previous study was not significantly different between supine and prone (P = 0.09). Finally, SFCT measured here did not change between the supine and prone postures (P = 0.7). In the previous study, prone choroidal area was greater than baseline (P < 0.006), but not in this study for choroidal volume (P = 0.33) and SFCT (P = 0.5). It not known why we did not find significance between the prone and seated position in this study. Subjects in the previous study had taken scopolomine for parabolic flight, and it is not known if this would have an effect. The previous study included choroids from 20 subjects, while the current study has 10 subjects.

Sustained changes.

Evaluating the time course of changes over 60 min allows the transient effects of shifting posture to be separated from the sustained changes. IOP, axial length, corneal thickness, and choroidal volume each changed with time but only in one posture. The time constant is a measure of exponential rise of a physical system. Longer time constants imply a slower change. These data show that IOP responds more rapidly to changes in posture than axial length and some ocular measures may not reach steady state within an hour. The time for IOP to reach steady state was consistent with that reported elsewhere for the time course of changes in IOP (10, 44). Compared with the seated position, IOP has been shown to increase in the supine position and is even further elevated in the prone position (7, 23, 30). The authors are unaware of published time-course information for axial length over these timescales.

Elevated IOP could cause expansion of the globe or stretching of ocular tissues, resulting in a measured change in axial length with time. There is an established relationship between elevated IOP and elongated axial length over long timescales (16, 17). Phillips and McBrien (32) showed a positive correlation of IOP and axial length in animals with ocular injection on short timescales (1 h) which increased IOP by 85 mmHg. Francis et al. (17) showed a group average decrease in axial length of 0.15 mm with a decrease in IOP of 8.8 mmHg 1 wk after glaucoma surgery. Our subjects showed an average increase in axial length from baseline of 0.03 mm after an hour in the prone position with an average increase in IOP of 8.4 mmHg. The effect of tissue weight could also be a relevant factor, since the force of gravity is acting on the eye and “pushes” the eye into the retro-orbital tissues in the supine position. The globe might be slightly flattened when it is resting in the bony orbit within the retro-orbital tissue. This would serve to counteract an IOP-driven change in axial length. In the prone position, the eye may be hanging from the connective tissue in the retro-orbital space and so could lengthen more easily than in the supine position. The different durations of the time constants for IOP and axial length may reflect the fact that axial length, dominated by tissue stiffness, changes more slowly than IOP, dominated by ocular fluid dynamics.

Age and baseline axial length were also investigated for correlations with IOP and axial length changes. Scleral stiffness increases with age (18). Baseline axial length is used as measure of myopia, emmotropia, and hyperopia, where myopic eyes have reduced scleral stiffness compared with emmotropic or hyperopic eyes (35). Age was correlated with changes in the supine posture after 60 min for IOP (R = −0.94) and axial length (R = −0.7). This means the youngest subjects saw increases in IOP in the supine posture, while older subjects saw either no change in IOP or a decrease. Older subjects also saw a decrease in supine axial length, while younger subjects tended to increase. In the prone position, there was no correlation with age at 60 min in the posture (IOP R = −0.3, axial length R = −0.3). Similarly, there were no correlations between baseline axial length and changes in IOP at 60 min (R supine = 0.3, R prone = 0.04) or changes in axial length at 60 min (supine R = 0.4, prone R = −0.1).

Shorter aqueous depths were also measured in the prone posture. A shorter aqueous depth implies the lens-iris diaphragm shifted closer to the cornea. In our subjects, aqueous depth was shortened immediately and did not change with time. We hypothesize this is due to tissue weight. The lens has greater density than the surrounding humor (15, 37), so it would shift closer to the cornea in the prone position due to gravity. Also, the vitreous humor is a viscous liquid and is subject to hydrostatic gradients, adding pressure to the flexible lens iris diaphragm and shifting it forward (14). A shorter aqueous depth implies a narrower anterior chamber angle, which could restrict aqueous humor drainage into the trabecular meshwork. Drainage through the uveoscleral pathway might also be affected due to the hydrostatic pressures within the circulation. Additionally, when IOP is elevated, aqueous humor flow is reduced through pseudofacility, and ultrafiltration is mitigated resulting in a longstanding decrease in production (3, 4, 20). This accounts for an estimated 27% of the outflow facility (3). The rate cited by Beneyto Martin et al. (3) is a reduction in flow by 0.081 μl·min⁻¹·mmHg. Estimating using the average increase in IOP of 8.4 mmHg yields reduced flow by 0.68 μl/min after elevated IOP is reached. These feedback mechanisms may be consistent with the logarithmic response of IOP with time observed in this study. Efforts to estimate these changes with numerical modeling are ongoing.

Corneal thickness changed with time in the prone posture but did not reach steady state. Mild corneal edema may occur as increased IOP causes a mild decrease in corneal endothelial cell pump function (47). An increase in IOP initially causes corneal thinning followed by edema if the pump function is overwhelmed (42). Our results are consistent with this trend. Alternatively, one may hypothesize that the modest increase we observe in the corneal thickness was related to gravitational redistribution of the tear film in the central cornea in the prone position rather than inferiorly in the upright position. While this is plausible it does not explain the increase in corneal thickness over time. Although the changes measured in this study are small and subclinical, the elevated IOP and corneal thickness act in concert with one another in the prone posture. It is known that thicker corneas elevate IOP values when measured with tonometry (38), but since the thickening of the cornea here does not likely represent tissue remodeling leading to gross structural changes on such short timescales, it is unlikely to contribute to elevated IOP measurements. We did not measure the changes in the thickness of the individual layers of the tear film or cornea (such as tear film, epithelium, stroma, and endothelium) and thus we cannot speculate as to which of these layers is increasing with prone positioning. In future studies anterior segment OCT might be helpful in defining subtle changes in corneal structure.

Choroidal volume increased with time while in the supine position but did not reach steady state. The maximum increase measured in an individual as a percentage of seated baseline was 5.6% after 60 min. The choroid has no autoregulatory mechanism (5); therefore, its volume may be expected to increase in supine due to the headward fluid shift, which would elevate venous pressures in the head. Choroidal volume in the prone position did not increase with time, unlike the changes seen in the supine position. Interestingly, below the fovea, choroidal thickness increased with time, regardless of the posture. It is possible there was an interaction between forces seeking to expand the choroid (elevated venous pressures due to the fluid shift) and other forces that would keep it from expanding (IOP). We hypothesize the substantially elevated prone IOP causes the fluid of the vitreous body to exert an increased force on the choroid, which could inhibit its expansion. This mechanism may have greater influence on the peripheral choroid than the subfoveal choroid. Choroidal thicknesses are known to vary with location in the posterior pole, age, and refractive status (40). For the choroid to expand, there needs to be both available volume (e.g., from the headward fluid shift) and no impediment to expansion. If IOP increases more than choroidal pressure, then the choroid will decrease or remain unchanged in volume. Pressure exerted on the choroid by increased IOP may overcome the expansion expected due to the fluid shift.

Relevance to microgravity.

Multiple factors that are affected by microgravity influence the eye: fluid shifts, tissue weight, hydrostatic gradients, aqueous humor dynamics, and cranial vascular dynamics. Two factors from the current study are relevant for understanding microgravity effects: the time course of the changes and the changes that occur in the supine and prone postures.

The changes that develop in space likely require a set of persistent, steady-state conditions that lead to tissue remodeling and cardiovascular adaptions over time. Although there may be differences in how the body responds to a headward fluid shift in space compared with on Earth, the results from this study show the transient effects were complete for IOP and axial length within ~15 min, while others, choroidal volume and corneal thickness, continued to develop. Although the changes found in all measures were relatively small and subclinical, if sustained over weeks or months, they may be enough to induce ocular remodeling in microgravity.

Although we cannot extrapolate directly from these results how microgravity will affect the eye, these results imply that both the force of gravity and time are important factors in eye physiology. We hypothesize that microgravity effects may represent an intermediate state between the supine and prone results, because they represent the two extremes of directional force on the eye, while in microgravity the gravitational vector is removed. If this is the case, we would expect that, at least in the early portion of microgravity exposure, IOP would be elevated above supine levels, axial length may be increased, aqueous depth may be shorter, corneal thickness may be increased, and choroidal volume may be increased.

Data from spaceflight and parabolic flight indicate IOP is initially increased in microgravity above the seated baseline (11, 12, 24) but is between supine and prone values (1). Aqueous depth is also measured to be between the supine and prone values in parabolic flight microgravity (1), potentially since tissue weight and hydrostatic gradients no longer act on the lens. Due to tissue offloading and removal of hydrostatic gradients, axial length, corneal thickness, and globe geometry may also be altered in microgravity, allowing the globe to settle into its least energy shape. To the authors’ knowledge, these measurements have not been made on timescales longer than parabolic flight.

How the eye changes from these early initial conditions in microgravity to the alterations in long-duration spaceflight seen weeks or months later is not known. Changes in globe geometry may alter volume and tissue compliance, and subsequently the pressure of the fluid inside the eye. Over time in microgravity, total blood volume decreases, diminishing the effect of the fluid shift (6). This may affect the choroid as well as IOP and pressures within and around the eye. Microgravity may affect aqueous humor production or outflow in ways that are not currently understood.

Looking at ocular changes in the lateral decubitus posture (LDP) may inform these hypotheses and help us to understand ocular changes in the intermediate between supine and prone postures. LDP would align the gravity vector orthogonally with the primary axis of the eye while inducing a fluid shift in the body. Therefore, changes in globe shape due to tissue weight would not be in the axis of measurement. Malihi et al. (26) measured IOP in subjects in the seated, supine, LDP, and in seated position with neck bent, causing the eye to align with the gravity vector as in the prone position but without inducing a fluid shift lying down would cause. The average IOP change from seated baseline is consistent with our results in the supine and head prone postures. IOP measured in LDP was between these two conditions, with the dependent eye being elevated over the nondependent eye (26). Lee et al. (22) similarly showed that LDP IOPs were between supine and prone values. IOP in the prone position was also made with the head turned, and in both prone and LDP, the dependent eye was elevated over the nondependent eye. This supports the dependence of IOP on the influence of the gravity vector beyond changes caused by fluid shift. Axial length and aqueous depth in LDP have not been studied to the author’s knowledge, but is an area of future work.

Limitations.

There are several relevant limitations to this study. The cornea is a very thin structure, and the optical biometer has resolution on the order of the changes seen in this experiment with high standard deviation. Nonetheless, the measurements show statistical changes within subjects. To measure aqueous humor dynamics, more invasive techniques are required (41). We did not control the fluid shift to assess changes in choroidal blood volume, but future work includes a study with lower body negative and positive pressure to test our hypothesis on choroidal volume and IOP interaction. Ocular geometry was measured on the right eye, while IOP was measured on the left eye. Measuring IOP requires the use of fluorescein, making it difficult to take the optical biometry and OCT measurements on the same eye. It is not known to what degree bilateral differences of eyes and pressure in the eye may have affected our results. The measured IOP values are low due to the negative bias of the Perkins of 1–2 mmHg from Goldmann applanation tonometry (2, 13, 45). Repeated-measures statistics, however, are not sensitive to this bias, since each subject’s baseline acts as the control; therefore, the statistical results are not affected. This study was not sufficiently powered to assess the influence of gender on the results, but eye structural and visual acuity changes with long-duration spaceflight are most profound in men, despite incidence rates that are not statistically different (27). Future studies may be designed to include gender as a study factor. Finally, there were some ocular measures that showed a main effect of time but did not reach a sustained level of change. It is possible with additional time in posture these measures may also have exhibited an exponential response.

In this study we measured the time constant for IOP and axial length but were not able to measure the steady-state response of other ocular variables. Additional time in the posture may be needed to measure the steady-state response. It is possible that IOP and axial length may continue to change after 60 min, but these changes may be due to diurnal variation or the body’s adaptive response to the altered state measured herein. We did not attempt to measure subsequent adaptation or diurnal variation since the objective was to measure the nature and time course of ocular changes to a discrete stimulus, changing the direction of the gravity vector.

Conclusion.

This study showed that the interaction of the direction of the gravity vector and time has profound effects on ocular structures. The data detail the time course of ocular changes with alterations in the direction of the force of gravity acting on the body. Changing posture from supine to prone reverses the direction of hydrostatic gradients and alters the forces on the eye due to tissue weight. Moving from the upright to supine or prone postures produces a fluid shift, which alters aqueous humor dynamics and induces changes in structures such as the choroid. These results show the perturbations that can be expected from the seated baseline to the supine and prone positions over 60 min. We found profound alterations of IOP, axial length, corneal thickness, and aqueous depth in the prone position but no change in choroidal volume. For each variable, the opposite is true in the supine position. When a variable did change, IOP, axial length, corneal thickness, and choroid volume changed progressively with time, while aqueous depth was altered immediately and did not change with time. These data also inform our understanding of how gravitational forces can affect ocular structures beyond what would be expected with a fluid shift alone. These data are essential for interpreting the ocular changes that occur with exposure to microgravity. The interaction among IOP, aqueous depth, axial length, corneal thickness, and choroidal volume points to the importance of numerical modeling in understanding the influence of gravity and time on the eye. The relative contribution of these potentially competing factors has to be understood to reach a full understanding of the changes. We are developing a structural finite element model of the eye and a cranial vascular circulation model to predict how removing the force of gravity in space may lead to subsequent ocular structural changes over longer durations on orbit. These results are also relevant in clinical applications for Earth-bound patients, for example, in surgery where understanding the effects on the eye of maintaining a given posture over time are relevant.

GRANTS

This work was supported by New Hampshire National Aeronautics and Space Administration Experimental Program to Stimulate Competitive Research Grant NNX13AD35A and the National Space Biomedical Research Institute through NCC 9–58.

DISCLAIMERS

The funding organizations had no role in the design or conduct of this research.

DISCLOSURES

No conflicts of interest, financial or otherwise, are declared by the authors.

AUTHOR CONTRIBUTIONS

J.C.B. conceived and designed research; A.P.A., G.B., J.G.S., C.M.T.-K., and A.M.F. performed experiments; A.P.A., G.B., and J.G. analyzed data; A.P.A., G.B., S.D.P., D.A.K., C.M.T.-K., and J.C.B. interpreted results of experiments; A.P.A. prepared figures; A.P.A., G.B., and J.C.B. drafted manuscript; A.P.A., G.B., J.G.S., S.D.P., D.A.K., C.M.T.-K., M.E.Z., A.M.F., J.G., and J.C.B. edited and revised manuscript; A.P.A., G.B., J.G.S., S.D.P., D.A.K., C.M.T.-K., M.E.Z., A.M.F., J.G., and J.C.B. approved final version of manuscript.