Presynaptic Calcium En Passage through the Axon

Main Text

The vast majority of the synapses in our brain are built by en passant (French for “in passing”) boutons, in which axons enlarge their diameter from ∼200 nm to ∼1 μm. Action potentials propagating along the axon trigger the opening of voltage-gated Ca²⁺ channels, and within a few hundred μs, ∼10,000 Ca²⁺ ions rush into the bouton, driven by a 70-mV electrical gradient and a 20,000-fold concentration gradient (due to 50 nM intra- and 1 mM extracellular [Ca²⁺]). In the vicinity of the Ca²⁺ channels, the intracellular Ca²⁺ concentration therefore rises rapidly to ∼10 μM and triggers the fusion of neurotransmitter-filled vesicles (1). After the Ca²⁺ channels close, diffusion of Ca²⁺ ions into the bouton cytoplasm leads to the collapse of local Ca²⁺ microdomains and an increase in the average (residual) Ca²⁺ concentration ranging from 100 nM to 1 μM.

But what happens to all of these Ca²⁺ ions in the bouton? This question is important because accumulation of Ca²⁺ within the bouton controls the probability and synchronicity of neurotransmitter release (2). Furthermore, Ca²⁺ accelerates vesicle recruitment (3), influences endocytosis (4), and controls various forms of presynaptic long-term plasticity (5).

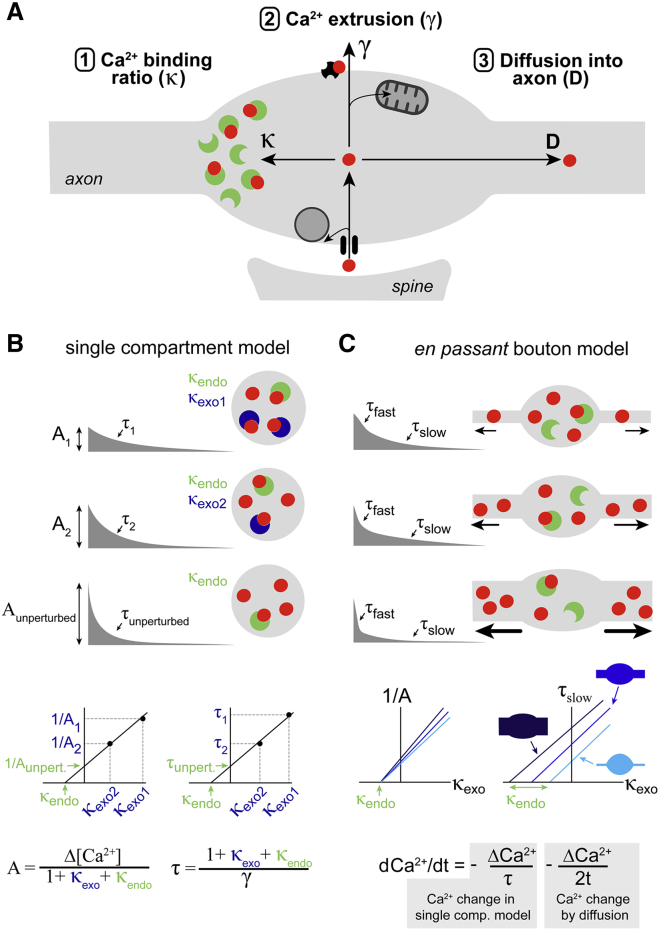
The fate of Ca²⁺ inside the bouton is determined by at least three processes (Fig. 1 A). First, the majority of Ca²⁺ ions will bind to endogenous Ca²⁺ buffers. The Ca²⁺ binding ratio κ is defined as the ratio of Ca²⁺ ions that, upon entering the bouton, bind to buffers to the ones that remain free (6). For example, κ = 4 means that four of five entering Ca²⁺ ions are buffer-bound and one remains free (cf. Fig. 1 A). In a single-compartment spherical cell, κ can be determined by perfusing the cell with different concentrations of fluorescent Ca²⁺ indicator dyes and measuring the action potential-evoked Ca²⁺ transients (6; Fig. 1 B). The dye acts as an exogenous buffer (dark blue) that adds to the endogenous buffer (green). With increasing dye concentration, the Ca²⁺ transients become smaller and slower. Plotting the inverse of the Ca²⁺ transient amplitude (1/A) or the decay time constant (τ) against the buffer-binding ratio of the dye (κ_exo) yields a linear relationship (see equations in Fig. 1 B). The endogenous Ca²⁺ binding ratio (κ_endo) can be extrapolated from the x-intercepts and the amplitude and the time constant in the unperturbed bouton from the y-intercepts of the relevant graphs.

Figure 1.

Diffusion into the axon shapes presynaptic Ca²⁺ signals. (A) An illustration of the three major determinants of Ca²⁺ signals in en passant presynaptic boutons is shown. Tran and Stricker (8) found that, besides buffering and extrusion, diffusion into the axon significantly impacts Ca²⁺ transients. (B) In a single-compartment model, the endogenous Ca²⁺ dynamics can be back extrapolated from amplitudes and time constants of Ca²⁺ dye signals. (C) With increasing axon diameter, the Ca²⁺ decay accelerates, becomes biexponential, and contaminates τ back extrapolation of κ.

The second factor determining the Ca²⁺ time course is the removal of Ca²⁺ from the cytoplasm. This is described by the extrusion rate γ (Fig. 1 A) and can be mediated by transport across the plasma membrane and/or by uptake into organelles. Mitochondrial uptake appears to be important when intracellular Ca²⁺ concentration is strongly increased, e.g., during trains of action potentials (7).

In this issue of Biophysical Journal, Tran and Stricker (8) provide an elegant analysis and convincingly demonstrate the importance of a third mechanism that controls presynaptic Ca²⁺ dynamics at small en passant boutons, namely, the diffusion of Ca²⁺ into the axon (Fig. 1 A). By thoroughly investigating boutons of neocortical layer 5 pyramidal neurons in acute brain slices, they found that diffusion into the axon significantly accelerates the decay of Ca²⁺ concentration. When recording presynaptic Ca²⁺ signals, the authors observed biexponential decays of Ca²⁺ transients (τ_fast and τ_slow). Furthermore, they found a low value of κ_endo = 10 from 1/A back extrapolation but larger values from τ_slow back extrapolation (Fig. 1 C). They then excluded nonlinear Ca²⁺ removal, saturation of fast buffers, and release from internal Ca²⁺ stores as potential underlying processes. However, they resolved axonal Ca²⁺ signals with a delay depending on the distance from the bouton. When extending a computational model of a bouton with an axon of varying diameter, they could quantitatively explain the biexponential decay kinetics and the differential κ_endo estimates observed experimentally. With a large diameter at the upper border of experimentally determined values (9), the model nicely reproduced the experimental findings. Finally, the authors derived an analytical equation of the contribution of diffusion into the axon that explains the biexponential decay kinetics (see equation in Fig. 1 C). These results convincingly demonstrate that the efflux of Ca²⁺ into the axon critically affects the Ca²⁺ transient in small en passant boutons.

In a previous seminal study published in Biophysical Journal, the Ca²⁺ transients at a large presynaptic terminal, the calyx of Held, could be described without considering diffusion into the axon (10). This difference can be readily explained by the large volume of these terminals in relation to the corresponding axon. Consistently, a previous study at small en passant boutons of layer 2/3 pyramidal neurons observed biexponential Ca²⁺ transients in a subset of boutons (11). However, in contrast to Tran and Stricker (8), prominent Ca²⁺ signals could not be resolved in adjacent axons. The large κ_endo of 140 obtained from τ back extrapolation (11) is probably an overestimation because Tran and Stricker show that axonal diffusion contaminates the τ back extrapolation but less so the 1/A back extrapolation (cf. Fig. 1 C). Interestingly, the washout of a slow endogenous buffer also contaminates the τ back extrapolation, although in the opposite direction (12). Thus, 1/A back extrapolation of κ seems to be more robust but requires quantitative Ca²⁺ measurements. Both τ and 1/A back extrapolation critically depend on the exact binding constants of the dyes, as beautifully demonstrated by Stricker and colleagues in a recent study (13). Finally, presynaptic whole-cell patch-clamp recordings from small en passant boutons have recently become possible (14, 15) and may provide the tools for a more robust quantification of Ca²⁺ concentration and a better control over the washout of endogenous buffers. It will therefore be interesting to see if such recordings confirm the low κ_endo and the large contribution of diffusion to Ca²⁺ dynamics at en passant boutons.

To explain presynaptic function and modifications during short- and long-term plasticity, a detailed knowledge of presynaptic Ca²⁺ dynamics is required (16). Tran and Stricker have now revealed the relevance of the passage of presynaptic Ca²⁺ through the axon. The study represents an important step toward a quantitative understanding of presynaptic Ca²⁺ signaling in the 10¹⁴ small boutons of our brain.

Editor: Jane Dyson.