Abstract
Oxygen is essential for animal life, and while geochemical proxies have been instrumental in determining the broad evolutionary history of oxygen on Earth, much of our insight into Phanerozoic oxygen comes from biogeochemical modelling. The GEOCARBSULF model utilizes carbon and sulphur isotope records to produce the most detailed history of Phanerozoic atmospheric O2 currently available. However, its predictions for the Paleozoic disagree with geochemical proxies, and with non-isotope modelling. Here we show that GEOCARBSULF oversimplifies the geochemistry of sulphur isotope fractionation, returning unrealistic values for the O2 sourced from pyrite burial when oxygen is low. We rebuild the model from first principles, utilizing an improved numerical scheme, the latest carbon isotope data, and we replace the sulphur cycle equations in line with forwards modelling approaches. Our new model, GEOCARBSULFOR, produces a revised, highly-detailed prediction for Phanerozoic O2 that is consistent with available proxy data, and independently supports a Paleozoic Oxygenation Event, which likely contributed to the observed radiation of complex, diverse fauna at this time.
The GEOCARBSULF model provides the most detailed reconstructions of Phanerozoic O2, but its predictions are not supported by geochemical data. Here, a GEOCARBSULF model rebuilt from first principles, with the addition of an amended sulphur cycle and the latest isotope records, supports a Paleozoic Oxygenation Event.
Introduction
Oxygen plays a vital role in planetary habitability, but the precise history of atmospheric oxygen on Earth remains a puzzle. The first rise in atmospheric O2 concentration to appreciable levels around 2.45–2.32 Ga, termed the Great Oxidation Event (GOE), is well-defined due to the loss of mass-independent sulphur isotope fractionation as stratospheric ozone abundance increased1. The presence of fossilized charcoal (inertinite) in sediments younger than 419 Ma defines another oxygen threshold, indicating that pO2 exceeded the levels required for combustion during this period2–4. However, the absence of fossil charcoal before 419 Ma does not necessarily point to lower atmospheric O2, and may simply reflect the absence of readily-combustible fuel before the evolution of woody plants (lignophytes)3.
A variety of geochemical methodologies have been developed and applied to constrain the redox state of the ancient oceans and atmosphere between the GOE and the Devonian period5–15. Several studies point to a Neoproterozoic Oxygenation Event16, but geochemical proxies also show widespread anoxia in early Paleozoic oceans. However, while marine geochemical proxies provide insightful baseline observations, it is difficult to quantitatively infer atmospheric oxygen levels from reconstructions of ocean redox chemistry17. Nevertheless, such data may be utilized and enhanced by whole Earth system biogeochemical models.
Biogeochemical ‘box models’ calculate how atmospheric oxygen concentrations may have fluctuated over the Phanerozoic Eon (541-0 Ma), with a particular strength lying in the potential for high-resolution temporal reconstruction of variability in atmospheric oxygen levels. These models make predictions by estimating source and sink fluxes in the geological oxygen cycle, which are the burial, weathering and tectonic recycling of organic carbon and pyrite sulphur. This is possible because the residence time of O2 in the surface system (i.e., atmosphere and ocean) is very long (>1 Myr). Box models for Phanerozoic oxygen can be computed in a number of ways with regard to the representation of elemental cycles, and how they calculate weathering and burial fluxes that transfer mass between the crust and surface system. The most prominent of these models, GEOCARBSULFvolc18–21, hereafter referred to simply as GEOCARBSULF, provides a detailed O2 reconstruction, as it uses the extensive carbon and sulphur isotope records from sedimentary rocks, as well as time-dependent, normalized Earth system parameters (such as river runoff) to derive changes to carbon and sulphur cycling18. Other prominent box models (e.g., COPSE22, MAGic23) opt instead to calculate productivity and burial of organic C and pyrite through an internal scheme of nutrient delivery and recycling. This gives them greater power to interrogate possible drivers of Earth system change, but reduces the ability to make detailed predictions directly from known geological data. For example, the COPSE model’s reconstructions of O2 levels are in large part driven by the evolution of the terrestrial biosphere, through changes to the C:P ratio of biomass and rates of primary productivity on the continents, which is imposed in the model based on paleobotanical evidence22,24,25. The MAGic model23 is similarly biosphere-driven, utilizing a dataset of the organic carbon content of the Phanerozoic sedimentary rock record26 to derive a terrestrial organic carbon burial flux, rather than using isotopic data. Because these models are driven by broad, long-term changes, they produce O2 curves that have less detail than GEOCARBSULF. The GEOCARBSULF reconstructions, or other isotope-derived curves, are most commonly used beyond the immediate field of O2 modelling, for example when considering O2 impacts on animal evolution27–30.
Despite differences in the way these models operate, GEOCARBSULF, COPSE, and MAGic generally agree on the broad changes in atmospheric O2 over the last ~400 Myrs, whereby pO2 rose to a peak of around 25–30% atm in the Permo-Carboniferous (~300 Ma), and remained above ~18% atm thereafter21–24,31,32. This broad picture is also supported by the inertinite record3. In contrast, there is considerable uncertainty in atmospheric oxygen levels during the early Paleozoic. Here, GEOCARBSULF predicts roughly present levels of atmospheric oxygen, which are not borne out by other models22,24. More importantly, these predictions conflict both with proxy evidence for widespread anoxia, which indicate that pO2 was below roughly half the present level7,11,14, and with the growing body of evidence for a step-change in surface oxygen levels during the Paleozoic (Fig. 1). This problem is further highlighted by recent work updating carbon isotope inputs in GEOCARBSULF30, which cause the model to predict even higher levels of oxygen in the early Paleozoic, with 37 and ~35% atm in the Cambrian and Silurian, respectively (see Fig. 1). These results suggest that the original GEOCARBSULF model produces increasingly unstable oxygen predictions during the Carboniferous period, back through to the Precambrian, as the pO2 oscillations become larger.
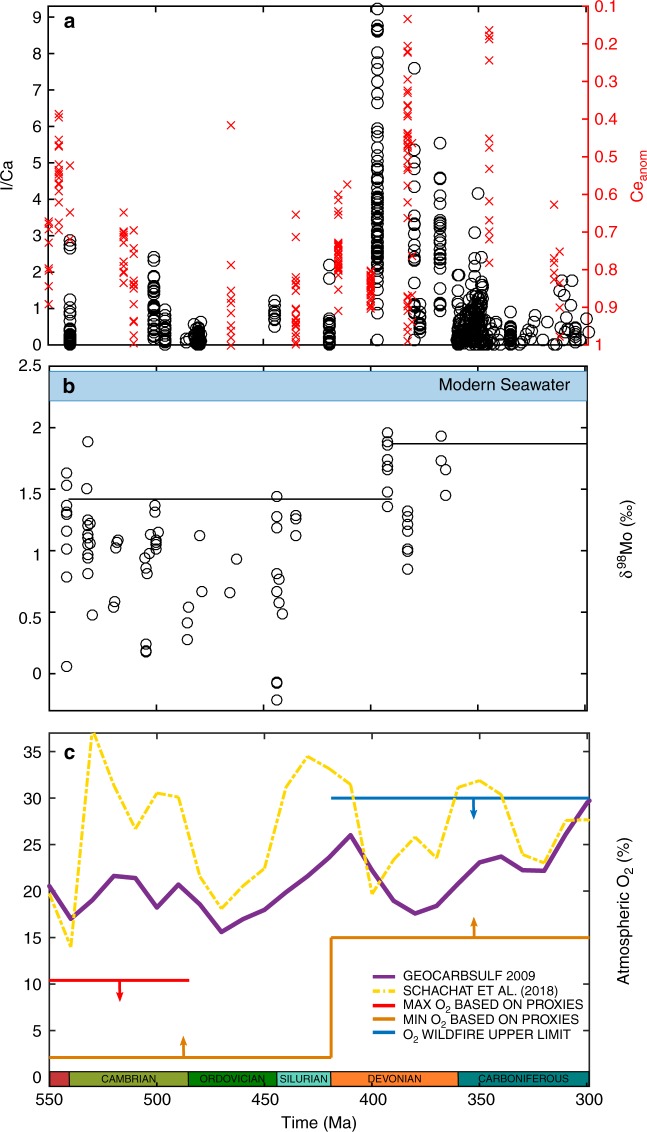
Fig. 1.
Atmospheric O2 levels during the early-mid Paleozoic predicted by GEOCARBSULF, compared to geochemical proxy data. a Iodine to calcium ratios50, and cerium anomaly data15, taken from marine carbonates. Note: higher iodine to calcium ratios, and lower cerium anomaly values indicate oceanic oxygenation. b Molybdenum isotope values for the early to mid-Paleozoic, where the solid black lines represent 90% percentiles for the two time periods11. c The purple line represents the atmospheric O2 output from GEOCARBSULF21, the yellow dot-dash line shows recent work to update the δ13C record in GEOCARBSULF30, and the red line shows the approximate maximum atmospheric O2 (10.4% atm) for the Cambrian and earlier, based on geochemical water column redox data and allowing for modelling uncertainties14,72,73. The orange line is the approximate O2 minimum, based on the same redox data, as well as Cambrian biota and wildfire oxygen requirements2,4,14. The blue line is a likely oxygen maximum, based on wildfire feedbacks, but geochemical mass balance studies suggest pO2 levels as high as 35% atm may be permissible4,68,74
To summarize, GEOCARBSULF is the most widely-used resource for reconstructing Phanerozoic oxygen concentrations, but the severe conflict with proxy records for the Paleozoic highlights that there may be a major problem in the model construction. Here we revisit GEOCARBSULF and challenge some of the underlying assumptions of the model, to ultimately produce a revised model and a new high-resolution reconstruction of Phanerozoic atmospheric O2. Our new reconstruction differs considerably from the original and is compatible with available proxy records, supporting a step-change in O2 during the Paleozoic.
Results
Paleozoic oxygen controls
Box models calculate the concentration of atmospheric oxygen by estimating the geological source and sink terms. These sources and sinks are related to the cycling of carbon and sulphur: both cycles produce reduced species (photosynthetic organic carbon and pyrite sulphur derived from microbially-produced H2S) which, when buried in sediments, leads to net oxygenation of the surface system, following Eqs. (1) and (2):33
| 1 |
| 2 |
When these sediments are oxidatively weathered—usually following atmospheric exposure due to uplift—or are subject to metamorphism/diagenesis, the reverse of Eqs. (1) and (2) takes place, and pyrite sulphur and organic carbon are oxidised, thus decreasing the amount of free oxygen in the system. Ultimately, the concentration of atmospheric oxygen is determined by the rates of burial of organic C and pyrite S, versus the consumption of oxygen by the weathering of these same reduced species over geologic time34.
The GEOCARBSULF model18,19, and its predecessors33,35, calculate these burial rates using isotope mass balance (IMB). Both photosynthesis and microbial sulphate reduction bestow significant isotopic fractionation effects, allowing their rates to be ‘inverted’ from the known isotopic compositions of sedimentary carbonates and sulphates. However, a major uncertainty in these models is the degree to which these isotopic fractionation effects are dependent on global O2 levels. Such a dependence of fractionation on oxygen concentration provides stabilizing negative feedback in the model, whereby higher O2 levels imply a higher degree of carbon and sulphur isotopic fractionation, which decreases the rate of organic C and pyrite burial calculated by the inversion approach, as less organic C or pyrite needs to be buried to achieve the same change in global isotope ratios.
The fractionation between carbonates and organic carbon is reasonably well understood from plant and plankton growth experiments and theory36–38, and thus a simple equation fitted to the data is possible. By contrast, the fractionation of sulphur isotopes between sulphate and sulphide (αs) due to bacterial sulphate reduction, is a complex process. Only ~5–20% of sulphide produced by sulphate reduction in shelf sediments is permanently buried as pyrite, with the remainder subject to disproportionation or reoxidation to sulphate via numerous byzantine pathways, with each step likely impacting upon the isotopic signature35,39–41.
For GEOCARBSULF, the sulphur isotope system was simplified and a sulphur isotope fractionation equation was derived (see supplementary equation (56)) based on the simple observation of larger fractionations at higher O2 levels42, with the underlying principle being that recycling of sulphur via reoxidation and disproportionation produced the high sulphur fractionations that were not observed in existing laboratory experiments of bacterial sulphate reduction43–45. More recent experimental studies (e.g., Sim et al.46) have, however, shown that large sulphur isotope fractionations can be obtained from microbial sulphate reduction alone, without the need for disproportionation, although the significance of this contribution to the global sulphur isotope fractionation record over geologic time remains unclear. Furthermore, as Jørgensen and Nelson39 note, pyrite can be oxidized in anoxic settings through abiotic pathways (e.g., via manganese oxides), and thus the disproportionation pathway for large sulphur isotope fractionation is not necessarily dependent on higher pO2 levels. Additionally, the source of sulphate and its isotopic signature are important to know, in order to derive fractionation values. While most sulphate in marine settings comes from bottom water sulphate, some fraction comes from the oxidation of organic sulphur compounds in particulate organic matter47. A lot of work has gone into elucidating the cryptic nature of the marine sulphur cycle, but it remains difficult to determine a quantitative relationship between oxygen concentration and sulphur isotope fractionation on a global scale, due to the nature of this cycling, which may have also changed over geologic time. Consequently, the equation used in GEOCARBSULF contains a considerable amount of uncertainty.
Crucially, the formulation chosen results in a very strong O2 feedback from the sulphur cycle in GEOCARBSULF. As pO2 levels begin to decrease, the sulphur isotope fractionation value calculated by the model also decreases. This smaller fractionation results in an increase in pyrite burial calculated from IMB, and subsequently, a rise in O2 production (see Fig. 2). Atmospheric oxygen levels <10% atm therefore result in an unrealistically low sulphur isotope fractionation (less than 10‰), and generate extremely large (~1–2 orders of magnitude higher than present day) amounts of pyrite burial. The concentration of atmospheric oxygen is thus tightly constrained by the sulphur cycle in the model, resulting in Paleozoic pO2 values that are close to present day (see Fig. 1), with low values of atmospheric oxygen being unobtainable.
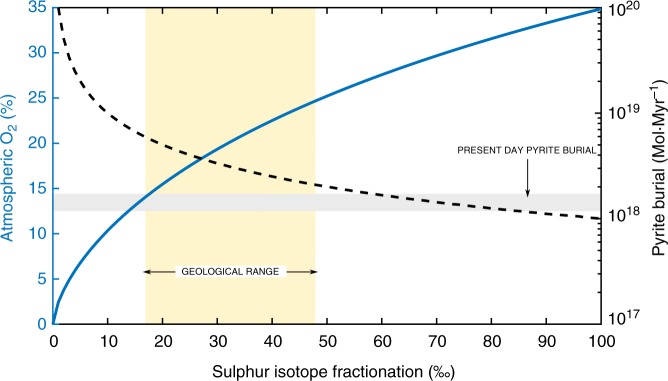
Fig. 2.
The relationship between sulphur isotope fractionation and oxygen concentration in GEOCARBSULF. The blue line shows the sulphur isotope fractionation (αs) generated by the equation used in the original GEOCARBSULF model for different concentrations of atmospheric O2, and the dashed black line shows the resultant pyrite burial flux. The yellow box represents the bounds of the geologic record for sulphur isotope fractionation between sulphate and pyrite over the last 570 Myrs, based on datasets from Wu et al.66 and the grey box represents estimated modern day pyrite burial levels75,76
Model development
We update the GEOCARBSULF model to incorporate a process-based sulphur cycle that does not depend on inverting the δ34S isotope record to calculate fluxes, and therefore does not rely on estimating oxygen-related changes in the fractionation factor. To do this we replace the equations which calculate the weathering and burial rates of both reduced (i.e., pyrite) and oxidized (i.e., gypsum) sulphur species with those used in the COPSE model24. In this modified system, the O2 source from pyrite burial depends on the concentration of oceanic sulphate, the supply of organic matter to sediments, and the average oceanic dissolved oxygen concentration, thus retaining some level of pO2-dependent feedback (see ‘Methods’ and Supplementary Note 3 for formulations). We also make modifications to the model which follow previous work: the carbon isotope record used to drive the model is updated to the latest compilation32,48, and oxidative weathering of fossil organic carbon is assumed to be sensitive to pO249. Rather than alter existing code, we build the new model from scratch in MATLAB, incorporating a variable-step, variable-order, stiff ODE solver which improves the resilience of the model. We name the new model GEOCARBSULFOR, indicating that it uses a ‘forwards’ sulphur system, wherein sulphur cycle dynamics are calculated from other model processes, instead of the δ34S record. The isotope record is used instead for model validation. See ‘Methods’ for full details and model equations for the amended sulphur system, and Supplementary Notes 2 and 3 for the full list of model parameters and equations.
Model predictions
Our GEOCARBSULFOR model generates significantly different predictions for Phanerozoic pO2 levels, relative to those generated by the original GEOCARBSULF model (Fig. 3). This is particularly evident in the early Paleozoic, where O2 levels from GEOCARBSULFOR are much lower than the modern-day levels predicted by the original, and generally agree with geochemical redox proxies for this time period11,14,15,50. A number of O2 variations in the new model can be linked to geobiological events, denoted with numbers in Fig. 3. The model calculates a transient rise in oxygen coincident with the Cambrian explosion (#1), which relaxes as bioturbation intensifies during Cambrian Stages 2 to 451 (#2). Bioturbation likely resulted in the increased burial of bioavailable phosphorus in sediments, thus limiting primary productivity and oxygen production, and exposed organic carbon and pyrite buried in anoxic sediments, to the overlying oxic water column, which led to oxidation51,52. A second transient increase in pO2 begins around 500 Ma, driven in the model by the Steptoean positive carbon isotope excursion (#3), which suggests high rates of organic carbon burial and subsequently oxygen production53.
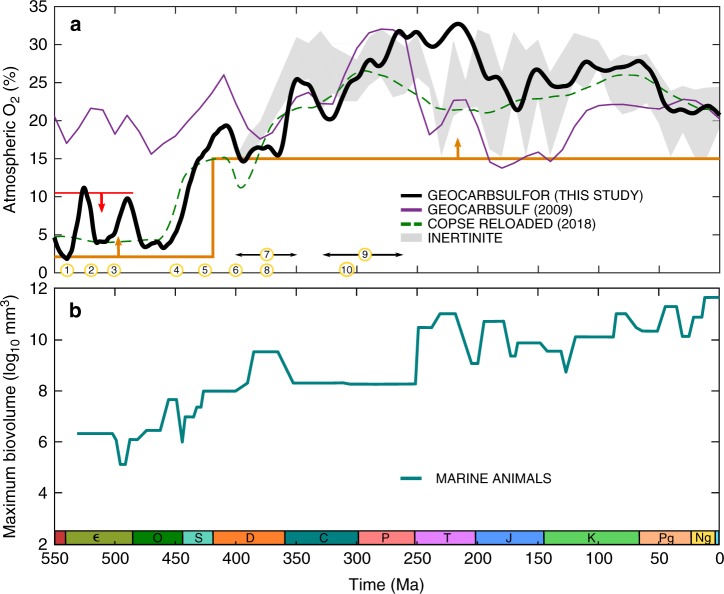
Fig. 3.
Results of GEOCARBSULFOR compared to GEOCARBSULF, the COPSE model, and to geochemical proxies. a The black line represents the results from GEOCARBSULFOR, the purple line is the original GEOCARBSULF21 and the green dashed line is COPSE22. The grey envelope shows pO2 predictions from inertinite data3, and the arrowed lines show the boundaries from Fig. 1. The numbered circles denote: (1) the beginning of the Cambrian explosion and a decrease in cerium anomaly values, (2) bioturbation intensification, (3) the Steptoean positive carbon isotope excursion event, (4) the Great Ordovician Biodiversity Event and evidence of the first land plant spores, (5) earliest evidence for vascular land plants, (6) initial evidence for a rise in δ98Mo and I/Ca and a decrease in cerium anomaly values, (7) a reduction in abundance of fossilized charcoal, (8) the first forests, (9) widespread mires and (10) a collapse in coal forests, and a rise to dominance of tree ferns4,15,50,51,53,55,56,61. b Marine animal body size54 evolution over the Phanerozoic. Є Cambrian, O Ordovician, S Silurian, D Devonian, C Carboniferous, P Permian, T Triassic, J Jurassic, K Cretaceous, Pg Paleogene, Ng Neogene
GEOCARBSULFOR predicts a clear rise in pO2 during the mid-Paleozoic at the time of the Great Ordovician Biodiversity Event (#4). At roughly the same time, the maximum biovolume of marine animals began to increase from 106 mm3 to >109 mm3 in the Devonian (Fig. 3b), which ties in with the radiation of large predatory fish that had increased oxygen demands11,54. While atmospheric O2 concentration oscillated between ~2 and 11% atm during the Cambrian to mid-Ordovician, the mid-Paleozoic rise (the timing of which is in broad agreement with recent work7,22,25,55,) to near modern-day levels in the Devonian coincides with the rise of vascular plants (#5). This marked a transition to an Earth system with pO2 levels generally above 15%—the level required to sustain smouldering fires2 and a level complemented by the results from several novel geochemical proxies (#6)11,15,50. A nadir of ~14.5% atm in the mid-Devonian (across the Emsian-Eifelian boundary at ~393 Ma) and generally lower pO2 predictions during the mid-Emsian to end Famennian stages (~400–360 Ma) are compatible with the scarcity (but not total absence) of fossilized charcoal (#7) during this period, and with both modelling and experimental work exhibiting the low probability of achieving combustion when pO2 < 19% atm2,4,56,57.
GEOCARBSULFOR, and the original GEOCARBSULF, are driven primarily by the carbon isotope record, and as such, the model distinguishes a major change in the carbon cycle from the Ordovician to Carboniferous periods, which we attribute to the expansion of land plants and the rise of the first forests (#8), due in part, to terrestrial biomass having a much greater C:P ratio than marine organic matter24. This is consistent with the biosphere driven method of COPSE22, which in a recent iteration of the model, assumes an increase in total organic carbon burial with the rise of land plants from ~470 Ma onwards, generating a rise in pO2 at this time (green dashed line in Fig. 3). The MAGic model, on the other hand, assumes that land plant material makes a relatively small contribution to total organic carbon burial today—which contrasts with data suggesting a large contribution58,59. Therefore, in MAGic, land plant evolution has little effect on organic carbon burial, and thus does not replicate this oxygenation event23.
In the mid-Carboniferous (Bashkirian, 323 Ma), the pO2 output from GEOCARBSULFOR briefly dips to ~20%, having been >25% in the early Mississippian. This decrease generally matches a drop in sea level at the time60, which possibly exposed previously buried organic C to oxidative weathering. Following this, pO2 levels rise once more to >20%, and this is concurrent with the appearance of widespread mires (#9), firstly in Euramerica, and then in Gondwana, Cathaysia and Angara, which provided ideal conditions for enhanced preservation of buried organic C4,57. Towards the end of the Carboniferous there was a collapse in the coal forests (#10), and opportunistic tree ferns took over as the dominant land plant species, probably due to aridification61. Despite this change to vegetation, pO2 levels in our model do not drop, as swamp and mire conditions remained prevalent until near the end of the Permian, and thus organic C continued to be preserved4.
The model results match well those from the oxygen proxy from inertinite data3—which back calculates pO2 based on charcoal abundance in mire settings—for most of the Phanerozoic, and provides a better fit to this proxy than the original GEOCARBSULF. However, there is a large discrepancy between GEOCARBSULFOR and this inertinite proxy, and between both GEOCARBSULFOR and GEOCARBSULF/COPSE, in the Triassic. The original GEOCARBSULF predicts a rapid decline in pO2 at the Permian-Triassic boundary, and while no early Triassic coals have yet been found, the inertinite proxy suggests that pO2 would have declined substantially (to ~18.5%) by the mid-Triassic3. Yet, GEOCARBSULFOR suggests that atmospheric oxygen was around 30% for this entire period.
There are two reasons for this conflict: first, the isotope record used by the original GEOCARBSULF indicated a ~4‰ decrease in δ13C at the end of the Permian, whereas the more recent records used for GEOCARBSULFOR show a decrease of only ~1.5‰. As such, there is a rapid decline in the amount of organic carbon buried in GEOCARBSULF, which is not matched to the same degree in GEOCARBSULFOR. Uncertainties surrounding the carbon isotope record are discussed further below. Secondly: pyrite burial in GEOCARBSULFOR is now partially dependent on the amount of sulphate in the ocean. During the Triassic, GEOCARBSULFOR predicts—and is validated by geochemical proxies and other modelling work (see Supplementary Fig. 4)—quite high levels of sulphate, with only a moderate decline over this period. This means pyrite burial rates for GEOCARBSULFOR do not decrease as substantially as they did in GEOCARBSULF, as sulphate levels buffer, to a degree, the changes to the organic carbon burial rates at this time. In the latest version of COPSE22, the C:P land plant stoichiometry was updated, to factor in a decrease in C:P (from 2000:1 to 1000:1) over 345–300 Ma, and a new forcing: coal basin depositional area, was introduced, with a high depositional area in the Carboniferous and a sharp decline at the end of the Permian. These forcings in the COPSE model serve to keep predicted pO2 levels much lower than those by GEOCARBSULFOR, over the end of the Permian and the Triassic.
Discussion
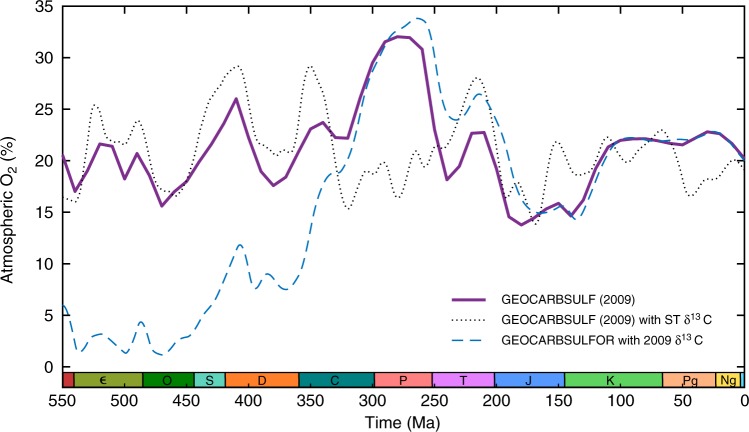
The key differences between the Paleozoic predictions of GEOCARBSULFOR and the original GEOCARBSULF model arise from the modified sulphur cycle, rather than the new isotope data. We tested this by inputting the new δ13C record, taken from Saltzman and Thomas48, into the original GEOCARBSULF model, and then using the δ13C record from GEOCARBSULF21 to drive GEOCARBSULFOR (see Fig. 4). Using the new C isotope data in the original model results in early Paleozoic pO2 levels which are broadly similar to those from the original model. However, using the older isotope record in our new model produces low pO2 in the early Paleozoic, followed by near identical results from the mid-Carboniferous onwards. This leads us to conclude that atmospheric oxygen levels in the early Paleozoic are highly dependent on the sulphur cycle, although the carbon cycle helps to constrain some of the timings and magnitude of changes to pO2.
Fig. 4.
Sulphur cycle versus carbon isotope modifications. The purple line is the original GEOCARBSULF pO2 prediction21, the black dotted line is the original GEOCARBSULF model but with the δ13C record updated to use data from Saltzman and Thomas48, and the blue dashed line is GEOCARBSULFOR but with the isotope data from the original GEOCARBSULF model
As our model computes the sulphur cycle mechanistically, rather than relying on isotope inversion, it can output a synthetic δ34S record, which may be compared to geological data. The model output does not reproduce the full degree of variability in measured δ34S, but it does remain within, or very close to, the data range defined by the record, giving confidence that the modified sulphur cycle is an accurate representation (Supplementary Fig. 1).
Several uncertainties regarding O2 levels are still inherent within the model; including the definitions of the mass fluxes and uncertainties in the carbon isotope record itself. The carbon cycle, primarily via organic carbon burial, in GEOCARBSULF is heavily dependent on the δ13C record input into the model. The compilation by Saltzman and Thomas48 provides a comprehensive set of data for all geologic time, but as they acknowledge, differences in the materials analysed (calcareous organisms, bulk versus component), sediment sources (epeiric seas vs. open oceans), temperature, diagenetic alteration (in the case of high Mg calcite brachiopod shells) etc., can demonstrate variability in the isotopic signature they produce32,62,63.
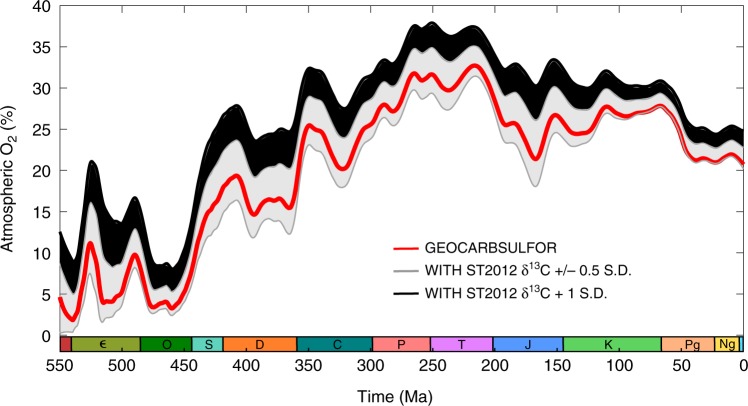
We conducted a sensitivity analysis to test the effect of variations in the δ13C dataset on pO2 predictions for our model (see Fig. 5). An initial attempt was made to run the model with a ±1 standard deviation to the δ13C record, with all other parameters remaining as they were in our baseline run (GEOCARBSULFOR—red line in Fig. 5). The model deals adequately with the plus one standard deviation change, however, the minus one standard deviation results in model failure almost immediately, as pO2 crashes to 0%. Although our treatment of the Saltzman and Thomas48 data has smoothed out some of the more extreme δ13C excursions, and our numerical method has made the model more resilient, the model may be missing some additional stabilizing feedbacks that can counter particularly negative δ13C values, especially at model initiation. The model thus remains highly sensitive to the δ13C record used, but the general trend in oxygenation remains the same. Further revisions to the Saltzman and Thomas48 compilation or a different treatment of the data may, however, result in significant differences to the computed evolution of Phanerozoic oxygen levels.
Fig. 5.
Atmospheric O2 sensitivity to changes in the δ13C record. The red line is our GEOCARBSULFOR model, the grey envelope is the atmospheric O2 generated by ±half a standard deviation change to the ocean-atmosphere δ13C record, based on the Saltzman and Thomas48 data, and the black envelope is the +1 standard deviation
We performed a further sensitivity analysis, by investigating the effect of changes to the parameter, J, which is used in the model to alter the effects of oxygen concentration on carbon isotope fractionation (see supplementary equation (23)). In our baseline run, following the original model, we use J = 4. A recent study by Edwards et al.55, has used this equation to estimate atmospheric oxygen concentrations in the early Paleozoic from the δ13C record in organic C and carbonates, using values for J of 2.5 to 7.5. We compare our results in Fig. 6. GEOCARBSULFOR does not show as great a temporal variability in pO2 levels during this period, and shows considerably less sensitivity to changes in the J parameter, due to the much greater complexity of the model adding negative feedbacks that buffer against change. Nevertheless, both methods show an increase in atmospheric O2 between the mid Ordovician and early Silurian. Additional sensitivity analyses and comparisons to other work were performed to confirm the robustness of low early Paleozoic O2 and provide further validation of our model. These can be found in the Supplementary Note 1.
Fig. 6.
The effect of the parameter, J, on GEOCARBSULFOR oxygen outputs, compared to another method of calculating atmospheric oxygen. The black line is our baseline model run with J = 4. The grey dashed line is J = 5, the grey dotted line is J = 2.5, and the grey dot-dash line is J = 7.5 for our GEOCARBSULFOR model. The blue line is J = 5, the dashed line is J = 7.5 and the dotted line is J = 2.5 from Edwards et al.55. The red line shows the approximate maximum atmospheric O2 (10.4% atm) for the Cambrian and earlier, based on geochemical water column redox data and allowing for modelling uncertainties14,72,73. The orange line is the approximate O2 minimum, based on the same redox data, as well as Cambrian biota and wildfire oxygen requirements2,4,14
Overall, GEOCARBSULFOR shows a clear transition from an Earth with relatively low pO2 (in terms of % atm), to a planet with an abundance of free atmospheric oxygen, in the process paving the way for the evolution of large terrestrial fauna. This well-defined, stepwise oxygenation—a Paleozoic Oxygenation Event (POE)—was coincident with the advent of terrestrial vascular plants, which fundamentally changed the geologic oxygen cycle. Our prediction of low pO2 in the early Paleozoic removes a central disagreement between the results of GEOCARBSULF with those of other models, and between the model and geochemical proxies. More importantly, we develop here a robust, detailed model for Phanerozoic O2 evolution that shows a number of potential links between atmospheric composition and biosphere evolution. This model provides a baseline against which to further investigate these ideas, and the opportunity to extend these approaches to investigate the Precambrian and the Neoproterozoic Oxygenation Event16.
Methods
Model reconstruction
The GEOCARBSULF18,21 model was reconstructed in MATLAB and, using a four-stage implicit Runge-Kutta method, was solved numerically64,65. The Runge-Kutta method allows the model to generate outcomes with a high accuracy, whilst retaining stability, thus permitting updates to several parameters, with a reduction in model failure compared to the original model code19,64,65.
Data updates
The δ13C record was updated to use data from Saltzman and Thomas48, to which we applied a 10 Myr moving average, and the data was smoothed to eliminate variations in the short term. The δ34S record, which was required for testing our model, was updated to use data from Wu et al.66. The dimensionless parameters: land area (f_A); river runoff (f_D); proportion of land underlain by carbonates (f_L); and effect of change of paleogeography on temperature (GEOG), as well as the temperature dependence parameter for carbonate, and silicate weathering (gcm), were revised to those employed by Royer et al.19, some of which are based on the work of Goddéris et al.67. An additional parameter: the fraction of land experiencing chemical weathering (f_AW), used by Royer et al.19. was included, but we observe that their original data for this parameter was not normalized to the present, as all other parameters for GEOCARBSULF are, thus we have updated these values.
Sulphur cycle
The weathering and burial equations for young pyrite and gypsum were altered to those used in the COPSE model24.
The weathering equations follow the reasoning that most gypsum is weathered with carbonate rocks, and most pyrite is weathered with silicate rocks, therefore they can include a dependency on the carbonate and silicate weathering functions68. As COPSE doesn’t utilize the rapid recycling inherent in GEOCARBSULF, changes were made to the young weathering fluxes. The ancient weathering fluxes remained as per GEOCARBSULF, but with an oxidative feedback function added to the weathering of ancient pyrite.
The pyrite burial equation was altered to eliminate the reliance on the sulphur isotope fractionation present in GEOCARBSULF, whilst retaining an oxygen dependent feedback on the amount of pyrite buried at each time step. As the model remains close to steady state throughout its run, the gypsum burial equation was modified to reduce the limitation the original equation imposed on pyrite burial. The new weathering and burial equations are as follows:
| 3 |
| 4 |
| 5 |
| 6 |
where:
F_wp_y is the young pyrite weathering flux; F_wgyp_y is the young gypsum weathering flux; F_bp is the pyrite burial flux; and F_bgyp is the gypsum burial flux.
F_ws is the silicate weathering flux at time (t); F_ws_0 is the present day silicate weathering flux; new_kwp is the pyrite weathering rate constant; Pyr_y(t) is the size of the young pyrite reservoir at time (t); Pyr_0 is the size of the young pyrite reservoir at present day. F_wc_y is the carbonate weathering flux at time (t); F_wc_y0 is the present day carbonate weathering flux; new_kwgyp is the gypsum weathering rate constant; Gyp_y(t) is the size of the young gypsum reservoir at time (t); and Gyp_0 is the size of the young gypsum reservoir at present day. OA_S(t) is the size of the ocean sulphate reservoir at time (t); OA_S_0 is the size of the ocean sulphate reservoir at present day; F_bg(t) is the organic carbon burial flux at time (t); F_bg_0 is the organic carbon burial flux at present day; k_bp is the pyrite burial rate constant; and k_bgyp is the gypsum burial rate constant.
Calc is the normalized calcium reservoir69, which is dimensionless, as is O2mr: the normalized amount of O2 in the ocean-atmosphere reservoir. All other reservoir sizes are in moles, and fluxes are in moles per Myr.
Finally, we make some alterations to the total amount of sulphur, and the apportioning of this to the various reservoirs, in the model. In the original GEOCARBSULF, the total amount of sulphur in the system is 638 × 1018 moles. First, we reduce the total amount of sulphur in the system to 418 × 1018 moles; we have conservation of mass in the model, so this reduction allows the model to run with a total sulphur value roughly equivalent to that used by Kump and Garrels70. Next, we challenge GEOCARBSULF’s assumption that the initial sizes of the pyrite and gypsum reservoirs are equal to each other (combined young and ancient pyrite is 300 × 1018 moles, as is the combined young and ancient gypsum). Following the work of Canfield and Farquhar71, who provide evidence for a Proterozoic dominated by pyrite burial, with low gypsum deposition across the Ediacaran-Cambrian boundary, we adjust the balance of the sulphur distribution across the sedimentary reservoirs. We retain GEOCARBSULF’s ocean-atmosphere reservoir value of 38 × 1018 moles, and then start the model run at 570 Ma, with total gypsum equal to 100 × 1018 moles, and total pyrite equal to 280 × 1018 moles (see Supplementary Note 1 and Supplementary Fig. 6 for further information), thus changing the pyrite to gypsum ratio from 1:1 to 2.8:1.
Changes to other fluxes and reservoirs
The changes we made to the sulphur cycle resulted in the need to update other fluxes in the model. In the original GEOCARBSULF, degassing fluxes are contingent on spreading rates at time (t) multiplied by the present day rate, while ancient reservoirs are forced to remain at steady state throughout an entire model run. These formulations introduce a rigidity to the model’s operations, which can be a source of failure, as the model cannot stabilize itself quickly enough following large perturbations. The following changes make the model more dynamic, allowing it to respond faster to fluctuations in the system.
We modified the original equations for the degassing of ancient reservoirs of pyrite, gypsum, organic carbon, and carbonate, so the degassing flux calculated at each time step was dependent on the total amount of material in each reservoir, multiplied by a rate constant and the spreading rate at time (t), with an additional dependence on the relative proportions of carbonates on shallow platforms or the deep ocean for carbonate degassing.
The weathering equations for ancient organic carbon and ancient carbonates were also updated: replacing the terms: F_wg_a0 and F_wc_a0—the modern day weathering fluxes for ancient organic carbon and ancient carbonates respectively—with a rate constant multiplied by the total amount of material in each reservoir at each time step; we also include an oxidative feedback to the weathering equations for young and ancient organic carbon.
Finally, the equations governing the flux of material from young to ancient reservoirs at each iteration were altered, to allow the total amount stored in the ancient reservoirs to vary, instead of remaining constant over geologic time. This young to ancient flux is now dependent on the total amount stored in the respective young reservoir multiplied by a rate constant. The model remains in a steady state, but the total mass apportioned to each reservoir at each time step, by the model, has greater variance.
Code availability
The code for GEOCARBSULF, reconstructed in MATLAB, and the code for GEOCARBSULFOR (also MATLAB), both of which support this study, are available from the corresponding author upon request.
Electronic supplementary material
Acknowledgements
A.J.K. is funded by a studentship from the NERC SPHERES Doctoral Training Partnership (NE/L002574/1). B.J.W.M. is funded by a University of Leeds Academic Fellowship. S.Z. acknowledges financial support from Yale University. N.J.P acknowledges funding from Alternative Earths NASA Astrobiology Institute. S.W.P. and T.M.L. are supported by NERC (NE/P013651) and by Royal Society Wolfson Research Merit Awards.
Author contributions
A.J.K. and B.J.W.M. designed the research. A.J.K. performed the modelling. A.J.K., B.J.W.M. and S.W.P. wrote the paper, with input from S.Z., N.J.P. and T.M.L.
Data availability
The datasets required to run the models, all of which support this study, are available from the corresponding author upon request.
Competing interests
The authors declare no competing interests.
Footnotes
Publisher's note: Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Electronic supplementary material
Supplementary Information accompanies this paper at 10.1038/s41467-018-06383-y.
References
- 1.Farquhar J, Bao H, Thiemens M. Atmospheric influence of Earth’s earliest sulfur cycle. Science. 2000;289:756–758. doi: 10.1126/science.289.5480.756. [DOI] [PubMed] [Google Scholar]
- 2.Belcher CM, McElwain JC. Limits for combustion in low O2 redefine paleoatmospheric predictions for the mesozoic. Science. 2008;321:1197–1201. doi: 10.1126/science.1160978. [DOI] [PubMed] [Google Scholar]
- 3.Glasspool IJ, Scott AC. Phanerozoic concentrations of atmospheric oxygen reconstructed from sedimentary charcoal. Nat. Geosci. 2010;3:627–630. doi: 10.1038/ngeo923. [DOI] [Google Scholar]
- 4.Glasspool IJ, Scott AC, Waltham D, Pronina N, Shao L. The impact of fire on the Late Paleozoic Earth system. Front. Plant Sci. 2015;6:1–13. doi: 10.3389/fpls.2015.00756. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 5.Arnold GL, Anbar AD, Barling J, Lyons TW. Molybdenum isotope evidence for widespread anoxia in mid-proterozoic oceans. Science. 2004;304:87–90. doi: 10.1126/science.1091785. [DOI] [PubMed] [Google Scholar]
- 6.Poulton SW, Fralick PW, Canfield DE. The transition to a sulphidic ocean ~1.84 billion years ago. Nature. 2004;431:173–177. doi: 10.1038/nature02912. [DOI] [PubMed] [Google Scholar]
- 7.Stolper DA, Keller CB. A record of deep-ocean dissolved O2 from the oxidation state of iron in submarine basalts. Nature. 2018;553:323–327. doi: 10.1038/nature25009. [DOI] [PubMed] [Google Scholar]
- 8.Brocks JJ, et al. Biomarker evidence for green and purple sulphur bacteria in a stratified Palaeoproterozoic sea. Nature. 2005;437:866–870. doi: 10.1038/nature04068. [DOI] [PubMed] [Google Scholar]
- 9.Scott C, et al. Tracing the stepwise oxygenation of the Proterozoic ocean. Nature. 2008;452:456–459. doi: 10.1038/nature06811. [DOI] [PubMed] [Google Scholar]
- 10.Frei R, Gaucher C, Poulton SW, Canfield DE. Fluctuations in Precambrian atmospheric oxygenation recorded by chromium isotopes. Nature. 2009;461:250–253. doi: 10.1038/nature08266. [DOI] [PubMed] [Google Scholar]
- 11.Dahl TW, et al. Devonian rise in atmospheric oxygen correlated to the radiations of terrestrial plants and large predatory fish. Proc. Natl Acad. Sci. 2010;107:17911–17915. doi: 10.1073/pnas.1011287107. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 12.Reinhard CT, et al. Proterozoic ocean redox and biogeochemical stasis. Proc. Natl Acad. Sci. 2013;110:5357–5362. doi: 10.1073/pnas.1208622110. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 13.Guilbaud R, Poulton SW, Butterfield NJ, Zhu M, Shields-Zhou GA. A global transition to ferruginous conditions in the early Neoproterozoic oceans. Nat. Geosci. 2015;8:466–470. doi: 10.1038/ngeo2434. [DOI] [Google Scholar]
- 14.Sperling EA, et al. Statistical analysis of iron geochemical data suggests limited late Proterozoic oxygenation. Nature. 2015;523:451–454. doi: 10.1038/nature14589. [DOI] [PubMed] [Google Scholar]
- 15.Wallace MW, et al. Oxygenation history of the Neoproterozoic to early Phanerozoic and the rise of land plants. Earth. Planet. Sci. Lett. 2017;466:12–19. doi: 10.1016/j.epsl.2017.02.046. [DOI] [Google Scholar]
- 16.Och LM, Shields-Zhou GA. The Neoproterozoic oxygenation event: environmental perturbations and biogeochemical cycling. Earth-Sci. Rev. 2012;110:26–57. doi: 10.1016/j.earscirev.2011.09.004. [DOI] [Google Scholar]
- 17.Lenton Timothy M., Daines Stuart J. Biogeochemical Transformations in the History of the Ocean. Annual Review of Marine Science. 2017;9(1):31–58. doi: 10.1146/annurev-marine-010816-060521. [DOI] [PubMed] [Google Scholar]
- 18.Berner RA. GEOCARBSULF: a combined model for Phanerozoic atmospheric O2 and CO2. Geochim. Cosmochim. Acta. 2006;70:5653–5664. doi: 10.1016/j.gca.2005.11.032. [DOI] [Google Scholar]
- 19.Royer DL, Donnadieu Y, Park J, Kowalczyk J, Goddéris Y. Error analysis of CO2 and O2 estimates from the long-term geochemical model GEOCARBSULF. Am. J. Sci. 2014;314:1259–1283. doi: 10.2475/09.2014.01. [DOI] [Google Scholar]
- 20.Berner RA. Inclusion of the weathering of volcanic rocks in the GEOCARBSULF model. Am. J. Sci. 2006;306:295–302. doi: 10.2475/05.2006.01. [DOI] [Google Scholar]
- 21.Berner RA. Phanerozoic atmospheric oxygen: new results using the geocarbsulf model. Am. J. Sci. 2009;309:603–606. doi: 10.2475/07.2009.03. [DOI] [Google Scholar]
- 22.Lenton TM, Daines SJ, Mills BJW. COPSE reloaded: an improved model of biogeochemical cycling over Phanerozoic time. Earth-Sci. Rev. 2018;178:1–28. doi: 10.1016/j.earscirev.2017.12.004. [DOI] [Google Scholar]
- 23.Arvidson RS, Mackenzie FT, Guidry MW. Geologic history of seawater: a MAGic approach to carbon chemistry and ocean ventilation. Chem. Geol. 2013;362:287–304. doi: 10.1016/j.chemgeo.2013.10.012. [DOI] [Google Scholar]
- 24.Bergman NM, Lenton TM, Watson AJ. COPSE: a new model of biogeochemical cycling over phanerozoic time. Am. J. Sci. 2004;304:397–437. doi: 10.2475/ajs.304.5.397. [DOI] [Google Scholar]
- 25.Lenton TM, et al. Earliest land plants created modern levels of atmospheric oxygen. Proc. Natl Acad. Sci. 2016;113:9704–9709. doi: 10.1073/pnas.1604787113. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 26.Budyko Michael I., Ronov Alexander B., Yanshin Alexander L. History of the Earth’s Atmosphere. Berlin, Heidelberg: Springer Berlin Heidelberg; 1987. [Google Scholar]
- 27.Falkowski PG, et al. The rise of oxygen over the past 205 million years and the evolution of large placental mammals. Science. 2005;309:2202–2204. doi: 10.1126/science.1116047. [DOI] [PubMed] [Google Scholar]
- 28.Clapham ME, Karr JA. Environmental and biotic controls on the evolutionary history of insect body size. Proc. Natl Acad. Sci. 2012;109:10927–10930. doi: 10.1073/pnas.1204026109. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 29.Lyons TW, Reinhard CT, Planavsky NJ. The rise of oxygen in Earth’s early ocean and atmosphere. Nature. 2014;506:307–315. doi: 10.1038/nature13068. [DOI] [PubMed] [Google Scholar]
- 30.Schachat SR, et al. Phanerozoic pO2 and the early evolution of terrestrial animals. Proc. R. Soc. B. 2018;285:1–9. doi: 10.1098/rspb.2017.2631. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 31.Hansen KW, Wallmann K. Cretaceous and cenozoic evolution of seawater composition, atmospheric O2 and CO2: a model perspective. Am. J. Sci. 2003;303:94–148. doi: 10.2475/ajs.303.2.94. [DOI] [Google Scholar]
- 32.Mills BJW, Belcher CM, Lenton TM, Newton RJ. A modeling case for high atmospheric oxygen concentrations during the Mesozoic and Cenozoic. Geology. 2016;44:1023–1026. doi: 10.1130/G38231.1. [DOI] [Google Scholar]
- 33.Berner RA. Models for carbon and sulfur cycles and atmospheric oxygen: application to Paleozoic geologic history. Am. J. Sci. 1987;287:177–196. doi: 10.2475/ajs.287.3.177. [DOI] [Google Scholar]
- 34.Garrels RM, Lerman A. Coupling of the sedimentary sulfur and carbon cycles: an improved model. Am. J. Sci. 1984;284:989–1007. doi: 10.2475/ajs.284.9.989. [DOI] [Google Scholar]
- 35.Berner RA. Modeling atmospheric O2 over Phanerozoic time. Geochim. Cosmochim. Acta. 2001;65:685–694. doi: 10.1016/S0016-7037(00)00572-X. [DOI] [Google Scholar]
- 36.Berner RA. Isotope fractionation and atmospheric oxygen: implications for Phanerozoic O2 evolution. Science. 2000;287:1630–1633. doi: 10.1126/science.287.5458.1630. [DOI] [PubMed] [Google Scholar]
- 37.Beerling DJ, Osborne CP, Chaloner WG. Evolution of leaf-form in land plants linked to atmospheric CO2 decline in the Late Palaeozoic era. Nature. 2001;410:352–354. doi: 10.1038/35066546. [DOI] [PubMed] [Google Scholar]
- 38.Beerling DJ, et al. Carbon isotope evidence implying high O2/CO2 ratios in the Permo-Carboniferous atmosphere. Geochim. Cosmochim. Acta. 2002;66:3757–3767. doi: 10.1016/S0016-7037(02)00901-8. [DOI] [Google Scholar]
- 39.Jørgensen BB, Nelson DC. Sulfide oxidation in marine sediments: geochemistry meets microbiology. Geol. Soc. Am. Spec. Pap. 2004;379:63–81. [Google Scholar]
- 40.Zopfi J, Ferdelman TG, Fossing H. Thiosulfate, and elemental sulfur in marine sediments. Geol. Soc. Am. Spec. Pap. 2004;379:97–116. [Google Scholar]
- 41.Johnston DT. Multiple sulfur isotopes and the evolution of Earth’s surface sulfur cycle. Earth-Sci. Rev. 2011;106:161–183. doi: 10.1016/j.earscirev.2011.02.003. [DOI] [Google Scholar]
- 42.Canfield DE, Teske A. Late Proterozoic rise in atmospheric oxygen concentration inferred from phylogenetic and sulphur-isotope studies. Nature. 1996;382:127–132. doi: 10.1038/382127a0. [DOI] [PubMed] [Google Scholar]
- 43.Canfield DE, Thamdrup B. The production of 34S-depleted sulfide during bacterial disproportionation of elemental sulfur. Science. 1994;266:1973–1975. doi: 10.1126/science.11540246. [DOI] [PubMed] [Google Scholar]
- 44.Habicht KS, Canfield DE, Rethmeier J. Sulfur isotope fractionation during bacterial reduction and disproportionation of thiosulfate and sulfite. Geochim. Cosmochim. Acta. 1998;62:2585–2595. doi: 10.1016/S0016-7037(98)00167-7. [DOI] [Google Scholar]
- 45.Sørensen KB, Canfield DE. Annual fluctuations in sulfur isotope fractionation in the water column of a euxinic marine basin. Geochim. Cosmochim. Acta. 2004;68:503–515. doi: 10.1016/S0016-7037(03)00387-9. [DOI] [Google Scholar]
- 46.Sim MS, Bosak T, Ono S. Large sulfur isotope fractionation does not require disproportionation. Science. 2011;333:4–6. doi: 10.1126/science.1205103. [DOI] [PubMed] [Google Scholar]
- 47.Fakhraee M, Li J, Katsev S. Significant role of organic sulfur in supporting sedimentary sulfate reduction in low-sulfate environments. Geochim. Cosmochim. Acta. 2017;213:502–516. doi: 10.1016/j.gca.2017.07.021. [DOI] [Google Scholar]
- 48.Saltzman MR, Thomas E. Carbon Isotope Stratigraphy. Geol. Time Scale 2012. 2012;1–2:207–232. doi: 10.1016/B978-0-444-59425-9.00011-1. [DOI] [Google Scholar]
- 49.Daines SJ, Mills BJW, Lenton TM. Atmospheric oxygen regulation at low Proterozoic levels by incomplete oxidative weathering of sedimentary organic carbon. Nat. Commun. 2017;8:1–11. doi: 10.1038/ncomms14379. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 50.Lu W, et al. Late inception of a resiliently oxygenated upper ocean. Science. 2018;5372:1–8. doi: 10.1126/science.aar5372. [DOI] [PubMed] [Google Scholar]
- 51.Boyle RA, et al. Stabilization of the coupled oxygen and phosphorus cycles by the evolution of bioturbation. Nat. Geosci. 2014;7:671–676. doi: 10.1038/ngeo2213. [DOI] [Google Scholar]
- 52.van de Velde S, Mills BJW, Meysman FJR, Lenton TM, Poulton SW. Early Palaeozoic ocean anoxia and global warming driven by the evolution of shallow burrowing. Nat. Commun. 2018;9:1–10. doi: 10.1038/s41467-017-02088-w. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 53.Saltzman MR, et al. Pulse of atmospheric oxygen during the late Cambrian. Proc. Natl Acad. Sci. 2011;108:3876–3881. doi: 10.1073/pnas.1011836108. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 54.Heim NA, Knope ML, Schaal EK, Wang SC, Payne JL. Cope’s rule in the evolution of marine animals. Science. 2015;347:867–871. doi: 10.1126/science.1260065. [DOI] [PubMed] [Google Scholar]
- 55.Edwards CT, Saltzman MR, Royer DL, Fike DA. Oxygenation as a driver of the Great Ordovician Biodiversification Event. Nat. Geosci. 2017;10:925–930. doi: 10.1038/s41561-017-0006-3. [DOI] [Google Scholar]
- 56.Belcher, C. M., Collinson, M. E. & Scott, A. C. in Fire Phenomena and the Earth System: An Interdisciplinary Guide to Fire Science (ed. Belcher, C. M.) 229–249 (Wiley-Blackwell, Hoboken, NJ, 2013).
- 57.Scott AC, Glasspool IJ. The diversification of Paleozoic fire systems and fluctuations in atmospheric oxygen concentration. Proc. Natl Acad. Sci. 2006;103:10861–10865. doi: 10.1073/pnas.0604090103. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 58.Burdige DJ. Burial of terrestrial organic matter in marine sediments: a re-assessment. Glob. Biogeochem. Cycles. 2005;19:1–7. doi: 10.1029/2004GB002368. [DOI] [Google Scholar]
- 59.Burdige DJ. Preservation of organic matter in marine sediments: controls, mechanisms, and an imbalance in sediment organic carbon budgets? Chem. Rev. 2007;107:467–485. doi: 10.1021/cr050347q. [DOI] [PubMed] [Google Scholar]
- 60.van der Meer DG, et al. Reconstructing first-order changes in sea level during the Phanerozoic and Neoproterozoic using strontium isotopes. Gondwana Res. 2017;44:22–34. doi: 10.1016/j.gr.2016.11.002. [DOI] [Google Scholar]
- 61.Sahney S, Benton MJ, Falcon-Lang HJ. Rainforest collapse triggered Carboniferous tetrapod diversification in Euramerica. Geology. 2010;38:1079–1082. doi: 10.1130/G31182.1. [DOI] [Google Scholar]
- 62.Buggisch W, Joachimski MM. Carbon isotope stratigraphy of the Devonian of Central and Southern Europe. Palaeogeogr. Palaeoclimatol. Palaeoecol. 2006;240:68–88. doi: 10.1016/j.palaeo.2006.03.046. [DOI] [Google Scholar]
- 63.Panchuk KM, Holmden CE, Leslie SA. Local controls on carbon cycling in the ordovician midcontinent region of North America, with implications for carbon isotope secular curves. J. Sediment. Res. 2006;76:200–211. doi: 10.2110/jsr.2006.017. [DOI] [Google Scholar]
- 64.Shampine LF, Reichelt MW. The MATLAB ODE Suite. SIAM J. Sci. Comput. 1997;18:1–22. doi: 10.1137/S1064827594276424. [DOI] [Google Scholar]
- 65.Ashino R, Nagase M, Vaillancourt R. Behind and beyond the MATLAB ODE Suite. Comput. Math. Appl. 2000;40:491–512. doi: 10.1016/S0898-1221(00)00175-9. [DOI] [Google Scholar]
- 66.Wu N, Farquhar J, Strauss H, Kim ST, Canfield DE. Evaluating the S-isotope fractionation associated with Phanerozoic pyrite burial. Geochim. Cosmochim. Acta. 2010;74:2053–2071. doi: 10.1016/j.gca.2009.12.012. [DOI] [Google Scholar]
- 67.Goddéris Y, Donnadieu Y, Lefebvre V, Le Hir G, Nardin E. Tectonic control of continental weathering, atmospheric CO2, and climate over Phanerozoic times. Comptes Rendus—Geosci. 2012;344:652–662. doi: 10.1016/j.crte.2012.08.009. [DOI] [Google Scholar]
- 68.Berner RA, Canfield DE. A new model for atmospheric oxygen over Phanerozoic time. Am. J. Sci. 1989;289:333–361. doi: 10.2475/ajs.289.4.333. [DOI] [PubMed] [Google Scholar]
- 69.Horita J, Zimmermann H, Holland HD. Chemical evolution of seawater during the Phanerozoic. Geochim. Cosmochim. Acta. 2002;66:3733–3756. doi: 10.1016/S0016-7037(01)00884-5. [DOI] [Google Scholar]
- 70.Kump LR, Garrels RM. Modeling atmospheric O2 in the global sedimentary redox cycle. Am. J. Sci. 1986;286:337–360. doi: 10.2475/ajs.286.5.337. [DOI] [Google Scholar]
- 71.Canfield DE, Farquhar J. Animal evolution, bioturbation, and the sulfate concentration of the oceans. Proc. Natl Acad. Sci. 2009;106:8123–8127. doi: 10.1073/pnas.0902037106. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 72.Canfield DE. A new model for Proterozoic ocean chemistry. Nature. 1998;396:450–453. doi: 10.1038/24839. [DOI] [Google Scholar]
- 73.Canfield, D. E. in Treatise on Geochemistry (eds Holland, H. D. & Turekian, K. K.) 197–216 (Elsevier, New York, 2014).
- 74.Watson, A. J. Consequences for the Biosphere of Forest and Grassland Fires. (University of Reading, 1978).
- 75.Berner RA. Burial of organic carbon and pyrite sulfur in the modern ocean: Its geochemical and environmental significance. Am. J. Sci. 1982;282:451–473. doi: 10.2475/ajs.282.4.451. [DOI] [Google Scholar]
- 76.Hu X, Cai WJ. An assessment of ocean margin anaerobic processes on oceanic alkalinity budget. Glob. Biogeochem. Cycles. 2011;25:1–11. doi: 10.1029/2010GB003859. [DOI] [Google Scholar]
Associated Data
This section collects any data citations, data availability statements, or supplementary materials included in this article.
Supplementary Materials
Data Availability Statement
The datasets required to run the models, all of which support this study, are available from the corresponding author upon request.