Stockpiled N95 Filtering Facepiece Respirator Polyisoprene Strap Performance

Abstract

Long term storage of personal protective equipment (PPE) in stockpiles is increasingly common in preparation for use during public health emergency responses. Confidence in PPE requires an understanding of the impact of time in storage on all aspects of PPE effectiveness, including protection against inward leakage. Disposable N95 filtering facepiece respirators (FFR) typically rely upon inexpensive elastomeric head straps to provide an effective seal between the filter body and the wearer’s face. Annual fit testing provides a measure of assurance that a model fresh from the manufacturer will prove effective, but seal quality may degrade during long term storage. This study examines the stability of a s election of polyisoprene elastomer straps taken from various ages of common N95 FFRs. The tension of the straps at a predetermined strain of 150% was found to differ according to age for one respirator model, though whether due to age or due to manufacturing variations could not be determined. The straps from one manufacturer were found to have notable variation in length, indicating that minor variations in strap tensile properties may not result in significant differences in respirator seal quality. Based on our observations, prolonged storage may affect the tensile properties of headstraps for some models of N95.

INTRODUCTION

Large scale respirator stockpiling began in earnest a decade ago in response to a number of high profile public health emergencies, such as the bird flu (H5N1) outbreak of 2006.[Patel et al., 2017; Toner and Waldhorn, 2006; Rebmann et al., 2009] Stockpiling will remain an integral part of preparation for future public health emergencies, but improvements in stockpile planning and implementation are still needed to ensure that public health is adequately protected. It is vital to know which supplies can be stored without becoming unreliable, and the storage conditions that can balance the longevity of the product and the economics of the stockpile holder. One method is to conduct a performance analysis of the product and determine the materials most likely to degrade and affect performance. For this work on N95 filtering facepiece respirators (N95 FFR), it was decided that the most likely component materials to degrade would be the elastomers, and the filtration media. Work is currently underway to analyze the performance of aged N95 FFR filtration media.

The population of people needing respiratory protection encompass a wide range of face sizes and shapes, and so a variety of shapes and sizes of respirators are available to provide a proper and protective fit. A poor match between the face and the respirator can allow excessive leakage of unfiltered air into the breathing space. To ensure that a selected respirator provides appropriate protection, annual fit testing is required for respirator use in Occupational Safety and Health Administration (OSHA) covered workplaces. Fit testing has been shown to be necessary to obtain high simulated workplace protection factors. [Coffey et al., 1999; Zhuang et al., 2003; Bergman et al., 2015] Fit testing must be performed on the same model and size respirator. If aging of the straps in the stockpiles considerably impacts the tensile properties, the fit on tested individuals might be compromised. For proper reliance on respirators during a public health emergency it is important to verify that aging of the straps during storage has not substantially changed the fit characteristics.

Necessary and common work activities, including head and body motions and talking, can affect the seal of a respirator as it is being worn. The standard fit test defined in OSHA respiratory protection regulations includes motions that may disrupt the faceseal, testing the ability of the masks to effect and maintain proper sealing force. [29 CFR 1910.134]

The authors are unaware of previous respirator strap studies examining the effect of storage time on strap properties. Roberge et al. [2012, 2014] performed an analysis of the tensile forces arising from N95 FFR straps, and later showed that FFR fit factors on test subjects are relatively insensitive to displacements of upper straps, which would be expected to affect not only the vector direction of the load effecting the respirator/skin seal but the overall strain on the strap- and therefore the magnitude of the load. Niezgoda et al. [2013] continued the work, using a 3-dimensional imager to determine the sealing area between the face and the N95 filtering facepiece respirators while being held in place by their straps. The tensile properties of stockpiled N95 FFR elastomeric straps must prove stable enough to provide adequate respirator sealing properties after prolonged storage. The effect of ultraviolet decontamination techniques on the breaking strength of straps was examined by Lindsley et al. [2015], finding a reduction in the tension required to break straps after exposure.

Elastomers can reversibly deform to several times the original length under an applied force but return to nearly the original length when the force is removed, making them well suited for use as filtering facepiece straps. The basis of the elastic properties of elastomers is fundamentally different from that of other elastic materials. While, for example, metal springs function by reversibly storing energy in the atomic interactions, elastomers depend on local entropy changes for their restorative force. The simplified view underlying all common models of rubber elasticity is that the important measure is the number of configurations the elastomeric network can sample at a given extension. Long sections of flexible polymer must be able to explore the local region through thermal motions of its constituent atoms. This can be connected to two aspects relevant to aging in elastomers. The requirement for relatively long linear polymer blocks makes the elastic response sensitive to relatively small changes in network connectivity and topology, while the requirement for accessible thermal motions is associated with larger free volume, with concomitant permeability to oxygen and other degradative contaminants. Both of these factors can affect the degradation rate of elastomers, changing the elastic properties of respirator straps in storage. Modern models of elastomer tensile properties relating the tension to the applied deformation typically include separate terms to account for direct crosslinks and entanglement (looping hindrances) of similar magnitude under typical conditions [Tobolsky et al., 1961]. The entanglement term is relatively unaffected by aging until late stages of degradation, with the bulk of the aging effect impacting the direct crosslinking term. [Rottach et al., 2006] Most elastomeric models treat the direct crosslinking term as a linear function of the number of crosslinks in excess of that required for bare network connectivity.

$$\text{τ}\left(\text{λ}\right)={\text{τ}}_{\text{crosslink}}\left(\text{λ},{\text{n}}_{\text{cl}}\right)+{\text{τ}}_{\text{entanglement}}\left(\text{λ}\right)$$ (1)

where τ is the tension, with crosslink and entanglement designating their separable contributions, ncl is the number of crosslinks after network formation, and λ is the uniaxial strain, itself defined as λ≡l_f/l_i, the ratio of the final and initial lengths l_f and l_i, respectively.

Elastomeric filtering facepiece straps can be divided into thermoplastics, in which the elastomeric network relies on temperature-dependent phase separation, and thermosets, in which the elastomeric network is fully connected with chemical bonds. The connections that bound the flexible sections are known as crosslinks, which can be chemical or, for thermoplastics, a polymer block which phase-separates from the elastic polymer sections. Thermoplastics are called such because if they are heated under the appropriate conditions the two polymer types will mix, eliminating the effective crosslink. While thermoplastics may change their fundamental network shape without affecting the chemistry of the component molecules, thermosets degrade through scission or creation of chemical bonds. The study focus is on straps composed of polyisoprene, a thermosetting elastomer that is the primary component of natural rubber. Test results of straps from an N95 FFR model using a thermoplastic strap will be briefly discussed.

The size and connectivity of polymer networks slow the thermodynamic equilibration of elastomers. When strained, the restorative force can decay for notable periods of time while the elastomeric network adjusts to the new configuration. [Curro and Pincus, 1983; Plazek, 1991] While the crosslinks (chemical or physical) prevent the stress from fully decaying, the stress can asymptotically approach the equilibrated value over a period that can vary from seconds to days or longer. For typical polyisoprene N95 FFR straps, measurements at 15 minutes are largely stable. [Roberge et al., 2012] The stress-strain response of the initial deformation for many elastomers, particularly those with fillers, displays a higher modulus than subsequent deformations in a phenomenon known as the Mullins effect. It is hypothesized that the decrease in modulus is due to non-reversible dewetting of the additives. The Mullins effect differs from chemical and entanglement effects in its strain dependence as well. The Mullins effect complicates the comparison of strap loads.

The tensile properties of thermosetting elastomers can be affected by both the breaking and formation of chemical bonds. The creation of new crosslinking chemical bonds tends to increase the modulus, i.e. making the material stiffer, while breaking bonds has the opposite effect and softens the elastomer. [Gillen et al., 2000] Polymers available for use in commercial products typically have a wide variety of additives to improve material stability, as well as to improve color, ease of processing, and other factors. In addition, excess curing agent may remain in an elastomer after product formation. [Rottach et al., 2006] Additives, through design or chance, impact the rate of the chemical changes in elastomers, complicating comparisons between elastomers from different sources.

The Basic Autoxidation Scheme (BAS) is a set of likely chemical reactions that describe oxidative polymer degradation. Free radicals form and react with oxygen, both disrupting polymer connectivity and forming new crosslinks. Antioxidant additives can disrupt crosslink formation and slow the process, making the degradation kinetics highly dependent upon the specific formulations of the additives in a particular sample. For thick polymers, diffusion can limit the amount of oxygen available in interior regions, although this effect is unlikely to be significant for the thickness of strands used in typical respirator straps. [Gillen et al., 1996]

The purpose of the work presented here is to evaluate the stability of the tensile properties of respirator straps. The strap forces exerted at a typical extension determined by Roberge et al. [2012] will be determined and compared.

MATERIALS AND METHODS

Samples were selected from sets of N95 FFRs with single band polyisoprene (synthetic rubber) stapled straps that had been previously purchased for testing and stored on-site for up to ten years. The storage location of the samples suffered an environmental control failure and was subject ted to higher than normal temperature and humidity for in excess of one year. Temperatures above 30◦C and humidity above 80% were observed. Samples from the first manufacturer (Manufacturer ‘A’) were from the same model, while due to limited supplies samples from the second manufacturer (Manufacturer ‘B’) were from three different models with visually similar straps. ‘A’ straps consisted of four thin separate strands (approximately 1 mm square in cross-section at rest) of polyisoprene surrounded by a braided jacket of a relatively inextensible polymer thread. One sample was carefully disassembled to compare the properties of the elastomeric strands with the jacket removed and the complete strap.

The straps were carefully cut as closely as possible to their attachment points, with care taken to avoid sample stretching during removal. The length was determined with a metal ruler, and the mass recorded using a Mettler AE 163 balance (Columbus, OH). 100 mm portions of the samples were marked using permanent ink, leaving approximately 15 mm on each side to allow for clamping in the tensile tester.

The pull profile was developed to approximate realistic extensions with a time profile designed to provide stable, repeatable, and still relevant results. An initial extension beyond the range likely to be found in a donned respirator simulates the donning process, and also allows fair comparison between samples that may have been handled differently during manufacturing and packing. The original length of the sample is 100 mm, and crosshead speed is set to 1 cm/second to match the proportional speed of the 0.5 cm/second ASTM D412 recommendation for a 5 cm sample. [ASTM D 412–16, 2016] The first pull extends the sample to 300 mm. The sample is held for 15 minutes while the tension relaxes. The sample is then alternatively returned to its original extension for 45 minutes and extended to 200 mm, twice its original length, for an additional 45 minutes, for two cycles. The pull profile was entered into the Bluehill software package controlling an Instron 5566A tensile tester.[Illinois Tool Works Inc., Norwood, MA, 2014] The results were analyzed using the F-test function for linear models in the R mathematical package, with the null hypothesis being that there is no age dependence on the measured tension.[R Core Team, 2017].

RESULTS

Straps samples from three FFR from each of nine cartons of Manufacturer ‘A’ FFR (upper and lower) and from eight lots of Manufacturer ‘B’ FFR (lower straps only) were selected. The length and mas s of straps from Manufacturer ‘A’ FFR are shown in Figure 1. The linear density of the straps were significantly more uniform than the separate measures, as seen in Figure 2 and Table I, suggesting that the differences in length are not due to post-assembly permanent set but rather manufacturing variations. The age of the FFR was determined through date codes on the cartons for those that had them and through analysis of the FFR lot number for those that did not.

Figure 1:

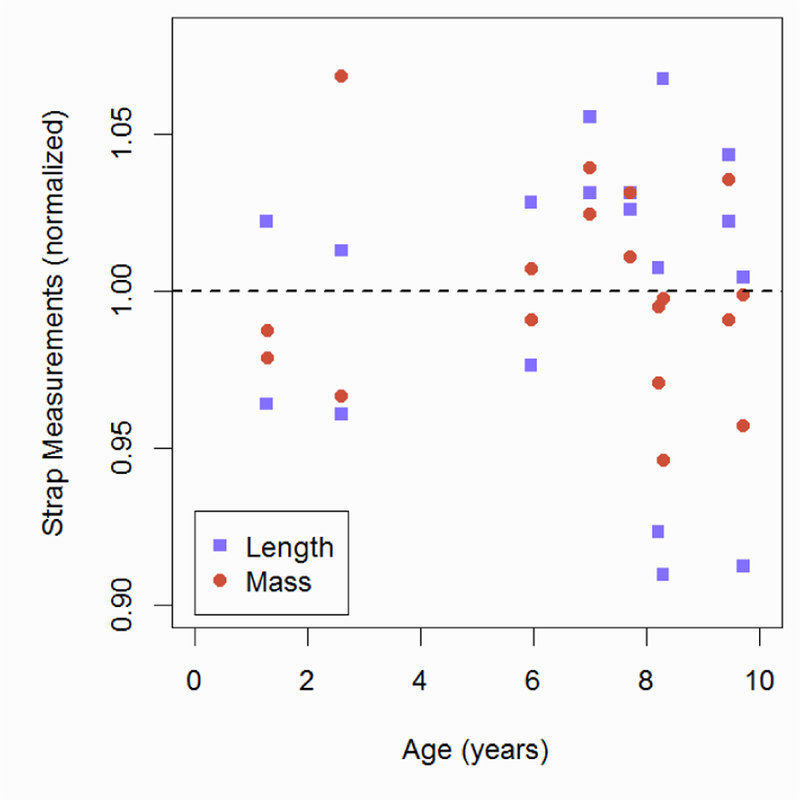
Normalized staple-to-staple length and mass for Manufacturer ‘A’ N95 respirators. Upper and lower values are normalized by averaging over the averages of the measurements for upper and lower respirator strap measurements, i.e. 29.7 mm length and 1.10 g mass for the upper, and 24.7 mm length and 0.92 g mass for the lower.

Figure 2:

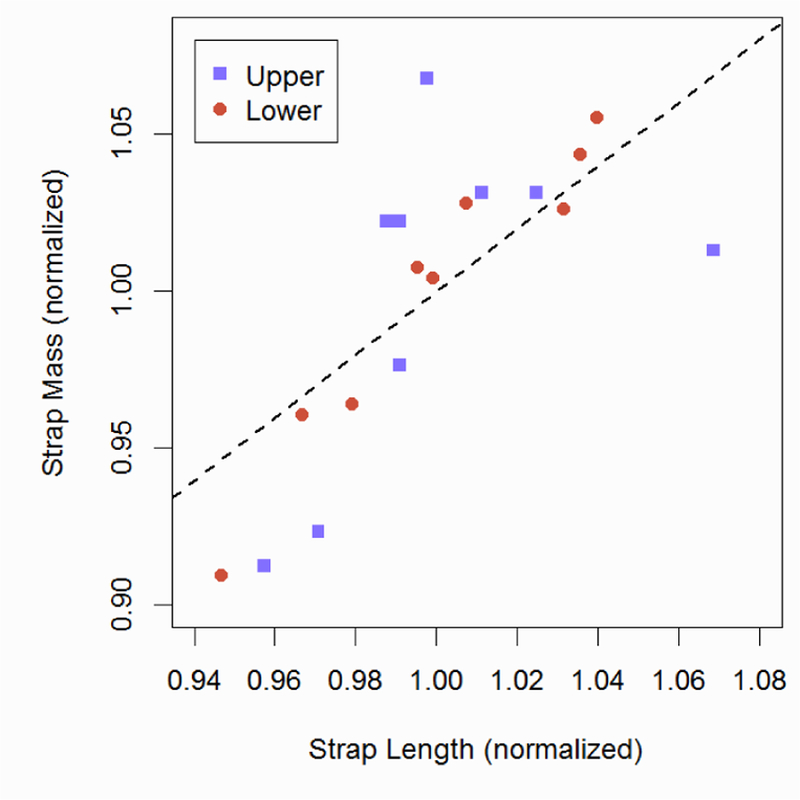
Normalized mass versus length ‘A’ straps showing a significant (p<0.01) correlation. The dotted line represents uniform density.

Table I.

Mean (±SD) Mass, Length, and Linear Density for Straps for Manufacturer ‘A’ N95 Respirators for Various Age Ranges

Age range (years)	N	Mass (g) Upper Lower		Length Upper	(mm) Lower	Linear density (g/mm) Upper Lower
All	54	1.10 (0.06)	0.92 0.04)	29.5 (0.8)	24.8 (0.8)	0.037 (0.002)	0.037(< 0.001)
1 -- 3	12	1.12 (0.01)	0.89(< 0.01)	29.9 (1.2)	24.1 (0.2)	0.037 (0.002)	0.037(< 0.001)
5 -- 8	18	1.11 (0.04)	0.95 (0.02)	29.9 (0.5)	25.4 (0.5)	0.037(< 0.001)	0.038(< 0.001)
8 -- 9	12	1.09 (0.09)	0.88 (0.05)	29.2 (0.5)	24.0 (0.7)	0.037 (0.002)	0.037 (0.001)
9 -- 10	12	1.06 (0.07)	0.94 (0.02)	28.9 (0.6)	25.1 (0.6)	0.037 (0.002)	0.037(< 0.001)

Load and extension data can be plotted against each other to produce the standard stress -strain comparison as in Figure 3. The initial pull displays typical Mullins behavior, with a higher tension at any given extension than the subsequent pulls. Roberge et al. [2012] reported a related effect when investigating strap tension over multiple donnings, finding higher tensions during initial simulated donnings than in subsequent both at the time of the pull and after 15 minutes viscoelastic relaxation. We found the subsequent pulls to be largely coincident, though the relaxations differ slightly. The non-zero extension at which the load rises is a result of a set at experimental time scales.

Figure 3:

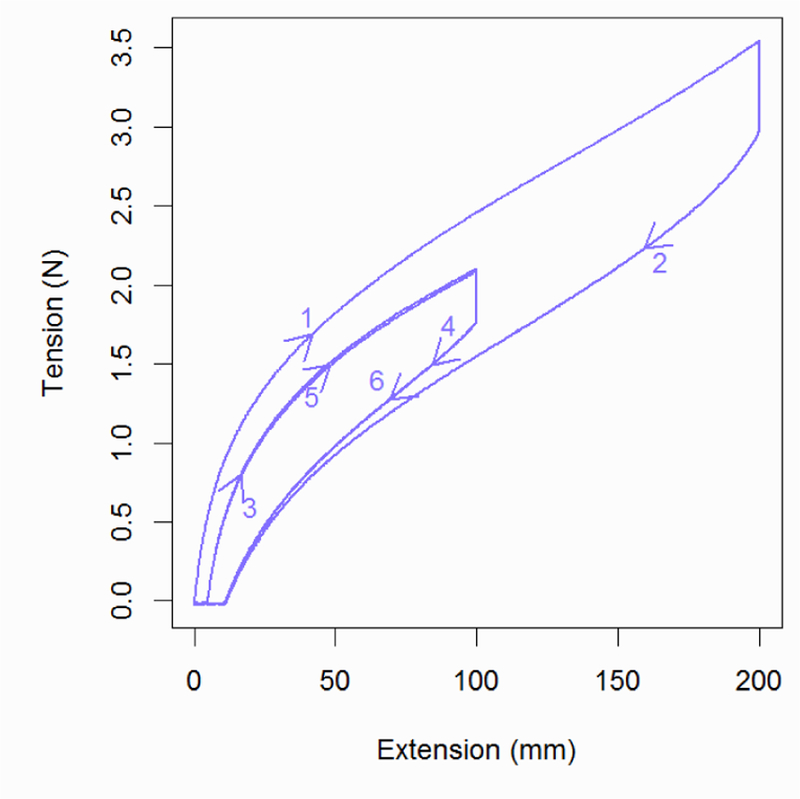
Stress-strain plot showing several stress-strain cycles for a sample from Manufacturer ‘A.’ Arrows and labels indicate the sequence, with odd numbered arrows indicating pulls and even indicating relaxations. Specific arrow placement along the curve was chosen solely for clarity. The two cycles after the first (3–4 and 5–6 on the graph) are nearly replicative.

It was found that when the jacket was removed from the Manufacturer ‘A’ samples, the strands contracted approximately 2%. At moderate strains of up to 100%, well under the ultimate limit of the inextensible jacket, the load for the complete strap was only slightly higher than the individual strands (see Figure 4). Manufacturer ‘B’ samples were single strips, approximately 8 mm × 0.5 mm at rest, with no fabric covering.

Figure 4:

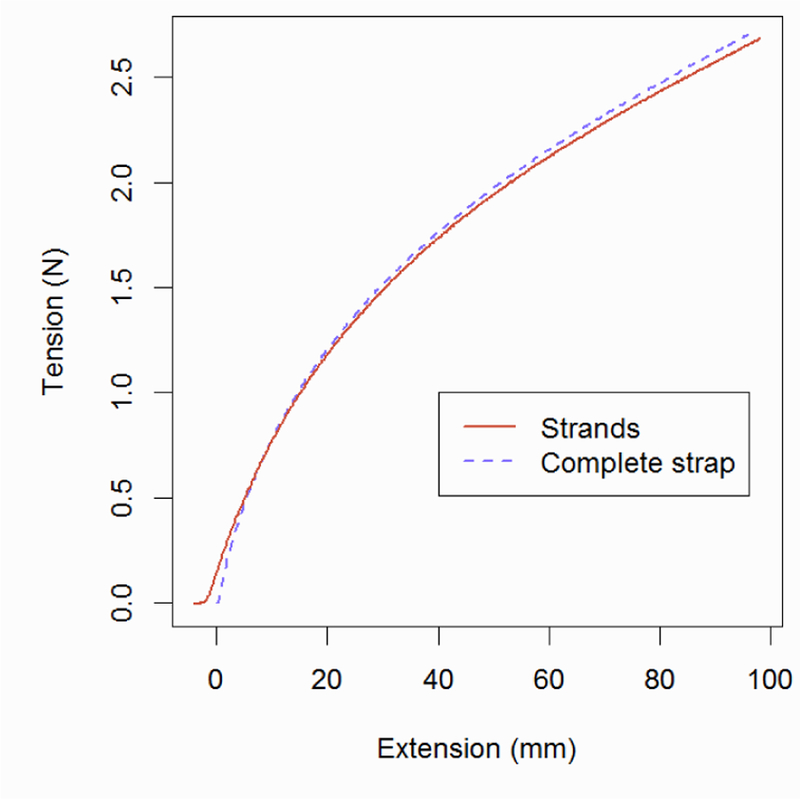
A comparison of the tensile force of the separated elastomeric component of Manufacturer ‘A’ strap with the full fabric-wrapped strap. The origins differ as the elastomeric strands contracted approximately 2% when the cover was removed.

Figure 5 shows a feather plot highlighting the extension memory characteristic of Mullins behavior. A series of pulls to increasing strains results in a set of stress -strain curves with an upper bound found by a single pull to the maximum strain and with a lower bound found by a second pull to the same maximum strain.

Figure 5:

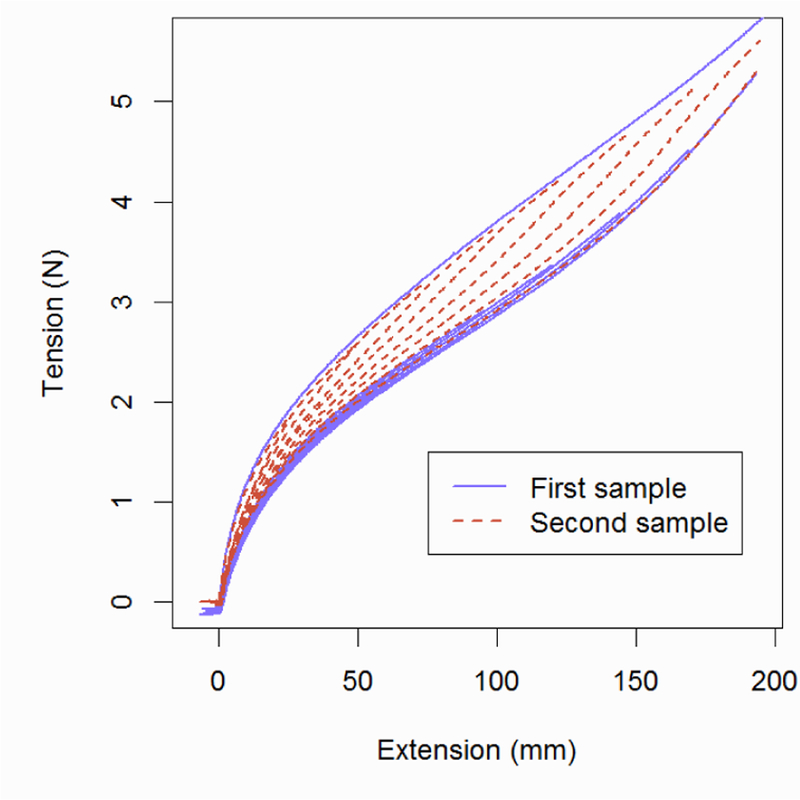
Stress-strain profile for Mullins wing plot. The outer upper line represents an initial pull for a first sample to λ=3 (nominal). Subsequent pulls of the same sample to λ=1.25 to 3 in 25% increments are largely coincident, at the lowest curves. A second sample is strained first to λ=1.25 with subsequent strains increasing in 25% increments until λ=3 on the final strain. Curves are adjusted to begin to rise at the origin, to correct for permanent set and instrument drift.

The load at an extension of 1.5 times the original length (i.e. λ=1.5) on the final pull was selected to be the point of comparison, with the results in Table II. However, given the variation in strap length, the strain needed to obtain the same overall staple-to-staple length varies between respirator samples. Figure 6 shows the load at an extension compensating for the variation in unstrained length, while keeping the average of the strains 1.5. A linear fit displays a slight but significant (p<0.01) trend toward higher tension with older samples (τ=0.060 Age N/years + 1.68 N). Higher tension with age suggests that the number of chemical crosslinks is higher in the older samples.

Table II.

Tension (±SD) at 150% Extension for Various Age Ranges of Manufacturer ‘A’ Straps

Age range	Tension (N
(years)	N	Upper	)	Combine d
All	54	2.13 (0.20)	2.07 (0.17)	2.10 (0.19)
1−−3	12	2.10 (0.09)	2.15 (0.09)	2.13 (0.07)
5−−8	18	2.09 (0.27)	2.06 (0.19)	2.08 (0.23)
8−−9	12	2.20 (0.07)	2.16 (0.07)	2.18 (0.07)
9–10	12	2.13 (0.23)	1.95 (0.22)	2.04 (0.23)

Figure 6:

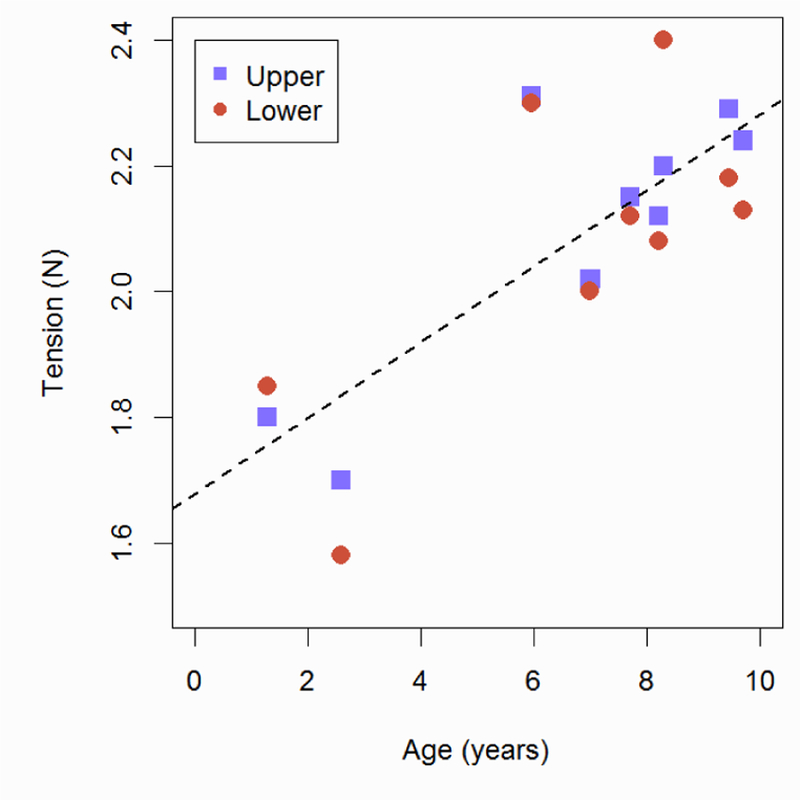
Strap load at the extension of a reference length, where the average strain is λ = 1.5, for Manufacturer ‘A’ N95 respirators.

Figure 7 shows the modulus at λ = 1.5 for straps from the ‘B’ manufacturer N95 respirators. No significant linear correlation was found at the p=0.05 confidence limit. The ‘B’ models had more uniform original strap mass, as seen in Figure 8, although the original length differed more than the mass. This may indicate that the straps were subjected to minor strains at some point before being removed from the box for testing, either during production or during packing.

Figure 7:

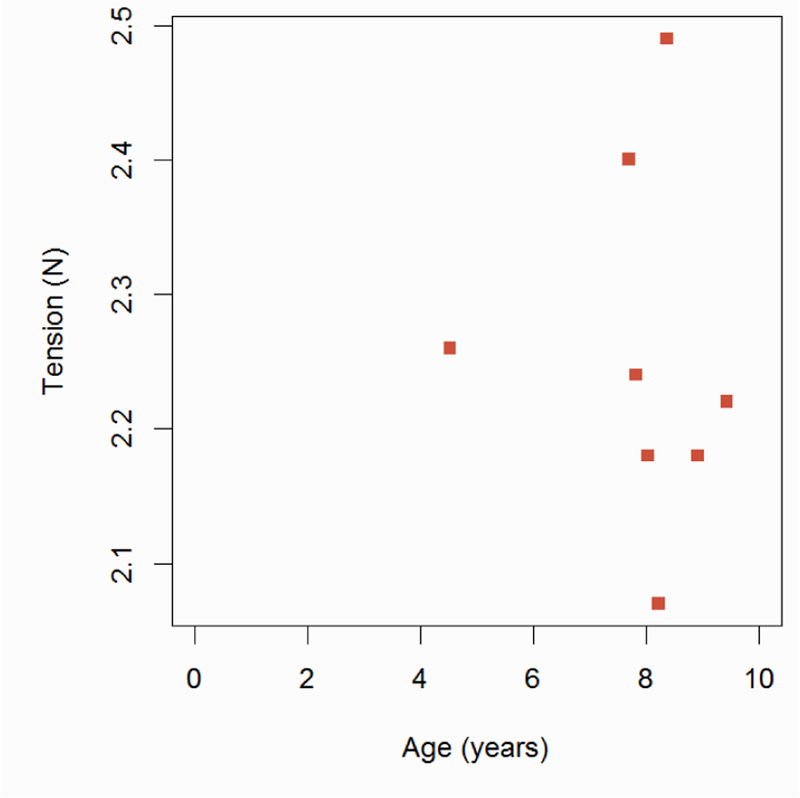
Load at λ = 1.5 for top straps of Manufacturer ‘B’ models at various ages. Three models with visually similar straps are used.

Figure 8:

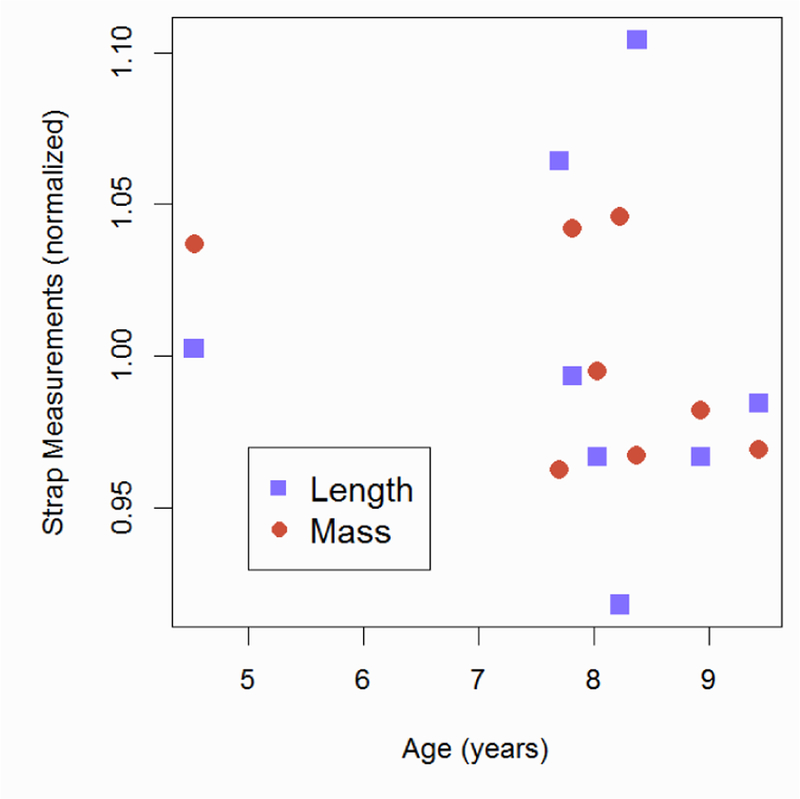
Length and mass of top strap of N95 samples of Manufacturer ‘B.’ Respirators varied in model and nominal size, but varied little in strap length. All straps were visually similar.

Thermoplastic respirator straps from a third manufacturer were not included in this study as the analysis was found to be unsuitable. The thermoplastic N95 FFR straps tested displayed a much longer viscoelastic relaxation time and more permanent set when compared with the polyisoprene straps. One sample tested over a period of several days continued to relax noticeably (as seen in Figure 9). This prolonged relaxation time prevented the easily repeatable comparison attainable with the models using thermosetting polyisoprene.

Figure 9:

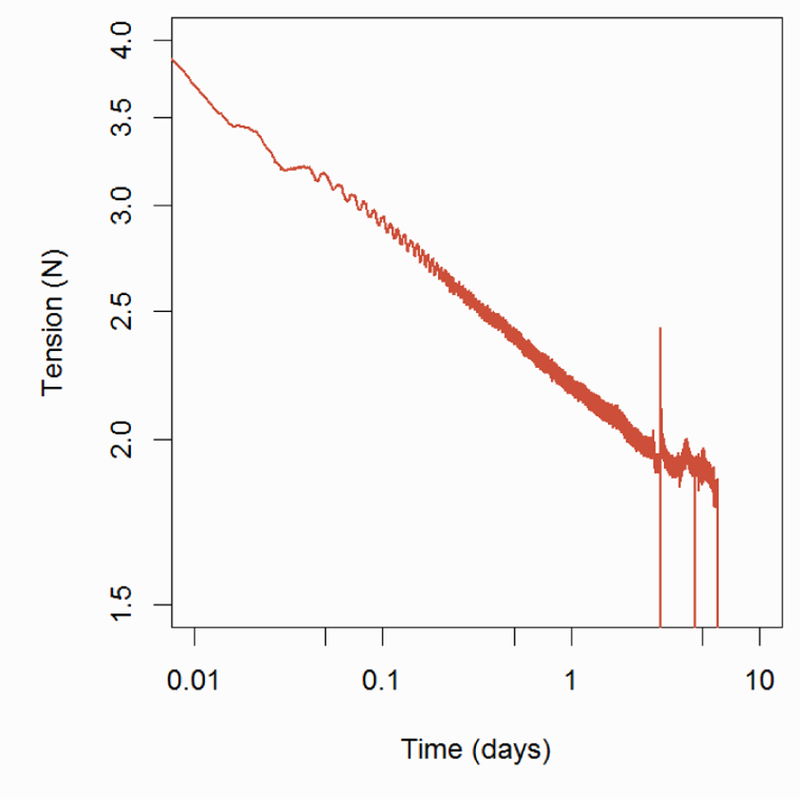
Force relaxation of a thermoplastic elastomer N95 FFR strap measured over a period of several days. The sample was held at λ = 1.5 for several days, with brief relaxation periods at 72 hours and 108 hours.

Roberge et al. [2014] studied the effect of varying the position of upper straps of respirators, and did not find a strong impact on measured fit factors. This, in addition to the variation in strap lengths at construction, implies that measured fit factors are likely insensitive to small changes in strap tension. It is likely that the minor variations in strap tension found would not lead to a measureable degradation in fit quality. Human subject testing is currently underway at NPPTL to evaluate fit factors using aged respirators.

This study has several notable study limitations. The tested samples were chosen by convenience from a storage location with poor, unmonitored environmental controls. High temperatures can increase the degradation rate of elastomers. The relationship between strap tensile properties and fit factor has not been determined. Differences in strap modulus may be counteracted during the donning process. The study compares different lots of each model without knowledge of the strap properties at the time of their manufacture. Differences in strap properties may be due to manufacturing or minor design differences between the lots. The study only focused on two types of polyisoprene straps. Other N95 FFR, including other models from the same manufacturers, use different elastic material and designs.

CONCLUSIONS

The effective tension of Manufacturer ‘A’ respirator straps shows a change over different ages, while Manufacturer ‘B’ respirator straps, also relying on polyisoprene for its elasticity shows no clear trend with age. Although there is an apparent change in the sealing force for one of the manufacturer models, the reason for the change and its effect on seal quality is yet unclear. The change may be due to either random or design variations during manufacturing, or may be due to chemical aging. Manufacturer ‘B’ straps also showed notice variation in strap masses and lengths.

The relatively high variation in strap length suggests that the respirator design would be somewhat insensitive to variation in the force at a given extension and therefore insensitive to minor variations in the modulus. Donning procedures include a ‘user seal check,’ typically performed by placing the hands over the filter surface and exhaling or inhaling sharply to verify the quality of the faceseal.[29 CFR 1910.134] This process may provide an opportunity for the user of the mask to use the feel of the mask to adjust the straps for their particular qualities, potentially mitigating the effect of minor differences in strap properties. Fit testing using human subjects is currently being performed to determine the effect of respirator age on their fit.