Binocular contrast summation and inhibition depends on spatial frequency, eccentricity and binocular disparity

Abstract

Purpose

When central vision is compromised, visually-guided behaviour becomes dependent on peripheral retina, often at a preferred retinal locus (PRL). Previous studies have examined adaptation to central vision loss with monocular 2D paradigms, whereas in real tasks, patients make binocular eye movements to targets of various sizes and depth in 3D environments.

Methods

We therefore examined monocular and binocular contrast sensitivity functions with a 26-AFC (alternate forced choice) band-pass filtered letter identification task at 2° or 6° eccentricity in observers with simulated central vision loss. Binocular stimuli were presented in corresponding or non-corresponding stereoscopic retinal locations. Gaze-contingent scotomas (0.5° radius disks of pink noise) were simulated independently in each eye with a 1000Hz eye tracker and 120Hz dichoptic shutter glasses.

Results

Contrast sensitivity was higher for binocular than monocular conditions, but only exceeded probability summation at low-mid spatial frequencies in corresponding retinal locations. At high spatial frequencies or non-corresponding retinal locations, binocular contrast sensitivity showed evidence of interocular suppression.

Conclusions

These results suggest that binocular vision deficits may be underestimated by monocular vision tests and identify a method that can be used to select a PRL based on binocular contrast summation.

Introduction

People with damaged foveae, as is the case in most patients with late-stage age-related macular degeneration (AMD), must use peripheral retina for viewing, known as the Preferred Retinal Locus (PRL) when a single location is dominant. The function of the PRL in AMD patients has generally been measured monocularly, and in the better eye, with simple tasks such fixation and perimetry dot detection (for review see ¹), or with complex tasks such as reading ² and face perception.³ However, both eyes are typically used for everyday activities and require the detection of targets at a range of sizes, contrasts and depths. The use of a PRL has rarely been investigated under these conditions. Where binocular vision has been studied, it has been shown that best monocular vision may underestimate the vision of the weaker eye ⁴ because the worse eye may be suppressed, ^5–8 that stereoacuity is impaired, ⁸ and that fixation patterns differ from those measured under monocular conditions. ⁹ These findings severely limit the conclusions that can be drawn from studies of monocular vision.

In normally-sighted observers, binocular vision has been studied extensively at the fovea and it is well-known that binocular visual function depends on a range of stimulus and task factors. ¹⁰ The simplest demonstration of binocular function is the comparison of detection thresholds under monocular and binocular viewing conditions. In central vision of healthy eyes, binocular contrast sensitivity is typically greater than the monocular contrast sensitivity of either eye and a stimulus can sometimes be detected binocularly when its contrast is too low to be detected by either of the two eyes independently. This phenomenon is known as binocular contrast summation. ^11–33 A binocular contrast summation factor of $\sqrt{2}$ is predicted in single channel systems with either linear summation of correlated signals and independent noise from each eye ¹²; or with a squaring non-linearity prior to linear combination. ¹⁷ Binocular summation may differ at and above contrast detection threshold ^{34, 35} and suprathreshold models often involve a summation stage and one or more gain control exponents; these stages can produce summation above $\sqrt{2}$. ^36–41 These models are relevant to healthy participants whose monocular foveal contrast sensitivity is similar in each eye. Previous results have demonstrated that binocular contrast summation may be reduced in older subjects ^{42, 43} or in peripheral visual field.⁴⁴

Furthermore, when interocular contrast sensitivity is dissimilar, for example with cataract, ⁴⁵ refractive error, ^{46, 47} amblyopia, ^48–50 or age-related macular degeneration, ⁵¹ binocular contrast sensitivity may be worse than the best monocular contrast sensitivity of either eye. Within the computational framework described above, a lack of binocular summation can arise from interocular suppression, ⁵² however these approaches do not account for binocular contrast summation factors that are less than 1, i.e., inhibition.

When central vision is intact, visual targets of interest are imaged at the fovea of both eyes and consequently have zero retinal disparity. Objects at depth planes that differ from that of the foveated target are imaged at non-corresponding retinal locations, either with crossed (positive) or uncrossed (negative) disparity for objects that are closer to or further from the horopter, respectively. Within a volume of space around the horopter known as Panum’s Fusional Area, objects with crossed or uncrossed disparity are seen as single, in depth ⁵³ and at full contrast. ³⁹ These observations require phase/disparity-dependent binocular gain control that modulates the apparent phase and contrast of the cyclopean image. ^{39, 54} In the present manuscript, we extend these findings to examine binocular contrast summation under conditions that are more relevant to people with central vision loss. We examine monocular and binocular contrast sensitivity as a function of spatial frequency, retinal eccentricity and retinal disparity.

Methods:

Participants

For 10 participants (age range= 19–35 years, mean = 24.1 years), we recorded the contrast sensitivity functions monocularly and binocularly in corresponding and non-corresponding retinal disparities at 2° eccentricity. One subject was author CA; two subjects were experienced psychophysical observers; and seven subjects were undergraduate students, naive to the purposes of the study, who had no previous experience with psychophysical research and completed the study for course credit. Five Subjects (two experienced and three naive observers who completed the 2° eccentricity experiment) repeated both the binocular and monocular conditions at 6° eccentricity in corresponding and non-corresponding retinal disparities. The same 10 subjects (three experienced and seven naive observers) completed a monocular control experiment. All participants passed binocular fusion Worth 4 dot screening tests (www.bernell.com/category/1064) and had normal range stereovision (Titmus Fly SO-001 StereoTest, www.stereooptical.com/product/fly/), (mean stereoacuity= 67 arcsecs). The study was approved by the Institutional Review Board at Northeastern University and conformed with the Declaration of Helsinki.

Apparatus:

Stimuli were generated on a PC using MathWorks MATLAB software (www.mathworks.com/matlabcentral/) with functions from the Psychtoolbox (www.psychtoolbox.org) ^{55, 56} and presented on an Asus VG248QE LCD monitor with screen resolution 1920×1080 pixels at 120Hz and a mean luminance of 240 cd/m², at 50 cm viewing distance. Shutter glasses (Nvidia GeForce 3D Vision, www.nvidia.com/object/3d-vision-main.html) were used to control stereoscopic stimulus presentation at 60 Hz per eye. The monitor was gamma-corrected to obtain linear output across RGB values and a bit-stealing algorithm ⁵⁷ was used to obtain 9.6 bits luminance resolution. Subpixel resolution via the graphics card provided a spatial positioning accuracy of .004 pixel, corresponding to a stereo display resolution of 0.2 arcsec.

A gaze-contingent scotoma was simulated ⁵⁸ independently for each eye by positioning a circular Gaussian (σ=0.5°) windowed patch of 100% contrast pink noise at the point of gaze of each eye on the computer display. The location of each eye was recorded with an EyeLink II eyetracker (www.sr-research.com/products/eyelink-ii) at 500Hz per eye.

Stimuli:

Contrast sensitivity was measured using a modified quickCSF algorithm ⁵⁹ that controlled the spatial frequency and contrast of 26 band-pass filtered Sloan font letters. We elected to use the full alphabet instead of a subset of letters because reducing the guessing rate greatly improves the efficiency of testing, ⁶⁰ this avoids forcing the subject to repeat a response when a target that is not in the subset is reported, and because identification differences are not avoided with subsets ^61–63 (see Hamm et al. ⁶⁴ for recent review). The quickCSF algorithm exploits the known 2D shape of the CSF, which conforms to an asymmetric log-parabola with four parameters ⁶⁵: peak gain, peak spatial frequency, low spatial frequency bandwidth and high spatial frequency bandwidth, and assumes the logarithm of the slope of the underlying psychometric function is constant across spatial frequencies.^{66, 67} Each trial, a one-step ahead search for all combinations of spatial frequency and contrast, then elects the stimulus that maximises the expected information gain. ^{68, 69} The quickCSF algorithm effectively simulates the next trial and evaluates responses to possible stimuli for their expected effects on the posterior. With this method regions of the stimulus space that are not likely to be useful to build the 2D contrast sensitivity function are avoided and trials at all spatial frequencies and contrasts contribute to the estimate of the underlying psychometric function at any spatial frequency. The quickCSF specifies the spatial frequency and contrast of the letter on each trial, each letter is digitally band-pass filtered with a raised cosine log filter with a peak spatial frequency of five cycles per letter ⁷⁰ and one octave bandwidth. The letters were scaled in size to produce the required spatial frequency on screen.

The binocular locations of the letters were either in corresponding (0° disparity), or non-corresponding retinal locations via crossed and uncrossed disparities. The horizontal disparity was scaled with stimulus size to maintain a ¼ cycle (90° phase) shift of the peak spatial frequency. This constant quadrature phase shift ensured constant depth sensitivity, ⁷¹ contrast summation ⁷² and perceived contrast ³⁹ across spatial frequencies. Corresponding and non-corresponding (crossed or uncrossed) conditions were randomly interleaved within a run.

Procedure:

Observers were instructed to align the simulated scotoma between two vertically aligned dots on the screen and then to click a mouse button to initiate a trial. Each trial began with a Nonius square presented 2° (Experiment 1) or 6° (Experiment 2) below fixation on separate runs. The lower visual field was chosen for PRL location as it is often selected as a PRL training location in low vision rehabilitation, primarily because it may prevent upcoming text from falling into the scotoma during reading. ^73–76

The Nonius square was twice the size of the unfiltered target letter to provide an indication of the size / spatial frequency of the upcoming target. Participants were required to fuse the Nonius squares presented within the Panum’s fusional areas and, once fusion was achieved, click a mouse button to trigger the presentation of the target letter. The target letter was randomly selected from the 26 letters of the alphabet and was presented in the centre of the Nonius square at a spatial frequency and contrast determined by the quickCSF algorithm described in the Stimuli section. The letter was presented within a Gaussian temporal envelope with standard deviation of 250 ms. This was followed by the response screen that contained all 26 letters of the alphabet arranged along the top of the screen. The observer’s task was to report the identity of the target by clicking on the corresponding letter in the response screen.

On monocular trials, the non-tested eye was occluded with an eye patch. On binocular trials, the target letter was the same identity, spatial frequency and contrast in both eyes. On corresponding trials, the letters were presented in the same position in the left eye and right eye. On non-corresponding trials, the letters were presented at a disparity corresponding to ¼ cycle (90° phase shift) of the peak spatial frequency of the letter target. The sign of the disparity was randomised across trials.

Eight CSFs were estimated within one session in a randomised order (four monocular CSFs and four binocular CSFs, two for stimuli in corresponding positions and two for stimuli in non-corresponding positions). Each CSF was measured with 50 trials. We derived monocular thresholds for each eye and binocular thresholds at each spatial frequency as well as two summary statistics from the CSF: (i) area under log CSF (AULCSF) which represents the overall contrast sensitivity of the patient, ⁷⁷ and (ii) CSF Acuity, the high spatial-frequency cutoff corresponding to the highest spatial frequency target than can be identified at full contrast.

Data Analysis

In order to quantify binocular summation, standard practice is to report the ratio of binocular and monocular detection thresholds^{11–19, 26, 28} under the assumption that the two eyes have the same detection threshold. However, when contrast sensitivity differs between the two eyes, as is the case for most clinical populations ^45–51, the two eyes have different detection thresholds. We therefore computed predicted binocular target detection probability based on the underlying observed monocular target detection probabilities. We then compared the predicted binocular target detection probability with the observed binocular target detection probability at each spatial frequency. From each monocular and binocular quickCSF, we extracted contrast sensitivity at 16 spatial frequencies, at evenly spaced log steps between 0.5 and 16 c/deg. This range was selected because of the physical constraints of the apparatus at the 50cm viewing distance and because of the low sensitivity to spatial frequencies above 16 c/deg in peripheral visual field. To examine binocular summation, we first calculated the contrast detection threshold for the binocular CSF as the reciprocal of contrast sensitivity at each spatial frequency. The quickCSF assumes a Weibull psychometric function:

$$P=1-\left(1-\gamma \right)\ast e^{-{\left(C/\alpha \right)}^{\beta }}$$ [Equation 1]

where C is stimulus contrast, α is threshold contrast, γ is guessing rate (γ =0.03 for our 26-AFC task) and β is the slope (β= 2;⁶⁶). For our 26AFC task, the binocular contrast detection threshold corresponds to the signal contrast at which the probability of detection (P_{Observed Binocular}) is 0.643. For each binocular contrast threshold, we calculated the probability of target detection by the left eye and by the right eye based on their monocular contrast sensitivity at the same spatial frequency. For each spatial frequency, the probability of monocular target detection (P) can be directly estimated at the binocular contrast threshold (C) given the monocular threshold (α), where γ and β are fixed. ^{78, 79}

The expected probability of binocular detection (P_{Expected Binocular}) based on the probabilistic summation of independent monocular signals is given by:

$$P_{\text{Expected Binocular}}=P_{\text{Left}\text{eye}}+P_{\text{Right}\text{eye}}-\left(P_{\text{Left}\text{eye}}*P_{\text{Right}\text{eye}}\right)$$ [Equation 2]

where P_{Left eye} and P_{Right eye} are the detection probabilities for the left and right eye. The ratio of P _{Observed Binocular} /P _{Expected binocular} provides an estimate of the binocular summation factor for each spatial frequency. A ratio of 1 indicates simple probability summation – i.e. the observed binocular detection threshold is equal to the threshold predicted by independent detection by the left eye and right eye. A ratio greater than 1 provides evidence for binocular contrast summation, and a value lower than 1 shows evidence of binocular inhibition.

Parametric analyses were used throughout the paper; analyses were run using lme package in R.2.5 (www.rdocumentation.org/packages/nlme/versions/3.1-137/topics/lme). Statistical comparisons were Bonferroni corrected for multiple comparisons.

Results

Contrast Sensitivity

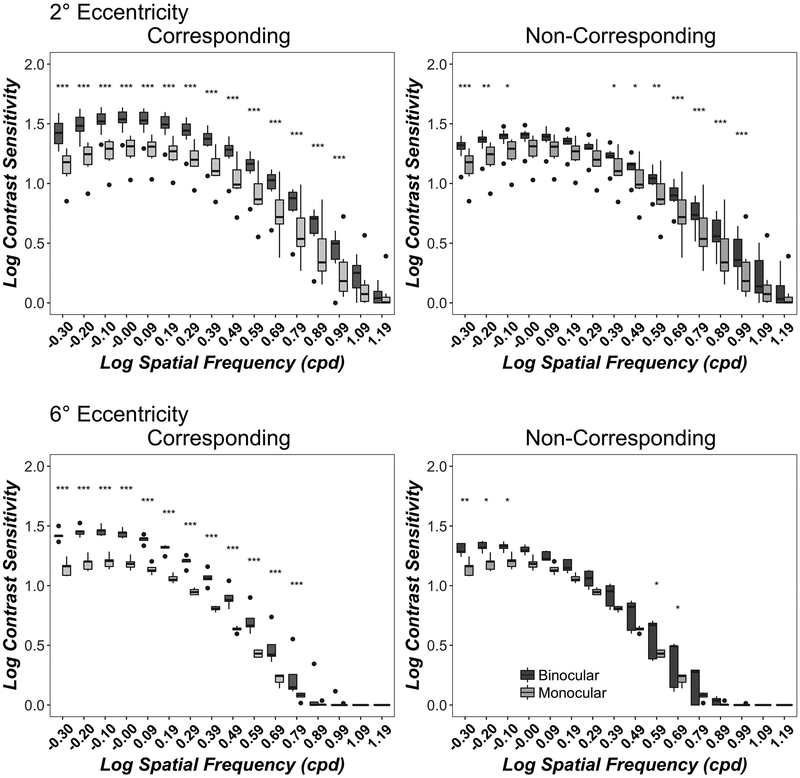
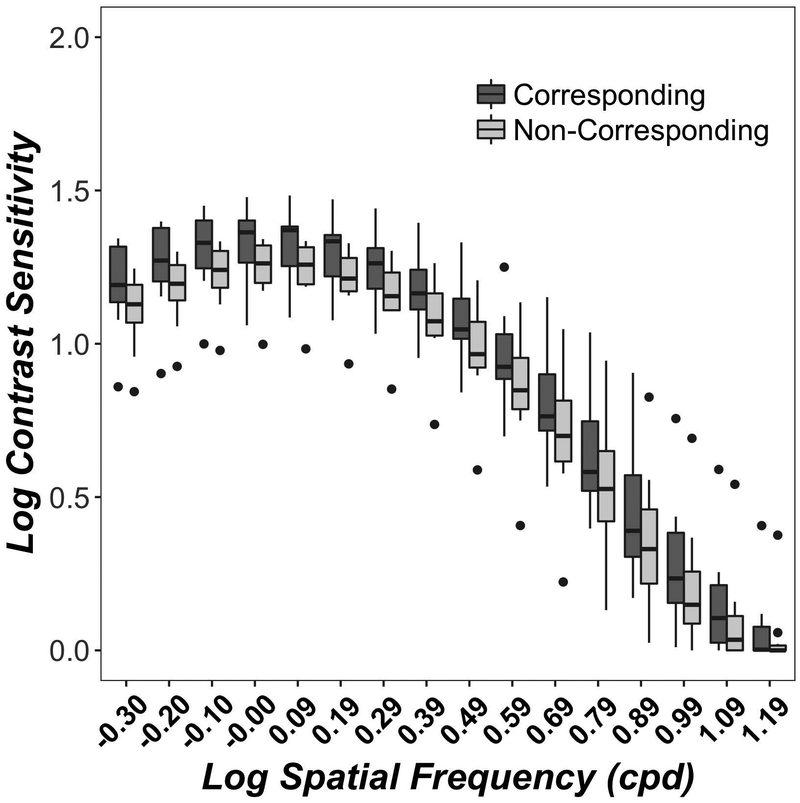
Figure 1 shows monocular (light grey) and binocular (dark grey) contrast sensitivity functions for corresponding (left column) and non-corresponding (right column) stimuli at 2° (top row) and 6° (bottom row) eccentricity. Contrast sensitivity was higher at 2° than 6° eccentricity in both corresponding (F_{(1, 124)}= 507, p<0.001) and non-corresponding (F_{(1, 124)}= 506, p<0.001) conditions, in good agreement with many previous studies. ⁸⁰ At both 2° and 6° eccentricities, binocular contrast sensitivity exceeded monocular contrast sensitivity for both corresponding (2° F_(1,279)= 573, p<0.001; 6° F_(1,124)= 410, p<0.001) and non-corresponding (2° F_(1,279)= 182, p<0.001; 6° F_(1,124)= 78, p<0.001) conditions. These findings confirm previous studies ^{16, 17} and extend them to a full spatial frequency range, to para-central visual field and to stimuli in depth.

Figure 1.

Monocular and Binocular Contrast Sensitivity Functions. Data show contrast sensitivity from the quickCSF algorithm at 16 spatial frequencies for corresponding (left column) and non-corresponding (right column) conditions at 2° (top row) and 6° (bottom row) eccentricities. Boxplots show medians and interquartile range (error bars) of ten observers (2° eccentricity) or 5 observers (6° eccentricity), with left eye and right eye data combined in the monocular data, dots show outliers. Monocular acuities are replotted for comparison in both columns. Significant binocular-monocular contrast sensitivity differences for each spatial Frequency are marked as asterisks, where *=p<0.05; **=p<0.01 and ***=p<0.001.

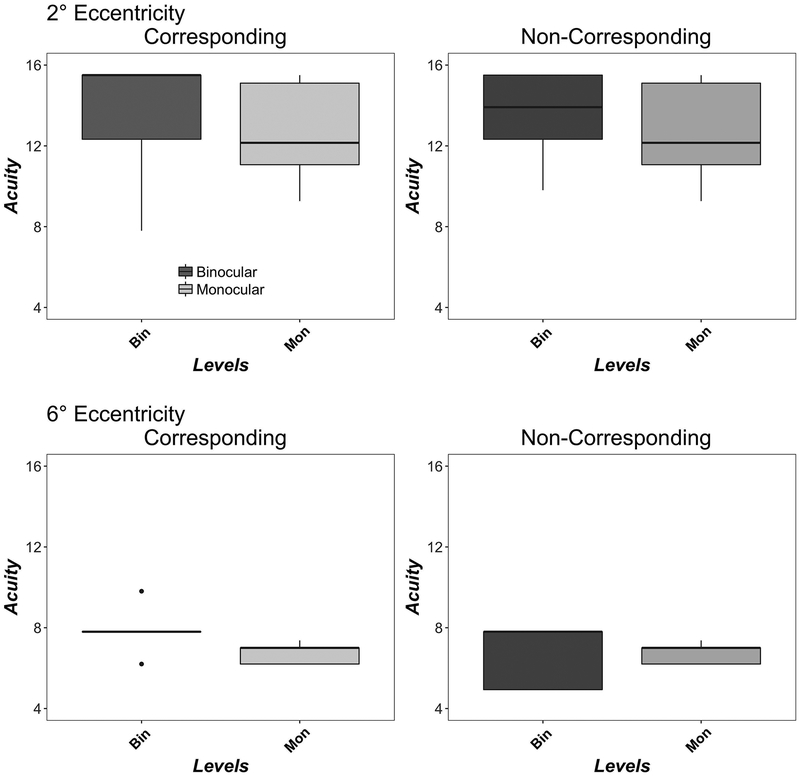
Figure 2 shows monocular (light grey) and binocular (dark grey) acuity for corresponding (left column) and non-corresponding (right column) stimuli at 2° (top row) and 6° (bottom row) eccentricity. Monocular acuities are replotted for comparison in both columns. Acuity was higher at 2° than 6° eccentricity in both corresponding (F_(1,13)=22, p<0.001), non-corresponding (F_(1,13)=43, p<0.001) and monocular conditions (F_(1,13)=28, p<0.001), in good agreement with previous studies. ⁸⁰ At both 2° and 6° eccentricities, binocular and monocular acuity did not significantly differ for either corresponding (2° F_(1,9)=3.82, p=0.08; 6° F_(1,4)=5.17, p=0.08) or non-corresponding (2° F_(1,9)=3.74, p=0.08; 6° F_(1,4)=0.02, p=0.88) conditions.

Figure 2.

Monocular and Binocular Acuity. Data show CSF Acuity (c/deg) for corresponding (left column) and non-corresponding (right column) Conditions at 2° (top row) and 6° (bottom row) eccentricities. Boxplots show medians and interquartile range (error bars) of ten observers (2°) or five observers (6°), with left eye and right eye data combined in the monocular data, dots show outliers. Monocular acuities are replotted for comparison in both columns.

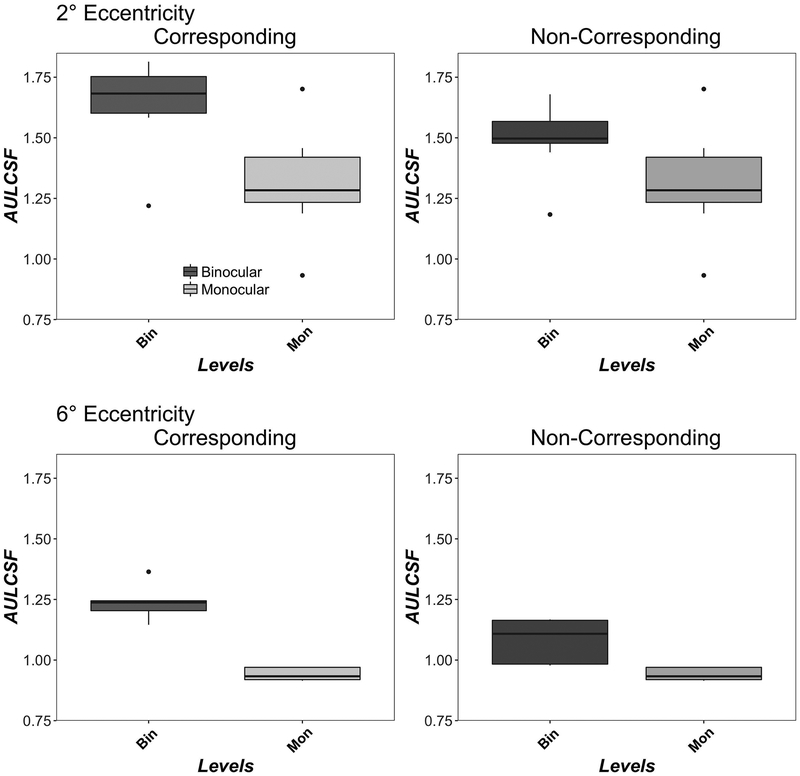
Figure 3 shows monocular (light grey) and binocular (dark grey) AULCSF for corresponding (left column) and non-corresponding (right column) stimuli at 2° (top row) and 6° (bottom row) eccentricity. Monocular AULCSF is replotted for comparison in both columns. AULCSF was higher at 2° than 6° eccentricity in both corresponding (F_(1,4)=25, p=0.007), non-corresponding (F_(1,4)=39, p=0.003) and monocular conditions (F_(1,4)=47, p=0.002), in good agreement with previous studies. ⁸⁰ At both 2° and 6° eccentricities, binocular contrast sensitivity exceeded monocular contrast sensitivity for both corresponding (2° F_(1,9)=77, p<0.001; 6° F_(1,4)=74, p<0.001) and non-corresponding (2° F_(1,9)=21, p=0.0012; 6° F_(1,4)=10, p=0.03) conditions.

Figure 3.

Monocular and Binocular AULCSF. Data show AULCSF for corresponding (left column) and non-corresponding (right column) conditions at 2° (top row) and 6° (bottom row) eccentricities. Boxplots show medians and interquartile range (error bars) of ten observers (2°) or five observers (6°), with left eye and right eye data in the monocular data, dots show outliers. Monocular AULCSF is replotted for comparison in both columns.

Binocular Summation

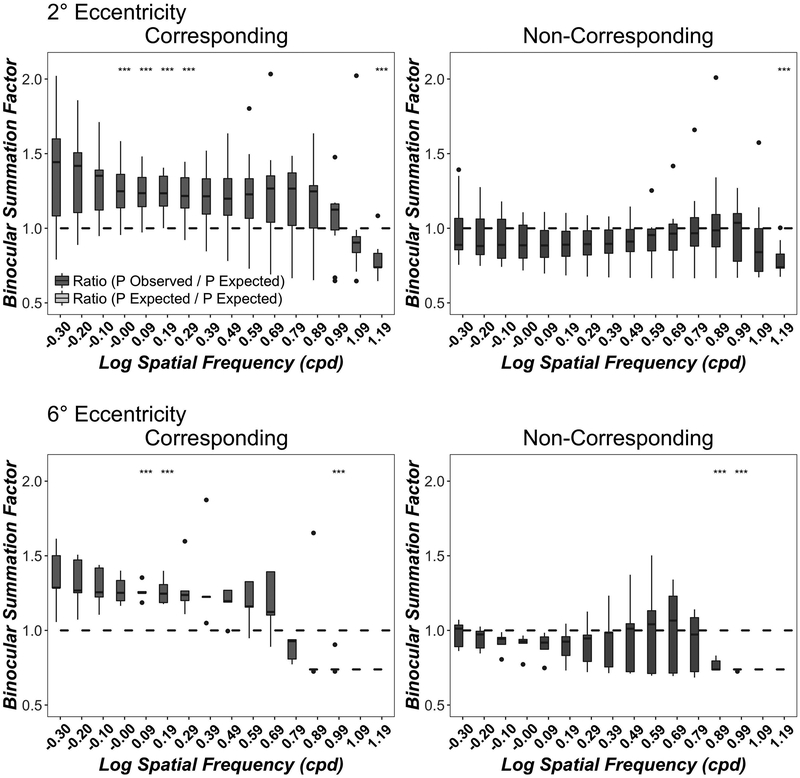
Figure 4 shows binocular summation ratios (P _{Observed Binocular} /P _{Expected binocular}) for corresponding (left column) and non-corresponding (right column) binocular stimuli at 2° (top row) and 6° (bottom row) eccentricity.

Figure 4.

Binocular Contrast Summation as a function of Spatial Frequency. Data show the ratio of observed binocular probability summation over expected binocular probability summation (P _{Observed Binocular} /P _{Expected binocular}) at 16 spatial frequencies for corresponding (left column) and non-corresponding (right column) conditions at 2° (top row) and 6° (bottom row) eccentricities. A value of 1 indicates simple probability summation between independent monocular sensors while a value greater than 1 or lower than 1 indicates a binocular integration mechanism or a binocular inhibition mechanism respectively. Boxplots show medians and interquartile range (error bars) of ten observers (2° eccentricity) or 5 observers (6° eccentricity). The dots are outlier points. Significant binocular contrast summation/inhibition vs probability summation t-tests for each spatial frequency are marked as asterisks.

In the corresponding conditions, binocular contrast summation exceed probability summation at both 2° eccentricity (F_{(1, 279)}= 75, p<0.001) and at 6° eccentricity (F_{(1, 124)}= 23, p<0.001). There was a significant interaction of spatial frequency and the type of ratio (observed versus predicted) at both 2° (F_{(15, 279)}= 2.06, p=0.01) and 6° (F_(15,124)= 2.67, p=0.0015) eccentricities, indicating that binocular summation is highly dependent on spatial frequency. Asterisks show the significance of pairwise t-tests and indicate which spatial frequencies showed, after correction for multiple comparisons, significant evidence of binocular summation (summation factor >1) or interocular inhibition (summation factor <1).

In the non-corresponding condition, binocular contrast thresholds were significantly below probability summation at both 2° eccentricity (F_(1,279)= 16, p<0.001) and at 6° eccentricity (F_(1,124)= 42, p<0.001). There was no significant interaction at 2° eccentricity (F_(15,279)= 1.42, p=0.13) but the interaction was significant at 6° eccentricity (F_(15,124)= 2.07, p=0.015) suggesting that at some spatial frequencies, contrast detection thresholds are consistent with binocular contrast inhibition. Asterisks show the significance of pairwise t-tests and indicate spatial frequencies that showed significant evidence of interocular inhibition (summation factor <1).

These results confirm and extend previous studies and provide evidence for binocular contrast summation. ^36–38 However, the present results demonstrate that such contrast summation is present only at low and intermediate spatial frequencies and only for stimuli in corresponding retinal locations.

Crosstalk control

When using liquid crystal shutter glasses to separate stereo half-images, interocular “cross-talk” can occur. It is therefore possible that the binocular contrast summation we observe for corresponding but not non-corresponding stimuli could arise from additive cross talk in successive displays. Simmons and Kingdom ⁸¹ report that at low stimulus contrasts (i.e., close to detection threshold, as in the present study) this cross-talk was undetectable. ^{19, 81} Furthermore, Figure 1 and Figure 4 show a strong spatial frequency dependence of correspondence, suggesting that the effect is not fully attributable to display cross-talk. Nevertheless, since TFT and LCD displays show non-linearities, ^{82, 83} to test if cross-talk contributed to our results, we compared the corresponding versus non-corresponding conditions at 2° eccentricity when the stimulus was viewed only monocularly, with the non-tested eye covered by an eye patch. In the corresponding condition, alternate fames (not presented to the test eye), contained an identical stimulus. In the non-corresponding conditions, alternate frames (not presented to the test eye), contained a ¼ cycle (90°) phase shifted stimulus. If crosstalk mediated our results, contrast sensitivity should be higher in the corresponding condition. Figure 5 shows monocular CSFs for 10 observers for corresponding and non-corresponding conditions. Contrast sensitivity was not significantly different between corresponding and non-corresponding conditions at any spatial frequency. We therefore conclude that cross-talk does not account for the differences in binocular and monocular contrast sensitivity in our main experiments.

Figure 5.

Contrast sensitivity functions for stimuli presented monocularly in binocular corresponding and non-corresponding conditions. Boxplots show medians and quartiles (error bars) of 10 observers. The dots are outlier points.

Discussion

We measured monocular and binocular contrast sensitivity functions at 2° and 6° eccentricity, with band-pass spatial frequency filtered letter stimuli that were presented in either corresponding or non-corresponding stereoscopic disparities. Consistent with many previous studies, under some conditions, binocular contrast sensitivity exceeded monocular contrast sensitivity by a factor that exceeded probability summation, and therefore provides evidence for binocular visual summation. Extending previous studies, we find that such super-probability summation occurs only at low spatial frequencies, low eccentricities and for corresponding retinal disparities. For non-corresponding stimuli, binocular contrast sensitivity does not exceed probability summation, and at high spatial frequencies there is evidence of interocular inhibition.

We found contrast summation in the central and para-central region only in the 0-disparity (corresponding) condition and at the lowest spatial frequencies, while contrast inhibition was found at high spatial for the non-corresponding condition. We found an average 1.4-fold increase in contrast sensitivity in a 26-AFC discrimination task in the corresponding condition with respect to the increase expected from simple probability summation alone. For low-intermediate spatial frequencies, contrast summation is slightly less at 6° than at 2° eccentricity. These results are in good agreement with earlier studies on binocular summation, where a 1.4 factor increase was observed in monocular detection contrast thresholds for sine-wave gratings compared to binocular contrast thresholds ^{12, 16}. Under standard explanations of these data, each monocular channel contains internal noise which determines signal detection threshold. However, since the standard error of n measurements of noisy processes will decrease in proportion to sqrt(n), with two channels an increase in sensitivity of $\sqrt{2}$=1.41 is expected. ^{11, 84}

In situations of non-zero-disparity (with or without diplopia), previous studies of foveal vision have reported that binocular summation fails. ^{18, 72, 85} Accordingly to Rose et al., ⁷² there is a range up to 3–4 degrees disparity for binocular summation to occur. As disparity increases, binocular summation decreases, such that at large disparities contrast summation was near 1.2, close to the value expected from probability summation alone. They also reported that disparity range limit was scaled with stimulus size to support binocular summation. In our experiment disparity was scaled, with stimulus size and spatial frequency in the range of 0.5° at 0.5 c/deg to 0.0625° at 16 c/deg; these values should be within the range suggested by Rose et al. ⁷²

Our results show that binocular visual acuity was higher than monocular visual acuity, however the effect was not significant. These results are consistent with previous studies of visual acuity which have reported relatively small (c. 10%) increases in binocular acuity ^86–88 and a lack of any benefit in visual acuity at the location of a PRL in AMD patients. ³⁵ In comparison, the AULCSF, an overall estimate of contrast sensitivity, was significantly higher under binocular viewing conditions, again consistent with previous studies. ⁸⁹ This result suggests that while acuity may not be improved at a binocular PRL ³⁵, contrast sensitivity may demonstrate a benefit from PRL training.

In most cases of pathological central vision loss, the scotoma size and location is asymmetrical. This complicates the selection of a PRL for best binocular function. Since contrast sensitivity decreases approximately linearly along each meridian with increases in eccentricity, ^20–33 a PRL closest to the fovea should support best visual function. Baldwin et al. ⁸⁹ proposed a witch’s hat model to describe this function:

$$S=lo{g}_{10}\left(\frac{{10}^{-m_{1}E}}{{10}^{\left(m_1-m_2\right)v}+{10}^{\left(m_1-m_2\right)E}}\right)+K$$ [Equation 3]

Where S is contrast sensitivity in dB, E is eccentricity in degrees, m₁ and m₂ control the slope of the first and second limbs of the function (with the constraint that m₁ ≤ m₂), v determines the location of the knee point and K controls the vertical position of the function. Baldwin et al. ⁸⁹ provide estimates of m₁, m₂, v and K for normally-sighted observers.

However, since such summation can provide a contrast sensitivity benefit of at least a factor of 1.4, depending on spatial frequency and disparity, best overall visual function may depend on the relative sizes of the scotomas in each eye rather than on best visual acuity (Bernard and Chung, 2018). Our results suggest that Equation 3 can be used to estimate the highest monocular contrast sensitivity in each eye, based on the size of the scotoma in that eye. In order to select the best overall function, it is relatively straightforward to compare monocular sensitivity at the lower eccentricity of the better eye with binocular sensitivity at the greater eccentricity of the worse eye. If sensitivity in the eye with the smaller scotoma exceeds sensitivity of the eye with the larger scotoma by more than a factor of 1.4, then this monocular location should be selected. Otherwise the more eccentric binocular location should be selected.

Limitations

In addition to reporting the ratios of acuity and AULCSF to estimate binocular summation, we implemented a simple, classic model of probability summation that is based on high threshold theory for detection of signal in independent channels. While this approach is limited for supra-threshold binocular vision ⁹⁰, its assumptions are reasonable at contrast detection threshold. ⁹¹ We also assumed that the underlying psychometric function was a Weibull function with a fixed slope. ^{66, 67} The slope parameter affects the predicted monocular detection probability, and we do not have sufficient data to constrain the slope at all spatial frequencies in the present study. However, predicted binocular summation at each spatial frequency was close to the values obtained for summation of the AULCSF. In more contemporary models of binocular function, summation is linear and determined by early non-linearities, while noise is late and additive. We therefore implemented the model proposed by ^{39, 40} and the results, shown in the Appendix, are in good agreement with the results reported with classic methods.

The present experiments were conducted in young healthy observers and we simulated central vision loss with a gaze-contingent scotoma. In instances of pathological central vision loss, there is evidence of longitudinal plasticity, reorganisation and attentional changes that might influence sensitivity and binocular summation. ⁹² Furthermore, the magnitude of binocular summation decreases with age.⁴⁴ Additionally, as we tested only in the inferior visual field, which is frequently the target for PRL training, it is possible that binocular summation may vary with visual field location. We also used an eye patch to occlude the untested eye, as is common practice, for monocular threshold measurement. However, given that binocular summation is affected by mean luminance ⁸⁴, different results may be obtained with a mean luminance target in the untested eye. Before our findings can be generalised to low vision rehabilitation for AMD patients, therefore, further testing involving visually impaired populations is necessary to support conclusions about binocular summation and best eccentric binocular location.

Appendix

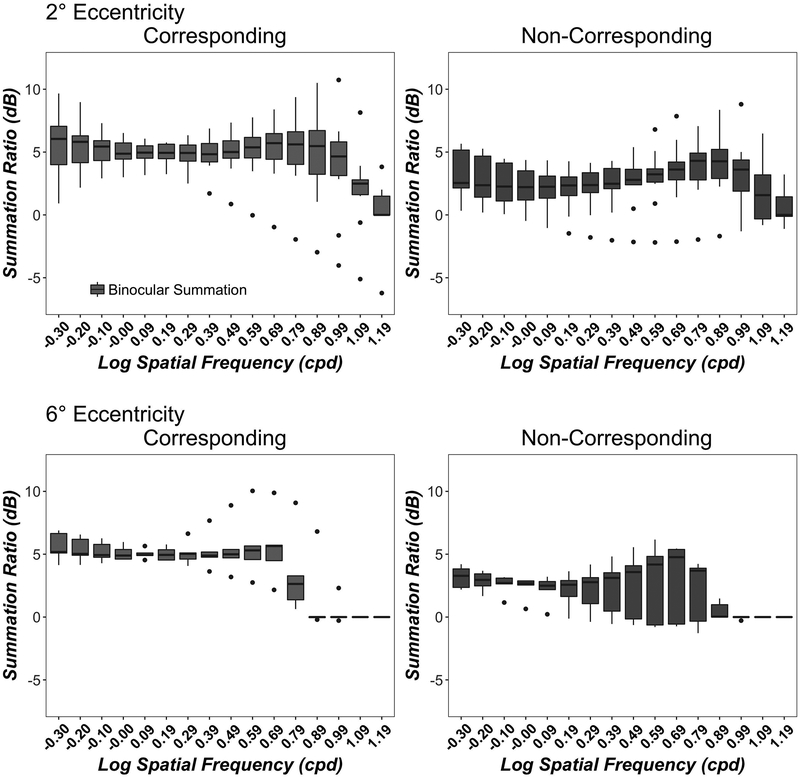
For comparison with alternative models of binocular contrast summation we calculated the Summation Ratio (SR) using Meese and Summer’s (2009) method:

$$\text{SR}=20{\text{log}}_{10}\left({\text{threshold}}_{\text{monocular}}/{\text{threshold}}_{\text{binocular}}\right)$$

Figure A1 expresses the summation ratio in decibels (dB). Since a larger ratio corresponds to a larger summation level, Figure A1 confirms the trend observed using the Binocular Summation Factor in Figure 4.

Figure A1.

Contrast Summation Ratio as a function of Spatial Frequency. Data show the summation ratio (in dB) between the monocular and the binocular thresholds at 16 spatial frequencies for corresponding (left column) and non-corresponding (right column) conditions at 2° (top row) and 6° (bottom row) eccentricities. Boxplots show medians and interquartile range (error bars) of ten observers (2° eccentricity) or 5 observers (6° eccentricity). The dots are outlier points.