A proposal to explain how the circatidal rhythm of the Arabidopsis thaliana root elongation rate could be mediated by the lunisolar gravitational force: a quantum physical approach

Abstract

Background and Aims

Roots of Arabidopsis thaliana exhibit a 24.8 h oscillation of elongation rate when grown under free-running conditions. This growth rhythm is synchronized with the time course of the local lunisolar tidal acceleration. The present study aims at a physiological/physical model to describe the interaction of weak gravitational fields with cellular water dynamics that mediate rhythmic root growth profiles.

Methods

Fundamental physical laws are applied to model the water dynamics within single plant cells in an attempt to mimic the 24.8 h oscillations in root elongation growth. In particular, a quantum gravitational description of the time course in root elongation is presented, central to which is the formation of coherent assemblies of mass due to the lunisolar gravitational field. Mathematical equations that characterize lunisolar gravity-induced coherent assemblies of water molecules are derived and related to the mass of cellular water within roots of A. thaliana.

Key Results

The derived physical model of gravitationally modulated water assemblies is capable of accounting for the experimentally observed arabidopsis root growth kinetics under free-running conditions. The close analogy between the derived time-dependent lunisolar effect upon coherent molecular states of water within single cells and the coherent assemblies of electrons that characterize the quantum Hall effect is emphasized.

Conclusions

The dynamics of the lunisolar-induced variation in coherent water assemblies provide a possible mechanism to describe the observed 24.8 h oscillation of root growth rate of A. thaliana. Therefore, this mechanism could function as an independent timekeeper to control cell elongation.

INTRODUCTION

Due to the rotation of the Earth around its axis, as well as the relative orbital motions of the Earth and its Moon around the Sun, the gravitational field of the Earth is continually modulated by a lunisolar gravitational force (Konopliv et al., 1998, 2001). On Earth, this modulation is readily evident and measurable in relation not only to the diurnal rise and fall of marine and oceanic tides but also to similar small elastic deformations of the Earth’s crust, the latter being expressed as incremental or decremental variations, ±δg, of Earthly 1G gravity. These are measurable by gravimetry (Xu et al., 2004; Crossley et al., 2005), and are expressed in units of micro-Gals, where 1G = 9.80 × 10⁸ μGals. In comparison with the solar day with a periodicity of 24.0 h, the lunar day, which is determined by the recurring marine and gravimetric tides, exhibits a periodicity of approx. 24.8 h.

Substantial evidence has been accumulated to show that the lunisolar gravitational force affects numerous physiological and behavioural processes in both plants and animals (Miles et al., 1977; Barlow, 1998, 2015; Denny and Paine, 1998; Zürcher et al., 1998; Endres and Schad, 2002; Helmuth et al., 2002; Engelmann, 2007; Barlow et al., 2008, 2010, 2013; Halberg et al., 2010; Connor and Gracey, 2011; Halberg et al., 2011; Barlow and Fisahn, 2012; Fisahn et al., 2012, 2015a, b; Moraes et al., 2012; Gallep et al., 2013, 2014, 2017; Zajączkowska and Barlow, 2017). When grown in free-running conditions of continuous low-level light, roots of the plant Arabidopsis thaliana exhibit oscillations of growth rate with the 24.8 h periodicity of the lunar day (Yazdanbakhsh and Fisahn, 2011; Yazdanbakhsh et al., 2011; Barlow and Fisahn, 2012; Fisahn et al., 2012; Barlow et al., 2013; Barlow, 2015). These oscillations are in phase with the lunisolar-driven gravimetric tidal variation. Intuitively one could consider that ‘tides’ might also occur within single plant cells induced by the lunisolar gravitational force. However, the gravitational effect of the lunar mass, as expressed by the variation δg, on water molecules within single cells is too weak to account sufficiently for the detected modulations in root growth rate (Dorda, 2010). Therefore, we investigated the applicability of alternative models related to lunisolar gravity that would be suited to account for the 24.8 h periodicity in root growth rate. One such approach is that adopted by Dorda (2010), who postulated an effect of lunar gravitation on organic structures. The concept underlying Dorda’s model is that the lunisolar gravitational field modulates the coherent state of water molecules within individual cells, and that these coherent assemblages respond to the diurnal variations in the lunisolar gravitational force in a defined manner. Whenever a change in lunisolar gravity is perceived, a certain mass of water molecules is either released from or entered into the coherent assemblage. It follows that, because the lunisolar gravity is continually varying, due to the orbital motion of the Earth and Moon, the number of water molecules associated with the coherent state varies similarly within cells.

Coherent units of particle assemblies are generally described by quantum electrodynamic equations (Von Klintzing et al., 1980; Del Giudice and Preparata, 1994; Arani et al., 1995; Dorda, 2004, 2010; Del Giudice et al., 2009). The units are characterized not only by their size and the total number of particles in the unit, but also by their unique dynamic behaviour, as enforced by surrounding electromagnetic or gravitational fields. In mathematical terms, the coherent state, in the gravity-induced case, is characterized by the inter-relationship of the de Broglie wave of the molecular unit and a derived orbital time unit of lunar movement (Dorda, 2004, 2010; Fisahn et al., 2012). In biological materials, this coherent molecular unit can be associated with intracellular water molecules (Dorda, 2004, 2010; Barlow and Fisahn, 2012). In the particular case of the arabidopsis root, a lunisolar tidal force-induced coherent unit of water molecules is sufficiently large to be capable of causing the observed 24.8 h rhythmicity of its elongation rate (Dorda, 2004, 2010; Barlow and Fisahn, 2012).

By analogy with the quantum Hall effect (QHE), which is related to the macroscopic coherent state of electrons (Von Klitzing et al., 1980; Prange and Girvin, 1987), we may assume that a feature of the lunar gravity effect (LGE) upon root growth rate is that there is a continually renewing collective mass state in the form of a coherent mass of water particles (Del Giudice and Preparata, 1994; Arani et al., 1995; Barlow and Fisahn, 2012). We propose, therefore, that one consequence of the inter-relationship between the quantized lunar gravity field and the coherent state is the conversion of lunisolar gravitational dynamics into a temporal change of the number of coherent water molecules within cells (Barlow and Fisahn, 2012). Thus, maintenance of the coherent mass state requires increases or decreases in the number of water molecules within the cells whenever the lunar gravitational quantum number is modulated due to the rotation of the Earth around its axis (Dorda, 2004, 2010). The quantized lunar gravitational field provides, therefore, a timer (Zeitgeber) with a periodicity of 24.8 h, matching exactly the timing of the described oscillations of root elongation, due to the temporal variations in the coherent mass state of cellular water.

Water and the associated turgor pressure of the cell is the driving force for cellular growth. The generally accepted equation for cellular growth, due to Lockhart (1965), is, in its basic form, specified by r = φ(P – Y), where r = growth rate, φ = cell wall extensibility, P = cellular turgor pressure and Y = yield stress threshold. Evidently, variation of the mass of the coherent water state within the cytoplasm, as modulated by the LGE, could affect r through an effect on P. If, however, the coherent water state also affected the transcription of genes (and thereby protein and enzyme amounts) through an accompanying alteration of the nucleoplasmic matrix, then the parameter Y, which directly relates to enzyme-driven cell wall turnover and biomechanical properties, could also be modulated by the LGE.

In the present study, we apply the quantum gravitational approach of lunisolar gravity-induced coherent particle assemblies (Del Giudice et al., 2009; Dorda, 2010, 2011) in an attempt to describe the detected 24.8 h oscillation in arabidopsis root growth kinetics. Mathematical equations are derived to address two basic questions. (1) Can the mathematical formalism of coherent water assembly formation due to the cyclical lunisolar tidal effect adequately account for the 24.8 h oscillation in arabidopsis root elongation rates? (2) Does the mechanism of lunisolar gravity-induced coherent water molecule assembly show any relationship to similar effects already known and described in physics: for example, the coherent units of electrons that account for the QHE?

MATHEMATICAL MODELLING AND RESULTS

To demonstrate that the 24.8 h oscillation in arabidopsis root elongation rate could emerge from the interaction of the diurnally modulated lunar gravitational force, with particle assemblies located within the roots of A. thaliana, we will consider the movement of a test mass M_x,E located on the surface of the Earth, as it might be affected by the temporarily variable lunar gravitational field (Fig. 1). As a first step, we need to derive an expression that describes the gravitational force between two masses in a quantized manner (Dorda, 2010). The plausibility of this procedure can then be demonstrated by the correspondence, in the limiting case, between the quantized and the classical formulation of gravitational attraction. An appropriate description of quantized gravitational mass interaction can be derived from the classical gravitational law of Newton (Dorda, 2010).

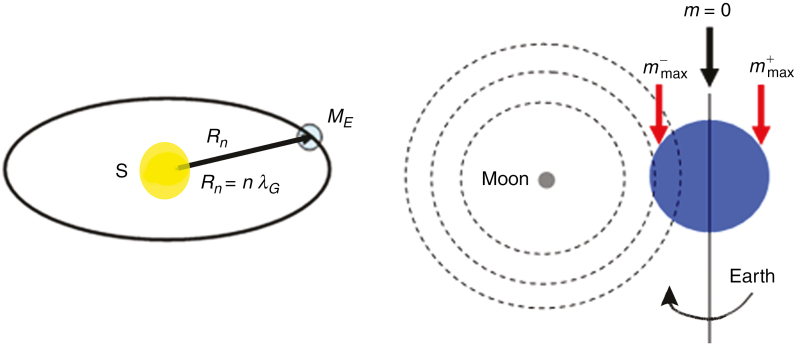
Fig. 1.

Schematic representation of the quantum numbers n and m used in the quantized expression of the gravitational force [eqn (4)].

In classical terms, the gravitational force between the Earth and the Moon can be described by

$$F_{M,E}=M_{E}G\frac{M_M}{R_{M,E}^2}$$ (1)

where M_E describes the mass of the Earth, M_M is the mass of the Moon, G is the gravitational constant given by G = c²L/M with L and M the Planck length and mass, c is the speed of light universal constant, and finally R_M,E denotes the mean distance between the Earth and the Moon, respectively (Vogel, 1993).

In general, a quantized formulation of Newton’s law follows from the quantized formulation of the distance R_n,y, given by

$$R_{n,y}=n{\lambda }_{G,y}$$ (2)

where n is the gravity-related quantum number, and where λ_G,y is given by

$${\lambda }_{G,y}=\frac{L}{M}{M}_y$$ (3)

this being the reference distance related to the gravitational mass M_y, the so-called Einstein–Schwarzschild gravitational radius (Kittel et al., 1965; Dorda 2010). It should be pointed out that this method of quantization of gravity was successfully applied to describe the time-related limits to visual and auditory discrimination in the context of a Sun-related gravity effect (Dorda, 2010).

In the case considered here, two different quantum numbers, n and m, are needed to describe the influence of the lunar gravity on organic structures located on the surface of the Earth. These quantum numbers are schematically shown in Fig. 1 where, in the left-hand panel, R_n ≡ R_n,y = R_E,S, i.e. R_E,S represents the distance between the Earth (together with the Moon) and the Sun, and λ_G ≡ λ_G,y is the reference length related to the mass of the Sun M_S Thus, the quantum number n is, in our case, the solar gravity-related quantum number, the average of which is given by n = 1.01 × 10⁸. The right-hand panel of Fig. 1 describes the quantum number m. During the daily rotation of the Earth around its axis, a test mass M_x,E, located on the surface of the Earth and indicated by the red arrow, will cross a defined interval of the quantized lunar gravity field denoted by the lunar field-associated quantum number m.

According to Dorda (2010) (see also the Supplementary Data Appendix I for a detailed derivation of the time-dependent gravitational law), eqn (1) can be transformed into an expression of the force exerted by the quantized lunar gravity field on a test mass M_x,E located on the surface of the Earth (Fig. 1, right-hand panel). It is given by

$$F_{(n,m),\lambda }=\frac{M_{x,E}{\upsilon }_{n,E}}{ab{t}_{M,(n,m)}}\cong \frac{h}{n_M{\lambda }_{(n,m)}{t}_{M,(n,m)}}$$ (4)

Here, M_x,E is the considered test mass; m and n are quantum numbers that characterize the quantized gravitational fields of the Moon and the Sun, respectively (see Fig. 1); a is the ratio of the Earth–Sun orbital time 2πt_E to the Moon–Earth-related orbital time, namely the lunar-day cycle 2πt_M; b is the ratio of the mean Sun–Earth gravitational force to the mean Moon–Earth gravitational force; n_M is a natural number, i.e. a quantum number, given approximately by n_M ≈ (ab); υ_n,E is the mean orbital velocity of the Earth around the Sun (υ_n,E = 2.977 × 10⁴ m s^–1); finally, t_M,(n,m) is the variable time.

Equation (4) describes the gravitational force due to the lunar gravitational field which acts on a test mass M_x,E on the surface of the Earth. In comparison with eqn (1), the lunar gravitational field in eqn. (4) is expressed in a quantized and temporally variable form (Dorda, 2010). The time dependence of the lunar gravitational field is a consequence of the rotation of the Earth around its axis that actually modulates the distance of the test mass located on the surface of the Earth to the centre of lunar gravitation within 24.8 h. Furthermore, eqn. (4) indicates that the mass M_x,E is in a wavelike state, with λ_(n,m), the de Broglie wave of the mass M_x,E, given by

$${\lambda }_{(n,m)}=\frac{h}{M_{x,E}{\upsilon }_{n,E}}$$ (5)

where h is the Planck constant.

As will be shown in the following discussion, the fundamental prerequisite of the LGE model is the assumption that the gravitational force F_(n,m),λ is constant within a coherent assembly of particles, i.e.

$$F_{(n,m),\lambda }=F_{n,\lambda }=const$$ (6)

Therefore, instead of eqn. (4) we can write

$$\left\{n_{M}const\right\}{\lambda }_{(n,m)}{t}_{M,(n,m)}=h$$ (7)

In other words, eqn. (7) shows only two variables, the wavelength λ_(n,m) and the (reversible) variable time t_M,(n,m), as set out above.

The question arises of whether the wave-like state of M_x,E is allowed for every mass size, or whether limiting conditions restrict these sizes. In Dorda (2010), it was demonstrated that the formation of a coherent state requires a particle assembly of n_M (n ± m) identical particles. [The Supplementary Appendices I and II contain a detailed derivation of the size requirements immanent to a coherent mass unit, according to Dorda (2010)]. As will be shown in the following section, the test mass M_x,E represents the coherent state only under the following limiting condition:

$$M_{x,E}=n_M(n\pm m)M_x$$ (8)

It follows that the lunar gravity-induced coherent unit M_x,E consists of n_M (n ± m) similar particles of mass M_x. Identical particles, whose number can be adjusted by the influence of the circatidal modulation in the gravitational field strength, cannot be charged particles. This particular standpoint already shows analogy between the LGE and the QHE where, in the latter, the charge independence of the electron gas system is also axiomatic. Thus, we suggest that the mass M_x can be represented by M_H2O, the mass of one water molecule.

Equation (7) provides a quantized rhythm generator, or a clock, inherent to mass particles and, hence, it reflects the periodicity of the lunar day, 24.8 h. As t_M,(n,m) in eqn (7) varies during the 24.8 h rotation of the Earth around its axis, constancy of eqn (7) requires an appropriate adjustment in the wavelength λ_(n,m). This modulation in the deBroglie wavelength λ_(n,m) implies an increase or decrease in the test mass M_x,E [eqns (3) and (5)]. The quantum number n refers to the combined orbital movement of the Earth and the Moon around the Sun; it can be approximately considered constant during a lunar day. The quantum number m refers to the rotation of the Earth around its axis, thus describing the quantized circatidal changes in lunar gravity and the associated gain and loss in the mass of the coherent state. Taken together, the number of mass particles forming the coherent unit changes with the quantum number m. Hence, the coherent unit shows a periodicity of 24.8 h.

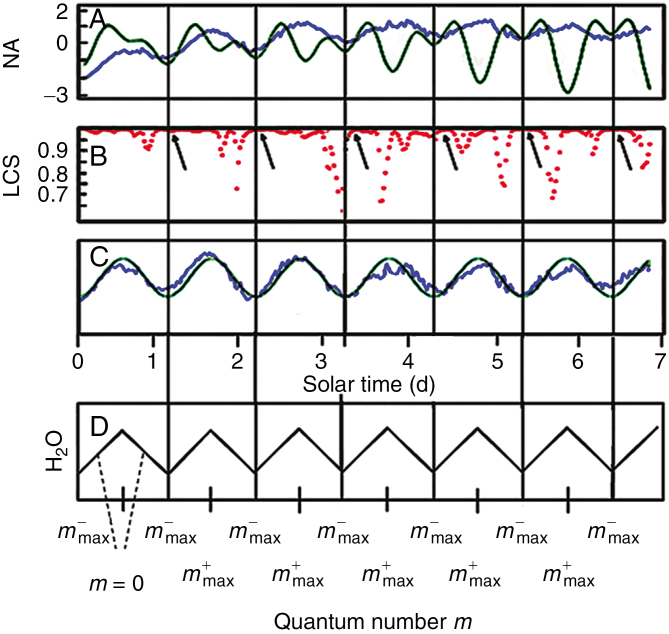
Figure 2 summarizes basic experimental observations (Barlow and Fisahn, 2012; Fisahn et al., 2012; Barlow et al., 2013) and illustrates the agreement of these findings with the predictions of the above model. The periodicity of the oscillations in root elongation rates of A. thaliana under free-running conditions has previously been described to be 24.8 h (Fig. 2A, C; blue line; Barlow and Fisahn, 2012; Fisahn et al., 2012; Barlow et al., 2013). This oscillation in root elongation rate was compared with the lunisolar tidal profile (Fig. 2A) and with a sine wave of 24.8 h periodicity (Fig. 2C). Convincing support for the congruence in phase of the tidal force profile and the growth rate profile was derived from a local correlation tracking analysis (Fig. 2B; Fisahn et al., 2012; Barlow et al., 2013). As demonstrated by the above equations, a coherent state of particles is generated by the interaction of the quantized lunar gravitational field with a defined amount of water molecules inside the roots of A. thaliana. This coherent state of water molecules expands and contracts in size, as depicted in Fig. 2D. Maximal and minimal expansion in the state of coherence exactly matches the peaks and troughs in the growth rate profile obtained from roots of A. thalliana.

Fig. 2.

Comparison between root elongation rates after transfer from long days of 16 h:8 h to continuous illumination, as well as the lunisolar tidal profiles and a 24.8 h sine wave. Roots of Arabidopsis thaliana seedlings were entrained using photoperiods of long days of 16 h:8 h, with n = 23 seedlings. On day 15, the light protocol was changed so that the seedlings were exposed to continuous illumination. (A) The blue trace denotes the averaged root elongation rate (µm h^–1) of seedlings (n = 23) in continuous illumination. The green trace indicates the lunisolar gravity profile (in µGal). Vertical lines specify the coincident positions of the troughs in both the root growth rate and the lunisolar gravity profile. (B) Local correlation score of the data profiles in (A). Arrows indicate sites of high local cross-correlation scores between the respective troughs in root growth rate kinetics and lunisolar tidal profiles. (C) Alignment of the average root growth rate with a 24.8 h sine wave (green trace). (D) Modulation in the particle number of the coherent water molecule unit as a function of the lunar gravitation-associated quantum number. m⁺ indicates an increase in the quantum number and m^– a decrease in the quantum numbers. During the daily rotation of the Earth around its axis, the test mass crosses the quantum numbers m up to a certain m⁺_max before it cycles back to m^–_max.

Lunar gravity effect (LGE) as an analogue of the quantum Hall effect (QHE)

The quantization of electron density in the QHE (Von Klitzing et al., 1980) is analogous to the quantization of the coherent state of water molecules, which we described in the previous section.

The fundamental equation describing QHE, derived by von Klitzing et al. (1980), can be reformulated to yield

$$i_{QHE}\frac{e{B}_x}{N_x}=h$$ (9)

where e is the electron charge, B_x (T) is the magnetic field perpendicular to the current within the semiconductor, and N_x (m^–2) is the electron density. Describing the coherent state of electrons in the QHE is the quantum number i_QHE (see also Dorda, 2010). It should be pointed out that the coherence state consists of a very high number of electrons with equal charges, forming a two-dimensional electron gas system, and that this resembles the high number of molecules which forms the coherent state of water in the LGE.

To enhance the possibility of describing the LGE on the basis of the QHE, four analogies (1–4, below) should be considered and discussed in detail:

• (1) At first glance, it is evident that eqn (7), as well as eqn (9), are related through the Planck constant h. Thus, in a manner similar to what is implicit in the Bohr equation, it is plausible to describe both effects – QHE and LGE – in a quantum manner. The quantization associated with the QHE appears in the form of the quantum number i_QHE, whereas in the LGE effect quantization is reflected by the quantum numbers n and m.

• (2) Analogy between QHE and LGE is discerned in the appearance of plateaus, schematically represented in Fig. 3, left panel. In the QHE, the plateaus result from coherent-state electrons. The density of the plateaus, reflecting the two-dimensional space, is in quantized correlation with the magnetic field, which is also related to the two-dimensional space (Dorda, 2010). Thus, these QHE plateaus are the result of the quantized relationship between the respective two-dimensional areas.

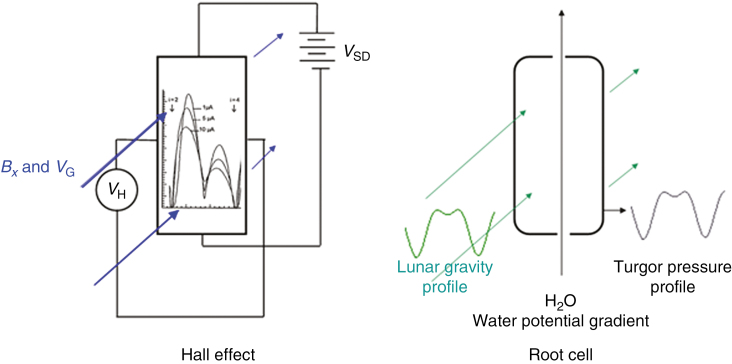
Fig. 3.

Schematic representation of the structural analogy between the QHE and LGE. Left. QHE: V_SD is the source-drain voltage, V_H the Hall voltage, B_x the magnetic field and V_G the gate voltage. Together, they induce the electron density N_x. The graph inset within the scheme of the QHE indicates the measured dependence of the specific resistivity of the sample (a Si-MOS transistor) on the gate voltage with the source-drain current as curve array parameter and i the quantum number of the QHE; see also Dorda (2010). Right. LGE: water molecules within a living root cell can form coherent units that are modulated in size by lunisolar gravity. An increase in the number of water molecules comprising the coherent state will give rise to increasing turgor pressure and thus could promote cell expansion.

• In comparison with these QHE plateaus, given by i_QHE– 1 < i_QHE < i_QHE+ 1, we have similar LGE plateaus, which, according to eqns (4) or (7), are given by n – m_max< n < n + m_max (Fig. 2D, and see also the schematic representation in Fig. 3, right panel). This means that the LGE plateaus result from the requirement to keep the gravitational force F_(n,m)λ constant [see eqns (6) and (7)]. In other words, the coherent state of the water molecules is a result of the endeavour to compensate the changes of the Moon-induced gravitational field caused by the rotation of the Earth around its axis, the so-called circatidal effect. It is evident that the variable stability between n – m and n + m provides the background for the postulated timekeeping rhythm within living organic structures. It should be pointed out that an absence of this lunar gravity-dependent timekeeping mechanism might cause some problems for future manned cosmic voyages, for example to Mars, due to alteration of time perception.

• To maintain the state of coherence in the QHE case, reservoirs of free electrons are required with electron densities above and below the electron density of the coherent state N_x. In the LGE case, a similar reservoir of free water molecules is needed. Two reservoirs, between which water might be exchanged, could be located within the protoplast (cytoplasm and nucleoplasm) and within the walls and apoplasm of the cells of interest, and should be independent of the coherent state; see Fig. 3. The independence of reservoir water suggests the possibility of the participation of bound and free water states.

• (3) The third analogy relates to the formation of coherent states of either electrons or water molecules, respectively. In the case of electrons, the coherence is observable in the independence of the QHE in relation to the size of the sample used, i.e. the coherent state of the electrons is independent of the total number of electrons. This noteworthy effect can be interpreted as a consequence of the coherent particles having, all together, the charge-less mass m_e of one single electron, thus realizing a two-dimensional electron gas system. In a similar manner, in LGE, we can interpret the state of the n + m or n – m water molecules, which create one single coherent water unit, as having quasi-independence of their total number. If taking into consideration eqns (4)–(7), this particular situation becomes recognizable by means of the following equation:

  $${\lambda }_{(n,m)}{t}_{(n,m)}={\lambda }_1\sqrt{n_G}{\tau }_G$$ (10)

• which represents the basic characteristic of the coherence state of the LGE (Dorda, 2010), where λ₁ is given by

  $${\lambda }_1=\frac{h}{M_x{\upsilon }_{n,E}}$$ (11)

• In this situation, and in agreement with eqn (8), M_x shows the mass of one water molecule, and in eqn (10), $\sqrt{n}{\tau }_G$ describes the quantum leap of the quantized circatidal dynamics (Dorda, 2010). The relationships implicit in eqn (10) demonstrate that the coherent state of LGE can, by analogy with the QHE, be described by the effect of only one single water molecule.

• (4) A very important argument for the close similarity between the LGE and the QHE is revealed in their basic background. As has been described, the main requirement for the realization of a coherent state consisting of water molecules is the constancy of the gravitational force induced by the moon within organic cells. This particular situation is described by eqn (7), where a proportional relationship is shown between only two variables, the wavelength λ and the reversible time t: generally speaking, that is between space and time.

• This specific circumstance of the unique relationship between the categories space and time, excluding any variability of the category mass, is also given in the case of the QHE. To demonstrate this assumption, we have to use for the description of the QHE the following equation:

  $$e*=\sqrt{n_{G,E}}{m}_e$$

• where n_G,E is the gravitational quantum number related to the surface of the Earth, the average of which is given by n_G,E = 1.4 × 10⁹. This important equation is obtained by applying the third law of Kepler to eqn (9), and it can be verified experimentally by analysing the critical current of the QHE, as demonstrated by Dorda (2010). Evidently, this equation describes a relationship between the so-called electron charge, an electromagnetic property, and mass, a gravitational attribute, modified by the proportionality factor $\sqrt{n_{G,E}}$ . Equation (12), as applied to the QHE, concerns the interpretation of the magnetic field B, which occurs in eqn (9). As shown in Dorda (2010), B should now be understood as frequency, i.e. it accords with the category ‘time’. Thus, we can draw the conclusion that the coherence of concepts displayed by both the LGE and the QHE represents a specific and basic relationship between space and time. Transformation of eqn (9) by eqn (12) can be further justified: the fundamental eqn (9) of the QHE does not consider the charge of the electron, a circumstance in accordance with the proposition that the coherent state deals solely with neutral particles.

DISCUSSION

A quantum gravitational model was derived that establishes a physical inter-relationship between the observed 24.8 h oscillations in the growth rate profiles of Arabidopsis thaliana roots and the circatidal variation of the lunisolar gravity field. The explicit dependence of the gravitational force on time [eqn (4)] suggests that the circatidal rhythms in the lunar gravitational field itself can serve as a fundamental timer (Zeitgeber) in biological organisms (Dorda, 2010). Central to this quantum gravitational approach was the formation of gravity-dependent coherent states of water molecules. According to eqns (4) and (5), these coherent states are induced by the inter-relationship of the de Broglie wave of the molecular unit comprised of water molecules and the given rate of rotation (orbital, i.e. reversible, time), which is related to the rotation of the Earth around its axis, in the presence of the also rotating Moon. A significant consequence of this inter-relationship is the conversion of lunisolar gravitational dynamics into a temporal modulation in the amount of water molecules that generate and aggregate as a coherent assembly. It is important to emphasize that this modulation takes place in accordance with a temporally ordered series of quantum leaps which relates to the respective orbiting bodies (Fig. 1). Thus, a coherent test mass located on the surface of the Earth will, due the rotation of the Earth around its axis, periodically cross equipotential lines of the quantized lunar gravitational field with a periodicity of 24.8 h. It is this periodicity in the size of the coherent assembly of water molecules that is able to provide a precise timekeeping device for living organic structures, and which also finds expression in a rhythm of growth rate.

The derived quantum gravitational model of time perception, represented by the gravitational force eqn (4) (Dorda, 2010), suggests a physical basis for the circatidal and circadian clock in biological organisms. Circatidal rhythmicity has been described for biological organisms that populate coastal regions (Wikelski and Hau, 1995; Connor and Gracey, 2011; Fisahn et al., 2012). In order to survive the extreme short-term environmental changes, from water-immersed to exposed, intertidal organisms need to anticipate the onset of wet or dry conditions (Connor and Gracey, 2011; Fisahn et al., 2012). However, up to now, molecular biological reactions which mediate circatidal rhythmicity within these organisms have not been described. Coherent water molecule assemblies mediated by the lunar gravity field could provide the basic mechanism necessary for time perception in biological structures.

The phylogenetic transition from growth in water to growth on land required innovations that aided reproductive success in a new, drier environment. Probably, this transition was associated with the development of a circadian clock in the aerial organs that could be entrained by the solar photoperiod. Underlying models of plant circadian clocks are comprised of several feedback loops in gene expression and metabolism (Yazdanbakhsh and Fisahn, 2009, 2010, 2011). Interestingly, significant differences in clock mechanisms occur between roots and shoots of A. thaliana (James et al., 2008). As described, the underground, dark-swelling roots exhibit a circatidal rhythm of 24.8 h in free-running conditions (Barlow and Fisahn, 2012; Fisahn et al., 2012). Thus, despite extended evolutionary time spans and the concomitant adaptation of plants to terrestrial conditions, roots still possess circatidal time perception, as described for their intertidal ancestors (Barlow and Fisahn, 2012; Fisahn et al., 2012; Barlow et al., 2013). Also of interest is that, in the halophytic archaeon, Halobacterium salinarum, an organism with an extremely ancient phylogeny and which continues to exist at the interface between aqueous and terrestrial environments, out of a total of 290 genes examined, 24 % of them showed an expression pattern with a periodicity of 25 h under free-running conditions (Whitehead et al., 2009); the present-day lunar periodicity ranges from 24.7 to 25.1 h, whereas the solar day is exactly 24.0 h.

Up to 95 % of a plant’s weight can be accounted for by water within the plant. Therefore, the formation of coherent assemblies of water molecules can occur in a variety of locations within plant organs, cells and specific organelles, such as plastids. Plants, and in particular their roots, are composed of a range of diverse cell types that differ tremendously in size. Thus, the formation of a coherent unit consisting of a well-defined amount of water molecules would be permissible in appropriately sized root cells (Barlow and Fisahn, 2012). The gel-like nature of the protoplast of such cells (Pollack, 2001) may be appropriate for the integration into its structure of a dynamic coherent unit of water. Because water-based plant cells and their organelles are surrounded by non-miscible lipid membranes, coherent water assemblies that match the size of these organelles or cells (Barlow and Fisahn, 2012) will not dissipate as a consequence of diffusion. Cells that greatly exceed the size exclusion limit for coherent water molecule formation could still harbour coherent units in appropriately sized cellular organelles, i.e. plastids, nuclei, vacuoles, etc. Moreover, it has been demonstrated (Bünning and Schöne-Schneiderhöhn, 1957; Biebl, 1974) that the nuclei present in mononucleate, non-dividing guard and epidermal cells of Phaseolus, Allium and several other species exhibited diurnal and circadian oscillation of volume. Through corresponding topological changes in the structure of the nucleoplasm and the configuration of chromatin (histones and DNA, etc.) brought about by variations in the mass of the coherent water aggregates, differential expression of diurnally regulated genes might be modulated in a circatidal manner. Diurnally regulated gene transcripts involved in cell growth or cell wall biosynthesis, as expressed by parameters in the Lockhart growth equation (Lockhart, 1965), might mediate some of the rheological constraints relevant to the observed diurnally modulated root growth relations (Barlow and Fisahn, 2012; Fisahn et al., 2012; Barlow et al., 2013). These would be in addition to variations in the water content and turgor pressure of the cells which could be related to the coherent water mass.

At present, proof of the postulated interaction of the lunisolar gravitational field with coherent assemblies of water molecules is mainly correlative (Fig. 2; see also Barlow and Fisahn, 2012; Fisahn et al., 2012; Gallep et al., 2012, 2017; Barlow et al., 2013). However, experiments have been designed which could compensate the effect of lunar gravitational fields on root growth. Although hypotheses and relevant experiments in this direction are immediately obvious, the financial and mechanical engineering constraints that would need to be overcome in order to conduct these experiments would seem to exceed present priorities, especially in view of their rather limited immediate practical applications. However, preliminary results obtained by artifical experimental modulation of the lunar gravitational field are in total support of the above derived model (J. Fisahn, pers. commun.). In particular, leaf movement amplitudes of bean leaves were significantly diminished upon artificially reducing the lunar gravitational field. Moreover, we have shown that theoretical considerations within the framework of already accepted physical phenomena, such as the QHE, can fruitfully pave the way towards the discovery and manifestation of novel concepts in the field of biological time perception.

The proposed theoretical model describing the influence of lunar gravity on organic structures is based on the application of the QHE to the LGE. The fundamental background for the idea to compare the LGE with the QHE is the absence of the electron charge interaction in the QHE; and the LGE also deals with a neutral particle system. As shown above, this application results: (a) in the Planck constant h in relation to eqns (4) or (7) for the gravity force; (b) in quantization, and therefore in the existence of plateaus; (c) in the existence of a Boson-like assembly of particles, a new state of matter, characterized by coherence with the option to describe the water assembly – analogous to the electron coherence state of QHE – by one coherent aggregate of mass; and (d) in the description of these two effects by a direct relationship between the categories space and time. The only difference between LGE and QHE appears in the direct proportionality between space and time at the LGE including the necessity for constancy of the gravitational force, whereas for the QHE we have an indirect proportionality of the space–time relationship.

Synergistic results as provided by the above-derived quantum gravitational model describing the lunar gravity effect on root growth dynamics can be obtained by consideration of the mathematical framework of stochastic resonance (Fokker–Planck equation; Risken 1996). Stochastic resonance in a living system was first demonstrated by Douglass et al. (1993; see also in Moss et al., 1994) in the mechanoreceptor cells located in the tail fan of crayfish. A similar experiment using the sensory hair cells of a cricket was performed by Levin and Miller (1996). A cricket can detect an approaching predator by the coherent motion of the air, although the coherence is buried under a huge random background. Fairly convincing arguments had been given by Levin and Miller that stochastic resonance is actually responsible for the emergence of coherence enabling a highly sensitive escape response. Coherence induced by stochastic resonance is equivalent to the coherence of water molecules due to lunar gravity as introduced above. Since the functionality of neurons is based on gating ion channels in the cell membrane, Bezrukov and Vodyanoy (1995) have studied the impact of stochastic resonance on ion channel gating. Participation of ion channel gating in the LGE would be consistent with the formation of coherent water molecule assemblies in the roots of A. thaliana (Barlow, 2012). Furthermore, stochastic resonance has been studied in visual perceptions (Chialvo and Apkarian, 1993; Riani and Simonotto, 1994, 1995; Simonotto et al., 1997) and in the synchronized response of neuronal assemblies to a global low-frequency field (Gluckman et al., 1996). These effects are in synergy with the formation of coherent assemblies of water molecules in the roots of A. thaliana due to the global low frequency lunar gravity field (Barlow, 2012).

CONCLUSIONS

Circatidal periodicity modulating the root elongation profiles of A. thaliana could emerge from the formation of coherent assemblies of water molecules within appropriately sized root cells or cellular organelles. The dynamics of these coherent units could directly affect the turgor pressure within root cells and hence modulate growth. They might also diurnally modulate the expression profiles of both nuclear and organellar genes. Experience with the macroscopic quantum effects of two-dimensional systems of solid-state physics (Dorda, 1971, 1988; Dorda et al., 1978) has proven to be useful in formulating the quantized gravitational model. The fundamental prerequisite for the coherence model, which was coupled to the LGE, was the assumption that the (n ± m) water molecules can form an independent, coherent unit. This would seem to be a novel type of matter, showing only variability in space and time. It has been pointed out that analogous conditions occur in the QHE, which is also a macroscopic quantum effect (Von Klitzing et al., 1980). By analogy with the function of water molecules in the lunar gravitational effect, which mediates the causal connection between the categories space and time in a direct proportionality, it is the function of electrons in the QHE to act as a binding element between categories, but in an indirect proportionality. Due to the circatidal periodicity of the modulation in the lunar gravitational field, the dynamics immanent to coherent mass particle assemblies establish a biological timer with a periodicity of 24.8 h which exactly matches the oscillation of the root elongation rate of A. thaliana under free-running conditions.

SUPPLEMENTARY DATA

Supplementary data are available online at https://academic.oup.com/aob and consist of the following. Appendix SI: introduction of a quantized time-dependent version of Newton’s law and size of coherent neutral particle unit. Appendix SII: introduction of the variable moon-related time t_M,(n,m).