Abstract
Molecular tessellations are often discovered serendipitously, and the mechanisms by which specific molecules can be tiled seamlessly to form periodic tessellation remain unclear. Fabrication of molecular tessellation with higher symmetry compared with traditional Bravais lattices promises potential applications as photonic crystals. Here, we demonstrate that highly complex tessellation can be constructed on Au(111) from a single molecular building block, hexakis(4-iodophenyl)benzene (HPBI). HPBI gives rise to two self-assembly phases on Au(111) that possess the same geometric symmetry but different packing densities, on account of the presence of halogen-bonded and halogen–metal coordinated networks. Sub-domains of these phases with self-similarity serve as tiles in the periodic tessellations to express polygons consisting of parallelograms and two types of triangles. Our work highlights the important principle of constructing multiple phases with self-similarity from a single building block, which may constitute a new route to construct complex tessellations.
Molecular tessellations of complex tilings are difficult to design and construct. Here, the authors show that molecular tessellations can be formed from a single building block that gives rise to two distinct supramolecular phases, whose self-similar subdomains serve as tiles in the periodic tessellations.
Introduction
Two-dimensional (2D) tessellation involves the tiling of a plane using one or more closed shapes without the formation of overlaps or gaps. The idea of tessellation, which is of great importance in aesthetics1, mathematics2,3, chemistry4, and molecular science5,6, can be traced back to building decorations used by the Sumerians in ancient times7. Archimedean tiling (AT), an archetypical tessellate form, is based on the tessellation of regular polygons and can be classified into three regular tiling types and eight semi-regular tiling types8; the former relies on basic tiling of one specific polygon (squares, triangles, or hexagons), while the latter consists of tessellations of more than one type of regular polygons. Compared with regular ATs, semi-regular ATs possess intriguing photonic9–13 and diffusion14 properties. Owing to their higher rotational symmetry than the traditional Bravais lattices, semi-regular ATs have been reported to exhibit isotropic photonic bandgaps, and the isotropy is enhanced with the increasing complexity of the structures9–11. Although it is trivial to demonstrate regular AT at the atomic level (e.g. the square lattice of Cu(100) and the honeycomb structure of graphene), it remains challenging to construct semi-regular ATs at the supramolecular level.
Supramolecular chemistry15, which relies on spontaneous and reversible non-covalent interactions16–22, is attractive as it enables highly versatile fabrication of molecular architectures on surfaces. In the past decade, great effort has been devoted to the development of semi-regular AT23–27 and complex tilings28–34 on surfaces using self-assembly approaches. For instance, semi-regular trihexagonal tiling, also known as the Kagomé lattice35, is frequently realized in two-dimensional (2D) self-assembly patterns23–26. To explore much more complex tessellations, Barth and co-workers reported rare earth metal-directed self-assembly structures, which were stabilized through metal–organic coordination interaction36,37. Taking advantage of the versatility of lanthanide coordination chemistry, a variety of coordination molecular motifs were constructed by tuning the metal-to-organic molecule ratio. Random tiling of the various molecular motifs generates quasicrystalline structures36. Non-planar rubrene molecule tessellation has also been reported; in addition to two ordered phases, a non-periodic phase was constructed via the random mixing of pentagonal, hexagonal, and heptagonal units38. The ability to form tessellated structures implies certain self-similarity and hierarchical order in the building blocks, but this organization can be expressed in different ways in multinuclear nodes, which are moderated by steric constraint and molecule–substrate interaction. Compared to the non-periodic tessellation, quasicrystal and periodic tessellations with high degree of rotational symmetry are promising candidates for 2D photonic crystals10. However, the construction of periodic higher-order tessellation from two-ordered phases has been reported only rarely39.
Herein hexakis(4-iodophenyl)benzene (HPBI), a D6h symmetric molecule, is used as a building block to fabricate two periodic supramolecular phase types on Au(111). These self-assembly patterns have the same lattice symmetry but different packing densities; the variation in packing density is attributed to networks formed by halogen bonds40,41 and halogen–Au coordination, as verified via scanning tunnelling microscopy (STM) and density function theory (DFT) calculations. Notably, the self-similarity of these two phases favours interweaving between the phases to form higher-order supramolecular phases; this mechanism constitutes a new method for constructing complex 2D tessellations through the interplay between intermolecular and molecule–substrate interactions42,43.
Results
Formation of two supramolecular phases
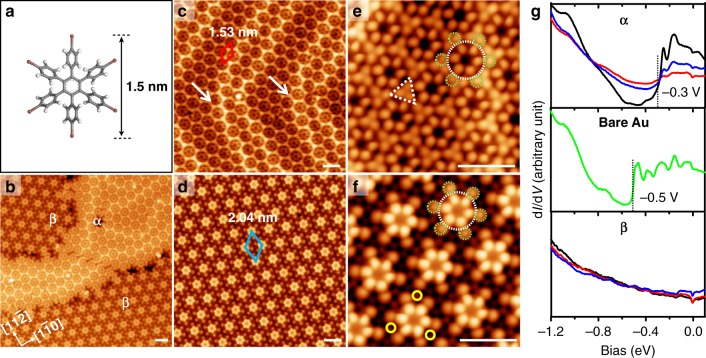
The mesogenic molecular building block, namely HPBI44,45 (synthesis details can be found in Supplementary Method), is a nonplanar molecule composed of a central benzene ring flanked by six out-of-plane p-iodophenyl groups with a lateral width of about 1.5 nm (Fig. 1a). Deposition of a submonolayer of HPBI on Au(111), followed by subsequent cooling to 0.4 K, generates two self-assembly phases with the same symmetry but different packing densities (Fig. 1b). The representative STM images of both phases (Fig. 1c, d) reveal lattice constants (red and blue unit cells) of 1.53 and 2.04 nm, giving rise to α and β phases with high (0.49 molecule nm−2) and low (0.28 molecule nm−2) packing densities, respectively. A herringbone-like reconstruction is clearly visible in the α phase (white arrows in Fig. 1c) but is not detected in β phase, which indicates different molecule–substrate interactions in the two phases.
Fig. 1.
The two-ordered self-assembly phases of HPBI on Au(111). a The chemical structure of HPBI. Carbon, grey; iodine, red; hydrogen, white. b Large-scale STM image of the coexisting α and β phases. The crystalline axes of the Au substrate are labelled. c, d STM images of the pure α (c) and β (d) phase domains. The white arrows in c designate the herringbone structure in the α phase; the α and β phase unit cells are represented by red and blue rhombi, respectively. e, f Magnified STM images of the α (e) and β (f) phases. HPBI molecules are labelled by the dashed white and green circles. The dashed white triangle in e highlights the I–I trimer. The solid yellow circles in f highlight the adatoms. g STS spectra acquired for the α (upper panel) and β (lower panel) phases and bare Au surface (middle panel). The black, blue, and red curves represent the spectra taken at the I atom, peripheral phenyl, and centre phenyl, respectively. Scale bars in all STM images: 2 nm
These two phases are detailed in Fig. 1e, f. A single HPBI molecule consists of a flower-shaped feature with six-fold symmetry, which is consistent with the C6 symmetry of the molecule. The central lobes (dashed white circle) are assigned to phenyl groups, where the contrast between the peripheral and central phenyl groups results from the nonplanar structure of HPBI; the peripheral protrusions (dashed green circles) are I atoms connected to the phenyl groups through C–I bonds. In the α phase (Fig. 1e), three I atoms from the adjacent molecules form a triangular arrangement called a halogen-3 synthon (i.e. the dashed white triangle), which is highly polarizable; owing to this polarizability, I atoms can develop σ-holes that enable them to form halogen bonds with nucleophilic I neighbours; similar halogen-bonded triangles have been reported previously46–48. The β phase can be considered as a derivative of the α phase, albeit with an enlarged intermolecular distance. Figure 1f reveals additional faint features adjacent to the I atoms (highlighted by solid yellow circles). These faint features should not be dissociated I atoms, as no dissociated aryl groups were found on the surface, which implies that HPBI was deposited intact herein. Previous studies have reported that the removal of the herringbone reconstruction is accompanied by the appearance of Au adatoms, and the formation of Au adatoms-mediated self-assembly49–51. Considering that the herringbone reconstruction is lifted in the β phase, it is reasonable to propose that the molecular network is stabilized by coordination between I atoms and Au adatoms.
The herringbone reconstruction on Au(111) is a manifestation of excess surface stress; the lifting of the reconstruction is commonly attributed to stress relief mechanism induced by molecular adsorbates52–54. Our molecular coverage experiments involving HPBI show that α phase first emerges at low molecular coverage (Fig. 2a), while the β phase appears as coverage increases and coexists with α phase (Fig. 2b). We speculate that, when the coverage of HPBI molecules reaches a critical threshold on the surface to affect the strain or charge density in one localized domain, the herringbone reconstruction is lifted and Au adatoms are ejected, leading to a phase transition to the β phase where Au adatoms participate in Au–I coordination network. The phase evolution of our supramolecular network is convoluted with the coverage-dependent evolution of surface stress on the Au(111) substrate.
Fig. 2.
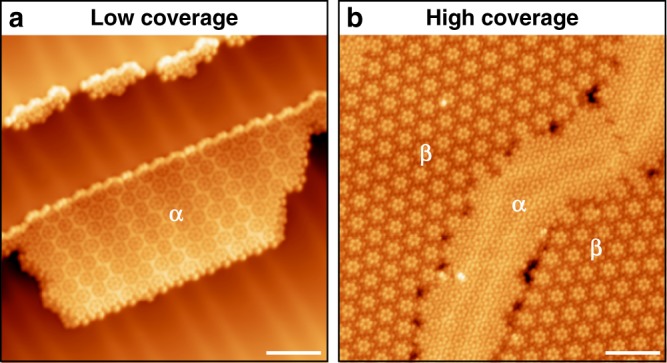
Coverage-dependent evolution of α and β phases. a STM image taken after the initial deposition of HPBI at low surface coverage. Molecules assemble to form close-packed α phase. b STM image taken at higher surface coverage of HPBI. Less compact β phase starts to appear on surface coexisting with α phase. Scale bar in all STM images: 5 nm
Scanning tunnelling spectroscopy (STS) was used at various molecular locations to investigate the interactions between the molecules and the Au substrate. As shown in Fig. 1g, dI/dV spectra of α phase HPBI display a step-like feature at –0.3 eV, while the surface state of the pristine Au(111) (middle panel) shows a characteristic step at –0.5 eV. The 0.2 eV upward shift is attributed to adsorption-induced modification of the surface state55–57. However, STS spectra of the β phase molecules show no evidence of the Au(111) surface state, this may be due to structural change or charge rearrangement58,59 at the Au–HPBI interface in the β phase, which is consistent with the appearance of Au adatoms on the substrate.
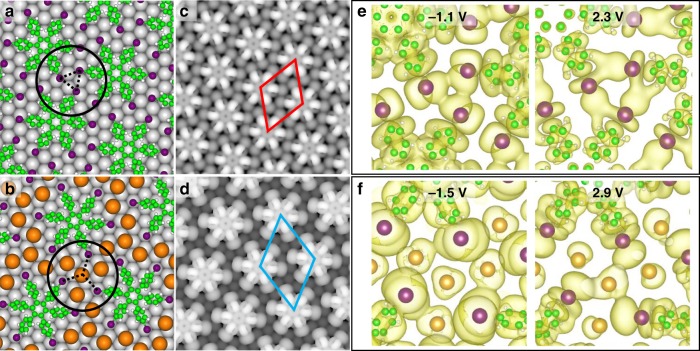
DFT calculations were performed to provide insight into the bonding interactions in both phases. The α phase model (Fig. 3a) shows that I atoms, which are adsorbed close to the Au top sites, form triple I–I halogen bonds (dashed black lines). The simulated STM image (Fig. 3c) shows excellent agreement with our experimental results (Fig. 1e). Figure 3b shows the fully relaxed β phase model, in which one Au adatom appears for every three neighbouring I atoms, consistenting with the STM results herein. In addition, the calculated unit cell lattice (2.07 nm, blue rhombus in Fig. 3d) agrees well with the experimental lattice constant (2.04 nm) determined for the β phase.
Fig. 3.
DFT calculations of the self-assembled α and β phases. a, b Calculated structural models for the α (a) and β (b) phases. c, d STM simulations of the α (c) and β (d) phases based on the structural models in a, b. e, f Real-space visualized wavefunctions of HOMO and LUMO in the α (e) and β (f) phases. Colour definitions: carbon, green; iodine, purple; Au adatom, orange
Frontier molecular orbitals were calculated to analyse intermolecular interactions. For the α phase, the highest occupied molecular orbitals (HOMO) and lowest unoccupied molecular orbitals (LUMO) have their projections on the I atoms at –1.1 eV and 2.3 eV, respectively (upper panel of Supplementary Fig. 1). Figure 3e shows that the wave functions of these states overlap within the I trimer region, which indicates I–I bonding. On the other hand, the distances between adjacent I atoms are much larger in the β phase due to coordination with Au adatoms to form I–Au–I interactions, as indicated by the plotted wavefunctions of HOMO (–1.5 V) and LUMO (2.9 V) shown in Fig. 3f. The three-centre orbital hybridization has been also reported in N–Au–N and biphenyl trimer systems previously60,61. Based on the structural models in Fig. 3a, b, the binding energies of the α and β phases are calculated to be –4.17 and –4.08 eV, respectively. The close similarity in binding energy between the two phases explains their coexistence in our experiments.
Although HPBI is an achiral molecule in the gas phase, two chiral isomers can be generated upon adsorption on surface as the tilted peripheral phenyls adopt clockwise or counter-clockwise rotations with respect to the axis perpendicular to the centre phenyl (Supplementary Fig. 2a). The chiral isomers can be resolved in high-resolution STM images, which show that the pure enantiomer usually appears in the α phase, while the racemic mixture is commonly observed in the β phase (Supplementary Fig. 2b and c). Beyond single molecules, the molecular arrangements in the α and β phases also exhibit chirality (Supplementary Fig. 3), which can be identified by the relative rotation between the molecule orientations and supramolecular organizations.
Higher-order interwoven tessellation
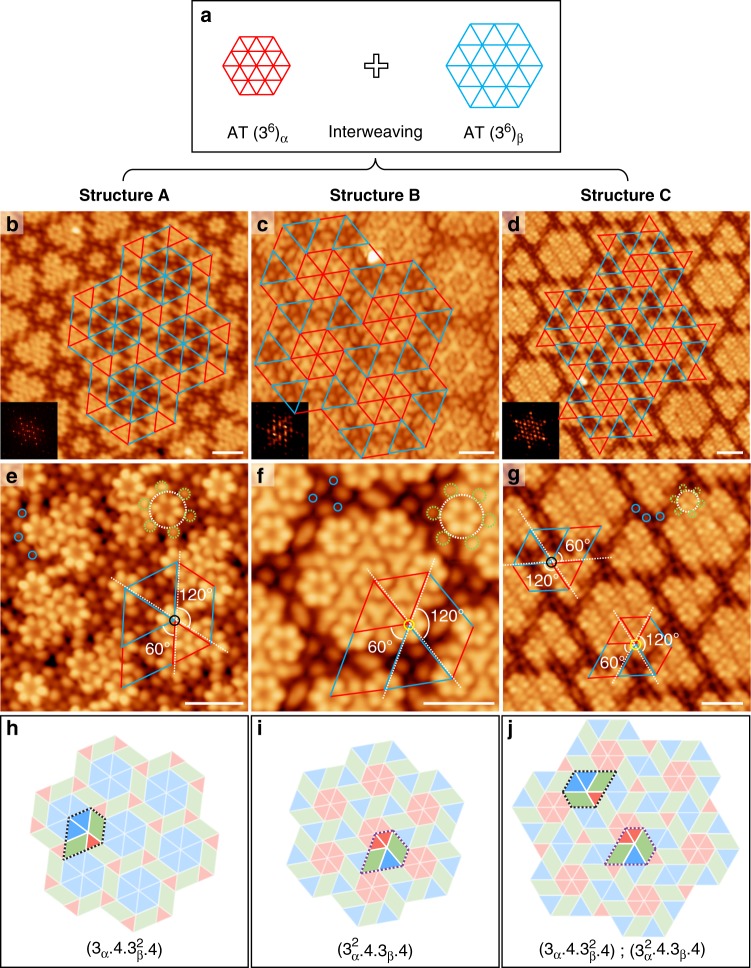
The α and β phase hexagonal arrangements can be viewed as geometric expressions of regular AT (36), where molecule centres are placed at the vertices of the polygons. Figure 4a shows a schematic illustration in which the distinct intermolecular distances connecting the adjacent vertices in the α and β phases are labelled by red and blue lines, respectively. One might ask whether these two phases can interweave to form a more complex architecture. Previous reports typically show formation of non-periodic structures when two or more ordered phases co-exist on the substrate38. In this work, in addition to the large-scale, phase-separated α and β phases, inhomogeneously mixed phases (Supplementary Figs. 4 and 5) are indeed observed in selected local areas as the HPBI coverage was increased above 0.55 ML (1 ML is defined as one monolayer of HPBI in α phase). Most intriguingly, three types of ordered mixed-phase structures (named A, B, and C) are discovered under high coverage regime; STM images and corresponding fast Fourier transform (FFT) patterns of these images show periodic arrangements of close-packed clusters with six-fold symmetry, as shown in Fig. 4b–d and insets therein. Three molecular cluster types can be resolved in these ordered structures, including monomers, triangular-shaped trimers, and hexagonal-shaped heptamers; the inter-molecular distances within the trimer and heptamer are equal to those in the α phase. Structure A is based on a monomer and a trimer, while structure B is based on a heptamer; structure C is based on a trimer and a heptamer. Interestingly, the distances between adjacent molecules located at the separated clusters (blue lines in Fig. 4b–d) is about 2.04 nm, equal to those in the β phase. Therefore, these complex structures can be interpreted as interwoven tessellations of the α and β phases. For a clearer view, the packing topologies were sketched and overlain on the STM images (Fig. 4b–d); the red and blue lines label intermolecular distances similar to those in the α and β phases, respectively. Careful comparison reveals that structures A and B can be interconverted through a simple exchange of red and blue lines. Structure C features a more complicated architecture containing units, which appear in structures A and B.
Fig. 4.
Periodic higher-order interwoven tessellations. a Schematic illustration of the AT in the α and β phases. b–d STM overviews of the three types of ordered interwoven structures with superimposed packing topologies. Insets: corresponding FFT patterns. e–g High-resolution STM images of structures A (e), B (f), and C (g). HPBI molecules are labelled by the dashed white and green circles, and the Au adatoms are labelled by blue circles. The interwoven vertices are marked by black and yellow circles. h–j Schematic tiling representations of structures A (h), B (i), and C (j) with highlighted interwoven vertex configurations. Triangles are red and blue, and parallelograms are green. In all images, intermolecular distances similar to those in the α and β phases are denoted by red and blue lines, respectively. Scale bars in all STM images: 2 nm
Enlarged views of STM images (Fig. 4e–g) reveal the detailed structures around the tessellation vertices. In structure A, the polygons that cross at the interwoven vertex (black circle in Fig. 4e) consist of one red triangle, two parallelograms, and two blue triangles. The red triangle is perfectly aligned with one of the blue triangles (highlighted by dashed white lines), leading to 60° and 120° interior angles in the parallelogram. The angular sum of the polygons involved is therefore 360°, resulting in perfect tessellation. The tile representation in Fig. 4h highlights the interwoven vertex, which is consistent with the observations in Fig. 4e. According to the nomenclature defined by Grünbaum and Shephard2, the lattice can be named using a set of integers in the form (n1α1.n2α2.···) to represent a vertex surrounded by polygons in a clockwise sequence; ni represents the number of sides on the polygon, and αi denotes the number of adjacent polygons of the same type14. Since we have two triangle types with different side lengths, we differentiate them as 3α and 3β. Therefore, structure A can be described as tiling (3α.4.3β2.4). Apparently, this packing geometry has a chiral structure. The STM images of two enantiomorphous domains are shown in Supplementary Fig. 6. Because structure B can be interconverted to structure A by exchanging red and blue lines, it can be described as tiling (3α2.4.3β.4) (see tile representation in Fig. 4i). Structure C contains two types of interwoven vertex configurations (Fig. 4j). As shown in Fig. 4g, the vertex marked by the yellow circle has an arrangement identical to that in structure B (Fig. 4f), while the vertex marked by the black circle is the chiral isomer of that shown in structure A (Fig. 4e). This complex configuration can be expressed as tiling (3α.4.3β2.4); (3α2.4.3β.4).
These complex tessellations can be regarded as two different phases interweaving in multiple ways. Although 2D molecular tessellation involving multiple polygon types has been demonstrated previously27,36–38, most studies reported construction from one specific assembly phase. In our case, owing to the co-existence of two distinct lattice constants originating from two phases, irregular polygons (parallelograms) are incorporated in the tessellation when two sub-domains with different lattices cross at a common vertex. Despite this complexity, the three higher-order structures maintain the six-fold symmetry of the α and β phases. Previous theoretical investigations have demonstrated that balanced entropy and enthalpy plays an important role in the formation of quasicrystalline and related complex structures62,63. From an entropic view point, random mixing of α and β sub-domains should occur; however, the highly oriented packing of α and β sub-domains with the gold substrate indicates considerable molecule–substrate interaction, which provide the enthalpy necessary for the generation of the periodic interweaving architectures. The formation of the manifold complex tessellations is most likely due to the competition between halogen–halogen, halogen–metal, and molecule–substrate interactions.
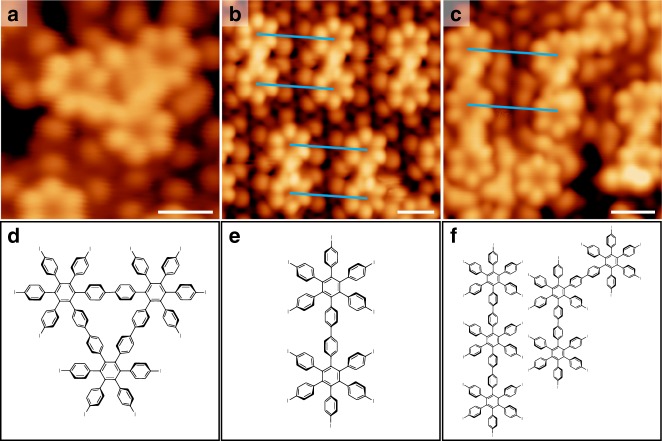
In order to determine whether the self-assembled network facilitates direct C–C coupling reactions, Ullmann coupling is initiated by annealing the sample containing various self-assembled phases to 200–400 °C, followed by cooling down and measuring at 0.4 K. Periodic complex tessellations are not detected after annealing, instead, triangle-shaped oligomers (Fig. 5a) are formed, which inherit the parental topology of the self-assembled triangular-shaped α domains. Enhanced local density of state observed at the junctions between molecules (Fig. 5a) indicates chemical bond formation (schematic model, Fig. 5d). Owing to molecular rotation and diffusion at elevated temperature, oligomers with different structures are also detected in our experiments. For example, dimer and trimer chains (STM images in Fig. 5b, c, schematic models in Fig. 5e, f) are observed. The distances between adjacent dimers or trimers (marked by blue lines in Fig. 5b, c) are the same as the intermolecular distance observed in β phase, which indicates that the surface reaction is guided by the pre-assembled structure. This reveals that on-surface reactions can be controlled by the pre-packed molecular assembly, which could constitute a promising strategy for the synthesis of C–C-coupled molecular clusters.
Fig. 5.
Ullmann reaction of HPBI on Au(111). a STM image of a triangular oligomer formed from three HPBI molecules. b STM image of linear oligomers formed from two HPBI molecules. c STM image of linear and angled oligomers formed from three HPBI molecules. The distances between the adjacent oligomer chains are the same as the intermolecular distances in the β phase, as indicated by the blue lines. d–f The corresponding structure models of STM images in a–c. Scale bar in all STM images: 1 nm
Discussion
We have demonstrated that ordered supramolecular architecture can be constructed from the tessellation of high- and low-density molecular phases. The two molecular phases arise from distinct intermolecular and molecule–substrate interactions; the high-density phase is constructed from halogen bonds, while the low-density phase is formed via a coordinated halogen–Au network. The evolution of these molecular phases are convoluted with strain-relieving mechanism on the reconstructed domains of Au(111). The self-similarity of the two molecular phases allows clusters from each to tessellate and form high-order supramolecular networks. This approach opens up new route to construct complex surface tessellation by considering the symmetry of building block and substrate, as well as by introducing multimode interactions. In addition, the complex tessellations in our work may provide new insights for understanding self-organized systems in biology and nanotechnology64.
Methods
Sample preparations
All experiments were carried out in an ultrahigh vacuum system (base pressure <3.0 × 10−10 mbar) equipped with a Unisoku low-temperature STM. A single Au(111) crystal was cleaned by repeated cycles of Ar+ sputtering (1.0 keV) followed by annealing at 600 °C, until no contamination could be detected by STM. Before use, the HPBI powder was outgassed at 300 °C for several hours. HPBI molecules were then deposited from a Knudsen cell at 420 °C; the substrate was kept at room temperature during molecule deposition.
STM/STS experiments
After the deposition of HPBI on the Au(111) substrate, the sample was transferred in situ to the analysis chamber equipped with a low-temperature STM. All STM and STS measurements were conducted at 0.4 K with electro-chemically etched tungsten tips. The STM images were collected under constant-current mode. The piezo was calibrated using an HOPG sample. The STS point spectra (dI/dV) were measured using standard lock-in techniques with a voltage modulation of 10 mV and frequency of 987 Hz. Before real-sample STS measurements, the tip was calibrated on a clean Au(111) sample or the bare Au area on a real sample. STM/STS data were analysed and rendered using the WSxM software.
Theoretical calculations
Calculations were performed using the Perdew–Burke–Ernzerhof function (for the exchange-correlation function)65, the projector augmented method66,67, and a plane-wave basis set, as implemented in the Vienna ab initio simulation package68; a semi-empirical dispersion correction (DFT-D3) proposed by Grimme et al. was used in the simulation69. The energy cutoff for the plane-wave basis was set to 400 eV. The optimized lattice parameter for the bulk Au was a = 4.097 Å. Slab models were used to simulate the Au(111) surface. The slab was constructed with 5 layers of Au atoms and a vacuum space of 20 Å in the z direction. Only gamma points were used to sample the first Brillouin zone of the supercell. All atoms except for the bottom layer were fully relaxed until the residual force for each atom was <0.02 eV Å−1. STM simulation was performed using the Tersoff–Hamann approximation70. The charge densities on the surface within a specific energy range, which are selected to match with the experimental bias, are calculated pixel by pixel. The constant charge density contour is generated as the simulated constant-current image, where the intensity of a specific pixel is determined by its vertical height. The interaction between tip and surface is ignored in the calculations.
Electronic supplementary material
Acknowledgements
K.P.L. acknowledges NRF-CRP grant “Two Dimensional Covalent Organic Framework: Synthesis and Applications”. Grant number NRF-CRP16-2015-02, funded by National Research Foundation, Prime Minister’s Office, Singapore. The authors gratefully acknowledge funding support from MOE Tier 3 programme (MOE2014-T3-1-004), the National Natural Science Foundation of China (Grant Nos. 91433103, 11622437, 11804247), the Fundamental Research Funds for the Central Universities of China (Grant Nos. 16XNLQ01 (RUC), 020514380162), and Strategic Priority Research Program of Chinese Academy of Sciences (Grant No. XDB30000000). Calculations were performed at the Physics Lab of High-Performance Computing of Renmin University of China and the Shanghai Supercomputer Center.
Author contributions
F.C., X.-J.W., and K.P.L. conceived the idea and designed the experiments. F.C., Y.S., and H.X. performed the STM and STS measurements. Z.H. and W.J. carried out the theoretical calculations. X.L. synthesized the molecule precursor under the supervision of J.W. F.C., X.-J.W., Z.H., Z.D., W.J., and K.P.L. analysed the data. F.C., X.-J.W., and K.P.L. wrote the paper with input from all the authors.
Data availability
All data are available from the authors upon reasonable request.
Competing interests
The authors declare no competing interests.
Footnotes
Publisher’s note: Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
These authors contributed equally: Fang Cheng, Xue-Jun Wu, Zhixin Hu.
Electronic supplementary material
Supplementary Information accompanies this paper at 10.1038/s41467-018-07323-6.
References
- 1.Escher, M. C. The World of M. C. Escher (Harry N. Abrams, New York, 1991).
- 2.Grünbaum, B. & Shephard, G. C. Tilings and Patterns (W. H. Freeman, New York, 1986).
- 3.Tóth LF. On shortest nets with meshes of equal area. Acta Math. Acad. Sci. Hungar. 1960;11:363–370. doi: 10.1007/BF02020950. [DOI] [Google Scholar]
- 4.Frank FC, Kasper JS. Complex alloy structures regarded as sphere packings. 1. Definitions and basic principles. Acta Cryst. 1958;11:184–190. doi: 10.1107/S0365110X58000487. [DOI] [Google Scholar]
- 5.Barth JV. Molecular architectonic on metal surfaces. Annu. Rev. Phys. Chem. 2007;58:375–407. doi: 10.1146/annurev.physchem.56.092503.141259. [DOI] [PubMed] [Google Scholar]
- 6.Bartels L. Tailoring molecular layers at metal surfaces. Nat. Chem. 2010;2:87–95. doi: 10.1038/nchem.517. [DOI] [PubMed] [Google Scholar]
- 7.Pickover, C. A. The Math Book: From Pythagoras to the 57th Dimension, 250 Milestones in the History of Mathematics (Sterling, New York, 2012).
- 8.Kepler, J. Harmonices Mundi (Johannes Planck, Linz, 1619).
- 9.David S, Chelnokov A, Lourtioz JM. Wide angularly isotropic photonic bandgaps obtained from two-dimensional photonic crystals with Archimedean-like tilings. Opt. Lett. 2000;25:1001–1003. doi: 10.1364/OL.25.001001. [DOI] [PubMed] [Google Scholar]
- 10.David S, Chelnikov A, Lourtioz JM. Isotropic photonic structures: Archimedean-like tilings and quasi-crystals. IEEE J. Quantum Electron. 2001;37:1427–1434. doi: 10.1109/3.958365. [DOI] [Google Scholar]
- 11.Jovanović Ɖ, Gajić R, Hingerl K. Refraction and band isotropy in 2D square-like Archimedean photonic crystal lattices. Opt. Express. 2008;16:4048–4058. doi: 10.1364/OE.16.004048. [DOI] [PubMed] [Google Scholar]
- 12.Ueda K, Dotera T, Gemma T. Photonic band structure calculations of two-dimensional Archimedean tiling patterns. Phys. Rev. B. 2007;75:195122. doi: 10.1103/PhysRevB.75.195122. [DOI] [Google Scholar]
- 13.Stelson AC, Britton WA, Watson CML. Photonic crystal properties of self-assembled Archimedean tilings. J. Appl. Phys. 2017;121:023101. doi: 10.1063/1.4973472. [DOI] [Google Scholar]
- 14.Basnarkov L, Urumov V. Diffusion on Archimedean lattices. Phys. Rev. E. 2006;73:046116. doi: 10.1103/PhysRevE.73.046116. [DOI] [PubMed] [Google Scholar]
- 15.Moulton B, Zaworotko MJ. From molecules to crystal engineering: supramolecular isomerism and polymorphism in network solids. Chem. Rev. 2001;101:1629–1658. doi: 10.1021/cr9900432. [DOI] [PubMed] [Google Scholar]
- 16.Rabe JP, Buchholz S. Commensurability and mobility in two-dimensional molecular patterns on graphite. Science. 1991;253:424–427. doi: 10.1126/science.253.5018.424. [DOI] [PubMed] [Google Scholar]
- 17.Stöhr M, et al. Self-assembly and two-dimensional spontaneous resolution of cyano-functionalized [7]helicenes on Cu(111) Angew. Chem. Int. Ed. 2011;50:9982–9986. doi: 10.1002/anie.201102627. [DOI] [PubMed] [Google Scholar]
- 18.Wasio NA, et al. Self-assembly of hydrogen-bonded two-dimensional quasicrystals. Nature. 2014;507:86–89. doi: 10.1038/nature12993. [DOI] [PubMed] [Google Scholar]
- 19.Zheng QN, et al. Formation of halogen bond-based 2D supramolecular assemblies by electric manipulation. J. Am. Chem. Soc. 2015;137:6128–6131. doi: 10.1021/jacs.5b02206. [DOI] [PubMed] [Google Scholar]
- 20.Kley CS, et al. Highly adaptable two-dimensional metal-organic coordination networks on metal surfaces. J. Am. Chem. Soc. 2012;134:6072–6075. doi: 10.1021/ja211749b. [DOI] [PubMed] [Google Scholar]
- 21.Ciesielski A, Samorì P. Supramolecular approaches to graphene: from self-assembly to molecule-assisted liquid-phase exfoliation. Adv. Mater. 2016;28:6030–6051. doi: 10.1002/adma.201505371. [DOI] [PubMed] [Google Scholar]
- 22.Otero R, et al. Elementary structural motifs in a random network of cytosine adsorbed on a Gold(111) Surf. Sci. 2008;319:312–315. doi: 10.1126/science.1150532. [DOI] [PubMed] [Google Scholar]
- 23.Schlickum U, et al. Chiral Kagomé lattice from simple ditopic molecular bricks. J. Am. Chem. Soc. 2008;130:11778–11782. doi: 10.1021/ja8028119. [DOI] [PubMed] [Google Scholar]
- 24.Tahara K, et al. Two-dimensional porous molecular networks of dehydrobenzo[12]annulene derivatives via alkyl chain interdigitation. J. Am. Chem. Soc. 2006;128:16613–16625. doi: 10.1021/ja0655441. [DOI] [PubMed] [Google Scholar]
- 25.Mao J, et al. Tunability of supramolecular kagome lattices of magnetic phthalocyanines using graphene-based moiré patterns as templates. J. Am. Chem. Soc. 2009;131:14136–14137. doi: 10.1021/ja904907z. [DOI] [PubMed] [Google Scholar]
- 26.Shi Z, Lin N. Porphyrin-based two-dimensional coordination Kagome lattice self-assembled on a Au(111) surface. J. Am. Chem. Soc. 2009;131:5376–5377. doi: 10.1021/ja900499b. [DOI] [PubMed] [Google Scholar]
- 27.Zhang YQ, et al. Complex supramolecular interfacial tessellation through convergent multi-step reaction of a dissymmetric simple organic precursor. Nat. Chem. 2018;10:296–304. doi: 10.1038/nchem.2924. [DOI] [PubMed] [Google Scholar]
- 28.Newkome GR, et al. Nanoassembly of a fractal polymer: a molecular “Sierpinski hexagonal gasket”. Science. 2006;312:1782–1785. doi: 10.1126/science.1125894. [DOI] [PubMed] [Google Scholar]
- 29.Shang J, et al. Assembling molecular Sierpinski triangle fractals. Nat. Chem. 2015;7:389–393. doi: 10.1038/nchem.2211. [DOI] [PubMed] [Google Scholar]
- 30.Blunt MO, et al. Random tiling and topological defects in a two-dimensional molecular network. Science. 2008;322:1077–1081. doi: 10.1126/science.1163338. [DOI] [PubMed] [Google Scholar]
- 31.Marschall M, et al. Random two-dimensional string networks based on divergent coordination assembly. Nat. Chem. 2010;2:131–137. doi: 10.1038/nchem.503. [DOI] [PubMed] [Google Scholar]
- 32.Bauert T, et al. Building 2D crystals from 5-fold-symmetric molecules. J. Am. Chem. Soc. 2009;131:3460–3461. doi: 10.1021/ja8101083. [DOI] [PubMed] [Google Scholar]
- 33.Stannard A, et al. Broken symmetry and the variation of critical properties in the phase behaviour of supramolecular rhombus tilings. Nat. Chem. 2012;4:112–117. doi: 10.1038/nchem.1199. [DOI] [PubMed] [Google Scholar]
- 34.Pfeiffer CR, Pearce N, Champness NR. Complexity of two-dimensional self-assembled arrays at surfaces. Chem. Commun. 2017;53:11528–11539. doi: 10.1039/C7CC06110B. [DOI] [PubMed] [Google Scholar]
- 35.Syôzi I. Statistics of Kagomé lattice. Prog. Theor. Phys. 1951;6:306–308. doi: 10.1143/ptp/6.3.306. [DOI] [Google Scholar]
- 36.Urgel JI, et al. Quasicrystallinity expressed in two-dimensional coordination networks. Nat. Chem. 2016;8:657–662. doi: 10.1038/nchem.2507. [DOI] [PubMed] [Google Scholar]
- 37.Ecija D, et al. Five-vertex Archimedean surface tessellation by lanthanide-directed molecular self-assembly. Proc. Natl. Acad. Sci. USA. 2013;110:6678–6681. doi: 10.1073/pnas.1222713110. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 38.Pivetta M, Blum MC, Patthey F, Schneider WD. Two-dimensional tiling by rubrene molecules self-assembled in supramolecular pentagons, hexagons, and heptagons on a Au(111) surface. Angew. Chem. Int. Ed. 2008;47:1076–1079. doi: 10.1002/anie.200704479. [DOI] [PubMed] [Google Scholar]
- 39.Guillermet O, et al. Self-assembly of fivefold-symmetric molecules on a threefold-symmetric surface. Angew. Chem. Int. Ed. 2009;48:1970–1973. doi: 10.1002/anie.200805689. [DOI] [PubMed] [Google Scholar]
- 40.Gutzler R, et al. Halogen bonds as stabilizing interactions in a chiral self-assembled molecular monolayer. Chem. Commun. 2011;47:9453–9455. doi: 10.1039/c1cc13114a. [DOI] [PubMed] [Google Scholar]
- 41.Gutzler R, et al. Halogen bonds in 2D supramolecular self-assembly of organic semiconductors. Nanoscale. 2012;4:5965–5971. doi: 10.1039/c2nr31648j. [DOI] [PubMed] [Google Scholar]
- 42.Bonifazi D, Mohnani S, Llanes-Pallas A. Supramolecular chemistry at interfaces: molecular recognition on nanopatterned porous surfaces. Chem. Eur. J. 2009;15:7004–7025. doi: 10.1002/chem.200900900. [DOI] [PubMed] [Google Scholar]
- 43.De Feyter S, De Schryver FC. Two-dimensional supramolecular self-assembly probed by scanning tunneling microscopy. Chem. Soc. Rev. 2003;32:139–150. doi: 10.1039/b206566p. [DOI] [PubMed] [Google Scholar]
- 44.Wu J, et al. Arylamine-substituted hexa-peri-hexabenzocoronenes: facile synthesis and their potential applications as “Coaxial” hole-transport materials. Angew. Chem. Int. Ed. 2004;43:5331–5335. doi: 10.1002/anie.200460174. [DOI] [PubMed] [Google Scholar]
- 45.Kobayashi K, et al. Syntheses of hexakis(4-functionalized-phenyl)benzenes and hexakis[4-(4‘-functionalized-phenylethynyl)phenyl]benzenes directed to host molecules for guest-inclusion networks. J. Org. Chem. 2005;70:749–752. doi: 10.1021/jo048521i. [DOI] [PubMed] [Google Scholar]
- 46.Wang W, et al. Single-molecule resolution of an organometallic intermediate in a surface-supported Ullmann coupling reaction. J. Am. Chem. Soc. 2011;133:13264–13267. doi: 10.1021/ja204956b. [DOI] [PubMed] [Google Scholar]
- 47.Han Z, et al. Imaging the halogen bond in self-assembled halogenbenzenes on silver. Science. 2017;358:206–210. doi: 10.1126/science.aai8625. [DOI] [PubMed] [Google Scholar]
- 48.Fan Q, et al. Surface adatom mediated structural transformation in bromoarene monolayers: precursor phases in surface Ullmann reaction. ACS Nano. 2018;12:2267–2274. doi: 10.1021/acsnano.7b06787. [DOI] [PubMed] [Google Scholar]
- 49.Maksymovych P, Sorescu DC, Yates JT., Jr. Gold-adatom-mediated bonding in self-assembled short-chain alkanethiolate species on the Au(111) surface. Phys. Rev. Lett. 2006;97:146103. doi: 10.1103/PhysRevLett.97.146103. [DOI] [PubMed] [Google Scholar]
- 50.Faraggi MN, et al. Bonding and charge transfer in metal–organic coordination networks on Au(111) with strong acceptor molecules. J. Phys. Chem. C. 2012;116:24558–24565. doi: 10.1021/jp306780n. [DOI] [Google Scholar]
- 51.Yang Z, et al. Orbital redistribution in molecular nanostructures mediated by metal–organic bonds. ACS Nano. 2014;8:10715–10722. doi: 10.1021/nn504431e. [DOI] [PubMed] [Google Scholar]
- 52.Driver SM, Zhang T, King DA. Massively cooperative adsorbate-induced surface restructuring and nanocluster formation. Angew. Chem. Int. Ed. 2007;46:700–703. doi: 10.1002/anie.200603325. [DOI] [PubMed] [Google Scholar]
- 53.Rossel F, et al. Modified herringbone reconstruction on Au(111) induced by self-assembled Azure A islands. Surf. Sci. 2008;602:L115–L117. doi: 10.1016/j.susc.2008.06.009. [DOI] [Google Scholar]
- 54.Baber AE, Jensen SC, Iski EV, Sykes ECH. Extraordinary atomic mobility of Au{111} at 80 Kelvin: effect of styrene adsorption. J. Am. Chem. Soc. 2006;128:15384–15385. doi: 10.1021/ja065904k. [DOI] [PubMed] [Google Scholar]
- 55.Andreev T, Barke I, Hövel H. Adsorbed rare-gas layers on Au(111): shift of the Shockley surface state studied with ultraviolet photoelectron spectroscopy and scanning tunneling spectroscopy. Phys. Rev. B. 2004;70:205426. doi: 10.1103/PhysRevB.70.205426. [DOI] [Google Scholar]
- 56.Repp J, Meyer G, Rieder KH. Snell’s law for surface electrons: refraction of an electron gas imaged in real space. Phys. Rev. Lett. 2004;92:036803. doi: 10.1103/PhysRevLett.92.036803. [DOI] [PubMed] [Google Scholar]
- 57.Gonzalez-Herrero H, et al. Graphene tunable transparency to tunneling electrons: a direct tool to measure the local coupling. ACS Nano. 2016;10:5131–5144. doi: 10.1021/acsnano.6b00322. [DOI] [PubMed] [Google Scholar]
- 58.Della Pia A, et al. Two-dimensional core-shell donor-acceptor assemblies at metal-organic interfaces promoted by surface-mediated charge transfer. Nanoscale. 2016;8:19004–19013. doi: 10.1039/C6NR06527A. [DOI] [PubMed] [Google Scholar]
- 59.Gerbert D, Tegeder P. Adsorbate-mediated relaxation dynamics of hot electrons at metal/organic interfaces. Phys. Rev. B. 2017;96:144304. doi: 10.1103/PhysRevB.96.144304. [DOI] [Google Scholar]
- 60.Wang CG, Cheng ZH, Qiu XH, Ji W. Unusually high electron density in an intermolecular non-bonding region: Role of metal substrate. Chin. Chem. Lett. 2017;28:759–764. doi: 10.1016/j.cclet.2016.08.004. [DOI] [Google Scholar]
- 61.Zhou X, et al. Steering surface reaction dynamics with a self-assembly strategy: Ullmann coupling on metal surfaces. Angew. Chem. Int. Ed. 2017;56:12852–12856. doi: 10.1002/anie.201705018. [DOI] [PubMed] [Google Scholar]
- 62.Iacovella CR, Keys AS, Glotzer SC. Self-assembly of soft-matter quasicrystals and their approximants. Proc. Natl. Acad. Sci. USA. 2011;108:20935–20940. doi: 10.1073/pnas.1019763108. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 63.Barkan K, Engel M, Lifshitz R. Controlled self-assembly of periodic and aperiodic cluster crystals. Phys. Rev. Lett. 2014;113:098304. doi: 10.1103/PhysRevLett.113.098304. [DOI] [PubMed] [Google Scholar]
- 64.Lehn JM. Perspectives in chemistry--steps towards complex matter. Angew. Chem. Int. Ed. 2013;52:2836–2850. doi: 10.1002/anie.201208397. [DOI] [PubMed] [Google Scholar]
- 65.Perdew JP, Burke K, Ernzerhof M. Generalized gradient approximation made simple. Phys. Rev. Lett. 1996;77:3865. doi: 10.1103/PhysRevLett.77.3865. [DOI] [PubMed] [Google Scholar]
- 66.Blöchl PE. Projector augmented-wave method. Phys. Rev. B. 1994;50:17953–17979. doi: 10.1103/PhysRevB.50.17953. [DOI] [PubMed] [Google Scholar]
- 67.Kresse G, Joubert D. From ultrasoft pseudopotentials to the projector augmented-wave method. Phys. Rev. B. 1999;59:1758–1775. doi: 10.1103/PhysRevB.59.1758. [DOI] [Google Scholar]
- 68.Kresse G, Furthmüller J. Efficient iterative schemes for ab initio total-energy calculations using a plane-wave basis set. Phys. Rev. B. 1996;54:11169–11186. doi: 10.1103/PhysRevB.54.11169. [DOI] [PubMed] [Google Scholar]
- 69.Grimme S, Antony J, Ehrlich S, Krieg H. A consistent and accurate ab initio parametrization of density functional dispersion correction (DFT-D) for the 94 elements H-Pu. J. Chem. Phys. 2010;132:154104. doi: 10.1063/1.3382344. [DOI] [PubMed] [Google Scholar]
- 70.Tersoff J, Hamann DR. Theory of the scanning tunneling microscope. Phys. Rev. B. 1985;31:805–813. doi: 10.1103/PhysRevB.31.805. [DOI] [PubMed] [Google Scholar]
Associated Data
This section collects any data citations, data availability statements, or supplementary materials included in this article.
Supplementary Materials
Data Availability Statement
All data are available from the authors upon reasonable request.