Is biasing offspring sex ratio adaptive? A test of Fisher's principle across multiple generations of a wild mammal in a fluctuating environment

Abstract

Fisher's principle explains that population sex ratio in sexually reproducing organisms is maintained at 1 : 1 owing to negative frequency-dependent selection, such that individuals of the rare sex realize greater reproductive opportunity than individuals of the more common sex until equilibrium is reached. If biasing offspring sex ratio towards the rare sex is adaptive, individuals that do so should have more grandoffspring. In a wild population of North American red squirrels (Tamiasciurus hudsonicus) that experiences fluctuations in resource abundance and population density, we show that overall across 26 years, the secondary sex ratio was 1 : 1; however, stretches of years during which adult sex ratio was biased did not yield offspring sex ratios biased towards the rare sex. Females that had litters biased towards the rare sex did not have more grandoffspring. Critically, the adult sex ratio was not temporally autocorrelated across years, thus the population sex ratio experienced by parents was independent of the population sex ratio experienced by their offspring at their primiparity. Expected fitness benefits of biasing offspring sex ratio may be masked or negated by fluctuating environments across years, which limit the predictive value of the current sex ratio.

1. Introduction

The 1 : 1 ratio of males : females in sexually reproducing populations and the selective forces acting to maintain it were first discussed by Darwin [1] and first mathematically described by Düsing [2]. Fisher further advanced the principle that the evolutionarily stable strategy in sexually reproducing organisms would be an equal investment into sons and daughters, maintaining a 1 : 1 ratio [3]. Fisher's principle assumes that there is random Mendelian segregation of the alleles controlling sex, and that equal investment in males and females will only result in a 1 : 1 ratio if male and female offspring are equally costly to produce [3–6]. Under these assumptions, an individual that biases their offspring sex ratio towards the sex that is rare in the population should experience an increase in fitness through negative frequency-dependent selection favouring individuals who invest more in the rare sex, as the rare sex offspring will have better mating prospects.

Determining the fitness consequences when individuals bias their offspring sex ratio requires a system in which the assumptions of the principle are expected to be met, but also in which deviations from the Fisherian 1 : 1 ratio and variation in offspring sex ratio may occur under certain conditions. For example, Trivers & Willard [7] hypothesized that in polygynous species the benefits of producing successful offspring of the sex with greater reproductive skew (and the risks of producing unsuccessful offspring) are much higher than the benefits of producing successful offspring of the sex with lower reproductive skew [8,9]. Therefore, in situations where maternal condition (such as age, body condition or social rank; [10]) influences offspring condition and reproductive success differentially between the offspring sexes, a parent should invest more in the sex which would provide a greater fitness benefit, either by allocating more energy into individuals of that sex or by producing more offspring of that sex. Alternatively, competition for local resources may favour the dispersing sex should there be a sex difference in dispersal patterns (local resource competition hypothesis [11]).

While the offspring sex ratios from which individuals may benefit can differ depending on species-specific ecologies, the fundamental predictions of adaptive sex ratio hypotheses are the same: parents that bias their offspring sex ratio (actively or passively) towards the ‘favourable’ sex will have more grandoffspring than parents that either do not bias their offspring sex ratio or who bias it towards the ‘unfavourable’ sex [5,6]. In a multi-species analysis of zoo breeding records, mammals that produced ‘favourably’ biased offspring sex ratios had more grandoffspring [6]. In the context of Fisher's principle, biased production of the rare sex occurs in wild mammals including black rhinoceros (Diceros bicornis) [12] and mountain goats (Oreamnos americanus) [13], but whether a mother has more grandoffspring by biasing offspring sex ratios towards the rare sex has not been tested in a wild mammal species in which we expect equal investment in males and females and where social hierarchy is not expected.

Here, we investigate whether the sex ratios of a wild population of North American red squirrels (Tamiasciurus hudsonicus, hereafter squirrels) support the assumptions of Fisher's principle, and whether it is adaptive for individuals to bias their offspring sex ratio. We first asked if the population exhibited the expected 1 : 1 adult sex ratio and if there was annual variation in adult and litter sex ratios. We next investigated whether environmental factors (food abundance and population density) or maternal quality (age and parturition date) influenced litter sex ratios. Finally, we determined whether females that produced litters biased towards the rare sex ultimately had more grandoffspring.

2. Methods

(a). Red squirrel life history

Female squirrels typically have one litter with an average litter size of three pups (range: 1–7 pups) in a year, but may breed again following an unsuccessful litter or in years where they anticipate high resource abundance [14,15]. We investigated all litters born each year. Age-related variation in reproductive output by females, shifts in population density and variation in environmental resource abundance have been shown to influence offspring sex ratio in other systems (e.g. [16–18]). Red squirrels exhibit variation in all of these factors as follows, and thus we deemed it important to investigate their effects on litter sex ratio. Younger inexperienced females (1–2 years old) and older females (greater than 4 years old) produce fewer recruits than prime-age females [15,19,20]. White spruce (Picea glauca), which provides the main source of food for squirrels at our study site in southwest Yukon [21,22], is a masting species with years of superabundant cone production interspersed with multiple years of low or no cone production [21]. Owing to this fluctuation in annual food abundance, squirrel density also fluctuates [23].

There is little evidence in this system suggesting one sex is more costly to produce than the other. Growth and survival of males and females are similar to each other across fluctuating levels of food abundance [24], suggesting that parental investment may not be allocated differently across gradients of maternal quality (in as much as maternal quality is related to food abundance). Dispersal distance is also similar between the sexes [25], though in situations when mothers bequeath some or all of their territory to offspring, daughters are more likely to be recipients [26]. Squirrels are essentially sexually monomorphic (adult males are 5–10% larger than females, but mass distributions overlap considerably [24]), and body mass is not correlated with male mating success; [27]. Instead, the maintenance of individual territories by both sexes [28] leads to a polygynandrous, scramble-competition mating system in which males and females have multiple mates [29]. Because sexual selection favours male search effort and ability rather than sexual dimorphism (reproductive output is higher for males that range farther and locate more oestrous females [27]), it is not clear whether maternal investment in male offspring has an effect on reproductive skew in adulthood.

We did not expect to see Trivers–Willard-like effects on litter sex ratios, but nevertheless identified maternal-level factors including age, parturition date and dam identity (ID) for the following reasons. Earlier parturition dates are favoured overall, although there is variation in this effect [30,31], and females supplemented with food breed earlier in spring [32]. It is conceivable that females which are prime age [15] and/or able to breed earlier in the year may be of higher ‘quality’ than non-prime aged or later-breeding females. Finally, the social ranking of the mothers as a metric of the condition is generally not applicable in this system given their asocial territoriality.

(b). Study location and population monitoring

We used targeted live-trapping of individuals to completely enumerate a population near Kluane National Park in southwest Yukon, Canada (61°N 138°W) from 1990 to 2015. We tracked population demographics and density, litter sex ratio and breeding phenology on two approximately 40 ha study grids named Kloo (KL) and Sulphur (SU; for complete details, see [15,30]). We determined annual population density using the number of squirrels that occupied and defended territories during a two-week interval each spring in May, based on trapping and behavioural observations (territorial ‘rattle’ calls) of colour-marked individuals [33]. We used the number of individuals of each sex recorded during the spring census each year to calculate annual adult sex ratio and the overall adult sex ratio for each grid across the 26 years.

We monitored the reproductive status of females each year by directly enumerating their offspring, accessed in natal nests within days of parturition [15]. We located nests and determined maternity by following females to active nests using radiotelemetry and/or behavioural observations. We weighed and sexed all pups and collected a small tissue biopsy from the outer margin of the ear. We estimated parturition dates using a combination of maternal mass or behaviour (e.g. if we observed her oestrous date) and mass of pups [34]. Litter sex ratios in this population are very close to secondary sex ratios (determined at 6.8 ± 0.2 d post-parturition; median = 5 d; mode = 2 d; [15]). We returned to nests approximately 25 days later to tag and weigh the pups that had survived to this date. Ages of breeding females were known with certainty for those who were born within the study area (n = 503). For immigrant females (n = 195), we defined the minimum age at first capture to be 1 year if nipple morphology suggested that they had never bred, and two years if they appeared to have previously bred [15]. Maternal age ranged from 1 to 8 years of age. As there were only four females that reproduced at age 8 (one litter per female in this age class), these litters were collapsed into the next lower maternal age category (7+ years).

Squirrels cache white spruce cones in late summer/early autumn to fuel reproduction during the following winter/spring [35]. We estimated the abundance of cones on a sample of trees (n = 81–168 trees) each year on each grid by counting the number of cones visible on one side in the top 3 m of the crown of each tree in August. This ln-transformed index of cone production [21] is predictive of the timing of breeding the following spring (i.e. the effect of cones in year t − 1; [14]).

(c). Determining maternity and paternity

We knew maternity with confidence from behaviour as described above and confirmed genetically. We determined paternity genetically commencing in 2003 [29]. Briefly, we took tissue samples from the outer margin of the squirrel's ear using a sterile biopsy punch and stored in 95% ethanol. We extracted DNA from preserved tissue using either an acetate-alcohol precipitation protocol [36] or DNeasy Tissue extraction kits (QIAGEN, Venlo, The Netherlands). We performed polymerase chain reaction amplification for a panel of 16 microsatellite loci (see [37] and [38] for details of the loci used). We assigned genetic parentage with 99% confidence using Cervus v. 3.0 [39].

(d). Adult and litter sex ratios

To determine whether the adult sex ratio at birth was predictive of what the sex ratio would be when offspring begin to breed, we tested for temporal autocorrelation in adult sex ratio across years [40]. Approximately 65% of breeding females reproduce for the first time as yearlings [15], so for adult sex ratio to be a reliable cue there must be a significant lag in temporal autocorrelation of 1 year minimum. We performed autocorrelation analysis of annual population adult sex ratio with lag time in years using the ‘acf’ function from the package stats included in base R (v. 3.3.2; [41]).

(e). Sex-specific reproductive success

To investigate whether squirrels show sex-specific variance in reproductive success, we examined the number of offspring produced by each sex between 2003 (the year in which paternity testing commenced) and 2010 (the last birth year of candidate squirrels who were deceased by year-end 2014). We investigated skewness and kurtosis for the frequency distributions of number of offspring for both sexes using the ‘moments’ package (v. 0.14; [42]) in R.

(f). Factors influencing litter sex ratio

We investigated environmental and maternal factors known to influence litter sex ratio in other systems to confirm that squirrels are an appropriate system in which to test Fisher's principle. We analysed the full dataset covering 26 years (1990–2015) to develop a suite of candidate models. We selected models using Akaike's information criterion [43]. We used a generalized linear mixed-effects model with a binomial error distribution (logit link, models fitted with Laplace approximation) using the lme4 package (v. 1.1–17; [44]) with litter sex ratio as the response variable (number of males and females per litter bound as a single object). We found two pairs of correlated independent variables and managed them as follows. Litter number (corresponding to the order in which a given litter was born to a mother in a year if she had multiple litters within that year) was positively correlated with litter size (r = 0.196, p < 0.001) and parturition date (Z-score within year; r = 0.503, p < 0.001), and was negatively correlated with spring density (r = −0.133, p < 0.001). As multiple litters are uncommon outside of mast years, and litter number and parturition are necessarily correlated because of the chronological nature of multiple litters in a year, parturition date, litter size and spring density were included and litter number was not considered further. Spring density was correlated with spruce cone abundance in the previous year (r = 0.683, p < 0.001) and candidate models were thus run with one or the other. We accounted for the repeated sampling of the same dams across years and within years, and multiple dams within a year, by including dam ID and year as random effects. Fixed effects at the annual level included grid, adult sex ratio and spring adult population density. Fixed effects at the litter level included litter size, parturition date (Z-score within a year) and maternal age. We fitted age of mother as both a linear and a quadratic effect because of evidence of maternal senescence in this population [15,20,45]. The null model was run without fixed or random effects in a generalized linear model with litter sex ratio as the response variable to represent random Mendelian sex chromosome assortment.

(g). Fitness consequences of litter sex ratio variation

We used the total number of grandoffspring as our fitness metric. Because squirrels have overlapping generations and individuals can breed in multiple years, we analysed a number of grandoffspring on an individual basis rather than selecting cohorts. To count the number of grandoffspring attributed to a female squirrel (F₀ generation, or granddam), we first defined the F₁ generation as individuals who satisfied the criteria detailed below. We then defined the F₀ generation as the parents of F₁ individuals, and subsequently defined the F₂ generation as the offspring produced by the F₁ individuals. The inclusion criteria for the F₁ generation were that individuals were: (i) born in years in which paternity had been determined (2003 onward), and (ii) no longer alive, so that lifetime number of offspring for each squirrel would be complete (last F₁ birth year was thus 2010). This yielded 1879 squirrels in the F₁ generation. The number of offspring for which we could not assign paternity (735) was greater than the number for which we could not assign maternity (364); therefore, we further increased the stringency of F₁ inclusion criteria to include only F₁ and F₂ squirrels who had both dam and sire assigned genetically. This ensured all grandoffspring would be attributed to F₀ granddams. This yielded a final total of 1658 unique F₁ individuals born to 284 unique F₀ granddams. When we had DNA samples for both offspring and parent, genotyping accuracy was high (99% of genotype calls match the true biological genotype; D. W. Coltman 2017, unpublished data). Therefore, we assumed missing paternity assignments are most probably owing to pups being sired by males that live off-grid, and less frequently owing to missing tissue from the pup.

To determine whether a female biasing her litter sex ratio towards the rare sex has more grandoffspring, we gave each litter a score to indicate the degree of deviation from the adult sex ratio on that grid within the year. If the adult sex ratio was greater than 0.5 (i.e. male biased), we subtracted litter sex ratio from adult sex ratio. If adult sex ratio was less than 0.5 (i.e. female-biased), we subtracted adult sex ratio from litter sex ratio. This yielded positive values when the litter was biased towards the common sex in the adult population, and negative values when biased towards the rare sex. We averaged each granddam's litter bias scores accrued over her lifetime (arithmetic mean) to yield her lifetime mean litter bias score. We ran a Poisson regression using the ‘pscl’ package (v. 1.5.2; [46]) to determine whether the number of grandoffspring attributed to a granddam was associated with her litter bias score.

3. Results

(a). Adult and litter sex ratio

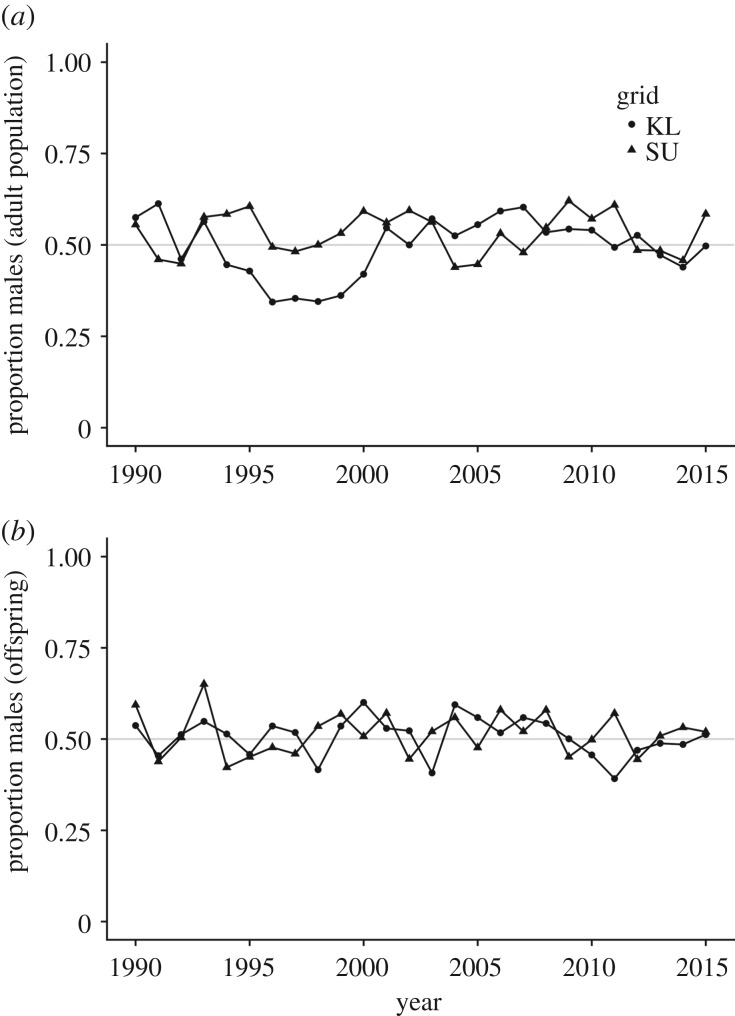
Over 26 years (1990–2015), we recorded 3533 squirrels owning territories: 1749 (49.5%) females and 1784 males (50.5%). Overall, the adult sex ratio did not differ from a 1 : 1 ratio (two-tailed binomial test; p = 0.57); however, we observed annual variation in adult sex ratio (figure 1a). During this time, we counted 5431 juveniles in 1728 litters, which ranged in size from one to seven offspring per litter (mean: 3.14 ± 0.02) with 94.4% of litters comprising between two and five offspring. Of these pups, overall survival between birth and tagging (at approx. one month of age) was 24.14%, and did not differ between the sexes. While there was variation in the litter sex ratios (figure 2b), males made up 51.1% (2776) of offspring, a proportion that did not differ from parity (two-tailed binomial test; p = 0.51; electronic supplementary material, figure S1).

Figure 1.

Annual sex ratios of North American red squirrels expressed as the proportion of males on each study grid (1990–2015) for (a) the adult population and (b) offspring born each year. Adult sex ratio on grid KL was significantly female-biased from 1996 to 2000 and significantly male-biased from 2003 to 2010. Grey horizontal lines indicate sex parity.

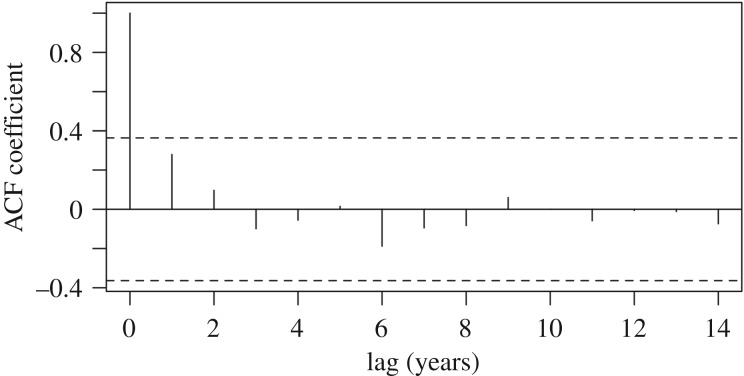
Figure 2.

The ACF plot for North American red squirrel adult sex ratio does not show evidence of temporal autocorrelation across years. Dashed lines indicate the 95% confidence interval. The significant ACF coefficient at a lag time of 0 years corresponds to a perfect correlation of adult sex ratio values against themselves within the same year.

Between the years 1996–2000, the adult sex ratio of one grid (KL) was significantly female-biased (287 unique female census entries out of 454 entries; proportion male 0.37, p < 0.001). Given this opportunity to investigate whether litter sex ratios would be male-biased in a female-biased environment, we looked at the 772 juveniles born in 264 litters on this grid. Males accounted for 394 of these pups (proportion male 0.49), which was not different from parity (p = 0.59). Conversely, from 2003 to 2010, the adult sex ratio on this grid was male-biased (231 males census entries of 410 entries, proportion male 0.56; p = 0.01); and still the litter sex ratio did not differ significantly from parity (414 males of 813 pups; proportion male 49.1; p = 0.62).

Adult sex ratio did not show evidence of temporal autocorrelation for any number of years of lag time when plotted using the autocorrelation function (ACF; figure 3). The highest level of autocorrelation was with a 1 year lag (0.279), however even this value was within the 95% confidence intervals.

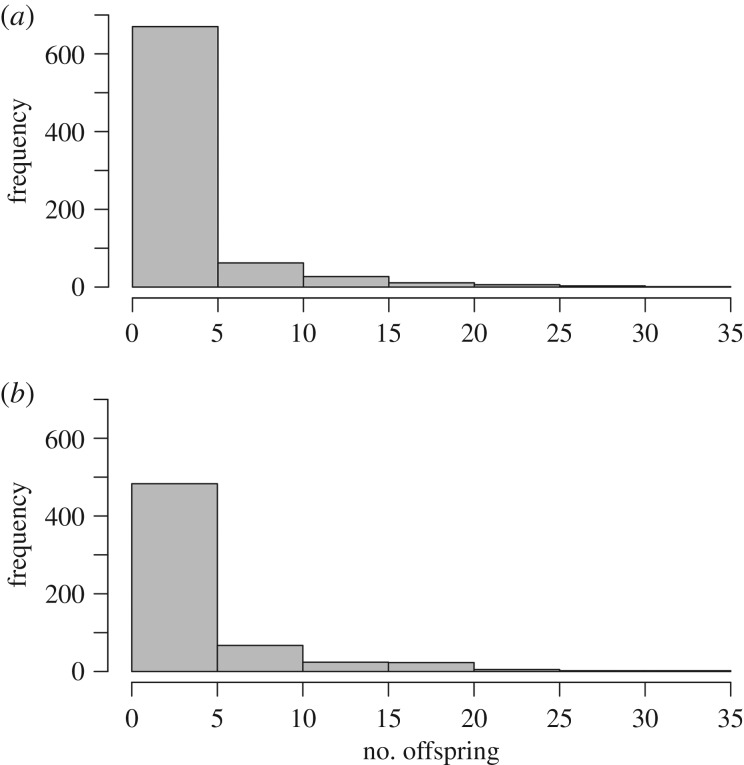
Figure 3.

Frequency distribution of lifetime reproductive output of (a) male (n = 780) and (b) female (n = 606) North American red squirrels prior to excluding individuals based on missing paternity or maternity assignments.

(b). Sex-specific reproductive success of F₁ squirrels

Lifetime reproductive success of F₁ squirrels as measured by total number of F₂ offspring produced (prior to filtering out individuals missing dam or sire identification) was higher for dams (median = 0, mean = 2.95 ± 0.22) than sires (median = 0, mean = 2.09 ± 0.17; Kruskal–Wallis rank-sum test, p = 0.03). While lifetime reproductive output is right skewed for both males and females, the skewness and kurtosis of the frequency distributions of reproductive output are higher for males than females (male lifetime reproductive output skewness = 3.15, kurtosis = 14.61; female skewness = 2.31, kurtosis = 8.89; figure 3).

(c). Factors affecting litter sex ratio

The best fitting model for predicting litter sex ratio was the null model representing random Mendelian chromosomal segregation as determined by lowest Akaike information criterion (table 1). Exclusion of interaction terms and/or addition of a quadratic maternal age effect did not change the results.

Table 1.

Results of the model selection for factors influencing litter sex ratios in North American red squirrels. (All models were run with individual litter sex ratio as the response variable (number of males and females per litter bound as a single object). Fixed and random effects fitted in each model are listed below the model name. Only first-order models are shown. K indicates the number of parameters estimated in each model; Akaike's information criterion adjusted for small sample size (AICc), ΔAICc denotes the difference in AICc values from the top-ranked model; and weight denotes the weight of the focal model among all models presented.)

model	K	AICc	ΔAICc	weight
null ∼ (1 | year) + (1 | dam ID)	3	4383.17	0.00	0.26
grid ∼ + grid + (1 | year) + (1 | dam ID)	4	4383.71	0.53	0.20
spring density ∼ + spring density + (1 | year) + (1 | dam ID)	4	4384.39	1.22	0.14
adult sex ratio ∼ + adult sex ratio + (1 | year) + (1 | dam ID)	4	4384.79	1.62	0.12
food ∼ + cone abundance + (1 | year) + (1 | dam ID)	4	4385.05	1.88	0.10
maternal age (quadratic) ∼ + maternal age2 + (1 | year) + (1 | dam ID)	4	4385.11	1.94	0.10
litter ∼ + parturition date (Z-score) + litter size + grid + (1 | year) + (1 | dam ID)	6	4386.97	3.80	0.04
environment ∼ + spring density + adult sex ratio + cone abundance + (1 | year) + (1 | dam ID)	6	4387.77	4.60	0.03
mother ∼ + maternal age2 + parturition date + cone abundance + (1 | year) + (1 | dam ID)	7	4390.65	7.48	0.01
full model ∼ + adult sex ratio + maternal age2 + spring density + parturition date + litter size + cone abundance + grid + (1 | year) + (1 | dam ID)	10	4394.17	10.99	0.00

(d). Number of grandoffspring

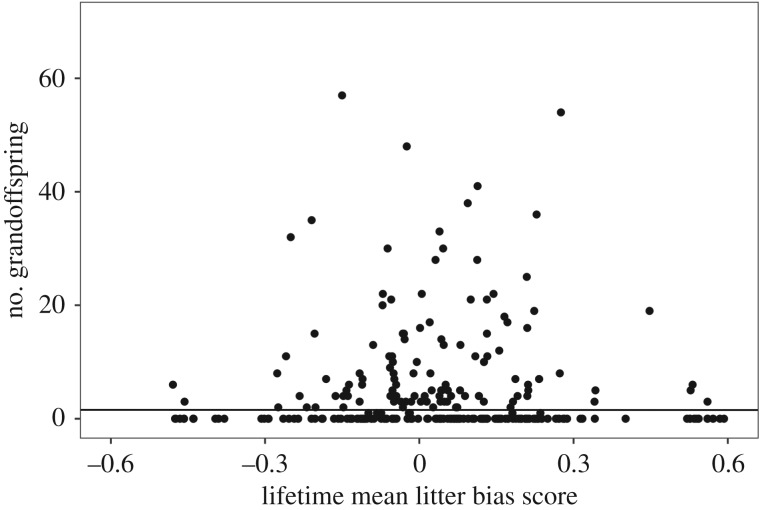
Litter bias score was not a significant predictor of the number of grandoffspring (p = 0.85, figure 4). While number of grandoffspring was positively correlated with lifespan (p < 0.001), controlling for maternal lifespan did not change the nonsignificant relationship between number of grandoffspring and lifetime mean litter bias score (data not shown).

Figure 4.

Number of grandoffspring attributed to female North American red squirrels (n = 284) as a function of the female's average litter bias score over her lifetime. Litter bias scores were calculated as the difference between the adult sex ratio (proportion male) within a given year and the sex ratio (proportion male) of an individual litter. The difference was assigned a positive value if the litter sex ratio was biased towards the rare sex in the adult population, and assigned a negative value if biased towards the common sex.

4. Discussion

As predicted, the overall adult sex ratio in our red squirrel population across 26 years was 1 : 1. Despite this overall parity, we did find transient sex ratio biases in the adult population. When these inequalities exist in the adult population, Fisher's principle predicts that the individuals who produce offspring of the rare sex will experience increased fitness until the ratio is in equilibrium again [3]. Our results did not support this prediction when quantifying increased fitness as the number of grandoffspring produced by mothers who had litters biased towards the rare sex. Adult sex ratios are not temporally autocorrelated across years, rendering the adult population sex ratio in the season of the birth of F₁ individuals not predictive of the population the F₁ individuals will encounter by the time they begin to breed.

The absence of an observable relationship between biased litter sex ratios and number of grandoffspring was at first surprising. However, this effect is explained by a lack of temporal autocorrelation in adult sex ratio, and this absence of a predictive pattern may be driven by environmental factors. Selective pressures acting on squirrels in this region can vary year-to-year as a result of the pulsed nature of food abundance in this system [14,20,23,30,31,47], leading to variation in population density, territory size, and cohort effects in reproductive success [14,20,48,49]. Any incremental fitness benefit that may have been achieved by biasing litters towards the rare sex during the two time periods (1996–2000 and 2003–2010) in which adult sex ratio was significantly non-equal may have been lost over the course of F₁ lifespans as a result of any of these factors influencing squirrel survival, recruitment or reproductive success.

We speculate that, while our populations are nearly closed (owing to the territorial nature of squirrels and because our grids are placed on the highest quality squirrel habitat in the surrounding area), some non-permanent movement during the mating season may contribute to lower success in assignment of paternity than maternity. Male travel distance is correlated with reproductive output [27], so the present analysis may not have captured some highly successful sires that may have immigrated into our study area. This should not affect the conclusions drawn here, as we included only F₁ and F₂ squirrels for which we could ascertain both dam and sire, and restricted analysis of grandoffspring to F₀ females only. Ultimately, given the very low slope and high variation in the relationship between lifetime litter bias and number of grandoffspring produced, this would probably not alter the count of grandoffspring significantly. The high inter-annual variation in food abundance and population density is likely to exert a larger effect, perhaps through variation across the sexes in allocation of resources towards survival versus reproduction (resource elasticity) [50].

The opportunity to test whether litter sex ratios were biased towards the rare sex in response to a biased adult population arose naturally within one grid (KL). A female-biased adult sex ratio failed to yield a male-biased litter sex ratio, and the absence of an effect held in a male-biased environment on the same grid some years later. While squirrels can adjust reproductive output based on perceived increases in population density (experimentally achieved by acoustic playbacks; [23]), it has not yet been shown that squirrels can perceive population sex ratio. Regardless, the lack of temporal autocorrelation in adult sex ratio indicates that adult sex ratio is not a reliable cue to for predicting the sex ratio environment that a juvenile will subsequently experience during adulthood. It is likely that although persistent for several years, this male bias is simply transient [51] when one considers the temporal scale that may be required to observe adaptive litter sex ratio adjustment [52].

While we did not directly measure female body condition at conception [13,53], our investigation of other indices of environmental and maternal condition yielded no significant predictors of litter sex ratio. Rather, the model that best explained the observed litter sex ratios was the null model representing random Mendelian assortment. One of the primary assumptions regarding adaptive sex ratio adjustment is that environmental or maternal traits affect the condition of offspring at the end of rearing, and that this variation in condition is maintained into adulthood [7]. It is plausible that the lack of effects of environment and maternal traits on sex ratios in the present study occurs because conditions experienced prior to weaning have little or no effect on male reproductive skew later in life, though cohort effects have been observed in females in this population [49]. If within-year variability in maternal or environmental quality were important in squirrels, as it is in laboratory mice [54], we would have expected an effect of reproductive timing as represented by parturition date on litter sex ratio [30,31]. Physiological mechanisms for facultative litter sex ratio adjustment have been identified in some mammalian species [55], yet whether such mechanisms have evolved in squirrels is unknown. The lack of sex differences in pup survival, dispersal distance, adult morphometrics and number of mates suggest that one sex is not more costly to produce than the other. The potential for paternal effects to influence sex ratios as demonstrated in other monomorphic mammals such as white-footed mice (Peromyscus leucopus) [56] remains to be explored in squirrels.

Progress in understanding the conditions under which sex ratio adjustment evolves also requires data from systems in which sex ratio adjustment is not observed (e.g. [57,58]). Because Fisher's predictions are frequency-dependent rather than condition-dependent, animals such as squirrels allow us to explore other conditions under which sex ratios may evolve. Although we did not find support for increased fitness of females who biased their litter sex ratio, we suggest environmental variability leading to temporally variable adult sex ratios are likely to obscure any adaptive benefit of adjusting sex ratios. Studying fitness returns in a sexually monomorphic animal predicted to support Fisher's principle provides critical insight into the role of ecological processes—particularly high annual variation in food abundance—in the maintenance of Fisher's predicted 1 : 1 sex ratio.

Ethics

All procedures were approved by the University of Alberta Animal Care and Use Committee.

Data accessibility

Data available from the Dryad Digital Repository: http://dx.doi.org/10.5061/dryad.347tv64 [59].

Authors' contributions

A.E.W. performed data and statistical analysis, participated in the design of this study and drafted the manuscript. C.T.W. conceived of the study, participated in the design and helped draft the manuscript. A.G.M. and J.E.L. participated in the design and analyses. All authors assisted in collecting data and provided valuable discussion and contributions to the manuscript. All authors gave final approval for publication.

Competing interests

We declare we have no competing interests

Funding

Data collection were supported by Discovery Grants and Northern Research Supplements from the Natural Sciences and Engineering Research Council of Canada (NSERC) grants to A.G.M. (RGPIN 371579-2009, RGPNS 377988-2009), S.B. (RGPIN 05874-2014), M.M.H. (RGPIN 262015-2013) and D.W.C. (RGPIN 312207-2011). While writing, J.E.L. was supported by NSERC Discovery Grant RGPIN 04093-2014 and Northern Research Supplement RGPNS 459038-2014. A.E.W. was supported by a Dean's Scholarship (University of Saskatchewan). This is paper no. 92 of the Kluane Red Squirrel Project.