Harris explores the development of our current understanding of electrical coupling between cells and the channels that mediate it, highlighting the contributions of the Journal of General Physiology.
Abstract
As the physiology of synapses began to be explored in the 1950s, it became clear that electrical communication between neurons could not always be explained by chemical transmission. Instead, careful studies pointed to a direct intercellular pathway of current flow and to the anatomical structure that was (eventually) called the gap junction. The mechanism of intercellular current flow was simple compared with chemical transmission, but the consequences of electrical signaling in excitable tissues were not. With the recognition that channels were a means of passive ion movement across membranes, the character and behavior of gap junction channels came under scrutiny. It became evident that these gated channels mediated intercellular transfer of small molecules as well as atomic ions, thereby mediating chemical, as well as electrical, signaling. Members of the responsible protein family in vertebrates—connexins—were cloned and their channels studied by many of the increasingly biophysical techniques that were being applied to other channels. As described here, much of the evolution of the field, from electrical coupling to channel structure–function, has appeared in the pages of the Journal of General Physiology.
Introduction
The mission of the Journal of General Physiology (JGP), as thoughtfully reviewed in previous articles in this series, is to publish research that explores the “basic biological, chemical, or physical mechanisms of broad physiological significance.” Essential to this definition is the meaning of “physiological.” The Physiological Society website cites the Oxford dictionary definition of physiology as “the science of the functions of living organisms and their parts.” This is a forgivable paraphrase of the definition in the actual Oxford English Dictionary (OED), which is “the branch of science that deals with the normal functioning of living organisms and their systems and organs.”
Additional insight may be gained by noting the earlier definition of physiology, designated by the OED as obsolete, as “natural philosophy, natural science.” The OED provides a chronological set of examples of published uses of the term in this “obsolete” sense. The most recent is from an article in Science in 1881 that critically examined the research on Torpedo electric organs to inform the contemporaneous controversy between Volta’s “physical” electricity and so-called Galvanic “natural electricity,” arising from living organisms. In that example, the quoted use of “physiology” is: “In order to obtain a thorough comprehension of the electric organs and their action, it is necessary to have recourse to a third science, experimental physiology, to unite anatomy with natural philosophy, and thus make the result of one answer for the other” (Lanza, 1881).1
The quoted sentence makes an essential point regarding the underpinning of JGP. Natural philosophy is the philosophical study of nature—in other words, theory and conceptualization—and “anatomy” can be updated to refer to the physical manifestations of nature, or in the context of the time, aspects of nature that can be seen and/or measured. Thus, the “third science,” physiology, seeks to unite and demand resolution between physical reality and theory.
In this light, the special mission of JGP is to explore the basics of both the theory and the reality of living organisms and to demand that they coincide, or at least not contradict. By this definition, physiology and JGP are never purely descriptive of either theory or biology.
This article emphasizes the contributions of JGP to understanding the physiology of gap junctions, the signaling they mediate, and the molecular structures responsible in vertebrates (connexin channels). Obviously, much seminal work in these areas was published in other journals. In many instances, a key finding was initially published elsewhere and afterward explored in a more detailed, analytic, and physiologically relevant manner in JGP. This article refers to first reports of seminal findings published in other journals but will focus mainly on the contributions and development of the field in the pages of JGP. As such, it is easy to fail to mention, intentionally or not, key contributions published in other journals, for which I apologize in advance. Particularly for the earlier work, verbatim quotations of key points from the articles are included to convey the perspective of the time.
The trajectory of the study of what are now known as “gap junctions” and “connexin channels” in JGP, like that of other topics, evolved in ways unlikely to have been predicted from the early work. The early studies of electrical coupling were inseparable from early studies of neuronal activity and synaptic function, and often were performed by the same investigators. Of necessity, this is a somewhat selective review; broader reviews of gap junction biophysics, physiology, biochemistry, cell biology, and relation to human pathology are available elsewhere (physiology, biophysics, cell biology: Harris, 2001; Bennett and Zukin, 2004; Bukauskas and Verselis, 2004; Harris and Contreras, 2014; Solan and Lampe, 2014; Miller and Pereda, 2017; Bargiello et al., 2018; connexins and disease: García et al., 2016; Belousov et al., 2017; Delmar et al., 2018; Laird and Lampe, 2018; Srinivas et al., 2018). Scholarly works on the role of electrical coupling in the philosophy of nature, particularly as applied to the nervous system, provide insight and perspective (Bennett, 1985, 1997, 2000, 2002).
A review of JGP publications in this area reveals three major categories of investigation: (1) gap junctions as mediators of intercellular electrical signaling; (2) gap junctions and connexin channels as mediators of molecular permeability and signaling; and (3) the structure–function of connexin channel gating, modulation, and permeability. Early studies of electrical signaling and intercellular molecular transfer involve invertebrates as well as vertebrates. These are considered together, without distinction, in the sections dealing with the physiology of intercellular signaling, since the key physiological properties are similar. However, in discussion of structure–function aspects, the focus is on channels formed by connexin protein, which is the only protein that forms gap junctions in vertebrates. Connexins are not found in invertebrates, in which gap junctions are formed by innexin proteins, which have no sequence similarity to connexins, and therefore the specifics of structure–function of gating and permeability necessarily differ.
Pre-JGP molecular and electrical coupling
Direct intercellular current flow was first explicitly proposed by Engelmann in the 1870s on the basis of patterns of excitation in heart tissue following targeted lesions (Engelmann, 1875, 1877). The author stated, “All facts are irresistible to the idea that the excitation process proceeds directly from cell to cell, without mediation of special anatomical elements;” and “muscle cells are by contact physiologically held conductively connected with each other.” These initial observations of intercellular electrical coupling apparently went unnoticed, as neither article is found in the Web of Science citation databases. The 1875 article stresses that the tissue is not an actual syncytium, but only a functional one when healthy; based on the observed patterns of cell death after tissue injury, the article stated, “In consideration of life and death in the collective animal organism one can summarize a fundamental relationship in brief: Cells live together but die individually.”
The first experimental evidence for a direct intercellular pathway permeable to molecules came in 1925 as a minor part of the habilitation dissertation of Martha Schmidtmann at the University of Leipzig that investigated differences in intracellular pH in cells and organs using neutral red and bromophenol blue as colorimetric pH reporters (Schmidtmann, 1925). Placement of these dyes inside one cell in an epithelium was followed by rapid spread to other cells. The author noted, “While we are accustomed to regard the cell as a self-contained unit from the histological point of view, the experiment shows us that for certain physiological functions such a separation of the cells does not exist…" and "this rapid distribution of staining over larger cell complexes raises some concern about the cell membrane being an ever-present envelope surrounding the cell on all sides.” This paper also reports that the efficacy of dye coupling (“Farbstoffübergang”) was different in different issues. This first report of dye coupling seems to have been ignored as well.
The first firm experimental evidence for direct intercellular current flow was obtained in the early 1950s in studies of action potential propagation in ox heart (Curtis and Travis, 1951). The authors stated, “All the facts presented here indicate clearly that there is a functional protoplasmic continuity between adjacent Purkinje cells, both longitudinally and transversely. One can only conclude then that the cell membrane which appears microscopically to separate adjacent cells is merely a vestige of a true cell membrane.” However, presciently, they went on to say, “A functional protoplasmic continuity need not mean a free exchange of all substances between cells, but merely a relatively low electrical resistance of the membranes at the point of contact between cells.” The next year, Weidmann (1952) showed that the electrical length constant of Purkinje fibers was greater than that of the individual fibers, demonstrating electrical continuity between them.
In 1957, Furshpan and Potter (1957) published in Nature what they later referred to as a “preliminary account” of a rectifying intercellular electrical connection at the giant motor synapse of the crayfish. Though indeed a brief report, it made clear that the synaptic signaling they found could not be adequately explained by chemical transmission.
In 1958, Watanabe (1958) published what is believed to be the first rigorously demonstrated instance of direct (nonrectifying) electrotonic coupling between neurons. This paper explored the basis of a slow oscillatory potential in the lobster cardiac ganglion that leads to periodic bursting. A series of elegant studies showed clear cell-to-cell propagation of depolarizing and hyperpolarizing potentials between the “large/follower” cells (Fig. 1). The experiments included multiple controls to eliminate possibilities other than intercellular spread of electrical current. “The foregoing results indicate that the potential change observed in the second cell is an electrotonic spread from the first cell,” the authors wrote. “This conclusion suggests the presence of protoplasmic connection among the large cells of the present ganglion.” This work foreshadows one of the themes of electrotonic coupling in neuronal circuits, which is that it can act as a low-pass filter of electrical events (in this paper, the junctions passed slowly changing currents with fidelity but only occasionally were able to transmit fast events such as action potentials), a property largely a function of the time constant of the cell receiving the current. In this system, the slow potentials in the coupled cells arise from coincident chemical synaptic inputs from “small/pacemaker” cells. The author considered whether the pacemaker cells were also electrically coupled to the follower cells. He could not prove this, but provided indirect evidence for it (this coupling would be demonstrated by Watanabe and Bullock 2 years later in JGP; below).
Figure 1.
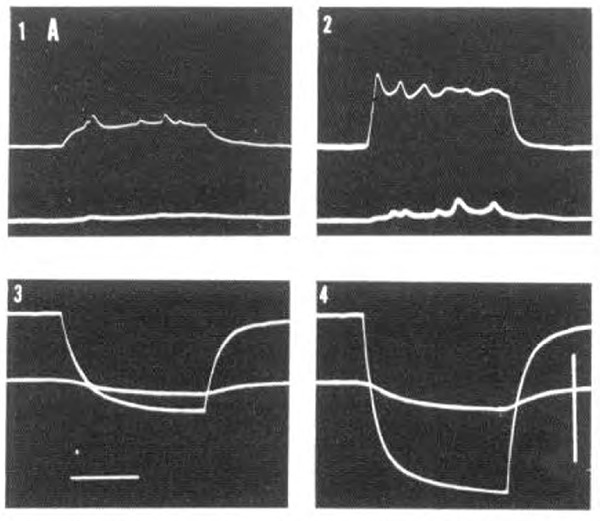
Initial recordings of electrical transmission between coupled cells of the lobster cardiac ganglion. In each panel, polarizing current is applied to the cell whose voltage is shown in the upper trace. The voltage in the cell coupled to it is the lower trace. The upper panels show the effect of depolarizing current, and the lower panels, the effect of hyperpolarizing current. Voltage calibration, 50 mV; time calibration, 25 ms (from Hagiwara et al. [1959]). Fig. 1 is reprinted with permission from the Journal of Neurophysiology.
The coupling between the follower cells was further explored in a subsequent paper that implemented the first use of voltage-clamp to study electrical coupling (Hagiwara et al., 1959). The frequency dependence of the coupling between the cells was explicitly measured and accurately modeled. The coupling resistance was framed in cellular terms: “The observed protoplasmic resistance of the [intercellular] route corresponds to a fiber diameter of 1–3 µm when the intercellular route is assumed to be a single fiber which runs a linear course between the cells and has a specific resistance of 100 Ω-cm.” This paper also presented, for the first time, I believe, the equation for coupling coefficient (V2/V1, where V1 is the voltage in the “presynaptic” cell and V2 the voltage in the cell coupled to it), expressed as a function of the junctional resistance (Ri), the resistance and capacitance of the nonjunctional membrane of the coupled cell (Rm and Cm, respectively), and time (t) (Fig. 2). This formulation made explicit and quantitative that the degree of coupling depends on the electrical properties of the coupled cell, in addition to the resistance of the coupling pathway itself, and that coupling may be modulated by changes in either parameter.
Figure 2.
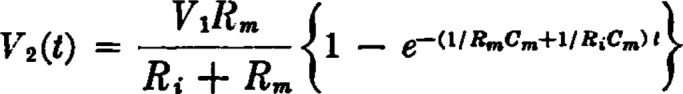
Expression describing the potential in a cell (V2) as a function of the clamp voltage in the cell coupled to it (V1). The relation between V1 and V2 is a function of junctional and nonjunctional membrane properties. See text for definition of parameters (from Hagiwara et al. [1959]). Fig. 2 is reprinted with permission from the Journal of Neurophysiology.
This paper was submitted to the Journal of Neurophysiology in September of 1958 but was not published until a year later, in the September 1959 issue. In the interim, two papers were published extensively characterizing rectifying electrical coupling and its effects on action potential transmission.
The lesser known was that of Arvanitaki and Chalazonitis (1959) showing rectifying coupling between neighboring somata in the pleurobranchial ganglion of Aplysia. This report followed up their earlier observation in the same system that showed, by paired intracellular recordings, that spontaneous activity in one neuronal soma led to simultaneous depolarizations or action potentials in a neighboring one (Chalazonitis and Arvanitaki-Chalazonitis, 1958).
The 1959 paper performed extensive analyses of the transmission of potentials and in particular the effects of different “presynaptic” stimuli on initiation of action potentials in the coupled cell, and the “synergique” versus “antergique” effects of different spiking patterns in the coupled cells. Particular note was made that the delay between the prejunctional spike and the postjunctional activity was not assignable to a delay in the process of transmission itself, but rather to the delay in the growth of the postsynaptic response (i.e., the charging of the postsynaptic membrane). No threshold for electrical transmission was found. A role for electrical coupling in synchronization of electrical activity in pathological conditions such as epilepsy was suggested. Interestingly, the paper makes a distinction between a “synaptic” connection explicitly analogous to that described by Furshpan and Potter (1957) and a “simple ephapse,” here apparently referring to transmission caused by close membrane apposition only. As in the earlier Furshpan and Potter report, action potentials propagated in one direction only, but hyperpolarizations could transmit in the opposite direction.
The more widely known Furshpan and Potter paper (Furshpan and Potter, 1959) came out at virtually the same time, with a detailed characterization of electrotonic coupling at the giant motor synapse of the crayfish, clearly showing rectifying intercellular current spread, in which current flowed in one direction across the intercellular junction and not the other. In addition to evoking puzzlement (e.g., “How does one make an instantaneous current rectifier from cellular materials?”), this paper showed that electrical coupling might be mediated by something other than simple holes in membranes, or rather, that the holes could have interesting properties.
None of this work was published in JGP. That would soon change, but first…
Matching function to structure
Understanding intercellular communication by gap junctions co-evolved in both the physiological and anatomical/cell biological worlds; the gap junction is defined by anatomy, and the ionic and molecular coupling it mediates is a physiological phenomenon. As a result, much of the work to identify the junctional structures that mediate electrical coupling was published in the Journal of Cell Biology (JCB), a sister journal of JGP at Rockefeller University Press. These structural and biochemical studies, and some functional studies, were published in JCB over the same period that JGP was publishing the fundamental studies of electrotonic coupling. Identification of the structures that became known as gap junctions as sites of electrical coupling enabled their purification and biochemical analysis (also largely published in JCB) and eventually to cloning of connexins.
As noted above, by the mid-nineteenth century, it was widely accepted that the heart was an electrical syncytium as an explanation for the rapid spread of excitation throughout the tissue, well beyond where nerve fibers could be seen. Light microscopy showed intercalated disk structures, which were presumed to contain a region in which the apposed cell membranes were not intact, allowing intercellular flow of current.
Perhaps the first correlation between intercellular current flow and morphology in neurons was in the giant nerve fibers of the crayfish and earthworm. It was known that excitation flowed rapidly and apparently unimpeded down the fibers, but in 1924 and 1926, it was firmly established that the axons were segmental in structure, with clear membranous septa interrupting what had been considered continuous cytoplasm (Johnson, 1924; Stough, 1926). These studies demonstrated the separateness of the segments bounded by septa, showing that degeneration after injury did cross not segmental boundaries, taken as evidence against fusion of adjacent segments. The lipidic nature of the septa was inferred from osmic acid staining. The giant fibers were initially viewed as being able to conduct action potentials in only one direction, but later work by Bullock (1945) showed that in fact the electrical communication across the septa was not rectifying, making two insightful observations about their functional properties: “…recent speculation that many of the apparently fundamental physiological properties of synapses, properties common used in the definition of this entity, are really not inherent in the nature of the junction,” and “the physiological evidence is quite definite that these fibers, which appear to be chains of compound axons separated by macrosynapses, are unpolarized, not only experimentally but in normal functioning. Furthermore, there must be no significant synaptic delay…” (Bullock, 1945). These observations laid the groundwork for first detailed studies of electrotonic coupling in this system (by Dewey and colleagues) and their function (Brink and colleagues), discussed later below.
Identification of the structural substrate of electrical and/or dye coupling between cells was made possible by EM, which provided the resolution and sample preparation techniques required to identify and distinguish among different types of intercellular junctions. The first reports got some things right and some things wrong. In a brief report in 1954, followed by a more extensive report in 1958 (Sjöstrand and Andersson, 1954; Sjöstrand et al., 1958), Sjöstrand and colleagues showed in EM studies that the plasma membranes were intact at the intercalated disk: “The plasma membrane at the cell junction appears structurally organized in a similar way as the plasma membrane covering the rest of the cell surface.” Also, “cardiac muscle tissue is subdivided into units representing cell territories without any anastomoses” (Sjöstrand and Andersson, 1954; Sjöstrand et al., 1958). Moreover, they found a space of constant width separating the membranes (70 Å in some animals and 150 Å in others). The only specializations noted were densely osmiophilic zones, likely desmosomes. It was noted that there were filamentous lines crossing the intermembrane space in these so-called S-regions. To reconcile these findings with syncytial spread of excitation, two proposals were made: “A rather free, unsupported speculation is that the S-regions would represent areas with a lower ohmic resistance and would form paths with a greater safety factor for conduction across the cell junction.” An alternative was that, based on a report of metabolic enzymes (succinic hydrolase and alkaline phosphatase) localized at the disk (Bourne, 1953), “considerable metabolic activity…might act as boosters of the contraction wave.” The clear absence of fused plasma membranes in this electrically coupled system was not noted in several subsequent papers on the subject.
A few years later, Dewey and Barr (1962) focused on what they termed the “nexus,” defined as a region of membrane fusion between electrically coupled smooth muscle cells, as the site of electrical coupling. EM images were interpreted to show “a true fusion of the outer lamellae of adjacent cell membranes…[which]…implies a lack of extracellular fluid between the cells.” Such structures were proposed to allow a “direct electrical connection between cell interiors without intervening extracellular space.” The mechanism by which membrane fusion would lead to a low resistance current pathway was not addressed. Soon afterward, Bennett et al. (1963) combined electrophysiology with EM of teleost electromotor neurons to similarly point to regions of membrane fusion as sites of coupling.
In 1963, structures referred to as “synaptic disks” composed of closely packed, hexagonally arranged hexameric and/or pentameric polygons (periodic spacing of 90 Å) were resolved at goldfish Mauthner cell synaptic regions (Robertson, 1963). These structures were obviously distinct from chemical synapses. It was unclear whether the membranes had fused, but the author wrote that “the evidence is against complete fusion.” In retrospect, these were clear gap junctions, with the first images of what we now know are the component intercellular channels. The hexagonal packing, the hexagonal nature of the particles, and the interparticle spacing turned out to be exactly correct.
The first paper in JGP to include EM analysis of gap junctions was an extensive correlative ultrastructural and electrophysiological study of the nexus in frog atrial muscle by Barr et al. (1965). This study used a modified sucrose gap technique (Stämpfli, 1954) to show that propagation between the cells was entirely electrically mediated. In this method, a wide region of nonconducting extracellular medium was imposed along the muscle, blocking action potential propagation across that segment. Action potentials propagated across that segment only when a shunt resistor was placed across it, showing that transmission across the nexal membranes was purely electrical.2 EM of the muscle located the cellular site and structure of the electrical coupling at the nexus. Hyperosmotic solutions caused the nexal membranes to pull apart and the electrical coupling to disappear. The site of the coupling was regarded as a place where “the opposing cell membranes are fused along their outer lamellae.” This article provided an informative overview of the attempts to correlate structure and function of coupling up until that time.
The pivotal conundrum regarding the structure mediating electrical coupling was nicely expressed by Dewey (1965) as two alternatives: “electrical transmission across an intercellular gap” or “electrotonic coupling between…cells across a region of contact between cells.” As was soon discovered, it was both—there is an intercellular gap, and the proteins in the two membranes are in contact with each other.
The same authors (Barr et al., 1968) later contributed an analogous study of coupling and its structural basis in smooth muscle that doubled down on the fusion of the outer lamellae on the basis that in permanganate-fixed EM material, the width of the nexal junction was less than the sum of the two component plasma membranes.
A strong case for specialized EM-identified junctions mediating electrotonic coupling between neurons in a variety of vertebrates was made in 1966 by Pappas and Bennett (1966). These are referred to as “electrotonic junctions” rather than “nexi,” but they are similarly described as sites of fusion of the apposing plasma membranes.3 It was acknowledged that “the nature of the membrane modification that leads to decreased resistivity remains to be determined.” Based on flux of fluorescein across the septa of the crayfish giant axon, the authors suggested that the minimum diameter of the low-resistance pathway between cells was just under ∼10 Å and that the maximum diameter could not be much greater. This turned out to be accurate.
Using a different approach in the same year, Weidmann (1966), in an article with a mathematical Appendix by A.F. Hodgkin, came very close to resolving the issue by showing that intracellular radiolabeled K+ diffused freely in the longitudinal direction between heart cells across intercalated disks. Explicit proof that the pathway was the disk was lacking, but the disks were identified as the only regions of contact between the cells that could provide a low-resistance intercellular pathway. In the description of membrane appositions, the paper refrained from referring to them as fused.
Much was resolved the next year by Revel and Karnovsky (1967) in detailed EM studies of mouse heart and liver. This work cleanly distinguished the junctions thought to mediate electrotonic transmission from other types of “occluding” junctions (e.g., tight junctions and fused membrane structures). Instead of the membranes being fused at these junctions, they are seen be separated by a ∼20-Å “gap.” The hexagonal packing of hexagonal particles reported by Robertson (1963) was seen, with the same center-to-center spacing. Strikingly, in lanthanum-stained material, “in the center of each particle there appeared to be an electron-dense core 10 Å or less in diameter…it would appear that each subunit is a hollow prism some 50 Å tall.” This may be the first image and physical description of the pore of any membrane channel. The paper ends, “A hexagonal pattern [of particle packing] such as described here may prove to be characteristic of some, but not necessarily all, junctions involved in electrical interconnection between cells.” The term “gap junction” first appears in an abstract by the same authors in the same year (Revel et al., 1967).
The definitive structure and characterization of gap junctions were presented by Brightman and Reese (1969) in vertebrate brain and Goodenough and Revel (1970) in mouse liver. These papers provided high-resolution EM images of gap junctions and insightful analysis using various sample preparation and staining techniques. Goodenough and Revel (1970) used the freeze-cleave technique to show the collections of particles (i.e., channels) and corresponding “pits” in the two leaflets of junctional membranes. It summarized the literature correlating observations of electrical coupling with gap junctions: “There is evidence that the gap junction is the site of electrotonic coupling between cells.”
These papers were cited in subsequent work that visualized faint Procion Yellow fluorescence within the junctional regions of the crayfish septate axon after extracellular application of the dye (Payton et al., 1969a). This, together with EM images of lanthanum-stained material that showed a network of hexagonal arrays within the septa, inspired the authors to propose that cell-to-cell channels existed through the center of the hexagons, and that the hexagons themselves were closed tubular structures continuous with extracellular space (i.e., filled with lanthanum). An innovative drawing was made of this scheme (Fig. 3). In retrospect, the hexagonal structures are themselves the channels, and the lanthanum stain surrounds them rather than fills them. In any case, this appears to be the first diagram of the fine structure of junctional channels.
Figure 3.
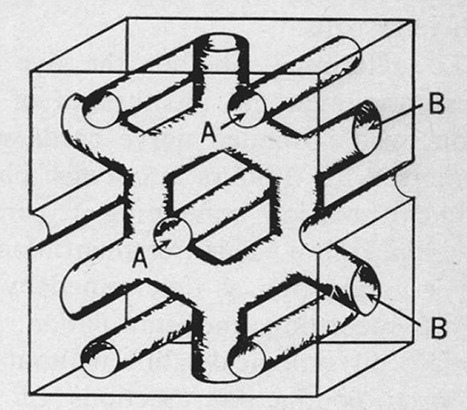
An early drawing of junctional structure derived from lanthanum-stained EM material. “A” indicates the intercellular pathway; “B” indicates continuity with lanthanum-filled extracellular space (from Payton et al. [1969a]). Fig. 3 is reprinted with permission from Science.
Exploration of electrical coupling
In reviewing the early work on electrical coupling in JGP, as for other aspects of physiology, one is struck by the extent to which investigators explored explanations and contingencies to explain the observed phenomena that one would dismiss out of hand today, but at the time were no less unreasonable than the inferences or conclusions to which they eventually came. These explorations are not discussed here, but make interesting reading.
The debut of direct electrical coupling in JGP came in a “Note Added in Proof” in 1959 (Bennett et al., 1959). The paper to which it was appended had pretty much concluded that direct electrical coupling between cells was the most reasonable explanation for the observed and manipulated patterns of excitability among a set of teleost neurons. The note stated, “Recent experiments (Bennett, unpublished) disclose that both hyperpolarization and depolarization of one SMC [supramedullary neuron] may cause polarization in adjacent cells. This potential is of the same sign, but much reduced and slowed, as would be expected from electrotonic conduction. These observations suggest the existence of electrical connections between cells which might be responsible for the synchronization of SMC.”4 The bulk of the work in JGP in the immediately following years grappled with what this direct current pathway meant for spread of excitability and circuit behavior and how it was used to achieve and modulate electrical signal processing. JGP provided a robust forum of exploration of these properties for several decades.
The first paper in JGP to explicitly study electrical coupling set this in motion, describing for the first time how electrical coupling was used in generating desired “circuit-level” neuronal behavior (Watanabe and Bullock, 1960). This paper built directly on the previous work by Watanabe (1958) and Hagiwara et al. (1959) on the relation between pacemaker and follower cells in the lobster cardiac ganglion. It documented and characterized the electrotonic coupling between these two cell types suggested in the previous work and showed how this coupling permitted the slow oscillatory voltage changes in the follower cells to be conducted “antidromically” back to the pacemaker cells, where they modulated the firing pattern. The effect of this reciprocal signaling (chemical transmission from pacemaker to follower, and electrical transmission in the reverse direction) was to effectively synchronize and stabilize the pacemaker firing pattern. It also provided a way for follower cells to modulate their own input from pacemaking cells. A key point was that the voltages of the action potentials of the follower cells did not propagate back to the pacemaker cells because of the low-pass filter characteristic of the electrotonic junctions (and because the follower cell somata were electrically inexcitable). Thus the interactions between follower cells and pacemaker cells ensured stable and oscillatory inputs while preventing the action potentials in the follower cells from affecting those inputs.
This was the first demonstration that “subthreshold cross-talk” between neurons, not mediated by extracellular field effects, could modulate neural activity. Such subthreshold modulatory influences had been proposed, but not demonstrated, by Gerard (1941) and Bullock (1958). The results are summed up: “Some kind of direct, low resistance pathway for electrotonic spread, discriminating against spikes because of their brevity, is inferred, providing a basis for subthreshold interaction which is specific and not by way of a field effect.” Perhaps reflecting the collegial nature of scientific publishing at the time, this paper also contained a “Note Added in Proof” referring not only to the previous paper by Bennett et al. (1959) but also to even more recent work published in abstract form (Bennett, 1960), thereby recognizing the work of colleagues who had gotten a key result into print a bit earlier, though in abbreviated form.
This was followed by a paper in JGP the next year (Watanabe and Grundfest, 1961) studying septal junctions in crayfish giant axons. In this work, extensive electrophysiological studies examined electrotonic spread across the septa and its consequences for spike transmission across them. The paper elaborated several features of the physiology of electrotonic coupling, including differences between transmission of action potentials and of current steps, an apparent synaptic delay, and the effects of polarization across the septa on these properties. The paper extensively tested and validated an equivalent circuit for the system.
In a reflection of the historical context of electrotonic coupling, transmission across the septa in this paper was termed “ephaptic.” The term “ephapse” was first used to describe electrical interactions between neurons mediated by close proximity or contact of the cells, but without the morphology or properties of a chemical synapse (Arvanitaki, 1942).5 It was invented to describe electrical interaction between adjacent axons, first shown by Ewald Hering in 1882 (Hering, 1882), and later by Jasper and Monnier (1938) and Katz and Schmitt (1940).6 At the time, the idea of intercellular current flow between cells was most readily understood as arising from close apposition of low-resistance membranes without any specialized structures. In such a configuration, current flowing out through the membrane on one side of the ephapse divides between extracellular leak, via whatever space there is between the membranes, and entry into the closely apposed, low-resistance membrane of the neighboring cell. Some fraction of the current leaving one cell would enter the other, the fraction depending on the relative resistances of the “leak” and “apposed cell” pathways. Efficient coupling required only that the leak resistance be much greater than the resistance of the path across the apposed membrane and any interposed space. In this view, transmission across fused low-resistance membranes would be highly efficient, as there would be no leak.
At the time, the potential difficulty posed by membrane conductivity requiring discrete protein channels, and how these channels would function in fused membranes, were below the radar. Initially, ephaptic transmission included transmission across “fused” membranes as well as across a narrow intercellular gap, but usage soon became limited to the latter case. In current usage, the ephaptic transmission includes both the effects of current leaving one cell and passing through extracellular space, however narrow, on its way into the other cell, as well as explicit extracellular field effects. Neither occurs at gap junctions, but at the time, in the absence of evidence for specialized structures, electrotonic transmission was considered to be ephaptic in nature. Ephaptic transmission can of course mediate electrical coupling, and can occur on its own or in conjunction with gap junction–mediated electrotonic coupling (see Veeraraghavan et al. [2014]).
Another instance of coupling was explored in JGP by Watanabe and Takeda (1963), who demonstrated high-fidelity electrotonic coupling between the axons of the heart ganglion of the mantis shrimp. Action potentials in one axon always produced action potentials in other axons. They stated, “…the electrical connections among neurons have developed to the extent that the independence of the individual neurons is almost abolished.” These studies localized the site of coupling as axonal–axonal, rather than involving the somata.
In 1966, Ito and Hori (1966) published a study in JGP showing strong electrical coupling between blastomeres of amphibian embryos, starting at the first cleavage, and continuing through the morula stage. They showed that “the resistance of the junctional membrane…formed at the former planes of cleavage, is small in relation to that of the cell surface in contact with the exterior.” The ratio of these resistances accounted for the electrical coupling, because the greater portion of injected current would pass from cell to cell rather than exit to the outside of the embryo (the paper demonstrated that the enveloping chorionic membrane did not contribute significant resistance). In recognizing the limits of the study, the authors concisely articulated a key feature of electrotonic coupling, the importance of the ratio of resistances of junctional and nonjunctional membranes: “The difference in the pattern of electrical coupling, as revealed by the method of measuring voltage attenuation, can reflect differences (a) in resistivity of the junctional membranes; (b) in resistance of the nonjunctional membrane surfaces; and (c) differences in shunting by the intercellular medium.” They could not distinguish among these factors and were implicitly agnostic about whether the current pathway between cells involved flux across a (narrow) extracellular junctional space.
Shortly afterward, Tanaka and Sasaki (1966) presented a mathematical model of 2-D cardiac muscle and compared its predictions for how potentials were distributed longitudinally, transversely, and within muscle cell fibers to experimental measurements. It had long been known that potentials spread throughout cardiac tissue, but quantitative analysis of the electrical properties, and the contribution of the junctions between the cells (intercalated disks) to this process, had been unresolved. A model with predictive value was generated. To make the measurements needed to validate the model, the authors made substantial technical innovations.7 The model and experimental data were presented in sequential sections headed “Theory” and “Evidence,” in what now appears a quaint distinction. Theory and evidence were in agreement, and additionally, “the resistance per unit area of intercalated disc can be calculated to be approximately 500 to 2,000 times lower than that of the fiber membrane.”
In 1966 a seminal paper was published (not in JGP) by Bennett (1966). In addition to providing a comprehensive review of the findings on electrical coupling up to that time, the analysis and principles in the paper essentially defined the physiology of gap junctions and electrotonic coupling. It fully analyzed the equivalent circuits represented by two coupled cells for steady-state and transient presynaptic voltages. For the former, building on the earlier work by Watanabe and Grundfest (1961) in JGP, it presented a rigorous method by which one could analyze electrophysiological data to assess coupling resistance independently of changes in nonjunctional resistances. It described what became known as the “π-t” transform of the equivalent circuit that enabled real-time unambiguous assessment of junctional resistance during current-clamp. This method was used extensively to assess junctional conductance until the application of voltage-clamp techniques in an initial report in 1979 and full reports published in JGP in 1981 (below).
The kinetic analysis revealed several nonintuitive insights about the potential for diversity and plasticity of electrical signal transmission. Solutions were derived for presynaptic potentials the shape of action potentials and for various time constants of the postsynaptic cell, with and without the contribution of junctional membrane capacitance. Among the observations were that for electrical synapses there could be significant “synaptic delay,” delay of postsynaptic time to peak, differences in magnitude of peak, and altered relaxation of the postsynaptic voltage, depending on the time course of the presynaptic potential relative to the time constant of the postsynaptic cell and the coupling coefficient (which, as noted above, is a function of both the junctional resistance and the resistance of the postsynaptic cell). In other words, observing the character of the postsynaptic potentials did not allow one to conclude a priori that the transmission was chemical versus electrical. The paper analyzed the effects of a “leaky” junction (essentially an ephapse) and considered whether the junctional membrane can be excitable, how changes in membrane voltage can alter transmission of action potentials, spatial and temporal summation, and how they relate to initiation of a postsynaptic action potential.8 There is also discussion of the various ways that unidirectional coupling might be generated.
With the exception of an instantaneously rectifying junction (e.g., Furshpan and Potter, 1959), the analysis presumed that the junctional membrane is “electrically inexcitable in the most rigorous sense” and that the coupling resistance is linear, at least during the time frames of action potentials and typical current injections. Yet, the door is left open for other possibilities: “...it should be emphasized that symmetry is not the same as linearity. The actual potentials produced across junctions when impulses are present on only one side are usually considerably larger and briefer than can be tested experimentally.…Thus, linearity remains unproved in a significant time and voltage range, and either increased or decreased resistance might well occur.” This statement was prescient.
Early electrical coupling studies in JGP
In 1963, Roger Eckert explored electrical coupling between giant segmental ganglion cells of the leech, with particular attention to the effects of different degrees of coupling on transmission of action potentials and subthreshold potentials (Eckert, 1963). Other early characterizations and analyses of electrical coupling in JGP include those of Bennett in guinea pig smooth muscle (Bennett, 1967) and Kriebel in tunicate heart (Kriebel, 1967, 1968).
Shortly after the first report of propagating electrical events in epithelia (Mackie, 1965), active conduction through epithelia was explored in JGP (Mackie and Passano, 1968), although the mechanism of intercellular propagation was not attributed to gap junctions until some years later. The subsequent work on gap junction coupling in excitable epithelia reported in JGP almost exclusively concerned hydrozoan coelenterates (Mackie, 1976; Josephson and Schwab, 1979).
Reuss and Finn (1974) performed a detailed study of intercellular and transepithelial current flow pathways in toad urinary bladder epithelium. The epithelial cells were found to be highly coupled, and the resistance of the paracellular pathway to be determined by the tight junctions.
Brink and Barr (1977) conducted a detailed and quantitative analysis of the junctional resistance of the septa in the median giant axon of the earthworm and presented a mathematical model of the axon. The conductance of the septal membrane was found to be three orders of magnitude greater than that of the nonjunctional membrane. Based on an estimated unitary conductance (∼100 pS) and morphometry of the septa and channel spacing, the authors calculated a septal conductance much lower than the septal conductance they measured. They suggested that frictional forces in the pore could account for the difference. In a first “pharmacological” test of junctional conductance, the authors applied TEA to the inside of the axons to see whether the intercellular channels were K+ channels (they found no effect).
Electrical coupling in physiological function in JGP
Each section below describes a role (or proposed role) of electrical coupling in a physiological process and makes a point or two about the properties of gap junctions (in parentheses in the heading).
Invertebrate “swallowing” (modulation of electrical coupling by chemical synapses)
The physiology of electrical coupling and how it interacts with chemical synapses was explored by Levitan et al. (1970). In the buccal ganglion of the opisthobranch mollusk Navanax inermis (a smaller and prettier cousin of Aplysia californica), the low-pass filter characteristic of electrical coupling was demonstrated using sinusoidal current stimuli and by showing that action potentials were more attenuated than postsynaptic potentials or direct current inputs. Of greater interest was demonstration that large cells that were not connected by chemical synapses were electrically coupled to each other, and also to “interneurons” that provided inhibitory feedback to them via chemical synapses. The effect of the former ensured that the large cells fired synchronously only when receiving simultaneous strong inputs, and the latter provided a delayed inhibitory input to terminate the burst. This coordination of chemical and electrotonic influences was proposed to underlie the rapid, brief pharyngeal expansion that allows the animal to swallow prey whole.
The circuitry and the effects of electrotonic coupling in this paper provided the basis for a paradigmatic exemplar of dynamic modulation of electrotonic coupling via chemical synapses (Spira and Bennett, 1972; Spira et al., 1980). Modulation of electrical coupling by chemical synaptic activity is a key component of feeding behavior in Navanax. Efficacy of coupling between motor neurons is controlled by chemical synaptic activity (inhibitory postsynaptic potentials with reversal potential near the resting potential) that increases nonjunctional conductance without significant effect on membrane potential, thereby shunting to extracellular space current that would otherwise cross the gap junctions. Activity of these chemical synapses determines whether the cells tend to fire synchronously or independently. An analogous mechanism is thought to operate in the inferior olive (Llinás et al., 1974) and elsewhere in the central nervous system (Connors, 2017).
Cardiac function (sometimes only a few are needed; interplay between action potentials)
Aside from the early work by Tanaka and Sasaki (1966), JGP published surprisingly few studies on the role of electrical coupling in cardiac conduction, given its prominent role in that process. In 1970, Freygang and Trautwein (1970) performed an extensive analysis of the frequency-dependent impedance of strands of Purkinje fibers and its role in signal transmission. Much of the quantitative analysis was correct, but the proposed capacitative component to longitudinal conduction was later disproven (Levis, 1981; Levis et al., 1983). See also Lieberman et al. (1975).
In 1975, Dehaan and Fozzard (1975) showed that heart cells grown as spherical aggregates were strongly electrically coupled and virtually isopotential, enabling study of their action potentials without the complications caused by the geometrical complexities of the cells in situ. The isopotentiality of the cells in the aggregates, imparted by the gap junctions, enabled the aggregates to be voltage clamped by Nathan and DeHaan (1979) to analyze the currents underlying the action potentials. The voltage control was assessed to have a 12% error in the first several milliseconds of a voltage step pulse and good control thereafter, which precluded detailed study of the role of voltage activated Na+ currents but allowed analysis of slower currents.
This system was used by Clapham et al. (1980) to study the development of electrical coupling between aggregates of cardiac cells and its role in the propagation of action potentials between the aggregates. This may be the first assessment of the time course of development of coupling between cardiac cells. The study detailed correlations among electrical coupling, junctional conductance, and latency of action potentials between the two aggregates. The result: “The simple linear relationship between Rc [coupling resistance] and L [latency of action potentials], with no changes in action potential parameters as coupling progressed, suggests that the junctional resistance had a dominant effect in causing action potential delays across the junction in this preparation.” Of particular interest was the finding that a very low degree of coupling was sufficient to synchronize the beats between the aggregates. Synchronization began when the junctional resistance dropped below 20 times that of the input resistance of the aggregate (corresponding to a coupling coefficient less than 0.1). Using a reasonably accurate estimate of the unitary conductance of a gap junction channel (100 pS) and an estimate of the number of cells in initial contact between the aggregates, it was calculated that only five junctional channels per contacting cell would be sufficient to permit synchrony between two 160-µm-diameter aggregates. Relevant to the dominant paradigm at the time regarding cardiac conduction defects, the authors made the point that although "...the mechanisms that underlie conduction disturbances [to date] have generally been sought in terms of altered excitability or membrane parameters,…we have shown…changes in action potential delay resulting purely from alterations in junctional resistance.”
In 1994, Rohr and Salzberg (1994) explored, using optical recording of membrane voltages, the propagation of action potentials in cultured neonatal rat heart cells across patterned transitions of different character. The idea was to recapitulate the essentials of impulse propagation across a transition from a narrow to a larger region of tissue (i.e., to a larger current sink), as would occur at the Purkinje fiber-to-ventricle junction and other sites in the heart. Multisite optical recording allowed detailed analysis of the shapes of the propagated action potentials on both sides of the transition, and of the potentials seen after the transition when propagation failed. Though electrical coupling was not directly assessed, its effects were evident in the observed physiology. Key findings were that (a) as the region of increased capacitative load was approached, the upstroke of the incoming action potential flattened out as current that would have maintained its rise flowed into the region of greater membrane area through the junctions, and (b) if an action potential was then initiated on the other side of the transition, current from it flowed back toward the incoming action potential to enhance its (compromised) upstroke; there was “bidirectional electrotonic interaction between the segments separated by the narrowing of the strand.” In more mechanistic terms, “the spatiotemporal evolution of the first phase of the upstroke of successfully propagating impulses is entirely determined by the electrotonic interaction between the activated proximal cell strand and the as yet still well-polarized large distal cell area (i.e.,…imposes a polarizing clamp on the transmembrane potential of the region upstream). Conversely, the second phase,…present only during successful propagation, could be explained by the delayed release of this ‘clamp’ during distal activation, which caused a secondary depolarization, supported by the electrotonic interaction in the upstream direction.” This paper demonstrated the reciprocal effects of gap junction coupling on the shapes and magnitudes of potentials and excitability upstream and downstream of a “source-sink” discontinuity caused by tissue geometry or damage.
An interesting and unique investigation of how changes in gap junction coupling affect propagation of cardiac action potentials was published by Verheijck et al. (1998) in which a digital “coupling clamp” provided the intercellular coupling currents to spontaneously active isolated sinoatrial (SA) node cells located and impaled in separate electrophysiology rigs. The digital system monitored the voltage difference between the cells in real time and, for each experimenter-determined “junctional” conductance, calculated the junctional current that would pass between the cells, and deliver it, with opposite sign, to each cell. Various parameters of the action potentials were assessed as functions of the junctional conductance, including their shapes, magnitudes, kinetics, activation delay, and interbeat intervals.
A notable finding was that 1:1 action potential synchronization could occur at very low coupling conductances, corresponding to two to three gap junction channels. This was in line with estimates derived from SA cells coupled by real gap junctions. Below this level of junctional conductance, there was clear evidence of “subthreshold” depolarizations that tended to have a phase-resetting effect on firing. This produced a mutual entrainment of excitability, which increased with increasing junctional conductance until synchrony was attained. It was noted that when frequency entrainment was achieved, the action potentials in the cells could still have somewhat different waveforms. This was caused by current flow between the cells during the diastolic interval—the time between the most negative value of the membrane potential and on the upstroke of the next action potential. During this time, coupling current leaves the cell with the decreasing potential, compromising its own depolarization, and increasing the depolarization of the other cell, distorting the voltage waveforms in both cells (analogous to the findings above in Rohr and Salzberg [1994]). As junctional conductance is increased, the efficiency of the transfer and coordination of the waveforms increases; both frequency and waveform were identical when junctional conductance was an order of magnitude greater than the minimum required for action potential synchronization. The summative finds were that “at low Gc [coupling conductance] mutual pacemaker synchronization results mainly from the phase-resetting effects of the action potential of one cell on the depolarization phase of the other. At high Gc, the tonic, diastolic interaction prevails [due to the increased efficiency of current flow between the cells].”
This study reasonably presumed that the junctional conductance was ohmic and did not change during the voltage transients. Through the work of Veenstra and colleagues, we know that this is not the case (Lin et al., 2003, 2005; Lin and Veenstra, 2004). It was shown for two cardiac connexins (Cx43 and Cx40) that there can be substantial reductions in junctional conductance during cardiac action potentials, because of the voltage sensitivity of the connexins involved. Cx45 is the dominant connexin expressed in the human SA node, and it is more voltage sensitive than Cx43. It remains to be seen whether in the SA node the junctional conductance decreases during action potentials, and the effect that would have on spike propagation.
Invertebrate vision (first indication of voltage sensitivity of coupling)
JGP has a strong record of publications in invertebrate visual physiology and especially on the photoreceptors of Limulus polyphemus. The first report in JGP of electrical coupling in a visual system was an abstract presented at the 1967 Society of General Physiologists meeting on Sensory Processes in Woods Hole, Massachusetts (Shaw, 1967). In 1972, Nolte and Brown (1972) published a study on the Limulus median ocellus. They showed that this primitive eye contained two types of photoreceptors, one sensitive to green light and the other to UV light. The latter were found to be electrically coupled to each other and to a second-order neuron. The study focused on the differences between these photoreceptors and their functional organization relative to the Limulus lateral eye. No particular inferences were drawn regarding the role of the coupling in visual processing. However, this work demonstrated that the UV-sensitive photoreceptors, like those of the retinular cells of the Limulus lateral eye, responded to strong hyperpolarization with an active process termed a “hyperpolarizing response.” With maintained constant hyperpolarizing current, the cell became more hyperpolarized, while in parallel the voltage in the cells coupled to it decreased. This uncoupling phenomenon had been observed and explored extensively in the Limulus lateral eye and attributed to a hyperpolarization-driven decrease in junctional conductance and the consequent increase in input resistance of the stimulated cell (Smith et al., 1965; Smith and Baumann, 1969). This “hyperpolarizing response” is the earliest example of a voltage-dependent junctional conductance, and this article in 1972 its first mention in JGP. More about this later.
Taste (capacitance and coupling)
The physiology of taste has also been prominent in JGP. Electrotonic coupling was examined in the taste buds of the mudpuppy Necturus maculosus (West and Bernard, 1978). In this initial report, the coupling was described as unsurprising and there was little speculation on its functional effect. Years later, Bigiani and Roper published two papers that used voltage clamp to explore electrotonic coupling in taste receptor cells in N. maculosus, using coordinate changes in membrane capacitance and input resistance to assess the degree of coupling (Bigiani and Roper, 1993, 1995). This method of assessing coupling among groups of cells had been worked out by Santos-Sacchi (1991) a few years earlier. The electrical measurements were complemented by dye-coupling studies using Lucifer yellow and validated by treating the tissue with 1-octanol, which decreases gap junction coupling. The measurements were incorporated into a model of the coupling network used to estimate junctional resistance. It was noted that, in this system, membrane capacitance was a more sensitive reporter of junctional coupling than was input conductance or degree of detectible dye coupling. It was speculated that the coupling favored synchronous activity and integration of subthreshold receptor potentials. Modeling suggested that the median level of junctional resistance fell on the steep part of the curve relating it to degree of coupling, so that relatively small changes in junctional resistance would have large effects on the intercellular spread of potentials.
Parturition (hormonal regulation of gap junctions)
Sims et al. (1982) performed a rigorous and comprehensive study of the basis of development of synchronous activity of uterine smooth muscle at the end of pregnancy. During most of gestation, uterine smooth muscle, although reasonably well coupled, does not exhibit notable synchronous activity. As birth approaches, the activity becomes highly synchronous to facilitate delivery. This study performed detailed electrophysiological studies (assessing membrane potential, length constant, and internal resistance) and longitudinal frequency-dependent impedance studies of rat uterine muscle before, during, and after delivery, in addition to EM. The authors were able to separate out changes in junctional, myoplasmic, and membrane resistances. The results showed that the myoplasmic resistance remained constant throughout, but that the junctional resistance decreased ∼60% leading up to delivery, along with a ∼50% increase in nonjunctional membrane resistance (each of these changes enhances coupling). The changes reversed after delivery. The decreased junctional resistance correlated with a dramatic up-regulation in morphological gap junctions and the expected changes in length constant. The authors were somewhat skeptical about whether gap junctions were the sole mechanism for electrical coupling, largely because of negative EM findings in some coupled tissues. However, they were convinced of the role of gap junctions in this particular instance, stating: “Whether gap junctions are necessary for cell coupling is a subject of debate, but available evidence including results described in this investigation suggests that the junctions are sufficient for coupling.…These data support, but do not prove, our hypothesis that gap junction formation causes an improvement in electrical coupling in parturient myometrium.”
Exocrine gland function (amplifying the effects of chemical synapses)
The role of electrical coupling in activation of an invertebrate salivary gland was explored by Senseman et al. (1983) using multisite optical recordings. The innervation of the salivary gland in the snail Helisoma trivolvis is such that action potentials are stimulated in only some cells in the gland, and in a variable manner. Electrical coupling within the gland had been demonstrated by others (Kater et al., 1978), but this study examined the interplay between chemical synaptic input and electrotonic coupling in the spread of excitation. By analyzing the spread of activity at multiple positions after stimulation, it was evident that action potential initiation in distal gland regions was propagated via electrotonic transmission rather than by direct chemical stimulation. The electrotonic transmission was essential for the gland to be fully activated; in only one of more than 100 trials did full activation not involve electrotonic transmission.
Vertebrate vision (diverse modulatory influences and functional properties)
By the mid-1980s, it was known that the central visual receptive fields of bipolar cells in teleost and amphibian retinas were much larger than could be accounted for by their dendritic field diameters and direct synaptic connections to photoreceptors. In 1986, Kujiraoka and Saito (1986) showed in Proceedings of the National Academy of Sciences (PNAS) that carp bipolar cells were electrically coupled to each other. In 1988, they expanded on this work in JGP, showing that this coupling enabled spatial summation of the inputs to the cells and that this contributed to the enhanced central visual field (Saito and Kujiraoka, 1988). They further showed that bipolar cells separated by as much as 130 µm could be electrically coupled, and that the coupling was only within each category of bipolar cells (ON and OFF cells) and not between them. Of note, the electrically coupled cells were not dye-coupled using Lucifer yellow. In the paper it was speculated that this was because of a detection issue (e.g., low number of junctional channels), but we know now that impermeability to larger dyes such as Lucifer yellow is characteristic of the particular connexin expressed in these cells (Cx35 or its murine orthologue Cx36).
Kamermans et al. (1989a,b) conducted extensive studies to understand the determinants of the lateral feedback from horizontal cells back to the red- and the green-sensitive cones from which they receive their synaptic input. As for the bipolar cells above, it was known that electrical coupling among cone-driven horizontal cells was responsible for their receptive fields being larger than their dendritic fields. Furthermore, it was known that the coupling resistance between them could be modified by background illumination and various neurotransmitters. This study sought to characterize the changes in receptive field of the horizontal cells that occurred as functions of wavelength, spot size, intensity, and light and dark adaptation and identify the responsible mechanisms. The conclusion from the study and modeling was that the synaptic inputs from the cones modulated the electrical coupling among the horizontal cells by decreasing the membrane resistance, thereby decreasing the electrical coupling. This and other aspects of the circuitry explained the findings. This modulation of coupling by chemical synapse activity is functionally similar to that described above, under “swallowing.”
Turning to rod-driven horizontal cells, Qian and Ripps (1992) investigated whether the receptive fields of cone-driven horizontal cells were modulated by background light and transmitters in the same way as were rod-driven horizontal cells, using the all-rod retinas of the skate. They found that indeed the rod-driven horizontal cells were coupled, but that the coupling and receptive fields were strikingly unaffected by background illumination or exposure to the wide variety of transmitters and pharmacological agents to which the coupling of cone-driven horizontal cells in retinas of a variety of other species were highly sensitive. The basis for this difference is still unknown, but the results show that modulatory sensitivities of gap junctions, and their functional effects, may differ, even within the same tissue.
Lens circulation (circulation of water and ions)
Much of what is known about the physiology of lens comes from the studies of Mathias and collaborators published in JGP. In 1991, they showed that the cells of the lens were extensively coupled, but that the character and modulatory sensitivities of the coupling differed with depth within the tissue (Mathias et al., 1991). Progressing from the periphery to the deeper layers, lens cells have somewhat lower junctional conductance and become insensitive to decreases in pH. The intracellular pH of the deeper cells was more acidic than that of the peripheral cells. Because ion transport out of the lens, including that of H+, occurs exclusively at the surface, it was proposed that the only way for H+ to be transported out of the deeper cells is via a circulating current that brings it to the surface cells through gap junctions. Like most gap junctions, those of the surface cells are inhibited by acidification, but for the H+ to circulate to the surface there must be a concentration gradient of H+, which required that the inner cells have a lower pH. For the system to work, the gap junctions of the inner cells must be relatively insensitive to pH, which was observed. It was postulated that the elimination of their pH sensitivity was caused by a modification of the gap junctions, with aging and/or position.
The basis of these differences was presented in 2001, after identification of the two connexins involved and study of the lenses of corresponding knockout animals (Baldo et al., 2001). In the peripheral layers, coupling was partially reduced by knockout of either Cx46 or Cx50, suggesting that both connexins contribute to the coupling in that region. In the Cx50 knockout, the junctions in the deeper layers were pH insensitive, as in wild type, suggesting that Cx50 did not contribute to pH insensitivity in that region. It was inferred that Cx50 imparted pH sensitivity to the peripheral layers and Cx46 imparted pH insensitivity to the deeper layers. Knockout of either connexin caused cataracts of different types.
In spite of the clear and satisfying results of this study, there was a conundrum: When exogenously expressed in oocytes, Cx46 junctions are sensitive to pH (White et al., 1994b), in contrast to the pH insensitivity of Cx46 in the deeper layers of the lens. We now know that at the transition between the peripheral and deeper layers, the C termini of both connexins are cleaved by calpain (Kistler and Bullivant, 1987; Lin et al., 1997). It was proposed that deletion of this domain of Cx46, thought to mediate the pH sensitivity, might render the channels pH insensitive. It was later shown that truncation of Cx46 did not actually eliminate the sensitivity but shifted the pKa 0.2 units in the acidic direction, from 6.8 to 6.6 (Eckert, 2002). Truncation of Cx50 dramatically down-regulates its channel activity (DeRosa et al., 2006), consistent with its minimal contribution to coupling in the deep layers of the lens.
In 2004, the notion of a circulation system in the lens, which allowed metabolic products generated in the deeper layers to exit the tissue, was explored in the context of Ca2+ regulation (Gao et al., 2004). It was suspected that elevated Ca2+ levels facilitated development of cataracts via activation of a Ca2+-dependent protease that cleaved crystallins, which then aggregated. As for H+ and other ions, transport out of the lens is mediated only by transporters in the cells at the surface, so a circulatory system would be essential. Using wild-type, Cx46 knockout, and Cx46-for-Cx50 knock-in lenses, intracellular Ca2+ was assessed using FURA2 at calibrated depths, and the Ca2+ levels and gradients were correlated with previously published studies of gap junction coupling conductances for the wild-type and knock-in mice. The findings supported the role of connexin coupling on the circulation of Ca2+ that established its proper homeostasis in the lens. Because of the knock-in lenses having greater coupling conductances than wild-type, the Ca2+ levels increased less steeply with depth than in wild-type. The Cx46 knockout lenses had dramatically increased Ca2+ at all levels. The increased coupling in the knock-in provided support for the idea that in wild-type lenses, the Cx50 was essentially nonfunctional in the deeper layers, a consequence of its C-terminal cleavage.
The role of connexins in lens physiology was elevated to a greater level of sophistication in work that explored the basis of volume flow in the lens circulatory system (Gao et al., 2011). This paper addressed a fundamental mechanism by which stratified epithelia can transport fluid. The ionic circulatory system described above was suspected to drive fluid movement as well as ion movement, and there was some supportive evidence. Previous work had established that the ionic circulation from the interior to the surface (driven by Na+/K+ ATPase at the surface) involved movement through gap junctions. If the water and the ions moved together, then the outward movement must be driven by hydrostatic rather than osmotic pressure. The experiments asked whether there is a such a pressure and whether the outward fluid flow is through gap junctions. An intracellular microelectrode-based manometer was devised, and hydrostatic pressure was measured as a function of depth from the surface in wild-type lenses and lenses from a heterozygous Cx46 knockout and the Cx46-for-Cx50 knock-in. There was indeed substantial hydrostatic pressure at the center of the lenses, which declined toward the surface. In the mutant lenses, the pressure profiles changed in accordance with the levels of coupling conductance—with greater conductance, the pressure was less, and with less conductance, the pressure was greater. Reduction of the Na+/K+ pumping capacity at the surface dropped the pressure, showing that the energy reflected by the hydrostatic pressure was ultimately provided by the Na+ circulation. On the basis of size and charge criteria, there is every reason to think that water can permeate gap junction channels and no reason to think otherwise (all-atom molecular dynamics simulations of connexin pores show freely mobile water; see Luo et al. [2016]). In fact, early modeling of the effects of steady-state junctional currents on ion accumulation could not reproduce experimental results without incorporating water flux through the junctional channels as well (Brink et al., 1988).
Overall, the demonstration that the transport properties and organization of a tissue can generate hydrostatic pressure that drives intercellular water flux through gap junctions is a fundamental insight with potential application to any fluid-transporting tissue. This gap junction–mediated circulation of water and ions, its modulation, and the role of cataract formation continue to be explored and their importance extended (Gao et al., 2015, 2018).
Swimming (different strokes)
Synaptic analysis, dye coupling, electrophysiology, and physiological stimulation in vertebrate skeletal muscle were integrated to show how electrical coupling was used (and not used) to generate swimming behavior in zebrafish larvae (Luna and Brehm, 2006). Luna and Brehm initially characterized the differences in electrotonic coupling in fast and slow muscle by application of sinusoidal stimuli over a range of frequencies, complemented by dye-coupling studies. They found a low degree of coupling in fast muscle and much greater coupling in slow muscle, and they defined the low-pass filtering characteristics of each. Building on this information, they were able to kinetically distinguish primary synaptic PSPs from electrotonically transmitted events in each type of muscle. To control for possible error in voltage clamp resulting from the coupling in the slow muscles, they compared the time courses of the clamped currents with those of extracellular field potentials. They confirmed that what they had identified as electrotonically transmitted transients were specifically eliminated by a gap junction inhibitor, and that they did not generate detectible extracellular field potentials. Further studies defined the size of coupled networks in both muscle types.
To understand how the electrotonic spread of PSP-generated currents played out in swimming, they used a mutant strain of zebrafish (relaxed) in which muscle contraction did not occur, but in which neuronal patterns of fictive swimming could be induced by photic stimulation. The synaptic and electrotonic activity in the muscle was characterized with and without the gap junction inhibitor. Control studies with formamide-paralyzed WT larvae were also performed. Detailed analysis showed that the highly coupled low-pass filter property of the coupling in slow muscle (1) allowed effective but slowed transmission of potentials from PSPs, allowing temporal integration of depolarizations/contractions with a segment, and (2) imparted a fixed delay in transmission between segments to define the rostral-caudal wave of depolarizations/contractions that produces undulatory swimming. This is a rigorous and insightful example of how exploration of the temporal and spatial dynamics of electrical coupling can inform the mechanism of a physiological behavior.
Pre-cloning physiology of gap junctions
This section summarizes the early work, mostly in JGP, (1) revealing that junctional coupling is a modifiable cellular property, and (2) providing indications of what could move between coupled cells through the junctions. It includes studies of invertebrate junctions, which are formed by innexin, not connexin, proteins. Although the specifics of modulation and permeability differ, these fundamental properties overlap.
Modulation
Initial work addressed changes in coupling in response to manipulations of the extracellular and intracellular milieu, using the salivary gland of Chironomus thummi (Loewenstein et al., 1967). It was found that extracellular Ca2+ chelators, pH 10, trypsin, or hypertonic media caused rapid uncoupling, with only the effect of high pH being reversible. Iontophoretic injection of Ca2+ also uncoupled the cells. Injury of a cell in the presence of millimolar Ca2+ caused the input resistance of the cell coupled to it to rise (suggesting an “uncoupling” action of the junctional membrane thus exposed to high Ca2+), but this did not occur in nominally Ca2+-free medium. The latter two studies suggested that the junctional membrane conductance was reduced by high Ca2+ levels. In much of this work, it was difficult to discern whether the changes in coupling were caused by direct effects of the manipulations on the junctional membrane or were downstream of cellular responses to the manipulations. Exploration of regulation of Chironomus gap junctions by cytosolic Ca2+ was pursued in other journals, using aequorin imaging combined with focal Ca2+ injections (Rose and Loewenstein, 1975, 1976).
In what may be the first “biophysical” study of electrical coupling, Payton et al. (1969b) examined the temperature dependence of junctional conductance in the crayfish septate giant axon. Low-chloride solutions had been shown to reversibly uncouple the cells and separate the septal membranes. This led to the somewhat dubious conjecture that the cell movements that pulled septal membranes apart might be eliminated by cooling. The effects of cooling and rate of temperature change on the relevant conductances, as well as septate membrane separation, were examined (the motivating low-chloride experiments seem to have been forgotten). With cooling over a 15°C range, junctional resistance increased with average Q10 of 3.1, whereas the nonjunctional resistance increased with Q10 close to that of aqueous diffusion. These changes were readily reversible, showed little hysteresis, and were not accompanied by any detectible separation of the septal membranes as assessed by EM. The authors speculated that cooling changed a feature of the septal membrane structure not visible by EM, causing it to revert to the high-resistance state of nonjunctional membrane. There was no comment on the implications of a Q10 in the range of 3.0. The junctions are described as “tight junctions,” but a “Note Added in Proof” confirmed that they were gap junctions as described by Revel and Karnovsky (1967) and Brightman and Reese (1969).9
The temperature dependence of junctional conductance has not been significantly pursued. A 1980 abstract showed a low Q10 for the conductance between blastomeres in the absence of junctional voltage but a substantial Q10 for voltage-dependent transitions (Harris et al., 1980). A study at the single-channel level using embryonic cardiac cells showed multiple unitary conductance levels (substates) with occupancies with different temperature dependencies (Chen and DeHaan, 1993).
Turin and Warner (1977) were the first to show regulation of gap junctions by pH, using Xenopus laevis blastomeres. Electrical coupling was shown to be a reversible function of intracellular pH (pHi), assessed by an intracellular pH electrode. Injection of Ca2+ up to micromolar levels had no effect. It was suggested that in the earlier studies increased Ca2+ levels may have been accompanied by a drop in pHi, as had been seen in other cellular systems. This initial report was followed by more detailed studies of pH regulation and the relative influence of Ca2+, sometimes coming to different conclusions in different systems (Rose and Rick, 1978; Turin and Warner, 1980; Spray et al., 1981b, 1982).
After these early studies, study of regulation of junctional coupling by cytosolic pH and Ca2+ moved largely to other journals and focused on specific tissues and connexins. In 1990, it was addressed in JGP by White et al. (1990) in ventricular myocytes, with an answer that might have been anticipated: both pH and Ca2+ can be involved. Using electrophysiology of paired myocytes, Fura-2 imaging, and manipulations of intracellular pH and Ca2+, the results showed that decreases in pHi or increases in intracellular Ca2+ alone did not reduce junctional conductance. There must be at least normal intracellular Ca2+ levels for decreases in pHi to reduce the conductance, and there must be acidic pHi for increases in intracellular Ca2+ to do so; thus, Ca2+ and H+ act synergistically. However, the relations are such that in a normal extracellular milieu, a drop in pHi will decrease junctional conductance, but increased intracellular Ca2+ in the absence of decreased pHi will not.
Voltage dependence
As noted above, the first suggestion of voltage sensitivity of junctional conductance was reported in 1965 by Smith et al. (1965) in the ommatidium of the Limulus lateral eye. This paper showed that with hyperpolarizing current pulses delivered to a retinular cell, its input resistance increased as the electrotonic coupling to the eccentric cell decreased. Both changes are most simply explained by an increase in junctional resistance. The phenomenon was more fully analyzed by Smith and Baumann (1969), who showed that the same phenomenon occurred symmetrically between retinular cells: “...the coupling is a function of the potential difference across the junction, and uncoupling by appropriate adjustment of the junctional potential difference is quite reversible.”
The same regenerative hyperpolarization in stimulated retinular cells was studied by Wasserman (1968), but without looking at the coupling. Instead, he focused on the observation that “steady current holds the cell in one of two stable states, depending on current strength.” Brief stimuli of opposite polarity could trigger a cell into either state, defined by a stable difference in membrane potential. As a result of not examining changes in coupling, the mechanism for the increased resistance is mistakenly attributed to nonjunctional voltage dependencies. The bistability of membrane potential of a cell coupled to neighbors by a voltage-dependent junctional conductance was recapitulated, by experiment and computation, in later studies based on the kinetics of junctional voltage dependence (Harris et al., 1983).
These early indications of voltage-dependent junctional conductance went unnoticed until ∼10 yr later when the phenomenon was rediscovered by Spray et al. (1979) (Fig. 4, upper panels). Using current clamp, they showed that Ambystoma mexicanum, X. laevis, and Rana pipiens blastomeres uncoupled during hyperpolarizing or depolarizing current pulses, essentially reproducing the traces shown by Smith and Baumann (1969). The electrical uncoupling correlated with loss of Lucifer yellow spread between the cells. Two coupled cells were individually voltage clamped so that junctional currents could be directly observed and analyzed as functions of junctional voltage. This report included the first direct measurements of junctional currents and set the stage for study of junctional conductance using the electrophysiological tools being applied to other membrane conductances.
Figure 4.
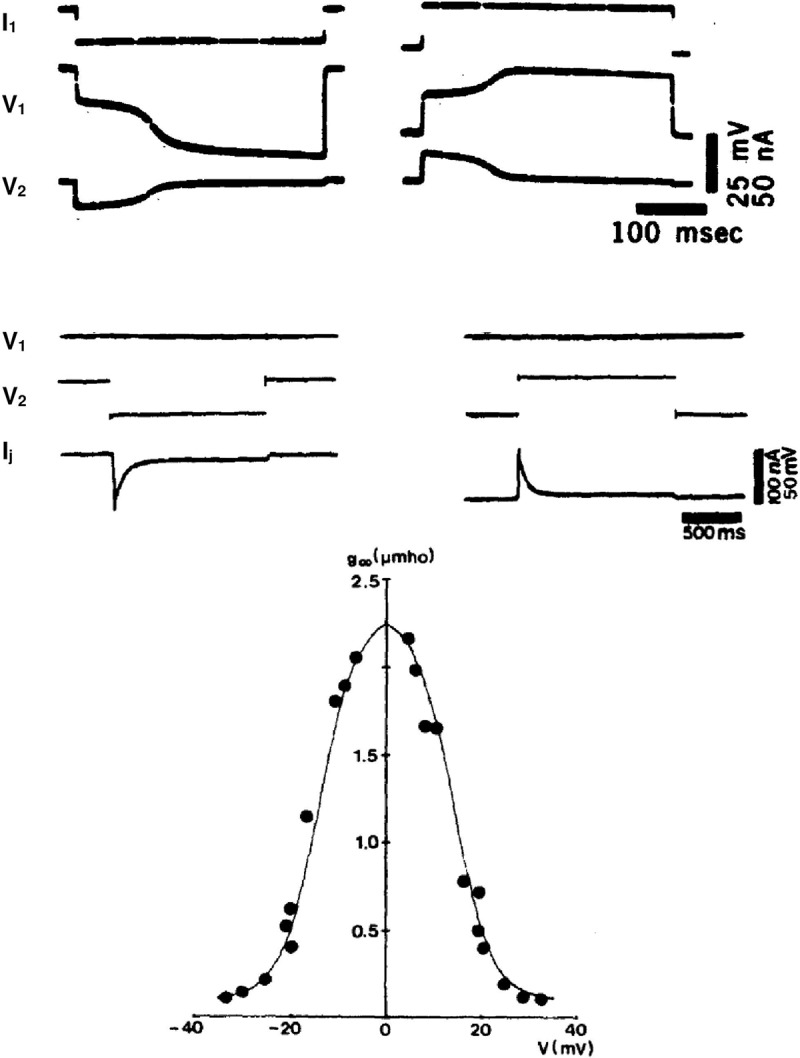
Early recordings of voltage dependence of junctional conductance of pairs of Ambystoma blastomeres. Upper panels: Application of polarizing current (I1) to one cell (V1) of a coupled pair (V1, V2) causes the cells to uncouple (from Spray et al. [1979]). Middle panels: Voltage clamp of two coupled blastomeres. Voltage of one cell (V1) is kept constant, while the other cell (V2) is stepped to positive or negative voltages. The junctional current (Ij) is recorded as the clamp current applied to cell 1 (from Spray et al. [1981a]). Bottom panel: Plot of the steady-state junctional conductance–voltage relation of a pair of coupled blastomeres. Lines are Boltzmann fits to each polarity of voltage (from Spray et al. [1981a]). Fig. 4 top is reprinted with permission from Science.
The first full characterization of voltage-dependent junctional currents was in back-to-back papers in JGP (Fig. 4, lower panels; Harris et al., 1981; Spray et al., 1981a). These papers showed that nearly all of the junctional conductance was steeply voltage dependent, with a small voltage-insensitive component. The conductance was insensitive to the voltage across each plasma membrane, responding only to differences in voltage across the junctional membrane. The relaxations were voltage dependent and apparently first order. The steady-state and kinetic effects were analyzed using the Boltzmann distribution and standard rate theory, drawing explicitly on the analysis applied to excitability-inducing material10 (EIM) conductances in bilayers published in JGP by Ehrenstein and colleagues (Ehrenstein et al., 1970, 1974; Ehrenstein and Lecar, 1977). For each polarity of voltage, the relations among voltage, time constant, and steady-state conductance were well modeled by a reversible two-state reaction scheme. The data suggested that each oppositely oriented hemichannel contained a gating mechanism sensitive to one polarity of junctional voltage. The operation of the two voltage-sensitive gates in series seemed to be contingent on one another, perhaps via modulation of the voltage profile within the pore, whereby a “closed” gate reduces the portion of the junctional voltage that drops across the sensor of the other gate. These studies established the vocabulary and basis for the analysis and description of junctional voltage dependence that are still in use, although often in more complex form.
Permeability
To account for non-ephaptic electrical coupling, it was presumed that the junctions were highly permeable to small, mobile intracellular ions. Movement of molecules directly between cells was apparently not investigated after the work of Schmidtmann in the 1920s until Kanno and Loewenstein (1964) showed that Drosophila melanogaster epithelial cell junctions mediated intercellular diffusion of fluorescein. This initial report was followed by a more detailed study in JCB (Loewenstein and Kanno, 1964).
The observation that the “impalability” of cultured chick heart cells (the ability to survive being impaled by a microelectrode) correlated with degree of electrical coupling inspired DeHaan and Gottlieb (1968) to make a prescient speculation in JGP: that electrical coupling allowed cells to replace the ions lost during impalement by diffusion through the intercellular coupling pathway from its neighbors. The paper stated that “those neighboring cells might serve as a source of current and of intracellular ions which would tend to maintain the resting potential of the impaled cell.” They continued, “The adjoining cells might also serve as a source of other substances which could hasten membrane repair or synthesis.” This is an assertion of what would later be called “metabolic cooperation” mediated by gap junctions, and may be the first suggestion in print, inferred from experiment, that gap junctions may have roles other than intercellular spread of electrical potential.
As noted above, Pappas and Bennett (1966) showed that fluorescein freely traversed the septate junctions of the crayfish giant axon. However, there was concern that fluorescein might also cross nonjunctional membranes, so studies were performed using Procion Yellow, which is slightly larger, known to not cross cell membranes, and could be imaged by EM after tissue fixation (Payton et al., 1969a). Using the septate giant axon of crayfish, junctional transfer of Procion Yellow was seen, and control studies confirmed absence of its permeation of nonjunctional membrane. A footnote refers to studies showing that sucrose permeates the junctions, but this work apparently remained unpublished except in abstract form (Bennett and Dunham, 1970). It would be the first report of a specific biological molecule permeating an electrotonic junction.
In 1972, Gilula et al. (1972) demonstrated that gap junctions enabled intercellular transfer of biological molecules and that this transfer could have functional effects. The phenomenon of “metabolic cooperation” had been described previously as dependent on cell contact, but this paper firmly established that, in the cells studied, it occurred only when cells were coupled electrically and morphologically by gap junctions. Donor cells with functional hypoxanthine-guanine phosphoribosyl transferase (HGPRT), which converts hypoxanthine to inosine monophosphate, were co-cultured with each of two types of HGPRT-negative cells, one of which was able to form electrical and morphological gap junctions with the donor cells and one that was unable to do so, in the presence of radiolabeled hypoxanthine. Only the former showed radiolabeling of its nuclei when cultured with the donor cells. Neither of the HGPRT-negative cells were radiolabeled when cultured without the donor cells. This was definitive evidence that gap junctions could mediate both electrical coupling and metabolic cooperation.
As part of the Brink and Barr (1977) study of the septa of the earthworm giant axon, they showed that fluorescein and TEA permeated the junctions. The evidence for TEA permeation was that it significantly lengthened action potential durations on the noninjected side, thereby showing a functional effect on the K+ channels of the axon. That fluorescein and TEA have opposite charge suggested that the junctional pathway was not highly charge selective, even among large permeants.
This work was expanded the next year in a combined freeze-cleave EM and quantitative dye-flux study of fluorescein derivatives of increasing size across the septal junctions (Brink and Dewey, 1978). The septal permeability decreased linearly with molecular weight over the range of 333 D (fluorescein) to 652 D (tetrabromofluorescein). Extensive controls and modeling were used to deal with cytosolic binding and potential technical and analytical confounders. The limiting dimensions of the probes ranged from 9 to 13 Å. Based on the “channel” surface area seen in freeze-cleave images, the specific resistance of junctional channels was calculated to be 50-fold greater than cytoplasm. The only negatively charged dye was aminofluorescein, which was less permeable than the cationic dyes, suggesting some degree of charge selectivity.
It’s a channel
For much of the 1960s, the existence of membrane channels, probably composed of protein, was largely theoretical but nevertheless widely accepted.11 By the early 1980s, it was dogma that ionic permeability of membranes was conferred by proteins that formed “aqueous” pathways through which ions could move from one side to the other. No channel proteins had been yet cloned, but single-channel recordings from cells had been obtained in 1976 by Neher and Sakmann (1976); as noted above, single-channel recordings had been seen in planar bilayers since 1969.
As understanding of mechanisms of membrane permeability evolved, it was recognized that the pathway for current flow between cells was most likely a channel of some sort. This raised the question of what sort of channels they were. Were they similar to those thought to mediate action potentials, only spanning two membranes instead of one? They would have to be permeable to molecules at least the size of fluorescein, unlike other known channels. Furthermore, could they be “gated”? In spite of skepticism about just how channel-like the intercellular pathway was, it was exciting to think that the concepts, and analytic and technical tools then being applied to voltage-dependent ion channels could be used to explore the structure–function and biophysics of junctional channels and perhaps their single-membrane subunits (hemichannels). The uneasy relationship to the concepts and mechanisms of so-called “real” ion channels has continued to the present day. The dual voltage clamp studies demonstrating that junctional conductance was gated by voltage provided empirical evidence that “real” channels were involved (Harris et al., 1981; Spray et al., 1981a).
The first recordings of single junctional channels were obtained by the dual voltage clamp technique in 1985 by Neyton and Trautmann (1985), from pairs of isolated lacrimal gland cells. The authors noted high unitary conductance, a near absence of charge electivity, a plethora of conductance states, and a remarkable slowness of transitions among them (tens of milliseconds). These would be hallmarks of connexin channel behavior. This paper was followed shortly by a more detailed biophysical characterization of single junctional channel behavior in coupled chick ventricle cells (Fig. 5; Veenstra and DeHaan, 1986).
Figure 5.
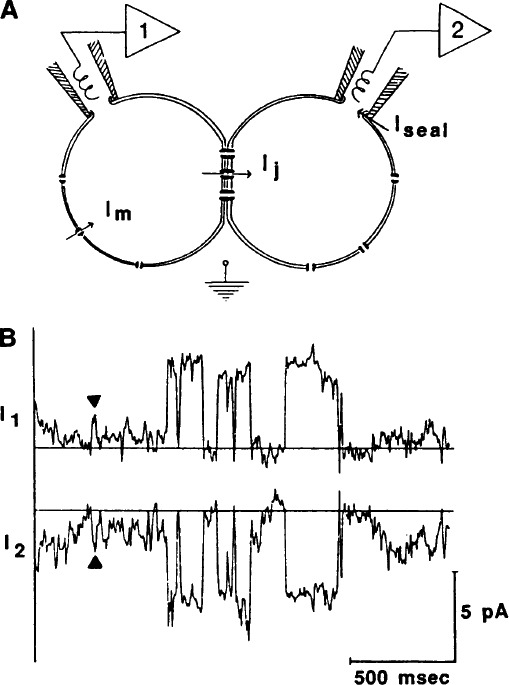
Early recording of single gap junction channels. (A) Diagram of dual whole-cell patch clamp of chick ventricle cells. (B) Currents recorded by each patch clamp for a junctional voltage of 40 mV. V1 = −40 mV, V2 = −80 mV. Junctional currents are of equal and opposite magnitude in the two clamp current traces; nonjunctional currents are not correlated. Unitary junctional conductances of two sizes are seen (from Veenstra and DeHaan [1986]). Fig. 5 is reprinted with permission from Science.
The first recordings of single junctional channels published in JGP were part of a detailed comparison of macroscopic junctional voltage-dependent currents with the underlying single junctional channel behavior (Chanson et al., 1993). This was achieved using pairs of neonatal rat Schwann cells in primary culture, some of which had sufficiently low coupling that single channels could be resolved by the dual voltage clamp technique, without use of pharmacological agents or blockers. Fortunately, even though the cells likely expressed more than one connexin, most of the junctional channels appeared to be of a single type (i.e., had a single unitary conductance). The analysis of macroscopic voltage-dependent junctional behavior essentially reproduced that of Spray et al. (1981a) and Harris et al. (1981), finding similar overall behavior with some differences in specific parameters, but the paper went on to characterize the voltage-dependent steady-state and kinetic features of the single channels. Overall, there was a good match, and a qualitative validation that the first-order kinetics seen in macroscopic currents were reflected at the single-channel level. That such a characterization could be made using a native tissue expressing multiple connexins was an impressive achievement.
Exploration of the properties of junctional channels was greatly advanced by the cloning of connexin proteins and their expression in heterologous systems such as Xenopus oocytes and mammalian cells that lack endogenous connexins. There are ∼20 redundant human connexin isoforms, each with specific tissue and developmental distributions. The cloning of a full-length connexin was first achieved by Paul (1986). This was followed the next year by the first recording of junctional currents from a cloned connexin (Dahl et al., 1987). In 1990, Eghbali et al. (1990) made the first single-channel recordings of junctional channels formed by an identified, cloned connexin.
Concurrent with electrophysiological studies of junctional channels came interest in the physiology of hemichannels, the hexameric single-membrane subunits of the junctional channel. Because they span only one membrane, they were more amenable to the techniques being applied to other channels. Also, the work on voltage sensitivity, in particular, suggested that each hemichannel contained its own gating mechanisms. There were several reports, starting in 1984, that material from isolated gap junctions formed channels in planar bilayers. Identifying the channel activity as arising from hemichannels was difficult because of the high sensitivity of bilayers to contaminants and the absence of specific pharmacological activators or inhibitors. Characterization typically relied on correlation with properties loosely inferred from cellular work on junctional channels. In reconstitution studies, permeability to molecules rather than atomic ions was used as an identifier in 1992 (Harris et al., 1992). The first use of a cloned connexin in bilayers was in 1995 (Buehler et al., 1995), and the first application of immunoaffinity purification of connexin to bilayer work was in 1996 (Rhee et al., 1996). It must be said that planar bilayer studies of connexin channels have not (yet) fulfilled their initial promise.
Meanwhile, evidence for hemichannel activity in nonjunctional plasma membranes was obtained by Paul et al. (1991) in the oocyte expression system. Expression of Cx46 caused oocytes to lyse, which could be osmotically inhibited. The oocytes took up Lucifer yellow but not BSA from the extracellular medium. The cells had low resting potentials, and voltage clamp to potentials more positive than −10 mV induced large, slowly activating currents. Interestingly, expression of a different connexin (Cx43) did not cause any of these changes, suggesting that different connexins had different abilities to form open hemichannels under the conditions of these experiments.
At about the same time, DeVries and Schwartz (1992) provided compelling evidence for functional hemichannels in horizontal cells of the catfish—a first demonstration of naturally occurring functional hemichannels. The currents were activated by reduced extracellular Ca2+ and strong depolarization, hallmarks of hemichannel activation. This work was the first to indicate that normal extracellular Ca2+ levels contribute to keeping undocked hemichannels closed. The steady-state and temporal responses to voltage were carefully characterized and found to correlate well with those of junctional currents in the same cells. Although the channels were not biochemically identified, this paper likely reported the first single-channel recordings of a connexin hemichannel in a cell membrane. The first report of hemichannel unitary currents from a cloned connexin was in 1996 (Trexler et al., 1996).
The first study of a cloned connexin in JGP was also the first study of hemichannel currents in JGP (Ebihara and Steiner, 1993). This paper defined the fundamental properties of hemichannels and how to study them. Using Cx46 expressed in oocytes, hemichannel activity was activated by long depolarizations above −20 mV. Reducing extracellular Ca2+ from 0.5 mM to nominally Ca2+-free shifted the steady-state voltage–activation curve ∼40 mV to the left, without change in slope. It also accelerated activation and deactivation kinetics and increased the maximal current. This was the first quantitative investigation of the effects of both Ca2+ and voltage in regulation of hemichannel activity.
Molecular structure and function
These advances in the study of junctional channels and hemichannels made possible exploration of their detailed structure–function. As for other channels, these studies were initially lumped into two general categories: permeation and gating. For most channels, until recently, these were considered distinct processes. However, for connexin channels, there is inherent overlap between these two aspects of channel function, because voltage sensing and permeation occur in the same physical space, the lumen of the pore. That is, alterations of structural and/or electrostatic aspects of the pore are likely to affect permeation characteristics, voltage sensing, and even the gating structures themselves, and conversely, any change within the pore caused by gating is likely to affect both voltage sensing and the character of any permeation that remains (e.g., charge selectivity; Harris and Contreras, 2014). Therefore, genetic manipulations targeted to investigate one process are very likely to affect other processes, directly or indirectly. More than other channels, the connexin channel appears to operate as a single highly coupled allosteric unit. Nevertheless, the discussion below will attempt to deal with pore and gating issues separately, until that becomes untenable.
Each member of the connexin protein family forms channels with distinct permeability properties and gating sensitivities. For example, the unitary conductance of junctional channels ranges from ∼6 to ∼300 pS, depending on the isoform. This alone makes clear that the pores are very different. Because the defining feature of this channel family is formation of wide, intercellular (or plasma membrane) channels, rather than a specific ion selectivity or activation by a specific ligand, one should not presume that the determinants of permeability or even gating are the same across the different family members. The inability to rigorously generalize across connexins has been a frustration for investigators inside and outside the field. The presentation below will emphasize “coarse-grained” rather than “atomistic” themes and understandings, as developed in JGP.
Ionic conductance and its consequences
In 1997, Veenstra and colleagues published a rigorous and comprehensive analysis of ionic selectivity of two cardiac connexins, Cx40 and Cx43 (Beblo and Veenstra, 1997; Wang and Veenstra, 1997). Pairs of transfected N2A cells were dual whole-cell voltage-clamped through patch pipettes filled with various electrolyte solutions. Single-channel slope conductances were measured for symmetric solutions of different salts, normalized by aqueous mobility. Relative permeabilities were determined from reversal potential measurements in asymmetric solutions. For Cx43, the K+:Cl− permeability ratio was ∼8:1. The relative permeabilities among cations was well fitted using the Levitt continuum theory for multi-ion pores (Levitt, 1975, 1991a,b) for a limiting pore radius of ∼6.3 Å. The selectivity corresponded to Eisenman sequence II or I (Eisenman, 1962; Eisenman and Horn, 1983). For Cx40, the K+:Cl− permeability ratio was ∼7:1, the corresponding limiting pore radius ∼6.6 Å, and the selectivity corresponding to Eisenman sequence I. In an extensive discussion of the finding that the anion conductance and permeability sequences differed greatly from the aqueous mobility sequences, it was suggested that the anion permeability is modulated by cation interaction with anionic sites within the pore, similar to a mechanism proposed by Franciolini and Nonner in JGP (Franciolini and Nonner, 1987, 1994).
The larger point made by these papers, beyond the specifics of ion/charge selectivity of these particular connexins, was that the conceptual framework typically applied to narrow pores to account for selectivity, permeability, and conductance cannot be applied to these wide multi-ion occupancy pores. Interactions among mobile ions of both signs with each other and the electrostatic environment within the pore are likely to be dominant. Such effects also imply that the energies involved in permeation of connexin channels are likely to be much lower than those involved in ion-specific channels in which there is substantial dehydration of ions and the consequent involvement of large energies in the permeation process, as recently explored in Luo et al. (2016).
As noted above, one of the earliest demonstrations of electrotonic coupling was one of the most difficult to understand. Furshpan and Potter showed that current rectified dramatically and instantaneously at the giant motor synapse of the crayfish (Furshpan and Potter, 1957, 1959), whereas almost all other instances of coupling described over the next decade were clearly nonrectifying. In 1969, Auerbach and Bennett (1969) described in JGP a rectifying electrotonic synapse in a vertebrate, the giant fiber-to-motor neuron synapse of the hatchetfish. The rectification of the junctional current was such that transmission from the giant fiber to the motor neuron was facilitated and transmission in the opposite direction was inhibited. The rectification was essentially instantaneous and largely attributable to an (unknown) electrical feature of the junctional conductance pathway itself, and not to differences in input resistance or membrane properties of the coupled cells.
In 1983, Giaume and Korn (1983) showed that rectification at the crayfish giant motor synapse (the Furshpan and Potter synapse) could be reduced and eventually eliminated by hyperpolarizing the postsynaptic fiber, thereby demonstrating a voltage dependence of the junctional resistance. Given the near-instantaneous nature of the change in junctional resistance with voltage, it was suggested that these gap junctions “have either the voltage-dependence typical of many aqueous channels, the electron transfer properties of solid-state elements, or both.” Later the same year, Margiotta and Walcott (1983) showed quantitatively that the steady-state rectification at this synapse could be well described by very rapid voltage-dependent gating. This junction was studied by voltage-clamp by Jaslove and Brink (1986). They were able to resolve kinetics of the junctional conductance changes by cooling the preparation, observing a 7.5-ms relaxation at 9.4°C for voltage jumps steps between −85 and +85 mV, and a Q10 of ∼11. The kinetics were not observable at room temperature. The next year, a voltage clamp study by Giaume et al. (1987) extensively explored and modeled the conductance changes as exclusive functions of the junctional voltage. Kinetics could not be resolved at room temperature, even with clamp resolution of 0.8 ms. A major conclusion, supported by the previous studies, was that there was an inherent asymmetry of the effect of voltage at this junction, in spite of there being no indication of asymmetry in its observable structure. The striking asymmetry of behavior and vastly more rapid kinetics of the junctional conductance changes in this system were in stark contrast to the much slower and symmetric kinetics in other systems.
Expression of specific cloned connexins permitted exploration of the basis of the rectification. In 1991, Barrio et al. (1991) found that the instantaneous junctional conductance between an oocyte expressing Cx26 and an oocyte expressing Cx32 (a “heterotypic” junctional configuration) was highly rectifying. In 1995, the basis for this was provided in recordings of single Cx26:Cx32 heterotypic junctional channels; the main state unitary conductance of these channels rectified. Over a junctional voltage range from −100 to +100 mV, the unitary conductance increased linearly from ∼20 to ∼80 pS (a slope of 32 pS/100 mV; Bukauskas et al., 1995). The mechanism was unknown, but it was suggested, based on unpublished experiments, that differences in ion selectivity of the two component hemichannels might be involved.
This, and more, was established in an experimental and modeling paper in JGP that provided a mechanistic explanation for instantaneously rectifying junctional conductances (Oh et al., 1999). The experiments to tease out the mechanism from differences in function of two connexins in heterotypic and homotypic configurations, and the contributions of different parts of each connexin protein to unitary conductances and current–voltage (I-V) relations, were complex. Based on many mutations and domain swaps, it was concluded that Cx26 and Cx32 hemichannels have opposite voltage sensitivities (discussed below). Previous work by this group showed that this difference was a function of differences of charged residues near the N-terminal end of the first transmembrane helix (TM1), at the cytoplasmic end of the pore, and residues at the extracellular border of TM1 (at the beginning of the first extracellular loop, EL1; Fig. 6). It was also known that Cx26 was somewhat cation selective and Cx32 somewhat anion selective. Experiments showed that changing the amino acids at the same positions that controlled the voltage sensitivity also altered the single channel I-V rectification, and mutations/swaps elsewhere did not. With these results in hand, pore charge distribution profiles were generated that reproduced the I-V relations and anion/cation selectivities of homotypic Cx26 and Cx32 channels using the electrodiffusion model of Chen and Eisenberg, which provided a 1-D numerical solution of the Poisson-Nernst-Planck (PNP) equations (Chen and Eisenberg, 1993; Chen et al., 1997). The charge profiles for each hemichannel were concatenated to generate a profile to correspond to a heterotypic Cx26:Cx32 channel. The resulting calculated I-V showed rectification that qualitatively reproduced the rectification of the single-channel currents.
Figure 6.
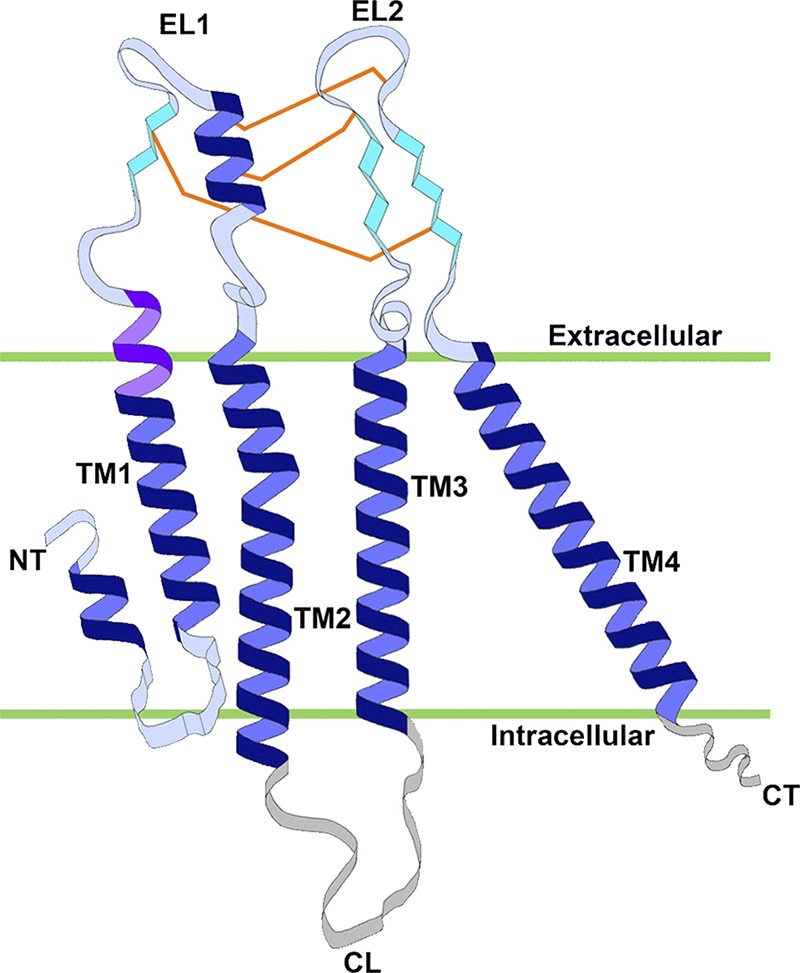
A connexin monomer indicating the domains. Consensus/approximate residue numbers for the indicated domains are NT, 1–20, with the bend roughly around position 12; TM1, 21–41; EL1, 42–71; TM2, 72–97; and CL, 98–129. Red lines indicate disulfide linkages (from Beyer and Berthoud [2017]). Fig. 6 is reprinted with permission from F1000Research.
The rectifying conductance of Cx26:Cx32 junctional channels thus appears to be due to the different charge distributions in each half of the channel. An essential insight: The experimental manipulations that altered the charge distributions that defined ionic flux were the same ones that control voltage sensing. Therefore, voltage gating and permeation were unlikely to be separable phenomena, as both were functions of the electrostatic environment in the pore.
Later the same year, Suchyna et al. (1999) investigated the same phenomenon in the same Cx26:Cx32 heterotypic channels and applied a similar analytic method. In their implementation of the PNP electrodiffusion approach, the different ionic selectivities in the two hemichannels were represented computationally by asymmetric Donnan potentials at the entrances of the pores rather than by asymmetric positions of fixed charge within the pore. This approximation also reproduced the single-channel rectification seen experimentally, although not to the same degree.
This explanation for the profound rectification of unitary currents in this heterotypic junctional channel provided insight regarding the more modest rectification of unitary conductances of some hemichannels in symmetric solutions. Hemichannel rectification was seen in Cx46 by Trexler et al. (1996) and Pfahnl and Dahl (1998) and later in Cx30, Cx45, and Cx50 by Valiunas and Weingart (2000). One may infer that the rectification in these hemichannels also arises from asymmetric distributions of charge that affect flux of atomic ions. A corollary is that the primary energetic determinant of ionic flux in these wide pores is not a centrally located energy barrier.
Molecular permeation
Molecular permeability through connexin channels was initially used more as a diagnostic feature of coupling than a means to explore the properties of the pathway. Every time a new connexin was cloned and expressed, its permeability to dyes (usually Lucifer yellow) was documented. This provided a rough estimate of the limiting pore width (∼12 Å), taken to infer that cytoplasmic molecules of that dimension or smaller ought to be permeant as well. As noted above, evidence for purine permeation of gap junctions was provided by Gilula et al. (1972). Use of fluorescent tracers of different size and charge led to inferences about relative pore width and charge preference for different connexin channels (reviewed in Harris and Locke, 2009). The relatively few studies of molecular permeability of connexin channels published in JGP have focused on implications for pore structure–function and permeation by biological molecules.
In 2002, Valiunas (2002) presented a detailed quantitative analysis of Cx45 hemichannels expressed in connexin-deficient mammalian cells, including studies of dye uptake and leakage of Lucifer yellow and propidium. The dye flux was correlated with magnitude of hemichannel currents and compared with junctional flux. Quantitative analysis of the flux of propidium was compromised by its binding to DNA, but it was clear that although highly anionic Lucifer yellow and highly cationic propidium both permeate Cx45 hemichannels, Lucifer yellow was the more permeable. The flux rates of Lucifer yellow through hemichannels was estimated from its concentration and estimation of the number of open channels from measured macroscopic and unitary conductances. Normalized to concentrations, the Lucifer yellow junctional permeability was 16-fold less than that of hemichannels, a greater reduction than expected for a doubling of pore length alone. Possible reasons were extensively discussed and illustrated the factors that could come into play, from alteration of the limiting pore diameter with hemichannel docking, to flux coupling, to altered occupancy of substates.
Regarding molecular permeability, it is the ability of endogenous signaling molecules to permeate connexin channels that is of biological importance. In this context, it becomes important to know (1) the degree to which a specific type of connexin channel can distinguish among biological permeants, and (2) the different selectivities among biological permeants exhibited by different connexin channels. Early non-JGP studies demonstrated surprising degrees of both types of selectivity among biological permeants for connexin channels (Bevans et al., 1998; Goldberg et al., 1999, 2002; Hernandez et al., 2007).
Using a cyclic nucleotide modulated channel (SpIH), in JGP, Kanaporis et al. (2008) obtained relative and absolute per-channel permeabilities of cAMP through junctional channels formed by each of three different connexins (Cx26, Cx40, and Cx43). SpIH tail currents reported real-time junctional flux of cAMP from a donor cell containing a known concentration of cAMP. During cAMP flux, junctional conductance was monitored by dual voltage clamp. As one would imagine, this experiment is difficult and prone to confounding factors and cellular behaviors. The data showed that the cAMP per-channel permeability of Cx43 junctional channels was 3.2-fold greater than that of Cx26 junctional channels and 5.2-fold greater than that of Cx40 junctional channels. The per-channel fluxes were normalized to cation flux and compared with previous work on flux of Lucifer yellow. The bottom line: Different connexin channels have different permeabilities to biological signaling molecules.
The Na+/K+ permeability ratios were essentially the same for all three connexins, in spite of a range of unitary conductances from 55 to 125 pS. The Cl−/K+ permeability ratio for the lowest conductance channel (Cx43; 55 pS) and the highest conductance channel (Cx40; 125 pS) also were about the same (0.13), whereas that of the intermediate conductance channel (Cx26; 110 pS) was significantly greater (0.38). The permeabilities to the molecules differ, as one may expect, but do not correlate with unitary conductance; the channel with the lowest unitary conductance had the highest permeabilities to cAMP and Lucifer yellow. Lack of correlation among unitary conductance, molecular permeability, and charge selectivity had been reported previously (Veenstra et al., 1995), extended here to a biological signaling molecule. Speculation as to the responsible underlying mechanisms included different combinations of effective channel lengths and limiting widths. The continuum theory of Levitt (Levitt, 1975, 1991a,b) previously used by Veenstra and colleagues (Beblo and Veenstra, 1997; Wang and Veenstra, 1997), applied to the data in this paper produced different estimates of pore diameter for the same connexin when based on the Lucifer yellow or the cAMP permeabilities. These estimates also differed from the pore diameters based on monovalent cation data. This theory essentially scales the ratio of the diffusion constant of a permeant within the pore to that in cytoplasm as a function of the relation between the minimal radius of the permeant to the radius of the pore. That it results in different calculated pore widths for the same channels based on data for atomic ions (K+), a fluorescent dye (Lucifer yellow), and a biological signaling molecule (cAMP) says that other factors are involved in the permeation process.
Although its inapplicability seems like a trivial point in retrospect, the notion that the junctional pore was a featureless right-cylinder had been the default idea in the minds of many, mostly a product of the idea that junctional channels were just holes that let anything through below a certain size. The data showing there was little correlation among unitary conductance, size of permeable molecules, charge selectivity, and limiting width forced an end to this simplistic notion.
The pore width emerging from the Levitt formulation based on the cAMP permeability numbers was narrower than that based on the much larger molecule Lucifer yellow. This raised the possibility that the smaller, biological permeant interacted more strongly with the pore (i.e., decreasing its effective “diffusion constant” within the pore) than did the nonbiological tracer molecule. Rephrased, one must be cautious about inferring permeability of biological molecules from that of nonbiological molecules; in this case, cAMP permeability revealed something about the pore that Lucifer yellow did not. It is clear that each connexin has its own preferences and selectivities among biological molecular permeants, and they are not explained by simple considerations of permeant size and charge (reviewed in Harris, 2007). Although the specific mechanisms by which connexin channels select among molecular permeants are as yet unknown, the forces that come into play in the relatively low-energy interactions of molecules, with many degrees of freedom (configurational, orientational), within the connexin pore have been explored computationally (Fig. 7; Luo et al., 2016).
Figure 7.
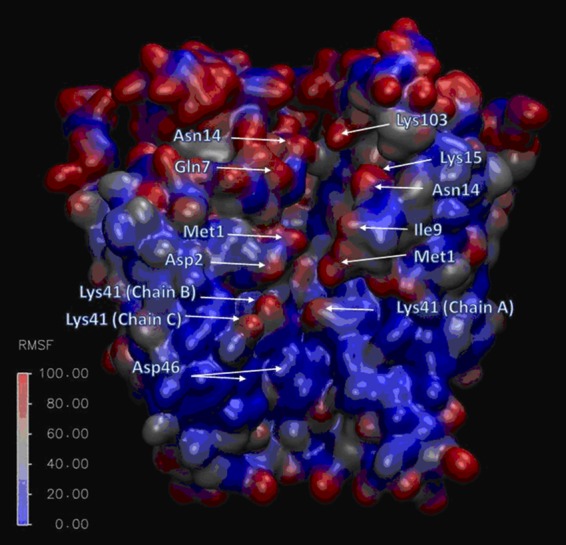
Flexibility of residue side chains lining the connexin pore. Cutaway of Cx26 hemichannel color-coded by per-side-chain RMSF values obtained from umbrella sampling Hamiltonian replica exchange molecular dynamics. Three of the connexin subunits are removed so that the pore can be seen. The cytoplasmic end of the hemichannel is at the top. Most of the residues lining the pore have high RMSF values; in contrast, the ASP46 residue, which corresponds to the peak of the PMF for an impermeant molecule, is relatively rigid (from Luo et al. [2016]). Fig. 7 is reprinted with permission from Biophysical Journal.
In addition to its biological importance, the permeability of connexin channels to small cytoplasmic molecules creates a problem for application of a commonly used experimental strategy. In 2015, Tong et al. (2015) discovered that the MTS-based accessibility scanning technique was compromised by the permeability of connexin hemichannels to cellular glutathione. While performing a pore accessibility scan on a connexin expressed in oocytes, they noticed that the effect of 2-sulfonatoethyl methanethiosulfonate sodium salt (MTSES) modification of pore-lining engineered cysteines slowly reversed. This is usually interpreted to indicate an effect of the MTS reagent unrelated to cysteine modification. In this case, however, it turned out that the reversal was a result of oocyte glutathione gaining access to the MTS-modified residue via the hemichannel pore itself and reducing the MTS-thiol linkage. Thiol modifications made with linkages that could not be reversed by reductants were stable.
The same year, a study in JGP explored the effects of pathogenic point mutants of a connexin on unitary conductance and dye permeability (Santa Cruz et al., 2015). The intriguing results illustrate the difficulty of facile explanations for the determinants of connexin pore properties. Cx40 is primarily found in the atrial myocardium. Junctional channels composed of three point mutants of Cx40 that are associated with atrial fibrillation but retain function were assessed by dual voltage clamp and dye permeation studies. Mutant A96S (in TM2) had unchanged unitary conductance and single-channel permeability to (anionic) Lucifer yellow but enhanced permeability to (cationic) ethidium. Mutant M163V (at the TM3/EL2 border) also had unchanged unitary conductance but greater permeability to both Lucifer yellow and ethidium. The third mutant (G38D), the only one in the pore-lining helix, had a significantly greater unitary conductance, greater permeability to Lucifer yellow, and essentially no permeability to ethidium. Each of these effects, while informative regarding the effects of each pathological mutation on unitary conductance and charge selectivity, are instructive in the inability to account for the effects in simple terms based on the location or character of the mutation.
What lines the pore?
The first studies to directly identify pore-lining segments of connexin channels were performed by Dahl and colleagues (Zhou et al., 1997; Pfahnl and Dahl, 1998, 1999; Hu and Dahl, 1999). Using cysteine-scanning accessibility mutagenesis (SCAM; Akabas et al., 1992) with extracellular application of a relatively large thiol reagent (maleimido-butyryl-biocytin; MBB), Zhou et al. showed modification at two positions deep in TM1 in two hemichannels (positions 33 and 34 in a Cx32 chimera and the corresponding positions in Cx46). The findings were inconclusive for positions in TM3, which had been predicted to be pore lining because it was the only overtly amphipathic transmembrane domain. Pfahnl and Dahl showed that position 35 was also accessible. Hu and Dahl generated chimeric channels in which TM1 was exchanged between the same two connexins as above. Several pore properties, including unitary conductance, were transferred, strongly suggesting that TM1 was a major contributor to the pore.
As hinted above in the discussion of rectification, mutations near the N-terminus and at the beginning of EL1 affect voltage dependence of junctional channels. Because early studies indicated that the voltage being sensed was that in the pore, it seemed reasonable that the voltage-sensing moieties were exposed to the pore lumen, and therefore could also affect permeation. The involvement of EL1 in charge selectivity was explored in Cx46 hemichannels by Trexler et al. (2000). In the key experiment, the EL1 of Cx46, a cation-preferring high-conductance channel, was replaced by that of Cx32, an anion-preferring, lower-conductance channel. This substitution changed the channel from cation to anion preferring, reduced the conductance, and changed the single-channel I-V relation from inwardly rectifying to outwardly rectifying. In the native Cx46 channel, high-ionic-strength solutions applied to the extracellular face of the channel, but not to the intracellular face, reduced its cation selectivity, also suggesting that a determinant of the charge selectivity was near the extracellular end of the pore. Many other chimeric channels were made, with functional ones giving results consistent with the EL1 domain being a key determinant of charge selectivity and permeability. The rectification was reproduced by the 1-D PNP equations, as previously used by Oh et al. (1999) in studies of single-channel rectification.
The paper that firmly identified TM1 (with the N-terminal [NT] domain at one end and EL1 at the other) as the primary pore-lining segment was published in JGP by Kronengold et al. (2003). Cysteine-scanning mutagenesis was performed on Cx46 in excised patches, using MTS reagents of different charge. Modification was seen with application from either side of the pore at positions at the N-terminal end of EL1 (i.e., closest to TM1), which altered single-channel rectification in opposite directions for oppositely charged modifying reagents. In addition, accessibility was seen at residues in TM1 about halfway through the membrane-spanning region from the extracellular side. Intriguingly, stepwise reductions in channel conductance were seen, suggesting sequential modification of cysteines in several of the subunits of the hexameric channel. No evidence of modification was seen when scanning TM3, which had earlier been suggested to be prelining on the basis of its amphipathicity and more recently by SCAM studies on junctional channels (Skerrett et al., 2002).
Confirming evidence for the direct contribution of TM1 to the pore, particularly its C-terminal half, came from expanded chimeric and mutagenesis studies from Dahl and colleagues (Hu et al., 2006). This study also showed that replacing the leucine at position 35 in the middle of TM1 with the smaller glycine residue greatly increased unitary conductance. Structural proof that TM1 was the primary pore-lining segment came with the x-ray structure of Cx26 in 2009, confirmed by a second set of structures in 2016 (Maeda et al., 2009; Bennett et al., 2016).
The 2009 x-ray structure of Cx26 (Maeda et al., 2009) provided the basis for a computational and experimental study in JGP that pulled together various themes to produce a validated structural model (Kwon et al., 2011). The crystal structure was lacking two cytosolic domains (the intracellular loop [CL] and the C-terminal tail [CT] as well as the N-terminal methionine [Met1]). These were added and the structure energy minimized, but Monte Carlo Brownian dynamics (grand canonical Monte Carlo/Brownian dynamics [GCMC/BD]) simulations showed that the pore was essentially nonconductive, mainly because of the position of Met1 deep within the pore. The system, including explicit water, ions, and lipid membrane, was equilibrated by MD. GCMC/BD of the equilibrated structure showed ion permeability, largely as result of widening of the pore in the region of Met1, but a strongly anion-selective channel and inward current rectification, unlike the native channel. Previous mass spectrometry (MS) studies had indicated a number of posttranslational modifications, some of which were charge-changing (Locke et al., 2009). Tandem MS/MS showed that Met1 was acetylated (an irreversible cotranslational modification that eliminates the charge of the terminal amine), consistent with known sequence determinants of this modification. Neutralizing the charge of the Met1 terminal amine to mimic the effect of acetylation produced I-V relations and charge selectivity that closely matched that of the native channel. Other charge changes corresponding to lysine acetylations (which are reversible) suggested by single MS caused minor modifications to selectivity and rectification, supported by variability in these parameters in channel recordings.
Lessons from this include the importance of using MD-equilibrated structures, incorporating known cotranslational modifications (and perhaps posttranslational modifications) and explicit experimental validation, in generating meaningful models. This model was later used to explore mechanisms of gating (below) and the energetics of permeation of a molecular permeant and impermeant through the Cx26 hemichannel (Luo et al., 2016).
Gating
Gating of connexin channels started out simple but became complex. Initial studies showed regulation by voltage, Ca2+ and pH. Soon it became clear that there were two separable varieties of voltage sensitivity in each hemichannel, which seemed to involve different parts of the protein. Then gating by Ca2+ appeared to involve the same or nearby parts of the protein, and one of the voltage-sensitive processes. The locations of the physical gates are uncertain, and what operates them unclear. Much of this complexity follows from the fact, mentioned above, that almost everything happens in the pore. These processes might be best viewed as different aspects of a single mechanistically integrated molecular machine (the fable of the elephant and blind men comes to mind). The initial observations of voltage gating and the influence of Ca2+ on hemichannel gating were discussed above. Below we start with the initial characterizations of modulation by pH followed by structure–function studies in which the various mechanisms become increasingly intertwined.
pH dependence
Trexler et al. (1999) published in JGP a study of the gating of Cx46 channels by pH at the single-channel level, in what remains one of the most detailed and informative analyses of this process. It was also the first report of unitary hemichannel currents in JGP. This paper unequivocally showed that Cx46 junctional channels and hemichannels in excised patches were readily, directly, and reversibly closed by acidic pH acting at the cytoplasmic end of the pore (with pK 6.4). This action did not require Ca2+ or any other cellular components. The state dependence of low pH effects was examined using fast perfusion. Low pH was found to act from the cytoplasmic side whether the channels were open or closed by voltage. The effect of low pH was voltage dependent, but in a direction opposite to that which would enhance H+ flux into the pore. One of the more remarkable features was that the single-channel closing transitions induced by low pH were slow (10–100 ms) and appeared to involve transitions among numerous, short-lived subconductance levels. This kind of transition had been seen previously in a specific type of voltage-induced transition (see “loop-gating” below), suggesting action on a common mechanism. Work on another connexin (Cx43) had shown that an intact CL domain, and a specific histidine, were involved in pH sensing (Ek et al., 1994; Morley et al., 1996). However, in Cx46, pH sensing was unaffected by deletion of most of the CL or mutation of the histidine. There is clear diversity in the pH-sensing mechanisms in different connexins.
A different kind of pH regulation of hemichannels and junctional channels was described by Locke et al. (2011) for a different connexin, Cx26. It had been shown that the apparent pH sensitivity of liposome-reconstituted Cx26-containing channels was a function of the pKas of the Good buffers (e.g., HEPES, MES) used to buffer the pH, and not the pH itself (Bevans and Harris, 1999). That is, the pH–activity relation shifted with the pKa of the buffer, and the inhibition of channel activity correlated with the concentration of the protonated form of the buffer. The same effect was seen for taurine, a cellular aminosulfonate, which is nearly fully protonated at intracellular pH. When non-aminosulfonate pH buffers were used, the channels showed no pH sensitivity. The structural basis of this regulation was explored by additions to the CT domain, NMR and ELISA binding assays of the relevant peptides, and channel permeability assays. An addition to the CT eliminated sensitivity to taurine in reconstituted channels and cells, suggesting CT involvement. However, NMR studies showed no interaction between taurine and the CT, suggesting that the CT interacted with another domain in this process, the CL being the most likely. ELISA studies of interaction between the CT peptide and segments of the CL showed that the CT interacted only with the segment of the CL closest to TM3, that low pH enhanced this interaction, and that taurine could disrupt it. NMR studies showed that taurine interacted with the target CL peptide at several of the same sites at which the CT peptide interacted, providing a basis for its disruption of the CL–CT interaction.
Several scenarios could explain the findings, but the simplest is that in this connexin, a CL–CT interaction keeps the channel open, low pH enhances this interaction, and protonated aminosulfonates disrupt it, leading to channel closure. Thus the “pH sensitivity” is really a balance between a pH-driven interdomain association, which keeps the channel open, and the protonated aminosulfonate-driven disruption of that association, which closes the channel. In this context, it is interesting to note that other cytosolic aminosulfonates have the same effect as taurine (e.g., l-cysteic acid, l-homocysteic acid, and hypotaurine) and that other cytosolic compounds, which lack the sulfonate moiety, competitively antagonize the effect of taurine on these channels (β-alanine, glycine, and γ-aminobutyric acid; Tao and Harris, 2004).
Voltage dependence (i.e., the usual source of confusion)
Perhaps the most confusing and skepticism-inducing aspect of connexin channel function is the character of voltage gating. It is unlike voltage gating in any other channel, even at its simplest level—there is no S4 equivalent, yet the conductance–voltage (G-V) curve can be as steep as that of voltage-gated Na+ channels, and the kinetics are at least an order of magnitude slower than for other voltage-gated channels. The basics were described in two non-JGP articles.
In 1994, Verselis et al. (1994) obtained two major insights about voltage gating of connexin hemichannels, deduced from the behavior of junctional channels: (a) some connexins form hemichannels that close in response to depolarization and others form hemichannels that close in response to hyperpolarization, and (b) the polarity to which a hemichannel responds is determined, and can even be reversed, by the presence or absence of charged residues at position 2 (one in from the N-terminal residue) and at the border of TM1 and EL1. These insights were derived from analysis of G-V relations of junctional channels formed by different combinations of wild-type and mutant hemichannels (point mutants and chimeras). Specifically, this paper showed that Cx26 hemichannels, when part of junctional channels, close in response to a positive junctional voltage and Cx32 hemichannels close in response to negative junctional voltage. If Cx26 and Cx32 hemichannels are paired, the junctional conductance is sensitive to only one polarity of voltage, since one hemichannel sees a positive voltage and the other a negative voltage but the hemichannels are oppositely oriented. Similarly, if a mutation that reverses the polarity of voltage sensing is made in either connexin, and it is paired with a wild-type hemichannel of the same connexin, the junctional channel will also close with only one polarity of voltage.
That the polarity of voltage sensing is determined at two locations separated by a transmembrane domain was surprising. A straightforward inference is that these positions must be within the junctional voltage field. This leads to the structural inference that the NT domain folds into the pore so that its initial segment can directly sense voltage. Later work showed that charge changes at positions 1 to 10 but no further could reverse the polarity to which a hemichannel responds, supporting the idea that the end region of the NT is in the voltage field and therefore inside the pore (Purnick et al., 2000). Because charge changes in the NT can reverse the polarity of voltage to which a hemichannel responds, it is thought that the sensor moves in the same direction in each case to effect closure.
This characterization of hemichannel voltage sensitivity was correct but incomplete, as revealed by Trexler et al. (1996) in a study of single hemichannel voltage-dependent gating. This study of on-cell and excised patches showed that as voltage is ramped from large negative voltages to large positive voltages, the channels transition from mostly closed, to mostly open, to “closed” to a substate. That is, single Cx46 hemichannels gate in response to both large positive and large negative voltages. This single-channel behavior matched the changes in macroscopic hemichannel currents. Closing at negative voltages accounts for the need to depolarize undocked hemichannels to open them. Closing to a substate at positive voltages provides an explanation for why, in junctional channels at large voltages, the conductance does not go to zero. To reinforce this point, others had shown in studies analogous to those of Verselis et al. (1994) above, that, like Cx26, Cx46 hemichannels in a junctional configuration gate in response to positive junctional voltage (White et al., 1994a), demonstrating that hemichannels have the same sensitivities to voltage when undocked and when part of junctional channels. Empirically, then, each hemichannel has two voltage-sensitive gating mechanisms, one fully closing the channel at negative voltages, and the other closing the channel to a substate at positive or negative voltages (the former in Cx26 and Cx46, and the latter in Cx32).
A second defining characteristic of these two responses to voltage was the nature of the gating transitions seen in single-channel records. The transitions into and out of the substate were rapid. However, transitions into and out of the fully closed state were slow (in this study lasting 10–20 ms) and appeared to involve multiple unstable intermediate states. Thus the two responses to voltage could be distinguished by both the conductance state to which the channels close and by the kinetics of the transitions. The fast transitions to the substate, since they corresponded to the characterizations of junctional voltage sensitivity, are referred to as “Vj-gating” or “fast gating.” The multi-stepped slower transitions to and from the fully closed state resembled transitions seen when two hemichannels initially dock to each other and open, a process inferred to involve the extracellular loops that mediate the docking interaction (Bukauskas and Weingart, 1994). For this reason, these transitions are called “loop-gating” or “slow gating.” All these terms remain in use (this article will use the Vj- and loop-gating terms). Subsequent work showed that loop-gating always closes at negative voltages, whereas Vj-gating can close to positive or negative voltages depending on the connexin (as in the Cx26 and Cx32 examples above) and that the polarity to which Vj-gating responds can be switched by mutation without affecting loop-gating.
One may ask, why at large voltages in junctional channels, when one of the two hemichannels is at a negative voltage, the channels are still conductive—shouldn’t the loop-gate of that hemichannel close the channel? A possible answer was provided by the notion, suggested by Harris et al. (1981) as a mechanism for “contingent gating” of hemichannels, that because voltage is sensed within the pore, the “closing” of one gate (e.g., to generate a substate) would alter the voltage field within the pore so that a greater portion of the field drops across that gate, reducing the portion of the field that drops across an “open” gate in the apposed hemichannel. In this scenario, in Cx46 when a Vj-gate closes to a substate, the loop-gate in the apposed hemichannel then sees a voltage insufficiently negative to induce its closure. Detailed modeling of this type of interaction has shown its essential empirical validity (Paulauskas et al., 2009), but other explanations are possible, such as the docking of hemichannels specifically destabilizing the loop-gate closed state.
Vj-gating
In 2000, Oh et al. (2000) applied and tested these ideas on Cx32 at the junctional and single hemichannel levels and explored the behavior of heteromeric channels formed of wild-type Cx32 and a Cx32 mutant in which the Vj-gating polarity was reversed. This was done on the background of a chimeric channel in which the EL1 of Cx32 was replaced by that of Cx43 (Cx32*43E1), which readily forms active undocked hemichannels as well as junctional channels while retaining the voltage-gating properties of wild-type Cx32 (first shown by Pfahnl et al. [1997]). Coexpressing this chimera with its N2D mutation variant (which reverses the Vj-gating polarity) yielded hemichannels that gated to substates at both voltage polarities. The frequency of gating to the reversed polarity caused by the N2D mutant correlated with the degree of its expression. In other words, it seemed that each connexin monomer within the hemichannel could sense and respond to voltage (i.e., adopt a conformation leading to a substate) separately from the other monomers. Though there may be interactions among the subunits in response to voltage, these findings disfavor a concerted gating model in which all six monomers need to be activated to close the channel to a Vj-gated substate.
In 2002, Bukauskas et al. (2002) published a detailed analysis of the properties of the substate to which Vj-gating closes the channel, which also yielded additional insights about the mechanism of Vj-gating. These studies used Cx43 junctional channels in which gating to the Vj substate by one of the hemichannels was blocked by a C-terminal addition, enabling clean recording of the currents through the substate induced by Vj-gate of the other hemichannel. The conductance of the substate rectified with larger conductances at negative potentials (∼30 pS at −70 mV and ∼18 pS at +35 mV; the main state conductance is ∼115 pS). Ion substitution studies showed that the rectification was accompanied by a substantial increase in anion selectivity (the main state of Cx43 being essentially nonselective between cations and anions). Furthermore, anionic and cationic dyes that readily permeate the main state did not permeate the substate.
These changes in single channel I-V and selectivity were consistent with Vj-gating narrowing the pore and introducing a positive charge at the cytoplasmic end. This was computationally reproduced by application of the 1-D PNP equations, as in Oh et al. (1999) and Trexler et al. (2000) above. It was noted that in Cx43 negative voltage induces Vj-gating, which was accounted for by movement of a positively charged moiety in the NT into the pore (compare Oh et al. [1999]), which also shows rectification of the Cx32 Vj-gating substate). This led to the inference that the positive charge mediating gating is the same positive charge that causes the single-channel rectification and anion selectivity. The suggestion is that a key part of the Vj-gating voltage sensor is also the Vj-gating gate, in the sense that it moves into the pore lumen. This is an example of gating and permeability being mechanistically interrelated in connexin channels.
Bukauskas et al. (2002) above had speculated on the physiological importance of a voltage-induced narrowing but not closure of the pore, noting that the dyes that were excluded from the substate were about the size of biological signaling molecules. The paper ended with the statement, “Consequently, the Vj-gating mechanism can serve as a selectivity filter that preserves electrical cell–cell communication but can limit the communication of metabolic or biological signaling molecules.” In fact, 6 d before this article appeared online, this precise point was demonstrated by Qu and Dahl (2002) in PNAS. Using different connexins, they showed that Vj-gating could dramatically restrict intercellular movement of dyes and cAMP while having little effect on electrical coupling.
Loop-gating and Ca2+
Loop-gating is defined as the mechanism by which negative voltages drive hemichannels toward a fully closed state. Extracellular Ca2+ shifts the loop-gating steady-state G-V relation to the right, empirically having the effect of stabilizing the closed state. The absence of a significant change in slope suggests that the effect of Ca2+ is not on the voltage sensitive process itself, but on the energy difference between the open and voltage-closed states (an effect of Ca2+ on surface charge would tend to shift the relations in the opposite direction, and would be the same for all divalent cations, which it is not). In highly schematic terms, this could be achieved by Ca2+ either binding to a closed state and lowering its energy, and/or binding to the open state and raising its energy.
The biological and biomedical importance of loop-gating is that normal extracellular Ca2+ ensures, in the context of a normal resting potential, that undocked hemichannels remain closed. Mutational changes in loop-gating that reduce the apparent affinity for Ca2+ result in aberrantly open hemichannels, with pathological consequences. Therefore, understanding the mechanisms of loop-gating, what acts on it, and the effects of Ca2+ are of compelling biophysical and biomedical importance. The path toward this understanding has not been smooth and the ultimate goal has not yet been reached, though substantial progress has been made.
The first study of the mechanism of Ca2+ effects on hemichannels was that of Pfahnl and Dahl (1999). Using Cx46 in whole-cell and excised patch configurations, they showed that the effect of Ca2+ was strongly voltage dependent and consistent with Ca2+ having its effect by entering the pore, more readily from the extracellular side. A Hill plot had slope greater than 2, suggesting that several Ca2+ ions were required. The paper concluded, “If calcium were to act on its own specific gate, that gate would need to interact strongly with the voltage gate to account for the strict interrelation of calcium and voltage effects on channel function.… Thus voltage and calcium may act on the same gating structure or on two separate structures that interact with one another.”
In 2003, a mechanism for Ca2+ effects was proposed that involved sequential binding at two sites, one that exerts voltage-dependent pore block and one that stabilizes the channel once blocked (Ebihara et al., 2003). This study also used Cx46, with Mg2+ as a surrogate for Ca2+ in most experiments, to avoid contamination by Ca2+-activated conductances in the oocyte. Confirmatory studies were performed using Ca2+, which gave similar results, but with greater apparent affinities than Mg2+. Surprisingly, it was reported that in divalent-free solutions there was no voltage dependence of these channels. In this scheme, the voltage dependence arises from movement of Mg2+/Ca2+ through the field to its blocking sites.
A similar mechanism was proposed in JGP for Ca2+ effects on Cx37 hemichannels (Puljung et al., 2004). As above, the voltage dependence was proposed to arise from the effect of voltage to drive Ca2+ into the pore to reach binding sites near the cytoplasmic end, where block occurred. Also as above, it was reported that removal of divalent cations resulted in an essentially linear G-V curve across negative and positive voltages, with little evidence of voltage-dependent gating. In this scenario, the channel has no intrinsic voltage-dependent gating processes, only open pore block that requires the Ca2+ to traverse the full membrane voltage. In this model, there was no second, stabilizing Ca2+ binding site, but kinetic considerations required that upon relief of block (depolarizing voltages) there is an accumulation of Ca2+ at the extracellular entrance of the pore.
Voltage-dependent divalent block was an intriguing idea, but the hemichannel behavior in these papers is difficult to reconcile with other work. The low Ca2+ concentration at which no voltage dependence seen was ∼20 µM, well above that in cytoplasm, yet Cx37 junctional channels have strong voltage dependence. Cx37 hemichannels exposed to much lower Ca2+ concentrations show voltage-sensitive gating at both positive and negative voltages (Derouette et al., 2009). A possible explanation is that the above studies were of macroscopic hemichannel currents, which can become large enough in low Ca2+ to produce series resistance effects on the voltage clamp that can make the hemichannels appear insensitive to voltage.
The relation between loop-gating and divalent cations was largely resolved by Verselis and Srinivas (2008) in JGP, in single-hemichannel studies of Cx46. Vj-gating in Cx46 occurs at positive voltages only, so loop-gating could be studied cleanly at negative voltages. The bottom line from this detailed work is that loop-gating is an intrinsic property of the hemichannel, occurring in the absence of divalent cations. Divalent cations were proposed to stabilize the loop-gate closed state, shifting the G-V relation to the right, as originally reported by Ebihara and Steiner (1993), as well as the Po–V relation. Effects at the macroscopic and single-channel levels showed that without divalent ions, the closures caused by loop-gating are short lived and unstable, seemingly to rapidly transit among substates and a closed state. The effect of divalent cations is to promote relatively long-lived closed states. In these effects, Ca2+ is ∼10-fold more effective than Mg2+. The divalent cations were effective only from the extracellular side of the pore. The relation between loop-gating and Ca2+ has been further explored recently by others (Pinto et al., 2017).
Modulators of loop gating
Several reagents are widely used to inhibit the activity of connexin channels. Most are not specific for connexin channels and are nonselective among connexin channels, and their modes of action are unclear. Articles in JGP have largely steered clear of relying on these agents. When it comes to the pharmacology of connexin channels, two studies have explored connexin isoform–specific modulators of loop-gating.
In 2006, Srinivas et al. (2006) unexpectedly discovered that monovalent cations compete with the action of Ca2+ to close hemichannels, and in only one of two closely related connexins. As mentioned above, Cx46 and Cx50 are the two major connexins in the lens and have a high degree of sequence homology. Replacement of extracellular Na+ with K+ dramatically blocked the ability of Ca2+ to keep Cx50 (but not Cx46) hemichannels closed. Specifically, monovalent cations, with potency Cs+ > Rb+, K+ >> Li+, Na+, greatly increased Cx50 hemichannel currents, and this was Ca2+-dependent; the effects decreased as Ca2+ was lowered. The potentiation of the currents was not attributable to changes in unitary conductance, permeability, or voltage-gating properties. Ca2+ regulation of Cx50 seems to be uniquely sensitive to monovalent cations. Studies with chimeric channels showed that this sensitivity was determined by the amino-terminal half of the protein. Beyond this, the molecular basis of this effect is unknown, although it makes the most sense for the monovalent ions to be acting extracellularly (perhaps on EL1) but not within the permeation pathway. A potential biological relevance is that modest changes in extracellular K+ could alter the sensitivity of Cx50 hemichannels, specifically, to regulation by Ca2+.
Quinine and its derivatives were found to robustly inhibit the activity of Cx36 and Cx50 channels, and have far less effect on channels composed of Cx26, Cx32, Cx40, Cx43, or Cx46 (the closest analogue of Cx50; Srinivas et al., 2001). In 2012, their detailed mechanism was studied in JGP by Rubinos et al. (2012) using macroscopic currents and excised patch single-channel recordings). The compound tested was a quaternary derivative of quinine (N-benzylquininium; BQ+). BQ+ inhibited Cx50 junctional and hemichannel activity. In excised patches, it inhibited channel opening from either side of the channel without a corresponding effect on unitary conductance. The inhibition was voltage dependent from each side in a manner consistent with entry into the pore. BQ+ also decreased mean open time as a function of concentration. Although these effects are expected of simple open-pore block from either side of the channel, the transitions between the open and closed/blocked states were indistinguishable from the characteristic slow (20–50 ms) and noisy transitions of the loop-gating mechanism that occurred in the absence of BQ+. Moreover, the mean closed times also increased with BQ+ concentration, inconsistent with simple open pore block. These data can be explained by BQ+ acting within the pore toward the cytoplasmic end to exert a relative stabilization of the (loop-gated) closed state. Rubinos et al. (2012) showed that the channel is intrinsically voltage dependent, but suggested that one BQ+ enters the pore to close it via loop-gating and a second stabilizes the closed state (a Hill plot suggested that more than one BQ+ was involved). An intriguing aspect is that BQ+ appears to affect the loop-gating mechanism from within the pore, but electrically much closer to the cytoplasmic end. Replacement of the NT of Cx50 with that of Cx46 essentially eliminated inhibition by BQ+, strongly suggesting that its effects on loop-gating involve the physical cytoplasmic end of the pore. The actions of BQ+ suggest that the loop-gating process likely involves the whole channel, or at least that it cannot be localized to one end or the other.
Loop-gating mechanisms
The first studies to provide insight into the structural changes associated with loop-gating were those of Pfahnl and Dahl (1998, 1999). These studies, using Cx46 and the large thiol-reactive reagent MBB on whole-cell and excised patches, showed that position 35 in TM1 was accessible from both sides of the channel when it was open but was inaccessible from the extracellular side when the channel was closed by negative voltages (which close only the loop-gate in Cx46) or by extracellular Ca2+. It was concluded that the physical gate of the loop-gating mechanism was extracellular to position 35. Also, this was evidence that the loop-gate caused a local change in the pore, not a global pore narrowing.
2009 saw the first explorations of what was happening at the extracellular end of the pore during loop-gating of hemichannels, in two complementary papers on two different connexins, the first published in JCB and the second in JGP (Tang et al., 2009; Verselis et al., 2009). These studies used Cx50 and the chimeric channel Cx32*43E1 (used previously in studies of Vj-gating, above), probed by state-dependent cysteine modification and formation of metal binding sites. There were some minor differences in the two studies, but the big picture is that loop-gating involves movement of residues at the border of the TM1 and EL1 domains (Fig. 8).
Figure 8.
Schematic depiction of the extracellular domains of a Cx26 hemichannel. The box in the left panel shows the region depicted in detail on the right. The front three connexin subunits are not shown. Each subunit is represented by a different color. Cylinders inside the subunits represent the transmembrane domains. TM1 denotes the first transmembrane domain, which is exposed to the pore lumen. E1 and E2 denote the first and second extracellular loop domains, respectively. The parahelix region mentioned in the text corresponds to residues 43–51, comprising the uppermost residues of TM1 and the contiguous residues with white labels in E1. The illustration is not drawn to scale or strictly according to the atomic structure (from Sanchez et al. [2013]).
Specifically, in Cx46, cysteine substitutions at positions 43 and 46 (and to a lesser extent 51) resulted in closed channels stabilized by Cd2+ under conditions that favored the loop-gate closed state. Cysteine substitutions at positions 40 or 41, shown by SCAM not to be exposed to the pore, had no effect on loop-gating. A straightforward interpretation is that loop-gating causes the pore to narrow at least at positions 43 and 46, and perhaps 51.
For the Cx32 chimera, cysteine substitutions at positions 38 and 45 (but not positions 37, 39, 40, or 43) were accessible to 2-aminoethyl methanethiosulfonate hydrobromide (MTSEA)-biotin from either end of the pore when the channels were open, indicating that the former set were exposed to the lumen and the latter set were not. Stepwise reductions in unitary conductance were seen for positions 38 and 45 (as in the Kronengold et al. [2003] studies on pore-lining residues in Cx46), suggesting sequential modification of intrapore cysteines in different subunits. However, with loop-gate closing, cysteines at positions 40 and/or 43 could be linked by dibromobimane, suggesting that they became accessible to the lumen, at close proximity, with loop-gating closure. This suggested that loop-gating involved a rotation of this portion of the TM1/EL1 structure, placing positions 40 and 43 into the pore. This was supported by studies showing that cysteine substitutions at 43 were able to bind Cd2+ with high affinity and form disulfide linkages with loop-gating. Under the same conditions, cysteine at position 40 could bind Cd2+, but at lower affinity. Small and reversible effects were seen for Cd2+ at positions 38 and 45, suggesting little change with loop-gating, and cysteines at positions 37 and 39 were not modified under any of the conditions.
These two studies identified the TM1/EL1 region as involved in the dynamics of loop-gating. While Tang et al. (2009) was in press, the first x-ray crystal structure of a connexin was published (Maeda et al., 2009). In an Appendix to Tang et al. (2009), the findings of both papers were discussed in the context of the new structural information. Drawing on the structure showing a bend at the TM1/EL1 transition at about position 43, and an apparent loss of α-helicity in the following segment (positions 43–48 and perhaps extending to 51, soon to be called the “parahelix”), it was suggested that rather than a rotation, loop-gating could involve a relaxation of the TM1/EL1 bend, which could constrict the extracellular entrance of the channel pore and alter the helical periodicity of the TM1/EL1 segment sufficiently to put positions 40 and 43 into the pore.
In 2012, an MD study characterized the fluctuations (in the open state) of the parahelix, its interactions, and the TM1/EL1 bend (Kwon et al., 2012). This work showed that the parahelix was part of an intersubunit electrostatic network whose dynamic changes correlated with changes in the configuration of the parahelix. Fluctuations in the network and parahelix affected the stability of the TM1/EL1 bend angle. It was suggested that the electrostatic network was the voltage sensor for loop-gating, and that voltage-driven changes in the network determined the configuration of the parahelix (proposed as the physical gate) and also drove changes elsewhere in the protein, including the TM1/EL1 bend angle.
The implications of changes in the TM1/EL1 bend angle during loop-gating motivated studies to examine changes at the extreme intracellular and extracellular ends of the pore (Kwon et al., 2013). State-dependent formation of Cd2+-thiolate metal bridges was used to probe changes with loop-gating in the Cx32 chimeric channel. Cysteine substitutions were made at positions 108 and 109 in the CL, which were seen in MD-equilibrated structures to be at the cytoplasmic entrance to the pore, outside the membrane-spanning domains. In these mutants, Cd2+ stabilized the loop-closed state but had no effect on the open channels. The effect of Cd2+ on the 109C mutant could be lessened if the channel was first exposed to MTSEA. From the coordination distances, it was estimated that loop-gating narrowed the pore diameter at these positions from ∼15 to ∼10 Å, which would be insufficient to occlude the pore. Notably, Cd2+ had no effect in response to Vj-gating. Similar studies were performed with cysteine substitution at position 56 in EL1, shown in the MD simulations to be at the furthest extracellular pore-facing position. No effects of Cd2+ were seen under conditions of loop-gating or Vj-gating. It was a negative result but suggested that this portion of the protein either does not narrow with loop-gating or does not narrow sufficiently for Cd2+ coordination.
Taken together with the previous work showing that loop-gating caused narrowing in the region of positions 43 up to position 47 and possibly to position 51 (these position numbers are approximate because of slight numbering differences in the two connexins), it was proposed that loop-gating narrowed but did not occlude the cytoplasmic pore entrance as a result of a straightening of the TM1/EL1 bend angle.
Putting (most of) it together
In 2013, two papers from different groups were published back to back in JGP, each addressing the fundamentals of how Ca2+ interacted with the Cx26 hemichannel to favor the closed state (Lopez et al., 2013; Sanchez et al., 2013). Both studies targeted mutations at D50, which cause syndromic deafness in humans, as a starting point. Mutations at this site in the parahelix reduce the ability of extracellular Ca2+ to keep the hemichannels closed, which is no doubt the proximate cause of the human pathology. In these papers, similar, but different, experimental strategies were used to investigate how and why mutations at this position compromised Ca2+ sensitivity. The big picture emerging from these studies was largely consistent, though there were specific areas of disagreement.
Both studies found that a negative charge at position 50 was required for full sensitivity to Ca2+. Both studies suggested that D50 was not necessarily part of the binding site for Ca2+, but was intimately involved in its effects. Both studies suggested that Ca2+ interaction with the channel resulted in disruption of specific electrostatic interactions involving D50, and that these disruptions destabilized the open state—but that the specific disrupted interactions were different (D50–K61 for Lopez et al. and D50–Q48 for Sanchez et al.). The different conclusions on this score were based on different sets of mutations, different ways of dealing with the optimal but nonfunctional mutants, and double mutant cycle analyses using different parameters of the effects of the mutations. Later work by Lopez et al. (2014) indicated a D50–Q48 interaction that stabilized the open state, but it was relatively insensitive to Ca2+.
Each study had unique aspects and conclusions. The kinetic data in Lopez et al. (2013) suggested that Ca2+ not only interacts with open channels to destabilize the open state, but also interacts with closed channels to stabilize the closed state. Activity of the channels was determined from peak tail currents following a depolarizing step to a single voltage maintained long enough for activation to reach steady state, and the mutant cycle analysis was performed on directly observable apparent Ca2+ affinities and rate constants. In Sanchez et al. (2013), the effects of cysteine substitution at position 48 indicated that the EL1 region was remarkably flexible, and that this could factor into control of several aspects of channel function. This work included studies of single channels as well as macroscopic currents, which showed that the transitions being affected were indeed loop-gating transitions, and also that D50 was pore lining. It also characterized the Ca2+ sensitivity of the mutants as a function of voltage. Both papers noted that further studies were needed to fully explain all the observations. Nevertheless, these papers make the essential point that Ca2+ induces a change or reorganization of interactions in the parahelix region to favor the loop-gate closed state of the channel.
In early 2016, Yeager and colleagues (Bennett et al., 2016) published x-ray crystal structures of Cx26 junctional channels in the presence and absence of bound Ca2+, with resolution that matched or exceeded that of the previous structure (Maeda et al., 2009), which did not have Ca2+ bound. These structures were largely consistent with the 2009 structure, but showed that the bound Ca2+ did not induce a major structural change in the pore. There was a modest main chain rearrangement, in which the backbone between residues W44 and Q48 (the parahelix, again) shifted an average of 0.8 Å toward the pore center; the biggest changes with Ca2+ coordination were side-chain reorientations. The Ca2+ was coordinated by the carboxylate of E47 and the carbonyl oxygen of G45 of one subunit, and the carboxylate of E42 in the adjacent subunit. The carboxylates of the glutamate residues act as bidentate ligands. The resulting hemispheric coordination of the Ca2+ leaves it exposed to the aqueous channel. Electrostatic and MD computations showed that the effect of this was to dramatically alter the electrostatic environment within the pore such that cations were effectively excluded, creating an electrostatic rather than steric barrier.
Unsurprisingly, these structures answered several questions and generated several more: The site of Ca2+ coordination is within the parahelix region, at the TM1/EL1 border, the same region that previous studies indicated was intimately involved in loop gating and Ca2+ regulation. The structure solved was that of a junctional channel (two docked hemichannels), whereas nearly all the work on loop-gating and Ca2+ regulation was on hemichannels. One does not know whether or how docking of hemichannels may affect Ca2+ coordination or its consequences; definitive answers require analogous structural solutions of undocked hemichannels, which are no doubt being pursued. It should be mentioned, however, that MD simulations of the hemichannel portion of the Maeda et al. (2009) structure (thought to be of a docked hemichannel) showed almost no change in the structure or configurations of the extracellular loops (Kwon et al., 2011).
In further examination of the effects of Ca2+, Lopez et al. (2016) published work in PNAS that explored the parahelix electrostatic network idea presented in Kwon et al. (2012) in the context of Ca2+ regulation of loop-gating. In an MD simulation, several Ca2+ ions were placed near the extracellular end of the channel, and the system was allowed to run for 50 ns. Even in this short simulation, interaction of the channel with two Ca2+ ions resulted in a number of rearrangements in the electrostatic network involving the parahelix and surrounding regions. These changes involved several subunits and extended deeper into the pore than the sites of Ca2+ coordination. The simulations suggested specific sites at which mutations might affect the process, which were experimentally tested. Some of the mutations produced kinetic changes and others eliminated Ca2+ dependence. Together, the results suggested that the effect of Ca2+ on hemichannel closing is via relative destabilization of the open state involving disruption of (likely electrostatic) interactions of specific residues. The data suggest that the electrostatic interactions of residues D50 and E47 in Cx26, and the corresponding residues D51 and E48 in Cx46, are major contributors to the stability of the closed state induced by interaction with Ca2+. Further studies were performed to assess whether the site of Ca2+ coordination was the physical gate. It was found that MTSES and Cd2+ had extracellular access to residue 45, when the Ca2+-activated gate was closed and ionic conduction eliminated. This suggests that the physical loop-gate is below position 45, and is not at the level of Ca2+ binding.
In the loop-gating narrative thus far, one would be forgiven for thinking that Ca2+ regulation is nicely confined to the parahelix and nearby regions. However, it has been known for some time that several point mutations in the NT domain, at the other end of the channel, produce aberrant hemichannel activity. One of these mutations, N14K, was studied in detail by Sanchez et al. (2016) in JGP. This mutation essentially eliminated the voltage sensitivity of loop-gating at low Ca2+ with no effect on Vj-gating (or on unitary conductance). The results were interpreted to indicate that the mutation causes relative destabilization of the loop-gate closed state. Curiously, the channels remained normally sensitive to regulation by Ca2+. Based on the crystal structures, an interaction between N14K and a specific residue at the TM2/CL border (H100) was assessed. The double mutant N14K + H100A restored the voltage sensitivity of loop-gating. This suggested that the effects of the N14K mutation to eliminate the voltage sensitivity of loop-gating (at low Ca2+) and/or destabilize the loop-gate closed state were mediated by an interdomain interaction involving N14K and H100.
The main import of this study is that it shows that loop-gating can be modulated by changes at the cytoplasmic end of the channel, and may involve NT–CL interactions. The former point is consistent with the study of Kwon et al. (2013) above suggesting that loop-gating narrows at the cytoplasmic end of the pore, and with the study of Rubinos et al. (2012) indicating that BQ+ affected loop-gating at a site near the cytoplasmic entrance.
In a recent paper, García et al. (2018) studied the mutation G12R in Cx26, a different NT gain-of-function mutation. This mutation essentially eliminated gating at large positive voltages, which is mediated by the Vj-gating process in this channel. Loop-gating retained normal sensitivity to Ca2+ but accelerated the deactivation kinetics in Ca2+ and rendered them insensitive to Ca2+ (they normally increase as Ca2+ is reduced). Based on MD simulations of the mutant channel, an interaction between G12R and R99 at the TM2/CL border (adjacent to H100 studied in Sanchez et al. [2016]) was investigated. The double mutant G12R + R99K did not appear to affect channel function, but the double mutant G12R + R99A recovered the wild-type single channel and macroscopic gating behavior at positive voltages. Curiously, the R99A mutation by itself appeared to increase gating at large positive voltages (it is unclear if this was Vj-gating). The inference drawn from the data is that interaction between G12R and R99 (perhaps via guanidinium group stacking) keeps the NT away from the mouth of the pore, thereby disabling Vj-gating. It is unclear why the R99K mutation would not disrupt that interaction. An effect on the deactivation kinetics of loop-gating was not evident for the G12R + R99 mutants, which suggests that the effects of G12R on Vj-gating and loop-gating kinetics are separable. This paper supports that position 12 is involved in Vj-gating and can affect the Ca2+ sensitivity of closing of the loop-gate, and suggests that NT–CL interactions can play a role in the former.
In G12R, the full closures in single-channel records at positive voltages do not have the kinetic hallmarks of loop-gating transitions, which take place over tens of milliseconds; the transitions are rapid, characteristic of Vj-gating, but not to a substate. Charge changes in the NT up to position 10 have been shown to reverse the polarity of Vj-gating in Cx32 (Purnick et al., 2000); if the G12R mutation did so, it could explain the apparent absence of Vj-gating at large positive potentials. Because both Vj-gating and loop-gating operate on the same open state, a mutation that lowers the energy of the open state would shift the G-V relations of both loop-gating and Vj-gating to the right, but not necessarily to the same degree, because the dominant energy barrier to each closed state is likely different. The voltage dependence of loop-gating was not examined directly; such a rightward shift could contribute to the reduced macroscopic conductance of G12R relative to wild type at moderate positive potentials. In any case, the G12R mutation may affect several aspects of channel function, and its effects at large positive voltages may involve interaction with the CL, but how or why it exerts its other effects remain to be elucidated. The integrated structural and energetic complexities presented by this channel inspire both creativity and caution.
Back to the future
This article began with the basics of electrical coupling and what it might mean for signaling, mostly among neurons. I would like to end it with an article in JGP that shows just how far we have come in that regard. This is the work of Feliksas Bukauskas and colleagues (Maciunas et al., 2016). It pulls together all that has been learned about voltage-gated behavior in junctional channels and applies it to a 2-D network of coupled cells whose excitability is explicitly described by the Hodgkin–Huxley equations. The spread of excitation is interrogated for the effects of different patterns of intrinsic activity and junctional channels of different types. The modeling of the junctional channels includes the single-channel behavior of two loop-gates that fully close the channel and two Vj-gates that close the channel to a substate, in series in each junctional channel, the effects of each gate’s activity on the voltage sensed by the others, and the observed rectification of single hemichannel currents. This model was developed and validated over several iterations of increasing complexity and has continued to be refined in subsequent publications (Snipas et al., 2016, 2017).
For all phenomena studied, the effects of greater or lesser degrees of junctional voltage dependence were examined, using Cx45 parameters for the former and Cx36 parameters for the latter, as well as different sites of coupling (somata–somata vs. dendro–dendritic). It was found that with action potential bursting there can be accumulating decreases in junctional conductance because of the voltage dependence of the junctions, which can have a multitude of specific effects on patterns of activity. The process by which phase-shifting effects caused by coupling can lead to firing synchrony when cells in the network have different intrinsic patterns of firing was examined. Determinants of action potential synchrony were analyzed for how they were affected by junctional conductance, size of firing clusters, intrinsic firing rates, etc. It was found that even a slight and local rectification of junctional channel I-V in the network could cause strong unidirectional action potential transmission, and eventually lead to reverberation of firing. The conditions for generation of reverberating action potentials were described, as were conditions for its initiation and termination.
This paper provides quantitative validation and understanding of phenomena seen in early studies of electrical coupling, particularly regarding action potential propagation, phase shifting, development of synchrony, and the low levels of initial coupling needed for these effects (compare Watanabe and Bullock, 1960; Eckert, 1963; Bennett, 1966; Clapham et al., 1980; Rohr and Salzberg, 1994; Verheijck et al., 1998; Lin et al., 2005). An extensive discussion relates the findings to what is known about diverse coupled neuronal systems, drawing on the experimental work performed by the senior author and others over several decades. This paper reflects an integration of physiological and modeling insights derived from extensive work in experiment and theory, and insistence that each answer to the other, as stated at the beginning of this review.
Conclusion
The physiology of electrical coupling of excitable cells has been quantitatively analyzed and extensively studied. The fundamental process is well understood, and the functional and dynamic roles of this coupling in various systems remain active areas of inquiry. At this point in time, understanding of the molecular and energetic mechanisms of gating, molecular permeation, and modulation of connexin channels is still incomplete. One hopes that with the application of increasingly sophisticated biophysical, structural, and computational approaches, further and unique insights will be gained. No doubt the answers for these unusual wide pores will be both intriguing and informative.

Acknowledgments
By rights this article should be dedicated exclusively to my mentor Michael Bennett, but I am sure he will not mind sharing this appreciation with the late Feliksas Bukauskas. Mike had a seminal role in the development of the field, from its early days to the present, as it evolved across many disciplinary lines on its way from the ephapse to molecular thermodynamics. He provided to the field an expectation of high standards and thorough reasoning that could stand up to informed challenge. He attracted to the Dominic Purpura Department of Neuroscience at Albert Einstein College of Medicine many of the key contributors to the field (and to JGP), including David Spray, Vytas Verselis, Ted Bargiello, and Feliks Bukauskas. Mike has an exceedingly informed, deep, and broad biological perspective. He insists on quantitative rigor, but more strikingly, insightful qualitative rigor in exploring the natural world. Feliks, simply put, was one of the good guys. He was an extraordinary, creative, and productive scientist, in the best Old World tradition. Unfortunately, these qualities tend to be undervalued in today’s increasingly corporatist university culture. Feliks was unfailingly thoughtful and considerate, without an ounce of self promotion. He is greatly missed by all who knew him. See photo: Mike Bennett (left) and Feliks Bukauskas (right). Left photo from Pereda et al. (2013), reprinted with permission from Neuron. Right photo reproduced with permission of the Bukauskas family.
This article has benefited from decades-long relationships with colleagues inside and outside the connexin field, of which there are too many to name. I am grateful for those relationships, which include many of the people mentioned in this article, particularly those investigators who shaped the field in the “early years,” when the gap junction world was new and connexins were undiscovered. These include (aside from Mike Bennett and the others at Einstein), in roughly chronological order, Ted Bullock, who taught my first neurophysiology class, Susumu Hagiwara, Roger Eckert, Harry Grundfest, Mahlon Kriebel, Joel Brown, Jean-Paul Revel, Dan Goodenough, Brian Salzberg, Bernie Gilula, Peter Brink, Bob DeHaan, Harry Fozzard, Rick Mathias, and Brian Payton (whom I never met, but with whom I had an entertaining email correspondence a few years ago). In reading the older literature, I was struck by how different scientific writing was then compared to now. It was more like narrative storytelling than the impersonal, nearly telegraphic exposition that dominates today. The former enabled the reader to be carried along in the thought processes of investigators as they puzzled their way through a problem. This window into the process of discovery and the development of insight was integral to the scientific discourse. One wonders if as a result, today’s younger scientific readers are missing something important about how to approach the surprising and the unknown.
The writing of this article was partially supported by National Institutes of Health grant R01GM101950.
The author declares no competing financial interests.
Olaf S. Andersen served as editor.
Footnotes
One may find it interesting to note that the author of this sentence, and the insightful article about electric fish that contains it, was not a scientist, but a female journalist soon to be a successful novelist, writing what was called “realistic fiction,” with titles such as “A Righteous Apostate,” “Basil Morton's Transgression,” and “A Modern Marriage.”
The technique of replacing a damaged/nonconductive segment by an electrically conductive pathway was first published in JGP by Osterhout and Hill (1930), using the alga Nitella.
The two terms “electrotonic synapse/junction” and “electrical synapse” do not denote a distinction. In early years, the former was promoted by Bennett in opposition to the term “ephapse” used by his postdoctoral mentor Grundfest and to make explicit that the transmission occurred by passive spread of potential across the junction (Bennett, 2002).
A revealing narrative of how these observations came about is in Bennett (2000).
“It [‘ephapse’] would therefore differ from the word ‘synapse’ whose meaning is narrower and designates surfaces of contact…anatomically differentiated and functionally specialized for the transmission of the liminal excitations from one element to the following in an irreciprocal direction.”
The Katz and Schmitt (1940) article pointed out that such interactions “are entirely consistent with the ‘local circuit’ theory of nervous conduction as proposed by Hermann and Cremer, and recently confirmed by Hodgkin and others.”
A key technical innovation required to make the measurements was use of “a pencil type coaxial superfine microelectrode,” consisting of two coaxial glass microelectrodes, one inside the other, in which the internal electrode could be advanced in front of the other so that resistance between the two tips separated by known distances could be recorded (Tomita and Kaneko, 1965).
Consideration of the latter may have inspired an intriguing and unique analysis of the relations among shape, time course, and magnitude of potentials on action potential initiation (Bennett et al., 1970).
It may interest some readers to know that the corresponding author of this paper, and of another paper the same year cited previously (Payton et al., 1969a), Brian Payton, went on to become well known in certain circles as a cultural twin of the Monty Python brand of satire, as applied to physiology. His most well-known production is the film “Proprioceptive Receptor Potentials of Oscillatory Form” (https://www.youtube.com/watch?v=NWvF4-1wztM), a collaboration with John Nicholls, as was “Goodbye Mr. Ipecac.” He was also responsible for the instructional film “An Artificial Lung and Thorax Model or the Smoking Condom” and a number of exciting and memorable “demonstrations” of key concepts in medical physiology for the benefit of students at the Medical School of Memorial University of Newfoundland.
EIM was a bacterial protein shown in the early 1960s to impart a voltage-dependent macroscopic conductance to planar bilayers (Mueller et al., 1962). In 1969, JGP published stepwise increasing conductances produced by EIM in bilayers, providing clear evidence it was a channel and not a carrier (Bean et al., 1969). It remains unidentified but is probably a porin.
At the time, it was actively debated—in the pages of JGP—whether the action potential was produced by a single type of channel with voltage-driven changes in selectivity (Mullins, 1959, 1960, 1968a,b; Sjodin and Mullins, 1967; Narahashi and Moore, 1968) and/or whether the voltage changes of the action potential resulted from a voltage-driven ion-exchange process within a fundamentally nonselective but charged membrane (Tasaki and Hagiwara, 1957; Tasaki, 1963; Tasaki et al., 1965, 1967).
References
- Akabas M.H., Stauffer D.A., Xu M., and Karlin A.. 1992. Acetylcholine receptor channel structure probed in cysteine-substitution mutants. Science. 258:307–310. 10.1126/science.1384130 [DOI] [PubMed] [Google Scholar]
- Arvanitaki A. 1942. Effects evoked in an axon by the activity of a contiguous one. J. Neurophysiol. 5:89–108. 10.1152/jn.1942.5.2.89 [DOI] [Google Scholar]
- Arvanitaki A., and Chalazonitis N.. 1959. [Electrical interactions between the giant soma A and the immediately contiguous somata (pleuro-branchial ganglion of Aplysia ] Bull. Inst. Oceanogr. (1143):1–30. [Google Scholar]
- Auerbach A.A., and Bennett M.V.L.. 1969. A rectifying electrotonic synapse in the central nervous system of a vertebrate. J. Gen. Physiol. 53:211–237. 10.1085/jgp.53.2.211 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Baldo G.J., Gong X., Martinez-Wittinghan F.J., Kumar N.M., Gilula N.B., and Mathias R.T.. 2001. Gap junctional coupling in lenses from alpha(8) connexin knockout mice. J. Gen. Physiol. 118:447–456. 10.1085/jgp.118.5.447 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Bargiello T.A., Oh S., Tang Q., Bargiello N.K., Dowd T.L., and Kwon T.. 2018. Gating of Connexin Channels by transjunctional-voltage: Conformations and models of open and closed states. Biochim Biophys Acta Biomembr. 1860:22–39. 10.1016/j.bbamem.2017.04.028 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Barr L., Dewey M.M., and Berger W.. 1965. Propagation of action potentials and the structure of the nexus in cardiac muscle. J. Gen. Physiol. 48:797–823. 10.1085/jgp.48.5.797 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Barr L., Berger W., and Dewey M.M.. 1968. Electrical transmission at the nexus between smooth muscle cells. J. Gen. Physiol. 51:347–368. 10.1085/jgp.51.3.347 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Barrio L.C., Suchyna T., Bargiello T., Xu L.X., Roginski R.S., Bennett M.V.L., and Nicholson B.J.. 1991. Gap junctions formed by connexins 26 and 32 alone and in combination are differently affected by applied voltage. Proc. Natl. Acad. Sci. USA. 88:8410–8414. 10.1073/pnas.88.19.8410 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Bean R.C., Shepherd W.C., Chan H., and Eichner J.. 1969. Discrete conductance fluctuations in lipid bilayer protein membranes. J. Gen. Physiol. 53:741–757. 10.1085/jgp.53.6.741 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Beblo D.A., and Veenstra R.D.. 1997. Monovalent cation permeation through the connexin40 gap junction channel. Cs, Rb, K, Na, Li, TEA, TMA, TBA, and effects of anions Br, Cl, F, acetate, aspartate, glutamate, and NO3. J. Gen. Physiol. 109:509–522. 10.1085/jgp.109.4.509 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Belousov A.B., Fontes J.D., Freitas-Andrade M., and Naus C.C.. 2017. Gap junctions and hemichannels: communicating cell death in neurodevelopment and disease. BMC Cell Biol. 18(S1, Suppl 1):4 10.1186/s12860-016-0120-x [DOI] [PMC free article] [PubMed] [Google Scholar]
- Bennett B.C., Purdy M.D., Baker K.A., Acharya C., McIntire W.E., Stevens R.C., Zhang Q., Harris A.L., Abagyan R., and Yeager M.. 2016. An electrostatic mechanism for Ca(2+)-mediated regulation of gap junction channels. Nat. Commun. 7:8770 10.1038/ncomms9770 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Bennett M.R. 1967. The effect of intracellular current pulses in smooth muscle cells of the guinea pig vas deferens at rest and during transmission. J. Gen. Physiol. 50:2459–2475. 10.1085/jgp.50.10.2459 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Bennett M.V.L. 1960. Electrical connections between supramedullary neurons. Fed. Proc. 19:282. [Google Scholar]
- Bennett M.V.L. 1966. Physiology of electrotonic junctions. Ann. N. Y. Acad. Sci. 137(2 Biological Me):509–539. 10.1111/j.1749-6632.1966.tb50178.x [DOI] [PubMed] [Google Scholar]
- Bennett M.V.L. 1985. Nicked by Occam’s razor: Unitarianism in the investigation of synaptic transmission. Biol. Bull. 168(3S):159–167. 10.2307/1541329 [DOI] [Google Scholar]
- Bennett M.V.L. 1997. Gap junctions as electrical synapses. J. Neurocytol. 26:349–366. 10.1023/A:1018560803261 [DOI] [PubMed] [Google Scholar]
- Bennett M.V.L. 2000. Electrical synapses, a personal perspective (or history). Brain Res. Brain Res. Rev. 32:16–28. 10.1016/S0165-0173(99)00065-X [DOI] [PubMed] [Google Scholar]
- Bennett M.V.L. 2002. Neoreticularism and neuronal polarization. Prog. Brain Res. 136:189–201. 10.1016/S0079-6123(02)36017-5 [DOI] [PubMed] [Google Scholar]
- Bennett M.V.L., and Dunham P.B.. 1970. Sucrose permeability of junctional membrane at an electrotonic synapse. Biophys. Soc. Ann. Mtg. Abstr. 14:114A. [Google Scholar]
- Bennett M.V.L., and Zukin R.S.. 2004. Electrical coupling and neuronal synchronization in the Mammalian brain. Neuron. 41:495–511. 10.1016/S0896-6273(04)00043-1 [DOI] [PubMed] [Google Scholar]
- Bennett M.V.L., Crain S.M., and Grundfest H.. 1959. Electrophysiology of supramedullary neurons in Spheroides maculatus. III. Organization of the supramedullary neurons. J. Gen. Physiol. 43:221–250. 10.1085/jgp.43.1.221 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Bennett M.V.L., Aljure E., Nakajima Y., and Pappas G.D.. 1963. Electrotonic junctions between teleost spinal neurons: electrophysiology and ultrastructure. Science. 141:262–264. 10.1126/science.141.3577.262 [DOI] [PubMed] [Google Scholar]
- Bennett M.V.L., Hille B., and Obara S.. 1970. Voltage threshold in excitable cells depends on stimulus form. J. Neurophysiol. 33:585–594. 10.1152/jn.1970.33.5.585 [DOI] [PubMed] [Google Scholar]
- Bevans C.G., and Harris A.L.. 1999. Regulation of connexin channels by pH. Direct action of the protonated form of taurine and other aminosulfonates. J. Biol. Chem. 274:3711–3719. 10.1074/jbc.274.6.3711 [DOI] [PubMed] [Google Scholar]
- Bevans C.G., Kordel M., Rhee S.K., and Harris A.L.. 1998. Isoform composition of connexin channels determines selectivity among second messengers and uncharged molecules. J. Biol. Chem. 273:2808–2816. 10.1074/jbc.273.5.2808 [DOI] [PubMed] [Google Scholar]
- Beyer E.C., and Berthoud V.M.. 2017. Gap junction structure: unraveled, but not fully revealed. F1000 Res. 6:568 10.12688/f1000research.10490.1 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Bigiani A., and Roper S.D.. 1993. Identification of electrophysiologically distinct cell subpopulations in Necturus taste buds. J. Gen. Physiol. 102:143–170. 10.1085/jgp.102.1.143 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Bigiani A., and Roper S.D.. 1995. Estimation of the junctional resistance between electrically coupled receptor cells in Necturus taste buds. J. Gen. Physiol. 106:705–725. 10.1085/jgp.106.4.705 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Bourne G.H. 1953. Enzymes of the intercalated disks of heart muscle fibres. Nature. 172:588–589. 10.1038/172588a0 [DOI] [PubMed] [Google Scholar]
- Brightman M.W., and Reese T.S.. 1969. Junctions between intimately apposed cell membranes in the vertebrate brain. J. Cell Biol. 40:648–677. 10.1083/jcb.40.3.648 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Brink P., and Barr L.. 1977. The resistance of the septum of the median giant axon of the earthworm. J. Gen. Physiol. 69:517–536. 10.1085/jgp.69.5.517 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Brink P.R., and Dewey M.M.. 1978. Nexal membrane permeability to anions. J. Gen. Physiol. 72:67–86. 10.1085/jgp.72.1.67 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Brink P.R., Mathias R.T., Jaslove S.W., and Baldo G.J.. 1988. Steady-state current flow through gap junctions. Effects on intracellular ion concentrations and fluid movement. Biophys. J. 53:795–807. 10.1016/S0006-3495(88)83159-X [DOI] [PMC free article] [PubMed] [Google Scholar]
- Buehler L.K., Stauffer K.A., Gilula N.B., and Kumar N.M.. 1995. Single channel behavior of recombinant beta 2 gap junction connexons reconstituted into planar lipid bilayers. Biophys. J. 68:1767–1775. 10.1016/S0006-3495(95)80353-X [DOI] [PMC free article] [PubMed] [Google Scholar]
- Bukauskas F.F., and Verselis V.K.. 2004. Gap junction channel gating. Biochim. Biophys. Acta. 1662:42–60. 10.1016/j.bbamem.2004.01.008 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Bukauskas F.F., and Weingart R.. 1994. Voltage-dependent gating of single gap junction channels in an insect cell line. Biophys. J. 67:613–625. 10.1016/S0006-3495(94)80521-1 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Bukauskas F.F., Elfgang C., Willecke K., and Weingart R.. 1995. Heterotypic gap junction channels (connexin26-connexin32) violate the paradigm of unitary conductance. Pflugers Arch. 429:870–872. 10.1007/BF00374812 [DOI] [PubMed] [Google Scholar]
- Bukauskas F.F., Bukauskiene A., and Verselis V.K.. 2002. Conductance and permeability of the residual state of connexin43 gap junction channels. J. Gen. Physiol. 119:171–185. 10.1085/jgp.119.2.171 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Bullock T.H. 1945. Functional organization of the giant fiber system of Lumbricus. J. Neurophysiol. 8:55–71. 10.1152/jn.1945.8.1.55 [DOI] [Google Scholar]
- Bullock T.H. 1958. Parameters of integrative action of the nervous system at the neuronal level. Exp. Cell Res. 14(Suppl 5):323–337. [PubMed] [Google Scholar]
- Chalazonitis N., and Arvanitaki-Chalazonitis A.. 1958. [Simultaneous Intracellular recording of simultaneous activity of different neurons in situ ] C. R. Hebd. Seances Acad. Sci. 246:161–163. [PubMed] [Google Scholar]
- Chanson M., Chandross K.J., Rook M.B., Kessler J.A., and Spray D.C.. 1993. Gating characteristics of a steeply voltage-dependent gap junction channel in rat Schwann cells. J. Gen. Physiol. 102:925–946. 10.1085/jgp.102.5.925 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Chen D., and Eisenberg R.. 1993. Charges, currents, and potentials in ionic channels of one conformation. Biophys. J. 64:1405–1421. 10.1016/S0006-3495(93)81507-8 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Chen Y.H., and DeHaan R.L.. 1993. Temperature dependence of embryonic cardiac gap junction conductance and channel kinetics. J. Membr. Biol. 136:125–134. 10.1007/BF02505757 [DOI] [PubMed] [Google Scholar]
- Chen D., Lear J., and Eisenberg B.. 1997. Permeation through an open channel: Poisson-Nernst-Planck theory of a synthetic ionic channel. Biophys. J. 72:97–116. 10.1016/S0006-3495(97)78650-8 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Clapham D.E., Shrier A., and DeHaan R.L.. 1980. Junctional resistance and action potential delay between embryonic heart cell aggregates. J. Gen. Physiol. 75:633–654. 10.1085/jgp.75.6.633 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Connors BW. 2017. Synchrony and so much more: Diverse roles for electrical synapses in neural circuits. Dev. Neurobiol. 77 (Special Issue: SI):610-624. [DOI] [PMC free article] [PubMed]
- Curtis H.J., and Travis D.M.. 1951. Conduction in Purkinje tissue of the ox heart. Am. J. Physiol. 165:173–178. [DOI] [PubMed] [Google Scholar]
- Dahl G., Miller T., Paul D., Voellmy R., and Werner R.. 1987. Expression of functional cell-cell channels from cloned rat liver gap junction complementary DNA. Science. 236:1290–1293. 10.1126/science.3035715 [DOI] [PubMed] [Google Scholar]
- Dehaan R.L., and Fozzard H.A.. 1975. Membrane response to current pulses in spheroidal aggregates of embryonic heart cells. J. Gen. Physiol. 65:207–222. 10.1085/jgp.65.2.207 [DOI] [PMC free article] [PubMed] [Google Scholar]
- DeHaan R.L., and Gottlieb S.H.. 1968. The electrical activity of embryonic chick heart cells isolated in tissue culture singly or in interconnected cell sheets. J. Gen. Physiol. 52:643–665. 10.1085/jgp.52.4.643 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Delmar M., Laird D.W., Naus C.C., Nielsen M.S., Verselis V.K., and White T.W.. 2018. Connexins and Disease. Cold Spring Harb. Perspect. Biol. 10:a029348 10.1101/cshperspect.a029348 [DOI] [PMC free article] [PubMed] [Google Scholar]
- DeRosa A.M., Mui R., Srinivas M., and White T.W.. 2006. Functional characterization of a naturally occurring Cx50 truncation. Invest. Ophthalmol. Vis. Sci. 47:4474–4481. 10.1167/iovs.05-1582 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Derouette J.P., Desplantez T., Wong C.W., Roth I., Kwak B.R., and Weingart R.. 2009. Functional differences between human Cx37 polymorphic hemichannels. J. Mol. Cell. Cardiol. 46:499–507. 10.1016/j.yjmcc.2008.12.018 [DOI] [PubMed] [Google Scholar]
- DeVries S.H., and Schwartz E.A.. 1992. Hemi-gap-junction channels in solitary horizontal cells of the catfish retina. J. Physiol. 445:201–230. 10.1113/jphysiol.1992.sp018920 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Dewey M.M. 1965. The anatomical basis of propagation in smooth muscle. Gastroenterology. 49:395–402. [PubMed] [Google Scholar]
- Dewey M.M., and Barr L.. 1962. Intercellular connection between smooth muscle cells: The nexus. Science. 137:670–672. 10.1126/science.137.3531.670-a [DOI] [PubMed] [Google Scholar]
- Ebihara L., and Steiner E.. 1993. Properties of a nonjunctional current expressed from a rat connexin46 cDNA in Xenopus oocytes. J. Gen. Physiol. 102:59–74. 10.1085/jgp.102.1.59 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Ebihara L., Liu X., and Pal J.D.. 2003. Effect of external magnesium and calcium on human connexin46 hemichannels. Biophys. J. 84:277–286. 10.1016/S0006-3495(03)74848-6 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Eckert R. 1963. Electrical interaction of paired ganglion cells in the leech. J. Gen. Physiol. 46:573–587. 10.1085/jgp.46.3.573 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Eckert R. 2002. pH gating of lens fibre connexins. Pflugers Arch. 443:843–851. 10.1007/s00424-001-0760-2 [DOI] [PubMed] [Google Scholar]
- Eghbali B., Kessler J.A., and Spray D.C.. 1990. Expression of gap junction channels in communication-incompetent cells after stable transfection with cDNA encoding connexin 32. Proc. Natl. Acad. Sci. USA. 87:1328–1331. 10.1073/pnas.87.4.1328 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Ehrenstein G., and Lecar H.. 1977. Electrically gated ionic channels in lipid bilayers. Q. Rev. Biophys. 10:1–34. 10.1017/S0033583500000123 [DOI] [PubMed] [Google Scholar]
- Ehrenstein G., Lecar H., and Nossal R.. 1970. The nature of the negative resistance in bimolecular lipid membranes containing excitability-inducing material. J. Gen. Physiol. 55:119–133. 10.1085/jgp.55.1.119 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Ehrenstein G., Blumenthal R., Latorre R., and Lecar H.. 1974. Kinetics of the opening and closing of individual excitability-inducing material channels in a lipid bilayer. J. Gen. Physiol. 63:707–721. 10.1085/jgp.63.6.707 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Eisenman G. 1962. Cation selective glass electrodes and their mode of operation. Biophys. J. 2:259–323. 10.1016/S0006-3495(62)86959-8 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Eisenman G., and Horn R.. 1983. Ionic selectivity revisited: the role of kinetic and equilibrium processes in ion permeation through channels. J. Membr. Biol. 76:197–225. 10.1007/BF01870364 [DOI] [PubMed] [Google Scholar]
- Ek J.F., Delmar M., Perzova R., and Taffet S.M.. 1994. Role of histidine 95 on pH gating of the cardiac gap junction protein connexin43. Circ. Res. 74:1058–1064. 10.1161/01.RES.74.6.1058 [DOI] [PubMed] [Google Scholar]
- Engelmann T.W. 1875. Ueber die Leitung der Erregung im Herzmuskel. Pflugers Arch. 11:465–480 (On the direction of excitement in heart muscle) 10.1007/BF01659313 [DOI] [Google Scholar]
- Engelmann T.W. 1877. Vergleichende Untersuchungen zur Lehre von der Muskel- und Nervenelektricitat. Pflugers Arch. 15:116–148 (Comparative investigation of muscle and nerve electricity) 10.1007/BF01628342 [DOI] [Google Scholar]
- Franciolini F., and Nonner W.. 1987. Anion and cation permeability of a chloride channel in rat hippocampal neurons. J. Gen. Physiol. 90:453–478. 10.1085/jgp.90.4.453 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Franciolini F., and Nonner W.. 1994. Anion-cation interactions in the pore of neuronal background chloride channels. J. Gen. Physiol. 104:711–723. 10.1085/jgp.104.4.711 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Freygang W.H., and Trautwein W.. 1970. The structural implications of the linear electrical properties of cardiac Purkinje strands. J. Gen. Physiol. 55:524–547. 10.1085/jgp.55.4.524 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Furshpan E.J., and Potter D.D.. 1957. Mechanism of nerve-impulse transmission at a crayfish synapse. Nature. 180:342–343. 10.1038/180342a0 [DOI] [PubMed] [Google Scholar]
- Furshpan E.J., and Potter D.D.. 1959. Transmission at the giant motor synapses of the crayfish. J. Physiol. 145:289–325. 10.1113/jphysiol.1959.sp006143 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Gao J., Sun X., Martinez-Wittinghan F.J., Gong X., White T.W., and Mathias R.T.. 2004. Connections between connexins, calcium, and cataracts in the lens. J. Gen. Physiol. 124:289–300. 10.1085/jgp.200409121 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Gao J., Sun X., Moore L.C., White T.W., Brink P.R., and Mathias R.T.. 2011. Lens intracellular hydrostatic pressure is generated by the circulation of sodium and modulated by gap junction coupling. J. Gen. Physiol. 137:507–520. 10.1085/jgp.201010538 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Gao J., Sun X., White T.W., Delamere N.A., and Mathias R.T.. 2015. Feedback regulation of intracellular hydrostatic pressure in surface cells of the lens. Biophys. J. 109:1830–1839. 10.1016/j.bpj.2015.09.018 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Gao J., Minogue P.J., Beyer E.C., Mathias R.T., and Berthoud V.M.. 2018. Disruption of the lens circulation causes calcium accumulation and precipitates in connexin mutant mice. Am. J. Physiol. Cell Physiol. 314:C492–C503. 10.1152/ajpcell.00277.2017 [DOI] [PMC free article] [PubMed] [Google Scholar]
- García I.E., Prado P., Pupo A., Jara O., Rojas-Gómez D., Mujica P., Flores-Muñoz C., González-Casanova J., Soto-Riveros C., Pinto B.I., et al. 2016. Connexinopathies: a structural and functional glimpse. BMC Cell Biol. 17(S1, Suppl 1):17 10.1186/s12860-016-0092-x [DOI] [PMC free article] [PubMed] [Google Scholar]
- García I.E., Villanelo F., Contreras G.F., Pupo A., Pinto B.I., Contreras J.E., Pérez-Acle T., Alvarez O., Latorre R., Martínez A.D., and González C.. 2018. The syndromic deafness mutation G12R impairs fast and slow gating in Cx26 hemichannels. J. Gen. Physiol. 150:697–711. 10.1085/jgp.201711782 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Gerard R.W. 1941. The interaction of neurons. Ohio J. Sci. 41:160–172. [Google Scholar]
- Giaume C., and Korn H.. 1983. Bidirectional transmission at the rectifying electrotonic synapse: a voltage-dependent process. Science. 220:84–87. 10.1126/science.6298940 [DOI] [PubMed] [Google Scholar]
- Giaume C., Kado R.T., and Korn H.. 1987. Voltage-clamp analysis of a crayfish rectifying synapse. J. Physiol. 386:91–112. 10.1113/jphysiol.1987.sp016524 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Gilula N.B., Reeves O.R., and Steinbach A.. 1972. Metabolic coupling, ionic coupling and cell contacts. Nature. 235:262–265. 10.1038/235262a0 [DOI] [PubMed] [Google Scholar]
- Goldberg G.S., Lampe P.D., and Nicholson B.J.. 1999. Selective transfer of endogenous metabolites through gap junctions composed of different connexins. Nat. Cell Biol. 1:457–459. 10.1038/15693 [DOI] [PubMed] [Google Scholar]
- Goldberg G.S., Moreno A.P., and Lampe P.D.. 2002. Gap junctions between cells expressing connexin 43 or 32 show inverse permselectivity to adenosine and ATP. J. Biol. Chem. 277:36725–36730. 10.1074/jbc.M109797200 [DOI] [PubMed] [Google Scholar]
- Goodenough D.A., and Revel J.P.. 1970. A fine structural analysis of intercellular junctions in the mouse liver. J. Cell Biol. 45:272–290. 10.1083/jcb.45.2.272 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Hagiwara S., Watanabe A., and Saito N.. 1959. Potential changes in syncytial neurons of lobster cardiac ganglion. J. Neurophysiol. 22:554–572. 10.1152/jn.1959.22.5.554 [DOI] [PubMed] [Google Scholar]
- Harris A.L. 2001. Emerging issues of connexin channels: biophysics fills the gap. Q. Rev. Biophys. 34:325–472. 10.1017/S0033583501003705 [DOI] [PubMed] [Google Scholar]
- Harris A.L. 2007. Connexin channel permeability to cytoplasmic molecules. Prog. Biophys. Mol. Biol. 94:120–143. 10.1016/j.pbiomolbio.2007.03.011 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Harris A.L., and Contreras J.E.. 2014. Motifs in the permeation pathway of connexin channels mediate voltage and Ca (2+) sensing. Front. Physiol. 5:113 10.3389/fphys.2014.00113 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Harris A.L., and Locke D.. 2009. Permeability of Connexin Channels. In Connexins: A Guide. Harris A.L., and Locke D., editors. Humana-Springer, New York: 165–206. 10.1007/978-1-59745-489-6_7 [DOI] [Google Scholar]
- Harris A.L., Spray D.C., and Bennett M.V.L.. 1980. Temperature dependence of a voltage dependent junctional conductance. Soc. Neurosci. Abstr. 6:96. [Google Scholar]
- Harris A.L., Spray D.C., and Bennett M.V.L.. 1981. Kinetic properties of a voltage-dependent junctional conductance. J. Gen. Physiol. 77:95–117. 10.1085/jgp.77.1.95 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Harris A.L., Spray D.C., and Bennett M.V.L.. 1983. Control of intercellular communication by voltage dependence of gap junctional conductance. J. Neurosci. 3:79–100. 10.1523/JNEUROSCI.03-01-00079.1983 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Harris A.L., Walter A., Paul D., Goodenough D.A., and Zimmerberg J.. 1992. Ion channels in single bilayers induced by rat connexin32. Brain Res. Mol. Brain Res. 15:269–280. 10.1016/0169-328X(92)90118-U [DOI] [PubMed] [Google Scholar]
- Hering E. 1882. [On nerve–excitation by the nerve-current ] Sitzungsber. Kaiser. Akad. Wiss. 85:237–275 (). [Google Scholar]
- Hernandez V.H., Bortolozzi M., Pertegato V., Beltramello M., Giarin M., Zaccolo M., Pantano S., and Mammano F.. 2007. Unitary permeability of gap junction channels to second messengers measured by FRET microscopy. Nat. Methods. 4:353–358. 10.1038/nmeth1031 [DOI] [PubMed] [Google Scholar]
- Hu X., and Dahl G.. 1999. Exchange of conductance and gating properties between gap junction hemichannels. FEBS Lett. 451:113–117. 10.1016/S0014-5793(99)00558-X [DOI] [PubMed] [Google Scholar]
- Hu X., Ma M., and Dahl G.. 2006. Conductance of connexin hemichannels segregates with the first transmembrane segment. Biophys. J. 90:140–150. 10.1529/biophysj.105.066373 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Ito S., and Hori N.. 1966. Electrical characteristics of Triturus egg cells during cleavage. J. Gen. Physiol. 49:1019–1027. 10.1085/jgp.49.5.1019 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Jaslove S.W., and Brink P.R.. 1986. The mechanism of rectification at the electrotonic motor giant synapse of the crayfish. Nature. 323:63–65. 10.1038/323063a0 [DOI] [PubMed] [Google Scholar]
- Jasper H.H., and Monnier A.M.. 1938. Transmission of excitation between excised non-myelinated nerves. An artificial synapse. J. Cell. Comp. Physiol. 11:259–277. 10.1002/jcp.1030110210 [DOI] [Google Scholar]
- Johnson G.E. 1924. Giant nerve fibers in crustaceans with special reference to Cambarus and Palaemonetes. J. Comp. Neurol. 36:323–373. 10.1002/cne.900360402 [DOI] [Google Scholar]
- Josephson R.K., and Schwab W.E.. 1979. Electrical properties of an excitable epithelium. J. Gen. Physiol. 74:213–236. 10.1085/jgp.74.2.213 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Kamermans M., van Dijk B.W., Spekreijse H., and Zweypfenning R.C.. 1989a Lateral feedback from monophasic horizontal cells to cones in carp retina. I. Experiments. J. Gen. Physiol. 93:681–694. 10.1085/jgp.93.4.681 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Kamermans M., van Dijk B.W., and Spekreijse H.. 1989b Lateral feedback from monophasic horizontal cells to cones in carp retina. II. A quantitative model. J. Gen. Physiol. 93:695–714. 10.1085/jgp.93.4.695 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Kanaporis G., Mese G., Valiuniene L., White T.W., Brink P.R., and Valiunas V.. 2008. Gap junction channels exhibit connexin-specific permeability to cyclic nucleotides. J. Gen. Physiol. 131:293–305. 10.1085/jgp.200709934 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Kanno Y., and Loewenstein W.R.. 1964. Intercellular diffusion. Science. 143:959–960. 10.1126/science.143.3609.959 [DOI] [PubMed] [Google Scholar]
- Kater S.B., Rued J.R., and Murphy A.D.. 1978. Propagation of action potentials through electrotonic junctions in the salivary glands of the pulmonate mollusc, Helisoma trivolvis. J. Exp. Biol. 72:77–90. [DOI] [PubMed] [Google Scholar]
- Katz B., and Schmitt O.H.. 1940. Electric interaction between two adjacent nerve fibres. J. Physiol. 97:471–488. 10.1113/jphysiol.1940.sp003823 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Kistler J., and Bullivant S.. 1987. Protein processing in lens intercellular junctions: cleavage of MP70 to MP38. Invest. Ophthalmol. Vis. Sci. 28:1687–1692. [PubMed] [Google Scholar]
- Kriebel M.E. 1967. Conduction velocity and intracellular action potentials of the tunicate heart. J. Gen. Physiol. 50:2097–2107. 10.1085/jgp.50.8.2097 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Kriebel M.E. 1968. Electrical characteristics of tunicate heart cell membranes and nexuses. J. Gen. Physiol. 52:46–59. 10.1085/jgp.52.1.46 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Kronengold J., Trexler E.B., Bukauskas F.F., Bargiello T.A., and Verselis V.K.. 2003. Single-channel SCAM identifies pore-lining residues in the first extracellular loop and first transmembrane domains of Cx46 hemichannels. J. Gen. Physiol. 122:389–405. 10.1085/jgp.200308861 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Kujiraoka T., and Saito T.. 1986. Electrical coupling between bipolar cells in carp retina. Proc. Natl. Acad. Sci. USA. 83:4063–4066. 10.1073/pnas.83.11.4063 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Kwon T., Harris A.L., Rossi A., and Bargiello T.A.. 2011. Molecular dynamics simulations of the Cx26 hemichannel: evaluation of structural models with Brownian dynamics. J. Gen. Physiol. 138:475–493. 10.1085/jgp.201110679 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Kwon T., Roux B., Jo S., Klauda J.B., Harris A.L., and Bargiello T.A.. 2012. Molecular dynamics simulations of the Cx26 hemichannel: insights into voltage-dependent loop-gating. Biophys. J. 102:1341–1351. 10.1016/j.bpj.2012.02.009 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Kwon T., Tang Q., and Bargiello T.A.. 2013. Voltage-dependent gating of the Cx32*43E1 hemichannel: conformational changes at the channel entrances. J. Gen. Physiol. 141:243–259. 10.1085/jgp.201210839 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Laird D.W., and Lampe P.D.. 2018. Therapeutic strategies targeting connexins. Nat. Rev. 10.1038/nrd.2018.1.38 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Lanza The Marchioness Clara. 1881. An experiment upon electric fish made by Galvani. Science. os-2:26–31. [DOI] [PubMed]
- Levis R.1981. Patch and axial wire voltage clamp techniques. Ph.D. Dissertation, University California, Los Angeles, CA. pp. 47-66. [Google Scholar]
- Levis R.A., Mathias R.T., and Eisenberg R.S.. 1983. Electrical properties of sheep Purkinje strands. Electrical and chemical potentials in the clefts. Biophys. J. 44:225–248. 10.1016/S0006-3495(83)84295-7 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Levitan H., Tauc L., and Segundo J.P.. 1970. Electrical transmission among neurons in the buccal ganglion of a mollusc, Navanax inermis. J. Gen. Physiol. 55:484–496. 10.1085/jgp.55.4.484 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Levitt D.G. 1975. General continuum analysis of transport through pores. I. Proof of Onsager’s reciprocity postulate for uniform pore. Biophys. J. 15:533–551. 10.1016/S0006-3495(75)85836-X [DOI] [PMC free article] [PubMed] [Google Scholar]
- Levitt D.G. 1991a General continuum theory for multiion channel. I. Theory. Biophys. J. 59:271–277. 10.1016/S0006-3495(91)82220-2 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Levitt D.G. 1991b General continuum theory for multiion channel. II. Application to acetylcholine channel. Biophys. J. 59:278–288. 10.1016/S0006-3495(91)82221-4 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Lieberman M., Sawanobori T., Kootsey J.M., and Johnson E.A.. 1975. A synthetic strand of cardiac muscle: its passive electrical properties. J. Gen. Physiol. 65:527–550. 10.1085/jgp.65.4.527 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Lin X., and Veenstra R.D.. 2004. Action potential modulation of connexin40 gap junctional conductance. Am. J. Physiol. Heart Circ. Physiol. 286:H1726–H1735. 10.1152/ajpheart.00943.2003 [DOI] [PubMed] [Google Scholar]
- Lin J.S., Fitzgerald S., Dong Y., Knight C., Donaldson P., and Kistler J.. 1997. Processing of the gap junction protein connexin50 in the ocular lens is accomplished by calpain. Eur. J. Cell Biol. 73:141–149. [PubMed] [Google Scholar]
- Lin X., Crye M., and Veenstra R.D.. 2003. Regulation of connexin43 gap junctional conductance by ventricular action potentials. Circ. Res. 93:e63–e73. 10.1161/01.RES.0000093379.61888.35 [DOI] [PubMed] [Google Scholar]
- Lin X., Gemel J., Beyer E.C., and Veenstra R.D.. 2005. Dynamic model for ventricular junctional conductance during the cardiac action potential. Am. J. Physiol. Heart Circ. Physiol. 288:H1113–H1123. 10.1152/ajpheart.00882.2004 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Llinás R., Baker R., and Sotelo C.. 1974. Electrotonic coupling between neurons in cat inferior olive. J. Neurophysiol. 37:560–571. 10.1152/jn.1974.37.3.560 [DOI] [PubMed] [Google Scholar]
- Locke D., Bian S., Li H., and Harris A.L.. 2009. Post-translational modifications of connexin26 revealed by mass spectrometry. Biochem. J. 424:385–398. 10.1042/BJ20091140 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Locke D., Kieken F., Tao L., Sorgen P.L., and Harris A.L.. 2011. Mechanism for modulation of gating of connexin26-containing channels by taurine. J. Gen. Physiol. 138:321–339. 10.1085/jgp.201110634 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Loewenstein W.R., and Kanno Y.. 1964. Studies on an epithelial (gland) cell junction. I. Modifications of surface membrane permeability. J. Cell Biol. 22:565–586. 10.1083/jcb.22.3.565 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Loewenstein W.R., Nakas M., and Socolar S.J.. 1967. Junctional membrane uncoupling. Permeability transformations at a cell membrane junction. J. Gen. Physiol. 50:1865–1891. 10.1085/jgp.50.7.1865 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Lopez W., Gonzalez J., Liu Y., Harris A.L., and Contreras J.E.. 2013. Insights on the mechanisms of Ca(2+) regulation of connexin26 hemichannels revealed by human pathogenic mutations (D50N/Y). J. Gen. Physiol. 142:23–35. 10.1085/jgp.201210893 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Lopez W., Liu Y., Harris A.L., and Contreras J.E.. 2014. Divalent regulation and intersubunit interactions of human connexin26 (Cx26) hemichannels. Channels (Austin). 8:1–4. 10.4161/chan.26789 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Lopez W., Ramachandran J., Alsamarah A., Luo Y., Harris A.L., and Contreras J.E.. 2016. Mechanism of gating by calcium in connexin hemichannels. Proc. Natl. Acad. Sci. USA. 113:E7986–E7995. 10.1073/pnas.1609378113 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Luna V.M., and Brehm P.. 2006. An electrically coupled network of skeletal muscle in zebrafish distributes synaptic current. J. Gen. Physiol. 128:89–102. 10.1085/jgp.200609501 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Luo Y., Rossi A.R., and Harris A.L.. 2016. Computational studies of molecular permeation through connexin26 channels. Biophys. J. 110:584–599. 10.1016/j.bpj.2015.11.3528 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Maciunas K., Snipas M., Paulauskas N., and Bukauskas F.F.. 2016. Reverberation of excitation in neuronal networks interconnected through voltage-gated gap junction channels. J. Gen. Physiol. 147:273–288. 10.1085/jgp.201511488 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Mackie G.O. 1965. Conduction in the nerve-free epithelia of siphonophores. Am. Zool. 5:439–453. 10.1093/icb/5.3.439 [DOI] [PubMed] [Google Scholar]
- Mackie G.O. 1976. Propagated spikes and secretion in a coelenterate glandular epithelium. J. Gen. Physiol. 68:313–325. 10.1085/jgp.68.3.313 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Mackie G.O., and Passano L.M.. 1968. Epithelial conduction in hydromedusae. J. Gen. Physiol. 52:600–621. 10.1085/jgp.52.4.600 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Maeda S., Nakagawa S., Suga M., Yamashita E., Oshima A., Fujiyoshi Y., and Tsukihara T.. 2009. Structure of the connexin 26 gap junction channel at 3.5 A resolution. Nature. 458:597–602. 10.1038/nature07869 [DOI] [PubMed] [Google Scholar]
- Margiotta J.F., and Walcott B.. 1983. Conductance and dye permeability of a rectifying electrical synapse. Nature. 305:52–55. 10.1038/305052a0 [DOI] [PubMed] [Google Scholar]
- Mathias R.T., Riquelme G., and Rae J.L.. 1991. Cell to cell communication and pH in the frog lens. J. Gen. Physiol. 98:1085–1103. 10.1085/jgp.98.6.1085 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Miller A.C., and Pereda A.E.. 2017. The electrical synapse: Molecular complexities at the gap and beyond. Dev. Neurobiol. 77:562–574. 10.1002/dneu.22484 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Morley G.E., Taffet S.M., and Delmar M.. 1996. Intramolecular interactions mediate pH regulation of connexin43 channels. Biophys. J. 70:1294–1302. 10.1016/S0006-3495(96)79686-8 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Mueller P., Rudin D.O., Tien H.T., and Wescott W.C.. 1962. Reconstitution of cell membrane structure in vitro and its transformation into an excitable system. Nature. 194:979–980. 10.1038/194979a0 [DOI] [PubMed] [Google Scholar]
- Mullins L.J. 1959. An analysis of conductance changes in squid axon. J. Gen. Physiol. 42:1013–1035. 10.1085/jgp.42.5.1013 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Mullins L.J. 1960. An analysis of pore size in excitable membranes. J. Gen. Physiol. 43:105–117. 10.1085/jgp.43.5.105 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Mullins L.J. 1968a A single channel or a dual channel mechanism for nerve excitation. J. Gen. Physiol. 52:550–556. 10.1085/jgp.52.3.550 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Mullins L.J. 1968b Single or dual channel mechanisms. J. Gen. Physiol. 52:555–556. 10.1085/jgp.52.3.555 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Narahashi T., and Moore J.W.. 1968. A single or dual channel in nerve membranes. J. Gen. Physiol. 52:553–555. 10.1085/jgp.52.3.553 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Nathan R.D., and DeHaan R.L.. 1979. Voltage clamp analysis of embryonic heart cell aggregates. J. Gen. Physiol. 73:175–198. 10.1085/jgp.73.2.175 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Neher E., and Sakmann B.. 1976. Single-channel currents recorded from membrane of denervated frog muscle fibres. Nature. 260:799–802. 10.1038/260799a0 [DOI] [PubMed] [Google Scholar]
- Neyton J., and Trautmann A.. 1985. Single-channel currents of an intercellular junction. Nature. 317:331–335. 10.1038/317331a0 [DOI] [PubMed] [Google Scholar]
- Nolte J., and Brown J.E.. 1972. Electrophysiological properties of cells in the median ocellus of Limulus. J. Gen. Physiol. 59:167–185. 10.1085/jgp.59.2.167 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Oh S., Rubin J.B., Bennett M.V.L., Verselis V.K., and Bargiello T.A.. 1999. Molecular determinants of electrical rectification of single channel conductance in gap junctions formed by connexins 26 and 32. J. Gen. Physiol. 114:339–364. 10.1085/jgp.114.3.339 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Oh S., Abrams C.K., Verselis V.K., and Bargiello T.A.. 2000. Stoichiometry of transjunctional voltage-gating polarity reversal by a negative charge substitution in the amino terminus of a connexin32 chimera. J. Gen. Physiol. 116:13–31. 10.1085/jgp.116.1.13 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Osterhout W.J.V., and Hill S.E.. 1930. Salt bridges and negative variations. J. Gen. Physiol. 13:547–552. 10.1085/jgp.13.5.547 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Pappas G.D., and Bennett M.V.L.. 1966. Specialized junctions involved in electrical transmission between neurons. Ann. N. Y. Acad. Sci. 137(2 Biological Me):495–508. 10.1111/j.1749-6632.1966.tb50177.x [DOI] [PubMed] [Google Scholar]
- Paul D.L. 1986. Molecular cloning of cDNA for rat liver gap junction protein. J. Cell Biol. 103:123–134. 10.1083/jcb.103.1.123 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Paul D.L., Ebihara L., Takemoto L.J., Swenson K.I., and Goodenough D.A.. 1991. Connexin46, a novel lens gap junction protein, induces voltage-gated currents in nonjunctional plasma membrane of Xenopus oocytes. J. Cell Biol. 115:1077–1089. 10.1083/jcb.115.4.1077 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Paulauskas N., Pranevicius M., Pranevicius H., and Bukauskas F.F.. 2009. A stochastic four-state model of contingent gating of gap junction channels containing two “fast” gates sensitive to transjunctional voltage. Biophys. J. 96:3936–3948. 10.1016/j.bpj.2009.01.059 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Payton B.1959. Proprioceptive Receptor Potentials of Oscillatory Form. https://www.youtube.com/watch?v=NWvF4-1wztM (accessed October 24, 2018).
- Payton B.W., Bennett M.V.L., and Pappas G.D.. 1969a Permeability and structure of junctional membranes at an electrotonic synapse. Science. 166:1641–1643. 10.1126/science.166.3913.1641 [DOI] [PubMed] [Google Scholar]
- Payton B.W., Bennett M.V.L., and Pappas G.D.. 1969b Temperature-dependence of resistance at an electrotonic synapse. Science. 165:594–597. 10.1126/science.165.3893.594 [DOI] [PubMed] [Google Scholar]
- Pereda A.E., Schwiezer E.S., and Zottoli S.J.. 2013. On the training of future neuroscientists: Insights from the Grass laboratory. Neuron. 79:12–15. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Pfahnl A., and Dahl G.. 1998. Localization of a voltage gate in connexin46 gap junction hemichannels. Biophys. J. 75:2323–2331. 10.1016/S0006-3495(98)77676-3 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Pfahnl A., and Dahl G.. 1999. Gating of cx46 gap junction hemichannels by calcium and voltage. Pflugers Arch. 437:345–353. 10.1007/s004240050788 [DOI] [PubMed] [Google Scholar]
- Pfahnl A., Zhou X.W., Werner R., and Dahl G.. 1997. A chimeric connexin forming gap junction hemichannels. Pflugers Arch. 433:773–779. 10.1007/s004240050344 [DOI] [PubMed] [Google Scholar]
- Pinto B.I., Pupo A., García I.E., Mena-Ulecia K., Martínez A.D., Latorre R., and Gonzalez C.. 2017. Calcium binding and voltage gating in Cx46 hemichannels. Sci. Rep. 7:15851 10.1038/s41598-017-15975-5 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Puljung M.C., Berthoud V.M., Beyer E.C., and Hanck D.A.. 2004. Polyvalent cations constitute the voltage gating particle in human connexin37 hemichannels. J. Gen. Physiol. 124:587–603. 10.1085/jgp.200409023 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Purnick P.E., Oh S., Abrams C.K., Verselis V.K., and Bargiello T.A.. 2000. Reversal of the gating polarity of gap junctions by negative charge substitutions in the N-terminus of connexin 32. Biophys. J. 79:2403–2415. 10.1016/S0006-3495(00)76485-X [DOI] [PMC free article] [PubMed] [Google Scholar]
- Qian H., and Ripps H.. 1992. Receptive field properties of rod-driven horizontal cells in the skate retina. J. Gen. Physiol. 100:457–478. 10.1085/jgp.100.3.457 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Qu Y., and Dahl G.. 2002. Function of the voltage gate of gap junction channels: selective exclusion of molecules. Proc. Natl. Acad. Sci. USA. 99:697–702. 10.1073/pnas.022324499 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Reuss L., and Finn A.L.. 1974. Passive electrical properties of toad urinary bladder epithelium. Intercellular electrical coupling and transepithelial cellular and shunt conductances. J. Gen. Physiol. 64:1–25. 10.1085/jgp.64.1.1 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Revel J.P., and Karnovsky M.J.. 1967. Hexagonal array of subunits in intercellular junctions of the mouse heart and liver. J. Cell Biol. 33:C7–C12. 10.1083/jcb.33.3.C7 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Revel J.P., Olson W., and Karnovsky M.J.. 1967. A twenty-Å gap junction with a hexagonal array of subunits in smooth muscle. J. Cell Biol. 35:112A. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Rhee S.K., Bevans C.G., and Harris A.L.. 1996. Channel-forming activity of immunoaffinity-purified connexin32 in single phospholipid membranes. Biochemistry. 35:9212–9223. 10.1021/bi960295m [DOI] [PubMed] [Google Scholar]
- Robertson J.D. 1963. The occurrence of a subunit pattern in the unit membranes of club endings in the Mauthner cell synapses in goldfish brains. J. Cell Biol. 19:201–221. 10.1083/jcb.19.1.201 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Rohr S., and Salzberg B.M.. 1994. Characterization of impulse propagation at the microscopic level across geometrically defined expansions of excitable tissue: multiple site optical recording of transmembrane voltage (MSORTV) in patterned growth heart cell cultures. J. Gen. Physiol. 104:287–309. 10.1085/jgp.104.2.287 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Rose B., and Loewenstein W.R.. 1975. Permeability of cell junction depends on local cytoplasmic calcium activity. Nature. 254:250–252. 10.1038/254250a0 [DOI] [PubMed] [Google Scholar]
- Rose B., and Loewenstein W.R.. 1976. Permeability of a cell junction and the local cytoplasmic free ionized calcium concentration: a study with aequorin. J. Membr. Biol. 28:87–119. 10.1007/BF01869692 [DOI] [PubMed] [Google Scholar]
- Rose B., and Rick R.. 1978. Intracellular pH, intracellular free Ca, and junctional cell-cell coupling. J. Membr. Biol. 44:377–415. 10.1007/BF01944230 [DOI] [PubMed] [Google Scholar]
- Rubinos C., Sánchez H.A., Verselis V.K., and Srinivas M.. 2012. Mechanism of inhibition of connexin channels by the quinine derivative N-benzylquininium. J. Gen. Physiol. 139:69–82. 10.1085/jgp.201110678 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Saito T., and Kujiraoka T.. 1988. Characteristics of bipolar-bipolar coupling in the carp retina. J. Gen. Physiol. 91:275–287. 10.1085/jgp.91.2.275 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Sanchez H.A., Villone K., Srinivas M., and Verselis V.K.. 2013. The D50N mutation and syndromic deafness: altered Cx26 hemichannel properties caused by effects on the pore and intersubunit interactions. J. Gen. Physiol. 142:3–22. 10.1085/jgp.201310962 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Sanchez H.A., Slavi N., Srinivas M., and Verselis V.K.. 2016. Syndromic deafness mutations at Asn 14 differentially alter the open stability of Cx26 hemichannels. J. Gen. Physiol. 148:25–42. 10.1085/jgp.201611585 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Santa Cruz A., Meşe G., Valiuniene L., Brink P.R., White T.W., and Valiunas V.. 2015. Altered conductance and permeability of Cx40 mutations associated with atrial fibrillation. J. Gen. Physiol. 146:387–398. 10.1085/jgp.201511475 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Santos-Sacchi J. 1991. Isolated supporting cells from the organ of Corti: some whole cell electrical characteristics and estimates of gap junctional conductance. Hear. Res. 52:89–98. 10.1016/0378-5955(91)90190-K [DOI] [PubMed] [Google Scholar]
- Schmidtmann M. 1925. [About the intracellular hydrogen ion concentration in physiological and in some pathological conditions]. Z. ges. Exp. Med. 45:714–742. 10.1007/BF02626087 [DOI] [Google Scholar]
- Senseman D.M., Shimizu H., Horwitz I.S., and Salzberg B.M.. 1983. Multiple-site optical recording of membrane potential from a salivary gland. Interaction of synaptic and electrotonic excitation. J. Gen. Physiol. 81:887–908. 10.1085/jgp.81.6.887 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Shaw S.R. 1967. Coupling between receptors in the eye of the drone honeybee. J. Gen. Physiol. 50:2480–2481. [Google Scholar]
- Sims S.M., Daniel E.E., and Garfield R.E.. 1982. Improved electrical coupling in uterine smooth muscle is associated with increased numbers of gap junctions at parturition. J. Gen. Physiol. 80:353–375. 10.1085/jgp.80.3.353 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Sjodin R.A., and Mullins L.J.. 1967. Tracer and nontracer potassium fluxes in squid giant axons and the effects of changes in external potassium concentration and membrane potential. J. Gen. Physiol. 50:533–549. 10.1085/jgp.50.3.533 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Sjöstrand F.S., and Andersson E.. 1954. Electron microscopy of the intercalated discs of cardiac muscle tissue. Experientia. 10:369–370. 10.1007/BF02160542 [DOI] [PubMed] [Google Scholar]
- Sjöstrand F.S., Andersson-Cedergren E., and Dewey M.M.. 1958. The ultrastructure of the intercalated discs of frog, mouse and guinea pig cardiac muscle. J. Ultrastruct. Res. 1:271–287. 10.1016/S0022-5320(58)80008-8 [DOI] [PubMed] [Google Scholar]
- Skerrett I.M., Aronowitz J., Shin J.H., Cymes G., Kasperek E., Cao F.L., and Nicholson B.J.. 2002. Identification of amino acid residues lining the pore of a gap junction channel. J. Cell Biol. 159:349–360. 10.1083/jcb.200207060 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Smith T.G., and Baumann F.. 1969. The functional organization within the ommatidium of the lateral eye of limulus. Prog. Brain Res. 31:313–349. 10.1016/S0079-6123(08)63248-3 [DOI] [PubMed] [Google Scholar]
- Smith T.G., Baumann F., and Fuortes M.G.. 1965. Electrical connections between visual cells in the ommatidium of Limulus. Science. 147:1446–1448. 10.1126/science.147.3664.1446 [DOI] [PubMed] [Google Scholar]
- Snipas M., Kraujalis T., Paulauskas N., Maciunas K., and Bukauskas F.F.. 2016. Stochastic model of gap junctions exhibiting rectification and multiple closed states of slow gates. Biophys. J. 110:1322–1333. 10.1016/j.bpj.2016.01.035 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Snipas M., Rimkute L., Kraujalis T., Maciunas K., and Bukauskas F.F.. 2017. Functional asymmetry and plasticity of electrical synapses interconnecting neurons through a 36-state model of gap junction channel gating. PLOS Comput. Biol. 13:e1005464 10.1371/journal.pcbi.1005464 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Solan J.L., and Lampe P.D.. 2014. Specific Cx43 phosphorylation events regulate gap junction turnover in vivo. FEBS Lett. 588:1423–1429. 10.1016/j.febslet.2014.01.049 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Spira M.E., and Bennett M.V.L.. 1972. Synaptic control of electrotonic coupling between neurons. Brain Res. 37:294–300. 10.1016/0006-8993(72)90674-9 [DOI] [PubMed] [Google Scholar]
- Spira M.E., Spray D.C., and Bennett M.V.L.. 1980. Synaptic organization of expansion motoneurons of Navanax inermis. Brain Res. 195:241–269. 10.1016/0006-8993(80)90063-3 [DOI] [PubMed] [Google Scholar]
- Spray D.C., Harris A.L., and Bennett M.V.L.. 1979. Voltage dependence of junctional conductance in early amphibian embryos. Science. 204:432–434. 10.1126/science.312530 [DOI] [PubMed] [Google Scholar]
- Spray D.C., Harris A.L., and Bennett M.V.L.. 1981a Equilibrium properties of a voltage-dependent junctional conductance. J. Gen. Physiol. 77:77–93. 10.1085/jgp.77.1.77 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Spray D.C., Harris A.L., and Bennett M.V.L.. 1981b Gap junctional conductance is a simple and sensitive function of intracellular pH. Science. 211:712–715. 10.1126/science.6779379 [DOI] [PubMed] [Google Scholar]
- Spray D.C., Stern J.H., Harris A.L., and Bennett M.V.L.. 1982. Gap junctional conductance: comparison of sensitivities to H and Ca ions. Proc. Natl. Acad. Sci. USA. 79:441–445. 10.1073/pnas.79.2.441 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Srinivas M., Hopperstad M.G., and Spray D.C.. 2001. Quinine blocks specific gap junction channel subtypes. Proc. Natl. Acad. Sci. USA. 98:10942–10947. 10.1073/pnas.191206198 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Srinivas M., Calderon D.P., Kronengold J., and Verselis V.K.. 2006. Regulation of connexin hemichannels by monovalent cations. J. Gen. Physiol. 127:67–75. 10.1085/jgp.200509397 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Srinivas M., Verselis V.K., and White T.W.. 2018. Human diseases associated with connexin mutations. Biochim Biophys Acta Biomembr. 1860:192–201. 10.1016/j.bbamem.2017.04.024 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Stämpfli R. 1954. A new method for measuring membrane potentials with external electrodes. Experientia. 10:508–509. 10.1007/BF02166189 [DOI] [PubMed] [Google Scholar]
- Stough H.B. 1926. Giant fibers of the earthworm. J. Comp. Neurol. 40:409–463. 10.1002/cne.900400302 [DOI] [Google Scholar]
- Suchyna T.M., Nitsche J.M., Chilton M., Harris A.L., Veenstra R.D., and Nicholson B.J.. 1999. Different ionic selectivities for connexins 26 and 32 produce rectifying gap junction channels. Biophys. J. 77:2968–2987. 10.1016/S0006-3495(99)77129-8 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Tanaka I., and Sasaki Y.. 1966. On the electrotonic spread in cardiac muscle of the mouse. J. Gen. Physiol. 49:1089–1110. 10.1085/jgp.0491089 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Tang Q., Dowd T.L., Verselis V.K., and Bargiello T.A.. 2009. Conformational changes in a pore-forming region underlie voltage-dependent “loop gating” of an unapposed connexin hemichannel. J. Gen. Physiol. 133:555–570. 10.1085/jgp.200910207 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Tao L., and Harris A.L.. 2004. Biochemical requirements for inhibition of Connexin26-containing channels by natural and synthetic taurine analogs. J. Biol. Chem. 279:38544–38554. 10.1074/jbc.M405654200 [DOI] [PubMed] [Google Scholar]
- Tasaki I. 1963. Permeability of squid axon membrane to various ions. J. Gen. Physiol. 46:755–772. 10.1085/jgp.46.4.755 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Tasaki I., and Hagiwara S.. 1957. Demonstration of two stable potential states in the squid giant axon under tetraethylammonium chloride. J. Gen. Physiol. 40:859–885. 10.1085/jgp.40.6.859 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Tasaki I., Singer I., and Takenaka T.. 1965. Effects of internal and external ionic environment on excitability of squid giant axon. A macromolecular approach. J. Gen. Physiol. 48:1095–1123. 10.1085/jgp.48.6.1095 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Tasaki I., Singer I., and Watanabe A.. 1967. Cation interdiffusion in squid giant axons. J. Gen. Physiol. 50:989–1007. 10.1085/jgp.50.4.989 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Tomita T., and Kaneko A.. 1965. An intracellular coaxial microelectrode--its construction and application. Med. Electron. Biol. Eng. 3:367–376. 10.1007/BF02476131 [DOI] [PubMed] [Google Scholar]
- Tong X., Lopez W., Ramachandran J., Ayad W.A., Liu Y., Lopez-Rodriguez A., Harris A.L., and Contreras J.E.. 2015. Glutathione release through connexin hemichannels: Implications for chemical modification of pores permeable to large molecules. J. Gen. Physiol. 146:245–254. 10.1085/jgp.201511375 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Trexler E.B., Bennett M.V.L., Bargiello T.A., and Verselis V.K.. 1996. Voltage gating and permeation in a gap junction hemichannel. Proc. Natl. Acad. Sci. USA. 93:5836–5841. 10.1073/pnas.93.12.5836 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Trexler E.B., Bukauskas F.F., Bennett M.V.L., Bargiello T.A., and Verselis V.K.. 1999. Rapid and direct effects of pH on connexins revealed by the connexin46 hemichannel preparation. J. Gen. Physiol. 113:721–742. 10.1085/jgp.113.5.721 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Trexler E.B., Bukauskas F.F., Kronengold J., Bargiello T.A., and Verselis V.K.. 2000. The first extracellular loop domain is a major determinant of charge selectivity in connexin46 channels. Biophys. J. 79:3036–3051. 10.1016/S0006-3495(00)76539-8 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Turin L., and Warner A.. 1977. Carbon dioxide reversibly abolishes ionic communication between cells of early amphibian embryo. Nature. 270:56–57. 10.1038/270056a0 [DOI] [PubMed] [Google Scholar]
- Turin L., and Warner A.E.. 1980. Intracellular pH in early Xenopus embryos: its effect on current flow between blastomeres. J. Physiol. 300:489–504. 10.1113/jphysiol.1980.sp013174 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Valiunas V. 2002. Biophysical properties of connexin-45 gap junction hemichannels studied in vertebrate cells. J. Gen. Physiol. 119:147–164. 10.1085/jgp.119.2.147 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Valiunas V., and Weingart R.. 2000. Electrical properties of gap junction hemichannels identified in transfected HeLa cells. Pflugers Arch. 440:366–379. 10.1007/s004240000294 [DOI] [PubMed] [Google Scholar]
- Veenstra R.D., and DeHaan R.L.. 1986. Measurement of single channel currents from cardiac gap junctions. Science. 233:972–974. 10.1126/science.2426781 [DOI] [PubMed] [Google Scholar]
- Veenstra R.D., Wang H.Z., Beblo D.A., Chilton M.G., Harris A.L., Beyer E.C., and Brink P.R.. 1995. Selectivity of connexin-specific gap junctions does not correlate with channel conductance. Circ. Res. 77:1156–1165. 10.1161/01.RES.77.6.1156 [DOI] [PubMed] [Google Scholar]
- Veeraraghavan R., Gourdie R.G., and Poelzing S.. 2014. Mechanisms of cardiac conduction: a history of revisions. Am. J. Physiol. Heart Circ. Physiol. 306:H619–H627. 10.1152/ajpheart.00760.2013 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Verheijck E.E., Wilders R., Joyner R.W., Golod D.A., Kumar R., Jongsma H.J., Bouman L.N., and van Ginneken A.C.. 1998. Pacemaker synchronization of electrically coupled rabbit sinoatrial node cells. J. Gen. Physiol. 111:95–112. 10.1085/jgp.111.1.95 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Verselis V.K., and Srinivas M.. 2008. Divalent cations regulate connexin hemichannels by modulating intrinsic voltage-dependent gating. J. Gen. Physiol. 132:315–327. 10.1085/jgp.200810029 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Verselis V.K., Ginter C.S., and Bargiello T.A.. 1994. Opposite voltage gating polarities of two closely related connexins. Nature. 368:348–351. 10.1038/368348a0 [DOI] [PubMed] [Google Scholar]
- Verselis V.K., Trelles M.P., Rubinos C., Bargiello T.A., and Srinivas M.. 2009. Loop gating of connexin hemichannels involves movement of pore-lining residues in the first extracellular loop domain. J. Biol. Chem. 284:4484–4493. 10.1074/jbc.M807430200 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Wang H.Z., and Veenstra R.D.. 1997. Monovalent ion selectivity sequences of the rat connexin43 gap junction channel. J. Gen. Physiol. 109:491–507. 10.1085/jgp.109.4.491 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Wasserman G.S. 1968. Persistent effects of brief stimuli interacting with the hyperpolarizing response. Physiol. Behav. 3:845–847. 10.1016/0031-9384(68)90165-0 [DOI] [Google Scholar]
- Watanabe A. 1958. The interaction of electrical activity among neurons of lobster cardiac ganglion. Jpn. J. Physiol. 8:305–318. 10.2170/jjphysiol.8.305 [DOI] [PubMed] [Google Scholar]
- Watanabe A., and Bullock T.H.. 1960. Modulation of activity of one neuron by subthreshold slow potentials in another in lobster cardiac ganglion. J. Gen. Physiol. 43:1031–1045. 10.1085/jgp.43.6.1031 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Watanabe A., and Grundfest H.. 1961. Impulse propagation at the septal and commissural junctions of crayfish lateral giant axons. J. Gen. Physiol. 45:267–308. 10.1085/jgp.45.2.267 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Watanabe A., and Takeda K.. 1963. The spread of excitation among neurons in the heart ganglion of the stomatopod, Squillia oratoria. J. Gen. Physiol. 46:773–801. 10.1085/jgp.46.4.773 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Weidmann S. 1952. The electrical constants of Purkinje fibres. J. Physiol. 118:348–360. 10.1113/jphysiol.1952.sp004799 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Weidmann S. 1966. The diffusion of radiopotassium across intercalated disks of mammalian cardiac muscle. J. Physiol. 187:323–342. 10.1113/jphysiol.1966.sp008092 [DOI] [PMC free article] [PubMed] [Google Scholar]
- West C.H.K., and Bernard R.A.. 1978. Intracellular characteristics and responses of taste bud and lingual cells of the mudpuppy. J. Gen. Physiol. 72:305–326. 10.1085/jgp.72.3.305 [DOI] [PMC free article] [PubMed] [Google Scholar]
- White R.L., Doeller J.E., Verselis V.K., and Wittenberg B.A.. 1990. Gap junctional conductance between pairs of ventricular myocytes is modulated synergistically by H+ and Ca++. J. Gen. Physiol. 95:1061–1075. 10.1085/jgp.95.6.1061 [DOI] [PMC free article] [PubMed] [Google Scholar]
- White T.W., Bruzzone R., Goodenough D.A., and Paul D.L.. 1994a. Voltage gating of connexins. Nature. 371:208–209. 10.1038/371208a0 [DOI] [PubMed] [Google Scholar]
- White T.W., Bruzzone R., Wolfram S., Paul D.L., and Goodenough D.A.. 1994b. Selective interactions among the multiple connexin proteins expressed in the vertebrate lens: the second extracellular domain is a determinant of compatibility between connexins. J. Cell Biol. 125:879–892. 10.1083/jcb.125.4.879 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Zhou X.W., Pfahnl A., Werner R., Hudder A., Llanes A., Luebke A., and Dahl G.. 1997. Identification of a pore lining segment in gap junction hemichannels. Biophys. J. 72:1946–1953. 10.1016/S0006-3495(97)78840-4 [DOI] [PMC free article] [PubMed] [Google Scholar]