Effects of GluN2B-selective antagonists on delay and probability discounting in male rats: Modulation by delay/probability presentation order

Abstract

The contribution of the GluN2B subunit of the NMDA receptor to impulsive/risky choice has recently been examined. Ro 63–1908, a highly selective antagonist for GluN2B-containing NMDA receptors, decreases impulsive choice. Because the order in which delays are presented modulates drug effects in discounting procedures, one goal of the current study was to determine the effects of Ro 63–1908 in delay discounting procedures in which the delays to obtaining the large reinforcer either increase or decrease across the session. We also determined if Ro 63–1908 differentially alters risky choice in probability discounting procedures that use ascending/descending schedules. Male rats were trained in either delay (n = 24) or probability (n = 24) discounting in which the delay to/odds against reinforcement were presented in either ascending or descending order (n = 12 each schedule). Following training, rats received the GluN2B antagonists Ro 63–1908 (0, 0.1, 0.3, 1.0 mg/kg) and CP-101,606 (0, 0.3, 1.0, 3.0 mg/kg) in a counterbalanced order. In delay discounting, Ro 63–1908 (1.0 mg/kg), but not CP-101,606, decreased choice for the large reinforcer, but only when the delays decreased across the session. In probability discounting, Ro 63–1908 (0.3 mg/kg)/CP-101,606 (1.0 mg/kg) increased choice for the large reinforcer when the probability of obtaining this alternative decreased across the session, but Ro 63–1908 (1.0 mg/kg)/CP-101,606 (3.0 mg/kg) decreased choice when the probabilities increased. These results show that the GluN2B is a mediator of impulsive/risky choice, but the effects of GluN2B antagonists are dependent on the order in which delays/probabilities are presented.

Because impulsive and risky decision making are associated with several psychiatric conditions, such as pathological gambling and substance use disorders, numerous studies have attempted to identify the neuromechanisms of impulsive/risky choice. To measure impulsive/risky choice, delay and probability discounting procedures are often used. In behavioral pharmacology experiments, the delay to (delay discounting) or the odds against (probability discounting; note: odds against = [1/probability-1]; Rachlin, Raineri, & Cross, 1991) receiving reinforcement often increase across a session (Cardinal & Howes, 2005; Evenden & Ryan, 1996). Recent research has focused on the role of the glutamate N-methyl-D-aspartate (NMDA) receptor in delay and probability discounting, and results have been somewhat mixed. Specifically, the NMDA receptor channel blockers ketamine (Cottone et al., 2013; Floresco, Tse, & Ghods-Sharifi, 2008) and memantine (Cottone et al., 2013) increase impulsive choice (but see Yates, Gunkel, Rogers, Hughes, & Prior, 2017 for an alternative explanation), whereas MK-801, another channel blocker, decreases impulsive choice (Higgins et al., 2016; Yates, Batten, Bardo, & Beckmann, 2015; but see Yates, Gunkel, et al., 2017 for null effects). The results with GluN2B subunit antagonists have also been mixed, as ifenprodil decreases preference for the large magnitude reinforcer when its delivery is immediate without altering impulsive choice (Yates, Gunkel, et al., 2017), whereas Ro 63–1908 decreases impulsive choice (Higgins et al., 2016). Similar to Ro 63–1908, CP-101,606 tends to decrease impulsive choice, although this effect was not statistically significant (Higgins et al., 2016). Concerning risky choice, MK-801 increases risky choice (Yates et al., 2015; Yates et al., 2016), whereas ketamine has been shown to decrease preference for the large magnitude reinforcer when its delivery is guaranteed (Yates et al., 2015; but see Yates et al., 2016). Finally, ifenprodil does not alter responses for the large, probabilistic reinforcer when the odds against receiving reinforcement increase across the session (Yates et al., 2016). Table 1 summarizes the effects of NMDA receptor ligands on discounting procedures.

Table 1:

Effects of NMDA receptor ligands on delay discounting and probability discounting.

Delay Discounting
Drug	Doses	Mechanism of Action	Procedure	Delay Presentation	Delays	Effect onlmpulsve Choice	Citation
D-cyclo serine	3.25–30.0 mg/kg	Partial agonist at GluNl	Evenden and Ryan (1996)	Ascending	0–40 s	No effect	van den Berg et al. (2006)
D-cyclo serine	3.25–30.0 mg/kg	Partial agonist at GluNl	Evenden and Ryan (1996)	Ascending	0–100s	No effect	Yates. Gunkel, et al. (2017)
CSG 19755	2.5–20.0 mg/kg	C ompetitive antagora st at GluN2	Modifi ed Adj usting Delay	N/A	Titrated by subject	↑ impulsive choice (20.0 mgkg)a	Cottone et al. (2013)
CSG 19755	2.5–20.0 mg/kg	C ompetitive antagora st at GluN2	Evenden and Ryan (1996)	Ascending	0–100s	↑ impulsive choi c e ( 5.0 m g kg)	Yates. GunkeL et al. (2017)
Ketamine	2.5–20.0 mg/kg	Channel blocker	Modifi ed Adj usting Delay	N/A	Titrated by subject	↑ impulsive choice ( 10.0 & 20.0 mgkg)	Cottone et al. (2013)
Ketamine	5.0 mg/kg	Channel blocker	Evenden and Ryan (1996)	Ascending	0.4–6.5 s	↑ impulsive choice	Floresco et al. (2008)
Ketamine	2.5–10.0 mg/kg	Channel blocker	Evenden and Ryan (1996)	Ascending	0–50 s	↓ choiceforLL at 0-s delay( 10.0mgkg)	Yates et al. (2015)
Ketamine	2.5–10.0 mg/kg	Channel blocker	Evenden and Ryan (1996)	Ascending	0–100s	↓ choice forLL at 0-s delay( 10.0mgkg)	Yates. GunkeL et al. (2017)
Memantine	1.25–10.0 mg/kg	Channel blocker	Modifi ed Adj usting Delay	N/A	Titrated by subject	↑ impulsive choice (5.0 & 10.0 mgkg)	Cottone et al. (2013)
Memantine	2.5–10.0 mg/kg	Channel blocker	Evenden and Ryan (1996)	Ascending	0–100s	↓ choice for LL at 0-s delay(5.0 mgkg)	Yates. Gunkel, et al. (2017)
MK-S01	0.01–0.06 mg/kg	Channel blocker	Evenden and Ryan (1996)	Ascending	0–40 s	↓impulsivechoice(0.03 & 0.06mgkg)	Higgins et al. (2016)
MK-S01	0.01–0.3 mg/kg	Channel blocker	Evenden and Ryan (1996)	Ascending	0–50 s	↓impulsivechoice(0.03 mgkg)	Yates et al. (2015)
MK-S01	0.003–0.03 mg/kg	Channel blocker	Evenden and Ryan (1996)	Ascending	0–100s	No effect	Yates. GunkeL et al. (2017)
Ifenprodil	1.0–10.0 mg/kg	Noncompetitive antagonist at GluN2B	Evenden and Ryan (1996)	Ascending	0–100 s	↓ choice for LL at 0-s del ay ( 10.0 mgkg)	Yates. GunkeL et al. (2017)
CP-101.606	1.0 & 3.0 mg/kg	Noncompetitive antagonist at GluN2B	Evenden and Ryan (1996)	Ascending	0–40 s	No effect	Higgins et al. (2016)
Ro 63–190S	0.l-l.0 mg/kg	Noncompetitive antagonist at GluN2B	Evenden and Ryan (1996)	Ascending	0–40 s	↓ impulsive choice (1.0 mgkg)	Higgins et al. (2016)
Probability Discounting
Drug	Doses	Mechanism of Action	Procedure	OA Presentation	Odds Against	Effect on Risky Choice	Citation
Ketamine	2.5–10.0 mg/kg	Channel blocker	Cardinal & Howes (2005)	Ascending	0–15	↓ choice for LR at 0 OA(10.0 mgkg)	Yates et al. (2015)
Ketamine	2.5–10.0 mg/kg	Channel blocker	Cardinal & Howes (2005)	Ascending	0–31	No effect	Yates et al. (2016)
Ketamine	2.5–10.0 mg/kg	Channel blocker	Cardinal & Howes (2005)	Descending	0–31	↓ risky choice (10.0 mgkg)	Yates et al. (2016)
MK-S01	0.01–0.3 mg/kg	Channel blocker	Cardinal & Howes (2005)	Ascending	0–15	↑ risky choi ce (0.03 mgkg)	Yates et al. (2015)
MK-S01	0.01–0.3 mg/kg	Channel blocker	Cardinal & Howes (2005)	Ascending	0–31	↑ risky choice (0.03 mgkg)	Yates et al. (2016)
MK-S01	0.01–0.3 mg/kg	Channel blocker	Cardinal & Howes (2005)	Descending	0–31	↓ risky choice (10.0 mgkg)	Yates et al. (2016)
Ifenprodil	1.0–10.0 mg/kg	Noncompetitive antagonist at GluN2B	Cardinal & Howes (2005)	Ascending	0–31	No effect	Yates et al. (2016)
Ifenprodil	1.0–10.0 mg/kg	Noncompetitive antagonist at GluN2B	Cardinal & Howes (2005)	Descending	0–31	↓risky choice (10.0 mgkg)	Yates et al. (2016)

^aCaused an increase in response latencies; as such, Cottone et al. (2013) argued that CGS 19755 did not selectively alter impulsive choice.

Abbreviations: LL = larger later; LR = larger risky.

Comparing the results across the NMDA receptor channel blockers (MK-801, ketamine, memantine) can be difficult because these drugs have high affinity for other receptors, such as adrenergic and serotonergic receptors (see Yates et al., 2015 for a discussion). Similarly, comparing the effects of the GluN2B antagonists ifenprodil and Ro 63–1908/CP-101,606 can be difficult, as ifenprodil has high affinity for adrenergic and serotonergic receptors (Chenard et al., 1991). Thus, the alterations in delay and probability discounting performance observed with these drugs may due to their interactions on other molecular targets as opposed to the NMDA receptor.

Another limitation to previous discounting studies is that they often use an ascending schedule (increase the delay to or odds against receiving reinforcement). Previous studies have shown that the order in which delays/odds against are presented modulates drug effects in discounting procedures. The psychostimulants d-amphetamine and methylphenidate decrease impulsive choice when the delay to reinforcement increases across the session, but increase impulsive choice when the delays decrease across trial blocks (Tanno, Maguire, Henson, & France, 2014). In probability discounting, amphetamine decreases preference for the large magnitude reinforcer when the probability of obtaining that alternative decreases across the session, but increases choice for that reinforcer when the probabilities increase across the session (St Onge, Chiu, & Floresco, 2010). The effects of ketamine and ifenprodil on probability discounting are also dependent on the order in which probabilities are presented. When the odds against receiving reinforcement decrease across the session, ketamine and ifenprodil decrease risky choice; however, these drugs do not alter task performance when the odds against increase (Yates et al., 2016). Thus, NMDA receptor ligands may differentially alter delay discounting in a paradigm that decreases the delay to delivery of the large magnitude reinforcer across the session.

Considering the limitations discussed above, the goal of the current study was to examine the effects of Ro 63–1908 and CP-101,606 in both delay and probability discounting using ascending and descending schedules. We used Ro 63–1908 and CP-101,606 for several reasons. First, GluN2B-selective antagonists lack the psychotomimetic side effects observed with channel blockers (Jiménez-Sánchez, Campa, Auberson, & Adell, 2014; Lima-Ojeda et al., 2013). Second, the GluN2B is an important mediator of substance use disorders (Gipson et al., 2013, Go, Barry, & McGinty, 2016; Li et al., 2016; Ma et al., 2006; Schumann, Matzner, Michaeli, & Yaka, 2009; Shen, Moussawi, Zhou, Toda, & Kalivas, 2011), which are correlated with impulsive and risky decision making (see Bickel, Koffarnus, Moody, & Wilson, 2014; Grant & Chamberlain, 2014; Verdejo-Garcia, Chong, Stout, Yücel, & London, 2018 for reviews). Directly related to the second point, GluN2B antagonists do not appear to have rewarding properties (e.g., Ma et al., 2006) as opposed to channel blockers such as phencyclidine (PCP) or ketamine (e.g., Carroll, Carmona, & Rodefer, 1994; Moreton, Meisch, Stark, & Thompson, 1977). Third, Ro 63–1908 and CP-101,606 are more selective for the GluN2B subunit compared to ifenprodil (Gill et al., 2002; Mony, Kew, Gunthorpe, & Paoletti, 2009). We predicted that Ro 63–1908 would decrease impulsive choice when an ascending schedule was used but would have no effect on task performance when a descending schedule was used. This hypothesis was made because previous research has shown a decrease in impulsive choice following Ro 63–1908 administration when an ascending schedule is used (Higgins et al., 2016). Additionally, our lab has shown that rats trained on a descending schedule have lower baseline levels of impulsive choice (Yates, Rogers, et al., 2017); therefore, we did not expect to see further increases in preference for the large, delayed reinforcer because of a ceiling effect. Regarding probability discounting, we hypothesized that Ro 63–1908 would decrease risk aversion when a descending schedule was used only. We based this hypothesis on the finding that ifenprodil decreases risk-taking behavior in a descending schedule but has no effect on behavior when an ascending schedule is used (Yates et al., 2016). Thus, if the effects observed with ifenprodil were due to its actions on the GluN2B subunit, one would expect Ro 63–1908 to exert similar effects in probability discounting. However, considering ifenprodil and Ro 63–1908 differentially alter delay discounting performance (Higgins et al., 2016; Yates, Gunkel, et al., 2017), it was important to test Ro 63–1908/CP-101,606 in probability discounting.

Method

Subjects

A total of 48 experimentally naïve, male Sprague Dawley rats (200–224 g upon arrival [approximately 45–52 days of age]; Envigo, Indianapolis, IN) was used in the current study. They were acclimated to an animal housing room and handled for six days before testing began. The housing room was maintained on a 12:12-h cycle (lights on at 630 h), and rats were tested in the light phase (approximately 1400–1600 h). Rats were individually housed in clear polypropylene cages (51 cm long × 26.5 cm wide × 32 cm high) with metal tops containing food and a water bottle. Rats were food restricted (approximately 80% free feed body weight) but had ad libitum access to water. All experimental procedures were carried out according to the Current Guide for the Care and Use of Laboratory Animals (USPHS) under a protocol approved by the Northern Kentucky University Institutional Animal Care and Use Committee.

Apparatus

Eight operant-conditioning chambers (28 × 21 × 21 cm; ENV-008; MED Associates, St. Albans, VT) located inside sound attenuating chambers (ENV-018M; MED Associates) were used. The front and back walls of the chambers were made of aluminum, while the side walls were made of Plexiglas. There was a recessed food tray (5 × 4.2 cm) located 2 cm above the floor in the bottom-center of the front wall. An infrared photobeam was used to record head entries into the food tray. Retractable levers (4.5 cm) were located 6 cm above the floor on each side of the food tray. A 28-V white stimulus light was located 2.54 cm above each response lever. A 28-V white house light was mounted in the center of the back wall of the chamber. A nosepoke aperture was located 2 cm above the floor in the bottom-center of the back wall (the aperture was never used in the current experiment). All responses and scheduled consequences were recorded and controlled by a computer interface. A computer controlled the experimental session using Med-IV software.

Drugs

1-[2-(4-hydroxyphenoxy)-ethyl]-4-[(4-methylphenyl)methyl]-4-piperidinol hydrochloride (Ro 63–1908) was purchased from Tocris Bioscience (Ellisville, MO), and (1S,2S)-1-(4-hydroxy-phenyl)-2-(4-hydroxy-4-phenylpiperidino)-1-propanol (CP-101,606) was purchased from Sigma Aldrich (St. Louis, MO). Ro 63–1908 was prepared in 5% Tween 80 in saline. CP-101,606 was prepared in distilled water containing 10% glacial acetic acid (pH = 5.6–5.8). Drug concentrations were calculated based on salt weight. Each drug was administered in a volume of 1 ml/kg via the subcutaneous route.

Procedure

Magazine training.

Each rat received three sessions of magazine training, in which 20 food pellets (45 mg dustless precision pellets; Bio-Serv, Frenchtown, NJ) were non-contingently delivered into the food tray according to a variable-time 30 s schedule of reinforcement.

Lever-press training.

Rats received six sessions of lever-press training. Each session began with illumination of the house light. A head entry into the food tray resulted in presentation of one lever; each lever was presented pseudo-randomly, with no more than two consecutive presentations of the same lever. A response on either lever (fixed ratio [FR] 1) resulted in delivery of one food pellet. Following a response on either lever, the house light was extinguished, and the lever was retracted for 5 s. After 5 s, the house light was illuminated. Each session ended after a rat earned 40 reinforcers or after 30 min, whichever came first. Following the first three sessions, the FR requirement increased each session to a terminal FR 10.

Magnitude discrimination.

Rats received five sessions of magnitude discrimination training. Similar to lever-press training, each session consisted of 40 trials, and the beginning of each trial was signaled by illumination of the house light. A head entry into the food tray extended one of the levers (the order of presentation between the two levers was pseudo-random, with no more than two consecutive presentations of the same lever). Responses (FR 10) on one lever resulted in immediate delivery of one pellet, whereas responses (FR 10) on the other lever resulted in immediate delivery of four pellets (the lever associated with the large magnitude reinforcer was counterbalanced across rats; the lever associated with each alternative never changed for individual subjects). Following completion of the response requirement on either lever, the house light was extinguished, and the lever was retracted for the remainder of the trial.

Delay discounting.

Half of the rats (n = 24) completed a delay discounting task as previously described (Yates, Gunkel, et al., 2017). Delay discounting sessions consisted of five blocks of nine trials. The stimuli used to signal the beginning of each trial differed across blocks of trials (0-s delay: house light; 10-s delay: house light and left stimulus light; 30-s delay: house light and right stimulus light; 60-s delay: house light and both stimulus lights; 100-s delay: both stimulus lights). The first four trials in a block were forced-choice trials, in which only one lever was pseudo-randomly presented (no more than two consecutive presentations of the same lever). The remaining trials were free-choice trials, in which both levers were extended. Completion of the response requirement (FR 10) on one lever always resulted in immediate delivery of one food pellet, whereas completion of the response requirement (FR 10) on the other lever resulted in delayed delivery of four pellets. The responses did not have to occur consecutively; for example, if a rat responded on the left lever 9 times and then responded on the right lever 8 times, the tenth response on the left lever would lead to reinforcement. For 12 rats, the delay to delivery of the large magnitude reinforcer increased across blocks of trials (0, 10, 30, 60, 100 s); for 12 rats, the delay decreased across the session (100, 60, 30, 10, 0 s). Following the response requirement on either lever, the stimuli used to signal the beginning of each trial were extinguished, and each lever was retracted for the remainder of the trial. To compensate for the delay to the large magnitude reinforcer, the length of each trial increased across blocks of trials (0-s delay: 30 s; 10-s delay: 40 s; 30-s delay: 60 s; 60-s delay: 90 s; 100-s delay; 130 s). Each trial within a block of trials was the same length, regardless if the rat chose the small, immediate reinforcer or large, delayed reinforcer. If a response was not made within 20 s, the trial was scored as an omission, and all stimuli were extinguished for the remainder of the trial. Each session lasted 52.5 min.

Probability discounting.

Half of the rats (n = 24) completed a probability discounting procedure as previously described (Yates et al., 2016). Probability discounting sessions were similar to the delay discounting sessions described above, with the following exceptions: 1) the odds against (odds against = [1/probability-1]; Rachlin, Raineri, & Cross, 1991) increased (0, 3, 7, 15, 31) across the session for half of the rats (n = 12), but decreased across the session for the other half (31, 15, 7, 3, 0); 2) during each block of trials, there were eight forced-choice trials and 10 free-choice trials; and 3) each trial lasted 30 s, and the entire session lasted 45 min. It is important to note that we did not signal wins or losses in this variant of the probability discounting procedure.

Drug Treatments.

Training was considered completed when the following requirements were met: (1) rats could not show exclusive preference for one reinforcer relative to the other across each block of trials (e.g., as the delay/odds against increased across the session, the proportion of responses for the large magnitude reinforcer should decrease); and (2) no increasing or decreasing trends in the proportion of responses for the large magnitude reinforcer were observed across 3 sessions. Following training (32 sessions for delay discounting; 36 sessions for probability discounting; note: one rat trained on the descending schedule of the probability discounting procedure needed 44 sessions to reach stability), rats received injections of Ro 63–1908 (0, 0.1, 0.3, 1.0 mg/kg; s.c.) or CP-101,606 (0, 0.3, 1.0, 3.0 mg/kg; s.c.) 30 minutes prior to the session. The vehicle for Ro 63–1908 was saline with 5% Tween 80, whereas the vehicle for CP-101,606 was distilled water with 10% glacial acetic acid (pH = 5.8). Half of the rats received Ro 63–1908 injections first, and half received CP-101,606 injections first. The order in which each dose was administered was counterbalanced across rats. The doses and treatment time were chosen based on previous research, as the highest dose of each drug has been shown to be behaviorally active in measures of impulsivity (Higgins et al., 2016). Injections occurred once every four sessions. During the three sessions in between each injection, rats were tested in delay/probability discounting as normal.

Statistical Analyses

Delay/probability discounting data were quantified two different ways (see subsections below for analyses of baseline discounting and drug effects on impulsive/risky choice). First, the raw proportion of responses for the large magnitude reinforcer was plotted as a function of delay/odds against. Greater responding for the large magnitude reinforcer is considered to reflect self-control in delay discounting, but is interpreted as increased risky choice in probability discounting. Second, area under the curve (AUC; Myerson, Green, & Warusawitharana, 2001) was calculated as previously described (Borges, Kuang, Milhorn, & Yi, 2016). Specifically, an ordinal scale transformation of delay/odds against was performed before calculating AUCs. AUC values range from 0–1, with 0 indicating exclusive preference for the small magnitude reinforcer and 1 indicating exclusive preference for the large magnitude reinforcer. Smaller AUC values indicate greater impulsive choice in delay discounting but decreased risky choice in probability discounting.

Baseline discounting performance.

The raw proportion of responses was analyzed with a two-way mixed factor ANOVA (SPSS version 22.0; Armonk, NY), with schedule as a between-subjects factor and delay/probability as a within-subjects factor. When the assumption of sphericity was violated, degrees of freedom were corrected using Greenhouse-Geisser estimates of sphericity. A significant interaction was probed by conducting independent-samples t tests (with Bonferroni correction) across each schedule for each delay/probability. For the independent-samples t tests, if homogeneity of variance was violated, degrees of freedom were corrected using the Welch-Satterthwaite method. Statistical significance was defined as p < .05, except for cases in which independent-samples t tests were used, in which a Bonferroni adjustment was used, resulting in an adjusted alpha of .01. Partial eta squared was reported as a measure of effect size.

Baseline AUCs were analyzed with independent-samples t tests, as this variable was normally distributed. If homogeneity of variance was violated, degrees of freedom were corrected using the Welch-Satterthwaite method. Statistical significance was defined as p < .05. Cohen’s d was reported as a measure of effect size.

Drug effects on impulsive/risky choice.

First, the raw proportion of responses was analyzed with a three-way mixed factorial ANOVA, with schedule as a between-subjects factor and dose and delay/probability as within-subjects factors. When necessary, degrees of freedom were corrected using Greenhouse-Geisser estimates of sphericity. A main effect of dose was probed with a Bonferroni post hoc test, and significant interactions were probed with two-way ANOVAs and/or paired-samples t tests, when appropriate. If a rat did not respond during one block of trials following drug treatment, this subject’s data for all drug doses were excluded due to the fact that repeated measures ANOVA uses listwise deletion when there are missing data (note: this happened to one rat trained on the descending schedule following treatment of the highest dose of CP-101,606). Statistical significance was defined as p < .05, except for cases in which paired-samples t tests were used, in which a Bonferroni adjustment was used, resulting in an adjusted alpha level of .01. Partial eta squared was reported as a measure of effect size.

Second, AUCs were analyzed with linear mixed effects (LME) models using the NLME package (Pinheiro, Bates, DebRoy, Sarkar, & R Core Team, 2016) in R (version 3.3.1.; R Core Team, 2016). LME is an extension of repeated measures ANOVA that accounts for partially missing data and offers several advantages over ANOVA, such as increased power and decreased Type I error (see Young, Clark, Goffus, & Hoane, 2009 for a discussion on LME). The LME models defined schedule as a fixed, nominal between-subjects factor, dose as a fixed, nominal within-subjects variable, and subject as a random factor. Identical LME models were used to analyze the effects of Ro 63–1908 and CP-101,606 on discounting. Significant effects were probed using contrasts in R. Statistical significance was defined as p < .05. Although one rat did not respond during one block of trials following CP-101,606 (3.0 mg/kg), AUC was calculated for this subject and was included in the analysis.

Omissions were analyzed using Friedman tests (in SPSS) because the residuals of this variable are rarely normally distributed. Separate Friedman tests were conducted for each schedule; therefore, alpha levels were adjusted to .025. If there was a main effect of dose, separate Wilcoxon signed-rank tests were conducted to compare each dose to vehicle. A Bonferroni correction was used, such that statistical significance was defined as p < .017.

Results

For simplicity, the outcomes of each inferential test (ANOVA, LME, Friedman tests) are presented in Table 2 (baseline performance), Table 3 (drug effects on delay discounting), and Table 4 (drug effects on probability discounting). The results of each analysis will be briefly presented below.

Table 2:

Results of the inferential tests used to analyze the raw proportion of responses for the large magnitude reinforcer and AUCs at the end of baseline training for rats trained in either delay discounting or probability discounting.

Delay Discounting					Probability Discounting
Raw Proportion of Responses					Raw Proportion of Responses
Factor	DF	F Statistic	P	ηp2	Factor	DF	F Statistic	P	ηp2
Delay	1.714, 37.703	118.543	<.001	.843	Delay	1.701, 37.412	166.274	<.001	.883
Schedule	1, 22	98.025	<.001	.817	Schedule	1, 22	6.574	.018	.230
Delay × Schedule	1.714, 37.703	16.513	< .001a	.429	Delay × Schedule	1.701, 37.412	3.869	0.036b	.150
AUC					AUC
Factor	DF	t Statistic	P	d	Factor	DF	t Statistic	P	d
Schedule	22	−9.802	<.001	−4.002	Schedule	22	−2.666	.014	−1.089

^aTo probe the significant interaction, independent-samples t tests (with Bonferroni correction) were used to compare the proportion of responses between rats trained on the ascending and descending schedules at each delay. Rats trained on the descending schedule showed greater responding for the large magnitude reinforcer at each delay (all t’s ≥ −4.905, all p’s < .001, all d’s ≥ 2.002), with the exception of the 0-s delay, t(11.000) = −2.756, p = .019, d = 1.166

^bTo probe the significant interaction, independent-samples t tests (with Bonferroni correction) were used to compare the proportion of responses between rats trained on the ascending and descending schedules at each odds against. There were no significant differences between rats trained on the ascending and descending schedules at any of the odds against (all t’s ≤ −2.817, all p’s > .011, all d’s ≥ .063).

Table 3:

Results of the inferential tests used to analyze the raw proportion of responses for the large magnitude reinforcer, AUCs, and omissions following administration of Ro 63–1908 and CP-101,606 in delay discounting.

Ro 63–1908					CP-101,606
Raw Proportion of Responses					Raw Proportion of Responses
Factor	DF	F Statistic	P	ηp2	Factor	DF	F Statistic	P	ηp2
Delay	1.932, 42.496	161.156	<.001	.880	Delay	1.807, 33.945	192.045	< .001	.901
Schedule	1,22	95.619	<.001	.813	Schedule	1,22	115.905	< .001	.847
Dose	3,66	8.030	<.001	.267	Dose	3,63	0.853	.470	.039
Delay × Schedule	1.932, 42.496	17.407	<.001	.442	Delay × Schedule	1.807, 37.945	27.776	< .001	.569
Delay × Dose	5.831, 128.282	1.606	.153	.068	Delay × Dose	6.103, 128.168	1.050	.403	.048
Schedule × Dose	3,66	7.845	< .001a	.263	Schedule × Dose	3,63	0.455	.470	.021
Delay × Dose × Schedule	5.831, 128.282	1.646	.142	.070	Delay × Dose × Schedule	6.103, 128.168	0.911	.536	.042
AUC					AUC
Factor	DF	F Statistic	P		Factor	DF	F Statistic	P
Schedule	1,22	98.519	< .001		Schedule	1,22	63.645	< .001
Dose	3,66	7.244	< .001		Dose	3,66	0.987	.405
Schedule × Dose	3,66	7.520	< .00 lb		Schedule × Dose	3,66	0.636	.594
Omissions					Omissions
Schedule	DF	χ2	n	P	Schedule	DF	χ2	n	P
Ascending	3	4.263	12	.234	Ascending	3	2.000	12	.572
Descending	3	0.143	12	.986	Descending	3	3.667	12	.300

^aTo probe the significant interaction, separate two-way ANOVAs were conducted for each schedule, with delay and dose as within-subjects factors. For rats tested in the ascending schedule, there was a main effect of delay only, F(1.212, 13.332) = 83.157, p < .001, η_p² = .883. For rats tested in the descending schedule, there were main effects of delay, F(1.820, 20.019) = 98.174, p < .001, η_p² = .899, and dose, F(3, 33) = 11.334, p < .001, η_p² = .507, as well as a significant delay × dose interaction, F(12, 132) = 2.256, p = .012, η_p² = .170. Ro 63–1908 (1.0 mg/kg) significantly decreased responding at the 30-s, t(11) = 3.261, p = .008, d = −1.040, and 60-s, t(11) = 3.207, p = .008, d = −1.158, delays relative to vehicle.

^bContrasts showed that Ro 63−1908 did not affect AUCs in rats trained on the ascending schedule (all p’s ≥.208), whereas Ro 63−1908 (1.0 mg/kg) significantly decreased AUCs (p = .008).

Table 4:

Results of the inferential tests used to analyze the raw proportion of responses for the large magnitude reinforcer, AUCs, and omissions following administration of Ro 63–1908 and CP-101,606 in probability discounting.

Ro 63–1908					CP-101,606
Raw Proportion of Responses					Raw Proportion of Responses
Factor	DF	F Statistic	P	ηp2	Factor	DF	F Statistic	P	ηp2
Probability	1.985, 43.666	155.276	<.001	.876	Probability	1.533, 33.717	198.688	< .001	.900
Schedule	1,22	3.247	.085	.129	Schedule	1.22	16.037	.001	.422
Dose	2.273, 49.999	1.188	.317	.051	Dose	2.101. 46.233	0.132	.886	.006
Probability × Schedule	1.985, 43.666	4.401	.018	.167	Probability × Schedule	1.533, 33.717	12.104	< .001	.355
Probability × Dose	5.182, 114.010	0.668	.782	.029	Probability × Dose	5.160, 113.526	1.536	.182	.065
Schedule × Dose	2.273, 49.999	4.234	.016a	.161	Schedule × Dose	2.101, 46.233	3.048	.055	.122
Probability × Dose × Schedule	5.182, 114.010	1.620	.158	.069	Probability × Dose × Schedule	5.160, 113.526	2.209	.056	.091
AUC					AUC
Factor	DF	F Statistic	P		Factor	DF	F Statistic	P
Schedule	1.22	10.157	.004		Schedule	1,22	24.946	< .001
Dose	3. 66	1.074	.366		Dose	3,66	0.093	.964
Schedule × Dose	3.66	4.249	.008b		Schedule × Dose	3,66	3.156	0.031c
Omissions					Omissions
Schedule	DF	χ2	n	P	Schedule	DF	χ2	n	P
Ascending	3	1.462	12	.691	Ascending	3	3.000	12	.392
Descending	3	6.000	12	.122	Descending	3	0.000	12	1.000

^aTo probe the significant interaction, separate two-way ANOVAs were conducted for each schedule, with probability and dose as within-subjects factors. For rats tested in the ascending schedule, there was a main effect of probability only, F(1.454, 15.990) = 77.862, p < .001, η_p² = .876, although there was a trend for Ro 63-1908 to increase responses for the large, probabilistic reinforcer, F(3, 33) = 2.706, p = .061, η_p² = .197. For rats tested in the descending schedule, there was a main effect of probability only, F(1.717, 18.883) = 81.503, p < .001, η_p² = .881, although there was a trend Ro 63-1908 to decrease responses for the large, probabilistic reinforcer, F(3, 33) = 2.720, p = .060, η_p² = .198.

^bContrasts showed that Ro 63-1908 (0.3 mg/kg) significantly increased AUCs when an ascending schedule was used (p = .004), whereas Ro 63-1908 (1.0 mg/kg) significantly decreased AUCs when a descending schedule was used.

^cContrasts showed that CP-101,606 (1.0 mg/kg) significantly increased AUCs when an ascending schedule was used (p = .040), whereas CP-101,606 (3.0 mg/kg) significantly decreased AUCs when a descending schedule was used (p = .046).

Baseline Discounting Performance

Overall, the proportion of responses for the large magnitude reinforcer decreased as the delay to or odds against obtaining reinforcement increased. In delay discounting, rats trained on the ascending schedule showed greater impulsive choice relative to rats trained on the descending schedule (Figs. 1a and 1b). In probability discounting, rats trained on the ascending schedule showed greater risk aversion relative to rats trained on the descending schedule (Figs. 1c and 1d).

Figure 1.

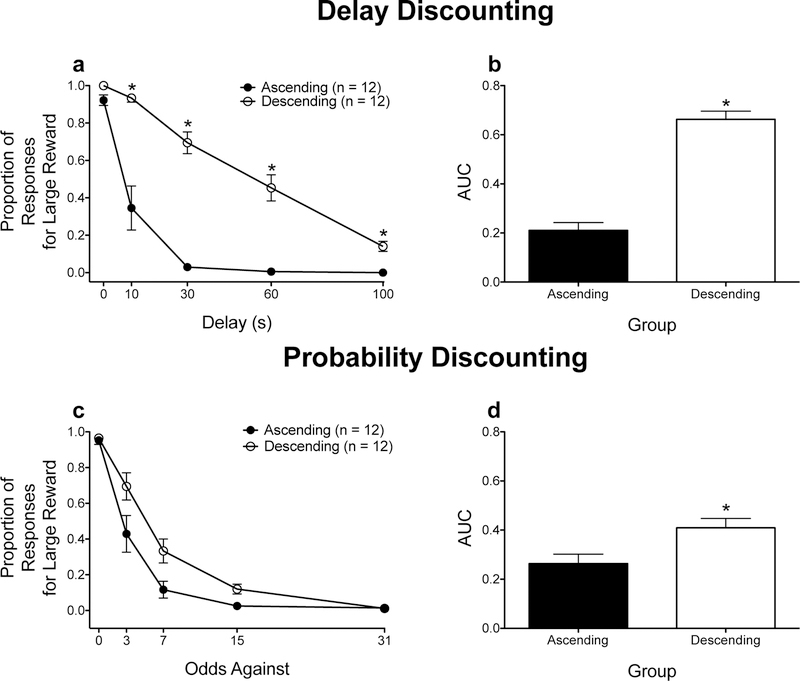
Discounting performance at the end of baseline training for rats trained in either delay discounting (a-b) or probability discounting (c-d). Mean (± SEM) raw proportion of responses for the large magnitude reinforcer (a and c). *p < .01, relative to rats trained on the ascending schedule (Bonferroni correction). Mean (± SEM) AUC values (b and d). *p < .05, relative to rats trained on the ascending schedule.

Drug Effects on Impulsive/Risky Choice

Delay discounting.

The order in which delays were presented modulated the effects of Ro 63–1908 on impulsive choice. When delays increased across the session, Ro 63–1908 did not alter either the raw proportion of responses for the large magnitude reinforcer at any of the delays tested or AUCs (Figs. 2a and 2c). However, Ro 63–1908 (1.0 mg/kg) significantly increased impulsive choice in rats trained on the descending schedule (Figs. 2b and 2d). CP-101, 606 did not affect impulsive choice in either schedule (Fig. 3).

Figure 2.

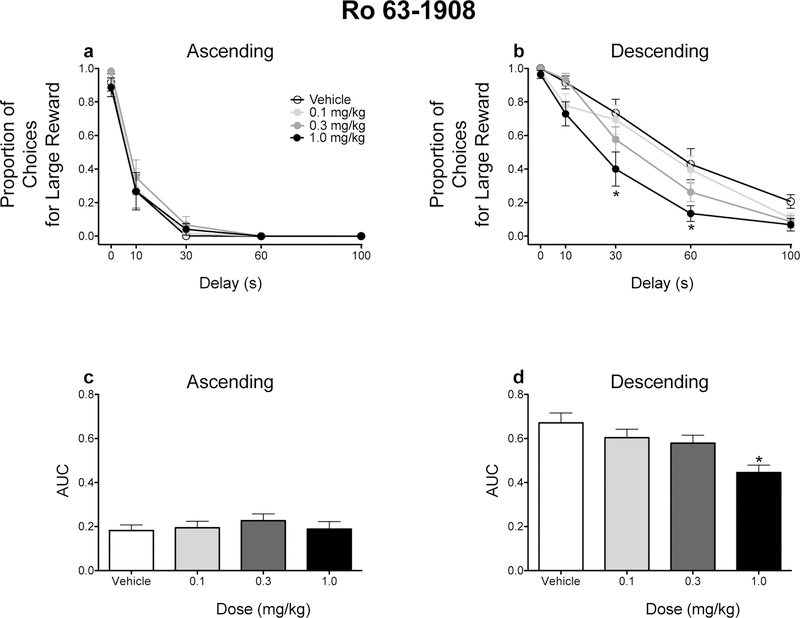
Effects of Ro-63–1908 on delay discounting performance. Mean (± SEM) raw proportion of responses for the large, delayed reinforcer for rats trained in the ascending (a) and descending (b) schedules. p < .01, relative to vehicle (Bonferroni correction). Mean (± SEM) AUC values for rats trained in the ascending (c) and descending (d) schedules. *p < .05, relative to vehicle. n =12 each dose and each schedule.

Figure 3.

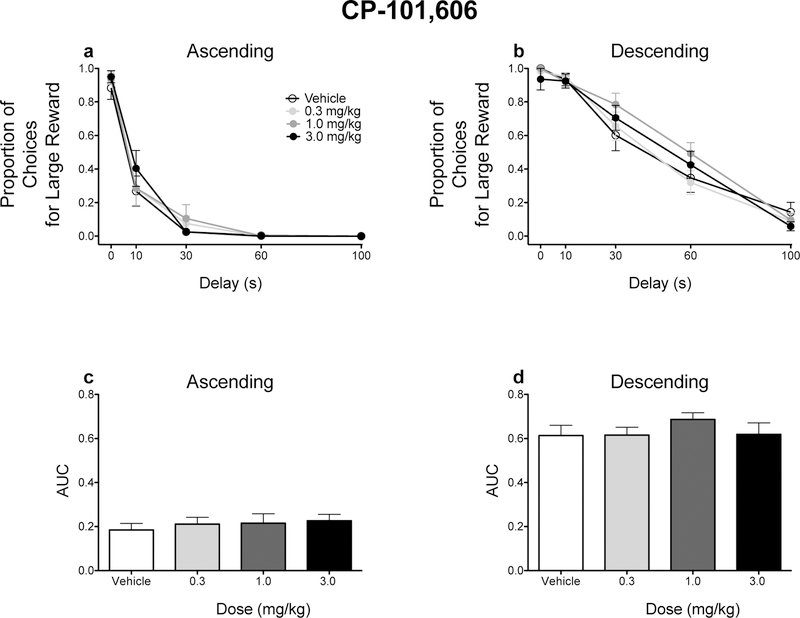
Effects of CP-101,606 on delay discounting performance. Mean (± SEM) raw proportion of responses for the large, delayed reinforcer for rats trained in the ascending (a) and descending (b) schedules. Mean (± SEM) AUC values for rats trained in the ascending (c) and descending (d) schedules. n =12 each dose and each schedule. Note: in panel b, one rat did not respond during the 10-s delay following administration of CP-101,606 (3.0 mg/kg).

Probability discounting.

Although Ro 63–1908 did not significantly alter the raw proportion of responses for the large magnitude reinforcer (Figs. 4a and 4b), the order in which probabilities were presented modulated the effects of Ro 63–1908 on risky choice as assessed with AUCs. When the odds against obtaining reinforcement increased (e.g., probabilities decreased) across the session, an intermediate dose of Ro 63–1908 (0.3 mg/kg) increased AUCs (Fig. 4c). Conversely, the highest dose of Ro 63–1908 (1.0 mg/kg) significantly decreased AUCs (Fig. 4d). Similar effects were observed following CP-101,606 administration (Fig. 5).

Figure 4.

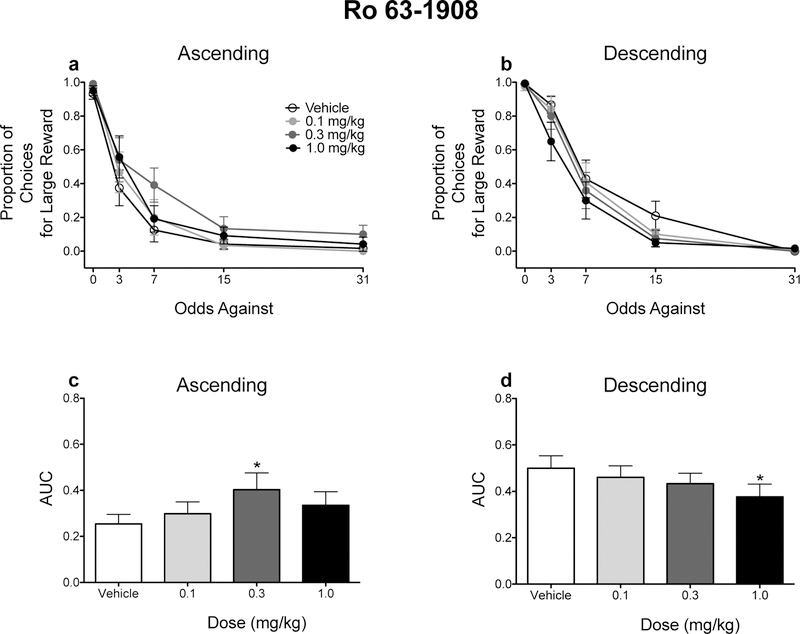
Effects of Ro-63–1908 on probability discounting performance. Mean (± SEM) raw proportion of responses for the large, probabilistic reinforcer for rats trained in the ascending (a) and descending (b) schedules. Mean (± SEM) AUC values for rats trained in the ascending (c) and descending (d) schedules. *p < .05, relative to vehicle. n =12 each dose and each schedule.

Figure 5.

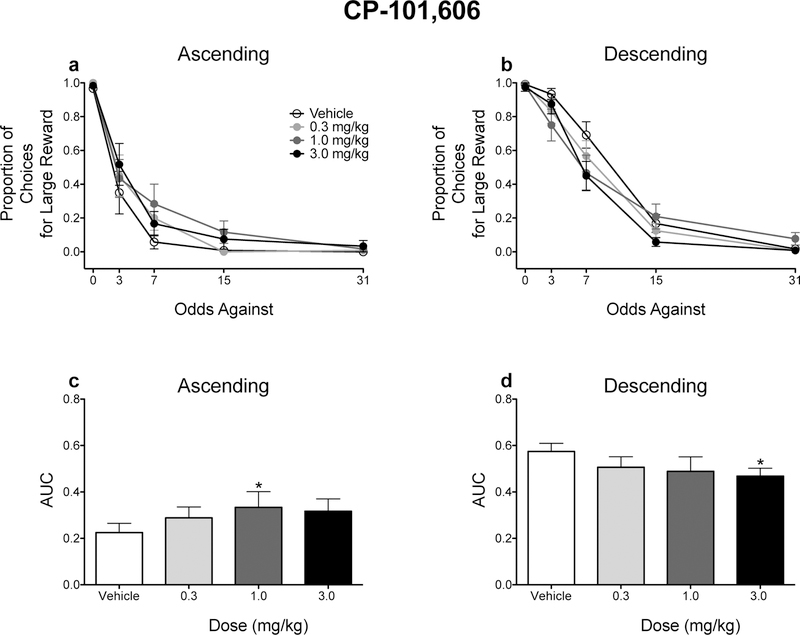
Effects of CP-101,606 on probability discounting performance. Mean (± SEM) raw proportion of responses for the large, probabilistic reinforcer for rats trained in the ascending (a) and descending (b) schedules. Mean (± SEM) AUC values for rats trained in the ascending (c) and descending (d) schedules. *p < .05, relative to vehicle. n =12 each dose and each schedule.

Omissions.

Table 5 shows the number of omissions following administration of Ro 63–1908 and CP-101,606. Neither drug significantly altered omissions in either delay or probability discounting.

Table 5:

Mean (± SEM) omissions in delay and probability discounting for rats trained on the ascending and descending schedules following administration of Ro 63–1908 and CP-101,606.

Delay Discounting						Probability Discounting
		Ascending		Descending		Ascending		Descending
Drug	Dose (mg/kg)	M	SEM	M	SEM	M	SEM	M	SEM
Ro 63–1908	0	0.250	0.131	0.083	0.083	0.250	0.179	0.167	0.112
Ro 63–1908	0.1	0.083	0.083	0.083	0.083	0.250	0.131	0.000	0.000
Ro 63–1908	0.3	0.000	0.000	0.333	0.333	0.083	0.083	0.000	0.000
Ro 63–1908	1.0	0.250	0.131	0.167	0.167	0.167	0.112	0.000	0.000
CP-101.606	0	0.417	0.336	0.000	0.000	0.417	0.149	0.000	0.000
CP-101.606	0.3	0.083	0.083	0.000	0.000	0.833	0.501	0.000	0.000
CP-101.606	1.0	0.000	0.000	0.083	0.083	0.583	0.500	0.000	0.000
CP-101.606	3.0	0.083	0.083	1.000	0.913	0.167	0.112	0.000	0.000

Discussion

Our hypotheses were not supported. Ro 63–1908 (1.0 mg/kg) increased impulsive choice, but only when the delay to obtaining the large magnitude reinforcer decreased across the session. However, we did not observe a decrease in impulsive choice when the delays increased across the session as previously shown (Higgins et al., 2016). In probability discounting, Ro 63–1908 (0.3 mg/kg) and CP-101,606 (1.0 mg/kg) increased risky choice when the odds against obtaining the large magnitude reinforcer increased across the session, but Ro 63–1908 (1.0 mg/kg)/CP-101,606 (3.0 mg/kg) increased risk aversion when the odds against decreased across the session. Overall, the results of this study show that the order in which delays/probabilities are presented modulates the effects of GluN2B-selective antagonists on impulsive and risky decision making.

Baseline Differences in Ascending and Descending Schedules

Some studies report differential baseline performance in delay discounting when ascending and descending schedules are used (e.g., Aparicio, Elcoro, & Alonso-Alvarez, 2015; Fox, Hand, & Reilly, 2008; Yates, Rogers, et al., 2017), whereas others show no differences in impulsive choice (Maguire, Henson, & France, 2014; Slezak & Anderson, 2009; Tanno et al., 2014). Interestingly, Fox et al. (2008) show that descending schedules lead to decreased preference for the large, delayed reinforcer, whereas our work (current study; Yates, Rogers, et al., 2017) shows that descending schedules lead to increased preference for this alternative. In probability discounting, the present results are consistent with our previous work (Yates et al., 2016) showing that descending schedules lead to increased responses for the large, probabilistic reinforcer, but are inconsistent with the findings reported by St Onge et al. (2010), who showed no differences in ascending and descending schedules (although they did report a larger proportion of responses for the large magnitude reinforcer when probabilities were randomized across the session). It is not entirely clear why these inconsistencies exist. Perhaps, these inconsistent findings reflect idiosyncratic differences across studies (e.g., using different delays/probabilities, using different reinforcer magnitudes [1 vs. 3 or 1 vs. 4], using a different number of training sessions, etc.). Considering numerous factors can influence responding for large, delayed/probabilistic reinforcers (see Yates, 2018 for a discussion), future studies are needed to systematically determine when ascending and descending schedules modulate impulsive/risky choice.

Contribution of the GluN2B to Impulsive Choice

Although we cannot ascertain as to why baseline differences occur when ascending and descending schedules are used, an important finding of the current study is that the order in which delays are presented influences the effects of Ro 63–1908 on impulsive choice, as rats trained on the descending schedule responded less for the large magnitude reinforcer following Ro 63–1908 (1.0 mg/kg) treatment. These results are consistent with other reports showing that delay presentation order alters the effects of amphetamine (Maguire et al., 2014; Tanno et al., 2014; but see Slezak & Anderson, 2009), methylphenidate (Tanno et al., 2014), and JNJ 16259685, a metabotropic glutamate receptor (mGluR) 1 antagonist (Yates, Rogers, et al., 2017), on delay discounting performance. Importantly, the effects reported with Ro 63–1908 in the current study are not due to increased perseverative responding, as Ro 63–1908 did not increase responses for the large magnitude reinforcer when the delays increased across the session. Because rats trained on the descending schedule responded more for the large magnitude reinforcer relative to rats trained on the ascending schedule, the present results could reflect a baseline effect, as opposed to a delay presentation order effect. However, this seems unlikely, as Maguire et al. (2014) and Tanno et al. (2014) report differential effects of psychostimulants on delay discounting performance even though baseline performance was similar across each schedule. Related to this potential caveat, rats exhibited near exclusive preference for the small, immediate reinforcer for three of the five delays tested; thus, we cannot rule out the possibility that Ro 63–1908 causes a general increase in impulsive choice. Specifically, we cannot observe a decrease in responding for the large reinforcer at the 30-s, 60-s, and 100-s delays due to a floor effect. Furthermore, rats, when treated with vehicle, respond for the large magnitude reinforcer only ~25% of the time at the 10-s delay, thus making further decreases in responding at this delay somewhat difficult to observe. However, this potential explanation seems unlikely, as Higgins et al. (2016) found that Ro 63–1908 decreases impulsive choice when an ascending schedule is used.

The finding that Ro 63–1908 did not alter impulsive choice when the delay to reinforcement increased across the session is surprising, considering blocking GluN2B-containing NMDA receptors increases preference for the large, delayed reinforcer (Higgins et al., 2016). There are two major procedural difference across studies that may account for the discrepant findings observed in the current study and those reported by Higgins et al. (2016). First, the delays used by Higgins et al. (2016) (0–40 s) are shorter than those used in the current study (0–100 s). Perhaps using delays consistent to those reported in Higgins et al. (2016) would have allowed us to observe increases in the proportion of responses for the large magnitude reinforcer following Ro 63–1908 administration. Second, whereas Higgins et al. (2016) used an FR 1 schedule of reinforcement, we used an FR 10. Manipulating the response requirement can alter an animal’s sensitivity to delayed reinforcement, as higher FR values promote increased self-control (Huskinson & Anderson, 2013). There is some evidence, albeit indirect, that suggests different response requirements can modulate drug effects in delay discounting procedures. Specifically, MK-801 decreases impulsive choice when an FR 1 schedule of reinforcement is used (Higgins et al., 2016; Yates et al., 2015) but has no effect on choice when an FR 10 is used (Yates, Gunkel, et al., 2017). Also, when delays are increased across the session, ketamine decreases preference for a large magnitude reinforcer at the 0-s delay when an FR 1 schedule is used (Yates et al., 2015) but does not alter performance in this task when an FR 10 is used (Yates, Gunkel, et al., 2017). Thus, future studies examining if the range of delays and response requirement modulates drug effects in discounting paradigms are of interest.

Contribution of the GluN2B to Risky Choice

Similar to the effects observed in delay discounting, Ro 63–1908 differentially affected probability discounting performance in rats trained on the ascending and descending schedules. When the odds against obtaining reinforcement increased across the session, Ro 63–1908 (0.3 mg/kg) significantly increased risky decision making; however, when the odds against decreased across the session, Ro 63–1908 (1.0 mg/kg) increased risk aversion. Similar effects were observed with CP-101,606, as the intermediate dose (1.0 mg/kg) increased risky choice when the odds against increased across the session, but the highest dose (3.0 mg/kg) increased risk aversion when the odds against decreased. Explaining the biphasic dose effect curve observed for the ascending schedule (i.e., only the intermediate dose significantly altered AUCs) is somewhat difficult. Even though Ro 63–1908/CP-101,606 are highly selective for the GluN2B subunit compared to ifenprodil (Ro 63–1908: GluN2B IC₅₀ of ~0.003–0.01 μM vs. GluN2A IC₅₀ of > 100 μM; Gill et al., 2002; CP-101,606: GluN2B IC₅₀ of 0.008–0.06 μM vs. GluN2A IC₅₀ of > 10 μM; see Mony et al., 2009 for a review; ifenprodil: GluN2B IC₅₀ of 0.21–0.81 μM vs. GluN2A IC₅₀ of 92.5–324.8 μM; Avenet et al., 1996), these drugs still show some non-selectivity. Ro 63–1908/CP-101,606 have some affinity for adrenergic receptors (although the IC₅₀ values are higher for Ro 63–1908 [~3.5 μM; Gill et al., 2002] and CP-101,60 [~20 μM; Chenard et al., 1995] compared to ifenprodil [~.11 μM; Chenard et al., 1991]). Ro 63–1908 also has shows affinity for sigma receptors and dopamine D₂-like receptors (Gill et al., 2002). Thus, the biphasic dose effect curve observed with Ro 63–1908/CP-101,606 may potentially be explained by their actions on other neurotransmitter systems at a lower dose. However, Montes, Stopper, and Floresco (2015) show that blocking adrenergic α2 receptors decreases responding for the large magnitude reinforcer at the 100% block of trials when the probabilities decrease within a session, which is inconsistent with what we observed with Ro 63–1908/CP-101,606. Although Ro 63–1908 has weak antagonistic effects on D₂-like receptors (Gill et al., 2002), St Onge and Floresco (2009) show that blocking D₂/D₃ receptors decreases preference for a large magnitude reinforcer when the probabilities of obtaining that alternative decrease across the session, which is the opposite of what we observed with Ro 63–1908 in this schedule. Overall, the effects of Ro 63–1908 on probability discounting do not appear to be due to its actions on noradrenergic or dopamine receptors. Because the contribution of sigma receptors to impulsive/risky decision making has not been elucidated, we cannot rule out the possibility that the results observed with Ro 63–1908 are due, at least partly, to these receptors.

Regardless of this potential limitation, the current findings add to previous studies showing differential drug effects across probability discounting paradigms using ascending and descending schedules (e.g., St Onge et al., 2010; Yates et al., 2016). Specific to the glutamatergic system, MK-801 increases risk-taking behavior when the odds against obtaining reinforcement increase across the session (Yates et al., 2015; Yates et al., 2016), but increases risk aversion when a descending schedule is used (Yates et al., 2016). Somewhat similarly, ifenprodil, which has some affinity for GluN2B-containing NMDA receptors, increases risk aversion, but only when the odds against decrease across the session (Yates et al., 2016). One potential explanation for the differential effects observed following Ro 63–1908 treatment in probability discounting is an increase in perseverative responding. Because rats trained on the ascending schedule initially respond for the large magnitude reinforcer when its delivery is certain, they develop a bias for the lever associated with this reward alternative, even as the probability of obtaining that reinforcer decreases. Conversely, when rats are trained on the descending schedule, they are more likely to choose the small, certain reinforcer at the beginning of the session; thus, they continue to respond on the lever associated with this alternative, even as the probability of earning that reinforcer increases across the session. An increase in perseverative responding does not seem to provide a full account of the current results, as Ro 63–1908 did not produce opposite effects in the ascending/descending delay discounting procedures. Instead, the results may reflect increased perseveration on the perceived value of the probabilistic reinforcer. For example, in the ascending schedule, the large reinforcer is delivered with a probability of 1 (odds against of 0). As the odds against obtaining reinforcement increase, rats continue to respond as if this reward alternative is more advantageous, despite the decreased probability of earning that reward alternative (see St Onge et al., 2010 for a further discussion).

Limitations

Although CP-101,606 similarly altered probability discounting performance compared to Ro 63–1908, it did not significantly alter choice for the large, delayed reinforcer in delay discounting. This finding is consistent with a previous study showing no statistically significant effect of CP-101,606 on impulsive choice (Higgins et al., 2016). Even though CP-101,606 and Ro 63–1908 are antagonists at GluN2B-containing NMDA receptors, there are differences across these ligands that could account for the null effects observed with CP-101,606 in delay discounting. Ro 63–1908 is more selective for the GluN2B subunit compared to CP-101,606 (see previous subsection for IC₅₀ values). Additionally, compared to other antagonists at GluN2B-containing NMDA receptors, CP-101,606 shows higher affinity for NMDA receptors that contain two GluN2B subunits compared to NMDA receptors that contain GluN2A and GluN2B subunits (Chazot, Lawrence, & Thompson, 2002). However, these differences still do not provide an account as to why CP-101,606 is capable of altering choice between large, probabilistic and small, guaranteed alternatives but not capable of altering delay discounting performance. This highlights one weakness of behavioral pharmacology experiments that aim to ascertain the contribution of a neurotransmitter system to impulsive/risky choice: the use of acute, systemic injections of Ro 63–1908/CP-101,606 does not allow us to fully elucidate the contribution of the GluN2B subunit to delay/probability discounting performance. Specifically, systemic injections do not allow us to isolate which GluN2B-containing brain regions are important mediators of impulsive/risky choice. Future studies can better determine the precise role of the GluN2B subunit to impulsive/risky decision making by using techniques that selectively reduce the number of GluN2B-containing NMDA receptors in distinct regions of the brain (“knockdown” models). So far, research has demonstrated that rats injected with siRNAs that reduce GluN2B expression in nucleus accumbens show attenuated cue- and drug-induced reinstatement of heroin seeking (Shen et al., 2011) and show decreased conditioned place preference for morphine (Kao, Huang, & Tao, 2011). Considering brain regions such as the prefrontal cortex and nucleus accumbens are implicated in impulsive (see Cardinal, 2006 for a review) and risky choice (Orsini, Trotta, Bizon, & Setlow, 2015; St Onge & Floresco, 2010; Stopper & Floresco, 2011; Stopper, Green, & Floresco, 2014; Zeeb, Baarendse, Vanderschuren, & Winstanley, 2015) and are critical mediators of substance use disorders (see Volkow, Fowler, & Wang, 2003 for a review), injecting siRNA against the GluN2B subunit in these regions would be a logical next step to further understanding the role of this subunit on delay/probability discounting.

Another limitation to the current study is the use of male rats only. In order to be consistent with previous studies examining the contribution of the GluN2B subunit to impulsive choice (Higgins et al., 2016) and risky choice (Yates et al., 2016), we did not test female rats. Whereas females/women show decreased risky choice relative to males/men (Orsini, Willis, Gilbert, Bizon, & Setlow, 2016; Sidlauskaite et al., in press), sex differences are less conclusive in measures of impulsive choice. When sex differences are observed in delay discounting paradigms, females/women tend to be more impulsive than males/men (see Weafer & de Wit, 2014 for a review). However, other studies have not observed sex differences in impulsive choice (Eubig, Noe, Floresco, Sable, & Schantz, 2014; Perry, Nelson, & Carroll, 2008; Smethells, Swalve, Eberly, & Carroll, 2016; Weston, Weston, Allen, & Cory-Slechta, 2014). Even when baseline differences are not observed across sex, studies have shown that pharmacological manipulations differentially alter impulsive/risky choice in males and females. For example, in delay discounting, atomoxetine decreases impulsive choice in male rats but not in female rats (Smethells et al., 2016; note: this effect is only observed when cocaine is used as the reinforcer). Additionally, ethanol increases risky choice in male rats but not in female rats (Wallin-Miller, Chesley, Castrillon, & Wood, 2017). One potential future direction is to determine if sex differences observed in impulsive/risky choice are due to differential GluN2B expression. Another future direction is to determine if GluN2B-selective ligands differentially alter choice between large, delayed/probabilistic and small, immediate/guaranteed alternatives in male and female rats.

One limitation of the procedure used in the current experiment is that dissociating a drug’s effects on sensitivity to delayed reinforcement from other behavioral processes can be difficult. For example, if a drug decreases impulsive choice, this is usually interpreted as a decrease in delay discounting. However, the drug may have altered response bias (e.g., rats show a preference for the lever associated with the large magnitude reinforcer that is independent of delay). Because the proportion of responses is capped at 1.0, one cannot observe an appreciable increase in the proportion of responses for the large magnitude reinforcer when its delivery is immediate (due to a ceiling effect). Thus, we cannot see a parallel upward shift in the discounting function. One way to avoid this limitation is to use a procedure that does not allow an animal to show exclusive preference for one alternative relative to another at each delay/probability (e.g., Aparicio et al., 2015).

Related to the previous point, in the Evenden and Ryan (1996) procedure, the ratio between the large and small magnitude reinforcer is kept constant throughout the experiment (in this case, 1 vs. 4 pellets); therefore, determining if pharmacological manipulations alter sensitivity to reinforcer magnitude is not feasible. To determine if a drug alters sensitivity to reinforcer magnitude, control experiments can be conducted in which subjects respond for reinforcers that differ in magnitude only. For example, Pitts, Cummings, Cummings, Woodcock, and Hughes (2016) tested the effects of methylphenidate on sensitivity to delayed reinforcement and sensitivity to reinforcer magnitude by conducting two experiments. In experiment 1, the delay to reinforcement differed across two reinforcer alternatives but magnitude was held constant. In experiment 2, the magnitude of each alternative differed but delay was held constant. Pitts et al. (2016) found that methylphenidate decreased sensitivity to delayed reinforcement and sensitivity to reinforcer magnitude. One potential future direction will be to determine if GluN2B-selective antagonists alter sensitivity to delayed reinforcement and/or sensitivity to reinforcer magnitude.

Conclusions

In the current experiment, we used discounting procedures that are modeled after those developed by Evenden and Ryan (1996). This procedure has been used extensively to measure the neurochemical underpinnings of impulsive/risky choice (see Yates, 2018 for a list of references using this procedure to measure impulsive choice). Because the order in which delays/probabilities are presented can modulate drug effects, determining the neurochemical basis of impulsive and risky decision making becomes challenging when using this procedure. Historically, delay/probability discounting procedures increase the delays to/odds against reinforcement across the session. For example, studies that have examined the contribution of the glutamatergic system to impulsive choice have primarily used ascending schedules (Floresco et al., 2008; Higgins et al., 2016; Yates et al., 2015; Yates, Gunkel, et al., 2017). This can be especially problematic when trying to determine if a drug is a potential pharmacotherapy for impulse-control disorders. Related to the current results, Higgins et al. (2016) reported that Ro 63–1908 increases choice for a large, delayed reinforcer. At first glance, these results suggest that Ro 63–1908 is effective at reducing impulsive choice. However, when a descending schedule is used, Ro 63–1908 increases impulsive choice. To fully elucidate the neurobiological basis of impulsive/risky choice, studies should include conditions in which delays/probabilities are increased and decreased across the session, as opposed to using just one schedule, or they should randomize the order in which delays/probabilities are presented within a session.