Mathematical modelling of in-situ microaerobic desulfurization of biogas from sewage sludge digestion

Highlights

• •An extension of the ADM1 model for the microaeration process is proposed.
• •The model was tested with data from pilot scale digester operated for 200 d.
• •Results indicate that the model can be used to predict the digester behavior.
• •The addition of a retention parameter for the SOB improved the model performance.

Abstract

Microaeration can be used to cost-effectively remove in-situ H₂S from the biogas generated in anaerobic digesters. This study is aimed at developing and validating an extension of the Anaerobic Digestion Model n°1 capable of incorporating the main phenomena which occurs during microaeration. This innovative model was implemented and tested with data from a pilot scale digester microaerated for ∼ 200 d. The results showed that despite the model’s initial ability to predict the digester’s behavior, its predicted performance was improved by calibrating the most influential parameters. The model’s prediction potential was largely enhanced by adding retention parameters that account for the activity of sulfide oxidizing bacteria retained inside the anaerobic digester, which have been consistently shown to be responsible for a large share of the H₂S removed.

1. Introduction

Microaeration, which consists of dosing of a limited amount of air to anaerobic digesters, has emerged as one of the most cost-effective technologies for H₂S removal from biogas. The microaerophilic conditions created by air supply support the partial oxidation of H₂S to S° by the action of sulfide-oxidizing bacteria. In Europe, this process is gaining increasing attention and several full-scale plants have already implemented this technology to remove H₂S from biogas [1]. Indeed, microaeration has been traditionally employed to control H₂S in full-scale digesters treating agricultural waste. Recently, this technology has been successfully applied to the treatment of a broad range of biogas flow rates (7 L d⁻¹- 250 m³ h⁻¹), H₂S concentrations (2500 - 67,000 ppm_v) [2] and substrates (from industrial wastewaters to WWTP sludge) [3,4]. Interestingly, microaeration does not inhibit organic matter removal nor CH₄ productivity [5,6]. On the contrary, significant enhancements in organic matter hydrolysis and methanogenic activity have been reported, likely due to the suppression of the inhibition caused by sulfide [1,7,8]. Both air and O₂ can be used to support biogas desulfurization with similar H₂S removal efficiencies. In this context, the use of concentrated O₂ resulted in lower operating costs when compared to ferric salt addition for biogas desulfurization in wastewater treatment plants (WWTP) [9].

Mathematical modeling of (bio)chemical processes is nowadays considered of paramount importance for process analysis, control and optimization. Modeling anaerobic digestion (AD) allows us to get more insight on process performance, evaluate different scenarios and hypotheses, facilitates a virtual plant for assessment and training, and represents a valuable tool for process control or experimental design. Therefore, process modelling helps minimize the experimental work needed, which translates into resource savings and risk minimization. Furthermore, modeling is recognized as one the future needs to be addressed in AD [10]. Recent studies have developed models for the microaerobic process in AD, for both liquid effluents and for systems with immobilized biomass, UASB [11] and biotrickling filters [12]. Therefore, to the best of our knowledge, no mathematical model has been so far adapted and implemented for the microaerobic H₂S removal during sewage sludge AD in a continuous stirred reactor. The anaerobic digestion model 1 (ADM1) developed by the IWA task group [13] is the most recognized and widely used model to describe the AD process. In this context, extensions of the ADM1 have also been published in order to describe particular processes not considered in the original model. These extensions have tackled biological sulfate reduction [14,15], inorganic compounds and solid precipitation [16] and phenolic compounds biodegradation [17], among others.

This study is aimed at developing, implementing and testing a mathematical model of the microaerobic digestion process based on the ADM1 model using experimental data from pilot-scale anaerobic digesters operated under microaerobic conditions.

2. Material and methods

2.1. Experimental data

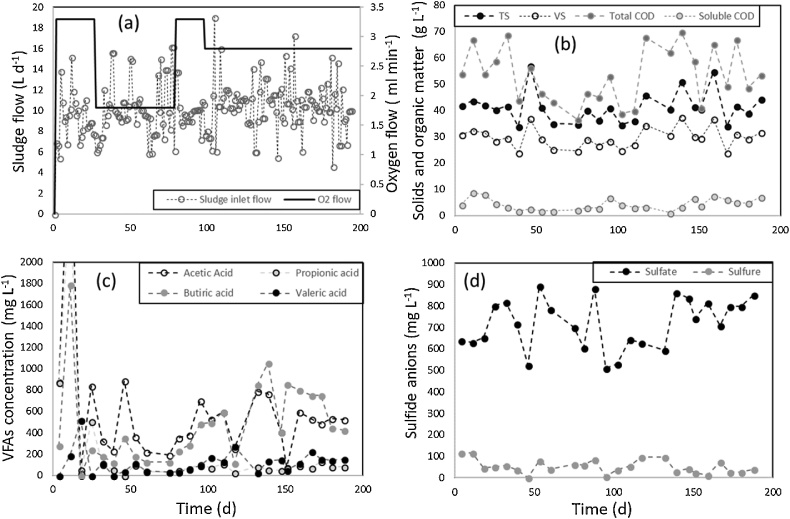
The pilot-plant scale digester (working volume of 200 L) was operated under mesophilic conditions with thickened mixed sewage sludge at a hydraulic retention time (HRT) of 20 d. Microaeration was performed in the sludge recirculation line. The O₂ flow rate (Fig. 1a) was manually adjusted to the variable biogas production rate resulting from the unsteady organic load of sludge feeding. Fluctuations in the organic loading rate of anaerobic digesters are inherent to the variations in sludge compositions in WWTPs and were here observed in all parameters monitored, namely, total COD (between 70 and 37 g_COD L⁻¹), soluble COD, TS, VS (Fig. 1b) and VFA concentrations (Fig. 1c). Additionally, Na₂SO₄ was added to the sludge feed (∼1090 mg L⁻¹) with the aim of increasing the formation of H₂S during anaerobic digestion. Therefore, the concentration of sulfate and dissolved sulfide were monitored (Fig. 1d). First, the digester was in operation for 70 d in strict anaerobic conditions (data not shown) before the microaerobic period began. More details about the experimental set-up and operation can be found elsewhere [18]. The biogas production was measured by liquid displacement and the biogas composition was determined by GC-TCD. Sulfate concentration was measured by HPLC-IC. VFA concentrations were quantified by GC-FID. Alkalinity, ammonium, TKN, COD, TS, VS, total dissolved sulfide, pH and ORP were determined according to standard methods [19].

Fig. 1.

Time course of (a) the sludge feeding rates and O₂ flow rate, (b) solids and COD concentrations, (c) VFA concentrations and (d) S²⁻ and SO₄²⁻ concentration in the digester.

2.2. Model description

2.2.1. Model implementation and ADM1 modification

ADM1 was implemented and solved in Matlab®2015b along with the general modification suggested by Rosen and Jeppsson [20]. Suggested parameters by the ADM1 report [21] were maintained, although the minimum and maximum values of the pH inhibition function for H₂ consumers were adapted to the primary sludge. Additionally, a VS output was also included, together with the COD output, by using a conversion COD/mass ratio derived from the generalized mineralization equation [22]. The composite concentration (Xc) was set equal to zero so that the particulate carbohydrates, proteins, lipids and inerts were the input conditions to the ADM1 model. The elimination of the disintegration step originally considered in the ADM1 has been lately suggested as necessary based on the recently reported drawbacks derived from the use of a two-hydrolysis step during the anaerobic digestion of sewage sludge [10]

2.2.2. Assumptions and microaeration process rationale

The mathematical description of the sulfide oxidation process involves the following assumptions:

• ■The levels of dissolved oxygen are always maintained below the inhibition threshold. Therefore, no inhibition function due to the presence of oxygen was added. In fact, the redox potential of the pilot digester cultivation broth remained always below −494 mV under process operation with and without microaeration [23].
• ■Sulfide oxidizing bacteria X_SOB is the only microbial community that consumes oxygen.
• ■The conversion of H₂S to S° is the only reaction considered. In this context, the conversion of H₂S to SO₄²⁻ requires 4-fold more O₂ than the oxidation to S°, and therefore the O₂ limiting conditions prevailing in the cultivation broth of the digester do not promote the complete oxidation of H₂S.
• ■No spontaneous H₂S or S° chemical oxidation (redox) reactions occur.
• ■The dissolved oxygen present in the sludge feeding is negligible compared to the O₂ transferred from the gas phase, which governs the growth of X_SOB.
• ■The new process included into the ADM1 along with its stoichiometry are shown in Table 1 based on the findings of [24]. The sulfur oxidation process involves elemental sulfur (S), sulfide oxidizing bacteria (X_SOB), dissolved oxygen and oxygen in the gas phase as new state variables (ordinary differential equations, ODE). The kinetic parameters of the sulfate reducing bacteria (SRB) were taken from Barrera et al [14].
• ■An O₂ laden gas stream is injected into the digester and partially transferred to the liquid phase according to Eq. (1), where K_H stand for the Henrys law constant (K_H = 0.0013 at 37 °C), k_La the volumetric mass transfer coefficient, p_pO2 the O₂ partial pressure and S_O2 the dissolved O₂ concentration in the anaerobic broth:

$$O_{2}Transferrate=k_{L}a\bullet (K_H\bullet p_{p{O}_2}-S_{O_{2\_l}})$$ (1)

• ■The value of the volumetric mass transfer coefficient, k_La, depends on reactor design and operating conditions. A unique k_La value was assumed because the HRT and mixing rate were constant in the experimental period considered, so were the temperature and the operating pressure. Changes in mixing conditions, such as switching sludge for biogas recirculation, would result in a different k_La value.
• ■Two additional ODEs were added to describe the O₂ mass balance in the anaerobic cultivation broth (Eqs. (2), (3)

$$\frac{d{S}_{02\_l}}{dt}=O_{2}Transferrate-{SOB}_{consumption}-S_{O_{2\_l}}$$ (2)

Where SOB_consumption stands for the O₂ consumption rate by X_SOB and S_O2 _l the O₂ mass flow rate in the effluent of the anaerobic digestion.

• ■Similarly, the O₂ mass balance in the headspace of the digester can be described as follows:

$$\frac{d{S}_{02\_g}}{dt}=S_{02in\_g}-O_{2}Transferrate-S_{02\_g}$$ (3)

Where S_{O2_g} represents the O₂ concentration in the gas phase, S_{O2in_g} the gas phase O₂ mass flow rate supplied to the digester and S_{O2_g} the gas phase O₂ mass flow rate leaving the digester together with the biogas effluent.

Table 1.

Petersen matrix of the new biochemical reactions added to the ADM1.

Process	SH2S	SS	S02_l	SIC	SIN	XC	XSOB	Rate
Uptake of H2S by SOB	−1	(1- YSOB)	- (1-YSOB)/64	-Σ Civi	-YSOB*NBAC		YSOB	kmSOB*(SH2S /(KsH2S + SH2S))*(S02/(KsO2 + S02))* XSOB;
Decay of SOB				-CBAC+ CXC	-NBAC+ NXC	1	−1	kdecSOB* XSOB
	Hydrogen sulfide (kmol m−3)	Elemental sulfur (S°) (kmol m−3)	Dissolved oxygen (kmol m−3)	Inorganic carbon (kmol m−3)	Inorganic nitrogen (kmol m−3)	Composites (kgCOD m−3)	Sulfur oxidizing bacteria (kgCOD m−3)

2.3. Model implementation

A manual calibration of the most sensitive model parameters was carried out. Several simulations were performed in order to identify the parameters that would influence the experimental outputs the most. The initial conditions of the model resolution were obtained from simulations until steady state conditions were reached and process parameters (mainly COD concentrations and pH) were similar to the initial values of the experimental data. This is a widely accepted method for estimating the initial conditions in anaerobic digesters [25].

2.4. Input conditions

A complete characterization of the sewage sludge was carried out weekly, along with the collection of fresh sludge from Valladolid WWTP. This characterization was assumed to hold until the next characterization and only the inlet flow was measured daily. The concentrations of H₂S and HS⁻ were estimated from the measurement of the total sulfur and the equilibria equation as a function of the pH. The content of carbohydrates, proteins and lipids was assumed to correspond to 20%, 65%, 15% of the degradable organic matter, respectively [26]. Similarly, inerts were assumed to be 30% of the total COD. The dissolved VFAs, inorganic nitrogen, sulfate, sulfide and oxygen concentrations were taken directly from the weekly experimental characterization of the sewage sludge.

3. Results and discussion

3.1. Reactor operation conditions

Fig. 1 shows the characteristics of the feed to the anaerobic digester throughout the period modelled. The sludge flow rate averaged 9 - 12 L d⁻¹, although significant drops and peaks occurred during digester operation. These fluctuations are necessary in order to test the model’s response to transient conditions and unstable reactor loads. Likewise, the solid content and the organic matter concentration inside the digester showed variations during the experimental period. Although the total COD concentration seems to experience the greatest fluctuation, this variability is strongly related to the experimental error of the COD measurement method when analyzing a semi-solid substrate such as sewage sludge. The VS concentration was correlated to the total COD concentration. It is worth stressing that the sewage sludge used in this study exhibited a significant concentration of VFAs and sulfur compounds, which are crucial to generate an adequate environment for SRB bacteria to thrive. Dissolved sulfide was present in the sewage and consequently in the sludge.

3.2. Parameters calibration

The sensitivity analysis (data not shown) indicated that only few parameters could be calibrated in a dependable way. Thus, some kinetic parameters of X_SOB were set as: K_{S_H2S} and K_{S_O2} (affinity constants) = 3·10⁻³ g L^-1; Y_SOB (biomass yield) = 0.25 g g^-1; k_dec (decay coefficient) = 0.02 d^-1. These values were chosen based on previous simulations in order to avoid the washout of the SOB from the digester and lied in the same order of magnitude as those microorganisms present in the anaerobic digestion process. Furthermore, these values were in agreement with the fact that a significant fraction of the SOB population was present over the layers of sulfur accumulated in the digester headspace according to a DGGE analysis [23]. The hydrolysis parameters were optimised by trial and error to minimize the squared value of the difference between predicted and experimental methane production curves. The values of the calibrated parameters are presented in Table 2, which compiles five stoichiometric coefficients and one kinetic parameter calibrated. This difference was induced by the type of experimental data since a continuous operation without controlled changes in the inlet conditions (such as hydraulic or organic overloads) suits better the estimation of stoichiometric parameters compared to that of kinetic parameters [25]. Indeed, a stable continuous operation is basically driven by process stoichiometry rather than by process kinetics.

Table 2.

Calibrated parameters of the model.

Type of parameter	Units	Calibrated value
Kinetic
km_SOB	d−1	160
Stoichiometric
Cch4	kmoleC kgCOD−1	0.0187
Nxc	kmoleN kgCOD−1	0.001
NI	kmoleN kgCOD−1	8 × 10−4
Naa	kmoleN kgCOD−1	6 × 10−3
Nbac	kmoleN kgCOD−1	0.019
Physical-chemical
kP	m3 d−1 bar−1	7 × 104

The calibrated parameter k_{m_SOB}, related to the maximum growth rate of sulfur oxidizing bacteria, showed that this type of microorganism grows 5–6 and 10-12-fold faster than acidogenic and methanogenic communities, respectively, which is in agreement with the difference between the metabolism of aerobic and anaerobic/facultative microorganisms. The stoichiometric value of the methane conversion (C_CH4) increased by 20% compared to the reference value [20] since a constant bias was observed in regards to the methane composition of the biogas generated in our pilot digesters. The coefficient parameters associated to N metabolism were also calibrated due to different properties of sludge used in this study compared to those used in the original ADM1 model. In fact, several authors recommend the calibration of the stoichiometric parameters associated to the N metabolisms for every substrate fed into the digester [21]. The only physical-chemical parameter calibrated was the pipe resistance coefficient (k_P), which governs the biogas flow estimation and determines the head loss of the outlet gas. This expression, which assumes an overpressure in the headspace of the digester, represents the preferred expression since it yields smoother values of the biogas flow than the original one [20]. The value of this parameter depends on the physical properties of the digester and should be modified according to the specific properties of the target set-up. In our particular case, overpressures ranging from 10 to 20 mbar were recorded throughout the digester’s operation, which resulted in a k_P of 7 × 10⁴ (Table 2).

3.3. Model performance

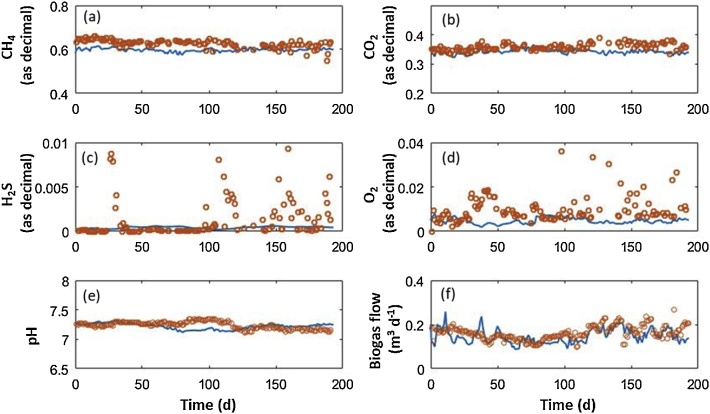
Fig. 2, Fig. 3 show the simulation results of the modified ADM1 model along with the experimental offline (i.e measurements in the anaerobic broth) and online data (biogas production and composition data and pH in the anaerobic broth), respectively. The online data corresponds to the composition of the biogas produced and the pH of the anaerobic broth in the digester. Both the methane and CO₂ content simulation matched the experimental data (Figure a,b) as well as the pH value and remained constant because microaeration did not affect them significantly. Even though the pH was slightly overestimated by the model’s predictions, it remained at ∼ 7.5 (close to the typical pH value of anaerobic digesters) (Fig. 2e). The biogas flow rate was very well predicted by the model’s simulation, although there were some random under and overestimation of this variable during digester operations (Fig. 2f). Therefore, the model was able to reproduce the most common on-line variables monitored during AD operation. On the other hand, the average H₂S and O₂ concentrations predicted in the gas phase was in agreement with the average empirical concentrations measured (Fig. 2c and d). However, the model was not able to reproduce the random H₂S peaks observed during the period evaluated. Interestingly, those H₂S peaks did not match any sudden rise in the sulfate inlet concentration or in soluble H₂S concentrations in the digester broth (Fig. 3). Previous works have hypothesized that the biological oxidation of H₂S may also occur in the headspace of the digester and in the superior layer of the anaerobic broth based on the high abundance of SOB bacteria recorded by the DGGE analysis [23]. A simple consideration of biomass retention was consequently proposed to partially overcome the limitation identified, while maintaining the model’s complexity. The biomass retention approach, which is presented in the next section, separates the hydraulic retention time and the biomass retention time, in this case, for the SOB microorganism.

Fig. 2.

Time course of the online measurements of the concentration of methane (a), carbon dioxide (b), hydrogen sulphide (c) and oxygen (d) in the biogas, pH in the anaerobic digestion broth (e) and biogas flow rate (f). Experimental data (circles), model simulation (continuous line).

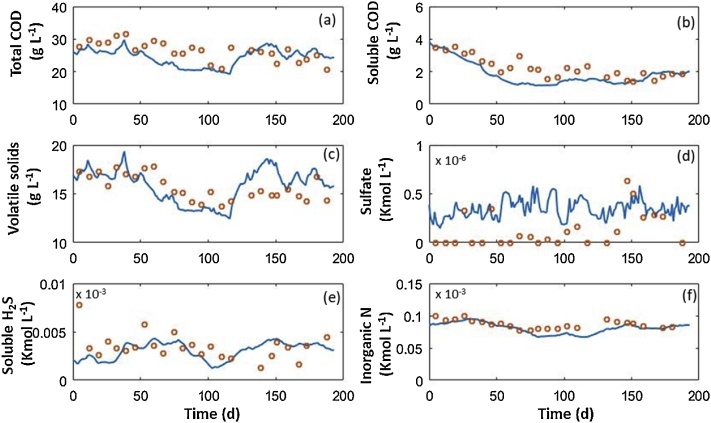
Fig. 3.

Time course of the offline measurements of the total COD (a), soluble COD (b), volatile solids (c), sulfate (d), soluble hydrogen sulfide (e) and inorganic nitrogen (f) in the digester. Experimental data (circles), model simulation (continuous line).

The decreasing trends in total organic matter (measured as total COD and VS concentrations) observed up to day 115th as well as the slight bounce back from this day onwards were both properly represented by the model (Fig. 3a, c). Likewise, the simulation of the evolution of the soluble COD concentration was in agreement with the empirical values throughout the entire operational time (Fig. 3b). Likewise, the inorganic nitrogen concentration was also properly predicted by the model, thus validating the calibration of the stoichiometric parameters conducted in the previous section. Fig. 3e also confirmed the ability of the ADM1 extension to describe the soluble H₂S concentration in the anaerobic broth. In contrast, the soluble sulfate concentration in the anaerobic broth was overpredicted and only matched when values were above 0.3 kmol L⁻¹, which were in agreement with the results reported by [14].

3.4. Effect of the biomass retention

Empirical observations of anaerobic digesters operated under microaerobic conditions have shown that SOB may form a biofilm inside the digester headspace, thus part of H2S oxidation may be carried out at the biofilm surface. Hence, Kobayashi et al [27] identified SOB in microbial mats located on the top of the biodigester, where elemental sulfur accumulated. Therefore, a new parameter in the S mass balance equation was proposed in order to take this biofilm based H₂S oxidation into account. This parameter, defined as “alpha”, multiplies the X_SOB leaving the system and thus modifies the retention time of this microbial population. This is conceptually described in the mass balance shown in Eq. (4)

$$\frac{d{X}_{SOB}}{dt}=-alpha*D*X_{SOB}+growthrate-decay$$ (4)

Where D is the dilution rate or the inverse of the hydraulic retention time (HRT)

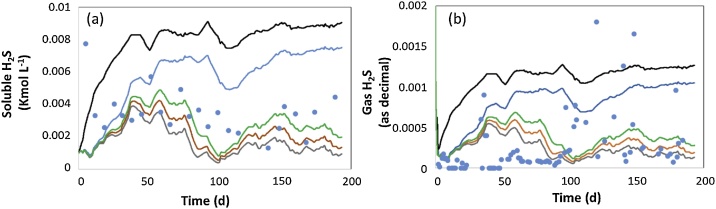
Fig. 4 depicts the new model predictions of the soluble and gaseous H₂S as a function of different alpha values compared to the experimental data. The influence of the presence of SOB (blue line) in the anaerobic broth compared to the scenario without these H₂S oxidizing microorganisms (black line) in both soluble and gas phases was significant. These new simulations also showed the impact of variations in biomass retention on the soluble and gas H₂S. Model simulation of these two variables improved significantly when the alpha value decreased (= enhanced retention of the SOB in the digester). Hence, the formation of biofilms at the digester headspace maybe partially modeled by adding this biomass retention artifact. This model approach has been successfully performed in anaerobic filters treating vinasse wastewater [28]. In brief, despite more physico-chemical reactions should be considered when describing H₂S oxidation in anaerobic digesters operated under microaerobic conditions [16,29]; the approach here validated represents a good trade-off between complexity and reality.

Fig. 4.

Time course of the H₂S concentration in the liquid (a) and gas (b) phases at different alpha values. Experimental values (symbols), Black line = No SOB, blue line = alpha 1 (no retention), Green line = alpha 0,4, orange line = alpha 0.25 and grey line = alpha 0.1 (For interpretation of the references to colour in this figure legend, the reader is referred to the web version of this article).

3.5. Elemental sulfur accumulation

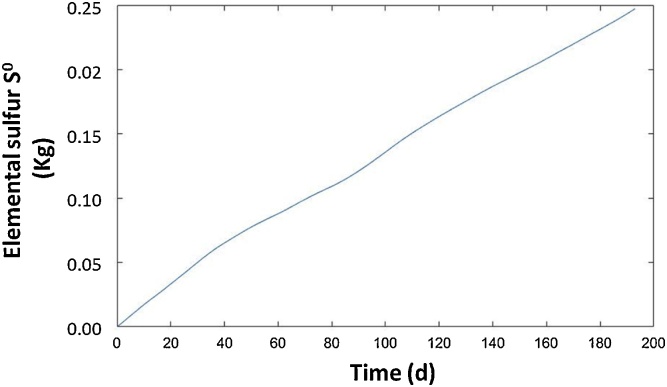
It is known that one of the main shortcomings of the application of microaeration, as a H₂S removal method, is the accumulation of elemental sulfur in the digester. The build-up of this compound may lead to multiple operational hurdles such as pipeline clogging, hindered mixing or even digester damage due to an excessive weight increase. Based on the negligible aqueous solubility of elemental sulfur, an estimation of the accumulation of this element in the reactor can be carried out using the ADM1 extension developed here. Fig. 5 shows the time course of the mass of S° generated in the pilot reactor during the operational period analyzed here. Five kg of elemental sulfur could have potentially accumulated in the pilot digester over a period of ∼ 200 days of operation. This number should be deemed as a theoretical maximum since part of the S° produced was dragged out with the outlet digestate. This estimation of the S° accumulation in the digester can be used to plan the necessary maintenance measures. Therefore, the model developed in this study represents a useful operational tool for AD.

Fig. 5.

Elemental sulfur accumulation in the experimental set-up over the operational period under microaerobic conditions.

4. Conclusions

An extension of the ADM1 capable of describing H₂S removal from biogas based on microaeration was developed and evaluated using experimental data from a pilot anaerobic digester. The maximum specific growth rate of the SOB along with four stoichiometric coefficients involved in nitrogen metabolism were estimated during model calibration. The model accurately described the most conventional variables monitored in anaerobic digestion processes (i.e biogas flow, CH₄ and CO₂ concentrations, pH and organic matter removal). The average concentrations of the S-related compound (i.e. soluble SO₄ and H₂S in the gas and liquid phase) were properly described. Unfortunately, the model extension provided a poor description of the variations in the concentration of S compounds under transient conditions. Further model improvements may be carried out by separating biological H₂S oxidation in different sections of the digester or by even considering H₂S oxidation in the headspace biofilm on top of the digester.