Skip to main content
PMC home page
Social Cognitive and Affective Neuroscience logoLink to Social Cognitive and Affective Neuroscience
. 2018 Nov 27;14(1):59–71. doi: 10.1093/scan/nsy106

Transcranial stimulation over right inferior frontal gyrus increases the weight given to private information during sequential decision-making

Xiaofei Niu 1, Jianbiao Li 1,2,3,✉, Glenn J Browne 4, Dahui Li 5, Qian Cao 1, Xiaoli Liu 1, Guangrong Wang 6, Pengcheng Wang 7
PMCID: PMC6318474  PMID: 30496572

Abstract

Decision makers often follow other similarly situated people in making decisions, creating a sequential decision-making context. Although rational behavior is often to make the same choice as previous decision makers, which can result in an information cascade, people may assign inappropriately higher weight to their own private information and discount public information about predecessors’ choices. Recent findings suggest that overweighting private information may be associated with increased activities in the inferior frontal gyrus (IFG). In the present study, we employed transcranial direct current stimulation (tDCS) and developed a computational model to examine the causal relationship between right IFG (rIFG) and overweighting private information. Specifically, we applied three types of tDCS over rIFG while participants were completing a sequential decision-making task. Our results showed that anodal stimulation significantly increased the weight given to private information and decreased the response time in making a decision when private information conflicted with public information, but cathodal stimulation did not have such impacts. Importantly, the effect of anodal stimulation was significant in some conditions when information conflict or task difficulty reached a threshold that might trigger cognitive control-related processes. Our findings revealed the important role of rIFG in trade-off between considering private and public information during sequential decision-making.

Keywords: inferior frontal gyrus, weight of evidence, tDCS, sequential decision-making, information cascades

Introduction

Individual decision makers are often faced with choices for which previous similarly situated decision makers have already made selections. If the choices of the previous decision makers are known, the new decision makers have two types of information—their own private information (which could consist of data, previous experiences, opinions, etc.) and the knowledge of the choices of the previous decision makers. Private information is nearly always incomplete and imperfect; such information will have some degree of validity and the decision maker must choose whether to rely on that information. Public information in terms of choices made by previous decision makers is assumed to be known and fully valid. In such situations, a rational decision maker will combine the public and private information using Bayes’ rule and will often make the same choice as previous decision makers even if the choice conflicts with what his or her private information suggests. When numerous sequential decision makers rationally follow previous decision makers and disregard their own private signals, the resulting sequence is known as an ‘information cascade’ (Banerjee, 1992; Bikhchandani et al., 1992). Information cascades have been observed in a variety of settings, such as technology adoption (Walden and Browne, 2009), movie ratings (Lee et al., 2015) and revolutionary regime transitions (Ellis and Fender, 2011).

However, the literature concerning information cascades has found that there exist systematic deviations from the Bayesian Nash Equilibrium (BNE), which prescribes how a rational individual should condition his or her decision based on predecessors’ actions (Huck and Oechssler, 2000; Nöth and Weber, 2003; Kübler and Weizsäcker, 2004; Goeree et al., 2007). These findings demonstrate that an individual may assign higher weight to his or her own private information relative to the publicly observable information from the decisions made by others. In fact, overweighting private information can lead to fewer cascades than predicted by BNE (Weizsäcker, 2010; see also, Walden and Browne, 2009). These results have important implications for theories of rational choice, suggesting that rational choice models often are not descriptive of human behavior (Simon, 1996). For this reason, investigating neural mechanisms to improve our understanding of overweighting private information is useful and can provide important insights into information cascades, weighting of evidence and decision-making more generally.

A recent functional magnetic resonance imaging (fMRI) study provided some initial insights into the neural mechanism to explain how an individual updates his or her private information as compared with public information in information cascades situations (Huber et al., 2015). This study showed that the more a participant overweights private information, the higher the activation in the inferior frontal gyrus (IFG). Moreover, an individual’s tendency to overweight his or her private signal was associated with his or her degree of overconfidence (Nöth and Weber, 2003). Overconfident participants exhibited a tendency to allocate their initial attention to private signals (Innocenti et al., 2010), indicating an attentional preference for these individuals of private over public information. Li et al.(2018) found in an event-related potential (ERP) study that the percentage of choices consistent with private information was correlated with frontal N200, which plays a key role in cognitive control (Folstein and Van Petten, 2008). Attention and cognitive control were found to be represented in the right IFG (rIFG) (Aron et al., 2004; Hampshire et al., 2010). Additionally, patients who had attention deficit hyperactivity disorder were able to improve interference control with anodal transcranial direct current stimulation (tDCS) over rIFG (Breitling et al., 2016). The interference effect was significantly decreased after anodal tDCS over rIFG in an imitation-inhibition task (Hogeveen et al., 2015).

These findings suggest that the activity of rIFG might be causally involved in overweighting private information during sequential decision-making. However, there is no experimental evidence so far, to the best of our knowledge, to support this speculation. In addition, prior findings of overweighting private information in the neuroscience literature have been based solely on fMRI or ERP (Huber et al., 2015; Li et al., 2018) and are not sufficient to reveal causal relationships between brain activity and human behavior. Establishing a causal relationship between rIFG and the overweighting of private information is crucial for our understanding of the neural mechanism that causes people to make non-rational choices in sequential decision-making situations.

To formally test the causal relationship, we conducted an experiment to study whether tDCS, a method of non-invasive stimulation of the human brain by means of weak currents, over rIFG affected overweighting of private information. In addition, we developed an information heteroweighting model (IHM) to describe the weight given to private information.

In the experiment, participants performed a sequential decision-making task adapted from the information cascades experiment of Anderson and Holt (1997). While participants were performing the task, we applied anodal, cathodal or sham stimulation over rIFG. Anodal and cathodal tDCSs are known to increase or decrease the resting potential, which leads to an increase or decrease of neural excitability in the targeted regions, while sham tDCS, which mimics the peripheral effects (i.e. tactile sensations), does not affect any neural processing (Nitsche et al.2008). Huber et al.(2015) found that people who tend to overweight private as compared with public information show increased activity in the IFG. Li et al.(2018) also found overweighting private information was correlated with control-related N200, which may stem from the rIFG region (Aron et al., 2004; Hogeveen et al., 2015; Breitling et al., 2016). We therefore expected that, compared with sham stimulation, anodal stimulation over rIFG would increase rIFG activity (and possibly other connected areas) so that a decision maker would increase the weight given to private information, whereas cathodal stimulation might have the opposite effect.

Materials and methods

Participants

Ninety-eight healthy students from Nankai University volunteered to participate in this study [mean age, 22.3 years; standard error of mean (SEM), 0.19 years; range, 19–28 years; 31 men]. Each participant was given a 5 Chinese yuan ($0.76 US) participation fee and also received a variable amount of money at the end of the experiment based on his or her performance during the task. The university’s institutional review board approved all the experimental procedures and protocols.

Participants were randomly assigned to one of three stimulation groups: anodal (n = 34; 11 men), cathodal (n = 32; 12 men) or sham (n = 32; 8 men). We excluded two female participants from the anodal group because one participant reported discomfort with the stimulation and another failed to report answers within the response time (RT) limit in a majority of the trials. Overall, 96 participants successfully performed the task in the experiment.

All experimental sessions were conducted in a group room at the Reinhard Selten Laboratory. The group room was laid out with several enclosed cubicles, each of which was equipped with a computer that was connected to a local area network. All the computers had the same hardware and software configuration. This setting was designed to conduct anonymous and randomized experiments.

Experimental tasks and procedure

Our sequential decision-making task was adapted from the information cascade experiment designed by Anderson and Holt (1997). In our study, a participant was asked to draw a conditionally independent private signal and predict which of two equally likely events had happened in the presence of known predictions made by prior participants. The events were denoted as A box and B box, and the signal was either a ball or b ball. Each of the two boxes (A and B) contained three balls (a or b), with the A box including two a balls and one b ball and the B box including one a ball and two b balls. In a total of 12 experimental trials, a participant received public information (i.e. the box designated but not the ball drawn) presented in an accumulated random order about the decisions made by predecessors.

At the beginning of a trial, one of the two boxes was randomly assigned to all participants from which to draw a ball and participants were then asked to predict from which box the ball was drawn. Predictions made by seven predecessors, representing public information, were then shown to the participant sequentially. The seven predecessors were not physically present in the experiment but had made their choices sequentially in a prior session and could observe each other’s choices. These people agreed that their choices could be used in subsequent sessions. A similar design was reported in Ruff et al.(2013). We arranged the seven people in a random order and indexed them as P1–P7. The participant in the experimental session then acted as P8 and drew a ball from the box assigned to all the predecessors in the trial. The participant was informed that P1–P7 also received their own conditionally independent private signals by drawing a ball from the same box. At the end of each trial, P8 made a prediction about which box the ball was drawn from after observing his or her own private signal (a ball or b ball) and the seven predecessors’ public information (A box or B box).

The procedure of a single trial is depicted in Figure 1. In each trial, the predictions or choices made by P1–P7 were displayed at the center of the computer screen in a sequential order with an interval of 2 s between 2 predictions. The displays were cumulative in nature. Specifically, the choice made by P1 was first displayed. After 2 s, P2’s choice was presented together with that of P1. Then, the choices made by P3–P7 were displayed after every 2 s in a sequential order, while the choices made by the predecessors were still shown in the computer screen. After all the seven predecessors’ predictions were displayed, P8 pressed the ‘Draw ball’ button to receive his or her private signal. Once P8 received his or her private signal (a or b), he or she was given 10 s to designate from which box (A or B) he or she drew the ball. We recorded the prediction made by P8 and the RT for making the prediction. We programmed the experiment in z-Tree (Fischbacher, 2007).

Fig. 1.

Fig. 1

Open in a new tab

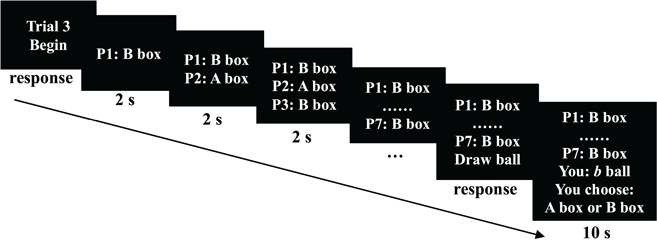
Time course of a single trial. At the beginning of each trial, a participant was presented with the current trial number followed by choices made by P1–P7. Once a participant pressed the ‘Begin’ button, P1’s choice was displayed on the screen. After 2 s, P2’s choice was displayed and so on. The seven predecessors’ choices were displayed sequentially, and the interval between displaying every predecessor’s choice was fixed at 2 s. After P7’s choice was displayed, P8 drew a ball by pressing the ‘Draw ball’ button. P8 then had 10 s to make a decision after observing his or her private signal.

Following a similar design (Huber et al., 2015), we manipulated P8’s draw in two conditions (congruent vs incongruent) so that P8’s private signal was congruent with a majority of the seven predecessors’ choices in six trials and incongruent in the other six trials. We also followed Frydman and Krajbich (2017) and created a variable called Net Public Information for Pi as 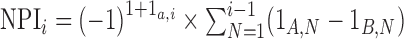Inline graphic, i ϵ [1,7]. The first term, (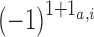Inline graphic), is 1 if Pi received private signal a and −1 if otherwise. The second term, (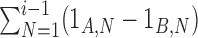Inline graphic), is the difference between the number of observed ‘A’ choices (or boxes) and those of ‘B’ choices. NPIi > 0 indicates that Pi received a congruent private signal, while NPIi < 0 indicates an incongruent private signal. NPI had six distinct values in our study, which were 1, 3 and 5 in the congruent condition and −1, −3 and −5 in the incongruent condition.

We created a design matrix (2 × 3 × 2 = 12 trials; Table 1) by using all combinations of condition (incongruent vs congruent), absolute NPI (the difference between the number of ‘A’ choice and ‘B’ choice; 5 vs 3 vs 1) and P8’s private signal (ball a vs b).

Table 1.

Stimuli in the main experiment

Condition Absolute NPI P1–P7’s choices (box) P8’s private signal (ball)
Incongruent (NPI < 0) 5 A-A-B-A-A-A-A b
B-B-A-B-B-B-B a
3 A-A-B-B-A-A-A b
B-B-A-A-B-B-B a
1 B-A-B-B-A-A-A b
A-B-A-A-B-B-B a
Congruent (NPI > 0) 5 A-B-A-A-A-A-A a
B-A-B-B-B-B-B b
3 B-A-A-B-A-A-A a
A-B-B-A-B-B-B b
1 B-A-A-B-B-A-A a
A-B-B-A-A-B-B b
Open in a new tab

Compared with the design of short sequences of decisions in Huber et al.(2015), we report the neural correlates of overweighting private information from long sequences of decisions in sequential decision-making situations. In particular, a participant such as P8 would weigh the evidence from predecessors’ choices as well as his or her private draw. This weight of the evidence design makes it possible to rigorously examine the effects of tDCS over rIFG on overweighting private information in the different conditions of NPI. NPI measures the degree of information conflict between public and private signals. The trade-off between these two types of information underlies the tendency to start an information cascade (Walden and Browne, 2009; Weizsäcker, 2010).

In the experiment design of Huber et al.(2015), a participant was the third decision maker and the proportions were two-thirds or four-fifths that the ball (a or b) matched the label of the box (A or B). After receiving his or her private signal, a participant could update his or her belief, which corresponded to six different posterior probabilities. However, the NPI value was only 2 or −2. Thus, Huber et al.(2015) examined the neural activity of information cascades only for these levels of uncertainty and did not provide any neural evidence of whether the degree of information conflict moderated the overweighting of private information, which would lead to fewer cascades than predicted by BNE.

In our experimental design, we explore the differing weights given to private and public information. Importantly, we employ tDCS to examine the casual relationship between overweighting private information and rIFG for the eighth decision maker using various NPI values.

Transcranial direct current stimulation

In the experiment, we applied tDCS in a double-blind sham-controlled setting by means of a battery-driven stimulator (Neuro Conn, Germany) in which weak currents were used to modulate regional neural excitability by increasing (anodal) or decreasing (cathodal) the resting membrane potential depending on the position and polarity of the electrode. In the present study, we applied anodal, cathodal and sham tDCS over an IFG region that was found to be activated in a prior fMRI study (Huber et al., 2015). In line with previous tDCS studies that focused on IFG (Holland et al., 2011; Hogeveen et al., 2015), we centered the stimulation electrode on electrode site FC6, according to the standard 10–10 system that corresponds to rIFG. We used an electroencephalograph (EEG) cap to determine the electrode position for each participant, with the reference electrode posterior to the left mastoid (Breitling et al., 2016). This reference electrode position minimizes its influence on other cortical areas that might be relevant to the top-down control of behaviors (e.g. other prefrontal regions). We applied tDCS using a set of standard electrodes fixed by rubber straps (5 × 7 cm; current density, 0.029 mA/cm2). These standard electrodes were chosen over customized focal electrodes because we planned to ensure that the large electrode covered all neural rIFG regions.

tDCS was applied for 20 min with 1 mA current strength for the anodal group and the cathodal group. The arrangement of the stimulation electrode was identical in all three groups except that the stimulator was turned off after 30 s for the sham group. In all groups, the current was applied with a 15 s ramp up and down.

Prior to the experiment, we randomly assigned each participant to a cubicle. While waiting for the installation of the experiment equipment, a participant filled out one questionnaire that included basic demographic information (e.g. age, gender and major) and another questionnaire that measured a person’s trust and respect, reciprocity, belief in fairness of others and norm of honesty based on the World Values Survey. After the installation of the experimental equipment, each participant received 5 min of tDCS to ensure stable stimulation effects (Nitsche et al., 2008). During this stage, we explained the experimental instructions to the participant again and conducted three training trials.

The experiment was composed of 12 trials presented in a random order. At the end of the experimental task, the true box (A or B) from which a participant drew his or her private signal in each trial was revealed to the participant. Participants who predicted correctly in a trial received 4 Chinese yuan ($0.30 US) and 0 otherwise. The average pay-off for all participants over all trials was 40 Chinese yuan ($6.08) (range, $4.31–$7.16; s.d., $1.05).

In addition, we also asked each participant to fill out a questionnaire that measured the participant’s conformity tendency and personality traits while the tDCS stimulation was still ongoing. The conformity tendency was measured by asking the participant to report what choice P3 would make (A or B box) if P3 received an a ball after observing that both P1 and P2 chose the B box. The questions about personality traits included the cognitive reflection test (Frederick, 2005), degree of overconfidence (Ren and Croson, 2013), risk-taking attitudes (Holt and Laury, 2002) and loss aversion (Gächter et al., 2007; Rau, 2014). Finally, participants indicated how much they perceived the stimulation to affect their behavior (using a 5-point Likert scale).

A computational model and model fitting

To investigate further a decision maker’s weights given to private information and public information, we developed a computational model to describe how a decision maker updates his or her beliefs during sequential decision-making.

Bayesian Nash Equilibrium

We use BNE to describe how a rational decision maker updates his or her beliefs in a sequential process, following Anderson and Holt (1997). We posit that P1, whose only information is his or her own private signal, will predict A box if he or she receives an a ball and B box if a b ball is received. Thus, P1 reveals his or her private signal (e.g. a ball) as public information (e.g. A box). If P2 receives a private signal (e.g. a ball) that matches P1’s choice (e.g. A box), P2 will reveal his or her private signal as public information by stating the A box also. If P2 receives a private signal (e.g. b ball) that does not match P1’s choice (e.g. A box), this will result in a posterior probability of one-half because the prior probability is one-half and the sample is balanced. Thus, P2 will likely state that he or she drew from the B box since his or her private signal was b. If P3 observes both P1 and P2 choosing the same box (e.g. A), and P3 receives a b ball, P3 will respond to an inferred sample of a balls on the first two draws and the b ball on his or her own draw. Let n be the number of relevant signals a, and m the number of relevant signals b. Bayes’ rule can be used to calculate the posterior probability of event A:

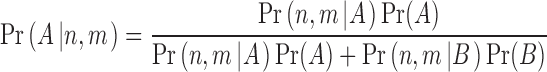graphic file with name M4.gif (1)

In the example above, the posterior probability of A is greater than one-half and P3 should choose the A box in line with the public signal, disregarding his or her private signal. This generates an information cascade, with players i = 4, …, 8 adopting the same logic and selecting A box regardless of their own private signals. In sum, according to the prediction of BNE, the rational behavior for all subsequent decision makers is to choose the same event regardless of their private signals.

In our experiment, a participant could update his or her beliefs after receiving a private signal, which should correspond to six posterior probabilities in the different NPI values. Specifically, the posterior probability is 0.0588 (NPI = −5), 0.2 (NPI = −3) and 0.5 (NPI = −1) in the incongruent trials, and 0.8 (NPI = 1), 0.9412 (NPI = 3) and 0.9846 (NPI = 5) in the congruent trials. It may not be easy for participants to calculate the Bayes’ posterior probabilities of equation (1). But participants can simply count the numbers of observed ‘A’ choices (or boxes) and those of ‘B’ choices to approximate optimal decision-making in this situation.

Information heteroweighting model

Despite the rationality assumptions noted above, previous studies using a similar experimental design have shown systematic deviations from predictions based on BNE (see Weizsäcker, 2010, for a review). Here we develop an IHM to identify how people weight private information in their decision-making. Bayes’ theorem assumes that people weight evidence according to normative principles. In our IHM, we measure how people actually weight private and public information, and β represents the weight given to private information. This model is consistent with previous experimental results that people overweight private information (e.g. Nöth and Weber, 2003; Goeree et al., 2007). Our model attempts to describe how people actually weight information rather than making the normative assumption. Ours is thus a descriptive model that captures potential deviations from the normative ideal.

Suppose that Pi receives an a ball, which denotes his or her private signal. The number of predecessors’ choices of A box is n − 1 and B box is m. That is, the number of relevant public signals a is n − 1, and the number of relevant public signals b is m. Let β be the weight Pi gives to his or her private signal a. γa and γb refer to the weights Pi gives to each relevant public signal a and b. Assume that Pi has no bias toward public information and weights each relevant public signal a and b with 1 (i.e. 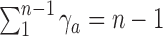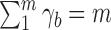γa = γb = 1),Inline graphic and Inline graphic. Then, given Pi’s private signal a and predecessors’ choices, the posterior probability of event A is

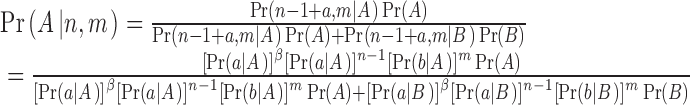graphic file with name M7.gif (2)

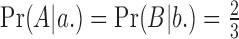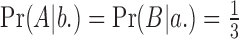In our experiment design, Inline graphic, Inline graphic and Inline graphic. So equation (2) can be simplified as

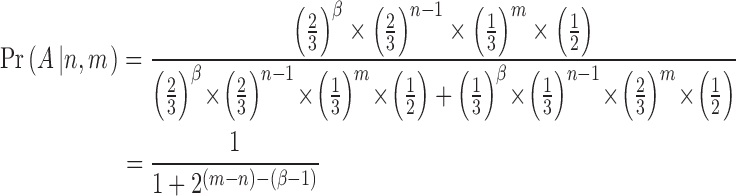graphic file with name M11.gif
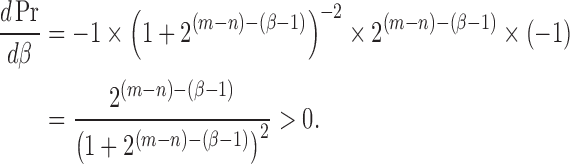graphic file with name M12.gif

If a participant weights public and private information equally, i.e. β = 1, equation (2) is identical to equation (1). However, if a participant gives higher weight to private information, β > 1; otherwise, β < 1.

Model fitting

We fitted the computational model that described how much weight each participant gave to private information (β) in each condition of NPI. The parameter β in each NPI was computed by putting a participant’s percentage of choices consistent with his or her own private signal to the left side of equation (3).

Data analyses and results

We analyzed the choice made by a participant and RT as dependent variables in two mixed-design analyses of variance (ANOVAs) with four factors. Condition (incongruent vs congruent), absolute NPI (5 vs 3 vs 1) and P8’s private signal (a vs b) were within-subject factors, and stimulation (anodal vs sham vs cathodal) was a between-subject factor. Choice was coded as a dummy variable and was set to 1 if a participant made a choice consistent with his or her own private signal and 0 if otherwise. Significant main and interaction effects were further analyzed using Bonferroni-corrected post hoc tests. In addition, we performed ordinary least squares (OLS) regression analyses with robust standard errors clustered at the individual (participant) level. All reported P-values are two-tailed and corrected for multiple comparisons.

With choice as the dependent variable, we found that the main effects of condition (F1,93 = 448.802; P < 0.001; partial η2 = 0.828) and absolute NPI (F1.80,167.77 = 65.097; P < 0.001; partial η2 = 0.412) were significant. The interaction effect of condition × absolute NPI (F1.84,170.94 = 59.86; P < 0.001; partial η2 = 0.392) was also significant.

The significant interaction effect of condition × absolute NPI suggests that a participant’s choice was strongly influenced by the congruency between P8’s private signal and the seven public choices made by predecessors. The tendency for P8 to make a choice consistent with his or her private information only held for trials in the incongruent condition (NPI < 0: F2,92 = 57.703, P < 0.001, partial η2 = 0.556; NPI = −5 vs NPI = −1: P < 0.001; NPI = −3 vs NPI = −1: P < 0.001; NPI = −5 vs NPI = −3: P = 0.97). P8 was more likely to make a choice in line with his or her private signal as NPI increased (NPI = −5, 19.791 ± 3.2%; NPI = −3, 22.917 ± 3.4%; NPI = −1, 63.021 ± 3.7%) in the incongruent condition. For the congruent condition (NPI > 0), almost all the participants (96%) made choices consistent with their private signals.

In addition, there was a significant main effect of P8’s private signal (F1,93 = 8.259; P = 0.005; partial η2 = 0.082), but the interaction effects between the private signal and other factors were not significant (all Ps > 0.10). The surprising main effect of P8’s private signal suggests that P8 was more likely to make a choice in line with his or her private signal when receiving an a ball than a b ball (68.23 ± 1.51 vs 64.06 ± 1.48%). We return to this surprising result later.

The most important findings were the main effect of stimulation (F2,93 = 4.643; P = 0.012; partial η2 = 0.091) and the condition × stimulation interaction effect (F2,93 = 6.165; P = 0.003; partial η2 = 0.117). The simple effects analysis suggested that stimulation over rIFG did not change P8’s choice when P8 received a congruent signal (main effect of stimulation in the congruent condition: F2,93 = 1.068; P = 0.348; partial η2 = 0.022) but increased the number of choices consistent with private signal when the participant received an incongruent signal (main effect of stimulation in the incongruent condition: F2,93 = 5.995; P = 0.004; partial η2 = 0.114).

Figure 2 shows the impact of stimulation on the percentage of choices consistent with the private signal. It shows that for the incongruent condition anodal rIFG stimulation resulted in a significantly higher percentage of choices in line with the private signal than both the sham stimulation (47.916 vs 30.729%; P = 0.025; Cohen’s d = 0.48) and the cathodal stimulation (47.916 vs 27.083%; P = 0.006; Cohen’s d = 0.64). The sham stimulation and cathodal stimulation did not differ (30.729 vs 27.083%; P = 0.687; Figure 2A). For the congruent condition, the anodal stimulation decreased the percentage of choices consistent with private signals more than the cathodal and sham stimulations (Figure 2B). However, this result was not significant (all Ps > 0.60).

Fig. 2.

Fig. 2

Open in a new tab

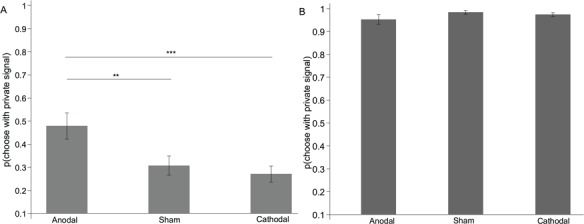
Impact of stimulation on the percentage of choices consistent with private signals. Anodal stimulation led to a higher percentage of choices consistent with private signals than both cathodal and sham stimulation in the incongruent condition (A). There was no significant difference among the stimulation in the congruent condition (B). Error bars indicate ±1 SEM. Asterisks indicate significance levels **P < 0.05 and ***P < 0.01. (A) was for the incongruent condition; (B) was for the congruent condition.

Critically, model fits of the weight given to private information, as shown in Figure 3, indicated that participants deviated from BNE in all the stimulation groups. In particular, participants in the anodal group gave higher weight to private information in the incongruent condition. Further, the weight given to private information was significantly higher in the anodal group (β = 3.25 ± 0.505) than in the sham group (β = 1.698 ± 0.363; P = 0.015; Cohen’s d = 0.51) and the cathodal group (β = 1.406 ± 0.267; P = 0.002; Cohen’s d = 0.55), indicating anodal tDCS stimulation increased the weight given to private information. However, there were no significant differences among the three stimulation groups in the congruent condition (anodal, β = 0.167 ± 0.161; sham, β = −0.073 ± 0.044; cathodal, 0.052 ± 0.111; all Ps > 0.15).

Fig. 3.

Fig. 3

Open in a new tab

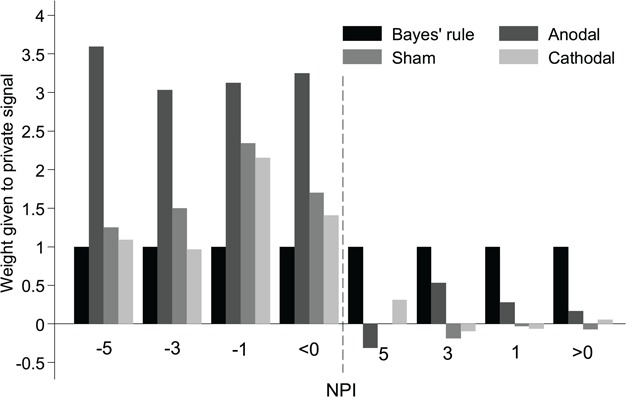
Model fits of the weight given to private information depend on NPI and rIFG stimulation. Anodal stimulation of the rIFG led to a higher weight given to private signals, as evident in the significantly higher β in the trials for NPI < 0.

When testing RT as the dependent variable, a mixed-design ANOVA indicated that there were significant main effects of condition (F1,93 = 203.531; P < 0.001; partial η2 = 0.686) and absolute NPI (F1.78,165.62 = 24.884; P < 0.001; partial η2 = 0.211). The interaction effect of condition × absolute NPI was also significant (F1.99,3.98 = 6.484; P = 0.002; partial η2 = 0.065). Post hoc tests suggested that RT was significant between NPI = −5 (M = 1.979 ± 0.178 s) and NPI = −3 (M = 2.609 ± 0.212 s; P = 0.035), NPI = −5 and NPI = −1 (M = 3.661 ± 0.248 s; P < 0.001) and NPI = −3 and NPI = −1 (P = 0.001) in the incongruent condition. RT was also significant between NPI = 5 (M = 0.443 ± 0.080 s) and NPI = 3 (M = 0.880 ± 0.125 s; P = 0.002) and NPI = 5 and NPI = 1 (M = 1.182 ± 0.157 s; P < 0.001) in the congruent condition. RT significantly increased when NPI increased in the incongruent condition and decreased when NPI increased in the congruent condition. The highest RT was found when NPI was −1 (63.021% of participants chose consistent with their private signals). In addition, the main and interaction effects of P8’s private signal were insignificant (all Ps > 0.10).

Importantly, we found a significant main effect of stimulation (F2,93 = 3.505; P = 0.034; partial η2 = 0.070) as well as a significant interaction effect of condition × stimulation (F2,93 = 3.833; P = 0.025; partial η2 = 0.076) on RT. The interaction effect revealed that stimulation had a significant effect on a participant’s RT in the incongruent condition (F2,93 = 4.814; P = 0.010; partial η2 = 0.094). Specifically, anodal stimulation resulted in shorter RT than both sham stimulation (2.172 vs 2.807 s; P = 0.062; Cohen’s d = 0.35) and cathodal stimulation (2.172 vs 3.270 s; P = 0.001; Cohen’s d = 0.47), while sham and cathodal stimulation did not differ (P = 0.143; Figure 4). In addition, stimulation did not significantly affect RT in the congruent condition (anodal, 0.745 ± 0.115 s; sham, 0.818 ± 0.169 s; cathodal, 0.943 ± 0.173 s; P > 0.10).

Fig. 4.

Fig. 4

Open in a new tab

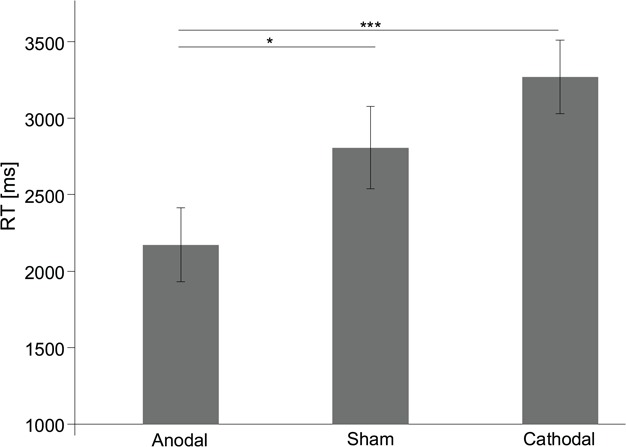
Impact of stimulation on the RT in the incongruent condition. Anodal stimulation led to a shorter RT than both cathodal and sham stimulations in the incongruent condition. Error bars indicate ±1 SEM. Asterisks indicate significance levels *P < 0.10 and ***P < 0.01.

In addition, we did not find a significant three-way interaction effect of condition × absolute NPI × stimulation on the participant’s choice and RT (all Ps > 0.10). However, Tables 2 and 3 show that anodal stimulation strongly affected the number of choices consistent with private signals and RT when NPI < 0. We therefore performed two additional ANOVAs with NPI (−5 vs −3 vs −1 vs 5 vs 3 vs 1) × P8’s private signal × stimulation as the factors.

Table 2.

Effect of tDCS on percentage of choices consistent with private signal in different NPI

NPI Stimulation (M ± SEM) Post hoc tests
1: Anodal 2: Sham 3: Cathodal
−5 0.359 ± 0.055 0.125 ± 0.055 0.109 ± 0.055 1 and 2**, 1–3*** and 2 and 3
−3 0.359 ± 0.060 0.188 ± 0.060 0.141 ± 0.060 1 and 2*, 1–3** and 2 and 3
−1 0.719 ± 0.064 0.609 ± 0.064 0.563 ± 0.064 1 and 2, 1–3 and 2 and 3
5 0.969 ± 0.022 1 ± 0.022 0.969 ± 0.022 1 and 2, 1–3 and 2 and 3
3 0.938 ± 0.026 0.969 ± 0.026 0.984 ± 0.026 1 and 2, 1–3 and 2 and 3
1 0.953 ± 0.025 0.984 ± 0.025 0.969 ± 0.025 1 and 2, 1–3 and 2 and 3
Open in a new tab

*P < 0.10; **P < 0.05; ***P < 0.01.

Table 3.

Effect of tDCS on RT in different NPI

NPI Stimulation [M ± SEM (seconds)] Post hoc tests
1: Anodal 2: Sham 3: Cathodal
−5 1.765 ± 0.292 2 ± 0.308 2.171 ± 0.323 1 and 2, 1–3 and 2 and 3
−3 1.968 ± 0.319 2.812 ± 0.353 3.046 ± 0.421 1 and 2*, 1–3** and 2 and 3
−1 2.781 ± 0.364 3.609 ± 0.446 4.593 ± 0.468 1 and 2*, 1–3*** and 2 and 3
5 0.484 ± 0.122 0.422 ± 0.121 0.422 ± 0.169 1 and 2, 1–3 and 2 and 3
3 0.937 ± 0.199 0.906 ± 0.238 0.796 ± 0.210 1 and 2, 1–3 and 2 and 3
1 0.813 ± 0.143 1.125 ± 0.270 1.609 ± 0.356 1 and 2, 1–3 and 2 and 3
Open in a new tab

*P < 0.10; **P < 0.05; ***P < 0.01.

The results confirmed that the impact of anodal stimulation depended on NPI (NPI × stimulation interaction: for choice, F6.58,306.23 = 2.774, P = 0.010, partial η2 = 0.056; for RT, F7.22,335.64 = 1.933, P = 0.062, partial η2 = 0.040). Specifically, anodal stimulation only increased the number of choices consistent with private signals when NPI = −3 (F2,93 = 3.721, P = 0.028, partial η2 = 0.074; anodal, 35.9 ± 6.0 vs sham, 18.8 ± 6.0%, P = 0.053, Cohen’s d = 0.46) and NPI = −5 (F2,93 = 6.497, P = 0.002, partial η2 = 0.123; anodal, 35.9 ± 5.5 vs sham, 12.5 ± 5.5%, P = 0.010, Cohen’s d = 0.67; Table 2) and decreased RT when NPI = −1 (F2,93 = 4.475, P = 0.014, partial η2 = 0.088; anodal, 2.781 ± 0.364 vs sham, 3.609 ± 0.446 s, P = 0.061, Cohen’s d = 0.36) and NPI = −3 (F2,93 = 2.383, P = 0.098, partial η2 = 0.049; anodal, 1.968 ± 0.319 vs sham, 2.812 ± 0.353 s, P = 0.073, Cohen’s d = 0.44; Table 3).

To further assess the effects of anodal and cathodal brain stimulations on participants’ number of choices consistent with their private signals and RT, we ran OLS regression analyses in which we defined ‘anodal’ and ‘cathodal’ as dummy variables. The variable ‘anodal’ was set to 1 if the individual received anodal stimulation or to 0 in all other cases. The variable ‘cathodal’ was set to 1 if the individual received cathodal stimulation or to 0 in all other cases. As shown in Supplementary data, Table S1, these analyses indicate that anodal tDCS increased the number of choices consistent with the private signal (coefficient, 0.172; P = 0.017) and decreased RT (coefficient, −0.635; P = 0.082) in the incongruent conditions but had no significant effect in the congruent condition. In the cathodal group, coefficients in the incongruent and congruent conditions were all insignificant (all Ps > 0.10).

Finally, we ran four robustness checks to control the perception of tDCS and background variables (Robustness check I), cognitive complexity and conformity tendency (Robustness check II), overconfidence (Robustness check III) and risk-taking attitude and loss aversion (Robustness check IV; see the Supplementary data).

Supplementary tDCS experiments

We also performed two additional tDCS experiments. The first tDCS experiment had 36 trials. This experiment tested the order effect to examine how much a participant relied on the anterior, middle or posterior public information to be consistent (or inconsistent) with his or her private signal. The second tDCS experiment had 24 trials in which a participant was the seventh person (P7) to make a choice. This experiment tested the effect of sequential order, examining whether our results were robust when we fixed participants’ position at P7.

Supplementary tDCS experiment with 36 trials

In the first supplementary tDCS experiment, we utilized 36 trials for which a design matrix (2 × 3 × 3 × 2 = 36 trials; Table 4) was created by using all combinations of condition (incongruent vs congruent), absolute NPI (the difference between the number of ‘A’ choices and ‘B’ choices; 5 vs 3 vs 1), order of (in)consistent public information (order of public information consistent with the private signal in the incongruent condition and inconsistent with the private signal in the congruent condition; anterior vs middle vs posterior) and P8’s private signal (ball a vs b). All other aspects of the experimental design and procedure were identical to the main experiment. Thirty-six students (anodal, n = 18; sham, n = 18) participated in this experiment.

Table 4.

Stimuli in the supplementary experiment with 36 trials

Condition Absolute NPI P1–P7’s choices (box) P8’s private signal (ball)
Incongruent (NPI < 0) 5 Anterior A-B-A-A-A-A-A b
B-A-B-B-B-B-B a
Middle A-A-B-A-A-A-A b
B-B-A-B-B-B-B a
Posterior A-A-A-A-A-B-A b
B-B-B-B-B-A-B a
3 Anterior B-A-A-B-A-A-A b
A-B-B-A-B-B-B a
Middle A-A-B-B-A-A-A b
B-B-A-A-B-B-B a
Posterior A-A-A-A-B-B-A b
B-B-B-B-A-A-B a
1 Anterior B-A-B-B-A-A-A b
A-B-A-A-B-B-B a
Middle B-A-A-B-B-A-A b
A-B-B-A-A-B-B a
Posterior B-A-A-A-B-B-A b
A-B-B-B-A-A-B a
Congruent (NPI > 0) 5 Anterior A-B-A-A-A-A-A a
B-A-B-B-B-B-B b
Middle A-A-B-A-A-A-A a
B-B-A-B-B-B-B b
Posterior A-A-A-A-A-B-A a
B-B-B-B-B-A-B b
3 Anterior B-A-A-B-A-A-A a
A-B-B-A-B-B-B b
Middle A-A-B-B-A-A-A a
B-B-A-A-B-B-B b
Posterior A-A-A-A-B-B-A a
B-B-B-B-A-A-B b
1 Anterior B-A-B-B-A-A-A a
A-B-A-A-B-B-B b
Middle B-A-A-B-B-A-A a
A-B-B-A-A-B-B b
Posterior B-A-A-A-B-B-A a
A-B-B-B-A-A-B b
Open in a new tab

Consistent with previous findings, when testing choice as the dependent variable we found a significant main effect of stimulation (F1,34 = 5.422; P = 0.026; partial η2 = 0.138) and an interaction effect of condition × stimulation (F1,34 = 9.039; P = 0.005; partial η2 = 0.210). For the incongruent condition, participants undergoing anodal tDCS had a higher percentage of choices consistent with the private signal than those undergoing sham tDCS (55.864 ± 6.454 vs 34.568 ± 3.799%; P = 0.007; Figure 5). But for the congruent condition anodal and sham, tDCS did not differ (95.444 ± 2.541 vs 99.074 ± 2.502%; P = 0.231). We also found that participants in the anodal group gave higher weight to private information in the incongruent condition (anodal, β = 3.909 ± 0.652; sham, β = 1.733 ± 0.323; P = 0.005), but in the congruent condition no significant difference was found between the anodal and sham stimulation groups (P = 0.421).

Fig. 5.

Fig. 5

Open in a new tab

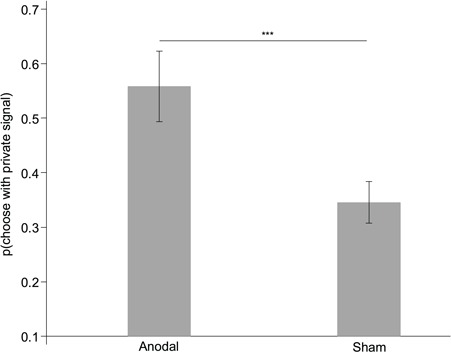
Impact of stimulation on the percentage of choices consistent with private signals in the incongruent condition for the supplementary tDCS experiment with 36 trials. Error bars indicate ±1 SEM. Asterisks indicate significance level ***P < 0.01.

In addition, we found a significant main effect of order of (in)consistent public information (F1.95,66.34 = 3.882; P = 0.026; partial η2 = 0.102) as well as a marginal significant main effect of P8’s private signal (F1,34 = 3.114; P = 0.087; partial η2 = 0.084) on choice, but the interaction effects between order of (in) consistent public information (or P8’s private signal) and other factors were not significant (all Ps > 0.10). Participants were more likely to make a choice in line with their private signal when the order of (in) consistent public information was in the posterior (74.074 ± 2.189%) than in the anterior (68.981 ± 2.312%; P = 0.025), while the middle (69.907 ± 1.805%) and the posterior (or anterior) did not differ (all Ps > 0.10). The percentage of choices consistent with the private signal was higher when participants received an a ball than a b ball (72.07 ± 1.82 vs 69.91 ± 1.96%).

With RT as the dependent variable, there was a marginal interaction effect of condition × stimulation (F1,34 = 3.283; P = 0.079; partial η2 = 0.088). For the incongruent condition, RT in the anodal stimulation was shorter than that in the sham stimulation (1.336 ± 0.338 vs 2.114 ± 0.338 s; P = 0.052; Cohen’s d = 0.38). For the congruent condition, RT did not differ between the anodal and sham stimulations (0.448 ± 0.120 vs 0.509 ± 0.120 s; P = 0.605).

The results of two additional ANOVAs on choice and RT with NPI (−5 vs −3 vs −1 vs 5 vs 3 vs 1) × order of consistent or inconsistent choice × P8’s private signal × stimulation as the factors showed that there was a significant interaction effect of NPI × stimulation on choice (F1.82,61.79 = 5.705; P = 0.007; partial η2 = 0.144) as well as a marginal significant interaction effect of NPI × stimulation on RT (F2.52,85.82 = 2.815; P = 0.081; partial η2 = 0.060). Consistent with previous findings, anodal stimulation only increased the percentage of choices consistent with the private signal when NPI = −3 (anodal, 0.398 ± 0.078; sham, 0.194 ± 0.078; P = 0.013; Cohen’s d = 0.66) and NPI = −5 (anodal, 0.380 ± 0.065; sham, 0.194 ± 0.065; P = 0.052; Cohen’s d = 0.32) and decreased RT when NPI = −1 (anodal, 1.694 ± 0.459 s; sham, 2.852 ± 0.459 s; P = 0.021; Cohen’s d = 0.44) and NPI = −3 (anodal, 1.426 ± 0.404 s; sham, 2.213 ± 0.404 s; P = 0.061; Cohen’s d = 0.30).

Supplementary tDCS experiment with participants in the seventh position

To test the effect of sequential order, we conducted a supplementary tDCS experiment in which a participant was the seventh person (P7) to make a choice. We created a design matrix (2 × 2 × 3 × 2 = 24 trials; Table 5) by using all combinations of condition (incongruent vs congruent), absolute NPI (the difference between the number of ‘A’ choice and ‘B’ choice; 4 vs 2), order of (in)consistent public information (order of public information consistent with the private signal in the incongruent condition or inconsistent with the private signal in the congruent condition; anterior vs middle vs posterior) and P7’s private signal (ball a vs b). All other aspects of the experimental design and procedure were identical to the main experiment. Thirty-one students (anodal, n = 15; sham, n = 16) participated in this experiment.

Table 5.

Stimuli in the supplementary experiment with the seventh position

Condition Absolute NPI P1–P6’s choices (box) P7’s private signal (ball)
Incongruent (NPI < 0) 4 Anterior A-B-A-A-A-A b
B-A-B-B-B-B a
Middle A-A-B-A-A-A b
B-B-A-B-B-B a
Posterior A-A-A-A-A-B b
B-B-B-B-B-A a
2 Anterior B-B-A-A-A-A b
A-A-B-B-B-B a
Middle A-A-B-B-A-A b
B-B-A-A-B-B a
Posterior A-A-A-A-B-B b
B-B-B-B-A-A a
Congruent (NPI > 0) 4 Anterior A-B-A-A-A-A a
B-A-B-B-B-B b
Middle A-A-B-A-A-A a
B-B-A-B-B-B b
Posterior A-A-A-A-A-B a
B-B-B-B-B-A b
2 Anterior B-B-A-A-A-A a
A-A-B-B-B-B b
Middle A-A-B-B-A-A a
B-B-A-A-B-B b
Posterior A-A-A-A-B-B a
B-B-B-B-A-A b
Open in a new tab

With choice as the dependent variable, we found a significant main effect of stimulation (F1,29 = 118.96; P < 0.001; partial η2 = 0.804) and an interaction effect of condition × stimulation (F1,29 = 7.043; P = 0.013; partial η2 = 0.195). For the incongruent condition, participants undergoing anodal tDCS had a higher percentage of choices consistent with their private signals than those undergoing the sham tDCS (42.222 ± 7.947 vs 15.104 ± 7.692%: P < 0.001; Figure 6). But for the congruent condition anodal and sham, tDCS did not differ (93.889 ± 2.361 vs 99.821 ± 2.286%: P = 0.214). We also found that participants in the anodal group gave higher weight to private information in the incongruent condition (anodal, β = 3.531 ± 0.534; sham, β = 1.973 ± 0.417; P = 0.011), but in the congruent condition no significant difference was found between the anodal and sham stimulation groups (P = 0.688).

Fig. 6.

Fig. 6

Open in a new tab

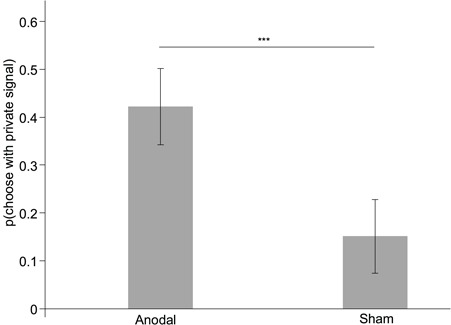
Impact of stimulation on the percentage of choices consistent with private signals in the incongruent condition for the supplementary tDCS experiment with participants in the seventh position. Error bars indicate ±1 SEM. Asterisks indicate significance level ***P < 0.01.

In addition, we found a significant main effect of order of (in)consistent public information (F1.86,53.99 = 4.647; P = 0.016; partial η2 = 0.138) as well as a marginal significant main effect of P7’s private signal (F1,29 = 2.981; P = 0.091; partial η2 = 0.071) on choice, but the interaction effects between order of (in)consistent public information (or P7’s private signal) and other factors were not significant (all Ps > 0.10). Participants were more likely to make a choice in line with the private signal when the order of (in)consistent public information was in the posterior (66.276 ± 3.129%) than in the anterior (62.239 ± 2.981%; P = 0.094) and in the middle (59.895 ± 2.514%; P = 0.024), but the latter two did not differ (P = 0.934). The percentage of choices consistent with the private signal was higher when participants received an a ball than a b ball (65.21 ± 2.81 vs 61.48 ± 2.76%).

With RT as the dependent variable, there was a marginal interaction effect of condition × stimulation (F1,29 = 3.455; P = 0.073; partial η2 = 0.106). For the incongruent condition, RT in the anodal stimulation was shorter than that in the sham stimulation (1.128 ± 0.378 vs 1.911 ± 0.338 s; P = 0.041; Cohen’s d = 0.51). For the congruent condition, RT did not differ between the anodal and sham stimulation (0.394 ± 0.093 vs 0.297 ± 0.090 s; P = 0.329).

The results of two additional ANOVAs on choice and RT with NPI (−4 vs −2 vs 4 vs 2) × order of consistent or inconsistent choice × P7’s private signal × stimulation as the factors showed that there was a significant interaction effect of NPI × stimulation on choice (F1.27,36.91 = 6.395; P = 0.011; partial η2 = 0.181) as well as a marginal significant interaction effect of NPI × stimulation on RT (F1.41,40.77 = 2.953; P = 0.080; partial η2 = 0.092). Anodal stimulation increased the percentage of choices consistent with the private signal when NPI = −4 (anodal, 0.400 ± 0.076; sham, 0.083 ± 0.073; P < 0.001; Cohen’s d = 0.61) and NPI = −2 (anodal, 0.444 ± 0.089; sham, 0.219 ± 0.086; P = 0.021; Cohen’s d = 0.53) and decreased RT when NPI = −4 (anodal, 0.856 ± 0.330 s; sham, 1.406 ± 0.320 s; P = 0.071; Cohen’s d = 0.33) and NPI = −2 (anodal, 1.400 ± 0.458 s; sham, 2.417 ± 0.444 s; P = 0.068; Cohen’s d = 0.28).

Discussion

Information may be weighted in various ways by individuals in sequential decision-making situations. An information cascade occurs when it is optimal for someone who has observed the choices made by others before him or her to follow the behavior of the preceding individuals regardless of the content of his or her own private information. The probability that an information cascade starts depends primarily on how people weight their own private information as compared with public information. Several studies have found that people tend to overweight private information even when following others is the rational choice (e.g. Nöth and Weber, 2003; Goeree et al., 2007). In the present experiment, our goal was to reveal the neural mechanism causing people to overweight private information. Specifically, we employed tDCS over rIFG to assess the role of the stimulated brain area in an individual’s tendency to overweight his or her private signal during a sequential decision-making task.

We found that anodal stimulation of rIFG significantly increased the weight given to private information and decreased RT in the incongruent condition (NPI < 0). This effect could not be attributed to individual differences in personality traits, such as conformity tendency and overconfidence, and it did not occur in the cathodal stimulation group. This finding supported the proposition in our IHM that people tend to give different weights to public and private information, which would have moderated the probability that a cascade occurs. We also found that a participant made more choices consistent with his or her own private signal when receiving an a signal than when receiving a b signal. Because the letter a is prior to the letter b in alphabetical order, it is possible that this order effect might have led to a preference for a. Thus, future studies might design an experiment to control for this potential effect. Furthermore, the results of our two additional tDCS experiments indicated that more weight was given to the private signal, and there was a shorter RT for anodal tDCS over rIFG in the incongruent condition when we controlled the order of P1–P7’s choices and when we fixed participants’ position to P7. This contributes to the robustness of our findings.

Our findings are consistent with the view that rIFG plays an important role in cognitive control (Aron et al., 2004). The increased weight given to private information and decreased RT after anodal stimulation in the incongruent condition might have been due to a more general influence on cognitive control processes required for performing the task. Li et al.(2018) found that frontal N200 (250–340 ms) was more negative in the incongruent condition than in the congruent condition, and the percentage of choices consistent with private signals was correlated with the N200 amplitude. N200 was interpreted as a correlate of cognitive control and conflict processing (see Folstein and Van Petten, 2008, for a review). Previous ERP studies have found that non-matching stimuli elicit larger N200 than matching stimuli, and the detection of conflicts is central to eliciting N200 (Wang et al., 2004). Moreover, comparing non-matching trials with matching trials produces rIFG activation (Hazeltine et al., 2003). In our study, anodal stimulation over rIFG might have led to the higher weight given to private information only when NPI = −5 and NPI = −3, in which a participant encountered greater information conflict. However, if a participant had not received sufficient conflict between private and public information, choices made in the anodal group would have been similar to the other two stimulation groups.

Alternatively, assigning higher weight to a private signal after anodal stimulation of rIFG might have been explained by attentional control. Hampshire et al.(2010) suggested that rIFG plays a role in attentional control. Participants who assigned higher weight to private information allocated initial attention to private signals in 81% of the cases, while participants who followed BNE allocated gaze direction uniformly (Innocenti et al., 2010). Anodal stimulation over rIFG might lead to higher weight given to private signals by allocating more attention to them while allocating less attention to public information. On the other hand, neuroimaging evidence has demonstrated the role of IFG in imitation learning (Buccino et al., 2004). Anodal tDCS over rIFG showed a decrease in the interference effect in an imitation-inhibition task (Hogeveen et al., 2015). Thus, anodal stimulation over rIFG might have ceased observational learning by enhancing the influence of private signals and reducing the influence of public signals. However, these two alternatives are not mutually exclusive and may work together to exert an effective overall influence.

Our findings also showed that anodal stimulation over rIFG decreased RT in incongruent trials, but this effect was only marginally significant when NPI = −3 and NPI = −1. According to sequential sampling models (SSMs; Ratcliff and Smith, 2004), shorter RT indicates easy decisions and longer RT indicates difficult decisions (Rubinstein, 2007). Our results supported the SSM prediction that the highest RT occurred when NPI = −1, while the shortest RT occurred when NPI = 5 (Table 3). Task difficulty might have explained why anodal stimulation over rIFG only decreased RT when NPI = −3 and NPI = −1. Our findings are consistent with recent tDCS studies (Jacobson et al., 2011; Cunillera et al., 2014; Stramaccia et al., 2015), which have found that anodal stimulation over rIFG leads to significant reduction in the stop-signal RT (SSRT) in SS tasks. The SSRT reflects task difficulty and response inhibition level (Logan and Cowan, 1984).

Taken together, we found that the impact of anodal stimulation of rIFG on choices consistent with private signals and RT was specific to situations in which information conflict or task difficulty reached a threshold that might trigger cognitive control-related processing, presumably representing attentional control or attenuated observational learning. It is noteworthy that NPI = −3 showed a trade-off of information conflict and task difficulty. Anodal stimulation over rIFG both increased the weight given to private information and decreased RT when NPI = −3.

Although anodal stimulation over rIFG had a critical impact on the strength of overweighting private information, we posit that it is unlikely that rIFG is the only factor that drives this effect. Instead, rIFG stimulation might have altered the crosstalk of rIFG and other areas that are critical for decision-making, in particular the medial prefrontal cortex (mPFC) and ventromeadial PFC (vmPFC). mPFC has been shown to be involved in the valuation during value-based decision-making (Tom et al., 2007) and in the integration of prior knowledge and likelihood information in calculating Bayesian posterior probabilities (Ting et al., 2015). Campbell-Meiklejohn et al.(2017) used an urn task in which predecessors reported their choices as well as their associated confidence and showed that the value computed from private information and predecessors’ choices was indiscriminately represented across vmPFC. But the value derived from predecessors’ choices weighted by their confidence was represented with specificity for the ventromedial area 10, which may be explained by inferential and integrative processes. Similar to their urn task, in the present sequential decision-making experiment a participant should first observe predecessors’ choices and update his or her belief by forming an evaluation of the probability of the box being A or B. Our results provided evidence that rIFG might override mPFC and vmPFC activities and thus hinder an individual’s decision-making consistent with his or her private information when private information conflicted with public information. Thus, we conjecture that anodal stimulation of rIFG might moderate the interplay of prefrontal areas with areas involved in valuation and opinion formation so that an individual’s decision-making is biased toward cognitive control-related processes at the expense of ‘rational’ decision-making based on Bayes’ rule. That is, rIFG might interfere with Bayes inferential and integrative processes and mediate the weight given to each piece of information, which is accumulated by mPFC or vmPFC. This indicates that the moderating influence of rIFG on mPFC and vmPFC may not be ‘beneficial’. Further studies are needed to explore this relationship more carefully.

tDCS is a safe and non-invasive method that allows us to assess the role of cortical brain areas in cognitive processes such as decision-making. Based on previous fMRI results that identified IFG as a critical area for overweighting private information (Huber et al., 2015), we chose an electrode position (FC6 in the standard EEG 10–10 system) that has been used in previous studies that targeted IFG (Holland et al., 2011; Hogeveen et al., 2015; Nobusako et al., 2017). The reference electrode was positioned posterior to the left mastoid (Breitling et al., 2016). It is important to note, however, that we did not exclusively stimulate rIFG, since the spatial resolution of tDCS is very limited. Using large electrodes (surface of 35 cm2) might produce wide spreading changes in cortical excitability, especially in the neighboring or other connected areas such as the dorsolateral prefrontal cortex (DLPFC). Previous studies have revealed the functions of DLPFC in cognitive control (MacDonald et al., 2000) and self-control (Hare et al., 2009). It is unlikely, however, that any unspecified tDCS effects that are independent of electrode position are responsible for our results because there was no significant improvement from the cathodal stimulation. In addition, small current density values predicted on brain and scalp model surface in Breitling et al.(2016) showed that shunting through low-resistive head tissues diminished largely when injecting through two large remote patch electrodes.

Finally, we did not find any significant effect of cathodal stimulation in spite of evidence of physiologically inhibitory influences by cathodal stimulation (Nitsche et al., 2008). There are two plausible explanations for the lack of behavioral effects by cathodal stimulation. First, several studies proposed that the effect of cathodal stimulation may be task-dependent and induce less stable cognitive behavioral effects than anodal tDCS (e.g. Jacobson et al., 2012). Second, it might reflect a floor effect, as the number of choices consistent with private signals in the sham condition was very small, especially when NPI = −3 and NPI = −5. This might have left little room for cathodal tDCS to decrease the number of choices.

To conclude, we have demonstrated in this study that anodal stimulation over rIFG increased the weight given to private information and decreased RT for the incongruent trials during sequential decision-making. This suggests that the stimulated brain area may play a critical role in reducing rational choice. Our findings of a malleable neural mechanism that influences the overweighting of private information are important to understand the cognitive process that causes individuals to violate rational decision-making in the form of information cascades. It is noteworthy that anodal tDCS over rIFG did not influence choices consistent with the private signal and RT in general but only occurred when information conflict or task difficulty reached a threshold that might trigger cognitive control-related processes. This is one of the most important findings in the present study.

Funding

This work was supported by the National Natural Science Foundation of China (71673152 and 71533002) and National Social Science Foundation of China (16BJY035).

Conflict of interest. None declared.

References

  1. Anderson L.R., Holt C.A. (1997). Information cascades in the laboratory. American Economic Review, 87(5), 847–62. [Google Scholar]
  2. Aron A.R., Robbins T.W., Poldrack R.A. (2004). Inhibition and the right inferior frontal cortex. Trends in Cognitive Sciences, 8(4), 170–7. [DOI] [PubMed] [Google Scholar]
  3. Banerjee A.V. (1992). A simple model of herd behavior. Quarterly Journal of Economics, 107(3), 797–817. [Google Scholar]
  4. Bikhchandani S., Hirshleifer D., Welch I. (1992). A theory of fads, fashion, custom, and cultural change as informational cascades. Journal of Political Economy, 100(5), 992–1026. [Google Scholar]
  5. Breitling C., Zaehle T., Dannhauer M., et al. (2016). Improving interference control in ADHD patients with transcranial direct current stimulation (tDCS). Frontiers in Cellular Neuroscience, 10, 72. [DOI] [PMC free article] [PubMed] [Google Scholar]
  6. Buccino G., Vogt S., Ritzl A., et al. (2004). Neural circuits underlying imitation learning of hand actions: an event-related fMRI study. Neuron, 42(2), 323–34. [DOI] [PubMed] [Google Scholar]
  7. Campbell-Meiklejohn D., Simonsen A., Frith C.D., Daw N.D. (2017). Independent neural computation of value from other people’s confidence. Journal of Neuroscience, 37(3), 673–84. [DOI] [PMC free article] [PubMed] [Google Scholar]
  8. Cunillera T., Fuentemilla L., Brignani D., Cucurell D., Miniussi C. (2014). A simultaneous modulation of reactive and proactive inhibition processes by anodal tDCS on the right inferior frontal cortex. PLoS One, 9(11), e113537. [DOI] [PMC free article] [PubMed] [Google Scholar]
  9. Ellis C.J., Fender J. (2011). Information cascades and revolutionary regime transitions. Economic Journal, 121(553), 763–92. [Google Scholar]
  10. Fischbacher U. (2007). z-Tree: Zurich toolbox for ready-made economic experiments. Experimental Economics, 10(2), 171–8. [Google Scholar]
  11. Folstein J.R., Van Petten C. (2008). Influence of cognitive control and mismatch on the N2 component of the ERP: a review. Psychophysiology, 45(1), 152–70. [DOI] [PMC free article] [PubMed] [Google Scholar]
  12. Frederick S. (2005). Cognitive reflection and decision making. Journal of Economic Perspectives, 19(4), 25–42. [Google Scholar]
  13. Frydman C., Krajbich I. (2017). Using response times to infer others’ beliefs: an application to information cascades. Available: https://ssrn.com/abstract=2817026 Accessed August 2017.
  14. Gächter S., Johnson E.J., Herrmann A. (2007). Individual-level loss aversion in riskless and risky choices. Institute for the Study of Labor Discussion Paper No. 2961, Bonn, Germany: IZA. [Google Scholar]
  15. Goeree J.K., Palfrey T.R., Rogers B.W., McKelvey R.D. (2007). Self-correcting information cascades. Review of Economic Studies, 74(3), 733–62. [Google Scholar]
  16. Hampshire A., Chamberlain S.R., Monti M.M., Duncan J., Owen A.M. (2010). The role of the right inferior frontal gyrus: inhibition and attentional control. NeuroImage, 50(3), 1313–9. [DOI] [PMC free article] [PubMed] [Google Scholar]
  17. Hare T.A., Camerer C.F., Rangel A. (2009). Self-control in decision-making involves modulation of the vmPFC valuation system. Science, 324(5927), 646–8. [DOI] [PubMed] [Google Scholar]
  18. Hazeltine E., Bunge S.A., Scanlon M.D., Gabrieli J.D. (2003). Material-dependent and material-independent selection processes in the frontal and parietal lobes: an event-related fMRI investigation of response competition. Neuropsychologia, 41(9), 1208–17. [DOI] [PubMed] [Google Scholar]
  19. Hogeveen J., Obhi S.S., Banissy M.J., et al. (2015). Task-dependent and distinct roles of the temporoparietal junction and inferior frontal cortex in the control of imitation. Social Cognitive and Affective Neuroscience, 10(7), 1003–9. [DOI] [PMC free article] [PubMed] [Google Scholar]
  20. Holland R., Leff A.P., Josephs O., et al. (2011). Speech facilitation by left inferior frontal cortex stimulation. Current Biology, 21(16), 1403–7. [DOI] [PMC free article] [PubMed] [Google Scholar]
  21. Holt C.A., Laury S.K. (2002). Risk aversion and incentive effects. American Economic Review, 92(5), 1644–55. [Google Scholar]
  22. Huber R.E., Klucharev V., Rieskamp J. (2015). Neural correlates of informational cascades: brain mechanisms of social influence on belief updating. Social Cognitive and Affective Neuroscience, 10(4), 589–97. [DOI] [PMC free article] [PubMed] [Google Scholar]
  23. Huck S., Oechssler J. (2000). Informational cascades in the laboratory: do they occur for the right reasons? Journal of Economic Psychology, 21(6), 661–71. [Google Scholar]
  24. Innocenti A., Rufa A., Semmoloni J. (2010). Overconfident behavior in informational cascades: an eye-tracking study. Journal of Neuroscience, Psychology, and Economics, 3(2), 74–82. [Google Scholar]
  25. Jacobson L., Javitt D.C., Lavidor M. (2011). Activation of inhibition: diminishing impulsive behavior by direct current stimulation over the inferior frontal gyrus. Journal of Cognitive Neuroscience, 23(11), 3380–7. [DOI] [PubMed] [Google Scholar]
  26. Jacobson L., Koslowsky M., Lavidor M. (2012). tDCS polarity effects in motor and cognitive domains: a meta-analytical review. Experimental Brain Research, 216(1), 1–10. [DOI] [PubMed] [Google Scholar]
  27. Kübler D., Weizsäcker G. (2004). Limited depth of reasoning and failure of cascade formation in the laboratory. Review of Economic Studies, 71(2), 425–41. [Google Scholar]
  28. Lee Y.J., Hosanagar K., Tan Y. (2015). Do I follow my friends or the crowd? Information cascades in online movie ratings. Management Science, 61(9), 2241–58. [Google Scholar]
  29. Li J., Niu X., Zhu C., et al. (2018). Electrophysiological precursor of information cascade: evidence from N200. ZBW-German National Library of Economics. Available: http://hdl.handle.net/10419/179966 Accessed May 2018.
  30. Logan G.D., Cowan W.B. (1984). On the ability to inhibit thought and action: a theory of an act of control. Psychological Review, 91(3), 295–327. [DOI] [PubMed] [Google Scholar]
  31. MacDonald A.W., 3rd, Cohen J.D., Stenger V.A., Carter C.S. (2000). Dissociating the role of the dorsolateral prefrontal and anteriorcingulate cortex in cognitive control. Science, 288(5472), 1835–8. [DOI] [PubMed] [Google Scholar]
  32. Nitsche M.A., Cohen L.G., Wassermann E.M., et al. (2008). Transcranial direct current stimulation: state of the art 2008. Brain Stimulation, 1(3), 206–23. [DOI] [PubMed] [Google Scholar]
  33. Nobusako S., Nishi Y., Y., et al. (2017). Transcranial direct current stimulation of the temporoparietal junction and inferior frontal cortex improves imitation-inhibition and perspective-taking with no effect on the Autism-Spectrum Quotient score. Frontiers in Behavioral Neuroscience, 11, 84. [DOI] [PMC free article] [PubMed] [Google Scholar]
  34. Nöth M., Weber M. (2003). Information aggregation with random ordering: cascades and overconfidence. Economic Journal, 113(484), 166–89. [Google Scholar]
  35. Ratcliff R., Smith P.L. (2004). A comparison of sequential sampling models for two-choice reaction time. Psychological Review, 111(2), 333–67. [DOI] [PMC free article] [PubMed] [Google Scholar]
  36. Rau H.A. (2014). The disposition effect and loss aversion: do gender differences matter? Economics Letters, 123(1), 33–6. [Google Scholar]
  37. Ren Y., Croson R. (2013). Overconfidence in newsvendor orders: an experimental study. Management Science, 59(11), 2502–17. [Google Scholar]
  38. Rubinstein A. (2007). Instinctive and cognitive reasoning: a study of response times. Economic Journal, 117(523), 1243–59. [Google Scholar]
  39. Ruff C.C., Ugazio G., Fehr E. (2013). Changing social norm compliance with noninvasive brain stimulation. Science, 342(6157), 482–4. [DOI] [PubMed] [Google Scholar]
  40. Simon H.A. (1996). The Sciences of the Artificial, Cambridge, MA: MIT Press. [Google Scholar]
  41. Stramaccia D.F., Penolazzi B., Sartori G., Braga M., Mondini S., Galfano G. (2015). Assessing the effects of tDCS over a delayed response inhibition task by targeting the right inferior frontal gyrus and right dorsolateral prefrontal cortex. Experimental Brain Research, 233(8), 2283–90. [DOI] [PubMed] [Google Scholar]
  42. Ting C.C., Yu C.C., Maloney L.T., Wu S.W. (2015). Neural mechanisms for integrating prior knowledge and likelihood in value-based probabilistic inference. Journal of Neuroscience, 35(4), 1792–805. [DOI] [PMC free article] [PubMed] [Google Scholar]
  43. Tom S.M., Fox C.R., Trepel C., Poldrack R.A. (2007). The neural basis of loss aversion in decision-making under risk. Science, 315(5811), 515–8. [DOI] [PubMed] [Google Scholar]
  44. Walden E.A., Browne G.J. (2009). Sequential adoption theory: a theory for understanding herding behavior in early adoption of novel technologies. Journal of the Association for Information Systems, 10(1), 31–62. [Google Scholar]
  45. Wang Y., Cui L., Wang H., Tian S., Zhang X. (2004). The sequential processing of visual feature conjunction mismatches in the human brain. Psychophysiology, 41(1), 21–9. [DOI] [PubMed] [Google Scholar]
  46. Weizsäcker G. (2010). Do we follow others when we should? A simple test of rational expectations. American Economic Review, 100(5), 2340–60. [Google Scholar]

Articles from Social Cognitive and Affective Neuroscience are provided here courtesy of Oxford University Press

RESOURCES