Abstract
The aim of this study was to evaluate dehydrated murta berries enriched with probiotic (Lactobacillus casei var. rhamnosus) bacteria. L. casei was incorporated to fresh murta by vacuum impregnation at alternative conditions (pressure 50, 150 and 300 mbar; time 5, 10 and 15 min; temperature 20 ± 0.2 °C) and impregnated murta samples were dehydrated by two drying methods at 40 °C, vacuum and convective drying. Both drying processes were modeled by three mathematical models (Weibull, Page and modified Page). According to the statistical tests applied, the Weilbull model obtained the best-fit quality on experimental data. Effective moisture diffusivity varied between 1.23–1.75 × 10−10 m2/s and 1.16–1.44 × 10−10 m2/s for vacuum and convective drying, respectively. After impregnation, murta berries contained approximately 107 CFU/g L. casei although maximum counts were found at 150 mbar for 15 min. Drying decreased L. casei viability in 1.5–1.9 log and 0.5–1.2 log for vacuum and convective drying, respectively. Thus, impregnation at 150 mbar for 15 min followed by convective drying at 40 °C appears as the method of choice to produce probiotic enriched murta berries that can be commercialized as probiotic dried snacks.
Keywords: Murta berries, Lactobacillus casei, Vacuum impregnation, Drying, Process modelling, Probiotics
Introduction
During the last decades, increasing concern of consumers about foods and their related health effects has boosted globally the consumption of berries and probiotics (Rascón et al. 2018). To date, probiotic-enriched foods are mostly dairy products (yogurt, yogurt drinks) that have the disadvantage of requiring cold storage conditions and cannot be consumed by the lactose intolerant population. Enriching foods with probiotics is challenging as cell viability in the food matrix depends on several factors, including intrinsic (bacterial species, presence of competing microorganisms and inhibitors) and extrinsic (impregnation method, pH, process temperature, oxygen levels among others) (Betoret et al. 2017; Mattila-Sandholm et al. 2002). Lactic acid bacteria such as Lactobacillus acidophilus and Lactobacillus casei are among the most widely used probiotics, conferring beneficial effects on the host when administered in appropriate amounts (Chaikham et al. 2013; Kligler and Cohrssen 2008). Probiotics can be added either to fresh or dehydrated foods although dried foods normally have a longer shelf-life than fresh foods (Weinbreck et al. 2010).
Murta or murtilla (Ugni molinae Turcz) berry comes from a wild-growing native shrub of the southern regions of Chile. The potential of murta berries as beneficial foods is given by the abundance of bioactive compounds with health-promoting properties (Ruiz et al. 2010). Among these are vitamins, minerals and fiber (Scheuermann et al. 2008). At present, industrial murta-based food products include jam, jellies, tea and liquor. Given the benefits of berries and probiotics, a combination of both products would result in a functional food product without the side effects of milk-based products (Betoret et al. 2012).
Vacuum impregnation can be considered as a useful technology to introduce solutes into the structural food matrix, thus modifying its composition. This technology is generally employed to enrich foods with bioactive compounds, resulting in foods with increased nutritional functionality (Zhao and Xie 2004). Fruits have been successfully impregnated with probiotics using this technology. For example, dried apple snacks were enriched with L. salivarius spp. (Betoret et al. 2017), Bifidobacterium spp., and Phoma glomerata (Alzamora et al. 2005) and fresh apple cylinders fortified with S. cerevisiae and L. casei subsp. rhamnosus (Betoret et al. 2003). In all these reports, impregnated apple samples contained around 107 CFU/g of the studied microorganism. As vacuum impregnation is generally performed in fresh (non-dehydrated) foods, after impregnation, fruit and vegetables are highly unstable due to their high water activity (aw). To increase their shelf life, perishable foods can be processed by drying (Betoret et al. 2012). Thus, vacuum impregnation followed by drying appears as a good combination to obtain stable and enriched dried foods with high functional properties.
Dehydrated foods have the benefit of retaining the impregnated probiotics as well as conserving and prolonging the product’s shelf life (Wu et al. 2007). Many drying processes have been used to dehydrate food after vacuum impregnation. Among these are hot-air (Betoret et al. 2003), vacuum (Wirjantoro et al. 2015) and microwave drying (Contreras et al. 2005). In addition, spherical shaped foods are commonly dried with fluidized-bed dryers (Poós and Szabó 2018). However, there are several disadvantages associated to drying processes, which include damage to the product, excessive energy consumption, excessive wear on the drying equipment and low product yields. In order to predict an optimum drying process that minimizes all these variables, mathematical modeling of drying kinetics behavior can be applied (Babalis and Belessiotis 2004). There are several empirical equations that are usually used to study and model the drying kinetics of food, such as Newton, Page, Henderson–Pabis, modified Page, Logarithmic and Midilli-Kucuk, all of which are ultimately governed by the diffusional model of Fick’s second law (Doymaz 2005). In this work we evaluated the impregnation efficiency of L. casei subsp. rhamnosus into murta berries under diverse vacuum impregnation conditions and the viability of the same probiotic L. casei subsp. rhamnosus after dehydration by convective and vacuum drying.
Materials and methods
Raw material and proximal composition
Murta berries (Ugni molinae T.) var. Red Pearl-INIA were purchased from a berry distributor (Hortifrut Chile S.A.). Samples were selected to provide a homogeneous group, based on their color, size and freshness according to visual analysis. They were stored at 4.0 ± 0.1 °C and 90.1 ± 0.2% humidity in a refrigerator (Samsung SR-34RMB, Seoul, South Korea) for a maximum of 24 h before processing. The moisture content (AOAC no. 934.06), crude protein (AOAC no. 960.52), lipid content (AOAC no. 960.39), crude fiber (AOAC no. 962.09) and crude ash (AOAC no. 923.03) were determined following the AOAC methods. Refractive index was measured with a refractometer (Abbe, ATAGO1-T, Tokyo, Japan), water activity with a water activity meter (AW-Sprint Novasina, TH-500, Neuheimstrasse, Lachen, Switzerland) and pH with a potentiometer (Extech Instruments, Microcomputer pH-Vision 246,072, Waltham, MA, USA). All determinations were performed in triplicate and expressed in g/100 g sample.
Microorganisms and vacuum impregnation
Lactobacillus casei subsp. Rahmnosus (LCR35) was isolated from commercial probiotic capsules “Lactil” (Laboratorio Chile) which contain 108 CFU/g lyophilized L. casei. Single colonies were isolated, grown in Man, Rogosa and Sharpe (MRS) broth and stored as 50% glycerol stocks at a 0.5 McFarland turbidity in sterile cryogenic vials. Glycerol stocks were stored at − 80 °C until use. Previous to the experiments, cultures were activated on MRS agar plates at 37 °C for 72 h. One colony was inoculated in 10 mL of MRS broth and incubated overnight at 37 °C. For impregnation, 4 mL of an overnight grown culture in MRS media were transferred to 1 L commercial apple juice and the pH was adjusted to 5.8–6.0 by adding sodium bicarbonate (approximately 5 g/L). The impregnation solution was incubated at 37 °C for 24 h. Murta berries were impregnated with 108 CFU/g L. casei, as described in Betoret et al. (2012). Briefly, the berries were immersed in impregnation solution in a 1/5 (w/w) fruit/impregnation solution ratio. The impregnation process was performed at 20.0 ± 0.2 °C in a vacuum oven (Memmert, model VO 400, Frankfurt, Germany) at three different pressure intensities and times. Pressurization conditions were 50, 150 and 300 mbar for 5, 10 and 15 min. These conditions were shown to minimally affect the viability of probiotic bacteria (Betoret et al. 2012; Krasaekoopt and Suthanwong 2008; Noorbakhsh et al. 2013; Alzamora et al. 2005). In all treatments atmospheric pressure was restored for additional 10 min. Immediately after impregnation, probiotic-enriched murta samples (heretofore impregnated murta) were packed in polyethylene bags and stored at 4.0 ± 0.1 °C in a refrigerator (Samsung SR-34RMB, Seoul, South Korea) until subsequent drying treatment.
Drying methods
Drying was performed immediately after impregnation. Probiotic-enriched murta samples were dehydrated by two drying methods, vacuum and convective drying. Vacuum drying was carried out in a vacuum oven (Memmert, VO 400, Frankfurt, Germany) at a temperature of 40.0 ± 0.2 °C under a vacuum pressure of 150 mbar. Convective drying was performed in a hot-air dryer at an air velocity of 1.2 ± 0.1 m/s and air temperature of 40 ± 0.2 °C. Samples were distributed as a thin layer on a stainless-steel basket and placed inside both dryers under the aforementioned conditions. Sample weight was controlled with an analytical balance (Mettler Toledo, XS205 DU, Schwerzenbach, Switzerland) from the beginning until the end of each drying process at defined time intervals until samples reached a constant weight (equilibrium condition). Dried samples were packed in polyethylene bags and refrigerated at 4.0 ± 0.1 °C. Immediately after drying, murta samples were analyzed by plate counts for viable microorganisms as described below.
Viable plate counts
Microbial counts were determined from the impregnation solution and impregnated murta before and after drying. Counts from the impregnation solution were performed by plating 10−1–10−8 dilutions of impregnation solution on MRS agar plates and incubating at 37 °C for 72 h. Total viable counts from impregnated murta and impregnated-dried murta were measured by mixing 5 g sample with 45 mL sterile 0.1% peptone water and homogenized in a stomacher for 2 min. Homogenized samples were serially diluted (to 10−10) in 0.1% peptone water and bacteria were counted on MRS agar after incubation at 37 °C for 72 h.
Effective moisture diffusivity (De)
For a better comprehension and simplicity regarding drying phenomena, the following assumptions were considered: (1) murta berries have a spherical shape with a radius (r), (2) effective moisture diffusivity is homogeneous throughout the berry, (3) moisture moves radially from inside of the berry to the surface, (4) shrinkage during drying is negligible, (5) mass transfer is symmetric and (6) moisture distribution is uniform in the whole berry after impregnation and before drying. Since moisture diffusion is one of the main mass transport mechanisms that describe drying processes (Babalis and Belessiotis 2004), Fick’s second law of diffusion (Eq. 1) was applied to describe the drying process based on the above-mentioned assumptions. The mathematical solution, when internal mass transfer is the controlling mechanism and transport in a sphere is assumed, is given by Eq. 2 (Vega-Gálvez et al. 2014).
| 1 |
| 2 |
Mathematical modeling
To fit the experimental drying data of probiotic-enriched murta samples, three mathematical models were applied: Weibull, Page and modified Page corresponding to Eqs. 3, 4 and 5, respectively (Lemus-Mondaca et al. 2009; Corzo and Bracho 2008). The parameter k (1/min) is known as a kinetic parameter or drying constant (Doymaz 2007). The constant n is known as an empirical parameter (dimensionless) that could depend on the existence of an external skin or shrinkage phenomena (Babalis and Belessiotis 2004). However, this study did not evaluate these parameters since the mass transport inside the berry is assumed to be an isothermal phenomenon (Simal et al. 2005). The shape parameter α is dimensionless and is related to the mass transfer rate at the beginning, e.g. the lower the α value, the faster the drying rate at the beginning (Corzo and Bracho 2008). The scale parameter β (min) can be interpreted as a kinetic reaction constant and represents the time when concentration, in this case, Xt–Xe attains a value corresponding to 36.8% of Xo–Xe (Corzo and Bracho 2008).
| 3 |
| 4 |
| 5 |
Statistical analysis
Fit goodness between predicted and experimental data was evaluated based on statistical analyses including sum squared error (Eq. 6) and Chi square (Eq. 7) (Doymaz 2007). The effect of vacuum impregnation conditions and drying methods on water diffusion coefficients, empirical parameters and viable microorganisms was estimated using StatgraphicsPlus® 5.1 (Statistical Graphics Corp., Herndon, VA, USA) with analysis of variance (ANOVA). Differences between the media were analyzed using the least significant difference test with a significance level of α = 0.05 and a confidence interval of 95% (p < 0.05). In addition, the multiple range test (MRT) was used to demonstrate the existence of homogeneous groups.
| 6 |
| 7 |
Results and discussion
Proximal composition
Fresh murta samples (var. Red Pearl-INIA) presented a moisture content of 80.26 ± 0.95 g/100 g crude protein (N × 6.25) of 1.11 ± 0.01 g/100 g total lipids of 0.30 ± 0.02 g/100 g crude fiber of 3.41 ± 0.14 g/100 g crude ash of 0.66 ± 0.06 g/100 g and available carbohydrates (by difference) of 17.67 ± 0.93 g/100 g. Water activity, soluble solids, pH and titrimetric acidity values were 0.98 ± 0.00, 16.48 ± 0.19° Brix, 3.82 ± 0.27 and 1.01 ± 0.14%, respectively. These results were comparable to those reported for murta var. Red Pearl-INIA (Seguel and Montenegro 2010). It should be noted, however, that the water content of the murta berries may vary during harvest season.
Evaluation of drying kinetics of impregnated murta
Murta berries were vacuum impregnated at 50, 150 and 300 mbar for 5, 10 and 15 min. Following vacuum impregnation, the moisture of murta samples ranged between 73.27 and 78.73 g water/100 g sample and the water activity (aw) averaged 0.98 ± 0.01 (Table 1). At these high values of moisture and aw, murta berries are susceptible to spoilage and chemical degradation during storage. Drying would increase the shelf-life and reduce chemical degradation as well as reducing moisture until a level near to the bond water, improving the characteristic crunchiness obtained at this level. Impregnated and dehydrated murta can be consumed as a snack or as an ingredient in breakfast products. Since drying temperature may have a negative effect on probiotics viability, impregnated murta berries were dried at 40 ± 0.2 °C by both vacuum and convective drying. The selection of drying temperature was based in the optimum for L. casei (37 °C). Previous reports have also successfully impregnated L. casei in fruits demonstrating that 40 °C is an adequate temperature for drying of this bacterium (Betoret et al. 2012; Krasaekoopt and Suthanwong 2008; Noorbakhsh et al. 2013).
Table 1.
Water activity and moisture of impregnated and impregnated-dehydrated murta berries
| Drying methods | Impregnation time (min) | Water activity | Moisture | ||||
|---|---|---|---|---|---|---|---|
| Impregnation pressure (mbar) | Impregnation pressure (mbar) | ||||||
| 50 | 150 | 300 | 50 | 150 | 300 | ||
| Vacuum drying | |||||||
| 5 | 0.9774 ± 0.0075aA | 0.9809 ± 0.0203aA | 0.9736 ± 0.0374aA | 75.69 ± 0.34aA | 77.57 ± 0.48aB | 76.01 ± 0.72aA | |
| Impregnated | 10 | 0.9811 ± 0.0035aA | 0.9752 ± 0.0205aA | 0.9824 ± 0.0349bA | 77.35 ± 1.01bA | 77.26 ± 0.26aA | 78.5 ± 1.58bA |
| 15 | 0.9773 ± 0.0345aA | 0.9785 ± 0.0114aA | 0.9714 ± 0.0162aA | 78.73 ± 0.65bA | 76.53 ± 1.01aB | 77 ± 1.21abAB | |
| 5 | 0.6441 ± 0.0075aA | 0.6182 ± 0.0203aA | 0.6523 ± 0.0374aA | 22.19 ± 0.88bA | 22.57 ± 0.82abA | 22.63 ± 0.75aA | |
| Dehydrated | 10 | 0.6511 ± 0.0035aA | 0.6390 ± 0.0205aA | 0.6349 ± 0.0349aA | 20.32 ± 0.84aA | 22.73 ± 0.74bB | 21.5 ± 0.42aAB |
| 15 | 0.6550 ± 0.0345aA | 0.6455 ± 0.0114aA | 0.6683 ± 0.0162aA | 22.39 ± 0.36bA | 21.17 ± 0.6aB | 21.94 ± 0.58aAB | |
| Convective drying | |||||||
| 5 | 0.9851 ± 0.0003cA | 0.9766 ± 0.0013aB | 0.9813 ± 0.0018bA | 73.79 ± 0.23aA | 76.46 ± 0.84aB | 76.92 ± 1.12aB | |
| Impregnated | 10 | 0.9769 ± 0.0001aA | 0.9800 ± 0.0033aA | 0.9748 ± 0.0026aA | 75.22 ± 0.23bA | 77.6 ± 0.53aB | 75.76 ± 0.25aA |
| 15 | 0.9809 ± 0.0000bA | 0.9742 ± 0.0016aB | 0.9789 ± 0.0002abB | 73.27 ± 0.50aA | 75.87 ± 0.12aB | 77.2 ± 0.70aA | |
| 5 | 0.5819 ± 0.0016bA | 0.5038 ± 0.0026aC | 0.5340 ± 0.0018bB | 18.52 ± 0.83cA | 15.85 ± 0.55aB | 17.98 ± 0.19aA | |
| Dehydrated | 10 | 0.5434 ± 0.0048aA | 0.5031 ± 0.0022aB | 0.5453 ± 0.0002cA | 13.45 ± 0.05aA | 18.12 ± 0.43bB | 16.72 ± 0.90aB |
| 15 | 0.5738 ± 0.0031bA | 0.5303 ± 0.0020bB | 0.5155 ± 0.0016aC | 16.66 ± 0.54bA | 18.63 ± 0.37bB | 18.47 ± 0.33aB | |
Lowercase letters (a, b and c) show the effect of impregnation time at constant pressure
Uppercase letters (A, B and C) show the effect of impregnation pressure at constant time
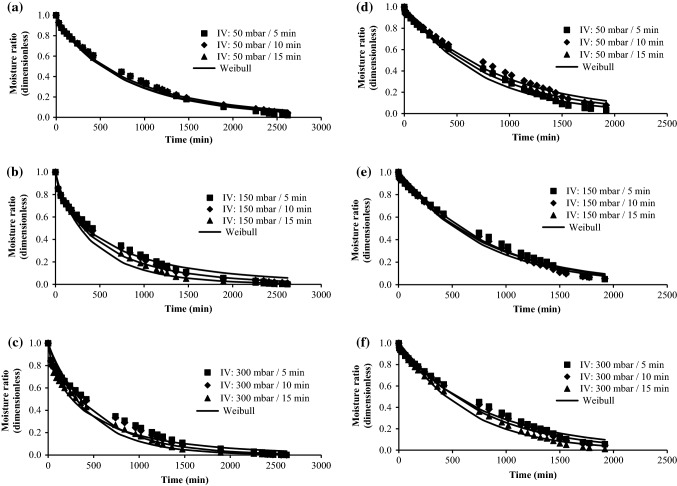
Drying curves of impregnated murta obtained from both vacuum and convective drying, showed that at any impregnation condition and drying method, equilibrium moisture is reached at the end of the drying process (Fig. 1). As such, an increase in impregnation pressure and time is accompanied by a decrease in drying time. Based on the foregoing, the drying time needed to achieve equilibrium moisture content was between 2000–2400 min for vacuum drying and between 1800–2000 min for convective drying. Samples had a final moisture content of 20–22 g water/100 g sample for vacuum and 13–18 g water/100 g sample for convective drying and an aw of 0.61–0.66 for vacuum and 0.50–0.58 for convective drying (Table 1), values at which the growth of spoilage microorganisms is inhibited (ICMSF 2011). In addition, vacuum and convective drying curves showed an extremely prolonged period of falling drying rate. Similar results were obtained for other foods e.g. for convective-dried silverside fish, (Toujani et al. 2012), air-dried peach slices, (Zhu and Shen 2014), vacuum-dried carrots (Chen et al. 2016) and vacuum dried strawberry (Orak et al. 2011).
Fig. 1.
Drying kinetics and modeling of murta berries as a function of vacuum impregnation conditions (time and pressure). a–c vacuum drying. d–f convective drying. Conditions were 40 °C for vacuum and convective drying. Models correspond to Weibull
Vacuum impregnation at 50 mbar for 5 min resulted in the longest drying times for both drying methods, 2400 min for vacuum and 2000 min for convective, while the shortest drying time was observed at 300 mbar for 15 min for both drying methods (2000 min for vacuum and 1800 min for convective). Independent of the similarities between methods, drying time was slightly but significantly shorter (p < 0.05) after convective drying compared to vacuum drying. This phenomenon can be explained by the hydrodynamic mechanism that occurs during vacuum impregnation, as samples subjected to vacuum impregnation suffer deformations and contractions of the fruit matrix due to the replacement of impregnation liquid in the pore spaces that were filled with air (Alzamora et al. 2005). Thus, application of vacuum to a food matrix where air was replaced by liquid would avoid vacuum-induced air expansion leading to a reduced area to volume ratio reducing consequently mass transfer.
Determination of effective moisture diffusivity
The diffusivity values obtained using Eq. 2 at different impregnation conditions and drying processes (vacuum and convective drying) are presented in Table 2. Effective moisture diffusivity varied in the range of 1.23–1.75 × 10−10 m2/s after vacuum drying and between 1.16 and 1.44 × 10−10 m2/s after convective drying. Both drying techniques were operated at 40 °C. In general, diffusivity values increased progressively as the impregnation pressure increased from 50 to 300 mbar. However, values were significantly (p < 0.05) higher after vacuum drying at 150 and 300 mbar at all impregnation times, compared to convective drying. Only at impregnation conditions of 50 mbar for any impregnation time, De values were slightly higher for convective than for vacuum drying. The highest De value (1.75 ± 0.06 × 10−10 m2/s) was obtained after vacuum drying at 300 mbar/15 min, followed by treatment at 150 mbar/15 min (De = 1.69 ± 0.08 × 10−10 m2/s); while the lowest De value (1.23 ± 0.06 × 10−10 m2/s) occurred at 50 mbar and 10 min. The maximum and minimum De values for convective drying were 1.44 ± 0.08 × 10−10 m2/s and 1.16 ± 0.07 × 10−10 m2/s, at 300 mbar/15 min and 50 mbar/10 min, respectively. Several reports on fruits and vegetables subjected to similar convective drying conditions showed De values between 3.91 and 6.65 × 10−10 m2/s for tomato (Doymaz 2007) and 6.25–24.32 × 10−10 m2/s for Chilean papaya (Lemus-Mondaca et al. 2009). To evaluate the influence of impregnation pressure at a fixed impregnation time, a bifactorial ANOVA was conducted on the De media of the impregnated-dried murta samples at each drying process (Table 2). The values showed significant differences (p < 0.05). Similarly, studying the influence of impregnation time at a constant impregnation pressure, bifactorial ANOVA on the media of De of the impregnated-dried murta samples showed significant differences (p < 0.05). Additionally, a multiple lineal regression test carried out on effective moisture diffusivities for the impregnated-dried murta samples resulted in Eq. 8 for vacuum drying and Eq. 9 for convective drying, which showed the interaction of moisture diffusivity with respect to impregnation pressure and time under these drying methods. According to these equations with a high coefficient of determination, r2 > 0.99, the impregnation time (T) seems to be a more relevant factor compared to impregnation pressure (P). This showed also that impregnation accelerated both drying processes.
| 8 |
| 9 |
Table 2.
Effective moisture diffusivity (× 10−10 m2/s) during the drying of murta at different impregnation conditions
| Drying methods | Impregnation time (min) | Impregnation pressure (mbar) | ||
|---|---|---|---|---|
| 50 | 150 | 300 | ||
| Vacuum drying | 5 | 1.24 ± 0.01aA | 1.38 ± 0.04bA | 1.60 ± 0.08cA |
| 10 | 1.23 ± 0.06aA | 1.50 ± 0.07bB | 1.42 ± 0.05bB | |
| 15 | 1.25 ± 0.01aA | 1.69 ± 0.08bB | 1.75 ± 0.06bC | |
| Convective drying | 5 | 1.35 ± 0.00aA | 1.21 ± 0.04bA | 1.24 ± 0.06bA |
| 10 | 1.16 ± 0.07aB | 1.41 ± 0.08bB | 1.31 ± 0.05bAB | |
| 15 | 1.29 ± 0.01aC | 1.29 ± 0.08aAB | 1.44 ± 0.08bB | |
Lowercase letters (a, b and c) show the effect of impregnation time at constant pressure
Uppercase letters (A, B and C) show the effect of impregnation pressure at constant time
Drying models and kinetic parameters
Several factors influence the drying process, e.g. air flow velocity and temperature, rate of water diffusion through the material, load density, thickness and shape of the product. Rapid removal of water during drying can decrease the flavor and nutritional value of foods (Lemus-Mondaca et al. 2009). The high temperatures of some processes destroy the semipermeable cell membranes that form fruit and vegetable tissues and that are essential to maintain their turgescence resulting in case hardening and shrinkage (Simal et al. 2005). All these negative factors can be minimized by studying the drying kinetics of fruits or vegetables and applying a rational design of the drying process to obtain high-quality dehydrated products (Vega-Gálvez et al. 2008). Table 3 shows the average and deviation values of the k and n parameters obtained from Page, modified Page and α and β for the Weibull models.
Table 3.
Kinetics and empirical parameters of the selected models used to model the drying curves under different impregnation conditions
| Empirical model | Impregnation time (min) | Impregnation pressure (mbar) | |||
|---|---|---|---|---|---|
| 50 | 150 | 300 | |||
| Vacuum drying | |||||
| Page | n | 5 | 0.8619 ± 0.0148 | 0.6963 ± 0.0030 | 0.6851 ± 0.0071 |
| 10 | 0.8544 ± 0.0310 | 0.7806 ± 0.0068 | 0.9300 ± 0.0098 | ||
| 15 | 0.8911 ± 0.0423 | 0.8566 ± 0.0799 | 0.9386 ± 0.0204 | ||
| k | 5 | 0.0032 ± 0.0001 | 0.0120 ± 0.0011 | 0.0156 ± 0.0021 | |
| 10 | 0.0033 ± 0.0001 | 0.0078 ± 0.0013 | 0.0030 ± 0.0000 | ||
| 15 | 0.0027 ± 0.0008 | 0.0058 ± 0.0023 | 0.0032 ± 0.0007 | ||
| Modified Page | n | 5 | 0.8619 ± 0.0148 | 0.6962 ± 0.0030 | 0.6850 ± 0.0071 |
| 10 | 0.8544 ± 0.0310 | 0.7865 ± 0.0069 | 0.9300 ± 0.0098 | ||
| 15 | 0.8911 ± 0.0423 | 0.8565 ± 0.0800 | 0.9385 ± 0.0204 | ||
| k | 5 | 0.0013 ± 0.0001 | 0.0017 ± 0.0001 | 0.0023 ± 0.0006 | |
| 10 | 0.0012 ± 0.0003 | 0.0020 ± 0.0003 | 0.0019 ± 0.0001 | ||
| 15 | 0.0013 ± 0.0000 | 0.0023 ± 0.0002 | 0.0022 ± 0.0002 | ||
| Weibull | α | 5 | 0.8619 ± 0.0148 | 0.6962 ± 0.0030 | 0.6851 ± 0.0071 |
| 10 | 0.8544 ± 0.0310 | 0.7865 ± 0.0069 | 0.9300 ± 0.0098 | ||
| 15 | 0.8911 ± 0.0423 | 0.8565 ± 0.0799 | 0.9386 ± 0.0204 | ||
| β | 5 | 779.18 ± 62.08 | 579.98 ± 61.35 | 446.40 ± 114.53 | |
| 10 | 829.17 ± 176.69 | 505.97 ± 76.40 | 525.80 ± 25.44 | ||
| 15 | 774.38 ± 12.85 | 428.39 ± 39.29 | 453.42 ± 40.08 | ||
| Convective drying | |||||
| Page | n | 5 | 1.0334 ± 0.0432 | 1.0614 ± 0.0435 | 0.9519 ± 0.0018 |
| 10 | 0.9732 ± 0.0196 | 1.0159 ± 0.0374 | 1.0383 ± 0.0033 | ||
| 15 | 0.9970 ± 0.0368 | 0.9794 ± 0.0055 | 1.0420 ± 0.0298 | ||
| k | 5 | 0.0011 ± 0.0003 | 0.0008 ± 0.0003 | 0.0018 ± 0.0001 | |
| 10 | 0.0014 ± 0.0003 | 0.0012 ± 0.0001 | 0.0010 ± 0.0000 | ||
| 15 | 0.0013 ± 0.0003 | 0.0014 ± 0.0001 | 0.0012 ± 0.0002 | ||
| Modified Page | n | 5 | 1.0334 ± 0.0432 | 1.0614 ± 0.0435 | 0.9519 ± 0.0018 |
| 10 | 0.9732 ± 0.0196 | 1.0159 ± 0.0374 | 1.0383 ± 0.0033 | ||
| 15 | 0.9970 ± 0.0368 | 0.9794 ± 0.0055 | 1.0420 ± 0.0298 | ||
| k | 5 | 0.0014 ± 0.0001 | 0.0012 ± 0.0000 | 0.0013 ± 0.0001 | |
| 10 | 0.0011 ± 0.0001 | 0.0013 ± 0.0002 | 0.0013 ± 0.0001 | ||
| 15 | 0.0013 ± 0.000 | 0.0013 ± 0.0001 | 0.0016 ± 0.0000 | ||
| Weibull | α | 5 | 1.0334 ± 0.0432 | 1.0614 ± 0.0435 | 0.9519 ± 0.0018 |
| 10 | 0.9732 ± 0.0196 | 1.0159 ± 0.0374 | 1.0383 ± 0.0033 | ||
| 15 | 0.9970 ± 0.0368 | 0.9794 ± 0.0055 | 1.0420 ± 0.0298 | ||
| β | 5 | 713.30 ± 41.30 | 832.06 ± 27.71 | 789.35 ± 33.37 | |
| 10 | 885.96 ± 73.21 | 756.49 ± 125.17 | 746.40 ± 31.82 | ||
| 15 | 798.82 ± 28.32 | 803.96 ± 60.64 | 626.76 ± 14.90 | ||
In none of the three models there was a positive correlation (R2 < 0.70) between the kinetics (k and β) or empirical (n and α) parameters and impregnation conditions (pressure and time). ANOVA from the averages of the k, β, n and α parameters at a 95% confidence level, revealed that these parameters were not statistically different (p > 0.05) for each impregnation conditions used. Thus, n and α parameters may depend mainly on characteristics of the cellular tissue, drying air velocity, relative humidity, and initial moisture content (Babalis and Belessiotis 2004). However, by performing a comparison between kinetic parameters of each drying method, the α parameter evidenced a higher drying rate for convective drying (from 0.68 to 0.93), than for vacuum drying (from 0.95 to 1.03). Likewise, the β parameter from vacuum dried samples ranged from 428 to 829 min showing a relatively long drying period compared to drying by hot air (626–885 min). The modified Page model, which is an alternate form of the Weibull model, has been applied by many authors to describe drying kinetics of different foods (Doymaz 2005; Simal et al. 2005; Vega-Gálvez et al. 2008). Considering that an important aspect of the drying kinetic models is to predict drying rate and drying time, the Weibull model can be used with good accuracy in the case of impregnated-dried murta berries.
As kinetic models can be used with good accuracy to predict drying rates and drying times, SSE and χ2 tests were applied to support these models. Our results showed a good fit for the three models. Among these, Weibull showed the best fit quality for both drying methods, vacuum: 0.0001 ≤ SSE ≤ 0.0006 and 0.0001 ≤ χ2 ≤ 0.0007 and convective: 0.0001 ≤ SSE ≤ 0.0006 and 0.0001 ≤ χ2 ≤ 0.0007. Page and modified Page models are also statistically solid models with low values for two drying techniques, vacuum: 0.0006 ≤ SSE ≤ 0.0011 and 0.0006 ≤ χ2 ≤ 0.0012 and convective: 0.0008 ≤ SSE ≤ 0.0013 and 0.0008 ≤ χ2 ≤ 0.0014. In addition, the three models obtained on average a value of R2 > 0.95, which confirms the goodness of fit of the models applied.
Viability of probiotic bacteria in impregnated-dried murta
Murta samples were impregnated with commercial apple juice at a pressure of 50, 150 and 300 mbar for 5, 10 and 15 min. Probiotic counts were measured at all stages, pre and post impregnation and pre and post drying. In general, at all impregnation conditions, murta was enriched with > 107 CFU/g L. casei (Table 4). These values represent less than 1 log cycle reduction in the initial L. casei content (108 CFU/g) from the impregnation solution and are similar to the reduction reported after vacuum impregnation of probiotics in several fruits and vegetables (Betoret et al. 2003; Oliveira et al. 2017; Noorbakhsh et al. 2013; Rêgo et al. 2013; Wirjantoro et al. 2015). The initial counts ranged from 1.46 ± 0.14 × 107 to 2.34 ± 0.06 × 107 CFU/g in vacuum dried samples and from 1.22 ± 0.09 × 107 to 2.53 ± 0.17 × 107 CFU/g in samples dried by convective drying (Table 4). The highest L. casei counts were quantified in murta impregnated at the highest pressure and longest pressurization time (150 mbar for 15 min). A similar effect of increasing from 5 to 15 min impregnation time was reported in guavas and papayas impregnated with L. casei (Krasaekoopt and Suthanwong 2008).
Table 4.
Effect of drying on L. casei viable counts from impregnated murta
| Pressure (mbar) | Time (min) | Impregnated murta × 107 (CFU/g) |
Impregnated dried murta × 106 (CFU/g) |
Log reduction |
|---|---|---|---|---|
| Vacuum drying | ||||
| 50 | 5 | 1.75 ± 0.10aA | 0.21 ± 0.15aA | 1.9 |
| 10 | 1.98 ± 0.08abA | 0.24 ± 0.25aB | 1.9 | |
| 15 | 2.22 ± 0.35bA | 0.65 ± 0.45bA | 1.5 | |
| 150 | 5 | 1.46 ± 0.14aB | 0.40 ± 0.80aA | 1.6 |
| 10 | 2.27 ± 0.03bB | 0.54 ± 0.44bB | 1.6 | |
| 15 | 2.34 ± 0.06bA | 0.66 ± 0.71bC | 1.5 | |
| 300 | 5 | 1.51 ± 0.04aB | 0.21 ± 0.85aA | 1.9 |
| 10 | 1.54 ± 0.03abC | 0.38 ± 0.65bA | 1.6 | |
| 15 | 1.60 ± 0.05bB | 0.34 ± 0.30bB | 1.7 | |
| Convective drying | ||||
| 50 | 5 | 1.32 ± 0.06aAB | 3.03 ± 0.21aA | 0.6 |
| 10 | 1.64 ± 0.09bA | 4.43 ± 0.50bA | 0.6 | |
| 15 | 2.53 ± 0.17Cb | 7.43 ± 0.47cB | 0.5 | |
| 150 | 5 | 1.22 ± 0.09aA | 2.80 ± 0.36aA | 0.6 |
| 10 | 2.04 ± 0.23bB | 4.30 ± 0.46bA | 0.7 | |
| 15 | 2.27 ± 0.21bA | 6.80 ± 0.20cB | 0.5 | |
| 300 | 5 | 1.45 ± 0.08aA | 8.67 ± 0.15aA | 1.2 |
| 10 | 1.47 ± 0.08aA | 2.70 ± 0.69bA | 0.7 | |
| 15 | 1.76 ± 0.13bB | 4.23 ± 0.59cB | 0.6 | |
Lowercase letters (a, b and c) show the effect of impregnation time at constant pressure
Uppercase letters (A, B and C) show the effect of impregnation pressure at constant time
Post-impregnation drying negatively affected L. casei counts. Viable counts decreased in 1.5–1.9 log after vacuum drying and in 0.5–1.2 after convective drying. Despite the decrease in microbial counts, murta samples dehydrated by convective drying retained 106 CFU/g L. casei, values that correspond to the average found in commercial probiotic dairy products (Betoret et al. 2003) while the 105 CFU/g L. casei obtained in vacuum dried murta are below the commercialized numbers from probiotic enriched foods. Most of the works aiming to obtain probiotic enriched dehydrated products have used vacuum impregnation followed by vacuum drying or convective (hot air) drying. However, convective drying should be carried out at temperatures below 40 °C to maintain viability of probiotic bacteria (Betoret et al. 2003; Cui et al. 2018; Rêgo et al. 2013; Rodrigues et al. 2018). Our results show that at the tested temperature (40 °C), convective drying is less deleterious to L. casei viability than vacuum drying while drying time does not differ greatly in both methods (Fig. 1). Marketed probiotics contain from 106 CFU/g, although studies have shown that health benefits are associated at a dosage of at least 109 CFU/g viable cells (Kligler and Cohrssen 2008) as microorganisms must be able to survive the passage through the digestive tract and be able to colonize and proliferate in the gut. Thus, vacuum impregnation at 150 mbar combined with convective drying appears as the best method to produce probiotic enriched murta.
Conclusion
The current consumers’ needs have led to the development of novel probiotic enriched foods with longer shelf life and increased nutritional attributes. A combination of an impregnation process followed by convective drying would produce murta berries impregnated with a high total count of viable cells of L. casei var. rhamnosus (106 CFU/g), although effective moisture diffusivity during convective drying was lower than during vacuum drying. Mathematical modeling of drying kinetics revealed the Weibull model as having the best fit among all selected models according to statistical tests applied. Our results showed that murta berries may be used as a carrier of probiotic bacteria, offering an alternative to consumers of non-dairy probiotic products, and impregnated dried murta berries may be considered as a novel functional food.
Acknowledgements
The authors gratefully acknowledge the financial support from the Department of Food Engineering and CONICY-PFCHA/National Doctorate/2016-21161718. This work was part of Liliana Zura-Bravo thesis, as a requisite to obtain the doctoral degree in Food Engineering and Bioprocesses at the University of La Serena, Chile.
Abbreviations
- MRc
Calculated moisture ratio
- r2
Determination coefficient
- t
Drying time (s)
- De
Effective moisture diffusivity (m2/s)
- n
Empirical parameters (dimensionless)
- Xe
Equilibrium moisture content (g water/100 g sample)
- MRe
Experimental moisture ratio
- P
Impregnation pressure (mbar)
- T
Impregnation time (min)
- Xo
Initial moisture content (g water/100 g sample)
- k
Kinetic parameter (1/min)
- r
Mean radius of the berry (m)
- Xt
Moisture content (g water/100 g sample)
- MR
Moisture ratio (dimensionless)
- z
Number of constants
- N
Number of data values
- i
Number of terms
- α
Shape parameter (dimensionless)
- β
Scale parameter (min)
- aw
Water activity (dimensionless)
References
- Alzamora SM, Salvatori D, Tapia MS, López-Malo A, Welti-Chanes J, Fito P. Novel functional foods from vegetable matrices impregnated with biologically active compounds. J Food Eng. 2005;67(1–2):205–214. doi: 10.1016/j.jfoodeng.2004.05.067. [DOI] [Google Scholar]
- Babalis SJ, Belessiotis VG. Influence of the drying conditions on the drying constants and moisture diffusivity during the thin-layer drying of figs. J Food Eng. 2004;65(3):449–458. doi: 10.1016/j.jfoodeng.2004.02.005. [DOI] [Google Scholar]
- Betoret N, Puente L, Díaz MJ, Pagán MJ, García MJ, Gras ML, Martínez-Monzó J, Fito P. Development of probiotic-enriched dried fruits by vacuum impregnation. J Food Eng. 2003;56(2–3):273–277. doi: 10.1016/S0260-8774(02)00268-6. [DOI] [Google Scholar]
- Betoret E, Betoret N, Arilla A, Bennár M, Barrera C, Codoñer P, Fito P. No invasive methodology to produce a probiotic low humid apple snack with potential effect against Helicobacter pylori. J Food Eng. 2012;110(2):289–293. doi: 10.1016/j.jfoodeng.2011.04.027. [DOI] [Google Scholar]
- Betoret E, Calabuig-Jiménez L, Patrignani F, Lanciotti R, Dalla Rosa M. Effect of high pressure processing and trehalose addition on functional properties of mandarin juice enriched with probiotic microorganisms. LWT Food Sci Technol. 2017;85:418–422. doi: 10.1016/j.lwt.2016.10.036. [DOI] [Google Scholar]
- Chaikham P, Apichartsrangkoon A, George T, Jirarattanarangsri W. Efficacy of polymer coating of probiotic beads suspended in pressurized and pasteurized longan juices on the exposure to simulated gastrointestinal environment. Int J Food Sci Nutr. 2013;64(7):862–869. doi: 10.3109/09637486.2013.799124. [DOI] [PubMed] [Google Scholar]
- Chen Z-G, Guo X-Y, Wu T. A novel dehydration technique for carrot slices implementing ultrasound and vacuum drying methods. Ultrason Sonochem. 2016;30:28–34. doi: 10.1016/j.ultsonch.2015.11.026. [DOI] [PubMed] [Google Scholar]
- Contreras C, Martín ME, Martínez-Navarrete N, Chiralt A. Effect of vacuum impregnation and microwave application on structural changes which occurred during air-drying of apple. LWT Food Sci Technol. 2005;38(5):471–477. doi: 10.1016/j.lwt.2004.07.017. [DOI] [Google Scholar]
- Corzo O, Bracho N. Application of Weibull distribution model to describe the vacuum pulse osmotic dehydration of sardine sheets. LWT Food Sci Technol. 2008;41(6):1108–1115. doi: 10.1016/j.lwt.2007.06.018. [DOI] [Google Scholar]
- Cui L, Niu L-Y, Li D-J, Liu C-Q, Liu Y-P, Liu C-J, Song J-F. Effects of different drying methods on quality, bacterial viability and storage stability of probiotic enriched apple snacks. J Integr Agric. 2018;17(1):247–255. doi: 10.1016/S2095-3119(17)61742-8. [DOI] [Google Scholar]
- Doymaz İ. Drying characteristics and kinetics of Okra. J Food Eng. 2005;69(3):275–279. doi: 10.1016/j.jfoodeng.2004.08.019. [DOI] [Google Scholar]
- Doymaz İ. Air-drying characteristics of tomatoes. J Food Eng. 2007;78(4):1291–1297. doi: 10.1016/j.jfoodeng.2005.12.047. [DOI] [Google Scholar]
- ICMSF . Microorganisms in foods 8: use of data for assessing process control and product acceptance. Berlin: Springer; 2011. [Google Scholar]
- Kligler B, Cohrssen A. Probiotics. Am Fam Phys. 2008;78(9):1073–1078. [PubMed] [Google Scholar]
- Krasaekoopt W, Suthanwong B. Vacuum impregnation of probiotics in fruit pieces and their survival during refrigerated storage. Kasetsart J (Nat Sci) 2008;42(4):723–731. [Google Scholar]
- Lemus-Mondaca R, Betoret N, Vega-Gálvez A, Lara-Aravena E. Dehydration characteristics of papaya (Carica pubenscens): Determination of equilibrium moisture content and difusion coefficient. J Food Process Eng. 2009;32(5):645–663. doi: 10.1111/j.1745-4530.2007.00236.x. [DOI] [Google Scholar]
- Mattila-Sandholm T, Myllärinen P, Crittenden R, Mogensen G, Fondén R, Saarela M. Technological challenges for future probiotic foods. Int Dairy J. 2002;12(2–3):173–182. doi: 10.1016/S0958-6946(01)00099-1. [DOI] [Google Scholar]
- Noorbakhsh R, Yaghmaee P, Durance T. Radiant energy under vacuum (REV) technology: a novel approach for producing probiotic enriched apple snacks. J Funct Foods. 2013;5(3):1049–1056. doi: 10.1016/j.jff.2013.02.011. [DOI] [Google Scholar]
- Oliveira PM, Ramos AM, Martins EMF, Vieira ÉNR, Soares AS, Noronha MC. Comparison of vacuum impregnation and soaking techniques for addition of Lactobacillus acidophilus to minimally processed melon. Int J Food Sci Technol. 2017;52(12):2547–2554. doi: 10.1111/ijfs.13540. [DOI] [Google Scholar]
- Orak HH, Aktas T, Yagar H, Isbilir SS, Ekinci N, Sahin FH. Antioxidant activity, some nutritional and colour properties of vacuum dried strawberry tree (Arbutus unedo L.) fruit. Acta Sci Pol Technol Aliment. 2011;10(3):327–338. doi: 10.1177/1082013211428213. [DOI] [PubMed] [Google Scholar]
- Poós T, Szabó V. Volumetric heat transfer coefficient in fluidized-bed dryers. Chem Eng Technol. 2018 [Google Scholar]
- Rascón MP, Huerta-Vera K, Pascual-Pineda LA, Contreras-Oliva A, Flores-Andrade E, Castillo-Morales M, Bonilla E, González-Morales I. Osmotic dehydration assisted impregnation of Lactobacillus rhamnosus in banana and effect of water activity on the storage stability of probiotic in the freeze-dried product. LWT Food Sci Technol. 2018;92:490–496. doi: 10.1016/j.lwt.2018.02.074. [DOI] [Google Scholar]
- Rêgo A, Freixo R, Silva J, Gibbs P, Morais A, Teixeira P. A functional dried fruit matrix incorporated with probiotic strains: Lactobacillus plantarum and Lactobacillus kefir. Focus Mod Food Ind FMFI. 2013;2(3):138–143. [Google Scholar]
- Rodrigues S, Silva LCA, Mulet A, Cárcel JA, Fernandes FAN. Development of dried probiotic apple cubes incorporated with Lactobacillus casei NRRL B-442. J Funct Foods. 2018;41:48–54. doi: 10.1016/j.jff.2017.12.042. [DOI] [Google Scholar]
- Ruiz A, Hermosín-Gutiérrez I, Mardones C, Vergara C, Herlitz E, Vega M, Dorau C, Winterhalter P, von Baer D. Polyphenols and antioxidant activity of calafate (Berberis microphylla) fruits and other native berries from southern Chile. J Agric Food Chem. 2010;58(10):6081–6089. doi: 10.1021/jf100173x. [DOI] [PubMed] [Google Scholar]
- Scheuermann E, Seguel I, Montenegro A, Bustos RO, Hormazábal E, Quiroz A. Evolution of aroma compounds of murtilla fruits (Ugni molinae Turcz) during storage. J Sci Food Agric. 2008;88(3):485–492. doi: 10.1002/jsfa.3111. [DOI] [Google Scholar]
- Seguel I, Montenegro A (2010) Murtilla plant named “Red Pearl-INIA”. Patent No. PP2, 273 P3, USA
- Simal S, Femenia A, Garau MC, Rosselló C. Use of exponential, Page’s and diffusional models to simulate the drying kinetics of kiwi fruit. J Food Eng. 2005;66(3):323–328. doi: 10.1016/j.jfoodeng.2004.03.025. [DOI] [Google Scholar]
- Toujani M, Hassini L, Azzouz S, Belghith A. Experimental study and mathematical modeling of silverside fish convective drying. J Food Process Preserv. 2012;37(5):930–938. doi: 10.1111/j.1745-4549.2012.00729.x. [DOI] [Google Scholar]
- Vega-Gálvez A, Lemus-Mondaca R, Bilbao-Sáinz C, Yagnam F, Rojas A. Mass transfer kinetics during convective drying of red pepper var. Hungarian (Capsicum annuum L.): mathematical modeling and evaluation of kinetic parameters. J Food Process Eng. 2008;31(1):120–137. doi: 10.1111/j.1745-4530.2007.00145.x. [DOI] [Google Scholar]
- Vega-Gálvez A, Puente-Diaz L, Lemus-Mondaca R, Miranda M, Torres MJ. Mathematical modeling of thin-layer drying kinetics of cape gooseberry (physalis peruviana l.) J Food Process Preserv. 2014;38:728–736. doi: 10.1111/jfpp.12024. [DOI] [Google Scholar]
- Weinbreck F, Bodnár I, Marco ML. Can encapsulation lengthen the shelf-life of probiotic bacteria in dry products? Int J Food Microbiol. 2010;136(3):364–367. doi: 10.1016/j.ijfoodmicro.2009.11.004. [DOI] [PubMed] [Google Scholar]
- Wirjantoro TI, Phianmongkhol A, Rongkom H. Lactobacillus enriched intermediate-moisture fruit products. CMU J Nat Sci. 2015;14(2):1–10. [Google Scholar]
- Wu L, Orikasa T, Ogawa Y, Tagawa A. Vacuum drying characteristics of eggplants. J Food Eng. 2007;83(3):422–429. doi: 10.1016/j.jfoodeng.2007.03.030. [DOI] [Google Scholar]
- Zhao Y, Xie J. Practical applications of vacuum impregnation in fruit and vegetable processing. Trends Food Sci Technol. 2004;15(9):434–451. doi: 10.1016/j.tifs.2004.01.008. [DOI] [Google Scholar]
- Zhu A, Shen X. The model and mass transfer characteristics of convection drying of peach slices. Int J Heat Mass Transf. 2014;72:345–351. doi: 10.1016/j.ijheatmasstransfer.2014.01.001. [DOI] [Google Scholar]