Survivorship pattern inaccuracies and inappropriate anthropomorphism in scholarly pursuits of killer whale ( Orcinus orca ) life history: a response to Franks et al. (2016)

Abstract

Franks et al. (2016) consider that the degree of error in estimated ages used to define survivorship patterns of northern and southern resident killer whale ( Orcinus orca ) populations is of insignificant impact to estimates of the species’ postreproductive lifespan (PRLS). We provide evidence that survival probabilities for killer whales using a dataset comprising estimated age animals differ significantly from that determined using data collected from known-age animals in the Pacific Northwest over the past 40 years. Consequently, our findings indicate that the degree of error in age estimates and ensuing survivorship patterns do not support the notion by Franks et al. (2016) of a prolonged PRLS in the female killer whale that is comparable to the PRLS observed in humans.

The topic that appears to be the foundation for the rebuttal letter from Franks et al. (2016), is our recent assertion ( Robeck et al. 2015 ) that “…reproductive and actuarial senescence is common in mammalian species… and it therefore should not be considered an unexpected finding in killer whales.” Franks et al. (2016) cite publications ( Foote 2008 ; Ward et al. 2009 ; Foster et al. 2012 ; Brent et al. 2015 ; Croft et al. 2015 ; Whitehead 2015 ), which have relied on data from animals of estimated age to support their contention that female killer whales are unique among mammals in having a prolonged postreproductive lifespan (PRLS) of up to 40 years, which verges upon that which can be found in humans. The estimated maximum longevity of 80–90 years used repeatedly in the literature (e.g., Olesiuk et al. 2005 ) and by Franks et al. (2016) clearly contrasts to our previously proposed female killer whale maximum longevity of 60–70 years ( Robeck et al. 2015 ). What is the reason for this discrepancy in proposed killer whale longevity and how may this discrepancy affect the duration of the PRLS of killer whales? We provide a review of the available evidence on female killer whale survivorship and clarify how using adults of estimated age has resulted in inaccurate longevity estimates and the impact of such estimates on the proposed duration of PRLS in the killer whale.

The evidence we present is derived from 3 areas of analysis: 1) inherent error in age estimations for NR and SR populations; 2) age-specific survival probabilities for Pacific Northwest resident killer whales; and 3) population demographics of NR and SR killer whales.

I nherent E rror in A ge E stimates

In our original article, we did not go into detail regarding the issue of error derived from age estimates of Pacific Northwest animals who were adults at the beginning of the photo-identification study in 1973. Instead we stated that “Given these odds and the population structure, it appears more likely that the estimated ages assigned to these animals (3 animals, W03, L25 and J02) at the start of the study period (1973— Bigg et al. 1990 ; Olesiuk et al. 1990 ) were inaccurate.” However, given the criticism of Franks et al. (2016) on our proposed maximum longevity, and their assertion that their analysis “..clearly demonstrates that the post-reproductive lifespan in resident killer whales is a substantial and significant life history stage and not simply an artifact due age estimation errors as proposed by Robeck et al.,” we feel that elaboration of methods by Olesiuk et al. (1990) , with which Franks et al. (2016) base their assertions on killer whale PRLS, along with other groups ( Cohen 2004 ; Foote 2008 ; Johnstone and Cant 2010 ; Foster et al. 2012 ; Croft et al. 2015 ) is thoroughly warranted. With respect to the use by Olesiuk et al. (1990) of a correction factor to adjust ages of females that may have had calves that died prior to observation, we would like to quote a previously published critique by Matkin et al. (2013) :

We found this method flawed because ….the correction factor also had extremely wide confidence limits, typically ranging from < 0 to > 20 yr, and failed to impart the actual effect of not observing the oldest offspring, pushing a small number of females into a much older age category rather than incrementing the ages of most older females by 1 – 3 yr. Eliminating the correction factor slightly decreases the age-specific reproductive and survival estimates in the older female categories but has negligible effect on classification of females into postreproductive age classes.

In other words, in the absence of applying the correlation factor used by Olesiuk et al. (1990) , postreproductive females are still classified as postreproductive, but they are not assigned inflated and unjustified birth dates. For additional evidence concerning the limitations of early aging methods, we can also look at available data for 2 of the oldest known females J02 and K07—both of whom would have been included in the probabilities used by the previously cited papers including Franks et al. (2016) to establish postreproductive representation values.

J02 is currently the most famous of these oldest living whales, as her birth date is listed by the Center for Whale Research ( CWR 2015 ) as 1911. In 2011, the assumptions used to assign J02’s (also known as “Granny”) birth data in 1971 were discussed in an interview with one of the coauthors of Franks et al. (2016; K. Balcomb, http://www.orcawatcher.com/2011/07/j2-granny-celebrating-100-years.html ):

One interesting thing Ken explained was how they arrived at 1911 as the estimated birth year for Granny, something I had always wondered about but a story I had never heard. I guess they had photos of both J1 Ruffles and J2 Granny in 1971 and both were already full grown adults. Since orcas reach full size around the age of 20, they made the estimated birth year for J1 Ruffles as 1951 (1971 - 20 years). Due to the way Granny and Ruffles associated with one another, they suspected that she might be Ruffles’ mother. Since Granny was never seen with a new calf since the study began, they assumed she was post-reproductive, and that perhaps Ruffles was her last calf. Females generally stop reproducing around the age of 40, so if she had Ruffles when she was 40, her birth year would be about 1911 (1951 - 40 years).

Despite the fact that this association was believed to be in error as early as 1987 ( Bigg et al. 1990 ) and it was genetically confirmed that they were not a mother–son pair ( Ford et al. 2011 ), the age originally assigned to J02 remains unchanged. In another account, J12 was assigned as a daughter of J02 in 1990 ( Bigg et al. 1990 ), however, more recently her dam is listed as unknown with a high degree of probability ( Ford et al. 2011 ). Regardless of the final genealogy, once the information which was used to define J02’s age had been disproven or put into question, her documented age should have been updated or flagged as unreliable, yet it continues to remain as it was assigned at the beginning of the research.

Another of the oldest well-documented females, K7 (born 1910), was aged based on a relationship, whereby K7 was believed to be the dam of K11 (estimated to be born 1933— Bigg et al. 1990 ). Recent DNA analysis of this relationship disproved this presumption ( Ford et al. 2011 ); however, despite this evidence, the assigned age for K7 has remained unchanged. It should be acknowledged that the photo-identification studies and ensuing publications by Olesiuk et al. (1990) and Bigg et al. (1990) are unquestionably seminal work on killer whale biology. Clearly though, some of the ages assigned to adult animals at the start of field studies were in error, and thus the accuracy of the estimates of life history traits is improved by the use of data from known-age animals.

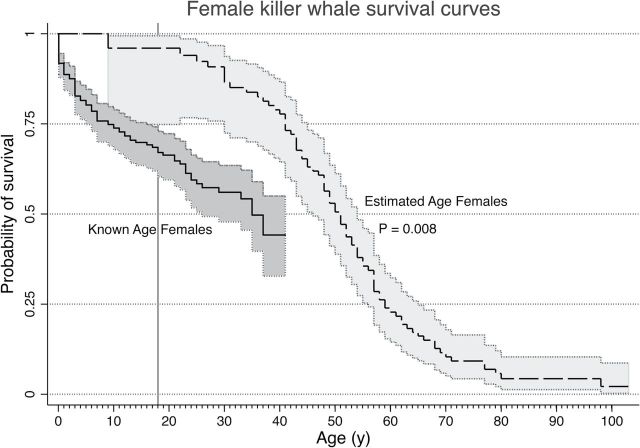
What other evidence do we have that indicates that there is a problem with the age estimates, and more importantly how this problem may affect PRLS estimates? Using methods previously described for Kaplan–Meier ( Kaplan and Meier, 1958 ) survivorship analysis of known-age animals ( Robeck et al. 2015 ), we determined the survivorship patterns of the known-age and then estimated age female killer whales from the northern and southern resident populations ( Supplementary Data ) and compared their survivorship curves using the log-rank test. Based on a log-rank test between survival curves, no significant differences ( P > 0.05) were detected in survivorship patterns between known-age SR and NR females or between estimated age NR and SR females, so we combined the populations (NSR) across the 2 groups (i.e., known-age and estimated age) for further analysis. For the combined population, overall survivorship patterns of known-age and estimated age animals were significantly different ( X² = 7.13, P = 0.008) with median survivorship estimated at 35.0±2.1 years (known-age females) and 51.0±1.8 years (estimated age females; Fig. 1 ). Although comparisons of survivorship at individual age points within each curve cannot be conducted using this analysis, the area of nonoverlapping 95% confidence interval ( CI ) indicates probable significant differences in survival patterns during these time periods. For the analysis depicted in Fig. 1 , the area of nonoverlapping 95% CI occurred from the 18-year mark and onward. We contend that the significant right shift of the Kaplan–Meier curve for animals of estimated age as compared to that of known age is compelling evidence of inaccurate (overestimated) age designation for the NSR population, for those animals identified as approximately 18 years of age and older in 1973.

Fig. 1.

Kaplan–Meier survival curves with their respective 95% CI of the proportion of known-age northern and southern resident female killer whales (NSR, solid line) or estimated age NSR (dashed line) alive over time (years) from 1 January 1975 to 1 January 2015. Significant differences ( X² = 7.13, P = 0.008) were detected between the overall survivorship rates of the 2 populations. Note the nonoverlapping CI s of the 2 populations from ~18 years of age (as identified by a vertical line), indicating probable significant differences in survival rates between known-age and estimated age females from this age and onward.

A ge-specific S urvival

In response to the age-specific survival probabilities we determined by relying on published ( Matkin et al. 2013 ) survival probabilities beyond age 50 for the southern Alaska resident (SAR), Franks et al. (2016) suggest that “…that there are either differences in the life histories of the Alaskan population compared to the Northern and Southern resident populations, or that differences in age estimates have come about due to differences in the length of observation of these populations (40 years for the Southern/Northern residents, 20 years plus 5 partial years for the Alaskan residents) and that future work is needed to unravel this.” The authors further state that “extrapolating between populations can be problematic.” We agree with the assertion of Franks et al. (2016) that extrapolating between ecotypes can be problematic; however, we are not aware of this principal being applied within similar ecotypes, such as the resident populations of the Pacific Northwest. We therefore used data from Matkin et al. (2013) on the SAR population to determine survivorship beyond age 50 for the following reasons: 1) it was the most recent publication on survival probabilities for resident killer whales of the North Pacific; 2) the population as a whole and for females have some of the highest (nonsignificantly) average life expectancy (ALE— Robeck et al. 2015 ) for any killer whale population; and 3) the age estimation methodology of adult members of the SAR population did not use the additional correction factors which we contend contribute to substantial error in survivorship estimates.

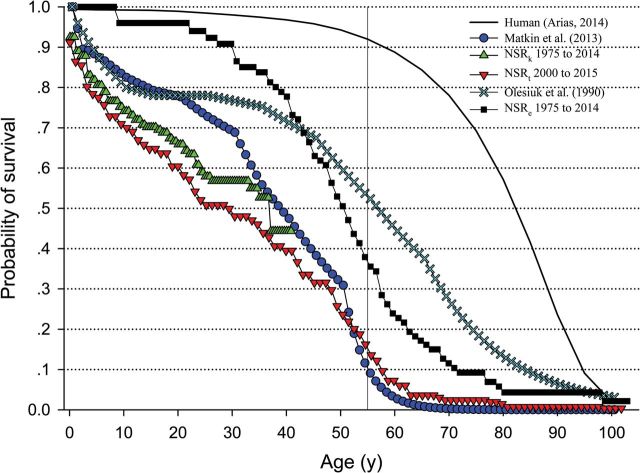
Since, as stated above, that values of ALE of the SAR are considered to be maximum across wild killer whale populations studied to date, the only differences we can appreciate (between the SAR and NSR populations) appear to be survival probabilities after age 50. Although data by Matkin et al. (2013) demonstrate the expected reduction in survival rates with age, Franks et al. (2016) use probabilities that imply no change in the survival probability with age beyond age 50. Franks et al. (2016) support this concept using probabilities to reach age 60.5 (0.245, or 1/4— Olesiuk et al. 2005 ) from data for wild killer whales only collected from 1975 until 1996 (20 years), ignoring the current data that extend to 2014. The authors then apply an annual survival rate (ASR) of 0.96 to yield new probabilities of female survival until age 80 (Franks et al. 2016; Table 1 ). Probabilities can easily be obtained using the Kaplan–Meier estimate; however, since Kaplan–Meier curves become flat when the numbers of animals become low and only 9 estimated age animals (currently living or dead) have ever reached beyond age 70, we applied the ASR of 0.93 that Franks et al. (2016) suggested they had used, and the new estimated probability of reaching the age milestones for NR and SR data is roughly twice those quoted by Franks et al. (2016; Table 1 ). Table 1 illustrates the difficulties encountered when working with data concerning the life history of a population that has been observed for less than 1 maximum lifespan, and the powerful effect that a flat ASR has on survival rates. Table 1 also highlights the vastly different probabilities for humans reaching these same age milestones and demonstrates that the ASR decreases at a rapid rate from 0.976 at age 70 to 0.938 at age 80 and to 0.89 at age 85 as humans reach the ALE (81 years) and beyond ( Arias 2014 ). This trend is similar to what Matkin et al. (2013) proposed for the SAR females once they reached their ALE of 49 years, and is in stark contrast to the flat ASR in older aged animals as proposed by Olesiuk et al. (1990 , 2005 ) and Franks et al. (2016). In addition, clear similarities can be observed between survival probabilities by Matkin et al. (2013) with those which we obtained from known-age animals ( Robeck et al. 2015 ), and with the new Kaplan–Meier analysis provided in Fig. 2 of all animals (estimated + known age) from 2000 to 2014. Finally, the dissimilarity between these survival curves and the survival curves produced by Olesiuk et al. (1990 ; used by Foote 2008 ; Johnstone and Cant 2010 ; Croft et al. 2015 ) and modern man ( Arias 2014 ) are also quite clear ( Fig. 2 ). What then is the correct ALE and survival probabilities for the SR and NR killer whales? We believe that combined evidence supports our hypothesis that values of these parameters are closer to those which were published by Matkin et al. (2013) , and since the issue at hand is based on survival probabilities after age 50 and the fact that the longest followed known-age females are in their early 40s, the question of the true ALE will be answered in the next 15 years and not “..up to another 60 years” as proposed by Franks et al. (2016).

Table 1.

Comparisons of the probabilities (Prob.) of females reaching age milestones based on the annual probability of survival. ASR = annual survival rate; NR = northern resident killer whales; SR = southern resident killer whales.

Age	Robeck et al. (2015) a		Olesiuk et al. (2005) b		Franks et al. (2016)		SR and NR Prob. c		Humans ( Arias 2014)
	ASR	Prob.	ASR	Prob.	ASR	Prob.	ASR	Prob.	ASR	Prob.
60	0.783	1/37	0.938	1/4	0.9603	1/4	0.93	1/7	0.990	1/1.1
65	0.783	1/127	0.938	1/6	0.9603	1/5	0.93	1/10	0.985	1/1.2
70	0.783	1/431	0.938	1/8	0.9603	1/6	0.93	1/14	0.976	1/1.3
75	0.783	1/1,465	0.938	1/11	0.9603	1/8	0.93	1/20	0.962	1/1.5
80	0.783	1/5,000	0.938	1/15	0.9603	1/9	0.93	1/28	0.938	1/1.9
85	0.783	1/17,000	0.938	1/20	0.9603	1/11	0.93	1/41	0.893	1/2.7
90	0.783	1/57,000	0.938	1/28	0.9603	1/14	0.93	1/58	0.826	1/5.1

^a Survival probabilities are from Matkin et al. (2013) for the southern Alaska resident killer whales.

^b Olesiuk et al. (2005).

^c Probabilities until age 60 from Kaplan–Meier survival analysis of combined known-age and estimated age northern and southern (NSR) resident females from 1 January 1975 to 1 January 2014 (NR) and 1 January 2015 (SR). Data after age 60 use a flat annual survival rate of 0.93 as proposed by Franks et al. (2016).

Fig. 2.

Kaplan–Meier survival curves for known-age northern resident (NR) and southern resident (SR) killer whales (▲, NSR _k ) from 1 January 1975 to 1 January 2014, estimated and known-age NR and SR killer whales (▼, NSR _t ) from 1 January 2000 to 1 January 2014, and estimated age NR and SR killer whales from 1 January 1975 to 1 January 2014 (□, NSR _e ). Survival curves created from published probabilities for the female southern Alaskan resident killer whales (□— Matkin et al. 2013 ), female NR and SR killer whales (NSR) from 1973 to 1987 (— Olesiuk et al. 1990 ), and United States human females in 2010 (black line— Arias 2014 ). Data from Olesiuk et al. (1990) were recently used to compare PRLS between humans and killer whales by Foote (2008) , Johnston and Cant (2010) , and Croft et al. (2015) . Note that the vertical line at 55 years (i.e., 1 interbirth interval plus 2 SD s from the mean age at last parturition— Cohen 2004 ) represents the age at which greater than 5% of the female killer whale population must survive in order to indicate significant PRLS for the species.

P opulation D emographics

In our paper ( Robeck et al. 2015 ), we provide clear evidence based on population structure that few animals (~3%) of estimated age males and females are alive beyond age 50 ( Robeck et al. 2015 ). However, Franks et al. (2016) contend that a significant number of females are living beyond age 70 giving support for an “extraordinarily long” PRLS in killer whales. As mentioned above, if the biology of these animals did indeed lend itself toward a proportion of super-aged females that lived twice the reproductive lifespan for the species, and whose mortality rates did not change after age 50, we should expect to see a predictable number of animals within each age group. Based on the probabilities presented by Franks et al. (2016) and the total population numbers of females in 2014/2015 (215), the predicted number of females (and actual number of estimated age females within parentheses) that should be present in each age class (for calculations see Supplementary Data ) is as follows: > 50 years, 38 (8); > 60 years, 22 (5); > 70 years, 13 (3); and > 80 years, 7 (2). Looking at the actual numbers of females, it is clear that the predicted number of older animals simply does not exist.

According to Croft et al. (2015) , the trait of PRLS is considered as a rare occurrence for species where “fewer than 5% of adult females survive to a post-fertile age.” In addition, Cohen (2004) states that “PRLS is significant only if it exceeds one inter birth interval plus two standard deviations” (the 95% CI for the mean). For the NSR killer whale population, the upper limit for the 95% CI of a birthing interval is 10 years ( Robeck et al. 2015 ). Since the updated data [updated to reflect recent birth data since the Robeck et al. (2015) publication] from the NSR population indicate that 20% of known-age females at age 38 have reproduced, 12% at age 39, 10% at age 42 (the current maximum age for known-age females), a value of 45 years is generously proposed ( Olesiuk et al. 2005 state no females reproduce after an estimated age of 46) as the age cutoff beyond which 95% of the females still alive will not reproduce. It therefore follows that the age at which > 5% of the female killer whale population must exceed to enable the species to be considered as having a significant PRLS is 55 years.

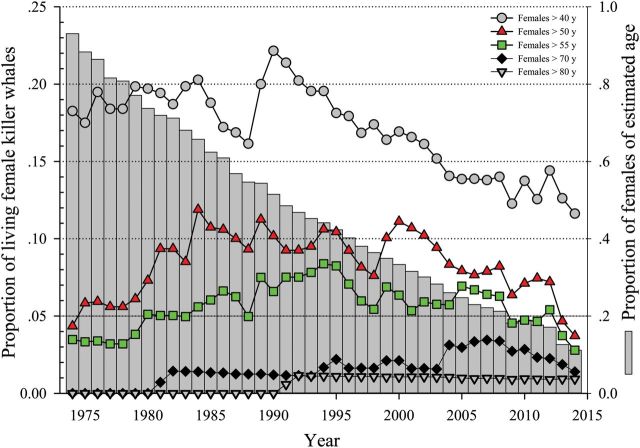
Across the entire study period, the mean annual percentage of NSR females (known and estimated age) over age 55 is 5.6%, which is just above the 5% cutoff cited by Croft et al. (2015) . Even when ignoring the inherent error in estimating the age of animals in the study, these results would be difficult to classify as “strong evidence” of resident killer whales exhibiting a substantial PRLS, and is far from comparable to that determined for human populations where 80% of adult females live 20–30 years beyond reproductive cessation ( Cohen 2004 ). Lack of a significant, prolonged PRLS for resident killer whales is also strongly indicated by other demographic data, namely by trends of the percentages of females within the older age classes over time. For example, for the > 40-year age class, the data clearly show that after a peak of 22% of the female population being present in this group in 1990 (during which 51% of the total animals were estimated age animals) a steady decline in this proportion has occurred running approximately in parallel to the decline in the percentage of estimated age animals remaining in the population ( Fig. 3 ). This trend was occurring despite continual population growth in the NR killer whales through the latest census ( Towers et al. 2015 ). Although increased mortality was observed in 1996–2004, believed to be due to naturally occurring cyclic changes ( Beamish and Mahnken 2001 ; Beamish et al. 2009 ) in the available food supplies ( Ford et al. 2009 ), these mortalities were spread equally across all age groups ( Olesiuk et al. 2005 ) and therefore should not have affected the percentage observed within each age group.

Fig. 3.

The population age structure for free-ranging killer whales ( Orcinus orca ) of the eastern North Pacific (NSR, northern and southern resident population from 1975 to 2014) for all females as reported by October 2015 ( Center for Whale Research 2015 ; Towers et al. 2015 ). The vertical bars represent the proportion of animals within the population that are of estimated age. Note that the horizontal line at 5% of the total females living denotes the threshold for which any values less than this reflect the absence of a significant prolonged PRLS for the species (i.e., for females > 55 years).

This is an additional evidence of the overestimation of animal ages, and therefore the most accurate estimate of killer whale population demographics should be the population during the most recent block of time which by default contains the least possible number of estimated age animals. If we look at the last 5 years, 13% of living females are > 40 years, 6% are > 50 years, and only 4% are > 55 years ( Fig. 3 ). More to the point, while the proportion of females above age 40 has continued to fall between 11% and 14% in the last decade (as opposed to the 37% predicted by probabilities created in 1990 [ Olesiuk et al. 1990 ] and used by Foote [2008] , Croft et al. [2015] , and Franks et al. [2016]), the percentage of animals exceeding 55 years has continued to decrease to the current percentage of 2.7%, which falls well below the 5% cutoff for a prolonged PRLS to be considered of significance ( Fig. 3 ).

Franks et al. (2016) and Croft et al. (2015) calculate the postreproductive representation value (PrR, based on a formula by Levitis and Bingham 2011 ) for killer whales. This is a modification of the method used by Cohen (2004) and using our results and the 5% threshold ( Croft et al. 2015 ), we find a PrR value of 0.15 that is similar to the PrR 0.157 that Franks et al. (2016) found for killer whales with a maximum age of 50 years. While according to Franks et al. (2016), this still indicates a significant ( P = 0.038) PRLS for killer whales and is worthy of discussion, it is less than convincing in terms of comparisons toward humans (PrR: 0.315–0.760) and does not far exceed the PrR value of 0.128 proposed for the Asian elephant ( Croft et al. 2015 ). Interestingly, neither Franks et al. (2016) nor Croft et al. (2015) attempt to put their results in context toward other nonprimate (except for elephant and pilot whale) mammalian taxa despite Cohen (2004) pointing to a large number of species (beagles, cattle, red deer, polar bear, horses, rabbits, and cats) that could have significant PRLS. For a quick example, brown bears ( Ursus arctos ) produce litters starting at 4 years, are reproductively senescent by 29 years, and can live to at least 34 years ( Schwartz et al. 2003 )—this gives a PrR estimate of 0.214. Clearly, more monitoring of known-age females is required to accurately place killer whales within the continuum of mammalian PRLS and before a statement similar to the one made by Franks et al. (2016) “the evidence that resident killer whales exhibit a post-reproductive lifespan approaching that of humans is overwhelming…” can be justified.

M enopause or R eproductively S enescent?

Franks et al. (2016) claim they do not want to get into a debate about the use of the term menopause, but then dedicate a considerable amount of text doing just that. Although the use of the term menopause is a convenient tool for anthropomorphisms commonly documented in the popular press, its lack of occurrence in the vast majority of species (i.e., in that only a small number of species, primarily primates, actually menstruate) ultimately creates confusion and is inappropriate in scholarly pursuits. Franks et al. (2016) cite 3 references to support their supposition of recent broad use of the term. One of the references ( Brent et al. 2015 ) was published by their own research group and therefore cannot be considered as valid evidence to support the broad use of menopause in nonhuman species. Another reference, by Cohen (2004) , uses a restricted definition of menopause:

Menopause is a term attached to a primarily human trait, so trying to identify in a comparative analysis which species do or do not have menopause would result more in a linguistic morass than a scientific analysis.

Cohen (2004) then goes on to state that “it is important to indicate that PRLS, not menopause, is the appropriate parameter for study.” As reproductive physiologists (Robeck and O’Brien), we are concerned with the biology behind reproductive senescence (see O’Brien and Robeck 2012 ), and consider it inappropriate to apply the term menopause toward species without menses (the vast majority of mammals). In fact, there already exists an adequate and universally accepted term for describing the cessation of reproduction in nonmenstruating mammals—which is “reproductively senescent.” A quick review of the literature will find thousands of scientific papers using this terminology. Why then change it now? It does make for easier headlines, similar to the use of the scientifically invalid “male menopause” a term which has only gained any traction in the popular press. However, for scientific endeavors, we strongly feel the use of the well-established term “reproductively senescent” to be appropriate.

S upporting I nformation

The Supporting Information documents are linked to this manuscript and are available at Journal of Mammalogy online ( Supplementary Data ). The materials consist of data provided by the author that are published to benefit the reader. The posted materials are not copyedited. The contents of all supporting data are the sole responsibility of the authors. Questions or messages regarding errors should be addressed to the author.

Supplementary Data .—Composition of the total population of northern resident (NR) and southern resident (SR) killer whales ( Orcinus orca ) of the eastern North Pacific, as of 1 January 2015. Only animals born during or after 1972 and that were alive on 1 January 1975 were included in reproductive or survivorship data analyses. For living animals, the date last observed alive is designated as 1 January in the year they were last sighted. For deceased animals, the date of death was assigned as 1 January in the 1st year of which they were not observed.

Supplementary Data .—Illustrates the calculations, based on the survival probabilities proposed by Franks et al. (2016), used to determine the predicted number of animals, which should be present within each age group in the current NSR population.