Figure - PMC

Skip to main content

An official website of the United States government

Here's how you know

Here's how you know

Official websites use .gov
A .gov website belongs to an official government organization in the United States.

Secure .gov websites use HTTPS
A lock ( ) or https:// means you've safely connected to the .gov website. Share sensitive information only on official, secure websites.

View full-text article in PMC

. 2019 Feb 18;5(2):e01181. doi: 10.1016/j.heliyon.2019.e01181

Search in PMC
Search in PubMed
View in NLM Catalog
Add to search

© 2019 The Authors

This is an open access article under the CC BY-NC-ND license (http://creativecommons.org/licenses/by-nc-nd/4.0/).

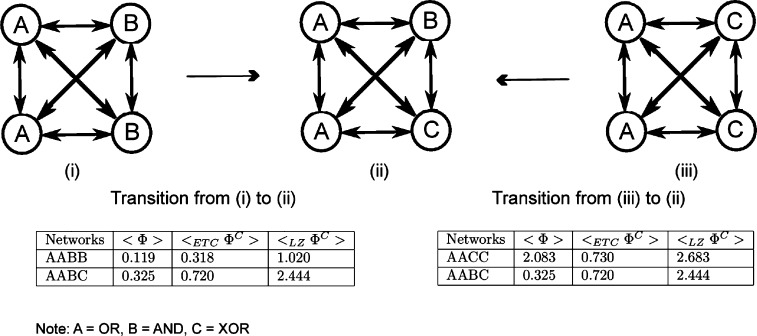
PMC Copyright notice

Interplay between differentiation, integration and entropy. (i) AABB has <Φ > =0.119, <_ETCΦ^C > =0.318, <_LZΦ^C > =1.020, (ii) AABC has <Φ > =0.325, <_ETCΦ^C > =0.720, <_LZΦ^C > =2.444, (iii) AACC has <Φ > =2.083, <_LZΦ^C > =0.730, <_ETCΦ^C > =2.683. The complexity of the simulated network AABB is lower than that of AABC, which is lesser than the complexity of AACC. This may seem counter-intuitive, but it is not, since the entropy of C (XOR gate) is higher than the entropies of both B (AND gate) and A (OR) gate. Thus, heterogeneity alone is insufficient to increase the value of integrated information of the network; the entropy of the individual nodes and their number in the network also matter.