A comparison of species specific sensitivities to changing light and carbonate chemistry in calcifying marine phytoplankton

Abstract

Coccolithophores are unicellular marine phytoplankton and important contributors to global carbon cycling. Most work on coccolithophore sensitivity to climate change has been on the small, abundant bloom-forming species Emiliania huxleyi and Gephyrocapsa oceanica. However, large coccolithophore species can be major contributors to coccolithophore community production even in low abundances. Here we fit an analytical equation, accounting for simultaneous changes in CO₂ and light intensity, to rates of photosynthesis, calcification and growth in Scyphosphaera apsteinii. Comparison of responses to G. oceanica and E. huxleyi revealed S. apsteinii is a low-light adapted species and, in contrast, becomes more sensitive to changing environmental conditions when exposed to unfavourable CO₂ or light. Additionally, all three species decreased their light requirement for optimal growth as CO₂ levels increased. Our analysis suggests that this is driven by a drop in maximum rates and, in G. oceanica, increased substrate uptake efficiency. Increasing light intensity resulted in a higher proportion of muroliths (plate-shaped) to lopadoliths (vase shaped) and liths became richer in calcium carbonate as calcification rates increased. Light and CO₂ driven changes in response sensitivity and maximum rates are likely to considerably alter coccolithophore community structure and productivity under future climate conditions.

Introduction

Coccolithophores are a group of phytoplankton (division Haptophyta, class Prymnesiophyceae), which produce both particulate organic carbon through photosynthesis and particulate inorganic carbon through calcification^1,2. They are found in almost all ocean ecosystems from the equator to sub-polar latitudes, from nutrient rich upwelling zones to nutrient poor oligotrophic regions and from surface waters to over 100 m in depth^3–7. Within ocean ecosystems coccolithophores play an important role in the production and cycling of carbon contributing up to 20% to total organic carbon fixation^8,9 and up to 50% of calcium carbonate flux to marine sediments^10–12. Because of this, coccolithophores have become amongst the most intensively studied organisms in terms of the effects of ocean acidification in particular and climate change in general (e.g.^13–16). Recent work, suggests that coccolithophores have a common over-arching response to changes in carbonate chemistry^16–18. However, these responses vary between species, strains and additionally can be modulated by changes in light intensity and temperature^16,19,20.

Of approximately 200 extant species of coccolithophores (e.g.²¹), the majority of research into coccolithophore responses to climate change come from only a few species such as Emiliania huxleyi, Gephyrocapsa oceanica, Calcidiscus leptoporus, and Coccolithus pelagicus spp. braarudii. Of these most studies have been on E. huxleyi which is considered a keystone species due to its ability to form massive blooms²², its dominance within the coccolithophore assemblage^3,6,23, and its almost global distribution³ (North and South Atlantic),²² (North Atlantic),⁶ (Equatorial and sub-equatorial Pacific),²⁴ (East Mediterranean),^25,26 (Southern Ocean),²⁷ (North Sea). While potentially dominant in terms of abundance E. huxleyi s contribution to both the organic and inorganic carbon cycle is likely less of importance. Among the coccolithophores E. huxleyi is quite small (cell size ∼5 μm) and has a fairly low cellular calcium carbonate (CaCO₃) content (2.88 to 12.12 pg C cell⁻¹)^16,28–30 (Fig. 1). Meanwhile larger and less abundant species such as C. pelagicus (∼16 μm), C. leptoporus (∼13.8 μm), Helicosphaera carteri or Scyphosphaera apsteinii (∼17.6 μm) produce more than 20 times this amount^29,31,32. Recent research suggests that these larger and more CaCO₃ rich species may be the dominant contributors to carbon production and export in the coccolithophore assemblage^{10,29,33–35}. Despite this, little is known about the effects of climate change on these larger species. As such the aim of this study was to examine the effects of changing carbonate chemistry and light intensity on the large coccolithophore S. apsteinii.

Figure 1.

Scanning electron microscope images of a E. huxleyi, S. apsteinii and a Coccolithus species showcasing size and cellular calcium carbonate quota differences between species. Species shown were either isolated or cultured by the authors.

Results

Growth of S. apsteinii cultures was observed between starting conditions of 50–5000 μatm, but not at 7000 μatm. The fit to combined light and carbonate chemistry conditions (Eq. 2) explained up to 75% of the variability in measured response rates across a range of carbonate chemistry (47–2570 μatm) and light (50–515 μmol photons m⁻² s⁻¹) conditions (Table 1). The equation was able to capture the limiting, stimulating and inhibiting effects of changing carbonate chemistry (Fig. 2) and light intensity (Fig. 3) on all physiological rates.

Table 1.

Fit coefficients (k₁, k₂, k₃, k₄, k₅, k₆), R², p-values, F-values and degrees of freedom obtained from fit equation (2) for calcification (pg C cell⁻¹ d⁻¹), photosynthetic carbon fixation (pg C cell⁻¹ d⁻¹) and growth rate (d⁻¹) fits to all data. Note that for calcification and photosynthetic carbon fixation rates the unit of k₁ is pg C cell⁻¹ day⁻¹ and for growth rates day⁻¹.

	Calcification	Photosynthesis	Growth rate
k1 (pg C cell−1 day−1 or day−1)	5.77E + 13	−1.18E + 05	−7.57E + 04
k2 (μmol photons m−2 s−1)	2.56E + 18	−1.79E + 10	−1.97E + 12
k3 (kg mol−1 μmol photons m−2 s−1)	−1.00E + 03	6.21E + 12	4.78E + 14
k4 (mol kg−1)	2.29E + 09	−2.81	−569.84
k5 (dimensionless)	−1.15E + 12	1.35E + 03	2.12E + 05
k6 (kg mol−1 μmol photons−1 m2 s)	7.95E + 16	−1.09E + 08	−2.69E + 10
R2	0.7501	0.7012	0.5727
(p-value)	(4.43E-12)	(3.00E-11)	(2.41E-8)
F-value	105.05	86.91	49.74
Degrees of freedom	35	37	37

Figure 2.

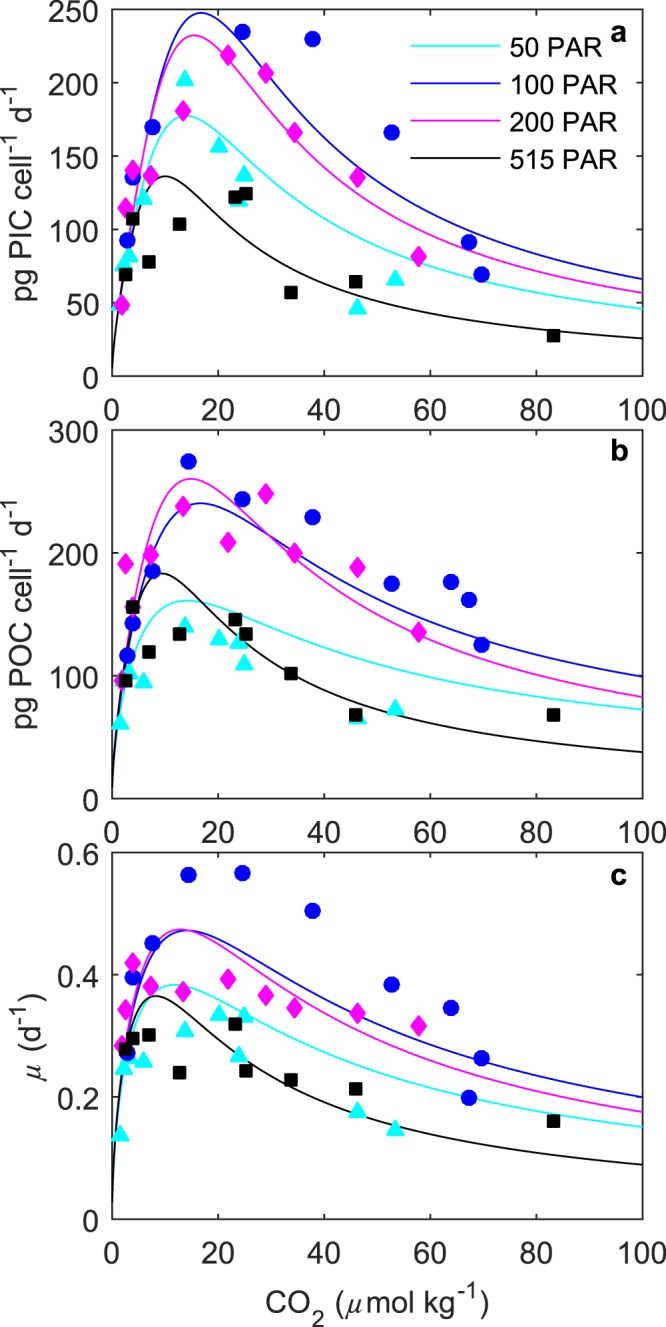
Fitted (solid lines) and measured (symbols) (a) particulate inorganic carbon (PIC), (b) particulate organic carbon (POC) production, and (c) growth rates in response to changes in CO₂ concentration at six different light intensities using equation 2 and fit coefficients from Table 1. Symbols represent rate measurements at a constant temperature (20 °C) and 50 (Δ), 100 (◯), 200 (◊) and 515 (□) μmol photons m⁻² s⁻¹.

Figure 3.

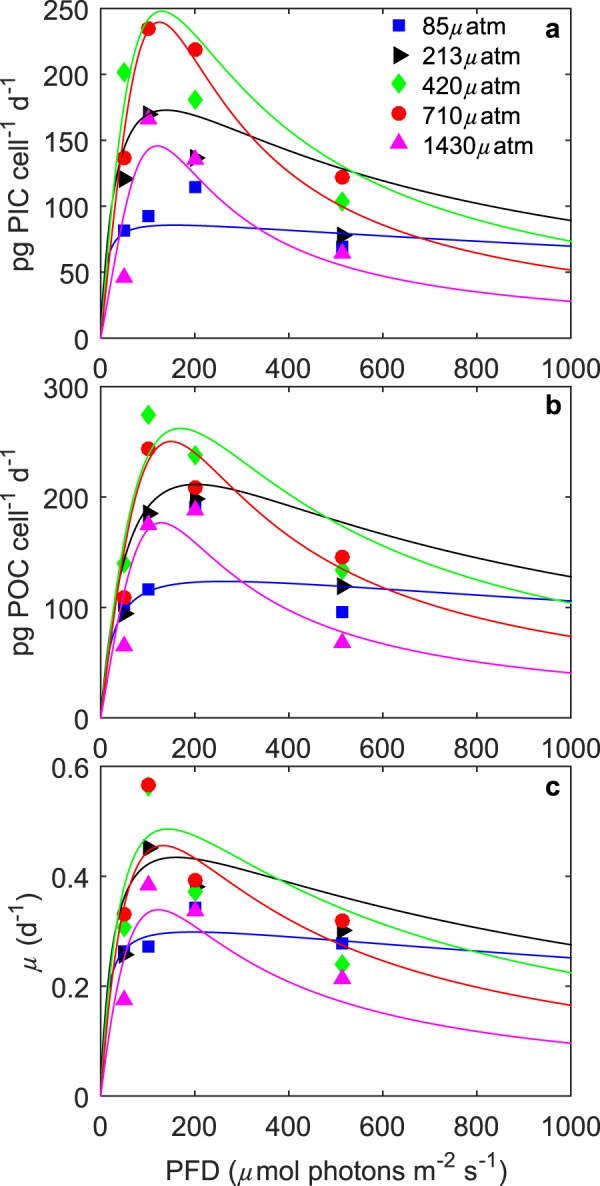
(a) Particulate inorganic carbon (PIC) and (b) particulate organic carbon (POC) production, and (c) growth rates of S. apsteinii in response to changes in light intensity at five different f CO₂ levels at a constant temperature (20 °C). Symbols denote measured data at f CO₂ levels of ∼85 (□), ∼213 (⊳), ∼420 (◊), and ∼710 (◯) and ∼1430 (Δ) μatm and lines represent calculated rates using equation 2 and fit coefficients in Table 1.

Responses to changing carbonate chemistry

Growth, photosynthetic carbon fixation and calcification rates all had an optimum curve response to increasing CO₂ (Fig. 2, Table S1) at all light intensities. Depending upon CO₂ level and light intensity, rates varied between 28–234 pg C cell⁻¹ d⁻¹ for calcification, 60–243 pg C cell⁻¹ d⁻¹ for photosynthesis and 0.14–0.57 d⁻¹ for growth (Table S1). At all light intensities, particulate inorganic to organic carbon ratios PIC:POC ratios increased with CO₂ to an optimal point before declining with further increases in CO₂ (Table S1). PIC:POC ratios varied from 0.42 to 1.44 depending upon CO₂ level and light intensity (Table S1).

Calcification, photosynthetic carbon fixation and growth rates had similar CO₂ requirements to achieve half saturation $\left(\mathrm{K}\frac{1}{2}{}_{{\mathrm{CO}}_2}\mathrm{s}\mathrm{at}\right)$ and optimal rates (Table 2). Calcification rates were more sensitive to increasing [H⁺] $\left(\mathrm{K}\frac{1}{2}{}_{{\mathrm{CO}}_2}\mathrm{i}\mathrm{nhib}\right)$ than photosynthetic carbon fixation or growth rates (Table 2, Fig. 2). Optimum CO₂ and CO₂ half-saturation concentrations $\left(\mathrm{K}\frac{1}{2}{}_{{\mathrm{CO}}_2}\mathrm{s}\mathrm{at}\right)$ changed little (in comparison to the overall CO₂ response range and uncertainties in the model fit) with increasing light (Table 2). CO₂ half inhibition concentrations $\left(\mathrm{K}\frac{1}{2}{}_{{\mathrm{CO}}_2}\mathrm{i}\mathrm{nhib}\right)$ changed little with increasing light for calcification, followed a slight optimum curve response for growth, and decreased with increasing light for photosynthesis (Table 2). Differences in sensitivity to [H⁺] between the three rates decreased with increasing light intensity.

Table 2.

Optimum CO₂ concentrations, ${\mathrm{CO}}_2\mathrm{K}\frac{1}{2}$ concentrations and maximum rates (V_max) of S. apsteinii from fit equation (2) at 50–515 μmol photons m⁻² s⁻¹ and 20 °C using fit coefficients from Table 1.

CO2	50 PAR	100 PAR	200 PAR	515 PAR
CO 2 optima ( μ mol kg −1 )
Calcification	13.9	16.8	15.5	10.0
Photosynthesis	14.2	16.7	14.8	9.4
Growth rate	11.8	14.1	12.9	8.2
V max (pg C cell −1 d −1 or d −1 )
Calcification	177.4	247.6	232.3	136.2
Photosynthesis	161.1	240.3	260.2	183.2
Growth rate	0.38	0.47	0.47	0.37
$\mathbf{K}\frac{1}{2}{}_{\mathbf{C}{\mathbf{O}}_2}\mathbf{i}\mathbf{nhib}$ ( μ mol kg −1 )
Calcification	49.9	53.8	49.7	36.7
Photosynthesis	84.1	77.2	59.5	38.3
Growth rate	71.3	79.4	68.0	42.6
$\mathbf{K}\frac{1}{2}{}_{\mathbf{C}{\mathbf{O}}_2}\mathbf{s}\mathbf{at}$ ( μ mol kg −1 )
Calcification	3.2	4.5	4.1	2.2
Photosynthesis	1.9	3.0	3.0	1.8
Growth rate	1.4	1.8	1.8	1.1

Responses to changing light intensity

Maximum rates for growth, calcification and photosynthetic carbon fixation were observed to increase up to an optimum light intensity before declining with further increases in light (Fig. 3). Calcification rates increased 39% between 50 and 100 μmol photons m⁻² s⁻¹ before declining by 45% at 515 μmol photons m⁻² s⁻¹. Photosynthetic carbon fixation rates increased 62% between 50 and 200 μmol photons m⁻² s⁻¹ and declined by 30% at 515 μmol photons m⁻² s⁻¹. Growth rates increased 24% between 50 and 200 μmol photons m⁻² s⁻¹ and declined by 23% at 515 μmol photons m⁻² s⁻¹ (Table 2). The effect of carbonate chemistry (i.e. the combined change in concentrations of CO₂, ${\mathrm{HCO}}_3^{-}$, ${\mathrm{CO}}_3^{2-}$ and H⁺ as a result of rising f CO₂) on metabolic rates was greatest between 100–400 μmol photons m⁻² s⁻¹ and decreased towards more extreme light intensities (Fig. 3).

Increasing f CO₂ resulted in an increase in light half-saturation intensities $\left(\mathrm{K}\frac{1}{2}{}_{\mathrm{PAR}}\mathrm{s}\mathrm{at}\right)$, a decrease in optimal light requirements, and an increase in sensitivity to high light $\left(\mathrm{K}\frac{1}{2}{}_{\mathrm{PAR}}\mathrm{i}\mathrm{nhib}\right)$ for all rates (Table 3). Calcification reached optimal rates at lower light intensities (120–157 μmol photons m⁻² s⁻¹) than growth (122–196 μmol photons m⁻² s⁻¹) or photosynthesis (128–263 μmol photons m⁻² s⁻¹), though differences between the rates decreased with increasing f CO₂ (Table 3). Inhibiting light levels were lowest for calcification (385–1050 μmol photons m⁻² s⁻¹), higher for photosynthesis (447–1346 μmol photons m⁻² s⁻¹) and highest for growth (521–1524 μmol photons m⁻² s⁻¹).

Table 3.

Calculated optimum light intensities, maximum rates (V_max) and light $\mathrm{K}\frac{1}{2}$ values of S. apsteinii at 20 °C and 85, 213, 420, 710 and 1430 μatm using equation 2 and fit coefficients from Table 1.

Light	85 μatm	213 μatm	420 μatm	710 μatm	1430 μatm
Optima ( μ mol photons m − 2 s − 1 )
Calcification	157	139	130	125	120
Photosynthesis	263	203	169	149	129
Growth rate	196	161	143	133	123
V max (pg C cell −1 d −1 or d −1 )
Calcification	85.6	172.9	247.9	239.6	145.7
Photosynthesis	123.6	211.4	262.1	250.3	176.6
Growth rate	0.30	0.43	0.49	0.46	0.34
$\mathbf{K}\frac{1}{2}{}_{\mathbf{PAR}}\mathbf{i}\mathbf{nhib}$ ( μ mol photons m − 2 s − 1 )
Calcification	>2500	1050	548	423	386
Photosynthesis	>2500	1346	754	561	448
Growth rate	>2500	1524	894	667	521
$\mathbf{K}\frac{1}{2}{}_{\mathbf{PAR}}\mathbf{s}\mathbf{at}$ ( μ mol photons m − 2 s − 1 )
Calcification	7.2	18.3	30.6	36.8	37.4
Photosynthesis	18.4	30.6	38.0	39.7	37.1
Growth rate	9.9	17.0	22.8	26.4	28.8

Coccolith morphology

Between the different light and CO₂ treatments, coccosphere size ranged from 12.0–22.4 μm (average 17.2, Table S2). The number of muroliths per cell varied between five and 23 (average 12.4), while the number of lopadoliths per cell varied between zero and six (average 2.3). The ratio of muroliths to lopadoliths on a cell varied from zero to 22 (average 6.4). The length of muroliths was 6.1–10.9 μm (average 8.3) while the width was 4.0–9.1 μm (average 6.1). The length of lopadoliths varied between 2.8–13.9 μm (average 8.1) and the width 6.1–16.3 μm (average 10.5). The ratio of muroliths to lopadoliths was observed to increase with increasing light at 100 (n = 479 df = 2, Chi-sq = 14.34, p = 7.69E-4) and 400 μatm CO₂ (n = 357 df = 2, Chi-sq = 11.44, p = 3.28E-3). There was also a significant effect of calcification rate on PIC per lith, with liths containing more PIC in treatments with higher calcification rates (n = 8 R² = 0.80, F = 23.45 p = 2.87E-3, Fig. 4). Other than this, no environmentally significant patterns were observed in any morphological features with changing CO₂ or light (see supplementary information for discussion on these results).

Figure 4.

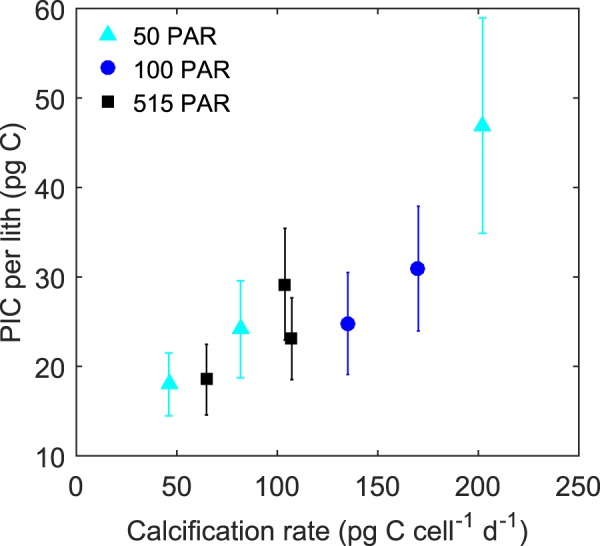
PIC content, by weight, of average S. apsteinii coccolith (mix of muroliths and lopadoliths) versus calcification rate under different light and CO₂ conditions. Error bars represent standard deviation for each treatment condition.

Discussion

Increasing CO₂ concentrations resulted in an optimum curve response in all physiological rates for S. apsteinii (Fig. 2). This response pattern has now been observed for multiple coccolithophore species^16,18, and is most likely driven by the combined effects of physiological rate stimulation by increasing substrate (CO₂ and ${\mathrm{HCO}}_3^{-}$) availability and physiological rate inhibition by increasing [H⁺]^14,17,18,36.

Larger species generally require more substrate than smaller species to sustain growth. However, larger species have less surface area, relative to their volume, over which to take up essential materials and nutrients³⁷. As such, it is expected that larger species, with a lower surface area to volume ratio, would require either a higher substrate concentration or a faster uptake rate in order to support their higher cellular requirements³⁷. If higher substrate concentrations were needed then it could be expected that S. apsteinii might have higher CO₂ half-saturation and optimum requirements than E. huxleyi and G. oceanica for all rates. However, S. apsteinii had a similar $\mathrm{K}\frac{1}{2}{}_{{\mathrm{CO}}_2}\mathrm{s}\mathrm{at}$ and CO₂ optimum as the two smaller species for growth and photosynthetic carbon fixation rates and a slightly higher CO₂ optimum requirement for calcification rates (Table 2,^20,30). This suggests that S. apsteinii would need to support its greater substrate demand by fixing more carbon per unit surface area than smaller species. To see if this was the case, daily carbon fixation per unit surface area was calculated using carbon fixation rates (POC, PIC and TPC from^16,19,20 and this paper at 20 °C) and average cell diameters of 5.59, 9.33, and 17.59 μm for E. huxleyi, G. oceanica and S. apsteinii, respectively.

Based on these calculations, S. apsteinii fixes more carbon per unit surface area per day than either E. huxleyi or G. oceanica under most CO₂ conditions at low to moderate light intensities (Fig. 5a and b). However, at higher light intensities it fixes approximately the same amount of carbon per unit surface area per day (Fig. 5c). The relative decrease in carbon fixation at these higher light intensities in comparison to E. huxleyi and G. oceanica is likely due to a higher light sensitivity (see below). Meanwhile below this threshold, the generally higher fixation of carbon per unit surface area per day by S. apsteinii can be achieved by higher inorganic carbon transporter density (higher substrate uptake), or by less diffusive CO₂ leakage from the cell. It may be that by having a proportionally lower amount of the cells internal volume interacting with the surrounding media (due to a much lower surface area to volume ratio) S. apsteinii loses proportionally less CO₂ through leakage. While decreased leakage in larger coccolithophore species has been suggested previously³⁷, this difference in leakage was thought to be driven by the uptake of different proportions of CO₂ and ${\mathrm{HCO}}_3^{-}$ between the species rather than size alone. While evidence suggests that carbon uptake efficiency is higher in some species and phytoplankton groups (i.e.^38,39), it is not yet clear if cell size has a consistent effect on carbon uptake efficiencies.

Figure 5.

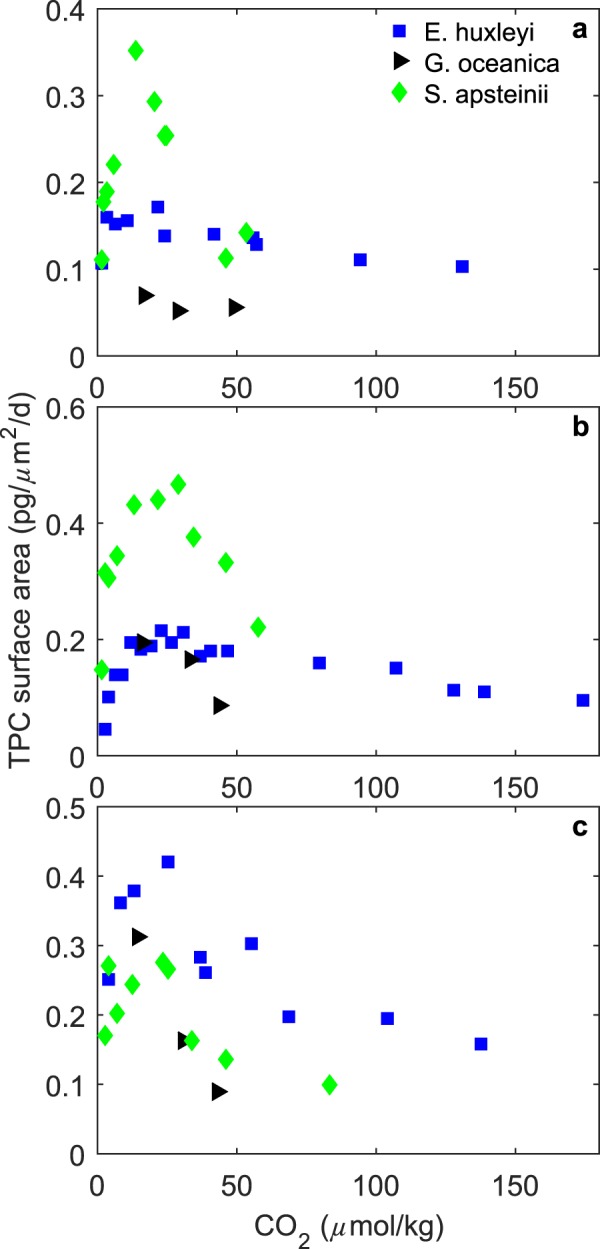
Total particulate carbon production rate per unit surface area (μm²) for E. huxleyi, G. oceanica and S. apsteinii across a range of CO₂ concentrations and at (a) 50, (b) 150–200, and (c) 515–600 μmol photons m⁻² s⁻¹.

Sensitivity to high CO₂/low pH varied between the three rates with calcification being more sensitive than photosynthesis and growth (Table 2). This agrees with previous work on G. oceanica, E. huxleyi and C. pelagicus, and provides additional evidence for the general notion that calcification by coccolithophores will be negatively impacted by future ocean changes^16,18–20. Sensitivity of calcification rates to high CO₂ $\left(\mathrm{K}\frac{1}{2}{}_{{\mathrm{CO}}_2}\mathrm{i}\mathrm{nhib}\right)$ are similar between S. apsteinii and E. huxleyi, with G. oceanica being much more sensitive^20,30. The steeper decline of calcification rates, beyond optimum CO₂, of G. oceanica compared to E. huxleyi has previously been speculated to be connected to a higher degree of inorganic versus organic carbon production (PIC:POC) in G. oceanica^18,20. The relatively greater production of intracellular H⁺, via calcification, makes it more difficult for species species with higher PIC content to maintain intracellular pH homeostasis¹⁷. In this regard, S. apsteinii with a similar PIC:POC as E. huxleyi would fit into this concept.

Significant inhibition of all rates by light was observed in S. apsteinii when CO₂ levels exceeded 100 μatm (Fig. 3, Table 3). That is in sharp contrast to rates in E. huxleyi and G. oceanica for which little light inhibition is observed regardless of f CO₂ levels^19,40–45. Optimal light intensities for growth, calcification and photosynthesis of S. apsteinii also differ from those in E. huxleyi and G. oceanica. While optimal physiological rates for S. apsteinii are observed at light intensities between ∼120–263 μmol photons m⁻² s⁻¹, depending upon f CO₂ level, optimal physiological rates for G. oceanica and E. huxleyi, under comparable experimental conditions, have been observed at light intensities of 400–800 μmol photons m⁻² s⁻¹ and 500–1200 μmol photons m⁻² s⁻¹, respectively (Table 2,^19,20). S. apsteinii also tends to half-saturate its rates $\left(\mathrm{K}\frac{1}{2}{}_{\mathrm{PAR}}\mathrm{s}\mathrm{at}\right)$ at lower light intensities than either E. huxleyi or G. oceanica under most CO₂ conditions (Table 2,^20,30, unpublished E. huxleyi data). This suggests that S. apsteinii may be a lower light adapted species which is also supported by the fact that it has been observed to grow at light intensities as low as 5 μmol photons m⁻² s^−1 46. Oceanic abundance data for S. apsteinii is sparse, as a result it is currently not possible to confirm if similar low light preferences are observed in the natural environment.

As f CO₂ levels increased S. apsteinii needed lower light intensities to sustain optimal rates. These results agree with those for G. oceanica, which also required less light to support optimum calcification and photosynthetic carbon fixation rates as f CO₂ increased³⁰. The results also agree with those for E. huxleyi growth rates, which required less light with rising f CO₂, but not calcification or photosynthetic carbon fixation rates which were insensitive to changing light intensity²⁰ (Table S3). In all three-above species, the increase in f CO₂ levels also resulted in an overall decrease in maximum rates^20,30 (Table 2). Most phytoplankton acquire inorganic carbon through a combination of passive CO₂ diffusion into the cell, and CO₂ concentrating mechanisms (CCMs) which actively transport inorganic carbon into the cell^47,48. As CO₂ concentrating mechanisms are an active process, they require energy to function^39,48. The efficiency of passive uptake depends upon the cell to seawater CO₂ concentration gradient (uptake versus leakage rates), membrane permeability and area³⁹. So, assuming that cell size and membrane permeability stay constant, increasing seawater CO₂ concentrations increase the diffusive influx and reduce loss through leakage. This would reduce the need for an active CCM thus lowering the cell’s energy requirements. As a result, higher substrate uptake efficiency could be indicated by a decrease in light levels needed to reach half-saturated and optimal rates (see Fig. 6 dotted line). Another explanation, for reduced light requirements could be the inhibiting effects of high CO₂/H⁺. As CO₂/H⁺ concentrations increase to above optimum levels it suppresses maximum rates to lower and lower absolute rates. Lower rates result in decreased demand for resources such as carbon. This lower substrate demand could result in decreased CCM activity thereby decreasing optimum light requirements under elevated CO₂/H⁺ (Fig. 6 dashed line). All three species show decreased maximum rates under elevated CO₂/H⁺. As a result, decreased energy requirements because of inhibited maximum rates could explain decreased optimum light requirements in E. huxleyi, G. oceanica and S. apsteinii. However, G. oceanica also shows indications of increased substrate uptake efficiency, through decreasing $\mathrm{K}\frac{1}{2}{}_{\mathrm{PAR}}\mathrm{s}\mathrm{at}$ and optimal light requirements³⁰. So, for G. oceanica it appears that increasing CO₂/H⁺ may decrease light optimal requirements through a combination of increased substrate uptake efficiency and through inhibition of maximum rates (Fig. 6 dot-dashed line).

Figure 6.

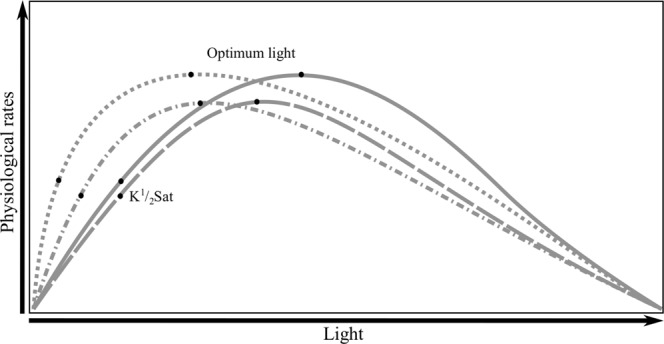
Conceptual diagram depicting how the light intensities required to support half-saturated $\left(\mathrm{K}\frac{1}{2}\mathrm{sat}\right)$ and optimum rates change with changing CO₂. While the solid line represents the default response to rising light levels, the dotted line represents an increase in substrate uptake efficiency with rising CO₂, the dashed line represents an increase in H⁺ inhibition with rising CO₂ and the dot-dashed line represents an increase in both substrate uptake efficiency and H⁺ inhibition with rising CO₂.

As well as decreasing light intensities for optimal rates, increasing f CO₂ also resulted in a narrowing of the light tolerances (light niche) of S. apsteinii. This was driven by a combined increase in light intensities required for half saturation of rates, and a decrease in light intensities required to half inhibit rates with rising f CO₂ (Table 3). The CO₂ niche of this species was also observed to narrow with increasing light intensity, with an increased sensitivity to high CO₂ and a decrease in CO₂ requirements for optimal physiological rates (Table 2). So S. apsteinii, when already under stress from one environmental condition, becomes less tolerant to other extreme environmental conditions, similar to E. huxleyi and G. oceanica for high CO₂ sensitivity under rising temperature^20,30. However, when exposed to the same stress of rising light intensities, E. huxleyi becomes less sensitive to high CO₂ conditions, while the sensitivity of G. oceanica to high CO₂ depends upon the rate considered^20,30. Similarly, when exposed to the stress of rising CO₂ conditions, neither E. huxleyi or G. oceanica show any change in sensitivity to high light conditions^20,30 (Table S3). So, it would appear that the response to multiple environmental stressors is species-specific. This adds weight to the suggestion that coccolithophore assemblages may undergo shifts in species composition, under future ocean change.

Depending on the emission scenario, p CO₂ levels are projected to reach between 420 to 985 μatm by 2100 resulting in ocean temperature increases of 2.6 to 4.8 °C (RCP 2.6–8.5)^49,50. Ocean warming is expected to strengthen stratification of the water column, resulting in a shallower mixed layer and thus a higher average light availability within the mixed layer^2,51. The dependence of light responses on f CO₂ levels, and vice-versa, could have important implications for S. apsteinii under future ocean conditions where both light and f CO₂ availabilities are expected to change. S. apsteinii already appears to be a low light adapted species with rates saturating at relatively low light intensities under current day f CO₂ conditions (<170 μmol photons m⁻² s⁻¹). Considering that light levels to saturate and inhibit rates for this species increase and decrease, respectively, with rising f CO₂ (Table 3), this species could become restricted to a narrower light range under predicted future ocean conditions.

Conclusion

S. apsteinii appears to be a low light adapted species, with a similar optimum curve response to rising CO₂ as E. huxleyi and G. oceanica, C. pelagicus and C. leptoporus. Calculations suggest that for S. apsteinii, a single unfavourable growth condition (CO₂, or light) will result in increased sensitivity to changes in other environmental variables. This contrasts with E. huxleyi, which either becomes less sensitive or shows no change in sensitivity to changes in other environmental variables when at extreme CO₂ or light conditions, and G. oceanica whose change in sensitivity varies between different physiological rates. With light and CO₂ levels both set to increase over the coming century^2,51 S. apsteinii could become restricted to narrower light and CO₂ ranges while under rising temperature, E. huxleyi and G. oceanica could become more sensitive to high CO₂. These species-specific changes in temperature/light/CO₂ sensitivity are likely to affect coccolithophore community composition under future ocean conditions.

Data from E. huxleyi, G. oceanica and S. apsteinii indicate that rising CO₂ levels will also result in decreased optimum light requirements of all species as a result of reduced maximum rates and, in the case of G. oceanica, increased substrate uptake efficiency. This adds further evidence to the idea that rising CO₂ will not only result in changes in species composition, but also in community-wide shifts in total organic and inorganic carbon production by coccolithophores, with consequent effects on local carbon cycling and sequestration.

Methods

Experimental set-up

Mono-specific cultures of S. apsteinii (strain RCC1456 isolated from the Mediterranean Sea, Spain) were grown in artificial seawater (ASW)⁵² at a salinity of 35 and temperature of 20 °C across a p CO₂ gradient of ∼50–7000 μatm. Cultures were incubated at 50, 100, 200 and 515 μmol photons m⁻² s⁻¹ of photosynthetically active radiation (PAR) on a 16:8 h light-dark cycle in a Panasonic Versatile Environmental Test Chamber (MLR-352-PE). Light intensities, for bottle placement, within the chamber were measured using a LI-193 spherical sensor (LI-COR). Cells were pre-acclimated to experimental conditions for 7–13 days depending upon cell division rates. Initial cell densities for each bottle varied between 35–50 cells ml⁻¹ depending upon the treatment conditions.

Media preparation

Artificial seawater (ASW), salinity 35, was prepared according to⁵², minus the initial addition of bicarbonate. The ASW was enriched with f/8 trace metals and vitamins⁵³, 64 μmol kg⁻¹ nitrate (${\mathrm{NO}}_3^{-}$), 4 μmol kg⁻¹ phosphate (${\mathrm{PO}}_4^{3-}$), 10 nmol kg⁻¹ SeO₂ and 1 ml kg⁻¹ of sterile filtered seawater collected from Shelly beach, Ballina. ASW medium was sterile-filtered (0.2 μm pore size, Whatman^TM Polycap 75 AS) into autoclaved polycarbonate bottles, Nalgene for acclimation (0.5 L) or experimentation (2 L), with a small head-space left for the adjustment of carbonate chemistry conditions.

Carbonate chemistry manipulation

Total alkalinity (TA) and dissolved inorganic carbon (DIC) for each treatment was adjusted through calculated additions of hydrochloric acid (certified 3.571 mol L⁻¹ HCl, Merck) and 1.4 mol L⁻¹ sodium carbonate (Na₂ CO₃ Sigma-Aldrich, TraceSELECT quality dried for 2 hours at 240 °C). At the end of the experiment samples for TA and DIC were taken, stored and measured following methods used in²⁰. Carbonate chemistry speciation (${\mathrm{HCO}}_3^{-}$, ${\mathrm{CO}}_3^{2-}$, CO₂, pH) for each treatment was calculated from measured TA, DIC, temperature, salinity and [${\mathrm{PO}}_4^{3-}$] using the program CO2SYS⁵⁴, the stoichiometric equilibrium constants (K₁ and K₂) for carbonic acid determined by⁵⁵ and refitted by⁵⁶, K_S for sulphuric acid determined by⁵⁷ and K_B for boric acid following⁵⁸.

Cell densities and particulate and dissolved carbon

Cell densities for each treatment were checked every 2–3 days using a flow cytometer (Becton Dickinson FACSCalibur). Living cells were detected by scatter plots of red autofluorescence in relation to orange fluorescence of the cells (FL3 vs. FL2). Specific growth rate (μ) was determined using cell density counts from the beginning and at the end of the experiment. Growth rate was calculated as:

$$\mu =\frac{\ln ({\mathrm{C}}_{\mathrm{t}})-\ln ({\mathrm{C}}_{\mathrm{0}})}{\mathrm{d}}$$ 1

where C_t and C₀ are the cell densities at the end and the beginning of the experiment respectively and d is incubation length in days. Calcification and photosynthetic rates were obtained by multiplying cellular quotas of particulate inorganic (calcification) or particulate organic (photosynthesis) carbon with growth rates. End of experiment sampling started approximately two hours after the onset of the light period and lasted no longer than 3 hours. Duplicate samples for total and organic particulate carbon (TPC and POC) were filtered (−200 mbar) onto pre-combusted (500 °C for 4 hours) Whatmann GF/F filters and stored in pre-combusted (500 °C for 4 hours) glass petri-dishes at −20 °C. Prior to analysis, POC filters were treated with 37% fuming HCl in a desiccator for 2 hours to remove all particulate inorganic carbon (PIC). TPC and POC filters were dried and analysed for carbon content and carbon isotopic signatures on an elemental analyser (Flash EA, Thermo Fisher) coupled to an isotope ratio mass spectrometer (IRMS, Delta V plus, Thermo Fisher) according to⁵⁹. PIC content was calculated by subtracting measured values of particulate organic carbon (POC) from total particulate carbon (TPC).

Data fitting

Cellular metabolic rates (n = 39 for calcification and photosynthesis and n = 37 for growth) were fitted to a non-linear equation derived in³⁰ as

$$\mathrm{MR}(\mathit{S},\mathit{I},\mathit{H})=\frac{{\mathrm{k}}_1\mathrm{SI}}{{\mathrm{k}}_{\mathrm{2}}\mathrm{H}+{\mathrm{k}}_{\mathrm{3}}\mathrm{SH}+{\mathrm{k}}_{\mathrm{4}}\mathrm{I}+{\mathrm{k}}_{\mathrm{5}}\mathrm{SI}+{\mathrm{k}}_{\mathrm{6}}{\mathrm{SHI}}^{\mathrm{2}}}$$ 2

where the metabolic rate (MR) of photosynthesis, calcification or growth is dependent on substrate (S = CO₂ and ${\mathrm{HCO}}_3^{-}$), [H⁺] (H) and light (I) conditions, and fit coefficients k₁, k₂, k₃, k₄, k₅ and k₆. For the experiment, a higher number of treatment levels was used at the expense of replication within treatments. This approach provides more information on the functional relationship, and tipping points, between carbonate chemistry and cellular rates without a significant loss of statistical power (see⁶⁰). Statistical results (R², fit coefficients, p-values, F-values and degrees of freedom), as well as V_max (maximum production rates), $\mathrm{K}\frac{1}{2}{}_{{\mathrm{CO}}_2}\mathrm{i}\mathrm{nhib}$ (conditions under which rates are reduced to half of maximum by either high light or high CO₂ concentrations), $\mathrm{K}\frac{1}{2}{}_{{\mathrm{CO}}_2}\mathrm{s}\mathrm{at}$ (conditions where low light or low CO₂ availability reduces rates to half of maximum) and optima (CO₂ or light conditions where V_max is reached), are presented in Tables 1, 2 and 3, respectively. Please note changes in CO₂ optima and half-saturation of less than 10 μmol kg⁻¹ were considered to be within the uncertainties of the model fit.

Coccolith morphology

S. apsteinii is unusual in that it bears two types of coccoliths; the plate-like muroliths and the vase-shaped lopadoliths⁶¹. To assess potential changes in coccolith morphology under different treatments, samples for scanning electron microscopy (SEM) were filtered onto Nucleopore^TM polycarbonate membrane filters (25 mm diameter, 0.8 μm pore size) and air-dried at room temperature over 12 hours. Samples were then stored in a desiccator until analysis. Samples were mounted onto metallic stubs using sticky tabs and sputter-coated with gold before being visualized using a ZEISS EVO/LS15 scanning electron microscope. For each treatment 80–100 cells were examined and the number of muroliths and lopadoliths per cell, average of murolith and lopadolith length and width per cell, and the ratio of muroliths to lopadoliths were recorded. Due to time constraints, only a sub-set of treatments could be examined. These were; 100, 400 and 2000 μatm f CO₂ at 50 and 515 μmol photons m⁻² s⁻¹ and 100, 200, 400 and 2000 μatm at 100 μmol photons m⁻² s⁻¹ of PAR. Comparisons between treatments were made using a Kruskal-Wallis ANOVA with an alpha level of significance of 0.05. The Kruskall-Wallis ANOVA was chosen firstly to avoid an inflated Type I error rate which can occur if making multiple comparisons between groups, and secondly to account for the slight non-normal distribution of the SEM data. The mass of PIC per coccolith was calculated by dividing PIC quota per cell by the total number of liths (muroliths + lopadoliths) for each treatment. Patterns in PIC per coccolith were tested using a linear regression with an alpha of significance of 0.05. Linear regressions were chosen for the reasons explained in Section ‘Cell densities and particulate and dissolved carbon’ above.

Author Contributions

All authors conceived and designed the experiments, N.G. conducted the experiments, K.S. and N.G. analysed the data. All authors reviewed the manuscript.

Data Availability

Datasets on physiological rate responses generated and compared during the current study are available in this published article (and its Supplementary Information files) and in^20,30. Datasets containing the raw scanning electron microscopy values are available from the corresponding author on reasonable request.

Competing Interests

The authors declare no competing interests.

Electronic supplementary material

Supplementary information accompanies this paper at 10.1038/s41598-019-38661-0.