Effect of calcium ions and pH on the morphology and mechanical properties of hyaluronan brushes

Abstract

Hyaluronan (HA) is a linear, regular polysaccharide that plays as a chief structural and functional component in peri- and extracellular matrices, thus contributing significantly to many basic cellular processes. To understand more comprehensively the response of the supramolecular organization of HA polymers to changes in their aqueous environment, we study the effects of Ca²⁺ concentration and pH on the morphology and rigidity of films of end-grafted HA polymers on planar supports (HA brushes), as a well-defined in vitro model system of HA-rich matrices, by reflection interference contrast microscopy and quartz crystal microbalance. The thickness and softness of HA brushes decrease significantly with Ca²⁺ concentration but do not change with pH, within the physiological ranges of these parameters. The effect of Ca²⁺ on HA brush thickness is virtually identical to the effect of Na⁺ at 10-fold higher concentrations. Moreover, the thickness and softness of HA brushes decrease appreciably upon HA protonation at pH less than 6. Effects of pH and calcium ions are fully reversible over large parameter ranges. These findings are relevant for understanding the supramolecular organization and dynamics of HA-rich matrices in biological systems and will also benefit the rational design of synthetic HA-rich materials with tailored properties.

1. Introduction

As a chief component of the extracellular matrix, hyaluronan (HA) is ubiquitous in the extracellular space of vertebrates and plays various biological roles. It is a linear, unbranched polysaccharide composed of identical disaccharide units containing glucuronic acid and N-acetylglucosamine [1]. Each disaccharide, which is 1.0 nm long [2], carries one chargeable carboxylic group. The pK_a of polymeric HA is approximately 3, which implies that HA is negatively charged at physiological pH [3].

Cells use HA synthases (HASs) in the plasma membrane to synthesize HA and extrude it into the extracellular space [4]. HA molecules produced can thus reach a very high molecular weight, up to millions of daltons [1]. HA can either be released into the extracellular space or remain grafted to the cell surface via HASs [4] or otherwise attached through HA receptors, such as CD44 [5], to form so-called pericellular coats (PCCs). HA-rich PCCs fulfil crucial functions in basic cellular processes such as proliferation and migration [6], intercellular adhesion [7] and mechanosensing [8]. To modulate these functions, the morphology and physical properties of HA-rich PCCs are remodelled by changes in the extracellular environment. HA-binding proteins, for example, can induce condensation and rigidification (by cross-linking, such as with the inflammation-associated protein TSG-6 [9]) or swelling of HA-rich matrices (by intercalation of swollen proteoglycans such as aggrecan [10–12]). On a more basic level, the physico-chemical properties of the aqueous solution such as pH and ionic strength also affect the properties of HA and the assemblies that it forms. This is relevant for the function of HA-rich matrices around cells and in biological tissues, where changes in salt concentration and pH modulate HA-related functions [13–16]. Artificial HA-rich matrices [17] and surface coatings [18] have become popular in bioengineering research, and a good knowledge of the effect of pH and salts on the ultra-structure and mechanical properties of HA-rich matrices is thus also important for technological applications. Previous studies focusing on HA solutions have shown that besides monovalent cations such as sodium [19,20], divalent calcium ions and pH also modulate the molecular structure and viscoelasticity of HA [21–27]. In biological and synthetic materials, however, HA is confined by cross-linking (in hydrogels) or by grafting or adsorption (in interfacial films), and there is still very limited information about the impact of environmental conditions such as pH and salts on such supramolecular HA assemblies.

Here, we study the effect of environmental conditions on films of HA polymers that are grafted via their reducing end to a planar support (figure 1a). These films are less complex than native PCCs yet they reproduce salient features such as the confinement of HA to a surface, the HA density in and typical thickness of, PCCs. Moreover, they are well defined in that the molecular weight and arrangement of HA are tightly controlled. This enables quantitative studies and correlation with polymer physics theory that would not be possible with native PCCs. The repulsion between neighbouring HA chains induces their stretching away from the surface and films of end-grafted HA are adequately described as polyelectrolyte brushes. In previous work, we quantified the mechanical properties of such HA brushes at physiological ionic strength and pH [28], and also how the monovalent salt NaCl affects HA brush morphology and mechanical properties [29]. These data revealed how the strong charge and the intrinsic stiffness of HA lead to brush properties that are distinct from those observed for synthetic polyelectrolytes. In this study, we employ two surface-sensitive analysis techniques, reflection interference contrast microscopy (RICM) and quartz crystal microbalance with dissipation monitoring (QCM-D), to quantify the effects of calcium ions and pH on the thickness and softness of HA brushes systematically over an extensive parameter range, that is, calcium concentrations between 1 µM and 1 M, and pH values between 1.0 and 9.0. The study sheds light on the effect of calcium and pH on the supramolecular organization and physical properties of surface-confined HA chains and highlights HA to be exquisitely responsive to these stimuli, both in terms of the magnitude and the reversibility of the response.

Figure 1.

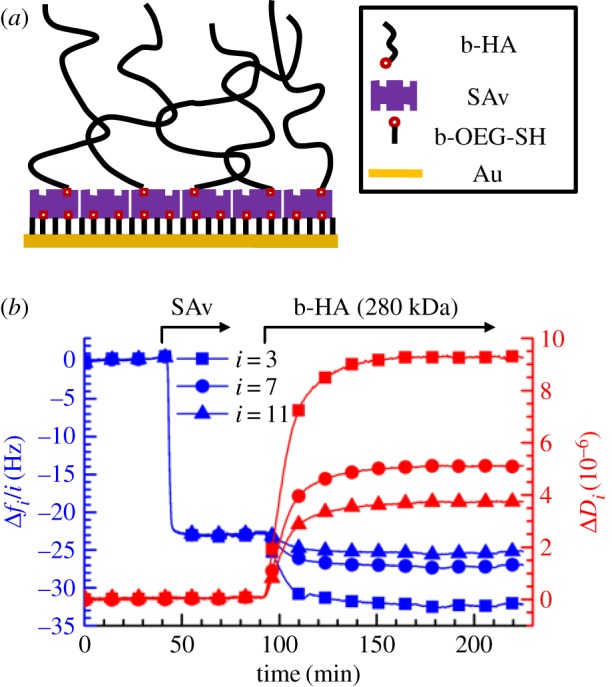
(a) Schematic of the build-up of HA brushes (not to scale). (b) Assembly of an HA brush; a biotinylated OEG monolayer on gold was formed ex situ and all subsequent assembly steps were followed by QCM-D. Normalized frequency shifts (Δf_i/i) and dissipation shifts (ΔD_i) at overtones i = 3, 7, 11 are presented. The start and duration of incubation of each sample (20 µg ml⁻¹ SAv and 5 µg ml⁻¹ b-HA) are indicated by arrows on top; during remaining times, the surface was exposed to sample preparation buffer (10 mM HEPES, 150 mM NaCl, pH 7.4). (Online version in colour.)

2. Experimental section

2.1. Materials

The oligo-ethylene glycol (OEG) solution was a mixture of 99% of 1 mM OEG thiol (M_w 386.5 Da) and 1% of 1 mM biotinylated OEG thiol (788 Da; both from Polypure, Oslo, Norway) in synthesis grade ethanol, stored in the dark at 4°C. Lyophilized streptavidin (SAv; 60 kDa; Sigma-Aldrich) was dissolved in ultrapure water. The stock solution at 1 mg ml⁻¹ was stored as aliquots at −20°C. Lyophilized hyaluronan, biotinylated at its reducing end (b-HA) and with a well-defined molecular masses of 280 ± 14 kDa (Select-HA B250) and 58 ± 3 kDa (Select-HA B50), as well as non-biotinylated HA of 237 ± 12 kDa (Select-HA 250), were from Hyalose (Oklahoma City, USA). HA was suspended in ultrapure water at 1 mg ml⁻¹ (HA) or 0.5 mg ml⁻¹ (b-HA), gently shaken at 4°C for 2 h for reconstitution and stored at −20°C.

All buffer and salt solutions were prepared in ultrapure water (resistance 18.2 MΩ cm⁻¹) and degassed before use. The sample preparation buffer contained 10 mM HEPES and 150 mM NaCl at pH 7.4. NaCl and CaCl₂ · 2H₂O were from Sigma-Aldrich. Potassium phosphate solutions with pH values ranging from 9.0 to 5.0 were prepared by mixing desired ratios of stock solutions, 100 mM KH₂PO₄ (Sigma-Aldrich) and 50 mM K₂HPO₄ (Fluka, Germany), prior to use. The potassium phosphate solution with pH 5.0 was further titrated by concentrated HCl (37%, reagent grade; Scharlab, Spain) to obtain solutions with pH values down to 1.0.

Polystyrene microspheres with 23.7 ± 1.5 µm diameter (Polysciences, Hirschberg, Germany) were washed in ethanol and ultrapure water and stored at 4°C in ultrapure water.

2.2. Preparation of substrates

Glass coverslips (#1.5, 24 × 24 mm²; Menzel Gläser, Germany) were gently wiped with lint-free tissue (Kimtech Science, Surrey, UK), blow-cleaned with N₂ gas, immersed in freshly prepared piranha solution composed of concentrated H₂SO₄ (95–98%) and H₂O₂ (50%; both Scharlab) at a volume ratio of 3 : 1 for 3 h, thoroughly rinsed with ultrapure water, blow-dried with N₂ gas and stored in sealed Petri dishes. To obtain gold (Au) coated substrates, clean glass coverslips underwent 10 min plasma cleaning in the vacuum chamber of a magnetron sputter system (ATC 1800 UHV; AJA International, Scituate, MA) before deposition of an adhesive titanium (Ti; 0.5 nm) and a 5 nm Au layer. The layer thickness was determined from the deposition rate which was calibrated by spectroscopic ellipsometry on reference silicon wafers. Au-coated QCM-D sensors (QSX301; Biolin Scientific, Västra Frölunda, Sweden) were rinsed with ethanol and ultrapure water and blow-dried with N₂ gas. Prior to use, all substrates were UV-ozone treated (ProCleaner; Bioforce Nanoscience, Ames, IA) for 30 min.

2.3. Preparation of hyaluronan brushes

Cleaned gold-coated substrates were immersed in OEG solution overnight, rinsed with ethanol and ultrapure water, blow-dried with N₂ gas, installed in fluidic devices for QCM-D or RICM and covered with sample preparation buffer. The surfaces were then sequentially exposed to SAv (20 µg ml⁻¹; 30 min) and b-HA (5 µg ml⁻¹ for QCM-D, 20 µg ml⁻¹ for RICM; 2 h) in sample preparation buffer, with each incubation step followed by abundant rinsing to remove excess molecules from the solution phase.

2.4. Quartz crystal microbalance with dissipation monitoring

QCM-D measurements were performed with a QSense E4 system (Biolin Scientific) in flow mode (5–20 µl min⁻¹, adjusted with a syringe pump; KD Scientific, Holliston, MA) at a working temperature of 24°C on Au-coated QCM-D sensors with an OEG monolayer. Changes in the sensor resonance frequency (Δf_i) and dissipation (ΔD_i) were collected at six overtones (i = 3, 5, 7, 9, 11, 13, corresponding to resonance frequencies of f_i ≈ 15, 25, 35, 45, 55, 65 MHz); for simplicity, only selected overtones (i = 3, 7, 11) are presented. As controls, measurements were also performed on surfaces covered by SAv, with or without 10 µg ml⁻¹ of non-biotinylated HA in the ambient solution.

2.5. Reflection interference contrast microscopy

For RICM, we used fluidic chambers in the form of cylindrical open cuvettes (5 mm diameter) made from a tailored Teflon holder and a glass coverslip at the bottom, glued together with two-component glue (Twinsil; Picodent, Germany). Cuvettes were used with a liquid volume of 50 µl. Samples were injected and the solution was rapidly homogenized with a pipette. Excess molecules were removed from the solution phase by repeated dilution and aspiration of the cuvette content until a concentration reduction of at least 10⁵ fold was reached. Care was taken to keep the glass coverslip wet throughout all solution exchanges. A separate cuvette was used to test for the effect of each salt (CaCl₂ or NaCl) concentration or pH on HA brush thickness. Polystyrene microspheres for RICM analysis were added 15 min after incubation of a given test solution.

Interferographs were obtained with an inverted microscope (Axio Observer Z1; Zeiss, Oberkochen, Germany) in epi-illumination reflection mode, using an XBO xenon short-arc lamp as light source, an antiflex oil immersion objective (EC Plan Neofluar Antiflex 63×/1.25; Zeiss) and a filter cube with two crossed polarizers (AHF Analysentechnik, Germany) [30]. The reflected light passed through a custom-built beam splitter unit [28] with band pass filters which in combination with two CCD cameras (ORCA-ER; Hamamatsu Photonics, France) permitted simultaneous acquisition of interferographs at wavelengths λ = 630, 546 and 490 nm. The opening of the aperture diaphragm was reduced to its minimum throughout all measurements.

To quantify the distance between the polystyrene sphere and the substrate surface, we adapted a previously established algorithm implemented in Matlab [28]. Briefly, the method is based on the determination of the position of extrema in the radial intensity profile of interferographs [30] and has an estimated accuracy of ±5 nm. The method was here adapted to account for the presence of the metallic coating and the OEG and SAv layers (see electronic supplementary material, methods and figure S1). Ten polystyrene beads were analysed per sample, and mean distances ± standard deviation were used for further analysis.

3. Results

3.1. Assembly of hyaluronan brushes

HA brushes were formed, as schematically shown in figure 1a, by grafting of HA with well-defined molecular weight (280 ± 14 kDa) via a biotin at the reducing end to a monolayer of SAv supported by a biotinylated thiol-OEG monolayer on gold. This approach has been established previously [9,31]. QCM-D was used to follow the main assembly steps (figure 1b). To a first approximation, a decrease in frequency shift Δf indicates an increase in the surface-bound mass (including hydrodynamically coupled water), whereas the dissipation shift ΔD reflects the softness of the surface-associated film [31].

The frequency and dissipation shifts upon SAv injection (figure 1b, 40–70 min; final shifts Δf₃/3 = −24 ± 1 Hz and ΔD₃ = 0.2 ± 0.2 × 10⁻⁶) were in good agreement with previous studies [9] and confirm the formation of a dense and stably anchored SAv monolayer. Subsequent incubation with biotinylated HA (figure 1b, 90–210 min) led to an additional decrease in the normalized frequency shift (Δf₃/3 = −9 ± 1 Hz) and a large increase in dissipation (ΔD₃ = 9 ± 1 × 10⁻⁶). The latter observation, together with the significant spreading of the responses between different overtones, is consistent with the formation of a highly hydrated and soft film, as expected for HA brushes [10]. The rate of frequency and dissipation changes gradually decreased with progressing b-HA incubation time and virtually stabilized after 2 h; these responses are consistent with the formation of a polyelectrolyte brush, where the HA film increasingly hampers penetration and surface-binding of new molecules as it becomes denser. HA molecules were also stably grafted as demonstrated by the lack of response upon rinsing with buffer (figure 1b; greater than 210 min).

3.2. Hyaluronan film thickness, response to NaCl and grafting density

Colloidal probe RICM was used to quantify the thickness of the HA film. The method measures the distance between a colloidal sphere and a planar transparent substrate [30], with a resolution of a few nanometres by the analysis of interference patterns arising from partial reflection of light at the interfaces between the planar SAv-covered substrate and the solution, and between the solution and the colloidal probe. Analogous to previous work by us and others [31,32], we used triple wavelength RICM to unambiguously resolve the thickness over a range of 1 µm, but we here refined the analysis method to account for the presence of the semi-transparent gold coating and the OEG and SAv layers (see electronic supplementary material, methods and figure S1).

The mean thickness of the HA film in sample preparation buffer (containing 150 mM NaCl and pH 7.4, close to physiological ionic strength and pH) was 207 nm. The standard deviation across 10 colloidal probes randomly positioned on the same surface was typically ±10 nm, and the standard deviation of the mean values of 5 measurements on independently prepared HA films was ±5 nm. This demonstrates that the HA brushes are laterally homogeneous and that their preparation is reproducible. With a contour length of 1.0 nm per disaccharide [2] and a molecular mass of 378 Da per disaccharide, the contour length of 280 ± 14 kDa HA is l_c = 740 ± 37 nm, and at physiological ionic strength and pH, an unperturbed HA chain of this length is expected to form a random coil with a radius of gyration of R_g ≈ 40 nm [33]. The HA chains are thus significantly stretched compared to their dimensions in solution, confirming that a polyelectrolyte brush is effectively formed.

To further validate the integrity of the HA brush, we quantified the brush thickness as a function of NaCl concentration. We have previously reported a detailed analysis of such a dependence for brushes of HA with a distinct molecular weight (1083 kDa) [29], which serves as a benchmark for the present work. The distance H between polystyrene spheres floating on the HA brush and the SAv-coated substrate was again used as an approximate measure of the brush thickness, and the results are shown in figure 2a (filled circles). Consistent with our previous findings [29], H decreased monotonically with increasing NaCl concentration. The shape of the curve was qualitatively consistent with theoretical predictions for brushes of strongly charged polyelectrolytes [34–36]. Specifically, at low ionic strength ([NaCl] ≤ 0.5 mM), the measured thickness varied only weakly, and this regime corresponds to the ‘osmotic brush’ regime [35] where the brush thickness is determined by the balance of the osmotic pressure of counterions trapped in the brush and the entropy of chain stretching [34]. At higher ionic strengths (1 mM ≤ [NaCl] ≤ 1000 mM), salt screens the electrostatic interactions (‘salted brush’ regime) [35] and leads to a progressive decrease in brush thickness. At very high ionic strength, it is expected that the screening becomes so strong that the excluded volume repulsion of the uncharged polymer backbone (intrinsic excluded volume) dominates the brush behaviour and the dependence on ionic strength again weakens. This ‘quasi-neutral’ brush regime is barely reached in the experiment presented here. We note that the slope for the salted brush regime in the log–log plot is −0.22, which deviates from the power of −1/3 expected by simple theory [34–36]. We had previously demonstrated that the apparent attenuation of the salt dependence compared to the theoretical prediction is expected and due to two distinct effects [29]: towards low ionic strength, HA brushes become strongly stretched (with H/l_c larger than 0.5) and the resistance to stretching thus becomes stronger than what is predicted according to the Gaussian chain approximation used in the theory; towards high ionic strength, on the other hand, the intrinsic excluded volume of the uncharged polymer backbone, which is positive for HA but not considered in the scaling theory, increasingly contributes to inter-chain repulsion and thus limits the screening effect of the salt.

Figure 2.

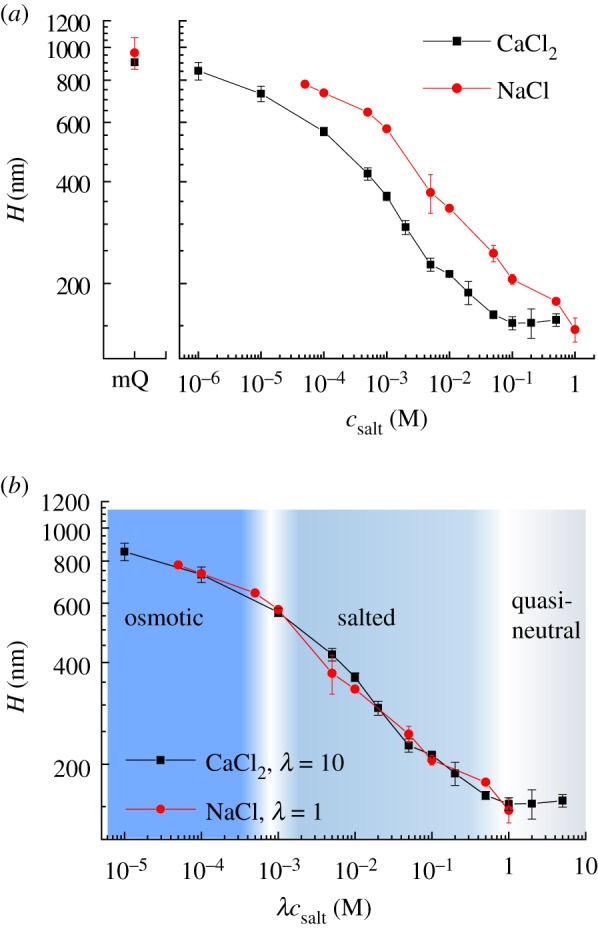
Effect of sodium and calcium ions on HA brush thickness. (a) The distance H of colloidal probes (polystyrene beads, around 24 µm diameter, hovering on top of HA brushes due to gravitation) from the SAv-coated substrate was quantified by RICM and is a measure of the HA film thickness. HA brushes were prepared as shown in figure 1b, and data represent means and standard deviations from two independent experiments. Next to CaCl₂ (squares), results for NaCl (circles) are also shown, together with results in ultrapure water without added salt (mQ). (b) Both datasets superpose when the salt concentration is re-scaled, by multiplication with λ = 1 for NaCl and λ = 10 for CaCl₂. The three canonical brush regimes—osmotic, salted and quasi-neutral—can be identified and are indicated with the shaded background. (Online version in colour.)

In our previous work, we also showed that the combined effect of intrinsic excluded volume and electrostatic repulsion on HA brush morphology can be quantitatively reproduced in the limit of high ionic strength (NaCl greater than or equal to 50 mM) with an analytical self-consistent mean-field theory [29]. With this approach, we can estimate the grafting density of the HA brush studied here (electronic supplementary material, figure S2), and the root-mean-square (RMS) distance between anchor points is s ≈ 50 nm. From the HA molecular weight, the brush thickness and the grafting density, one can estimate the concentration of chargeable groups in the HA brush to match the concentration of added salt at around 0.8 mM NaCl. This value is consistent with the transition between the osmotic and salted brush regimes identified above.

Detailed inspection of the bead–substrate distances H in figure 2a reveals that these exceed the contour length of the HA chain without added salt or at very low NaCl concentrations. Specifically, in ultrapure water, H exceeded l_c by approximately 220 nm. This result was unexpected and was not observed in the earlier study with HA brushes made from approximately four times larger HA chains [29]. On the other hand, we found the bead–substrate distance on HA brushes made from smaller HA chains (58 ± 3 kDa) to be 579 ± 8 nm in ultrapure water, that is, the discrepancy with the contour length (153 ± 8 nm) is even larger than in the case of 280 kDa b-HA. Clearly, the polystyrene bead hovers at a certain distance above the HA brush and we propose that this is due to repulsive electrostatic interactions between the negatively charged polystyrene bead and an excess negative charge displayed by the HA brush. The grafting density of the HA brush is expected to increase with decreasing HA size in our assays, because the kinetic limitations associated with brush formation become less severe for shorter chains [37]. The stretching of the HA chains and also the excess charge of the brush [29] would increase with grafting density, and the thickness trends as a function of HA molecular weight would thus be consistent with such a scenario.

Our finding thus indicates that H can significantly overestimate the brush thickness in the limit of low ionic strength. A quantitative estimation of the magnitude of the discrepancy appears difficult, because we lack the surface charge density on the colloidal sphere. However, with increasing salt concentration, the charges are screened, and we thus expect that the error is progressively decreasing as salt is added. At the same time, the colloidal probe will exert some pressure on the brush due to gravitation and H may thus effectively underestimate the thickness of the unperturbed brush. However, the forces acting on the brushes are small, less than 4 pN considering that gravitational forces are offset by buoyancy, and we thus estimate this effect to be small [29].

3.3. Impact of calcium ions on hyaluronan films

3.3.1. Morphology

We used colloidal probe RICM to analyse the impact of CaCl₂ on HA film thickness (figure 2a, filled squares). As for NaCl, the HA film thickness decreased monotonically with increasing CaCl₂ concentration, with the salt dependence being most pronounced at intermediate CaCl₂ concentrations (0.1 mM ≤ [CaCl₂] ≤ 100 mM) and a trend towards plateaus at higher and lower CaCl₂ concentrations. Over the entire range of CaCl₂ concentrations, the film thickness was reproducible to within a few nanometres across the measured surfaces, and the film thickness remained well above the RMS distance between grafting points. Thus, the HA polymers retain their hydrated and stretched, homogeneous brush conformation even at the highest CaCl₂ concentration.

Closer inspection revealed a high degree of similarity in the responses of HA brushes to the two salts. The curves for CaCl₂ and NaCl could be virtually overlaid on a single master curve through a simple re-scaling of the CaCl₂ concentration by a factor of 10 (figure 2b). This indicates that the effect of calcium ions on the interaction between HA chains in the brush, and apparently also the repulsion between the HA brush and the colloidal probe in the limit of low ion concentrations, is equivalent to the effect of sodium ions at 10-fold higher concentration. Such a simple scaling is remarkable, considering that the two cations have different valency and that the dependence of brush thickness on ion concentration is rather complex. Moreover, the scaling factor of 10 is substantially larger than what would be predicted for simple charge screening: the ionic strength, defined as $I=(1/2){\sum }_i{z}_i^2{c}_i$ where c_i are the molar concentrations of ions of valency z_i, is increased by three-fold for CaCl₂ as compared to NaCl at the same molar cation concentration. This comparison implies that the simple scaling by a factor 10 must arise from a combination of several distinct effects, which we shall discuss later (vide infra).

3.3.2. Viscoelastic properties

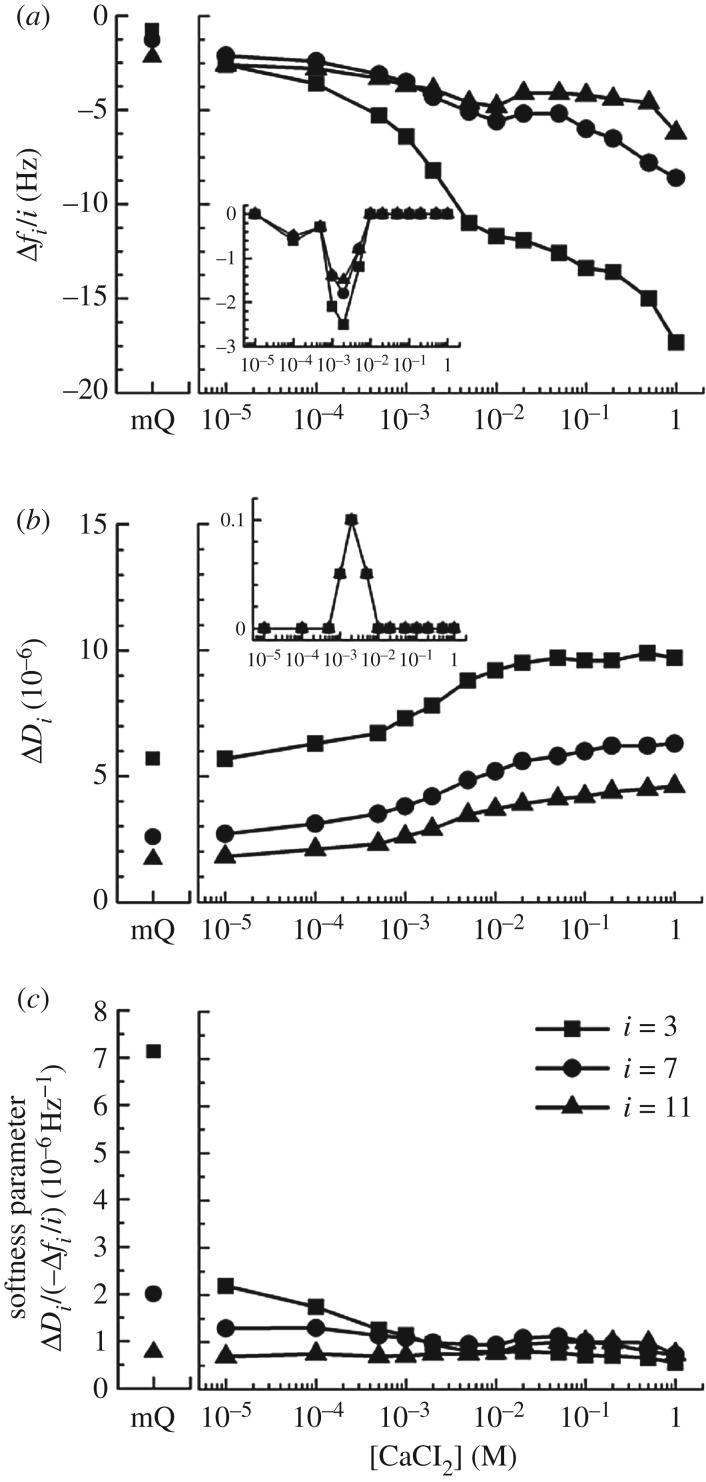
The effect of Ca²⁺ on the viscoelastic properties of HA brushes was characterized by QCM-D. To this end, frequency and dissipation shifts upon variation of the ambient CaCl₂ concentration were monitored on HA brushes (electronic supplementary material, figure S3A) as well as reference surfaces (bare SAv without HA; electronic supplementary material, figure S3B). By subtracting the responses on the reference surfaces from those on the HA brushes, the effect of the HA brush could be discriminated from the effects of the ions on the density and viscosity of the solution phase, to which the QCM-D is also sensitive. These data are shown in figure 3a,b. In addition, we also examined over the full range of CaCl₂ concentrations if non-biotinylated HA polymers (237 ± 12 kDa, at 10 µg ml⁻¹) bind to the reference surface (electronic supplementary material, figure S3C), and these effects are shown as insets in figure 3a,b. Figure 3c shows the ratio of dissipation and frequency shifts ΔD_i/(−Δf_i/i). This parameter is related to the mechanical properties of the surface-confined films: for very thin films, it is proportional to the elastic compliance, a measure of film softness [38]. The HA films studied here are too thick for this proportionality to hold, and the parameter should thus be considered an effective and relative measure of HA brush softness.

Figure 3.

Effect of calcium ions on HA brush rigidity, characterized by QCM-D. Normalized frequency shifts (Δf_i/i; (a)), dissipation shifts (ΔD_i; (b)) and softness parameter (ΔD_i/(−Δf_i/i); (c)) are displayed for selected overtones (i = 3, 7 and 11, as indicated in (c)). HA brushes were prepared as in figure 1b, and data represent responses at equilibrium of the HA film compared to a reference SAv monolayer film as a function of the CaCl₂ concentration in ultrapure water (see electronic supplementary material, figure S3, for details; data in ultrapure water without added salt are also shown, as mQ). Responses upon exposure of non-biotinylated HA (10 µg ml⁻¹) to the SAv monolayer are shown for comparison in the insets in (a,b); these show minor non-specific binding at intermediate CaCl₂ concentrations and correlate with transient minima in the frequency shifts for the HA brush. The softness parameter indicates HA brush stiffening with CaCl₂ concentration.

Overall, the film softness decreased with increasing calcium concentration, a trend that is expected as the salt-induced contraction of the HA brush (figure 2) makes the film denser. The dependence is monotonic except for a shallow local minimum around 2 mM CaCl₂. At this salt concentration, we also found HA to show minor yet significant non-specific interaction with the SAv substrate which was not present at lower and higher CaCl₂ concentrations (figure 3a, inset). The local minimum in the softness parameter, therefore, most likely is the consequence of a slight perturbation of the HA brush morphology by binding of a fraction of the HA chains to the SAv substrate in addition to the biotin anchorage. It is also notable that the softness parameter (figure 3c) does not show the pronounced sigmoidal dependence on the calcium concentration that we had observed for the HA brush thickness (figure 2). In particular, the rapid decrease in softness in the low calcium concentration regime (osmotic brush) suggests that the mechanical properties of the HA brush are sensitive to calcium concentration in the osmotic brush regime even though the thickness remains roughly unchanged.

We also note that the effect of calcium on the HA brushes was fully reversible for most of the calcium concentrations investigated, that is, QCM-D responses in ultrapure water before and after exposure to each calcium concentration were comparable (electronic supplementary material, figure S3A). Exceptions in this regard were the highest calcium concentrations (greater than or equal to 100 mM), for which a fraction of the frequency and dissipation shifts was not recovered upon rinsing in ultrapure water, as shown in detail in electronic supplementary material, figure S4. Apparently, very high calcium concentrations can promote irreversible (though minor) changes to the HA brush.

3.4. Impact of pH on hyaluronan films

3.4.1. Morphology

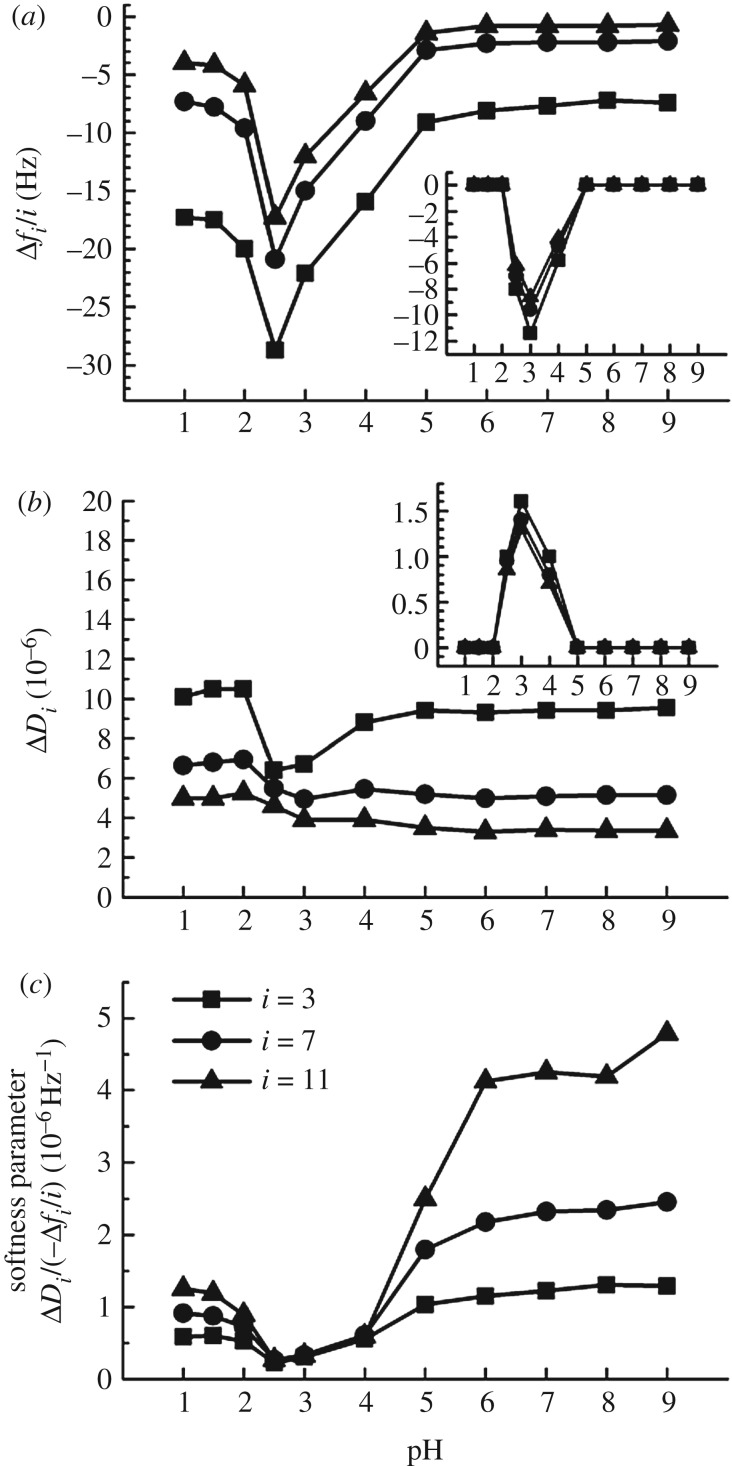
The effect of pH on the thickness of HA brushes was again characterized by RICM (figure 4a). We used potassium phosphate as buffering agent throughout, to avoid any effects due to variations in cation species or concentration. Phosphate buffer has pK_a values of 2.1, 7.2 and 12.3 [39], and we found the buffering capacity at a concentration of 100 mM K⁺ to be satisfactory over the tested pH range (1.0 to 9.0).

Figure 4.

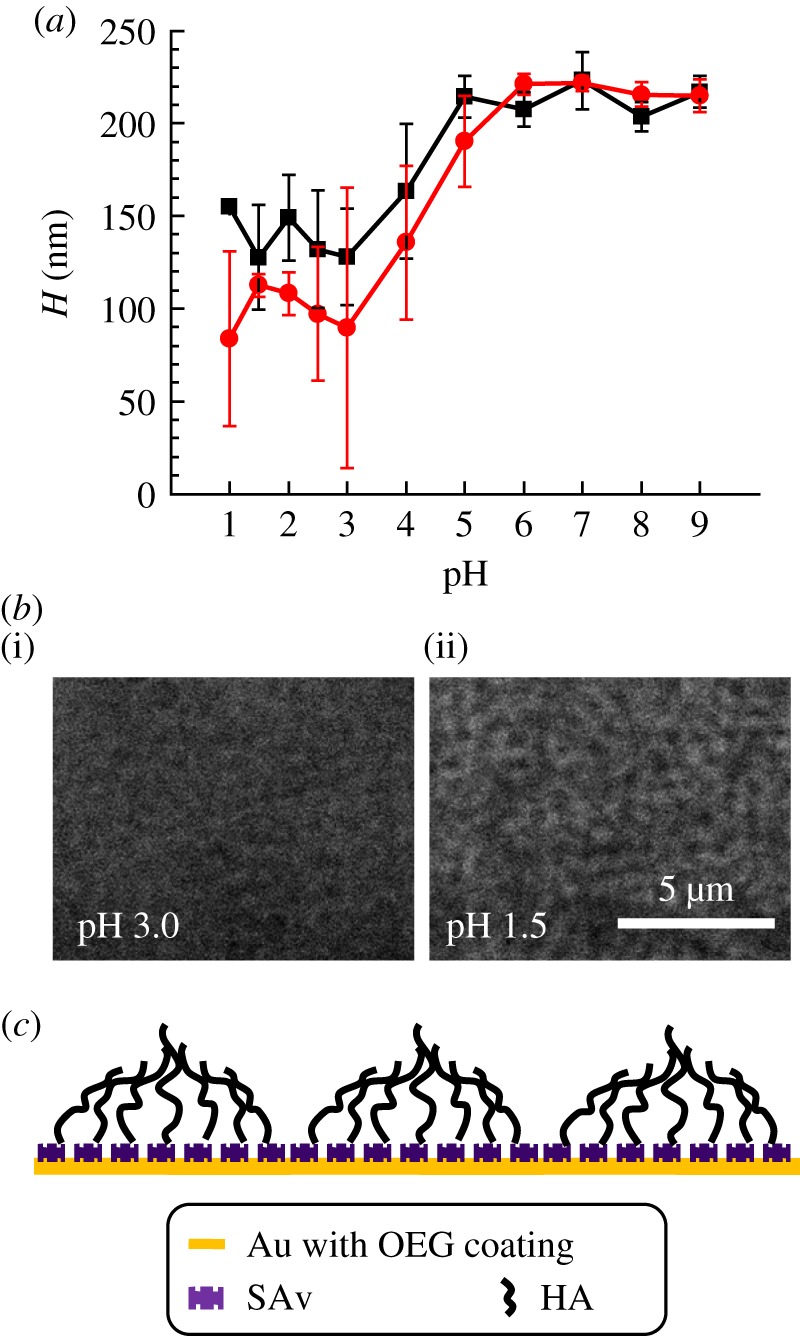
(a) Effect of pH on HA film thickness, quantified by RICM analogous to figure 2. Two independent experiments are shown. (b) Representative interferographs (without colloidal probe) of an HA film in the presence of pH 3 (i) and pH 1.5 (ii). Interferographs at pH 1.5 reveal a distinctive pattern, that is not (or only faintly) visible at pH greater than or equal to 3. The contrast of both images was adjusted consistently, and interferographs acquired at pH greater than 3 were comparable to what is shown here for pH 3. (c) Schematic of the hypothesized microphase formation in end-grafted HA films at low pH (not to scale). (Online version in colour.)

Over a broad range around neutral pH (6.0 ≤ pH ≤ 9.0), H remained virtually constant at 216 ± 7 nm. This value matches the results for 100 mM NaCl (206 ± 7 nm; figure 2), indicating that potassium and sodium have very similar effects on the HA brush. The mean H decreased by roughly two-fold between pH 6.0 and pH 3.0, and then remained roughly constant down to pH 1.0. The carboxyl groups in HA have a pK_a around 3 [3], and the reduction in film thickness is thus likely due to the protonation of carboxyl groups and loss of the polyelectrolyte character of the HA chains.

It is notable that the standard deviations in H increased markedly at pH values less than 5 (figure 4a). This indicates that the HA film loses its homogeneity under strongly acidic conditions, and instead forms a film that is heterogeneous on the microscale. Circumstantial evidence for microscale heterogeneities also comes from interferographs taken on HA films in the absence of a colloidal probe. These revealed a distinctive pattern at pH 1.5 that was not (or only faintly) visible at pH values larger than 3 (figure 4b). The contrast and spatial resolution of the interferographs are too low to assign a specific structure. However, the fact that we observe heterogeneities indicates that protonated HA becomes sticky, and we tentatively propose that the patterns arise from the clustering of sticky HA polymers into microdomains as schematically indicated in figure 4c. The formation of microphases is indeed predicted by theory [40,41] and was experimentally observed for brushes of cross-linked HA [12] and other polymers [42].

3.4.2. Viscoelastic properties

QCM-D was again used to study the influence of pH on HA film softness (electronic supplementary material, figure S5; and figure 5) following the method used for the characterization of the effect of CaCl₂ solutions (electronic supplementary material, figure S3; and figure 3). The softness parameter (figure 5c) was not sensitive to pH between pH 6.0 and 9.0 but decreased substantially at lower pH values. The most rigid films (i.e. with the smallest softness parameter) occurred at pH 2.5. Below this pH value, the HA films became again somewhat softer but clearly remained more rigid than at neutral pH. The effect of pH changes on HA brushes was fully reversible over the large range of pH variations studied, indicating that pH can be used for the reversible switching of HA brush properties. Notably, we did not find any indications for the degradation of HA upon exposure to pH down to 1.0, in contrast to some earlier reports studying HA in the solution phase [26,27,43].

Figure 5.

Effect of pH on HA brush rigidity, characterized by QCM-D. Data are displayed analogous to figure 3, in potassium phosphate buffer (containing 100 mM potassium) at various pH. The insets in (a,b) show strong non-specific binding of non-biotinylated HA at pH 4.0, 3.0 and 2.5. The softness parameter indicates that HA brush stiffening is most pronounced at these pH values.

It is notable that HA polymers also showed rather strong non-specific binding to the SAv-coated substrate for pH values ranging between 2.5 and 4.0, whereas no such binding was detectable at lower and higher pH values (figure 5a,b, insets). The electrostatic potential of SAv is positive below pH 6 [44], and attractive electrostatic interactions between the (partially) de-protonated HA and the acidic SAv may well be responsible for the selective non-specific binding of HA between pH 2.5 and 4.0. Moreover, it is likely that the transient minimum in the softness parameter at pH 2.5 arises from a combination of inter-HA chain attraction (after HA protonation) and HA–substrate attraction, whereas the values measured at pH 1.0 and 1.5 may well represent the genuine softness of the fully protonated HA brush (in the absence of attractive HA–substrate interactions).

4. Discussion

On the qualitative level, the results of our systematic analysis of the response of HA brushes to calcium ions and pH are in line with the known properties of HA in the solution phase. In particular, the response of HA brushes to sodium and calcium ions (figure 2) confirms that HA around neutral pH behaves as a strongly charged and semi-flexible polyelectrolyte in a good solvent (we here refer to semi-flexible as the statistical polymer segment length being much larger than the size of the chemical monomer). Moreover, a ‘putty’ gel state has been reported for HA solutions around pH 2.5 [26,45,46], and the collapse of HA brushes at low pH (figure 4a), accompanied by microphase formation (figure 4b,c) and film rigidification (figure 5), would be consistent with a transition from repulsive to attractive inter- and intra-chain interactions upon HA protonation.

On the quantitative level, a striking observation that to the best of our knowledge has not previously been reported is that divalent calcium ions affect the HA brush thickness in much the same way as monovalent sodium ions do at 10-fold higher concentrations. Can we explain such a similar behaviour? It is well known that divalent ions are more effective than monovalent ions in screening electrostatic interactions. However, this effect can only account for a three-fold potentiation. We propose that calcium ions affect the measured brush thickness (figure 2b) by several distinct mechanisms that come into play in the different brush regimes.

Osmotic brush regimes (low calcium concentrations). For a polyelectrolyte brush in the osmotic regime, the brush morphology has been predicted to be sensitive to the valency of added ions [47]: ions with multiple charges can substitute several monovalent ions, thus reducing the number of mobile counterions that are necessary to compensate the polymer charge within the brush and thereby diminishing the osmotic pressure of the counterion gas. Specifically, with calcium and sodium having valencies of z_Ca = 2 and z_Na = 1, respectively, the brush thickness would be expected to decrease by a factor of $\sqrt{z_{\mathrm{Ca}}/z_{\mathrm{Na}}}\approx 1.4$ for divalent counterions compared to monovalent counterions at the same molar ion concentration [48]. Our experimental data at 0.1 and 0.5 mM added salt showed a decrease in H by 1.3 and 1.5, respectively, which is in reasonably good agreement with the theoretical prediction. In addition, divalent counterions can decrease the surface potential more dramatically compared to monovalent ions [49], or may even decrease the surface charge more effectively by counterion condensation. This would result in a decrease in the electrostatic repulsion between the HA brush and the surface of the polystyrene bead, which we have seen to be important at low ionic strength.

Salted and quasi-neutral brush regimes (high calcium concentrations). The thickness of a polyelectrolyte brush is expected to scale with the Debye screening length κ⁻¹ as H ∼ κ^−2/3 in the salted regime if the thickness changes were solely driven by electrostatic screening by free ions in the solution phase [48]. The Debye length scales as ${\kappa }^{-1}\sim 0.304\sqrt{[\mathrm{NaCl}]}$ and as ${\kappa }^{-1}\sim 0.176\sqrt{[\mathrm{CaC}{\mathrm{l}}_2]}$ [49], and consequently, a reduction in thickness by a factor of (0.304/0.176)^2/3 ≈ 1.4 would be expected when replacing NaCl by CaCl₂ at a given salt concentration. Inspection of figure 2a reveals that the factor is significantly larger in our experiments (1.6 ± 0.1 between 1 and 50 mM salt). This is equivalent to our earlier statement that the three-fold increased potency of CaCl₂ to screen electrostatic interactions is much smaller than the 10-fold potentiation observed in our experiments. Several additional mechanisms may play a role in enhancing the potency of CaCl₂. First, the polymer charge may be reduced due to the condensation of calcium ions on the HA chains [22]. For polymers with monovalent charges such as HA, Manning theory [50] predicts partial condensation of counterions if the distance between charges on the polymer is smaller than zλ_B, where z is the counterion valency and λ_B = 0.71 nm the Bjerrum length. The distance of carboxyl groups on HA is 1.0 nm, implying that partial condensation is likely to ensue for calcium but not for sodium ions. Second, calcium ions may potentially also bridge carboxylic groups on HA owing to the bivalency of the calcium ions [22]. However, the stability (i.e. homogeneity and solvation) of the brush at very high calcium concentrations as observed here (figure 2) and the stability (i.e. lack of precipitation) of HA solutions at very high calcium concentrations observed by others [19] suggest that such a cross-linking effect would be rather weak. Third, calcium may reduce the intrinsic stiffness of HA. This has indeed been suggested in previous works [23,51] although the magnitude of this effect has not been quantified to our knowledge. The thickness of a polymer brush scales with the intrinsic stiffness of the polymer p as H ∼ p^1/3 [29]. A decrease in intrinsic stiffness (or equivalently, the polymer's persistence length) by 32% would hence be required to produce a thickness change by a factor 1.4/1.6 ≈ 0.88 if the intrinsic stiffness alone was to account for the impact of calcium on brush thickness beyond what is achieved by simple electrostatic screening. Obviously, the above described mechanisms may also act together, to different extents depending on the calcium concentration.

Taken together, the mechanisms underlying the 10-fold potentiation of calcium over sodium ions with regard to brush thickness variations remain to be further elucidated. Future comparative studies with different divalent ions, and possibly also monovalent and trivalent ions, may help resolving this question. It will here also be interesting to quantify the intrinsic stiffness of HA chains as a function of ion concentration and type, for example, by single chain stretching experiments [52].

The results of this study are relevant for rationalizing the basic physical properties of HA-rich matrices in biological systems. We learn that protonation of carboxyl groups affects HA brush morphology and mechanical properties only at pH values below 6.0 and is thus unlikely to be relevant under physiological conditions. Electrostatic screening, on the other hand, has a dramatic effect on HA brushes. This effect is exerted by all free ions, although with a threefold higher potency by CaCl₂ as compared to NaCl. The concentration of calcium ions in the extracellular space is of the order of 1 mM [53], and thus more than 100-fold lower than the physiological concentration of the monovalent ions (mostly Na⁺ and to a much lesser extent K⁺). Therefore, sodium ions will account for most of the electrostatic screening while the contribution of calcium ions is rather marginal, of the order of a few per cent. As discussed above, calcium clearly exerts effects additional to the electrostatic screening. In the future, it will be interesting to explore how important these additional effects are if the relatively low physiological calcium concentration is combined with the much higher physiological sodium concentrations. Naturally, the situation in native HA-rich matrices will be even more complex, for example, because HA-binding and other proteins also contribute to defining matrix assembly and dynamic reorganization, and their function may also be modulated by calcium ions. However, the simplified yet well-defined model system studied here provides an idea of the magnitude of effects that can occur and provides a reference for studies with more complex systems. Future studies should also consider if HA brushes respond in specific ways to other divalent ions (e.g. Mg²⁺ and Zn²⁺). Work with HA solutions has proposed, for instance, that zinc(II) ions coordinate with HA [54], and that copper(II) chloride may degrade HA [55], and it will be interesting to see if such effects can also be observed in HA brushes.

Our results also provide information that should be useful for the rational design of HA-based materials with tailored properties. Here, HA brushes appear particularly attractive for the development of stimuli-responsive surface coatings: they combine a large dynamic range—such as a five-fold thickness change in response to ions (figure 2) and a two-fold thickness change in response to pH (figure 4)—with a high degree of reversibility in the morphological changes, thus allowing for strong and repeatable switching by external stimuli. Changes in thickness can be exploited directly as a readout, or as a mechanical actuator, for sensing applications. Associated changes in brush concentration and hence physical properties such as viscoelasticity (figures 3c and 5c) or permeability could also be exploited for applications. An important parameter defining permeability is the correlation length ξ, a statistical measure of the distance between segments on neighbouring chains [56]. In homogeneous meshworks of flexible polymers, the correlation length is an effective measure for the mesh size, and in a good solvent, it is predicted to decrease with polymer concentration c with a power of −3/4 [57]. For a given HA brush (i.e. at constant grafting density), we have thus ξ ∼ c^−3/4 ∼ H^−3/4 to a first approximation, and a five-fold reduction in brush thickness would thus entail a more than three-fold reduction in the size of particles that can effectively permeate the HA film.

5. Conclusion

In this work, we have systematically analysed the variations in thickness (by colloidal probe RICM) and softness (by QCM-D) of HA brushes over a broad range of Ca²⁺ concentrations and pH. Within the physiologically relevant ranges of these two parameters, we found the softness and thickness of HA brushes to be exquisitely sensitive to Ca²⁺ concentrations but essentially insensitive to pH. More broadly, we discovered that the effect of divalent calcium ions on HA brush thickness is virtually identical to the effect of monovalent sodium ions at 10-fold higher concentrations and that HA brushes collapse upon protonation below pH 6.0. These findings are relevant for understanding the supramolecular organization and dynamics of HA-rich extracellular matrices and for the design of stimuli-responsive surface coatings with tailored properties.

Data accessibility

The electronic supplementary material contains methods and figures S1–S5. Figures S3–S5 represent datasets supporting this article.

Authors' contributions

R.P.R. conceived the study; X.C. and R.P.R. designed the experiments; X.C. performed the experiments; X.C. and R.P.R. analysed the data and wrote the manuscript. All authors gave final approval for publication.

Competing interests

We have no competing interests.

Funding

This work was supported by the European Research Council (Starting Grant ‘JELLY’, FP7-ERC-2012-StG-306435 to R.P.R.), the Spanish Ministry for Economy and Competitiveness (MAT2014-54867-R to R.P.R) and the UK Biotechnology and Biological Sciences Research Council (BB/R000174/1 to R.P.R.).