Abstract
Horse locomotion is remarkably economical. Here, we measure external mechanical work of the galloping horse and relate it to published measurements of metabolic cost. Seven Thoroughbred horses were galloped (ridden) over force plates, under a racing surface. Twenty-six full strides of force data were recorded and used to calculate the external mechanical work of galloping. The mean sum of decrements of mechanical energy was −876 J (±280 J) per stride and increments were 2163 J (±538 J) per stride as horses were accelerating. Combination with published values for internal work and metabolic costs for galloping yields an apparent muscular efficiency of 37–46% for galloping, which would be reduced by energy storage in leg tendons. Knowledge about external work of galloping provides further insight into the mechanics of galloping from both an evolutionary and performance standpoint.
Keywords: horse, gallop, biomechanics, energetics
1. Introduction
Like many cursors, the horse (Equus caballus) has evolved to locomote economically over long distances. The horse has a very low metabolic cost of transport [1,2] (the amount of energy consumed to cover a given distance, COT) and has been selectively bred for increased speed and endurance. Adaptive specializations for running in the horse include a distal limb that is slim and light, with an extended single digit and no musculature below the carpus and tarsus. The reduced mass of the distal fore- and hindlimbs reduces the energy required to swing the limbs between stances [3]. Further, long, elastic distal tendons allow elastic energy storage and return [4,5], contributing to economical locomotion [6,7]. Conversely, the proximal portions of the limbs are made up of large, bulky muscles which allow rapid limb swinging and propulsion with the further aid of a large tendon within the biceps muscle of the forelimb that acts as a catapult to give rapid limb protraction [8].
External work in the galloping horse has been modelled [9] and measured, through kinematics [1] and inertial sensors [10] and the published values are high, 10 500 J (515 kg at 12 m s−1) [1] and 8000 J (480 kg at 12 m s−1) [10] per stride, and exceed the metabolic cost of galloping. This mismatch has been attributed to energy-storing springs, along with effects of skin movement, movement of the centre of mass (COM) within the reference frame of the subject, digitizing errors and sensor placement. Here, we set out to make a more direct measure of external mechanical work using force plates as ergometers, as outlined elsewhere [11,12].
2. Material and methods
Data were collected from seven Thoroughbred racehorses at the British Racing School (BRS), Newmarket, UK. All subjects were weighed on the in-house scales (477 ± 25 kg) (though weights for calculations were taken as the integral of the vertical force across a stride) and limb lengths were taken to the top of the scapula (1.63 ± 0.04 m) using a standard tape measure. The same professional jockey (jockey + equipment = 70.1 kg) rode all horses for all trials.
Ten 0.6 × 0.9 m Hall-effect force plates (AMTI custom build, Watertown, MA, USA) were placed in a custom steel frame in the racing track at the BRS on a base layer of chalk to yield a 6 × 0.9 m array. Plates were covered with a membrane and protective metal/resin top-plates. Approximately 0.1 m depth of oiled sand was then layered over the plates to obscure them and provide a surface over which the horses could gallop safely. The sand was smoothed between runs. The sand compacts at low force and is then relatively firm so while a small amount of work would be performed at foot-on, this was mostly vertical—the footprints showed no evidence of horizontal foot displacement (slipping) through stance. On the left-hand side of the track were two AOS high-speed cameras (X-Pri, AOS Technologies AG, Switzerland) set to 1280 × 560 pixels, filming at 500 Hz.
Each horse was acclimatized to the set-up. Three-dimensional limb force data were collected at 500 Hz from the 10 plates. Each horse performed four to six galloping trials.
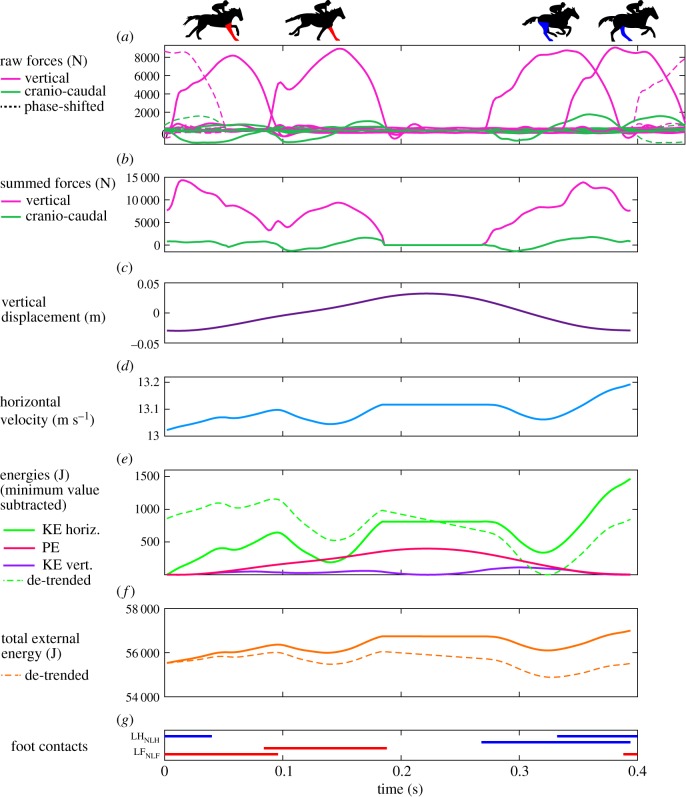
Footfall timings were taken from the high-speed video using VirtualDub (version 1.9.11), and initial velocity conditions were taken from the video using custom digitization software [13]. Raw force data were analysed in a custom-script written in MATLAB (Mathworks, Natick, MA, USA). Forces, for strides in which four complete footfalls were captured, were summed across plates with respect to time in the vertical and cranio-caudal directions. Single-stride data were cut using a custom-script written in MATLAB, using stride time from the high-speed video. Integration was stopped at foot-off to prevent force plate resonance giving spurious work calculations. In the majority of trials, there were simultaneous hoof contacts occurring during the stride of interest that were not on the plates and would therefore confound external work calculations. As such, the force traces for the four limb contacts were phase shifted, using stride time from the high-speed video, and over-lapped to account for these hoof contacts, under the assumption that horses were in consistent gallop and all strides were equivalent. This procedure is illustrated in the top panel of figure 1 and in the electronic supplementary material.
Figure 1.
Forces and energy fluctuations during a typical galloping trial (13 m s−1). (a) Raw force data (dashed lines show phase-shifted overlay), while (b) shows summed force data used in the calculations. (c) Vertical displacement of the COM in metres and (d) horizontal velocity throughout the stride. (e) Horizontal kinetic (dashed line shows de-trended data), potential and vertical energy fluctuations throughout the stride. (f) The total energy fluctuations throughout the stride, with the dashed line representing the de-trended values. Stance times are represented in (g), corresponding to the images at the top. Energy traces in (e) have had the offset of their minimum value removed.
External work was calculated by summing, separately, decrements (negative increments) and (positive) increments of potential and kinetic energy of the COM using the series of equations as outlined in the literature [11,12]. Initial velocity conditions were taken as the average velocity across the stride from the high-speed video data and the mass of the individual was taken as the average vertical force across the stride.
Horses accelerated in every recorded stride with a submaximal mean acceleration of approximately 0.45 m s−2 (maximum capacity is around 3 m s−2 [14]). This corresponds to an average net velocity increase over the stride of 0.18 m s−1, and a resultant increase in horizontal kinetic energy of 0.016 J kg−1. During galloping, COM kinetic energy should reduce early in the stance phase of each limb (owing to forward limb configuration) and such fluctuations would be ameliorated during the period of hindlimb stance by the hip torques used for acceleration. We approach the effect of the net acceleration on work calculations in two ways. One, we de-trend the acceleration by calculating the mean horizontal acceleration through the stride and subtracting that mean from the data before re-running the analysis and then calculating positive increments in external work. Two, we also sum the decrements in mechanical work in the non-de-trended data. In steady-state galloping, summing positive increments and summing decrements would yield the same work values, but with acceleration the calculated work will be higher for summed increments and may be underestimated when summing decrements, because fluctuations will be reduced by the underlying upward trend. Further notes on methods can be found in the electronic supplementary material.
3. Results
Twenty-six complete strides were used in the analysis with speeds between 10.2 and 13.1 m s−1. In 12 of the 26 trials, the non-lead forelimb contacted the plates first.
The mean (±s.d.) vertical displacement of the COM was 0.06 m (±0.02 m) and the mean fluctuation in horizontal velocity was 0.18 m s−1 (±0.07 m s−1). The mean fluctuation (amplitude) in mechanical work was 1510 J (±479 J), equivalent to 2.7 J kg−1, which was reduced to 1007 J in the de-trended data. The mean sum of positive increments of work was 2163 J (±538 J), equivalent to 3.9 J kg−1 (1537 J in the de-trended data), and the mean sum of decrements was −876 J (±280 J), equivalent to −1.6 J kg−1 (−1544 J in the de-trended data). A typical plot of COM energies during one trial (one stride) is displayed in figure 1. Data for further trials are displayed in the electronic supplementary material.
4. Discussion
Until now, the available data for external mechanical work in the galloping horse have been limited to calculations from kinematic data [1,10], resulting in values for mechanical work similar to the total metabolic energy expenditure [1,15], but much of the energy is recycled rather than dissipated and performed de novo each stride. Muscle initial efficiency of doing mechanical work is around 20–63% [16], but this is from breakdown of the existing ATP. The actual (apparent) efficiency of mechanical work determined from oxygen consumption will be considerably lower [17], but the difference has not been measured for horses. Cursorial mammals are adapted for fast and efficient locomotion [3] and explanations for this, such as energy storage in tendons [4,6,18] and minimizing energy losses [9,19] have been described.
Internal work was not calculated in this study, but published values of 2000 J per stride at 12 m s−1 exist [1]. This is approximately double the external work seen in this study when considering the decrements and close to the de-trended values. This is consistent with humans, where internal work exceeds external work at higher speeds [20]. The high proportion of total work being internal work reinforces the evolutionary selection pressure for light distal limbs and adaptive mechanisms for efficient locomotion.
To calculate apparent efficiency, we took the metabolic (oxygen) cost of galloping to be 2.5 J kg−1 m−1 [1,21], equating to a metabolic cost of transport (for a 550 kg horse) of 1375 J m−1. This would result in a cost per stride (stride frequency 2.13 s−1, so stride length 5.63 m [22]) of 7740 J (at 12 m s−1). Taking the external work (decrements) from this study of 876 J and adding internal work of 2000 J per stride at 12 m s−1 [1] gives an apparent muscular efficiency of 37%. This would require net efficiency higher than most published results for muscle (of smaller animals), which are around 25% [23]. When we consider the de-trended data, this gives an apparent efficiency value of 46%. The true muscle work will be lower because energy is stored and returned by limb tendons during stance [4] and during swing [8], hence reducing muscle contributions to internal and external work. Using the example trial shown in figure 1, we can approximate elastic strain energy from the force data, using resultant force as axial limb force and a published leg stiffness value of 55 kN m−1 [6,7]. Figure 2 shows this strain energy and the effect on total energy throughout the stride. This reduces the total positive and negative increments of work for much of the trial and shows a net positive work produced by the hindlimbs at the end of the stride.
Figure 2.
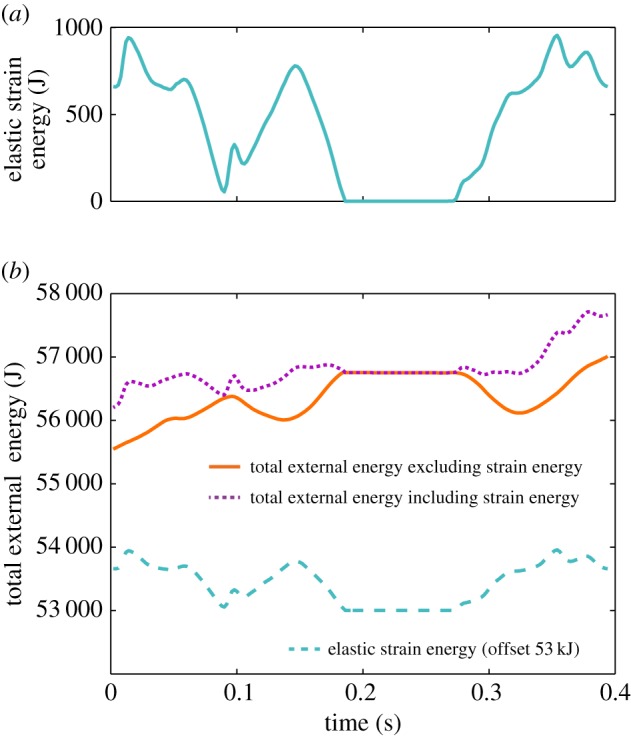
(a) Calculated elastic strain energy throughout one stride. (b) Effect of elastic strain energy on total energy (elastic strain energy is shown again here to scale but absolute values have been offset by 53 kJ).
Factoring in aerodynamic drag, at speeds (v) of 12 m s−1, the contribution of drag to COT is 0.15 J kg−1 m−1 (½ CDρ Av2/bodymass: CD = 0.9, ρ = 1.29 kg m−3, A = 1 m2, 550 kg mass), equivalent to around 464 J per stride, which is a considerable proportion of the mechanical work being performed and will increase the cost of galloping and yield a muscle efficiency of 43% (52% for de-trended data). While often considered to be negligible, this is a larger proportion of total mechanical work than previously considered, and likely explains the importance of aerodynamic drafting in winning horse races [24], especially as it is proportional to v2 and will be much higher at racing speeds (17–20 m s−1).
With regard to this study being performed on ridden horses, 13% of the total mass is the rider who, in racing posture, will add weight but limited inertia. As the rider can move horizontally somewhat out of phase with the horse's COM [25], horizontal work (by the horse) on the rider is reduced while their weight is still supported. Calculating mechanical works for horse mass alone would result in an 11% increase in mass-specific work.
Knowledge of the mechanics of galloping can give insight into the increase in total work as a result of perturbations which may impose a power limit to maximum speed. For example, moving up a 10% incline at 12 m s−1 (i.e. 1.2 m s−1 vertical velocity) equates to 706 J kg−1 min−1 (12 W kg−1) potential energy power, equivalent to approximately 3000 J per stride [26]. Given external work values from this study, galloping on a 10% incline would increase total mechanical work by over 100%, which is concomitant with the increase in metabolic cost [21,27]. Energy supply may eventually become limiting, which may become apparent from measurements of maximum speed on different gradients.
Between-trial variability is somewhat high in this dataset, which can be attributed to the nature of the set-up and excitability of racehorses. While every effort was made to ensure steady-state locomotion, horizontal kinetic energy increased in most strides, e.g. in the stride shown in figure 1; however, this only represents an increase in absolute velocity of 0.2 m s−1, which may be as close to steady state as possible for overground locomotion outside of the laboratory.
5. Conclusion
Large cursorial animals are known to be uniquely economical with a low COT. Understanding of the costs and efficiency of high-speed locomotion in large cursorial animals gives insight into how they have evolved anatomically and physiologically to meet the evolutionary selective pressures that result from ranging to find resources in open grasslands and the need for high-speed predator evasion. These adaptations underpin the metabolic and mechanical factors affecting and limiting athletic performance in racehorses.
The results show that external work is a small fraction of the total mechanical work of galloping, less than that of internal work and similar in magnitude to the aerodynamic drag costs. Apparent muscle efficiencies are of 37–46% and exceed net efficiencies, demonstrating the importance of elastic cycling of energy in limb tendons.
Supplementary Material
Acknowledgements
The authors would like to thank members of the Structure and Motion Laboratory for their assistance in the data collection, Jeffery Rankin for help with data analysis, and Richard Perham and staff at the British Racing School for their time.
Ethics
Ethical approval for this study was granted by the RVC Welfare and Ethics Committee (URN 2011 1107).
Data accessibility
Data are available from the Dryad Digital Repository: http://dx.doi.org/10.5061/dryad.db543 [28]. Further details of data analysis and additional figures have been uploaded as electronic supplementary material.
Authors' contributions
Z.T.S.D., A.J.S. and A.M.W. designed the study, collected and analysed data and wrote and revised the manuscript. All authors approved the final manuscript and agree to be accountable for all aspects of the study.
Competing interests
The authors have no competing interests to declare.
Funding
This study was funded by a BBSRC UK CASE studentship to Z.T.S.D. sponsored by Turftrax Ltd.
References
- 1.Minetti AE, Ardigò LP, Reinach E, Saibene F. 1999. The relationship between mechanical work and energy expenditure of locomotion in horses. J. Exp. Biol. 202, 2329–2338. [DOI] [PubMed] [Google Scholar]
- 2.Thornton J, Pagan J, Persson S. 1987. The oxygen cost of weight loading and inclined treadmill exercise in the horse. In Equine exercise physiology (eds Gillespie JR, Robinson NE), vol. 2, pp. 206–215. Davis, CA: ICEEP Publications. [Google Scholar]
- 3.Hildebrand M. 1988. Analysis of vertebrate structure. New York, NY: John Wiley and Sons. [Google Scholar]
- 4.Biewener AA. 1998. Muscle-tendon stresses and elastic energy storage during locomotion in the horse. Comp. Biochem. Physiol. B 120, 73–87. ( 10.1016/S0305-0491(98)00024-8) [DOI] [PubMed] [Google Scholar]
- 5.Payne RC, Hutchinson JR, Robilliard JJ, Smith NC, Wilson AM. 2005. Functional specialisation of pelvic limb anatomy in horses (Equus caballus). J. Anat. 206, 557–574. ( 10.1111/j.1469-7580.2005.00420.x) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 6.McGuigan MP, Wilson AM. 2003. The effect of gait and digital flexor muscle activation on limb compliance in the forelimb of the horse Equus caballus. J. Exp. Biol. 206, 1325–1336. ( 10.1242/jeb.00254) [DOI] [PubMed] [Google Scholar]
- 7.Wilson AM, McGuigan MP, Su A, van den Bogert AJ. 2001. Horses damp the spring in their step. Nature 414, 895 ( 10.1038/414895a) [DOI] [PubMed] [Google Scholar]
- 8.Wilson AM, Watson JC, Lichtwark GA. 2003. Biomechanics: a catapult action for rapid limb protraction. Nature 421, 35–36. ( 10.1038/421035a) [DOI] [PubMed] [Google Scholar]
- 9.Bertram JEA, Gutmann A. 2009. Motions of the running horse and cheetah revisited: fundamental mechanics of the transverse and rotary gallop. J. R. Soc. Interface 6, 549–559. ( 10.1098/rsif.2008.0328) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 10.Pfau T, Witte TH, Wilson AM. 2006. Centre of mass movement and mechanical energy fluctuation during gallop locomotion in the Thoroughbred racehorse. J. Exp. Biol. 209, 3742–3757. ( 10.1242/jeb.02439) [DOI] [PubMed] [Google Scholar]
- 11.Cavagna GA. 1975. Force platforms as ergometers. J. Appl. Physiol. 39, 174–179. ( 10.1152/jappl.1975.39.1.174) [DOI] [PubMed] [Google Scholar]
- 12.McGowan CP, Baudinette RV, Usherwood JR, Biewener AA. 2005. The mechanics of jumping versus steady hopping in yellow-footed rock wallabies. J. Exp. Biol. 208, 2741–2751. ( 10.1242/jeb.01702) [DOI] [PubMed] [Google Scholar]
- 13.Hedrick TL. 2008. Software techniques for two- and three-dimensional kinematic measurements of biological and biomimetic systems. Bioinspir. Biomim. 3, 034001 ( 10.1088/1748-3182/3/3/034001) [DOI] [PubMed] [Google Scholar]
- 14.Williams SB, Tan HL, Usherwood JR, Wilson AM. 2009. Pitch then power: limitations to acceleration in quadrupeds. Biol. Lett. 5, 610–613. ( 10.1098/rsbl.2009.0360) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 15.Hoyt DF, Taylor CR. 1981. Gait and the energetics of locomotion in horses. Nature 292, 239–240. ( 10.1038/292239a0) [DOI] [Google Scholar]
- 16.Curtin NA, Bartlam-Brooks HLA, Hubel TY, Gardner-Medwin AR, Bennitt E, Lorenc M, West TG, Wilson AM. 2018. Remarkable muscles, remarkable locomotion in desert dwelling wildebeest. Nature 563, 393–396. ( 10.1038/s41586-018-0602-4) [DOI] [PubMed] [Google Scholar]
- 17.Smith NP, Barclay CJ, Loiselle DS. 2005. The efficiency of muscle contraction. Prog. Biophys. Mol. Biol. 88, 1–58. ( 10.1016/j.pbiomolbio.2003.11.014) [DOI] [PubMed] [Google Scholar]
- 18.Alexander RM, Dimery NJ, Ker RF. 1985. Elastic structures in the back and their role in galloping in some mammals. J. Zool. 207, 467–482. ( 10.1111/j.1469-7998.1985.tb04944.x) [DOI] [Google Scholar]
- 19.Ruina A, Bertram JE, Srinivasan M. 2005. A collisional model of the energetic cost of support work qualitatively explains leg sequencing in walking and galloping, pseudo-elastic leg behavior in running and the walk-to-run transition. J. Theor. Biol. 237, 170–192. ( 10.1016/j.jtbi.2005.04.004) [DOI] [PubMed] [Google Scholar]
- 20.Cavagna GA, Kaneko M. 1977. Mechanical work and efficiency in level walking and running. J. Physiol. Lond. 268, 467–481. ( 10.1113/jphysiol.1977.sp011866) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 21.Eaton MD, Evans DL, Hodgson DR, Rose RJ. 1995. Effect of treadmill incline and speed on metabolic-rate during exercise in Thoroughbred horses. J. Appl. Physiol. 79, 951–957. ( 10.1152/jappl.1995.79.3.951) [DOI] [PubMed] [Google Scholar]
- 22.Witte TH, Hirst CV, Wilson AM. 2006. Effect of speed on stride parameters in racehorses at gallop in field conditions. J. Exp. Biol. 209, 4389–4397. ( 10.1242/jeb.02518) [DOI] [PubMed] [Google Scholar]
- 23.Heglund NC, Cavagna GA. 1985. Efficiency of vertebrate locomotory muscles. J. Exp. Biol. 115, 283–292. [DOI] [PubMed] [Google Scholar]
- 24.Spence AJ, Thurman AS, Maher MJ, Wilson AM. 2012. Speed, pacing strategy and aerodynamic drafting in Thoroughbred horse racing. Biol. Lett. 8, 678–681. ( 10.1098/rsbl.2011.1120) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 25.Pfau T, Spence A, Starke S, Ferrari M, Wilson A. 2009. Modern riding style improves horse racing times. Science 325, 289 ( 10.1126/science.1174605) [DOI] [PubMed] [Google Scholar]
- 26.Self ZT, Spence AJ, Wilson AM. 2012. Speed and incline during Thoroughbred horse racing: racehorse speed supports a metabolic power constraint to incline running but not to decline running. J. Appl. Physiol. 113, 602–607. ( 10.1152/japplphysiol.00560.2011) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 27.Wickler SJ, Hoyt DF, Cogger EA, Hirschbein MH. 2000. Preferred speed and cost of transport: the effect of incline. J. Exp. Biol. 203, 2195–2200. [DOI] [PubMed] [Google Scholar]
- 28.Self Davies ZT, Spence AJ, Wilson AM. 2019. Data from: External mechanical work in the galloping racehorse Dryad Digital Repository. ( 10.5061/dryad.db543) [DOI] [PMC free article] [PubMed]
Associated Data
This section collects any data citations, data availability statements, or supplementary materials included in this article.
Data Citations
- Self Davies ZT, Spence AJ, Wilson AM. 2019. Data from: External mechanical work in the galloping racehorse Dryad Digital Repository. ( 10.5061/dryad.db543) [DOI] [PMC free article] [PubMed]
Supplementary Materials
Data Availability Statement
Data are available from the Dryad Digital Repository: http://dx.doi.org/10.5061/dryad.db543 [28]. Further details of data analysis and additional figures have been uploaded as electronic supplementary material.