Psychological Constellations Assessed at Age 13 Predict Distinct Forms of Eminence 35 Years Later

Abstract

This investigation examined whether math/scientific and verbal/humanistic ability and preference constellations, developed on intellectually talented 13-year-olds to predict their educational outcomes at age 23, continue to maintain their longitudinal potency by distinguishing distinct forms of eminence 35 years later. Eminent individuals were defined as those who, by age 50, had accomplished something rare: creative and highly impactful careers (e.g., full professors at research-intensive universities, Fortune 500 executives, distinguished judges and lawyers, leaders in biomedicine, award-winning journalists and writers). Study 1 consisted of 677 intellectually precocious youths, assessed at age 13, whose leadership and creative accomplishments were assessed 35 years later. Study 2 constituted a constructive replication—an analysis of 605 top science, technology, engineering, and math (STEM) graduate students, assessed on the same predictor constructs early in graduate school and assessed again 25 years later. In both samples, the same ability and preference parameter values, which defined math/scientific versus verbal/humanistic constellations, discriminated participants who ultimately achieved distinct forms of eminence from their peers pursuing other life endeavors.

How early in development do promising signs for differing forms of eminence emerge? Given the ever-increasing emphasis on human capital in our conceptual economy and the importance of staying economically competitive in a “flat world” (Friedman, 2007; Rindermann & Thompson, 2011; Zakaria, 2011), this question has implications beyond a theoretical understanding of how exceptional careers develop (Lubinski & Benbow, 2000; Murray, 2003; Simonton, 2014). Studies of intellectually precocious youth have already revealed that assessing the level and pattern of abilities within the top 1% (which constitutes over one third of the ability range) is critical for understanding contrasting creative accomplishments and high-impact careers (Lubinski, 2016; Makel, Kell, Lubinski, Putallaz, & Benbow, 2016). More ability matters, as does the ability pattern. The former is related to the magnitude of accomplishment, whereas the latter is related to the nature of the fields pursued. In addition, information regarding personal preferences is also essential to accurately model the unfolding of exceptional careers that maintain and advance modern cultures.

This investigation examined the extent to which two distinct ability and preference constellations (math/scientific and verbal/humanistic) assessed at age 13 in intellectually precocious youth predict distinct forms of eminence 35 years later. In past research with intellectually precocious 13-year-olds, Achter, Lubinski, Benbow, and Eftekhari-Sanjani (1999) showed that psychometric assessments of abilities and preferences each add value to the prediction of concrete educational outcomes 10 years later. Subsequently, Wai, Lubinski, and Benbow (2005) successfully used the math/scientific and verbal/humanistic functions derived from this analysis to predict contrasting occupational outcomes 20 years later.

We do not know, however, whether these early assessments continue to maintain their longitudinal potency over subsequent decades in predicting eminence, concrete forms of creativity, and extraordinary careers. These are the rare life achievements and outcomes that few attain. Do these early assessments hold predictive validity for distinguishing, for example, full professors in different disciplines at research-intensive universities, CEOs at Fortune 500 companies, prestigious judges and lawyers, leaders in biomedicine, distinguished journalists, and award-winning writers? This article reports on whether different forms of eminence and leadership in cutting-edge careers can be anticipated by early psychological assessments of ability and preference constellations among intellectually talented youth.

Study 1 uses information from the discriminant-function analysis conducted on 432 intellectually talented 13-year-olds that used ability and preference assessments to predict contrasting college degree outcomes at age 23 (Achter et al., 1999). We applied weights derived from this analysis to determine whether the distinct constellations captured by these functions discriminate different types of eminence 35 years later.

Although Study 1 provided a compelling test of differential validity of the math/scientific and verbal/humanistic constellations derived by Achter et al. (1999) in the prediction of distinct classes of professional eminence, a constructive replication (Lykken, 1968, 1991) would afford further and more definitive support. Constructive replications vary as many of the nonessential design features of the initial study as possible while maintaining focus on the substantive constructs of interest. For example, a constructive replication of Study 1 would vary measures, sample, and time of identification while maintaining focus on the essential ability and preference and criterion constructs.

Study 2 provided such a replication. This study analyzed an independent sample of high-potential students identified at a different time, at different ages, and with different selection procedures from those of Study 1. Yet the focal constructs were conceptually equivalent. Study 2 applied the same ability and preference weights derived on intellectually precocious youth by Achter et al. (1999) to a sample of 605 top science, technology, engineering, and math (STEM) graduate students who were identified in 1992 at age 25 and assessed on the same ability and preference constructs. This study tested whether the same math/scientific and verbal/humanistic functions are generalizable to top STEM graduate students. If so, those functions should distinguish students who become genuine STEM leaders from those pursuing less distinguished STEM careers or other endeavors in life 25 years later.

Study 1

Method

Participants and procedure

Participants were drawn from the first three cohorts of the Study of Mathematically Precocious Youth (SMPY; Lubinski & Benbow, 2006). Participants were identified as 13-year-olds between 1972 and 1983 by talent searches using the SAT-Mathematical Reasoning (SAT-M) and SAT-Verbal Reasoning (SAT-V) subtests. These participants scored in the top 1% on either one or both of the measures. Only participants with complete data on the SAT-M, SAT-V, and the Study of Values (SOV; Allport, Vernon, & Lindzey, 1970) qualified for this investigation (n = 677; 433 males, 244 females: 89% Caucasian, 1% Hispanic, 1% African American, and 9% Asian American). See the Supplemental Material available online for further background characteristics of these participants, our selection methods, and analyses.

Assessment instruments at age 13

Ability

The SAT-M and SAT-V subtests were used to identify participants through talent searches conducted by SMPY between 1972 and 1983 (Lubinski & Benbow, 2006). The mean scores at age 13 were 569 (SD = 95) for the SAT-M and 450 (SD = 88) for the SAT-V. (These scores were obtained before the SAT’s 1995 recentering; at the time, the mean SAT scores for college-bound high school seniors were 500 for the SAT-M and 430 for the SAT-V.) See Lubinski and Benbow (2006) for further details.

Preferences

The SOV is an ipsative measure with six scales: Theoretical, Aesthetic, Social, Economic, Political, and Religious. Scale scores sum to 240, and there is a mean of 40 on each scale. Thus, these assessments provide an intraindividual appraisal of participants’ value orientations and approaches to life (Dawis, 1991). The SOV’s test-retest reliability for intellectually talented populations has been documented over a 20-year interval (ages 13 to 33; Lubinski, Schmidt, & Benbow, 1996). The generalizability of the SOV’s internal structure and construct validity for external criteria, ranging from hobbies, interests, personality, and preferences, has been documented for intellectually precocious adolescents and young adults (Lubinski & Benbow, 2006; Schmidt, Lubinski, & Benbow, 1998).

Criterion assessments of eminence at age 50

We defined eminence using ultimate criteria (Simonton, 2014; Thorndike, 1949, pp. 121–124). Rather than defining eminence in terms of “potential,” we wanted to identify those participants who by age 50 had attained the rare outcome of highly consequential and creative careers. To refine our criteria, we consulted with distinguished experts in engineering, law, and physics as well as with individuals having extensive knowledge of prestigious government positions (see Acknowledgments); in addition, we consulted the literature on this topic (Murray, 2003; Simonton, 2014; Zuckerman, 1977).

We classified participants as eminent if they met one of the criteria found in the three broad classes in Table 1. To secure these data, we aligned detailed Internet searches with up-to-date SMPY database personal information (e.g., last known occupation or change of names; Lubinski & Benbow, 2006). This method maximized follow-up of our entire sample of 677 participants—those who had provided complete SAT and SOV assessments at age 13—as opposed to relying solely on outcome criteria from participants responding to follow-up surveys. Of the 677, we were unable to find 27 males and 15 females in our Internet searches (we could not confirm their identities in our contact-information database). Given our method and criteria (Table 1), however, we believe it is unlikely that we missed an individual with a truly illustrious career. While tedious and time-consuming, this procedure for collecting criterion information enabled us to document objectively and with confidence the creative impact and leadership of our participants. Our reasoning was that, if someone were truly eminent, then that person should be publicly conspicuous and therefore discoverable through online searches. This is especially true given that we had extensive biographical knowledge of each participant from the initial assessment and from subsequent periodic SMPY surveys (Lubinski & Benbow, 2006).

Table 1.

Results From Study 1: Descriptive Statistics for Participants Classified as Eminent in Science, Technology, Engineering, and Math (STEM); Humanities and Social Sciences; and Other Fields (n = 83)

Group and criterion	Participants (n)			Percentage of eminent participants
	Males	Females	Total
Science, technology, engineering, and math (STEM)	37	1	38	45.8
R1 associate professor	1	0	1	1.2
R1 full professor	17	0	17	20.5
NIH/NSF grants ≥ $2.75 million	1	0	1	1.2
Fortune 500 executive	6	1	7	8.4
Number of patents ≥ 20	10	0	10	12.0
Emmy winner in visual effects	1	0	1	1.2
STEM exceptiona	1	0	1	1.2
Humanities and social sciences	10	1	11	13.3
Social sciences R1 full professors	2	1	3	3.6
Social sciences R1 associate professors	2	0	2	2.4
Humanities R1 full professors	2	0	2	2.4
Humanities R1 associate professors	2	0	2	2.4
Pulitzer Prize winner	1	0	1	1.2
Humanities exceptionb	1	0	1	1.2
Other	25	9	34	41.0
Law professors, partners at major firms, judges	8	2	10	12.0
Financial executives, hedge fund founders	4	0	4	4.8
Medical professors, directors of major divisions in hospitals	11	6	17	20.5
Military leadership	1	0	1	1.2
National cable channel executive	0	1	1	1.2
CEO of major health care organization	1	0	1	1.2

Note: R1 = research-intensive university; NIH = National Institutes of Health; NSF = National Science Foundation.

^aThe STEM exception was a professor in a small honors engineering college with a visiting position at a top STEM university in the United States. This individual is the author of several STEM books and is highly visible as an online STEM educator. ^bThe humanities exception was a professor in a non-research-intensive college with a publication record comparable with that of other individuals in the humanities/social-sciences group.

We used Publish or Perish software (Harzing, 2007) to collect information on each participant’s number of publications, number of patents, and h-index (an indication of scholarly influence based on the frequency with which an article is cited and the productivity of the author). When possible, we cross-referenced these Publish or Perish results with other online information (e.g., Research Gate, Microsoft Academic, participant’s website, participant’s curriculum vitae). We also searched the National Institutes of Health (NIH) and National Science Foundation (NSF) databases for the number of grants and amount of grant funding awarded to participants. Information on these accomplishments is in Table 1.

Of the initial 677 participants, 12.3% were deemed eminent and placed in three classes: humanities/social sciences, STEM, and other (e.g., Fortune 500 CEOs, leaders in medicine, prestigious judges and lawyers). Two participants classified as eminent had accomplishments that were exceptions to the three broad classes of criteria in Table 1. They were classified as eminent on the basis of our judgment combined with the expertise of our consultants; we list their accomplishments in the Table 1 note.

Background and analysis

Achter et al. (1999) showed that among intellectually precocious youth (the top 1% in ability), assessing preferences adds value to ability assessments in the prediction of college degree categories 10 years later. Using a group of 432 intellectually talented youth who were assessed on the SAT and SOV at age 13, Achter et al. conducted a discriminant-function analysis to predict three classes of four-year college degrees: humanities/social sciences, STEM, and other. They found that SAT-M and SAT-V scores accounted for 10% of the variance among these educational outcomes, and when the SOV was added to abilities, an additional 13% of the variance was accounted for. The discriminant-function structure matrix derived in this analysis is shown in Figure 1.

Fig. 1.

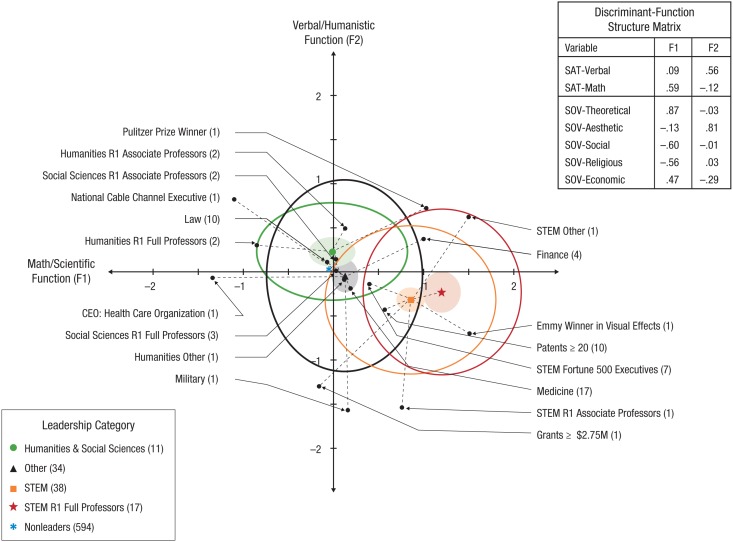
Results from Study 1: bivariate means for the math/scientific function and the verbal/humanistic function for the eminence/leadership categories. Three major categories of eminence are graphed: science, technology, engineering, and math (STEM); humanities/social sciences; and other disciplines. A subset of the STEM group—full professors in research-intensive universities (R1s)—is also graphed. Surrounding these major centroids are ellipses indicating ±1 SEM (inner, shaded ellipse) and ±1 SD (outer, open ellipse) for scores for both functions. Sample sizes appear in parentheses. Broken lines connect idiographic data points to their major centroids. Values in the structure matrix represent correlations between the discriminant functions and the measures of abilities and preferences derived from the Achter, Lubinski, Benbow, and Eftekhari-Sanjani (1999) study. SOV = Study of Values.

Achter et al. (1999) labeled the two functions in Figure 1 math/scientific (salient positive loadings on mathematical ability and theoretical values; salient negative loadings on social and religious values) and verbal/humanistic (salient positive loadings on verbal ability and aesthetic values). In addition to the Achter et al. 10-year follow-up of educational outcomes, which developed these functions, Wai et al. (2005) applied this same structure matrix to 511 SMPY participants in their 20-year follow-up. Wai et al. (2005) used the same weights to determine whether the math/scientific and verbal/humanistic functions would discriminate three conceptually distinct occupational categories, mirroring those of Achter et al. They found that the weights derived by Achter et al. to predict educational outcomes did generalize to the occupational outcomes 20 years later. In the current investigation, we examined participants 35 years after they were initially assessed. Would the Achter et al. (1999) math/scientific and verbal/humanistic functions maintain their longitudinal potency by distinguishing distinct and rare forms of eminence 35 years later?

Results

Using standardized SAT and SOV scores in the full data set, we computed the math/scientific and verbal/humanistic function scores for all 677 participants. Three broad categories for the 83 participants classified as eminent were then formed: humanities/social sciences (n = 11), STEM (n = 38), and other (n = 34). Bivariate points on these two functions are plotted in Figure 1 for all three eminent groups and the remainder of our participants. Because of the rarity of these outcomes, we also plotted data for the individual constituents in each group. For constituents occupied by multiple participants, bivariate points were averaged and their sample sizes included in Figure 1 in parentheses; these constituents connect to their broad categories with dotted lines. For example, the bivariate location of a Pulitzer Prize winner (n = 1) is connected to its broader centroid (humanities/social sciences). This affords both an idiographic and normative depiction of these outcomes through a qualitative and quantitative mixed-methods approach. Finally, because of the current interest in STEM leadership in the professoriate, a fourth centroid was plotted for full professors in STEM at research-intensive universities (n = 17). Correlations among all variables used in the analyses and background variables can be found in Table S4 in the Supplemental Material.

Few of the 83 eminent participants are located in the lower-left quadrant of the two-dimensional space defined by these functions. Eminent participants typically score high on one or both of these functions relative to the total sample. This is likely due to two factors: the relationship between individual differences (within the top 1% in ability) and more impressive accomplishments (Lubinski, 2016; Makel et al., 2016; Park, Lubinski, & Benbow, 2008) and the fact that each function has a salient weight on either mathematical ability (math/scientific function) or verbal ability (verbal/humanistic function).

The math/scientific function clearly separates the STEM leaders from the noneminent participants, d = 0.95, 95% confidence interval (CI) = [0.62, 1.28], t(630) = 5.56, p < .001. They are psychologically removed from the eminent groupings of humanities/social sciences, d = 0.97, 95% CI = [0.29, 1.66], t(47) = 2.75, p = .008, and other, d = 0.81, 95% CI = [0.34, 1.28], t(70) = 3.41, p = .001. However, the full professors in STEM at research-intensive universities are especially distinguished on this function from the noneminent participants, d = 1.35, 95% CI = [0.87, 1.83], t(609) = 5.20, p < .001; they were also distinguishable from the humanities/social-sciences, d = 1.42, 95% CI = [0.62, 2.21], t(26) = 3.64, p = .001, and other, d = 1.23, 95% CI = [0.64, 1.83], t(49) = 4.18, p < .001, eminent participants.

The humanists/social scientists are more distinct on the verbal/humanistic function relative to the noneminent participants and other groupings of eminence. Differences on this function between the eminent humanities/social sciences and STEM, other, and the noneminent were, respectively, d = 0.77, 95% CI = [0.08, 1.46], t(47) = 2.03, p = .048; d = 0.32, 95% CI = [–0.38, 1.02], t(43) = 0.80, p = .426; and d = 0.26, 95% CI = [–0.34, 0.86], t(603) = 0.69, p = .491. Individuals who are eminent in the other disciplines show a relatively balanced profile between the math/scientific and verbal/humanistic functions, reflecting the need for balance in the fields of medicine, law, and finance. These results suggest that psychological assessments of ability and preference constellations among intellectually talented youth possess differential predictive validity for distinct forms of eminence decades later.

Study 1’s results are psychologically meaningful and promising. Each of the three groups achieving eminence occupied a distinct region of the space defined by the math/scientific and verbal/humanistic functions in psychologically interpretable ways. However, replication is needed (Open Science Collaboration, 2015). The criterion groupings of eminence for Study 1, while impressive in terms of accomplishments, are composed of relatively small numbers of individuals because of the rarity of these accomplishments: only 11 individuals in the humanities/social-sciences grouping, 38 in the STEM grouping, and 34 in the other grouping. Study 2 was designed to test the robustness of Study 1’s findings.

Study 2

Study 2 was designed as a generalization probe. It analyzed another high-potential sample identified as having promise for eminence but using different criteria. In 1992, SMPY surveyed a group of 714 STEM graduate students attending 1 of the top 15 STEM graduate training programs in the United States (Lubinski, Benbow, Shea, Eftekhari-Sanjani, & Halvorson, 2001). Of these participants, 605 had complete data available on the SOV along with GRE score reports: GRE-Quantitative (GRE-Q) and GRE-Verbal (GRE-V). This provided the opportunity for constructive replication (Lykken, 1968, 1991).

In Lykken’s (1968) three-tiered nomenclature for conducting replications—literal, operational, and constructive—the latter is the most scientifically compelling. Constructive replications vary as many of the irrelevant design features of the initial study as possible, while the focal constructs under analysis remain the same. For Study 2, we drew on a different sample at promise for eminence, measured at different points in time, and used unique measures (the GRE rather than the SAT) but the same ability and preference constructs. The focus of Study 2 was to determine whether the same ability and preference constellations—math/scientific and verbal/humanistic—and weights developed on intellectually precocious youth, differentiated contrasting outcomes among a group of elite STEM graduate students. Can these functions distinguish those who would ultimately become eminent STEM leaders from those pursuing more typical STEM careers or other endeavors 25 years after they were initially identified and assessed?

Method

Participants and procedures

Study 2 consisted of 605 participants (313 males, 292 females; 82.2% Caucasian, 2.2% African American, 2.3% Hispanic, 10.1% Asian, and 3.2% other) who were drawn from SMPY’s Cohort 5 (Lubinski & Benbow, 2006; McCabe, Lubinski, & Benbow, in press). They were identified in 1992 as first- and second-year graduate students who were enrolled in STEM doctoral programs ranked among the top 15 in the United States (according to the National Research Council; see Lubinski et al., 2001, p. 301). Their mean age at the time they were identified and assessed was 24.5 years (SD = 1.7 years). Only participants with complete SOV profiles and GRE score reports were included in this study.

Criterion assessments of STEM eminence at age 50

The same criteria employed in Study 1 for identifying eminence in STEM were utilized in Study 2 (see Table 2). In addition, five individuals who did not meet any of the criteria found in Table 2 were included in our grouping of STEM eminence. In our judgment, combined with that of our consultants, their accomplishments warranted inclusion; their level of stature and impact is detailed in the Table 2 note. Of the 605 participants, 124 (20.5%) were deemed eminent in STEM. Using standardized GRE and SOV scores from the full sample, we computed scores on the math/scientific and verbal/humanistic functions for all 605 participants (Fig. 2).

Table 2.

Results From Study 2: Descriptive Statistics for Participants Classified as Eminent in Science, Technology, Engineering, and Math (STEM; n = 124)

STEM leader criterion	Participants (n)			Percentage of eminent participants
	Males	Females	Total
Tenured professor at R1 or international equivalent	40	21	61	49.2
Associate professor	8	7	15	12.1
Full professor	32	14	46	37.1
STEM Fortune 500 company senior executive	2	2	4	3.2
Senior position in government ≥ GS-14 or equivalent scale	6	7	13	10.5
GS-14	0	1	1	.8
GS-15	2	4	6	4.8
Executive scale	0	1	1	.8
Other scales	4	1	5	4.0
Patents ≥ 20	12	5	17	13.7
Publications ≥ 75	35	15	50	40.3
Median number of publications			60
Median h-index			22
NIH/NSF grants ≥ $2.75 million	31	12	43	34.7
Median grant number			3
Median grant total			$825K
Othera	3	2	5	4.0

Note: Individuals who met multiple criteria (e.g., a full professor with 75 or more publications and government funding of at least $2.75 million) were counted in all categories for which they met the criteria. R1 = research-intensive university; NIH = National Institutes of Health; NSF = National Science Foundation.

^aThe “other” group includes STEM leaders who are exceptions to the six criteria. These include an astronaut, a researcher who has multiple Nature and Science publications and over $3 million in nongovernment grants, a senior executive at a company that works on high-impact government projects, a full professor at a higher-research-activity university (R2) with both publication and funding totals just below our cutoffs, and a research supervisor in a national research laboratory who has over 60 publications.

Fig. 2.

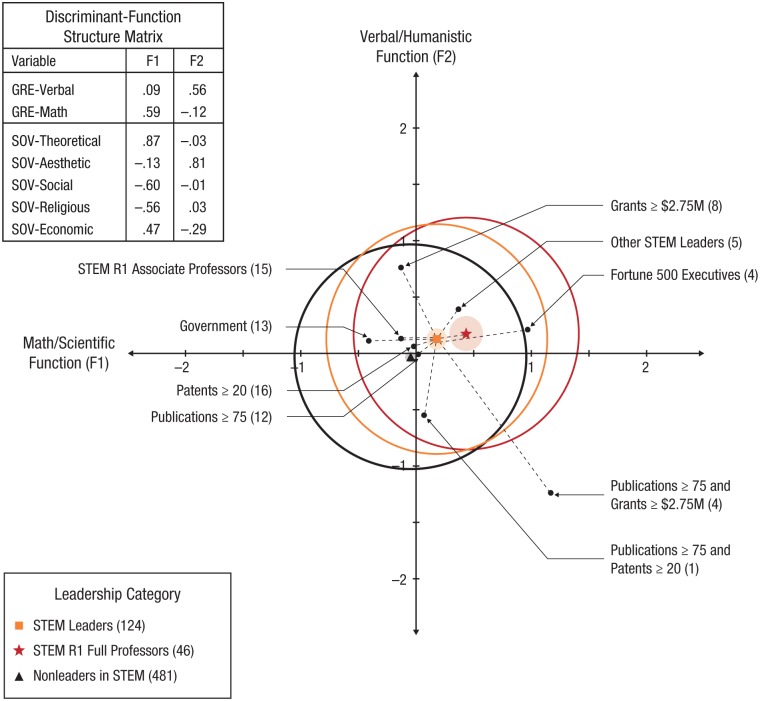
Results from Study 2: bivariate means for the math/scientific function and the verbal/humanistic function plotted for three groups: science, technology, engineering, and math (STEM) leaders, non-STEM leaders, and a subset of STEM leaders (full professors in research-intensive universities, or R1s). Surrounding these major centroids are ellipses indicating ±1 SEM (inner, shaded ellipse) and ±1 SD (outer, open ellipse) for scores for both functions. Sample sizes for constituent categories appear in parentheses; those for major groupings are in the key. Broken lines connect constituent data points comprising the STEM leaders grouping. Values in the structure matrix represent correlations between the discriminant functions and the measures of abilities and preferences derived from the Achter, Lubinski, Benbow, and Eftekhari-Sanjani (1999) study. SOV = Study of Values.

Results

Figure 2 shows the math/scientific and verbal/humanistic bivariate means of participants in Study 2 who became eminent in STEM relative to their peers who did not meet our criteria for STEM eminence. Individuals who became full professors in STEM disciplines at research-intensive universities, a subset of those deemed eminent in STEM, are also plotted separately. For idiographic detail, we plotted subsets of the constituents of the STEM leaders to reveal in which regions of the graph they fell. Correlations among all variables used in the analyses and background variables can be found in Table S4.

Figure 2 shows that those individuals who achieved eminence in STEM occupy higher status on the math/scientific function relative to their graduate student peers, d = 0.23, 95% CI = [0.03, 0.43], t(603) = 2.25, p = .025; this is particularly true for those who went on to become full professors in STEM at research-intensive universities, d = 0.49, 95% CI = [0.18, 0.79], t(525) = 3.11, p = .002. Once again, the longitudinal potency of these early assessments and the robustness of the weights derived on intellectually precocious youth (Achter et al., 1999) are demonstrated. The statistically and substantively meaningful differences on the math/scientific function show that even among these highly select graduate students, this function isolates those with outstanding careers in STEM from the remainder of their peers. This finding on the math/scientific function, combined with the absence of meaningful differences on the verbal/humanistic function, forms a clear convergent/discriminant pattern. Because the functions used in this analysis held up in a different population of high-potential students at different time points and with different ability measures (GRE vs. SAT), Study 2 fulfills the requirements of a constructive replication. Moreover, because the same weights were used in Study 1 and Study 2, Study 2 constitutes a constructive replication with a cross-validation component embedded in the design, which adds confidence to the robustness of these functions.

Discussion

Contrasting ability and preference constellations among intellectually talented young adolescents give rise to distinct forms of eminence by age 50. Past research has shown that math/scientific and verbal/humanistic functions, whose weights were based on ability and preference assessments at age 13 and calibrated against educational outcomes at age 23 (Achter et al., 1999), generalize to occupational outcomes at age 33 (Wai et al., 2005). The current research shows that these same functions maintain longitudinal potency over more distal stages of development. Among intellectually precocious youths, they distinguish different forms of creativity and eminence 35 years later. They are also psychologically interpretable in meaningful ways. For example, these two functions provide a psychological basis to the validity of C. P. Snow’s (1959) original formulation of the “two cultures”—that is, a humanistic versus a scientific orientation to the nature of the world and universe. These two functions appear to operate by structuring qualitatively different accomplishments over the course of participants’ professional lives.

In Study 1 (Fig. 1), intellectually precocious youths who ultimately achieved eminence in the humanities and the social sciences scored higher on the verbal/humanistic function at age 13 relative to their peers who became eminent in STEM fields and other fields. Moreover, participants in the eminent STEM group scored highest on the math/scientific function; at age 13, they were nearly a full standard deviation above the norm of their intellectual peers on this function. Given the current interest in the development of STEM leaders in the professoriate (Ceci, Ginther, Kahn, & Williams, 2014; Ceci & Williams, 2011; Gino, Wilmuth, & Brooks, 2015), and because sample sizes made this a reasonable statistic to compute, a separate bivariate point was plotted for the 17 full professors in STEM fields. At age 13, this subsample of STEM leaders was 1.2 standard deviations above the norm of intellectually precocious youths on the math/scientific function.

The math/scientific function appears to be especially well suited for identifying intellectually precocious youths who hold promise for exceptional STEM accomplishments. Study 2, a constructive replication, supports this conclusion.

In Study 2 (Fig. 2), the same math/scientific and verbal/humanistic function weights were applied to a sample of 605 top STEM graduate students assessed on the GRE and the SOV when they were in their mid-20s. When their professional status was evaluated 25 years later, 124 (20.5%) were deemed eminent in STEM. As young graduate students, the effect-size difference between those ultimately achieving eminence in STEM and the remainder of their peers was significant on the math/scientific function. Further, the effect-size difference between the full professors in STEM at research-intensive universities and the non-STEM leaders was even more distinctive. In this highly select sample, the math/scientific function, initially developed on intellectually precocious 13-year-olds, captured meaningful individual differences among top STEM graduate students assessed at age 25.

Collectively, Study 1 and Study 2 suggest that early signs of different kinds of eminence are detectable in high-potential populations early in their development. However, given the limitations of Study 1 and Study 2, these findings could be more compelling than they initially appear. For example, in Study 1, the average SAT-M scores of the future STEM leaders and the full professors in STEM at research-intensive universities were 636 and 671, respectively. Individual-differences assessments are compromised when a scale’s mean is within 2 standard deviations of its ceiling (Lubinski & Humphreys, 1990), and these two values are 1.4 and 1.2 standard-deviation units, respectively, from the SAT-M’s top possible score (800). The mean SAT-M score for the non-STEM leaders in Study 1 was 563.8 (2.6 SD units away from the SAT-M’s ceiling, which is psychometrically adequate). In Study 2, the GRE-Q scores for the STEM leaders and the full professors in STEM at research-intensive universities were even more problematic: 748 and 757, respectively. Here, both scores are less than a standard deviation (0.95 and 0.91, respectively) from the top possible GRE-Q score (800). The mean GRE-Q for the non-STEM leaders in Study 2 was 740 (1.0 SD unit below the GRE-Q’s ceiling and thus still psychometrically compromising).

This investigation is limited in yet another way. Research suggests that, after mathematical-reasoning ability, spatial ability is the most important intellectual attribute for developing expertise and distinction in STEM (Kell, Lubinski, Benbow, & Steiger, 2013; Uttal et al., 2013; Wai, Lubinski, & Benbow, 2009). Further, relatively lower spatial scores are characteristic of people excelling in non-STEM disciplines and help refine predictions among them (Kell et al., 2013; Wai et al., 2009).

Because quantitative reasoning ability was not assessed in its full scope and spatial ability was neglected entirely, the psychological distances between the eminence groupings in Study 1 and Study 2 are probably underestimated. Given these constraints, the findings in Figures 1 and 2 should be viewed in terms of their overall pattern and functional form (Steen, 1988), which Meehl (1990) has suggested are more important than precise estimates of statistical significance during the early stages of model testing. Future studies employing measures of the constructs utilized here with appropriate ceilings and assessments of spatial ability are likely to isolate these groups even more clearly. With larger samples, additional refinements become possible; for example, the psychological differences between exceptional engineers and physical scientists, or between outstanding social scientists and writers, might be discernable.

Psychological models in education (Corno et al., 2002) and the world of work (Dawis, 1991; Dawis & Lofquist, 1984) have stressed the importance of jointly assessing ability and preference amalgams for modeling performance in learning and occupational environments. Individual differences in the constituents of these “trait complexes” (R. E. Snow, Corno, & Jackson, 1996), “taxons” (Dawis & Lofquist, 1984), or “trait clusters” (von Stumm & Ackerman, 2013) reflect differential promise and are critical for modeling contrasting developmental trajectories (Roberts, Caspi, & Moffitt, 2003; Scarr, 1996). In this investigation, assessing these constellations among intellectually talented youths was found to be meaningful as early as age 13. Early signs of different kinds of creativity and eminence that emerge decades later are evident. Furthermore, the predictive validity of ability and preference patterns of intellectually talented adolescents generalizes to top STEM graduate students. These constellations distinguish individuals who ultimately become STEM leaders from their graduate-student peers. In sum, individuals who hold promise for distinct forms of creativity and eminence appear to manifest different psychological signs of such promise early in life.

Conclusion

Developing sufficient expertise to advance knowledge and achieve eminence is a lifelong process. Creative advances and significant careers do not happen independently of one’s individuality or opportunity; they emerge over time among individuals with the ability and passion to embrace opportunity at each developmental milestone and make the most of that opportunity. Each developmental stage builds on skills mastered in previous phases (education → occupation → creativity/eminence), yet each stage also brings unique challenges that can redirect and refine professional expertise for more noteworthy accomplishments. For intellectually talented youths and other promising populations, ability and preference constellations early in life do affect how they respond to opportunities, and some do embrace the challenge of developing extraordinary careers. By the time children are 13 years old, we can predict who is likely to become eminent and the ways in which their eminence is likely to be expressed in modern economies fueled by innovative products and ideas.