Abstract
The conformational properties of flexible and semiflexible polymers exposed to active noise are studied theoretically. The noise may originate from the interaction of the polymer with surrounding active (Brownian) particles or from the inherent motion of the polymer itself, which may be composed of active Brownian particles. In the latter case, the respective monomers are independently propelled in directions changing diffusively. For the description of the polymer, we adopt the continuous Gaussian semiflexible polymer model. Specifically, the finite polymer extensibility is taken into account, which turns out to be essential for the polymer conformations. Our analytical calculations predict a strong dependence of the relaxation times on the activity. In particular, semiflexible polymers exhibit a crossover from a bending elasticity-dominated dynamics to the flexible polymer dynamics with increasing activity. This leads to a significant activity-induced polymer shrinkage over a large range of self-propulsion velocities. For large activities, the polymers swell and their extension becomes comparable to the contour length. The scaling properties of the mean square end-to-end distance with respect to the polymer length and monomer activity are discussed.
Keywords: semiflexible polymer, active Brownian particle, active polymer, polymer conformations, polymer dynamics
1. Introduction
A distinctive characteristic of active matter is the conversion of internal chemical energy into, or utilization of energy from the environment for, directed motion [1,2,3,4,5,6,7,8,9]. The spectrum of biological active systems is wide and ranges from the macroscopic scale of flocks of birds and mammalian herds [3], the cytoskeleton in living cells [2,5,10,11,12,13,14,15,16,17], down to moving bacteria [2,6,18] on the micrometer scale. Thereby, nature employs various propulsion strategies. Bacteria are typically propelled by helical flagella [6,18,19,20,21]. The actin filaments of the cytoskeleton are driven forward by molecular motors [5,14,15,16,17,22]. Alike, microtubules in motility assays are propelled by surface-bound dyneins [23]. For synthetic active particles, chemical or physical propulsion mechanism are exploited [24,25,26,27].
Various features are common to all active systems [28], and the challenge of a theoretical description is to find a suitable approach capturing these characteristics. Generically, the activity-induced hydrodynamic flow field of a microswimmer is described by a force dipole [1,29,30]. Experiments, theoretical calculations and computer simulations, e.g., for Escherichia coli bacteria [30,31,32,33,34] and Chlamydomonas reinhardtii algae [31,32,35,36] confirm such a description for the far-field flow. However, the near-field flow can be distinctively different from the flow field of a force dipole [31,32,34,35,36].
Microswimmers are often described as active Brownian particles (ABPs) [4,24,28,37,38,39,40,41,42], neglecting hydrodynamics. This minimal stochastic model already yields interesting propulsion and excluded volume-induced emerging structures [4,38,39,40,41]. Moreover, ABPs are an extremely useful model to unravel the out-of-equilibrium statistical features of active systems [43,44,45,46,47,48,49,50,51].
The properties of connected active particles, such as linear chains [28,52,53,54,55,56,57,58,59,60,61,62,63,64,65,66] or other arrangements [67], are particular interesting systems, because of the coupling of their conformational properties and propulsion. Similar to external forces, the intrinsic activity leads to significant conformational changes, as shown in [28,57,68]. In this context, we also like to mention the conformational modulations of polymers embedded in a bath of active Brownian particles [69,70]. Activity also affects other polymer properties. An example is the linear viscoelastic response of an entangled, isotropic solution of semiflexible polymers as a model systems for myosin-driven actin filaments [52]. Here, activity leads to novel time-dependent regimes of the shear modulus. Other aspects are emerging beat patterns [54], activity-induced ring closure [53,71], aggregation of individual polymers in two dimensions [57] and collective phenomena [55]. Moreover, the internal dynamics of active dumbbells [28] and polymers [56,71] has been addressed. The influence of hydrodynamic interactions on the dynamical properties of active polymer properties have been analyzed in [59,60,62,72].
The (theoretical) analysis of the nonequilibrium behavior of flexible and semiflexible polymers, e.g., under shear flow [73,74,75,76,77,78] or during stretching [79,80,81,82,83,84,85,86,87,88,89,90,91,92,93], reveals the paramount importance of the finite polymer extensibility. We expect this intrinsic polymer property to be essential also for polymers comprising active monomers. Most theoretical studies have neglected finite polymer extensibility [56,68,71]. Only in the analytical treatment of the dynamics of an active dumbbell in [28] has the finite extensibility been taken into account and its fundamental importance for the dumbbell dynamics been demonstrated.
In this article, the conformational properties of flexible and semiflexible active Brownian polymers (ABPO) are studied analytically. Thereby, we consider a polymer composed of active Brownian particles, which are assembled in a linear chain. The diffusive motion of the propulsion velocity of the monomers is described by a Gaussian, but non-Markovian process. The emphasize is on the conformational properties due to the intimate coupling of the entropic polymer degrees of freedom and the activity of the monomers. We adopt the Gaussian semiflexible polymer model [82,94], which allows us to treat the problem analytically. As an important extension to previous studies, we account for the finite polymer extensibility and demonstrate that it strongly affects the out-of-equilibrium properties of an active polymer. Evaluation of the polymer relaxation times shows a drastic influence of that constraint on the polymer dynamics. In general, the relaxation times decrease with increasing activity, whereby the decline is more pronounced for stiffer polymers. Here, activity induces a transition from semiflexible-polymer behavior, determined by bending elasticity, to the entropy-dominated behavior of flexible polymers with increasing activity. Correspondingly, the conformational properties depend on activity. In the simpler case of flexible polymers, activity leads to their swelling over a wide range of activities. Thereby, the dependence on activity is very different from the theoretical prediction of a Rouse model [68]. Interestingly, semiflexible polymers exhibit an activity-induced shrinkage. However, for large activities the polymer conformations are ultimately comparable with those of flexible polymers. The shrinkage of active polymers in two dimensions has been observed by simulations in [68]. However, that shrinkage is due to excluded-volume effects and is unrelated to our observations for semiflexible polymers, where excluded-volume interactions are negligible.
Our theoretical considerations shed light on the nonequilibrium properties of semiflexible polymers and underline the importance of an adequate description already for moderate activities. Models without the constraint of a finite contour length, e.g., the standard Rouse model [95], would by no means be able to reproduce and capture the correct structural and dynamical aspects.
2. Model of Active Polymer
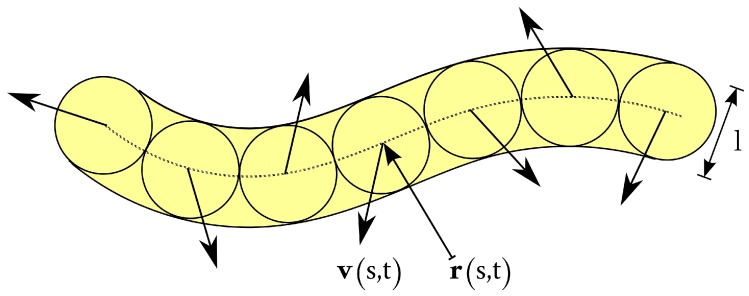
We adopt a mean-field model for a semiflexible polymer [82,94,96,97,98,99], which is denoted as Gaussian semiflexible polymer (GSFP), complemented by the activity of the monomers (GSFAP). We describe the GSFP as a continuous, differentiable space curve , where s () is the contour coordinate along the chain of length L and t is the time. Activity is added by assigning the self-propulsion velocity to every point , as typical for active Brownian particles (cf. Figure 1) [6,7,8,38,39,41]. The equation of motion of the GSFAP is then given by the Langevin equation [78,100,101,102,103]:
| (1) |
with the boundary conditions:
| (2) |
Figure 1.
Model of the continuous semiflexible active polymer.
The terms with the second and fourth derivative in Equation (1) account for the entropic degrees of freedom and bending restrictions, respectively. Formally, the entropic part looks like a stretching energy due to harmonic bonds along the polymer contour with and as the Hookean spring constants [79,104] of the continuous chain. In the following, we will denote λ and as stretching and ϵ as the bending coefficient. Note that λ and are in general different due to the broken symmetry at the chain ends. The stochastic force is assumed to be stationary, Markovian and Gaussian with zero mean and the second moments:
| (3) |
where T is the temperature, the Boltzmann constant, γ the translational friction coefficient per length and . The Lagrangian multipliers λ, and ϵ are determined by constraints [80,82]. In general, we find and for a polymer in three dimensions, where p is related to the persistence length via [80,82], i.e., the bending coefficient is solely determined by the persistence length, as is well known [103,105,106]. In Equation (1), we apply a mean-field value for the Lagrangian multiplier λ. Strictly, we expect the Lagrangian multiplier to depend on the contour coordinate for the active system, because, as shown in [76,78,80,82,83], λ strongly depends on the presence of an external force, i.e., , since it is determined by the local inextensibility condition . However, in Equation (1), we neglected this aspect and assume that λ is constant along the polymer contour. Hence, we imply the global constraint of a finite contour length:
| (4) |
corresponding to a mean-field approach. As a consequence, the polymer conformations may be inhomogeneous along its contour as, e.g., in the stretching of the GSFP [82]. However, the full solution of a discrete free-draining polymer model with individual Lagrangian multipliers for every bond and bond angle [80,82,94] yields expectation values for global quantities, such as viscosity, which deviate only very little from those determined with the constraint (4) in the limit of a nearly continuous polymer. Hence, the solution of the equations of motion with the constraint (4) suffices for many practical purposes.
We regard the self-propulsion velocity as a non-Markovian stochastic process in time with the correlation function:
| (5) |
Here, is the magnitude of the propulsion velocity and the damping factor of the rotational motion. The velocity correlation function arises, on the one hand, from the independent stochastic process for the propulsion velocity:
| (6) |
where is a Gaussian and Markovian stochastic forces with zero mean and the second moment:
| (7) |
in three dimensions; is the rotational diffusion coefficient. On the other hand, the correlation function (5) also follows for the active force , with a constant self-propulsion velocity and the unit vector e of the propulsion direction, where e performs a random walk according to [6,8,28,51]:
| (8) |
Here, is a Gaussian and Markovian stochastic process with zero mean and the second moment:
| (9) |
Since we will need and apply only the correlation function (5) in the following, the exact nature of the underlying process is irrelevant and our considerations apply for both type of processes.
Note that the continuum representation of the semiflexible polymer requires introducing a length scale l in Equations (5) and (7). With a touching-bead model in mind for a discrete polymer, this minimum length corresponds to the bead diameter and bond length of that model (cf. Figure 1). Strictly speaking, l is a free parameter in the continuum model. For a flexible polymer, we regard as the Kuhn length [107,108].
In the above description, we consider the velocity v as an intrinsic property of the active polymer. However, we may also consider v as an external stochastic process with an exponential correlation (colored noise) [6,8,28,71]. Such a correlated noise may be exerted by active Brownian particles on an embedded polymer [63,69,70].
3. Solution of Equation of Motion
To solve the equation of motion (1), we apply an eigenfunction expansion in terms of the eigenfunctions of the eigenvalue equation [76,100]:
| (10) |
The resulting eigenfunctions are given by [76,100]:
| (11) |
| (12) |
| (13) |
with:
| (14) |
The s follow from the normalization condition, and the wave numbers and are determined by the boundary conditions (2). describes the translational motion of the whole molecule.
Inserting the eigenfunction expansions:
| (15) |
into Equation (1) yields the equation of motion for the mode amplitudes :
| (16) |
with the relaxation times:
| (17) |
The stationary-state solution of Equation (16) is:
| (18) |
The time correlation functions of the mode amplitudes, which are useful in the further analysis, are obtained as , with [28]:
| (19) |
4. Results
4.1. Center-of-Mass Motion
The center-of-mass position is given by [100,102]:
| (20) |
With the solution of Equation (16) for the zeroth’s mode:
| (21) |
we obtain the center-of-mass mean square displacement:
| (22) |
As for an active Brownian particle, the term linear in time on the right-hand side accounts for the translational Brownian motion [6]. As a generalization, the total friction coefficient appears. The second term represents the contribution of activity. Again, it is similar to the term appearing for ABPs, aside from the ratio . We can identify the latter as the number of frictional sites or monomers N of diameter l, i.e., . Then, corresponds to an ABP with the friction coefficient and to a dumbbell [28,109].
The long-time diffusion coefficient follows as:
| (23) |
with the diffusion coefficient of a passive polymer, the Péclet number and the ratio Δ of the diffusion coefficients [6,28,110]:
| (24) |
Here, we introduce the diffusion coefficient as the diffusion coefficient of a segment of length l (cf. description of the model on page 3). In the following, we use the thermal translational and rotational diffusion coefficients of spherical particles of diameter l in solution, which yields .
4.2. Lagrangian Multiplier: Stretching Coefficient
Inextensibility is a fundamental property of a polymer and determines its conformational and dynamical characteristics. Hence, we have to calculate the Lagrangian multiplier λ first in order to relate other polymer aspects to the constraint Equation (4). Insertion of the eigenfunction expansion (15) for the position into Equation (4) yields:
| (25) |
which determines the Lagrangian multiplier λ. In terms of the Péclet number and Δ of Equation (24), this equation can be expressed as:
| (26) |
with the abbreviation:
| (27) |
Here, we introduce the Lagrangian multiplier μ via the relation , i.e., μ is the ratio between the stretching coefficients of the active and the passive polymer. In the integral, we substituted s by .
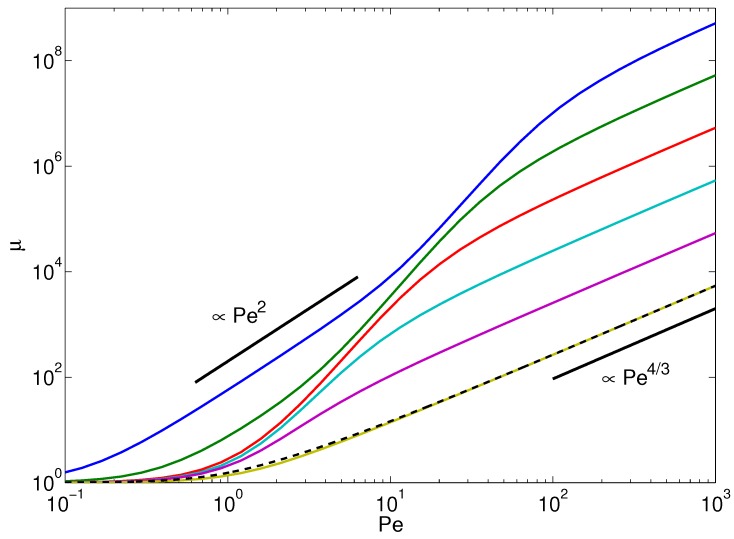
Figure 2 displays Lagrangian multipliers as a function of the Péclet number for various bending stiffnesses (at constant polymer length L, variation of corresponds to a variation of the polymer persistence length). Evidently, activity leads to an increase of the multiplier μ with increasing . Thereby, semiflexible polymers with exhibit a pronounced dependence on already for moderate Péclet numbers. In the limit , the multiplier assumes the value of a passive polymer . Over the considered range of Péclet numbers, the curves exhibit the asymptotic dependence for large , independent of the polymer stiffness. For polymers with , an intermediate regime appears, where , with . Very stiff polymers () even exhibit another power-law regime for small , where . The various activity-induced features reflected in the Lagrangian multiplier imply pronounced effects on the conformations and internal dynamics of an active polymer.
Figure 2.
Normalized stretching coefficient (Lagrangian multiplier) as a function of the Péclet number for the polymer bending stiffnesses , , 10, 1, and (bottom to top). For the other parameters, we set and . The dashed line for represents the solution of the asymptotic Equation (31). The straight lines indicate the power-law dependencies for and , and (cf. Equation (32)), respectively.
Flexible-polymer limit: An analytical solution of Equation (25) can easily be obtained for a flexible polymer, where . In this case, the wavenumbers are given by , and the eigenfunctions reduce to trigonometric functions [100], such that:
| (28) |
Hence, Equation (25) turns into:
| (29) |
including modes up to order . Evaluation of the sum yields:
| (30) |
or in terms of the Péclet number and Δ (Equation (24)),
| (31) |
The solution of this equation is compared to the exact solution of Equation (25) in Figure 2. Evidently, we find good agreement for and . Taking into account modes of order or even leads to a better agreement between the results of the two equations.
Equation (31) yields the following asymptotic dependencies:
For a passive polymer, implies .
- For and , i.e., ,
which yields (cf. Figure 3). Here, there remains a polymer-length dependence for , namely .(33)
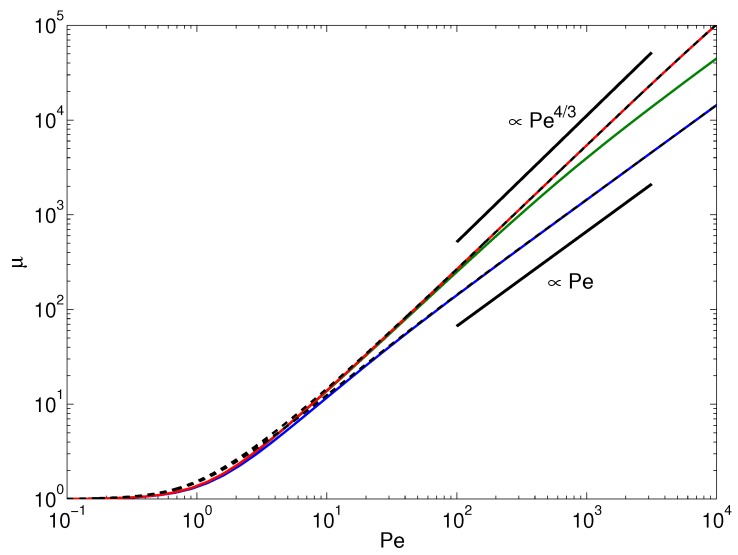
In the asymptotic limit , we find a crossover of the Lagrangian multiplier from the power-law dependence to . In the latter regime, the Lagrangian multiplier depends on polymer length. The crossover behavior is illustrated in Figure 3. The figure presents results for flexible polymers of various lengths, where the Kuhn segment length is identified with l, i.e., . The power-law dependence is specific to the large number of internal degrees of freedom of a polymer. This applies to flexible, as well as semiflexible polymers. As is discussed in the next section, activity changes the properties of semiflexible polymers, and they exhibit flexible polymer behavior at large Péclet numbers. However, in the asymptotic limit , activity causes a stretching of the polymer and a crossover to the dependence appears. The same relation is obtained for a finite-extensible active dumbbell, which lacks internal degrees of freedom [28]. Hence, the dynamical properties of active polymers are not only determined by the longest relaxation time, as is often the case for passive polymers, but the internal degrees of freedom play a much more significant role than for passive polymers.
Figure 3.
Normalized stretching coefficient as function of the Péclet number for , and (bottom to top). In all cases, we set , which corresponds to and . The dashed lines represent the solution of the asymptotic Equation (31). The straight lines indicate the power-law dependencies for and for (cf. Equations (32) and (33), respectively).
4.3. Relaxation Times
The relaxation times (Equation (17)):
| (34) |
depend via μ on the activity (or ). We like to emphasize once more that this is a consequence of the finite extensibility of a polymer [28]. Neglecting this intrinsic property implies , and the relaxation times are independent of the activity [68,71]. The presence of the factor μ gives rise to a particular dynamical behavior, specifically for semiflexible polymers.
In the limit of a flexible polymer, the relaxation times become:
| (35) |
with the Rouse relaxation time [95,100]. Since, is a monotonically increasing function of , activity accelerates the relaxation process and the relaxation times become shorter. However, the mode-number dependence is not affected.
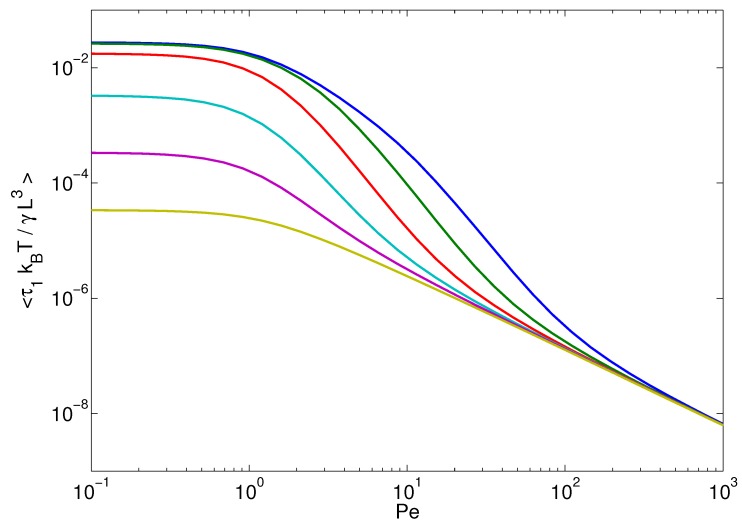
The influence of activity on semiflexible polymers is much more substantial. For such polymers, , and the -dependence (bending modes) typically dominates the relaxation behavior. However, with increasing activity, and hence μ, the flexible modes () in Equation (34) dominate over the bending modes. Thus, the contribution determines the relaxation behavior of the polymer for . Only for larger modes, semiflexibility matters. As a consequence, starting from the large length-scale dynamics, activity induces a transition from semiflexible to flexible polymer behavior, which extends to smaller and smaller length scales with increasing . This behavior is illustrated in Figure 4 for the longest polymer relaxation time . For , exhibits the predicted behavior (cf. Equation (35)), with for large . At , the relaxation times of the stiffer polymers are determined by the bending modes, and approaches the persistence-length and independent value:
| (36) |
with decreasing . The increase of μ with increasing Péclet number causes a decrease of the relaxation time , and in the limit , the relaxation times assume the same asymptotic value of Equation (17) independent of the stiffness. Quantitatively, as soon as . The latter is already satisfied for rather moderate Péclet numbers on the order of .
Figure 4.
Longest polymer relaxation times as a function of the Péclet number for the bending stiffnesses (L is fixed) , , 10, 1, and (bottom to top). The other parameters are the same as in Figure 2.
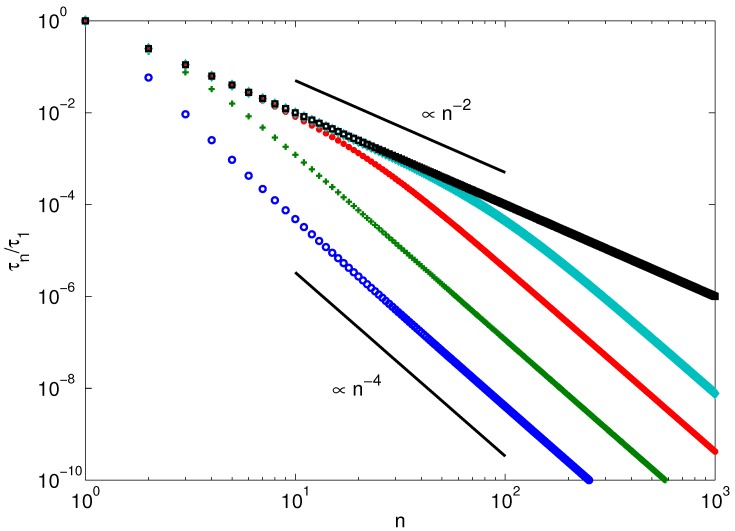
Figure 5 displays the dependence of the relaxation times of a stiff polymer on the mode number for various Péclet numbers. At low , we find the well-known dependence valid for semiflexible polymers [100,103,106]. With increasing , the relaxation times increase, and for , the small-mode-number relaxation times exhibit the dependence of flexible polymers. At larger n, the relaxation times cross over to the semiflexible behavior again. However, the crossover point shifts to larger mode numbers with increasing activity. Taking the wavenumbers for flexible polymers, Equation (34) yields the condition for the dominance of bending modes. Hence, active polymers at large Péclet numbers appear flexible on large length and long time scales and only exhibit semiflexible behavior at small length scales.
Figure 5.
Mode-number dependence of the relaxation times of active polymers with for the Péclet numbers , , and (bottom to top). The black squares (top) show the mode-number dependence of a flexible polymer with . The other parameters are and . The solid lines indicate the relations for flexible () and semiflexible () polymers, respectively. is the longest relaxation time.
4.4. Mean Square End-to-End Distance
To characterize the conformational properties of the polymers, we consider the mean square end-to-end distance , which is given by:
| (37) |
in terms of the eigenfunction expansion (15), where:
| (38) |
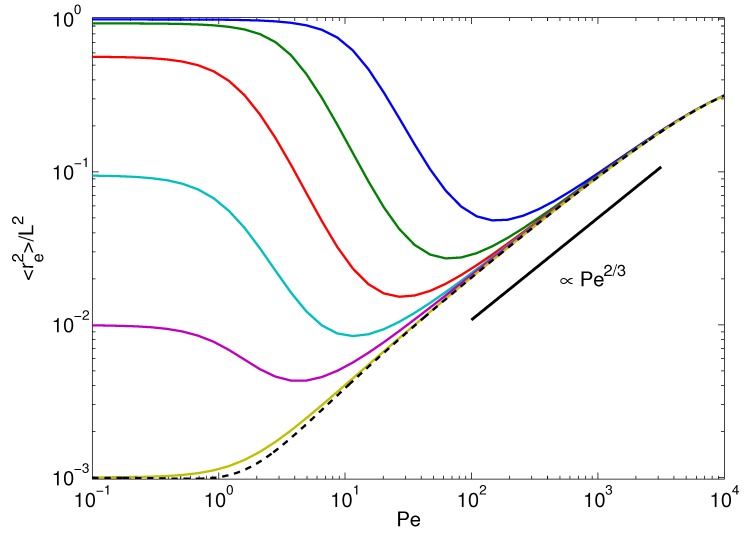
If the stretching coefficient λ and, hence, the relaxation times were independent of the activity, the average mean square mode amplitudes (38) would increase quadratically with the Péclet number for (cf. the second term in the right-hand side of Equation (38)). Thus, the mean square end-to-end distance would increase quadratically with [68]. As shown in Figure 6, the constraint of a constant contour length drastically changes the activity dependence of the polymer conformations. In the limit of a flexible polymer (bottom curve of Figure 6), increases with increasing Péclet number as from the passive equilibrium value . The mean square end-to-end distances of passive polymers itself increases with increasing persistence length, until the limit is reached for . For bending stiffnesses and , activity causes a significant shrinkage of the polymer over a wide range of Péclet numbers. Above a certain Péclet number, the actual value depends on the stiffness, the polymer swells again, but now, similar to a flexible polymer, and the asymptotic value is assumed for . This reflects the above-mentioned activity-induced transition from semiflexible to flexible-polymer behavior.
Figure 6.
Mean square end-to-end distances as a function of the Péclet number for the polymer bending stiffnesses , , 10, 1, and (bottom to top at ). The other parameters are the same as in Figure 2. The dashed line represents the analytical solution of Equation (40) with the Lagrangian multiplier of Equation (31).
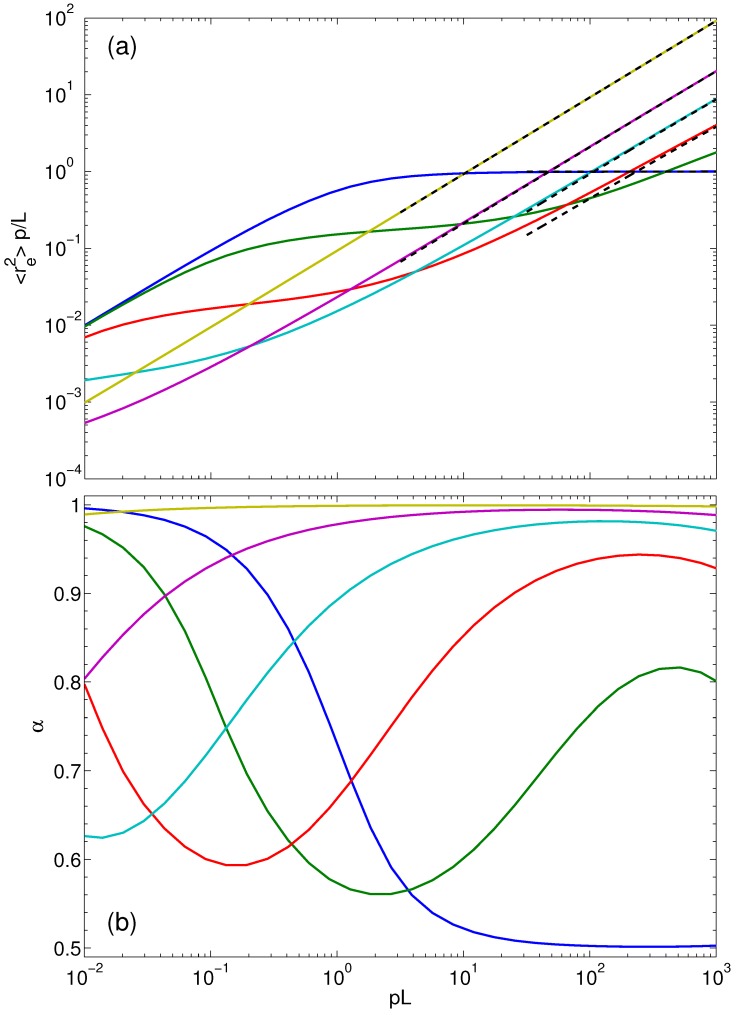
The scaling properties of as a function of polymer length () are illustrated in Figure 7a. In addition, Figure 7b shows the local slope:
| (39) |
In the passive case , increases quadratically with increasing for (, rod-like scaling). In the limit , the flexible Gaussian polymer scaling is obtained, where (), as is well know. In an active system, the local slope assumes the asymptotic value for , independent of the Péclet number . At a given , the mean square end-to-end distance exhibits a monotonic progression with increasing , but the local slope is non-monotonic. Starting from the asymptotic value , the local slope decreases first with increasing flexibility, i.e., , passes through a minimum, which depends on , and increases again. This is illustrated in Figure 7b for and 30. The intermediate regime is rather broad, with local slopes almost as small as the value for simple Gaussian polymers. In terms of scaling, we can identify a -regime for —the actual range depends on —where α gradually increases with increasing Péclet number from the flexible polymer value to the rod limit . In addition, (smaller) scaling regimes exist in the crossover region, which shift to smaller values with increasing , with local slopes increasing from with increasing Péclet number. The slopes for decrease for large values. This is related to the selected density of active sites along the polymer. For , a polymer is stiff on the length scale . In contrast, for , the polymer becomes flexible on lengths scales smaller than l, which gives rise to the decrease of the local slope.
Figure 7.
(a) Mean square end-to-end distances and (b) local slopes (Equation (39)) as function of the polymer length () for the Péclet numbers , 3, 10, 30, and (bottom to top at ). The other parameters are the same as in Figure 2. The dashed lines in (a) represent the analytical solution of Equation (40) with the Lagrangian multiplier of Equation (31).
Flexible-polymer behavior: Evaluation of Equation (37) in the limit of flexible polymers taking into account modes up to , but neglecting all ϵ terms, yields:
| (40) |
This equation exhibits the asymptotic behaviors:
- For finite and , the argument of the hyperbolic tangent function becomes small, and Taylor expansion gives:
Insertion of the asymptotic behavior of Equation (33) for the Lagrangian multiplier yields . Hence, the polymers assume nearly stretched conformations independent of the persistence length. This is visible in Figure 6.(41) - For , such that and , the argument of the hyperbolic tangent function becomes large. By setting the hyperbolic tangent to unity, we obtain:
Insertion of the asymptotics of Equation (32) for the stretching coefficient yields . This dependence on the Péclet number is shown in Figure 6 for the polymer with .(42)
5. Summary and Conclusions
We have presented an analytical approach to study the conformational and dynamical properties of active semiflexible polymers. We have adopted a continuum representation of a polymer with a certain number of active segments. Each of the segments is considered as an active Brownian particle whose orientation changes independently in a diffusive manner. Alternatively, the active random process can be considered as an additional external correlated (colored) noise acting on the polymer [6,8,28,71]. Active polymers have been considered before, both theoretically and by simulations [52,53,56,57,68,71]. As an important extension of the previous studies, we have taken into account the finite polymer extensibility due to its finite contour length. As has been shown, this constraint changes the dynamical behavior of active dumbbells drastically [28]. Taking into account the constraint by a Lagrangian multiplier leads to a linear equation, which is analytically tractable.
Evaluation of the polymer relaxation times shows a major influence of the finite contour length on the polymer dynamics. Models without such a constraint, e.g., the standard Rouse model [95], would not be able to reproduce and capture the correct dynamics, as reflected in the strong dependence of the stretching coefficient (Lagrangian multiplier) on the Péclet number already for moderate values. In particular, the relaxation times decrease with increasing activity (Péclet number). Thereby, the influence of activity on stiff polymers is much more severe. Here, activity induces a transition from semiflexible-polymer behavior, characterized by bending modes, to flexible-polymer behavior, characterized by stretching modes, with increasing activity. Thereby, the affected length scale depends on the activity. For activities , the large length-scale and low-mode number properties are altered. With increasing , an increasing number of modes and hence smaller length scales are affected. Due to the continuous nature of the considered polymer model, the (very) small-scale properties will always be dominated by bending modes.
The effect on the relaxation times translates to the conformational properties. In the simpler case of flexible polymers, activity leads to a monotonous swelling of the polymers over a wide range of Péclet numbers in a power-law manner, which is dictated by the constraint. Hence, our theoretical prediction is very different from the relation of a Rouse model derived in [68] for any flexibility and Péclet number. For semiflexible polymers, with , activity leads to shrinkage over a wide, stiffness-dependent range of Péclet numbers. At large , the polymer conformations are comparable to those of flexible polymers. An activity-induced shrinkage of semiflexible passive polymers embedded in a fluid of ABPs has been observed in simulations of two-dimensional systems [69,70], in qualitative agreement with our theoretical predictions. This supports the equivalence between intramolecular activity and the impact of external colored noise on the properties of semiflexible polymers (cf. Section 2).
The simulation studies of [68] for two-dimensional ABPO predict an activity-induced shrinkage of self-avoiding polymers. These kinds of shrinkage may be particular for 2D ABPS in combination with self-avoidance. As stated in [68], the polymer shrinkage at moderate Péclet numbers can be attributed to activity-induced encaging by neighboring ABPs. The particular relevance of excluded-volume interactions in 2D systems is also reflected in other studies, e.g., in References [57,69,70]. The activity-induced shrinkage of our 3D semiflexible polymers is of different origin. Here, self-avoidance does not play any role. In general, self-avoidance is less important in 3D than in 2D systems. Nevertheless, we expect interesting collective dynamical effects in 3D systems based on our studies of suspensions of 3D ABPs [41]. Moreover, the 2D simulations of [68] suggest that the scaling relation of the mean square end-to-end distance with polymer length is unperturbed by the activity. However, this should only apply to (very) small Péclet numbers, as is evident from Figure 7, which suggest swelling of the polymer already for and an activity-induced modified scaling behavior for large values. Note that the Péclet number of [68] is larger than ours due to the different definitions in terms of the translational and rotational diffusion coefficient, respectively. We definitely find for a wide crossover regime to the asymptotic scaling behavior of rod-like polymers, namely (cf. Figure 7).
Our studies illustrated the usefulness of basic polymer models for the understanding of the complex interplay between polymer entropy, stiffness and activity. Extension of the current studies toward further dynamical properties and other propulsion preferences, e.g., along the tangent of the polymer contour, are under way.
Experimentally, chains of ABPs can be synthesized by linearly-connecting self-propelling Janus particles [7] by a flexible linker. A random distribution of linker sites on the colloid surface yields a random orientation of the propulsion directions of the individual “monomers”. The ensemble average over various realizations corresponds to our description.
Acknowledgments
Financial support by the Deutsche Forschungsgemeinschaft (DFG) within the priority program SPP1726 “Microswimmers – from Single Particle Motion to Collective Behaviour” is gratefully acknowledged.
Author Contributions
Roland G. Winkler and Gerhard Gompper conceived of and designed the theoretical study. Thomas Eisenstecken and Roland G. Winkler performed the analytical calculations. Roland G. Winkler, THomas Eisenstecken and Gerhard Gompper wrote the paper.
Conflicts of Interest
The authors declare no conflict of interest. The founding sponsors had no role in the design of the study; in the collection, analyses or interpretation of data; in the writing of the manuscript; nor in the decision to publish the results.
References and Notes
- 1.Lauga E., Powers T.R. The hydrodynamics of swimming microorganisms. Rep. Prog. Phys. 2009 doi: 10.1088/0034-4885/72/9/096601. [DOI] [Google Scholar]
- 2.Ramaswamy S. The mechanics and statistics of active matter. Annu. Rev. Condens. Matter Phys. 2010;1:323–345. doi: 10.1146/annurev-conmatphys-070909-104101. [DOI] [Google Scholar]
- 3.Vicsek T., Zafeiris A. Collective motion. Phys. Rep. 2012 doi: 10.1016/j.physrep.2012.03.004. [DOI] [Google Scholar]
- 4.Romanczuk P., Bär M., Ebeling W., Lindner B., Schimansky-Geier L. Active brownian particles. Eur. Phys. J. Spec. Top. 2012;202:1–162. doi: 10.1140/epjst/e2012-01529-y. [DOI] [Google Scholar]
- 5.Marchetti M.C., Joanny J.F., Ramaswamy S., Liverpool T.B., Prost J., Rao M., Simha R.A. Hydrodynamics of soft active matter. Rev. Mod. Phys. 2013;85:1143. doi: 10.1103/RevModPhys.85.1143. [DOI] [Google Scholar]
- 6.Elgeti J., Winkler R.G., Gompper G. Physics of microswimmers—single particle motion and collective behavior: A review. Rep. Prog. Phys. 2015 doi: 10.1088/0034-4885/78/5/056601. [DOI] [PubMed] [Google Scholar]
- 7.Bechinger C., Di Leonardo R., Löwen H., Reichhardt C., Volpe G., Volpe G. Active Brownian Particles in Complex and Crowded Environments. 2016. [(accessed on 6 June 2016)]. Available online: https://arxiv.org/abs/1602.00081.
- 8.Marchetti M.C., Fily Y., Henkes S., Patch A., Yllanes D. Minimal model of active colloids highlights the role of mechanical interactions in controlling the emergent behavior of active matter. Curr. Opin. Colloid Interface Sci. 2016;21:34–43. doi: 10.1016/j.cocis.2016.01.003. [DOI] [Google Scholar]
- 9.Zöttl A., Stark H. Emergent behavior in active colloids. J. Phys. Condens. Matter. 2016 doi: 10.1088/0953-8984/28/25/253001. [DOI] [Google Scholar]
- 10.Nédélec F.J., Surrey T., Maggs A.C., Leibler S. Self-organization of microtubules and motors. Nature. 1997;389:305–308. doi: 10.1038/38532. [DOI] [PubMed] [Google Scholar]
- 11.Howard J. Mechanics of Motor Proteins and the Cytoskeleton. Sinauer Associates; Sunderland, MA, USA: 2001. [Google Scholar]
- 12.Kruse K., Joanny J.F., Jülicher F., Prost J., Sekimoto K. Asters, vortices, and rotating spirals in active gels of polar filaments. Phys. Rev. Lett. 2004 doi: 10.1103/PhysRevLett.92.078101. [DOI] [PubMed] [Google Scholar]
- 13.Bausch A.R., Kroy K. A bottom-up approach to cell mechanics. Nat. Phys. 2006;2:231–238. doi: 10.1038/nphys260. [DOI] [Google Scholar]
- 14.Jülicher F., Kruse K., Prost J., Joanny J.F. Active behavior of the cytoskeleton. Phys. Rep. 2007;449:3–28. doi: 10.1016/j.physrep.2007.02.018. [DOI] [Google Scholar]
- 15.Harada Y., Noguchi A., Kishino A., Yanagida T. Sliding movement of single actin filaments on one-headed myosin filaments. Nature. 1987;326:805–808. doi: 10.1038/326805a0. [DOI] [PubMed] [Google Scholar]
- 16.Schaller V., Weber C., Semmrich C., Frey E., Bausch A.R. Polar patterns of driven filaments. Nature. 2010;467:73–77. doi: 10.1038/nature09312. [DOI] [PubMed] [Google Scholar]
- 17.Prost J., Jülicher F., Joanny J.F. Active gel physics. Nat. Phys. 2015;11:111–117. doi: 10.1038/nphys3224. [DOI] [Google Scholar]
- 18.Berg H.C. E. Coli in Motion. Springer; New York, NY, USA: 2004. (Biological and Medical Physics Series). [Google Scholar]
- 19.Scharf B. Real-time imaging of fluorescent flagellar filaments of rhizobium lupini H13-3: Flagellar rotation and ph-induced polymorphic transitions. J. Bacteriol. 2002;184:5979–5986. doi: 10.1128/JB.184.21.5979-5986.2002. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 20.Copeland M.F., Weibel D.B. Bacterial swarming: A model system for studying dynamic self-assembly. Soft Matter. 2009;5:1174–1187. doi: 10.1039/b812146j. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 21.Kearns D.B. A field guide to bacterial swarming motility. Nat. Rev. Microbiol. 2010;8:634–644. doi: 10.1038/nrmicro2405. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 22.Cordoba A., Schieber J.D., Indei T. A single-chain model for active gels I: active dumbbell model. RSC Adv. 2014;4:17935–17949. doi: 10.1039/c4ra02262a. [DOI] [Google Scholar]
- 23.Sumino Y., Nagai K.H., Shitaka Y., Tanaka D., Yoshikawa K., Chate H., Oiwa K. Large-scale vortex lattice emerging from collectively moving microtubules. Nature. 2012;483:448–452. doi: 10.1038/nature10874. [DOI] [PubMed] [Google Scholar]
- 24.Howse J.R., Jones R.A.L., Ryan A.J., Gough T., Vafabakhsh R., Golestanian R. Self-motile colloidal particles: From directed propulsion to random walk. Phys. Rev. Lett. 2007 doi: 10.1103/PhysRevLett.99.048102. [DOI] [PubMed] [Google Scholar]
- 25.Volpe G., Buttinoni I., Vogt D., Kümmerer H.J., Bechinger C. Microswimmers in patterned environments. Soft Matter. 2011;7:8810–8815. doi: 10.1039/c1sm05960b. [DOI] [Google Scholar]
- 26.Buttinoni I., Bialké J., Kümmel F., Löwen H., Bechinger C., Speck T. Dynamical clustering and phase separation in suspensions of self-propelled colloidal particles. Phys. Rev. Lett. 2013 doi: 10.1103/PhysRevLett.110.238301. [DOI] [PubMed] [Google Scholar]
- 27.Ten Hagen B., Kümmel F., Wittkowski R., Takagi D., Löwen H., Bechinger C. Gravitaxis of asymmetric self-propelled colloidal particles. Nat. Commun. 2014 doi: 10.1038/ncomms5829. [DOI] [PubMed] [Google Scholar]
- 28.Winkler R.G. Dynamics of flexible active Brownian dumbbells in the absence and the presence of shear flow. Soft Matter. 2016;12:3737–3749. doi: 10.1039/C5SM02965A. [DOI] [PubMed] [Google Scholar]
- 29.Kim S., Karrila S.J. Microhydrodynamics: Principles and Selected Applications. Butterworth-Heinemann; Boston, MA, USA: 1991. [Google Scholar]
- 30.Drescher K., Dunkel J., Cisneros L.H., Ganguly S., Goldstein R.E. Fluid dynamics and noise in bacterial cell-cell and cell-surface scattering. Proc. Natl. Acad. Sci. USA. 2011;108:10940–10945. doi: 10.1073/pnas.1019079108. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 31.Drescher K., Goldstein R.E., Michel N., Polin M., Tuval I. Direct measurement of the flow field around swimming microorganisms. Phys. Rev. Lett. 2010 doi: 10.1103/PhysRevLett.105.168101. [DOI] [PubMed] [Google Scholar]
- 32.Guasto J.S., Johnson K.A., Gollub J.P. Oscillatory Flows Induced by Microorganisms Swimming in Two Dimensions. Phys. Rev. Lett. 2010 doi: 10.1103/PhysRevLett.105.168102. [DOI] [PubMed] [Google Scholar]
- 33.Watari N., Larson R.G. The hydrodynamics of a run-and-tumble bacterium propelled by polymorphic helical flagella. Biophys. J. 2010;98:12–17. doi: 10.1016/j.bpj.2009.09.044. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 34.Hu J., Yang M., Gompper G., Winkler R.G. Modelling the mechanics and hydrodynamics of swimming E. coli. Soft Matter. 2015;11:7867–7876. doi: 10.1039/C5SM01678A. [DOI] [PubMed] [Google Scholar]
- 35.Ghose S., Adhikari R. Irreducible representations of oscillatory and swirling flows in active soft matter. Phys. Rev. Lett. 2014 doi: 10.1103/PhysRevLett.112.118102. [DOI] [PubMed] [Google Scholar]
- 36.Klindt G.S., Friedrich B.M. Flagellar swimmers oscillate between pusher- and puller-type swimming. Phys. Rev. E. 2015 doi: 10.1103/PhysRevE.92.063019. [DOI] [PubMed] [Google Scholar]
- 37.Peruani F., Schimansky-Geier L., Bär M. Cluster dynamics and cluster size distributions in systems of self-propelled particles. Eur. Phys. J. Spec. Top. 2010;191:173–185. doi: 10.1140/epjst/e2010-01349-1. [DOI] [Google Scholar]
- 38.Fily Y., Marchetti M.C. Athermal phase separation of self-propelled particles with no alignment. Phys. Rev. Lett. 2012 doi: 10.1103/PhysRevLett.108.235702. [DOI] [PubMed] [Google Scholar]
- 39.Bialké J., Speck T., Löwen H. Crystallization in a dense suspension of self-propelled particles. Phys. Rev. Lett. 2012 doi: 10.1103/PhysRevLett.108.168301. [DOI] [PubMed] [Google Scholar]
- 40.Redner G.S., Hagan M.F., Baskaran A. Structure and dynamics of a phase-separating active colloidal fluid. Phys. Rev. Lett. 2013 doi: 10.1103/PhysRevLett.110.055701. [DOI] [PubMed] [Google Scholar]
- 41.Wysocki A., Winkler R.G., Gompper G. Cooperative motion of active Brownian spheres in three-dimensional dense suspensions. EPL. 2014 doi: 10.1209/0295-5075/105/48004. [DOI] [Google Scholar]
- 42.Ten Hagen B., Wittkowski R., Takagi D., Kümmel F., Bechinger C., Löwen H. Can the self-propulsion of anisotropic microswimmers be described by using forces and torques? J. Phys. 2015 doi: 10.1088/0953-8984/27/19/194110. [DOI] [PubMed] [Google Scholar]
- 43.Yang M., Ripoll M. A self-propelled thermophoretic microgear. Soft Matter. 2014;10:1006–1011. doi: 10.1039/c3sm52417e. [DOI] [PubMed] [Google Scholar]
- 44.Solon A.P., Stenhammar J., Wittkowski R., Kardar M., Kafri Y., Cates M.E., Tailleur J. Pressure and phase equilibria in interacting active brownian spheres. Phys. Rev. Lett. 2015 doi: 10.1103/PhysRevLett.114.198301. [DOI] [PubMed] [Google Scholar]
- 45.Solon A.P., Fily Y., Baskaran A., Cates M.E., Kafri Y., Kardar M., Tailleur J. Pressure is not a state function for generic active fluids. Nat. Phys. 2015;11:673–678. doi: 10.1038/nphys3377. [DOI] [Google Scholar]
- 46.Takatori S.C., Yan W., Brady J.F. Swim pressure: Stress generation in active matter. Phys. Rev. Lett. 2014 doi: 10.1103/PhysRevLett.113.028103. [DOI] [PubMed] [Google Scholar]
- 47.Maggi C., Marconi U.M.B., Gnan N., Di Leonardo R. Multidimensional stationary probability distribution for interacting active particles. Sci. Rep. 2015 doi: 10.1038/srep10742. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 48.Ginot F., Theurkauff I., Levis D., Ybert C., Bocquet L., Berthier L., Cottin-Bizonne C. Nonequilibrium equation of state in suspensions of active colloids. Phys. Rev. X. 2015 doi: 10.1103/PhysRevX.5.011004. [DOI] [Google Scholar]
- 49.Bertin E. An equation of state for active matter. Physics. 2015;8:44. doi: 10.1103/Physics.8.44. [DOI] [Google Scholar]
- 50.Speck T., Menzel A.M., Bialké J., Löwen H. Dynamical mean-field theory and weakly non-linear analysis for the phase separation of active Brownian particles. J. Chem. Phys. 2015 doi: 10.1063/1.4922324. [DOI] [PubMed] [Google Scholar]
- 51.Winkler R.G., Wysocki A., Gompper G. Virial pressure in systems of spherical active Brownian particles. Soft Matter. 2015;11:6680–6691. doi: 10.1039/C5SM01412C. [DOI] [PubMed] [Google Scholar]
- 52.Liverpool T.B., Maggs A.C., Ajdari A. Viscoelasticity of solutions of motile polymers. Phys. Rev. Lett. 2001;86:4171–4174. doi: 10.1103/PhysRevLett.86.4171. [DOI] [PubMed] [Google Scholar]
- 53.Sarkar D., Thakur S., Tao Y.G., Kapral R. Ring closure dynamics for a chemically active polymer. Soft Matter. 2014;10:9577–9584. doi: 10.1039/C4SM01941E. [DOI] [PubMed] [Google Scholar]
- 54.Chelakkot R., Gopinath A., Mahadevan L., Hagan M.F. Flagellar dynamics of a connected chain of active, polar, Brownian particles. J. R. Soc. Interface. 2013 doi: 10.1098/rsif.2013.0884. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 55.Loi D., Mossa S., Cugliandolo L.F. Non-conservative forces and effective temperatures in active polymers. Soft Matter. 2011;7:10193–10209. doi: 10.1039/c1sm05819c. [DOI] [Google Scholar]
- 56.Ghosh A., Gov N.S. Dynamics of active semiflexible polymers. Biophys. J. 2014;107:1065–1073. doi: 10.1016/j.bpj.2014.07.034. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 57.Isele-Holder R.E., Elgeti J., Gompper G. Self-propelled worm-like filaments: Spontaneous spiral formation, structure, and dynamics. Soft Matter. 2015;11:7181–7190. doi: 10.1039/C5SM01683E. [DOI] [PubMed] [Google Scholar]
- 58.Isele-Holder R.E., Jäger J., Saggiorato G., Elgeti J., Gompper G. Dynamics of self-propelled filaments pushing a load. Soft Matter. 2016 doi: 10.1039/C6SM01094F. [DOI] [PubMed] [Google Scholar]
- 59.Laskar A., Singh R., Ghose S., Jayaraman G., Kumar P.B.S., Adhikari R. Hydrodynamic instabilities provide a generic route to spontaneous biomimetic oscillations in chemomechanically active filaments. Sci. Rep. 2013 doi: 10.1038/srep01964. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 60.Jayaraman G., Ramachandran S., Ghose S., Laskar A., Bhamla M.S., Kumar P.B.S., Adhikari R. Autonomous motility of active filaments due to spontaneous flow-symmetry breaking. Phys. Rev. Lett. 2012 doi: 10.1103/PhysRevLett.109.158302. [DOI] [PubMed] [Google Scholar]
- 61.Jiang H., Hou Z. Motion transition of active filaments: Rotation without hydrodynamic interactions. Soft Matter. 2014;10:1012–1017. doi: 10.1039/c3sm52291a. [DOI] [PubMed] [Google Scholar]
- 62.Babel S., Löwen H., Menzel A.M. Dynamics of a linear magnetic “microswimmer molecule”. EPL. 2016 doi: 10.1209/0295-5075/113/58003. [DOI] [Google Scholar]
- 63.Kaiser A., Löwen H. Unusual swelling of a polymer in a bacterial bath. J. Chem. Phys. 2014 doi: 10.1063/1.4891095. [DOI] [PubMed] [Google Scholar]
- 64.Valeriani C., Li M., Novosel J., Arlt J., Marenduzzo D. Colloids in a bacterial bath: Simulations and experiments. Soft Matter. 2011;7:5228–5238. doi: 10.1039/c1sm05260h. [DOI] [Google Scholar]
- 65.Suma A., Gonnella G., Marenduzzo D., Orlandini E. Motility-induced phase separation in an active dumbbell fluid. EPL. 2014 doi: 10.1209/0295-5075/108/56004. [DOI] [Google Scholar]
- 66.Cugliandolo L.F., Gonnella G., Suma A. Rotational and translational diffusion in an interacting active dumbbell system. Phys. Rev. E. 2015 doi: 10.1103/PhysRevE.91.062124. [DOI] [PubMed] [Google Scholar]
- 67.Küchler N., Löwen H., Menzel A.M. Getting drowned in a swirl: Deformable bead-spring model microswimmers in external flow fields. Phys. Rev. E. 2016 doi: 10.1103/PhysRevE.93.022610. [DOI] [PubMed] [Google Scholar]
- 68.Kaiser A., Babel S., ten Hagen B., von Ferber C., Löwen H. How does a flexible chain of active particles swell? J. Chem. Phys. 2015 doi: 10.1063/1.4916134. [DOI] [PubMed] [Google Scholar]
- 69.Harder J., Valeriani C., Cacciuto A. Activity-induced collapse and reexpansion of rigid polymers. Phys. Rev. E. 2014 doi: 10.1103/PhysRevE.90.062312. [DOI] [PubMed] [Google Scholar]
- 70.Shin J., Cherstvy A.G., Kim W.K., Metzler R. Facilitation of polymer looping and giant polymer diffusivity in crowded solutions of active particles. New J. Phys. 2015 doi: 10.1088/1367-2630/17/11/113008. [DOI] [Google Scholar]
- 71.Samanta N., Chakrabarti R. Chain reconfiguration in active noise. J. Phys. A Math. Theor. 2016 doi: 10.1088/1751-8113/49/19/195601. [DOI] [Google Scholar]
- 72.Laskar A., Adhikari R. Brownian microhydrodynamics of active filaments. Soft Matter. 2015;11:9073–9085. doi: 10.1039/C5SM02021B. [DOI] [PubMed] [Google Scholar]
- 73.Dua A., Cherayil B.J. Chain dynamics in steady shear flow. J. Chem. Phys. 2000 doi: 10.1063/1.481487. [DOI] [PubMed] [Google Scholar]
- 74.Prabhakar R., Prakash J.R. Gaussian approximation for finitely extensible bead-spring chains with hydrodynamic interactions. J. Rheol. 2006;50:561–593. doi: 10.1122/1.2206715. [DOI] [Google Scholar]
- 75.Dua A., Cherayil B.J. Effect of stiffness on the flow behavior of polymers. J. Chem. Phys. 2000 doi: 10.1063/1.1324710. [DOI] [Google Scholar]
- 76.Winkler R.G., Keller S., Rädler J.O. Intramolecular dynamics of linear macromolecules by fluorescence correlation spectroscopy. Phys. Rev. E. 2006 doi: 10.1103/PhysRevE.73.041919. [DOI] [PubMed] [Google Scholar]
- 77.Munk T., Hallatschek O., Wiggins C.H., Frey E. Dynamics of semiflexible polymers in a flow field. Phys. Rev. E. 2006 doi: 10.1103/PhysRevE.74.041911. [DOI] [PubMed] [Google Scholar]
- 78.Winkler R.G. Conformational and rheological properties of semiflexible polymers in shear flow. J. Chem. Phys. 2010 doi: 10.1063/1.3497642. [DOI] [PubMed] [Google Scholar]
- 79.Bird R.B., Curtiss C.F., Armstrong R.C., Hassager O. Dynamics of Polymer Liquids. John Wiley & Sons; New York, NY, USA: 1987. [Google Scholar]
- 80.Winkler R.G., Reineker P. Finite size distribution and partition functions of gaussian chains: Maximum entropy approach. Macromolecules. 1992;25:6891–6896. doi: 10.1021/ma00051a026. [DOI] [Google Scholar]
- 81.Marko J.F., Siggia E.D. Stretching DNA. Macromolecules. 1995;28:8759–8770. doi: 10.1021/ma00130a008. [DOI] [Google Scholar]
- 82.Winkler R.G. Deformation of semiflexible chains. J. Chem. Phys. 2003 doi: 10.1063/1.1537247. [DOI] [PubMed] [Google Scholar]
- 83.Winkler R.G. Equivalence of statistical ensembles in stretching single flexible polymers. Soft Matter. 2010;6:6183–6191. doi: 10.1039/c0sm00488j. [DOI] [Google Scholar]
- 84.Kierfeld J., Niamploy O., Sa-yakanit V., Lipowsky R. Stretching of semiflexible polymers with elastic bonds. Eur. Phys. J. E. 2004;14:17–34. doi: 10.1140/epje/i2003-10089-3. [DOI] [PubMed] [Google Scholar]
- 85.Salomo M., Kegel K., Gutsche C., Struhalla M., Reinmuth J., Skokow W., Hahn U., Kremer F. The elastic properties of single double-stranded DNA chains of different lengths as measured with optical tweezers. Colloid Polym. Sci. 2006;284:1325–1331. doi: 10.1007/s00396-006-1517-4. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 86.Blundell J.R., Terentjev E.M. Stretching semiflexible filaments and their networks. Macromolecules. 2009;42:5388–5394. doi: 10.1021/ma9004633. [DOI] [Google Scholar]
- 87.Lamura A., Winkler R.G. Semiflexible polymers under external fields confined to two dimensions. J. Chem. Phys. 2012 doi: 10.1063/1.4772748. [DOI] [PubMed] [Google Scholar]
- 88.Hsu H.P., Binder K. Stretching semiflexible polymer chains: Evidence for the importance of excluded volume effects from Monte Carlo simulation. J. Chem. Phys. 2012 doi: 10.1063/1.3674303. [DOI] [PubMed] [Google Scholar]
- 89.Radhakrishnan R., Underhill P.T. Models of flexible polymers in good solvents: Relaxation and coil–stretch transition. Soft Matter. 2012;8:6991–7003. doi: 10.1039/c2sm25802a. [DOI] [PubMed] [Google Scholar]
- 90.Manca F., Giordano S., Palla P.L., Cleri F., Colombo L. Theory and Monte Carlo simulations for the stretching of flexible and semiflexible single polymer chains under external fields. J. Chem. Phys. 2012 doi: 10.1063/1.4772656. [DOI] [PubMed] [Google Scholar]
- 91.Manca F., Giordano S., Palla P.L., Cleri F., Colombo L. Response to “Comment on ’Elasticity of flexible and semiflexible polymers with extensible bonds in the Gibbs and Helmholtz ensembles”’. J. Chem. Phys. 2013 doi: 10.1063/1.4801656. [DOI] [PubMed] [Google Scholar]
- 92.Iliafar S., Vezenov D., Jagota A. In-plane force–extension response of a polymer confined to a surface. Eur. Polym. J. 2014;51:151–158. doi: 10.1016/j.eurpolymj.2013.12.001. [DOI] [Google Scholar]
- 93.Alexeev A.V., Maltseva D.V., Ivanov V.A., Klushin L.I., Skvortsov A.M. Force-extension curves for broken-rod macromolecules: Dramatic effects of different probing methods for two and three rods. J. Chem. Phys. 2015 doi: 10.1063/1.4919295. [DOI] [PubMed] [Google Scholar]
- 94.Winkler R.G., Reineker P., Harnau L. Models and equilibrium properties of stiff molecular chains. J. Chem. Phys. 1994 doi: 10.1063/1.468239. [DOI] [Google Scholar]
- 95.Doi M., Edwards S.F. The Theory of Polymer Dynamics. Clarendon Press; Oxford, UK: 1986. [Google Scholar]
- 96.Bawendi M.G., Freed K.F. A Wiener integral model for stiff polymer chains. J. Chem. Phys. 1985 doi: 10.1063/1.449296. [DOI] [Google Scholar]
- 97.Battacharjee S.M., Muthukumar M. Statistical mechanics of solutions of semiflexible chains: A path integral formulation. J. Chem. Phys. 1987 doi: 10.1063/1.452579. [DOI] [Google Scholar]
- 98.Langowski J.B., Noolandi J., Nickel B. Stiff chain model—Functional integral approach. J. Chem. Phys. 1991;95:1266. doi: 10.1063/1.461106. [DOI] [Google Scholar]
- 99.Ha B.Y., Thirumalai D. A mean-field model for semiflexible chains. J. Chem. Phys. 1995;103:9408–9412. doi: 10.1063/1.470001. [DOI] [Google Scholar]
- 100.Harnau L., Winkler R.G., Reineker P. Dynamic properties of molecular chains with variable stiffness. J. Chem. Phys. 1995;102:7750. doi: 10.1063/1.469027. [DOI] [Google Scholar]
- 101.Winkler R.G., Harnau L., Reineker P. Distribution functions and dynamical properties of stiff macromolecules. Macromol. Theory Simul. 1997;6:1007–1035. doi: 10.1002/mats.1997.040060603. [DOI] [Google Scholar]
- 102.Winkler R.G. Semiflexible polymers in shear flow. Phys. Rev. Lett. 2006 doi: 10.1103/PhysRevLett.97.128301. [DOI] [PubMed] [Google Scholar]
- 103.Winkler R.G. Diffusion and segmental dynamics of rod-like molecules by fluorescence correlation spectroscopy. J. Chem. Phys. 2007 doi: 10.1063/1.2753160. [DOI] [PubMed] [Google Scholar]
- 104.Öttinger H.C. Stochastic Processes in Polymeric Fluids. Springer; Berlin, Germany: 1996. [Google Scholar]
- 105.Kratky O., Porod G. Röntgenuntersuchung gelöster Fadenmoleküle. Recl. Trav. Chim. PaysBas. 1949 doi: 10.1002/recl.19490681203. [DOI] [Google Scholar]
- 106.Aragón S.R., Pecora R. Dynamics of wormlike chains. Macromolecules. 1985;18:1868. doi: 10.1021/ma00152a014. [DOI] [Google Scholar]
- 107.Flory P.J. Statistical Mechanics of Polymer Chains. John Wiley & Sons; New York, NY, USA: 1989. [Google Scholar]
- 108.Rubinstein M., Colby R.C. Polymer Physics. Oxford University Press; Oxford, UK: 2003. [Google Scholar]
- 109.The Equation (10) of Reference 28 contains an error. The factor 2 in front of should be replaced by unity.
- 110.Stenhammar J., Marenduzzo D., Allen R.J., Cates M.E. Phase behaviour of active Brownian particles: The role of dimensionality. Soft Matter. 2014;10:1489–1499. doi: 10.1039/C3SM52813H. [DOI] [PubMed] [Google Scholar]