The Diagnosis and Treatment of Dyscalculia

Abstract

Background

3–7% of all children, adolescents, and adults suffer from dyscalculia. Severe, persistent difficulty performing arithmetical calculations leads to marked impairment in school, at work, and in everyday life and elevates the risk of comorbid mental disorders. The state of the evidence underlying various methods of diagnosing and treating this condition is unclear.

Methods

Systematic literature searches were carried out from April 2015 to June 2016 in the PsycInfo, PSYNDEX, MEDLINE, ProQuest, ERIC, Cochrane Library, ICTRP, and MathEduc databases. The main search terms on dyscalculia were the German terms “Rechenstörung,” “Rechenschwäche,” and “Dyskalkulie” and the English terms “dyscalculia,” “math disorder, and “math disability.” The data from the retrieved studies were evaluated in a meta-analysis, and corresponding recommendations on the diagnosis and treatment of dyscalculia were jointly issued by the 20 societies and associations that participated in the creation of this guideline.

Results

The diagnosis of dyscalculia should only be made if the person in question displays below-average mathematical performance when seen in the context of relevant information from the individual history, test findings, clinical examination, and further psychosocial assessment. The treatment should be directed toward the individual mathematical problem areas. The mean effect size found across all intervention trials was 0.52 (95% confidence interval [0.42; 0.62]). Treatment should be initiated early on in the primary-school years and carried out by trained specialists in an individual setting; comorbid symptoms and disorders should also receive attention. Persons with dyscalculia are at elevated risk of having dyslexia as well (odds ratio [OR]: 12.25); the same holds for attention deficit/hyperactivity disorder and for other mental disorders, both internalizing (such as anxiety and depression) and externalizing (e.g., disorders characterized by aggression and rule-breaking).

Conclusion

Symptom-specific interventions involving the training of specific mathematical content yield the best results. There is still a need for high-quality intervention trials and for suitable tests and learning programs for older adolescents and adults.

Three to seven percent of all children, adolescents, and adults suffer from dyscalculia. This figure corresponds to some 84 000 to 195 750 primary-school pupils in Germany (1– 3). The significance of dyscalculia is still underappreciated. Poor mathematical ability places a major burden on society and on the affected individual (4). A large-scale cohort study in England revealed that poor mathematical ability is associated with major psychosocial and economic risks: 70–90% of the affected persons ended their schooling prematurely at age 16; at age 30, very few of them were employed full-time. Their probability of being unemployed and of developing depressive symptoms was twice as high as that of persons without dyscalculia (5). The costs arising from severe impairment of mathematical ability in Great Britain have been estimated at £2.4 billion per year (6).

Persons with dyscalculia have marked, persistent problems in applying the basic methods of arithmetic and in knowledge of math facts (the multiplication table); according to the ICD-10 definition of the disorder (code F81.2), these problems are not merely due to low intelligence or inadequate schooling. These problems are often associated with impaired basic processing of numbers and quantities (7– 10) (box). The sex ratio of sufferers is approximately even, with a trend toward a higher prevalence among girls (11).

BOX. Typical features of dyscalculia.

• Difficulties in processing numbers and quantities, starting in the preschool years

  • The connection between a number (e.g., 2) and the quantity it represents (e.g., 2 apples) is made only with difficulty.

  • The relation between numbers and quantities (two apples and one apple = 2 + 1) is inadequately understood.

  • Ensuing difficulties in counting, comparing two numbers or quantities, rapid assessment and naming of small quantities of dots, determining the position of a number on the number line, understanding the place-value system, and transcoding.

• Difficulties with basic arithmetic operations and with further mathematical tasks

  • Computation rules are not understood because the underlying comprehension of numbers and quantities is lacking or insufficiently developed (17 + 14 = 1 + 1 and 7 + 4 = 13 or 211).

  • Deficits in retrieval of math facts (e.g., the multiplication table) with which the answers to simple calculation problems can be recalled directly from memory, rather than needing to be calculated anew each time.

  • Lack of transition from computation by counting (8 + 4 = 9, 10, 11, 12 = 12) to non-counting strategies (8 + 4 = 8 + 2 and 2 = 12).

  • These difficulties become worse with increasing mathematical complexity (larger number range, written computations, multiple calculating operations, word problems).

• Important:

  • Finger-counting per se is not a sign of dyscalculia, but rather a normal aid to the memorization of math facts and the learning of efficient calculating strategies. Persistent finger-counting, particularly for frequently repeated, easy calculating tasks, does indeed indicate a problem in calculation.

    Not the mere presence of calculating errors, but rather their variety, persistence, and frequency are determinative.

When dyscalculia is not recognized as such (as often happens), negative school experiences and repeated lack of success in mathematical tasks generate fears of failure as well as diminished self-esteem. The affected children and adolescents develop diverse mental symptoms and disorders (12). Symptoms are common (ca. 10–40%), both of the externalizing type (such as aggressiveness and agitation) and of the internalizing type (such as anxiety and depressed mood) (13– 15). Dyscalculia also displays high comorbidity with reading and/or spelling disorder (dyslexia; ca. 30–40%) as well as with attention deficit/hyperactivity disorder (ADHD; ca. 10–20%) (1, 2, 16, 17). Without specific intervention, dyscalculia often leads to scholastic failure and school absenteeism (12, 18).

The past two decades have seen markedly increased interest in dyscalculia, both among specialists studying the problem and among the general public (11, 19). Nonetheless, the new knowledge gained during this time has not been systematically integrated into medical, psychological, learning-therapeutic, and educational practice. A variety of procedures, criteria, and tests are used in the diagnostic evaluation of dyscalculia (7). Although a broad spectrum of therapeutic and learning programs is available, their effectiveness has generally not been studied or else remains unclear (20). Moreover, the classification of dyscalculia in the ICD-10, which is based exclusively on deficits in carrying out basic arithmetical tasks, must be questioned on the basis of recently acquired scientific knowledge.

The above considerations indicate the need for a guideline in which the current state of research in the field is systematically assessed and the participating scientific and professional organizations, working in collaboration, jointly issue clear and empirically well-grounded recommendations for the uniform and valid diagnostic evaluation and effective treatment of this condition. Twenty societies and associations under the leadership of the German Society of Child and Adolescent Psychiatry, Psychosomatics and Psychotherapy (ebox 1) have now created the world’s first evidence- and consensus-based S3 guideline on the diagnosis and treatment of dyscalculia (guideline No. 028-046 of the Association of the Scientific Medical Societies in Germany, Arbeitsgemeinschaft der Wissenschaftlichen Medizinischen Fachgesellschaften [AWMF]).

Key Messages.

• Persons with dyscalculia perform poorly in all areas of mathematics, particularly in the processing of numbers and quantities, in basic arithmetic operations, and in the solving of word problems.

• The diagnosis of dyscalculia requires mathematical performance as assessed by a standardized test to be at least one standard deviation below the age- or grade-appropriate mean. In addition, the history and the findings from clinical examination and further psychosocial assessment should clearly support the diagnosis.

• The treatment should be disorder-specific, should be initiated as early as possible, and should be provided by an appropriately trained expert in an individual setting.

• Comorbid symptoms and disorders must be kept in mind during the diagnosis and treatment of dyscalculia, particularly comorbid dyslexia, attention deficit/hyperactivity disorder, and disorders of either the internalizing type (anxiety, depression) or the externalizing type (characterized by aggression or rule-breaking).

• Health-insurance carriers should assume the costs for the treatment of dyscalculia. Analogously to dyslexia, dyscalculia should be officially recognized in education law, and supportive treatment measures should be made available in the schools to all who need them.

Methods

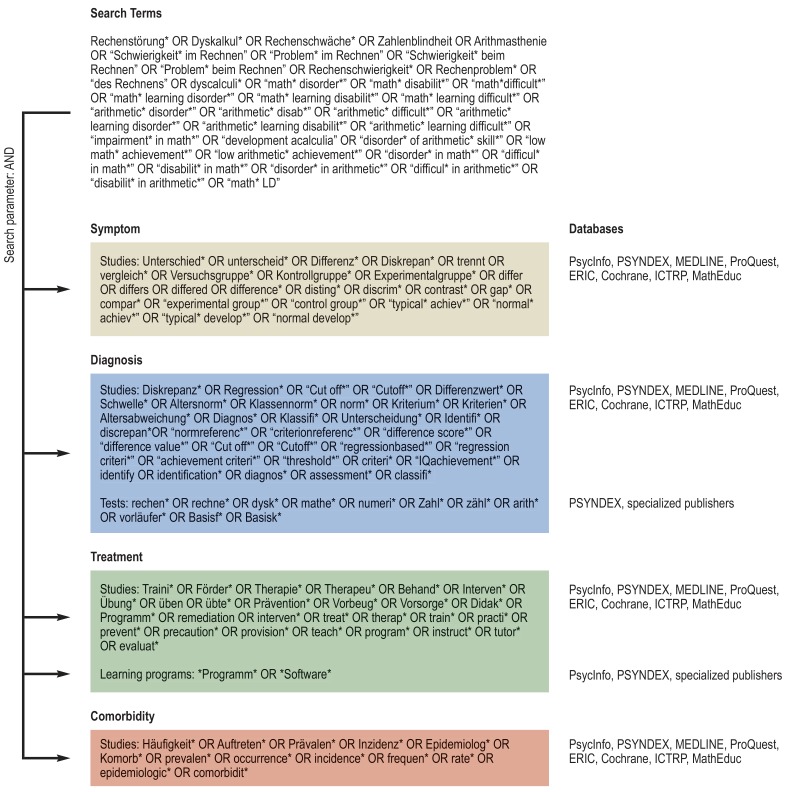
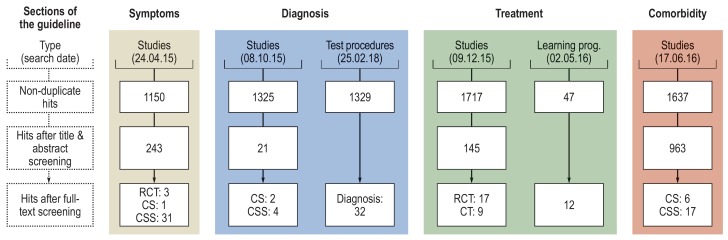
This guideline is divided into four sections concerning, respectively, the symptoms, diagnosis, treatment, and comorbidities of dyscalculia. The current state of research in the field was evaluated by systematic literature searches in multiple scientific databases (PsycINFO, Medline, ProQuest, ERIC, Cochrane, ICTRP, PSYNDEX, MathEduc). With regard to tests and learning programs, further searches were carried out for materials issued by relevant specialized publishers. The retrieved publications were selected on the basis of multiple inclusion criteria for each of the four sections of the guideline (ebox 2). The overall procedure and flow charts for the literature search in each of the four areas are shown in eFigures 1 and 2.

eBOX 1. Participating organizations (voting representant*).

• Coordinating specialty society

  • German Society of Child and Adolescent Psychiatry, Psychosomatics and Psychotherapy (Deutsche Gesellschaft für Kinder- und Jugendpsychiatrie, Psychosomatik und Psychotherapie e. V.) (DGKJP) (Prof. Dr. med. Gerd Schulte-Körne*)

• Participating specialty societies and associations (listed alphabetically by German name) and experts

  • Professional Association of Special Education (Berufsverband der Heilpädagoginnen und Heilpädagogen Fachverband für Heilpädagogik e. V.) (BHP) (Dr. phil. Miriam Stiehler*)

  • Professional Association of Pediatricians (Berufsverband der Kinder- und Jugendärzte e. V.) (BVKJ) (Dr. med. Harald Tegtmeyer-Metzdorf*)

  • Professional Association of Child and Adolescent Psychotherapists (Berufsverband der Kinder- und Jugendlichenpsychotherapeutinnen und Kinder- und Jugendlichenpsychotherapeuten e. V.) (bkj) (Christina Jung*)

  • Professional Association of Child and Adolescent Psychiatrists, Psychosomatic Therapists and Psychotherapists in Germany (Berufsverband für Kinder- und Jugendpsychiatrie, Psychosomatik und Psychotherapie in Deutschland e. V.) (BKJPP) (Dr. med. Gisela Schimansky*)

  • Board of Department Heads in Child and Adolescent Psychiatry, Psychosomatics and Psychotherapy (Bundesarbeitsgemeinschaft der Leitenden Klinikärzte für Kinder- und Jugendpsychiatrie, Psychosomatik und Psychotherapie e. V.) (BAG) (Dr. med. Astrid Passavant*)

  • Federal Chamber of Psychotherapists (BPtK) (Wolfgang Schreck*, Peter Lehndorfer, Dr. phil. Johannes Klein-Heßling)

  • German Dyslexia and Dyscalculia Association (Bundesverband Legasthenie & Dyskalkulie e. V.) (BVL) (Christine Sczygiel*)

  • German Educational Research Association (Deutsche Gesellschaft für Erziehungswissenschaft e. V.) (DGfE) (Prof. Dr. phil. Frank Hellmich*)

  • German Society of Pediatrics and Adolescent Medicine (Deutsche Gesellschaft für Kinder- und Jugendmedizin e. V.) (DGKJ) (Dr. med. Burkhard Lawrenz*)

  • German Society of Phoniatrics and Pediatric Audiology (Deutsche Gesellschaft für Phoniatrie und Pädaudiologie e. V.) (DGPP) (Prof. Dr. med. Rudolf Reiter*)

  • German Association for Psychiatry, Psychotherapy and Psychosomatics (Deutsche Gesellschaft für Psychiatrie und Psychotherapie, Psychosomatik und Nervenheilkunde e.V.) (DGPPN) (Prof. Dr. med. Ludger Tebartz van Elst*)

  • German Psychological Society (Deutsche Gesellschaft für Psychologie e. V.) (DGPs) (Prof. Dr. phil. Wolfgang Schneider*)

  • German Society of Social Pediatrics and Adolescent Medicine (Deutsche Gesellschaft für Sozialpädiatrie und Jugendmedizin e. V.) (DGSPJ) (Manfred Mickley*)

  • German Teachers’ Association (Deutscher Lehrerverband e. V.) (DL) (Florian Borges*)

  • German Association of Occupational Therapists (Deutscher Verband der Ergotherapeuten e. V.) (DVE) (Christine Priß*, Kerstin Hamm)

  • Association for Integrative Learning Therapy (Fachverband integrative Lerntherapie e. V.) (FiL) (Marlis Lipka*)

  • Society of Didactics of Mathematics (Gesellschaft für Didaktik der Mathematik e. V.) (GDM) (Prof. Dr. Jens-Holger Lorenz*)

  • Society for Neuropsychology (Gesellschaft für Neuropsychologie e. V.) (GNP) (Prof. Dr. rer. nat. Klaus Willmes-von Hinckeldey*)

  • Special Education Association (Verband Sonderpädagogik e. V.) (vds) (Stephan Prändl*)

  • Prof. Dr. Jörg-Tobias Kuhn (expert)

  • Prof. Dr. Michael von Aster (expert)

eFigure 1.

The employed search terms and databases

eFigure 2.

Flow chart of the literature search

CS: cohort or longitudinal study; CSS: cross-sectional study; CT: controlled trial; Diagnosis: standardized tests (from the end of the 1^st grade onward); RCT: randomized controlled trial; Learning prog.: learning programs

The methodological assessment of study quality was performed with the aid of checklists for each relevant study type (e.g., randomized controlled trial, non-randomized controlled trial) (21, 22). On this basis, each study was assigned an evidence grade, according to the scheme of the Oxford Center for Evidence-Based Medicine (OCEBM) (23). The datasets for each of the four areas were meta-analytically evaluated.

The methodological quality of diagnostic tests was evaluated with the aid of a specially developed rank-ordering procedure. For all tests, a rank order was determined on the basis of how well each test fulfilled a set of quality criteria in comparison to the other tests.

Learning programs were only considered if they had been evaluated in at least one trial involving a control group, an intervention group, and a pre–post design. These programs were assessed with respect to the quality of the evaluative trials providing evidence for their efficacy. The main criterion was whether the evaluative trials had been subjected to peer review and displayed a correspondingly high scientific quality, and/or whether they were carried out in persons with dyscalculia and were therefore valid for the purposes of this guideline.

On the basis of the findings of the literature search and evaluation, all of the participating organizations developed and agreed upon the recommendations of the guideline in a consensus conference under the neutral moderation of the AWMF. For each recommendation, the degree of consensus was rated as a strong consensus (>95%), a consensus (76–95%), or majority approval (51–75%).

Findings

All recommendations emerging from the consensus conference were strong (i.e., recommendation grade A) and supported by a broad consensus (i.e., at least 76% approval). Moreover, the findings of further studies published after the end of the literature search period and the publication of the guideline were still consistent with the guideline’s recommendations (ebox 3).

eBOX 2. Inclusion criteria for studies, tests, and learning programs.

• Symptoms of dyscalculia

  Studies:

  • Comparison of groups with and without dyscalculia with respect to performance-related outcomes (e.g., working memory)

  • Groups are age- and sex-matched

  • Exclusion of major reading deficit by reading ability above the 16^th percentile and exclusion of low intelligence by IQ = 70, or else by the non-fulfillment of the ICD-10 or DSM-IV/-5 criteria for dyslexia or low intelligence

  • The diagnosis of dyscalculia has been made from mathematical ability at or below the 25^th percentile or fulfillment of the ICD-10 or DSM-IV/-5 criteria for dyscalculia.

• Diagnosis of dyscalculia

  Studies:

  • Comparison of groups with dyscalculia whose diagnoses are based on: the simple IQ discrepancy criterion (i.e., the difference between the IQ and mathematical ability is greater than a given threshold amount, e.g., 1.5 standard deviations); the double discrepancy criterion (i.e., the difference between the IQ and mathematical ability is greater than a given threshold amount and, at the same time, mathematical ability is at or below a given percentile rank, e.g., the 16^th percentile); and/or the age/grade norm discrepancy (i.e., mathematical ability is lower than a given percentile rank for the subject’s age or grade)

  • The diagnosis of dyscalculia has been made from mathematical ability at or below the 25^th percentile or from the fulfillment of the ICD-10 or DSM-IV/-5 criteria for dyscalculia (except for persons diagnosed by the IQ discrepancy criterion)

  Tests:

  • Tests in the German language assessing mathematical ability with at least two different subtests (e.g., number comparisons, basic arithmetic operations) that were designed for the diagnosis of dyscalculia in subjects from the end of the 1^st grade onward

  • Use of up-to-date grade- and/or age-based norms (defined from the year 2000 onward)

• Treatment of dyscalculia

  Studies:

  • Comparison of groups with dyscalculia with respect to the improvement of mathematical performance after either an intervention intended to improve such performance or a control intervention (i.e., a nonspecific intervention or none at all); pre-post design with a test group and a control group

  • The groups are age- and sex-matched

  • The diagnosis of dyscalculia has been made from mathematical ability at or below the 25^th percentile or from the fulfillment of the ICD-10 or DSM-IV/-5 criteria for dyscalculia

  Supportive treatment programs:

  • Learning programs for which evaluation studies are available

  • Pre-post design with a treatment group and a control group

• Comorbidity of dyscalculia

  Studies:

  • Studies with epidemiological and selected samples for the estimation of the prevalence and/or relative frequency and/or odds ratios of other disorders or clinically relevant symptoms in association with dyscalculia

  • Diagnosis of other disorders, or documentation of clinically relevant symptoms, with standardized tests and according to the diagnostic criteria of the ICD-10 or the DSM-IV/-5 for the disorder in question

  • The diagnosis of dyscalculia has been made from mathematical ability at or below the 25^th percentile or from the fulfillment of the ICD-10 or DSM-IV/-5 criteria for dyscalculia

DSM, Diagnostic and Statistical Manual of Mental Disorders; ICD, International Classification of Diseases

Manifestations and diagnosis

Persons with dyscalculia have major difficulties in all areas of arithmetic (basic arithmetic operations, fact retrieval, word problems) (table 1) and in the processing of numbers and quantities. They need much more time than persons without dyscalculia to solve problems. In addition to these mathematics-specific deficits, they have markedly impaired performance in visuospatial working memory (e.g., remembering the position of dots in a matrix) and in the suppression of distracting stimuli (inhibition).

Table 1. Meta-analysis: persons with vs. persons without dyscalculia(the analysis shows positive effect sizes favoring persons without dyscalculia).

		Accuracy *1		Response time *2
Parameter	Description and examples	ES (SE)	References	ES (SE)	References
Mathematics		0.66 (0.13)	e1–e18	0.84 (0.21)	e3–e6, e8, e9, e12, e18, e19
Numerical and quantitative processing	Understanding of numbers and magnitudes (e.g. symbolic/non-symbolic comparisons, number line)	0.45 (0.17)	e1, e3, e5, e7, e9, e13, e15, e17, e18	0.64 (0.16)	e3, e5, e6, e9, e18, e19
Basic arithmetic operations	Math facts fluency (e.g. multiplication table)	0.74 (0.16)	e2, e6, e8, e11–13, e18	0.84 (0.15)	e6, e8, e12, e18
Word problems	Mathematical deductions from a text	0.97 (0.28)	e10. e14–16
Working memory		0.52 (0.07)	e2, e6, e11, e13–e18, e20–e28
Phonological loop	Short-term storage of linguistic information (e.g., forward letter span)	0.37 (0.09)	e6, e11, e13, e15, e17, e18, e21, e23–e25, e27, e28
Visuospatial sketchpad	Short-term storage of visuospatial information(e.g., matrix/block span)	0.84 (0.07)	e11, e13, e17, e21, e25, e28
Central executive function	Short-term storage and (further) processing of ‧information (e.g., backward letter span)	0.65 (0.08)	e2, e11, e13–e17, e20–e22, e24–e28
Executive functions		0.36 (0.16)	e2, e14–e16, e24, e26, e29	0.59 (0.18)	e2, e6, e24, e30–e32
Inhibition	Suppression of distracting stimuli while performing a task (e.g., the Stroop effect)	0.27 (0.07)	e2, e15, e26, e29	0.50 (0.11)	e2, e6, e24, e30–e32
Updating	Use of new information while performing a task	0.61 (0.33)	e14, e16, e24
Attention	Assessment of attention performanceby onself or others	0.73 (0.30)	e18, e26, e33
Processing speed	Processing of simple cognitive tasks (e.g., judging whether two symbols are identical)	0.68 (0.16)	e17, e20	0.28 (0.27)	e17, e18, e31
Reading and writing		0.21 (0.12)	e2, e6, e11, e18, e27, e29	0.30 (0.22)	e2, e6, e15, e18, e22, e23, e31
Phonological processing	Rapid naming (RAN) of letters, numbers, objects; phonological awareness (e.g., breaking down words into constituent syllables and phonemes)	0.21 (0.20)	e2, e6, e18, e27, e29	0.33 (0.14)	e2, e6, e15, e22, e23, e31
Reading and writing	Rapid, correct reading of words and non- or pseudowords; questions on the comprehension of a text; correct writing of words	0.34 (0.13)	e11, e18
Intelligence	Logical deductions (reasoning) (e.g., matrix tasks)	0.85 (0.19)	e20, e34

*¹ Number of solved problems, or number of errors; *² time needed for problem-solving ES, effect size, in Hedges’ g; SE, standard error

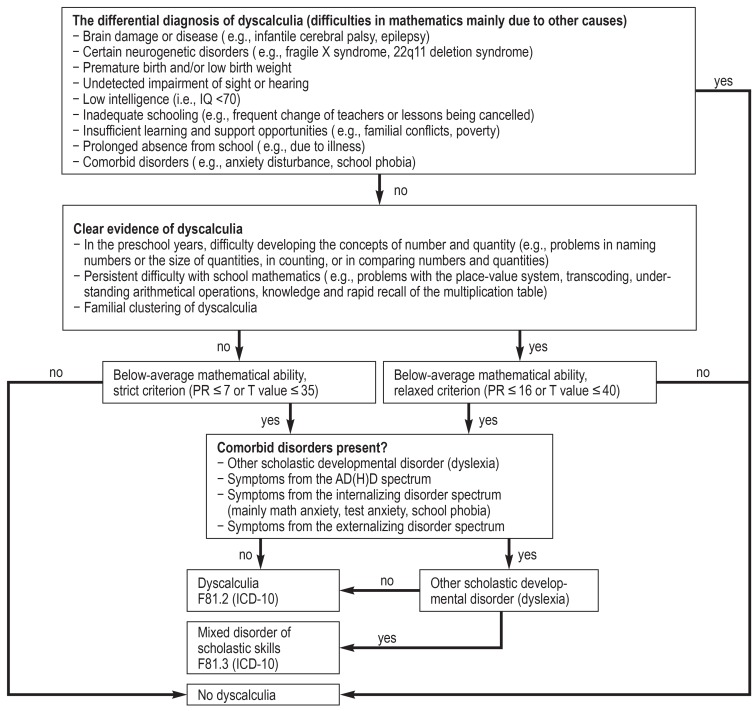
The diagnosis of dyscalculia involves not only obligatory psychometric (arithmetical) testing, but also a clinical examination, thorough history-taking, and further psychosocial assessment. The medical diagnostic algorithm is shown in the Figure.

Figure.

Algorithm for the diagnosis of dyscalculia

ADHD, attention deficit/hyperactivity disorder; ICD, International Classification of Diseases; PR, percentile rank

Clinical examination

The clinical diagnostic evaluation consists of a physical examination (including a neurological examination with testing of vision and hearing) and a standardized intelligence test. The diagnostic criteria for dyscalculia specify that impaired performance on mathematical tasks must not be attributable to low intelligence as defined by the ICD-10 (IQ <70), brain damage, brain disease (e.g., infantile cerebral palsy, epilepsy), or a previously undetected impairment of sight or hearing (24). The effects of neurogenetic disorders (e.g., fragile-X syndrome, 22q11 deletion syndrome) and other factors that can impair performance on mathematical tasks (e.g., premature birth, low birth weight) should also be taken into account in the differential diagnosis (25– 27).

History and further psychosocial assessment

The person’s biographical course of development and his or her familial and scholastic situation should be documented systematically, as should the effects of the performance deficits on mental and social development, school integration, and social participation (psychosocial integration).

In the differential diagnosis, other potential causes of difficulty in performing mathematical tasks must be ruled out, e.g.: inadequate schooling (frequent change of teachers or lessons being cancelled), insufficient learning and support opportunities (family conflicts, learning disorders in the family, poverty), prolonged absence from school, or the effects of other disorders on mathematical performance ability, such as anxiety disorders (school phobia).

To assure a correct diagnosis, supportive criteria for the diagnosis of dyscalculia should be applied, including family clustering of dyscalculia (28, 29) or difficulty in developing the concepts of number and quantity in the preschool years (10, 30).

Consideration of multiple risk factors sometimes enables prediction of the course and stability of dyscalculia: comorbid mental disorders, psychosocial stress factors (e.g., unsuccessful integration in school), and low family socioeconomic status should be taken into account, as these can affect the course of the disorder and the efficacy of interventions (16).

Psychometric testing

Psychometric tests (of mathematical performance) should be used to document, as completely as possible, the overall picture of the deficits. All tests were evaluated for methodological quality and assigned a rank order in a list (etable 1). The tests at the top of the list are recommended; those in the top half of the list are considered to be of better quality than those in the bottom half. If no test from the top half of the list is suitable for measuring the particular deficits of the person to be tested, a test from the next (i.e., third) quarter of the list can be chosen. This would be the case, for example, if the subject is in the sixth grade and none of the tests in the top half of the list is normed for this level, but one from the third quarter of the list is so normed. The tests in the lowest quarter of the list should not be used. An abbreviated listing of the best tests is given in Table 2.

eTable 1. Tests for the diagnosis of dyscalculia (from the end of the first grade onward).

		Areas Assessed			Range of Applicability		Time (min)
Overall Test	Subtest	P	B	W	From	To	From	To	Rank	Reference
Recommended diagnostic tests
CODY-M 2–4: CODY math test: mathematics test for the 2 nd –4 th grades		X	X		2nd grade (beginning)	4th grade (end)	30	45	1	(e81)
MBK 1+: test of basic mathematical competences at the beginning of schooling	1st grade, 3rd-4th quartile	X	X	X	1st grade (middle)	1st grade (end)	45	60	2	(e82)
BADYS 1–4+ (R): Bamberg Dyscalculia Diagnostic Test 1–4+ (R)	BADYS 2+ (R)	X	X	X	2nd grade (end)	3rd grade (middle)	60	75	3	(e83)
DEMAT 4: German mathematics test for the 4 th grade		X	X	X	4th grade (middle)	4th grade (end)	45	45	4	(e84)
BADYS 1–4+ (R): Bamberg Dyscalculia Diagnostic Test 1–4+ (R)	BADYS 4+ (R)	X	X	X	4th grade (end)	5th grade (middle)	60	75	5	(e83)
ERT 3+: Eggenberg Calculation Test 3+		X	X	X	3rd grade (end)	4th grade (middle)	30	90	6	(e85)
BADYS 1–4+ (R): Bamberg Dyscalculia Diagnostic Test 1–4+ (R)	BADYS 3+ (R)	X	X	X	3rd grade (end)	4th grade (middle)	60	75	7	(e83)
DEMAT 1+: German mathematics test for the 1 st grade		X	X	X	1st grade (end)	2nd grade (beginning)	45	45	8	(e86)
DEMAT 6+: German mathematics test for the 6 th grade		X	X	X	6th grade (end)	7th grade (middle)	35	35	9	(e87)
DEMAT 5+: German mathematics test for the 5 th grade		X	X	X	5th grade (end)	6th grade (middle)	35	35	10	(e88)
ERT 2+: Eggenberg Calculation Test 2+		X	X	X	2nd grade (end)	3rd grade (middle)	14	70	11	(e89)
DEMAT 2+: German mathematics test for the 2 nd grade		X	X	X	2nd grade (end)	3rd grade (beginning)	45	45	12	(e90)
ERT 4+: Eggenberg Calculation Test 4+		X	X	X	4th grade (end)	5th grade (middle)	20	85	13	(e91)
BADYS 5–8+: Bamberg Dyscalculia Diagnostic Test 5–8+	BADYS 5+	X	X	X	5th grade (end)	6th grade (middle)	45	90	14	(e92)
DEMAT 3+: German mathematics test for the 3 rd grade		X	X	X	3rd grade (end)	4th grade (middle)	45	45	15	(e93)
BADYS 5–8+: Bamberg Dyscalculia Diagnostic Test 5–8+	BADYS 7+	X	X	X	7th grade (middle)	8th grade (middle)	45	90	16	(e92)
HRT 1–4: Heidelberg ArithmeticTest	HRT 2 (end) –4	X	X		2nd grade (end)	4th grade (end)	40	60	17	(e94)
BADYS 5–8+: Bamberg Dyscalculia Diagnostic Test 5–8+	BADYS 6+	X	X	X	6th grade (middle)	7th grade (middle)	45	90	18	(e92)
BIRTE 2: Bielefeld Arithmetic Test for the 2nd grade		X	X	X	2nd grade (beginning)	2nd grade (end)	45	60	19	(e95)
BADYS 5–8+: Bamberg Dyscalculia Diagnostic Test 5–8+	BADYS 8+	X	X	X	8th grade (middle)	9th grade (middle)	45	90	20	(e92)
ERT 1+: Eggenberg Calculation Test 1+		X	X	X	1st grade (end)	2nd grade (middle)	14	70	21	(e96)
TEDI-MATH: Test of numerical calculating skills from kindergaten to the 3rd grade	core battery, 2_1	X	X	X	2nd grade (beginning)	2nd grade (middle)	45	45	22	(e97)
KEKS: Competence Assessment in Kindergarten and School	KEKS 3: mathematics	X	X	X	3rd grade (beginning)	3rd grade (middle)	45	45	23	(e98)
BADYS 1–4+ (R): Bamberg Dyscalculia Diagnostic Test 1–4+ (R)	BADYS 1+ (R)	X	X	X	1st grade (end)	2nd grade (middle)	60	75	24	(e83)
KEKS: Competence Assessment in Kindergarten and School	KEKS 4: mathematics	X	X	X	4th grade (beginning)	4th grade (middle)	45	45	25.5	(e98)
KEKS: Competence Assessment in Kindergarten and School	KEKS 4 ‧transition: mathematics	X	X	X	4th grade (end)	4th grade (end)	45	45	25.5	(e98)
DIRG: Diagnostic Inventory for Arithmetic Skills in Primary School	BASIS		X		1st grade (end) 2 nd grade (middle) 3 rd grade (middle) 4 th grade (middle)	1st grade (end) 2 nd grade (end) 3 rd grade (end) 4 th grade (end)	16	30	27.5	(e99)
KEKS: Competence Assessment in Kindergarten and School	KEKS 2: mathematics	X	X	X	2nd grade (beginning)	2nd grade (middle)	45	45	27.5	(e98)
MARKO-D1+: Mathematical and Arithmetical Concepts in First-Graders—Diagnosis		X	X	X	1st grade (middle)	2nd grade (beginning)	30	40	29	(e100)
Optionally recommended diagnostic tests (only in case none of the tests recommended above is suitable)
ZAREKI-R: Neuropsychological Test Battery for Numerical Processing and Arithmetic in Children		X	X	X	1st grade (middle) 2 nd grade (middle) 3 rd grade (middle) 4 th grade (middle)	1st grade (middle) 2 nd grade (middle) 3 rd grade (middle) 4 th grade (middle)	35	35	30	(e101)
TEDI-MATH: Test of numerical calculating skills from kindergarten to the 3 rd grade	core battery, 3_1	X	X	X	3rd grade (middle)	3rd grade (end)	45	45	31	(e97)
TeDDy-PC: Test for the Diagnosis of Dyscalculia	TeDDy-PC 2+	X	X	X	2nd grade (end)	3rd grade (beginning)	25	30	32	(e102)
HRT 1–4: Heidelberg ArithmeticTest	HRT 1–2 (middle)	X	X		1st grade (end)	2nd grade (middle)	40	60	33	(e94)
DEMAT 9: German mathematics test for the 9 th grade			X	X	9th grade (end)	9th grade (end)	35	35	34	(e103)
LVD-M 2–4: Longitudinal Learning Diagnosis— Mathe‧matics for 2 nd –4 th graders	LVD-M 4		X		4th grade (beginning)	4th grade (end)	15	15	35	(e104)
TeDDy-PC: Test for the Diagnosis of Dyscalculia	TeDDy-PC 3+	X	X	X	3rd grade (end)	4th grade (beginning)	25	30	36	(e102)
TEDI-MATH: Test of numerical calculating skills from kindergarten to the 3 rd grade	core battery, 1_2	X	X	X	1st grade (middle)	1st grade (end)	45	45	37	(e97)
RZD 2–6: Diagnostic Instrument for Arithmetical Skills and Number Processing in the 2 nd to 6 th Grades	RZD 6	X	X	X	6th grade (beginning)	6th grade (middle)	44	44	38	(e105)
KLASSE 4: Combined Performance Inventory for General Scholastic Performance and School Achievement Recommendations in the 4 th Grade	mathematics	X		X	4th grade (middle)	4th grade (middle)	20	20	39	(e106)
RZD 2–6: Diagnostic Instrument for Arithmetical Skills and Number Processing in the 2 nd to 6 th Grades	RZD 3–4	X	X	X	3rd grade (end)	4th grade (middle)	34	34	40	(e105)
TeDDy-PC: Test for the Diagnosis of Dyscalculia	TeDDy-PC 1+	X	X	X	1st grade (end)	2nd grade (beginning)	25	30	41	(e102)
ERT JE: Eggenberg Calculation Test for adolescents and adults		X	X	X	7th grade (beginning)	8th grade (end)	20	100	42	(e107)
RZD 2–6: Diagnostic Instrument for Arithmetical Skills and Number Processing in the 2 nd to 6 th Grades	RZD 4–5	X	X	X	4th grade (end)	5th grade (middle)	42	42	43	(e105)

P, numerical and quantitaive processing; B, basic arithmetic operations W, word problems; area of application, and duration, according to the corresponding test manuals

Table 2. Recommended psychometric tests of mathematical performance (in alphabetical order)*.

Test	Areas assessed			Range of applicability		Administration & scoring	Time (min)
	P	A	W	From	To		From	To
BADYS 1–4+ (R) (e83)	X	X	X	1st grade (end)	5th grade (middle)	PP (S)	60	75
BADYS 5–8+ (e92)	X	X	X	5th grade (end)	9th grade (middle)	PP (S)	45	90
BIRTE 2 (e95)	X	X	X	2nd grade (beginning)	2nd grade (end)	PC	45	60
CODY-M 2–4 (e81)	X	X		2nd grade (beginning)	4th grade (end)	PC	30	45
DEMAT 1+ (e86)	X	X	X	1st grade (end)	2nd grade (beginning)	PP	45	45
DEMAT 2+ (e90)	X	X	X	2nd grade (end)	3rd grade (beginning)	PP	45	45
DEMAT 3+ (e93)	X	X	X	3rd grade (end)	4th grade (middle)	PP	45	45
DEMAT 4 (e84)	X	X	X	4th grade (middle)	4th grade (end)	PP	45	45
DEMAT 5+ (e88)	X	X	X	5th grade (end)	6th grade (middle)	PP	35	35
DEMAT 6+ (e87)	X	X	X	6th grade (end)	7th grade (middle)	PP	35	35
DIRG (e99)		X		1st grade (end)	4th grade (end)	PP	16	30
ERT 1+ (e96)	X	X	X	1st grade (end)	2nd grade (middle)	PP	14	70
ERT 2+ (e89)	X	X	X	2nd grade (end)	3rd grade (middle)	PP	14	70
ERT 3+ (e85)	X	X	X	3rd grade (end)	4th grade (middle)	PP	30	90
ERT 4+ (e91)	X	X	X	4th grade (end)	5th grade (middle)	PP	20	85
HRT 1–4 (e94)	X	X		1st grade (end)	4th grade (end)	PP	40	60
KEKS (e98)	X	X	X	1st grade (beginning)	4th grade (end)	PP (S)	45	45
MARKO-D1+ (e100)	X	X	X	1st grade (middle)	2nd grade (beginning)	PP	30	40
MBK 1+ (e82)	X	X	X	1st grade (beginning)	4th grade (end)	PP	45	60

A, basic arithmetic operations; P, numerical and quantitative processing; PC, testing on a PC or tablet; PP, paper-and-pencil test; S, optional scoring program available for paper-based test (applicability and duration according to test manual); W, word problems

* abbreviated representation, for a detailed listing of these tests and their rankings, cf. eTable 1

Establishment of the diagnosis

The diagnosis is established on the basis of information from all three sources (testing, clinical examination, and history, including further psychosocial assessment) (figure). Whatever test is used for mathematical performance, below-average performance (= 16^th percentile) in mathematics must be documented, particularly in basic arithmetic operations and numerical and quantitative processing. The threshold value on the test that should be used as a criterion for the diagnosis depends on the degree to which the clinical examination, the history, and the psychosocial assessment support the suspected diagnosis of dyscalculia. If they do not do so, then a strict (low) threshold of 1.5 standard deviations below the age- or grade-appropriate mean is to be used (i.e., = 7^th percentile or T-value = 35). On the other hand, if there is already evidence to support the diagnosis of dyscalculia (e.g., preschool difficulties with the concepts of number and quantity), the threshold test value need not be so strict and can be set at 1 standard deviation below the age- or grade-appropriate mean (i.e., = 16^th percentile or T-value = 40).

The treatment of dyscalculia

All proposed interventional methods for dyscalculia must be scientifically evaluated with respect to their content and the conceptions of support and treatment that they embody. This is the only way to ensure that any positive effects are independent of other factors (e.g., the therapist–patient relationship). Evidence-based treatments are not yet available for all age groups, and there may thus be deviations in the treatment plan. The areas of mathematical performance that the diagnostic evaluation has shown to be problematic are the main targets of the therapeutic intervention. A meta-analysis on this topic has shown that symptom-specific interventions, in which persons with dyscalculia are mainly given mathematical tasks to practice, yields markedly better improvement in all areas of mathematical performance than no intervention at all or non–symptom-specific interventions that mainly train other skills (e.g., working memory). The mean effect size (Hedges’ g) in all intervention trials was 0.52 (95% confidence interval [0.42; 0.62]) (e14, e35–e59). Performance in numerical and quantitative processing improved by 0.30 [0.08; 0.52], in basic arithmetic operations by 0.44 [0.14; 0.58], and in word problems by 0.47 [0.14; 0.61]. Other clinically relevant symptoms and disorders that might affect mathematical performance should also be taken into account in choosing suitable interventions. If such symptoms/disorders are present, it is important to differentiate whether they are functionally linked to dyscalculia (e.g., math anxiety) or not (e.g., ADHD). In all cases, any comorbid mental disorder must be considered in the design of a suitable treatment plan.

In persons with dyscalculia, mathematical abilities should be reinforced through the application of standardized, disorder-specific interventions whose efficacy has been scientifically demonstrated (etable 2). These, however, should only be applied if they are appropriate in the context of the individual treatment plan. If the patient, for example, simultaneously suffers from an attention deficit that makes it impossible for him or her to follow a standardized program, then that program cannot be used.

eTable 2. Evaluated learning programs in German (names not translated).

Program	Applicability	Reference
recommended (peer-reviewed or evaluated for individuals with dyscalculia)
Dortmunder Zahlbegriffstraining	1st to 4th grade	(e108)
Dybuster Calcularis	1st to 5th grade	(e109)
MARKO-T	Kindergarten to 4th grade	(e110)
Meister Cody – Talasia	1st to 4th grade	(e111)
Mengen, Zählen, Zahlen	Kindergarten to 1st grade	(e112)
Rechenspiele mit Elfe und Mathis I	Kindergarten to 3rd grade	(e113)
Wasserglasmethode	Kindergarten to primary school	(e114)
optionally recommended (not peer-reviewed or evaluated for individuals with dyscalculia)
ALFONS Lernwelt Mathematik 1 und 2	1st to 2nd grade	(e115, e116)
Das Zahlenbuch 1	1st grade	(e117)
Mathematik im Vorschulalter	Kindergarten	(e118)
Merlins Rechenmühle	1st to 5th grade	(e119)
Mina und der Maulwurf	Kindergarten to 2nd grade	(e120)

Programs listed in alphabetical order by German name; applicability according to the relevant manuals

Treatment should be provided only by specialized personnel who have received appropriate pedagogical-therapeutic training in the development of mathematical ability and its disorders, according to the standards established by the relevant specialty associations (the German Dyslexia and Dyscalculia Association [BVL] and the Association for Integrative Learning Therapy [FiL]), or who have undertaken a course of university study centering on learning therapy.

Treatment should be provided in individual sessions of at least 45 minutes’ duration. Treatment was found to have a weaker effect if provided in a group setting (-0.19 [-0.37; -0.01]) or in sessions lasting less than 45 minutes (-0.49 [-1.02; 0.04]).

Preschool children who are held to be at risk for developing dyscalculia should receive supportive treatment as early as possible, as this has been found to have a beneficial effect on the later development of mathematical competence and on scholastic performance (31, 32). The decision when to end treatment depends on the course of the response and on changing individual factors (e.g., the severity of comorbid symptoms). Treatment should thus be continued as long as it is appropriate and necessary in the judgment of the interdisciplinary team caring for the child (e.g., therapist, teacher, and physician). The indication for continued treatment should be reevaluated at least once a year, with disorder-specific follow-up examinations carried out by independent specialists (i.e. not the person conducting therapy) who have the relevant expertise.

Comorbid disorders in persons with dyscalculia

Dyscalculia has high comorbidity with other disorders and symptoms. The prevalences, odds ratios, and relative frequencies were determined in a meta-analysis (etable 3). The most common comorbidities were found to be dyslexia, symptoms from the ADHD spectrum (mainly attention deficits), and symptoms of either the internalizing type (mainly math anxiety, test anxiety, and school phobia) or the externalizing type (e.g., aggressive behavior). Any individual who is given a diagnosis of dyscalculia should, therefore, undergo diagnostic screening for these potentially comorbid disorders. If a screening test yields the suspicion of a comorbid disorder, then a corresponding diagnostic work-up should be performed, preferably as specified in the relevant guideline (33– 37).

eTable 3. Comorbidity of dyscalculia.

Dyscalculia and:	PREV	REF	OR	References
	%	%	Risk
Attention deficit/hyperactivity disorder
Attention deficit/hyperactivity disorder	1.19	11.08	1.11	PREV: e60–e63 / REF: e60–e 63 / OR: e62
Attention disorder	5.81	21.74	1.34	PREV: e62, e63 / REF: e62, e63 / OR: e62
Hyperactivity disorder	2.18	8.93	1.59	PREV: e62 / REF: e62, e63 / OR: e62
Attention problems	0.41	33.04		PREV: e64 / REF: e64, e65
Dyslexia (reading and/or spelling disorder)
Reading and spellingdisorder	1.97	33.78	12.25	PREV: e61, e62, e66–e72 / REF: e61, e62, e66–e72 / OR: e62, e67–e70
Reading disorder	4.70	40.28	6.71	PREV: e61–e63, e66–e70, e72–e80 / REF: e61–e63, e66–e70, e72–e75, e77–e80 / OR: e62, e67–e70, e73–e75, e77, e78, e80
Spelling disorder	3.55	42.67	5.49	PREV: e62, e67–e72, e75, e79 / REF: e62, e67–e72, e75, e79 / OR: e62, e67–e70, e75
Internalizing spectrum of disorders
Affective disorder	0.12	2.06		PREV: e60 / REF: e60
Generalized anxiety disorder	5.43	15.09		PREV: e63 / REF: e63
Anxious-depressive symptoms	0.39	10.69		PREV: e60, e64 / REF: e60, e64
Internalizing symptoms	0.52	28.98		PREV: e64 / REF: e64, e65
Physical symptoms	0.29	10.73		PREV: e64 / REF: e64
Major depression	4.56	12.67		PREV: e63 / REF: e63
Symptoms of withdrawnness	0.41	15.14		PREV: e64 / REF: e64
Externalizing spectrum of disorders
Aggressive behavior	0.19	6.92		PREV: e64 / REF: e64
Social conduct disorder	1.37	6.50		PREV: e60, e63 / REF: e60, e63
Rule-breaking behavior	0.22	8.51		PREV: e64 / REF: e64
Externalizing symptoms	0.37	19.44		PREV: e64 / REF: e64, e65
Oppositional-defiant disorder	6.40	17.79		PREV: e63 / REF: e63

OR, odds ratio; PREV, prevalence—the frequency of the combined occurrence of dyscalculia together with another disorder or symptom(s) in the overall population; REF, relative freqeuncy—the frequency with which another disorder or symptom(s) arise(s) among persons with dyscalculia

The need for action and further research

There is currently a lack of high-quality standardized tests and evidence-based learning programs for children and adolescents with dyscalculia from the fifth grade and up, and also, in particular, for adults. There is likewise a lack, for all age groups, of high-quality randomized controlled trials with multiple follow-up examinations that could inform us about the intermediate- and long-term effects of treatment. Research is also needed on the long-term course of dyscalculia onward into adulthood and on the development of comorbid disorders that interact with dyscalculia (above all, math anxiety and school phobia), which can be major impediments to scholastic achievement and to the success of treatment.

Action is needed, in particular, on the level of social policy, because dyscalculia persists through all age categories, with manifold negative consequences for its sufferers. At present, across Germany, pupils with dyscalculia are not given equal treatment to pupils with dyslexia. For example, supportive measures (e.g., deficit compensation) are available only at the primary-school level, or not at all. Moreover, if learning therapy is needed outside of school, the costs are not borne by the health-insurance carriers; this places a major financial burden on the affected families that can go on for years, often leading to inadequate support and treatment of the affected children and adolescents. Social policy in the areas of education and health thus faces the task of making evidence-based scholastic supportive treatment available to all who need it, and of providing financial support for the costs of treatment. These two aspects are explicitly stressed in the preamble to the guideline, reflecting the consensus of the groups that participated in its creation.

The practical application of the S3 guideline

With the issuance of this guideline on the diagnosis and treatment of dyscalculia, evidence- and consensus-based S3 guidelines are now available that cover the entire area of specific developmental disorders of scholastic skills (ICD F81). This guideline contains relevant information for children, adolescents, and adults suffering from dyscalculia and should be implemented in all areas of its diagnosis and treatment. To enable better implementation of the recommendations, the guideline also includes additional information on their application in school, in learning therapy, and in the treatment of adults with dyscalculia. It also includes case illustrations exemplifying the diagnostic process. Fact sheets on each test that indicate its parameters and the included subtests, as well as lecture slides detailing the contents of the guideline, are available for downloading on the AWMF website.

eBOX 3. Studies published since the end of the literature search period.

• Remark

  The studies mentioned here are only those meeting the inclusion criteria of the literature searches described in the guideline report. The study findings are, in general, consistent with the recommendations of the guidelines, with only a few exceptions.

• The symptoms of dyscalculia

  As for the symptom profile of dyscalculia, a number of studies appeared after the end of the literature search period in which groups with and without dyscalculia were compared with respect to various outcomes. Only the outcomes of the group comparisons (rather than the specific questions investigated in each study) are of interest for the purposes of this guideline; these are, therefore, briefly described here.

  • Mammarella et al. (e121): Children with dyscalculia (n = 24, 6^th to 8^th grade) performed significantly worse than children without dyscalculia (n = 23, 6^th to 8^th grade) on visual, but not verbal, working-memory tasks.

  • Moll et al. (e122): Children with dyscalculia (n = 17, age range: 6–12 years) performed significantly worse than children without dyscalculia (n = 32, age range: 7–11 years) on all numerical processing tasks (counting, transcoding, symbolic comparison), quantitative processing tasks (number line, non-symbolic comparison), and arithmetical tasks (addition, subtraction). No significant differences were found in tests of phonological awareness, rapid naming of numbers and letters (RAN), or processing speed.

  • Donker et al. (e123): Children with dyscalculia (n = 31, 2^nd to 4^th grade) performed significantly worse than children without dyscalculia (n = 34, 2^nd to 4^th grade) on the rapid naming of pictures and colors (RAN), fact retrieval, and word problems. No significant differences were found in the rapid naming of numbers and letters or in any outcome having to do with reading and spelling performance.

  • Maehler et al. (e124): Children with dyscalculia (n = 18, 2^nd to 4^th grade) performed significantly worse than children without dyscalculia (n = 31, 2^nd to 4^th grade) on visuospatial sketchpad tasks. No significant differences were found in phonological loop or central executive tasks.

  • Raddatz et al. (e125): Children with dyscalculia (n = 20, 2^nd to 4^th grade) performed significantly worse than children without dyscalculia (n = 40, 2^nd to 4^th grade) in counting, symbolic comparison, and symbolic/non-symbolic comparison. No significant differences were found in non-symbolic comparison, number line, transcoding, and visuospatial sketchpad tasks.

  • Karakonstantaki et al. (e126): Children with dyscalculia (n = 13, 5^th to 6^th grade) performed significantly worse than children without dyscalculia (n = 26, 5^th to 6^th grade) in addition, subtraction, and multiplication.

  • Lambert et al. (e127): Children with dyscalculia (n = 27, 3rd to 4th grade) performed significantly worse than children without dyscalculia (n = 60, 3rd to 4th grade) in quantitative processing and spatial imagery.

  • Mammarella et al. (e128): Children with dyscalculia (n = 24, 4^th to 5^th grade) performed significantly worse than children without dyscalculia (n = 24, 4^th to 5^th grade) on visuospatial working memory tasks.

  • McDonald et al. (e129): Children with dyscalculia (n = 20, 2^nd to 5^th grade) performed significantly worse than children without dyscalculia (n = 20, 2^nd to 5^th grade) in the inhibition of quantities or numbers (e.g., with the number 444, they named the number of characters [three] rather than the value of the numeral [four]), in shifting between tasks, and on visuospatial working memory tasks. No significant differences were found in verbal working memory or in the inhibition of colors or color words (e.g., the word “red” in blue ink being expressed out loud as “blue,” rather than “red”).

  • Morsanyi et al. (e130): Children with dyscalculia (n = 20, 5^th to 7^th grade) performed significantly worse than children without dyscalculia (n = 20, 5^th to 7^th grade) on tests of verbal and visuospatial working memory, counting, non-symbolic comparison, and number line. No significant differences were found in symbolic comparison or in inhibition.

• The treatment of dyscalculia

  • Koponen et al. (e131): A non-randomized controlled study comparing two groups (an intervention group and a waiting-list control group), each consisting of 62 Finnish children with dyscalculia, in the 2^nd to 4^th grades, to test the efficacy of rule and strategy training for to promote rapid recall of math facts. The intervention was in a small-group setting and consisted of two weekly sessions of 45 minutes each for a total of 12 weeks. The intervention group performed significantly better in recalling facts relating to addition tasks, as well as on a test of fact knowledge in the basic arithmetic operations. The improvement of performance on subtraction tasks was not significant. Within the intervention group, a switch was observed from counting strategies to breakdown strategies and direct fact retrieval.

  • Kohn et al. (e132): A non-randomized controlled study comparing three groups (an intervention group, a control training group, and a waiting-list control group), each consisting of 22 or 23 German children with dyscalculia, in the 2^nd to 5^th grades, to test the efficacy of computer-based training of numerical and quantitative processing, and of arithmetic. The intervention consisted of five practice sessions per week lasting 20 minutes each for a total of 6–8 weeks. The control training group received computer-based training in spelling. The intervention group performed significantly better than the other two groups on subtraction and number line tasks. The improvement of performance on addition tasks was not significant.

• Comorbid disorders of dyscalculia

  • Morsanyi et al. (e133): A prevalence study among 2421 2^nd- to 5^th-graders in Northern Ireland. The combined prevalence of dyscalculia and dyslexia was 2.64%. 46.04% of children with dyscalculia also had dyslexia.

  • Devine et al. (e134): A prevalence study among 1757 3^rd-, 6^th-, and 7^th graders in England. The combined prevalence of dyscalculia and math anxiety was 2.62%. 19.25% of children with dyscalculia also had math anxiety.

  • Moll et al. (e135): A prevalence study among 1454 3^rd-graders in Germany (Bavaria). The combined prevalence of dyscalculia and dyslexia was 5.98%. 45.31% of children with dyscalculia also had dyslexia.