Abstract
Purpose:
Our study was conducted to evaluate and compare the accuracy of the refractive prediction determined by the calculation formulas for different intraocular lens (IOL) powers for high myopia.
Methods:
This study reviewed 217 eyes from 135 patients who had received cataract aspiration treatment and IOL implantation. The refractive mean numerical error (MNE) and mean absolute error (MAE) of the IOL power calculation formulas (SRK/T, Haigis, Holladay, Hoffer Q, and Barrett Universal II) were examined and compared. The MNE and MAE at different axial lengths (AL) were compared, and the percentage of every refractive error absolute value for each formula was calculated at ±0.25D, ±0.50D, ±1.00D, and ±2.00D.
Results:
In all, 98 patients were recruited into this study and 98 eyes of them were analyzed. We found that Barrett Universal II formula had the lowest MNE and MAE, SRK/T and Haigis formulas arrived at similar MNE and MAE, and the MNE and MAE calculated by Holladay and Hoffer Q formula were the highest. Barrett Universal II formulas have the lowest MAE among different AL patients, whereas it reached the highest percentage of refractive error absolute value within 0.5D in this study. The MAE of each formula is positively correlated with AL.
Conclusion:
Barrett Universal II formula rendered the lowest predictive error compared with SRK/T, Haigis, Holladay, and Hoffer Q formulas. Thus, Barrett Universal II formula may be regarded as a more reliable formula for high myopia.
Keywords: Axial length, Barrett Universal II formula, high myopia, intraocular lens power calculation formulas, refractive errors
High myopia, one of the most prevalent eye diseases worldwide, has a higher risk of occurrence than other eye diseases. High myopia is associated with the elongation of axial length (AL).[1,2,3] Studies have shown that the incidence and progression of cataract among those with high myopia were significantly higher and faster than those with nonmyopic eyes.[4,5] At present, patients with high myopia and cataract were often treated with cataract phacoemulsification.[6] Cataract surgery mainly consists of three steps: preoperative preparation, operation and postoperative symptomatic treatment, and observation and follow-up.[7] In the recent years, as a treatment of eye diseases, the development of cataract surgery was applied to improve preoperative vision correction, and such an application demands a higher standard for all aspects of cataract surgery.[8]
The preoperative preparation of cataract surgery consists of two parts, that is, examination of biometrics of the eye and artificial IOL power calculation.[9] A large number of studies have confirmed that the accuracy of preoperative eyeball biometrics, the selection, and calculation of IOL power calculation formulas were the main factors for prediction error.[10,11] This creates a demand for a higher standard of accuracy on eyeball measuring method and the selection of IOL power calculation formulas.[12] With the invention and application of biological measuring instruments, the error of eyeball measurement reduces increasingly; however, the selection of IOL power calculation formulas remains a key to the prediction of postoperative refractive error.[13,14]
The IOL power calculation formula has been applied for more than 40 years clinically and has been developed to the fourth-generation formula.[15,16] At present, the most commonly used IOL power calculation formula are the second- and third-generation formulas. Although scholars have been studying the comparison of IOL power calculation formulas for many years, research has rarely probed into the accuracy and application conditions of various formulas.[17,18,19] Studies have proposed that 54% deviation between actual diopter and prediction of diopter after cataract surgery comes from AL measurement, whereas 38% predicted from postoperative anterior chamber depth (ACD) and another 8% came from the evaluation of corneal curvature. Therefore, the accuracy of preoperative eye AL measurement is of particular importance.[20]
This study was performed to evaluate and compare the accuracy of the refractive prediction determined by different intraocular lens (IOL) power calculation formulas for high myopia and to analyze the correlation among the mean absolute error (MAE) rendered by different formulas and AL. Thus, this study provided reference for surgical treatment of patients with high myopia.
Methods
Patients
Details about all the patients who had received cataract extraction and IOL implantation were collected from our hospital from July 2016 to December 2017, based on the following criteria to eliminate bias. The inclusion criteria were as follows: (1) patients who had high myopia combined with cataract and had completed preoperative examination data and postoperative follow-up; (2) those who underwent cataract surgery performed by phacoemulsification and had no complications; (3) computing tool use of AcrySof IOL; and (4) preoperative biological measurement using LENSTAR LS900 (Haag-Streit AG, Koeniz, Switzerland). The exclusion criteria were as follows: (1) patients with a history of previous intraocular surgery or those who had postoperative complications; (2) patients followed up less than 1 month; and (3) preexisting ocular diseases that may affect postoperative refraction, for instance, keratoconus, endothelial dystrophy, corneal scarring, macular edema or retinal detachment.
Inspection method
Before the operation, LENSTAR LS900 measuring instrument and contact-type A ultrasound were used to measure the AL, corneal curvature, and other related eye parameters of recruited patients, and the measurement was repeated five times to obtain the average figure. Meanwhile, slit lamp microscope examination, computer optometry, and pupil fundus examination were conducted. Patients were distributed into group 1 (24.5 mm ≤ AL <27 mm, n = 28), group 2 (27.0 mm ≤ AL < 30 mm, n = 47), and group 3 (AL ≥30 mm, n = 23) in terms of their ALs. After the operation, the number of diopter was measured, and the best-corrected visual acuity refractive state was determined by standard methods at least 1 month after surgery by the same professional automatic computer optometry instrument, namely, postoperative actual diopter. The MAE was calculated after operation.
Formulas and constants
The four formulas of SRK/T, Haigis, Holladay, and Hoffer Q were selected from LENSTAR LS900 optical biosensor. According to the recommended LENSTAR LS900 optical biological measuring instrument, SRK/T formula optimization of A constant is 121.00, A0 Haigis formula optimization constant is 3.151, A1 constant is 0.400, A2 constant is 0.100, Holladay formula optimization of SF constant is 2.92, and Hoffer Q formula optimization pACD constant is 6.72. Meanwhile, back-calculation with new-generation Barrett Universal II formula was performed using the online software (http://www.apacrs.org/barrett universal2/), and the constants recommended in this online software were used for back-calculation.
Evaluation of the accuracy in predicted refraction
Normally, patients with high myopia would reserve the target refraction of 0.75D–3.0D. The selected AcrySof IOL degrees are respectively substituted into SRK/T formula, Haigis formula, Holladay formula, Hoffer Q formula, and Barrett Universal II formula. The absolute refractive error was calculated in terms of the difference among the predicted refraction outcomes and the actual postoperative refractive (actual postoperative refraction − predicted refraction) rendered by each formula. The mean absolute refractive error was calculated by each formula. The refractive error absolute value (by percentage) of each formula was calculated at ±0.25D, ± 0.50D, ±1.00D, and ±2.00D.
Statistical analysis
SPSS (version 22.00; SPSS, Inc., IL, USA) was used for statistical analysis. Means were shown as mean ± standard deviation (SD). The MAE of SRK/T, Haigis, Holladay, Hoffer Q, and Barrett Universal II formula was compared among all patients with high myopia. The differences in the mean numerical error (MNE) and MAE rendered by the five formulas – SRK/T, Haigis, Holladay, Hoffer Q, and Barrett Universal II at different ALs, were calculated using single-factor variance analysis. The least significant difference was used in two or two comparisons, and the association between MAE and AL was assessed using Spearman's rank correlation. P values less than 0.05 were considered as statistically significant.
Results
This study selected 217 eyes of 135 patients who had received cataract aspiration and IOL implantation. In all, 98 eyes of 98 patients were eventually investigated in this study. Patient's basic information, mean values, and ranges measured by IOLMaster were as follows: 37 males (37.8%), 61 females (62.2%), age 65.23 ± 6.78 years (range 56.0–76.0 years), 52 right eyes (53.1%), 46 left eyes (46.9%), ALs were 29.63 ± 2.35 mm (range 24.61–33.28 mm), average keratometries were 44.24D ± 2.42D (range 42.03D–48.54D), and IOL powers were 10.14D ± 4.20D (range − 4.5D to − 22.5D) [Table 1].
Table 1.
Basic information of the study population
| Parameter | Value |
|---|---|
| Gender, n (%) | |
| Male | 37 (37.8) |
| Female | 61 (62.2) |
| Age (years) | |
| Mean±SD | 65.23±6.78 |
| Range | 56-76 |
| Eye used, n (%) | |
| Right | 52 (53.1) |
| Left | 46 (46.9) |
| AL (mm) | |
| Mean±SD | 29.63±2.35 |
| Range | 24.61-33.28 |
| K (D) | |
| Mean±SD | 44.24±2.42 |
| Range | 42.03-48.54 |
| IOL power (D) | |
| Mean±SD | 10.14±4.20 |
| Range | −4.5 to 22.5 |
SD: Standard deviation; AL: Axial length; IOL: Intraocular lens; K: Keratometry
Mean numerical error
We found that among all the eyes, the MNE values ranged from 0.05 to 0.38 [Table 2], and that all formulas had a positive MNE and yielded a considerably high interquartile range (IQR) [Table 2]. Barrett Universal II formula had the lowest MNE and IQR in the five formulas, whereas SRK/T and Haigis formulas had similar MNEs; however, the MNE of Holladay and Hoffer Q was the highest [Table 2].
Table 2.
MNE and MAE for all formulas
| Formula | All | |
|---|---|---|
| MNE (D) | MAE (D) | |
| SRK/T | ||
| Mean±SD | 0.10±0.60 | 0.46±0.39 |
| Range | −1.39 to 1.85 | 0.00-1.85 |
| Haigis | ||
| Mean±SD | 0.10±0.58 | 0.45±0.38 |
| Range | −1.56 to 1.88 | 0.00-1.88 |
| Holladay | ||
| Mean±SD | 0.38±0.73 | 0.65±0.50 |
| Range | −2.11 to 2.21 | 0.00-2.21 |
| Hoffer Q | ||
| Mean±SD | 0.33±0.79 | 0.67±0.53 |
| Range | −2.00 to 2.11 | 0.00-2.11 |
| Barrett Universal II | ||
| Mean±SD | 0.05±0.46 | 0.35±0.30 |
| Range | −1.49 to 1.66 | 0.00-1.66 |
MNE: Mean numerical error; MAE: Mean absolute error; SD: Standard deviation
In group 1, we calculated that the MNE ranged from 0.06 to 0.45, whereas the SD ranged from 0.33 to 0.56, and that SRK/T formula had the lowest MNE among all the formulas, whereas Barrett Universal II formula reached the smallest SD [Table 3]. However, in group 2, we identified that the MNE ranged from 0.04 to 0.36, whereas the SD ranged from 0.32 to 0.77. SRK/T formula had the lowest MNE among all the formulas; however, Barrett Universal II formula touched the smallest SD [Table 3]. In group 3, the MNE ranged from − 0.15 to 0.60, and the SD ranged from 0.64 to 1.04. Barrett Universal II formula had the lowest MNE among all formulas; however, Haigis formula had the smallest SD [Table 3].
Table 3.
MNE and MAE for formulas and groups
| Formula | Group 1 (24.5 mm ≤AL <27 mm, n=28) |
Group 2 (27.0 mm ≤AL <30 mm, n=47) |
Group 3 (AL ≥30 mm, n=23) |
|||
|---|---|---|---|---|---|---|
| MNE (D) | MAE (D) | MNE (D) | MAE (D) | MNE (D) | MAE (D) | |
| SRK/T | ||||||
| Mean±SD | 0.06±0.50 | 0.34±0.36 | 0.04±0.55 | 0.44±0.32 | 0.27±0.78 | 0.65±0.49 |
| Range | −1.11-1.52 | 0.00-1.52 | −1.33-1.20 | 0.00-1.33 | −1.41-1.92 | 0.00-1.92 |
| Haigis | ||||||
| Mean±SD | 0.09±0.53 | 0.41±0.34 | 0.17±0.58 | 0.44±0.41 | 0.00±0.64 | 0.59±0.41 |
| Range | −0.90-1.59 | 0.00-1.59 | −1.62-1.89 | 0.00-1.89 | −1.20-1.43 | 0.00-1.43 |
| Holladay | ||||||
| Mean±SD | 0.45±0.56 | 0.55±0.47 | 0.36±0.65 | 0.59±0.44 | 0.32±1.04 | 0.90±0.58 |
| Range | −0.51-1.91 | 0.00−1.91 | −1.11-2.00 | 0.00-2.00 | −2.11-2.20 | 0.00-2.20 |
| Hoffer Q | ||||||
| Mean±SD | 0.28±0.55 | 0.48±0.38 | 0.23±0.77 | 0.64±0.49 | 0.60±1.02 | 1.00±0.62 |
| Range | −1.31-1.22 | 0.00-1.31 | −1.49-1.91 | 0.00-1.91 | −2.00-2.08 | 0.1-2.08 |
| Barrett Universal II | ||||||
| Mean±SD | 0.07±0.33 | 0.27±0.19 | 0.14±0.32 | 0.29±0.21 | -0.15±0.70 | 0.55±0.44 |
| Range | −0.50-0.72 | 0.00-0.72 | −0.71-0.79 | 0.00-0.79 | −1.52-1.73 | 0.00-1.73 |
MNE: Mean numerical error; MAE: Mean absolute error; SD: Standard deviation
Median absolute error
The MAE values ranged from 0.35 to 0.67 [Table 2] among all the eyes examined. All the formulas had a positive MAE and similar IQRs [Table 2]. We found that Barrett Universal II formula had the lowest MAE and IQR among the five formulas, and that SRK/T and Haigis formulas had similar MAEs, and that the MAEs of Holladay and Hoffer Q were the highest [Table 2]. In formula subgroup in which refractive error absolute value was less than 0.5D, Barrett Universal II formula had the highest proportion, SRK/T and Haigis formulas had similar proportion, and the proportion of Holladay and Hoffer Q was the lowest. In formula subgroup in which refractive error absolute value was more than 0.5D, Barrett Universal II formula had the lowest proportion of value outcome reaching higher than 0.5D, SRK/T and Haigis formulas had similar proportion, and the proportions of Holladay and Hoffer Q were the highest [Fig. 1].
Figure 1.
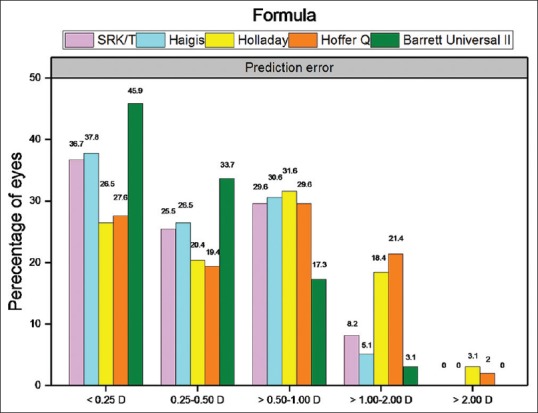
Percentages of the formulas of refractive error absolute value
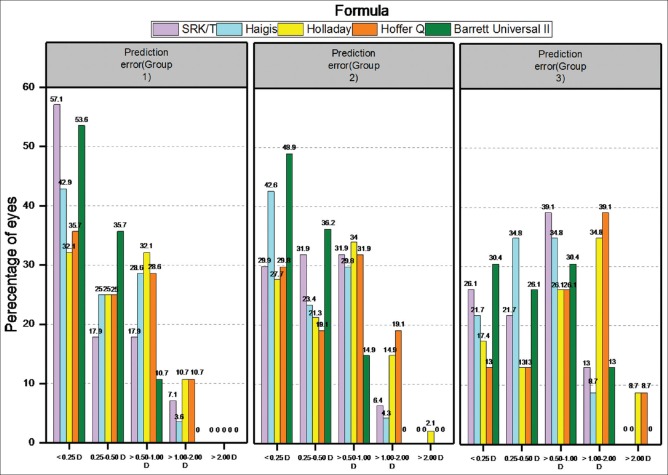
In group 1, the MAE ranged from 0.27 to 0.55, and the SD ranged from 0.19 to 0.47. The results showed that Barrett Universal II formula had the lowest MAE and IQR among all the formulas [Table 3]. In addition, we found that the proportion of Barrett Universal II formula was the highest when the refractive error absolute value was less than 0.5D, and that Barrett Universal II formula was the lowest when refractive error absolute value was more than 0.5D [Fig. 2]. In group 2, the MAE ranged from 0.29 to 0.64, whereas the SD ranged from 0.21 to 0.49. Barrett Universal II formula had the lowest MAE and IQR among all the formulas [Table 3]. Furthermore, the results indicated that the proportion of Barrett Universal II formula was the highest when the refractive error absolute value was less than 0.5 D, and that Barrett Universal II formula was the lowest when the refractive error absolute value was more than 0.5D [Fig. 2]. In group 3, the MAE ranged from 0.55 to 1.00, and the SD ranged from 0.41 to 0.62. Barrett Universal II formula had the lowest MAE among all formulas; however, Haigis formula has the smallest SD [Table 3]. Furthermore, the proportions of Barrett Universal II formula and Haigis were similar when the refractive error absolute value was less than 0.5D, and obviously higher than other formulas [Fig. 2].
Figure 2.
Percentages of the formulas of refractive error absolute value in different groups
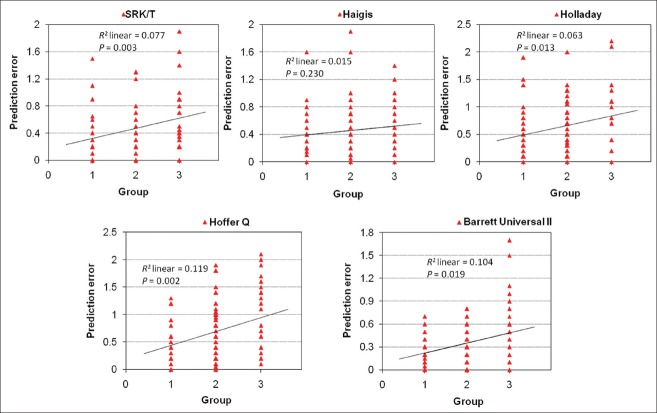
The correlation analysis showed that the prediction error of each formula was positively correlated with AL [Fig. 3].
Figure 3.
The association between MAE and AL. X-axis was AL; Y-axis was MAE of each formula. The MAE was higher as the AL became longer for all formulas
Discussion
In this study, we compared the accuracies of four widely used IOL power calculation formulas, namely, Holladay, SRK/T, Hoffer Q, and Haigis as well as a new-generation formula, that is, Barrett Universal II, applying for 98 high myopic eyes. The significance of this study is to reduce the prediction error of diopter, which greatly affects patients’ prognosis. In addition, this is one of the few studies supportive of the fact that the use of Barrett Universal II formula would enhance predictability in high myopia.
The cataract aspiration in combination with IOL implantation is the main method to treat cataract with high myopia.[21] In recent years, two main challenges in IOL power prediction for high myopia have emerged, that is, on one hand, there exists unexpected hyperopic outcomes with IOL power prediction formulas regardless of the IOL power (though this tendency for postoperative hyperopic outcomes is more marked for eyes with minus-power IOL), and on the other hand, the error rate of formula prediction increases gradually as the AL grows.[22,23] To avoid these postoperative hyperopic outcomes and improve patient satisfaction, surgeons usually empirically reserved − 0.75 D to − 3 D diopter for patients with high myopia.[24]
The most important factors that explain the prediction error in IOL calculations are the effective lens position assumption and the IOL constant adopted.[25,26] In this study, the formula constants were optimized, and the prediction results show that Barrett Universal II formula has the highest proportion of outcomes of refractive error absolute value being lower than 0.5D, SRK/T and Haigis formulas had similar proportion to Barrett Universal II formula, and the proportion of Holladay and Hoffer Q formula was the lowest among the three formulas. Meanwhile, Barrett Universal II formula has the lowest proportion, SRK/T and Haigis formulas had similar proportion, and the proportions of Holladay and Hoffer Q were the highest formula when the refractive error absolute value was more than 0.5D, indicating that Barrett Universal II formula has higher accuracy in the refractive prediction of patients with high myopia. The accuracy of SRK/T formula is similar to that of Haigis formula.
Another factor affecting the prediction error in IOL calculations was the error of AL measuring.[27,28] In this study, the accuracy of each formula under different ALs was analyzed. This study showed that the prediction error of SRK/T formula and Barrett Universal II formula was less when the AL was between 24.5 and 30 mm in comparison to that calculated by Haigis, Holladay, and Hoffer Q; the proportion of Barrett Universal II formula was higher than that of SRK/T formula when the refractive error absolute value was less than 0.5D; and Barrett Universal II formula was lower than SRK/T formula when the refractive error absolute value was more than 0.5D. These results showed that Barrett Universal II formula had the highest refractive prediction accuracy for patients with AL between 24.5 and 30 mm. Meanwhile, we found that when the AL was greater than 30 mm, the prediction error of Haigis formula and Barrett Universal II formula was less. However, the accuracy analysis shows that the prediction error of each formula was high. We observed that the proportion of Barrett Universal II formula was higher than Haigis formula when the refractive error absolute value was less than 0.5D, and that Barrett Universal II formula was lower than Holladay, Hoffer Q, and SRK/T when the refractive error absolute value was more than 0.5 D. This result suggests that with the increase in AL, the formula of refractive prediction accuracy in patients with high myopia went down, but Barrett Universal II formula still had a certain advantage. Therefore, we believe that Barrett Universal II formula has higher diopter prediction accuracy in patients with high myopia, especially for patients with longer AL have higher application value. Statistical analysis showed that the refractive error absolute value of SRK/T, Holladay, Hoffer Q formulas, and the Barrett Universal II formula was positively correlated with AL. That is, the longer the length of the AL of the patient's eye, the greater the refractive error absolute value. Therefore, in clinical treatment, we can measure the length of the AL of the patient's eye preoperatively by appropriate prediction formula to reduce the refractive error absolute value and improve the clinical treatment effect of patients with high myopia.
A limitation of this study was that we did not fully consider the IOL differences among patients, and that there were some differences in terms of the strength of the crystalline lens of different patients.[29] Another limitation was that each formula had its own applicability.[30] All IOL power calculations in this study were based on the measurements from LENSTAR LS900; however, Barrett Universal II formula was based on the measurements from another source. As zero-diopter IOL and minus-power IOL only affect a small number of the population. Thus, the number of research subjects with the two diseases recruited was small, which may hinder the accuracy of the prediction result calculated by each formula. Also, patients with ALs that were greater than 26 mm were relatively rare, and the sample cross section of patients being monitored was insufficient to give a conclusive result.
Conclusion
In conclusion, the results of this study suggested that Barrett Universal II formula achieves the highest accuracy for predicting diopter after surgery for high myopia. SRK/T and Haigis formulas achieved similar results that were more accurate than those calculated by Holladay and Hoffer Q formulas. In addition, Barrett Universal II formula had the highest value of refractive prediction for patients with high myopia with long AL. Thus, Barrett Universal II formula may be more applicable for patients with high myopia.
Financial support and sponsorship
This work was supported by Clinical Application and Evaluation of VERION Digital Navigation System in Refractive Cataract Surgery [Grant Number: CE20175039].
Conflicts of interest
There are no conflicts of interest.
Acknowledgements
Dong Zhou and Zhuo Sun contributed equally to this work.
References
- 1.Holden BA, Fricke TR, Wilson DA, Jong M, Naidoo KS, Sankaridurg P, et al. Global prevalence of myopia and high myopia and temporal trends from 2000 through 2050. Ophthalmology. 2016;123:1036–42. doi: 10.1016/j.ophtha.2016.01.006. [DOI] [PubMed] [Google Scholar]
- 2.Wang XG, Dong J, Pu YL, Liu HJ, Wu Q. Comparison axial length measurements from three biometric instruments in high myopia. Int J Ophthalmol. 2016;9:876–80. doi: 10.18240/ijo.2016.06.15. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 3.Jong M, Sankaridurg P, Li W, Resnikoff S, Naidoo K, He M. Reduced vision in highly myopic eyes without ocular pathology: The ZOC-BHVI high myopia study. Clin Exp Optometry. 2017:101. doi: 10.1111/cxo.12563. [DOI] [PubMed] [Google Scholar]
- 4.Yan J, Hu DN, Jing S, Zhou J. Correlations between MMPs and TIMPs levels in aqueous humor from high myopia and cataract patients. Curr Eye Res. 2017;42:600–3. doi: 10.1080/02713683.2016.1223317. [DOI] [PubMed] [Google Scholar]
- 5.Asonuma S, Hirota M, Kanda H, Haga H, Ikuno Y, Matsumoto T, et al. Quality of life of high myopia and influence of cataract surgery. Folia Japonica Ophthalmol Clin. 2017;10:373–9. [Google Scholar]
- 6.Chen X, Yu Y, Song X, Zhu Y, Wang W, Yao K. Clinical outcomes of femtosecond laser-assisted cataract surgery versus conventional phacoemulsification surgery for hard nuclear cataracts. J Cataract Refract Surg. 2017;43:486–91. doi: 10.1016/j.jcrs.2017.01.010. [DOI] [PubMed] [Google Scholar]
- 7.Czajka MP, Frajdenberg A, Johansson B. Comparison of 1.8-mm incision versus 2.75-mm incision cataract surgery in combined phacoemulsification and 23-gauge vitrectomy. Acta Ophthalmologica. 2016;94:507–13. doi: 10.1111/aos.12998. [DOI] [PubMed] [Google Scholar]
- 8.Ewe SY, Abell RG, Oakley CL, Lim CH, Allen PL, McPherson ZE, et al. A comparative cohort study of visual outcomes in femtosecond laser-assisted versus phacoemulsification cataract surgery. Ophthalmology. 2016;123:178–82. doi: 10.1016/j.ophtha.2015.09.026. [DOI] [PubMed] [Google Scholar]
- 9.Senthil S, Chinta S, Rao HL, Choudhari NS, Pathak-Ray V, Mandal AK, et al. Comparison of cataract surgery alone versus cataract surgery combined with trabeculectomy in the management of phacomorphic glaucoma. J Glaucoma. 2016:25. doi: 10.1097/IJG.0000000000000229. [DOI] [PubMed] [Google Scholar]
- 10.Abulafia A, Hill WE, Koch DD, Wang L, Barrett GD. Accuracy of the Barrett True-K formula for intraocular lens power prediction after laser in situ keratomileusis or photorefractive keratectomy for myopia. J Cataract Refract Surg. 2016;42:363–9. doi: 10.1016/j.jcrs.2015.11.039. [DOI] [PubMed] [Google Scholar]
- 11.Kang SI, Moon K, Jun JH. Accuracy of three intraocular lens-power formulas in predicting refractive outcomes in different intraocular lenses. J Korean Ophthalmol Soc. 2016;57:1891. [Google Scholar]
- 12.Mimouni M, Shapira Y, Jadon J, Frenkel S, Blumenthal EZ. Assessing visual function behind cataract: Preoperative predictive value of the Heine Lambda 100 retinometer. Eur J Ophthalmol. 2017;27:559. doi: 10.5301/ejo.5000993. [DOI] [PubMed] [Google Scholar]
- 13.Lenkova GA. Specific features of measuring the optical power of artificial refractive and diffractive–refractive eye lenses. Opt Spectrosc. 2016;121:310–21. [Google Scholar]
- 14.Zhang Y, Liang XY, Liu S, Lee JWY, Bhaskar S, Lam DSC. Accuracy of intraocular lens power calculation formulas for highly myopic eyes. J Ophthalmol. 2016;2016:1–7. doi: 10.1155/2016/1917268. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 15.Cooke DL, Cooke TL. Comparison of 9 intraocular lens power calculation formulas. J Cataract Refract Surg. 2016;42:1157–64. doi: 10.1016/j.jcrs.2016.06.029. [DOI] [PubMed] [Google Scholar]
- 16.Vasavada V, Shah SK, Vasavada VA, Vasavada AR, Trivedi RH, Srivastava S, et al. Comparison of IOL power calculation formulae for pediatric eyes. Eye. 2016;30:1242. doi: 10.1038/eye.2016.171. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 17.Doshi D, Limdi P, Parekh N, Gohil N. A comparative study to assess the predictability of different IOL power calculation formulas in eyes of short and long axial length. J Clin Diagn Res. 2017;11:NC01. doi: 10.7860/JCDR/2017/22095.9136. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 18.Roberts TV, Hodge C, Sutton G, Lawless M. Comparison of Hill-RBF, Barrett Universal and current third generation formulas for the calculation of intraocular lens power during cataract surgery. Clin Exp Ophthalmol. 2017;143:159–67. doi: 10.1111/ceo.13034. [DOI] [PubMed] [Google Scholar]
- 19.Ghoreyshi M, Khalilian A, Peyman M, Mohammadinia M, Peyman A. Comparison of OKULIX ray-tracing software with SRK-T and Hoffer-Q formula in intraocular lens power calculation. J Curr Ophthalmol. 2018;30:63–7. doi: 10.1016/j.joco.2017.06.008. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 20.Seo S, Lee CE, Kim YK, Lee SY, Jeoung JW, Park KH. Factors affecting refractive outcome after cataract surgery in primary angle-closure glaucoma. Clin Exp Ophthalmol. 2016;44:693–700. doi: 10.1111/ceo.12762. [DOI] [PubMed] [Google Scholar]
- 21.Liu X, Fan F, Wang X, Lu Y, Zheng T, Zhou P, et al. Long-term observation of triplex surgery for cataract after phakic 6h implantation for super high myopia. J Ophthalmol. 2016;2016:1–10. doi: 10.1155/2016/9569868. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 22.Wu Y, Liu S, Liao R. Prediction accuracy of intraocular lens power calculation methods after laser refractive surgery. BMC Ophthalmol. 2017;17:44. doi: 10.1186/s12886-017-0439-x. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 23.Chen X, Yuan F, Wu L. Metaanalysis of intraocular lens power calculation after laser refractive surgery in myopic eyes. J Cataract Refract Surg. 2016;42:163–70. doi: 10.1016/j.jcrs.2015.12.005. [DOI] [PubMed] [Google Scholar]
- 24.Reitblat O, Levy A, Kleinmann G, Lerman TT, Assia EI. Intraocular lens power calculation for eyes with high and low average keratometry readings: Comparison between various formulas. J Cataract Refract Surg. 2017;43:1149. doi: 10.1016/j.jcrs.2017.06.036. [DOI] [PubMed] [Google Scholar]
- 25.Savini G, Hoffer KJ, Lombardo M, Serrao S, Schiano-Lomoriello D, Ducoli P. Influence of the effective lens position, as predicted by axial length and keratometry, on the near add power of multifocal intraocular lenses. J Cataract Refract Surg. 2016;42:44. doi: 10.1016/j.jcrs.2015.07.044. [DOI] [PubMed] [Google Scholar]
- 26.Mckee HD, Jhanji V. Theoretical effect of lens position and corneal curvature on the near focal point of multifocal intraocular lenses. J Refract Surg. 2016;32:64. doi: 10.3928/1081597X-20151207-04. [DOI] [PubMed] [Google Scholar]
- 27.Raucau M, El HC, Agard E, Lagenaite C, Dot C. Toric lens implantation in cataract surgery: Automated versus manual horizontal axis marking, analysis of 50 cases. J Fr Ophtalmol. 2018:41. doi: 10.1016/j.jfo.2017.11.002. [DOI] [PubMed] [Google Scholar]
- 28.Vlachynska A, Oplatkova ZK, Sramka M, editors. The coordinate system of the eye in cataract surgery: Performance comparison of the circle Hough transform and Daugman; s algorithm. American Institute of Physics Conference Series. 2017 [Google Scholar]
- 29.Jiménez-Alfaro I, Gómez-Tellería G, Bueno JL, Puy P. Contrast sensitivity after posterior chamber phakic intraocular lens implantation for high myopia. J Refract Surg. 2016;17:641. doi: 10.3928/1081-597X-20011101-02. [DOI] [PubMed] [Google Scholar]
- 30.Kane JX, Van HA, Atik A, Petsoglou C. Accuracy of 3 new methods for intraocular lens power selection. J Cataract Refract Surg. 2017;43:333. doi: 10.1016/j.jcrs.2016.12.021. [DOI] [PubMed] [Google Scholar]