Abstract
Gas exchange parameters and stomatal physical properties were measured in Tradescantia virginiana plants grown under well-watered conditions and treated daily with either distilled water (control) or 3.0 mm abscisic acid (ABA). Photosynthetic capacity (CO2 assimilation rate for any given leaf intercellular CO2 concentration [ci]) and relative stomatal sensitivity to leaf-to-air vapor-pressure difference were unaffected by the ABA treatment. However, at an ambient CO2 concentration (ca) of 350 μmol mol−1, ABA-treated plants operated with significantly lower ci. ABA-treated plants had significantly smaller stomata and higher stomatal density in their lower epidermis. Stomatal aperture versus guard cell pressure (Pg) characteristics measured with a cell pressure probe showed that although the form of the relationship was similar in control and ABA-treated plants, stomata of ABA-treated plants exhibited more complete closure at Pg = 0 MPa and less than half the aperture of stomata in control plants at any given Pg. Scaling from stomatal aperture versus Pg to stomatal conductance versus Pg showed that plants grown under ABA treatment would have had significantly lower maximum stomatal conductance and would have operated with lower stomatal conductance for any given guard cell turgor. This is consistent with the observation of lower ci/ca in ABA-treated plants with a ca of 350 μmol mol−1. It is proposed that the ABA-induced changes in stomatal mechanics and stomatal conductance versus Pg characteristics constitute an improvement in water-use efficiency that may be invoked under prolonged drought conditions.
Although the plant growth regulator abscisic acid (ABA) was first identified for its role in abscision of fruits (Okhuma et al., 1963), it has since been widely recognized for its ability to regulate stomatal aperture (Little and Eidt, 1968; Mittelheuser and van Steveninck, 1969; Jones and Mansfield, 1970; Raschke, 1987; for review, see Leung and Giraudat, 1998). ABA is synthesized in several plant organs, and the increased concentrations to which stomata respond under conditions of water deficit are the result of not only synthesis and redistribution of ABA within leaves, but also synthesis and export from roots (Davies and Zhang, 1991; Dodd et al., 1996).
It has been shown that bulk leaf ABA concentration increases with increasing water stress (e.g. Wright and Hiron, 1969; Beardsell and Cohen, 1975; Pierce and Raschke, 1980), and that the stomatal conductance attainable for any given set of environmental conditions is negatively correlated with ABA concentration (Trejo et al., 1995; Tardieu et al., 1996). These and many other studies show that the short-term effects of elevated ABA concentrations are reversible, i.e. the return of ABA concentrations to those prevailing before water stress is accompanied by a return of stomatal function to near its full potential. In intact plants subjected to brief drought (several days or less) recovery of stomatal conductance and ABA concentrations to predrought levels can take 1 to 2 d (Ackerson, 1980; Henson, 1981). However, Trejo et al. (1995) showed that stomata in epidermal strips of Commelina communis that had been substantially closed by applying a 30-min pulse of 0.01 mm ABA returned to initial apertures within 3 h. These studies suggest that short periods (hours to days) of elevated leaf ABA concentrations have no permanent effect on stomatal function.
Much research into the action of ABA on stomata has focused on the mechanism by which changes in ABA concentrations in the vicinity of guard cells are transduced into changes in stomatal aperture via processes on the guard cell plasma membrane (Assmann, 1993; MacRobbie, 1995, 1998). However, little is known about the potential role of this growth regulator in promoting developmental changes in stomatal structure and arrangement within leaves, or of the functional significance of such changes. Work by McCree (1974) and Brown et al. (1976) showed that when plants were subjected to frequent or long-term drought, their stomata reopened more readily upon rewatering than did stomata in plants experiencing only a single, brief period of drought. Based on these observations and work by Cutler et al. (1977) that showed stomata grown under water stress were smaller than in well-watered plants, Spence et al. (1986) emphasized an important distinction between long-term anatomical and short-term physiological causes of a plant's reaction to water stress. Spence et al. (1986) showed mathematically that the smaller stomata that develop in water-stressed plants are likely to be mechanically different from those in well-watered plants and may achieve greater increases in aperture for a given change of guard cell turgor under certain conditions. However, there have been no measurements of guard cell mechanical properties to support these claims.
To investigate the effects of longer-term exposure to elevated ABA concentrations, Bradford et al. (1983) sprayed leaves of young tomato plants with ABA. They found that leaves that had developed under these conditions had the same photosynthetic capacity as control plants, despite operating with different stomatal conductances and having different stomatal sizes and frequencies. However, the effects of prolonged elevated ABA concentrations on leaf gas exchange and stomatal function remain poorly understood.
Elevated concentrations of ABA in leaf tissues are usually associated with water deficit in plants, and elevated tissue ABA concentrations alone tend to promote developmental changes in stomata and leaf anatomy that mimic the effects of water deficit (Quarrie and Jones, 1977). However, the functional significance of ABA-induced changes in stomatal structure has never been quantified in terms of stomatal guard cell inflation characteristics, so the true energetic cost or benefit of these changes is difficult to assess. Our aim in this study was to use the cell pressure probe to measure the effect of long-term elevated leaf ABA concentrations on the relationship between stomatal aperture and guard cell pressure in Tradescantia virginiana. We also sought to relate this information to the overall effect of this treatment on leaf gas exchange properties. The use of exogenous ABA to chemically simulate “drought” conditions in well-watered plants enabled investigation of the influence of ABA alone (i.e. without associated reductions in leaf water potential or turgor) in permanently modifying stomatal functional characteristics.
RESULTS
Gas Exchange
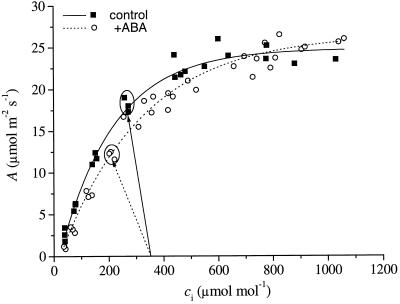
Photosynthetic capacity overall was unaltered by treatment with ABA. When the CO2 assimilation rate (A) is plotted against the leaf intercellular CO2 concentration (ci), the relationship for ABA-treated plants is similar to that for control plants (Fig. 1). The data in Figure 1 are well characterized by the photosynthesis model of Farquhar and von Caemmerer (1982), but for ease of comparison, in Figure 1 we have fitted a single exponential decay function to the data for control and ABA-treated plants (y = 24.8 − 27.4e(−x/198), r2 = 0.99 for control; y = 26.5 − 28.5e(−x/300), r2 = 0.98 for ABA-treated plants). Overall, the relationship between A and ci was similar between control and ABA-treated plants, suggesting ABA had little, if any, influence on overall photosynthetic capacity. However, steady-state operating points under the initial ambient conditions (ambient CO2 concentration, ca, = 350 μmol mol−1, leaf-to-air vapor pressure difference, VPD, = 1.0 kPa) did differ significantly, with ABA-treated plants operating at lower ci (points circled and indicated by arrows in Fig. 1). Mean ± se ci/ca was 0.764 ± 0.013 for control and 0.600 ± 0.016 for ABA-treated plants.
Figure 1.
Plot of A against ci for control and ABA-treated plants. Data are the combined results for three control and three ABA-treated plants. Solid and dotted lines are nonlinear least-squares fits of first-order exponential decay functions to data for control and ABA-treated plants, respectively (y = 24.8 − 27.4e(−x/198), r2 = 0.99 for control; y = 26.5 − 28.5e(−x/300), r2 = 0.98 for ABA-treated plants). Solid and dotted arrows point to initial operating points (circled) for control and ABA-treated plants, respectively (ca = 350 μmol mol−1, vapor-pressure difference [VPD] = 1.0 kPa). Leaf temperature 25°C, photosynthetically active radiation 800 μmol m−2 s−1.
Although transpiration rates (E) differed between control and ABA-treated plants, on account of different stomatal conductances, changes in E following a step change in VPD were similar when expressed as a percentage reduction from the value at VPD = 1.0 kPa. Mean ± se A (μmol m−2 s−1), stomatal conductance to water vapor (gs; steady-state stomatal conductance at the initial 1.0 kPa, at 2.0 kPa, and the final 1.0 kPa are denoted gs1, gs2, and gs3, respectively; mol m−2 s−1), and E (mmol m−2 s−1) at the initial 1.0-kPa VPD were, respectively, 18.1 ± 0.49, 0.451 ± 0.03, and 3.60 ± 0.12 for control and 12.1 ± 0.27, 0.149 ± 0.01, and 1.42 ± 0.04 for ABA-treated plants. A step increase in VPD from 1.0 kPa to 2.0 kPa resulted in mean reductions in stomatal conductance of 18% and 14% for control and ABA-treated plants, respectively (gs2/gs1 = 0.82 ± 0.04 for control and 0.86 ± 0.05 for ABA-treated plants). Following a return from 2.0-kPa to 1.0-kPa VPD, stomatal conductances increased, but recovery was not complete, with stomatal conductance of control plants recovering up to 90% of the initial 1.0-kPa VPD value, and ABA-treated plants recovering up to 94% (gs3/gs1 = 0.90 ± 0.06 for control and 0.94 ± 0.03 for ABA-treated plants). Mean gs2/gs1 and gs3/gs1 did not differ significantly between control and ABA-treated plants at the 5% level.
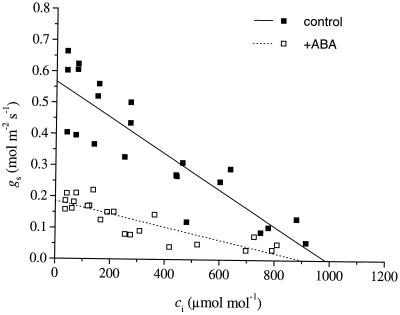
Steady-state relationships between gs and ci for control and ABA-treated plants, at 1.0-kPa VPD, are shown in Figure 2. Linear regressions were performed on the grouped data for the three control and three ABA-treated plants (y = 0.57 − 0.58 × 10−3x, r2 = 0.79 for control; y = 0.19 − 0. 20 × 10−3 x, r2 = 0.72 for ABA-treated plants). Fitting a linear model to these data gives a good approximation of the relationship between gs and ci, although there is evidence to suggest the relationship is more likely to be curvilinear (Wong et al., 1978; Morison and Jarvis, 1983). Some of the individual plants in this study exhibited a clear sigmoidal relationship between gs and ci (data not shown). The 95% confidence intervals calculated for the slopes and intercepts of the linear regressions in Figure 2 revealed significant differences, i.e. when averaged over the range 40 < ci < 900 μmol mol−1, the absolute sensitivity of stomata of ABA-treated plants to ci was significantly lower than the control plants under these experimental conditions. However, relative sensitivity remained unchanged. Also, when sensitivity to ci is measured only over the range of ci corresponding to a step reduction in ca from 350 to 200 μmol mol−1, there is evidently a high degree of variability in the sensitivities between individual plants (Δgs/Δci = −1053, −534, and −357 mol m−2 s−1 for the three controls and Δgs/Δci = −3393, −213, and −264 mol m−2 s−1 for the three ABA-treated plants).
Figure 2.
Steady-state relationships between gs and ci for control and ABA-treated plants, at 1.0-kPa VPD. Data are composites for three control and three ABA-treated plants. Leaf temperature 25°C, photosynthetically active radiation 800 μmol m−2 s−1.
Leaf Turgor and Anatomical Measurements
Epidermal turgor (Pe), stomatal density in upper (nsu) and lower (nsl) epidermis, guard cell length in upper (Lsu) and lower (Lsl) epidermis, and mean stomatal ratio (S) are summarized in Table I. The significance of difference between means was tested using analysis of variance. Within treatments, both control and ABA-treated plants showed significantly higher stomatal densities in lower versus upper epidermes (variance ratio [F] = 490, P < 0.001, and n = 30). Overall, stomatal densities that we measured are typical of T. virginiana, which is known to have very low stomatal densities (Willmer, 1983). Unlike control plants, the ABA-treated plants had significantly longer guard cells in upper versus lower epidermes (F = 37.7, P < 0.001, and n = 45). Between treatments, ABA-treated plants maintained significantly lower epidermal cell turgor in vitro, compared with control plants (F = 26.8, P < 0.001, and n = 30). nsu did not differ significantly between control and ABA-treated plants, but nsl of ABA-treated plants was significantly higher than in control plants (F = 524, P < 0.001, and n = 30); hence, the stomatal ratio was lower in ABA-treated plants. Compared with control plants, mean guard cell length was significantly shorter in both upper and lower epidermes of ABA-treated plants (F = 253, P < 0.001, and n = 45 for upper; F = 443, P < 0.001, and n = 45 for lower).
Table I.
Pe, nsu, nsl, Lsu, Lsl, and S (S = nsu/nsl) in control and ABA-treated plants
| Treatment | Pea MPa | nsl | nsu | Lsu | Lsl | S |
|---|---|---|---|---|---|---|
| mm−2 | μm | |||||
| Control | 0.56 ± 0.01 | 11.4 ± 0.27 | 4.24 ± 0.17 | 86.6 ± 0.75 | 88.6 ± 1.11 | 0.37 ± 0.01 |
| ABA-treated plants | 0.47 ± 0.02 | 19.7 ± 1.28 | 4.47 ± 0.25 | 68.4 ± 0.87 | 62.0 ± 0.61 | 0.23 ± 0.01 |
All figures are mean ± se; n = 30 for Pe, nsu, nsl, S; n = 45 for Lsu, Lsl.
As measured in peels from lower epidermes.
Guard Cell Aperture/Pressure Characteristics
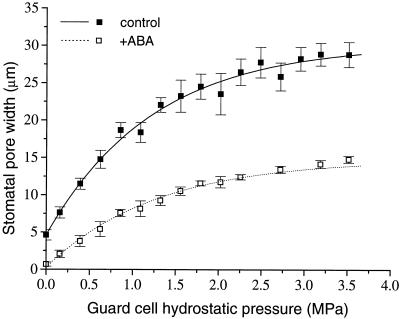
Stomatal pore width as a function of guard cell hydrostatic pressure is shown in Figure 3. The form of this relationship in both ABA-treated and control plants is the same, i.e. continuous negative curvature over the range of 0 to 4 MPa. The smaller physical size of guard cells in ABA-treated plants resulted in apertures of less than half the width of control plants for any given guard cell pressure. First-order exponential decay functions were fitted to the data using a nonlinear least-squares fitting procedure. These functions provide an accurate mathematical description of the relationship between stomatal aperture and guard cell hydrostatic pressure (or turgor) in T. virginiana under conditions of 0 Pe (for control plants y = 30.2 − 25.6e−x/1.23, r2 = 0.988; for ABA-treated plants y = 14.8 − 14.6e−x/1.27, r2 = 0.991). The smaller maximum possible aperture of stomata in ABA-treated plants is due largely to shorter guard cell length because the width of guard cells was similar in both control and ABA-treated plants (approximately 18 μm).
Figure 3.
The relationship between stomatal aperture and guard cell hydrostatic pressure, measured at 0 Pe, for control and ABA-treated plants. Each datum point is the mean ± se for between three and 13 stomata.
DISCUSSION
Measurement of A as a function of ci (Fig. 1) revealed exogenous ABA had no significant effect on the photosynthetic capacity of T. virginiana (defined here as A for a given ci). A versus ci characteristics did not differ overall between ABA-treated and control plants. However, plants grown under ABA treatment operated at lower ci/ca and hence with higher water-use efficiency due to lower stomatal conductance.
There has been mixed opinion as to whether ABA has a direct effect on photosynthetic capacity. Several studies have concluded that ABA fed to the transpiration stream has a direct effect on carbon fixation (Cornic and Miginiac, 1983; Raschke and Hedrich, 1985; Ward and Bunce, 1987). However, other studies of isolated mesophyll cells (Mawson et al., 1981) or whole-leaf gas exchange (Dubbe et al., 1978; Bradford et al., 1983) have found no evidence of reduced photosynthetic capacity following ABA treatment. It has been proposed that in cases where an effect is observed, ABA could be acting (probably indirectly) to inhibit the activity of ribulose-1,5-bisphosphate carboxylase (Fischer et al., 1986; Popova et al., 1996). It has been shown that patchy distribution of stomatal conductance, which can be induced by application of ABA, can give the illusion of reduced photosynthetic capacity (Terashima et al., 1988; Mott, 1995). It should be noted that many of these studies have dealt with only short-term responses. Our results (and those of Bradford et al. [1983] with which our data agree) relate to long-term exposure to elevated ABA concentrations where leaves have grown and matured under such conditions.
The similarity of response to VPD for control and ABA-treated plants suggests that either (a) The VPD response mechanism is independent of physical changes in guard cell structure induced by growth under elevated ABA concentrations, or (b) the VPD response mechanism is a highly conservative property and that changes in stomatal structure associated with elevated ABA concentrations (or water stress) are linked to the maintenance of this property. There are insufficient published data with which to explore these possibilities. Several studies have observed altered stomatal sensitivities to VPD following imposition of water stress on fully developed leaves (Schulze and Küppers, 1979; Turner et al., 1985; Nonami et al., 1990), but it remains unclear as to how the VPD response is affected in leaves that develop entirely under water stress and/or elevated ABA concentrations.
Our observations of increased stomatal density and smaller stomatal dimensions in ABA-treated plants (Table I) are similar to those of Bradford et al. (1983) who grew tomato under artificially elevated ABA (leaves sprayed daily with 10 or 30 μm ABA). Quarrie and Jones (1977) observed similar trends in wheat leaves injected with ABA. The same effect has been observed in studies where plants were grown under water stress without any artificial manipulation of ABA concentrations (Cutler et al., 1977; Quarrie and Jones, 1977; Spence et al., 1986; Xia, 1994). However, it remains to be determined whether ABA is the main chemical influencing developmental changes in stomatal properties under water stress.
Increased nsl of ABA-treated leaves could amount to a shifting of greater transpiration control to the lower epidermis, but the full advantage of this is unclear. Several morphological adaptations associated with growth under water stress are likely to contribute to an overall improvement in water-use efficiency. Although we did not measure leaf areas precisely, leaves from ABA-treated plants were noticeably smaller, and this may have reduced total plant transpirational losses (Quarrie and Jones, 1977). However, it is this in combination with stomatal and hydraulic properties that will determine plant water status.
The results in Figure 3 show that the application of ABA has altered the physical properties of stomata in T. virginiana. For any given guard cell hydrostatic pressure, mean stomatal pore width in the ABA-treated plants is less than half that in control plants. To assess whether this was likely to result in different stomatal conductances, we applied an adaptation of the original Brown and Escombe (1900) model, which has been shown to give a reliable approximation of stomatal conductance using stomatal dimensions (Penman and Schofield, 1951; Bange, 1953; Lee and Gates, 1964). Using the molar terms of Cowan (1977), this model may be written as:
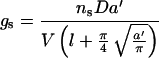 |
1 |
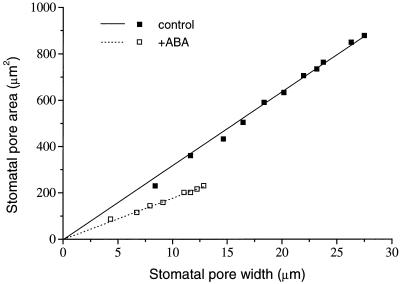
where gs is stomatal conductance to water vapor (excluding boundary layer), ns = stomata per unit epidermal area (m−2; using nsu for calculating conductance of upper leaf surface, and nsl for conductance of lower leaf surface), D = diffusivity of water in air (m2 s−1), a ' = mean stomatal pore area (m2), V = molar volume of air (m3 mol−1), l = depth of stomatal pore (m), and π is 3.142. In T. virginiana, a ' is linearly related to stomatal pore width (Fig. 4). Similar linear relationships have also been observed in Vicia faba (Raschke, 1979) and C. communis (Weyers and Meidner, 1990). The term:
 |
2 |
is the “end correction” accounting for diffusion shells at the outside end of stomatal pores. There is some difference of opinion as to the calculation of this end correction (see discussions by Nobel, 1983; Weyers and Meidner, 1990) and also whether or not to apply the same correction to both the inside and outside ends of the stomatal pore. It has been suggested (e.g. Bange, 1953) that in some cases the end correction on the inside of stomatal pores will be negligible for water vapor diffusing out of leaves, and we found that applying the above-end correction to both ends of the stomatal pore tended to underestimate stomatal conductance. Underestimates of similar magnitude also resulted from application of the end correction developed by Parlange and Waggoner (1970), although it was used successfully with Avena fatua (van Gardingen et al., 1989).
Figure 4.
The relationship between stomatal pore area a ' (μm2) and stomatal pore width a (μm), as measured for typical stomata from control and ABA-treated T. virginiana plants. Solid and dotted lines are linear regressions of a ' on a for control and ABA-treated plants, respectively. For control, a ' = 31.7a, r2 = 0.998; for ABA-treated plants, a ' = 17.8a, r2 = 0.991.
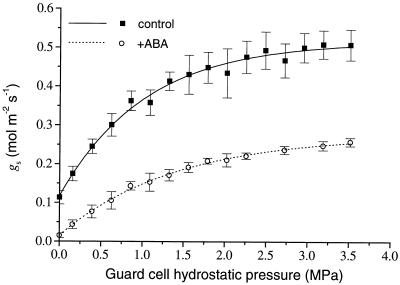
Estimates of stomatal conductance for T. virginiana at 0 Pe (Fig. 5) show that ABA-treated plants would have operated with substantially lower stomatal conductance for any given guard cell turgor, and would also have had a significantly lower maximum stomatal conductance. Even if epidermal cells in ABA-treated plants had a greater mechanical advantage over guard cells than those in control plants (due to the smaller physical dimensions of guard cells in ABA-treated plants), the ABA-treated plants were still likely to have operated with lower stomatal conductances for any given combination of epidermal and guard cell turgor. This situation may not hold if, for any given set of environmental conditions, guard cell turgor and Pe are substantially different between control and ABA-treated plants. This possibility awaits further experimental verification. However, our gas exchange results show that plants grown under ABA treatment operated with lower stomatal conductance over a range of ci and VPD conditions, which is consistent with the information in Figure 5.
Figure 5.
An estimate of the relationship between stomatal conductance (gs) and guard cell hydrostatic pressure (Pg) at 0 Pe, for control and ABA-treated plants. gs was calculated using Equation 1 and information from Table I and Figures 3 4. Each point is the calculated mean ± se. See Table II for an example calculation. Solid and dotted lines are nonlinear least-squares fits of first-order exponential decay functions to data for control and ABA-treated plants, respectively (y = 0.52 − 0.40e(−x/1.03), r2 = 0.990 for control; y = 0.28 − 0.26e(−x/1.39), r2 = 0.998 for ABA-treated plants).
We conclude that ABA applied daily to leaf surfaces of T. virginiana under well-watered conditions produced leaves with much-reduced stomatal size and potential stomatal conductance, but with unaltered photosynthetic capacity. Although this is likely to indicate to some extent the effect of drought-induced increases in leaf ABA concentrations during leaf development, the results presented here will help to clarify the role of ABA alone in the more complex mechanism of plant adaptation to long-term drought. Hence, in terms of “stomatal” and “non-stomatal” components of plant response to drought, it would appear that ABA operates directly and, at least in this case, exclusively on the “stomatal” component. Therefore, ABA not only regulates short-term, reversible adjustments to rates of carbon uptake and water loss, but through its effect on stomatal structure has the potential also to permanently alter the leaf photosynthetic operating point in the direction of improved water-use efficiency.
MATERIALS AND METHODS
Plant Material
Tradescantia virginiana plants were cloned by detaching plantlets (roots plus shoots) from parent plants and placing them immediately in 3-L pots filled with soil (compost:sand:peat:perlite, 5:2:2:1). Existing mature leaves were trimmed to a height of 4 cm and the plants were grown in a greenhouse (day/night air temperature 30°C/25°C, high humidity) under 50% shade cloth. Plants were well watered at all times. A slow-release fertilizer (Osmocote, Scotts Australia Pty Ltd, Castle Hill, Australia) was added in one application after 2 weeks (15 g/pot). After 3 weeks, when new shoots had begun to appear, one-half of the pots were selected at random and treated with 1 mL of 3.0 mm ABA (Sigma-Aldrich Pty Ltd, Castle Hill, Australia) twice daily at the base of an emerging leaf. Treatment lasted 14 d in total. The ABA solution formed a well between the base of the emerging leaf and the base of an adjacent mature leaf. The remaining untreated plants (control) were given 1 mL distilled water in the same manner as treated plants. Leaves that had emerged and matured after commencement of treatments were used for subsequent gas exchange, pressure probe, and anatomical measurements.
Gas Exchange
Leaf gas exchange measurements were performed with a commercial, open-flow gas exchange measurement system (LI-6400P, LI-COR Inc., Lincoln, NE). All experiments were carried out during the natural daylight photoperiod, with plants brought to the laboratory on the evening prior to measurements. ABA treatment was stopped 24 h prior to gas exchange measurements, and leaves were thoroughly rinsed with tap water. For all measurements leaf temperature was 25°C and leaf irradiance was 800 μmol m−2 s−1. Measurements were taken on attached, mature leaves approximately halfway along their length, with about 3.6 cm2 of the leaf inside the measurement cuvette. Stomatal response to leaf-to-air VPD was assessed by first allowing the leaf to reach a steady state at 1.0-kPa VPD, 350 μmol mol−1 ca, and then stepping VPD up to 2.0 kPa. After steady state had again been reached, VPD was returned to 1.0 kPa. The time between these steady states was at least 40 min, and in most cases 60 min, following the step reduction from 2.0 to 1.0 kPa. At each steady state, A, gs, E, and ci were obtained. Steady-state stomatal conductance at the initial 1.0 kPa, at 2.0 kPa, and the final 1.0 kPa are denoted gs1, gs2, and gs3, respectively. Stomatal sensitivity to the step increase in VPD, for these particular conditions, was quantified as gs2/gs1. With the same leaf, the relationship between A and ci, and the steady-state relationship between gs and ci, were obtained by first decreasing and then increasing ca in steps, beginning with steady state at 350 μmol mol−1.
Leaf Anatomical Measurements
Epidermal peels were prepared and viewed as for Pe measurements. Means of nsu and nsl were obtained by counting stomata in 10 different circular 2.0-mm diameter fields on upper and lower epidermes of three plants from each treatment (n = 30). Means of Lsu and Lsl were obtained by sampling 15 stomata in upper and lower epidermes of three plants for each treatment (n = 45). Stomatal ratio was obtained by dividing nsu by nsl.
Pressure Probe
Pe
Epidermal peels were prepared by carefully separating epidermal tissue from sections of leaf with the aid of a dissecting microscope. Peels were stuck with the cuticle up on a well slide using a drop of “vallap” (vaseline:lanolin:paraffin, 1:1:1) and the well filled with a bathing solution comprising 25 mm MES [2-(N-morpholino) ethane-sulfonic acid] at pH 6.5 (adjusted with NaOH), 1 mm KCl, and 0.1 mm CaCl2. Epidermal cell turgor was measured in the conventional manner (for review, see Steudle, 1993) using a pressure probe of the type described below. Mean Pe was obtained by sampling 10 cells in peels from three different plants (n = 30).
Guard Cell Aperture/Pressure Characteristics
Epidermal peels were obtained as for Pe measurements and incubated for 1 h in the dark in a bathing medium having the same composition as that used for Pe measurements, except for the addition of 400 mm mannitol. The mannitol was added to induce a state of mild plasmolysis in epidermal cells so that guard cell aperture/pressure characteristics could be compared independent of Pe (Franks et al., 1998). Peels were then mounted as for Pe measurements and the slide well filled with incubating medium. The relationship between guard cell hydrostatic pressure and stomatal aperture was obtained using the equipment and technique described by Franks et al. (1995, 1998). This involved the use of a specially modified pressure probe capable of operating at high pressures (at least 6.0 MPa). In brief, using a micromanipulator (Narishige Scientific Instrument Laboratory, Tokyo) and an inverted microscope (Zeiss Axiovert 35M, Carl Zeiss, Oberkochen, Germany), the glass microcapillary of the pressure probe was inserted into the guard cells of a stoma in a manner that allowed injection of silicone oil into both cells. While ensuring no leakage of oil from the guard cells, pressure in the guard cells was increased and decreased in steps and steady-state aperture was recorded for each pressure. Images for each pressure increment were recorded digitally for later analysis using a charge-coupled device camera (RTE/CCD-1300-Y/HS, Princeton Instruments Inc., Trenton, NJ) and image capture software (Metamorph 3.51, Universal Imaging Corp., West Chester, PA).
Table II.
An example of how total stomatal conductance to gs was calculated in control and ABA-treated leaves
| Quantity | Control Leaf | ABA-Treated Leaf | Units |
|---|---|---|---|
| Pg | 1.09 | 1.09 | MPa |
| a | 18.4 × 10−6 | 8.15 × 10−6 | m |
| a′ | 0.582 × 10−9 | 0.145 × 10−9 | m2 |
| nsu | 4.24 × 10−6 | 4.47 × 10−6 | m−2 |
| nsl | 11.4 × 10−6 | 19.7 × 10−6 | m−2 |
| D | 24.9 × 10−6 | 24.9 × 10−6 | m2 s−1@ 25°C |
| l | 18 × 10−6 | 18 × 10−6 | m |
| V | 24.4 × 10−3 | 24.4 × 10−3 | m3 mol−1@ 25°C, 101.3 kPa |
| gs(upper) | 0.122 | 0.028 | mol m−2 s−1@ 25°C, 101.3 kPa |
| gs(lower) | 0.236 | 0.125 | mol m−2 s−1@ 25°C, 101.3 kPa |
| gs(total) | 0.358 | 0.153 | mol m−2 s−1@ 25°C, 101.3 kPa |
For this example, Pg is 1.09 MPa. Pe = 0. From Figure 4, a′ = 31.7 × 10−6a for control; a′ = 17.8 × 10−6a for ABA-treated plants. Note that in this table a and a′ are in units of m and m2, respectively, whereas in Figure 4, units are μm and μm2. Stomatal conductances for upper leaf surface (gs(upper)) and lower leaf surface (gs(lower)) were obtained from Equation 1 using values of nsu and nsl from Table I. gs(total) = gs(upper) + gs(lower).
ACKNOWLEDGMENTS
We thank O. Schwartz and S.C. Wong for excellent technical assistance.
LITERATURE CITED
- Ackerson RC. Stomatal response of cotton to water stress and abscisic acid as affected by water stress history. Plant Physiol. 1980;65:455–459. doi: 10.1104/pp.65.3.455. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Assmann SM. Signal transduction in guard cells. Annu Rev Cell Biol. 1993;9:345–375. doi: 10.1146/annurev.cb.09.110193.002021. [DOI] [PubMed] [Google Scholar]
- Bange GGJ. On the quantitative explanation of stomatal transpiration. Acta Bot Neerl. 1953;2:255–297. [Google Scholar]
- Beardsell MF, Cohen D. Relationship between leaf water status, abscisic acid levels, and stomatal resistance in maize and sorghum. Plant Physiol. 1975;56:207–212. doi: 10.1104/pp.56.2.207. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Bradford KJ, Sharkey TD, Farquhar GD. Gas exchange, stomatal behavior, and δ13C values of the flacca tomato mutant in relation to abscisic acid. Plant Physiol. 1983;72:245–250. doi: 10.1104/pp.72.1.245. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Brown HT, Escombe F. Static diffusion of gases and liquids in relation to the assimilation of carbon and translocation in plants. Philos Trans R Soc Ser B. 1900;193:223–291. [Google Scholar]
- Brown KW, Jordan WR, Thomas JC. Water stress induced alterations of the stomatal response to decreases in leaf water potential. Physiol Plant. 1976;37:1–5. [Google Scholar]
- Cornic G, Miginiac E. Nonstomatal inhibition of net CO2 uptake by (±) abscisic acid in Pharbitis nil. Plant Physiol. 1983;73:529–533. doi: 10.1104/pp.73.3.529. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Cowan IR. Stomatal behavior and environment. Adv Bot Res. 1977;4:117–228. [Google Scholar]
- Cutler JM, Rains DW, Loomis RS. The importance of cell size in the water relations of plants. Physiol Plant. 1977;40:255–260. [Google Scholar]
- Davies WJ, Zhang JH. Root signals and the regulation of growth and development of plants in drying soil. Annu Rev Plant Physiol Plant Mol Biol. 1991;42:55–76. [Google Scholar]
- Dodd IC, Stikic R, Davies WJ. Chemical regulation of gas exchange and growth of plants in drying soil in the field. J Exp Bot. 1996;47:1475–1490. [Google Scholar]
- Dubbe DR, Farquhar GD, Raschke K. Effect of abscisic acid on the gain of the feedback loop involving carbon dioxide and stomata. Plant Physiol. 1978;62:413–417. doi: 10.1104/pp.62.3.413. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Farquhar DD, von Caemmerer S. Modeling of photosynthetic response to environmental conditions. In: Lange OL, Nobel PS, Osmond CB, Ziegler H, editors. Encyclopedia of Plant Physiology New Series. 12B. Berlin: Springer-Verlag; 1982. pp. 550–587. [Google Scholar]
- Fischer EK, Raschke K, Stitt Effects of abscisic acid on photosynthesis in whole leaves: changes in CO2 assimilation, levels of carbon reduction cycle intermediates, and activity of ribulose 1,5-bisphosphate carboxylase. Planta. 1986;169:536–545. doi: 10.1007/BF00392104. [DOI] [PubMed] [Google Scholar]
- Franks PJ, Cowan IR, Farquhar GD. A study of stomatal mechanics using the cell pressure probe. Plant Cell Environ. 1998;21:94–100. [Google Scholar]
- Franks PJ, Cowan IR, Tyerman SD, Cleary AL, Lloyd J, Farquhar GD. Guard cell pressure/aperture characteristics measured with the pressure probe. Plant Cell Environ. 1995;18:795–800. [Google Scholar]
- Henson IE. Abscisic acid and after-effects of water stress in pearl millet (Pennisetum americanum (L.) Leeke) Plant Sci Lett. 1981;21:129–135. [Google Scholar]
- Jones RJ, Mansfield TA. Suppression of stomatal opening in leaves treated with abscisic acid. J Exp Bot. 1970;21:714–719. [Google Scholar]
- Lee R, Gates DM. Diffusion resistance in leaves as related to their stomatal anatomy and microstructure. Am J Bot. 1964;51:963–975. [Google Scholar]
- Leung J, Giraudat J. Abscisic acid signal transduction. Annu Rev Plant Physiol Plant Mol Biol. 1998;49:199–222. doi: 10.1146/annurev.arplant.49.1.199. [DOI] [PubMed] [Google Scholar]
- Little CHA, Eidt DC. Effect of abscisic acid on bud break and transpiration in woody species. Nature. 1968;220:498–499. [Google Scholar]
- MacRobbie EAC. ABA-induced ion efflux in stomatal guard cells: multiple actions of ABA inside and outside the cell. Plant J. 1995;7:565–576. [Google Scholar]
- MacRobbie EAC. Signal transduction and ion channels in guard cells. Philos Trans R Soc Lond Ser B. 1998;353:1475–1488. doi: 10.1098/rstb.1998.0303. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Mawson BT, Colman B, Cummins WR. Abscisic acid and photosynthesis in isolated leaf mesophyll cell. Plant Physiol. 1981;67:233–236. doi: 10.1104/pp.67.2.233. [DOI] [PMC free article] [PubMed] [Google Scholar]
- McCree KJ. Changes in stomatal response characteristics of grain sorghum produced by water stress during growth. Crop Sci. 1974;14:273–278. [Google Scholar]
- Mittelheuser CJ, van Steveninck RFM. Stomatal closure and inhibition of transpiration induced by (RS)-abscisic acid. Nature. 1969;221:281–282. [Google Scholar]
- Morison JIL, Jarvis PG. Direct and indirect effects of light on stomata: II. In Commelina communis L. Plant Cell Environ. 1983;6:103–109. [Google Scholar]
- Mott KA. Effects of patchy stomatal closure on gas exchange measurements following abscisic acid treatment. Plant Cell Environ. 1995;18:1291–1300. [Google Scholar]
- Nobel PS. Biophysical Plant Physiology and Ecology. New York: WH Freeman and Company; 1983. [Google Scholar]
- Nonami H, Schulze E-D, Ziegler H. Mechanisms of stomatal movement in response to air humidity, irradiance and xylem water potential. Planta. 1990;183:57–64. doi: 10.1007/BF00197567. [DOI] [PubMed] [Google Scholar]
- Okhuma K, Lyon JL, Addicott FT, Smith OE. Abscisin II, an abscission accelerating substance from young cotton fruit. Science. 1963;142:1592–1593. doi: 10.1126/science.142.3599.1592. [DOI] [PubMed] [Google Scholar]
- Parlange J-Y, Waggoner PE. Stomatal dimensions and resistance to diffusion. Plant Physiol. 1970;46:337–342. doi: 10.1104/pp.46.2.337. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Penman HL, Schofield RK. Some physical aspects of assimilation and transpiration. Symp Soc Exp Biol. 1951;5:115–129. [Google Scholar]
- Pierce M, Raschke K. Correlation between loss of turgor and accumulation of abscisic acid in detached leaves. Planta. 1980;148:174–182. doi: 10.1007/BF00386419. [DOI] [PubMed] [Google Scholar]
- Popova LP, Tsonev TD, Lazova GN, Stoinova ZG. Drought and ABA-induced changes in photosynthesis of barley plants. Physiol Plant. 1996;96:623–629. [Google Scholar]
- Quarrie SA, Jones HG. Effects of abscisic acid and water stress on development and morphology of wheat. J Exp Bot. 1977;28:192–203. [Google Scholar]
- Raschke K. Movements of stomata. In: Hampt W, Feinleib ME, editors. Encyclopedia of Plant Physiology. Vol. 7. Berlin: Springer; 1979. pp. 381–441. [Google Scholar]
- Raschke K. Action of abscisic acid on guard cells. In: Zeiger E, Farquhar GD, Cowan IR, editors. Stomatal Function. Stanford, CA: Stanford University Press; 1987. pp. 253–279. [Google Scholar]
- Raschke K, Hedrich R. Simultaneous and independent effects of abscisic acid on stomata and the photosynthetic apparatus in whole leaves. Planta. 1985;163:105–118. doi: 10.1007/BF00395904. [DOI] [PubMed] [Google Scholar]
- Schulze E-D, Küppers M. Short-term and long-term effects of plant water deficits on stomatal response to humidity in Corylus avellana L. Planta. 1979;146:319–326. doi: 10.1007/BF00387804. [DOI] [PubMed] [Google Scholar]
- Spence RD, Wu H, Sharpe PJH, Clark KG. Water stress effects on guard cell anatomy and the mechanical advantage of the epidermal cells. Plant Cell Environ. 1986;9:197–202. [Google Scholar]
- Steudle E. Pressure probe techniques: basic principles and application to studies of water and solute relations at the cell, tissue and organ level. In: Smith JAC, Griffiths H, editors. Water Deficits: Plant Responses from Cell to Community. Oxford: BIOS Scientific Publishers; 1993. pp. 5–36. [Google Scholar]
- Tardieu F, Lafarge T, Simonneau TH. Stomatal control by fed or endogenous xylem ABA in sunflower: interpretation of correlations between leaf water potential and stomatal conductance in anisohydric species. Plant Cell Environ. 1996;19:75–84. [Google Scholar]
- Terashima I, Wong SC, Osmond CB, Farquhar GD. Characterization of non-uniform photosynthesis induced by abscisic acid in leaves having different mesophyll anatomies. Plant Cell Physiol. 1988;29:385–394. [Google Scholar]
- Trejo CL, Clephan AL, Davies WJ. How do stomata read abscisic acid signals? Plant Physiol. 1995;109:803–811. doi: 10.1104/pp.109.3.803. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Turner NC, Schulze E-D, Gollan T. The response of stomata and leaf gas exchange to vapor pressure deficits and soil water content: II. In the mesophytic herbaceous species Helianthus annuus. Oecologia. 1985;65:348–355. doi: 10.1007/BF00378908. [DOI] [PubMed] [Google Scholar]
- van Gardingen PR, Jeffree CE, Grace J. Variation in stomatal aperture in leaves of Avena fatua L. observed by low temperature scanning electron microscopy. Plant Cell Environ. 1989;12:887–898. [Google Scholar]
- Ward DA, Bunce JA. Abscisic acid simultaneously decreases carboxylation efficiency and quantum yield in attached soybean leaves. J Exp Bot. 1987;38:1182–1192. [Google Scholar]
- Weyers J, Meidner H. Methods in Stomatal Research. Harlow, UK: Longman Scientific and Technical; 1990. [Google Scholar]
- Willmer CM. Stomata. New York: Longman; 1983. [Google Scholar]
- Wong SC, Cowan IR, Farquhar GD. Leaf conductance in relation to assimilation in Eucalyptus pauciflora Sieb. Ex Spreng. Plant Physiol. 1978;62:670–674. doi: 10.1104/pp.62.4.670. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Wright STC, Hiron RWP. (+)- Abscisic acid, the growth inhibitor induced in detached wheat leaves by a period of wilting. Nature. 1969;224:719–720. [Google Scholar]
- Xia MZ. Effects of soil drought during the generative development phase of faba bean (Vicia faba) on photosynthetic characteristics and biomass production. J Agric Sci. 1994;122:67–72. [Google Scholar]