Parasite resource manipulation drives bimodal variation in infection duration

Abstract

Over a billion people on earth are infected with helminth parasites and show remarkable variation in parasite burden and chronicity. These parasite distributions are captured well by classic statistics, such as the negative binomial distribution. But the within-host processes underlying this variation are not well understood. In this study, we explain variation in macroparasite infection outcomes on the basis of resource flows within hosts. Resource flows realize the interactions between parasites and host immunity and metabolism. When host metabolism is modulated by parasites, we find a positive feedback of parasites on their own resources. While this positive feedback results in parasites improving their resource availability at high burdens, giving rise to chronic infections, it also results in a threshold biomass required for parasites to establish in the host, giving rise to acute infections when biomass fails to clear the threshold. Our finding of chronic and acute outcomes in bistability contrasts with classic theory, yet is congruent with the variation in helminth burdens observed in human and wildlife populations.

1. Background

Over one billion people are infected with parasitic worms and suffer the health costs of hosting helminths [1,2]. Yet hosts vary tremendously in their duration of infection, parasite burden [3] and subsequent morbidity outcomes [1,4]. Because of this variation, host populations typically have a few individuals with many parasites while most individuals have few or no parasites [3]. Variation in parasite burden has been classically formalized by the overdispersed negative binomial distribution [3,5], but the biology underlying this pattern remains unclear [6]. Many of the hypotheses to explain variation in parasite burden relate to host heterogeneity in susceptibility, recovery or exposure [7,8]. In this study we focus on infection duration, the inverse of recovery, and show that variation in infection duration can generate realistic variation in parasite burden. We then identify key within-host processes that lead to divergence in duration. Because parasite burden and infection duration drive many health costs of infection [1,2], it is crucial to understand the within-host processes that lead some hosts to expel parasites quickly in acute infections while others suffer persistent infections.

To elucidate these critical within-host processes, we integrate the two dynamic perspectives that have dominated within-host theory: resource competition between host and parasite [9], and immunity-driven top-down control of parasite growth by the host [10]. Since ultimately parasite growth as well as the host immune response relies on resources [11,12], we focus on within-host resource flows and the impact of the parasite on host metabolism.

Parasite modulation of the immune system is well-studied [13–15], but parasites are also capable of modulating host energy dynamics through altered resource uptake or reduced digestive transit time [16]. However, the implications of these manipulations are poorly understood, despite the fact that resource modulation by parasites is widespread (e.g. [17]) and is probably decisive for infection outcome [12], especially under food-limited conditions [18–21].

Here we represent host resource dynamics using dynamic energy budget theory [22,23], and build a novel framework to analyse the within-host interaction between parasite and host. Our model accounts for within-organism resource flows by integrating the dynamics of food intake, metabolism, and growth for hosts, and by including the resource-dependent immune response and parasite growth. We formulate the model on the basis of the outcomes from mouse rewilding studies that have examined the relationships between resources, immunity and infection in a realistic setting, yet with controlled parasite exposure [24,25].

In this framework, parasites modulate within-host resources, which results in a positive feedback of the parasite population on its own resource availability (i.e. an Allee effect). This positive feedback gives rise to outcomes that vary in infection duration, parasite burden and host health. Because these outcomes are emergent results of the within-host interactions, we avoid making any a priori assumption of an acute or chronic outcome, which is the norm in theoretical epidemiology [10]. In this study we will use the terms acute and chronic to indicate infections of short and (life-) long duration. In the biomedical literature, descriptions of infections as acute or chronic often also carry connotations about both the nature of the immune response (e.g. acute implies inflammatory) and the severity of infection. Here we use these words only as a shorthand to describe the duration of infection, although we note that our modelling approach could be extended to consider the health costs of infections of short- versus long-duration.

Crucially, we find that when parasites modulate host resource flow, both acute and chronic infections are possible for identical model settings. Acute and chronic infections encompass the most extreme variation in infection outcomes, while this variation emerges from the resource-driven interaction between parasite and host. In population settings, the bistability underlying these outcomes moreover drives parasite burdens that follow negative binomial distributions, as so frequently observed in nature.

2. Methods

(a). Model formulation

To analyse how host metabolism, immunity and parasite growth interact with parasite modulation of resources, we formulate and analyse a mathematical model of these within-host processes. Using dynamic energy budget (DEB) theory [22,23] to account for resource-driven processes (where all processes and flows are translated into units of biomass), we extend the baseline DEB model to include parasite and immune processes.

As a case study for model formulation, we use a mouse-gastrointestinal nematode system (the Mus musculus–Trichuris muris interaction). A widely studied system, murine growth and immunity to infection (reviewed in [26]), allow for empirically grounded model assumptions. The host organism is modelled using biomass ‘pools’, including structural mass (S), reversible mass (R) and ingesta (G). We explicitly account for the biomass in the colon, referred to as ‘egesta’ (C), and for induced immunity (I_i, electronic supplementary material, figure S2). Structural mass and reversible mass form the two major components of total body mass. Structural mass represents essential body components, such as bone, muscle and organs, whereas reversible mass can be metabolized when food supply is low, such as fat tissue, liver glycogen stores and non-essential muscular tissue.

We use a standard demand-driven, net-production DEB model [27,28]. This model structure is representative for mammal hosts and deviates (here and elsewhere) from the model defined in [29]. The model is parametrized for M. musculus [23,30,31] and its baseline settings (host-only) result in realistic growth curves [30], as does varying food availability (electronic supplementary material, figure S3; cf. fig. 3 in [30]). With this validation, we use the full-grown mouse as the initial state for parasite infection. This assumption facilitates equilibrium analyses and is consistent with mature mice being used in the rewilding field experiments (electronic supplementary material, figure S1). All model flows and processes are discussed below, full model equations and variables are given in electronic supplementary material, figure S2, and parameter definitions and values are given in electronic supplementary material, table S1.

The host has a constant food availability, F [30], and intake is defined by size and body condition, according to $A(R,S)=(F{\iota }_{\max }{S}^{2/3})/(1+{\mathrm{e}}^{\eta ((R/S)-{\theta }_r)})$ [28]. Intake is then scaled with maximum ingestion rate, ${\iota }_{\max }$, and with structural mass to the power 2/3, following [28]. Intake is limited according to a target body condition, θ_r, the ideal ratio between reversible and structural mass (electronic supplementary material, figure S2 and table S1). The parameter η controls the steepness of the intake rate as it compensates for low body condition (electronic supplementary material, table S1).

We distinguish external ‘food’ from within-host ‘resources’, where ‘resources’ are used as the energy source for growth in either host or parasite biomass. Incoming resources (from food intake) flow into the pool of ingesta at the rate A(R,S), and flow out at rate ρ, with outflow subdivided between assimilated resources and egesta (electronic supplementary material, figure S2). The dynamics of ingesta are then

$$\frac{\mathrm{d}G}{\mathrm{d}t}=A(R,S)-\rho G.$$ 2.1

We assume that the flow processes in this compartment are fast, relative to other processes and in particular to the host–parasite interaction, such that

$$G^{\ast }=\frac{A(R,S)}{\rho }.$$ 2.2

From the resources in the ingesta, a fraction E_a(P) is assimilated (dependent on parasite biomass, P), with the remainder flowing to the egesta, C. Both the inflow (from ingesta) to and outflow from egesta occur at rate ρ, such that

$$\frac{\mathrm{d}C}{\mathrm{d}t}=\rho ((1-E_{\mathrm{a}}(P))G^{\ast }-C).$$ 2.3

Assimilated resources first cover maintenance demands, following M = m_w(S + R). M is here a ‘field’ metabolic rate, which is an average of active, resting, and moving maintenance levels. Surplus resources first go to structural mass according to the solved von Bertalanffy equation [23],

$$g(S)=3\gamma (s_{\max }^{1/3}{S}^{2/3}-S),$$ 2.4

where γ is the growth rate, and s_max the asymptotic size. Further surplus resources are allocated to reversible mass, R,

$$\frac{\mathrm{d}R}{\mathrm{d}t}={ϵ}_r(\rho E_{\mathrm{a}}(P)G^{\ast }-M(S,R)-g(S)),$$ 2.5

where ε_r is the conversion efficiency. Reversible mass decreases whenever the assimilate flow is insufficient for maintenance or structural growth costs. Equations (2.2), (2.3) and (2.5) define a balance between costs for metabolism and growth and energetic gains through feeding (electronic supplementary material, figure S2).

(b). Immune response dynamics

Host immunity consists of constitutive (baseline) immunity I_c, which equals a small fraction (c) of total mass, I_c = c(S + R) [32], and induced immunity I_i, which responds dynamically to parasite infection. Because the capacity to mount an induced immune response depends on host body condition and reserves, the resource input to induced immunity is taken directly from reversible biomass [32]. This assumption is supported by the observed correlation between measures of reversible mass (e.g. the fat-associated hormone leptin) and induced immunity (e.g. the cytokine interleukin (IL)-13, a promotor of helminth clearance) in our rewilded mouse experiment (electronic supplementary material, figure S1) [24,33], as well as by previous laboratory experiments (reviewed in [34]) and wildlife experiments [35].

The rate of resource flow to induced immunity is a function of response-fuelling reversible mass and response-provoking parasite biomass, defined as bRP, with b as the biomass flow rate per gram parasite [10]. Reversible biomass is converted to induced immunity with efficiency ε_i. Induced immunity decays at a constant rate, μ_i, so that

$$\frac{\mathrm{d}{I}_{\mathrm{i}}}{\mathrm{d}t}={ϵ}_{\mathrm{i}}bRP-{\mu }_{\mathrm{i}}{I}_{\mathrm{i}}.$$ 2.6

Because constituent and induced immunity impose additional maintenance costs to the host (captured by the parameters m_c and m_i), total maintenance cost M = m_w(S + R) + m_cI_c + m_iI_i (electronic supplementary material, table S1) [32] such that equation (2.5) becomes

$$\frac{\mathrm{d}R}{\mathrm{d}t}={ϵ}_r(\rho E_{\mathrm{a}}(P)G^{\ast }-M(S,R,I_{\mathrm{c}},I_{\mathrm{i}})-g(S))-bRP.$$ 2.7

(c). Parasite dynamics

Parasites exploit the biomass in the egesta (C) as resource, reflecting the biology of Trichuris spp. and other helminths that live in the colon [36]. Parasite resource intake rate follows a type II functional response, $({\sigma }_{\mathrm{c}}C)/(h_{\mathrm{c}}+C)$, with uptake rate σ_c and half-saturation constant h_c. Resources are converted into parasite biomass through the conversion factor ε_p. Parasite biomass decreases at background mortality rate, μ_p, reflecting mortality and metabolic losses, and at the immune-imposed rates, v_cI_c + v_iI_i, reflecting mortality or stunting of parasite growth by the immune response (electronic supplementary material, table S1). The parasite dynamics are then determined by growth from resources and mortality through top-down imposed immune responses:

$$\frac{\mathrm{d}P}{\mathrm{d}t}={ϵ}_{\mathrm{p}}\frac{{\sigma }_{\mathrm{c}}C}{h_{\mathrm{c}}+C}P-{\mu }_{\mathrm{p}}P-(v_{\mathrm{c}}{I}_{\mathrm{c}}+v_{\mathrm{i}}{I}_{\mathrm{i}})P.$$ 2.8

We explicitly account for modulation of resources by the parasite. Gastrointestinal worms can cause a decrease in the digestive efficiency of their host, either by directly stealing resources from the intestines [17], or by decreasing the assimilation efficiency [37]. To include this effect we use a saturating function of parasite biomass to reduce the proportion of ingested food that is assimilated by the host:

$$E_{\mathrm{a}}(P)={ϵ}_{\mathrm{a}}\left(1-\frac{{ϵ}_{\mathrm{Amin}}P}{h_{\mathrm{e}}+P}\right).$$ 2.9

This function simplifies to the default value of assimilation efficiency, ε_a, in absence of the parasite. The unassimilated resources that flow to the egesta are useless for the host, but exploitable by the parasite. As such, gastrointestinal parasites create a positive feedback on their own resource availability, controlled via the parameter ε_Amin, which is the fractional reduction in assimilation efficiency by the parasite (for example, ε_Amin = 0.05 translates into a 5% reduction in assimilation efficiency when the parasite burden is very high, and ε_Amin = 0.5 means that assimilation efficiency is reduced by 50%). Parameter h_e is the half-saturation level in the resource modulation by the parasite (electronic supplementary material, figure S2).

(d). Model analysis

We analysed transient (figures 1 and 2) and equilibrium (figure 3) dynamics of the system defined by equations (2.2), (2.3) and (2.6)–(2.8) with MatCont [38] (6p10), in MATLAB (version 2018b). In addition, we analysed two bifurcation points that characterize specific regions in parameter space (electronic supplementary material, figure S7) [39], which represent the persistence and invasion thresholds of the parasite. The interpretation and explanation of these techniques can be found in the electronic supplementary material.

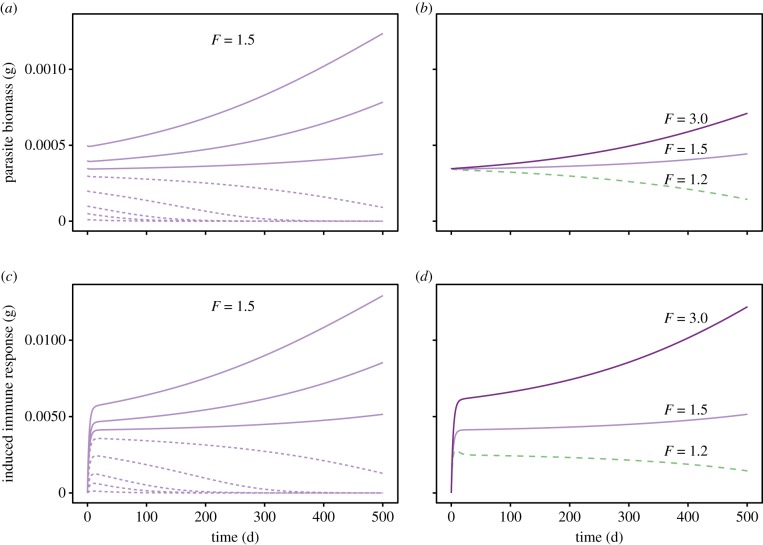
Figure 1.

Parasite biomass and induced immune response in time series starting from various initial conditions. Simulation of infections starting at eight different doses (a,c), and starting at different host food availability levels (b,d). σ_C = 0.52, other parameters have default values (electronic supplementary material, table S1). Only the first 500 time steps of the integration are shown, see electronic supplementary material, figure S4 for the extended time series with full transient dynamics up to the attractor states and for the dynamics of other system variables. (Online version in colour.)

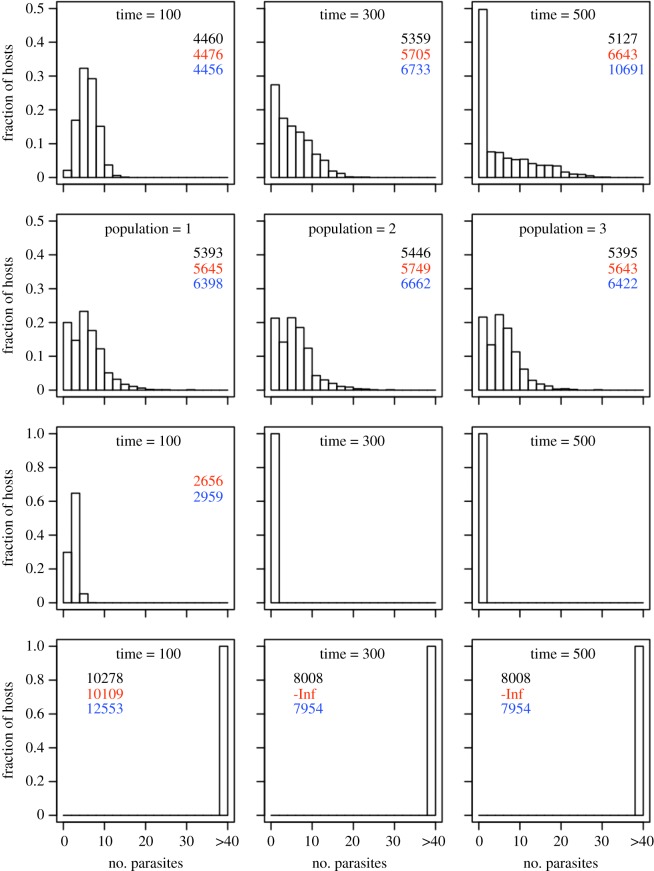
Figure 2.

Distribution of parasite burdens under different scenarios. For each scenario, 1000 hosts were infected with a parasite dose randomly drawn from a normal distribution with mean of 3 × 10⁻⁴ g and standard deviation of 8 × 10⁻⁵ g. With the default value σ_C = 0.52, this distribution centres around the unstable equilibrium in figure 3c. All parameters have default values (electronic supplementary material, table S1); to convert biomass into parasite numbers, we used the mass of an adult worm of 5 × 10⁻⁵ g. First scenario: every host is sampled at the same infection age (top row). Second scenario: each host is sampled at a different infection age, as in sampling a natural population (second row), which was repeated three times to simulate sampling three different populations. Third scenario: σ_C = 0.45, only acute infections are possible (third row). Last scenario: σ_C = 0.9, only chronic infections are possible (bottom row). Legend in panels: AIC scores from fitting different statistical distributions to distribution data using the ‘fitdistr’ function (MASS package in R; black—negative binomial, red—normal, blue—Poisson). (Online version in colour.)

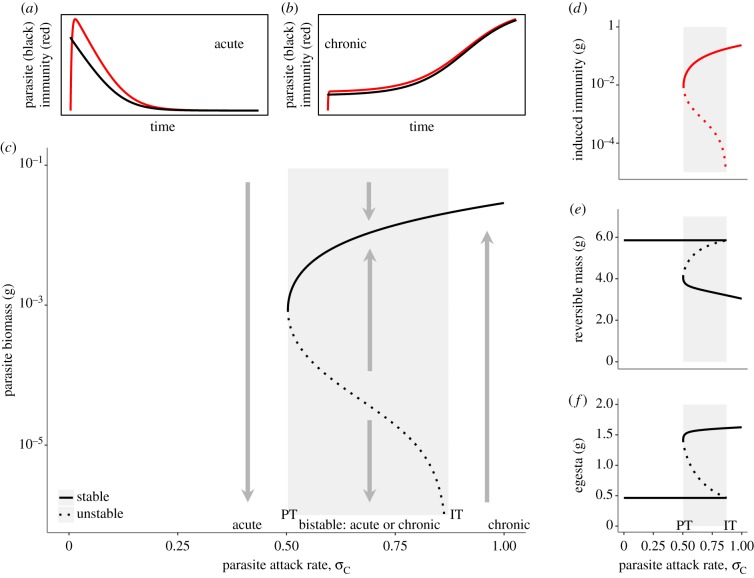
Figure 3.

Bistability with acute and chronic infections. Within-host biomass dynamics as a function of parasite attack rate (resource uptake rate). Low attack rate values (0 < σ_C < 0.5) exclusively allow for acute infections (no equilibrium with positive parasite biomass, a,c left of PT). High attack rate values (σ_C > 0.89) exclusively allow for chronic infections (only an equilibrium with high parasite biomass, b,c, right of IT). Intermediate attack rate values result in bistability between acute and chronic infection states, meaning that both the state with extinct parasite (acute) and high parasite biomass (chronic) are stable equilibria. The relationship between time series (a,b) and equilibrium solutions (c–f) is shown. Time series (a,b) illustrate the induction and waning of immune response in an acute infection (a) and the elevated endpoint in a chronic infection (b). Grey shaded area: bistable region, enclosed between the persistence threshold (PT) and invasion threshold (IT) (see electronic supplementary material). Bold, grey arrows (c) indicate dynamics in time as approaching equilibria. F = 1.5, ε_Amin = 0.4 (compare with electronic supplementary material, figure S7); other parameters have default values. (Online version in colour.)

To examine the consequences of bistability for parasite burden distributions, we projected the within-host model outcomes to the population level. We simulated infection dynamics for 1000 hosts, where the initial parasite dose (biomass) for each host was drawn from a normal distribution. Hosts were then sampled at the same or at different timepoints, to explore how the distribution of burden varied across hosts (figure 2). To fit statistical distributions to these data, including the negative binomial distribution, we used the mass of an adult T. muris parasite to convert the continuous parasite biomass measurement into a discrete number of parasites.

In addition to the extensive analysis of the DEB model (equations (2.2), (2.3), (2.6)–(2.8); electronic supplementary material, figure S2), we also analysed a simplified model that considers only parasite and resource dynamics. This simplified system is mathematically tractable and allowed us to corroborate our bistability findings analytically (electronic supplementary material).

3. Results

(a). Acute and chronic infections emerge from different initial conditions

Our model analysis shows both acute and chronic parasite infections as emerging outcomes of the within-host interactions among resource allocation, parasites and immunity (figure 1). These divergent outcomes were observed by changing the initial conditions of the model in terms of parasite dose and by changing the equilibrium configuration by adjusting the food level (see electronic supplementary material, figure S5). We do not change any assumptions about the mechanistic basis of the model, unlike standard theory in which infection duration is determined by the model's structural assumptions [10].

Parasite dose and food availability together determine infection outcome. Low parasite dose results in acute infections where the parasite is expelled, and high parasite dose results in chronic infections with parasite persistence (figure 1a). This divergence is caused by the potential for a positive feedback of the parasite on its own resource availability. Biologically, this positive feedback generates an Allee effect: if initial parasite biomass is too low, the host's immune response keeps parasite biomass low, preventing it from effectively modulating host resources and leading to acute infections, because parasites are rapidly expelled. If initial parasite biomass is high enough, the positive feedback allows the parasite to increase the resource flow towards egesta sufficiently to establish in the host, leading to chronic infections (figure 1; electronic supplementary material, figure S4).

We also simulated parasite infections using a constant initial parasite dose for three food levels. Higher food level resulted in chronic infections, whereas low food availability allowed the host to expel the parasite (figure 1c,d; electronic supplementary material, figure S4). This is somewhat counterintuitive, because higher food level improves host body condition, and therefore the strength of the immune response. Immunity was, however, counterbalanced by the fact that hosts with better body condition at the onset of infection (due to higher food levels) initially had higher resource availability for parasites (figure 1b). Parasites therefore expanded in biomass and strengthened the positive feedback on their own resources (through resource modulation), leading to a chronic infection. Low food levels provided a less profitable resource environment to the parasite. Parasites could not grow as fast and did not effectively enforce the positive feedback loop between parasite biomass and resource availability. Suppressed by the immune response, parasites were expelled and hosts experienced an acute infection (figure 1b,d; electronic supplementary material, figure S4).

The time series show diverging results and eventually stable system attractors that represent either acute or chronic outcomes for the within-host dynamics (figure 1; electronic supplementary material, figure S4). We hypothesized that such diverging outcomes for host individuals may contribute to the well-known pattern of overdispersed parasite distributions in host populations [3]. Assuming a population of hosts, each exposed to a unique parasite dose and sampled either at the same (figure 2, top row) or different (figure 2, second row) timepoints in infection, we find that the parasite burden distributions conform to a negative binomial distribution if the system state is bistable. Moreover, when parameter values are outside of the bistable region, parasite burden distributions approach entirely acute (figure 2, third row) or entirely chronic distributions (figure 2, bottom row). This final negative result confirms that a negative binomial distribution is unlikely to arise due to variation in exposure alone [3], but rather requires underlying dynamical variation. This negative binomial burden distribution also occurs when we include variation in the parametrization of host and parasite processes (electronic supplementary material, figure S6). Including this variation is more representative of natural systems, where genetic and phenotypic variation exists among both hosts and parasites.

(b). Stability analyses of acute and chronic equilibrium states

The two stable states we found in time simulations (figure 1) represent the core dynamics of our system. We studied these dynamics further through stability analysis of the stable states, using parameter bifurcations, which illustrate how the system stability changes as a function of parameters.

For this analysis we studied the equilibrium outcomes as a function of the parasite attack rate, σ_c, because this parameter quantifies a key process for the parasite (resource uptake) and is an important determinant of the interaction strength between host and parasite. We found three regions with qualitatively different dynamics (figure 3; electronic supplementary material, figure S7). Low attack rates (below the persistence threshold) only allow for acute infections (figure 3a), since the extinct-parasite equilibrium is the only stable attractor. Parasites were expelled upon infection by the host's immune response, and the final system state (equilibrium) did not contain the parasite. High attack rates (above the invasion threshold) only allow for chronic infections (figure 3b), since the positive-parasite biomass equilibrium is the only stable attractor. Here, parasites are able to invade even from very low biomass, resulting in a chronic infection. Intermediate attack rate values allow for both acute and chronic infections (figure 3; electronic supplementary material, figure S7), with the outcome being dependent on initial conditions (figures 1 and 3a,b). The acute and chronic equilibria are characterized by parasite biomass being zero or positive (figure 3c; electronic supplementary material), but these states also differed with respect to host body condition.

In the acute infection equilibrium, the parasite was absent, and because of this all host variables, S, R, C and I_i, were constant and independent of the parasite attack rate. The induced immune response was absent (figure 3d), reversible biomass was high (figure 3e) and biomass in egesta was low (figure 3f). Note that host irreversible mass is constant across the entire parameter range because we initiate simulations in adult-sized hosts.

In the chronic infection equilibrium, the host had an elevated induced immune response, triggered by the presence of the parasite (figure 3d). The induced immune response intensity increased with increasing parasite attack rate, in response to increasing parasite biomass (figure 3c). Because of the resource demands of the immune response and the reduction in assimilation efficiency due to the parasite, reversible biomass was reduced with respect to the acute infection state (figure 3e). In the chronic infection equilibrium, the parasite exerted a positive feedback on the biomass in the egesta, keeping the resource level high, and maintaining a profitable environment for itself (figure 3f).

We verified that bistability depends on resource modulation using a simplified model that only accounted for resources and parasites (electronic supplementary material). The analysis of this simple model revealed the importance of a nonlinear functional form for the resource modulation function, E_a(P) (equation (2.9)). We also carried out two-parameter bifurcation analysis to quantify the importance of the parameters ε_Amin, which determines how much parasites are able to modulate assimilation efficiency, and v_i, which determines the killing rate of the induced immune response. These analyses showed that the larger the impact a given parasite biomass has, the stronger the potential for positive feedback and for bistability (electronic supplementary material, figure S7a). Increasing the immune killing rate did not qualitatively affect the occurrence of bistability (electronic supplementary material, figure S7b).

4. Discussion

We show that the positive feedback of parasites on their own resources can produce both acute and chronic infections in a single within-host model. This positive feedback is comparable to an Allee effect in free-living consumer species [40], where per capita increase is positively correlated to population density at low densities. Accounting for bottom-up, resource-driven processes is essential to capture this possibility of acute and chronic infections emerging simultaneously in the same model.

Our results contrast with previous within-host theory that must define a priori whether a model represents an acute or chronic infection. In these classic models, one makes separate assumptions about the parasite–host interaction to produce differences in infection outcomes (acute versus chronic [9,10,41]). Even previous approaches where both resource-competition and immunity are explicitly accounted for were restricted to chronic infections [11]. In contrast, we only assume that all within-host processes rely on available resources (or energy). And we consequently find acute and chronic infections as emergent model outcomes, depending on the initial conditions in parasite dose or different food availability. These results fill a knowledge gap in within-host theory, by showing that acute and chronic infections can both be the outcome of infection in a single (general) within-host model [10,11].

Our assumption that parasites can reduce their host's assimilation efficiency is based on various mechanisms found in empirical systems. Helminths commonly reduce the host's ability to assimilate nutrients. For example, many species feed on intestinal cells [37], alter intestinal lining structure [42] and increase intestinal permeability [43]. While some immune defences ameliorate these effects [43], other defences reduce nutrient acquisition, thereby indirectly reducing assimilation [44,45]. Reduced digestive efficiency has substantial consequences [46–48]: calves infected with GI nematodes had nearly 40% lower conversion efficiency of feed to liveweight gain [49]. We capture all these sources of reduced assimilation efficiency in the term E_a(P) in our system.

The immune response was represented using a minimal form, where we did not do justice to many known aspects of immunology, such as the specific cell types involved in responses against nematodes [50] or the many positive and negative feedbacks inherent to the dynamics of the immune system [51]. Moreover, (genetic) variation in immune function is expected to alter host susceptibility and parasite infection outcome and may explain some discrepancies between our model predictions and empirical results [52]. For example, in our model, higher parasite doses are more likely to lead to chronic infections, whereas dose–response experiments suggest that lower parasite doses can produce chronic infections in otherwise resistant host strains [53–55]. In laboratory settings, this outcome is attributed to low-dose infections leading to a response biased towards immune activity that is ineffective against nematodes (instead of an effective response that follows high-level exposure [52]). In our model, effective or ineffective immune responses would bear the same resource costs, but we do not capture the parasite-dose dependence in the immune response type that is being triggered (effective or ineffective). Accounting for a more sophisticated representation of the parasite-dose dependence in the immune response could reveal the interplay with resource modulation, and how these processes in concert explain variability in infection duration.

Additionally, previous studies showed that immune modulation by parasites is a decisive factor of parasite–host interactions [13–15]. But the theoretical models accounting for this effect have not shown bistable outcomes [41], such as we present here. We included the resource-dependence of the immune response, providing an indirect route for immune modulation by parasites [12]. It is, however, likely that immune modulation by parasites can also directly produce bistability through a similar Allee effect. It would be a valuable extension to investigate the interplay of immune and resource modulation combined, and the relative potential of these processes in determining infection outcomes.

An important challenge to testing our model predictions against experimental data is that in laboratory environments high-dose exposure is usually performed in hosts fed to excess (see [18–20] for exceptions). To avoid confounding effects, we need direct, experimental manipulation to pair dose variation with diet variation in factorial designs. And quality matters also, as shown in a study where hosts fed on low-protein diets more rapidly expelled helminth parasites [20]. In line with our finding that the immune response to parasites strongly depends on available resources, [56] showed that lactating mice experimentally infected with T. muris had a suppressed immune response compared to non-lactating individuals. Our finding of bifurcating infection outcomes resonates with recent empirical studies in Drosophila and Tribolium, both of which showed that experimental infections could produce either short-duration, fatal infections or long duration, persistent infections [57,58]. Importantly, these divergent outcomes were observed even when host and pathogen genetic variation, dose, and host environment were tightly controlled. In the light of all these laboratory observations, note that field systems and real-world parasite infections are likely to be more resource-limited than laboratory conditions, and unravelling where and why differences occur between controlled and real-world systems is crucial for progress towards solving real-world parasite infection patterns.

The results we present may help explain the ubiquitous pattern of burden variation among individuals in field systems [3]. Our population level projection of the bistability between acute and chronic infections in individual hosts generated aggregated burden distributions starting with only stochastic variation in dose. But dose variation by itself is insufficient to generate heterogeneity in burden; at the same time, without a source of host heterogeneity, bistability alone does not produce burden variation. It is the combination of the two that drives burden variation.

For the population projection, we assumed that parasite biomass represents a fixed number of parasites of the same size, discounting the effects of different numbers of differently-sized parasites. This is an important simplifying assumption, since a few large parasites may have very different energetic requirements (both in terms of host resource ingestion rates and metabolic rates) than many small parasites of equal biomass, given the difference in surface-area-to-volume relationship.

Future work should incorporate knowledge of these heterogeneities into the DEB framework to tighten predictions for how parasite biomass and burden are related, and how these traits combine to affect host health and parasite transmission. This would also allow a more mechanistic analysis of processes like (biomass-dependent) parasite-induced host mortality [59], or biomass-driven infection by free-living parasite stages. Such a mechanistic model would build towards a nested approach including the energetic dependencies between parasites and their hosts, integrating from the within-host to the population level [60].

Previous adaptations of dynamic energy budgets for parasite–host interactions revealed the importance of host metabolism for parasite virulence [29], parasite production [11,21] and parasitic castration [61], but none of these systems accounted for bistability. Here we highlight the variability of infection duration and parasite burdens, which have wide-ranging implications for parasite transmission, infectivity and host mortality [21].

The dynamics we present here take place in an ecological dimension, where the interaction between host and parasite, as well as the functioning of the immune response, are all dependent on bottom-up resource availability. There is evidence that parasite modulation is dependent on parasite density or parasite biomass [62], but further studies should explore how this relationship differs between (resource-limited) field versus laboratory systems. The energy dependence of all processes in our modelling framework ensures that the outcomes we find emerge from low-level assumptions and calls for extensions from the within-host scale to considering the between-host dynamics of infectious disease.

Data accessibility

Data available from the Dryad Digital Repository: https://doi.org/10.5061/dryad.b2g21j1 [33].

Authors' contributions

All authors conceived of the project and developed the DEB model. A.v.L. analysed the DEB model, with input from C.E.C., S.A.B. and A.L.G. A.v.L., C.E.C. and S.A.B. developed the simplified model and C.E.C. analysed this model. A.L.G. and S.A.B. designed the rewilding study and performed the empirical work. A.v.L. wrote the first draft of the manuscript and all authors contributed substantially to revisions and gave final approval for publication.

Competing interests

We have no competing interests.

Funding

We acknowledge the National Science Foundation Research Coordination Network on Infectious Disease Evolution Across Scales (RCN-IDEAS) for research exchanges that led to the development of this theory and these models; Princeton University and the program on Research and Policy in Infectious Disease Dynamics (RAPIDD) of the Fogarty International Center for funding the empirical work; A.v.L. was supported by the National Science Foundation grant no. GEO-1211972.