Expansion history and environmental suitability shape effective population size in a plant invasion

Abstract

The margins of an expanding range are predicted to be challenging environments for adaptation. Marginal populations should often experience low effective population sizes (N_e) where genetic drift is high due to demographic expansion and/or census population size is low due to unfavorable environmental conditions. Nevertheless, invasive species demonstrate increasing evidence of rapid evolution and potential adaptation to novel environments encountered during colonization, calling into question whether significant reductions in N_e are realized during range expansions in nature. Here we report one of the first empirical tests of the joint effects of expansion dynamics and environment on effective population size variation during invasive range expansion. We estimate contemporary values of N_e using rates of linkage disequilibrium among genome-wide markers within introduced populations of the highly invasive plant Centaurea solstitialis (yellow starthistle) in North America (California, USA), and within native Eurasian populations. As predicted, we find that N_e within the invaded range is positively correlated with both expansion history (time since founding) and habitat quality (abiotic climate). History and climate had independent additive effects with similar effect sizes, indicating an important role for both factors in this invasion. These results support theoretical expectations for the population genetics of range expansion, though whether these processes can ultimately arrest the spread of an invasive species remains an unanswered question.

Introduction

Adaptation is expected to be a critical component of how species respond to novel environmental conditions, such as those encountered during colonization and range expansion (Mayr 1963; Kirkpatrick & Barton 1997; Griffith & Watson 2006; Colautti & Barrett 2013; Bock et al. 2015; Hamilton et al. 2015). At the same time, it has been suggested that colonizing species might experience small population sizes that limit the ability of founding populations to respond to natural selection (Elam et al. 2007; Dlugosch et al. 2015; Gonzalez-Martinez et al. 2017; Welles and Dlugosch 2018). Small population sizes could result from both founder events and maladaptation to novel environments. A failure to adapt under these conditions could slow or limit range expansion and contribute to the formation of range limits (Bridle and Vines 2007; Eckert et al. 2008; Sexton et al. 2009; Polechová and Barton 2015; Polechova 2018). These effects are currently an active area of theoretical and experimental research (Gilbert et al. 2017; Szűcs et al. 2017a; Szűcs et al. 2017b), but empirical observations of the dynamics of population size and its influence on evolution during ongoing range expansions is scant (Ramakrishnan et al. 2010; Wootton and Pfister 2015).

Population genetic models predict that deleterious alleles may become fixed during range expansion due to the strong effects of genetic drift during colonization (Lehe et al. 2012; Peischl et al. 2013; Peischl et al. 2015), ultimately resulting in failure to adapt (Henry et al. 2015; Polechová and Barton 2015; Polechova 2018). Range expansions are expected to involve a series of founding events (repeated sampling events) as new populations establish beyond the current range boundary, resulting in reduced effective population size (N_e) and increased sampling effects as the range boundary advances (Le Corre & Kremer 1998; Excoffier 2004; Slatkin & Excoffier 2012). In particular, low N_e at the leading edge can cause random alleles, including deleterious mutations, to ‘surf’ to high frequency regardless of patterns of selection (Travis et al. 2007; Excoffier and Ray 2008; Excoffier et al. 2009; Hallatschek and Nelson 2010; Moreau et al. 2011; Peischl et al. 2013). This can create an ‘expansion load’ of deleterious alleles at the wave front, although beneficial mutations can also surf to high frequency and aid in local adaptation (Peischl et al. 2013; Peischl et al. 2015). The effects of range expansion on adaptation have been empirically observed with greatest detail in bacterial culture, where manipulative experiments have shown that the strength of genetic drift is key to determining whether allele surfing promotes or hinders adaptation (Hallatschek and Nelson 2010; Gralka et al. 2016; Bosshard et al. 2017).

Environmental conditions should also shape N_e during range expansion via their impact on population (census) size and demography. If leading edge environments are different than those experienced by source populations, then founding genotypes will not be pre-adapted and are likely to experience lower absolute fitness. Unfavorable conditions and low fitness may lead to lower abundance and/or fluctuations in population size, reducing N_e relative to larger or more stable populations (Wright 1938; Crow & Morton 1955; Kimura & Crow 1963; Frankham 1996). In a rare empirical example, Micheletti & Storfer (2015) found that streamside salamander (Ambystoma barbouri) populations on the periphery of the range were also on the margins of their climatic niche and tended toward lower N_e. Similarly, peripheral populations of the North American annual plant Arabidopsis lyrata possess greater genetic load and appear to exist at their ecological, and perhaps evolutionary limits (Willi et al. 2018). These studies address a set of long-debated hypotheses proposing that range limits form in part because they consist of ecologically and/or genetically marginal populations (Kirkpatrick & Barton 1997; Phillips 2012; Chuang & Peterson 2016), which lack the capacity to acquire adaptations that are necessary to support further expansion (i.e. the ‘central-marginal’, ‘center-periphery’ and ‘abundant center’ hypotheses: (Sagarin and Gaines 2002; Eckert et al. 2008; Pironon et al. 2015). Importantly, all of these hypotheses share the prediction that colonization will be associated with reduced response to selection for ecological reasons without requiring additional population genetic changes caused by expansion alone. The relative importance of these two factors (environment and expansion) for shaping N_e at range margins is unknown, but both have the potential to reduce opportunities for local adaptation.

Although N_e has long been used as a fundamental measure of the scale of genetic drift in populations (Wright 1931; Robertson 1960; Kimura and Crow 1963; Kimura 1964; Ohta 1992; Charlesworth 2009), little is known about how N_e changes during the process of range expansion. Most empirical population-level estimates come from the field of conservation genetics, where N_e is used to infer the potential for genetic drift to exacerbate the decline of threatened populations (Lynch et al. 1995; Frankham 1996; Sung et al. 2012). These studies have demonstrated that N_e can be highly variable within species, sensitive to local demography and modes of reproduction, and poorly predicted by census size (Frankham 1995; Turner et al. 2002; Palstra and Ruzzante 2008). For example, in recovering Chinook salmon (Orcorhynchus tshawytscha) populations, Shrimpton and Heath (2003) found up to a three-fold difference in both N_e and its ratio with census size across spawning sites. While low N_e is generally expected in declining populations, many of the same demographic factors are likely to affect N_e in founding populations (Allendorf and Lundquist 2003; Colautti et al. 2017).

Despite the potential obstacle low N_e might pose to adaptation, many species -- including large numbers of invaders -- have been successful at colonization and show evidence of adaptive evolution during range expansion (Rice and Mack 1991; Dlugosch and Parker 2008; Linnen et al. 2009; Colautti and Barrett 2013; Vandepitte et al. 2014; Colautti and Lau 2015; Li et al. 2015). Additionally, detailed studies of range expansion have found evidence of serial founding events and associated increases in genetic drift (Ramakrishnan et al. 2010; Graciá et al. 2013; White et al. 2013; Pierce et al. 2014; Peischl et al. 2018), and it is notable that few invasions appear to have expanded beyond the fundamental niches of their native range (Petitpierre et al. 2012; Tingley et al. 2014). Taken together, it appears that adaptive evolution might be achievable in many invading species, but that perhaps expansion load and ecological mismatch may act, either independently or in concert, to prevent expansion in some cases. An understanding of how founding dynamics and marginal environments shape N_e in individual wave front populations is needed to connect theoretical expectations to observed patterns of successful range expansion.

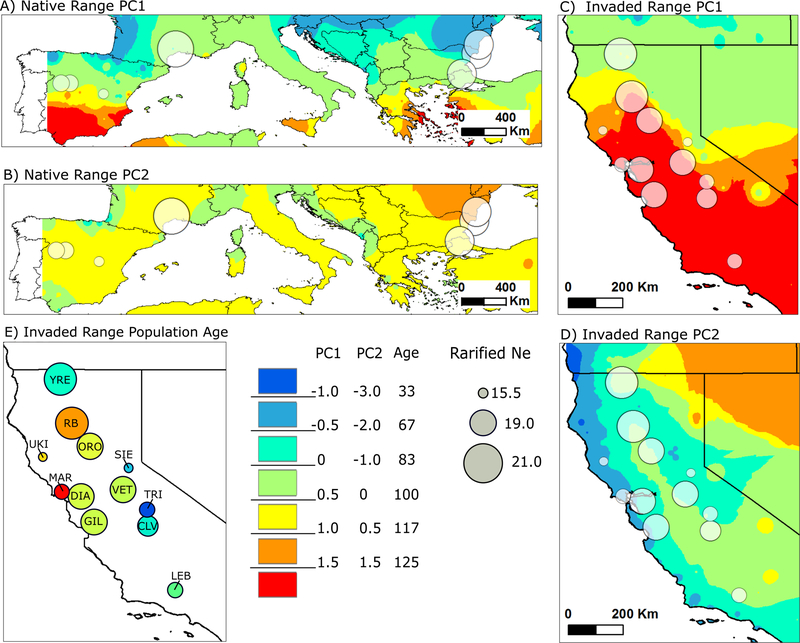
Here we estimate contemporary N_e for populations of the obligately outcrossing annual plant Centaurea solstitialis (yellow starthistle) across its invasion of California (USA) and its native range in Eurasia. In California, C. solstitialis was initially introduced in the mid 19th century into the San Francisco Bay area as a contaminant of alfalfa seed (Gerlach 1997; DiTomaso et al. 2006). Colonization by C. solstitialis resulted in a weak genetic bottleneck that is characterized by reduced private allele richness but no change in total allelic richness, nucleotide diversity, or observed heterozygosity (Barker et al. 2017). By the mid 20th century, the species was rapidly expanding through California’s Central Valley and Sierra Nevada foothill grasslands, and the current leading edge of this invasion lies above 4000 m in elevation along the west side of the Sierra Nevada Mountains (Pitcairn et al. 2006). In the North American invasion, habitat quality is often linked to the climatic environment, with warmer and drier habitats frequently supporting the densest C. solstitialis populations (Pitcairn et al. 2006; Swope and Parker 2010). During expansion, C. solstitialis has crossed climatic gradients that are largely independent in direction from the pathway of colonization (Fig. 1), allowing us to quantify the influence of both climatic environment and expansion history (time since founding) on estimates of N_e across populations.

Fig 1.

The distribution of rarefied effective population size (N_e), climatic principal component (PC) gradients, and population age (in years) across C. solstitialis populations in Eurasia and California. In all panels, circles indicate sampled populations with a diameter proportional to N_e. PC1 is positively correlated with annual temperature and temperature of the driest quarter and negatively correlated with seasonal differences in total radiation in the native (A) and invaded (C) ranges. PC2 is positively correlated with seasonal differences in temperature and negatively correlated with annual precipitation and seasonal differences in precipitation in the native (B) and invaded (D) ranges. In the California invasion, population age (E) reflects a history of expansion beginning in the San Francisco Bay area and expanding first to the North and then to the South and East of the state. Abbreviations in (E) correspond to populations in Table S1.

We used Restriction-site Associated DNA sequencing (RADseq) to estimate contemporary N_e in C. solstitialis populations sampled at a single time point. In addition to testing for the joint influence of expansion dynamics and climatic conditions on N_e in this system, we explored solutions for general problems associated with using large genome-wide marker data sets to estimate N_e. Linkage disequilibrium N_e (LD-N_e) is a powerful method for inferring contemporary N_e from single time sampled data, and does so by utilizing the frequency of statistical linkage across loci (Waples and Do 2008; Gilbert and Whitlock 2015). This method requires that loci segregate independently of each other, and while RADseq is widely used to produce population genetic datasets in non-model systems (Narum et al. 2013; Catchen et al. 2017), it is likely to violate this assumption of independence, resulting in biased calculations of N_e.

We used marker resampling and rarefaction approaches to improve inferences of variation in N_e across populations. We tested for effects of expansion history (time since founding) and habitat quality (climatic environment) on rarified N_e estimates, and compared these values to those from populations in the native range. We also explored whether estimates of genetic diversity could predict values of N_e, given that non-equilibrium population dynamics may in the short term decouple contemporary N_e from its expected long term effects on genetic variation (e.g. Nei et al. 1975; Varvio et al. 1986; Alcala et al. 2013; Epps and Keyghobadi 2015). By testing for evidence of historical and ecological effects on N_e, our goal is to shed light on the factors shaping fundamental parameters of evolution during colonization and range expansion.

Materials and Methods

Study Species

Yellow starthistle (Centaurea solstitialis) is an obligately outcrossing, diploid annual plant, native to a broad region of Eurasia. Plants grow as basal rosettes with a deep taproot, then bolt and produce up to several hundred flowering heads (capitula), which can collectively produce thousands of small (under 2mg) seeds per individual (Graebner et al. 2012; Hierro et al. 2012). Reproduction is by seed only (there is no clonal reproduction), and seeds are either unadorned (outer florets) or have a small (2mm) bristle-like pappus that appears to be better adapted for animal (including human) dispersal than for wind dispersal (Roche 1992; Gerlach 1997; Sun and Ritland 1998). Over 80% of seeds germinate within the first year, and while seeds can remain viable within the soil for up to ten years, most natural seed banks appear to be depleted in three years without new input (Joley et al. 1992; Callihan et al. 1993; Benefield et al. 2001).

Seeds of C. solstitialis were introduced to the Americas as a contaminant of alfalfa seed (Gerlach 1997), and have formed dense invading populations in mediterranean and semi arid grasslands of North and South America (DiTomaso et al. 2006). Invading populations are persistent and difficult to control (Aslan et al. 2009; Matzek and Hill 2012). Genotypes from invaded regions have evolved larger seeds, larger biomass, faster growth rates, shorter time to flowering, and greater reproductive output than those from the Eurasian native range (Eriksen et al. 2012; Widmer et al. 2007; Dlugosch et al. 2015). Invading populations in the Americas achieve densities that are more than an order of magnitude higher than those in the native range (Uygur et al. 2004; Andonian et al. 2011).

The first recorded introduction of C. solstitialis in North American occurred in the San Francisco Bay area of California, USA in 1869 (Pitcairn et al. 2006). Records indicate a subsequent expansion eastward into the Central Valley of California, then southward to San Diego, northward to southern Oregon, and further East to the Sierra Nevada mountains where the expansion remains active (Gerlach 1997; DiTomaso et al. 2006). There are also additional invading populations in the interior Pacific Northwest, but previous genetic work indicates that these are the product of separate introductions, and the California invasion is composed of a single expansion of genotypes originally from western Europe (Barker et al. 2017). Our work focuses on the California invasion.

Within California, coastal populations (closest to the initial introduction) are composed of smaller plants and reach densities that are an order of magnitude lower than those in the Central Valley and Sierra Nevadas (Swope and Parker 2010; Swope et al. 2017). Seed addition studies indicate that coastal populations are near carrying capacity despite their lower densities, while Central Valley and Sierra Nevada populations are seed limited and have the capacity to achieve higher densities (Swope and Parker 2010). Multiple biocontrol agents have been introduced to California, but have only been effective at controlling population growth in low density coastal populations, where a small decrease in vital rates has a large effect on population growth (Swope et al. 2017). In the Central Valley and Sierra Nevadas, compensatory growth and high plant densities limit the impact of biocontrol, and density dependent reproduction in C. solstitialis results in seed production that is independent of individual density across sites (Swope and Parker 2010).

Genomic Data

Genome-wide markers for C. solstitialis in this study were sampled from single nucleotide polymorphisms in double-digest RADseq (ddRADseq; (Peterson et al. 2012), previously published by Barker and colleagues (Barker et al. 2017; Dryad doi:10.5061/dryad.pf550). All sequences were obtained from C. solstitialis individuals germinated in the laboratory from wild collected seed. Seeds were sampled in 2008 from maternal plants along a linear transect in each population, with >1m separation between individuals. Populations included at least 14 individuals each grown from different maternal plants, from 12 invading populations in California and seven native populations in Europe (451 individuals total; Table S1). Sampled populations spanned the extent of the Californian invasion (Fig 1e).

Briefly, sequence data published by Barker and colleagues (2017) were generated as follows. Genomic DNA was extracted with a modified CTAB protocol (Webb and Knapp 1990) and fragmented using PstI and Mse1 restriction enzymes. Samples were individually barcoded, cleaned and size selected for fragments between 350 and 650 bp. Size selected fragments were amplified through 12 PCR cycles and sequenced on an Illumina HiSeq 2000 or 2500 platform (Illumina, Inc., San Diego, CA USA) to generate 100 bp paired-end reads. Reads were de-multiplexed with custom scripts and cleaned with the package SNOWHITE 2.0.2 (Dlugosch et al. 2013) to remove primer and adapter contaminants. Barcode and enzyme recognition sequences were removed from individual reads, and bases with phred quality scores below 20 were clipped from the 3’ end. Reads were trimmed to a uniform length of 76 base pairs. The R2 (reverse) reads from the data set were removed due to variable quality, and all analyses in this study were conducted using R1 (forward) reads only.

We used the denovo_map.pl pipeline in STACKS 1.20 (Catchen et al. 2011; Hohenlohe et al. 2011) to identify putative alleles within individuals, allowing a maximum of two nucleotide polymorphisms when merging stacks (-M parameter in STACKS), a maximum of two alleles per locus (-X), and a minimum coverage depth of five (-m). A catalog of loci and single nucleotide polymorphisms (SNPs) was generated across individuals, allowing two polymorphisms (-n) between individuals within a stack. The population.pl module in STACKS was used to calculate the population level nucleotide diversity (π) (Nei & Li 1979; Allendorf 1986). We restricted our analyses to loci that were sequenced in 80% of individuals within a population and in 90% of all populations (-r and -p parameters respectively).

Estimates of N_e

We used SNPs identified by STACKs to calculate N_e for each population using a method based on linkage disequilibrium among loci with a correction for missing data (Waples & Do 2008) implemented in the program NeEstimator v.2.01 (Do et al. 2014). This method derives estimates of N_e from the frequency of statistical linkage among loci and has been shown to be one of the best predictors of N_e (hereafter LD-N_e) for markers sampled at a single time point (Gilbert & Whitlock 2015; Wang 2016; Waples 2016). The LD-N_e method is not strongly influenced by the total genetic diversity in the sample (Charlesworth 2009; Do et al. 2014), making it particularly well suited to analyses of invading populations where low genetic diversity might arise from founder effects unrelated to the number of individuals currently reproducing in the population. We used a minimum allele frequency threshold of 0.05 for including a locus in the analyses, which was the lowest threshold that did not result in excessive loss of loci and infinite estimates of N_e at some study sites.

The ddRADseq dataset consisted of thousands of SNPs across the genome, 622 of which passed our screening requirements. Some of these loci were located in the same RAD 76bp sequence, and we expect that these and many others do not segregate independently in our data set, either due to physical proximity or the influence of selection on multi-locus allele combinations (C. solstitialis has a genome size of 850Mbp, distributed across eight chromosomes (Bancheva & Greilhuber 2005; Widmer et al. 2007). We generated an initial estimate of N_e using one randomly sampled locus from each sequence (Table S1). To minimize the likelihood of our estimates including physically linked loci, we re-sampled random sets of 20 polymorphic SNPs from unique sequences to obtain distributions of LD-N_e estimates for each population. We chose to use 20 loci because this is typical of previous studies that have estimated LD-N_e (England et al. 2006; Waples 2006 ; Waples & Do 2008; Gilbert & Whitlock 2015), and it is highly conservative relative to our genome size and chromosome number (Bancheva and Greilhuber 2006; Gaut et al. 2007; Widmer et al. 2007). Substantial increases in locus sampling would require a genetic map for C. solstitialis to ensure loci were not in physical linkage. Each population was resampled 30,000 times. Sampling distributions were generally lognormal and spanned at least four orders of magnitude (Supporting Information Fig. S1). We used median values from these distributions to identify the median estimate.

We observed a strong, positive effect of the number of individuals sampled in each population on median LD-N_e (F_1,17=9.36, P=0.007). Unequal sampling has been shown to decrease the accuracy of LD-N_e estimates (England et al. 2006; Waples 2006), and NeEstimator implements a corrective algorithm to address this problem (Do et al. 2014). To account for persistent sampling effects, we produced rarefaction curves of median N_e estimated by subsampling different numbers of individuals (10 to the maximum number available per population) after marker resampling. As above, each marker resampling consisted of 30,000 N_e estimates with 20 loci. Median estimates did not asymptote at our maximum sampling effort and increased linearly (see Results). We fit a linear mixed model with random intercept and slopes implemented in the Lme4 package in R (Bates et al. 2014) to obtain population specific functions which describe the relationships between the number of individuals sampled in each population and median LD-N_e values. The estimated slope and intercepts for each population were extracted from the model and used to calculate rarefied N_e for each population at a standard value of 10 individuals (our minimum rarefaction size). We explored the relationship between rarified N_e and measures of genome-wide marker variation using nucleotide diversity (π) at variable sites, as calculated in STACKS. We used linear regression to predict π from N_e among invading populations, and among native European populations for comparison.

Effects of Expansion History and Climate on N_e

We tested for an effect of population age since founding on the rarified N_e of invading populations. We estimated the date of colonization for each population by searching the Jepson Online Herbarium (http://ucjeps.berkeley.edu/) for records of C. solstitialis in California since its first record in 1869. For each sampling location, we used the earliest date on record for the county, or for an adjacent county when the sampling location was closer to older collection records there. These dates were subtracted from the year of our seed collections (2008) to produce values of population age used in subsequent analyses. Using herbaria records to assign population ages in this manner may not represent the true population age because of the time between population founding and the first records of the population. Nevertheless, C. solstitialis has a relatively well-documented invasion history in California (858 specimens on record, 577 records with GPS data, 61 records prior to 1930 in the Jepson Herbarium), and our population age estimates are in line with historical reconstructions of a general pattern of expansion out from initial establishment in the San Francisco Bay area first to the Central Valley and then to the North, East, and South (Gerlach 1997; DiTomaso et al. 2006; Pitcairn et al. 2006).

We also tested for the influence of the climatic environment on rarefied N_e in both invading and native populations. Increasingly severe droughts reduce fecundity and density in invading C. solstitialis populations (Sheley and Larson 1994; Swope and Parker 2012), implicating a role for climatic variation in demographic performance. To quantify the climatic gradients that might be most relevant to C. solstitialis ecology, we used principal component (PC) axes of climatic variation across C. solstitialis collection sites in North America and Europe, as previously identified by Dlugosch and colleagues (2015a; Supporting Information Fig. S1). This PCA was performed on CliMond variables at 18.5 × 18.5 km resolution (Kriticos et al. 2012), extracted from a spatially thinned set of occurrence records from western North America (185 records) and Eurasia (372 records). CliMond data were chosen for this analysis because they are available worldwide, and because they include variables that vary strongly across the range of C. solstitialis (particularly solar radiation;(Dlugosch et al. 2015a)). The full CliMond dataset (35 variables) included many strongly correlated climatic variables across the range of C. solstitialis, and these were reduced to seven representative variables (Supporting Information Fig. S1). The first two PC axes explained over 72% of variation in these variables (Dlugosch et al. 2015a). Larger values along the first PC climate axis (PC1) generally indicate sites with higher temperatures and lower seasonality in total solar radiation. Larger PC2 values indicate lower annual precipitation and greater seasonality in temperature. Greater seasonal variation in temperature has been shown to be related to ecologically important traits (plant size and drought tolerance) in C. solstitialis in both the native and invaded ranges (Dlugosch et al. 2015a).

To quantify the contributions of both population age and climatic environment to variation in rarified N_e for the invaded range, we used a general linear model with N_e as the dependent variable and population age, climate PC1, climate PC2, and their interactions as explanatory variables. We constructed a separate model of rarified N_e in native range populations using only PC1 and PC2 as variables, since no information about population age is available for the native range. We used model decomposition and F-scores to identify the best fit model. To explore the relative effect of each variable and their interactions on N_e, effect sizes were calculated as partial eta-squared values, which partition the total variance in a dependent variable among all independent variables (analogous to R² in multiple regression), using the best fit linear model with the function ‘etasq’ in the R package ‘heplots’ version 1.3–1 (Fox et al. 2008). Partial-eta squared values are standardized for differences in magnitude of the independent variables. We also tested for an overall difference in the rarified N_e of invading and native populations using a Wilcoxon signed-rank test and a Monte Carlo exact test implemented with the ‘coin’ package in R (Hothorn et al. 2006). All statistical analyses were conducted in R version 3.4.1 (R Core Team 2017).

Results

Estimates of LD-N_e varied widely depending on which set of 20 loci were subsampled (Supporting Information Fig. S2). Distributions of subsampled LD-N_e estimates spanned at least four orders of magnitude within each population. Distributions peaked strongly around median estimates (Supporting Information Fig. S2). Median estimates of LD-N_e prior to rarefaction varied from 19.5 to 38.5 across the California invasion (Supporting Information Table S1). In general, estimates were higher in central and northern California and decreased to the East and South (Fig. 1). In native populations, median N_e estimates ranged from 16.2 to 42.7, with three populations with lowest LD-N_e located on the western side of the range in Spain (Fig. 1). Median estimates were consistently lower than estimates based on all sequences, and differed in rank order among populations (Supporting Information Table S1).

We found a strong association between median LD-N_e and the number of individuals sampled across our populations (r²_adj=0.32, F_1,17= 9.36, P=0.007). Rarefaction sampling produced positive relationships between LD-N_e and the number of individuals resampled within each population (Fig. 2). Slopes in the linear mixed model ranged from ~0.11 to 1.19. Importantly, rarefaction removed the significant effect of sampling effort on N_e values (rarefied N_e vs. total sample size; r²_adj=0.12, F_1,17=3.47, P=0.08). Rarefaction fits predicted a consistent rank order of LD-N_e among populations, with differences among populations being the smallest in magnitude at our minimum sampling of 10 individuals (Supporting Information Fig S3). Therefore, we expect our rarified Ne index to be conservative for tests of relationships between Ne and explanatory variables.

Fig 2.

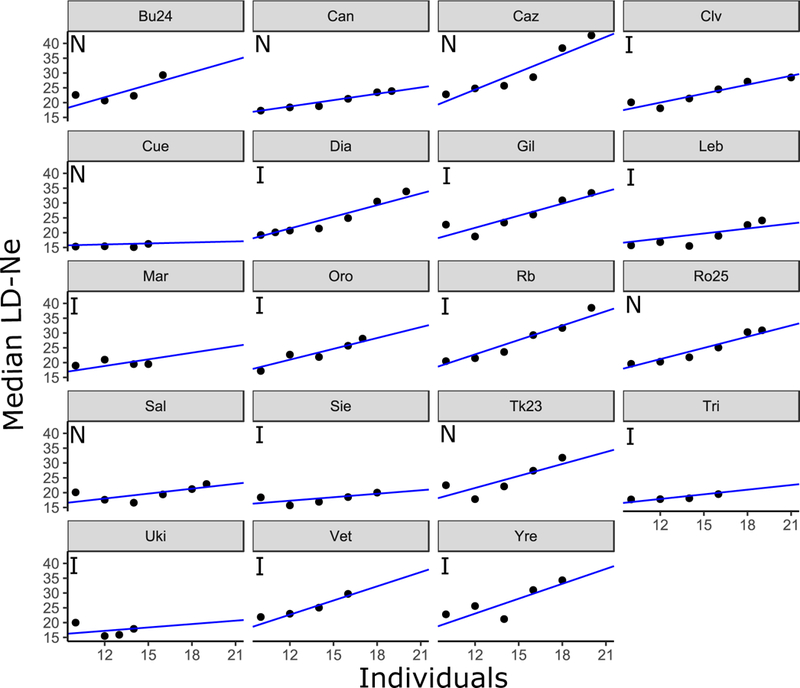
Relationships between median values of LD-N_e after locus resampling and the number of subsampled individuals for native (N) and invading (I) populations. Rarefaction was performed by linear mixed model with random slopes and intercepts.

Both climate and population age predicted rarified N_e in invading populations. The best fit linear model (Full model: r²_adj=0.46, F_(4,8)=4.09, P=0.0493) included significant, additive effects of population age and PC2 (Fig. 3; Table 1). Population age and PC2 were both positively correlated with rarified N_e values, indicating that N_e is largest in older populations and habitats with more temperature seasonality and lower precipitation. Age and PC2 were not significantly correlated (F1,10=2.42, P=0.15), and the model did not violate linear model assumptions of normality and no autocorrelation in the residuals (Supporting Information Fig. S4). PC2 had a greater influence on rarified N_e values than age, based on its larger standardized effect size (Table 1), although this difference was small. In contrast, rarified N_e of native range populations was not predicted by either climatic PC variable (Full model: r²_adj=0.2314, F_2,4=1.90, P=0.23) (Interactions: PC1: P=0.13, PC2: P=0.20).

Fig 3.

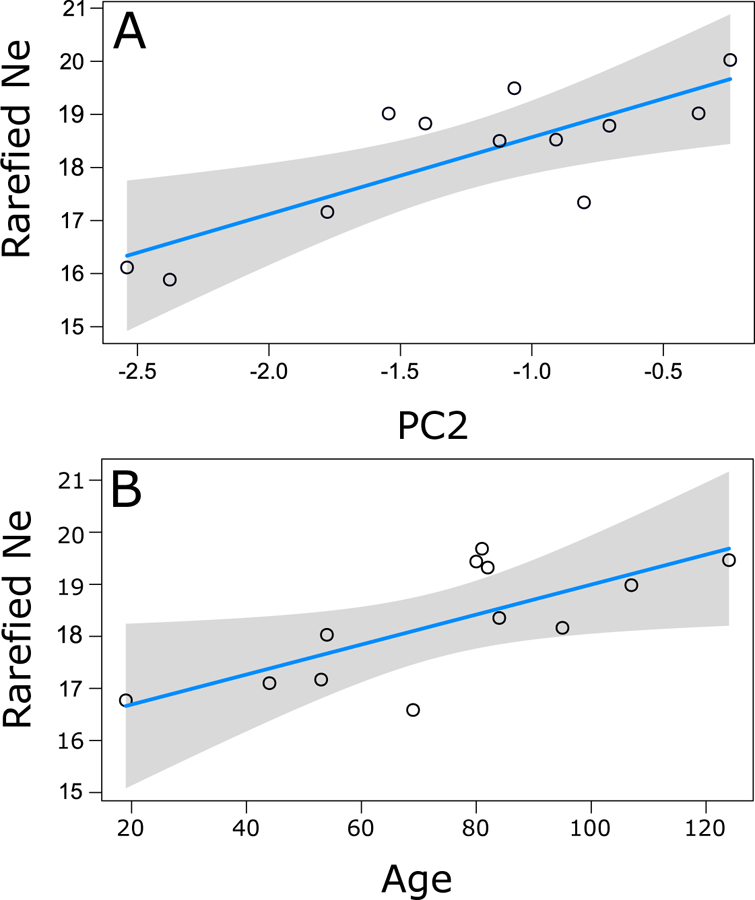
Rarefied N_e values are predicted by the second principal component (PC2) of climatic variability (A) and population age (B) in invading C. solstitialis populations. Rarefied N_e is positively correlated with PC2, for which larger values represent lower annual precipitation and greater seasonality in temperature (P=0.011), and with population age (P = 0.037). Lines show linear model fits and shading indicates the 95% confidence interval. Points represent partial residuals after accounting for other variables in the linear model.

Table 1.

Individual effects for the best fit linear model explaining rarefied effective population size (N_e) in invading populations of C. solstitialis, as a function of climatic principal component variables (PC1, PC2) and population age (Age).

Invaded Range Populations
Effect	Coefficient	Standard Error	t-value	p-value	Effect Size
PCA1	1.4503	0.4831	−0.66	0.5278	0.0516
PCA2	−0.3187	0.4376	2.50	0.0106	0.5786
Age	0.0288	0.0115	3.31	0.0369	0.4387

Invading populations included a narrower range of rarified N_e values, nested within the distribution observed for native populations (Supporting Information Fig S5), and there was no significant difference between rarified N_e values in the native and invaded ranges (Wilcoxon signed rank test: W = 43, P = 0.97; Monte-Carlo one-way exact test: P = 0.93). Nucleotide diversity (π) also did not differ between the native and invaded ranges (r²_adj=0.−0.04, F_(3,15)=0.78, P=0.55; region term: t_(2,15)=−1.5, P=0.15 ). There was no significant relationship between π and N_e in invading populations (r²_adj=−0.037, F_(1,10)=0.59, P=0.46) and there was a positive, marginally significant relationship between π and N_e in native populations (r²_adj=0.43, F_(1,5)=5.45, P=0.067), despite a smaller number of sampled populations from this range (Supporting Information Fig S6).

Discussion

Here we report empirical evidence for the joint effects of both range expansion and climatic environment on contemporary N_e in natural populations. We produced rarified estimates of LD-N_e across 12 populations in the invaded range of C. solstitialis and found a significant positive relationship between population age and N_e, a finding in line with theoretical expectations for the population genetics of expanding populations (Hallatschek et al. 2007; Excoffier & Ray 2008; Excoffier et al. 2009; Moreau et al. 2011; Lehe et al. 2012; Peischl et al. 2013; Peischl et al. 2015). We also found evidence that spatial variation in climatic conditions had a significant impact on N_e which was independent of population age. The effects of range expansion and climate were similar in magnitude in our study system, suggesting that both of these factors have been important for shaping evolutionary outcomes in invading populations (though their relative impact should be expected to vary across different scales of time and environment (e.g. the effect of age over time may diminish or the effect of climate may vary over both space and time) (Wegmann et al. 2006; Excoffier and Ray 2008; Gilbert et al. 2017).

We emphasize that our rarified LD-N_e values do not reflect a ‘true’ N_e value for the populations in our study. Rather, rarified estimates here represent relative values of N_e, and are useful for comparisons among populations. We expect asymptotic LD-N_e values for these populations to be larger, because we observed no asymptote with rarefaction for any of the populations in our study. However, our maximum estimates are similar to values reported in other plant and animal species using the same approach (e.g. Shrimpton & Heath 2003; Coyer et al. 2008; Wang et al. 2013; Álvarez et al. 2015). We note the LD-N_e estimation method itself also has a tendency to underestimate known values of N_e in simulations (Gilbert & Whitlock 2015), such that the true number of breeding individuals is likely higher than an asymptotic estimate.

Our resampling revealed that N_e estimates in C. solstitialis vary by at least four orders of magnitude when different sets of loci are used. This variation is expected given that particular sets of loci will capture different effects of physical linkage, history of selection, and chance sampling effects (Daly et al. 2001; Remington et al. 2001; Flint-Garcia et al. 2003). Resampling allowed us to leverage many combinations of loci across the genome to identify a well defined peak in the distribution of N_e estimates. A resampling approach is likely to be generally useful for RAD-seq and other popular methods used to generate genome-wide marker datasets, particularly where a complete reference genome is not available to determine the physical arrangement of loci.

After accounting for population and marker sampling, we found a significant effect of population age on differences in N_e across invading populations. Rarefied N_e estimates were lower in younger populations, which fits with expectations that a subset of individuals will contribute to range expansion (Excoffier & Ray 2008) and that genetic drift will be larger at the leading edge (Lehe et al. 2012; Peischl et al. 2013; Peischl et al. 2015). Estimates of contemporary N_e from C. solstitialis invading populations were within the distribution that we observed among native populations, which suggests that this species did not experience a large initial genetic bottleneck during its introduction to California, nor exceptionally low N_e during range expansion (relative to values observed in native populations). This lack of evidence for a strong genetic bottleneck is in line with models of historical demography by Barker and colleagues (2017), who inferred little reduction in N_e and maintenance of genetic diversity during the colonization of the Americas by C. solstitialis. In general, introduced species often lack strong genetic bottlenecks (Dlugosch & Parker 2008; Uller & Leimu 2011; Dlugosch et al. 2015b), and our results demonstrate that species which avoid genetic bottlenecks at introduction may still experience significant declines in N_e during range expansion. Importantly, invading populations of C. solstitialis in California are an order of magnitude higher in density than native populations (Uygur et al. 2004; Andonian et al. 2011), indicating that the fraction of the census population that is contributing to the evolutionary effective population in the invasion is much lower than in the native range.

We also observed an independent positive relationship between climatic PC2 and N_e of invading C. solstitialis populations, consistent with an impact of habitat suitability on N_e. High PC2 values reflect greater variation in seasonal temperatures and lower total annual precipitation, which typify areas of especially high C. solstitialis density in California (Dlugosch et al. 2015a). Previous studies in this system have proposed that C. solstitialis success stems from a lack of effective competitors in more drought prone habitats (Dlugosch et al. 2015a), due in part to the extensive conversion of these habitats to rangeland (Menke 1989; Stromberg and Griffin 1996). Other studies within the California invasion, however, have found that water availability (both naturally occurring and experimentally manipulated) is strongly and positively correlated with C. solstitialis density and fecundity (Enloe et al. 2004; Morghan & Rice 2006; Hulvey & Zavaleta 2012; Eskelinen & Harrison 2014), suggesting that fitness should be highest in wetter areas. Our results are most consistent with the landscape pattern of abundant C. solstitialis in drier areas, and might therefore reflect differences in human land use and the availability of native competitors across the invaded range. An underlying relationship between N_e and land use in the invasion could also explain why we did not find the same relationship with climate in the native range. Alternatively, native populations are more likely to be locally adapted, which could disrupt any relationships between climatic patterns, habitat quality, and N_e, particularly at the large geographic scale of our sampling in the native range.

Differences in rarified N_e among invading populations were not predicted by nucleotide diversity (π). Nonequilibrium populations such as the invasions here are unlikely to have had sufficient time to reach equilibrium diversity at a given N_e, and will also have been changing in size over time (Nei et al. 1975; Alcala et al. 2013; Epps & Keyghobadi 2015). Notably, we did find a marginally significant positive relationship between π and N_e in native range populations (despite a smaller population sample size), which have had more time to stabilize in population size and reach mutation-drift equilibrium. Moreover, rare alleles contribute important equilibrium genetic variation (Luikart et al. 1998) and native C. solstitialis populations have been previously shown to harbor more rare alleles than invading populations in North America (Barker et al. 2017). There is also a tendency for RAD-seq to underestimate π in more diverse genomes (Arnold et al. 2013; Cariou et al. 2016), although given the loss of rare alleles from invading populations, we might expect this to affect native populations more strongly than invading populations.

Our results support the prediction that both range expansion and habitat quality can increase the genetic drift experienced by leading edge populations. There is particular interest in whether these effects can hinder adaptation, slow further colonization, and establish static range boundaries (Bosshard et al. 2017; Lehe et al. 2012; Peischl et al. 2013; Peischl et al. 2015; Marculis et al. 2017; Birzu et al. 2018). Recent studies have demonstrated a link between differences in historical values of N_e and differences in efficacy of selection across species (e.g. (Slotte et al. 2010; Jensen & Bachtrog 2011; Strasburg et al. 2011), and both theoretical and experimental studies of bacteria have shown that the process of range expansion can reduce contemporary N_e and impose limits to adaptation and further colonization at the expansion front (Hallatschek & Nelson 2010; Lehe et al. 2012; Gralka et al. 2016; Peischl et al. 2013; Peischl et al. 2015). Natural populations of Arabidopsis lyrata demonstrate some of these effects, with greater genetic load in range edge populations associated with a lack of adaptation along an environmental cline (Willi et al. 2018). Limits to range expansion are expected to be sensitive to the specifics of evolutionary parameters in natural populations, including the magnitudes of N_e and selection, the amount and scale of gene flow across the expansion, and the genetic architecture of adaptive variation (Hallatschek & Nelson 2010; Lehe et al. 2012; Peischl et al. 2013; Peischl et al. 2015; Gralka et al. 2016).

The expansion ecology of C. solstitialis in California does not support the existence of maladapted edge populations. Populations of C. solstitialis close to the range edge can achieve higher densities than older, more interior populations (Swope et al. 2017), which runs counter to expectations of high genetic load. Additionally, evolution of increased growth and earlier flowering appears to be enhancing the invasiveness of C. solstitialis (Dlugosch et al. 2015a), suggesting that reduced N_e at the range edge has not created a barrier to adaptation and further expansion. Additional studies are needed to test for quantitative connections between expansion dynamics and the role of adaptation in this system, including detailed analyses of dispersal patterns (included biased dispersal of particular phenotypes, (Shine et al. 2011), trait and fitness differences, and demographic performance across populations. The availability of adaptive variation and the degree to which this is a limiting factor in species invasions is an active area of debate (Ellstrand & Schierenbeck 2000; Rius & Darling 2014; Bock et al. 2015), and should be particularly relevant to the colonization of habitats requiring significant niche evolution. The results reported here emphasize that an understanding of the evolutionary mechanisms that generate boundaries to range expansion in natural populations will require evaluating evidence not only for the availability of adaptive variation (Dlugosch et al. 2015a), but also for an effective response to selection.