Skip to main content
PMC home page
ACS AuthorChoice logoLink to ACS AuthorChoice
. 2019 May 29;31(12):4575–4583. doi: 10.1021/acs.chemmater.9b01395

Role of Surface Reduction in the Formation of Traps in n-Doped II–VI Semiconductor Nanocrystals: How to Charge without Reducing the Surface

Indy du Fossé †, Stephanie ten Brinck ‡, Ivan Infante ‡,§,*, Arjan J Houtepen †,*
PMCID: PMC6595709  PMID: 31274957

Abstract

graphic file with name cm-2019-013956_0005.jpg

The efficiency of nanocrystal (NC)-based devices is often limited by the presence of surface states that lead to localized energy levels in the bandgap. Yet, a complete understanding of the nature of these traps remains challenging. Although theoretical modeling has greatly improved our comprehension of the NC surface, several experimental studies suggest the existence of metal-based traps that have not yet been found with theoretical methods. Since there are indications that these metal-based traps form in the presence of excess electrons, the present work uses density functional theory (DFT) calculations to study the effects of charging II–VI semiconductor NCs with either full or imperfect surface passivation. It is found that charge injection can lead to trap-formation via two pathways: metal atom ejection from perfectly passivated NCs or metal–metal dimer-formation in imperfectly passivated NCs. Fully passivated CdTe NCs are observed to be stable up to a charge of two electrons. Further reduction leads to charge localization on a surface Cd atom and the formation of in-gap states. The effects of suboptimal passivation are probed by charging NCs where an X-type ligand is removed from the (100) plane. In this case, injection of even one electron leads to Cd-dimerization and trap-formation. Addition of an L-type amine ligand prevents this dimer-formation and is suggested to also prevent trapping of photoexcited electrons in charge neutral NCs. The results presented in this work are generalized to NCs of different sizes and other II–VI semiconductors. This has clear implications for n-doping II–VI semiconductor NCs without introducing surface traps due to metal ion reduction. The possible effect of these metal ion localized traps on the photoluminescence efficiency of neutral NCs is also discussed.

Introduction

Due to their size-dependent properties and solution processability, colloidal semiconductor nanocrystals (NCs) have proven to be very interesting for application in a wide range of optoelectronic devices,1−4 including solar cells,5,6 light-emitting diodes,7−9 and lasers.10−12 However, the efficiency of these devices is often hampered by localized electronic states within the bandgap, which can trap the generated charge carriers.13−15 Much work has been dedicated to passivating these trap states by, for example, the epitaxial growth of an inorganic shell around the NC core,16 the addition of various ligands,17−19 and the electrochemical filling of in-gap states.20−22 Nevertheless, due to the complexity of the NC surface and the plethora of possible interactions between the ligands and the various binding sites on the NC, a complete understanding of the microscopic nature of these trap states remains challenging.19,23,24

In its simplest form, a trap can be described as a stable nonbonding orbital of an undercoordinated (and hence often surface-located) atom. This orbital usually lies deep in the bandgap, where it can act as an electron or hole trap. Surface atoms can however be fully coordinated due to the interaction with surface ligands, forming bonding and antibonding orbital energy levels, which lie within respectively the valence band (VB) and the conduction band (CB).14,25 We recently used density functional theory (DFT) calculations to show that mostly 2-fold undercoordinated chalcogenide atoms are responsible for trap-formation in II–VI semiconductor NCs.23 These 2-coordinated chalcogenide sites feature one nonbonding p-orbital, which remains deep in the bandgap as a trap state. In contrast, the spherical symmetry of the s-orbital of the metal ensures it is always split in states that lie outside the bandgap, regardless of coordination number.23 In line with this description, we have recently shown that a very wide range of electron accepting Z-type ligands, which coordinate to the surface anions, can be used to increase the photoluminescence quantum yield (PL QY) of II–VI and III–V NCs.19

Contrary to these computational and experimental results, several experimental studies suggest that, besides the 2-coordinated chalcogenides, some traps may also be localized on the metal atoms. For instance, amine ligands have been found to increase the PL QY of NCs.19,26−28 Since these electron donating ligands cannot passivate the undercoordinated chalcogenides,23 amines have been suggested to passivate excess cadmium ions.27,28 In addition, spectroelectrochemical studies of CdSe core-only and core/shell-particles have shown the existence of in-gap states near the CB that act as hole traps upon raising of the Fermi level. These traps were ascribed to undercoordinated surface Cd and could be passivated by growth of a ZnS shell.29 Metal-based traps may be more complex and of a more dynamic nature than their chalcogenide counterparts29 and perhaps only form in the presence of excess electrons (vide infra), which can be injected into the NCs via chemical,30−32 electrochemical22,33,34 or photochemical35,36 doping but are also created upon photoexcitation of the NCs.

It is often tacitly assumed that charging NCs only leads to the filling or emptying of traps or band edge states, without affecting the structure of the NC. Yet, it is well-known that there are limits to charging of bulk semiconductor materials due to electrochemical degradation or photodecomposition,37−39 meaning that metal (chalcogenide) atoms can be reduced (oxidized) at certain electrochemical potentials. These effects will be strongly modified in NCs due to the high surface-to-volume ratio and the presence of stabilizing ligands. Yet it is to be expected that, even with full NC passivation, charging will eventually lead to degradation and consequent trap-formation; this process will likely be enhanced for imperfectly passivated NCs.

To study the effect of excess electrons on the NC surface, this work will focus on charge unbalanced NCs. To make a clear distinction between charge-balanced and charge unbalanced NCs, the number of excess electrons in this work is determined using the model of Voznyy et al., which has as its basic underlying assumption that each entire NC is charge neutral:40

graphic file with name cm-2019-013956_m001.jpg

Here, ⟨n⟩ is the number of excess electrons, Ni the number of chemical species of type i, and qi the oxidation state of species i. In this work it is assumed that the oxidation states of the constituents of the NCs are M2+ (M = Cd, Zn), X2– (X = S, Se, Te), K+ and Cl–. If ⟨n⟩ = 0, the NC is charge-balanced. If ⟨n⟩ > 0, electrons are injected in the CB, while holes occupy the VB when ⟨n⟩ < 0. Previous theoretical works have shown that making PbS NCs charge unbalanced can lead to traps localized on (partially) reduced surface Pb41,42 or on Pb–Pb dimers,43,44 which are different from the 2-fold undercoordinated chalcogenides found for charge-balanced NCs.

Although these previous studies show that disrupting the charge balance of NCs in different ways can lead to different surface states, no theoretical study has given a structured overview of what happens to the NC when it becomes increasingly charged. Yet, investigating the traps that form upon electron charging can be relevant for various fields of research that involve the redox chemistry of NCs. First of all, a better comprehension of the stability of charged NCs can open up new pathways to prevent trap-formation, which will be essential for the controllable doping of NCs that is required for NC-based optoelectronic devices.45,46 Defects in charged NCs are also relevant for NC-based catalysis, where reduction of surface Cd2+ to Cd0 has been found to create catalytically active metal sites.47,48 Understanding the surface of charged NCs may lead to the design of better NC-catalysts. Lastly, this work may help to explain the photoinstability and lower-than-unity PL QY of charge-balanced NCs. If injection of electrons can lead to trap-formation (as will be shown in this work), it is conceivable that a photoexcited electron in the CB could also lead to the formation of new surface states. Such a mechanism would lower the PL QY of uncharged NCs, without the need of (electro)chemical charge injection or doping.

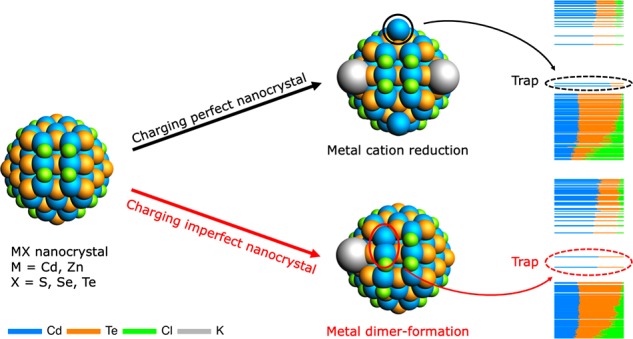
The aim of the current work is to provide an atomistic picture of what happens to the NC surface upon negative charging (⟨n⟩ > 0) and to show that, depending on the NC passivation, injection of electrons can lead to the formation of new surface states within the bandgap. DFT calculations are used to charge both fully passivated CdTe NCs and NCs with missing ligands. In the former case, the complete passivation prevents the occurrence of surface reorganization. Instead, it probes the maximum stability of the NCs. It is shown that, for small, perfectly passivated NCs, charging with more than two electrons will cause the (partial) reduction of surface Cd2+ to Cd0, leading to localized in-gap states that can act as hole-traps. However, if the NC is not perfectly passivated, charging with only one electron already leads to surface reconstruction in the form of Cd–Cd dimers, which manifest themselves as traps within the bandgap that are half-filled and hence can trap both electrons and holes. While both these results can be generalized to other Cd-chalcogenide materials, the increased electrochemical stability of zinc causes fully passivated Zn-chalcogenide to be significantly more stable upon electron injection. Upon ligand removal, Zn–Zn dimer-formation also leads to trap states in Zn-chalcogenide NCs. Lastly, it is shown that addition of L-type ligands can stabilize the surface and prevent dimerization, which may explain the positive effect of amines on the PL QY of charge-balanced NCs.

Results and Discussion

Model System

Following the example of Houtepen et al.,23 a zincblende Cd68Te55Cl26 NC of ca. 1.9 nm in diameter (see Figure 1A-i) was used as the model system for the calculations presented here. As also often found experimentally,49,50 the NC is cation-rich with a Cd/Te ratio of 1.24. Chloride anions were added to preserve charge balance, since they are electronically similar to the experimentally frequently employed oleate ligands43 but are computationally less demanding.51,52Figure 1B shows the electronic structure of these model NCs, computed at the DFT level (see Methods for further technical details). For these systems, the so-called PBE exchange-correlation function was employed, which provides reliable geometrical structures and the composition of the molecular orbitals (MOs) [i.e., the density of states (DOS)]. On the other hand, the bandgap tends to be underestimated.53 For the purpose of this work, one should keep in mind that the absolute energy of the calculated MOs may differ significantly from experimentally found values. Although energy levels can therefore not be directly related to, for example, electrochemical potentials, trends between different systems can still easily be observed. Also, for charged NCs, the energetic position of the trap state in the bandgap versus the CB and VB can only be qualitatively reproduced.

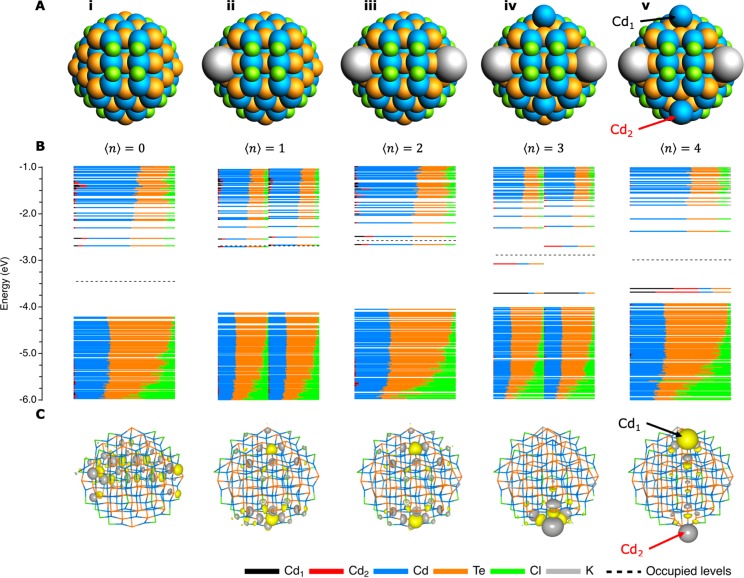
Figure 1.

Figure 1

Open in a new tab

Effect of charging fully passivated CdTe NCs on their structure and DOS. (A) Structure of the NCs when 0, 1, 2, 3, and 4 electrons are injected, respectively. The total system is kept neutral by the addition of a potassium cation for each added electron. (B) DOS for each of the NCs, where every line corresponds to an MO. The length of a colored line segment indicates the contribution of the corresponding atom or element to that MO. MOs below the dotted line are occupied, whereas the ones above are unoccupied. If the total number of electrons is even (i.e., for ⟨n⟩ = 0, 2, 4), every MO is occupied by two electrons with opposing spin. If the total number of electrons is odd (⟨n⟩ = 1, 3), the unrestricted calculation leads to a splitting of the spin-up and spin-down orbitals, which are plotted separately on the left- and right-hand side of the graph. As a result, each MO is only occupied by a single electron. (C) Contour plots of the HOMO level of each of the NCs, using a contour plot value of 0.02 e/bohr3.

Charging of Perfectly Passivated NCs

Perfectly passivated CdTe NCs were charged with up to four extra electrons, as shown in Figure 1. For this, neutral potassium atoms were added to the NC structure. Subsequent geometry optimization resulted in an overall charge neutral system, where one electron per potassium atom is donated to the NC. This gives an effectively negative NC, compensated by positive potassium ions. Since different counterions can be used to compensate for the negatively charged NCs,54 it was verified that the same results are obtained for other cations, as shown for Li+ and Cs+ in Figure S1. Throughout this work, the number of excess electrons in a NC is denoted by ⟨n⟩, as defined in the introduction.

Figure 1A shows that charging the NCs with up to two electrons results in no major structural changes. As indicated by the DOS in Figure 1B, the electrons are simply injected into the CB edge and no states are formed in the bandgap. Note that for ⟨n⟩ = 1, the odd total number of electrons in the system necessitates the use of an unrestricted DFT calculation, so that each MO holds only one electron (either spin-up or spin-down). This approach breaks the spatial symmetry between alpha and beta electrons, providing two distinct densities of states, a difference that is more pronounced near the band edge region. Apart from this, the unrestricted calculation for odd numbers of electrons does not lead to significantly different results compared to the restricted calculation for an even number of electrons. In Figure 1B and in all of the ensuing figures with an odd total number of electrons, the MOs belonging to the alpha electrons are displayed on the left half of the graph, while the beta electrons are plotted on the right half. For ⟨n⟩ = 2, the total number of electrons is even, so that no unrestricted calculation is required and each MO holds two electrons. Plotting the HOMO level for ⟨n⟩ = 1 and ⟨n⟩ = 2, as done in Figure 1C-ii and 1C-iii, respectively, shows that the electrons are delocalized over the NC. However, when three electrons are added to the NC, a Cd0 is ejected from the (111) plane (designated by Cd1 in Figure 1), which leads to the formation of multiple in-gap states (see Figure 1B-iv). Two electrons are injected into two closely spaced MOs around −3.7 eV. The black line segment in Figure 1B-iv indicates that these levels are mainly localized on the ejected Cd1. The third electron occupies the HOMO localized on a Cd from another (111) plane (Cd2 in Figure 1), as can be clearly seen in the contour plot of Figure 1C-iv. The MO localized on Cd1 is full and can hence act as a hole trap, while the MO on Cd2 is half-filled and can trap both electrons and holes. If four electrons are added to the NC (Figure 1-v), Cd2 will also be expelled from its facet (as Cd0), resulting in two filled in-gap states that are highly localized on the two ejected Cd0 atoms.

These results can be interpreted as the gradual reduction of surface Cd2+ and illustrate the instability of the fully chloride-passivated CdTe surface against negative charging. It shows that a limited number of electrons can be injected into the CB of the NCs without significant structural changes. However, if too many electrons are injected, localization of the excess charge on certain Cd2+ sites can lead to their reduction to Cd0. It should be noted that not all of the excess charge is localized on the Cd2+ sites and that the Cd is therefore not completely reduced to Cd0 but to a small noninteger oxidation state. However, for simplicity, the oxidation states of Cd will be limited to integers in this discussion. Since the CB consists of antibonding Cd 5s orbitals,55 charge localization will probably weaken the Cd–Te bonds of the Cd0 sites, thus leading to expulsion of the Cd0 from the lattice. This is in agreement with previous theoretical studies, which have shown that addition of ligands can lead to the ejection of Cd atoms from certain facets.56,57

It may be possible that the weakening of the Cd–Te bonds can cause Cd0 to entirely leave the NC. With the removal of the Cd0 atoms, the in-gap states would also be removed (see Figure S2), suggesting that the traps presented in this section are only transient. We performed molecular dynamics simulations that show that the Cd0 atoms are highly mobile. However, experimentally there are various factors that influence the stability of the Cd0, like the presence of L-type ligands on the surface and solvent effects, that are not considered in the current work and make it difficult to predict theoretically if the Cd0 remains bound to the surface. Spectroelectrochemical experiments show an induced sub-bandgap absorbance at negative potentials, which is attributed to the formation of metallic Cd0, and which disappears again at more positive potentials.29 This chemical reversibility suggests that the Cd0 remains on the NC surface, where it can be oxidized back upon application of more anodic potentials. In addition, stable surface Cd0 is suggested to play an important role in NC-based catalysis.47,48 On the basis of these experimental results, we conjecture that at least part of the Cd0 remains on the NC surface.

The reduction of Cd2+ has already been suggested in various previous experimental works on NCs that were chemically,30,58,59 photochemically,36 or electrochemically21,22,29,60 n-doped. The results presented in this section are in line with these experimental observations and provide the first atomistic picture of the surface during increasing reduction of the NC.

The results presented in this section can be generalized to charging with a different counterion (see Figure S1) and to certain larger NC sizes (see Figure S3). Interestingly, upon charging Cd152Te135Cl34 NCs with up to four electrons, no in-gap states are formed, whereas charging of Cd176Te147Cl58 NCs does lead to expulsion of Cd0 and trap-formation (see Figure S3). This suggests that the stability of the NCs also depends on the local coordination of the surface atoms. Only if suitable surface sites are present, does it become energetically favorable to expel one or multiple atoms from the lattice.

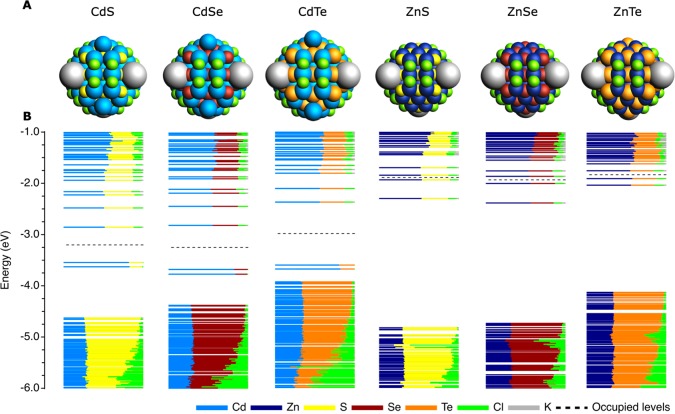
Lastly, the findings presented in this section can also be generalized to other II–VI NCs. Figure 2 shows the structures and DOS of six different M68X55Cl26 (M = Cd, Zn; X = S, Se, Te) zincblende semiconductor NCs after injection of four electrons (see Figure S4 for the structures and trap-free DOS of the uncharged NCs). It shows that the stability of a NC is highly dependent on the nature of the metal cation. While injection of four electrons leads to expulsion of surface Cd0 in the CdX NCs, the electrons in the ZnX NCs remain completely delocalized in the CB. This is in line with the thermodynamic reduction potentials of the bulk materials, which show that the Zn-chalcogenides are generally more stable against reduction than the Cd-based compounds.39 Although these potentials are based on aqueous media and neglect the details of the surface and surface passivation, they give an indication on why the injected charges are less prone to localize on Zn sites.

Figure 2.

Figure 2

Open in a new tab

Effect of charging different M68X55Cl26 (M = Cd, Zn; X = S, Se, Te) zincblende NCs on their structure and DOS. (A) Structure and (B) DOS of the NCs when charged with four electrons. The total system is kept neutral by the addition of a potassium cation for each added electron. The structure and DOS of the uncharged NCs are given in Figure S4.

We note that contrary to the observation presented here that ZnX NCs are more stable than CdX, charge injection into CdX NCs has been shown to be reversible,33,34 while reports on charging ZnX NCs are scarce. We conjecture that this may be due to the energetic position of the CB edge of the materials. Since the CB of ZnX lies higher than that of CdX,39,61 electrons injected into the CB of the Zn-based compounds have a higher electrochemical potential than electrons in the Cd-based materials. While the ZnX material would remain stable according to our calculations, the high energy of CB electrons could more easily lead to side-reactions with ligands, solvent, or impurities, which could consume the electrons. Lastly, as will be shown in the ensuing section, ligand passivation has a large influence on the stability of the NC and may also explain some of the differences found between charging Cd- and Zn-based NCs.

Charging of Imperfectly Passivated NCs

So far, perfectly passivated NCs have been considered. However, NCs often have some form of surface imperfections, like incomplete ligand passivation. Surface states resulting from such imperfections do not necessarily act as traps themselves (vide infra), but they can provide a pathway to the formation of trap states. Any ligand on the surface will stabilize the ions it is coordinated to and will hence make it harder for them to be reduced. Inversely, removing such ligands will lead to more facile reduction of the same ions.

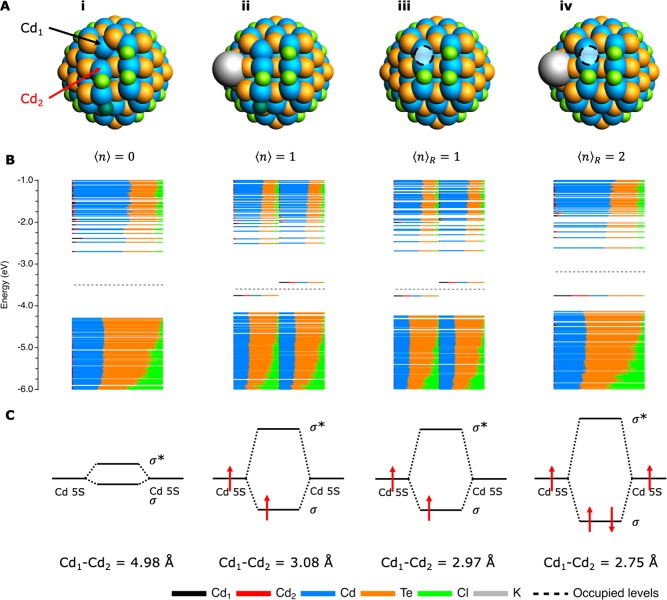
To investigate the effects of imperfect passivation, Figure 3 shows the results of charging a NC when a chloride ligand has either been removed from a (100) surface or moved to another facet. If a chloride is moved from a (100) to the neighboring (111) facet, as shown in Figure 3A-i, it can no longer stabilize Cd1 and Cd2. As a result, the structure will adapt a new geometry to minimize its energy. In Figure 3A-i, it can be seen that Cd1 moves deeper into the NC, so that its distance from Cd2 is increased to 4.98 Å (as compared to a distance of 3.95 Å for the perfectly passivated NC in Figure 1-i). Still, the bandgap remains clean (see Figure 3B-i). If, simultaneously with the displacement of this Cl–, the NC is charged with one electron (Figure 3-ii), Cd1 and Cd2 form a dimer that gives an energy state within the bandgap. We confirmed with molecular dynamics simulations that this dimer is stable (i.e., it is not removed by surface reconstruction). The same dimer-formation is observed when a chloride is removed as Cl– and an electron is injected into the NC (which is isoelectric with removing a chlorine, Cl0), as shown in Figure 3-iii. Further charging of this NC (Figure 3-iv) reduces the Cd1–Cd2 distance to 2.75 Å (as compared to a Cd1–Cd2 distance of 2.97 Å for the NC in Figure 3-iii).

Figure 3.

Figure 3

Open in a new tab

Cd–Cd dimerization upon charging of imperfectly passivated NCs. (A) Structure of the NCs when (i) a chloride (indicated in dark green) is moved from a (100) to an adjacent (111) facet (⟨n⟩ = 0); (ii) the NC is charged simultaneously with moving the chloride (⟨n⟩ = 1); (iii) a chloride is removed from the (100) facet (from the position indicated with the black circle) while an electron is injected, which is isoelectric with removing a Cl0 ligand (⟨n⟩R = 1); and (iv) when the same chloride is removed and two electrons are injected (⟨n⟩R = 2). Note that the R in ⟨n⟩R indicates that a Cl0 has been removed from the NC. For ⟨n⟩ = 1 and ⟨n⟩R = 2 a potassium cation has been added to keep the total system charge neutral. (B) The DOS for each of the NCs. (C) Simplified MO diagram, which shows that a Cd–Cd dimer is only formed when an extra electron is available to occupy the bonding MO, which in turn forms a trap state in the bandgap. The distance between Cd1 and Cd2 has been given for each of the structures to show the significant reduction in bond length upon dimerization (the Cd1–Cd2 distance for the fully passivated NC in Figure 1-i is 3.95 Å).

These results can be explained by considering the 5s orbitals of the Cd2+ ions, which could in theory overlap to form a Cd–Cd sigma-bond. However, this surface configuration is prevented by two factors. First of all, the stabilizing influence of the X-type ligands prevents the Cd2+ sites from moving closer to each other. Additionally, the 5s orbitals of Cd2+ are empty. As a result, even if the Cd2+ ions would be able to approach each other close enough to form a bond, there would be no electrons to populate the bonding MO. Thus, there would be no energetic gain by bond formation. If a chloride is moved from the (100) plane to the adjacent (111) facet (Figure 3-i), there is room for the Cd2+ ions to move toward each other. Yet, no extra electrons are present to occupy the bonding MO and no dimer is therefore formed. Only if the NC is charged (Figure 3-ii) can the extra electron occupy the bonding orbital and thus lead to dimerization. This bonding MO is then pushed out of the CB into the bandgap, where it forms a half-filled trap state, meaning that it can trap both holes and electrons. The same reasoning applies to the NC where a chlorine (Cl0) ligand has been removed (see Figure 3-iii). Charging this NC further (Figure 3-iv) will lead to the injection of a second electron in the bonding orbital, thus shortening the Cd–Cd bond, as shown in Figure 3C. Since the in-gap state is now completely filled, it can only lead to hole-trapping.

These results show that the passivation of the NC surface plays an important role in the stability of the NC in the presence of extra electrons. If the NC is perfectly passivated (as discussed in the previous section) the NC is initially stable upon reduction, with electrons occupying the CB. However, since ligands like acetates57 and Z-type complexes24 have been found to be mobile on the NC surface, there is a possibility of charging NCs with a suboptimal distribution of ligands on the surface. If a ligand diffuses from the (100) surface to another facet, injection of one electron (Figure 3-ii) can lead to the formation of a trap. Moreover, charging of NCs could force X-type ligands off the surface to retain charge neutrality58 and thus enable the formation of Cd–Cd dimers.

The results presented in this section can be generalized to larger NCs (see Figure S5) and other II–VI materials (see Figure S6). Figure S5 shows that the removal of chloride from certain sites on the (100) facet actually does not lead to dimer-formation. It is conjectured that dimerization on those specific sites may induce too high a strain on the surrounding structure to be energetically favorable.43Figure S6 shows that, contrary to the stable charging of fully passivated NCs, all singly charged Cd- and Zn-chalcogenide NCs form trap states when a chloride ligand is missing from the (100) facet, again emphasizing the importance of surface passivation for the NC stability.

Although various studies have shown that L-type amine ligands can increase the PL QY of NCs,19,26−28 this cannot be properly explained by the hypothesis that only undercoordinated chalcogenides can lead to trap states, as passivating those traps requires electron accepting Z-type ligands instead of electron donating L-type ligands.23 The atomistic picture of dimer-formation given in this section, however, may help to understand a possible mechanism of L-type passivation. Figure 4 shows that, if a Cl0 atom is replaced by an L-type methylamine ligand, Cd–Cd dimerization is prevented, even though the NC is effectively negatively charged (⟨n⟩ = 1). Instead, the extra electron remains delocalized in the CB (see Figure 4C), indicating that amines can stabilize NCs against surface reduction of metal ions.

Figure 4.

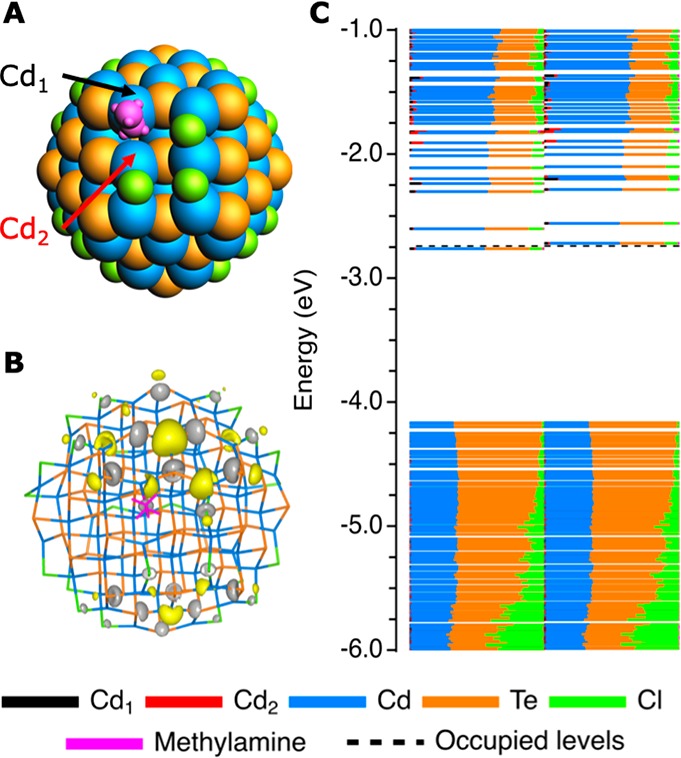
Figure 4

Open in a new tab

Influence of methylamine on the formation of Cd–Cd dimers. (A) Structure of the NC when a Cl0 is replaced by a methylamine ligand (this is isoelectric to removing a chloride and injecting one extra electron into the NC). (B) Contour plot of the HOMO level of the NC, using a contour plot value of 0.02 e/bohr3, indicating that the electron is delocalized over the NC. (C) DOS of the NC, showing a clean bandgap and one electron in the CB.

An interesting open question is whether the formation of traps on reduced surface metal ions is only relevant for charged NCs, or whether it also plays a role in neutral, photoexcited NCs and may explain a suboptimal PL QY in absence of stabilizing amine ligands. Here, we conjecture that if the time scale of dimer-formation is similar to the radiative lifetime of the photoexcited electron–hole pair, the electron could be trapped in the bonding MO of the Cd–Cd dimer before it recombines with the hole. If the time scale of dimer-formation is slow compared to the radiative lifetime, the event can still happen once in a while, and as long as the dimer exists (i.e., before recombining with a hole) it will act as a trap state, thus decreasing the PL of the NC. If an amine is now present to block the Cd-dimerization, as shown in Figure 4A, the photoexcited electron can no longer be trapped in the dimer and the PL QY remains unaffected. Although more research is required to test this hypothesis, such a mechanism may eventually help to understand not only the PL-increasing effect of amines19,26−28 but also perhaps processes like blinking and delayed photoluminescence.62

Conclusions

In conclusion, DFT calculations have been used to study the effects of charging fully and imperfectly passivated CdTe NCs on their electronic structure. It is shown that charge injection can lead to trap-formation via two pathways: metal atom ejection from perfectly passivated NCs or metal–metal dimer-formation in imperfectly passivated NCs. The small, fully passivated model NCs used here were found to be stable up to a charge of two electrons. Further charge injection led to charge localization and consequent reduction of Cd2+ ions. The resulting Cd0 atoms were ejected from the lattice and acted as localized in-gap states. The effects of surface passivation were probed by charging NCs with missing or displaced ligands. It was found that, if NCs miss an X-type ligand from a (100) facet, injection of one electron can already lead to Cd-dimerization and trap-formation. Addition of L-type ligands can prevent dimer-formation and is suggested to also prevent trapping of photoexcited electrons in charge neutral NCs, which emphasizes the importance of surface passivation for the stability of NCs. The results presented in this work can be generalized to NCs of different sizes and other Cd- and Zn-based zincblende chalcogenide NCs. They show that excess charges can lead to the formation of new surface defects and can therefore help to gain a better understanding of trap-formation during (electro)chemical doping or illumination of NCs.

Methods

All calculations have been carried out at the DFT level with a PBE exchange-correlation function63 and double-ζ basis set, as used in the CP2K quantum chemistry software package.64 Relativistic effects have been taken into account by means of effective core-potentials. All structures have been optimized to the lowest energy in the gas phase at 0 K. Unrestricted calculations were used for all systems with an odd total number of electrons. Further details are given in the main text.

Acknowledgments

A.J.H. acknowledges support from the European Research Council Horizon 2020 ERC Grant 678004 (Doping on Demand). I.I. acknowledges The Netherlands Organization of Scientific Research (NWO) for financial support through the Innovational Research Incentive (Vidi) Scheme (Grant 723.013.002). The computational work was carried out on the Dutch national e-infrastructure with the support of the SURF Cooperative.

Supporting Information Available

The Supporting Information is available free of charge on the ACS Publications website at DOI: 10.1021/acs.chemmater.9b01395.

  • Structures and DOS for the following: charging fully passivated NCs with Li+ and Cs+ counterions; removing the Cd0 sites from a charged NC; charging larger fully passivated NCs; different fully passivated neutral Cd- and Zn-based zincblende chalcogenide NCs; charging a larger CdTe NC when a chloride ligand has been removed from different sites; and charging different Cd- and Zn-based zincblende chalcogenide NCs when a chloride ligand has been removed (PDF)

Author Contributions

The manuscript was written through contributions of all authors. All authors have given approval to the final version of the manuscript.

The authors declare no competing financial interest.

Supplementary Material

cm9b01395_si_001.pdf (1.5MB, pdf)

References

  1. Talapin D. V.; Lee J.-S.; Kovalenko M. V.; Shevchenko E. V. Prospects of Colloidal Nanocrystals for Electronic and Optoelectronic Applications. Chem. Rev. 2010, 110, 389–458. 10.1021/cr900137k. [DOI] [PubMed] [Google Scholar]
  2. de Mello Donegaá C. Synthesis and properties of colloidal heteronanocrystals. Chem. Soc. Rev. 2011, 40, 1512–1546. 10.1039/C0CS00055H. [DOI] [PubMed] [Google Scholar]
  3. Shirasaki Y.; Supran G. J.; Bawendi M. G.; Bulović V. Emergence of colloidal quantum-dot light-emitting technologies. Nat. Photonics 2013, 7, 13. 10.1038/nphoton.2012.328. [DOI] [Google Scholar]
  4. Grim J. Q.; Manna L.; Moreels I. A sustainable future for photonic colloidal nanocrystals. Chem. Soc. Rev. 2015, 44, 5897–5914. 10.1039/C5CS00285K. [DOI] [PubMed] [Google Scholar]
  5. Carey G. H.; et al. Colloidal Quantum Dot Solar Cells. Chem. Rev. 2015, 115, 12732–12763. 10.1021/acs.chemrev.5b00063. [DOI] [PubMed] [Google Scholar]
  6. Ganesan A. A.; Houtepen A. J.; Crisp R. W. Quantum Dot Solar Cells: Small Beginnings Have Large Impacts. Appl. Sci. 2018, 8, 1867. 10.3390/app8101867. [DOI] [Google Scholar]
  7. Dai X.; Deng Y.; Peng X.; Jin Y. Quantum-Dot Light-Emitting Diodes for Large-Area Displays: Towards the Dawn of Commercialization. Adv. Mater. 2017, 29, 1607022. 10.1002/adma.201607022. [DOI] [PubMed] [Google Scholar]
  8. Li X.; et al. Bright colloidal quantum dot light-emitting diodes enabled by efficient chlorination. Nat. Photonics 2018, 12, 159. 10.1038/s41566-018-0105-8. [DOI] [Google Scholar]
  9. Choi M. K.; et al. Extremely Vivid, Highly Transparent, and Ultrathin Quantum Dot Light-Emitting Diodes. Adv. Mater. 2018, 30, 1703279. 10.1002/adma.201703279. [DOI] [PubMed] [Google Scholar]
  10. Wu K.; Park Y.-S.; Lim J.; Klimov V. I. Towards zero-threshold optical gain using charged semiconductor quantum dots. Nat. Nanotechnol. 2017, 12, 1140. 10.1038/nnano.2017.189. [DOI] [PubMed] [Google Scholar]
  11. Geiregat P.; et al. Continuous-wave infrared optical gain and amplified spontaneous emission at ultralow threshold by colloidal HgTe quantum dots. Nat. Mater. 2018, 17, 35. 10.1038/nmat5000. [DOI] [PubMed] [Google Scholar]
  12. Lim J.; Park Y.-S.; Klimov V. I. Optical gain in colloidal quantum dots achieved with direct-current electrical pumping. Nat. Mater. 2018, 17, 42. 10.1038/nmat5011. [DOI] [PubMed] [Google Scholar]
  13. Katsiev K.; et al. The Complete In-Gap Electronic Structure of Colloidal Quantum Dot Solids and Its Correlation with Electronic Transport and Photovoltaic Performance. Adv. Mater. 2014, 26, 937–942. 10.1002/adma.201304166. [DOI] [PubMed] [Google Scholar]
  14. Boles M. A.; Ling D.; Hyeon T.; Talapin D. V. The surface science of nanocrystals. Nat. Mater. 2016, 15, 141. 10.1038/nmat4526. [DOI] [PubMed] [Google Scholar]
  15. Kagan C. R.; Lifshitz E.; Sargent E. H.; Talapin D. V. Building devices from colloidal quantum dots. Science 2016, 353, aac5523 10.1126/science.aac5523. [DOI] [PubMed] [Google Scholar]
  16. Reiss P.; Protière M.; Li L. Core/shell semiconductor nanocrystals. Small 2009, 5, 154–168. 10.1002/smll.200800841. [DOI] [PubMed] [Google Scholar]
  17. Jasieniak J.; Mulvaney P. From Cd-Rich to Se-Rich - the Manipulation of CdSe Nanocrystal Surface Stoichiometry. J. Am. Chem. Soc. 2007, 129, 2841–2848. 10.1021/ja066205a. [DOI] [PubMed] [Google Scholar]
  18. Page R. C.; et al. Near-Unity Quantum Yields from Chloride Treated CdTe Colloidal Quantum Dots. Small 2015, 11, 1548–1554. 10.1002/smll.201402264. [DOI] [PMC free article] [PubMed] [Google Scholar]
  19. Kirkwood N.; et al. Finding and Fixing Traps in II-VI and III-V Colloidal Quantum Dots: The Importance of Z-type Ligand Passivation. J. Am. Chem. Soc. 2018, 140, 15712–15723. 10.1021/jacs.8b07783. [DOI] [PMC free article] [PubMed] [Google Scholar]
  20. Weaver A. L.; Gamelin D. R. Photoluminescence Brightening via Electrochemical Trap Passivation in ZnSe and Mn2+-Doped ZnSe Quantum Dots. J. Am. Chem. Soc. 2012, 134, 6819–6825. 10.1021/ja301102h. [DOI] [PubMed] [Google Scholar]
  21. Boehme S. C.; et al. Density of Trap States and Auger-mediated Electron Trapping in CdTe Quantum-Dot Solids. Nano Lett. 2015, 15, 3056–3066. 10.1021/acs.nanolett.5b00050. [DOI] [PubMed] [Google Scholar]
  22. van der Stam W.; et al. Spectroelectrochemical Signatures of Surface Trap Passivation on CdTe Nanocrystals. Chem. Mater. 2018, 30, 8052–8061. 10.1021/acs.chemmater.8b03893. [DOI] [PMC free article] [PubMed] [Google Scholar]
  23. Houtepen A. J.; Hens Z.; Owen J. S.; Infante I. On the Origin of Surface Traps in Colloidal II-VI Semiconductor Nanocrystals. Chem. Mater. 2017, 29, 752–761. 10.1021/acs.chemmater.6b04648. [DOI] [Google Scholar]
  24. Saniepay M.; Mi C.; Liu Z.; Abel E. P.; Beaulac R. Insights into the Structural Complexity of Colloidal CdSe Nanocrystal Surfaces: Correlating the Efficiency of Nonradiative Excited-State Processes to Specific Defects. J. Am. Chem. Soc. 2018, 140, 1725–1736. 10.1021/jacs.7b10649. [DOI] [PubMed] [Google Scholar]
  25. Knowles K. E.; Tice D. B.; McArthur E. A.; Solomon G. C.; Weiss E. A. Chemical Control of the Photoluminescence of CdSe Quantum Dot-Organic Complexes with a Series of Para-Substituted Aniline Ligands. J. Am. Chem. Soc. 2010, 132, 1041–1050. 10.1021/ja907253s. [DOI] [PubMed] [Google Scholar]
  26. Shen Y.; Tan R.; Gee M. Y.; Greytak A. B. Quantum Yield Regeneration: Influence of Neutral Ligand Binding on Photophysical Properties in Colloidal Core/Shell Quantum Dots. ACS Nano 2015, 9, 3345–3359. 10.1021/acsnano.5b00671. [DOI] [PubMed] [Google Scholar]
  27. Gao Y.; Peng X. Photogenerated Excitons in Plain Core CdSe Nanocrystals with Unity Radiative Decay in Single Channel: The Effects of Surface and Ligands. J. Am. Chem. Soc. 2015, 137, 4230–4235. 10.1021/jacs.5b01314. [DOI] [PubMed] [Google Scholar]
  28. Pu C.; Peng X. To Battle Surface Traps on CdSe/CdS Core/Shell Nanocrystals: Shell Isolation versus Surface Treatment. J. Am. Chem. Soc. 2016, 138, 8134–8142. 10.1021/jacs.6b02909. [DOI] [PubMed] [Google Scholar]
  29. van der Stam W.et al. Revealing Trap-Assisted Auger Recombination in CdSe/CdS Core/Shell Quantum Dots and its Elimination by ZnS Growth with Spectroelectrochemistry. Chem. Mater. 2019, submitted. [Google Scholar]
  30. Shim M.; Guyot-Sionnest P. n-type colloidal semiconductor nanocrystals. Nature 2000, 407, 981. 10.1038/35039577. [DOI] [PubMed] [Google Scholar]
  31. Koh W.-k.; Koposov A. Y.; Stewart J. T.; Pal B. N.; Robel I.; Pietryga J. M.; Klimov V. I.; et al. Heavily doped n-type PbSe and PbS nanocrystals using ground-state charge transfer from cobaltocene. Sci. Rep. 2013, 3, 2004. 10.1038/srep02004. [DOI] [PMC free article] [PubMed] [Google Scholar]
  32. Garcia G.; et al. Dynamically Modulating the Surface Plasmon Resonance of Doped Semiconductor Nanocrystals. Nano Lett. 2011, 11, 4415–4420. 10.1021/nl202597n. [DOI] [PubMed] [Google Scholar]
  33. Wang C.; Shim M.; Guyot-Sionnest P. Electrochromic Nanocrystal Quantum Dots. Science 2001, 291, 2390–2392. 10.1126/science.291.5512.2390. [DOI] [PubMed] [Google Scholar]
  34. Guyot-Sionnest P.; Wang C. Fast Voltammetric and Electrochromic Response of Semiconductor Nanocrystal Thin Films. J. Phys. Chem. B 2003, 107, 7355–7359. 10.1021/jp0275084. [DOI] [Google Scholar]
  35. Rinehart J. D.; Schimpf A. M.; Weaver A. L.; Cohn A. W.; Gamelin D. R. Photochemical Electronic Doping of Colloidal CdSe Nanocrystals. J. Am. Chem. Soc. 2013, 135, 18782–18785. 10.1021/ja410825c. [DOI] [PubMed] [Google Scholar]
  36. Tsui E. Y.; Carroll G. M.; Miller B.; Marchioro A.; Gamelin D. R. Extremely Slow Spontaneous Electron Trapping in Photodoped n-Type CdSe Nanocrystals. Chem. Mater. 2017, 29, 3754–3762. 10.1021/acs.chemmater.7b00839. [DOI] [PMC free article] [PubMed] [Google Scholar]
  37. Gerischer H. On the Stability of Semiconductor Electrodes Against Photodecomposition. J. Electroanal. Chem. Interfacial Electrochem. 1977, 82, 133–143. 10.1016/S0022-0728(77)80253-2. [DOI] [Google Scholar]
  38. Nadjo L. The characterization and behaviour of n-and p-CdTe electrodes in acetonitrile solutions. J. Electroanal. Chem. Interfacial Electrochem. 1980, 108, 29–47. 10.1016/S0022-0728(80)80090-8. [DOI] [Google Scholar]
  39. Chen S.; Wang L.-W. Thermodynamic Oxidation and Reduction Potentials of Photocatalytic Semiconductors in Aqueous Solution. Chem. Mater. 2012, 24, 3659–3666. 10.1021/cm302533s. [DOI] [Google Scholar]
  40. Voznyy O.; et al. A Charge-Orbital Balance Picture of Doping in Colloidal Quantum Dot Solids. ACS Nano 2012, 6, 8448–8455. 10.1021/nn303364d. [DOI] [PubMed] [Google Scholar]
  41. Zherebetskyy D.; Zhang Y.; Salmeron M.; Wang L.-W. Tolerance of Intrinsic Defects in PbS Quantum Dots. J. Phys. Chem. Lett. 2015, 6, 4711–4716. 10.1021/acs.jpclett.5b02202. [DOI] [PubMed] [Google Scholar]
  42. Hwang G. W.; et al. Identifying and Eliminating Emissive Sub-bandgap States in Thin Films of PbS Nanocrystals. Adv. Mater. 2015, 27, 4481–4486. 10.1002/adma.201501156. [DOI] [PMC free article] [PubMed] [Google Scholar]
  43. Voznyy O.; Thon S. M.; Ip A. H.; Sargent E. H. Dynamic Trap Formation and Elimination in Colloidal Quantum Dots. J. Phys. Chem. Lett. 2013, 4, 987–992. 10.1021/jz400125r. [DOI] [PubMed] [Google Scholar]
  44. Giansante C.; Infante I. Surface Traps in Colloidal Quantum Dots: A Combined Experimental and Theoretical Perspective. J. Phys. Chem. Lett. 2017, 8, 5209–5215. 10.1021/acs.jpclett.7b02193. [DOI] [PMC free article] [PubMed] [Google Scholar]
  45. Shim M.; Wang C.; Norris D. J.; Guyot-Sionnest P. Doping and Charging in Colloidal Semiconductor Nanocrystals. MRS Bull. 2001, 26, 1005–1008. 10.1557/mrs2001.257. [DOI] [Google Scholar]
  46. Mocatta D.; et al. Heavily Doped Semiconductor Nanocrystal Quantum Dots. Science 2011, 332, 77–81. 10.1126/science.1196321. [DOI] [PubMed] [Google Scholar]
  47. Shiragami T.; Ankyu H.; Fukami S.; Pac C.; Yanaglda S.; Mori H.; Fujita H. Semiconductor Photocatalysis: Visible Light lnduced Photoreduction of Aromatic Ketones and Electron-deficient Alkenes Catalysed by Quantised Cadmium Sulfide. J. Chem. Soc., Faraday Trans. 1992, 88, 1055–1061. 10.1039/ft9928801055. [DOI] [Google Scholar]
  48. Zhao J.; Holmes M. A.; Osterloh F. E. Quantum Confinement Controls Photocatalysis: A free Energy Analysis for Photocatalytic Proton Reduction at CdSe Nanocrystals. ACS Nano 2013, 7, 4316–4325. 10.1021/nn400826h. [DOI] [PubMed] [Google Scholar]
  49. Anderson N. C.; Hendricks M. P.; Choi J. J.; Owen J. S. Ligand Exchange and the Stoichiometry of Metal Chalcogenide Nanocrystals: Spectroscopic Observation of Facile Metal-Carboxylate Displacement and Binding. J. Am. Chem. Soc. 2013, 135, 18536–18548. 10.1021/ja4086758. [DOI] [PMC free article] [PubMed] [Google Scholar]
  50. Greaney M. J.; et al. Controlling the Trap State Landscape of Colloidal CdSe Nanocrystals with Cadmium Halide Ligands. Chem. Mater. 2015, 27, 744–756. 10.1021/cm503529j. [DOI] [Google Scholar]
  51. Drijvers E.; De Roo J.; Martins J. C.; Infante I.; Hens Z. Ligand Displacement Exposes Binding Site Heterogeneity on CdSe Nanocrystal Surfaces. Chem. Mater. 2018, 30, 1178–1186. 10.1021/acs.chemmater.7b05362. [DOI] [Google Scholar]
  52. Singh S.; et al. Colloidal CdSe Nanoplatelets, A Model for Surface Chemistry/Optoelectronic Property Relations in Semiconductor Nanocrystals. J. Am. Chem. Soc. 2018, 140, 13292–13300. 10.1021/jacs.8b07566. [DOI] [PubMed] [Google Scholar]
  53. Azpiroz J. M.; Ugalde J. M.; Infante I. Benchmark Assessment of Density Functional Methods on Group II-VI MX (M = Zn, Cd; X = S, Se, Te) Quantum Dots. J. Chem. Theory Comput. 2014, 10, 76–89. 10.1021/ct400513s. [DOI] [PubMed] [Google Scholar]
  54. Gudjonsdottir S.; van der Stam W.; Kirkwood N.; Evers W. H.; Houtepen A. J. The Role of Dopant Ions on Charge Injection and Transport in Electrochemically Doped Quantum Dot Films. J. Am. Chem. Soc. 2018, 140, 6582–6590. 10.1021/jacs.8b01347. [DOI] [PMC free article] [PubMed] [Google Scholar]
  55. Fox M.Optical properties of solids; Oxford University Press, 2010. [Google Scholar]
  56. Manna L.; Wang L. W.; Cingolani R.; Alivisatos A. P. First-Principles Modeling of Unpassivated and Surfactant-Passivated Bulk Facets of Wurtzite CdSe: A Model System for Studying the Anisotropic Growth of CdSe Nanocrystals. J. Phys. Chem. B 2005, 109, 6183–6192. 10.1021/jp0445573. [DOI] [PubMed] [Google Scholar]
  57. Voznyy O. Mobile Surface Traps in CdSe Nanocrystals with Carboxylic Acid Ligands. J. Phys. Chem. C 2011, 115, 15927–15932. 10.1021/jp205784g. [DOI] [Google Scholar]
  58. Hartley C. L.; Dempsey J. L. Electron-Promoted X-Type Ligand Displacement at CdSe Quantum Dot Surfaces. Nano Lett. 2019, 19, 1151–1157. 10.1021/acs.nanolett.8b04544. [DOI] [PubMed] [Google Scholar]
  59. Shim M.; Wang C.; Guyot-Sionnest P. Charge-Tunable Optical Properties in Colloidal Semiconductor Nanocrystals. J. Phys. Chem. B 2001, 105, 2369–2373. 10.1021/jp0035683. [DOI] [Google Scholar]
  60. Haram S. K.; Quinn B. M.; Bard A. J. Electrochemistry of CdS Nanoparticles: A Correlation between Optical and Electrochemical Band Gaps. J. Am. Chem. Soc. 2001, 123, 8860–8861. 10.1021/ja0158206. [DOI] [PubMed] [Google Scholar]
  61. Norris D. J.; Efros A. L.; Erwin S. C. Doped Nanocrystals. Science 2008, 319, 1776–1779. 10.1126/science.1143802. [DOI] [PubMed] [Google Scholar]
  62. Rabouw F. T.; et al. Delayed Exciton Emission and Its Relation to Blinking in CdSe Quantum Dots. Nano Lett. 2015, 15, 7718–7725. 10.1021/acs.nanolett.5b03818. [DOI] [PubMed] [Google Scholar]
  63. Perdew J. P.; Burke K.; Ernzerhof M. Generalized Gradient Approximation Made Simple. Phys. Rev. Lett. 1996, 77, 3865–3868. 10.1103/PhysRevLett.77.3865. [DOI] [PubMed] [Google Scholar]
  64. Hutter J.; Iannuzzi M.; Schiffmann F.; VandeVondele J. CP2K: atomistic simulations of condensed matter systems. Wiley Interdiscip. Rev. Comput. Mol. Sci. 2014, 4, 15–25. 10.1002/wcms.1159. [DOI] [Google Scholar]

Associated Data

This section collects any data citations, data availability statements, or supplementary materials included in this article.

Supplementary Materials

cm9b01395_si_001.pdf (1.5MB, pdf)

Articles from Chemistry of Materials are provided here courtesy of American Chemical Society

RESOURCES