Inventory management of surgical supplies and sterile instruments in hospitals: a literature review

ABSTRACT

Operating rooms are considered a significant revenue source, as well as the main source of waste and cost, among the hospital’s departments. Any cost savings in operating rooms will have a broad financial impact. Over the last decades, many researchers and practitioners have conducted studies to deal with the issue of managing surgical supplies and instruments, which are highly affected by surgeons’ preferences. The purpose of this article is to present an up-to-date review of research in the field of inventory management of surgical supplies and instruments. We have analysed the literature in a systematic manner and organised the identified papers into two groups: the papers that were published by scientific researchers and developed optimisation techniques and the papers that were published by practitioners and reported their observations of the current issues in the operating room. We also identify the future research directions leading to operating room inventory cost reduction.

1. Introduction

According to a report published by the Centers for Medicare and Medicaid Service (CMS, 2015), healthcare expenditures in the USA are growing at an average rate of 5.8% per year, 1.3% faster than the Gross Domestic Product (GDP), and are projected to account for about 20.1% of the GDP by 2025. This report also indicated that hospitals represented 32% of national health expenditures in 2015. Hospital operating rooms (ORs) are cost centres, which are responsible for about 60% of total hospital cost (Weiss et al., 2016), although they generate a large proportion of the hospital’s revenue as well (Cardoen, Beliën, & Vanhoucke, 2015). Therefore any systematic cost reduction in ORs would significantly impact the nation’s economy.

In an OR, in order to reduce cost through efficient utilisation of capacity, as well as enhance revenue by performing more cases, two domains have been studied: (1) planning and scheduling of resources; see extensive reviews of Cardoen, Demeulemeester, and Beliën (2010), Guerriero and Guido (2011), Demeulemeester, Beliën, Cardoen, and Samudra (2013), Erhard, Schoenfelder, Fügener, and Brunner (2017); and (2) inventory management of surgical supplies and sterile instruments. Despite numerous research studies and practitioners’ reports on the management of OR inventories, which play a significant role in hospital costs and wastes, to the best of our knowledge, there is no comprehensive review dedicated to OR inventory management of surgical supplies and sterile instruments. There exist some general reviews in application of operations research/management in healthcare (Fakhimi & Probert, 2013; Hulshof, Kortbeek, Boucherie, Hans, & Bakker, 2012; Rais & Vianaa, 2011), healthcare supply chain management (De Vries & Huijsman, 2011; Dobrzykowski, Saboori Deilami, Hong, & Kim, 2014), and hospital material logistics (Volland, Fügener, Schoenfelder, & Brunner, 2016), but these reviews do not deal with the unique challenges associated with operating room inventories.

OR inventory needs to be stocked in multiple locations and generally contains more items compared to other hospital inventories (Melson & Schultz, 1989). One of the difficulties of inventory management in an OR in comparison to a manufacturing setting is the trade-off between inventory costs and level of required service (availability of the supplies) that each surgeon expects to receive (De Vries, 2011). This leads to a conflict between inventory managers, who are attempting to lower inventory costs, and surgeons in the OR, who expect to have everything on hand (Reis & Fontaine, 1993). In addition, most hospitals lack a standardised process to manage materials. Especially when it comes to the OR, management of surgical supplies and instruments is highly affected by the surgeon “preference card”. The preference card is a list of all items and their quantity that a surgeon requires in the OR when performing a given procedure (Young & Conley, 1988). It also includes the number of each supply that the surgeons request to be opened before beginning a procedure. There is an inclination that surgeons request material “just in case”, not necessarily based on the patient needs. For example, a preference card examination of 10 surgeons who performed a laparoscopic cholecystectomy procedure revealed that the total disposable supply cost ranged from $92 to $637 with a mean of $333 (Allen & Polk, 2002).

It has been also shown that surgical supplies and instruments should be quickly available to staff for performing the diverse procedures (Little & Coughlan, 2008), and the high rate of material availability is often attained by stocking excessive quantities (Rappold, Van Roo, Di Maritinelly, & Riane, 2011). Lack of available supplies, especially for expensive items, requires emergency fulfilment that causes additional labour costs, delays in patient treatment, and potentially life-threatening situations for the patient. Since surgical supplies are typically selected by the surgeon without any comparative data (Burns, Lee, Bradlow, & Antonacci, 2007), eliminating or even changing an item on the preference card could cause surgeon dissatisfaction. Communication with surgeons and providing them with sufficient information about alternatives can create trust and overcome their concerns about the changes (Reis & Fontaine, 1993).

Another challenge in controlling surgical supplies and instruments is that inventory between the Core (a storage area surrounded by ORs that is used to support ORs during the procedures) and the ORs themselves is often not tracked using information systems (Rappold et al., 2011). Thus, there is a gap of information that makes control of inventory even more complicated.

In dealing with the aforementioned challenges, the primary objective of an inventory management system in a healthcare environment is to reduce inventory costs without sacrificing service level (Rossetti, Buyurgan, & Pohl, 2012).

The goal of this review article is threefold. First, we intend to provide a comprehensive review of all relevant publications about inventory management of surgical supplies and sterile instruments for the purpose of cost savings. Second, we underline the area of cost reduction related to the OR inventory that is specified by practitioners. Third, we want to highlight the OR inventory-related aspects of cost reduction that have not been sufficiently examined to identify issues for researchers to study, model, and analyse.

The rest of this article is structured as follows. The research methodology is covered in Section ‎2, followed by Section ‎3 containing the terminology and a framework for classifying the relevant literature in inventory management of surgical supplies and sterile instruments. The literature review along the framework is presented in Section ‎4 and Section ‎5. In the final section, a discussion, conclusion, and the interesting future research opportunities are presented.

2. Research methodology

2.1. Scope

The general questions that we pursue in this review are: (1) what decisions are made regarding inventory management of surgical supplies and sterile instruments; (2) what performance metrics are used in making these decisions; and (3) what constraints are considered when making inventory decisions. In addition to the research papers dedicated to OR inventory management, inventory control models implemented in other hospital areas, which can be extrapolated to the OR, are also part of this review. However, only supplies that are managed by the material management department and stocked in a central storage (CS) prior to being distributed to the point-of-use (POU) locations fall within the scope of this review. Therefore, pharmaceutical products that are often perishable and primarily managed by the pharmacy are out of the scope of our review. For more details about the management of perishable products see Bakker, Riezebos, and Teunter (2012); Janssen el al (Janssen, Claus, & Sauer, 2016); Pahl and Voß (2014), and about drug inventory management see Jebbor, El Afia, Chiheb, and Ouzayd (2016).

2.2. Bibliographic search

The strategy for searching and identifying the relevant articles followed the Boell et al. (2015) guidelines in four steps.

1. The first step was to develop a protocol that specifies the search criteria and appropriate search databases aiming to cover research questions. Table 1 shows our research protocol within three databases of Science Direct, Web of Science, and PubMed.

2. In the second step, a search within the selected databases was conducted.

3. In the third step, the title and abstract of the returned publications were screened to identify the appropriate papers for review.

4. The selected papers were reviewed and a backward search was conducted by references within each paper, as well as investigating the cited publications to the selected papers through Google Scholar.

Table 1.

Literature search criteria and number of papers found within each database (duplicates between databases are removed in relevant papers).

	Science direct				Web of science				PubMed				Google scholar
			Relevant papers				Relevant papers				Relevant papers		Backward search
Search criteria	Search fields	Result	Oa	Pb	Search fields	Result	O	P	Search fields	Result	O	P	O	P
(“operating room” OR “operating theatre” OR “surgical suite” OR “surgical supply” OR “surgical instrument” OR “sterile instrument” OR “sterile supply”) AND (“inventory management” OR “supply management” OR “material management” OR “instrument” OR “ supply” OR “custom pack” OR “tray” OR “kit” OR “package” OR “preference card” OR “physician preference” OR ”waste”)	All fields	199	2	2	All fields	592	2	6	Title, abstract	570	1	5	7	5
(“healthcare” OR “hospital”) AND (“inventory management” OR “supply management” OR “material management” OR “supply chain”) NOT (“Pharmacy” OR “medication” OR “pharmaceutical “ OR “medicine” OR “drug”)	Title, abstract, keyword	76	4	0	Title	89	1	0	Title, abstract	398	3	2

^a O refers to the number of papers that contain optimisation methods

^b P refers to the number of practitioners reports

The number of publications retrieved within each database with respect to the search criteria, as well as the relevant papers selected for review in two categories of optimisation and practitioner are shown in Table 1.

Considering the search criteria specified in Table 1, 1,924 papers were found. However, many of them were duplicated between databases. According to Step 3, after screening the titles and abstracts of the retrieved papers, as well as removing duplicates, 28 papers were selected as relevant papers and categorised in two groups of papers containing optimisation models and practitioners’ reports. Twelve papers were also added to the relevant papers in Step 4 through Google Scholar exploration. Finally, 40 papers (20 papers containing optimisation techniques and 20 papers containing practitioner reports) met all of our criteria for being reviewed. Two journals – Health Care Management Science and European Journal of Operational Research – published papers that incorporated optimisation methods (see Table 2). Practitioners, on the other hand, were inclined to publish in the AORN Journal (see Table 3).

Table 2.

Distribution of publications containing optimisation techniques across different journals.

	Time period
Journal name	Before 2003	2003–2007	2008–2012	2013–2017	Total
Health Care Management Science	0	0	2	1	3
European Journal of Operational Research	0	1	0	2	3
Computers & Operations Research	1	1	0	0	2
International Journal of Production Economics	1	0	1	0	2
International Journal of Production Research	0	0	0	2	2
Conference proceedings	0	1	1	0	2
Decision Sciences	0	0	0	1	1
Hospital Material Management Quarterly	1	0	0	0	1
IIE Transactions on Healthcare Systems Engineering	0	0	0	1	1
Journal of Medical Systems	1	0	0	0	1
Journal of the Operational Research Society	0	0	1	0	1
Supply Chain Forum: An International Journal	0	0	1	0	1
Total	4	3	6	7	20

Table 3.

Distribution of publications containing practitioner reports across different journals.

	Time period
Journal name	Before 2003	2003–2007	2008–2012	2013–2017	Total
Association of periOperative Registered Nurses (AORN) Journal	1	1	1	2	5
Healthcare Financial Management	1	1	0	0	2
Canadian Operating Room Nursing Journal	0	0	1	0	1
Canadian Journal of Surgery	0	0	0	1	1
Current Opinion in Anesthesiology	0	0	1	0	1
Journal for Healthcare Quality	0	0	0	1	1
Journal of Gynecologic Surgery	0	0	0	1	1
Journal of Hospital Administration	0	0	0	1	1
Journal of Minimally Invasive Gynecology	0	0	0	1	1
Journal of Neurosurgery	0	0	0	1	1
Journal of Pediatric Urology	0	0	0	1	1
Journal of Perioperative Practice	0	0	0	1	1
Journal of the American College of Surgeons	0	0	0	1	1
Surgery (United States)	0	0	0	1	1
Conference proceedings	0	0	0	1	1
Total	2	2	3	13	20

It also can be observed in Figure 1 that there is an increasing trend in the number of publications addressing issues of managing surgical supplies and sterile instruments in hospitals. Given the important of cost containment pointed out by practitioners in the recent years, it is estimated that this area will attract more researchers’ attention to develop scientific methods.

Figure 1.

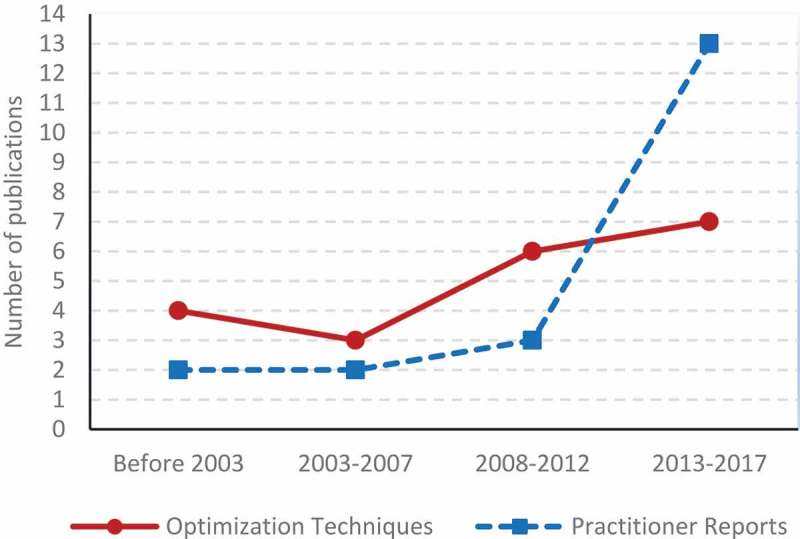
Trend of number of publications.

3. Literature classification scheme

In order to shape a framework for classifying the identified relevant papers, we briefly discuss the terminology of inventory management in healthcare, concentrating on surgical supplies and sterile instruments.

3.1. Surgical supplies

There are two prevailing decision-making approaches in the inventory management context: (1) inventory-oriented models such as traditional reorder point and Economic Order Quantity (EOQ) models, which are only based on the costs (like order cost and holding cost) and (2) supply-chain oriented models, which account for limited storage capacity and constrained human resources (Lapierre & Ruiz, 2007). Deployment of pure inventory models is not practical in an OR since, in some cases, service given to the POUs (eg, operating theatre, laboratory, care unit), is a greater concern than the cost (Lapierre & Ruiz, 2007).

Hugos (2011) defines supply chain management as “the coordination of production, inventory, location, and transportation among the participants in a supply chain to achieve the best mix of responsiveness and efficiency for the market being served” (p. 4). The healthcare supply chain, which can be considered as an external chain and an internal chain, consists of managing medical and surgical supplies and pharmaceutical products (Landry & Beaulieu, 2013). A general framework of a hospital supply chain is presented in Figure 2. In some systems, the distributor may directly deliver products to the department storerooms, known as a “semi-direct delivery” system, or the supplier may take responsibility for meeting the patient demand and refill supplies at the POUs, known as “direct delivery” (Aptel & Pourjalali, 2001; Volland et al., 2016). From a hospital’s point-of-view, the products that are not stocked in the hospital central storage (ie, either semi-direct delivery or direct delivery), are called “non-stock” items (Landry & Beaulieu, 2013).

Figure 2.

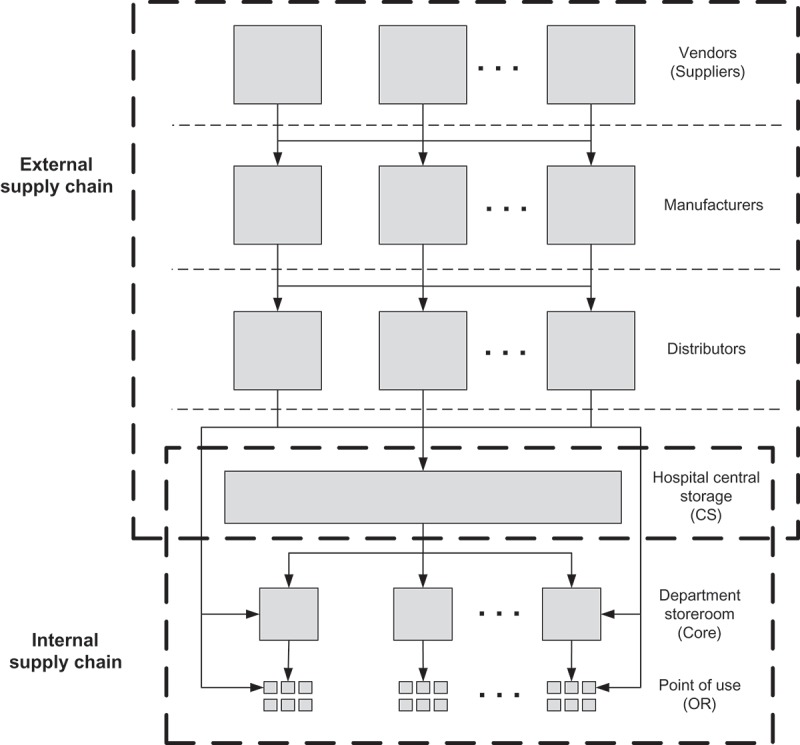
Hospital supply chain. Source: Adopted from Rivard‐Royer, Landry, and Beaulieu (2002). For surgical supplies, “Hospital central storage” is CS, “Department storeroom” is Core, and “Point of use” is OR.

The internal supply chain has been less-covered in the literature, whereas the external supply chain is the dominating one (Landry & Philippe, 2004; Volland et al., 2016). Inventories in the central storage, which are controlled by the procurement department, are known as “global inventory”. The inventories in the POUs that are often controlled by each department are known as “local inventory” (Dellaert & van de Poel, 1996). For the local inventory, the lead time is very short due to the availability of products at upstream inventory (Bijvank & Vis, 2012), which is often a central storage. In a case of a stock-out (ie, there is a demand for an item but there is no inventory on hand at the POU), either a substitute product would be used or the item would be obtained from another location through an emergency delivery. This situation imposes additional costs to the system and should be avoided, which is why shortage in healthcare inventory management is considered to be similar to lost sales (Bijvank & Vis, 2012).

Figure 2 shows a three-echelon internal supply chain. Echelon refers to the physical location where supplies are being stocked (Vila-Parrish & Ivy, 2013). However, depending on the system under consideration, some systems may be modelled as a two-echelon supply chain. Figure 2 illustrates the internal supply chain in general. Regarding the OR inventory, supplies are usually stocked in the CSs, Cores and within ORs themselves. Before performing the procedure, a picking list would be generated based on the preference card to build the case cart in the CSs. The Cores and within-OR storage support ORs during the procedures. After a case is completed, items that are unused (but still usable) would be restocked to the CSs.

In the literature, there are five fundamental inventory control policies, which are applicable to both global and local inventories, as shown in Table 4 (Rossetti et al., 2012). These policies are to answer when and how much of each item should be replenished.

Table 4.

Different types of inventory control policies.

Inventory control policy	Symbol	Description
Periodic review (periodic inventory system)	(R, s, S)	Every review cycle (R), if inventory level is equal to or less than reorder point (s), replenishment would be triggered to increase inventory up-to-level (S). The amount ordered is not constant and depends on the current inventory.
	(R, r, Q)	Every review cycle (R), if inventory level is equal to or less than reorder point (r) replenishment would be triggered with a constant amount (Q).
	(R, S)	In every review cycle (R), an order is placed to increase inventory to the up-to-level (S).
Continuous review (perpetual inventory system)	(s, S)	Whenever the inventory level for a given item goes below the reorder point (s), a replenishment process would be triggered to increase inventory to up-to-level (S).
	(r, Q)	Whenever the inventory level for a given item goes below the reorder point (r), an order is placed with a constant size (Q).

In the healthcare setting, the (R, S) policy – known as Periodic Automatic Replenishment (PAR) level – is widely used because of its simplicity and consolidation of orders in replenishment periods (Rossetti et al., 2012). If the holding and shortage costs are linear, the optimal model for periodic review is the (R, s, S) policy (Scarf, 1959) and for continuous review it is the (r, Q) policy (Zheng, 1992). The periodic review policy includes four replenishment methods as described in Table 5.

Table 5.

Different replenishment methods, adopted from Landry and Beaulieu (2010).

Replenishment method	Description
Requisition	Regular counting and replenishment operations in the POUs are done, usually by clinical staff. After identifying the supplies that need to be replenished, they send a requisition form to the CS.
Exchange cart	In the POU locations, each supply is stocked in a cart. The carts are exchanged with fully stocked carts in the periodic review. This method decreases clinical staff involvement. The main problem with such a method is that extra supplies are stocked since a cart must be ready to be delivered to the units in the next period (Taylor, 1990).
PAR level	In the periodic review, the quantity of each supply is counted to generate a picking list with the quantities that bring inventories up-to-level S. The main concern with this system is that it needs the information of how much is on the shelf and often requires two trips for replenishment (Taylor, 1990).
Two-bin (no-count, Kanban)	The quantity of each supply that is required to be stocked in POUs is evenly distributed between two separate bins (or two compartments within a bin). The clinical staff consumes from the front bin until it is completely exhausted. The empty bin is placed on top of the shelf to trigger replenishment in the next review period. This procedure can be more automated by virtue of advanced technology like bar code and Radio-Frequency Identification (RFID). The staff then pull the second bin to the front for consumption.

In some papers, the term “PAR level” is used instead of “up-to-level” (eg, Landry & Beaulieu, 2010; Nicholson, Vakharia, & Selcuk Erenguc, 2004; Rosales, 2011; Rosales, Magazine, & Rao, 2014). In the rest of this article, these two terms are considered to be equivalent.

Regardless of the policy chosen, inventory parameters for surgical items – particularly PAR level – are often set by personal experience rather than engineering judgment (Rappold et al., 2011). Dubey (1991) and Veral and Rosen (2001) show how experience-based decisions yielded overstocking.

3.2. Sterile instruments

Inventory management of sterile instruments is different from that of surgical supplies. Surgical instruments are grouped into containers that are referred to as trays (some researchers named the containers as kits) and since they are reused, instruments typically go through the process illustrated in Figure 3.

Figure 3.

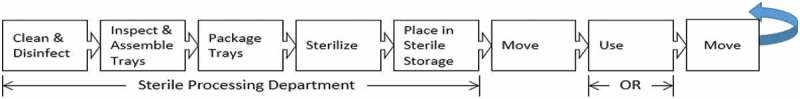
Sterile instrument cycle within a hospital. Adopted from (Reymondon, Pellet, & Marcon, 2008).

Once a tray is opened in the OR, all items in the tray must go through every step in the sterile processing department (SPD), even if an item was not used. A single procedure might require multiple tray types and each tray type might be requested for more than one procedure. Management of sterile instruments requires answering three questions: (1) which instruments and in what quantity should be placed in each tray type, (2) which tray type should be used for a given surgeon and procedure, and (3) how many of each tray type should be stocked in inventory. The two former questions are driven by the surgeon preference cards, while the latter question depends on the frequency and scheduling of the cases. In the literature, this problem is known as the tray optimisation problem (TOP) (van der Kooij & Glorie, 2015).

In the following sections, the publications are classified into two categories: papers that incorporated optimisation methods, with subcategories of surgical supplies and sterile instruments, and practitioner studies in cost/waste reduction related to OR inventories.

4. Literature review of papers containing optimisation techniques

In this section, the publications that applied optimisation methods for management of surgical supplies and management of sterile instruments are discussed.

4.1. Inventory management of surgical supplies

A very basic inventory control approach is ABC classification, where the classification is based on the cost of the supply. More attention should be paid to the A class that absorbs a high portion of the budget (70%) but accounts for a low percentage of the total items (10%). Around 20% of items fall in group B, which consumes 20% of the budget. The remaining 70% of items are Group C and they absorb 10% of the budget (Gupta, Gupta, Jain, & Garg, 2007). In conjunction with the ABC analysis, Gupta et al. (2007) propose VED analysis, which relies on the criticality of the items. “V” stands for the vital items that the function of a hospital highly depends on. “E” stands for essential items; the quality of the service depends on this group. “D” indicates desirable items that do not inhibit a hospital’s operation if they are not available. The item classification is then extended by Al-Qatawneh and Hafeez (2011) such that in addition to the cost and criticality, usage frequency is taken into account.

Given the most important items, extracted using the above-discussed methods, inventory models need to be established to decide on the inventory control parameters for these items. We have classified the optimisation problems of inventory management in hospitals in three major categories: (1) the global inventory comprises the papers that only address inventory management in the CS; (2) the local inventory consists of research that investigates methods applicable to department storerooms or POUs; and (3) the papers that consider both local and global inventories.

4.1.1. Global inventory

Two relevant studies have been found in the category of global inventory (see Table 6)

Table 6.

Global inventory models for a healthcare setting.

Study	Stochastic/deterministic	Method	Objective (cost component)	Decision	Stationary/non-stationary	Constraint	Type of good
Fineman and Kapadia (1978)	Deterministic	EOQ	Purchase cost, order cost, holding cost	Replenishment amount	Stationary	-	Sterile supplies
Dellaert and van de Poel (1996)	Stochastic	Simple rules	Holding cost, ordering cost	s (reorder point), c (can-order), S (up-to-level)	Stationary	-	Not specified

Fineman and Kapadia (1978) are the first to study a closed-loop chain known as the “sterilisation processing cycle”. This process involves receiving contaminated medical devices used to perform a surgical procedure, then cleaning, inspecting, packaging, and storing the grouped items. The authors divide sterile stock into two categories: the processing stock and the replacement stock. The first one is required to support the processing cycle described above and the second one is required to replace items that are lost, damaged, or worn out. In analysing the two categories, demand is assumed to be constant, which simplifies the problem significantly. Thus, they use an EOQ model to determine the inventory requirements for replacement stock.

Dellaert and van de Poel (1996) address the global inventories in a hospital which follow the (R, s, c, S) inventory control policy with stochastic demand. In the (R, s, c, S) model, if the inventory level for an item of a supplier (in R review cycle) goes below the reorder point (s), all other items of this supplier that are below the can-order (c) level are also ordered to increase the inventory to the up-to-level (S). They propose a simple rule for using a given R to calculate s, c, and S in an intuitive way with the aim of minimising total cost, including holding cost and ordering cost. In their evaluation, demands follow the Poisson distribution with normally distributed transaction size.

These early studies examine very classical inventory control models, which rely on assumptions that highly simplify the problem. Therefore, the provided solutions are far from practical and can only be considered as a general rule of thumb.

4.1.2. Local inventory

As can be seen in Table 7, seven research papers are dedicated to inventory models for the POU locations.

Table 7.

Local inventory models for a healthcare setting.

Study	Stochastic/deterministic	Method	Objective (cost component)	Decision	Stationary/non-stationary	Constraint	Type of good
Burns, Cote, and Tucker (2001)	Deterministic	EOQ	Order cost, holding cost	Order quantity	Stationary	-	Injectable supplies
Little and Coughlan (2008)	Stochastic	Constraint programing	Service level	Delivery cycle, service level	Stationary	Space	Sterile items
van de Klundert, Muls, and Schadd (2008)	Deterministic	Integer programming	Delivery cost, storage cost	Delivery time	Stationary	Space	Sterile instrument
Bijvank and Vis (2012)	Stochastic	Markov chain	Minimising the capacity, maximising service level	Reorder point and order quantity	Stationary	Service level, capacity	Disposable products
Rosales et al. (2014)	Stochastic	Simulation	Order cost	Reorder point, order quantity, order up-to level	Stationary	-	Not specified
Rosales, Magazine, and Rao (2015)	Stochastic	Semi-Markov process	Stock-out cost, replenishment cost	Review cycle, number of empty bins to trigger replenishment	Stationary	Inventory balance	Not specified
Diamant, Milner, Quereshy, and Xu (2017)	Stochastic	Markov chain	Stock-out cost	Inventory level for each instrument kit	Stationary	Service level	Sterile instrument

An application of ABC inventory analysis to the injectable supplies in a care centre along with a classical EOQ model is demonstrated by D. M. Burns et al. (2001). However, such a model accounts only for the cost without considering other important elements in healthcare such as storage space, demand variability, service level, etc. Because the main goal of any healthcare organisation is to provide high-quality patient care, any effort for inventory cost reduction should not compromise the quality of care. In the context of healthcare inventory management, not having the supplies in stock when needed indeed has serious impact on the quality of care (Moons, Waeyenbergh, & Pintelon, 2018), which might lead to loss of life (Guerrero, Yeung, & Guéret, 2013). Measuring impact of such an inventory shortage on patients is difficult, if not impossible. Therefore, the occurrence of a shortage can be preventable by introducing service level as a constraint (Bijvank & Vis, 2012; Diamant et al., 2017; Guerrero et al., 2013; Nicholson et al., 2004) or an objective function (Little & Coughlan, 2008). Service level is usually defined as the fraction of the demand that is satisfied by on-hand inventory, without substitution or emergency delivery (Bijvank & Vis, 2012).

In this context, a general multi-product, multi-period optimisation model is developed by Little and Coughlan (2008), in which the CS requires delivery of a variety of items to different departments such as the operating theatre or laboratory. Constraint programming is utilised to determine the number of units of each item that needs to be stocked in the POUs, the frequency of delivery, and the best service level subject to the limited space. In their model, a range of desired service levels and delivery frequencies for each item is specified by the user and the model is validated by sterile and bulk items in an intensive care unit within a hospital in Ireland. This model is further developed by Bijvank and Vis (2012) with the consideration of both service requirements and the capacity limitation. The authors provide a capacity model with the objective of maximising the service-level subject to the limited capacity. They also examine a service model with the objective of minimising the required capacity by considering the service level as a constraint.

van de Klundert et al. (2008) address managing of reusable instrument kits to improve their flow between the central sterilisation locations and ORs. They determine the optimum delivery time with the objective of minimising the delivery cost and the storage cost. The storage capacity at the ORs and the capacity of the transportation vehicle are restricted. Since they consider deterministic demand, no stock-out cost is taken into account. However, they suggest keeping safety stock and proposed four replenishment policies to cover the shortage caused by the variation in demand. Diamant et al. (2017) further address the problem of managing reusable instrument kits by considering the stochastic daily demand for instruments. They focus on determining the number of instrument kits that need to be stocked to maintain high service levels. Their model does not deal with the problem of kit configuration (ie, the required instruments to be included in each kit). Instead, the optimal inventory level for each instrument kit, given the predetermined composition of kits is provided.

Emerging advanced identification technologies such as automated dispensing machines (ADM), barcode, and RFID have encouraged researchers to investigate hybrid replenishment policies. Rosales et al. (2014) describe a hybrid model for a single item where inventories in the POUs were replenished periodically according to the (s, S) policy at the beginning of the shift. However, between two consecutive periods, whenever the inventory level reaches a threshold R, an out-of-cycle replenishment would be triggered with the size of Q (ie, a continuous (R, Q) policy). Their results show that the hybrid policy is better than pure periodic review or continuous review policy in terms of the cost, inventory and reduction in the number of replenishments. The single item model is then extended by Rosales et al. (2015) to a multi-item one. In addition they propose a methodology to compare two inventory systems in POU locations: a two-bin system, which is a periodic review policy and is widely used in POU locations, and a bin-level RFID-enabled tag, which is a continuous review policy in the bin level. In the two-bin system, they try to find the optimal value of the reviewing cycle, called parameter optimisation, and the bin-level RFID system aims to find the optimal number of empty bins to trigger a replenishment. They compare the performance of the two policies, called policy optimisation, in terms of the cost per unit time. The objective function minimises the stock-out cost and replenishment cost with the assumption of a fixed size for the bins. Unlike the previously discussed studies, Rosales et al. (2015) directly measure the stock-out cost by estimating the time spent by nurses to request and receive the required items. The implication of such a stock-out on quality of care, however, has not been taken into account.

4.1.3. Global and local inventory

In hospitals, the inventory decisions at downstream locations of the internal supply chain (ie, point-of-use locations) are connected to the inventory decisions at upstream locations (ie, central storage) and vice versa. Therefore, an integrated approach of local and global inventory optimisation models is necessary to reach a more practical model. A summary of the publications containing the integrated approach along with their specifications is presented in Table 8.

Table 8.

Integrated approach of local and global inventory optimisation models for a healthcare setting.

Study	Stochastic/deterministic	Method	Objective (cost component)	Decision	Stationary/non-stationary	Constraint	Type of good
Nicholson et al. (2004)	Stochastic	Mathematical programing	Holding cost, stock-out cost	Par-level (up-to-level)	Stationary	Service level	Not specified
Hammami, Ruis, Ladet, and Hadj Alouane (2006)	Deterministic	Linear and non-linear programming	Handling cost, holding cost	Period and the quantity of replenishment	Stationary	Space	Surgical supplies
Lapierre and Ruiz (2007)	Deterministic	Linear Programming	Holding cost	Period and the quantity of replenishment	Stationary	Space, human resource	Not specified
Rappold et al. (2011)	Stochastic	Linear programing	Holding and Shortage (backorder) cost	Purchase quantity, number of prepared items (kit), transferring unprepared and prepared amount to the OT	Non-stationary	Availability of stock in CS	Surgical supplies, surgical kit
Guerrero et al. (2013)	Stochastic	Markov chain	Holding cost in CS and POUs	s and S for central warehouse and POUs	Stationary	Service level, ordered quantity by CS, Storage capacity	Infusion solutions
Wang, Cheng, Tseng, and Liu (2015)	Stochastic	System dynamic	Inventory cost	Replenishment quantity, safety stock	Stationary	-	Not specified

The procurement department in hospitals has to make scheduling decisions in terms of when and how often each point-of-use should be visited for replenishment. These decisions would indeed affect the staffing decisions (eg, how many workers are required and when they should work). Lapierre and Ruiz (2007) consider a scheduling approach to address a multi-product, multi-period, two-echelon internal supply chain system where the CS purchases supplies from external suppliers and is responsible for delivering the required amount to the POUs. In addition to the primary objective of minimising the total inventory (holding) cost, limited availability of human resources led them to define a secondary objective of balancing the workload over the weekdays. The model decides when the POUs should be visited and how much of each product is delivered to the POUs.

Despite the papers discussed in the previous section that defined the service level as the percentage of demand coverage, Lapierre and Ruiz (2007) describe the service level as the frequency of visits to POUs. They assume that minimisation of the inventory cost would force the model to increase the service level. Guerrero et al. (2013) use a constraint to provide a minimum service level (ie, probability of avoiding stock-out in a given period) in a stochastic, multi-product, two-echelon (s, S) inventory control system. In their model, a central warehouse receives infusion solutions from the external suppliers and distributes them to the POU locations in different hospitals that all belong to the hospital’s network. Wang et al. (2015) incorporate a system dynamic approach, in which a set of decisions is changed in response to changing of the input information, to minimise the inventory cost without occurrence of stock-out.

Nicholson et al. (2004) go beyond the internal supply chain for addressing inventory management in hospitals. They consider a healthcare provider network in which a central warehouse, owned by the provider, receives supplies and distributes to the hospitals inside the network. Each hospital has its own central storage and distributes stock to its departments. The authors formulate two models. The first model is a three-echelon system containing a central warehouse, a central storage room in each hospital and POU locations in the departments. The second model contains a central warehouse and POU locations with no central storage room, in which the distribution of the non-critical items are outsourced to a third party. They conclude that outsourcing will reduce the inventory cost without having a negative impact on the quality of services. This finding is consistent with the benefits of outsourcing some logistics activities reported in the literature (Beaulieu, Roy, & Landry, 2018).

Hammami et al. (2006) consider a classical (R, Q) inventory model, as well as a supply chain approach for surgical supplies in a system where supplies are stocked in ORs, block warehouses (Cores) and CSs. However, they simplify the model by excluding the ORs from investigation because the inventory level in the ORs is highly dependent on surgeons’ estimates of need. This is due to the fact that patient condition may unexpectedly change during their stay in the hospital, and consequently induce unplanned requisition for some supplies. Modelling the system in this way (ie, removing ORs from the model) would over-simplify the problem, which leads to formulating an unpractical model. Vila-Parrish, Ivy, and King (2008) describe an inventory model as a Markov decision process to manage perishable drugs by considering the possible changes in the patient condition. Although addressing perishable products goes beyond the scope of our review, incorporating patient condition in the study of Vila-Parrish et al. (2008) is an interesting issue. In their model, patients are classified into N types. Each patient type has an associated profile of prescription drug usage (which resembles a BOM). They assume that patient condition (type) changes stochastically overtime. In the event of a stock-out, demand would be satisfied from another location (eg, other hospitals).

Some research has incorporated the concept of the Material Requirements Planning (MRP) and Manufacturing Resource Planning (MRP II) to address the material planning problem in hospitals. The backbone of MRP relies on the Master Production Schedule (MPS) and the Bill of Materials (BOM). Stevenson (2018) defines the MPS as “which end items are to be produced, when these are needed, and in what quantities” (p. 502) and the BOM as “a listing of all of the raw materials, parts, subassemblies, and assemblies needed to produce one unit of a product” (p. 503). In the healthcare context, the master surgery schedule (MSS) can serve as the MPS (Roth & Van Dierdonck, 1995). The BOM can be created through a system called diagnostic-related groups (DRGs). DRG classifies patients into clinically similar groups often based on the similarity of the procedure (or a group of procedures) and their ages (Roth & Van Dierdonck, 1995). Patients in the same group require similar treatment and therefore a similar BOM. Showalter (1987) is the first one who used the MRP concept for material management in hospitals. Roth and Van Dierdonck (1995) discuss that the traditional MRP has shortcomings when applied in hospitals. They develop a control system called Hospital Resource Planning (HRP) based on the MRP II concept in a deterministic condition. Van Merode, Groothuis, and Hasman (2004) suggest using Enterprise Resource Planning (ERP) (ie, the next generation of MRP II), in planning and controlling a hospital’s deterministic processes.

The flow of surgical supplies and challenges in the ORs are referenced by Rappold et al. (2011). They utilise MRP to address the material planning problem in the OR and discuss that the MSS, and consequently the scheduled procedures, are usually known weeks in advance. In this context, they consider two types of uncertainty in the OR. A stochastic number of surgical procedures performed in a day (resulting in a stochastic number of surgical cases) and a stochastic BOM (SBOM) (resulting in a stochastic usage of supplies). The source of the SBOM is the surgeon “preference card”. Although it is determined by the surgeon, the actual usage amount would be different case by case depending on the condition of the patient during the procedure, even for a given surgeon and specific procedure. The authors take uncertainties into account and formulate a model that provides an optimal purchase quantity from the supplier, as well as transferred unprepared and prepared (kitted) quantity to the Cores subject to the available stock in the CS. Finally, they quantify and evaluate the impact of information sharing between the surgical scheduling department and the material management department (to decrease schedule uncertainty), as well as the consequence of BOM standardisation (to decrease BOM uncertainty) among the physicians by varying their corresponding Variance-to-Mean Ratio (VTMR).

One important aspect of inventory control models, especially in the highly uncertain environment of healthcare, is how the models address the uncertainty involved in the system. In the supply chain context, there are two main sources of uncertainty, which can result in undesirable system performance, eg, shortage of required supplies and shortage of capacity. The first source, which is called disruption risk, is caused by the occurrence of natural disasters such as earthquakes, floods, epidemic diseases, environmental crises, and other sources of loss. The second source, operational risk, is caused by the intrinsic uncertainties of supply chain parameters such as uncertainty in demand, transportation time and cost, and lead time (Farrokh, Azar, Jandaghi, & Ahmadi, 2017; Tang, 2006).

Prior research that incorporates stochastic models (discussed in Sections ‎4.1.1, ‎4.1.2, and ‎4.1.3) does not clearly specify which sources of uncertainty were considered. According to the formulations, which often fitted a probability distribution to the historical data to model demand structure, we conclude that these prior studies just dealt with the operational risk. However, Tang (2006) discusses that the impact of the disruption risk on the supply chain is greater than the operational risk. The scope of this article does not review the different methods that are applicable to deal with each type of uncertainty. To read more details about the methods of stochastic programming and the papers that dealt with either operational risk or disruption risk in a general supply chain, one can refer to the review paper by Govindan, Fattahi, and Keyvanshokooh (2017) and for a method to cope with the hybrid uncertainty (both operational and disruption risks), we refer readers to Farrokh et al. (2017).

4.2. Inventory management of sterile instruments

Farrokhi, Gunther, Williams, and Blackmore (2015) show that appropriate configuration of surgical trays, which contain predefined groups of instruments, offers opportunities for substantial cost savings. Optimum surgical tray configuration leads to decreasing OR time (so more cases can be scheduled), decreasing instrument reprocessing costs and decreasing unnecessary wear and tear of instruments, which provides potentially millions of dollars of cost savings per year (Weiss et al., 2016).

Although the potential cost savings in the area of managing sterile instruments is highlighted by many studies (see the next section for a detailed review of these studies), tray configuration is less investigated in the literature. There are only five research papers that proposed optimisation methods for the tray optimisation problem or the similar problem of packaging of surgical supplies. A summary of these papers is reported in Table 9.

Table 9.

Literature overview of the tray configuration problem and packaging of supplies.

				Decision
Study	Stochastic/deterministic	Solution method	Objective (cost component)	Number of trays/packs required for each surgical case	Type of instruments within each tray	Number of instruments within each tray	Constraint
O’Neill, Murphy, Gray, and Stoner (2001)	Deterministic	Exact	Holding cost and reprocessing (packing) cost	✓			Demand coverage
van de Klundert et al. (2008)	Deterministic	Not provided	Storage and usage costs	✓	✓		Demand coverage
Reymondon et al. (2008)	Deterministic	Heuristic	Storage and reprocessing costs	✓	✓	✓	Demand coverage
Dobson, Seidmann, Tilson, and Froix (2015)	Deterministic	Heuristic	Holding and reprocessing costs	✓	✓		Demand coverage
Cardoen et al. (2015)	Deterministic	Exact and heuristic	Point of touch	✓	✓	✓	Demand coverage, budget

van de Klundert et al. (2008) design an integer linear programming model for TOP and prove that TOP is NP-hard in the strong sense. Reymondon et al. (2008) propose a mathematical model with non-linear constraints to deal with the TOP. Their model determines the number of trays required for each surgical case, as well as the type and number of instruments within each package, with the aim of minimising storage and reprocessing costs. They also discuss the computational challenges of their model when large instances of the problem are considered. They report that it takes 30 minutes finding a near-optimum solution for an instance that comprises 15 surgical cases and 200 instruments. Dobson et al. (2015) consider the same problem with a different formulation and propose a heuristic to obtain a near-optimal solution in considerably less time.

Preparing a custom pack for disposable/reusable supplies (ie, grouping surgical supplies into predefined packages, to be used for performing the procedure in the operating room) is a problem with some similarities to the surgical tray configuration problem. On one hand, custom packs reduce the material handling effort, which directly decreases the risk that an individual product becomes contaminated (Cardoen et al., 2015). On the other hand, this may increase the wasted amount if a pack becomes contaminated (eg, due to the handling) (Gellman, 1988). Un-optimised custom packs may also increase the wasted amount, in terms of the number required during the procedure (Akridge, 2005; Reis & Fontaine, 1993). In order to help a hospital in configuring their custom packs, Cardoen et al. (2015) develop a mathematical programing approach with the objective of minimising the points-of-touch, which is a measure defined as the number of physical contacts between staff and medical materials. Machline (2008) adjusts the classical inventory models of determining reorder point and inventory reviewing period for an inventory system that has implemented custom packs.

Surgical linen (known as green linen) is required to be packed and stocked before performing a procedure. In order to determine the number of packs needed in each period, O’Neill et al. (2001) formulate a linear programme to minimise holding cost and reprocessing (packing) cost. They evaluate two scenarios: packing the required amount in each period and packing a constant amount. They conclude that the latter approach would lead to lower cost than the former.

In all the above papers, it is assumed that the number of items and instruments required to perform a procedure is known in advance, which is not the case in practise. Moreover, safety of preoperative personnel in carrying and lifting of trays has not been taken into account. In an effort to keep cost down, surgical trays may end up being inappropriately prepared and may be too heavy to lift or carry safely (Nelson, Waters, Spratt, Petersen, & Hughes, 2007). In the preoperative environment, staff are frequently required to carry heavy sets or trays of sterile instruments, which puts them at risk for developing work-related musculoskeletal disorders (WRMSDs) (Garb & Dockery, 1995; Ogg, 2011). According to the Association of periOperative Registered Nurses (AORN), lifting and carrying supplies and equipment is one of the seven tasks related to ORs which is classified as a high-risk task (Nelson et al., 2007). Seavey (2013) also listed the weight of the trays as one of the main issues in the SPD and OR. Attempts to optimise tray configuration may exacerbate the problem of overweight trays, so a method should consider weight as a constraint in order to be practical.

5. Literature review of practitioner reports about OR cost/waste reduction

Some practitioners report case studies of improvement projects implemented in ORs with the aim of increasing the availability of supplies and instruments for scheduled surgery, tracking of supplies through the network, and quantifying the usage/waste of surgical supplies and sterile instruments. They do not present optimisation methods, but rather they compare their results before and after implementing a new technology/scenario, and also provide some general recommendations to improve inventory control of surgical supplies. A summary of these papers with respect to three criteria is chronologically presented in Table 10.

Table 10.

Practitioner reports about cost/waste reduction in ORs.

Category	References
Descriptive recommendation for inventory management (eg, preference cards improvement)	Young and Conley (1988), Melson and Schultz (1989), Nilsen (2005), Bilyk (2008), Park and Dickerson (2009), Ryan, Lewis, Doster, and Daily (2014), Goh, Tan, and Leong (2016), Hall (2016), Putnam (2016)
Quantified usage/waste of surgical supplies	Williams (2007), Conrardy, Hillanbrand, Myers, and Nussbaum (2010), Stall, Kagoma, Bondy, and Naudie (2013), Eiferman, Bhakta, and Khan (2015), Zygourakis et al. (2016), Harvey, Smith, and Curlin (2017b)
Quantified usage/waste of sterile instruments	Stockert and Langerman (2014), Mhlaba, Stockert, Coronel, and Langerman (2015), Farrokhi et al. (2015), Harvey et al. (2017a), Koyle et al. (2017), Harvey et al. (2017b)

In the following subsections, the practitioners’ studies are reviewed in more depth.

5.1. Review of descriptive reports

Although optimal inventory solutions (eg, item choice, quantity, location, etc.) are found in many studies, effective strategies for implementing changes without sacrificing quality of care or causing errors has been neglected. In this regard, Young and Conley (1988) and Nilsen (2005) suggest a framework to improve the inventory control system in the OR. The approach contains four steps: (1) identifying the current inventory; the items that are operationally important should be tracked accurately; (2) mapping the current flow of material; using the flow charts is an appropriate way to understand deviation from the previously established standard; (3) identifying a method of managing items; a group of employees from different departments should work together to establish a method that works better for managing equipment; and (4) analysing and validating the various inventory levels with the actual usage. In line with the above mentioned steps, Bilyk (2008) adds another step as educating all stakeholders (ie, management, physicians, and clinical staff) about the importance of supply chain management. Ryan et al. (2014) evaluate that over 50% of a hospital’s inventory assets are related to the surgical supplies and instruments. As a general rule of thumb, driven from the descriptive analysis, it is recommended that no more than 20% of inventory be stocked in the OR and sterile storage room (Core) (Melson & Schultz, 1989). However, no analytical method is employed to reach such a conclusion.

One of the most important prerequisites for improving management of surgical supplies and instruments, which is highlighted by practitioners (Goh et al., 2016; Park & Dickerson, 2009) and academics (Rosales et al., 2014; Yazici, 2014), is that the healthcare industry utilises information technology systems to collect data and share information. Using data capture technologies (eg, barcode and RFID) enables hospitals to benefit from perpetual inventory system by reducing delays in delivery to the OR, reducing labour costs in restocking unused items, sharing information between involved departments, and reducing inventory levels. In addition, through implementation of such a system, items’ utilisation patterns on the preference cards can be captured. Therefore, frequently used items can be identified to share with surgeons for standardisation purposes (Park & Dickerson, 2009). However, using barcode and RFID technologies have their own limitations. Barcode requires personnel time for scanning items and also manual cycle count should be performed to capture supply usage and match the inventory level in the system with physical on-hand inventory. RFID tags and readers can be a substitute for the labour-intensive barcode technology, but it is indeed capital-intensive and requires high investment in technology infrastructure (Moons et al., 2018).

One of the issues raised from the lack of information technology, especially an inventory management system, is that clinical staff are unaware about the cost of items (Hall, 2016; Putnam, 2016). Informing surgeons and nurses about the price of items not only enables them to decide to open it immediately or hold it until it is actually required, but they can also think about alternative products with a lower cost and the same quality. Another step towards cost reduction is providing the surgeon with the information of the average cost of a procedure when performed by other surgeons. Having access to this information, surgeons start to communicate with each other about their techniques to learn about less costly options. This step would initiate standardisation of the preference card as well (Putnam, 2016).

5.2. Review of quantified studies about usage/waste of surgical supplies

Weiss et al. (2016) provide extensive statistics about different types of waste in ORs. A major source of supply waste in ORs is the items that are requested by surgeons to be opened on the sterile field before the procedure, but are not used during the procedure. This group of items are referred to as “overage” (Rosenblatt, Chavez, Tenney, & Silverman, 1997). Zygourakis et al. (2016) identify the costs and the quantities of overage for 58 neurosurgical cases. They observe that the average cost of unused supplies is $653 (ranging from $89 to $3640), which accounts for 13.1% of the total surgical supply cost. They estimate that there is approximately $2.9 million of OR waste per year for the neurosurgical department alone at a single hospital.

Collaboration with physicians for preference card standardisation, without a doubt, is the most effective way towards cost reduction in the ORs. It has been shown by Williams (2007) that a close collaboration with physicians in the Medical Center of Central Georgia (just focusing on Cardiology Medical Supplies) and St. Tammany Parish Hospital (focusing on Cardiac Cath Lab Supplies) can save $1.7 million and $987,000 in a year, respectively. Another project of preference card standardisation performed by Eiferman et al. (2015) in a hospital in the USA results in a cost savings of $893,865 in nine months by standardising three different surgical supplies (cranial plating systems, biologic mesh, and neurostimulators). In a recent study, Harvey et al. (2017b) examine five frequently performed procedures across 21 surgeons to reduce the cost associated with disposable supplies and sterile instruments in the preference cards. Their collaboration with surgeons in revising 48 preference cards yields $16 in cost reduction per card, on average, for only disposable supplies. This savings is realised every time the procedures are performed. They also report a cost savings of about $925 over a three-month period in reprocessing of the sterile instruments that were eliminated from the trays.

One strategy for reducing waste in the OR is to replace the reusable items for disposable ones. Conrardy et al. (2010) carry out a project to compare potential cost saving of using reusable surgical linens (eg, gowns and drapes) instead of disposable ones and report about a 65% reduction in the regular medical waste. In addition, using reusable products has environmental benefits over disposable products (Overcash, 2012). However, some studies show conflicting results about the financial and environmental benefits of reusable products compared to the disposable ones (Stall et al., 2013). The reason for this is rooted in the fact that the studies were specific to the institutions considered and cannot be extrapolated to other institutions. Therefore, such a strategic decision requires further research before deciding whether to implement a reusable-based surgical inventory system or a disposable-based one.

5.3. Review of quantified studies about usage/waste of sterile instruments

As discussed in Section ‎3, inventory management of sterile instruments is different than the management of disposable surgical supplies. A series of studies has recently been conducted to make observations of surgical procedures and quantify the utilisation rate of the trays and potential cost savings through tray standardisation. Stockert and Langerman (2014) reveal that the percent of instruments in a tray that are used across four busy surgical services (Otolaryngology, Plastic Surgery, Bariatric Surgery, and Neurosurgery) is on average 17.1%, ranging from 13.0% to 21.9%. They conclude that improvement of tray configuration may result in immediate and significant cost savings. In another study, Mhlaba et al. (2015) quantify instrument utilisation and the cost associated with processing the instruments (ie, decontamination and packing). They observe that the average percent of utilisation is 14% and 29% for the Plastic Soft Tissue tray and Major Laparotomy tray, respectively. They estimate $29,900 per year savings by eliminating those instruments that were not used in the Plastic Soft Tissue tray alone.

Farrokhi et al. (2015) incorporate lean methodology to improve management of sterile instruments and report about 70% reduction in the number of instruments for the two procedures studied, which results in approximately $2.8 million cost savings in a year. Harvey et al. (2017a) observe five surgical trays for two procedures of Female Pelvic Medicine and Reconstructive Surgery and Minimally Invasive Surgery and report annual cost savings of $151,691 for eliminating unused instruments within associated surgical trays. In another recent observation of Pediatric Inguinal Hernia Repair for 56 cases, 86 instruments from a total of 147 instruments included in two trays, are never used (Koyle et al., 2017).

Although improvement in the management of surgical trays, which is underlined by both practitioners and academics, offers many benefits in cost reduction, its adoption is still not fully explored. Each of the discussed papers proposed a tailor-designed methodology and reported different ranges of cost containment. However, surgeon’s satisfaction and quality of care received by patients, as well as safety of preoperative nurses and technicians (ie, ergonomic injuries associated with frequently lifting and handling of surgical trays) are important aspects that should be taken into account to form a practical model.

6. Conclusion

In this article, a comprehensive review of publications on inventory management of surgical supplies and sterile instruments is presented. The publications are divided into two categories: the papers that proposed optimisation methods and the papers that contained practitioner reports about the reduction of cost or waste of supplies and instruments in the OR. The identified manuscripts are discussed from different perspectives such as costs, service levels, limited storage area, limited human resources, surgeon satisfaction, and lack of information.

Preference card optimisation (ie, the items and their quantities) is a topic that has been untouched thus far in the research literature. Preference cards have a direct impact on the inventory control parameters as well as the amount of wasted supplies. Another question that remains unanswered in the literature is the location and the quantity of surgical supplies that must be stocked according to the OR’s specific process. For example, a portion of the supplies that are required to perform the procedures should be stocked in the CS to set-up the case cart, a portion should be stocked in the core storage, and some should be stocked within the ORs to be used for augmentation during the procedure. A further question that needs to be answered is what methodology can help physicians to decide the appropriate quantity to be opened before the procedure with the aim of minimising waste without sacrificing patients’ quality of care.

From the methodology and modelling perspectives, one of the obvious future research directions is to develop stochastic models. The assumption of deterministic demand or even stochastic stationary demand hinders the models in being practical. In addition, deployment of a model that just considers the inventory cost without accounting for service level is not applicable. Stochastic models can be developed by considering either operational risk, disruption risk, or a hybrid of operational and disruptions risks. The operational risk is caused by the uncertain patient condition, which may change during a surgical procedure (or stay in hospital) and consequently requires a different type and quantity of supplies. Surgeons’ preferences also play a significant role in the need for stocking a variety of items. Disruptions risk, on the other hand, occurs due to sources of supply loss or delay due to earthquakes, floods, epidemic diseases, or other sources of supply disruption, would indeed affect the inventory decisions parameters. Therefore, by considering risk, developed models will reflect real-world problems, which will be more applicable in practise.

Optimal surgical tray configuration is another potential topic for future research. Surgical trays need to be frequently handled and the weight of trays is a concern for staff safety. Moreover, the low utilisation rate of instruments in the trays is a signal for the necessity of tray optimisation. It needs to be ensured that the right instrument is available to the surgeon at the right time, while also reducing the cost associated with the surgical case. There is also a need to ensure that the trays meet the safety requirements for lifting and handling. Advanced clustering techniques and mathematical programming can be utilised to deal with the tray configuration with respect to staff safety, cost reduction and surgeon satisfaction.

In order to cover each of the above-mentioned research gaps, the availability of data plays a significant role in developing analytical methods. Utilising advanced technologies such as barcode and RFID can help with capturing information. Advanced technologies are referenced as capital-intensive, which require high investment in technology infrastructure. However, since these technologies are always advancing and becoming more cost-effective, research will need to be updated accordingly.

Disclosure statement

No potential conflict of interest was reported by the authors.