Evolution towards minimum ovule size? Ovule size variations and the relative sizes of ovules to seeds

Abstract

Background and Aims

Is there selection minimizing the costs of ovule production? Such selection should lead to a smaller ovule size in relation to seed size and, at the same time, smaller variation in ovule size within plants, the latter because the minimum structures and resources for functioning of ovules should be the same among ovules. Additionally, within species, ovule size should not depend on the plant’s resource status.

Methods

To confirm these predictions, we examined ovule and seed production for a variety of species.

Key Results

Among the 27 species studied, we found a significant negative dependence of the species mean of the coefficient of variation for plant ovule size on the ratio of the mean species seed size/mean species ovule size. Thus, the smaller the ovule size as compared with seed size, the smaller the degree of variation in ovule size. Among the 49 species studied, only two species showed significant positive dependence of mean ovule size on plant size. Although larger plants should have greater resources for ovule production, selection has not enhanced the production of large ovules in most species.

Conclusions

These results suggest that there is selection minimizing the costs of ovule production.

INTRODUCTION

Ovules are reproductive organs that develop into seeds. Although ovules are much smaller than seeds, a certain amount of resources is necessary to produce them. Hence, the strategies of ovule production, as well as those of seed production, should also be subject to natural selection.

Many studies (e.g. Kozlowski and Stearns, 1989; Burd, 1995; Sakai and Sakai, 1995; Sakai, 1996, 2007; Porcher and Lande, 2005; Burd et al., 2009; Sakai and Kojima, 2009) have examined the adaptive significance of the number of ovules produced. For example, ovules are generally overproduced, but not all ovules develop into mature seeds; this overproduction may exist to compensate for the losses of developing embryos (Porcher and Lande, 2005), to anticipate favourable pollination and/or resources for seed production (Kozlowski and Stearns, 1989; Burd, 1995, 2008; Griffin and Barrett, 2002; Burd et al., 2009), for the selective abortion of low-quality embryos (Kozlowski and Stearns, 1989; Korbecka et al., 2002) and/or for uniform seed production via the selection of fertilized ovules with similar resource absorption rates (Sakai, 2007).

On the other hand, considering ovule size, allocation to individual ovules should be minimized because of the uncertainty of ovule fertilization, and the resources allocated to the unfertilized ovules are wasted (Lloyd, 1980; Westoby and Rice, 1982; Greenway and Harder, 2007). However, Greenway and Harder (2007) found that variations within species exist in ovule size and the number of ovules per flower; within a species, the coefficient of variation (CV) of ovule volume ranges from 0.153 to 0.663, and the CV of the number of ovules in a flower ranges from 0.040 to 0.378. This suggests that ovule size has not evolved to an equally minimum size in many species. The authors further reported equivocal evidence that selection minimizes the cost of ovule production; among species, mean ovule size increases with mean flower size but decreases with the mean number of ovules in a flower (Greenway and Harder, 2007). These results also suggest that ovule size depends on flower resource allocation and ovule number, and hence, ovule size has not evolved to a universally minimum size across species.

However, it is possible that selection minimizing the costs of ovule production might exist in many species, if not in all species. Here, minimal ovule size may not necessarily have the same origin among species; it may vary due to structural and/or morphological limits and post-fertilization maturation time limits. Hence, such selection minimizing costs should lead to a smaller ovule size in relation to seed size and, at the same time, smaller ovule size variation within plants, the latter because the minimum structures and resources for functioning of ovules should be the same among ovules. Also, such minimum costs should not depend on ovule positions within flowers and within plants; minimum costs are the same irrespective of the differences in the availability of resources or the differences in successful fertilization probability. Hence, if minimum allocation to individual ovules has been realized, there should be no variation in sizes among ovules. Thus, (1) the smaller the ovules are in relation to seeds, the smaller the CV of ovule size. Seed size is accounted for because minimum ovule size might be larger if seed size is larger, due to the above constraints. Also, variation in ovule size is not advantageous if saving resources is a strong selection factor. Additionally, (2) within species [but not among species, as observed by Greenway and Harder (2007)], ovule size should not depend on plant resource status. In this paper, to detect selection minimizing the costs of ovule production, we examine whether the first relationship is observed among 27 species and the second relationships are observed within each of 49 species.

MATERIAL AND METHODS

Study sites and study species

This study was conducted in the natural habitats in Aobayama (38.258°N, 140.837°E) and Izumigatake (38.247°N, 140.4252°E), Miyagi prefecture, and Hakkoda (40.396°N, 140.526°E), Aomori prefecture, in the northern region of Honshu, Japan, during 2015–2018. Details regarding the studied species and their sampling sites are provided in Supplementary Data Table S1. We studied 49 animal-pollinated herbaceous species with hermaphroditic flowers from 29 families. We avoided examining plant species that produce very small ovules, such as Orchidaceae, because the individual sizes of these ovules are difficult to measure. We sampled plants from a single population for each species, selecting populations with >60 plants.

Measurements of ovule sizes

We collected fresh flowers (in which fresh pollen remained) to avoid possible changes in ovule sizes due to fertilization. For each species, we collected one or two flowers from ~30 plants. However, for species whose individual flowers produce single ovules, i.e. Agrimonia pilosa var. viscidul, Sanguisorba tenuifolia, Melilotus officinalis and Eupatorium glehnii, we sampled 3–5 flowers from each plant. We avoided plants growing close (within ~10 m) to each other to minimize the chances of studying genetically identical plants. The samples were collected from April 2015 to September 2017.

We determined the sizes of the sampled ovules of each species using one or both of the following two measurements (Supplementary Data Table S1): ovule area and ovule mass. Before the measurements, we counted the number of ovules of each sample flower under a stereomicroscope. We sampled all ovules of each sampled flower for most species, but for the species whose individual flowers produce hundreds of ovules, i.e. Helonias orientalis, Chamerion angustifolium, Mimulus sessilifolius, Gentiana triflora var. japonica and Schizocodon soldanelloides var. soldanelloides, we randomly sampled ten ovules from each flower. For the measurements of ovule area, ovules were carefully placed between clear plates so that their largest surfaces faced upwards, they were then scanned using a GT-S630 scanner (Seiko Epson, Tokyo Japan; 2400 pixel/inch) and individual ovule areas were measured using ImageJ imaging software (Schneider et al., 2012). For the measurements of ovule mass, the samples collected were dried in an oven at 80 ℃ for 3 d and then weighed. Because the dimensions differ between area (two dimensions) and mass (three dimensions), we used mass^2/3 values for ovule mass for further calculations.

Plant size measurements

We measured the size of each plant whose ovules were sampled. For most species, we measured the shoot length and/or the basal diameter of the shoot (Supplementary Data Table S1), and the length, diameter, or length × diameter² were used as an index of plant size. For Erythronium japonicum, in which each fertile plant produces two leaves, the lengths and widths of the two leaves were measured, and the sum of the length × width of the two leaves was used as an index of plant size. For Drosera rotundifolia, Helonias orientalis and Iris gracilipes, the length of the longest leaf of each plant was measured and used as an index of plant size.

Seed size measurements

We collected mature fruits from 27 of the 49 species (Supplementary Data Table S1) from May 2015 to July 2018. For those species whose individual fruits produce many seeds, we collected one mature fruit from ~30 plants whose flowers had not been sampled. As an exception, several fruits were sampled from each plant of Nephrophyllidium japonicum because we were able to collect only part of the seeds produced in each fruit. We also sampled 3–5 fruits from each plant in those species whose individual fruits produce single seeds. We avoided plants growing close to each other to minimize the chances of studying genetically identical plants.

We determined mean seed size (mean area or mass) of each species using the same method as used for the ovule measurements. For most species, mean seed sizes were calculated using all sampled seeds, but in several species, mean seed sizes were calculated using five or ten seeds from each fruit (Supplementary Data Table S1). We also used mass^2/3 values for seed mass for further calculations.

Analysis

The following data analyses were conducted using R 3.5.0 (R Core Team, 2018).

Dependence of ovule size variation on the relative size of the ovule to the seed.

We examined the dependence of ovule size variation on the relative size of the ovule to the size of the seed for the 27 species (Supplementary Data Table S1) whose seed sizes were measured. The method of phylogenetic generalized least squares (PGLS) was applied as follows.

First, we calculated the CV of ovule sizes for each plant [individual ovule sizes (ovule areas) were available for all 27 species], and the mean CV of the species was obtained. We also calculated the ratio of mean seed size/mean ovule size for each species. Here, we used either seed area/ovule area or seed mass^2/3/ovule mass^2/3 (Supplementary Data Table S1); we did not use seed mass^2/3/ovule area nor seed area/ovule mass^2/3 because accurate relative size cannot be obtained based on these ratios.

We then obtained the phylogenetic relationships of the 27 species using the online program Phylomatic (version 3) (Webb and Donoghue, 2005; http://www.phylodiversity.net/phylomatic). For the ‘megatree’ from which the phylogenetic information was extracted, we used a tree (R20120829 Phylomatic tree for plants; https://github.com/camwebb/tree-of-trees) derived from the Angiosperm Phylogeny Group III (The Angiosperm Phylogeny Group, 2009) implemented in the program Phylomatic. After obtaining the tree topology, branch lengths were estimated as previously described (Grafen, 1989) using the compute.brlen function in the ape package of R (Paradis et al., 2004).

For PGLS calculations, gls in the nlme package (Pinheiro et al., 2018) of R was used. In this analysis, the ratio of mean species seed size/mean species ovule size was the explanatory variable, and the species mean of the CV of plant ovule size was the response variable. The phylogenetic model for covariance was the Brownian motion model.

Dependence of ovule size on plant size.

For each of the 49 species (Supplementary Data Table S1), we examined the dependence of mean ovule size on plant size. The generalized linear model (McCullagh and Nelder, 1989) with a gamma distribution and an inverse link was applied to these analyses. The P values obtained were adjusted using the Holm method (Holm, 1979).

RESULTS

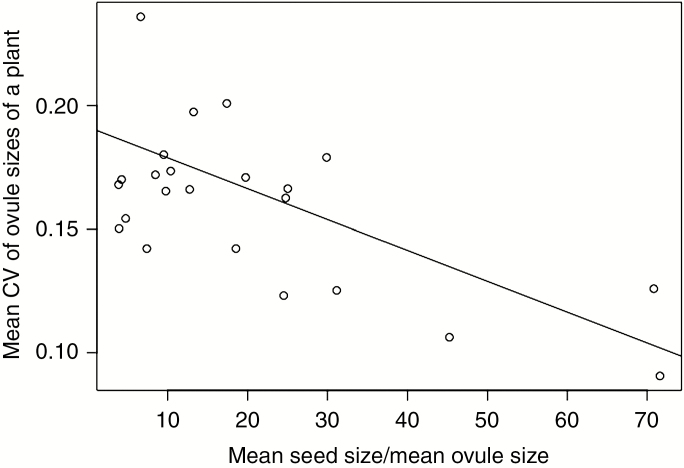
We found a significant negative dependence of the species mean of the CV of plant ovule sizes on the ratio of the mean species seed size/mean species ovule size (Fig. 1; estimate = −0.0012, s.e. = 0.0004, t = −3.0653 and P = 0.0059, obtained by PGLS). Thus, the smaller the ovule is compared to the seed, the smaller the degree of variation in ovule size.

Fig. 1.

The dependence of the species mean of the CV of plant ovule sizes on the ratio of the mean species seed size/mean species ovule size. The line shows the phylogenetic linear regression obtained by phylom in the phylom package of R.

There was no significant dependence of mean plant ovule size on plant size in 47 of the 49 species. Only two species (Agrimonia pilosa var. viscidula and Erythronium japonicum) showed significant positive dependence, and larger plants produced larger ovules in these two species (Table 1; full results for the 49 species studied are provided in Supplementary Data Table S2).

Table 1.

Species showing significant dependence of mean plant ovule size on plant size, obtained by generalized linear model analyses. In the models, a gamma distribution and an inverse link were used

Species	Estimate	Standard error	t values	Adjusted P values
Agrimonia pilosa var. viscidula	−18.6031	4.8273	−3.8537	0.0279
Erythronium japonicum	−1.3977	0.3823	−3.6558	0.0467

DISCUSSION

The present results suggest that there is a selection minimizing the costs of ovule production; in species producing small ovules relative to their seed size, the CV values of ovule sizes were also small (Fig. 1). Thus, small and uniform ovules have been selected for in these species. Additionally, larger plants did not produce larger ovules in most species examined (Table 1). Because larger plants should have greater resources for ovule production, they could increase their ovule size and/or number. Nevertheless, selection has not enhanced the production of large ovules. This result is consistent with the present hypothesis, although the production of ovules with uniform sizes is different from the production of ovules with minimum sizes. Thus, it is likely that some plants minimize allocation to individual ovules because of fertilization uncertainty.

Two species, Erythronium japonicum and Agrimonia pilosa var. viscidula, showed significant dependences of mean plant ovule size on plant size. Erythronium japonicum is a single-flowered species. In general, pollinator visits increase with flower size but with diminishing gains (e.g. Sakai and Sakai, 1995; Conner and Rush, 1996; Glaettli and Barrett, 2008). Because single-flowered species cannot enhance pollinator visits by increasing flower numbers, they may suffer a stronger diminishing gain of visits than multiflowered species at the plant level. Hence, as plant size increases, it is disadvantageous to proportionally increase ovule number because of the increasing pollen limitation, and hence plants increase ovule sizes using additional resources (Sakai and Sakai, 1995). On the other hand, plants of Agrimonia pilosa var. viscidula produce many small flowers with single ovules, and we suggest that parents can regulate the size and number of ovules at the whole plant level. Thus, parents seem to be able to produce ovules with a small uniform size, although it remains unclear why this species shows the above positive dependence.

The large variances in the species mean of the CV of plant ovule sizes and the ratio of the mean species seed size/mean species ovule size (Fig. 1) indicate that a minimum ovule size has not evolved in all species studied. In some species, other factors influence the evolution of ovule size. Greenway and Harder (2007) proposed that parent-–offspring conflict leads to ovule size variation, and strong parent–offspring conflict has in fact been reported (Cailleau et al., 2018). Ovules contain sporophytic tissues (integuments and nucellus), and these may control resource investment to the developing seeds (Westoby and Rice, 1982). Larger sporophytic tissues might have evolved to strongly control developing seeds, and the differences in the intensity of parent-–offspring conflict might be a causal factor in ovule size variation (Greenway and Harder, 2007). To examine whether this hypothesis is true, it is necessary to distinguish the size of the sporophytic tissues from other parts of the ovules and examine the dependence of the sporophytic tissue size on the intensity of the parent–offspring conflict. Species with high outcrossing rates, those with high mating diversity and/or those that produce many ovules may suffer strong parent–offspring conflict and produce ovules with large sporophytic tissues. Thus, it is possible that ovule size is determined by the relative importance of its minimization and of offspring control. On the other hand, it is also possible that post-fertilization maturation time can affect ovule size. This time varies among and within species; for example, it may be shorter if pollination occurs late in the growing season and for flowers produced later within inflorescences. Large ovules may be advantageous in these flowers so that ovules develop to seeds quickly. Effects of post-fertilization maturation time should also be included in future studies.

SUPPLEMENTARY DATA

Supplementary data are available online at https://academic.oup.com/aob and consist of the following. Table S1. Details of the studied species and their sampling sites. Table S2. Results of the generalized linear model analyses for the dependence of mean plant ovule size on plant size.