Comparison of Cardio-Ankle Vascular Index (CAVI) and CAVI₀ in Large Healthy and Hypertensive Populations

Abstract

Aim: The cardio-ankle vascular index (CAVI) represents the blood pressure-independent arterial stiffness from the origin of the aorta to the ankle. CAVI₀ has been proposed as a variant index. We aimed to clarify the difference between CAVI and CAVI₀ among large populations, and to explore reasons of the difference.

Methods: The subjects were 5,293 Japanese healthy and 3,338 hypertensive people. Simple and multiple regression analyses were performed using age, sex, body mass index, systolic, and diastolic blood pressure (Pd) as variables. Sub-group analysis was performed by sex and age. The CAVI values with and without adjustment by reference pressure were also compared.

Results: CAVI had a positive correlation with Pd, while CAVI₀ had a negative correlation with Pd in the healthy population. The CAVI values of the hypertensive group were higher than those of healthy group in both men and women, but the CAVI₀ values in women of the hypertensive group in the 30–39 age group was significantly lower than that of the corresponding healthy group. Differences of CAVI values with or without modification using the reference pressure were 1.09% ± 1.38% for the healthy group and 3.68% ± 1.66% for the hypertensive group.

Conclusion: CAVI showed the expected values, but CAVI₀ showed inexplicable results in the healthy and hypertensive populations. The differences were due to the strong dependency of CAVI₀ on Pd. Differences of CAVI values with or without reference pressure were negligible. These results indicate that CAVI obtained by the VaSera system is appropriate, but CAVI₀ is not.

See editorial vol. 26: 601–602

Introduction

It is known that arterial stiffness reflects arteriosclerosis and it is related to cardiovascular events. Measurements of arterial stiffness have been attempted from the late 19^th century¹⁾. It is established that the velocity of the pulse that arises from the heart is correlated with the stiffness of the artery²⁾. Consequently, pulse wave velocity (PWV) has been used as an index of arterial stiffness ³⁾. However, PWV essentially changes according to changes in blood pressure at the measuring time. This has been shown theoretically and also experimentally⁴⁾. Therefore, PWV is an inappropriate index for research studying the effect of blood pressure on arterial stiffness.

An attempt to establish an arterial stiffness index independent of blood pressure at measuring time was made by Hayashi et al.⁵⁾. They found that the relationship between a change in vascular diameter and internal blood pressure showed an exponential curve, using isolated arteries. Whereas, when blood pressure change was expressed as a natural logarithm, the relationship between diameter change and blood pressure change showed nearly a straight line. The inclination of this line, regarded as an arterial stiffness parameter which does not change according to changes in blood pressure at the measuring time, was defined as stiffness parameter β (Supplement Seq. 1)⁵⁾. Later, Kawasaki et al. showed a method to measure β using an ultrasonic diagnostic imaging apparatus by setting the diastolic pressure (Pd) in place of the reference pressure (Supplement Seq. 7)⁶⁾.

In 2004, the cardio–ankle vascular index (CAVI) was developed by applying the Bramwell–Hill equation to Kawasaki's β formula using PWV, as an index reflecting arterial stiffness of the arterial tree from the origin of the aorta to the ankle. The VaSera system was invented to measure CAVI. The precise methods were already published^{7, 8)} and the index was defined by eq. 1⁸⁾.

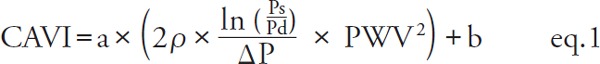[PWV: pulse wave velocity of the arterial tree from the origin of the aorta to the ankle, Ps: systolic blood pressure, Pd: diastolic blood pressure, ρ: blood density, Δ P: Ps–Pd, a, b: coefficients⁸⁾].

Using this index, many studies have been published over the last 15 years; these studies include various valuable findings confirmed by researchers throughout the world⁹⁾. Though both CAVI and PWV values increase with aging, the increase is more marked with CAVI¹⁰⁾. Further, CAVI is more closely correlated to aortic distensibility than PWV¹¹⁾. Patients with arteriosclerotic diseases, such as coronary arterial disease, cerebrovascular diseases, and chronic kidney disease show high CAVI values^12–14). Furthermore, CAVI is known to be high among those with coronary risk factors, such as hypertension^{15, 16)}, diabetes mellitus^{17, 18)}, dyslipidemia¹⁹⁾, and sleep apnea syndrome^{20, 21)}. Many studies have shown that CAVI is a predictor of cardiovascular events^22–25). In addition, several studies on the acute changes of CAVI showed reasonable changes as a result of the administration of metoprolol, doxazosin, and nitroglycerin^{8, 26)}.

However, recently, Spronck et al. proposed a similar index, termed CAVI₀, which is based on the assumption that CAVI is dependent on blood pressure, whereas, CAVI₀ is not. The calculation formula for CAVI₀ is defined in eq. 2 ^27–29).

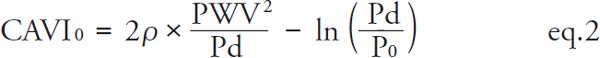[P₀: reference pressure (100 mmHg)]

According to Spronck et al., there are two reasons for the blood pressure dependence of CAVI. The first reason is that the slight difference between the β of Hayashi and that of Kawasaki et al. is expressed by the second term of the right side of eq. 2, which is related to the reference pressure, and this is the first element of blood pressure dependency. The second reason is the 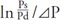of the CAVI expression, which is different from 1/Pd. Spronck et al. compared CAVI and CAVI₀ values based on mathematical simulations using blood pressure values from a randomized list, and showed the difference between CAVI and CAVI₀, as it related to the dependency on the blood pressure at measuring time²⁷⁾.

Based on the assumption that the artery is completely monotonous and blood pressure is the same at both sides of the artery, Spronck's equation (eq. 2) could be mathematically reasonable. However, Spronck's equation (eq. 2) includes only Pd. This raises one concern that CAVI₀ might be influenced too strongly by Pd³⁰⁾. The pulse transition time measured in CAVI is not just a foot-to-foot period of the pulse waves at the pressure level of Pd, and it is not suitable to apply the CAVI₀ theory simply to this condition.

To date, there has been no detailed report comparing CAVI and CAVI₀ with a large population; carotid-femoral PWV has been compared to CAVI and resulted in a relatively small correlation [r² = 0.18 ¹⁰⁾ or r² = 0.31 ³¹⁾].

Aim

The aim of this study was to compare and clarify the actual differences between CAVI and CAVI₀ in the large population. Both values were compared in a healthy group and a hypertensive group by simple and multiple regression analyses using age, sex, body mass index (BMI), Ps, and Pd as variables. Also, the comparison was performed in subgroups by sex and age. As a result, there were differences between CAVI and CAVI₀. The reason for the differences is discussed from the viewpoint of the properties of heart-ankle PWV (haPWV) used in the VaSera system.

Furthermore, Spronck et al. pointed out that the arterial stiffness value should be corrected with a reference pressure²⁷⁾, and 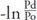(see eq. 2) was added in the CAVI₀ equation. In this article, the actual differences between the CAVI values with or without reference pressure were calculated and compared among the healthy group and the hypertensive group, respectively.

Methods

Subjects

The population-based sample used in the present analysis was comprised of n = 8,631 (n = 5,293, healthy and n =3,338, hypertensive) Japanese subjects aged ≥ 20 years residing in major cities nationwide who underwent a health check at the Japan Health Promotion Foundation with the complete data set n = 28,400 among n =32,627 of the published data^{32, 33)}. A flow diagram of the population is shown in Fig. 1.

Fig. 1.

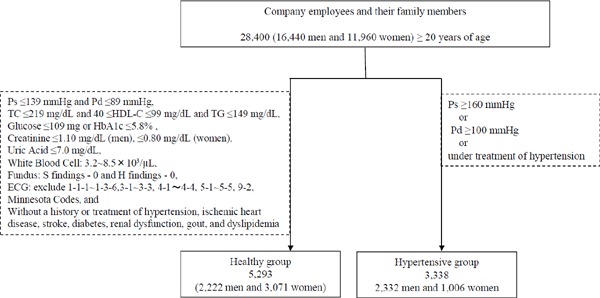
Flow diagram of the population

Abbreviations: Ps, systolic blood pressure; Pd, diastolic blood pressure; Pm, mid blood pressure; TC, total cholesterol; HDL-C, high-density lipoprotein cholesterol; TG, triglyceride.

Analysis Method

1. Comparison of CAVI and CAVI₀ by Simple and Multiple Regression Analysis

Firstly, in order to overview the basic feature of CAVI and CAVI₀ as a whole, the coefficients of correlation between CAVI and CAVI₀ were obtained in the healthy and hypertensive groups. Then, simple and multiple regression analyses were performed in the healthy and hypertensive groups using age, sex, BMI, Ps, and Pd as variables. Dummy variables were set for sex (men: 1, women: 2). To avoid confounding by blood pressure, regression analysis was performed in separate models with Ps and with Pd. The criteria of the healthy group and the hypertensive group are shown in Fig. 1.

2. Comparison of the Differences between CAVI and CAVI₀ among the Healthy and Hypertensive Groups in Sex and Age subgroups

To investigate the differences between CAVI and CAVI₀ in detail, subjects were divided into 12 groups by sex and age strata. The age groups were 20–29, 30–39, 40–49, 50–59, 60–69, and ≥ 70 years. The mean values of CAVI and CAVI₀ and the statistical significance between the healthy group and the hypertensive group were calculated, respectively, in men and women.

Also, in order to investigate the relationship between CAVI and CAVI₀ with blood pressure, Ps and Pd of the healthy group and the hypertensive group were obtained in the same manner in each age stratum in men and women.

3. Correlation between haPWV and Ps, Pd, and Mid Pressure (Pm) in the Healthy Group

Shirai et al. reported that CAVI without coefficient “a” and “b” can be described as indicated in eq. 3 ³⁴⁾:

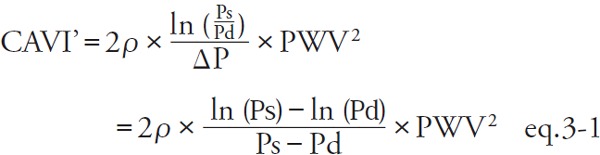[CAVI': CAVI without coefficients “a” and “b,” Pm: mid blood pressure ]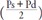

The reason why CAVI is based on Pm is as follows: CAVI is calculated from haPWV, which is the PWV of the arterial tree from the origin of the aorta to the ankle. As is well known, PWV has blood pressure dependency¹⁶⁾. Therefore, also in the cardiac cycle, PWV takes different values depending on the point of measurement between at Pd and at Ps; Shirai et al. showed that haPWV in CAVI corresponded mostly to Pm rather than Ps and Pd³⁴⁾. It means that the formula of CAVI is substantially based on Pm, whereas, Spronck et al. assumed that Pd should be used in CAVI₀ because PWV measured at the foot-to-foot of pulse wave corresponds to at Pd. This is the essential difference. The influence of the second term of CAVI₀, which is related to the reference pressure, will be described later.

In order to reconfirm this, the relationship of haPWV with Ps, Pd, and Pm were studied in a healthy group, which is considered to have less influence of vascular remodeling with hypertension, by age strata with simple regression analysis.

4. Analysis of the Over-Estimation of CAVI₀: Comparison of Pm/Pd in Men and Women

Since in CAVI₀, PWV squared is divided by incompatible Pd instead of compatible Pm, there is a concern that the CAVI₀ value is overestimated by Pm/Pd, which corresponds to CAVI₀/CAVI as a whole. In order to confirm the influence of this over-estimation, the values of Pm/Pd were calculated in the healthy group and the hypertensive group in each age stratum in men and women

5. Comparison of CAVI Values with and without Reference Pressure

A combination of Bramwell–Hill's equation and Stiffness Parameter β gives eq. 4 (Supplement Seq. 15).

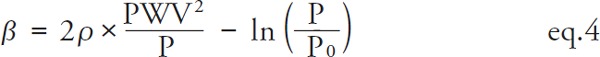When PWV corresponds to Pd, by substituting P=Pd, the CAVI₀ equation of eq. 2 is derived. For CAVI, PWV corresponds to Pm, and by substituting P= Pm, eq. 5 is derived.

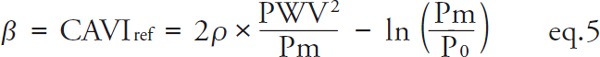[CAVI_ref: the value with reference pressure P₀.]

CAVI' described in eq. 3-1 is the actual measured value without the coefficients “a” and “b,” and is comparable to CAVI_ref, the mathematical value with reference pressure described in eq. 5. It has been reported that there is no discrepancy in the clinical significance between CAVI with and without the coefficients “a” and “b”⁸⁾.

In order to evaluate the differences of values, CAVI_ref, CAVI' and CAVI₀ were compared and the difference ratio to CAVI_ref were calculated in the healthy group and hypertensive group.

Statistical Analysis

Unpaired Welch's t-test was used to evaluate comparisons of CAVI and CAVI₀ among the groups. Results were expressed as the mean ± standard deviation, and p < 0.05 was considered significant.

In the regression analysis, the dependent variables were CAVI and CAVI₀, and the independent variables used for regression analysis were sex, age, body mass index (BMI), Ps, and Pd. Co-linearity between independent variables was confirmed by the Durbin-Watson test and Variance Inflator Factor.

In the simple regression analysis of haPWV with Ps, Pd, and Pm, the coefficient of correlation was obtained in each age stratum of the healthy group. In the comparison of βm, βm', and CAVI₀, the results were expressed as the mean ± standard deviation. All statistical analyses were performed using the SPSS software package (SPSS Inc., Chicago, IL, USA).

Results

1. Comparison of CAVI and CAVI₀ with Simple and Multiple Regression Analysis

The coefficients of correlation between CAVI and CAVI₀ in the healthy group and the hypertensive group were 0.923 and 0.955, respectively, with p < 0.001 for both. Subsequently, regression analyses with age, sex, BMI, Ps, and Pd in CAVI and CAVI₀ were studied. The results are shown in Table 1. In the relationship with Ps (Table 1a, b), no major discrepancy was seen between CAVI and CAVI₀, except for that in CAVI; Ps was not significant in the multiple regression analysis in the healthy group.

Table 1. Comparison of the relationship of CAVI and CAVI0 with blood pressure by simple and multiple regression analysis with Ps (a, b) and with Pd (c, d).

(a) Simple and multiple regression analysis of CAVI with Ps
	Healthy (N = 5,293)							Hypertension (N = 3,338)
	Simple Regression		Multiple Regression					Simple Regression		Multiple Regression
	r	p value	β	B	95%CI		p value	r	p value	β	B	95%CI		p value
Age	0.607	p < 0.001	0.644	0.050	0.048	0.051	p < 0.001	0.525	p < 0.001	0.515	0.064	0.060	0.067	p < 0.001
Sex	−0.117	p < 0.001	−0.182	−0.317	−0.353	−0.280	p < 0.001	−0.081	p < 0.001	−0.115	−0.292	−0.363	−0.221	p < 0.001
BMI	−0.049	p < 0.001	−0.197	−0.058	−0.064	−0.051	p < 0.001	−0.224	p < 0.001	−0.146	−0.050	−0.059	−0.040	p < 0.001
Ps	0.119	p < 0.001					NS	0.127	p < 0.001	0.166	0.010	0.009	0.012	p < 0.001

(b) Simple and multiple regression analysis of CAVI0 with Ps
	Healthy (N = 5,293)							Hypertension (N = 3,338)
	Simple Regression		Multiple Regression					Simple Regression		Multiple Regression
	r	p value	β	B	95%CI		p value	r	p value	β	B	95%CI		p value
Age	0.531	p < 0.001	0.564	0.084	0.081	0.088	p < 0.001	0.553	p < 0.001	0.548	0.182	0.173	0.191	p < 0.001
Sex	−0.080	p < 0.001	−0.143	−0.480	−0.558	−0.403	p < 0.001	−0.027	p = 0.122	−0.057	−0.388	−0.574	−0.202	p < 0.001
BMI	−0.088	p < 0.001	−0.221	−0.125	−0.139	−0.112	p < 0.001	−0.211	p < 0.001	−0.123	−0.112	−0.137	−0.087	p < 0.001
Ps	0.114	p < 0.001	0.027	0.004	0.000	0.008	p < 0.05	0.180	p < 0.001	0.224	0.038	0.033	0.042	p < 0.001

(c) Simple and multiple regression analysis of CAVI with Pd
	Healthy (N = 5,293)							Hypertension (N = 3,338)
	Simple Regression		Multiple Regression					Simple Regression		Multiple Regression
	r	p value	β	B	95%CI		p value	r	p value	β	B	95%CI		p value
Age	0.607	p < 0.001	0.633	0.049	0.047	0.051	p < 0.001	0.525	p < 0.001	0.548	0.068	0.064	0.071	p < 0.001
Sex	−0.117	p < 0.001	−0.175	−0.304	−0.341	−0.266	p < 0.001	−0.081	p < 0.001	−0.097	−0.246	−0.319	−0.173	p < 0.001
BMI	−0.049	p < 0.001	−0.205	−0.060	−0.066	−0.054	p < 0.001	−0.224	p < 0.001	−0.146	−0.049	−0.059	−0.040	p < 0.001
Pd	0.214	p < 0.001	0.040	0.004	0.002	0.006	p = 0.001	−0.031	p = 0.076	0.145	0.014	0.011	0.017	p < 0.001

(d) Simple and multiple regression analysis of CAVI0 with Pd
	Healthy (N = 5,293)							Hypertension (N = 3,338)
	Simple Regression		Multiple Regression					Simple Regression		Multiple Regression
	r	p value	β	B	95%CI		p value	r	p value	β	B	95%CI		p value
Age	0.531	p < 0.001	0.641	0.096	0.092	0.099	p < 0.001	0.553	p < 0.001	0.534	0.178	0.168	0.187	p < 0.001
Sex	−0.080	p < 0.001	−0.195	−0.655	−0.729	−0.580	p < 0.001	−0.027	p = 0.122	−0.069	−0.468	−0.661	−0.276	p < 0.001
BMI	−0.088	p < 0.001	−0.162	−0.092	−0.105	−0.079	p < 0.001	−0.211	p < 0.001	−0.114	−0.104	−0.130	−0.078	p < 0.001
Pd	−0.064	p < 0.001	−0.257	−0.050	−0.055	−0.045	p < 0.001	−0.166	p < 0.001					NS

Abbreviations: BMI, Body Mass Index; Ps, systolic blood pressure; Pd, diastolic blood pressure; r, regression coefficient; β, standardized partial regression coefficient; B, unstandardized partial regression coefficient; CI, confidential interval.

However, in the relationship with Pd (Table 1c, d), an inexplicable result was observed for CAVI₀. For CAVI, the correlation coefficient (r) in simple regression with Pd in the healthy group was 0.214 (p < 0.001). In multiple regression analysis, the standardized partial regression coefficient (β) was 0.040 (p = 0.001). In the hypertensive group, for a simple regression with Pd, the result was −0.031 (p = 0.076). In the multiple regression analysis, the standardized partial regression coefficient (β) was 0.145 (p < 0.001). From these results, a positive correlation with Pd was observed for CAVI.

For CAVI₀, the correlation coefficient (r) in the simple regression analysis with Pd in the healthy group was −0.064 (p < 0.001). In the multiple regression analysis, the standardized partial regression coefficient (β) was −0.257 (p < 0.001). In the hypertensive group, in the simple regression analysis with Pd, the result was −0.166 (p < 0.001). In multiple regression analysis, the standardized partial regression coefficient (β) was not significant. As shown above, a negative correlation with Pd was observed for CAVI₀.

2. Comparison of the Significant Differences of CAVI and CAVI₀ between the Healthy and the Hypertensive Groups in Sex and Age Subgroups

As shown in Fig. 2a–d, in general, a similarity was seen between CAVI and CAVI₀, but inexplicable results were found for CAVI₀. In the analysis of CAVI, the value of the hypertensive group exceeded the value of the healthy group in both men and women, and the differences were significant, except for women at the age of 30–39 years.

Fig. 2.

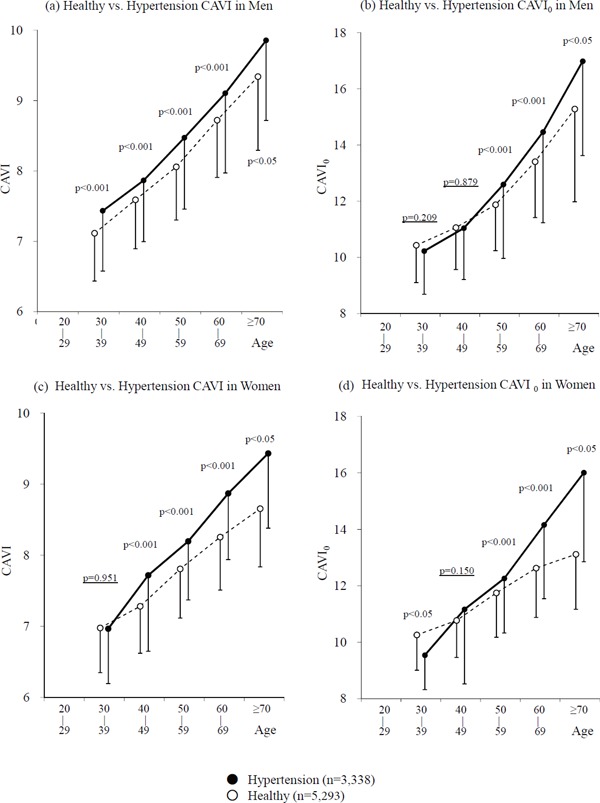
Comparison of the significant differences of CAVI and CAVI₀ between healthy group and the hypertensive group in each age stratum in men (a, b) and women (c, d)

However, in the analysis of CAVI₀, in the group with the age of 30–39 years, the values of the hypertensive group were less than the values of the healthy group in both men and women. (10.23 ± 1.54 vs. 10.43 ± 1.33, p = 0.21 in men, and 9.54 ± 1.22 vs. 10.26 ± 1.25, p = 0.024 in women, respectively). In the group with women at the age of 30–39 years, the values of the hypertensive group were significantly less than the values of the healthy group. Thus, a contradiction was observed between CAVI and CAVICAVI₀ among large healthy and hypertensive populations.

Additionally, the differences between the healthy and hypertensive groups were not significant in the age groups; 30–39 years and 40–49 years in men and 40–49 years in women (p = 0.209, p = 0.879, and p = 0.150, respectively), whereas in CAVI, the differences were significant with p < 0.001 or p < 0.05. The results of the blood pressure in each age stratum in men and women are shown in Fig. 3. In the healthy group, both Ps and Pd increased with age in both men and women. In the hypertensive group, both Ps and Pd decreased with age in both men and women, in conjunction with the antihypertensive treatments.

Fig. 3.

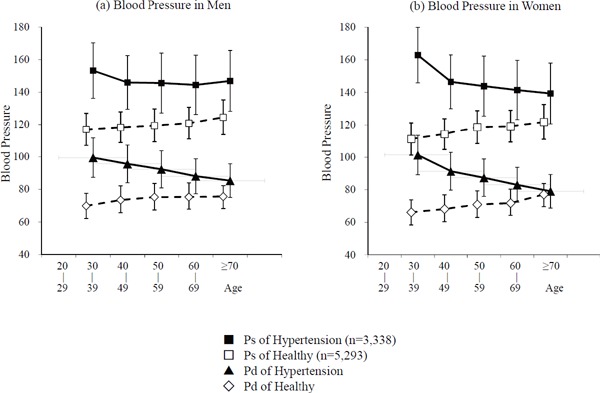
Blood pressure in each age stratum in men (a) and women (b)

Abbreviations: Ps, systolic blood pressure; Pd, diastolic blood pressure.

3. Correlation between haPWV and Ps, Pd, and Pm in the Healthy Group

Coefficients of correlation between haPWV and Ps, Pd, and Pm in the healthy group were obtained, and the results are shown in Table 2. In all age strata, Pm showed a stronger correlation with haPWV than Ps and Pd, indicating that Pm was a stronger factor in the determination of haPWV than Ps and Pd. This result was consistent with the previous report by Shirai et al.³⁴⁾.

Table 2. Correlation of coefficients between haPWV and Ps, Pd, and Pm in the healthy group (n = 5,293).

Age n	20–29 980	30–39 1,948	40–49 1,260	50–59 818	60–69 255	≥ 70 32	Total 5,593
Ps	.565 (***)	.529 (***)	.556 (***)	.520 (***)	.464 (***)	.332 (NS)	.544 (***)
Pd	.599 (***)	.587 (***)	.632 (***)	.567 (***)	.536 (***)	.082 (NS)	.624 (***)
Pm	.637 (***)	.604 (***)	.641 (***)	.599 (***)	.546 (***)	.290 (NS)	.631 (***)

***means p < 0.001 and NS means p ≥ 0.05

Abbreviations: Ps, systolic blood pressure; Pd, diastolic blood pressure; Pm, mid blood pressure.

4. Analysis of the Over-Estimation in CAVI₀; Comparison of Pm/Pd in Men and Women

The changes of Pm/Pd at each age group were studied to clarify how the contradictory results (i.e., higher CAVI₀ in healthy women than in hypertensive women at the age of 30–39 years), were obtained. The results are shown in Fig. 4. Pm/Pd values in the hypertensive group were less than those aged 30–39 and 40–49 in the healthy group in both men and women.

Fig. 4.

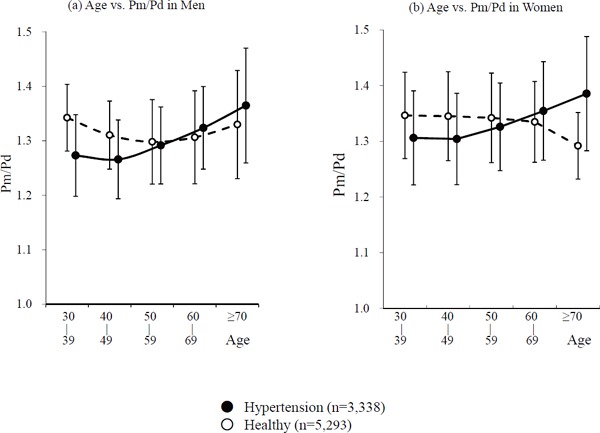
Comparison of the Pm/Pd between healthy group and the hypertensive group in each age stratum in men (a) and women (b)

Abbreviations: Pm, mid blood pressure; Pd, diastolic blood pressure.

5. Comparison between CAVI Values with and without Reference Pressure

The difference between CAVI_ref (with reference pressure P₀), CAVI' (actual measured value without the coefficients “a” and “b”), and CAVI₀ are shown in Fig. 5. The difference ratio of CAVI' and CAVI_ref in the healthy group and in the hypertensive group were 1.09% ± 1.39% and 3.68 ± 1.66%, respectively, while the difference ratio of CAVI₀ to CAVI_ref in the healthy group and in the hypertensive group were 37.58 ± 8.66% and 34.75 ± 9.48%, respectively.

Fig. 5.

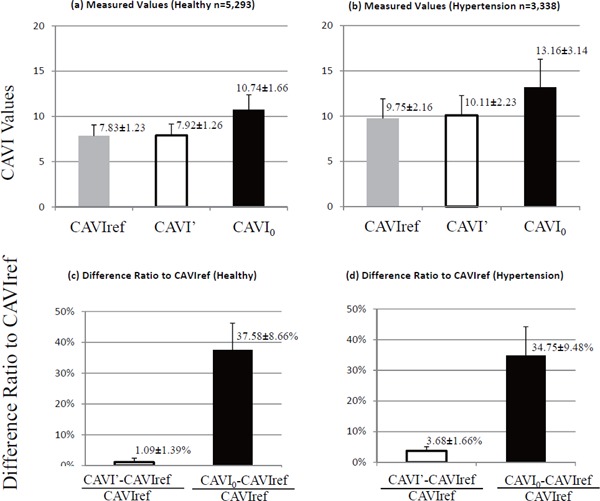
Comparison of measured values with and without the reference pressure

CAVI ref is with reference pressure and CAVI' is without reference pressure. (a) values in the healthy group, (b) values in the hypertensive group, (c) difference ratio of CAVI' and CAVI₀ with CAVIref in the healthy group, and (d) difference ratio in the hypertensive group.

Discussion

In general, some similarity was seen between CAVI and CAVI₀ with high values more than 0.9 in coefficients of correlation both in the healthy and hypertensive groups.

However, as shown in Table 1 (d), the Pd was a significantly negative contributing factor for CAVI₀ in both the healthy group and hypertensive group by simple regression analysis, and it was also a negatively contributing factor for CAVI₀ in the healthy group by multiple regression analysis. Generally, those results were unreasonable. Whereas the Pd was a significantly positive contributing factor for CAVI in the healthy group by simple regression analysis and multiple regression analysis, and there was no significance between Pd and CAVI in the hypertensive group by both simple and multiple regression analysis. These results were acceptable.

Next, CAVI and CAVI₀ values were compared in subgroups of sex and age, as shown in Fig. 2. a–d. The CAVI₀ values of the hypertensive young women group were significantly lower than the value of the healthy young women group, and the significantly higher CAVI₀ was observed age > 50 years in both men and women. Whereas, CAVI values of the hypertensive young women group were almost the same as those of the healthy young women group, and the significantly higher CAVI in the hypertensive group was observed in all age strata of men and women, except for young women in their 30s. It is unreasonable that arterial stiffness in young hypertensive women is lower than that of the young healthy women in their 30s. Therefore, we tried to clarify the reason.

At first, the equations of CAVI and CAVI₀ (see eq. 1 and eq. 2 as described in the introduction), were compared. The main component of CAVI₀ is composed of pwv²/pd, so it is supposed that CAVI₀ is overly negatively influenced by Pd. This is considered the reason for lower CAVI₀ with higher Pd and higher CAVI₀ with lower Pd, seen in Fig. 2–3.

Furthermore, in order to clarify another reason for these contradictory results between CAVI₀ and CAVI, the properties of PWV, which is an important component for CAVI and CAVI₀, were analyzed. As previously reported, PWV changes during the cardiac cycle according to the blood pressure^{35, 36)}.

Spronck et al. assumed in the analysis of CAVI₀ that haPWV should be the PWV at Pd based on the assumption that PWV was measured at the foot-to-foot of the pulse wave and used Pd in the CAVI₀ equation^27–29). To confirm this, the correlation of Ps, Pd, and Pm with haPWV used in the VaSera system were studied. Among Ps, Pd, and Pm, Pm was correlated with haPWV most strongly in the healthy group in all age strata as shown in Table 2. This data was consistent with the previously published data in all populations (n = 3,591)³⁴⁾. The reason is not clear yet; however, one of the reasons may be due to the detection system of PWV in the VaSera system. In order to identify the period from the starting time of the wave at the origin of the aorta, the time from the 2^nd heart sound to the corresponding notch of the upper brachial artery, and the time from the upper brachial artery to the ankle are measured. The former time is measured in the end-systolic period, and the pressure level is far higher than Pd. This detection system may render the strong dependency of haPWV on Pm rather than Pd. We have already reported this item³⁴⁾. Spronck et al. gave a further comment on previous data³⁴⁾ in an attempt to explain that the dependency of CAVI on Pm might be due to arterial remodeling in hypertensive patients because Pm is considered to be related to the remodeling. However, the data of Table 2 in this paper was obtained from the healthy group, and the results were consistent in all age strata, irrespective of the influence of age-related remodeling. This data was essentially consistent with the data in our previous report.

Then, it can be concluded that CAVI was obtained at Pm during the pulse cycle between Ps and Pd in the VaSera system.

Furthermore, to confirm the reason why CAVICAVI₀ in healthy women was higher than in hypertensive women at the age of 30–39 years, the contribution of Pd was studied. Fig. 4 shows Pm/Pd in men and women of all ages. Pm/Pd in the hypertensive group was significantly less than those of the healthy group aged 30–39 years and 40–49 years in both men and women. This corresponds to the results of the comparison of the significant contradiction between CAVI and CAVI₀ among women at the age of 30–39 years in the healthy group and the hypertensive group, and is considered to be the reason why the CAVI₀ values were reversed between the young hypertensive group and the healthy group, and that there were no significant differences between them in men.

Also, as Pd increases, Pm/Pd becomes relatively small, so the CAVI₀ value decreases. As a result, CAVI₀ correlated negatively with Pd. This is considered to be the main reason why CAVI₀ showed unreasonable results.

These results suggest that CAVI₀ was strongly dependent on Pd changes and the CAVI₀ value was underestimated in the case of the elevated Pd group.

Another important difference between CAVI and CAVI₀ is that CAVI₀ included 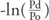to be corrected with P₀. The influence of the reference pressure on CAVI was studied using clinical data without coefficients “a” and “b.” As shown in Fig. 5, the difference ratio between CAVI' and CAVI_ref was small and practically negligible in clinical usage, while the difference ratio between CAVI₀ and CAVI_ref was large.

From the above results, the difference between CAVI and CAVI₀ is due to the application of Pm in place of Pd, rather than the difference with or without the reference pressure.

Recently, two studies comparing CAVI and CAVI₀ in pediatric subjects of Slovakia reported a slight blood pressure dependency of CAVI. One study compared between normotensive boys and those with white-coat hypertension (WCH) with higher CAVI³⁷⁾, and the other study compared between normotensive girls and boys with spurious systolic hypertension (SSH) with lower CAVI³⁸⁾. No significant differences were seen in CAVI₀ in both cases.

However, this article did not show any other standard of arterial stiffness, and it is actually difficult to decide which index is more proper. Only the values of CAVI and CAVI₀ were presented on the assumption that there was no difference in either group regarding arterial stiffness. Although it is known that WCH is associated with sympathetic hyperactivity³⁹⁾, which can modulate arterial stiffness⁴⁰⁾, and it is also known that SSH is seen in young boys with highly elastic arteries⁴¹⁾, some possibilities remain, in that arterial stiffness of the persons with WCH is actually high, and that of boys with SSH is actually low.

Conclusion

CAVI showed reasonable values, but CAVI₀ showed unreasonable values between healthy and hypertensive populations, especially in young women. This difference was due to the strong dependency of CAVI₀ on Pd. The differences in CAVI values with or without the reference pressure were negligible. These results indicate that CAVI obtained by the VaSera system is appropriate, whereas CAVI₀ is not. We concluded that the CAVI value obtained by using the VaSera system is reasonable and appropriate as a clinical index of blood pressure-independent arterial stiffness of the arterial tree from the origin of the aorta to the ankle, and the accumulated data published for the last 15 years is reliable.

Disclosures

Kohji Shirai, Kenji Suzuki, Kazuhiro Shimizu, and Masanobu Takata had no conflict of interest concerning this article.

Shinichi Tsuda, Tomoyuki Yamamoto, Mitsuya Maruyama, and Koji Takahashi belong to Fukuda Denshi Co., Ltd. and are involved in the development of CAVI.

Supplement

β Described with PWV and Blood Pressure

Hayashi defied the β as blood pressure-independent parameter of arterial stiffness in Seq. 1 ⁵⁾.

(β: specific stiffness of the blood vessel, P: blood pressure, P₀: reference pressure, D: blood vessel diameter, D₀: blood vessel diameter at P₀)

By dividing both side of Seq. 1 by P₀ and taking natural logarithm,

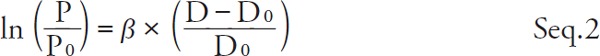Therefore,

By substituting P = Ps and P = Pd for Seq. 2 and transforming,

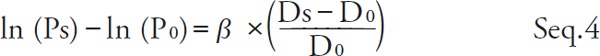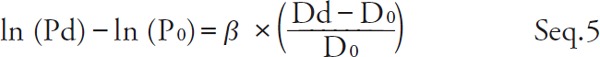By taking the difference between both sides of Seq. 4 and Seq. 5 and transforming,

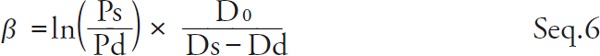Kawasaki et al. defined the β' as practically measurable stiffness parameter in Seq. 7 ⁶⁾.

From Seq. 6 and Seq. 7,

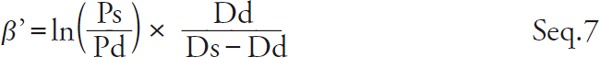From Seq. 2,

By substituting Seq. 9 for Seq. 8 with D = Dd

Since in the physiological range, the second term of right side of Seq. 10 is generally small compared to the first term, β is approximated by β'.

On the other hand, the Bramwell-Hill equation is represented by Seq. 11 ^{2, 3)}.

(ρ: blood density)

Here, 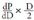is the volume elastic modulus, and Seq. 11 is a general equation expressing the relationship between the wave velocity and the volume elastic modulus. If D changes in proportion to P, the volume elastic modulus is constant and the wave velocity PWV is also constant. In blood vessels, however, D changes exponentially with respect to P, which is indicated by the Stiffness Parameter β equation of Seq. 1

The blood pressure dependency of PWV arises from the exponential nature of this blood vessel as follows:

When both sides of Seq. 1 are differentiated with D, the exponent 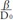becomes a coefficient from the differential formula of exponential,

Substituting Seq. 12 for the right side of Seq. 11

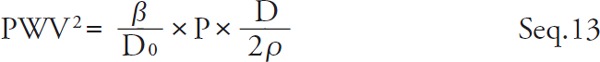β is represented by Seq. 14 by transforming Seq. 13.

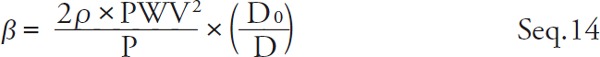Substitute Seq. 9 for Seq. 14 and organize it with β,

Since in the physiological range, the second term of right side of Seq. 15 is generally small compared to the first term, PWV² is approximately proportional to β and P.