Abstract
Is the variation in the compressive strength of concrete across the thickness of horizontally cast elements negligibly small or rather needs to be taken into account at the design stage? There are conflicting answers to this question. In order to determine if the compressive strength of concrete varies across the thickness of horizontally cast elements, ultrasonic tests and destructive tests were carried out on core samples taken from a 350 mm thick slab made of class C25/30 concrete. Special point-contact probes were used to measure the time taken for the longitudinal ultrasonic wave to pass through the tested sample. The correlation between the velocity of the longitudinal ultrasonic wave and the compressive strength of the concrete in the slab was determined. The structure of the concrete across the thickness of the slab was evaluated using GIMP 2.10.4. It was found that the destructively determined compressive strength varied only slightly (by 3%) across the thickness of the placed layer of concrete. Whereas the averaged ultrasonically determined strength of the concrete in the same samples does not vary across the thickness of the analyzed slab. Therefore, it was concluded that the slight increase in concrete compressive strength with depth below the top surface is a natural thing and need not be taken into account in the assessment of the strength of concrete in the structure.
Keywords: concrete slabs and floorings, horizontal casting, compressive strength, ultrasonic tests
1. Introduction
The view that the compressive strength of concrete varies across the thickness of horizontally cast elements (concrete slabs, floorings, etc.) is seldom expressed in the literature on the subject. Opinions as to the significance of this variation are widely divided, as the following survey of literature indicates.
The research published by Stawiski [1,2,3] provided the direct incentive for this study of the distribution of concrete compressive strength along the height of horizontally cast elements. On the basis of ultrasonic tests of core samples taken from concrete, Stawiski found the compressive strength of the concrete to be lower in the top zone than in the bottom zone by as much as 40–50% [1,2,3]. The variation in compressive strength along the height of the cross section was approximately linear. The fall in ultrasonic wave velocity at the sample’s top surface is ascribed by Stawiski [1] to the surface weakening effect connected with concrete consolidation resulting in the segregation of concrete components. The main factor responsible for the decrease in concrete compressive strength is considered to be porosity, which very strongly affects ultrasonic wave velocity. Also the inadequate curing of fresh concrete, damage to the structure of concrete caused by corrosion, and mechanical damage to the top surface of the concrete which can arise in the course of the service life of the element are also possible factors.
Stawiski [3] proposed to introduce (besides the grade of concrete) strength gradient ∇fc into the evaluation of concrete in horizontally cast elements (e.g., floor toppings). On the basis of his research [3] Stawiski pointed out that in, e.g., an approximately 15 cm thick element the strength gradient of the concrete at the depth of 10 cm from the bottom amounted to 0.7 MPa/cm, whereas in the layers situated closer to the top surface it varied markedly (−3.0, −4.5, −8.0 MPa/cm). Therefore, Stawiski calls for [3] defining allowable variations in concrete compressive strength, e.g., ∇fc ≤ 1.0 MPa/cm. The increase of 1.0 MPa/cm in the strength of concrete in the lower situated layers relative to the top layer suggested by Stawiski [3] seems to be very large.
On the basis of ultrasonic tests of the compressive strength across the thickness of samples taken from cut out pieces of 40, 45 and 60 mm thick floorings made of cement mortars, Hoła, Sadowski and Hoła A. [4] found the strength was not identical and varied across the thickness. The lowest strength was in the top zone, the highest in the bottom zone, while in the middle zone, it was close to the destructively determined compressive strength. In the considered case, the strength gradient of the mortar across the thickness of the flooring amounted to 6–7 MPa/cm.
Petersons in [5] found the compressive strength of the lower situated layers to be higher than that of the top layer, but only by 10–20%. No further increase in concrete strength was observed in the layers situated below 300 mm. The difference in compressive strength between the top surface and the bottom surface in slabs was ascribed to the inadequate curing of the concrete [5].
In monograph [6], Dąbrowski, Stachurski and Zieliński found that the deeper situated layers of concrete had higher strength than the surface layer. Below 80 cm, this increase in strength stabilized at the level of approximately 10%. In the authors’ opinion [6], this is due to the well-known property of concrete—it reaches higher strength when hardening under a moderate pressure—and that is why this phenomenon does not occur in samples of low height.
Yuan, Ragab, Hill and Cook [7] found that the compressive strength of concrete along the height of the placed layer did not vary significantly. Suprenant [8] found that the compressive strength of concrete in slabs varied minimally, and only in a small upper part of the element. The most marked variation in concrete strength along element height has been observed in walls and beams. This is mainly due to the greater static pressure exerted by the concrete situated above.
Neville [9] found that the slight increase in concrete compressive strength below the top surface was a natural thing, but need not be taken into account. When testing a reinforced concrete wall and beam by means of the ultrasonic method, Watanabe, Hishikawa, Kamae and Namiki [10] found the compressive strength of concrete in samples taken from the lower part of the element was slightly higher than in samples taken from its upper part. They treated this as a natural thing which did not need to be taken into account.
Neville [11] ascribed the variation in the compressive strength of concrete along the height of the sample to the presence of retained water, occurring during concrete bleeding.
According to standard [12], the compressive strength of concrete in a structure can be lower in the top layer than in the bottom layer by as much as 25%. Concrete characterized by lower compressive strength usually occurs to a depth of 300 mm or to 20% of the height of the cross section, depending on which of the values is lower. According to standard [13], the range of variation in the compressive strength of concrete in a structure can differ between the particular portions of the structure. The variation is random and often forced (by, e.g., the relative density, the degree of compaction, the curing conditions, etc.).
Therefore, the questions arises: Is the variation in the compressive strength of concrete across the thickness of horizontally cast elements negligibly small or rather needs to be taken into account at the design stage?
2. Description of Author’s Investigations
2.1. Ultrasonic Tests of Concrete
In order to verify the phenomenon of concrete compressive strength variation across the thickness of horizontally cast elements, samples with diameter d = 100 mm height h = 350 mm (Table 1), taken from a specially cast slab made of concrete C25/30 (the grade of the concrete was determined using concrete cubes cast when casting the slab) were subjected to ultrasonic tests. CEMII/BS-32.5 cement (270 kg/m3), fly ash additive (60 kg/m3), plasticizer (2.43 kg/m3), water (170 kg/m3) and 1879 kg/m3 of natural aggregate (sand 0/2 mm −40%, gravel 2/8 mm −26%, gravel 8/16 mm −34%) were used in the concrete mix for the slab construction. The latter had been compacted by means of an immersion vibrator and cured for 28 in the laboratory conditions defined in standard [14]. The slab had been exposed to variable weather conditions for two years. Samples (01−06 in Figure 1) were drilled out of the slab perpendicularly to its top surface. For reference purposes specimens (07−12) were drilled out of the slab parallel with its top surface. Prior to the tests, the actual dimensions of the samples and their weight were determined (Table 1).
Table 1.
The mean dimensions of core samples and their weight.
| Sample Number | dśr | hśr | m | V | ρ |
|---|---|---|---|---|---|
| mm | g | cm3 | g/cm3 | ||
| 01 | 98.7 | 351.7 | 6158.5 | 2691 | 2.29 |
| 02 | 98.5 | 351.4 | 6167.5 | 2680 | 2.30 |
| 03 | 98.6 | 351.5 | 6138.0 | 2682 | 2.29 |
| 04 | 98.6 | 351.4 | 6176.5 | 2682 | 2.30 |
| 05 | 98.5 | 350.0 | 6121.5 | 2669 | 2.29 |
| 06 | 98.6 | 350.2 | 6121.0 | 2675 | 2.29 |
| 07 | 98.6 | 350.0 | 6122.0 | 2672 | 2.29 |
| 08 | 98.7 | 351.3 | 6172.5 | 2688 | 2.30 |
| 09 | 98.5 | 351.4 | 6168.2 | 2678 | 2.30 |
| 10 | 98.5 | 350.0 | 6122.4 | 2667 | 2.30 |
| 11 | 98.6 | 351.5 | 6140.0 | 2684 | 2.29 |
| 12 | 98.5 | 351.4 | 6137.6 | 2678 | 2.29 |
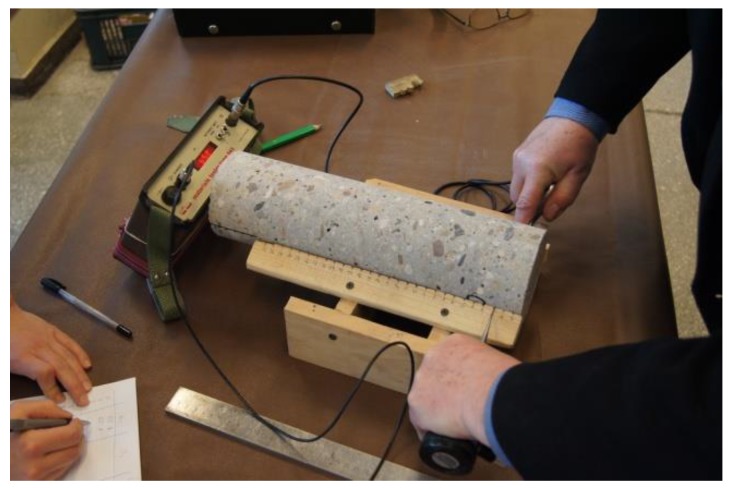
Figure 1.
The samples to be tested using ultrasonic device.
The measuring points spaced at every 1 cm (Figure 1 and Figure 2) were marked on the sides of the core samples. Velocity CL of ultrasonic wave passage through concrete was measured in two perpendicular directions. No concrete/probe coupling material was used. The probes were set perpendicularly to the tested side surface of the sample (Figure 2). The distributions of velocity CL of longitudinal ultrasonic wave passage through the concrete were obtained from the tests (Table 2 and Table 3, and Figure 3 and Figure 4).
Figure 2.
The measurement of velocity CL of longitudinal ultrasonic wave.
Table 2.
Velocities CL ultrasonic wave propagation, measured in two perpendicular directions in core samples taken perpendicularly to top surface of slab.
| Measuring Place Distance from Sample Top | Sample 01 | Sample 02 | Sample 03 | Sample 04 | Sample 05 | Sample 06 | ||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| CL 1 | CL 2 | CL 1 | CL 2 | CL 1 | CL 2 | CL 1 | CL 2 | CL 1 | CL 2 | CL 1 | CL 2 | |
| cm | km/s | |||||||||||
| 0.5 | 3.19 | 3.39 | 3.49 | 3.18 | 3.32 | 3.44 | 3.40 | 3.31 | 3.36 | 3.36 | 3.23 | 3.29 |
| 1.5 | 3.18 | 3.35 | 3.48 | 3.02 | 3.28 | 3.38 | 3.29 | 3.26 | 3.35 | 3.30 | 3.22 | 3.33 |
| 2.5 | 3.30 | 3.41 | 3.49 | 3.48 | 3.31 | 3.37 | 3.40 | 3.36 | 3.28 | 3.33 | 3.27 | 3.36 |
| 3.5 | 3.58 | 3.63 | 3.62 | 3.57 | 3.43 | 3.54 | 3.57 | 3.62 | 3.33 | 3.54 | 3.55 | 3.65 |
| 4.5 | 3.60 | 3.68 | 3.60 | 3.61 | 3.49 | 3.52 | 3.53 | 3.62 | 3.53 | 3.52 | 3.61 | 3.63 |
| 5.5 | 3.51 | 3.66 | 3.64 | 3.54 | 3.66 | 3.62 | 3.66 | 3.62 | 3.62 | 3.67 | 3.57 | 3.61 |
| 6.5 | 3.60 | 3.68 | 3.70 | 3.52 | 3.57 | 3.67 | 3.68 | 3.44 | 3.47 | 3.66 | 3.60 | 3.63 |
| 7.5 | 3.53 | 3.59 | 3.62 | 3.48 | 3.64 | 3.58 | 3.58 | 3.60 | 3.53 | 3.62 | 3.62 | 3.58 |
| 8.5 | 3.46 | 3.60 | 3.72 | 3.61 | 3.57 | 3.50 | 3.70 | 3.60 | 3.54 | 3.61 | 3.56 | 3.58 |
| 9.5 | 3.53 | 3.57 | 3.74 | 3.57 | 3.26 | 3.62 | 3.71 | 3.58 | 3.44 | 3.59 | 3.60 | 3.65 |
| 10.5 | 3.61 | 3.59 | 3.70 | 3.57 | 3.57 | 3.63 | 3.54 | 3.53 | 3.47 | 3.58 | 3.67 | 3.79 |
| 11.5 | 3.58 | 3.59 | 3.62 | 3.66 | 3.60 | 3.62 | 3.62 | 3.56 | 3.50 | 3.58 | 3.58 | 3.62 |
| 12.5 | 3.56 | 3.65 | 3.62 | 3.65 | 3.55 | 3.65 | 3.60 | 3.58 | 3.52 | 3.62 | 3.41 | 3.62 |
| 13.5 | 3.32 | 3.63 | 3.61 | 3.60 | 3.57 | 3.49 | 3.65 | 3.61 | 3.57 | 3.57 | 3.57 | 3.70 |
| 14.5 | 3.38 | 3.65 | 3.72 | 3.66 | 3.55 | 3.63 | 3.70 | 3.70 | 3.63 | 3.62 | 3.66 | 3.76 |
| 15.5 | 3.45 | 3.67 | 3.73 | 3.57 | 3.65 | 3.63 | 3.44 | 3.53 | 3.61 | 3.65 | 3.73 | 3.68 |
| 16.5 | 3.60 | 3.66 | 3.73 | 3.58 | 3.62 | 3.51 | 3.72 | 3.59 | 3.50 | 3.61 | 3.62 | 3.65 |
| 17.5 | 3.53 | 3.68 | 3.72 | 3.53 | 3.53 | 3.62 | 3.66 | 3.53 | 3.54 | 3.61 | 3.65 | 3.70 |
| 18.5 | 3.68 | 3.67 | 3.64 | 3.49 | 3.62 | 3.53 | 3.70 | 3.59 | 3.54 | 3.66 | 3.61 | 3.70 |
| 19.5 | 3.58 | 3.72 | 3.66 | 3.59 | 3.47 | 3.48 | 3.65 | 3.57 | 3.62 | 3.66 | 3.63 | 3.62 |
| 20.5 | 3.59 | 3.68 | 3.63 | 3.47 | 3.57 | 3.39 | 3.64 | 3.70 | 3.43 | 3.70 | 3.62 | 3.66 |
| 21.5 | 3.62 | 3.57 | 3.57 | 3.58 | 3.53 | 3.56 | 3.62 | 3.54 | 3.54 | 3.62 | 3.48 | 3.65 |
| 22.5 | 3.40 | 3.61 | 3.62 | 3.61 | 3.55 | 3.45 | 3.70 | 3.50 | 3.54 | 3.61 | 3.58 | 3.70 |
| 23.5 | 3.45 | 3.64 | 3.62 | 3.65 | 3.68 | 3.59 | 3.59 | 3.61 | 3.40 | 3.37 | 3.58 | 3.65 |
| 24.5 | 3.35 | 3.62 | 3.51 | 3.62 | 3.52 | 3.58 | 3.64 | 3.70 | 3.48 | 3.53 | 3.62 | 3.65 |
| 25.5 | 3.55 | 3.66 | 3.65 | 3.48 | 3.53 | 3.50 | 3.53 | 3.65 | 3.46 | 3.57 | 3.64 | 3.57 |
| 26.5 | 3.38 | 3.71 | 3.69 | 3.61 | 3.57 | 3.32 | 3.48 | 3.65 | 3.44 | 3.61 | 3.58 | 3.58 |
| 27.5 | 3.54 | 3.55 | 3.62 | 3.55 | 3.54 | 3.43 | 3.58 | 3.63 | 3.37 | 3.58 | 3.54 | 3.49 |
| 28.5 | 3.54 | 3.61 | 3.52 | 3.57 | 3.56 | 3.59 | 3.62 | 3.66 | 3.57 | 3.66 | 3.62 | 3.65 |
| 29.5 | 3.61 | 3.65 | 3.63 | 3.58 | 3.60 | 3.59 | 3.68 | 3.69 | 3.48 | 3.54 | 3.57 | 3.57 |
| 30.5 | 3.57 | 3.62 | 3.64 | 3.57 | 3.57 | 3.57 | 3.55 | 3.62 | 3.57 | 3.53 | 3.61 | 3.54 |
| 31.5 | 3.45 | 3.61 | 3.58 | 3.52 | 3.45 | 3.48 | 3.57 | 3.29 | 3.57 | 3.50 | 3.27 | 3.51 |
| 32.5 | 3.24 | 3.20 | 3.52 | 3.25 | 3.13 | 3.34 | 3.30 | 3.42 | 3.48 | 3.26 | 3.43 | 3.34 |
| 33.5 | 3.33 | 3.25 | 3.57 | 3.22 | 3.28 | 3.20 | 3.51 | 3.42 | 3.54 | 3.41 | 3.42 | 3.42 |
| 34.5 | 3.39 | 3.29 | 3.59 | 3.23 | 3.47 | 3.04 | 3.62 | 3.49 | 3.49 | 3.49 | 3.40 | 3.49 |
Table 3.
Velocities CL of longitudinal ultrasonic wave propagation, measured in two perpendicular directions in core samples taken parallel with the top surface of the slab.
| Measuring Place Distance from Sample Top | Sample 07 | Sample 08 | Sample 09 | Sample 10 | Sample 11 | Sample 12 | ||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| CL 1 | CL 2 | CL 1 | CL 2 | CL 1 | CL 2 | CL 1 | CL 2 | CL 1 | CL 2 | CL 1 | CL 2 | |
| cm | km/s | |||||||||||
| 0.5 | 3.31 | 3.42 | 3.60 | 3.40 | 3.34 | 3.60 | 3.60 | 3.35 | 3.30 | 3.28 | 3.50 | 3.38 |
| 1.5 | 3.27 | 3.33 | 3.50 | 3.28 | 3.25 | 3.30 | 3.32 | 3.34 | 3.29 | 3.26 | 3.40 | 3.31 |
| 2.5 | 3.55 | 3.47 | 3.31 | 3.60 | 3.36 | 3.56 | 3.41 | 3.51 | 3.32 | 3.41 | 3.45 | 3.33 |
| 3.5 | 3.56 | 3.45 | 3.25 | 3.46 | 3.59 | 3.42 | 3.62 | 3.53 | 3.52 | 3.54 | 3.43 | 3.59 |
| 4.5 | 3.54 | 3.49 | 3.54 | 3.42 | 3.45 | 3.55 | 3.50 | 3.51 | 3.40 | 3.41 | 3.46 | 3.56 |
| 5.5 | 3.55 | 3.46 | 3.46 | 3.60 | 3.40 | 3.50 | 3.48 | 3.44 | 3.41 | 3.55 | 3.42 | 3.36 |
| 6.5 | 3.57 | 3.50 | 3.41 | 3.45 | 3.51 | 3.44 | 3.41 | 3.44 | 3.28 | 3.38 | 3.46 | 3.37 |
| 7.5 | 3.49 | 3.50 | 3.57 | 3.42 | 3.51 | 3.53 | 3.44 | 3.45 | 3.39 | 3.50 | 3.56 | 3.43 |
| 8.5 | 3.45 | 3.43 | 3.56 | 3.60 | 3.47 | 3.54 | 3.55 | 3.41 | 3.49 | 3.51 | 3.51 | 3.49 |
| 9.5 | 3.55 | 3.43 | 3.60 | 3.52 | 3.44 | 3.57 | 3.48 | 3.51 | 3.50 | 3.44 | 3.45 | 3.50 |
| 10.5 | 3.40 | 3.47 | 3.49 | 3.50 | 3.45 | 3.50 | 3.40 | 3.45 | 3.41 | 3.49 | 3.39 | 3.39 |
| 11.5 | 3.56 | 3.43 | 3.46 | 3.51 | 3.54 | 3.46 | 3.41 | 3.55 | 3.36 | 3.46 | 3.44 | 3.50 |
| 12.5 | 3.55 | 3.38 | 3.42 | 3.50 | 3.54 | 3.46 | 3.42 | 3.47 | 3.52 | 3.55 | 3.43 | 3.43 |
| 13.5 | 3.57 | 3.43 | 3.38 | 3.46 | 3.51 | 3.56 | 3.37 | 3.40 | 3.39 | 3.50 | 3.55 | 3.51 |
| 14.5 | 3.48 | 3.47 | 3.41 | 3.55 | 3.57 | 3.51 | 3.38 | 3.40 | 3.51 | 3.55 | 3.46 | 3.52 |
| 15.5 | 3.59 | 3.47 | 3.55 | 3.51 | 3.43 | 3.59 | 3.42 | 3.41 | 3.48 | 3.58 | 3.44 | 3.45 |
| 16.5 | 3.54 | 3.45 | 3.56 | 3.46 | 3.42 | 3.46 | 3.38 | 3.38 | 3.41 | 3.45 | 3.56 | 3.40 |
| 17.5 | 3.50 | 3.40 | 3.53 | 3.35 | 3.57 | 3.56 | 3.49 | 3.44 | 3.40 | 3.38 | 3.41 | 3.39 |
| 18.5 | 3.47 | 3.44 | 3.46 | 3.47 | 3.49 | 3.45 | 3.40 | 3.36 | 3.42 | 3.39 | 3.38 | 3.40 |
| 19.5 | 3.42 | 3.43 | 3.41 | 3.55 | 3.45 | 3.43 | 3.40 | 3.46 | 3.42 | 3.50 | 3.43 | 3.54 |
| 20.5 | 3.40 | 3.48 | 3.47 | 3.46 | 3.44 | 3.50 | 3.44 | 3.43 | 3.38 | 3.40 | 3.38 | 3.55 |
| 21.5 | 3.45 | 3.50 | 3.50 | 3.39 | 3.42 | 3.42 | 3.44 | 3.56 | 3.48 | 3.44 | 3.44 | 3.49 |
| 22.5 | 3.40 | 3.41 | 3.41 | 3.42 | 3.46 | 3.42 | 3.40 | 3.44 | 3.50 | 3.43 | 3.42 | 3.43 |
| 23.5 | 3.43 | 3.43 | 3.41 | 3.45 | 3.54 | 3.41 | 3.41 | 3.49 | 3.33 | 3.50 | 3.37 | 3.41 |
| 24.5 | 3.50 | 3.38 | 3.53 | 3.50 | 3.38 | 3.42 | 3.49 | 3.42 | 3.52 | 3.38 | 3.51 | 3.50 |
| 25.5 | 3.42 | 3.50 | 3.51 | 3.38 | 3.40 | 3.57 | 3.46 | 3.40 | 3.46 | 3.41 | 3.45 | 3.53 |
| 26.5 | 3.56 | 3.38 | 3.45 | 3.36 | 3.44 | 3.37 | 3.45 | 3.25 | 3.55 | 3.45 | 3.49 | 3.44 |
| 27.5 | 3.54 | 3.45 | 3.51 | 3.47 | 3.39 | 3.31 | 3.30 | 3.36 | 3.36 | 3.43 | 3.41 | 3.46 |
| 28.5 | 3.42 | 3.37 | 3.55 | 3.46 | 3.40 | 3.38 | 3.48 | 3.36 | 3.37 | 3.37 | 3.50 | 3.55 |
| 29.5 | 3.50 | 3.43 | 3.46 | 3.34 | 3.43 | 3.36 | 3.44 | 3.35 | 3.43 | 3.35 | 3.53 | 3.50 |
| 30.5 | 3.56 | 3.50 | 3.42 | 3.43 | 3.45 | 3.45 | 3.44 | 3.53 | 3.50 | 3.37 | 3.37 | 3.32 |
| 31.5 | 3.57 | 3.40 | 3.55 | 3.33 | 3.44 | 3.43 | 3.46 | 3.50 | 3.40 | 3.37 | 3.37 | 3.45 |
| 32.5 | 3.40 | 3.34 | 3.40 | 3.53 | 3.40 | 3.41 | 3.50 | 3.56 | 3.40 | 3.45 | 3.45 | 3.44 |
| 33.5 | 3.50 | 3.40 | 3.59 | 3.51 | 3.47 | 3.45 | 3.42 | 3.49 | 3.51 | 3.40 | 3.44 | 3.47 |
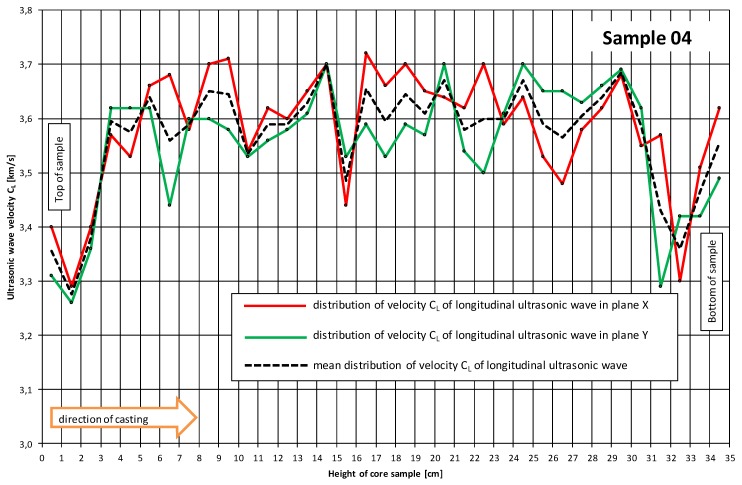
Figure 3.
Exemplary distribution of velocity CL of longitudinal ultrasonic wave along the height of sample 04 taken perpendicularly to the top surface of the slab.
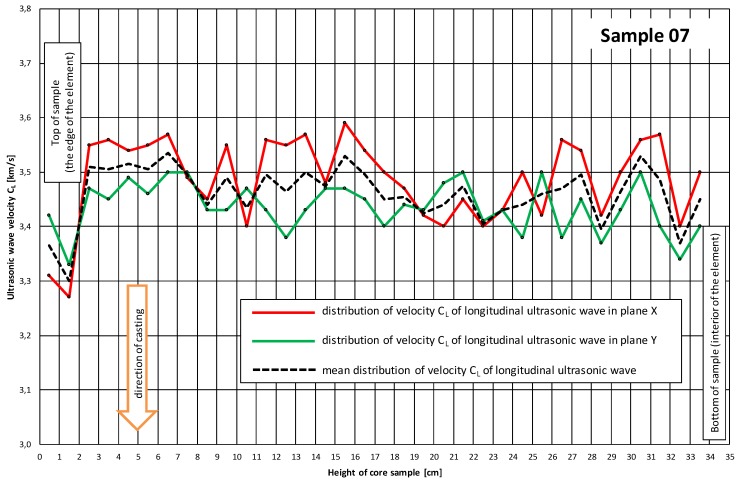
Figure 4.
Exemplary distribution of velocity CL of longitudinal ultrasonic wave along height of sample 07 taken parallel with the top surface of the slab.
A Unipan Materials Tester Type 543 with point-contact exponential probes [15] and a frequency of 40 kHz (Figure 2) was used to measure the time taken for the longitudinal ultrasonic wave to pass through the tested sample. Prior to the tests, the instrument had been calibrated to determine the time taken for the ultrasonic wave to pass through the probes alone (t0 = 36.6 μs). The details of the operation of exponential heads with point-to-point contact with the examined surface are described in detail in the paper [15].
Table 2 and Table 3 and Figure 3 and Figure 4 showed that the distributions of velocity CL of the longitudinal ultrasonic wave along the height of the core samples with d ≅ 100 mm were quite constant (except for the top layers). The mean velocity CL of ultrasonic wave propagation in the samples (01–06) taken perpendicularly to the top surface of the slab was CL1 = 3.54 km/s, a standard deviation sCL1 = 0.13 km/s and a variation coefficient νCL1 = 3.67%. In the case of the samples (07–12) taken parallel with the top of the slab, the following were obtained: CL2 = 3.45 km/s, sCL2 = 0.072 km/s and νCL2 = 2.09%. On the basis of the obtained velocities CL of the longitudinal ultrasonic wave in the range: CL = 3.5–4.5 km/s, it can be assessed [16] whether the quality of the concrete as good, whereas the velocities in the range of 3.0–3.5 km/s indicated dubious quality.
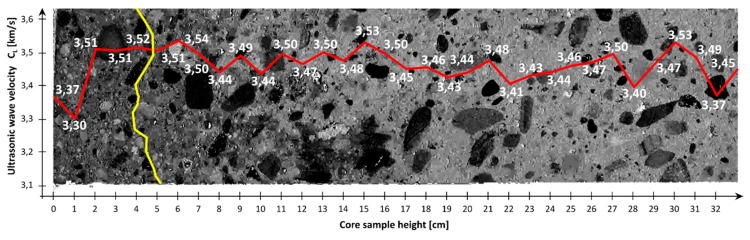
The observed slight fluctuations of velocity CL of the longitudinal ultrasonic wave in the inner layers of the concrete are due to local concrete defects (e.g., air voids) or local strengthening with larger aggregate grains. Lower velocities CL of the longitudinal ultrasonic wave were registered in the samples (01–06) taken perpendicularly to the top surface of the slab (Figure 3 and Figure 5). In the samples (07–12) taken parallel with the top of the slab, a decline in velocity CL of the longitudinal ultrasonic wave was observed only at the edge constituting the side edge of the slab (Figure 4 and Figure 6).
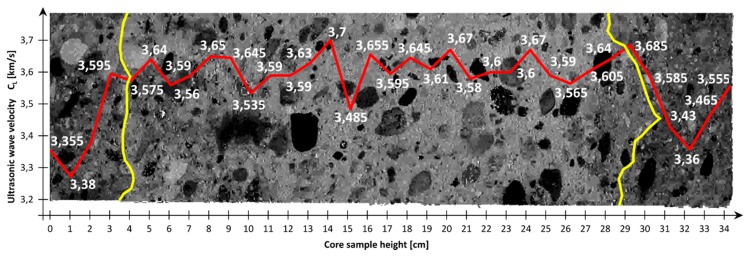
Figure 5.
The structure of concrete of the sample taken perpendicularly to the top surface of the slab (slab top surface on left). The image also shows distribution of the mean velocity CL of longitudinal ultrasonic wave (red line) and disturbance zone at the top and bottom surface of the slab (yellow line).
Figure 6.
The structure of concrete of the sample taken parallel with the top surface of the slab (slab top surface on left). The image also shows distribution of the mean velocity CL of longitudinal ultrasonic wave (red line) and zone of disturbance at the lateral surface of the slab (yellow line).
Initially, it was though that the falls in velocity CL of the longitudinal ultrasonic wave were due to the sample end effect. Ultimately, it was decided that this phenomenon in samples 01–06 was caused from the top by bleeding [17] and concrete sedimentation, and from the bottom by the improper vibration of the concrete by means of the immersion vibrator (the vibrator was not fully immersed in the freshly placed concrete). In samples 07–12, the fall in velocity CL of the longitudinal ultrasonic wave can be caused by the wall effect [9,10]. Figure 5 and Figure 6 show the structure of the concrete along the height of the samples taken perpendicularly to and parallel with the top surface of the slab. The image of the structure of the samples in Figure 5 and Figure 6 was prepared in GIMP 2.10.4 using the filter: LCHH(ab) component with a contrast of 50%. In Figure 5 the altered structure of the concrete is visible in the sample’s upper part (an approximately 30–40 mm thick layer) and lower part (an approximately 30–50 mm thick layer). In these places, reduced velocities of the longitudinal wave velocity were observed. In Figure 6, the altered structure of the concrete can be seen in an approximately 20–80 mm thick layer located at the side wall of the slab. Also in this layer, falls in the velocity of the longitudinal ultrasonic wave were recorded.
2.2. Ultrasonic Tests of Concrete
For further investigations the core samples (Table 1) were cut into smaller samples (Figure 7) with height h = 100 mm (h/d = 1). In the terminal samples (e.g., 01/1—the top of the sample, 01/3—the bottom of the sample) the actual end faces were left unchanged (or were slightly trimmed to make them level). The middle sample (e.g., 01/2) was cut to the required size of 100 mm. Then, the end faces were prepared by grinding for compressive strength tests (Figure 8).
Figure 7.
Core samples with height/diameter ratio h/d = 1, obtained from samples 01–06.
Figure 8.
One of the core samples during grinding of its end surface.
The compressive strength tests were carried out in conformance with standard [13] in the ZD100 strength testing machine (Figure 9a) satisfying the requirements of standard [18]. All the samples showed the same type of failure (Figure 9b). The parameters of the samples and the test results are presented in Table 2.
Figure 9.
The sample in strength testing machine: (a) during loading, (b) after failure.
The concrete strength values (Table 4) yielded by the tests carried out on the100 mm high samples were used to evaluate the class of the concrete and the variation of strength along the core sample height (h = 350 mm) and were correlated with the results obtained using the ultrasonic method. According to standard [19], the result of concrete compressive strength tests carried out on cylindrical specimens with diameter d = 100 mm and height h = 100 mm, cut out of a structure directly corresponded to the strength of concrete determined on 150 × 150 × 150 mm standard cubes (fck,is = fck,is,cube).
Table 4.
The results of concrete compressive strength tests.
| Samp. No. |
dm | hm | m | Ac | Vc | ρ | Fis | fis | Samp. No. |
dm | hm | m | Ac | Vc | ρ | Fis | fis |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| mm | g | cm2 | cm3 | g/cm3 | kN | MPa | mm | g | cm2 | cm3 | g/cm3 | kN | MPa | ||||
| 01/1 | 98.7 | 99.9 | 1725 | 76.51 | 764 | 2.26 | 236 | 30.85 | 07/1 | 98.6 | 100.0 | 1760 | 76.36 | 764 | 2.30 | 226 | 29.60 |
| 01/2 | 98.7 | 99.8 | 1743 | 76.51 | 764 | 2.28 | 240 | 31.37 | 07/2 | 98.6 | 99.9 | 1751 | 76.36 | 763 | 2.30 | 231 | 30.25 |
| 01/3 | 98.7 | 99.8 | 1765 | 76.51 | 764 | 2.31 | 242 | 31.63 | 07/3 | 98.6 | 99.8 | 1746 | 76.36 | 762 | 2.29 | 232 | 30.38 |
| 02/1 | 98.5 | 100.0 | 1724 | 76.20 | 762 | 2.26 | 236 | 30.97 | 08/1 | 98.7 | 99.9 | 1755 | 76.51 | 764 | 2.30 | 230 | 30.06 |
| 02/2 | 98.5 | 99.8 | 1761 | 76.20 | 760 | 2.32 | 238 | 31.23 | 08/2 | 98.7 | 99.9 | 1761 | 76.51 | 764 | 2.30 | 235 | 30.71 |
| 02/3 | 98.5 | 99.9 | 1762 | 76.20 | 761 | 2.31 | 243 | 31.89 | 08/3 | 98.7 | 99.9 | 1757 | 76.51 | 764 | 2.30 | 237 | 30.98 |
| 03/1 | 98.5 | 99.8 | 1711 | 76.20 | 760 | 2.25 | 233 | 30.58 | 09/1 | 98.5 | 99.7 | 1744 | 76.20 | 760 | 2.30 | 234 | 30.71 |
| 03/2 | 98.5 | 99.9 | 1741 | 76.20 | 761 | 2.29 | 241 | 31.63 | 09/2 | 98.5 | 99.9 | 1740 | 76.20 | 761 | 2.29 | 238 | 31.23 |
| 03/3 | 98.6 | 99.6 | 1768 | 76.36 | 761 | 2.32 | 248 | 32.48 | 09/3 | 98.5 | 100.0 | 1760 | 76.20 | 762 | 2.31 | 243 | 31.89 |
| 04/1 | 98.5 | 99.9 | 1731 | 76.20 | 761 | 2.27 | 237 | 31.10 | 10/1 | 98.5 | 99.6 | 1754 | 76.20 | 759 | 2.31 | 239 | 31.36 |
| 04/2 | 98.5 | 99.9 | 1755 | 76.20 | 761 | 2.31 | 240 | 31.50 | 10/2 | 98.5 | 99.8 | 1742 | 76.20 | 760 | 2.29 | 245 | 32.15 |
| 04/3 | 98.5 | 99.8 | 1779 | 76.20 | 760 | 2.34 | 240 | 31.50 | 10/3 | 98.5 | 99.8 | 1748 | 76.20 | 760 | 2.30 | 248 | 32.55 |
| 05/1 | 98.5 | 99.9 | 1717 | 76.20 | 761 | 2.26 | 234 | 30.71 | 11/1 | 98.6 | 99.7 | 1741 | 76.36 | 761 | 2.29 | 237 | 31.04 |
| 05/2 | 98.5 | 99.9 | 1745 | 76.20 | 761 | 2.29 | 240 | 31.50 | 11/2 | 98.6 | 99.7 | 1747 | 76.36 | 761 | 2.29 | 232 | 30.38 |
| 05/3 | 98.5 | 99.9 | 1769 | 76.20 | 761 | 2.32 | 242 | 31.76 | 11/3 | 98.6 | 99.9 | 1750 | 76.36 | 763 | 2.29 | 238 | 31.17 |
| 06/1 | 98.5 | 99.9 | 1716 | 76.20 | 761 | 2.25 | 240 | 31.50 | 12/1 | 98.5 | 99.9 | 1766 | 76.20 | 761 | 2.32 | 240 | 31.50 |
| 06/2 | 98.5 | 99.9 | 1741 | 76.20 | 761 | 2.29 | 242 | 31.76 | 12/2 | 98.5 | 99.8 | 1754 | 76.20 | 760 | 2.31 | 243 | 31.89 |
| 06/3 | 98.5 | 99.9 | 1762 | 76.20 | 761 | 2.31 | 245 | 32.15 | 12/3 | 98.5 | 100.1 | 1750 | 76.20 | 763 | 2.29 | 245 | 32.15 |
Standard [19] states that due to drilling, which undoubtedly can slightly damage the core’s material, the strengths of core samples determined in-situ are usually lower than the strengths of the standard samples. For this reason, it is allowed to use a correction factor of 0.85, understood as a ratio of the in-situ characteristic compressive strength to the characteristic compressive strength determined on the standard samples. As a result, the concrete compressive strength values coming directly from strength tests are increased.
The mean compressive strength of the concrete, determined on the 18 samples taken perpendicularly to the top surface of the slab, amounted to fm(18),is = 31.45 MPa (the minimum value fis,lowest = 30.58 MPa). The mean standard deviation amounted to s = 0.49 MPa. The coefficient k1 = 1.48 was assumed. The characteristic compressive strength of the concrete in the structure (fck,is) was determined on the basis of standard [13], from the condition: fck,is = min(fm(18),is − k1 × s; fis,lowest + 4) = (30.72 MPa, 34.58 MPa). Thus the characteristic cube compressive strength of the concrete determined on samples taken perpendicularly to the top surface of the slab amounted to fck,is = fck,is,cube = 30.72 MPa.
The mean compressive strength of the concrete determined on the 18 samples taken parallel with the top surface of the slab amounted to fm(18),is = 31.11 MPa (the minimum value fis,lowest = 29.60 MPa). The mean standard deviation amounted to s = 0.81 MPa. The characteristic cube compressive strength of the concrete, determined on the samples taken parallel with the top surface of the slab, amounted to fck,is = (29.91 MPa, 33.60 MPa) = 29.91 MPa. The cube strength determined on the samples taken parallel to the top surface of the slab was 3% lower than the strength determined on the samples taken perpendicularly to the top surface of the slab. This confirms the observation that the strength of core samples drilled out horizontally is lower (on average by 8% [12,13]) than that of core samples drilled out vertically.
On the basis of the obtained fck,is,cube values, the actual strength class of the concrete in the structure is estimated to be fck,is,cube = 30.72 MPa and 29.91 MPa, respectively. When the correction factor of 0.85 is applied, this gives the concrete strength class respectively fck,is,cube = 36.1 MPa and 35.2 MPa, which corresponds to at least concrete class C25/30.
2.3. Scaling of Correlation Curve
On the basis of the measurements, the mean longitudinal ultrasonic wave passage velocities CL were correlated with the mean compressive concrete strengths fis. When calculating the mean longitudinal ultrasonic wave passage velocity CL, the values from the areas of disturbances near the ends of the samples were rejected. The correlation curve was scaled according to the procedure described in standard [13] (version 2). In accordance with [16], a hypothetical base regression curve for ordinary concrete, i.e., fCL,b = 2.39CL2 − 7.06CL + 4.2 for CL = 2.4–5.0 km/s, was adopted. Then, the differences δf between experimental compressive strength fis and the strength obtained from base curve fCL, as well as the mean value δfm(n) of the differences and standard deviation s were determined. The shift of the base correlation curve was calculated from the relation Δf = δfm(n) − k1·s for coefficient k1 = 1.48 [13]. Ultimately, the corrected correlation curve fCL = fCL,b + Δf has the form fCL = 2.39CL2 − 7.06CL + 25.09. The obtained curve only slightly differs from the curves determined separately for samples 01 ÷ 06 and 07–12.
The obtained correlation was evaluated using two accuracy characteristics, i.e., the correlation coefficient η > 0.75 and the mean square relative deviation νk ≤ 12 ≤ %. The correlation coefficient amounted to:
| η = [0.25 × ∑(fCL,I − fCL(36),ν)2]1/2 ÷ [0.25 × ∑(fis − fm(36),is)2]1/2 η = [0.25 × 15.34]1/2 ÷ [0.25 × 16.33]1/2 = 0.97 > 0.75 |
(1) |
and the mean square relative deviation to:
| νk = 100 × {[1/(n − 1)] × ∑[(fCL,i − fis)/fCL,i]2}1/2 νk = 100 × [(1/35)] × 0.12555]1/2 = 5.99% < 12%. |
(2) |
Thus, it can be said that a good correlation between the mean longitudinal ultrasonic wave passage velocities CL and the mean concrete compressive strengths fis was obtained.
The class of the concrete in the structure was determined on the basis of the concrete compressive strength values obtained from the correlation curve fCL = 2.39 × CL2 − 7.06CL + 25.09 for the mean longitudinal ultrasonic wave passage velocities CL. The mean compressive strength of the concrete determined using the ultrasonic method amounted to fCL(36),is = 29.80 MPa (minimal fCL,is,lowest = 28.80 MPa) and the mean standard deviation to s = 0.66 MPa. Hence, the characteristic compressive strength of the concrete amounted to fck,is = fck,is,cube ≤ (29,80 – 1.48 × 0.66, 28.80 + 4) = (28.82 MPa, 32.80 MPa) = 28.82 MPa. When the correction factor of 0.85 was taken into account, concrete class C25/30 was obtained. The concrete class determined on the basis of the compressive strength values obtained from the correlation curve confirmed the destructively determined class of the concrete.
3. Analysis of Test Results
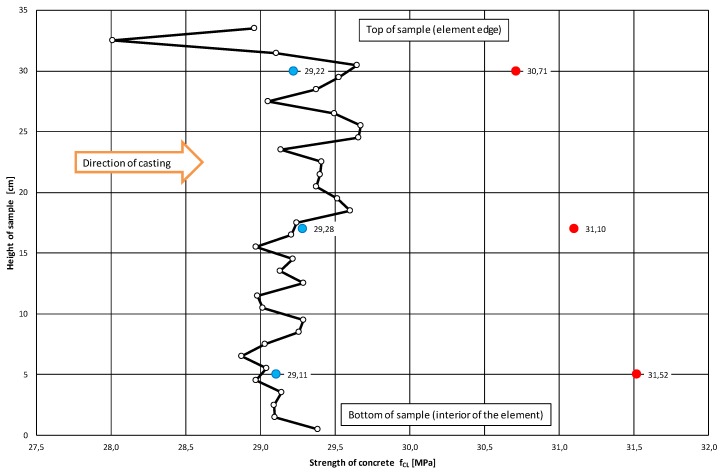
With the corrected correlation curve: fCL = 2.39·CL2 − 7.06·CL + 25.09, it was possible to trace the variation of the compressive strength of the concrete along the height of the analyzed samples. Figure 10 and Figure 11 show the variations of concrete compressive strength along the height of the core samples respectively, and perpendicular to (Figure 10) and parallel with (Figure 11) the top surface of the slab. Further, the results of the destructive tests and the averaged results of the ultrasonic tests for the particular samples with a height h = 100 mm are included in the diagrams.
Figure 10.
The mean distribution of compressive strength fCL of concrete along the height of the sample taken perpendicularly to top surface of slab. The diagram includes the mean concrete strengths for the top, middle and bottom samples, obtained from ultrasonic tests (marked blue) and destructive tests (marked red).
Figure 11.
The mean distribution of compressive strength fCL of concrete along the height of the sample taken parallel with the top surface of the slab. The diagram includes the mean concrete strengths for the top, middle and bottom samples obtained from ultrasonic tests (marked blue) and destructive tests (marked red).
An analysis of the concrete compressive strength values for the particular samples 01–06 (Figure 10) taken perpendicularly to the top plane of the slab indeed showed a slight increase (by 3%) in this strength in the sample’s lower part relative to its upper part. A similar phenomenon (also an increase by approximately 2.6%) was observed for samples 07–12 (Figure 11) taken parallel with the top plane of the slab. However, it should be noted that the compressive strength values were strongly averaged for the samples and included the effect of various factors connected with the destructive test itself.
The averaged compressive strength results obtained from the ultrasonic measurements showed (Figure 10 and Figure 11), however, that there was no increase in the compressive strength of the concrete along the height of the sample. This applies to the samples taken both perpendicularly to and parallel with the top plane of the slab.
The ultrasonic tests indicate that the variation in the compressive strength of concrete along the height of the sample is minimal and random. It can even be considered as negligible. The obtained results do not corroborate Stawiski’s theses [1,2,3], but confirm the results reported in [7,8,9,10].
The decreases in the compressive strength of the concrete occurring at the ends of the samples taken perpendicularly to the top plane of the slab (samples 01–06) and at the edge constituting the side edge of the slab for the samples taken parallel with the top plane of the slab (samples 07–12) were found to be interesting.
4. Conclusions
The following conclusions emerge from the investigations of the compressive strength of concrete carried out on core samples taken perpendicularly to and parallel with the top surface of the approximately 35 cm thick element, using different testing methods (the ultrasonic method and the destructive method):
The concrete compressive strength destructively determined along the height of the placed layer of concrete changed slightly (by 3%—samples 01–06) with a depth below the top surface of the element. The averaged concrete strength determined on the basis of the ultrasonic tests of the same samples did not vary across the thickness of the analyzed slab.
The obtained compressive strength increments across the thickness of the placed layer of concrete do not corroborate Stawiski’s theses [1,2,3], but confirm the results reported in, [7,8,9]. Therefore, there can be agreement with Neville’s statement [10] that the slight increase in concrete compressive strength with depth below the top surface is a natural thing and need not to be taken into account in the evaluation of the strength of concrete in the structure.
The concrete compressive strength determined on core samples only slightly depends on the depth of where the sample came from (provided the ingredients of the concrete do not segregate as it is being placed and compacted).
The use of the ultrasonic method for testing concrete with point-contact exponential probes showed the variation in concrete strength along the height of the core sample could be quite accurately evaluated and areas of lower quality concrete could be indicated. This was mainly from the thick layer (approximately 30–40 mm) extending from the top edge and the thick layer (approximately 30–50 mm) extending from the bottom edge of the samples 01–06 taken perpendicularly to the upper plane of the element. Further, from the thick layer (approximately 20–80 mm) extending from the edge constituting the side plane of the slab for the samples taken parallel with the top plane of the element (samples 07–12). The decline in the strength of the concrete in the upper part of samples 01–06 is caused by the bleeding of water from the concrete mixture (the bleeding phenomenon [17]) and the sedimentation of the latter. While in the lower part of the samples, it is due to the improper vibration of the placed layer of concrete mixture. The decrease in concrete strength at the side edge of the slab in the case of samples 07–12 can be due to the wall effect [9,10].
From the point of view of the assessment of the concrete structure, supplementary tests on the slab in the future should be carried out using ultrasonic tomography [20,21].
The ultrasonic method of testing concrete by means of point-contact exponential probes enables the accurate assessment of the quality of concrete (the segregation of concrete components, porosity, density, strength, etc.) along the height of a core sample drilled out perpendicularly to the placed layer.
Funding
This research received no external funding.
Conflicts of Interest
The authors declare no conflicts of interest.
References
- 1.Stawiski B. Ultrasonic Testing of Concrete and Mortar Using Point Probes. Wroclaw University of Technology; Wroclaw, Poland: 2009. p. 154. (In Polish) [Google Scholar]
- 2.Stawiski B. The heterogeneity of mechanical properties of concrete in formed constructions horizontally. Arch. Civ. Mech. Eng. 2012;12:90–94. doi: 10.1016/j.acme.2012.03.006. [DOI] [Google Scholar]
- 3.Stawiski B. Concrete strength gradients in industrial floors. Mater. Bud. 2017;543:22–24. (In Polish) [Google Scholar]
- 4.Hoła J., Sadowski L., Hoła A.M. The effect of failure to comply with technological and technical requirements on the condition of newly built cement mortar floors. Proc. Inst. Mech. Eng. Part L J. Mater. Des. Appl. 2019;233:268–275. doi: 10.1177/1464420718799378. [DOI] [Google Scholar]
- 5.Petersons N. Should standard cube test specimens be replaced by test specimens taken from structures? Mater. Struct. 1968;1:425–435. doi: 10.1007/BF02473740. [DOI] [Google Scholar]
- 6.Dąbrowski K., Stachurski W., Zieliński J.L. Concrete Constructions. Wydawnictwo Arkady; Warsaw, Poland: 1982. (In Polish) [Google Scholar]
- 7.Yuan R.L., Ragab M., Hill R.E., Cook J.E. Evaluation of core strength in high-strength concrete. Concr. Int. 1991;13:30–34. [Google Scholar]
- 8.Suprenant B.A. Core Strength Variation of in-Place Concrete. The Aberdeen Group; Boston, MA, USA: 1995. [Google Scholar]
- 9.Neville A. Core tests: Easy to perform, not easy to interpret. Concr. Int. 2001;11:59–68. [Google Scholar]
- 10.Watanabe S., Hishikawa K., Kamae K., Namiki S. Study on estimation of compressive strength of concrete in structure using ultrasonic method. J. Struct. Constr. Eng. 2016;81:191–198. doi: 10.3130/aijs.81.191. [DOI] [Google Scholar]
- 11.Neville A.M. Properties of Concrete. Polski Cement Sp. z o.o.; Cracow, Poland: 2000. (In Polish) [Google Scholar]
- 12.PN-EN 12390-2:2011 . Testing Hardened Concrete. Part 2. Making and Curing Specimens for Strength Tests. Polish Committee for Standardization; Warsaw, Poland: 2011. [Google Scholar]
- 13.PN-EN 13791:2008 . Assessment of In-Situ Compressive Strength in Structures and Precast Concrete Components. Polish Committee for Standardization; Warsaw, Poland: 2008. [Google Scholar]
- 14.PN-EN 12390-3:2011 . Testing Hardened Concrete. Part 3: Compressive Strength of Test Specimens. Polish Committee for Standardization; Warsaw, Poland: 2008. [Google Scholar]
- 15.Gudra T., Stawiski B. Non-destructive strength characterization of concrete using surface waves. NDT E Int. 2000;33:1–6. doi: 10.1016/S0963-8695(99)00028-6. [DOI] [Google Scholar]
- 16.Drobiec L., Jasiński R., Piekarczyk A. Diagnosis of Reinforced Concrete Structures. Methodology, Field Tests, Laboratory Tests of Concrete and Steel. Wydawnictwo Naukowe PWN; Warsaw, Poland: 2010. (In Polish) [Google Scholar]
- 17.Soshiroda T. Properties of Fresh Concrete. Taylor & Francis; Mild Park, UK: 1990. Effects of bleeding and segregation on the internal structure of hardened concrete; pp. 225–232. [Google Scholar]
- 18.PN-EN 12390-4:2001 . Testing Hardened Concrete. Part 4: Compressive Strength. Specification for Testing Machines. Polish Committee for Standardization; Warsaw, Poland: 2001. [Google Scholar]
- 19.PN-EN 12504-1:2011 . Testing Concrete in Structures. Part 1: Cored Specimens. Taking, Examining and Testing in Compression. Polish Committee for Standardization; Warsaw, Poland: 2011. [Google Scholar]
- 20.Schabowicz K. Ultrasonic tomography—The latest nondestructive technique for testing concrete members—Description, test methodology, application example. Arch. Civ. Mech. Eng. 2014;14:295–303. doi: 10.1016/j.acme.2013.10.006. [DOI] [Google Scholar]
- 21.Schabowicz K., Suvorov V.A. Nondestructive testing of a bottom surface and construction of its profile by ultrasonic tomography. Russ. J. Nondestruct. Test. 2014;50:109–119. doi: 10.1134/S1061830914020089. [DOI] [Google Scholar]