Dynamic Protonation Dramatically Affects the Membrane Permeability of Drug-like Molecules

Abstract

Permeability (P_m) across biological membranes is of fundamental importance and a key factor in drug absorption, distribution and development. Although the majority of drugs will be charged at some point during oral delivery, our understanding of membrane permeation by charged species is limited. The canonical model assumes that only neutral molecules partition into and passively permeate across membranes, but there is mounting evidence that these processes are also facile for certain charged species. However, it is unknown whether such ionizable permeants dynamically neutralize at the membrane surface or permeate in their charged form. To probe protonation-coupled permeation in atomic detail, we herein apply continuous constant-pH molecular dynamics along with free energy sampling to study the permeation of a weak base propranolol (PPL), and evaluate the impact of including dynamic protonation on P_m. The simulations reveal that PPL dynamically neutralizes at the lipid-tail interface, which dramatically influences the permeation free energy landscape and explains why the conventional model overestimates the assigned intrinsic permeability. We demonstrate how fixed-charge-state simulations can account for this effect, and propose a revised model that better describes pH-coupled partitioning and permeation. Our results demonstrate how dynamic changes in protonation state may play a critical role in the permeation of ionizable molecules, including pharmaceuticals and drug-like molecules, thus requiring a revision of the standard picture.

Graphical Abstract

INTRODUCTION

Permeation across biomembranes, the barriers that enable the compartmentalization and localization essential to life,¹ is generally a prerequisite for the effective delivery of pharmaceuticals to target sites. Thus, permeability (P_m) is an essential pharmacological property to assess in preclinical drug discovery.² It is often measured in vitro with cell-based assays (Caco-2³ and MDCK⁴) or the parallel artificial membrane permeability assay (PAMPA).⁵ More than 60% of the human pharmaceuticals contain at least one ionizable group^6–7 and ionize to varying magnitudes in response to the microenvironment pH, depending on the ionization constant (pK_a), which in turn affects their in vivo distribution and availability to affect biological reactions. Given the considerable pH gradient along the gastrointestinal tract,⁸ a significant proportion of these ionizable compounds are charged at one point or another during delivery to their target destination. Therefore, knowledge of the titration behavior of a pharmaceutical or drug-like molecule is essential to predict and optimize its pharmacokinetic profile.^9–10

The pH-partition hypothesis states that only the neutral form of an ionizable molecule can diffuse across lipophilic membranes.^11–12 In analogy to the Henderson-Hasselbalch equation, the pH-dependent apparent or effective permeability coefficient $\left(P_{\mathrm{m}}^{\mathrm{e}}\right)$ (for a monoprotic base) is expressed in the form:⁸

$$P_{\mathrm{m}}^{\mathrm{e}}=\frac{P_{\text{m}}^0}{1+{10}^{\text{p}{K}_{\text{a}}^{\text{aq}}-\text{pH}}}$$ (1)

where $\text{p}{K}_{\text{a}}^{\text{aq}}$ is the aqueous pK_a and $P_{\text{m}}^0$ is the P_m of the neutral form, or the “intrinsic” P_m. Yet, a growing list of studies has revealed violations of the pH-partition hypothesis. For example, it has been shown that the permanently charged quaternary ammonium compounds are able to permeate cell membranes.^13–14 The permeation in the charged form has also been reported for ionizable compounds, albeit at a much slower rate than the neutral form,^15–17 and the abundance of the charged species can make this a significant contribution to the total measured P_m.¹⁸ In the context of cells, molecules can permeate via multiple pathways (e.g., paracellular, transcellular carrier-mediated, and passive transport),¹⁹ making it difficult to evaluate unambiguously the contribution of the passive diffusion of charged species. Palm et al. compared Caco-2 permeation of two weak bases with approximately the same $\text{p}{K}_{\text{a}}^{\text{aq}}$ and found that the one with a larger molecular weight has a higher $P_{\mathrm{m}}^{\mathrm{e}}$ for the charged form,¹⁸_‘²⁰ suggesting that the transcellular passive diffusion is nonnegligible because it is believed that larger molecules are not expected to permeate substantially via the paracellular route.¹⁵ Violation of the pH-partition hypothesis was also observed in PAMPA and liposomal assays, where the paracellular and active transport are absent. Fischer et al. measured the PAMPA $P_{\mathrm{m}}^{\mathrm{e}}$ of 12 quaternary ammonium compounds and found that the permanently charged molecules can have high passive transmembrane permeability, potentially due to the charge delocalization.²¹ Regev et al. measured liposomal $P_{\mathrm{m}}^{\mathrm{e}}$ of doxorubicin, a primary amine with a $\text{p}{K}_{\text{a}}^{\text{aq}}$ of 9.7, and found the increase in $P_{\mathrm{m}}^{\mathrm{e}}$ at high pH is significantly smaller than what eq. 1 predicted,²² but the origin of the deviation is not clear. All of these violations suggest that the neutral permeant assumed in eq. 1 is oversimplified.

The pH-partition hypothesis of course neglects the possibility that an ionizable molecule might neutralize at the membrane surface before entry. This behavior would provide an obvious explanation for the violations of the pH-partition hypothesis described above. However, this explanation has been challenged by several results showing that amphiphilic molecules migrate into membranes in their charged form^23–31 and accumulate in the lipid headgroup and glycerol regions.^32–33 The ionizable sites of the bound amphiphiles position in the water-accessible³⁴ headgroup region^35–36 where they stabilize their ionized states by forming a surface ion pair with the zwitterionic lipid headgroups.^{8, 2} (Note here the interesting difference between bases, which associate lower in the membrane with the negatively charged phosphates, and acids, which are closer to the membrane-water interface associating with the positively charged trimethylammonium groups.^{8, 29}) By contrast, the neutralized species were observed in a deeper location,^23–24 suggesting that a titration event could indeed occur somewhere inside the membrane during permeation. Two questions naturally arise: Can ionized molecules really neutralize before permeating through membranes as these results suggest, and, if so, where exactly within the membrane does this neutralization occur? The solution to these questions requires insight into permeant-membrane interactions at the atomic level, which is not easily accessible in experiments.

Molecular dynamics (MD) simulations can, with care, predict P_m and offer atomistic details of permeation. Since the work by Marrink and Berendsen,³⁴ MD simulations have become a well-established method to study permeation.^37–49 On the basis of the pH-partition hypothesis, simulations are mostly performed with neutralized permeants from which $P_{\text{m}}^0$ can be computed. However, using a fixed ionization state, whether it is charged or neutral, faces several limitations. First, the choice of the neutral form overlooks the above-mentioned evidence supporting membrane partitioning of charged species. Second, it assumes an unperturbed pK_a inside the membrane, and thus no dynamic titration during permeation, despite the physically expected quite significant pK_a shift. Given the observed location-dependent ionization state for some molecules,²³ it could be more realistic to have multiple simulations at fixed locations along the permeation route with varying ionization states, which can be assigned by comparing the aqueous phase pH and the pK_a at the location of interest. The pK_a inside membrane might then be predicted by Poisson-Boltzmann (PB) electrostatic calculations, for example, the PB solver APBSmem.⁵⁰ However, this approach often gives large errors in pK_a predictions for highly buried ionizable sites.⁵¹ Moreover, calculations based on static structures suffer from the loss of the coupling between protonation and conformation equilibria, which has been shown to be essential in titration-coupled processes.^52–54 Alternatively, the pK_a inside a membrane can be derived from permeation free energies for both ionization states.⁵⁵ Yet, this approach is computationally expensive and fails to offer a dynamic picture of the protonation in response to varying the membrane environment. Consequently, better modeling of membrane permeation of ionizable molecules requires a tool enabling dynamic protonation in response to the changes in microenvironment.

The past decade has witnessed the development of constant-pH molecular dynamics (pHMD), a class of methods that determines the charge states of ionizable sites during the conformational dynamics. On the basis of how charge states are represented and sampled, pHMD methods are categorized into the discrete pHMD (DpHMD) and continuous pHMD (CpHMD). In DpHMD,⁵⁶ a standard MD simulation is periodically interrupted to update charge states using Monte Carlo methods, which determines if the randomly generated new charge states should be accepted or rejected based on the Metropolis criteria.⁵⁷ MD simulation will resume with the new charge states if accepted or old charge states if rejected. Different DpHMD formulations mostly vary in the solvation model for evaluating the energetics of titration^{56, 58–62}. Unlike DpHMD, CpHMD allows charge states to fluctuate in MD simulations by adding titration as an extra degree of freedom to an extended Hamiltonian.⁶³ In the CpHMD formalism^64–65 based on the λ-dynamics approach for free energy calculations,⁶⁶ each ionizable site is assigned a λ, which is bounded between 0 (protonated form) and 1 (deprotonated form), and propagates simultaneously with spatial coordinates. The pHMD methods have succeeded in modeling a variety of pH-coupled systems.^67–68 Using DpHMD, the Machuqueiro lab characterized the pK_a values of ionizable amino acid at the membrane surface⁶⁹ and the pK_a shift (ΔpK_a) in the pHLIP peptides during membrane insertion.⁷⁰ The Roux lab investigated the translocation of a pentapeptide AADAA across a POPC (1-palmitoyl-2-oleoyl-sn-glycero-3-phosphocholine) membrane and found that the pK_a of the central aspartic acid was shifted to 7.3 at the center of the membrane.⁷¹ The Brooks lab first applied CpHMD with an implicit description of solvent and membrane to study the protonation-linked structural rearrangement in rhodopsin activation.⁷² Later, the Brooks lab investigated the protonation equilibria of titratable amino acids in transmembrane helices using explicit-solvent CpHMD⁷³ and found that the neutral states of those titratable residues were significantly favored in the context of membranes.⁷⁴ More recently, the Shen lab developed hybrid-solvent CpHMD,⁷⁵ which combines the accuracy of explicit solvent and membrane for conformational sampling with the efficiency of the implicit-solvent model for calculating the solvation free energies or pK_a values of ionizable sites. This method has been validated in various pH-coupled systems, such as assembly and phase transition of fatty acids^76–77 as well as polysaccharides,⁷⁸ inhibitor-enzyme binding,^79–81 and protein folding.⁸² With membrane description enabled in the underlying implicit-solvent model,⁸³ the hybrid-solvent CpHMD has also afforded mechanistic insight into the proton-driven activation of the Na⁺/H⁺ antiporter NhaA,⁸³ the influenza A M2 proton channel,⁸⁴ and the multidrug efflux pump AcrB.⁸⁵

Inspired by these successes, we combined the membrane-enabled hybrid-solvent CpHMD technique^{75, 83} with the umbrella sampling (US) method⁸⁶ to investigate the permeation of a cationic amphiphile propranolol (PPL, Figure 1A) from the β-blocker family through a POPC bilayer. β-Blockers are a class of drugs primarily used to treat cardiovascular diseases. As the prototype of β-blockers, propranolol is listed by the World Health Organization as one of the most effective and safe medicines needed in a health system.⁸⁷ The POPC lipids were chosen because they were used to determine P_m in the liposomal fluorescence assay.^88–89 The liposome P_m measures the rate of passive permeation and is thus directly comparable to the MD-based P_m.⁹⁰ We sought to answer the following questions: (1) how does PPL adjust its ionization state in response to the changing environment during permeation; and (2) how does the dynamic protonation of PPL impact its permeability? We find that PPL enters the POPC bilayer in the charged form but neutralizes at the lipid-tail interface and then permeates in its neutral form. Including dynamic protonation in the MD free energy sampling simulations not only confirms the permeation pathway but also predicts P_m with very high accuracy (~1–3-fold the experimental measures). Accordingly, we propose a revised pH-partition model to better describe the ionization-linked permeation process. The major findings of the present work yield new insight into the membrane permeation process at the atomic level, and the overall methodology will be applicable to other important ionization-coupled permeation phenomena.

Figure 1. PPL structure and permeation thermodynamics.

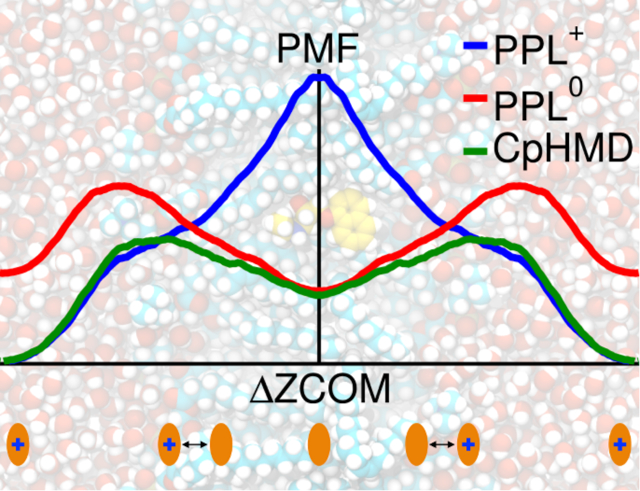
(A) Structure of PPL. The ionizable amine group is indicated by a blue circle. Experimental⁹¹ $\text{p}{K}_{\text{a}}^{\text{aq}}$ is shown below. (B) Potential of mean force (PMF) as a function of the distance from bilayer center ΔZCOM, which is defined as the projection of the center-of-mass (COM) distance between the heavy atoms of PPL and POPC on the bilayer normal (Z-axis). Charged (PPL⁺) and CpHMD (at pH 7) PMFs were set to zero in aqueous phase, while the neutral (PPL⁰) PMF was upshifted by the deprotonation free energy in aqueous phase at pH 7 (3.55 kcal/mol). Error bars indicate the standard deviations from block analysis and are displayed every 3 Å for clarity (see Figure S1 for details). A schematic POPC molecule is aligned below with its components colored and mapped along the membrane normal according to Figure 2B.

RESULTS AND DISCUSSION

Dynamic Protonation Delineates the Permeation Pathway.

We first investigated the thermodynamic impact of including dynamic titration on the PPL permeation. Figure 1B shows the potentials of mean force (PMFs), that is, free energy profiles, as a function of the distance from bilayer center ΔZCOM in both the charged (PPL⁺) and the neutral (PPL⁰) forms, as well as under the CpHMD environment. PPL⁺ experiences a large barrier of ~12 kcal/mol as it approaches the bilayer center, consistent with other computational work,^{55, 92–98} whereas PPL⁰ experiences a moderate barrier of ~3 kcal/mol around the POPC headgroups (ΔZCOM of 15–20 Å) where the charge and density of the system maximize.^{34, 55} The PPL⁺ and PPL⁰ PMFs experience a crossover at ΔZCOM of 12 Å. Consistent with previous computational studies,^{92, 95–98} this suggests a permeation path of the least effort (or lowest free energy), that is, entering the bilayer as PPL⁺ but permeating through it as PPL⁰. With CpHMD turned on, PPL was able to adjust its charge state in response to microenvironment. In the permeation direction, the CpHMD PMF coincides with PPL⁺ first until ΔZCOM of ~12 Å and then with PPL⁰ within statistical error. In other words, CpHMD reproduced the lowest free energy path of the combined fixed-ionization-state PMFs, a commonly adopted approach without rigorous validation.^{92, 95–98} Note that CpHMD delineated the path at one-half the computational cost.

PPL Neutralizes at the Hydrophobic Boundary of the POPC Bilayer.

Next, we examined the molecular details of the change of the PPL charge state during permeation. Although bound to biomembranes as a charged species,^26–31 it has been shown that the PPL trans-liposome partition increases the internal pH, suggesting permeation in the neutral form and reprotonation inside liposome.⁹⁹ To verify this, we plotted the free energy surface (FES) as a function of ΔZCOM and the titration coordinate of PPL (λ^PPL) in Figure 2A. λ^PPL describes the transition between the protonated (λ = 0, PPL⁺) and deprotonated states (λ = 1, PPL⁰). The distribution probabilities of various components of the system were computed on the basis of the scattering density profile model¹⁰⁰ and are displayed in Figure 2B. Figures 2A and B indicate PPL⁺ exists from water down to the carbonyl and glycerol groups of the lipids (ΔZCOM ≈ 16.5 Å), but a neutral PPL⁰ exists inside the lipid hydrocarbon tails (ΔZCOM ≤ 10.5 Å), in good agreement with the experiments (around physiological pH amphiphiles accumulate in the lipid headgroup and glycerol region in their charged forms^23–31 but become neutral once they migrate to deeper locations^23–24) and the lowest free energy permeation path (Figure 1B). Notably, PPL titrates (λ^PPL changes from 0 to 1) in the ΔZCOM range of 10.5–16.5 Å, where the bilayer transitions from the carbonyl and glycerol groups (CG) to the hydrocarbon tails (HC). That is to say, PPL neutralizes at the hydrophobic boundary of the bilayer (14.6 Å, D_C in Figure 2B) rather than at the bilayer surface. This finding, although contradicting the common notion that neutralization would occur at the water-membrane interface (20.7 Å, D_B/2 in Figure 2B), is not especially surprising. Considering the zwitterionic nature of the lipid headgroups, the membrane-bound ionized amphiphiles likely have their hydrophilic groups pointing toward and salt-bridging with the lipid headgroups, while their hydrophobic moieties are buried in the vicinity of carbonyl and glycerol groups or hydrocarbon tail region to reduce their water contact (i.e., they likely follow the surface ion pair model⁸). PPL also binds to membranes in the same fashion, as can be inferred from experiments.^{32, 35–36} In our simulations, PPL partitioned into the POPC bilayer with its naphthalene moiety localized close to the carbonyl and glycerol region and amine group ion-paired with the phosphate groups (Figure S2). Such a partition pattern emerges approximately between the hydrophobic boundary (D_C) and the bilayer surface (D_B/2), which agrees with the experiments and lends support to the surface ion pair model. Furthermore, amphiphiles bound to the carbonyl and glycerol groups of a bilayer³³ are likely to titrate there given the limited water accessibility in the region³⁴ (Figures 2B–D). In fact, Boulanger et al. studied the pH-dependent interactions of two local anesthetics (bases) with eggPC vesicles using NMR.^23–24 They found the ionized form at low pH preferred binding close to the membrane surface, while the neutral form at high pH strongly bound to a deeper site. This indicates an amphiphile can adjust its location inside the membrane (moving up and down) in response to varying ionization states induced by pH, leading to a “pH piston hypothesis”.²⁹ In fact, the position-dependent ionization state revealed in this work agrees with Boulanger et al.’s findings as well as previous CpHMD results,^{69–71, 74} supporting the “pH piston hypothesis”.

Figure 2. Change of PPL protonation state during permeation.

(A) Free energy surface (FES) as a function of insertion depth (ΔZCOM) and the titration coordinate of PPL (λ^PPL) at pH 7. Contour lines are displayed every 1 k_BT. The dashed vertical white lines mark where PPL titrates (λ^PPL changes from 0, i.e., PPL⁺, to 1, i.e., PPL⁰). The average standard deviation from block analysis is 0.3 k_BT. (B) Volume probability distributions for the density profile model¹⁰⁰ of components of the POPC bilayer. Componentization follows Kucerka et al.:¹⁰¹ water (H₂O, brown), phosphate and CH₂CH₂N part of choline (PCN, red), 3xCH₃ part of choline (CholCH₃, green), carbonyl and glycerol groups (CG, black), methine group (CH, cyan), methylene groups (CH₂, blue), methyl groups (CH₃, magenta), and the total hydrocarbon tails (HC, orange). Data collected from the umbrella sampling run at pH 7 with PPL at ΔZCOM = 13.5 Å. The vertical purple and dark green dot-dashed lines mark the hydrophobic boundary (D_C) and bilayer surface (D_B/2),¹⁰⁰ respectively. The background gray box highlights the titration region (same below). A schematic POPC molecule is mapped along the bilayer normal in the center with components colored accordingly. (C) The ΔpK_a of PPL as a function of ΔZCOM. Black line was calculated from the PMFs in Figure 1B with error bars calculated from error propagation. Red circles represent the pK_a values calculated from CpHMD with error bars calculated from three independent runs. (D) The hydration number of PPL, counted as the number of water oxygen atoms within 3.5 Å of the PPL titrating nitrogen (Figure 1A) as a function of ΔZCOM. Error bars indicate the standard deviations from block analysis and are shown every 3 Å for clarity.

From the permeation PMFs of PPL⁺ and PPL⁰ (Figure 1B), location-specific pK_a can be computed using a thermodynamic cycle⁵⁵ (Figure S3). As displayed in Figure 2C, the PPL pK_a smoothly decreases from 9.5 in water to ~1 in the bilayer center. This is expected because it is energetically unfavorable to charge a base like PPL inside membranes. Remarkably, the pK_a reaches 7 at ΔZCOM ≈ 12 Å, indicating this is where POPC-bound PPL deprotonates at pH 7, which agrees with Figure 2A. Using the Hill equation (eq. S3), we also calculated pK_a values from the membrane-enabled hybrid-solvent CpHMD combined with the pH-based replica-exchange (pHREX) protocol,^{75, 83} which has been shown to predict pK_a values with high accuracy and faster convergence.⁶⁸ Consistent with the PMF pK_a, the CpHMD pK_a (Figure 2C) decreases as permeation proceeds and reaches 7 at ΔZCOM ≈ 14 Å. The PMF and CpHMD pK_a values agree well at ΔZCOM ≥ 15 Å. Below 15 Å, the CpHMD pK_a values are shifted to lower values until they plateau at ΔZCOM ≤ 10 Å. The second plateau absent in the PMF pK_a maps to the hydrocarbon tail region of the bilayer (Figure 2B) and exhibits a pK_a of 2.5–3. The decrease in pK_a occurs in the ΔZCOM range of ~10–19.5 Å, consistent with the titration region revealed by Figure 2A. Moreover, the Hill coefficients calculated from the pH-REX CpHMD simulations are in the range of 0.7–0.8 inside the bilayer, an indication of anticooperativity in titration.¹⁰².

The pK_a inside a membrane can be inferred from the partition coefficient (logP)^{8, 103} (Figure S4). The pK_a of PPL has been reported to downshift by 0.5–1 pH units with respect to $\text{p}{K}_{\text{a}}^{\text{aq}}$ based on the PC liposome logP values (Table S2). The same ΔpK_a was reported by the cosolvent method using methanol/water mixture,¹⁰⁴ whose dielectric constant is close to that of the lipid headgroups.⁸ The PPL-POPC interactions involved in the PPL membrane partition pattern^35–36 discussed above become the most obvious in the ΔZCOM range of 14–16 Å (Figure S2). The predicted ΔpK_a at this location is 1–2 pH units, which is ~1 pH units larger than the experimental values. This can be attributed to the absent ionic effect in our simulations, because the presence of salt enhances the partition of the charged species by attenuating their electrostatic repulsion on the bilayer surface,¹⁰⁵ leading to a higher pK_a. As indicated in Table S2, ΔpK_a increases by ~0.5 pH units with the ionic strength reduced from 0.23 to 0.15 M. A further increase in ΔpK_a is thus expected in the absence of salt. Note some membranes listed in Table S2 are anionic, which should increase the partitioning of PPL⁺ and result in a reduced Δ pK_a as compared to the charge-neutral PC lipids. Octanol logP values are also frequently reported. Although octanol/water mixtures may resemble the bilayer surface better than the hydrophobic interior given their ability to form hydrogen bonds, the hydrocarbon chains create a solvent-inaccessible region with a dielectric constant similar to that of the bilayer interior.8 Thus, we reason that the octanol logP values could give some insights into the permeant pK_a around the entry of the hydrocarbon tail region (ΔZCOM of 7–14 Å). The octanol ΔpK_a has been measured to be ~3 pH units in the 0.15 M background ionic strength (Table S2) and may further increase by ~1.5 pH units for weak bases under marginal ionic strength.⁸ We then expect a ΔpK_a of ~4.5 pH units, which falls within the range of the calculated ΔpK_a values within the region. Collectively, the predicted ΔpK_a values are in agreement with the experiments, underling the robustness of our calculations.

We now consider the discrepancy between the two sets of calculated ΔpK_a values. As compared to the PMF approach, CpHMD predicted larger ΔpK_a values in the ΔZCOM range of 10–14 Å (Figure 2C). Because of the limited solvent accessibility in the region (Figures 2B,D), there exists an equilibrium between the “open” and “closed” microstates, each coupled to a separate protonation equilibrium. Also, the calculated pK_a values in Figure 2C are macroscopic values that incorporate the microscopic pK_a values of both conformations.^52–53 Because the effect of discrete water molecules around a buried site cannot be captured by the underlying generalized-Born (GB) model of the hybrid-solvent CpHMD, the error in the closed-state pK_a may be nonnegligible.⁶⁸ It has been shown that the closed-state pK_a for a buried lysine could be underestimated by ~1.5 pH units, resulting in an overestimated ΔpK_a by the same amount.⁵² Bearing this in mind, the CpHMD pK^a values agree well with the PMF ΔpK_a values in the lipid headgroup, carbonyl, and glycerol regions. The deviation becomes more significant in the hydrocarbon tail region that is devoid of water. On the CpHMD side, it is known that ΔpK_a is overestimated for deeply buried titration sites due to the inaccuracy in the desolvation penalties by GB models.^{51, 106} This systematic error is less likely to improve with longer simulations. On the PMF side, the error in the PPL⁺ PMF maximizes around the bilayer center (Figure 1B), possibly due to the water defect (Figure 2D), and propagates uncertainty into the resulting ΔpK_a values (Figure 2C). Although prolonged simulation may alleviate this error, within the present simulation length we have achieved qualitative agreement between the two sets of ΔpK_a in this region. We note that CpHMD is much less computationally demanding as compared to the US method (pK_a values converged within 3 ns per replica, Figure S5) and therefore a more suitable tool for predicting membrane pK_a values.

To better understand the cause of the PPL deprotonation inside the POPC bilayer, we quantified its hydration by computing a hydration number, which was defined as the number of water oxygen atoms within 3.5 Å (first solvation shell) of the PPL titrating nitrogen (Figure 1A), as a function of ΔZCOM (Figure 2D). Similar to the change in charge state (Figure 2A), the resulting hydration profile from the CpHMD coincides with that for PPL⁺ until the entry of the bilayer, decreases within the titration region, and eventually matches that for PPL⁰ near the bilayer center. This suggests that the decrease in pK_a is induced by the decreased hydration. To verify this, we investigated the correlation between λ^PPL and PPL hydration in the US window of ΔZCOM = 13.5 Å, the center of the titration region (Figure 2A) where the CpHMD pK_a decreases to ~7 (Figure 2C). As plotted in Figure S6A, λ^PPL mostly samples values close to 1 (PPL⁰) when PPL is dehydrated (blue bars), but flips to values close to 0 (PPL⁺) when there are two or more water molecules within the first solvation shell (red bars). This anticorrelation (reduced solvation favors proton release) is also reflected in the Hill coefficients (0.7–0.8). Interestingly, if there is only one water molecule around, PPL⁰ and PPL⁺ are equally sampled (brown bars), an indication of titration event. A similar finding was reported by Bonhenry et al., who studied the permeation of a lysine analogue through a POPC bilayer by constructing the 2D-PMF as a function of ΔZCOM as well as hydration and found the charge state was tightly coupled to the hydration number.⁹⁷ These results demonstrate that neutralization inside membrane correlates with desolvation.

A related question then arises: Where does the proton go after titrating off PPL⁺? In the absence of titratable lipid headgroups the obvious acceptor is water; but is that water also buried in the membrane, or can the proton diffuse into bulk? Note that the hydration number displayed in Figure 2D only quantifies the number of “solvating” water molecules in close proximity to the PPL titrating nitrogen and is insufficient to gauge the water distribution inside membrane or its connection to bulk water. Therefore, we counted the number of water molecules along the permeation pathway (N_water), which was defined as the number of water oxygen atoms in 1-Å slices within a radius of 6.5 Å (the second solvation shell of the PPL titrating nitrogen) centered along the membrane normal. Figure S6C shows that water molecules are always present above the hydrophobic boundary (D_C) and occasionally go below D_C. By adding N_water cumulatively, we find that on average there is always one water molecule slightly penetrating the POPC tails (4 Å below D_C), and above D_C the cumulative N_water increases rapidly (Figure S6D). These results suggest that there are ample water molecules distributed along the permeation pathway to pass the proton from PPL⁺ to bulk water (Figure S6B). It is of course possible that titratable lipid headgroups could be an intermediate resting place for the proton. Such intermediates would influence the rate or proton transport out to bulk, but would not influence the pK_a of PPL unless they were close enough to alter the relative stability of the charged/neutral drug, a perturbation that CpHMD would capture. No such headgroups were included in these simulations. Furthermore, on the basis of the assumption that the permeation profiles in both bilayer leaflets are symmetric, our results indicate there exists another titration region in the opposite leaflet (ΔZCOM from −16.5 to −10.5 Å) where PPL⁰ can reprotonate by the influx proton from the opposite bulk waters before leaving the POPC bilayer as PPL⁺, consistent with the experimental observation that PPL permeation increased the pH inside liposomes.⁹⁹

PPL Permeates in a “Triple-Flip” Manner.

Next, we examined the molecular-scale conformational dynamics of PPL during permeation. To describe the orientation of PPL with respect to the bilayer, we defined an order parameter θ, the angle between the principal axis of the bulky rigid PPL naphthalene moiety $\left({\overrightarrow{r}}_{\text{naph}}\right)$ and the bilayer normal $\left({\overrightarrow{r}}_{\text{Z}}\right)$ (Figure 3A). The FES as a function of ΔZCOM and θ (Figure 3B) shows that the naphthalene moiety adopts an orientation parallel to the membrane surface (θ of ~90°) beyond the hydrocarbon tail region (ΔZCOM ≥ 14.5 Å). In the ΔZCOM range of 5.5–14.5 Å, the naphthalene moiety prefers to align with ${\overrightarrow{r}}_{\text{Z}}$ (θ of ~30). This observation is consistent with a result reported by Badaoui et al. using an equivalent orientational reaction coordinate.¹⁰⁷ This region is where the cis double bonds in the POPC tails reside (CH in Figure 2B) and the contacts of naphthalene moiety with CH maximize (Figure S7). The same interaction was reported for amlodipine using NM R.¹⁰⁸ No preference in the orientation is seen near the bilayer center (ΔZCOM ≤ 5.5 Å), as suggested by a broad minimum. Furthermore, the aliphatic chain can stay either above or below the naphthalene moiety when PPL is located near the bilayer center but favors the former conformation if PPL moves closer to water (Figure S8). Given the symmetry in bilayer, in the context of the entire bilayer, these results collectively suggest one flip of the naphthalene moiety around the lipid CH region in each bilayer leaflet and a flip of the aliphatic chain near the bilayer center (Figure S9), which is consistent with the “triple-flip” mechanism reported in our previous work.⁴⁶ However, the accuracy of the permeation PMF as a function of ΔZCOM may be affected by hidden barriers involving permeant orientation,¹⁰⁹ which might be solved by adding an orientational collective variable. However, because the free energy barrier related to PPL orientation is marginal (< 1 k_BT, Figure 3B), using ΔZCOM alone should not cause significant error.

Figure 3. Change of PPL conformation during permeation.

(A) Variables characterizing PPL conformation. Angle θ is the angle between the principal axis of PPL naphthalene moiety $\left({\overrightarrow{r}}_{\text{naph}}\right)$ and the bilayer normal $\left({\overrightarrow{r}}_{\text{Z}}\right)$. Angle ϕ is the angle between ${\overrightarrow{r}}_{\text{naph}}$ and the vector pointing from PPL alkoxy oxygen (O1) to titrating nitrogen (N1) ${\overrightarrow{r}}_{01-\text{N}1\cdot }{\text{d}}_{01-\text{N}1}$ is the distance between O1 and N1. (B) FES as a function of ΔZCOM and θ. Contour lines are displayed every 0.5 k_BT. The vertical white dashed lines mark the region where the PPL naphthalene moiety is aligned with ${\overrightarrow{r}}_{\text{Z}}$ The average standard deviation from block analysis is 0.3 k_BT. (C) FES as a function of ϕ and d_O1−N1. Contour lines are displayed every 0.5 k_BT. Representative structures of the extended, twisted, and collapsed states are shown. Data collected from all US windows at pH 7. The average standard deviation from block analysis is 0.1 k_BT. (D) Occupancies of the E (orange), T (red), and C (blue) states as a function of ΔZCOM. See Figure S10 for state definitions.

The PPL conformation can be characterized by another two order parameters: ϕ and d_O1−N1 (Figure 3A).ϕ is the angle between the PPL aliphatic chain and naphthalene moiety and quantifies the intrapermeant orientation. d_O1−N1 is the distance between atoms O1 and N1 and describes the extension of the aliphatic chain. The FES as a function of ϕ and d_O1−N1 reveals three local minima (Figure 3C). We refer to the three states as the extended (E), twisted (T), and collapsed (C) states. In the E state centered at ϕ = 65° and d_O1−N1 = 5 Å, the dihedrals along the PPL aliphatic chain are in trans rotamers, resulting in an extended conformation. Separated from the E state by a barrier of 2 kcal/mol is the T state centered at ϕ = 95° and d_O1−N1 = 4.3 Å. The T state has a “twisted” aliphatic chain as compared to the E state. The conformation with the aliphatic chain bending toward the naphthalene ring corresponds to the C state, which is separated from the T state by a 3 kcal/mol barrier and centered at ϕ = 110° and d_O1−N1 = 2.8 Å. The occupancies of the three states display a ΔZCOM-dependent manner (Figure 3D). The T state (Figure 3D, red line) is dominant in water and about twice sampled as the C state (Figure 3D, blue line). After PPL enters the bilayer and deprotonates, the populations of the T and C states start to decrease and increase, respectively. Near the bilayer center, the C state is slightly favored over the T state. The E state (Figure 3D, orange line), although marginally sampled, becomes more populated inside the bilayer.

Dynamic Protonation Dramatically Affects the Membrane Permeability.

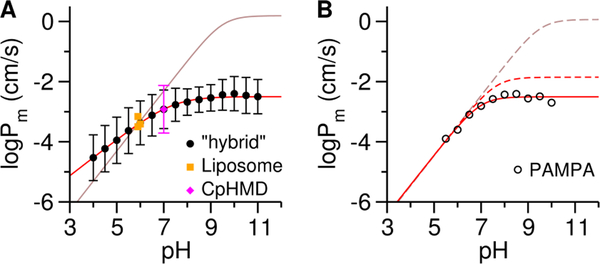
Finally, we examined the impact of including protonation dynamics on P_m prediction. The P_m values were calculated on the basis of the solubility-diffusion model from the permeation PMF and calculated local diffusivity D (eq. S4–S6).^{34, 47} We note that overall there is no significant difference in D values of PPL⁺ and PPL⁰ (Figure S11). Yet around the bilayer center PPL⁰ is twice as diffusive as PPL⁺ due to the increased population of the C state (Figure 3D). Because of the presence of a large energy barrier, P_m of PPL⁺ (−7.38, log scale, Table S3) is ~30,000 times smaller than that of PPL⁰ (−2.50), indicating protonation of PPL strongly disfavors permeation and likewise deprotonation greatly enhances it. This observation agrees with other computation studies^{92, 94} and the pH-dependent PAMPA P_m profile of PPL¹¹⁰ (Figure 4B). As compared to PPL⁰, CpHMD at pH 7 predicts a P_m ≈ 60% smaller (−2.92, magenta rhombus in Figure 4A), because PPL⁰ underestimates the permeation barrier of bilayer entry relative to its stability in bulk (Figure 1B). The P_m from liposomal fluorescence assay⁸⁸ (Figure 4A, orange squares) offers a good experimental measure of P^m solely due to passive diffusion.⁹⁰ The CpHMD P^m (−2.92) is 3.8-fold (or 2.8 times larger than) the P_m reported by Eyer et al. 88 (−3.50 log scale, Table S3), and 1.7-fold (or 0.74 times larger than) the P_m determined with a larger liposome (−3.16).⁸⁹ The origin of the deviation with experiment is mainly 2-fold: (1) the experiments were conducted at pH 6 while the CpHMD was run at pH 7; and (2) the titration dynamics in CpHMD are accelerated due to the underlying biasing potential, which effectively removes the aqueous titration free energy barrier.^64–65 In other words, CpHMD predicts P_m at the limit where the titration of a permeant becomes instantaneous relative to its permeation, which is the case when liposomes with sufficiently large radii (20 μm or larger) are used.¹¹¹ Thus, the P_m determined with a largest liposome to date (−3.16 was measured with a liposome radius of 239 nm)⁸⁹ is approaching this limit, and is expected to increase closer to our calculated value (−2.92) in even larger liposomes that better reflect most biological membranes.

Figure 4. pH-dependent permeability coefficient (P_m) of PPL.

(A) Comparison of the calculated “hybrid” (●) and experimental liposome P_m values (orange ■).^88–89 The red solid line is the best fit of the “hybrid” P_m to eq. 2. The brown solid line was predicted from eq. 1. (B) Reassessment of the experimental PAMPA P_m values .¹¹⁰ The solid red line is the best fit to eq. S10. The dashed lines refer to the $P_{\mathrm{m}}^{\mathrm{e}}$ corrected for the aqueous boundary layer (brown based on eq. 1 and red based on eq. 2, check the Supporting Information section 3.2 for details).

Following the lowest free energy path along the PMF (Figure 1B), we calculated P_m from PPL⁺ and PPL⁰.⁹² This “hybrid” P_m at pH 7 (−2.92) agrees with the CpHMD prediction and the value at pH 6 (−3.37) is approximately consistent with the liposome P_m values (Table S3). Consequently, we were able to build the pH-dependent profile (Figure 4A, ●) that qualitatively agrees with experiments (Figure 4B, ), i.e., $P_{\mathrm{m}}^{\mathrm{e}}$ increases at low pH values and plateaus at high pH values, resulting in a hyperbolic shape. Note that the PAMPA $P_{\mathrm{m}}^{\mathrm{e}}$ includes the aqueous boundary layer contribution ($P_{\text{m}}^{\text{ABL}}$, check Supporting Information section 3.2 for details). $P_{\text{m}}^{\text{ABL}}$, is absent in liposome method and simulations, making their P_m measures directly comparable. The calculated P_m values cannot fit to eq. 1 (Figure 4A, brown line), an indication of the violation of the pH partition hypothesis. However, by incorporating the Hill coefficient n and replacing $\text{p}{K}_{\text{a}}^{\text{aq}}$ with effective $\text{p}{K}_{\text{a}}\left(\text{p}{K}_{\text{a}}^{\text{e}}\right)$:

$$P_{\mathrm{m}}^{\mathrm{e}}=\frac{P_{\text{m}}^0}{1+{10}^{\text{n}\left(\text{p}{K}_{\mathrm{a}}^{\mathrm{e}}-\text{pH}\right)}}$$ (2)

the best fit (n = 0.61 and $\text{p}{K}_{\mathrm{a}}^{\mathrm{e}}=7.35$) was obtained (Figure 4A, red line). As revealed in this work, the assumption that only PPL⁰ contributes to $P_{\mathrm{m}}^{\mathrm{e}}$ underestimates the amount of “effective” PPL⁰ because PPL⁺ deprotonates at the hydrophobic boundary rather than at the bilayer surface (Figures 2A,B). Thus, a reduced pK_a is expected with the “effective” PPL⁰ taken into account. An n < 1 indicates anticooperativity in the pH-dependent permeation. Note that n is not just a scaling factor. Instead, it reflects the sensitivity of P_m to the change in pH, which originates from how the microenvironment affects the titration of the membrane-associated permeant (check Supporting Information section 3.3 for details). Using the revised model (eq. S10) based on eq. 2, we reassessed the experimental PAMPA $P_{\mathrm{m}}^{\mathrm{e}}$ values. The best fit (Figure 4B, red solid line) gives a $\text{p}{K}_{\text{a}}^{\text{e}}$ of 7.63 and an n of 0.99. The PAMPA $\text{p}{K}_{\text{a}}^{\text{e}}$ agrees with the “hybrid” prediction, but an n close to 1 indicates little anticooperativity, which can be attributed to the high ionic strength in the experiment. The new $P_{\text{m}}^0\left(-1.85\right)$ is 80 times smaller than the value (0.06) overestimated by the original model (eq. S9) based on eq. 1 and is 4.5-fold (3.5 times larger than) the “hybrid” value (−2.50), potentially due to the different composition in the PAMPA membrane.^{28, 112} Note that we cannot predict $P_{\text{m}}^0$ from the liposome measures at single pH using eq. 2, but we feel the pH-dependent $P_{\mathrm{m}}^{\mathrm{e}}$ profile should also have a $\text{p}{K}_{\text{a}}^{\text{e}}$ close to 7 as well as an n close to 1 under 0.2 M ionic strength, leading to an estimated $P_{\text{m}}^0$ around −2.

CONCLUDING DISCUSSION

We have employed CpHMD simulations combined with US free energy surface analysis to study the ionization-coupled permeation of the drug molecule PPL through a POPC lipid bilayer. Two fixed ionization states, PPL⁺ and PPL⁰, were also simulated for comparison. With dynamic protonation included, CpHMD largely reproduced the permeation path with the lowest free energy (Figure 1B) as delineated by jointly considering PPL⁺ and PPL⁰.^{92, 95–98} Contrary to what is commonly assumed, the permeation pathway indicates PPL ionizes inside membrane rather than at the surface, which was confirmed by the ΔZCOM-dependent pK_a values (Figure 2C). The pK_a values, either calculated from the permeation PMFs or predicted by pH-REX CpHMD, are in line with the experimental values inferred from logP values (Table S2). As the molecule partitions into the lipid bilayer, the ΔpK_a monotonically increases due to the desolvation effect (Figures 2D and S6A), in agreement with previous fixed-ionization-state^{55, 93} and CpHMD^{69–71, 74} simulations. Notably, the pK_a reaches 7 (the aqueous pH) in the neighborhood of the carbonyl and glycerol moieties of the lipids (Figures 2B,C). These results are consistent with the partitioning experiments showing that amphiphilic molecules migrate into membrane as ionized species but permeate in the neutral form,^{23–31, 99} and lend support to the surface ion pair model⁸ as well as the “pH piston hypothesis”, which states that ionizable molecules associate with either the zwitterionic headgroups or glycerols/lipid tails depending on their ionization states.²⁹ These findings do not necessarily override the pH-partition hypothesis (PPL still permeates through the lipid tail region in its neutral form) but instead point to an essential complementary understanding (that dynamic neutralization increases the permeable molecules to include some proportion of those charged in solution).

Collectively, this work demonstrates the importance of considering dynamic protonation in permeation studies. With dynamic protonation, CpHMD was able to predict passive diffusion P_m within a factor of ~1–3 of the experimental measurements^88–89 (Table S3). Following the lowest free energy permeation PMF from the fixed-ionization-state PMFs, which was validated by CpHMD simulations, we were able to delineate P_m as a function of pH. The resulting pH-dependent P_m values qualitatively agree with those determined by PAMPA,¹¹⁰ with some expected deviation due to the different PAMPA membrane composition.^{28, 112} Importantly, we found the pH-dependent P_m profile cannot be described by the conventional model (eq. 1). By assuming that dynamic ionization does not occur at the membrane surface, the conventional model underestimates the number of permeable molecules, which results in an overestimated $P_{\text{m}}^0$ based on the experimentally measured $P_{\mathrm{m}}^{\mathrm{e}}$. To better describe membrane partitioning and permeation of ionizable molecules, we modified eq. 1, adding the Hill coefficient n to reflect the cooperativity/anticooperativity in the proton-coupled permeation and replacing $\text{p}{K}_{\text{a}}^{\text{aq}}$ by $\text{p}{K}_{\text{a}}^{\text{e}}$ to reflect the molecule’s ionizability inside the membrane (eq. 2). This modified model helps explain violations of the pH-partition hypothesis. For example, Regev et al. found that the trans-liposome permeation of doxorubicin increased only 2-fold from pH 7.4 to 9.7, while eq. 1 predicted an increase of 68-fold.²² Such a deviation is generally treated as a clue of charged species permeation. However, eq. 2 estimates an increase of ~1.4 with $\text{p}{K}_{\text{a}}^{\text{e}}$ of 7 and n of 1 (0.2 M ionic strength), suggesting that this “pseudo-violation” originates from the assumption that only neutral species in solution permeate.

Note that our findings should be generalized with a degree of caution. Weak acids are also likely to bind the POPC bilayer as charged species, neutralize (protonate) before diffusing through lipid tails, and reionize (deprotonate) upon leaving lipid tails in the opposite leaflet. So the surface ion pair model⁸ and the “pH piston hypothesis”²⁹ should still apply. However, slight deviations are anticipated, given the association of acids with the positively charged trimethylammonium groups at the POPC-water interface, as opposed to the negatively charged and more buried phosphates to which weak bases associate. In the permeation direction, a continual increase of acid pK_a is expected, and the protonation occurs where the pK_a upshifts to 7 (aqueous pH). This has been demonstrated for acidic amino acids using the fixed-ionization-state⁵⁵ and DpHMD simulations.^69–71 Given that only neutral species diffuse through lipid tails, acid $P_{\mathrm{m}}^{\mathrm{e}}$ plateaus at low pH values and then decreases at high pH values.^{8, 112–114} Yet an overestimated $P_{\text{m}}^0$ by the conventional model (eqs. 1 and S9) is also anticipated by ignoring the membrane-associated charged species. Ampholytes (with at least one acidic and one basic group) are interesting cases because they may adopt three or more charge states. However, how an ampholyte adjusts its charge state during passive permeation cannot be readily deduced from the current work and may be the focus of future work. In the case of a strong acid with very low or a base with very high $\text{p}{K}_{\text{a}}^{\text{aq}}$, its pK_a inside the membrane may not be adequately shifted for it to neutralize. For instance, the p ^ a of arginine reduces from 12.5 in water to ~7 at the center of bilayer,^{55, 115–118} indicating that arginine could remain ionized inside membrane. Also, the permeation may be facilitated via transient water pores,¹¹⁹ which can stabilize the ionized species and reduce the permeation barrier for a charged molecule.^{93, 118, 120–121} For weak acids or bases, however, our findings suggest that they are likely to permeate biomembranes as neutral species, which is consistent with other computational studies^{55, 69, 71, 74, 93, 116} and explains why human drugs typically have a $\text{p}{K}_{\text{a}}^{\text{aq}}$ in the range of 3.5−10.5.⁶ Also note that this study was conducted using a pure bilayer with a single drug. Introducing ionizable membrane “impurities” may charge the bilayer and affect drug permeation. It has been demonstrated that the presence of anionic lipids or fatty acids increases the partition (Table S2) and permeability (Table S4) of PPL in PC liposomes,^{26, 28, 30, 114, 122} which is not surprising given the strong electrostatic attraction between PPL⁺ and the negatively charged membranes. If multiple PPLs were present in close proximity, their ionizations are likely to be coupled in an anticooperative manner due to the great electrostatic repulsion between PPL⁺s inside low-dielectric membrane. Smaller pK_a and the Hill coefficient are thus expected, which have been captured in the titration of the fatty acid^76–77 and polysaccharide⁷⁸ aggregates by CpHMD. However, how multiple drugs would affect the MD-based P_m prediction is not clear at this stage since the impact may be nonadditive.¹²³

As compared to the fixed-ionization-state simulations, pHMD not only offers a more realistic scenario via real-time update of charge states during permeation, but also is computationally more efficient, especially in the presence of ampholytic and/or multiple permeants whose charge states inside membranes may vary in an unpredictable way. There are, however, a few limitations of using pHMD in the permeation studies, such as the absence of explicit proton transfer because pHMD models the relative deprotonation free energy or ΔpK_a of an ionizable site relative to that in bulk. In reality, when deprotonation occurs inside membranes, an excess proton will be accepted by the surrounding water molecules and transported via Grotthuss shuttling¹²⁴ to bulk water or to an ionizable headgroup. However, the only way in which this process would alter the drug’s permeation free energy profile is if the protonated waters or lipid headgroup were attractive, long-lived, and in close enough proximity to shift the drug’s local stability in a kinetically significant way (i.e., to interact strongly with and thereby slow the drug’s permeation). This is unlikely in the presented scenario given the weak interaction expected between the neutral drug and such protonated groups. On the basis of our previous work on carbon nanotubes, a good mimic of permeation pathway inside membranes, using the multiscale reactive molecular dynamics (MS-RMD) simulations, an excess proton (H₃O⁺ delocalized over multiple waters) inside a hydrophobic environment is highly energetically unfavorable.¹²⁵ Given ample waters connecting the titrating drug and bulk water (Figures S6B-D), the release of H₃O⁺ to bulk should be fast. Thus, we estimated P_m on the basis of the assumption that the titration is fast relative to permeation. A more rigorous assessment can be done by adding explicit proton transfer using the MS-RMD technique developed in our group,^126–130 which may be the focus of future work. Because the phosphate groups of POPC lipids can occasionally ion-pair with the titrating nitrogen atom of PPL⁺ (Figure S2), it will be interesting to see how these moieties are involved in the excess proton’s release to bulk water. Turning on titration for lipids^131–132 and the drug-bound waters¹³³ in pHMD will be an additional way to explore their local stability. For the present work, above pH 4 (as in Figure 4A), the POPC lipids remain zwitterionic,^{25, 134} limiting their influence to an intermediate during proton release to bulk water.

Another limitation of pHMD is that it ignores the role of altered pH in local microenvironments, such as at the membrane surface.^135–140 Turning to the hybrid-solvent CpHMD method applied in this work, we note that it systematically overestimates the ΔpK_a inside membranes, especially near the center of membrane (Figure 2C), due to the underestimated desolvation of the underlying GB model.^{51, 75} However, on the basis of the previous CpHMD studies,^{51–52, 54, 75} we believe the direction of the ΔpK_a is robust. In addition, hybrid-solvent CpHMD neglects any explicit interactions with ions.⁷⁵ Although the current work is free of ions and not influenced by them, hybrid-solvent CpHMD may be less suitable to study the ion pair permeation.¹⁴¹ Other potential limitations, as in all MD-based permeation studies, are the need to include bilayer asymmetry, membrane undulations, and polarizability. The simulations in this study on a symmetric bilayer focused sampling on one-half of the system (one leaflet) and generated the permeation profile for the exiting leaflet by mirroring those in the entering leaflet (eq. S4). Given the symmetric lipid distribution and converged sampling (Figure S16), the permeation profiles should be symmetric.¹⁴² The reaction coordinate in this study (ΔZCOM) was defined on the basis of all lipid molecules, which might lead to size-dependent permeation PMFs due to the artifact from membrane undulations.¹⁰⁹ However, given the system size chosen in this work (26 lipids per leaflet), undulation should be insignificant, and the resulting PMFs should be relatively size insensitive.⁴² Molecules experience significant change in dielectric environment during permeation,⁸ so the description by polarizable force fields may be more physically realistic. Yet using nonpolarizable force fields should not impair the major findings of this work, that is, dynamic protonation should be considered when investigating facile membrane permeation of ionizable molecules, which is also supported by a study including the polarization effects.⁹⁶

In summary, we have applied CpHMD, where protonation and conformation equilibria are dynamically coupled, to study the permeation of an ionizable drug PPL through a POPC lipid bilayer. We find that PPL migrates into the bilayer in the charged form and deprotonates at the hydrophobic boundary, and that by involving dynamic protonation the P_m was predicted with high accuracy. What is commonly assumed in the permeation studies is that an ionizable molecule must neutralize in solution before membrane partitioning. As such, the amount of the “effective” permeant is underestimated, leading to an overestimated intrinsic P_m. We have also presented a revised model to better describe the pH-dependent partitioning and permeation. While the generality of our findings awaits further testing on a larger data set, these findings demonstrate how adding dynamic protonation can advance our understanding of the permeation of ionizable pharmaceuticals and drug-like molecules and hence improve the accuracy of MD-based P_m prediction. Last, this work demonstrates the utility of CpHMD in permeation studies.

METHODS

Unless otherwise stated, simulations were conducted and analyzed using the CHARMM package¹⁴³ (version c42b2). Lipids and waters were represented by CHARMM36¹⁴⁴ and CHARMM modified TIP3P model,¹⁴⁵ respectively. PPL was parametrized by CHARMM General Force Field¹⁴⁶ (CGenFF, version 3.0.1) using ParamChem^147–148 (version 1.0.0). The CpHMD parameters for PPL were derived using the protocol described by Lee et al.⁶⁴ A PPL⁰ molecule was inserted in an explicit POPC bilayer with a 15-Å water layer added to the top and bottom. No explicit ions were added. The system was equilibrated in three stages, on the basis of the protocol previously established.⁸⁵ In stage 1, after energy minimization, a 500 ps restrained simulation was performed to relax the system, following the CHARMM-GUI protocol.^149–150 In stage 2, the POPC bilayer was equilibrated for ~100 ns using the GROMACS package¹⁵¹ (version 5.1.4). In stage 3, 21 replicated systems were generated with PPL restrained at various locations along the membrane normal (Z-axis) and equilibrated for 26 ns with titration enabled on PPL at pH 7 using the membrane-enabled hybrid-solvent CpHMD method^{75, 83} implemented in CHARMM. Following the equilibration, production runs were performed at 310 K and 1 atm using the US method.⁸⁶ The equilibrated 21 configurations were further branched into 60 systems with PPL positioned from 0 to 29.5 Å with an interval of 0.5 Å. The reaction coordinate (ΔZCOM) was chosen as the Z-component of the COM distance between the PPL and POPC heavy atoms. US simulations were performed for PPL⁺, PPL⁰, and CpHMD at pH 7. All US windows were run for 70.5 ns. Detailed simulation protocols and additional analyses are given in the Supporting Information.