Asynchronous leaf and cambial phenology in a tree species of the Congo Basin requires space–time conversion of wood traits

Abstract

Background and Aims

Wood traits are increasingly being used to document tree performance. In the Congo Basin, however, weaker seasonality causes asynchrony of wood traits between trees. Here, we monitor growth and phenology data to date the formation of traits.

Methods

For two seasons, leaf and cambial phenology were monitored on four Terminalia superba trees (Mayombe) using cameras, cambial pinning and dendrometers. Subsequently, vessel lumen and parenchyma fractions as well as high-resolution isotopes (δ¹³C/δ¹⁸O) were quantified on the formed rings. All traits were dated and related to weather data.

Key Results

We observed between-tree differences in green-up of 45 d, with trees flushing before and after the rainy season. The lag between green-up and onset of xylem formation was 59 ± 21 d. The xylem growing season lasted 159 ± 17 d with between-tree differences of up to 53 d. Synchronized vessel, parenchyma and δ¹³C profiles were related to each other. Only parenchyma fraction and δ¹³C were correlated to weather variables, whereas the δ¹⁸O pattern showed no trend.

Conclusions

Asynchrony of leaf and cambial phenology complicates correct interpretation of environmental information recorded in wood. An integrated approach including high-resolution measurements of growth, stable isotopes and anatomical features allows exact dating of the formation of traits. This methodology offers a means to explore the asynchrony of growth in a rainforest and contribute to understanding this aspect of forest resilience.

Introduction

Future climate change is expected to push tropical ecosystems into novel, unprecedented states (Holm et al., 2017). Therefore, it is vital to understand how this will affect plant growth (Reyer et al., 2013; Cavaleri et al., 2015) in general and forests specifically, given the enormous value of this natural capital. The majority of forest biomass is stored in the wood and bark of trees (Beeckman, 2016) and studying radial growth is therefore imperative (Steppe et al., 2015) given the uncertainties in tropical tree carbon gain and specific vulnerability to drought (Zuidema et al., 2013; Gebrekirstos et al., 2014). Long-term growth, climate response and ecological information can be read from rings in trees.

In tropical trees, the classic matching of ring widths is often not possible due to discontinuous or wedging rings or unclear ring boundaries (Tarelkin et al., 2016), which often result in many uncertainties regarding growth rate and how these trees embed ecological and climate information into their wood structure. Consequently, there is a clear need for alternative approaches to interpreting and analysing tropical tree rings (Silva et al., 2019). One option is to study wood traits embedded within these rings, to tap into underexplored intra-annual ecological information from the past (Fonti et al., 2010; Battipaglia et al., 2014b; Sass-Klaassen, 2015; Van Camp et al., 2018).

Establishing such high-resolution or intra-annual chronologies (Eckstein, 2004; Rossi and Deslauriers, 2007) of wood traits [which can include elemental (Poussart et al., 2006; Cherubini et al., 2013; Hietz et al., 2015), wood stable isotope (Helle and Schleser, 2004; Verheyden et al., 2004; Poussart et al., 2004; Evans and Schrag, 2004; Fichtler et al., 2010; Managave and Ramesh, 2012) and wood anatomical traits (Fonti et al., 2010; Battipaglia et al., 2010; von Arx et al., 2016)] can reveal climatic control on tree growth and how these traits vary during tree development. Concentrations of cellulose stable isotopes in leaves and wood (δ¹³C, δ¹⁸O) are of particular interest in the tropics, as a proxy for physiological processes such as stomatal conductance, intrinsic water use efficiency (van der Sleen et al., 2014) and source water use. Especially at the intra-annual level, a clear link with weather conditions such as monsoon rains has been reported (Schollaen et al., 2014).

More and more studies in tropical regions are combining isotope analysis and wood anatomical traits to relate physiological processes to hydraulic properties (Li et al., 2011; Battipaglia et al., 2014a). However, weak seasonality causes these traits to be difficult to date. A sound interpretation of growth patterns of tropical trees depends on understanding phenological rhythms (Enquist and Leffler, 2001) at the primary meristem level (leaf phenology) and at the secondary meristem level (cambial phenology). It is therefore essential to study both simultaneously.

Landscape leaf phenology can be studied from satellite images (Ryan et al., 2017) but individual phenology variations are studied using detailed in situ field observations (Borchert et al., 2005; Fu et al., 2015) or digital repeat photography (Sonnentag et al., 2012). Cambial phenology, although more difficult to measure, is archived in the secondary xylem (Beeckman, 2016) via radial growth. Monitoring radial growth at high precision in the tropics (Krepkowski et al., 2010; Trouet et al., 2012; Mendivelso et al., 2016; Spannl et al., 2016; Butz et al., 2017; Raffelsbauer et al., 2019) provides a mechanistic understanding of seasonal wood formation as a function of weather variables.

Phenology varies due to climate (Borchert, 1999; Borchert et al., 2005; Lisi et al., 2008; Yáñez-Espinosa et al., 2010; Fauset et al., 2012) and growth strategies (Couralet et al., 2013; Wolfe and Kursar, 2015) at the species and even individual plant levels (Heinrich and Banks, 2006; Forrest and Miller-Rushing, 2010; Visser et al., 2010). Closely linked to leaf phenology variability, the exact period of tree ring formation within one growing season varies with age, site (Philipson et al., 1971; Rossi et al., 2008), species and between trees.

Fixing intra-annual wood traits on a seasonal time axis is essential to link the physiological mechanisms (Bouriaud et al., 2005; Steppe et al., 2015; Van Camp et al., 2018) to environmental conditions. The intra-annual curve can be used to convert wood traits to the time domain for some tree species in temperate regions (e.g. Wimmer et al., 2002; Bouriaud et al., 2005; Skomarkova et al., 2006; Stangler et al., 2016) but this combinatory approach is missing for species in the Congo Basin.

Given the challenging task of measuring tree performance in the past, there is a clear need to improve knowledge of xylem responses to climate variability in tropical trees. Intra-annual wood traits should be coupled to the growth rhythms which are determined based on leaf and cambial phenology.

This study reports on Terminalia superba, a deciduous tree (Couralet et al., 2013) with good potential for tree-ring analysis and considerable inter- and intra-annual anatomical variation (De Ridder et al., 2013; De Mil et al., 2016), but for which knowledge on the timing of the latter remains elusive.

Here, we hypothesize that the timing of leaf and cambial phenology are related and determine the synchrony of wood cellulose stable isotope δ¹³C and δ¹⁸O and wood anatomical traits. Hence, when these traits are synchronized accordingly by fixing on a time axis through the seasonal growth curve, their common signal could be increased, rather than assessing them in the spatial domain by simple averaging.

MATERIAL AND METHODS

Study site and species

The Luki reserve (5°37.31′S, 13°5.90′E) lies at the southern edge of the Mayombe, which is an Atlantic Coastal forest ranging from Gabon to western DR Congo (Supplementary Data Fig. S1a). The rainy season (average 1188 ± 245 mm) lasts from October to May, yet the dry season is less pronounced due to thick cloud cover and high relative humidity (Lubini, 1997).

Terminalia superba is a widespread pioneer in the Mayombe forest. Its distribution area ranges from Sierra Leone, along the West African coastline, and up to the northern boundary of Angola. The Luki reserve is at the edge of its distribution area. Four trees were selected on different locations around the Nkulapark in the Luki Reserve close to the research station, and monitored from August 2013 to December 2015, covering three season onsets and two full growing seasons. Height (H) and diameter (D) of the study trees and neighbouring trees were measured and crown area was calculated as well. Subsequently, the crowding index was calculated, as a measure of the influence of neighbouring trees (Michelot et al., 2011) (Table 1; Supplementary Data Fig. S2).

Table 1.

General characteristics of the sampled trees,with label (T), Tervuren Wood identification number at the xylarium (Tw), height (H), diameter (D), estimated tree age, projected crown area, crowding index (CI) as calculated by Michelot et al. (2011), and location and coordinates

Tree	Tw	H (m)	D (cm)	Age (years)*	Crown area (m2)	CI (–)	Location	Coordinates
T1	Tw68886	35	47	35	142.5	2.23	Young secondary forest	13.108E, −5.620S
T2	Tw68884	45	69	70	223.2	1.70	Old secondary forest	13.099E, −5.612S
T3	Tw68885	39	65	85	213.6	2.10	Old secondary forest	13.099E, −5.612S
T4	Tw68883	31	83	47	530.0	1.36	Free standing tree, ridge	13.100E, −5.624S

Three-point dendrometers (EcoMatik) were installed on each of the four trees to account for circumferential variation (Supplementary Data Fig. S2d). The dendrometers were installed at 4–5 m height, to reduce the influence of large buttresses. Stem diameter variations (SDVs) were logged every 30 min using ONSETComp HOBOloggers (Onset). Four time-lapse cameras (Wingscapes) (Sonnentag et al., 2012) were installed at 10–20 m height on adjacent trees and directed towards the studied trees for leaf phenology monitoring: two or three pictures per day were taken for two consecutive growing seasons. Time-lapse images were visually analysed and leaf events were classified into defined phenology phases (adapted from Derory et al., 2006). Daily precipitation (p, mm) was measured by the local weather station of Luki, and air temperature (T, °C), relative humidity (RH, %) and solar radiation (R, W m⁻²) were measured and logged with ONSETComp HOBOloggers (U23 and S-LIB-M003 respectively; Onset) near the station with a sampling interval of 30 min. Vapour pressure deficit (VPD) was calculated based on measured T and RH in the plant modelling software PhytoSim (Phyto-IT BVBA).

Cambial pinning

Cambial pinning was performed monthly by inserting a needle that wounds the cambium locally. Sampling was done at 0.4–1 m below the point dendrometers in a staggered pattern around the circumference of the tree (Supplementary Data Fig. S1c). Trees were felled and stem discs were harvested in December 2015. Samples of 5 × 5 × 5 cm³ containing the pinnings were extracted from the discs. All stem discs were given a Tervuren wood (Tw) number for storage in the xylarium. Growth between the previous ring boundary and the cambial wound was measured on fresh samples using a stereo microscope and a Lintab measuring stage in combination with TsapWin software (Rinntech; precision 1: 1000 mm). Relative growth (RG) was then calculated as the distance between the cambial pinning and the previous ring boundary, divided by ring width to cope with the high variability in absolute growth along the circumference (Seo et al., 2007; De Mil et al., 2017).

Vessel lumen fraction, parenchyma fraction and high-resolution isotopes

At the exact position of the point dendrometers, tree rings of the two monitored growing seasons were cut from the stem discs (15 mm × 20 mm), carefully sectioned (Microm HM 440E, thickness 15–25 µm), and cross-sections were stained with safranin and alcian blue to enhance the contrast between the different anatomical components (Jansen et al., 1998).

The cross-sections were imaged on a scanning stage (SCAN 100 × 100, Märzhäuser) with a camera (UC30, Olympus) mounted on a microscope (BX60, Olympus, magnification 5×) and stitched to full images (StreamMotion software, Olympus). Vessel lumina were delineated in ImageJ (Schneider et al., 2012) via manual selection with the magic wand tool, whereas parenchyma was manually indicated using the polygon tool. The vessel lumen fraction and parenchyma area were calculated as a fraction of the total wood cross-sectional area (Supplementary Data Fig. S1e). A pixel-by-pixel radial summation of vessel lumen and parenchyma area was made by dividing by the total width of the section, to obtain fractions. Hence, comparisons with the dendrometer series were made at high spatial resolution.

Intra-annual isotope samples (δ¹³C and δ¹⁸O) were taken adjacent to the point dendrometer positions. A Lintab measuring stage (Rinntech) with a stereomicroscope (SZX 12, Olympus) and a scalpel was used to cut the blocks (15 mm × width of the tree rings) into equal radial slices with an approximate thickness of 0.3 mm to comply with the minimum sample size required for isotope analysis. Cellulose extraction of the radial slices was performed at the ISOFYS Lab, according to a protocol adapted from Wieloch et al. (2011). δ¹³C was measured using an automated nitrogen carbon analyser – solid and liquids (ANCA-SL, SerCon) interfaced with an isotope ratio mass spectrometer (IRMS) (20-20, SerCon), whereas the same slices were used for δ¹⁸O determination using a thermal conversion elemental analyser (Sercon) interfaced with an IRMS (20-20, SerCon). The δ¹³C and δ¹⁸O isotope values are expressed against the international standard Vienna Pee Dee Belemnite and Vienna Standard Mean Ocean Water, respectively. Isotope measurements were not able to be processed from tree T1 over the 2014–2015 growing season.

Isotope and wood anatomical traits were further processed in Matlab r2016b for space–time conversion and statistical analysis. Data are available in Supplementary Data Information S1.

Space/time conversion

Xylem growth onset and cessation were derived from cambial pinning at a monthly resolution. Both onset and cessation were further resolved to a daily time step based on breakpoint correlation analyses (segmented regression) of the SDV series (Supplementary Data Figs S3 and S4).

On some radii of all trees, xylem growth exceeded the measurement range of the dendrometers. Therefore, saturated data were removed and gap-filled using Savitsky-Golay interpolation based on (1) the total tree ring width and (2) cessation dates from the other radii of the same tree which did not show saturation. A smoothed (Savitsky-Golay) curve, eliminating diel variations, was then fitted to the SDV and used to position wood traits on a time axis. All wood trait data were averaged within trees, and synchronized with weather data, followed by resampling to weekly resolution. Pearson correlation analyses were performed between the weekly averaged trait profiles and weather variables.

RESULTS

Leaf phenology

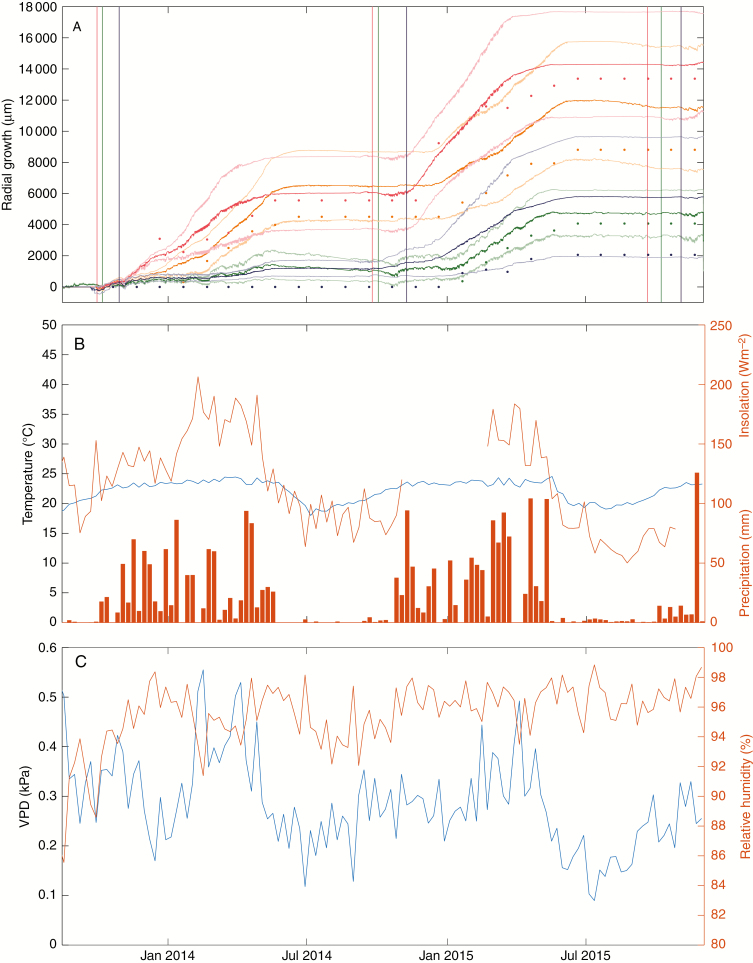
Green-up data are presented in Fig. 1A. Figure 1B includes rainfall data for the end of the dry season and the onset of the following rainy season. Trees show considerable variation in leafing timing up to 45 d (Fig. 1A, Table 2). For all three monitored onsets, flushing occurs either before (T1, T4) or after (T2, T3) the first major rains, except for rain onset in 2015, when all trees flushed before the late onset of rain (Fig. 1B). Unlike leaf flushing, leaf senescence and abscission are not sharply defined in T. superba (Supplementary Data Fig. S5). The full deciduous (i.e. leafless) period is short for all trees, ranging from 5 to 25 d. VPD is lowest in the dry season and peaks during the rainy season (Fig. 1C), when radial growth occurs.

Fig. 1.

Comparison of (A) radial growth, cambial pinning data and leaf onset during two seasons of four mature Terminalia superba trees in the Mayombe region of the Congo Basin, Central Africa (DR Congo) with (B) mean daily temperature, insolation as well as weekly precipitation for the measured period and (C) vapour pressure deficit (VPD) and relative humidity (RH). Gaps are missing data. In A, full lines are raw radius dendrometer data (three per tree), and each colour represents a tree (T1 green, T2 orange, T3 blue and T4 red). Circles at a given month are growth data derived from cambial pinnings. Vertical lines in A represent bud burst dates from the studied trees.

Table 2.

Overview of leaf (Bud burst ± 2 d) and cambial phenology (Onset Xy, ± 5 d) timing (day of the year), the lag between both events and length of the xylem growing season

Sample	2013–2014					2014–2015					2015–2016
	Bud burst	Onset Xy	Lag (d)	End Xy	Xy season (d)	Bud burst	Onset Xy	Lag (d)	End Xy	Xy season (d)	Bud burst	Onset Xy	Lag (d)
T1	280	–	–	–	–	276	11	100	138.0	127	280	–	–
T2	302	361	59	154	158	313	359	46	156.0	162	307	–	–
T3	302	–	–	–	–	313	361	48	157.0	161	307	–	–
T4	273	308	35	120	177	268	325	57	130.0	170	263	330	67

Two trees formed no xylem during the 2013–2014 season (indicated by –) and at the time of felling (December 2015) three out of four trees had not yet formed new xylem (see also Supporting Data Fig. S7).

Cambial phenology

Wood growth is shown in Fig. 1A for all radii of the four trees during two growing seasons. The original RG series are presented in Supplementary Data Fig. S6.

Wood growth starts between November and January for all trees, ranging from 35 to 100 d after bud burst (Table 2). The trees thus start xylem formation well into the wet season. When trees start growing earlier, growth cessation starts earlier in all monitored trees and throughout the monitored seasons. On wet sections taken just after tree felling in December 2015, (non-lignified) wood formation could be observed for the early-onset T4 tree, whereas all the other trees did not yet show cambial activity, which confirms the observed differences (Supplementary Data Fig. S7). The onset is identical for the different radii of a tree (Fig. 1A).

Total xylem growing season length is about 159 ± 17 d and is quite constant except for tree T1 with a shorter season of 127 d.

For trees T1 and T3, a missing ring occurred in the 2013–2014 growing season (Fig. 1A, blue and green triangles). The small net growth observed for some radii of these trees in the SDV series is merely due to water status (Fig. 1A), as well as a small amount of wound-induced xylem formation caused by the dendrometer support, which was confirmed visually on the stem discs (Supplementary Data Fig. S8). In the following growing season, these trees formed a ring on all radii.

Wood trait profiles

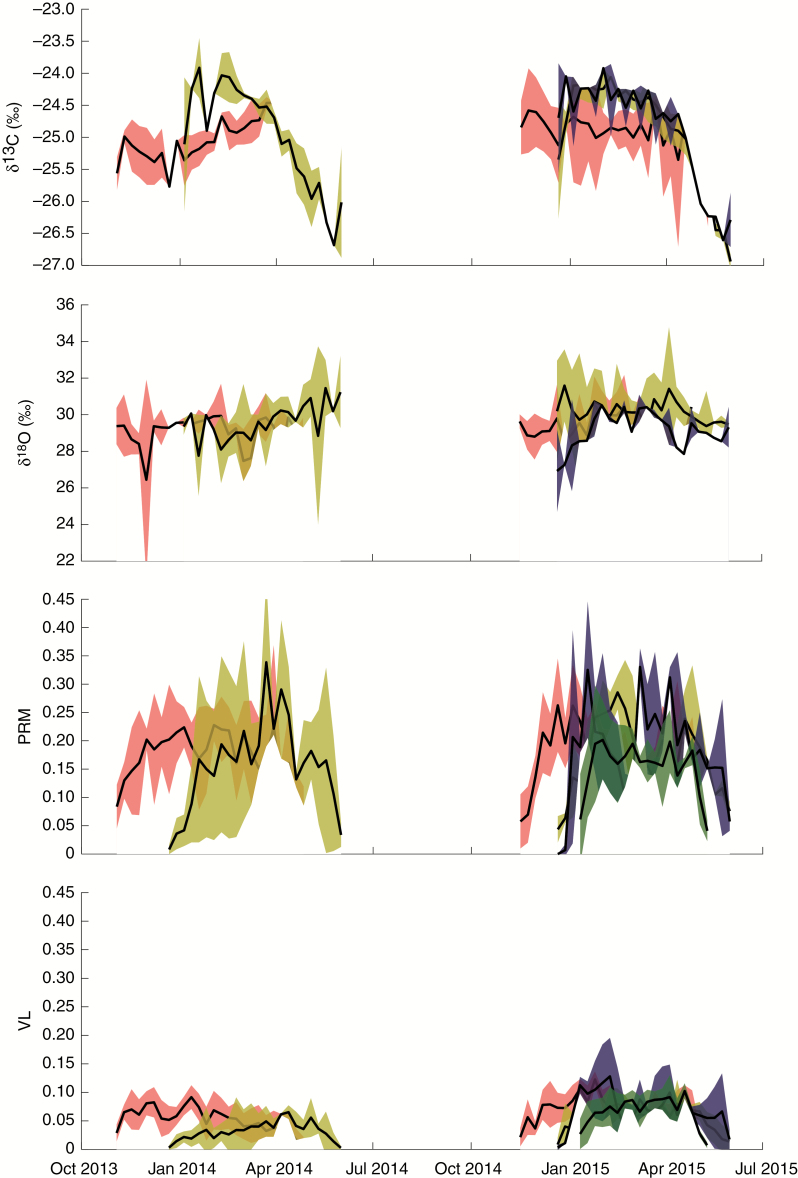
The δ¹³C and δ¹⁸O, vessel lumen and parenchyma profiles (Fig. 2) were positioned on a time axis. The lags in trait values can be seen as xylem onsets and cessations are different.

Fig. 2.

Synchronized and averaged trait profiles of four Terminalia superba trees from Central Africa for (A) cellulose stable δ¹³C pattern, (B) δ¹⁸O pattern, (C) parenchyma fraction (PRM) and (D) vessel lumen fraction (VL). Both fractions are unitless. Each colour represents a tree (T1 green, T2 orange, T3 blue and T4 red) and surface plot shows 1 s.e. Gaps represent the dry seasons. T4 starts developing traits first, while T2 and T3 develop traits further towards the end of the season.

In general, δ¹³C increases at the beginning of the growing season, culminates and begins to decrease around February–March (Fig. 2A) with a minimum in June followed by growth cessation. δ¹³C values range between −27.11 and −23.28 ‰ and the maximum and minimum intra-annual range within one ring is 3.42 ‰ and 1.76 ‰, respectively. The averaged δ¹³C profile is strongly positively correlated with solar radiation, temperature and VPD, and negatively correlated with relative humidity (Table 3). The δ¹⁸O profiles do not show a clear trend for either year in all trees (Fig. 2B), and the values range between 22.59 ‰ and 35.30 ‰ and the maximum and minimum intra-annual range within one ring is 9.24 ‰ and 6.35 ‰, respectively. Parenchyma fractions can locally add up to 45 % of the wood surface, and vessel lumen fractions reach up to 20 %. Due to shifts in the timing of growth, vessels in one tree appear to form 42 d earlier than the other tree and a similar pattern occurs for parenchyma (Fig. 2C, D). Both parenchyma and vessel lumen fractions gradually increase at the beginning of the season, culminate around February, then decrease towards the end of the season. Vessel lumen fractions and parenchyma fractions are positively related to each other as well as to the δ¹³C profile (Table 3). None of the traits is significantly correlated with precipitation.

Table 3.

Pearson correlation matrix of the measured wood traits, and simultaneously measured and calculated environmental variables at a weekly scale

Variables	δ13C	δ18O	VL	PRM	p	R	RH	T	VPD
δ13C		–0.26	0.46	0.60	0.23	0.72	–0.55	0.59	0.56
δ18O			–0.24	–0.20	–0.08	–0.06	0.15	–0.04	–0.07
VL				0.60	0.23	0.27	–0.11	0.15	0.18
PRM					0.05	0.55	–0.41	0.49	0.44
p						0.08	0.15	0.10	–0.06
R							–0.79	0.72	0.88
RH								–0.65	–0.90
T									0.68

δ¹³C and δ¹⁸O, high-resolution isotopes from wood cellulose (‰); VL, vessel lumen fraction (–); PRM, parenchyma fraction (–); p, precipitation (mm); R, solar radiation (W m⁻²), RH, relative humidity (%); T, temperature (°C), VPD, vapour pressure deficit (kPa). Bold type indicates significant correlations (P < 0.01).

Discussion

Leaf phenology and cambial phenology

Generally, asynchronous leaf and cambial phenology is observed. Pre-rain green-up in southern African systems (Ryan et al., 2017) is shown here for T. superba, where leaf flushing occurs before the onset of rain in some trees (Fig. 1A, B). While there are small differences in leaf flushing between seasons, large differences occur between individuals. Leaf onsets per tree for 2013, 2014 and 2015 are similar (Table 2), but considerable differences in rain onset (Fig. 1B) exist. Other drivers such as insolation or photoperiod might thus influence the timing of flushing as well (Borchert et al., 2015), yet do not explain the individual differences. Variations in leaf onset in T. superba (Fig. 1) have also been reported (Mariaux, 1969; Couralet et al., 2013).

Intrinsic factors in this study could thus overrule the effect of precipitation: leaf flushing could merely be a consequence of leaf shedding and resulting changes in tree water status (Borchert, 1999). Terminalia superba can thus be considered a brevi-deciduous tree, with a short leafless period and flushing following leaf shedding, and stored stem water could cause some trees to flush before the onset of the rainy season (Borchert, 1994) (Supplementary Data Fig. S5a,b). Additionally, an increase in temperature, from July to September (Fig. 1B), could also be of influence. In the specific case of the Mayombe, water loss during the dry season is probably limited due to reduced VPD (Fig. 1C), as well as occult precipitation (Lubini, 1997) (i.e. condensation drops on leaves from fog that cannot be measured with rain gauges), which could also explain the variability in leaf onset due to lower environmental forcing.

The delay between the onset of leaf and onset of cambial phenology (Table 2) can be explained by tree height. At up to 45 m (Table 1), T. superba is one of the largest trees in the Mayombe, and cambial triggering hormones need to migrate from crown to stem base. This hypothesis is also proposed for temperate regions (Schmitt et al., 2000). Differences in xylem formation onset between T. superba trees were observed by Mariaux (1969) and are presented in Fig. 1A. In this study, observed differences of up to 50 d are higher than those reported in temperate regions (e.g. Bouriaud et al., 2005; Rossi et al., 2008; Hetzer et al., 2014). Here, we also report ‘live’ observation of missing rings in two trees in 2013–2014 (Fig. 2), monitored via SDV and confirmed with cambial pinnings. Based on cambial pinnings, missing rings were also found in understorey trees of the same study area (De Mil et al., 2017).

Wood traits on a time axis

Intra-annual sampling of individual rings does represent growth increments, but not necessarily equivalent time increments (Poussart et al., 2004), which is confirmed by shifts in time between individual trait profiles (Fig. 2). In order to date δ¹³C (Soudant et al., 2016), δ¹⁸O and wood anatomical variables accurately within a tree ring, the space–time conversion approach is used to fix traits of T. superba rings on a time axis, and shows that between-tree cambial phenology differences requires shifting of traits to their proper time of formation. Using just spatial averaging per intra-annual position would result in incorrect interpretation of the data.

Large variability in the shape of the δ¹³C profiles between trees is also observed for temperate species (Skomarkova et al., 2006; Vaganov et al., 2009; Michelot et al., 2011). Variation between radii (Fig. 2A) of the trees in this study remains largely within general limits of 0.5–1 ‰ (Leavitt, 2010) for δ¹³C.

The general trend of the intra-annual δ¹³C profile for all trees combined (Fig. 2A) is similar for profiles observed in other tropical trees (Verheyden et al., 2004; Poussart et al., 2004; Fichtler et al., 2010). The increase in δ¹³C at the onset of the xylem-growing season is due to the use of starch reserves of the previous growing season, which is typically enriched in ¹³C (Michelot et al., 2011). The decrease towards the end of the growing season is due to the use of current year carbon assimilates to synthesize cellulose (Helle and Schleser, 2004). However, many of these studies assess the isotope pattern as a function of ring position, which makes it difficult to link the isotopic response to specific weather events for interpretation, thus missing the more complete environmental effect that can be seen in the δ¹³C profile (Soudant et al., 2016). By synchronizing and linking to weather variables, we obtain a positive relationship between δ¹³C and solar radiation and VPD, and a negative relationship with relative humidity (Table 3), which are common environmental factors affecting δ¹³C (Managave and Ramesh, 2012).

High-frequency variations are observed in the δ¹⁸O profile (Fig. 2B), without a clear trend. Variation between radii is higher than the general interval 0.5–2 ‰ (Leavitt, 2010). Mixing of water sources (Gessler et al., 2014), a constant high relative humidity (Roden and Siegwolf, 2012), the proximity of the Atlantic Ocean and occult precipitation during the dry season in the Mayombe might cause this variation.

Despite the space–time conversion of the δ¹³C and δ¹⁸O and link with weather variables, it is not evident to separate the signal from current-year photosynthesis and remobilization of previous-year storage components (Schleser et al., 1999).

Parenchyma and vessel lumen fractions (Fig. 2C, D) are rarely assessed at an intra-annual scale in trees of the Congo Basin. Vessel lumen fraction is related to δ¹³C and parenchyma fraction but has no direct link with weather parameters in this study (Table 3). Vessel lumen area is shown to be positively linked with δ¹³C in seasonally dry forests as well (Ohashi et al., 2009).

Parenchyma area (Fig. 2C) is related to vessel area because the axial parenchyma is centred around the vessels (i.e. aliform parenchyma). The main function of parenchyma is storage (Beeckman, 2016; Morris et al., 2016), and while parenchyma is being explored as a climate-sensitive trait (Olano et al., 2013), a mechanistic understanding of its use as a proxy is lacking, especially for axial parenchyma in angiosperms (Eckstein, 2013). A positive correlation between parenchyma and radiation, temperature and VPD, and a negative correlation with relative humidity is observed in this study (Table 3).

To correctly assess the resilience of tropical trees, asynchrony between trees should be further investigated, where more advanced pattern matching of wood traits could offer further prospects (Mann et al., 2018). Given the greater occurrence of extreme climatic events and the potential effect on leaf phenology, variation in tree-specific phenology presented here, as opposed to general phenology trends (Ryan et al., 2017), will impact the interpretation of wood traits considerably, and should be given more emphasis in ecological and biomass (Cuny et al., 2015) modelling of tropical forests.

SUPPLEMENTARY DATA

Supplementary data are available online at https://academic.oup.com/aob and consist of the following. Fig. S1: Study site and illustration of the cambial pinnings, cameras, dendrometers and wood anatomical measurements. Fig. S2: Information on the size and site characteristics of the studied trees. Fig. S3: Scheme of the space–time conversion and breakpoint analysis. Fig. S4: Results of breakpoint analysis for further fine-tuning the date of onset and cessation of xylem formation based on pinning and dendrometer data. Fig. S5: Leaf flushing and leaf shedding events, plotted together with stem diameter variations, at the end of the dry season and the end of the wet season, respectively. Fig. S6: Xylem growth season length of all trees. Fig. S7: Microsections at the beginning of the 2015–2016 season, with cambial stadia visible. Fig. S8: Observation of a missing ring on the stem disc, at the dendrometer position. Information S1: Data link to anatomical sections and data analysis.

FUNDING

This work was funded by a Special Research Grant PhD scholarship of T. De Mil from Ghent University (BOF.DOC.2014.0037.01), and by the Brain programme of the Belgian Federal Government (BR/143/A3/HERBAXYLAREDD). A travel grant for T. De Mil was obtained from the King Leopold III Fund for Nature Exploration and Conservation. Sensors were funded by the XYLAREDD (AG/LL/165) project. V. Deklerck and S. Maginet (VLIR-UOS funding) assisted with crown and site descriptions. Final editing and revisions were done by T. De Mil at the University of Arizona, who received a Léon Speeckaert Fund postdoctoral fellowship from the King Baudouin Foundation and the Belgian American Educational Foundation (BAEF).