Integration of single photon emitters in 2D layered materials with a silicon nitride photonic chip

Abstract

Photonic integrated circuits (PICs) enable the miniaturization of optical quantum circuits because several optic and electronic functionalities can be added on the same chip. Integrated single photon emitters (SPEs) are central building blocks for such quantum photonic circuits. SPEs embedded in 2D transition metal dichalcogenides have some unique properties that make them particularly appealing for large-scale integration. Here we report on the integration of a WSe₂ monolayer onto a Silicon Nitride (SiN) chip. We demonstrate the coupling of SPEs with the guided mode of a SiN waveguide and study how the on-chip single photon extraction can be maximized by interfacing the 2D-SPE with an integrated dielectric cavity. Our approach allows the use of optimized PIC platforms without the need for additional processing in the SPE host material. In combination with improved wafer-scale CVD growth of 2D materials, this approach provides a promising route towards scalable quantum photonic chips.

Integration of photonic circuits with single photon emitters provides a promising route towards scalable quantum photonic chips. Here, the authors integrate a WSe₂ monolayer onto a SiN chip and demonstrate the coupling of single photon emitters in WSe₂ with the guided mode of a SiN waveguide.

Introduction

Photonic integrated circuits (PICs) enable the miniaturizing of complex quantum optical circuits with large numbers of photonic devices connected with optimized insertion losses and phase stability¹. Photons in a PIC are routed in a single spatial mode of a low-loss single mode waveguide, consisting of a high index core surrounded by lower index cladding materials to provide confinement of the optical mode. Spatial mode matching, which is crucial for classical and quantum interference, can be nearly perfect for such an architecture¹. The use of PICs moreover allows integration of several functionalities on a single chip, including photonic cavities to enhance light-matter interaction, filters to block or select specific wavelengths, integrated photodetectors, etc. A central building block for such quantum photonic circuits are single photon emitters (SPEs)². Over the past decade a variety of material systems have been investigated to create on-chip SPEs, including III–V quantum dots³, carbon nanotubes⁴, GaSe crystals⁵, and crystal colour centers such as the NV⁶ or SiV⁷ centers in diamond.

Recently, SPEs were discovered in monolayer transition metal dichalcogenides (TMDCs)^8–12 and monolayer and multilayer hexagonal boron nitride (hBN)^13,14. It has been shown that nanoscale strain engineering can be used to scale up the creation of such 2D-SPEs^15–20, but integration with a PIC has not been achieved so far. This would however alleviate some important issues met with other approaches for quantum photonic applications. First of all, techniques to transfer 2D materials or stack them by Van der Waals epitaxy to create complex heterostructures are by now getting well established, enabling easy interfacing with high quality PICs^21–23. Secondly, it is possible to achieve very high light extraction efficiencies because the emitters are embedded in a monolayer, avoiding total internal reflection. This is a major issue for diamond and III–V based quantum technologies, where a separate photonic structure is typically made in the host material to allow efficient single photon transfer between the host and underlying PIC. This adds serious challenges because separate PICs have to be fabricated in the host material and moreover may require precise pick-and-place techniques to integrate both PICs together^6,24. Furthermore, 2D materials can easily be integrated with electrical contacts²⁵ to ultimately enable all-electrical single photon generation over a broad spectrum²⁶ or to tune the single photon wavelength and symmetry by the quantum-confined Stark effect^27,28. Finally, 2D materials grown with high wafer-scale uniformity are becoming widely available^29–31, such that they can be matched at the wafer level with underlying photonic circuitry. Since 2D-SPEs mainly emit in the visible, the standard silicon-on-insulator PIC platform cannot be used because it’s not transparent for these wavelengths. Silicon nitride (SiN) PICs on the other hand are a useful platform for routing photons that carry quantum information since they provide low-loss transmission in the visible and are also available in a CMOS-fab³².

Here we study the integration of a WSe₂ monolayer onto a SiN chip and demonstrate the coupling of 2D-based single photon sources with the guided mode of a SiN waveguide. We discuss how integrated cavity-emitter systems, evanescently coupled to a waveguide, should be designed to optimize single photon extraction into the waveguide. As such the full potential of a high quality and CMOS-compatible PIC platform can be exploited without the need for stringent processing in the host material itself. In combination with wafer-scale growth of 2D materials, this provides a promising route towards scaling of quantum photonic circuits.

Results

Device overview

Figure 1a shows a schematic of the device. A mechanically exfoliated WSe₂ flake is transferred by dry transfer onto a single mode SiN waveguide. After transfer, the sample was placed in an optical cryostat from Montana instruments and cooled down to 3.9 K. Photoluminescence (PL) from the WSe₂ can either couple to free-space radiation or to the guided mode of the waveguide. The radiation to free-space is collected by a top objective with NA = 0.65, while the waveguide-coupled PL is captured by a lensed fiber, aligned to the output facet of the waveguide. An impression of the fiber-coupled chip and a microscope image of the integrated WSe₂ flake are depicted in Fig. 1b. The typical 1/e single photon propagation length for our devices is 0.5–1 cm (≈4–10 dB cm⁻¹). See Supplementary Note 1–3 for more information on the device fabrication and experimental setup, as well as a plot of a typical spectrum of the flake showing the neutral exciton peak around 710 nm with the broad delocalized neutral exciton defect band.

Fig. 1.

Integrated WSe₂ quantum emitters. a Top view of the device: a WSe₂ flake is integrated on a 220 nm thick single mode SiN waveguide, separated by 2 air trenches from the bulk SiN. The waveguide ends are tapered to allow easier coupling with a lensed fiber. The orientation of the dipole moment of the WSe₂ emitters (red arrow) is random with respect to the quasi-TE polarization (approximately aligned along x−direction) of the fundamental waveguide mode (black arrow). A fraction η_wg of the total emission couples into the left-propagating waveguide mode (represented by red shaded areas). b Cross-section of the sample. The width of the air trenches and waveguide is 3 μm and 700 nm respectively. The generated PL of emitters near the waveguide couples both to free-space and to the waveguide (red shaded circles). A cross-sectional mode profile (at λ = 750 nm) of the waveguide, taken along the dotted black line in the top figure, is shown as well. c Impression of the fiber-coupled chip (inset shows light coupling from the fiber to the chip). The tapered lensed fiber is a standard SM630 fiber from Thorlabs with a focal spot size of 2 μm and an 8 μm working distance. d Microscope image of SiN chip with WSe₂ transferred on waveguide region. The flake is highlighted by the white triangle

To maximize the count rate of an integrated single photon source, the fraction η_wg of total PL that couples to the waveguide mode should be as close as possible to one. It is, however, impossible to achieve this with the simple waveguide geometry shown in Fig. 1a, but interaction with a cavity can significantly boost the overall coupling rate to the guided mode. As an extension of our experimental results we will therefore investigate for which cavity parameters near-unity waveguide extraction efficiencies can be obtained. An essential parameter in this calculation is the cavity-emitter coupling, which critically depends on the dipole moment strength of the integrated 2D-based emitter. For realistic estimates of this value, we will assess it from our experiments. As such we can get a clear overview of which cavity Q–factors and mode volumes V_c are required to maximize single photon extraction.

On-chip quantum emitters

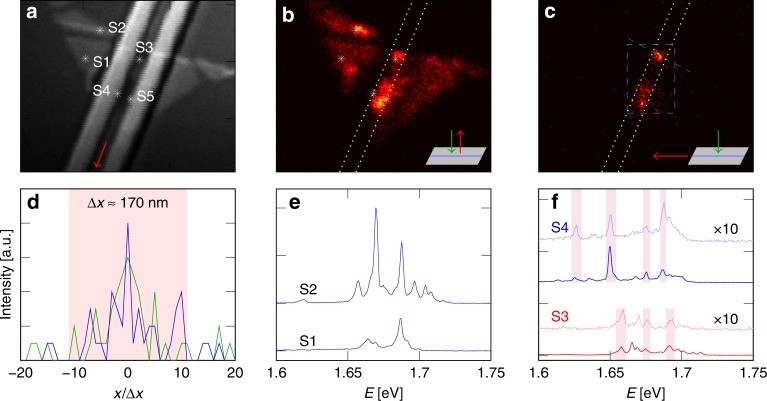
Figure 2 summarizes PL measurements on the flake. The excitation beam (λ = 532 nm) can be scanned over the sample through the top window of the cryostat by a set of two galvo-mirrors. The regions that light up in the PL scan of Fig. 2b, match with the area covered by the flake in the scanning confocal image of Fig. 2a. We will investigate five different spots on the flake, labeled S1 through S5. The spectra for two positions off the waveguide (S1 and S2) are shown in Fig. 2e. Spot S1 exhibits only two prominent peaks, which are relatively weaker compared to the spot S2 peaks. Spot S2 contains several narrower peaks with FWHM on the order of 3 meV in the 1.65–1.7 eV spectral region. This result is similar to observations made by Tonndorf et al.⁸. Spot S2 appears near a spatial non-uniformity in the flake (Fig. 2a), which could be due to e.g. a wrinkle in the monolayer, a crack in the material or a transition between a mono- and bi-layer. Such spatial non-uniformities usually lead to strong strain-gradient regions. Previous reports have shown that such regions are usually associated with the appearance of localized bright spots containing narrow linewidth emitters in TMDC monolayers^15–17. As such, the most likely mechanism for the appearance of multiple narrower peaks in the spectrum of spot S2 is strain (see also furtheron for the spectra of spots near the waveguide ridge). For all spectra in Fig. 2, the excitation power was set to 25 nW with an integration time of 60 s. Because the excitation power was low, the FWHM was not affected by power broadening. Spectral wandering during the long integration time could, however, result in inhomogeneous broadening of the FWHM of the emitters, as observed in earlier studies¹².

Fig. 2.

Waveguide-coupled WSe₂ quantum emitters. a Confocal laser scan (λ = 532 nm) of the relevant sample area. Spots S1 and S2 are spots off the waveguide, while spots S3 to S5 mark positions on the waveguide. The red arrow indicates the direction along which the fiber-coupled light is collected. b Confocal PL scan, by scanning the excitation beam over the sample from the top and collecting the PL from the top. c Waveguide PL scan, by scanning the excitation beam over the sample from the top and collecting the PL through the fiber. The white dotted lines mark the waveguide position. d Line scan along the the green and blue lines in Figure c, highlighting the estimated spatial region coupled to the waveguide (shaded red region). e PL spectra from spots S1 and S2, collected from the top. f PL spectra from spots S3 (red) and S4 (blue), collected from the top (solid color) and through the fiber (shaded color). Matching peaks are highlighted by shaded purple regions. Where necessary, the spectrum baseline is shifted for improved visualization. The waveguide-coupled spectra are multiplied by 10. The excitation power for all PL spectra was P_e ≈ 25 nW

The areas near spots S3, S4, and S5 exhibit brighter fluorescence compared to the surrounding region (see Fig. 2b) and are all located in the vicinity of the waveguide (region between the white dotted lines). This is similar to recent reports in which bright emission of a TMDC monolayer was observed at positions where the material was bend over a nanopillar and hints to the presence of strain-induced emitters coupled to the waveguide^16,17. To confirm that these spots are indeed coupled to the waveguide, we scan the excitation beam from the top, but collect the PL through the lensed fiber and indeed observe that only the waveguide region lights up (Fig. 2c). A small offset in the piezo position of the fiber from the waveguide results in an immediate loss of the signal, further confirming that we indeed collect light originating from the waveguide. The integrated intensity near the center of the waveguide is in general higher, which could be attributed to the fact that the electromagnetic overlap with the waveguide mode is higher near the center. As such, more radiation from the 2D material can couple into the waveguide mode. When the emitters are not located on the waveguide, it is interesting to estimate how far they can be away from the waveguide core and still generate PL that can couple into the waveguide. Figure 2d shows a line scan along two lines perpendicular to the waveguide to estimate the spatial extent over which the PL can still be coupled. Emitters located up to 1.9 μm on either side of the waveguide can couple into the waveguide. A closer examination of the confocal and waveguide-coupled spectra of spots S3 and S4 is shown in Fig. 2f. The spectra feature several narrow lines, with a typical linewidth ranging between 2.5 and 4 meV. This linewidth can be significantly broadened by the immediate surrounding of the WSe₂ (e.g. surface charges in the SiO₂ and SiN), but the broadening can be partially alleviated by encapsulation with hBN^33,34. A comparison between the spectrum of spot S1 and the other spots moreover shows more peaks near the waveguide or cracks in the sample, substantiating the argument that the emitters are indeed strain-induced. Data from a hyperspectral scan of the blue-dashed area in Fig. 2c, containing info on the spectral distribution of the PL and an estimation on the number of peaks, are included in Supplementary Note 4.

A comparison of the confocal and waveguide-coupled spectra shows that not all peaks appearing in the confocal spectra are present in the waveguide-coupled spectra. This can be understood from the fact that the coupling between the waveguide mode E_wg (quasi-TE-mode in our case) and the dipole moment of the quantum emitter p_d scales according to E_wg · p_d ∝ cosθ_d, with θ_d the angle between E_wg (black arrow in Fig. 1a) and p_d (red arrow in Fig. 1a). Hence, when ${\theta }_{\mathrm{d}}\to \frac{\pi }{2}$, the coupling vanishes. According to numerical simulations with Lumerical FDTD solutions, about η_wg = 7.3% of the total power radiated by a dipole (at E = 1.63 eV) with θ_d = 0 and centered on the top surface of the waveguide couples in the left-propagating guided TE-mode. For the same dipole emitter, η_NA≈ 6.5% radiates upwards in an NA = 0.65. A dipole at the same position on the waveguide but with ${\theta }_{\mathrm{d}}=\frac{\pi }{2}$ does not radiate into the TE-mode (as expected by the cosθ_d behaviour), while emitting ≈7.3% upwards in an NA = 0.65. So regardless of the orientation of the dipole, we expect about 7% of the total radiation to be captured in an NA of 0.65, while the light captured by the waveguide heavily depends on θ_d. As such, the large spread in relative strength between the confocal and waveguide-coupled signal of a certain peak stems from the fact that their ratio scales as η_wg/η_NA ∝ cosθ_d. The relative strength between different peaks depends both on the dipole polarization as well as on the absolute dipole moment of the emitter.

Waveguide-coupled single photon source

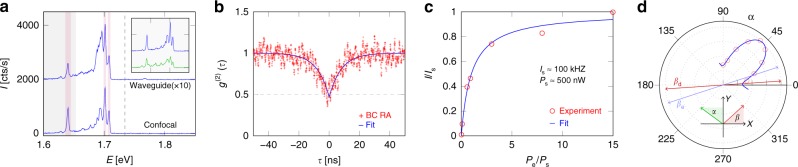
We will now focus on spot S5 of Fig. 2a and investigate the quantum nature of the observed emitters in more detail. The confocal and waveguide-coupled spectrum of spot S5 are shown in Fig. 3a. We observe a few peaks recurring in both the confocal and waveguide spectrum, confirming that these emitters are indeed coupled to the waveguide. A prominent and isolated waveguide-coupled peak (FWHM ≈3 meV) appears around 1.64 eV (756.5 nm). It has been shown that the PL of 2D-based quantum dots can be enhanced when the excitation laser wavelength is tuned close to the free excitonic resonance⁹. When we scan the excitation wavelength with a tunable Ti:saph laser around the free exciton wavelength, we also find a considerable increase in peak count rate and reduction in background compared to excitation with λ = 532 nm for the same excitation power (see inset Fig. 3a). An excitation wavelength of λ = 702 nm provided the most optimal ratio between peak count rate and background, and hence the emitter was pumped at this wavelength for all subsequent experiments.

Fig. 3.

On-chip single photon emission. a Confocal and waveguide-coupled spectrum of spot S5, excited with λ = 702 nm. The waveguide spectrum is multiplied by 10 and offset by 2000 cts/sec for improved visualization. Common peaks are highlighted by the shaded purple regions. A 715 nm (1.73 eV) longpass filter, marked by the dashed line, was used to filter the pump. For the g⁽²⁾(τ) measurement a 750 nm (1.65 eV) longpass filter (gray shaded area) was used to isolate the single emitter at 756.6 nm (1.64 eV). The inset figure shows confocal spectra obtained by either green (λ = 532 nm) excitation (green curve) or excitation with λ = 702 nm (blue curve). b–d Characterization of the 1.64 eV emitter. b Normalized background-corrected (BC) running average (RA) coincidence counts (red) and g⁽²⁾(τ) fit (blue). c Measured intensity saturation (red) and fit to saturation curve (blue). d Normalized SPD count of the emitter (red) as a function of half-wave plate rotation angle α and fit to intensity transmission curve (blue); α = 0 corresponds to a half-wave plate fast axis along the Y−direction. See Supplementary Fig. 1 for orientation of the half-wave plate with respect to the (X, Y, Z) axes. Based on the fit, the difference in polarization angle between the PL (β_d) and excitation (β_e) beam can be extracted; β = 0 corresponds to a polarization along the X−axis

A 750 nm longpass filter (gray shaded area in Fig. 3a) was used to spectrally isolate the 1.64 eV peak from the broad PL emission around 1.7 eV before the beam hits the Single Photon Detectors (SPDs). As such, the major contribution to the SPD count stems from the 1.64 eV peak and we can perform a g⁽²⁾−measurement to investigate whether single photons are emitted by this emitter. Due to the lower count rates of the waveguide-coupled PL, we use the free-space collected PL for the g⁽²⁾−measurement. Based on the spectrum we assess that the peak of interest (at 1.64 eV) contributes a fraction of about ρ = 0.76 to the total signal while the rest is due to uncorrelated background. The raw normalized coincidence counts without any background correction are reported in the Supplementary Note 5, while the plot in Fig. 3b shows the background-corrected g⁽²⁾(τ)−curve, on which moreover a running average is applied to reduce the noise on the data. The background corrected g_BC⁽²⁾(τ) value can be calculated according to g_BC⁽²⁾(τ) = (g⁽²⁾(τ) − (1 − ρ²))/ρ²³⁵. See Supplementary Note 5 for more details on the background correction and running average. Fitting the background-corrected data to the equation g_f⁽²⁾(τ) = 1 − Aexp(−|τ|/τ_f) yields g_f⁽²⁾(0) = 1 − A = 0.47 and τ_f = 7.99 ns³⁶. The minimum value in the background-corrected data without averaging is about 0.03, which would hint to almost perfect single photon emission. The fitted rise time τ_f = 7.99 ns is a lower limit for the PL decay time and is in the same order of magnitude as previously reported values for WSe₂⁸. The clear anti-bunching dip with a background corrected g⁽²⁾(0) < 0.5 confirms that the emitter indeed emits single photons.

A generic two-level system moreover exhibits saturation of the PL emission when the excitation rate increases, and this has been observed for WSe₂ emitters before^8–10,12. The PL saturation for our waveguide-coupled quantum emitter is shown in Fig. 3b. A fit of the PL intensity I = I_s(P_e/(P_e + P_s)) as a function of excitation power P_e yields a saturation power of P_s ≈ 500 nW (at λ = 702 nm) and a saturation intensity of I_s ≈ 100 kHz. The excitation efficiency of the emitter will, however, depend on the orientation between the dipole moment of the quantum emitter β_d and the excitation polarization β_e and will hence affect the measured intensity. We therefore perform polarization-dependent transmission measurements to determine Δβ = β_d − β_e. The normalized transmitted emitter count rate to SPD1 as a function of the polarization-rotating half-wave plate angle α is shown in Fig. 3d. By fitting this count rate one can determine Δβ and eventually assess the saturation count rate of the single photon source. When corrected for transmission and collection efficiencies of the system, the total saturation intensity is about 3 MHz (to all modes, guided and non-guided) while the estimated maximum waveguide-coupled count rate is about 100 kHz (see Supplementary Note 6). Further improvements consist of changes in the waveguide design³⁷ or interaction with plasmonic or dielectric cavities^38,39 to maximize the coupling efficiency into the guided mode and enhance non-classical light generation.

Optimized single photon extraction and indistinguishability

Apart from high single-photon extraction efficiency, various applications (linear optical quantum computing, quantum teleportation, quantum networks, etc.) require the single photons to be indistinguishable (i.e. identical spatial and spectral modes)⁴⁰. For an ideal single photon source, the product of extraction efficiency η and indistinguishability V should be ηV = 1. In this section we will assess how η and ηV of an integrated 2D quantum emitter can be optimized by cavity coupling. Figure 4a shows a schematic of the investigated platform. The emitter is coupled to a cavity with coupling strength Ω, while the cavity is evanescently coupled to the waveguide with a coupling strength κ. The intrinsic decay rate of the cavity γ_c contains both absorption losses and radiation to non-guided modes. The overall cavity decay rate (containing both intrinsic losses as well as coupling to the nearby waveguide) is given by γ_p = γ_c + κ. The rate γ_e incorporates decay of the emitter to all modes (radiative and non-radiative) other than the cavity and γ* is the emitter dephasing (which describes a decay of the atomic polarization S_x + iS_y, without changing the decay of S_z and is modeled by a coupling between S_z and a high temperature heat bath; S_x,y,z are the the Pauli matrices⁴¹). For our calculations we assume the emitter is resonant with the cavity (ω_e = ω_c) and is initialized in the excited state by a short excitation pulse (EXC) with no photons present in the cavity. The master equation governing the dynamics of this system is discussed in Supplementary Note 7. In the regime where γ^∗ ≪ γ_e + γ_p (which should be satisfied for low temperatures and moderate Q–factor cavities), the single photon extraction efficiency into the guided mode (η) is given by

$$\eta =\frac{\kappa }{\left({\gamma }_{\mathrm{e}}+{\gamma }_{\mathrm{c}}+\kappa \right)\left(1+\frac{{\gamma }_{\mathrm{e}}\left({\gamma }_{\mathrm{c}}+\kappa \right)}{4{\mathrm{\Omega }}^2}\right)}.$$ 1

Fig. 4.

Integrated cavity-emitter system. a Schematic of an integrated cavity-emitter system, evanescently coupled to a single mode waveguide. The coupling rate between an emitter with frequency ω_e and a cavity with resonance frequency ω_c is given by Ω. The decay rate from the cavity-emitter system to the guided mode is κ, while the other decay channels of the emitter and cavity are given by γ_e and γ_c, respectively. The emitter dephasing is described by γ*. The system is excited (EXC) by a short pulse and subsequently the single photon PL is collected. b, c Single photon extraction efficiency η (b) and extraction-indistinguishability product ηV (c) as a function of cavity mode volume V_c and cavity decay rate κ. The black solid lines represent lines of constant Purcell factor F_P, while the black dashed line represents (V_c, κ) combinations for which η is maximal. The parameter values used to generate plots b, c are Q_i = 10000, Γ = 3 MHz, γ_e = 300 MHz, cos²θ_d = 1/2 (i.e. average over different orientations of the quantum emitter), γ* = 100 GHz⁴⁴, n_d = 4 and λ_c = λ₀/n_eff with a free-space wavelength of λ₀ = 750 nm and an effective refractive index of n_eff = 1.6 for the fundamental TE-mode (which is calculated using a commercial FDTD solver from Lumerical). Both plots are on a log₁₀ color scale, i.e. 0 corresponds to perfect η = 1 or ηV = 1. d Optimum η_opt (evaluated at (V_c, κ) combinations for which η is maximal) as a function of F_P for different values of Γ ranging from 0.01γ_e to γ_e (different quantum yields)

The expressions for the indistinguishability V of photons coupled into the guided mode, as derived by Grange et al.⁴⁰, depend on the regime within which the system falls (see Supplementary Note 7). To assess η and ηV (as shown in Fig. 4b, c), we first need to determine the different coupling strengths. The coupling constant Ω depends on the cavity mode volume V_c through ${\mathrm{\Omega }}^2=\frac{3\pi c^3}{2n_{\mathrm{d}}{\omega }_{\mathrm{c}}^2}{\cos }^2{\theta }_{\mathrm{d}}\left(\frac{\mathrm{\Gamma }}{V_{\mathrm{c}}}\right)$, with Γ the free-space radiative decay rate in a uniform dielectric with index n_d, and θ_d the angle between the emitter dipole moment and the cavity field. For our calculations we assume n_d is the refractive index of a WSe₂ monolayer (n_d = 4)⁴². In our case, the radiative decay rate to non-guided modes will usually differ from Γ due to the non-uniform dielectric environment and may furthermore be influenced by the vicinity of the dielectric cavity, but as a simplifying assumption we set Γ ≈ γ_r with γ_r the radiative decay rate determined from our experiment, i.e. γ_r ≈ 3 MHz. Numerical simulations of dipole emission near a waveguide show that the total radiated dipole power (with polarization parallel to the top surface of the waveguide) is on the same order of magnitude as what the dipole would radiate in a homogeneous dielectric, so in a first approximation this is a valid assumption. To take into account different polarizations of the quantum emitter, we assume an average value for cos²θ_d over all possible orientations θ_d, i.e. < cos²θ_d > = 1/2. The decay rate γ_e also contains contributions to non-radiative modes (γ_e = γ_r + γ_nr), and can be approximated by γ_e = γ_r/ξ with ξ the quantum yield of the monolayer. Strain-induced quantum emitters in WSe₂ are reported to have a typical quantum yield of 1%⁴³, so we take γ_e ≈ 300 MHz for our calculations. It is important to note that the quantum yield of these emitters can however vary significantly depending on growth conditions. As such the 1% is only a first approximation. The effect of different quantum yields will be described furtheron. The final parameter is κ, which we express through the intrinsic cavity quality factor Q_i as $\kappa =\chi {\gamma }_{\mathrm{c}}=\chi \left(\frac{{\omega }_{\mathrm{c}}}{2Q_{\mathrm{i}}}\right)=\frac{{\omega }_{\mathrm{c}}}{2Q_{\kappa }}$ such that the loaded quality factor of the cavity is given by $Q={\left(Q_{\mathrm{i}}^{-1}+Q_{\kappa }^{-1}\right)}^{-1}=Q_{\mathrm{i}}∕\left(1+\chi \right)$. We use Q_i = 10000 for our calculations. The above parameter values are now used to estimate how η and ηV can be improved through cavity-assisted interaction as a function of the normalized cavity mode volume V_c/λ_c³ and waveguide-cavity coupling χ = κ/γ_c (Fig. 4b, c). The solid black lines represent lines of constant Purcell factor $F_{\mathrm{P}}=\frac{3}{4{\pi }^2}Q\left(\frac{{\lambda }_{\mathrm{c}}^3}{V_{\mathrm{c}}}\right)$, while the dashed black line represents the (V_c, κ) combinations for which η is optimized. For a given mode volume V_c (i.e. Ω), the coupling rate κ that maximizes η is given by

$${\kappa }_{\mathrm{opt}}={\gamma }_{\mathrm{c}}\sqrt{\left(1+\frac{{\gamma }_{\mathrm{e}}}{{\gamma }_{\mathrm{c}}}\right)\left(1+\frac{4{\mathrm{\Omega }}^2}{{\gamma }_{\mathrm{e}}{\gamma }_{\mathrm{c}}}\right)}.$$ 2

For this value of κ, the optimum $\eta \approx \mathcal{C}∕{\left(1+\sqrt{1+\mathcal{C}}\right)}^2$ if γ_e < γ_c, with $\mathcal{C}=4{\mathrm{\Omega }}^2∕\left({\gamma }_{\mathrm{e}}{\gamma }_{\mathrm{c}}\right)\propto \xi Q_{\mathrm{i}}\left({\lambda }_{\mathrm{c}}^3∕V_{\mathrm{c}}\right)$. As such, near-unity extraction requires a high intrinsic quality factor (while the loaded Q can be much lower), high quantum efficiency and small mode volume. The intersection of the F_P = 100 line with κ_opt yields η ≈ 34% for κ = 2γ_c (Q ≈ 3333) and V_c = 2.45λ_c³. For these parameter values, ηV is only 0.2% however. To achieve high ηV one typically needs much smaller V_c because the cooperativity $\mathcal{C}$ has to overcome the emitter dephasing γ*⁴⁴. If we decrease V_c to V_c = 0.01λ_c³, then a maximum ηV ≈ 25% is achieved for κ = 2.05γ_c (Q ≈ 3280). A near-unity extraction (η = 93%) can be achieved for κ = 29γ_c (Q ≈ 333) and V_c = 0.01λ_c³ (F_P ≈ 2530), with ηV ≈ 6.8%. By using the ultrasmall mode volume nanocavities reported in⁴⁵, we could hence achieve near perfect single photon extraction, even for a very low quantum yield emitter. However, the required cavity Purcell factor is still large. In order to achieve higher η for smaller F_P one can aim to increase the quantum yield as shown in Fig. 4d, which depicts η_opt (i.e. η evaluated at (V_c, κ) combinations for which η is maximal) as a function of F_P and ξ. For near-unity quantum yield, η already reaches 84% for a moderate Purcell factor of F_P = 10, while η = 98% for F_P = 100. Nevertheless, the corresponding ηV product is still far from the desired unity value. In most realistic cases, the system will be in the bad cavity limit (γ_p > γ_e + γ^∗), and achieving high ηV will require the Purcell factor to satisfy F_P≫(1/ξ + γ^*/Γ) (see Supplementary Note 7 for formulas of V in different limits). To relax the constraints on F_P one should hence aim to reduce the ratio γ*/Γ or increase ξ. However, usually γ*/Γ > 1/ξ so increasing ξ will have little effect as long as the dephasing rate is high. This analysis can be repeated for any dielectric cavity-emitter system that is evanescently coupled to the waveguide and as such can guide future design efforts to optimize single photon extraction and indistinguishability of photons coupled into the guided mode of the waveguide.

Discussion

In conclusion we have demonstrated that integration of a WSe₂ monolayer onto a SiN waveguide results in quantum emitters evanescently coupled to the waveguide. Second-order correlation measurements on a spectrally isolated quantum emitter confirm that single photons are emitted with a waveguide-coupled saturation count rate of 100 kHz. These results confirm previous claims that strain-induced quantum emitters could be coupled to photonic structures^16,17. A numerical analysis on the optimization of single photon extraction and indistinguishability using integrated dielectric cavity-emitter systems indicates that near-unity single photon extraction can be achieved, even for low quantum yield emitters. The presented approach for integration of strain-induced TMDC-based SPEs retains the favorable attributes of SiN PICs without the need for stringent processing in the quantum emitter host material itself. Recent progress in wafer-scale growth and patterning of identical 2D-material based devices^29–31 provides a promising route in combination with our waveguide-coupled 2D-SPEs to scale up quantum photonic circuits.

Author contributions

F.P. designed the chip, conducted the experiments, analyzed the data and developed the theoretical model. C.C. performed the transfer of the 2D material and helped with the experiments. M.M. fabricated the sample. D.V.T. and D.E. supervised the work.

Data availability

The data that support the findings of this study are available from the corresponding author upon reasonable request.

Competing interests

The authors declare no competing interests.

Supplementary information

Supplementary information is available for this paper at 10.1038/s41467-019-12421-0.