Abstract
OBJECTIVES
Accurate determination of ideal body weight (IBW) in pediatric patients is important for the proper dosing of many medications and the classification of nutritional status. There is no consensus on the best method to calculate IBW. The purpose of this study is to evaluate and compare 7 different methods used to calculate IBW in the pediatric population.
METHODS
This was a retrospective observational study. All subjects were pediatric inpatients at a 536-bed community teaching hospital between January 1, 2016, and June 30, 2017. Subjects were divided into 2 cohorts: cohort 1 was aged 12 months and 0 day to 35 months and 30 days, and cohort 2 was aged 36 months and 0 day to 17 years and 364 days. The McLaren method was used as the reference to compare with 6 other methods: Moore method, Devine method, American Dietetic Association (ADA) method, body mass index (BMI) method, Traub equation, and simplified Traub equation.
RESULTS
For cohort 1 (n = 347), the Moore method was not statistically different from the McLaren method with a mean difference of −0.07 kg (95% CI: −0.14 to 0.01, p = 0.07). For cohort 2 (n = 1095), the BMI method was not statistically different from the McLaren method with a mean difference of 0.17 kg (95% CI: −0.07 to 0.40, p = 0.17).
CONCLUSIONS
In both cohorts, the majority of methods used to calculate IBW in pediatric patients leads to statistically different results when compared with the McLaren method. For certain methods, these differences become pronounced at high and low height percentiles and in older age groups.
Keywords: dosing, ideal body weight, pediatrics
Introduction
Accurate determination of ideal body weight (IBW) in pediatric patients is important for the proper dosing of many medications, such as acyclovir, digoxin, and morphine.1 It is also crucial in other scenarios, such as tidal volumes prescribed in mechanically ventilated patients.2 If IBW is calculated inaccurately, it can lead to under or over dosing of medications, causing reduced efficacy or increased toxicity.1 It can also lead to discrepancies in the care of serious conditions such as acute respiratory distress syndrome.2 IBW is defined as being a reflection of lean body mass.1 Dosing considerations regarding IBW may be especially true for patients with obesity, and the obesity rate for patients ages 2 to 19 years from 2011 to 2014 was 17%.3 The Pediatric Pharmacy Advocacy Group recommends that clinicians should consider pharmacokinetic analysis for adjusting medications whenever possible in children with obesity to ensure the most effective and safe regimen. Incorrect dosing is the most commonly reported medication error in children and can lead to lack of efficacy if subtherapeutic or toxicity if supratherapeutic.4,5 Furthermore, IBW is important for the classification of nutritional status.6 Although many different IBW calculation methods exist, there is no consensus on the most accurate method for pediatric patients.7
The McLaren method was the first method published in 1972 and was originally developed to classify malnutrition. It is a growth chart-based method that compares weight and height in relation to a child's age. This method requires graphing a vertical line between the child's height on the height-for-age line and the corresponding 50th percentile weight to determine IBW.8
The most commonly used adult equation for IBW, the Devine method, was published in 1974 in order to aid in the dosing of gentamicin.9 For men, IBW = 50.0 + 2.3 × (ht – 60 in); whereas for women, IBW = 45.5 + 2.3 × (ht – 60 in).10 To our knowledge, there has not been a study that has evaluated if this method is appropriate for adolescents.
In 1983, the Traub equation for pediatric IBW was published as a way to calculate ideal body mass without graphing. Unlike other methods, the Traub equation only requires knowledge of the child's height in centimeters: IBW (kg) = 2.396e0.01863(ht).11 Lexicomp further simplifies the Traub equation to [(ht2) × 1.65] ÷ 1000.12
The Moore method, an additional growth chart-based method, determines IBW by looking at the same weight percentile line as the child's height percentile for that age. For example, if a child has a height in the fifth percentile, his IBW should be at the fifth percentile of weight for this age.13
In 2003, the American Dietetic Association (ADA) published an infrequently used method that determines the IBW by reviewing the growth chart and identifying the 50th percentile value on the weight-for-age chart.14
Finally, the body mass index (BMI) method was proposed in 2006 and used the following equation: IBW (kg) = [BMI at the 50th percentile for that child's age × (ht in meters)2]. Of note, it was originally developed to be a tool for screening individuals who may be overweight or underweight and not necessarily as a tool to calculate IBW.15
Previous research that compared multiple methods suggested that the McLaren, Moore, and BMI methods had good correlation at the 50th percentile through age 18 years and across all percentiles in children younger than 8 years old. However, these 3 different methods provided widely divergent results for IBW at the lowest and highest percentiles in patients aged 8 to 18 years.7 The objectives of this study, which focused on patients 12 months to younger than 18 years of age, were to evaluate and compare the 7 methods previously mentioned in estimating IBW in the pediatric population.
Materials and Methods
Patients and Study Design. All subjects in this retrospective observational study were inpatients at a 536-bed community teaching hospital between January 1, 2016, and June 30, 2017. Subjects aged 12 months and 0 day to 17 years and 364 days were included. Patients were excluded for the following reasons: 1) no height data, 2) no weight data, and 3) had a diagnosis of medical conditions, such as growth hormone deficiency or cerebral palsy, that would substantially affect their height and/or weight. The broad nature of the inclusion criteria was chosen to mimic actual patients who were admitted to the hospital.
The primary objective of this study was to compare the previously mentioned methods of calculating pediatric IBW with the McLaren method across all height percentiles and ages. Although there is no guideline defined as the gold standard, the McLaren method was chosen as a comparator because it is still commonly referenced as a primary method of calculating IBW in children and was the first of the 7 methods to be published in the literature.7,13,16,17 The McLaren method was also recommended as the preferred method of calculating IBW in mechanically ventilated patients, because it had the best agreement with other methods compared.2 Secondary objectives included determining the method that was the best comparator of the McLaren method for the following subgroups: 1) ≤5% height percentile, 2) ≥95% height percentile, 3) 3 to <8 years old, and 4) ≥8 years old. This study was approved by the institutional review board, and full waiver of informed consent was granted.
Data Collection. All patient charts were obtained from the institution's electronic medical record. Once inclusion criteria were met, data elements, including demographic information, were abstracted from each patient's medical chart. Height and weight data were obtained based on the most recent admission and were matched with the exact age of the patient at that admission.
Data analysis was separated into 2 cohorts because height is typically measured in the supine position when patients are <36 months old. Measuring in the supine position requires that the birth to 36 months' length-for-age and weight-for-age growth charts to be used.7 For patients ≥36 months, height is typically measured in the standing position. This requires that the children 2 to 20 years' stature-for-age and weight-for-age growth charts to be used. Cohort 1 included patients aged 12 months and 0 day to 35 months and 30 days. Cohort 2 included patients aged 3 years and 0 day to 17 years and 364 days. For cohort 1, IBW was calculated using the McLaren method, Moore method, Traub equation, simplified Traub equation, and ADA method. For cohort 2, IBW was calculated using the previous 5 methods in addition to the BMI method and the Devine method if the subjects were >60 inches tall. For all methods requiring growth charts, these were obtained from the Centers for Disease Control and Prevention website.18
Sample Size. Data from an initial pilot explored estimation precision of 95% CIs for the differences in calculated IBW between the McLaren and other methods. This demonstrated that for cohort 1, a sample size of 300 patients would provide 90% power to construct 95% CIs no wider than ± 0.1 kg, and for cohort 2, a sample size of 900 patients would provide 90% power to construct 95% CIs no wider than ± 0.5 kg.
Statistical Analysis. Analysis of variance was performed to assess the IBW differences between the McLaren method and other methods of calculating IBW. Confidence intervals and p values were reported. The Benjamini-Hochberg procedure was used to control the potential false discovery rate from multiple comparisons, maintaining a at 0.05.19 Graphical depictions of estimated IBW difference between the McLaren method and each of the alternative methods were constructed. All statistical tests were 2-sided. Computations were performed using Stata 15.1 (Stata Corp, College Station, TX).
Results
One thousand five hundred thirty-five subjects were screened, and 1442 were included in the study. Baseline demographics are listed in Table 1. The mean total body weight in cohort 1 was 11.9 kg, and the mean height percentile was 45.5%; whereas in cohort 2, 45.5 kg and 55.6%, respectively.
Table 1.
Baseline Characteristics of the Study Population (N = 1442)
| Characteristic | Value | |
|---|---|---|
| Cohort 1 (n = 347) | Cohort 2 (n = 1095) | |
| Boys, no. (%) | 188 (54.2) | 574 (52.4) |
| Age, yr, mean ± SD | 1.8 ± 0.6 | 10.6 ± 4.7 |
| 3 to <8 yr, no. (%) | — | 388 ± 35.4 |
| ≥8 yr, no. (%) | — | 707 ± 64.6 |
| Weight, kg, mean ± SD | 11.9 ± 2.3 | 45.5 ± 26.7 |
| 3 to <8 yr, mean ± SD | — | 20.3 ± 6.2 |
| ≥8 yr, mean ± SD | — | 59.3 ± 23.4 |
| Height, cm, mean ± SD | 83.8 ± 8.5 | 141.3 ± 27.0 |
| PICU admission, no. (%) | 43 (12.4) | 192 (17.5) |
| Race, no. (%) | ||
| White | 152 (43.8) | 441 (40.3) |
| Black | 142 (40.9) | 476 (43.5) |
| Asian | 8 (2.3) | 20 (1.8) |
| Other | 16 (4.6) | 72 (6.6) |
| Not specified | 29 (8.4) | 86 (7.9) |
| Height percentiles, no. (%) | ||
| ≤5% | 56 (16.1) | 100 (9.1) |
| >5%–10% | 22 (6.3) | 46 (4.2) |
| >10%–25% | 53 (15.3) | 123 (11.2) |
| >25%–50% | 62 (17.9) | 205 (18.7) |
| >50%–75% | 65 (18.7) | 233 (21.3) |
| >75%–90% | 39 (11.2) | 156 (14.2) |
| >90%–94% | 16 (4.6) | 77 (7.0) |
| ≥95% | 34 (9.8) | 155 (14.2) |
| BMI, kg/cm2 (≥2 yr), mean ± SD | 16.6 ± 2.4 | 20.7 ± 6.8 |
BMI, body mass index; cm, centimeters; PICU, pediatric intensive care unit
Cohort 1. For the primary outcome, all methods except the Moore method were statistically different when compared with the McLaren method across all data points. The mean difference between the Moore and McLaren methods was −0.07 kg (95% CI: −0.14 to 0.01, p = 0.07). Mean differences of all other methods are shown in Table 2. None of the methods had a mean difference >10% as compared with the mean weight of the cohort. The graphical depictions show the trend of all methods compared with the McLaren method across all height percentiles (Figure 1) and ages (Figure 2).
Table 2.
Cohort 1: Comparison of Different IBW Equations to the McLaren Method
| Methods | All Data Points (N = 347) | ≤5th Percentile (n = 56) | ≥95th percentile (n = 34) | ||||||
|---|---|---|---|---|---|---|---|---|---|
| Difference, kg (mean) | 95% CI | p value | Difference, kg (mean) | 95% CI | p value | Difference, kg (mean) | 95% CI | p value | |
| Simplified Traub12 | −0.24 | −0.31 to −0.17 | <0.001 | −0.63 | −0.80 to −0.46 | <0.001 | 0.24 | 0.06 to 0.41 | 0.008 |
| Traub11 | −0.3 | −0.37 to −0.22 | <0.001 | −0.26 | −0.43 to −0.09 | 0.003 | −0.2 | −0.37 to −0.03 | 0.03 |
| Moore13 | −0.07 | −0.14 to 0.01 | 0.07 | −0.82 | −0.99 to −0.65 | <0.001 | 1.42 | 1.25 to 1.60 | <0.001 |
| ADA14 | 0.33 | 0.26 to 0.41 | <0.001 | 1.96 | 1.79 to 2.13 | <0.001 | −1.41 | −1.59 to −1.24 | <0.001 |
ADA, American Dietetic Association; IBW, ideal body weight
Figure 1.
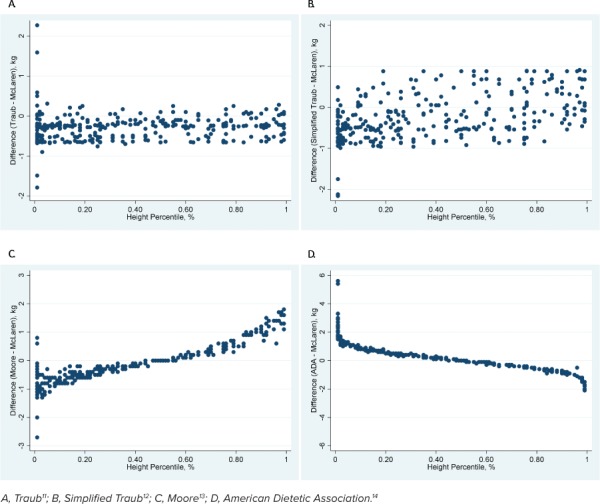
Comparison of the McLaren method with 4 other methods across height percentiles in cohort 1.
Figure 2.
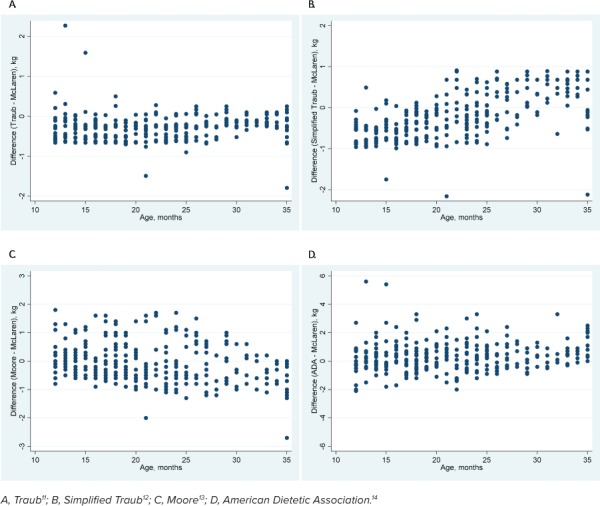
Comparison of the McLaren method with 4 other methods across ages in cohort 1.
For patients with height percentiles ≤5% and ≥95%, all methods were statistically different from the McLaren method, but the Traub equation was the closest approximation in both subgroups (Table 2). For the subgroup of patients with height percentiles ≤5%, the ADA method had a mean difference >10% as compared with the mean weight. For the subgroup of patients with height percentiles ≥95%, the Moore and ADA methods both had a mean difference >10% as compared with the mean weight.
Cohort 2. For the primary outcome, all methods except the BMI method were statistically different when compared with the McLaren method across all data points. The mean difference between the BMI and McLaren methods was 0.17 kg (95% CI: −0.07 to 0.40, p = 0.17). Mean differences for all methods are shown in Table 3. The Devine method had the largest mean difference, and this was >10% different from the mean weight. The graphical depictions show the trend of all methods compared with the McLaren method across all height percentiles (Figure 3) and ages (Figure 4).
Table 3.
Cohort 2: Comparison of Different IBW Equations to the McLaren Method
| Methods | All Data Points (N = 1095) | ≤5th Percentile (n = 100) | ≥95th Percentile (n = 155) | ||||||
|---|---|---|---|---|---|---|---|---|---|
| Difference, kg (mean) | 95% CI | p value | Difference, kg (mean) | 95% CI | p value | Difference, kg (mean) | 95% CI | p value | |
| Simplified Traub12 | −2.39 | −2.62 to −2.15 | <0.001 | −0.54 | −1.13 to 0.06 | 0.08 | −1.72 | −2.35 to −1.08 | <0.001 |
| Traub11 | −0.66 | −0.90 to −0.43 | <0.001 | −0.56 | −1.16 to 0.03 | 0.06 | −0.62 | −1.25 to 0.02 | 0.06 |
| Moore13 | 1.02 | 0.78 to 1.26 | <0.001 | −2.48 | −3.07 to −1.89 | <0.001 | 6.09 | 5.45 to 6.72 | <0.001 |
| ADA14 | 0.25 | 0.02 to 0.49 | 0.04 | 8.05 | 7.45 to 8.64 | <0.001 | −7.58 | −8.22 to −6.94 | <0.001 |
| Devine9 | 6.04 | 5.62 to 6.46 | <0.001 | 8.35 | 6.25 to 10.45 | <0.001 | 6.31 | 4.99 to 7.64 | <0.001 |
| BMI15 | 0.17 | −0.07 to 0.40 | 0.17 | 1.6 | 1.01 to 2.19 | <0.001 | −2.09 | −2.72 to −1.45 | <0.001 |
ADA, American Dietetic Association; BMI, body mass index; IBW, ideal body weight
Figure 3.
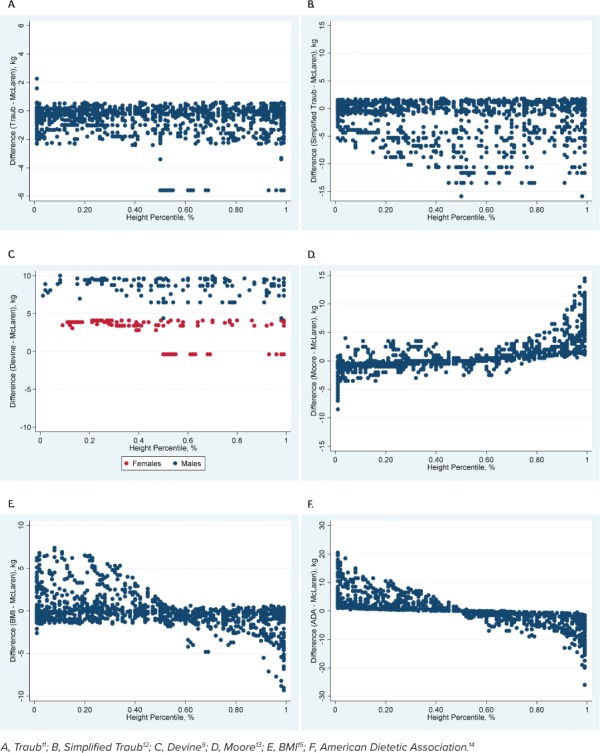
Comparison of the McLaren method with 6 other methods across height percentiles in cohort 2.
Figure 4.
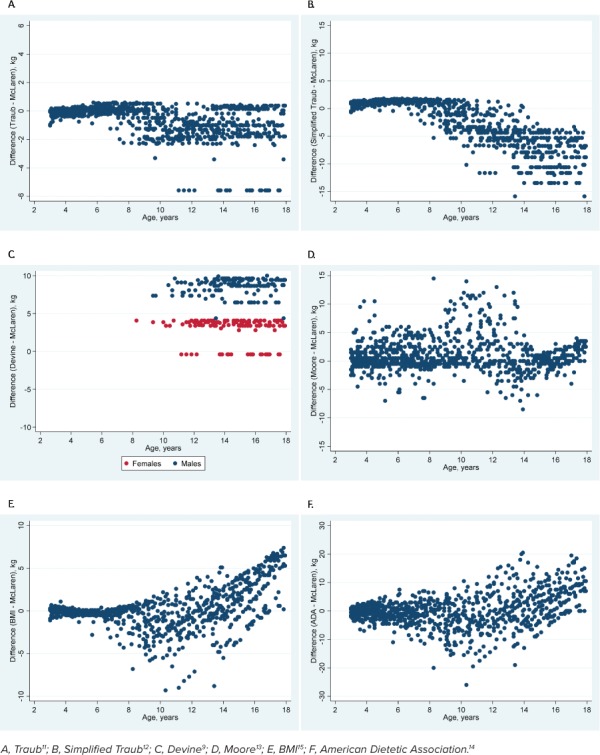
Comparison of the McLaren method with 6 other methods across ages in cohort 2.
For patients with height percentiles ≤5%, both the simplified Traub (p = 0.08) and Traub equation (p = 0.06) were not statistically different from the McLaren method. The ADA and Devine methods both had mean differences >10% as compared with the mean weight (Table 3). For patients with height percentiles ≥95%, only the Traub equation (p = 0.06) was not statistically different from the McLaren method. The ADA, Devine, and BMI methods all had mean differences >10% as compared with the mean weight (Table 3).
For patients 3 to <8 years old, the Traub equation (p = 0.82) and BMI method (p = 0.05) were not statistically different from the McLaren method (Table 4). For patients ≥8 years old, all methods were statistically different from the McLaren method, but the BMI method was the closest approximation (p = 0.02). The Devine method had a mean difference >10% as compared with the mean weight of patients ≥8 years old (Table 4).
Table 4.
Cohort 2: Sub-group Comparison of Different IBW Equations to the McLaren Method in Cohort 2
| Methods | 3 to <8 yr old (n = 388) | ≥ 8 yr old (n = 707) | ||||
|---|---|---|---|---|---|---|
| Difference, kg (mean) | 95% CI | p value | Difference, kg (mean) | 95% CI | p value | |
| Simplified Traub12 | 1.12 | 0.95 to 1.28 | <0.001 | −4.92 | −5.27 to −4.56 | <0.001 |
| Traub11 | −0.02 | −0.18 to 0.15 | 0.82 | −1.13 | −1.48 to −0.78 | <0.001 |
| Moore13 | 0.72 | 0.55 to 0.88 | <0.001 | 1.24 | 0.88 to 1.59 | <0.001 |
| ADA14 | −0.5 | −0.67 to −0.34 | <0.001 | 0.8 | 0.45 to 1.15 | <0.001 |
| BMI15 | −0.17 | −0.33 to −0.002 | 0.05* | 0.41 | 0.05 to 0.76 | 0.02 |
| Devine9 | N/A | N/A | N/A | 6.04 | 5.56 to 6.51 | <0.001 |
ADA, American Dietetic Association; BMI, body mass index; IBW, ideal body weight
* Nominal p value is 0.047, but not statistically significant after application of the Benjamini-Hochberg procedure for multiple comparisons.
Discussion
Accurate estimation of IBW in the pediatric population is vital for dosing of many medications, classifying nutritional status, and dosing of tidal volume in mechanically ventilated patients.1,2 With the prevalence of children with obesity reaching an epidemic level in the United States, significant challenges are present with these scenarios. In regards to medications, individuals with obesity generally have a larger volume of distribution for lipophilic medications. For hydrophilic medications, patients with obesity may have larger or smaller volume of distribution due to increased lean body mass, blood volume, and decreased percentage of total body volume.4,20,21 It is often impossible to know the true IBW of a patient, so multiple methods of estimation have been proposed in the literature. Previous research suggested that these various methods could produce different calculated IBWs, especially in older children and children further away from the 50th height percentile.7
The various methods also have different pros and cons to their use. The McLaren method offers the advantage of only needing a growth chart, but it does not account for patient's age in the final IBW determination. In addition, some children may be too tall to be estimated by this method. The Devine method does not require any graphing, but it is designed for adults and many pediatric patients are not more than 60 inches tall. The Traub and simplified Traub equations do not account for the patient's age. The Moore method offers the advantage of only needing a growth chart, but approximation is required when a patient's height does not fall exactly on one of the percentile lines on the growth chart. The ADA method can be graphed quicker than the McLaren or Moore methods, but it does not account for patient's height. The BMI method has the advantage of being able to account for normal patterns of body composition changes, but it requires multiple steps of both graphing on a BMI growth chart and calculating the value using an equation.
In this study, we assessed the different methods used to calculate IBW in pediatric patients and compared the results with the McLaren method. For patients in cohort 1, only the Moore method was not statistically different from the McLaren method across all data points. However, graphical depictions suggested that the Moore method would still underestimate IBW when compared with the McLaren method at height percentiles <50% and overestimate IBW at height percentiles >50%.
Although all methods in cohort 1 except the Moore method showed statistical differences, they might not have been clinically significant. The mean weight of this cohort was 11.9 kg. None of the methods had a mean difference >10% from the mean IBW. The electronic medical record at the institution where this study was conducted would alert the clinicians if an entered weight was >10% different from a previously entered weight during the same admission, so any 10% difference from the mean weight of the population for either cohort was considered a clinically significant difference. Thus, none of the methods fit the definition of clinical significance. These results also suggested that the Moore method would be the best comparator of the McLaren method. However, the ADA method, Traub equation, and simplified Traub equation might also provide clinically similar IBW calculations when compared with the McLaren method.
When looking closer at the subgroup of patients with height percentiles ≤5% and ≥95% in cohort 1, all methods were statistically different from the McLaren method, but the Traub equation served as the closest approximation. For the subgroup of patients with height percentile ≤5%, the ADA method showed clinically significant differences, and thus, would not be recommended as a reasonable comparator of the McLaren method. In the same manner, for the subgroup of patients with height percentile ≥95%, the ADA and Moore methods showed clinically significant differences.
For patients in cohort 2, only the BMI method was not statistically different from the McLaren method across all ages and height percentiles. As such, it would serve as a reasonable comparator of the McLaren method. However, the graphical depictions suggested that the BMI method would still overestimate IBW when compared with the McLaren method at height percentiles <50% and underestimate IBW at height percentiles >50%. In addition, the graphical depictions suggested that as patients get older, the BMI method produces larger differences in IBW when compared with the McLaren method.
Of note, the Devine method was the only method that had a clinically significant mean difference in cohort 2 and would not be a reasonable comparator of the McLaren method. The ADA method, Traub equation, simplified Traub equation, BMI method, and Moore method all had mean differences that were <10% than the mean weight of 45.5 kg in cohort 2. As such, all methods except the Devine method might provide clinically similar IBW calculations when compared with the McLaren method.
When looking closer at the subgroup of patients with height percentiles ≤5%, the Traub and simplified Traub equations were not statistically different. The simplified Traub equation was the closest approximation of the IBW calculated by the McLaren method. In this subgroup, the ADA and Devine methods showed clinically significant differences and would not be reasonable comparators of the McLaren method. For patients with height percentiles ≥95%, only the Traub equation was not statistically different from the McLaren method. In this subgroup, the ADA, Devine, and BMI methods showed clinically significant differences and would not be reasonable comparators of the McLaren method.
For patients 3 to <8 years old, the Traub equation and BMI method were not statistically different from the McLaren method. For patients ≥8 years old, all methods were statistically different, but the BMI method served as the closest approximation. In this subgroup, the Devine method showed clinically significant differences and would not be a reasonable comparator of the McLaren method.
One limitation of this study was that we were not able to compare patients who were “too tall” for the McLaren method. With how the growth charts are depicted, boys taller than 177 cm and girls taller than 163 cm cannot have an IBW estimated by the McLaren method.7 Another limitation was that measurements were done manually using growth charts. For patients who fell between pre-set percentile lines (3%, 10%, 25%, 50%, 75%, 90%, and 97%), the exact IBW calculated might not have been exact. However, this manual approach is generalizable to actual practice when growth-chart based methods are used. Finally, the lack of a gold standard in calculating IBW is also a limitation. Although the McLaren method was used as the comparator because it is still commonly used in literature and has been recommended as a preferred method, no studies have definitely proven that it is the most accurate method of calculating IBW in pediatrics.2,7,13,16,17
Future research in the area of pediatric IBWs should assess specific height percentiles and age subgroups with larger sample sizes to validate the differences seen in this study. Although our overall sample size was quite large, the sample sizes were a lot smaller when we looked at subgroups with our secondary outcomes. It is possible that larger sample sizes for those subgroups could produce alternate equations that could serve as a more accurate calculation for IBWs in this population. Although our research suggested that the Traub equation was clinically comparable with the McLaren method across all pediatric patients, it still might not be the most accurate. Furthermore, gender is another subgroup that was not explored in this study. Future studies may consider gender as another factor that can potentially contribute to differences in calculating IBWs in the pediatric population.
Conclusions
In this study, the Moore method was the most accurate comparator of the McLaren method in patients aged 12 to <36 months, whereas the BMI method was the most accurate comparator of the McLaren method in patients aged ≥3 years. However, this did not hold true for patients with height percentiles ≤5% or ≥95%, or for ages 3 to <8 years old or ≥8 years old where other methods were more accurate comparators of the McLaren methods depending on the cohort. The Traub and simplified Traub equations seemed to be the most clinically useful IBW methods for patients with height percentiles ≤5% or ≥95%. Ultimately, this study showed that the various methods used to calculate pediatric IBW lead to differences of varying statistical and clinical significance. Larger sample sizes in a separate cohort will need to validate the results of this study and further investigate the most accurate methods for specific subgroups of varying age and height percentiles.
Acknowledgment
Data from this manuscript were presented at the Southeastern Residency Conference in Athens, GA, on April 26, 2018.
ABBREVIATIONS
- ADA
American Dietetic Association
- BMI
body mass index
- IBW
ideal body weight
Footnotes
Disclosure The authors declare no conflicts or financial interest in any product or service mentioned in the manuscript, including grants, equipment, medications, employment, gifts, and honoraria. The primary investigator (KK) had full access to all the data in the study and takes responsibility for the integrity of the data and the accuracy of the data analysis.
REFERENCES
- 1.Ross EL, Heizer J, Mixon MA et al. Development of recommendations for dosing of commonly prescribed medications in critically ill obese children. Am J Health Syst Pharm. 2015;72(7):542–556. doi: 10.2146/ajhp140280. [DOI] [PubMed] [Google Scholar]
- 2.Ward SL, Quinn CM, Steurer MA et al. Variability in pediatric ideal body weight calculation: implications for lung-protective mechanical ventilation strategies in pediatric acute respiratory distress syndrome. Pediatr Crit Care Med. 2018;19(12):e643–e652. doi: 10.1097/PCC.0000000000001740. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 3.Ogden CL, Carroll MD, Lawman HG et al. Trends in obesity prevalence among children and adolescents in the United States, 1988–1994 through 2013–2014. JAMA. 2016;315(21):2292–2299. doi: 10.1001/jama.2016.6361. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 4.Matson KL, Horton ER, Capino AC. Medication dosage in overweight and obese children. J Pediatr Pharmacol Ther. 2017;22(1):81–83. doi: 10.5863/1551-6776-22.1.81. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 5.Rowe S, Siegel D, Benjamin DK et al. Gaps in drug dosing for obese children: a systematic review of commonly prescribed emergency care medications. Clin Ther. 2015;37(9):1924–1932. doi: 10.1016/j.clinthera.2015.08.006. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 6.Becker P, Carney LN, Corkins MR et al. Consensus statement of the Academy of Nutrition and Dietetics/American Society for parenteral and enteral nutrition: indicators recommended for the identification and documentation of pediatric malnutrition (undernutrition) Nutr Clin Pract. 2015;30(1):147–161. doi: 10.1177/0884533614557642. [DOI] [PubMed] [Google Scholar]
- 7.Phillips S, Edlbeck A, Kirby M et al. Ideal body weight in children. Nutr Clin Pract. 2007;22(2):240–245. doi: 10.1177/0115426507022002240. [DOI] [PubMed] [Google Scholar]
- 8.Mclaren DS, Read WW. Classification of nutritional status in early childhood. Lancet. 1972;2(7769):146–148. doi: 10.1016/s0140-6736(72)91324-4. [DOI] [PubMed] [Google Scholar]
- 9.Devine BJ. Gentamicin therapy. Drug Intell Clin Pharm. 1974;8:650–655. [Google Scholar]
- 10.Peterson CM, Thomas DM, Blackburn GL et al. Universal equation for estimating ideal body weight and body weight at any BMI. Am J Clin Nutr. 2016;103(5):1197–1203. doi: 10.3945/ajcn.115.121178. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 11.Traub SL, Kichen L. Estimating ideal body mass in children. Am J Hosp Pharm. 1983;40(1):107–110. [PubMed] [Google Scholar]
- 12.Taketomo CK, Hodding JH, Kraus DM. Pediatric & Neonatal Dosage Handbook. 24th ed. Alphen aan den Rijn, Netherlands: Wolters Kluwer; 2017. p. 2159. [Google Scholar]
- 13.Moore BJ, Durie PR, Forstner GG et al. The assessment of nutritional status in children. Nutr Res. 1985;57:97–99. [Google Scholar]
- 14.Klawitter BM. Nutrition assessment of infants and children. In: Nevin-Folino NL, editor. Pediatric Manual of Clinical Dietetics. 2nd ed. Chicago, IL: American Dietetic Association; 2003. p. 147. [Google Scholar]
- 15.Ringwald-Smith K, Cartwright C, Mosby T. Medical nutrition therapy in pediatric oncology. In: Elliott L, Molseed LL, McCallum PD, editors. The Clinical Guide to Oncology Nutrition. 2nd ed. Chicago, IL: American Dietetic Association; 2006. pp. 114–116. [Google Scholar]
- 16.Le grange D, Doyle PM, Swanson SA et al. Calculation of expected body weight in adolescents with eating disorders. Pediatrics. 2012;129(2):e438–e446. doi: 10.1542/peds.2011-1676. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 17.Collier H, Nasim M, Gandhi A. Prescribing in obese children: how good are paediatricians? Arch Dis Child. 2017;102(1):61–62. doi: 10.1136/archdischild-2016-310603. [DOI] [PubMed] [Google Scholar]
- 18.Clinical growth charts. Centers for Disease Control and Prevention website. https://www.cdc.gov/growthcharts/clinical_charts.htm Accessed September 1, 2017.
- 19.Benjamini Y, Hochberg Y. Controlling the false discovery rate: a practical and powerful approach to multiple testing. J R Statist Soc B. 1995;57(1):289–300. [Google Scholar]
- 20.Kendrick JG, Carr RR, Ensom MH. Pediatric obesity: pharmacokinetics and implications for drug dosing. Clin Ther. 2015;37(9):1897–1923. doi: 10.1016/j.clinthera.2015.05.495. [DOI] [PubMed] [Google Scholar]
- 21.Harskamp-van ginkel MW, Hill KD, Becker KC et al. Drug dosing and pharmacokinetics in children with obesity: a systematic review. JAMA Pediatr. 2015;169(7):678–685. doi: 10.1001/jamapediatrics.2015.132. [DOI] [PMC free article] [PubMed] [Google Scholar]


