Abstract
Non-random space use is common among animals across taxa and habitats. Social insects often use space non-randomly, outside as well as inside their nests. While such non-random space use outside the nest may improve foraging efficiency, inside the nest, it is often associated with the efficient division of labour. Non-random space use by adults on their nests has been hypothesized to result from dyadic dominance interactions, non-random distribution of tasks, differential activity levels, workers avoiding their queens or prophylactic avoidance of disease spread. These hypotheses are generally derived from species in which the tasks of the workers are themselves non-randomly distributed on the nest. Here, we study the primitively eusocial wasp Ropalidia marginata, in which tasks are not distributed non-randomly, and show that 62.4% ± 16.2% of the adults nevertheless use space on their nest non-randomly. In this species, we find that non-random space use may help optimizing nutritional exchange between individuals while prophylactically minimizing disease spread among nest-mates. We did not find evidence for the roles of dominance interactions, activity levels or location of larvae in non-random space use. Spatial organization appears to be a mechanism of minimizing the costs and maximizing the benefits of social life.
Keywords: social wasp, space use, spatial fidelity, social interactions, organizational immunity
1. Introduction
Non-random space use by animals is ubiquitous across taxa and habitats, and often arises as a result of concentrated use around areas rich in food [1], mates [2] or refuges [3]. Such non-random space use can affect information transfer [4], spread of disease [5] and reproductive fitness [6], and can in turn influence the landscape [7].
Social insects are known to use the space outside their nests non-randomly while foraging. For example, foragers of the ant species Paltothyreus tarsatus and Ectatomma ruidum repeatedly visit the same food patch in the habitat surrounding their nest using the same route [8,9]. Such non-random space use by foragers has been found to prevent disorientation, avoid predation and reduce time spent in searching for food [10]. In addition to using space outside their nests non-randomly, social insects also use space on their nests non-randomly. For instance, different age groups in the complex nests of harvester ants, Pogonomyrmex badius, disproportionately use nest areas at different depths such that the older workers are found closer to the nest entrance and younger individuals are found at incrementally greater depths while the brood is at the bottom [11]. Preferential use of parts of the nest by different workers has been suggested to lead to efficient division of labour. Similarly, workers of the ant Pheidole dentata preferentially perform sets of tasks that are spatially clustered in the nests potentially reducing the travel time between tasks [12]. Studies on the ants Odontomachus brunneus [13], Leptothorax unifasciatus [14] and Temnothorax albipennis [15,16], honeybees [17] and a bumblebee species [18] have shown that adult insects use space on the nest non-randomly, usually with nurses in the nest centre near the brood pile and foragers towards the periphery. At least five different hypotheses have been proposed to explain such non-random utilization of their nests by social insects.
Based on a study of the ant O. brunneus, the ‘interaction-based task allocation’ hypothesis proposes that dyadic dominance interactions among nest-mates result in dominant individuals manoeuvring subordinate individuals towards the periphery and thereby securing nursing opportunities near the central brood pile for themselves [13]. Based on a study of the ant L. unifasciatus, a second hypothesis, the ‘foraging for work’ hypothesis, attributes non-random space use to efficiency of task performance so that workers are in close proximity to the physical location of the tasks they have to perform; ants closer to the central brood pile perform nursing tasks while guards are at the entrance and foragers outside the nest [14]. Based on the observation that T. albipennis colonies are resilient with respect to spatial organization of workers on the nest even after the queen, the brood and a majority of the workers are removed, a third hypothesis, the ‘activity levels’ hypothesis, has been proposed. It states that inter-individual differences in activity levels of different individual ants lead to spatial sorting so that active individuals radiate outwards while less active ones remain in the centre [16]. A study on bumblebees showing that workers that maintained greater distance from their queens had better developed ovaries has led to a fourth hypothesis, which may be called ‘avoidance of queen’ hypothesis, and it states that by being farther away from the queen and avoiding her inhibitory influence, workers can develop their ovaries and have a reproductive potential that can be realized towards the end of the colony cycle [19]. A fifth hypothesis, the ‘organizational immunity’ hypothesis, posits that spatial compartmentalization within the high-density nests of social insects limits the interactions of susceptible entities like the reproductive and brood with incoming infections in the colony, which are often brought in by foraging workers [20].
Most of the above studies hypothesizing possible causes and consequences of non-random space use have used highly eusocial species such as ants and honeybees (with the exception of two studies in bumblebees [18,19]). At this stage, it is not clear if primitively eusocial species (other than bumblebees [18,21]) such as paper wasps exhibit non-random space use, and if so, what are its causes and consequences. We are aware of only one study on Polistes dominulus, which does not provide statistically significant evidence of non-random space use [22]. It is important to investigate non-random space use and test hypotheses to explain it in primitively eusocial species, because they differ in many features from highly eusocial species so that the hypotheses based on highly eusocial species may not be valid for them. For example, while highly eusocial species often have non-random distribution of tasks, primitively eusocial species generally do not; neither the distribution of brood nor the architecture of the nest suggests the need for non-random use of space. Here, we investigated the possible non-random space use and attempted to understand its causes and consequences in the primitively eusocial paper wasp Ropalidia marginata, a model system that is well suited for this purpose. Ropalidia marginata, being primitively eusocial, lacks queen-worker dimorphism, has small colonies (usually less than 100 individuals), a nearly two-dimensional gymnodomous (unenveloped) nest facilitating easy observation of the adults and the brood, and has a perennial nesting cycle. As expected of a primitively eusocial species, there is no evidence of non-random distribution of tasks. The single queen of the colony regulates worker reproduction by applying pheromones on the nest surface, rather than through physical dominance. Workers replace lost or dead queens rapidly although the queen's successors (potential queens, PQ) cannot be identified in the presence of the former queen; and yet, there is evidence that the workers themselves ‘know’ the identity of their successors. Besides, many other aspects of the biology of this species have been well documented over many years of study [23,24].
Having first ascertained that adult wasps of R. marginata use space on their nest non-randomly, we have attempted to understand its causes and consequences by checking (i) if dyadic dominance interactions are correlated with the spatial proximity between pairs of nest-mates, (ii) if proximity to larvae explains the location of workers who feed them, (iii) if active individuals are located at nest periphery while less active ones are in the colony centre, (iv) if spatial proximity to the queen is correlated with the ovarian development of the workers, and (v) if queens avoid foragers who potentially carry infections from their foraging trips.
2. Material and methods
We collected six nests of the paper wasp R. marginata from different locations within Bangalore, India, and transplanted them into the vespiary where the wasps were free to fly in and out for foraging. Video cameras were used to record all activities on the nest within the cage from 8.00 to 18.00 (10 h) for three consecutive days. All wasps were uniquely colour coded for identification. The videos were used to obtain behavioural profiles of the wasps, which included the data on rates of dominance behaviour, subordinate behaviour, feeding larvae, nest maintenance, soliciting (exchanging liquid food), snatching (exchanging solid food) and proportions of time spent in foraging (see electronic supplementary material, for details).
We manually located each wasp in each nest, every 6th min in 30 h of video recording, using the software ImageJ (1.50i) [25]. After scanning for the on-nest presence of each wasp in 300 frames, we extracted a total of 25 492 locations of all wasps. Areas of frequent use or ‘core areas’ were created as the 50% contour kernels for each of the 155 wasps from the 6 nests, and their area was recorded, using the smoothing parameter ‘href’ [26]. We define a wasp as displaying ‘spatial fidelity’ if it used its core area more intensively than expected by chance alone. To test if wasps used their core areas significantly more than expected by chance alone, it is not sufficient merely to ascertain that their core areas are less than 50% of the nest area. This is because the smoothing parameter is known to sometimes overestimate the core areas beyond 50% [26] (see electronic supplementary material). Thus, a wasp may be sighted more than 50% of the time in its core area by chance alone because the core area itself is more than 50%. To overcome this limitation imposed by the smoothing parameter, we calculated a test ratio for each wasp, which was the proportion of observed locations of the wasp that fell in the core area polygon, divided by the proportion of total nest area that was occupied by the core area polygon. If 50% of all sightings of a wasp lie in 50% of the nest area, then the test ratio would be one indicating no spatial fidelity. If a wasp shows spatial fidelity, i.e. uses its core area more than expected by chance alone, the test ratio should be significantly greater than 1.0. To ascertain if the observed test ratios were indeed significantly greater than 1.0, we compared the observed test ratio for each wasp with 1000 simulated test ratios. Each simulation for each wasp, involved sampling n locations with replacement (n being the number of frames in which the wasp was sighted on the nest), from all pooled locations of all wasps. A wasp was considered to show spatial fidelity if the observed test ratio was greater than 95% of the simulated test ratios.
We fitted a generalized linear mixed-effects model with binomial error family to identify the behaviours that predict the probability of a wasp showing spatial fidelity. Linear mixed effects models were used to address the following questions. Do the behavioural profiles of wasps that show spatial fidelity predict their core area sizes? Do the behavioural profiles of the wasps predict the Euclidean distance between the centroid of a wasp's spatial distribution and that of the collective colony distribution? Do the activity levels of the wasps predict their location with respect to the colony centre? The activity levels of wasps were measured as their step length (= the average distance each wasp moved in consecutive frames, as long as they were present in more than 10 frames in a day). To understand which dyadic interactions among nest-mates, such as dominance or dyadic food exchange (soliciting and exchanging food or building material), predict spatial overlap in core areas of pairs of wasps (see electronic supplementary material, figure S1), we used generalized linear mixed models using Template Model Builder (glmmTMB) with β-binomial error family and logit link [27].
We used larval feeding as a proxy for tasks and tested if larvae were non-randomly distributed using the Clark–Evans R measure [28] and a linear mixed-effects model. To test if larval locations dictated the core area location of wasps that fed them, we compared the density of larvae within the observed and five randomly placed core areas using mixed conditional logistic regression (often used for habitat selection studies; see electronic supplementary material, figure S2). To understand if the behaviour of wasps predicted their non-random distribution with respect to the colony centre (the colony centre was calculated as the centroid of the spatial distribution of all wasps pooled together; see electronic supplementary material, figure S3), we used a linear mixed-effects model. To understand the role of the queen in space use by the workers, we ranked all wasps from five nests (in one nest, the PQ was absent for 2 out of 3 days) based on their average real-time Euclidean distance from the queen whenever they were co-present on the nest. The PQ was identified after removing the queen on the fourth day, and all the wasps were then collected and stored in a −20°C refrigerator for calculating the ovarian index (see electronic supplementary material). To test if core area size influenced the number of social partners, we calculated the average number of social partners of each wasp with whom it had dyadic dominance or food exchange interactions using igraph [29] and used a linear mixed-effects model.
Confidence intervals for all models were computed using bootstrapping, and the p values were obtained by randomization. All mixed-effects models used nest ID as the random effect. All the statistical analysis was done in R (v. 1.1.453) [30].
3. Results
(a). Non-random space use
The core area of a wasp was identified as a densely used area on the nest in which the probability of sighting an individual was 50%. The overlap between core areas of a wasp across days was significantly higher than the overlap between core areas of different wasps (electronic supplementary material, figure S4; Wilcoxon rank-sum test, W = 272550, p < 0.001). When the core areas of each of the 155 wasps were determined by pooling data for each wasp over 3 days (figure 1), the size of the core areas ranged from 2.9 to 62.2% (mean ± s.d. = 30.6 ± 11.3%) of their nest areas. Of the 155 wasps studied from the 6 nests, 101 wasps used parts of the nest more than expected by chance, as determined by the test ratios (see Material and methods) and were thus classified as showing ‘spatial fidelity’. The proportions of wasps showing spatial fidelity in different nests ranged from 38.1 to 80.6% (mean ± s.d. = 62.4 ± 16.2%). The queen showed spatial fidelity in four of the six nests, and the PQ showed spatial fidelity in two of these nests. The wasps that showed spatial fidelity had smaller core areas (n = 101; mean ± s.d. = 25.3 ± 9.7%) than the wasps that did not show spatial fidelity (n = 54; mean ± s.d. = 40.4 ± 6.4%) (linear mixed-effects model: χ2 = 81.12, d.f. = 1, p < 0.001).
Figure 1.
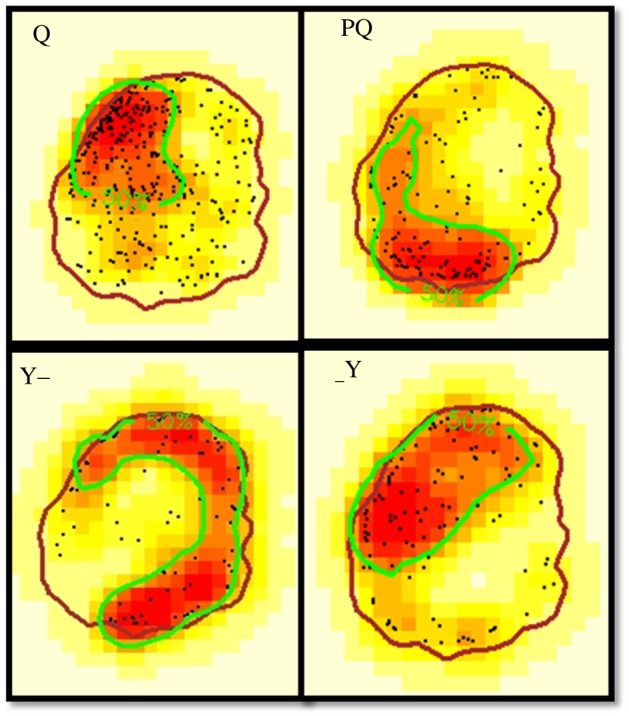
Kernel density estimation maps showing the core areas depicted for the queen, PQ and two workers in nest v57. The dots denote the locations occupied by the wasps in the 3 days, and the brown boundary denotes the nest boundary. (Online version in colour.)
(b). Predictors of non-random space use
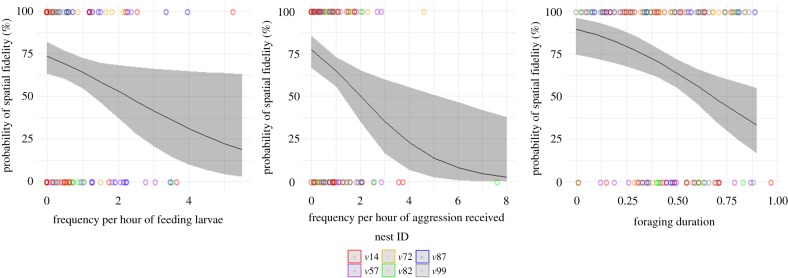
The frequencies per hour of feeding larvae and subordinate behaviour and the proportion of time spent foraging were strongly negatively correlated with the probability of a wasp showing spatial fidelity, while the frequencies of maintenance behaviour and dominance behaviour were not correlated with the tendency to show spatial fidelity (figure 2; electronic supplementary material, table S1; generalized mixed-effects model: FL: estimate = −0.45, χ2 = 4.52, p = 0.04; S: estimate = −0.61, χ2 = 8.9, p < 0.01; FG: estimate = −3.17, χ2 = 10.62, p = 0.002). The frequencies per hour of feeding larvae and subordinate behaviour were strongly positively correlated with the size of the core areas, while the frequencies per hour of maintenance behaviour, dominance behaviour and proportion of time spent in foraging were not correlated (electronic supplementary material, table S2; linear mixed-effects model: FL: estimate = 2.55, χ2 = 4.92, p = 0.03; S: estimate = 3.8, χ2 = 8.92, p = 0.003).
Figure 2.
Effect plots with data points colour coded by nest ID and grey bands around the regression line depicting the 95% confidence interval. Wasps (n = 155) that fed larvae frequently, those that frequently received aggression from nest-mates and those that spent a greater proportion of time foraging are less likely to display spatial fidelity. Generalized linear mixed-effects model (FL: estimate = −0.45, bootstrapped CI = −1.27 to −0.03, p = 0.04; S: estimate = −0.61, bootstrapped CI = −1.57 to −0.08, p = 0.01; FG: = −3.17, bootstrapped CI = −4.2 to −1.8, p = 0.002). (Online version in colour.)
The respective distances of different wasps from the colony centre were strongly positively correlated with the proportion of time that they spent in foraging. The frequency of feeding larvae had a strong negative correlation, while that of the subordinate behaviour was weakly negatively correlated with the distance of a wasp from the colony centre (electronic supplementary material, figure S5 and table S3; linear mixed-effects model: FL: estimate = −0.12, χ2 = 5.57, p = 0.02; FG: estimate = 0.63, χ2 = 8.86, p = 0.003). The frequency of nest maintenance and dominance behaviour did not significantly predict the distance of wasps from the colony centre.
(c). Testing hypotheses for non-random space use
(i). Hypothesis 1. Interaction-based task allocation: are dyadic dominance interactions correlated with the spatial proximity between nest-mates?
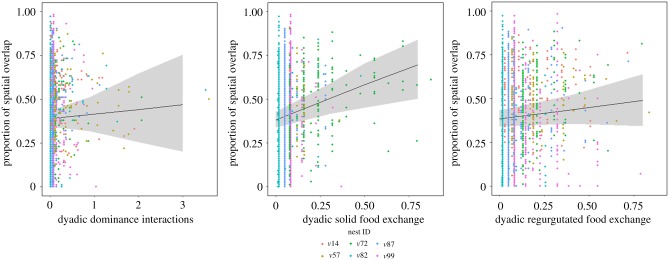
The centroids of core areas of wasps were significantly clumped on the nest (electronic supplementary material, table S4; R < 1, p < 0.001), but the extent of spatial overlap between pairs of wasps varied from 0 to 98%. Dyadic spatial overlap was not influenced by the dominance interactions between a pair of wasps but was strongly positively correlated with the dyadic exchange of food (figure 3; electronic supplementary material, table S5; linear mixed-effects model: SC: estimate = 0.45, bootstrapped CI = 0.08–1, p = 0.004; ST: estimate = 1.68, bootstrapped CI = 0.33–2.02, p < 0.001).
Figure 3.
Effect plots with data points colour coded by nest ID and grey bands around the regression line depicting the 95% confidence interval. Wasps (n = 155) that exchanged solid food (ST) frequently are located closer to each other on the nest, and exchange of regurgitated food (SC) is also weakly positively correlated with spatial overlap. Dyadic aggressive interactions are not correlated with dyadic spatial overlap. GlmmTMB with β-binomial error family and nest ID as random effect (SC: estimate = 0.45, bootstrapped CI = 0.08–1, p = 0.004; ST: estimate = 1.68, bootstrapped CI = 0.33–2.02, p < 0.001). (Online version in colour.)
(ii). Hypothesis 2. Non-random distribution of tasks drives spatial sorting of individuals: are core area locations of feeders correlated with their proximity to larvae?
The proportion of larvae inside the observed core area was not significantly different from the proportion of larvae in the five randomly placed core areas of the same size (mixed conditional logistic regression: coefficient: −0.85, z-value = −0.5, p = 0.62). Thus, the core areas were not necessarily situated close to the larvae. This could possibly be a result of the larvae themselves being randomly distributed on the nest surface because none of the brood stages were spatially clumped in any of the nests (electronic supplementary material, table S6; Clark–Evans R measure, p > 0.05). The larval cells were not found to be located significantly closer or farther from the nest centre when compared with non-larval cells (linear mixed-effects model; t = −0.55, χ2 = 0.313, p = 0.6).
(iii). Hypothesis 3. Inter-individual differences in activity levels: are active wasps located at nest periphery and inactive ones in colony centre?
Activity levels of wasps measured as average step lengths (distance travelled by a wasp between consecutive frames) were weakly negatively correlated with the distance from colony centre (electronic supplementary material, figure S6a; linear mixed-effects model: estimate = −0.39, bootstrapped CI = −0.82 to 0.008, p = 0.001).
(iv). Hypothesis 4. Avoidance of queen hypothesis: is worker ovarian development correlated with spatial proximity to queen?
The real-time Euclidean distance between the queen and a worker ranged from 1.6 to 3.9 cm (mean ± s.d. = 2.9 cm ± 0.37, n = 149 workers). When the Euclidean distance was ranked from 1 (closest to the queen) to 100 (farthest from the queen), the Euclidean distances of the PQs from their queens were intermediate and occupied ranks between 20.7 and 85.7 in the five nests. The PQ was also not observed to avoid the nest substrate more than other workers by sitting next to the nest (Wilcoxon rank-sum test with continuity correction; W = 429.5, p = 1) or foraging for extensive durations (Wilcoxon rank-sum test with continuity correction; W = 496.5, p = 0.52).
The ovarian development of the queen was, as expected, the highest among all nest-mates in each of the six nests (Kruskal–Wallis rank-sum test; χ2 = 17.34, d.f. = 2, p < 0.001) but, as shown in previous studies [23], the ovarian index of the PQ was not significantly different from the workers (Wilcoxon rank-sum test: W = 78.5, p = 0.07). The ovarian indices of workers were not predicted by their average real-time Euclidean distance from the queen within the nest (linear mixed-effects model: estimate = 0.25, bootstrapped CI = −1.3 to 3.3, randomized p = 0.8). The ovarian development of wasps was positively correlated with their core area size (electronic supplementary material, figure S7a; linear mixed-effects model: estimate = 1.3, χ2 = 10.8, d.f. = 1, p = 0.001) as well as their activity levels (electronic supplementary material, figure S7b; linear mixed-effects model: estimate = 0.07, χ2 = 22.7, d.f. = 1, p < 0.001).
(v). Hypothesis 5. Organizational immunity: does the queen avoid foragers and, more generally does limited space use reduce exposure to social partners?
We found that the real-time Euclidean distance between workers and their queen was strongly positively correlated with the proportion of time workers spent in foraging (figure 4; electronic supplementary material, table S7; linear mixed-effects model: FG: estimate = 0.41, χ2 = 11.94, p < 0.001). Queens also behaviourally avoided workers (moved away from approaching workers) more frequently than workers avoided other workers or their queens (electronic supplementary material, figure S8; mixed-effects logistic regression; z = 2.91, p = 0.003). The number of partners with whom the queen exchanged regurgitated food was significantly lower than of both PQ and other workers (electronic supplementary material, figure S9 and table S8; generalized linear mixed-effects model with the Poisson error distribution; queen: estimate = −0.94, z = −4.18, p < 0.001; PQ: estimate = 0.78, z = 8.5, p < 0.001). The core area size of a wasp, normalized by nest size, was positively correlated with the average number of socially interacting partners of a wasp (electronic supplementary material, figure S10; linear mixed-effects model; estimate = 0.22, bootstrapped CI = 0.13–0.36, χ2 = 16.4, p < 0.001).
Figure 4.
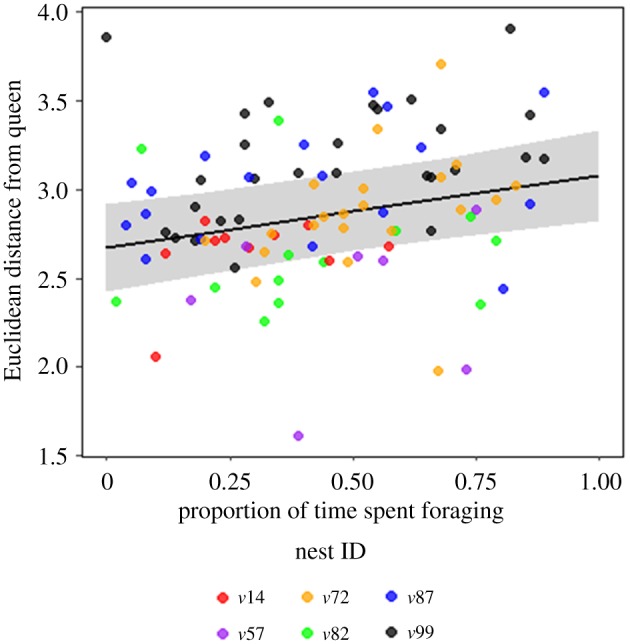
Effect plot with data points colour coded by nest ID and grey bands around the regression line depicting the 95% confidence interval. Foraging duration is strongly positively correlated with the average real-time Euclidean distance of workers (n = 149) from the queen. Linear mixed-effects model was used with nest ID as a random effect (FG: estimate = 0.41, bootstrapped CI = 0.2–0.5, p < 0.001). (Online version in colour.)
4. Discussion
In this study, we have measured the core areas of 155 wasps from six nests. Of these, only 101 satisfied the criterion of using their core areas more intensively than expected by chance alone. In the six nests studied, the percentage of wasps showing such spatial fidelity was 62.4 ± 16.2%. This value is intermediate between the ant L. unifasciatus [14], in which all individuals show spatial fidelity, and the ant T. albipennis [15] and the bumblebee Bombus impatiens [18], in which 45.4% and 12% of the individuals, respectively, show spatial fidelity. It may be noted that our experimental setup required the wasps to fly out for foraging, so that they showed normal activity levels. In the study with bumblebees, ad libitum food was provided, which may have caused lower than normal activity levels in that experiment [18]. Hence, we do not expect the number of wasps showing spatial fidelity to be exaggerated as has been suspected by the authors of the bumblebee study [18].
In an attempt to understand the causes and consequences of non-random space use, we have tested five hypotheses put forward by previous researchers studying other species of social insects. We did not find support for the ‘interaction-based task allocation’ hypothesis as proposed by Powell & Tschinkel [13] because in R. marginata, dyadic dominance interaction did not predict spatial overlap between pairs of wasps. However, the rates of dyadic food exchange did predict spatial overlap between pairs of wasps. Thus, although non-random space use in R. marginata may not be brought about by patterns of dominance–subordinate interactions, it may facilitate efficient nutritional exchange between wasps. Such spatial structuring resulting in efficient food exchange may be more widespread. For instance, exclusion of non-cooperative species in bacterial co-cultures is known to maintain cross-feedings between cooperating species [31].
We also did not find support for the ‘foraging for work’ hypothesis. Similar to ants and bumblebees, we find that wasps that fed larvae often were closer to the colony centre, while foragers were farther away. This may suggest some link between task performance and individual space use, but we provide evidence to suggest that the non-random arrangement of core areas of feeders is not related to maximizing their proximity to larvae. This we have done using larvae as a proxy for tasks (since feeding the larvae is an important task) and applying landscape-level habitat selection methods (see electronic supplementary material), with the spatial distribution of larvae as available mosaic of habitats to a wasp. This lack of correlation between locations of core areas and availability of larvae may be because we found that the larvae were themselves randomly located on the nest surface. We have shown that, rather than locating their core areas near the larvae, wasps that frequently fed larvae had larger core areas. This strategy of having larger core areas may help them to feed the larvae efficiently, not only by giving them access to randomly distributed larvae but also information about the dynamic nest landscape wherein eggs develop to larvae and larvae develop to pupae, asynchronously. This is reminiscent of alternative space use strategies observed in ovenbird populations in habitats with randomly distributed food resources where ‘floaters’ in the population hold bigger feeding areas and are thus better able to adapt to seasonal variation in food availability [32].
Furthermore, we did not find evidence in support of the ‘activity levels’ hypothesis, as proposed by Backen et al. [16]. Instead of inactive workers being in the centre and active ones in the periphery as in T. albipennis, we find the opposite pattern in R. marginata because activity levels measured as distance between locations in consecutive frames (step length) are negatively correlated with distance of the wasps from the colony centre. It is presently unclear why more active wasps are in the centre. Non-random space use in R. marginata does not support the ‘avoidance of queen’ hypothesis either, because the PQ is not farther away from the queen than other workers, unlike in the case of bumblebees [19]. This was ascertained by measuring the real-time distance between the queen and workers in co-occurring frames, which can detect both spatial and temporal avoidance. Besides, distance from the queen has no relation to levels of ovarian development. This may be because R. marginata queens do not use physical aggression to suppress their workers. They use pheromones to regulate worker reproduction, but they do so by rubbing their pheromones all over the nest. Therefore, it is not surprising that avoiding the queen is not a strategy to escape the inhibitory effects of the queen in this species.
Finally, we asked if non-random space use in R. marginata is consistent with the ‘organizational immunity’ hypothesis. Our results seem to support this hypothesis because the real-time Euclidean distance between queens and workers was positively correlated with the proportion of time workers spent in foraging. This may be either because queens avoided foragers or foragers avoided their queens. However, our behavioural observations show that queens actively avoided foragers who approached them and not the other way around. Foragers who spend time away from the nest and are exposed to the external environment are likely to be potential sources of infection, and it is known that irrespective of the infection status, animals display ‘disgust’ towards potentially infectious cues or individuals [33]. The fact that the majority of the wasps showed spatial fidelity is itself an indication that non-random space use is a prophylactic measure against infection because we found that individuals with smaller core areas interacted with significantly fewer social partners. Restricting space use is expected to help avoid infection spread. It has been shown, for example, that the number of partners in den-sharing possums (Trichosurus vulpecula) predicts tuberculosis infection [34]. In the case of social insects, interindividual interactions are even more likely to help spread infection because they are known to frequently exchange regurgitated food in a behaviour known as trophallaxis [35,36]. Therefore, we investigated whether queens of R. marginata avoid trophallactic interactions with multiple workers and found this to be the case. Perhaps counter-intuitively, the PQ had the most trophallactic partners. However, studies on humans find opposing effects of multiple contacts on stressed and non-stressed individuals [37], and we hypothesize that ovarian development in queens may compromise her immunity, making avoidance behaviour imperative. Owing to lower investment in ovarian development, the PQ might instead benefit from multiple trophallactic partners possibly by even gaining coalitionary support [38].
In summary, queens and workers in the primitively eusocial paper wasp R. marginata use space on their nests non-randomly with the majority of individuals showing spatial fidelity to small core areas, in spite of the brood itself being randomly distributed. Such non-random space use appears to be a prophylactic measure against the spread of infection as commensurate with nutritional exchange. However, variation in the space use patterns of individuals within a social group may fulfil various functional roles necessary for the ecological success of the collective. Further research is needed to examine how social groups maintain a balance between information and disease spread as commensurate with their need for frequent interactions and spatial proximity. Thus, non-random space use is a topic worthy of more intensive study in different kinds of social species.
Supplementary Material
Supplementary Material
Acknowledgements
We thank Kavita Isvaran, Vishwesha Guttal, Maria Thaker, Jenny Jandt and two anonymous reviewers for many helpful comments.
Ethics
Wasps in this study were collected and euthanized humanely, according to standard protocols.
Data accessibility
The raw data and R codes supporting this study have been provided as part of the electronic supplementary material.
Authors' contributions
R.G. and N.S. designed the study. N.S. conducted the study. R.G. and N.S. co-wrote the paper.
Competing interests
The authors declare no competing interests.
Funding
This work was supported by grants (to R.G.) from the Ministry of Environment, Forests and Climate Change, Government of India, Department of Science and Technology (including DST-FIST Program), the Science and Engineering Research Board (SERB), Department of Science and Technology, Department of Biotechnology (including DBT-IISc Partnership Program) and Council of Scientific and Industrial Research.
References
- 1.Avgar T, et al. 2015. Space-use behaviour of woodland caribou based on a cognitive movement model. J. Anim. Ecol. 84, 1059–1070. ( 10.1111/1365-2656.12357) [DOI] [PubMed] [Google Scholar]
- 2.Carranza J, Alvarez F, Redondo T. 1990. Territoriality as a mating strategy in red deer. Anim. Behav. 40, 79–88. ( 10.1016/S0003-3472(05)80667-0) [DOI] [Google Scholar]
- 3.Johnson JR, Mahan RD, Semlitsch RD. 2008. Seasonal terrestrial microhabitat use by gray treefrogs (Hyla versicolor) in Missouri oak-hickory forests. Herpetologica 64, 259–269. ( 10.1655/07-064.1) [DOI] [Google Scholar]
- 4.Jaffe AB, Trajtenberg M, Henderson R. 1993. Geographic localization of knowledge spillovers as evidenced by patent citations. Q. J. Econ. 108, 577–598. ( 10.2307/2118401) [DOI] [Google Scholar]
- 5.Viboud C, Bjørnstad ON, Smith DL, Simonsen L, Miller MA, Grenfell BT. 2006. Synchrony, waves, and spatial hierarchies in the spread of influenza. Science 312, 447–451. ( 10.1126/science.1125237) [DOI] [PubMed] [Google Scholar]
- 6.Gavin TA, Bollinger EK. 1988. Reproductive correlates of breeding-site fidelity in Bobolinks (Dolichonyx oryzivorus). Ecology 69, 96–103. ( 10.2307/1943164) [DOI] [Google Scholar]
- 7.Laundré JW, Hernández L, Ripple WJ. 2010. The landscape of fear: ecological implications of being afraid. Open Ecol. J. 3, 1–7. ( 10.2174/1874213001003030001) [DOI] [Google Scholar]
- 8.Déjean A, Beugnon G, Lachaud JP. 1993. Spatial components of foraging behavior in an African ponerine ant, Paltothyreus tarsatus. J. Insect Behav. 6, 271–285. ( 10.1007/BF01048109) [DOI] [Google Scholar]
- 9.Schatz B, Lachaud JP, Beugnon G. 1995. Spatial fidelity and individual foraging specializations in the Neotropical ponerine ant, Ectatomma ruidum Roger (Hymenoptera, Formicidae). Sociobiology 26, 269–282. ( 10.1007/bf01242563) [DOI] [Google Scholar]
- 10.Wehner R, Harkness RD, Schmid-Hempel P. 1983. Foraging strategies in individually searching ants, Cataglyphis bicolor (Hymenoptera, Formicidae). Akad. Wiss. Lit. Mainz, Math. Naturwiss. Kl. Stuttgart, Germany: Fischer. [Google Scholar]
- 11.Tschinkel WR. 1999. Sociometry and sociogenesis of colonies of the harvester ant, Pogonomyrmex badius: distribution of workers, brood and seeds within the nest in relation to colony size and season. Ecol. Entomol. 24, 222–237. ( 10.1046/j.1365-2311.1999.00184.x) [DOI] [Google Scholar]
- 12.Wilson EO. 1976. Behavioral discretization and the number of castes in an ant species. Behav. Ecol. Sociobiol. 1, 141–154. ( 10.1007/BF00299195) [DOI] [Google Scholar]
- 13.Powell S, Tschinkel WR. 1999. Ritualized conflict in Odontomachus brunneus and the generation of interaction-based task allocation: a new organizational mechanism in ants. Anim. Behav. 58, 965–972. ( 10.1006/anbe.1999.1238) [DOI] [PubMed] [Google Scholar]
- 14.Sendova-Franks AB, Franks NR. 1995. Spatial relationships within nests of the ant Leptothorax unifasciatus (Latr.) and their implications for the division of labour. Anim. Behav. 50, 121–136. ( 10.1006/anbe.1995.0226) [DOI] [Google Scholar]
- 15.Richardson TO, Giuggioli L, Franks NR, Sendova-Franks AB. 2017. Measuring site fidelity and spatial segregation within animal societies. Meth. Ecol. Evol. 8, 965–975. ( 10.1111/2041-210X.12751) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 16.Backen SJ, Sendova-Franks AB, Franks NR. 2000. Testing the limits of social resilience in ant colonies. Behav. Ecol. Sociobiol. 48, 125–131. ( 10.1007/s002650000219) [DOI] [Google Scholar]
- 17.Seeley TD. 1982. Adaptive significance of the age polyethism schedule in honeybee colonies. Behav. Ecol. Sociobiol. 11, 287–293. ( 10.1007/BF00299306) [DOI] [Google Scholar]
- 18.Jandt JM, Dornhaus A. 2009. Spatial organization and division of labour in the bumblebee Bombus impatiens. Anim. Behav. 77, 641–651. ( 10.1016/j.anbehav.2008.11.019) [DOI] [Google Scholar]
- 19.Jandt JM, Dornhaus A. 2011. Competition and cooperation: bumblebee spatial organization and division of labor may affect worker reproduction late in life. Behav. Ecol. Sociobiol. 65, 2341–2349. ( 10.1007/s00265-011-1244-9) [DOI] [Google Scholar]
- 20.Stroeymeyt N, Casillas-Pérez B, Cremer S. 2014. Organisational immunity in social insects. Curr. Opin. Insect Sci. 5, 1–5. ( 10.1016/j.cois.2014.09.001) [DOI] [PubMed] [Google Scholar]
- 21.Crall JD, Gravish N, Mountcastle AM, Kocher SD, Oppenheimer RL, Pierce NE, Combes SA. 2018. Spatial fidelity of workers predicts collective response to disturbance in a social insect. Nat. Commun. 9, 1201 ( 10.1038/s41467-018-03561-w) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 22.Baracchi D, Zaccaroni M, Cervo R, Turillazzi S. 2010. Home range analysis in the study of spatial organization on the comb in the paper wasp Polistes dominulus. Ethology 116, 579–587. ( 10.1111/j.1439-0310.2010.01770.x) [DOI] [Google Scholar]
- 23.Gadagkar R. 2001. The social biology of Ropalidia marginata: toward understanding the evolution of eusociality. Cambridge, MA: Harvard University Press. [Google Scholar]
- 24.Bhadra A, Gadagkar R. 2008. We know that the wasps ‘know’: cryptic successors to the queen in Ropalidia marginata. Biol. Lett. 4, 634–637. ( 10.1098/rsbl.2008.0455) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 25.Schneider CA, Rasband WS, Eliceiri KW. 2012. NIH Image to ImageJ: 25 years of image analysis. Nat. Methods 9, 671–675. ( 10.1038/nmeth.2089) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 26.Calenge C. 2011. Home range estimation in R: the adehabitatHR package. Saint Benoist, France: Office national de la classe et de la faune sauvage.
- 27.Magnusson A, et al. 2017. Package ‘glmmTMB’. R Package Version 0.2. 0.
- 28.Clark PJ, Evans FC. 1954. Distance to nearest neighbor as a measure of spatial relationships in populations. Ecology 35, 445–453. ( 10.2307/1931034) [DOI] [Google Scholar]
- 29.Csardi G, Nepusz T. 2006. The igraph software package for complex network research. InterJournal Complex Syst. 1695, 1–9. [Google Scholar]
- 30.R Core Team. 2016. R: a language and environment for statistical computing. Vienna, Austria: R Foundation for Statistical Computing; See https://www.R-project.org/. [Google Scholar]
- 31.Pande S, Kaftan F, Lang S, Svatoš A, Germerodt S, Kost C. 2016. Privatization of cooperative benefits stabilizes mutualistic cross-feeding interactions in spatially structured environments. ISME J. 10, 1413–1423. ( 10.1038/ismej.2015.212) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 32.Brown DR, Sherry TW. 2008. Solitary winter roosting of ovenbirds in core foraging area. Wilson J. Ornithol. 120, 455–460. ( 10.1676/07-084.1) [DOI] [Google Scholar]
- 33.Weinstein SB, Buck JC, Young HS. 2018. A landscape of disgust. Science 359, 1213–1214. ( 10.1126/science.aas8694) [DOI] [PubMed] [Google Scholar]
- 34.Corner LA, Pfeiffer DU, Morris RS. 2003. Social-network analysis of Mycobacterium bovis transmission among captive brushtail possums (Trichosurus vulpecula). Prev. Vet. Med. 59, 147–167. ( 10.1016/S0167-5877(03)00075-8) [DOI] [PubMed] [Google Scholar]
- 35.Bailey L, Gibbs J. 1964. Acute infection of bees with paralysis virus. J. Insect Pathol. 6, 395–407. [Google Scholar]
- 36.Jouvenaz DP. 1986. Diseases of fire ants: problems and opportunities. In Fire ants and leaf-cutting ants: biology and management (eds Lofgren CS, Vander Meer RK), pp. 327–338. Boulder, CO: Westview Press. [Google Scholar]
- 37.Hamrick N, Cohen S, Rodriguez MS. 2002. Being popular can be healthy or unhealthy: stress, social network diversity, and incidence of upper respiratory infection. Health Psychol. 21, 294 ( 10.1037/0278-6133.21.3.294) [DOI] [PubMed] [Google Scholar]
- 38.Patton JQ. 2005. Meat sharing for coalitional support. Evolution and human behavior. 26, 137–157. ( 10.1016/j.evolhumbehav.2004.08.008) [DOI] [Google Scholar]
Associated Data
This section collects any data citations, data availability statements, or supplementary materials included in this article.
Supplementary Materials
Data Availability Statement
The raw data and R codes supporting this study have been provided as part of the electronic supplementary material.