Abstract
Thirty years ago, Coullet et al. proposed that a special optical field exists in laser cavities bearing some analogy with the superfluid vortex. Since then, optical vortices have been widely studied, inspired by the hydrodynamics sharing similar mathematics. Akin to a fluid vortex with a central flow singularity, an optical vortex beam has a phase singularity with a certain topological charge, giving rise to a hollow intensity distribution. Such a beam with helical phase fronts and orbital angular momentum reveals a subtle connection between macroscopic physical optics and microscopic quantum optics. These amazing properties provide a new understanding of a wide range of optical and physical phenomena, including twisting photons, spin–orbital interactions, Bose–Einstein condensates, etc., while the associated technologies for manipulating optical vortices have become increasingly tunable and flexible. Hitherto, owing to these salient properties and optical manipulation technologies, tunable vortex beams have engendered tremendous advanced applications such as optical tweezers, high-order quantum entanglement, and nonlinear optics. This article reviews the recent progress in tunable vortex technologies along with their advanced applications.
Subject terms: Optical physics, Optical physics, Optical physics, Optical physics
Optical vortices: looking back at twisted light
Commemorating the 30th anniversary of the prediction of optical vortices by theoretical physicist Pierre Coullet and colleagues, researchers in China review the development of understanding and applications of these intriguing phenomena. Xing Fu at Tsinghua University, Xiaocong Yuan at Shenzhen University and co-authors, explain how the concept of optical vortices emerged from observed similarities between the behaviour of fluid vortices and some forms of laser light. The light waves of optical vortices are twisted around their direction of travel, with a point of zero intensity at their centre. The authors survey the steady refinement of techniques used to create optical vortices, and explore their applications. Prominent applications include sophisticated optical computing processes, novel microscopy and imaging techniques, the creation of ‘optical tweezers’ to trap particles of matter, and optical machining using light to pattern structures on the nanoscale.
Introduction
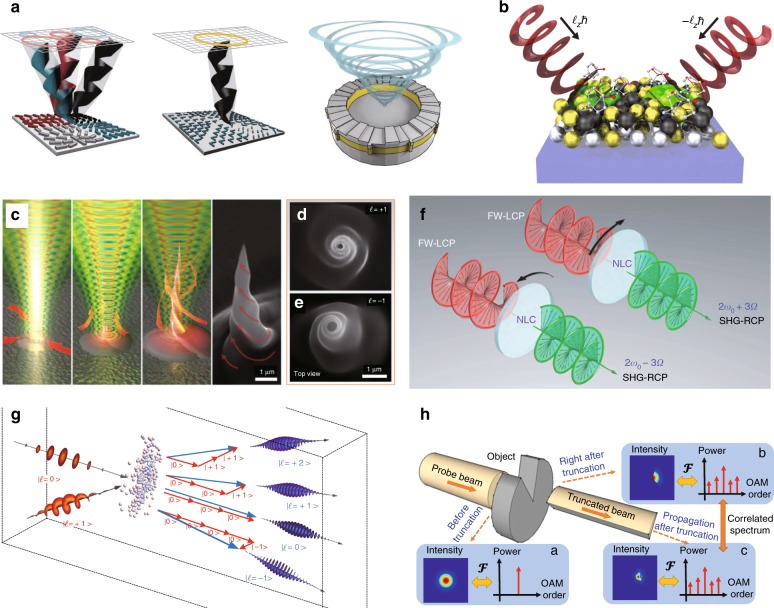
Vortices are common phenomena that widely exist in nature, from quantum vortices in liquid nitrogen to ocean circulation and typhoon vortices and even to spiral galaxies in the Milky Way, manifesting themselves not only in macroscopic matter but also in structured electromagnetic and optical fields. This year is the 30th anniversary of the birth of optical vortices (OVs). In 1989, Coullet et al.1 found the vortex solutions of the Maxwell-Bloch equations and created the concept of OVs, inspired by hydrodynamic vortices. Before the proposal of OVs, the analogy between laser physics and fluids/superfluids was already recognized2 as early as 1970 by reducing the laser equations to complex Ginzburg–Landau equations (CGLEs), which constitute a class of universal models describing pattern formation in a vast variety of phenomena such as superconductivity, superfluidity, and Bose-Einstein condensation3. Later, many hydrodynamic features, such as chaos, multistability, and turbulence, were analogically studied in optical fields4–6 and observed in laser systems7–9. Among the various hydrodynamic effects, the vortex soliton is quite attractive due to its distinctive structure carrying a singularity5–7. Analogous to the flow singularity in a fluid vortex, an optical vortex soliton has a phase singularity that appears as an isolated dark spot possessing the topological charges (TCs) of a helical phase5,10. Novel optical vortex solitons were intensively explored based on CGLEs. For instance, stable vortex solitons11 and dissipative vortex solitons with trapping potentials12 can be solved by two-dimensional CGLEs. Topologically multicharged rotating vortex solitons13 and vortex excitation with feedback14 in lasers were also studied by CGLEs. Moreover, complicated three-dimensional toroidal dissipative vortex solitons15 can also be characterized by CGLEs with high-order nonlinearity. In 1992, Allen et al.16 proposed the orbital angular momentum (OAM) in vortex beams (VBs) where the OVs propagate in paraxial beams, which unveiled a new understanding of the connection between macroscopic optics and quantum effects. As a typical representative of OVs, a VB has become a classical tool to study the properties of OVs because its generation can be easily realized in the laboratory17. VBs characterized by Hilbert factor exp(iℓθ), e.g., the Laguerre–Gaussian (LG) modes, can carry OAM equivalent to ℓℏ per photon (ℓ is an integer number), and this angular momentum (AM) can be much greater than the spin angular momentum (SAM) related to the photon spin10. The general results of these investigations created a new chapter of modern optics, i.e., singular optics18, which is a great leap forward in the development of traditional optics.
In the first 10 years, 1989–1999, the studies on OVs mainly focused on establishing fundamental theories and exploring basic physical phenomena, paving the way for further studies of the light–matter interaction, topological structures, and quantum nature of light. For instance, the dynamics of transverse pattern formation5,6, the interaction and OAM transfer between OVs and particles19–21, vortex solitons in a nonlinear medium22–24, nonlinear OAM-frequency transformation25,26, the topological phase in OVs27, the rotational Doppler effect28, and multi-singularity arrays or vortex crystals5,29 were thoroughly studied. These novel theories lay the foundation for extending further widespread applications by using the unique properties of OVs.
In the second 10 years, 1999–2009, with the development of OAM manipulation, tremendous new applications rapidly emerged. In 2001, Zeilinger’s group30 realized the OAM-entangled photon pair, bringing OVs or twisted photons into quantum applications31. In 2002, Dholakia’s group trapped particles with controlled rotation32 and a three-dimensional structure33 by VBs, expanding the applications of optical tweezers34. In 2003, Harwit35 demonstrated astrophysical OAM light generation and its applications in astrometry. In 2004, Zhuang36 used VBs as tweezers to assemble DNA biomolecules, opening up biomedical applications of OVs. In 2005, Ritsch-Marte’s group37 used OAM in microscopy and imaging, and Tamburini et al.38 reported a super-diffraction-limit imaging approach using OAM. In 2008, Barreiro et al.39 presented a coding technology using OAM, giving VBs great advantage for use in optical communications. During the decade, OVs were extended to almost every field of advanced optics.
In the last 10 years, 2009–2019, vortex and OAM applications have made many breakthroughs in rapid succession. In 2010, the optical lattice in far-field diffraction of OVs was unveiled as a very prompt and handy way to detect the TC40. In 2011, Capasso’s group41 proposed the generalized laws of reflection and refraction, guiding OV generation in nanoscale metasurfaces. In 2012, OAM beams were directly generated in a nanoscale emitter42. In 2013, Willner’s group demonstrated terabit-scale high-capacity optical communication via OAM multiplexing in both free space43 and fibres44. In 2016, Zeilinger’s group45 generated extreme OAM states of over 10,000ℏ and realized quantum entanglement of these states. In the recent three years, increasing numbers of tunable properties of OVs have been flexibly controlled at the nanoscale, including SAM–OAM conversion for classical46 and quantum light47, tunable wavelength from visible48,49 to X-ray light50, ultra-broadband tunable OAM51, and tunable chirality52. Moreover, the time-varying OAM was recently revealed in extreme-ultraviolet VB with time-delay-tunable high harmonic generation53. To date, OVs have brought about numerous innovations in various fields and are still enabling great novelties with improved tunability.
Throughout the roadmap summarized above and depicted in Fig. 1, we can divide the 30-year development into three stages: the first 10 years, the fundamental theories stage; the second, the application development stage; and the third, the technology breakthrough stage. These three stages share one common theme of pursuing improved tunability of OVs because the realization of a broader tunable range of OVs can always benefit the birth of new applications. Thus, we propose the tunability of OVs as a better way to describe state-of-the-art achievements of OVs. Traditionally, tunable light always means that the wavelength can be tuned and sometimes means that the pulse width can be tuned for a pulsed laser; however, the tunability of OVs should be expanded to more dimensions due to their exotic properties. The tunability of OVs includes not only the spectral and temporal tunability but also the OAM-, chirality-, TC-, and singularity-distribution tunability. We present reviews in succession on the historical progress in the new tunable methods of OVs driven by the fundamental theories and then the numerous novel applications engendered by the improved tunability of OVs. In the “Properties of OVs” section, we review the fundamental theories and properties of OVs, providing a better understanding of the corresponding applications enabled by the unique properties. In “Progress in vortex generation, tuning, and manipulation” section, we review the generation and manipulation methods of OVs, developed from tuning the TC of a single singularity to controlling a multi-singularity array, including wavelength-, temporal-, and OAM-tuning technologies. In “Advanced applications of tunable VBs” section, we comprehensively review various advanced applications derived from vortex manipulation. Concluding remarks and prospects are given in the “Conclusions and perspectives” section.
Fig. 1. Roadmap of the 30-year development of optical vortices from 1989 to 2019, including significant theoretical and technical breakthroughs with corresponding references.
Reprinted with permission from ref. 1, Copyright (2019), with permission from Elsevier. Reprinted with permission from refs. 5,16,19,25,27,28,38,40. Copyright (2019) by the American Physical Society. From refs. 29,32,33,41,42,44,46,47. Reprinted with permission from AAAS. Reprinted by permission from Springer Nature: Nature30,34,59,60, Nature Physics31,39, Nature Photonics43,50,52, Nature Communications48,49, Light: Science & Applications51, Copyright (2019). From ref. 57. Reproduced by permission of IOP Publishing
Properties of OVs
Singularity and topological charge
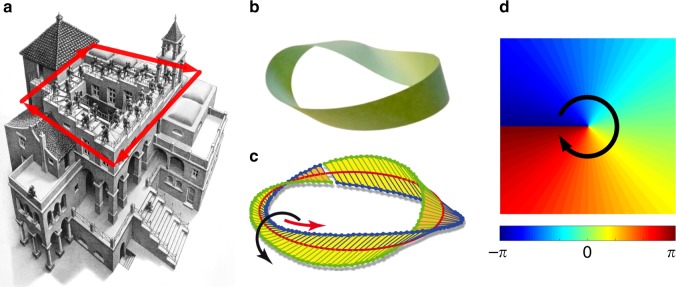
The salient properties of OVs are mostly related to the topological phase structure. Early in the 1970s before OVs were first observed, the topological structure in the wave phase was already under study. Nye and Berry54 demonstrated that wave trains with dislocations could induce a vortex structure where a singularity could be solved in the wave equation, which laid the foundation for the study of vortices in air, water, and even light waves, pushing the discovery of OVs. To understand the profound topology in a plain way, we can refer to a familiar artwork exhibiting a similar structure. Escher’s painting Ascending and Descending shows an impossible scenario where the stairs are ascending clockwise yet have a seamless connection to their origin after a roundtrip, which is an artistic implementation of the Penrose Stairs55, as illustrated in Fig. 2. This structure is impossible in real space but possible in phase space. If the phase angle continually increases clockwise along a closed loop from 0 to 2πℓ and returns to the origin, where the integer ℓ is called the TC, the angle zero is exactly equal to 2πℓ, forming a continuous phase distribution along the closed loop, similar to the topology of the well-known Möbius strip56. The centre spot of the closed loop where the phase cannot be defined is a phase singularity. The definition of the TC of a singularity for the phase distribution is given by:
| 1 |
where C is a tiny closed loop surrounding the singularity. For the light field with phase distribution exp(iℓθ) carrying OAM of ℓℏ per photon, the TC of the centre phase singularity is ℓ. The effect of TC is actually commonly seen in our daily life, e.g., the time distribution on earth has a singularity at the North Pole with a TC of 24 h, the duration that the earth takes to rotate one cycle. The continuous phase along the closed loop results in an integer TC. However, as a peculiar case, a non-integer TC was also experimentally and theoretically investigated in OVs57,58. A phase singularity with a certain TC is a representation of a very simple vortex soliton yet acts as an important unit element in that more complex hydrodynamic vortices with chaos, attractors, and turbulence can be seen as the combination of a set of various singularities. This basic description is widely applicable to air53, water4, light1, electron59, and neutron60 vortex fields.
Fig. 2. Basic topological structure of vortex from art to science.
The topological structures of a the Penrose Stair55, b, c a Möbius strip56, and d the phase of a vortex soliton (Hilbert factor) are isomorphic, i.e., a physical value (displacement or angle) continually increases along a closed loop and coincides exactly with the origin after a roundtrip. c From ref. 56. Reprinted with permission from AAAS
Orbital angular momentum and vortex beams
A VB is a paraxial light beam possessing Hilbert factor exp(iℓθ) and carrying OVs along the propagation axis. OVs are not restricted to VBs, yet as typical OVs, VBs carrying OAM, also called OAM beams, are almost the most attractive form of OVs due to their unique quantum-classical-connection properties. There are already many review articles on OAM, especially on vortex generation61,62, OAM on metasurfaces63, and basic OAM theories and applications64,65. However, few studies have focused on vortex tunability, which is the main theme of this article. For the introduction of basic theories of OAM, previous reviews usually used the well-known Poynting picture to describe the AM of the photon66,67, which leads to some difficulties, such as complex expressions of OAM and SAM, incompatibility with quantum optics, and the Abraham–Minkowski dilemma68. Here, we review the recently proposed new theory of the canonical picture69,70, which can overcome these difficulties, to introduce basic properties of OAM. The canonical momentum of light is represented as
| 2 |
where H is the magnetizing field. Gaussian units with , , and are used. The canonical SAM and OAM densities are expressed as
| 3 |
The total AM of light is J = S + L. For a light beam, a rotating polarization leads to SAM, while a rotating wavefront leads to OAM. Consider a VB propagating along the z-axis:
| 4 |
The average SAM and OAM can be derived as69,70
| 5 |
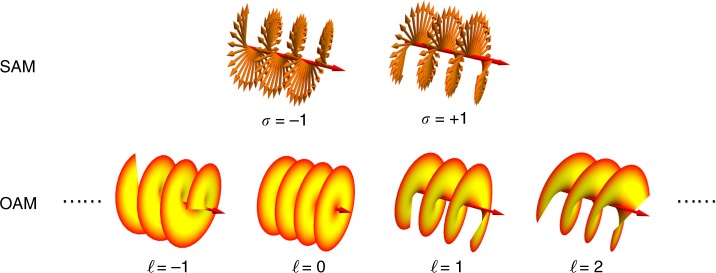
where the power density and . σ = +1 (−1) and 0 correspond to left (right) circularly polarized light and linearly polarized light, respectively. Thus, Eq. (4) reveals that left (right) circularly polarized light carries an SAM of + ℏ (−ℏ) per photon; the light with Hilbert factor exp(iℓθ) carries an OAM of ℓℏ (ℓ = 0, ±1, ±2, …) per photon, where “ ± ” reveals the chirality of the vortex, as demonstrated in Fig. 3. This is consistent with the AM quantization in quantum optics, i.e., the eigenvalues of SAM and OAM for the photon eigenstate yield and . Therefore, the phase factor exp(iℓφ) provides a basic frame of VBs.
Fig. 3. The OAM of light beams is revealed by the phase distributions and the SAM by the polarizations.
The phase distributions for various eigenstates of OAM and the polarizations (right- and left-handed circularly polarized) for the two eigenstates of SAM are illustrated
Polarization and vector vortices
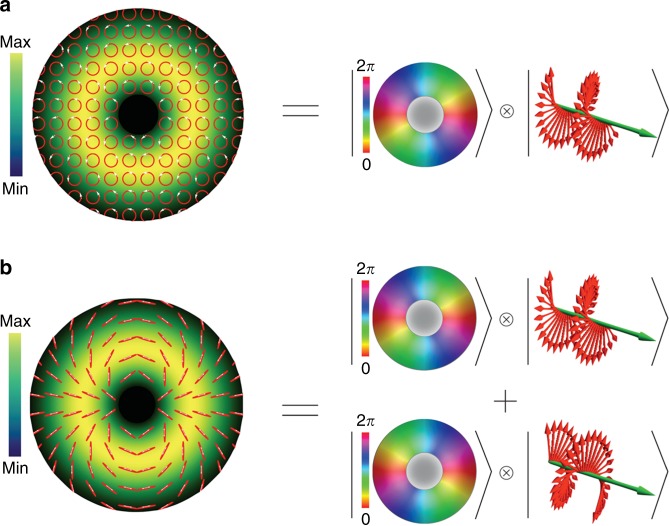
The previous part focuses on the scalar light field, where the polarization is separable from the space. In scalar vortices, there are topological spatial phase structures, but the polarization is unchanged; e.g., Fig. 4a shows that a circularly polarized OV can be expressed as the product of a spatially varying vortex phase state and a circular polarization state71. If the polarization state has a spatially varying vector distribution forming vortex-like patterns, then the corresponding optical field is called polarization vortices or vector vortices, and the corresponding singularity is called a polarization singularity or a vector singularity72,73. Based on the various topological disclinations of polarization, vector vortices can be categorized into many types, such as C-point, V-point, lemons, star, spider, and web, according to the actual vector morphology74. In contrast to the phase vortices carrying OAM, the vector vortices are always related to a complex SAM-OAM coupling; e.g., Fig. 4b shows a spider-like vector OV formed by the superposition of opposite phase variations and opposite circular polarizations, where the total OAM is zero due to the sum of the two opposite phase variations but there is a complex SAM entangled with the space71.
Fig. 4. Formation of vector beam with space-polarization nonseparability.
a Circularly polarized OV with an azimuthally varying phase distribution. Such a state is considered separable, as it can be represented as the product of a spatially varying vortex phase and a polarization state vector. b Spider-like vector vortex represented as the superposition of the state of a with another state with the opposite phase variation and the opposite circular polarization. From ref. 71. Reprinted with permission from AAAS
Classical models of OVs
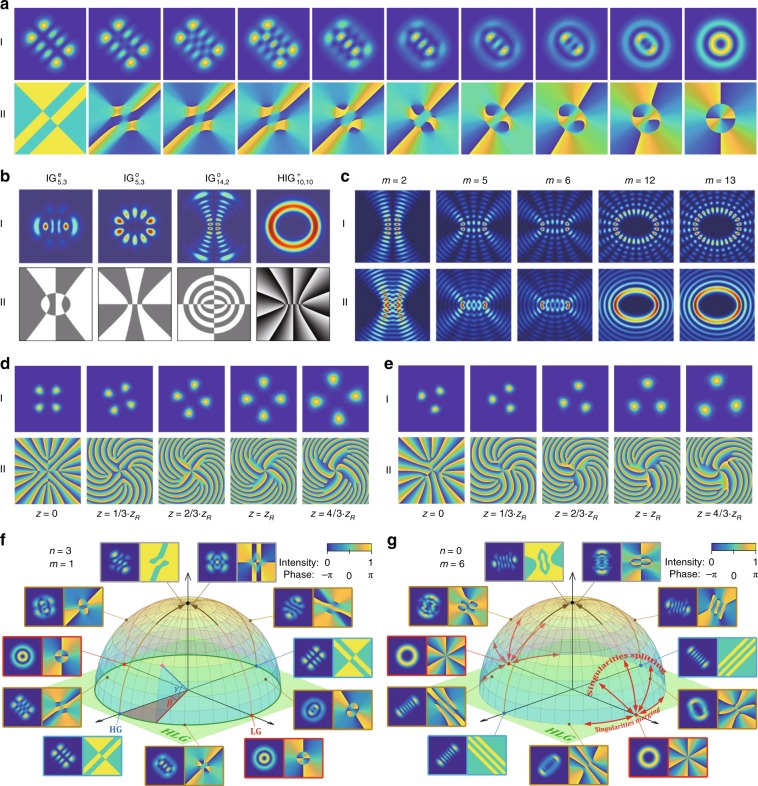
LG and Hermite–Laguerre–Gaussian modes
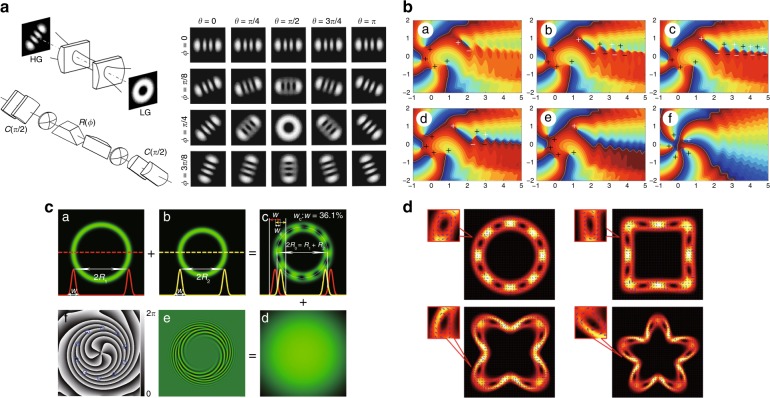
LG modes with circular symmetry are the earliest reported VBs carrying OAM16 and can be included in the general family of Hermite–Laguerre–Gaussian (HLG) modes with elliptical vortices75–77, thus accommodating the transform from the HG to LG mode, which has recently played increasingly important roles because the exploration of the more general structure of OVs always leads to novel applications:
| 6 |
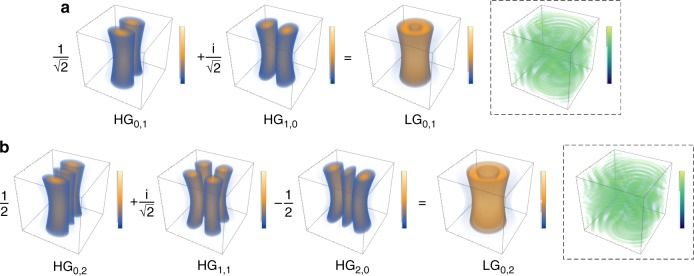
where HLn,m(·) is a Hermite–Laguerre (HL) polynomial75, , , , , and zR is the Rayleigh range. For α = 0 or π∕2, the HLGn,m mode is reduced to the HGn,m or HGm,n mode. For α = π∕4 or 3π∕4, the HLGn,m mode is reduced to LGp,±ℓ mode [, ]. For the other interposed states, the HLG mode has multiple singularities with a total TC of ℓ. As illustrated in Fig. 5, the LGp,ℓ mode can be decomposed into a set of Hermite–Gaussian (HG) modes16,17:
| 7 |
| 8 |
which also interprets the transformation to an LGp,ℓ mode from an HGn,m mode through an astigmatic mode converter (AMC)17.
Fig. 5. Decomposition of LG vortex beams.
Examples of the decomposition of LG modes (LG0,1 (a) and LG0,2 (b)) into HG modes according to Eq. (7), where the insets in the dotted box show the corresponding vortex phase distributions
Helical-Ince–Gaussian and singularities hybrid evolution nature mode
The Ince-Gaussian (IG) mode78 is the eigenmode of the paraxial wave equation (PWE) separable in elliptical coordinates (ξ, η)79:
| 9 |
where Ce,o are normalization constants (the superscripts e and o refer to even and odd modes), are the even and odd Ince polynomials, with for even functions, for odd functions, and for both, and is the eccentricity. The special superposition of these modes can form a multi-singularity array with OAM, named the helical-IG (HIG) modes80–82:
| 10 |
which carries multiple singularities with unit TC, having a total TC of v. Sharing the singularities hybrid evolution nature (SHEN) of the HIG and HLG modes, the SHEN mode is a very general family of structured Gaussian modes including the HG, LG, HLG, and HIG modes, the expression of which is83
| 11 |
The SHEN mode is reduced to the HIG mode when β = ±π/2, to the HLG mode when γ = 0, to the HG mode when (β, γ) = (0,0) or (π, 0), and to the LG mode when (β, γ) = (±π/2, 0). In addition, there is a graphical representation, the so-called SHEN sphere, to visualize the topological evolution of multi-singularity beams. Thus, the SHEN mode has great potential to characterize more general structure beams.
Bessel and Mathieu modes
Using the non-diffraction assumption in solving the PWE, we can also solve a set of eigenmodes. Under separable conditions in circular coordinates, the Bessel mode can be obtained as84
| 12 |
Bessel beams with ℓ ≠ 0 are VBs carrying ℓℏ OAM. Another non-diffraction solution separable in elliptical coordinates is the Mathieu modes85,
| 13 |
| 14 |
where Cm and Sm are normalization constants, Jem and Jom are radial Mathieu functions, and cem and sem are angular Mathieu functions. Analogous to deriving the HIG mode, a helical Mathieu (HM) beam86 can carry multiple singularities and complex OAM87.
| 15 |
High-order Bessel and HM beams are often called non-diffractive VBs, whose unique properties have been extended to a great number of applications, such as particle assembly and optical communication88,89.
SU(2) geometric modes
When a resonator cavity fulfils the reentrant condition of a coupled quantum harmonic oscillator in SU(2) Lie algebra90, the laser mode undergoes frequency degeneracy with a photon performing as an SU(2) quantum coherent state coupled with a classical periodic trajectory91, which is called an SU(2) geometric mode (GM)92. The frequency degeneracy means that should be a simple rational number, where P and Q are two coprime integers, and () is the longitudinal (transverse) mode spacing. The wave-packet function of a planar GM is given by92
| 16 |
where phase ϕ0 is related to the classical periodic trajectory. represents the HGn,m mode considering the frequency-dependent wavenumber , where . If the HG bases are transformed into LG bases, then the circular GM is obtained92:
| 17 |
where represents the LGp,ℓ mode considering the frequency-dependent wavenumber. The vortex circular GM has many unique properties, such as an exotic 3D structure, multiple singularities, and fractional OAM92,93. Note that there are other types of SU(2) modes related to OAM with special properties, such as Lissajous modes94, trochoidal modes95, polygonal VBs96, and SU(2) diffraction lattices97 as shown in Fig. 6d, e.
Fig. 6. Classical models of paraxial VBs.
a Evolution of the (I) intensity and (II) phase distributions of HLG modes as interposed states between HG and LG modes; b various (I) intensity and (II) phase distributions of IG and HLG modes82; c intensity distributions of a selection of (I) odd and (II) helical Mathieu beams88. (I) Intensity and (II) phase distributions of SU(2) vortex geometric modes for Ω = 1/4 (d) and Ω = 1/397 (e). SHEN spheres with orders of (n, m) = (3, 1) (f) and (n, m) = (0, 6) (g) along with represented mode (phase) fields at selected points83. b Reproduced from ref. 82, with the permission of AIP Publishing. c Reprinted with permission from ref. 88, OSA Publishing. d, e Reprinted with permission from ref. 97, OSA Publishing. f, g Reprinted with permission from ref. 83, OSA Publishing
The above forms are classical VBs in free space, which are just optical modes carrying OAM. In addition, there are OVs that are formed by non-OAM beams, as reviewed in the following.
Optical Möbius strips
A direct idea is to arrange the optical parameter into the form of Möbius strips, one of the classical topological models. This type of OV is called an optical Möbius strip (OMS). A simple vortex phase with integer TC can be seen as a phase OMS. In addition to phase vortices, more OMSs can be obtained by arranging the polarization: the major and minor axes of the polarization ellipses that surround singular lines of circular polarization in three-dimensional optical ellipse fields can be organized into an OMS, as theoretically proposed98,99 and experimentally observed49. Currently, multitwist OMSs can be controlled in both paraxial and nonparaxial vector beams56,100. By combining other spatial and optical parameters into OMSs, more complex structures, such as 3D solitons and topological knots, can be proposed for OVs101.
Vortex knots
The vortex core of an OV can not only be distributed along the propagation axis of a beam but also form closed loops, links and knots embedded in a light field102. As a new form of OVs, vortex knots have stimulated many experimental observation and theoretical studies on the dynamics of knotted vortices102,103. Vortex knots can also show many homologies, such as pigtail braid and Nodal trefoil knots104 as shown in Fig. 7c–f. Currently, researchers have realized the isolated manipulation and temporal control of optical vortex knots104,105.
Fig. 7. Classical models of spatial nonparaxial OVs.
Polarization topology of optical Möbius strips with twisted TCs of –1/2 and –3/2 (a, b)56. Nodal trefoil knot and pigtail braid knot OVs (c, d) and corresponding phase distributions (e, f)104. Optical vortex knots of a threefold distorted loop (g), a trefoil knot (h), and a pair of linked rings (i)103. a, b From ref. 56. Reprinted with permission from AAAS. c–f Reprinted by permission from Nature Physics104, Copyright (2019). g–i Reprinted by permission from Nature Physics103, Copyright (2019)
There are many other forms of OVs that cannot be fully covered in this paper. For instance, there are many free-space VB modes that carry OVs and OAM, such as elegant HLG beams106, Airy beams107, Pearcey beams108, and parabolic beams109. There are many morphologies of the non-beam spatial distribution of OVs with singularities fractality110. It is highly expected that many new formations of OVs will be reported and investigated in future explorations.
Properties of VBs
Reflection and refraction
The reflection of a VB generally does not satisfy the classical reflection law, i.e., the angle of incidence θi does not equal the angle of reflection θr. Instead, the reflected light has a spatial deflection effect related to the OAM of the VB111. The difference between θi and θr is related to the OAM of the beam, obeying the generalized law of reflection41
| 18 |
where λ and ϕ are the wavelength and phase of the light beam, respectively, and n is the refractive index of the medium. In addition, the refraction of VBs does not satisfy Snell’s law, i.e., ntsinθt ≠ nisinθi. The refraction is related not only to the angles of incidence and refraction (θi and θt) and the refractive indices but also to the OAM, obeying the generalized law of refraction41
| 19 |
Interference
For conventional laser beams, the equal-inclination interference pattern is equispaced fringes, and the equal-thickness interference pattern is Newton’s rings. However, for a VB, the pattern of equal-inclination interference with a plane wave is not equispaced fringes but fringes with bifurcation at the singularity of the vortex, and the morphology of the bifurcation is related to the OAM of the beam66. The equal-thickness interference pattern of a VB with a plane wave is not Newton’s rings but spiral stripes extending outward from the vortex singularity, the number of which is related to the OAM112. The self-interference pattern can also show some bifurcation fringes112. These special interference fringes can be used in detection and measurement methods of vortices.
Diffraction
VBs have unique diffraction properties, the aperture diffraction patterns of which are coupled with the actual OAM. Since Hickmann et al.40 unveiled in 2010 the exotic lattice pattern in triangular-aperture far-field diffraction of VBs, it has been used as an effective method for OAM detection and measurement of femtosecond vortices113, non-integer charge vortices114, and elliptical VBs115. Many other unique far-field diffraction patterns were investigated through a slit116, a square aperture117, a diamond-shaped aperture118, a circular aperture119, an off-axis circular aperture120, an isosceles right triangular aperture121, a sectorial screen122, and so on. The Fresnel diffraction of VBs was also studied123. Some special VBs, such as vector VBs124 and SU(2) VBs97, can even bring about special lattice structures in diffraction patterns. These special diffraction patterns can be used in vortex detection and measurement methods.
Polarization
The polarization states of conventional beams can be represented on the Poincaré sphere. VBs can have complex transverse structures involving polarization vortices. Upon combining structured polarization with VBs, the vector VBs can demonstrate more amazing properties and more extensive applications74. To characterize a classical family of vector VBs, Holleczek et al. proposed a classical-quantum-connection model to represent cylindrically polarized beams on the Poincaré sphere125; this model was then extended to the high-order Poincaré sphere (HPS)126, which can reveal SAM-OAM conversion and more exotic vector beams, including radial and azimuthal polarization beams. In an experiment, controlled generation of HPS beams was realized127 as illustrated in Fig. 8f. As an improved formation of the HPS, the hybrid-order Poincaré sphere was theoretically proposed128, and the corresponding experimental controlled generation methods were also presented129,130.
Fig. 8. Reflection, interference, diffraction, and polarization of VBs.
a Abnormal reflection of a VB111. b LG VBs with different TCs (first column) and corresponding interference patterns with a co-axis coherent planar wave (second column) and an inclined coherent planar wave (third column). Far-field diffraction patterns of VBs through a triangular aperture40 (c) and a single slit116 (d). e Near-field diffraction pattern of a VB123. f Polarization distribution of vector VBs on the HPS127. a Reprinted with permission from ref. 111. Copyright (2019) by the American Physical Society. c Reprinted with permission from ref. 40. Copyright (2019) by the American Physical Society. d Reprinted with permission from ref. 116, Copyright (2019), with permission from Elsevier. f Reprinted by permission from Nature Photonics127, Copyright (2019)
Quantum properties
Twisted photons31 are associated with the quantum behaviour of macroscopic VBs. Akin to the conventional Heisenberg uncertainty, there is also the formation of uncertainty for twisted photons; i.e., the product of the uncertainties in the angle and the OAM is bounded by Planck’s constant, ΔϕΔL ≥ ℏ/2131,132. The general Fourier relationship between the angle and the OAM of twisted photons was also investigated133. In contrast to the polarization-entangled state with two dimensions, the OAM-entangled state can be high dimensional as 134. Combining the polarization and OAM of the photon, more complex SAM–OAM entangled photon pairs were realized47,135. There are many other new quantum properties related to OAM beams, such as the spin–orbit interaction136–138, the Hanbury–Brown–Twiss effect139, quantum interference140,141, and the spin Hall effect142,143.
Measurements of OVs
As mentioned above, OVs can be measured by adopting the interference and diffraction properties of VBs. Counting the stripes and lattices in the special interferogram and diffraction patterns serves as a toolkit to measure the TC, OAM, and singularity distributions of corresponding OVs. In addition, for measuring phase vortices, one can use a spatial light modulator (SLM) to carry out phase transformations, reconstructing the target phase to detect the TC and OAM. Typical realizations include the forked diffraction grating detector144, the OAM sorter145, and spiral transformation146. For polarization vortices, the measurement should also consider the detection of the vector field. By introducing a space-variant structure into a half-wave plate to modulate the polarization, the TC of the polarization singularity in vector VBs can be measured147. For measuring more properties of vector OVs, Forbes’ group introduced quantum measurement methods to classical light and realized more precise measurement of properties such as the non-separability, SAM–OAM coupling, and vector factors of vector beams148,149, which is widely applicable to more structured OVs.
Progress in vortex generation, tuning, and manipulation
Brief review of vortex generation
The vortex generation methods can be divided into passive vortex generators (converting the fundamental Gaussian beams into VBs by using dynamic or geometric phase plates, metasurfaces, SLMs, etc.) and active vortex laser generators (such as free space or fibre vortex lasers and nanointegrated OAM generators)61,112,150. There have already been some recent reviews on vortex and OAM beam generation61–63,112,150. However, a review focused on vortex generation with tunable and multi-singularity properties is rare. Hereinafter, we specifically review active vortex generation with tunable properties, including wavelength-, temporal-, and OAM-tunable beams. In particular, the OAM-tunable beams include TC-tunable and multi-singularity-tunable beams.
Wavelength- and OAM-tunable VBs
OAM-tuning of VBs can be realized by gain-loss control151, off-axis pumping92,152, or the use of a spiral phase plate (SPP)153, a Q-plate154,155, or an SLM144. A wavelength-tunable VB can be achieved by designing special liquid crystal devices156, microcavities157, or on-chip gratings158 or using nonlinear frequency conversion159,160. However, more methods to simultaneously realize wavelength and OAM tuning for novel applications, such as high-capacity optical communication using wavelength- and mode-division multiplexing, are still required.
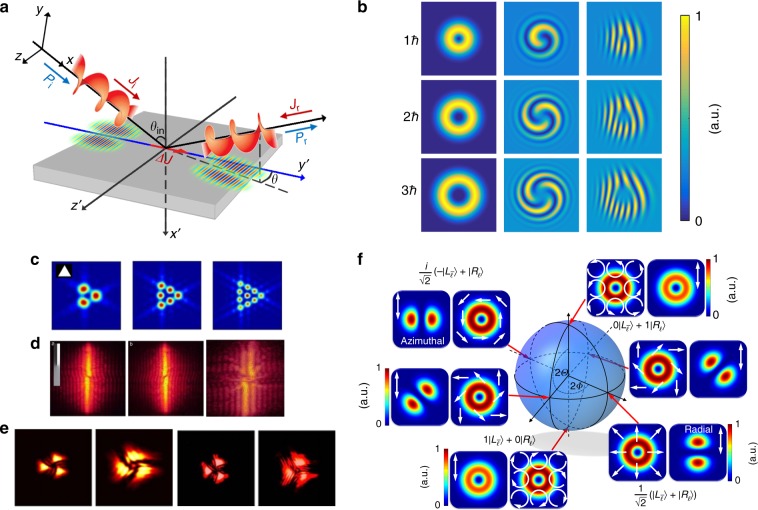
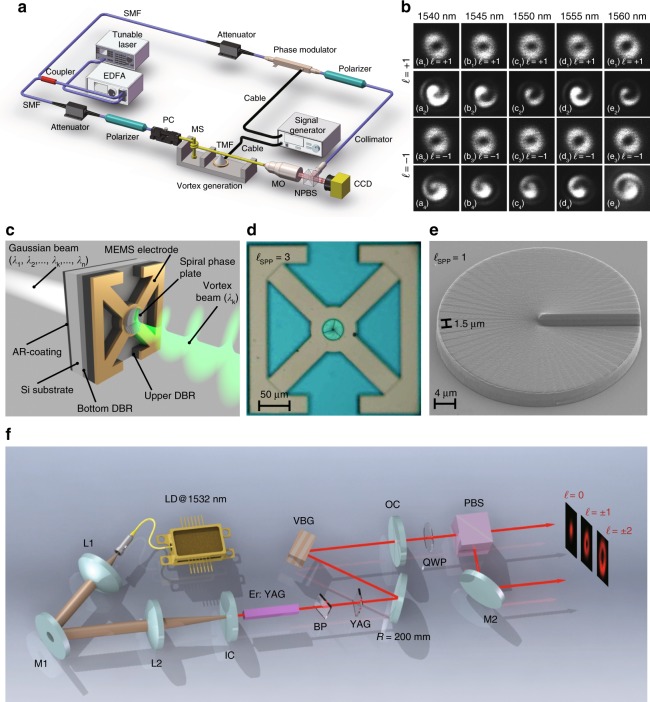
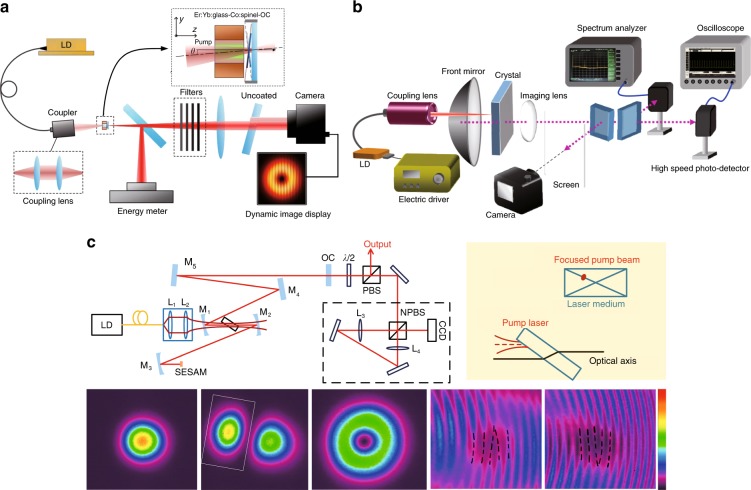
In 2016, Zhang’s group161 presented a wavelength- and OAM-tunable system by employing a tunable fibre laser with an acousto-optic fibre grating with a wavelength-tunable range of 1540–1560 nm and an OAM of ± 1ℏ, as shown in Fig. 9a, b. In 2017, Lyubopytov et al.162 designed a micro-electro-mechanical (MEMS) filter system realizing vortex generation with a wavelength-tunable width of 37.5 nm and an OAM of 0~3ℏ. In the same year, Liu et al.163 reported a ring-pumped Er:YAG solid-state laser generating an 8.4-nm wavelength-tunable width and 0~±2ℏ OAM-tunable VB. In 2018, Yao et al.164 invented a new optical fibre combiner for combining two polarization-controllable fundamental modes into a VB with chiral control, obtaining a 30-nm wavelength-tunable width and 0~±1ℏ OAM. Our group165 proposed solid-state vortex generation utilizing a dual-off-axis pumped ultra-wide-band Yb:CALGO laser, reaching a wavelength-tunable width of over 10 nm and an OAM range of 0~±15ℏ, as depicted in Fig. 10a. This system was adapted to generate tunable dual-wavelength VBs166. Recently, Wang et al.167 improved the output efficiency and reduced the threshold of a similar system by using a Z-cavity and a birefringent plate in the cavity design, and a 14.5-nm wavelength-tunable width and a 0~±14ℏ OAM range were achieved. Wang’s group168 designed and implemented a fibre-space coupling vortex laser system, where a wavelength-tunable range of 1530–1565 nm and an OAM of 0~±10ℏ were achieved.
Fig. 9. Generation of wavelength- and OAM-tunable CW VBs (I).
Generation methods of wavelength- and OAM-tunable VBs using an acousto-optic fibre grating (a), along with the experimental results161 (b), an SPP-embedded MEMS filter system162 (c–e), and a VBG in a hollow-pumped solid-state laser163 (f). Reprinted with permission from refs. 161–163, OSA Publishing
Fig. 10. Generation of wavelength- and OAM-tunable CW VBs (II).
Generation methods of wavelength- and OAM-tunable VBs using a dual-off-axis pumped Yb:CALGO laser system165,166 (a), intracavity birefringent plate rotation167 (b), and a “Gauss-OAM-Gauss” beam conversion system in a fibre laser168 (c). a From refs. 165,166. Reproduced by permission of IOP Publishing. b Reprinted with permission from ref. 167, OSA Publishing
In addition to the abovementioned wavelength- and OAM-tunable OVs from lasers, there are vortex generators that are known to be intrinsically broadband, which can also be used to obtain wavelength- and OAM-tunable OVs. For instance, vortex generation from anisotropic solid crystals, both uniaxial and biaxial, can be related to complex SAM–OAM coupling and gain competition effects, leading to the tunability of vector OVs72,73,169. Similar tunable OVs can be generated in chiral liquid crystals in the regime of circular Bragg reflection170,171. Taking advantage of space-variant anisotropic liquid crystals that can be electrically controlled, wavelength- and OAM-tunable OVs can be generated within a wide spectral tunable range156,172. Overall, a wider tunable range controlled by a more convenient OV generation method is still required in the current explorations.
Pulsed VBs
High-peak-power pulsed VBs with different levels of duration have great potential for use in advanced applications, such as optical machining173,174, nonlinear optics25,26,48,49, strong-field physics175, and optical tweezers176. Hereinafter, we review the recent compact and effective pulsed VB sources.
Nanosecond-level VBs
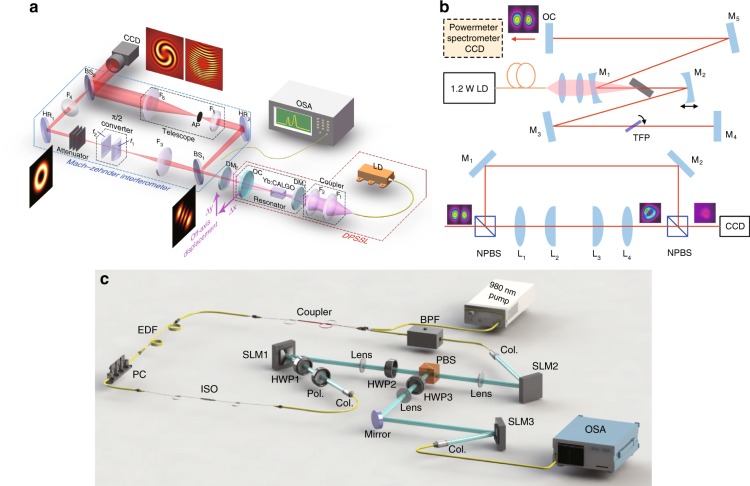
The generation of nanosecond-level VBs has always been combined with Q-switched lasers. An earlier method involved selecting the LG modes using an intracavity aperture in a Q-switched solid-state laser177, with which obtaining high mode purity is difficult. In 2013, Kim et al.178 used an etalon and a Brewster plate in an acoustic-optic Q-switched laser and generated high-quality chirality-controlled LG beams with an ~250 μJ pulse energy and an ~33 ns duration. In 2016, Zhao et al.179 controlled the pump position in an Er:YAG acoustic-optic Q-switched laser, generating a nearly 1-mJ and 50-ns pulsed VB. In 2017, Chen’s group180 designed a nanosecond vortex laser system employing a compact Nd:YVO4/Cr4+:YAG passively Q-switched laser with an external AMC. In 2018, He et al.181 presented a Cr,Nd:YAG self-Q-switched microchip laser to directly generate low-threshold nanosecond high-repetition-rate vortex pulses without an AMC, where the chirality was controlled by a tilted output mirror. Our group182 recently reported a pulsed vortex output directly from a room-temperature diode-pumped Er,Yb:glass microchip laser with an extremely compact structure.
Picosecond-level VBs
Picosecond-level VBs have always been realized in a mode-locking laser using a narrow-band gain medium. In 2011, Koyama et al.183 realized a VB in a stressed Yb-doped fibre amplifier seeded by a picosecond mode-locked Nd:YVO4 laser with a pulse width of 8.2 ps and a peak power of 34.2 kW. However, the master oscillator power-amplifier structure limited the compactness. The discovery of a self-mode-locking effect in neodymium-doped crystals provided an alternative way to generate picosecond pulses with a quite compact structure184. In 2009, Liang et al.185 reported an OV with a pulse width of 20–25 ps and a repetition rate of 3.5 GHz using off-axis-pumped self-mode-locked Nd:GdVO4 lasers with an AMC. In 2013, the same group186 improved this system via cavity control and realized the self-mode-locked SU(2) vortex GM with pulse widths of 22.2 ps and 21.1 ps for Ω = 1/4 and Ω = 1/3, respectively92. In 2017, Tung et al.187 reported the direct generation of picosecond large-OAM SU(2) vortex pulses in self-mode-locking Nd:YVO4 lasers without the help of an AMC, which largely enhanced the compactness. In 2018, Huang et al.188 reported an 8.5 ps pulsed VB generated from a mode-locked fibre laser, where the polarization could be controlled at arbitrary states on the HPS.
Femtosecond-level VBs
In contrast to picosecond pulses, femtosecond pulse generation always requires more extreme operating conditions, such as a tightly focused pumping spot, a wide emission band, and a high nonlinear coefficient of the gain medium. Utilizing the external modulation method, flexible temporal shaping of femtosecond VBs was recently realized189. Considering the improvements in compactness and cost, the self-mode-locking laser oscillator scheme is still desirable. In 2013, Chen’s group190 reported a self-mode-locked monolithic Yb:KGW laser with a duration of 850 fs and a repetition rate of 22.4 GHz. In 2016, they191 improved the system to directly generate a sub-picosecond VB carrying OAM by selective pumping. In 2018, Zhang et al.192 proposed an all-fibre mode-locked femtosecond LG0,±1 vortex laser with a pulse width of 398 fs. In the same year, Wang et al.193 realized direct emission of an ultrafast LG0,±1 VB via a z-type cavity design in an SESAM mode-locking Yb:QX laser with a pulse width of 360 fs, as shown in Fig. 11c. These structures have recently been improved by using a Yb:KYW oscillator with a defect-spot mirror, obtaining a 298-fs VB194. Direct generation of sub-100-fs VBs may be a future target.
Fig. 11. Generation of wavelength- and OAM-tunable pulsed VBs.
Generation methods of a room-temperature diode-pumped Er,Yb:glass microchip nanosecond laser182 (a), picosecond-level VBs in a self-mode-locked Nd:YVO4 laser187 (b), and femtosecond VBs in an SESAM mode-locking laser193 (c). a ©(2019) IEEE. Reprinted, with permission, from ref. 182. b Reprinted with permission from ref. 187, OSA Publishing. c From ref. 193. Reproduced by permission of IOP Publishing
Complex OAM manipulation
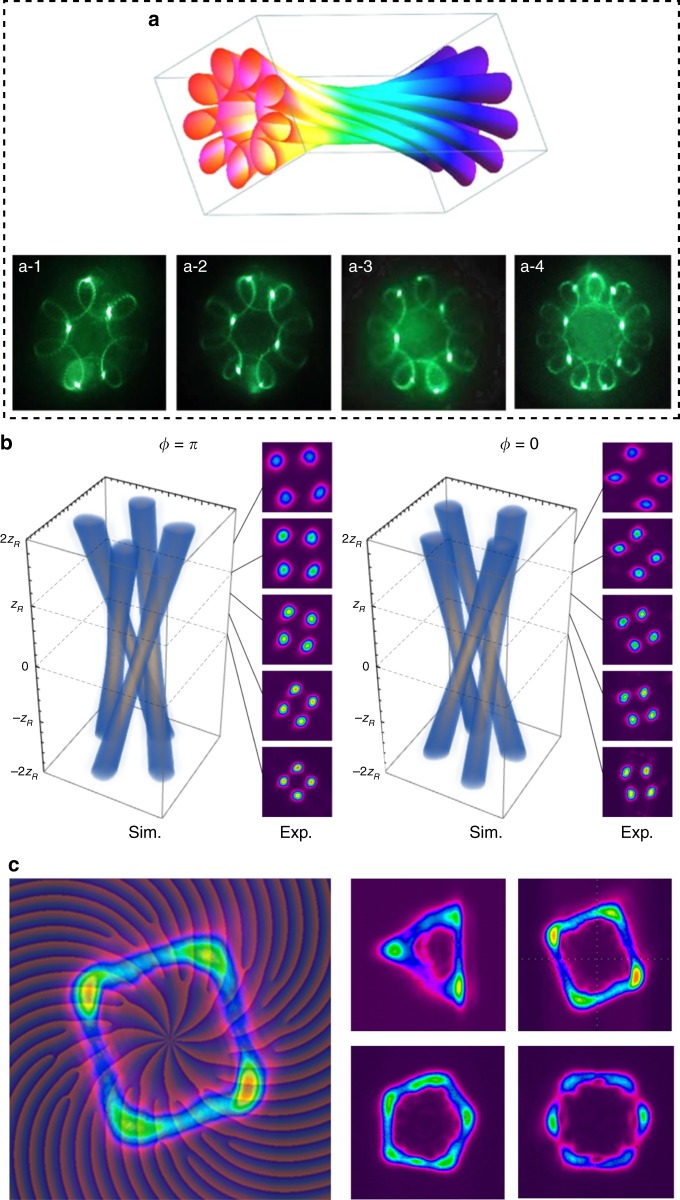
In addition to TC-tunable VBs, beams with multiple singularities can induce exotic tunable OAM. The multi-singularity optical field with a vortex array is also known as a vortex lattice or a vortex crystal5,29,73. Strong requirements of multi-singularity beams have been put forward because of the boom of special applications such as multiple particle manipulation82,195, 3D displays196, and optical modulation and communication197.
A singularity splitting phenomenon was found in an AMC when the phase matching condition in the AMC was not satisfied198,199. A large number of matrix optics theories were put forward200,201, deriving the HLG mode to describe the controllable generation of a vortex array in the AMC system76,77. Similar to the HLG mode, the HIG mode is also a multi-singularity VB, which can be generated in special cavities with selective pumping80 and an SLM81. Recently, our group proposed a method of tuning the periodic orbits of an SU(2) GM in a degenerate cavity and further tuning the multi-singularity OAM of SU(2) VBs202–204, as shown in Fig. 12b. In addition to HIG, HLG and SU(2) VBs, many other multi-singularity VBs with special mathematical formulations were generated with different control methods, such as trochoidal VBs95, transverse-mode-locking vortex lattices 202,203, and polygonal VBs96.
Fig. 12. Exotic SU(2) structured multi-singularity VBs.
Theoretical and experimental investigations of special multi-singularity VBs: a trochoidal vortex modes95, b the vortex SU(2) GM204, and c polygonal VBs96. a Reprinted with permission from ref. 95. Copyright (2019) by the American Physical Society. b Reprinted with permission from ref. 204, OSA Publishing. c © (2019) IEEE. Reprinted, with permission, from ref. 96
In addition to the above multi-singularity modes, people are pursuing more freely tailored methods for arbitrary singularity distributions. SLM modulation combined with a laser source for on-demand modes has been favoured205. Recently, increasing numbers of tailored singularity distributions have been designed and realized via SLMs, such as rectangular and circular multi-singularity arrays206,207 and arbitrary curvilinear arrays208, and quadrant-separable singularity control209, as presented in Fig. 13.
Fig. 13. Generation of multi-singularity VBs.
a Exploring the singularity splitting phenomenon in AMC systems200. b Multiple singularity formation in fractional OAM VBs211. Tailoring multi-singularity beams with c a circular vortex array207 and d an optical vortex array along an arbitrary curvilinear arrangement208. a Reprinted with permission from ref. 200, Copyright (2019), with permission from Elsevier. b Reprinted with permission from ref. 211, OSA Publishing. c Reprinted with permission from ref. 207. Copyright (2019) by John Wiley and Sons. d Reprinted with permission from ref. 208, OSA Publishing
There are still many novel methods of tuning the multi-singularity OAM in more types of exotic OVs. For instance, various OV arrays can be generated by coherent combining technology with digital control210. Infinite scalar and vector OV arrays can be realized in fractional OAM VBs211,212. On-demand multi-singularity VBs can be generated based on the appropriate combination of optical scattering and discrete rotational symmetries of optical isotropic masks213 and can be electrically and optically controlled via anisotropic masks214,215.
Despite the numerous multi-singularity manipulation methods, the realization of universal and versatile tunability will be the everlasting target in the future.
Advanced applications of tunable VBs
Optical tweezers
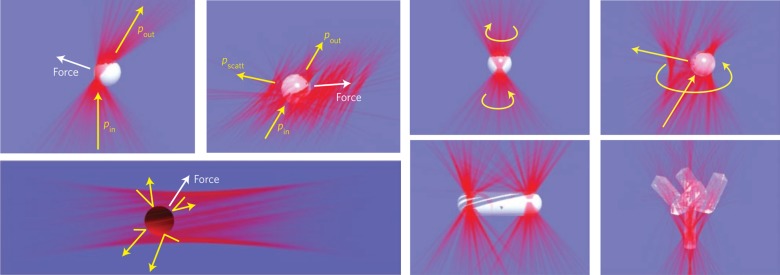
Optical tweezers that trap particles using an optical force were proposed by Ashkin216, who won the Nobel Prize in 2018. Benefitting from the study of OAM interactions with matter, OVs were first used in 1995 in optical tweezers and extended to the optical spanner19, where particles can be trapped and driven to move around the singularity. Then, the transformation from optical OAM to mechanical AM was widely studied32–34.
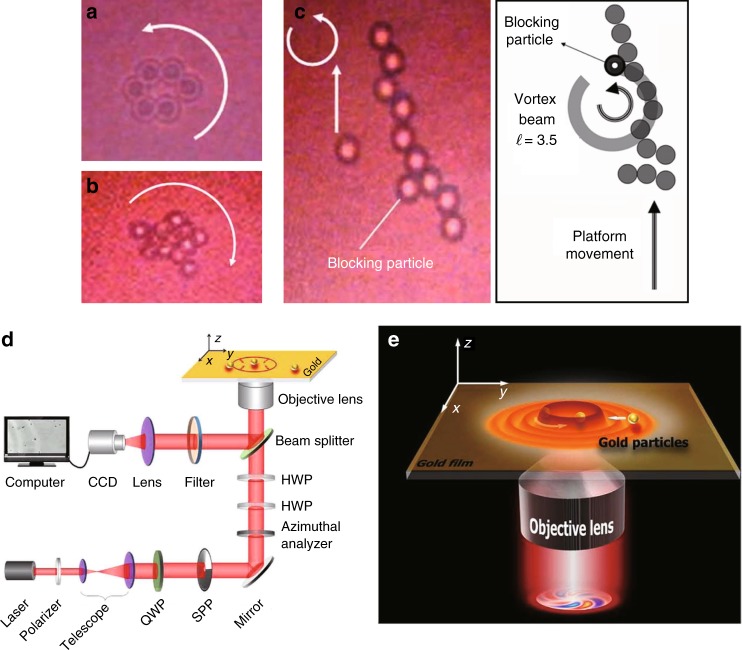
With the improvement of vortex tunability, new-generation tweezers with OVs have shown distinct advantages34,217. As demonstrated in Fig. 14, the novel vortex tweezers can conveniently manipulate not only the spatial positions of particles but also the multiple degrees of freedom of particles, largely extending the automated guiding, assembly, and sorting technology217,218. With the control of multi-singularity VBs, many new techniques were designed and applied to trap multiple particles82,217,218, including the fractional optical VB for optical tweezers219. With femtosecond VBs, the tweezers carrying special nonlinear properties can be used to manipulate optical Rayleigh particles220. Furthermore, with femtosecond vector VBs, nonlinearity-induced multiplexed optical trapping and manipulation was designed221, where the number of traps and their orientations could be flexibly controlled. In addition to dielectric particles, metal particles can also be manipulated by novel plasmonic vortex tweezers222, where the vortex field of surface plasmon polaritons can be generated by focusing vector VBs onto a metal film. Plasmonic vortex tweezers as depicted in Fig. 15d, e were shown to be superior in manipulating metal particles with large flexibility223.
Fig. 14. Optical tweezers with VBs can manipulate not only the positions of particles but also their motion, such as precession, nutation, spin, and more complicated orbital motion217.
Reprinted by permission from Springer Nature: Nature Photonics217, Copyright (2019)
Fig. 15. Particle manipulation using OVs.
a, b Manipulating the rotation of multiple particles and c aligning and transporting particles with fractional VBs219. d Setup and e schematic of plasmonic vortex tweezers for manipulating metal particles223. a–c Reprinted with permission from ref. 219, OSA Publishing
Optical communication
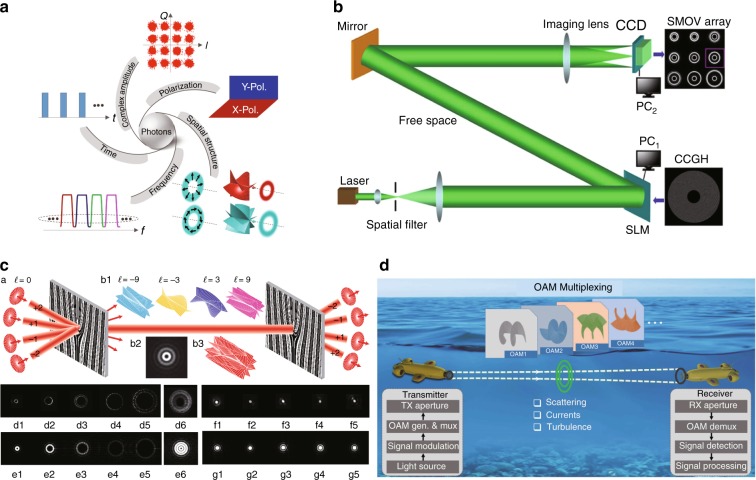
In addition to the polarization, amplitude, pulse shape, and wavelength of light, the OAM can be used as an alternative degree of freedom for multiplexing modulation, enlarging the capacity of optical communication39, which is also referred to as mode/spatial-division multiplexing (MDM/SDM)224. Optical communication by OAM multiplexing has enabled breaking the Tbit level43,44, much beyond the conventional scheme, thus greatly broadening the application scope225,226. With the study of VB propagation in the atmosphere, free-space communication using vortices was gradually improved227–229. Furthermore, a sidelobe-modulated OV method was proposed for free-space communication with a significant increase in the data transmission capacity230. With the development of multi-singularity-tunable VBs, the capacity and speed of communication can be further improved231. A variety of special fibres for OAM mode transmission were designed to enable fibre-based vortex communication technology232,233. Recently, a new OAM multiplexing technology using Dammann vortex gratings in fibre-free-space coupled systems realized massive OAM state parallel detection234, offering an opportunity to raise the communication capacity to the Pbit level. OAM-multiplexing-based communication was also demonstrated under many extreme circumstances, such as underwater communication235 illustrated in Fig. 16d, high-dimensional quantum communication236, and long-distance fibre communication237.
Fig. 16. Optical communication using OVs.
a Optical communication can be realized by modulating the time domain, frequency domain, amplitude, polarization, and OAM225. b Schematic of sidelobe-modulated OVs for free-space communication230. c Dammann-grating-enabled OAM multiplexing technology raising the large capacity to the Pbit level234. d Underwater optical communication using VBs235. b Reprinted with permission from ref. 230, OSA Publishing. c Reprinted by permission from Springer Nature: Light: Science & Applications234, Copyright (2019)
Quantum entanglement
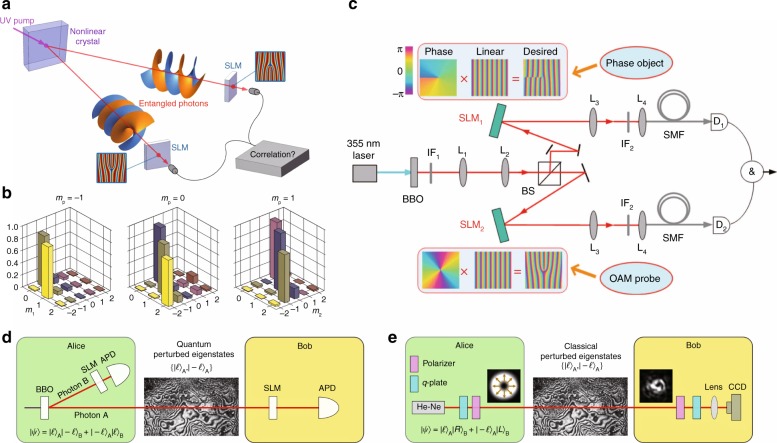
With the recent mature quantum descriptions of twisted photons31, OAM entanglement has engendered plenty of applications134. For instance, high-dimensional quantum key distribution (QKD) protocols can be designed based on mutually unbiased bases related to OAM photons238, which motivated high-dimensional quantum cryptography for high-security communication239. The quantum memory technology for OAM photonic qubits was recently proposed to provide an essential capability for future networks240. Because of the inherent infinite dimension of OAM, the OAM of photons has been successfully used to realize quantum storage in various systems, such as atomic ensembles241 and rare-earth-ion-doped crystals242, benefiting high-capacity communication. High-dimensional OAM entanglement was also successfully used in high-efficiency digital spiral imaging243. Employing the Hong–Ou–Mandel interference of OAM photons, quantum cloning technology for making copies of unknown quantum states was presented244. With the development of vector VB manipulation, SAM and OAM were combined for quantum communication to further scale the capacity and speed245. Quantum teleportation using OAM can largely improve the technical control of scalable and complicated quantum systems246. To date, the entangled photon system with the highest number of qubits (18 qubits with six entangled photons) with OAM as one degree of freedom has been produced247. Very recently, as a remarkable breakthrough, quantum entanglement between the SAM and OAM states was realized in a metamaterial47.
In addition to scalar phase OVs, vector polarization OVs also have fruitful quantum properties. The non-separable states between the polarization and space share common properties with the entangled state of photons, which is also called the classical entanglement state71,248. The quantum tomography, Bell parameter, concurrence count, and linear entropy can be realized in vector OVs akin to corresponding quantum measurements148,149,248. Taking advantage of the high-dimensional properties of the non-separable states, quantum walks can be implemented by vector OV modes of light, enlarging the scalable range249. Entanglement beating generated in vector VBs can be used to control spin–orbit coupling in free space135. High-dimensional entanglement has also been utilized in coding quantum channels to improve high-capacity optical communication250, as illustrated in Fig. 17d, e.
Fig. 17. Quantum technologies using OVs.
a OAM-entanglement photon pairs generated by the spontaneous parametric down conversion process. b Quantum tomography experimental data demonstrating that the OAM of the pump beam is transferred to the sum of the OAM of the generated photons (mp = m1 + m2)31. c Setup for quantum digital spiral imaging243. Comparison between quantum communication using d entangled photons and e non-separable states of vector OVs250. b Reprinted by permission from Springer Nature: Nature Physics31, Copyright (2019). c Reprinted by permission from Springer Nature: Light: Science & Applications243, Copyright (2019). d, e Reprinted by permission from Springer Nature: Nature Physics250, Copyright (2019)
Nonlinear optics
With the development of high-power and large-energy VBs92,180,251, the peak power can exceed the threshold of various nonlinear effects, providing conditions to explore novel nonlinear conversion phenomena related to OAM48,49,251. Conventionally, the development of nonlinear optics was based mainly on the scattering that obeys momentum conservation (Rayleigh scattering, Brillouin scattering, Raman scattering, etc.), and the corresponding development of nonlinear frequency transformation effects (frequency doubling, frequency summing, four-wave mixing, etc.) has benefited a myriad of applications. In the new century, new transverse nonlinear transformation effects have been developed based on AM conservation, such as TC variation during the processes of frequency doubling25,26, summing and mixing252,253, tunable OAM high-harmonic transform48,49, and OAM strong-field physics175. Recently, these OAM harmonic generations have been widely applied in nanomaterials for the control of nonlinear phases254, the Pancharatnam–Berry phase255 and beam shaping256. In addition, there are many novel physical phenomena coupled with nonlinear OAM-frequency conversion, such as the rotational Doppler effect257 and rotational nonlinear absorption258.
Nanotechnology
Due to the rapid development of nanofabrication and increasing demands for nanotechnology applications, nanointegrated on-chip vortex generators have emerged for emitting VBs at the nanoscale, such as integrated silicon-chip-based VB emitters259, vortex vertical-cavity surface-emitting lasers (VCSELs)260, angular gratings42, micro-nano-OAM laser emitters261, and various metasurface designs262. Taking advantage of nanoscale VBs, many novel phenomena related to OAM in nanophotonic materials have been demonstrated, such as non-dispersive vortices263 and SAM-to-OAM conversion effects46,47. Combined with new nanomaterials, many vortex-emitting materials and devices with unique functions have been invented, such as vector vortex on-chip generators264 and parallel OAM processors265. Combining quantum technology and nanotechnology, a photonic chip capable of purifying the OAM quantum states was recently produced, which possesses great potential to develop on-chip quantum calculation266.
Optical machining
Due to the nature of high-order modes, VBs show weaker capability in conventional machining processes, such as laser cutting and laser punching, than the fundamental Gaussian beam. However, in some special applications, vortex light has distinct advantages. When a metal surface is processed by different vector VBs, various intriguing new patterns can be selectively displayed under light illumination267,268. Moreover, the surface can exhibit different patterns when the illuminated light has different incidence angles269. In addition to the angular sensitivity, a polarization-sensitive surface was fabricated based on a similar technique using vortex processing, i.e., different patterns were exhibited when the surface was illuminated by light with different polarizations268. Utilizing nanophotonics technology, nanoscale VBs were used in nanostructure fabrication. For instance, the chiral nanoneedle structure can be easily fabricated by a perpendicular VB through the transfer of the consequential torque from OAM light to the object173,269,270. Similar methods can produce some other nanostructures, such as helical surface reliefs271 and monocrystalline silicon needles272. Recently, high-power ultrashort OAM-tunable VBs were combined with femtosecond laser direct writing technology to process more special structures, such as multi-waveguide266 and micro-pipe structures174.
Microscopy and imaging
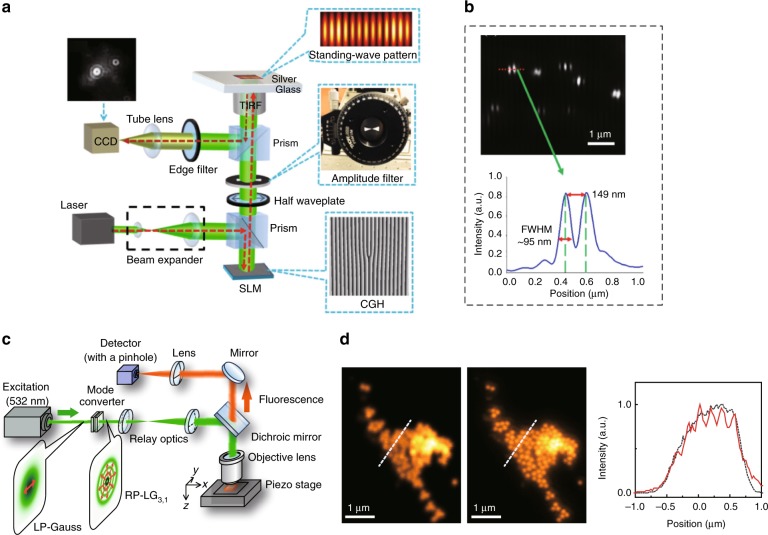
The unique spiral phase of VBs can be used in phase-contrast microscopy, demonstrating high-resolution micro-imaging37. Applying OAM analysis in the imaging method, the novel digital spiral imaging technique was proposed to improve the resolution273. Currently, imaging using OAM has already realized super-diffraction-limit resolution38. In recent years, a growing number of novel microscopy and imaging technologies using VBs have emerged, reaching increasingly higher resolution. For instance, plasmonic structured illumination microscopy using standing surface plasmon waves induced by OVs was proposed, realizing high-resolution wide-field imaging274. This microscopy was further improved by using perfect VBs (VBs with a controllable ring radius) to enhance the excitation efficiency and reduce the background noise275. With the development of multi-singularity beams, a vortex array was used to harness the point-spread function to realize high-resolution far-field microscopy276. Specifically, fractional VBs were also used for precise microscopy to reach sub-100-nm resolution277. With the advanced vector VBs having a special polarization structure, the super-resolution imaging reached an even higher resolution278, as shown in Fig. 18c, d. With the quantum properties of VBs, quantum ghost imaging was combined with twisted photons, opening new routes for imaging techniques243. As a remarkable breakthrough of microscopy using OVs, the stimulated emission depletion (STED) microscopy technique proposed by Willig et al.279, in which the vortex phase is modulated in STED beams to realize super-resolution, was awarded the 2014 Nobel Prize in Chemistry.
Fig. 18. High-resolution imaging using OVs.
a Setup of the plasmonic structured illumination microscopy technique, and b imaging results with super-resolution277. c Setup of superoscillation focusing imaging using a vector VB, and d imaging results with super-resolution278. a, b Reprinted with permission from ref. 277, OSA Publishing. c, d Reprinted with permission from ref. 278, OSA Publishing
Biomedicine and chemistry
Using OV tweezers, one can manipulate and assemble some proteins and other biomolecules, greatly advancing the development of structural chemistry and biomedical photonics34,36. Note that VBs and some organic molecules all have chirality, and the chirality of the vortex phase can interplay with that of a biomolecule, which has promoted a number of applications in biomedicine and chemistry280. For instance, VBs can be used to assemble DNA36 and resolve enantiomers281 due to the chirality coupling effect. By applying this method to chiral metamaterials, novel sensing technology was proposed to detect many enantiomers or biomolecules, such as amino acids, sugars, and nucleotides282. Additionally, the functionalities of transporting subcellular organelles and exerting less photodamage on the trapped particle was developed for vortex tweezers, which have been used in sophisticated single-cell nanosurgery283. The advanced microscopy brought about by VBs was also used for observing biological cell structures with high resolution279. Most recently, vortices were directly generated from organic materials284, with further development of organic illumination and chemical detection technologies expected in the future.
Metrology
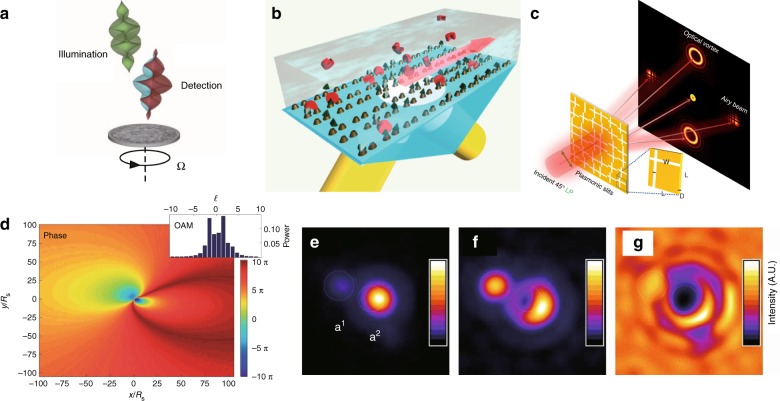
Based on the light-matter interaction through which the OAM of light can be coupled with the mechanical momentum, VBs can be used to detect object motion, including spin motion285 and lateral motion286. With recent advances in nanophotonics and nanofabrication, the precision of detection has reached the nanoscale, and VBs can be used for label-free single-molecule detection in metamaterials287. Recently, the OAM spectrum, acting as a new powerful tool, was used in optical detection, in which the difference between the OAM spectra of incident and outgoing light revealed the topography of the target288, as depicted in Fig. 19h. Similar OAM-spectrum methods have been successfully applied to detect complicated turbulence in the atmosphere289 and ocean290. Recently, with the study of the interaction between OVs and plasmonic nanoslits291, VBs have been used to detect the nanostructure on metal films, opening the door for onchip compact OAM detection292. There are also several devices and structures for detecting OAM states. For instance, a virtual rotational antenna structure was designed to generate the rotational Doppler effect, and the signal of the Doppler shift could be detected to reveal the OAM of the corresponding OV293. The on-chip plasmonic nanoslit structure can produce different scattering effects for OVs with different TCs, serving as a useful tool for the discrimination of OAM294. Moreover, some on-demand metasurface262 and liquid crystal170,171,265 devices have shown great potential for detecting OAM, enabling the further development of precise metrology technologies.
Fig. 19. Nanotechnology, nonlinear conversion, and detection using OVs.
a Various integrated nanoscale vortex emitters259. b Sorting and resolving enantiomers or molecules with different chiralities281. c Machining a nanoscale needle using VBs with d clockwise rotation and e counterclockwise rotation structures270. f Schematic diagram of the rotational Doppler effect in nonlinear optics257. g Schematic diagram of the OAM conservation process in the high-harmonic generation of VBs49. h Schematic diagram of object detection using the OAM spectrum288. a From ref. 259. Reprinted with permission from AAAS. b Reprinted/adapted from ref. 281. © The Authors, some rights reserved; exclusive licensee American Association for the Advancement of Science. Distributed under a Creative Commons Attribution NonCommercial License 4.0 (CC BY-NC) http://creativecommons.org/licenses/by-nc/4.0/. c–e Reprinted with permission from ref. 270, OSA Publishing. f Reprinted by permission from Springer Nature: Nature Physics257, Copyright (2019). g Reprinted by permission from Springer Nature: Nature Communications49, Copyright (2019). h Reprinted with permission from ref. 288, OSA Publishing
Astronomy
OVs not only have been artificially created in laser beams but also naturally exist in the cosmic microwave background35. In 2003, Harwit described astrophysical processes of OAM light generation, including photon scattering and vortex generation in the environments surrounding energetic sources, e.g., masers, pulsars, and quasars35. To make an astronomical survey that took advantage of OVs, an OV coronagraph was designed295 and experimentally verified296 by Swartzlander’s group, which has made many breakthroughs in astronomical demonstration297. In addition to the scalar vortex masks used in these coronagraph devices, vectorial masks were also implemented in coronagraphs at nearly the same time as Swartzlander’s work in 2005298. With the development of vector OVs, the vortex coronagraph implemented in international ground-based telescope facilities has been based on vectorial vortex masks to obtain higher sensitivity and lower aberrations299. With the recent development of multi-singularity tunability, adaptive multiple-vortex coronagraph masks have been developed for multiple-star detections300,301. In 2011, Tamburini et al.302 reported the OAM light effect around rotating black holes, which provided a new method to detect black holes, as shown in Fig. 20d. Interestingly, astronomical applications are always accompanied by sci-fi themes, and vortex light has been declared to be a fast, furious and perfect tool for talking to aliens and detecting alien civilizations due to its unique properties303.
Fig. 20. Metrology and astronomy using OVs.
a VBs can be used to detect the spin motion of an object285. b Twisted light can be used for label-free single-molecule detection287. c VBs can be used with plasmonic nanoslit structures for multi-channel OAM generation and detection291. d Vortex generation around a rotating black hole302. e–g Imaging of an OV coronagraph297. a From ref. 285. Reprinted with permission from AAAS. b Reprinted by permission from Springer Nature: Nature Materials287, Copyright (2019). c Reprinted with permission from ref. 291. Copyright (2019) by John Wiley and Sons. d Reprinted by permission from Springer Nature: Nature Physics302, Copyright (2019). e–g Reprinted with permission from ref. 297, OSA Publishing
Other advances
OVs indeed demonstrate various characteristics, not only as VBs analysed under the paraxial approximation but also as a general spatial singular field with fractality of singularities. In addition, OVs are not restricted to linear space but have been extensively studied in nonlinear media in connection with optical solitons7,22–24. Moreover, topological vortex waves can be studied in other spectra in addition to the light field, such as microwave vortices304, acoustic vortices305 and X-ray vortices50. Vortex electron beams59 and neutron beams60 with unique OAM properties were also produced and investigated. Very recently, gravitational waves with AM were observed and could be used for trapping and guiding cosmic bodies306. Overall, there are currently numerous promising and amazing applications related to OVs with unlimited possibilities that require further exploration.
Conclusions and perspectives
This review article is dedicated to commemorating the 30th anniversary of the birth of OVs, covering the development history from fundamental theories to tunable vortex techniques and then to widespread scientific applications. We first reviewed the theoretical foundation of OVs and emphasized the unique properties related to OAM, TC, and singularities. Then, we reviewed the recent advances in tunable VBs, where the tunability includes not only wavelength tunability and temporal tunability but also OAM tunability. Recent vortex generation methods with different kinds of tunability were reviewed, revealing the development of optical field manipulation. Taking advantage of the advanced vortex manipulation techniques, widespread novel applications have boomed in the new century. We reviewed the various applications in different branches of science as comprehensively as possible. The development tendency of OVs is a typical example that theories guide new applications and that application demands inspire new theories. To date, OVs are still hot topics and have high potential for both theories and applications.
Acknowledgements
This work was funded by The National Key Research and Development Program of China (Grant No. 2017YFB1104500), Natural Science Foundation of Beijing Municipality (4172030), Beijing Young Talents Support Project (2017000020124G044), Leading talents of Guangdong province program (00201505), National Natural Science Foundation of China (U1701661, 91750205, 61975133, 11604218, 61975087), and Natural Science Foundation of Guangdong Province (2016A030312010, 2017A030313351). The first author Y.S. would like to thank Prof. Andrew Forbes at Wits University for useful discussions and Xilin Yang at the Beijing Institute of Technology for assistance with the graphics.
Conflict of interest
The authors declare that they have no conflict of interest.
Contributor Information
Xing Fu, Email: fuxing@mail.tsinghua.edu.cn.
Xiaocong Yuan, Email: xcyuan@szu.edu.cn.
References
- 1.Coullet P, Gil L, Rocca F. Optical vortices. Opt. Commun. 1989;73:403–408. doi: 10.1016/0030-4018(89)90180-6. [DOI] [Google Scholar]
- 2.Graham R, Haken H. Laserlight—first example of a second-order phase transition far away from thermal equilibrium. Z. Phys. 1970;237:31–46. doi: 10.1007/BF01400474. [DOI] [Google Scholar]
- 3.Aranson IS, Kramer L. The world of the complex Ginzburg-Landau equation. Rev. Mod. Phys. 2002;74:99–143. doi: 10.1103/RevModPhys.74.99. [DOI] [Google Scholar]
- 4.Coullet P, Gil L, Lega J. Defect-mediated turbulence. Phys. Rev. Lett. 1989;62:1619–1622. doi: 10.1103/PhysRevLett.62.1619. [DOI] [PubMed] [Google Scholar]
- 5.Brambilla M, et al. Transverse laser patterns. I. Phase singularity crystals. Phys. Rev. A. 1991;43:5090–5113. doi: 10.1103/PhysRevA.43.5090. [DOI] [PubMed] [Google Scholar]
- 6.Brambilla M, et al. Transverse laser patterns. II. Variational principle for pattern selection, spatial multistability, and laser hydrodynamics. Phys. Rev. A. 1991;43:5114–5120. doi: 10.1103/PhysRevA.43.5114. [DOI] [PubMed] [Google Scholar]
- 7.Rosanov NN, Fedorov SV, Shatsev AN. Curvilinear motion of multivortex laser-soliton complexes with strong and weak coupling. Phys. Rev. Lett. 2005;95:053903. doi: 10.1103/PhysRevLett.95.053903. [DOI] [PubMed] [Google Scholar]
- 8.Genevet P, et al. Bistable and addressable localized vortices in semiconductor lasers. Phys. Rev. Lett. 2010;104:223902. doi: 10.1103/PhysRevLett.104.223902. [DOI] [PubMed] [Google Scholar]
- 9.Barland, S. et al. Observation of “true” optical vortices in a laser system. in Nonlinear Photonics and Novel Optical Phenomena (eds Chen, Z. G. & Morandotti, R.) 195–205 (Springer, New York, NY, 2012).
- 10.Bazhenov VY, Soskin MS, Vasnetsov MV. Screw dislocations in light wavefronts. J. Mod. Opt. 1992;39:985–990. doi: 10.1080/09500349214551011. [DOI] [Google Scholar]
- 11.Crasovan LC, Malomed BA, Mihalache D. Stable vortex solitons in the two-dimensional Ginzburg–Landau equation. Phys. Rev. E. 2000;63:016605. doi: 10.1103/PhysRevE.63.016605. [DOI] [PubMed] [Google Scholar]
- 12.Mihalache D, et al. Stable topological modes in two-dimensional Ginzburg-Landau models with trapping potentials. Phys. Rev. A. 2010;82:023813. doi: 10.1103/PhysRevA.82.023813. [DOI] [Google Scholar]
- 13.Fedorov SV, et al. Topologically multicharged and multihumped rotating solitons in wide-aperture lasers with a saturable absorber. IEEE J. Quantum Electron. 2003;39:197–205. doi: 10.1109/JQE.2002.807212. [DOI] [Google Scholar]
- 14.Paulau PV, et al. Vortex solitons in lasers with feedback. Opt. Express. 2010;18:8859–8866. doi: 10.1364/OE.18.008859. [DOI] [PubMed] [Google Scholar]
- 15.Mihalache D, et al. Stable vortex tori in the three-dimensional cubic-quintic Ginzburg-Landau equation. Phys. Rev. Lett. 2006;97:073904. doi: 10.1103/PhysRevLett.97.073904. [DOI] [PubMed] [Google Scholar]
- 16.Allen L, et al. Orbital angular momentum of light and the transformation of Laguerre-Gaussian laser modes. Phys. Rev. A. 1992;45:8185–8189. doi: 10.1103/PhysRevA.45.8185. [DOI] [PubMed] [Google Scholar]
- 17.Beijersbergen MW, et al. Astigmatic laser mode converters and transfer of orbital angular momentum. Opt. Commun. 1993;96:123–132. doi: 10.1016/0030-4018(93)90535-D. [DOI] [Google Scholar]
- 18.Dennis MR, O’Holleran K, Padgett MJ. Singular optics: optical vortices and polarization singularities. Prog. Opt. 2009;53:293–363. doi: 10.1016/S0079-6638(08)00205-9. [DOI] [Google Scholar]
- 19.He H, et al. Direct observation of transfer of angular momentum to absorptive particles from a laser beam with a phase singularity. Phys. Rev. Lett. 1995;75:826–829. doi: 10.1103/PhysRevLett.75.826. [DOI] [PubMed] [Google Scholar]
- 20.Gahagan KT, Swartzlander GA. Optical vortex trapping of particles. Opt. Lett. 1996;21:827–829. doi: 10.1364/OL.21.000827. [DOI] [PubMed] [Google Scholar]
- 21.Simpson NB, Allen L, Padgett MJ. Optical tweezers and optical spanners with Laguerre–Gaussian modes. J. Mod. Opt. 1996;43:2485–2491. doi: 10.1080/09500349608230675. [DOI] [Google Scholar]
- 22.Swartzlander GA, Jr., Law CT. Optical vortex solitons observed in Kerr nonlinear media. Phys. Rev. Lett. 1992;69:2503–2506. doi: 10.1103/PhysRevLett.69.2503. [DOI] [PubMed] [Google Scholar]
- 23.Tikhonenko V, Christou J, Luther-Daves B. Spiraling bright spatial solitons formed by the breakup of an optical vortex in a saturable self-focusing medium. J. Opt. Soc. Am. B. 1995;12:2046–2052. doi: 10.1364/JOSAB.12.002046. [DOI] [Google Scholar]
- 24.Firth WJ, Skryabin DV. Optical solitons carrying orbital angular momentum. Phys. Rev. Lett. 1997;79:2450–2453. doi: 10.1103/PhysRevLett.79.2450. [DOI] [Google Scholar]
- 25.Dholakia K, et al. Second-harmonic generation and the orbital angular momentum of light. Phys. Rev. A. 1996;54:R3742–R3745. doi: 10.1103/PhysRevA.54.R3742. [DOI] [PubMed] [Google Scholar]
- 26.Courtial J, et al. Second-harmonic generation and the conservation of orbital angular momentum with high-order Laguerre-Gaussian modes. Phys. Rev. A. 1997;56:4193–4196. doi: 10.1103/PhysRevA.56.4193. [DOI] [Google Scholar]
- 27.Soskin MS, et al. Topological charge and angular momentum of light beams carrying optical vortices. Phys. Rev. A. 1997;56:4064–4075. doi: 10.1103/PhysRevA.56.4064. [DOI] [Google Scholar]
- 28.Courtial J, et al. Rotational frequency shift of a light beam. Phys. Rev. Lett. 1998;81:4828–4830. doi: 10.1103/PhysRevLett.81.4828. [DOI] [Google Scholar]
- 29.Scheuer J, Orenstein M. Optical vortices crystals: spontaneous generation in nonlinear semiconductor microcavities. Science. 1999;285:230–233. doi: 10.1126/science.285.5425.230. [DOI] [PubMed] [Google Scholar]
- 30.Mair A, et al. Entanglement of the orbital angular momentum states of photons. Nature. 2001;412:313–316. doi: 10.1038/35085529. [DOI] [PubMed] [Google Scholar]
- 31.Molina-Terriza G, Torres JP, Torner L. Twisted photons. Nat. Phys. 2007;3:305–310. doi: 10.1038/nphys607. [DOI] [Google Scholar]
- 32.Paterson L, et al. Controlled rotation of optically trapped microscopic particles. Science. 2001;292:912–914. doi: 10.1126/science.1058591. [DOI] [PubMed] [Google Scholar]
- 33.MacDonald MP, et al. Creation and manipulation of three-dimensional optically trapped structures. Science. 2002;296:1101–1103. doi: 10.1126/science.1069571. [DOI] [PubMed] [Google Scholar]
- 34.Grier DG. A revolution in optical manipulation. Nature. 2003;424:810–816. doi: 10.1038/nature01935. [DOI] [PubMed] [Google Scholar]
- 35.Harwit M. Photon orbital angular momentum in astrophysics. Astrophys. J. 2003;597:1266–1270. doi: 10.1086/378623. [DOI] [Google Scholar]
- 36.Zhuang XW. Unraveling DNA condensation with optical tweezers. Science. 2004;305:188–190. doi: 10.1126/science.1100603. [DOI] [PubMed] [Google Scholar]
- 37.Fürhapter S, et al. Spiral phase contrast imaging in microscopy. Opt. Express. 2005;13:689–694. doi: 10.1364/OPEX.13.000689. [DOI] [PubMed] [Google Scholar]
- 38.Tamburini F, et al. Overcoming the Rayleigh criterion limit with optical vortices. Phys. Rev. Lett. 2006;97:163903. doi: 10.1103/PhysRevLett.97.163903. [DOI] [PubMed] [Google Scholar]
- 39.Barreiro JT, Wei TC, Kwiat PG. Beating the channel capacity limit for linear photonic superdense coding. Nat. Phys. 2008;4:282–286. doi: 10.1038/nphys919. [DOI] [Google Scholar]
- 40.Hickmann JM, et al. Unveiling a truncated optical lattice associated with a triangular aperture using light’s orbital angular momentum. Phys. Rev. Lett. 2010;105:053904. doi: 10.1103/PhysRevLett.105.053904. [DOI] [PubMed] [Google Scholar]
- 41.Yu NF, et al. Light propagation with phase discontinuities: generalized laws of reflection and refraction. Science. 2011;334:333–337. doi: 10.1126/science.1210713. [DOI] [PubMed] [Google Scholar]
- 42.Cai XL, et al. Integrated compact optical vortex beam emitters. Science. 2012;338:363–366. doi: 10.1126/science.1226528. [DOI] [PubMed] [Google Scholar]
- 43.Wang J, et al. Terabit free-space data transmission employing orbital angular momentum multiplexing. Nat, Photonics. 2012;6:488–496. doi: 10.1038/nphoton.2012.138. [DOI] [Google Scholar]
- 44.Bozinovic N, et al. Terabit-scale orbital angular momentum mode division multiplexing in fibers. Science. 2013;340:1545–1548. doi: 10.1126/science.1237861. [DOI] [PubMed] [Google Scholar]
- 45.Fickler R, et al. Quantum entanglement of angular momentum states with quantum numbers up to 10,010. Proc. Natl Acad. Sci. USA. 2016;113:13642–13647. doi: 10.1073/pnas.1616889113. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 46.Devlin RC, et al. Arbitrary spin-to–orbital angular momentum conversion of light. Science. 2017;358:896–901. doi: 10.1126/science.aao5392. [DOI] [PubMed] [Google Scholar]
- 47.Stav T, et al. Quantum entanglement of the spin and orbital angular momentum of photons using metamaterials. Science. 2018;361:1101–1104. doi: 10.1126/science.aat9042. [DOI] [PubMed] [Google Scholar]
- 48.Kong FQ, et al. Controlling the orbital angular momentum of high harmonic vortices. Nat. Commun. 2017;8:14970. doi: 10.1038/ncomms14970. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 49.Gauthier D, et al. Tunable orbital angular momentum in high-harmonic generation. Nat. Commun. 2017;8:14971. doi: 10.1038/ncomms14971. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 50.Lee JCT, et al. Laguerre–Gauss and Hermite–Gauss soft X-ray states generated using diffractive optics. Nat. Photonics. 2019;13:205–209. doi: 10.1038/s41566-018-0328-8. [DOI] [Google Scholar]
- 51.Xie ZW, et al. Ultra-broadband on-chip twisted light emitter for optical communications. Light Sci. Appl. 2018;7:18001. doi: 10.1038/lsa.2018.1. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 52.Zambon NC, et al. Optically controlling the emission chirality of microlasers. Nat. Photonics. 2019;13:283–288. doi: 10.1038/s41566-019-0380-z. [DOI] [Google Scholar]
- 53.Rego L, et al. Generation of extreme-ultraviolet beams with time-varying orbital angular momentum. Science. 2019;364:eaaw9486. doi: 10.1126/science.aaw9486. [DOI] [PubMed] [Google Scholar]
- 54.Nye JF, Berry MV. Dislocations in wave trains. Proc. R Soc A Math. Phys. Eng. Sci. 1974;336:165–190. doi: 10.1098/rspa.1974.0012. [DOI] [Google Scholar]
- 55.Penrose LS, Penrose R. Impossible objects: a special type of visual illusion. Br. J. Psychol. 1958;49:31–33. doi: 10.1111/j.2044-8295.1958.tb00634.x. [DOI] [PubMed] [Google Scholar]
- 56.Bauer T, et al. Observation of optical polarization Möbius strips. Science. 2015;347:964–966. doi: 10.1126/science.1260635. [DOI] [PubMed] [Google Scholar]
- 57.Leach J, Yao E, Padgett MJ. Observation of the vortex structure of a non-integer vortex beam. New J. Phys. 2004;6:71. doi: 10.1088/1367-2630/6/1/071. [DOI] [Google Scholar]
- 58.Berry MV. Optical vortices evolving from helicoidal integer and fractional phase steps. J. Opt. A Pure Appl. Opt. 2004;6:259–268. doi: 10.1088/1464-4258/6/2/018. [DOI] [Google Scholar]
- 59.Verbeeck J, Tian H, Schattschneider P. Production and application of electron vortex beams. Nature. 2010;467:301–304. doi: 10.1038/nature09366. [DOI] [PubMed] [Google Scholar]
- 60.Clark CW, et al. Controlling neutron orbital angular momentum. Nature. 2015;525:504–506. doi: 10.1038/nature15265. [DOI] [PubMed] [Google Scholar]
- 61.Wang XW, et al. Recent advances on optical vortex generation. Nanophotonics. 2018;7:1533–1556. doi: 10.1515/nanoph-2018-0072. [DOI] [Google Scholar]
- 62.Zhu L, Wang J. A review of multiple optical vortices generation: methods and applications. Front. Optoelectron. 2019;12:52–68. doi: 10.1007/s12200-019-0910-9. [DOI] [Google Scholar]
- 63.Chen MLM, Jiang LJ, Sha WEI. Orbital angular momentum generation and detection by geometric-phase based metasurfaces. Appl. Sci. 2018;8:362. doi: 10.3390/app8030362. [DOI] [Google Scholar]
- 64.Barnett SM, Babiker M, Padgett MJ. Optical orbital angular momentum. Philos. Trans. R Soc. A Math. Phys. Eng. Sci. 2017;375:20150444. doi: 10.1098/rsta.2015.0444. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 65.Padgett MJ. Orbital angular momentum 25 years on [Invited] Opt. Express. 2017;25:11265–11274. doi: 10.1364/OE.25.011265. [DOI] [PubMed] [Google Scholar]
- 66.Yao AM, Padgett MJ. Orbital angular momentum: origins, behavior and applications. Adv. Opti. Photonics. 2011;3:161–204. doi: 10.1364/AOP.3.000161. [DOI] [Google Scholar]
- 67.Milonni PW, Boyd RW. Momentum of light in a dielectric medium. Adv. Opt. Photonics. 2010;2:519–553. doi: 10.1364/AOP.2.000519. [DOI] [Google Scholar]
- 68.Nelson DF. Momentum, pseudomomentum, and wave momentum: toward resolving the Minkowski-Abraham controversy. Phys. Rev. A. 1991;44:3985–3996. doi: 10.1103/PhysRevA.44.3985. [DOI] [PubMed] [Google Scholar]
- 69.Bliokh KY, Bekshaev AY, Nori F. Optical momentum, spin, and angular momentum in dispersive media. Phys. Rev. Lett. 2017;119:073901. doi: 10.1103/PhysRevLett.119.073901. [DOI] [PubMed] [Google Scholar]
- 70.Bliokh KY, Nori F. Transverse and longitudinal angular momenta of light. Phys. Rep. 2015;592:1–38. doi: 10.1016/j.physrep.2015.06.003. [DOI] [Google Scholar]
- 71.Karimi E, Boyd RW. Classical entanglement? Science. 2015;350:1172–1173. doi: 10.1126/science.aad7174. [DOI] [PubMed] [Google Scholar]
- 72.Chen YF, Lu TH, Huang KF. Observation of spatially coherent polarization vector fields and visualization of vector singularities. Phys. Rev. Lett. 2006;96:033901. doi: 10.1103/PhysRevLett.96.033901. [DOI] [PubMed] [Google Scholar]
- 73.Chen YF, et al. Observation of vector vortex lattices in polarization states of an isotropic microcavity laser. Phys. Rev. Lett. 2003;90:053904. doi: 10.1103/PhysRevLett.90.053904. [DOI] [PubMed] [Google Scholar]
- 74.Rosales-Guzmán C, Ndagano B, Forbes A. A review of complex vector light fields and their applications. J. Opt. 2018;20:123001. doi: 10.1088/2040-8986/aaeb7d. [DOI] [Google Scholar]
- 75.Abramochkin E, Alieva T. Closed-form expression for mutual intensity evolution of Hermite–Laguerre–Gaussian Schell-model beams. Opt. Lett. 2017;42:4032–4035. doi: 10.1364/OL.42.004032. [DOI] [PubMed] [Google Scholar]
- 76.Alieva T, Bastiaans MJ. Mode mapping in paraxial lossless optics. Opt. Lett. 2005;30:1461–1463. doi: 10.1364/OL.30.001461. [DOI] [PubMed] [Google Scholar]
- 77.Abramochkin EG, Volostnikov VG. Generalized Hermite-Laguerre-Gauss beams. Phys. Wave Phenom. 2010;18:14–22. doi: 10.3103/S1541308X10010036. [DOI] [Google Scholar]
- 78.Bandres MA, Gutiérrez-Vega JC. Ince–Gaussian beams. Opt. Lett. 2004;29:144–146. doi: 10.1364/OL.29.000144. [DOI] [PubMed] [Google Scholar]
- 79.Bandres MA, Gutiérrez-Vega JC. Elliptical beams. Opt. Express. 2008;16:21087–21092. doi: 10.1364/OE.16.021087. [DOI] [PubMed] [Google Scholar]
- 80.Bandres MA, Gutiérrez-Vega JC. Ince–Gaussian modes of the paraxial wave equation and stable resonators. J. Opt. Soc. Am. A. 2004;21:873–880. doi: 10.1364/JOSAA.21.000873. [DOI] [PubMed] [Google Scholar]
- 81.Bentley JB, et al. Generation of helical Ince-Gaussian beams with a liquid-crystal display. Opt. Lett. 2006;31:649–651. doi: 10.1364/OL.31.000649. [DOI] [PubMed] [Google Scholar]
- 82.Woerdemann M, Alpmann C, Denz C. Optical assembly of microparticles into highly ordered structures using Ince–Gaussian beams. Appl. Phys. Lett. 2011;98:111101. doi: 10.1063/1.3561770. [DOI] [Google Scholar]
- 83.Shen YJ, et al. Hybrid topological evolution of multi-singularity vortex beams: generalized nature for helical-Ince-Gaussian and Hermite-Laguerre-Gaussian modes. J. Opt. Soc. Am. A. 2019;36:578–587. doi: 10.1364/JOSAA.36.000578. [DOI] [PubMed] [Google Scholar]
- 84.Volke-Sepulveda K, et al. Orbital angular momentum of a high-order Bessel light beam. J. Opt. B Quantum Semiclassical Opt. 2002;4:S82–S89. doi: 10.1088/1464-4266/4/2/373. [DOI] [Google Scholar]
- 85.Gutiérrez-Vega JC, Iturbe-Castillo MD, Chávez-Cerda S. Alternative formulation for invariant optical fields: Mathieu beams. Opt. Lett. 2000;25:1493–1495. doi: 10.1364/OL.25.001493. [DOI] [PubMed] [Google Scholar]
- 86.Lóxpez-Mariscal C, et al. Orbital angular momentum transfer in helical Mathieu beams. Opt. Express. 2006;14:4182–4187. doi: 10.1364/OE.14.004182. [DOI] [PubMed] [Google Scholar]
- 87.Chávez-Cerda S, et al. Holographic generation and orbital angular momentum of high-order Mathieu beams. J. Opt. B Quantum Semiclassical Opt. 2002;4:S52–S57. doi: 10.1088/1464-4266/4/2/368. [DOI] [Google Scholar]
- 88.Alpmann C, et al. Mathieu beams as versatile light moulds for 3D micro particle assemblies. Opt. Express. 2010;18:26084–26091. doi: 10.1364/OE.18.026084. [DOI] [PubMed] [Google Scholar]
- 89.Zhu L, Wang J. Demonstration of obstruction-free data-carrying N-fold Bessel modes multicasting from a single Gaussian mode. Opt. Lett. 2015;40:5463–5466. doi: 10.1364/OL.40.005463. [DOI] [PubMed] [Google Scholar]
- 90.Bužek V, Quang T. Generalized coherent state for bosonic realization of SU(2)Lie algebra. J. Opt. Soc. Am. B. 1989;6:2447–2449. doi: 10.1364/JOSAB.6.002447. [DOI] [Google Scholar]
- 91.Lin YC, et al. Model of commensurate harmonic oscillators with SU(2) coupling interactions: Analogous observation in laser transverse modes. Phys. Rev. E. 2012;85:046217. doi: 10.1103/PhysRevE.85.046217. [DOI] [PubMed] [Google Scholar]
- 92.Tuan PH, et al. Realizing high-pulse-energy large-angular-momentum beams by astigmatic transformation of geometric modes in an Nd:YAG/Cr4+:YAG laser. IEEE J. Sel. Top. Quantum Electron. 2018;24:1600809. doi: 10.1109/JSTQE.2018.2798999. [DOI] [Google Scholar]
- 93.Tung JC, et al. Exploring vortex structures in orbital-angular-momentum beams generated from planar geometric modes with a mode converter. Opt. Express. 2016;24:22796–22805. doi: 10.1364/OE.24.022796. [DOI] [PubMed] [Google Scholar]
- 94.Chen YF, et al. Devil’s staircase in three-dimensional coherent waves localized on Lissajous parametric surfaces. Phys. Rev. Lett. 2006;96:213902. doi: 10.1103/PhysRevLett.96.213902. [DOI] [PubMed] [Google Scholar]
- 95.Lu TH, et al. Three-dimensional coherent optical waves localized on trochoidal parametric surfaces. Phys. Rev. Lett. 2008;101:233901. doi: 10.1103/PhysRevLett.101.233901. [DOI] [PubMed] [Google Scholar]
- 96.Shen YJ, et al. Polygonal vortex beams. IEEE Photonics J. 2018;10:1503016. [Google Scholar]
- 97.Shen YJ, Fu X, Gong ML. Truncated triangular diffraction lattices and orbital-angular-momentum detection of vortex SU(2) geometric modes. Opt. Express. 2018;26:25545–25557. doi: 10.1364/OE.26.025545. [DOI] [PubMed] [Google Scholar]
- 98.Freund I. Optical Möbius strips in three-dimensional ellipse fields: I. Lines of circular polarization. Opt. Commun. 2010;283:1–15. doi: 10.1016/j.optcom.2009.09.042. [DOI] [Google Scholar]
- 99.Freund I. Optical Möbius strips in three dimensional ellipse fields: II. Lines of linear polarization. Opt. Commun. 2010;283:16–28. doi: 10.1016/j.optcom.2009.09.037. [DOI] [Google Scholar]
- 100.Galvez EJ, et al. Multitwist Möbius strips and twisted ribbons in the polarization of paraxial light beams. Sci. Rep. 2017;7:13653. doi: 10.1038/s41598-017-13199-1. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 101.Veretenov NA, Fedorov SV, Rosanov NN. Topological vortex and knotted dissipative optical 3D solitons generated by 2D vortex solitons. Phys. Rev. Lett. 2017;119:263901. doi: 10.1103/PhysRevLett.119.263901. [DOI] [PubMed] [Google Scholar]
- 102.Leach J, et al. Vortex knots in light. New J. Phys. 2005;7:55. doi: 10.1088/1367-2630/7/1/055. [DOI] [Google Scholar]
- 103.Kleckner D, Irvine WTM. Creation and dynamics of knotted vortices. Nat. Phys. 2013;9:253–258. doi: 10.1038/nphys2560. [DOI] [Google Scholar]
- 104.Dennis MR, et al. Isolated optical vortex knots. Nat. Phys. 2010;6:118–121. doi: 10.1038/nphys1504. [DOI] [Google Scholar]
- 105.Tempone-Wiltshire SJ, Johnstone SP, Helmerson K. Optical vortex knots–one photon at a time. Sci. Rep. 2016;6:24463. doi: 10.1038/srep24463. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 106.Cunzhi S, Pu JX, Chávez-Cerda S. Elegant Cartesian Laguerre–Hermite–Gaussian laser cavity modes. Opt. Lett. 2015;40:1105–1108. doi: 10.1364/OL.40.001105. [DOI] [PubMed] [Google Scholar]
- 107.Ellenbogen T, et al. Nonlinear generation and manipulation of Airy beams. Nat. Photonics. 2009;3:395–398. doi: 10.1038/nphoton.2009.95. [DOI] [Google Scholar]
- 108.Ring JD, et al. Auto-focusing and self-healing of Pearcey beams. Opt. Express. 2012;20:18955–18966. doi: 10.1364/OE.20.018955. [DOI] [PubMed] [Google Scholar]
- 109.Bandres MA, Gutiérrez-Vega JC, Chávez-Cerda S. Parabolic nondiffracting optical wave fields. Opt. Lett. 2004;29:44–46. doi: 10.1364/OL.29.000044. [DOI] [PubMed] [Google Scholar]
- 110.O’Holleran K, et al. Fractality of light’s darkness. Phys. Rev. Lett. 2008;100:053902. doi: 10.1103/PhysRevLett.100.053902. [DOI] [PubMed] [Google Scholar]
- 111.Zhang LG, et al. Deflection of a reflected intense vortex laser beam. Phys. Rev. Lett. 2016;117:113904. doi: 10.1103/PhysRevLett.117.113904. [DOI] [PubMed] [Google Scholar]
- 112.Omatsu T, Miyamoto K, Lee AJ. Wavelength-versatile optical vortex lasers. J. Opt. 2017;19:123002. doi: 10.1088/2040-8986/aa9445. [DOI] [Google Scholar]
- 113.de Araujo LEE, Anderson ME. Measuring vortex charge with a triangular aperture. Opt. Lett. 2011;36:787–789. doi: 10.1364/OL.36.000787. [DOI] [PubMed] [Google Scholar]
- 114.Mourka A, et al. Visualization of the birth of an optical vortex using diffraction from a triangular aperture. Opt. Express. 2011;19:5760–5771. doi: 10.1364/OE.19.005760. [DOI] [PubMed] [Google Scholar]
- 115.Melo LA, et al. Direct measurement of the topological charge in elliptical beams using diffraction by a triangular aperture. Sci. Rep. 2018;8:6370. doi: 10.1038/s41598-018-24928-5. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 116.Ghai DP, Senthilkumaran P, Sirohi RS. Single-slit diffraction of an optical beam with phase singularity. Opt. Lasers Eng. 2009;47:123–126. doi: 10.1016/j.optlaseng.2008.07.019. [DOI] [Google Scholar]
- 117.Mesquita PHF, et al. Engineering a square truncated lattice with light’s orbital angular momentum. Opt. Express. 2011;19:20616–20621. doi: 10.1364/OE.19.020616. [DOI] [PubMed] [Google Scholar]
- 118.Liu YX, et al. Propagation of an optical vortex beam through a diamond-shaped aperture. Opt. Laser Technol. 2013;45:473–479. doi: 10.1016/j.optlastec.2012.06.007. [DOI] [Google Scholar]
- 119.Ambuj A, Vyas R, Singh S. Diffraction of orbital angular momentum carrying optical beams by a circular aperture. Opt. Lett. 2014;39:5475–5478. doi: 10.1364/OL.39.005475. [DOI] [PubMed] [Google Scholar]
- 120.Taira Y, Zhang SK. Split in phase singularities of an optical vortex by off-axis diffraction through a simple circular aperture. Opt. Lett. 2017;42:1373–1376. doi: 10.1364/OL.42.001373. [DOI] [PubMed] [Google Scholar]
- 121.Bahl M, Senthilkumaran P. Energy circulations in singular beams diffracted through an isosceles right triangular aperture. Phys. Rev. A. 2015;92:013831. doi: 10.1103/PhysRevA.92.013831. [DOI] [Google Scholar]
- 122.Chen RS, et al. Detecting the topological charge of optical vortex beams using a sectorial screen. Appl. Opt. 2017;56:4868–4872. doi: 10.1364/AO.56.004868. [DOI] [PubMed] [Google Scholar]
- 123.Zhang WH, et al. Experimental demonstration of twisted light’s diffraction theory based on digital spiral imaging. Chin. Opt. Lett. 2016;14:110501. doi: 10.3788/COL201614.110501. [DOI] [Google Scholar]
- 124.Ram BSB, Sharma A, Senthilkumaran P. Diffraction of V-point singularities through triangular apertures. Opt. Express. 2017;25:10270–10275. doi: 10.1364/OE.25.010270. [DOI] [PubMed] [Google Scholar]
- 125.Holleczek A, et al. Classical and quantum properties of cylindrically polarized states of light. Opt. Express. 2011;19:9714–9736. doi: 10.1364/OE.19.009714. [DOI] [PubMed] [Google Scholar]
- 126.Milione G, et al. Higher-order Poincaré sphere, Stokes parameters, and the angular momentum of light. Phys. Rev. Lett. 2011;107:053601. doi: 10.1103/PhysRevLett.107.053601. [DOI] [PubMed] [Google Scholar]
- 127.Naidoo D, et al. Controlled generation of higher-order Poincaré sphere beams from a laser. Nat. Photonics. 2016;10:327–332. doi: 10.1038/nphoton.2016.37. [DOI] [Google Scholar]
- 128.Yi XN, et al. Hybrid-order Poincaré sphere. Phys. Rev. A. 2015;91:023801. doi: 10.1103/PhysRevA.91.023801. [DOI] [Google Scholar]
- 129.Liu ZX, et al. Generation of arbitrary vector vortex beams on hybrid-order Poincaré sphere. Photonics Res. 2017;5:15–21. doi: 10.1364/PRJ.5.000015. [DOI] [Google Scholar]
- 130.Wang RS, et al. Electrically driven generation of arbitrary vector vortex beams on the hybrid-order Poincaré sphere. Opt. Lett. 2018;43:3570–3573. doi: 10.1364/OL.43.003570. [DOI] [PubMed] [Google Scholar]
- 131.Franke-Arnold S, et al. Uncertainty principle for angular position and angular momentum. New J. Phys. 2004;6:103. doi: 10.1088/1367-2630/6/1/103. [DOI] [Google Scholar]
- 132.Leach J, et al. Quantum correlations in optical angle–orbital angular momentum variables. Science. 2010;329:662–665. doi: 10.1126/science.1190523. [DOI] [PubMed] [Google Scholar]
- 133.Jha AK, et al. Fourier relationship between the angle and angular momentum of entangled photons. Phys. Rev. A. 2008;78:043810. doi: 10.1103/PhysRevA.78.043810. [DOI] [Google Scholar]
- 134.Erhard M, et al. Twisted photons: new quantum perspectives in high dimensions. Light Sci. Appl. 2018;7:17146. doi: 10.1038/lsa.2017.146. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 135.Otte E, et al. Entanglement beating in free space through spin–orbit coupling. Light Sci. Appl. 2018;7:18009. doi: 10.1038/lsa.2018.9. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 136.Bliokh KY, et al. Spin–orbit interactions of light. Nat. Photonics. 2015;9:796–808. doi: 10.1038/nphoton.2015.201. [DOI] [Google Scholar]
- 137.Cardano F, Marrucci L. Spin–orbit photonics. Nat. Photonics. 2015;9:776–778. doi: 10.1038/nphoton.2015.232. [DOI] [Google Scholar]
- 138.Shao ZK, et al. Spin-orbit interaction of light induced by transverse spin angular momentum engineering. Nat. Commun. 2018;9:926. doi: 10.1038/s41467-018-03237-5. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 139.Magaña-Loaiza OS, et al. Hanbury brown and Twiss interferometry with twisted light. Sci. Adv. 2016;2:e1501143. doi: 10.1126/sciadv.1501143. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 140.Mohanty A, et al. Quantum interference between transverse spatial waveguide modes. Nat. Commun. 2017;8:14010. doi: 10.1038/ncomms14010. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 141.Zhang YW, et al. Engineering two-photon high-dimensional states through quantum interference. Sci. Adv. 2016;2:e1501165. doi: 10.1126/sciadv.1501165. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 142.Yin XB, et al. Photonic spin Hall effect at metasurfaces. Science. 2013;339:1405–1407. doi: 10.1126/science.1231758. [DOI] [PubMed] [Google Scholar]
- 143.Liu YC, et al. Photonic spin Hall effect in metasurfaces: a brief review. Nanophotonics. 2017;6:51–70. doi: 10.1515/nanoph-2015-0155. [DOI] [Google Scholar]
- 144.Forbes A, Dudley A, McLaren M. Creation and detection of optical modes with spatial light modulators. Adv. Opt. Photonics. 2016;8:200–227. doi: 10.1364/AOP.8.000200. [DOI] [Google Scholar]
- 145.Berkhout GCG, et al. Efficient sorting of orbital angular momentum states of light. Phys. Rev. Lett. 2010;105:153601. doi: 10.1103/PhysRevLett.105.153601. [DOI] [PubMed] [Google Scholar]
- 146.Wen YH, et al. Spiral transformation for high-resolution and efficient sorting of optical vortex modes. Phys. Rev. Lett. 2018;120:193904. doi: 10.1103/PhysRevLett.120.193904. [DOI] [PubMed] [Google Scholar]
- 147.Liu GG, et al. Measurement of the topological charge and index of vortex vector optical fields with a space-variant half-wave plate. Opt. Lett. 2018;43:823–826. doi: 10.1364/OL.43.000823. [DOI] [PubMed] [Google Scholar]
- 148.Ndagano B, et al. Beam quality measure for vector beams. Opt. Lett. 2016;41:3407–3410. doi: 10.1364/OL.41.003407. [DOI] [PubMed] [Google Scholar]
- 149.McLaren M, Konrad T, Forbes A. Measuring the nonseparability of vector vortex beams. Phys. Rev. A. 2015;92:023833. doi: 10.1103/PhysRevA.92.023833. [DOI] [Google Scholar]
- 150.Forbes A. Controlling light’s helicity at the source: orbital angular momentum states from lasers. Philos. Trans. R Soc. A Math. Phys. Eng. Sci. 2017;375:20150436. doi: 10.1098/rsta.2015.0436. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 151.Qiao Z, et al. Generating high-charge optical vortices directly from laser up to 288th order. Laser Photonics Rev. 2018;12:1800019. doi: 10.1002/lpor.201800019. [DOI] [Google Scholar]
- 152.Lee CY, et al. Generation of higher order vortex beams from a YVO4/Nd:YVO4 self-Raman laser via off-axis pumping with mode converter. IEEE J. Sel. Top Quantum Electron. 2015;21:1600305. doi: 10.1109/JSTQE.2015.2432022. [DOI] [Google Scholar]
- 153.Sueda K, et al. Laguerre-Gaussian beam generated with a multilevel spiral phase plate for high intensity laser pulses. Opt. Express. 2004;12:3548–3553. doi: 10.1364/OPEX.12.003548. [DOI] [PubMed] [Google Scholar]
- 154.Cardano F, et al. Polarization pattern of vector vortex beams generated by q-plates with different topological charges. Appl. Opt. 2012;51:C1–C6. doi: 10.1364/AO.51.0000C1. [DOI] [PubMed] [Google Scholar]
- 155.Marrucci L. The q-plate and its future. J. Nanophotonics. 2013;7:078598. doi: 10.1117/1.JNP.7.078598. [DOI] [Google Scholar]
- 156.Brasselet E. Tunable high-resolution macroscopic self-engineered geometric phase optical elements. Phys. Rev. Lett. 2018;121:033901. doi: 10.1103/PhysRevLett.121.033901. [DOI] [PubMed] [Google Scholar]
- 157.Mock A, Sounas D, Alù A. Tunable orbital angular momentum radiation from angular-momentum-biased microcavities. Phys. Rev. Lett. 2018;121:103901. doi: 10.1103/PhysRevLett.121.103901. [DOI] [PubMed] [Google Scholar]
- 158.Zhou N, et al. Generating and synthesizing ultrabroadband twisted light using a compact silicon chip. Opt. Lett. 2018;43:3140–3143. doi: 10.1364/OL.43.003140. [DOI] [PubMed] [Google Scholar]
- 159.Horikawa MT, et al. Handedness control in a tunable midinfrared (6.0-12.5 μm) vortex laser. J. Opt. Soc. Am. B. 2015;32:2406–2410. doi: 10.1364/JOSAB.32.002406. [DOI] [Google Scholar]
- 160.Abulikemu A, et al. Widely-tunable vortex output from a singly resonant optical parametric oscillator. Opt. Express. 2015;23:18338–18344. doi: 10.1364/OE.23.018338. [DOI] [PubMed] [Google Scholar]
- 161.Zhang WD, et al. Optical vortex generation with wavelength tunability based on an acoustically-induced fiber grating. Opt. Express. 2016;24:19278–19285. doi: 10.1364/OE.24.019278. [DOI] [PubMed] [Google Scholar]
- 162.Lyubopytov VS, et al. Simultaneous wavelength and orbital angular momentum demultiplexing using tunable MEMS-based Fabry-Perot filter. Opt. Express. 2017;25:9634–9646. doi: 10.1364/OE.25.009634. [DOI] [PubMed] [Google Scholar]
- 163.Liu QY, et al. Wavelength- and OAM-tunable vortex laser with a reflective volume Bragg grating. Opt. Express. 2017;25:23312–23319. doi: 10.1364/OE.25.023312. [DOI] [PubMed] [Google Scholar]
- 164.Yao SZ, et al. Tunable orbital angular momentum generation using all-fiber fused coupler. IEEE Photonics Technol. Lett. 2018;30:99–102. doi: 10.1109/LPT.2017.2776981. [DOI] [Google Scholar]
- 165.Shen YJ, et al. Wavelength-tunable Hermite–Gaussian modes and an orbital-angular-momentum-tunable vortex beam in a dual-off-axis pumped Yb:CALGO laser. Opt. Lett. 2018;43:291–294. doi: 10.1364/OL.43.000291. [DOI] [PubMed] [Google Scholar]
- 166.Shen YJ, et al. Dual-wavelength vortex beam with high stability in a diode-pumped Yb:CaGdAlO4 laser. Laser Phys. Lett. 2018;15:055803. doi: 10.1088/1612-202X/aaaa97. [DOI] [Google Scholar]
- 167.Wang S, et al. Generation of wavelength- and OAM-tunable vortex beam at low threshold. Opt. Express. 2018;26:18164–18170. doi: 10.1364/OE.26.018164. [DOI] [PubMed] [Google Scholar]
- 168.Zhou N, Liu J, Wang J. Reconfigurable and tunable twisted light laser. Sci. Rep. 2018;8:11394. doi: 10.1038/s41598-018-29868-8. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 169.Fadeyeva TA, et al. Spatially engineered polarization states and optical vortices in uniaxial crystals. Opt. Express. 2010;18:10848–10863. doi: 10.1364/OE.18.010848. [DOI] [PubMed] [Google Scholar]
- 170.Rafayelyan M, Tkachenko G, Brasselet E. Reflective spin-orbit geometric phase from chiral anisotropic optical media. Phys. Rev. Lett. 2016;116:253902. doi: 10.1103/PhysRevLett.116.253902. [DOI] [PubMed] [Google Scholar]
- 171.Kobashi J, Yoshida H, Ozaki M. Polychromatic optical vortex generation from patterned cholesteric liquid crystals. Phys. Rev. Lett. 2016;116:253903. doi: 10.1103/PhysRevLett.116.253903. [DOI] [PubMed] [Google Scholar]
- 172.Piccirillo B, et al. Photon spin-to-orbital angular momentum conversion via an electrically tunable q-plate. Appl. Phys. Lett. 2010;97:241104. doi: 10.1063/1.3527083. [DOI] [Google Scholar]
- 173.Toyoda K, et al. Using optical vortex to control the chirality of twisted metal nanostructures. Nano Lett. 2012;12:3645–3649. doi: 10.1021/nl301347j. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 174.Yang L, et al. Direct laser writing of complex microtubes using femtosecond vortex beams. Appl. Phys. Lett. 2017;110:221103. doi: 10.1063/1.4984744. [DOI] [Google Scholar]
- 175.Zürch M, et al. Strong-field physics with singular light beams. Nat. Phys. 2012;8:743–746. doi: 10.1038/nphys2397. [DOI] [Google Scholar]
- 176.Ran LL, Guo ZY, Qu SL. Rotational motions of optically trapped microscopic particles by a vortex femtosecond laser. Chin. Phys. B. 2012;21:104206. doi: 10.1088/1674-1056/21/10/104206. [DOI] [Google Scholar]
- 177.Ishaaya AA, et al. Efficient selection of high-order Laguerre-Gaussian modes in a Q-switched Nd:YAG laser. IEEE J. Quantum Electron. 2003;39:74–82. doi: 10.1109/JQE.2002.806164. [DOI] [Google Scholar]
- 178.Kim DJ, Kim JW, Clarkson WA. Q-switched Nd:YAG optical vortex lasers. Opt. Express. 2013;21:29449–29454. doi: 10.1364/OE.21.029449. [DOI] [PubMed] [Google Scholar]
- 179.Zhao YG, et al. 1 mJ pulsed vortex laser at 1645 nm with well-defined helicity. Opt. Express. 2016;24:15596–15602. doi: 10.1364/OE.24.015596. [DOI] [PubMed] [Google Scholar]
- 180.Chang CC, et al. Generating high-peak-power structured lights in selectively pumped passively Q-switched lasers with astigmatic mode transformations. Laser Phys. 2017;27:125805. doi: 10.1088/1555-6611/aa92e2. [DOI] [Google Scholar]
- 181.He HS, et al. Low-threshold, nanosecond, high-repetition-rate vortex pulses with controllable helicity generated in Cr, Nd:YAG self-Q-switched microchip laser. Laser Phys. 2018;28:055802. doi: 10.1088/1555-6611/aaad4c. [DOI] [Google Scholar]
- 182.Wang YB, et al. Generation of 1535-nm pulsed vortex beam in a diode-pumped Er, Yb:glass microchip laser. IEEE Photonics Technol. Lett. 2018;30:891–894. doi: 10.1109/LPT.2018.2822838. [DOI] [Google Scholar]
- 183.Koyama M, et al. Power scaling of a picosecond vortex laser based on a stressed Yb-doped fiber amplifier. Opt. Express. 2011;19:994–999. doi: 10.1364/OE.19.000994. [DOI] [PubMed] [Google Scholar]
- 184.Liang HC, et al. Compact efficient multi-GHz Kerr-lens mode-locked diode-pumped Nd:YVO4 laser. Opt. Express. 2008;16:21149–21154. doi: 10.1364/OE.16.021149. [DOI] [PubMed] [Google Scholar]
- 185.Liang HC, et al. Picosecond optical vortex converted from multigigahertz self-mode-locked high-order Hermite-Gaussian Nd:GdVO4 lasers. Opt. Letters. 2009;34:3842–3844. doi: 10.1364/OL.34.003842. [DOI] [PubMed] [Google Scholar]
- 186.Liang HC, et al. Total self-mode locking of multi-pass geometric modes in diode-pumped Nd:YVO4 lasers. Laser Phys. Lett. 2013;10:105804. doi: 10.1088/1612-2011/10/10/105804. [DOI] [Google Scholar]
- 187.Tung JC, et al. Exploring the self-mode locking and vortex structures of nonplanar elliptical modes in selectively end-pumped Nd:YVO4 lasers: manifestation of large fractional orbital angular momentum. Opt. Express. 2017;25:22769–22779. doi: 10.1364/OE.25.022769. [DOI] [PubMed] [Google Scholar]
- 188.Huang K, et al. Controlled generation of ultrafast vector vortex beams from a mode-locked fiber laser. Opt. Lett. 2018;43:3933–3936. doi: 10.1364/OL.43.003933. [DOI] [PubMed] [Google Scholar]
- 189.Bolze T, Nuernberger P. Temporally shaped Laguerre–Gaussian femtosecond laser beams. Appl. Opt. 2018;57:3624–3628. doi: 10.1364/AO.57.003624. [DOI] [PubMed] [Google Scholar]
- 190.Zhuang WZ, et al. High-power high-repetition-rate subpicosecond monolithic Yb:KGW laser with self-mode locking. Opt. Lett. 2013;38:2596–2599. doi: 10.1364/OL.38.002596. [DOI] [PubMed] [Google Scholar]
- 191.Chang MT, et al. Exploring transverse pattern formation in a dual-polarization self-mode-locked monolithic Yb: KGW laser and generating a 25-GHz sub-picosecond vortex beam via gain competition. Opt. Express. 2016;24:8754–8762. doi: 10.1364/OE.24.008754. [DOI] [PubMed] [Google Scholar]
- 192.Zhang ZM, et al. Generation of all-fiber femtosecond vortex laser based on NPR mode-locking and mechanical LPG. Chin. Opt. Lett. 2018;16:110501. doi: 10.3788/COL201816.110501. [DOI] [Google Scholar]
- 193.Wang S, et al. Direct emission of chirality controllable femtosecond LG01 vortex beam. Appl. Phys. Lett. 2018;112:201110. doi: 10.1063/1.5028477. [DOI] [Google Scholar]
- 194.Wang S, et al. Direct generation of femtosecond vortex beam from a Yb:KYW oscillator featuring a defect-spot mirror. OSA Contin. 2019;2:523–530. doi: 10.1364/OSAC.2.000523. [DOI] [Google Scholar]
- 195.Woerdemann M, et al. Advanced optical trapping by complex beam shaping. Laser Photonics Rev. 2013;7:839–854. doi: 10.1002/lpor.201200058. [DOI] [Google Scholar]
- 196.Li XF, et al. Automultiscopic displays based on orbital angular momentum of light. J. Opt. 2016;18:085608. doi: 10.1088/2040-8978/18/8/085608. [DOI] [Google Scholar]
- 197.Anguita JA, Herreros J, Djordjevic IB. Coherent multimode OAM superpositions for multidimensional modulation. IEEE Photonics J. 2014;6:7900811. doi: 10.1109/JPHOT.2014.2309645. [DOI] [Google Scholar]
- 198.Padgett M, et al. An experiment to observe the intensity and phase structure of Laguerre–Gaussian laser modes. Am. J. Phys. 1996;64:77–82. doi: 10.1119/1.18283. [DOI] [Google Scholar]
- 199.Courtial J, Padgett MJ. Performance of a cylindrical lens mode converter for producing Laguerre–Gaussian laser modes. Opt. Commun. 1999;159:13–18. doi: 10.1016/S0030-4018(98)00599-9. [DOI] [Google Scholar]
- 200.O’Neil AT, Courtial J. Mode transformations in terms of the constituent Hermite–Gaussian or Laguerre–Gaussian modes and the variable-phase mode converter. Opt. Commun. 2000;181:35–45. doi: 10.1016/S0030-4018(00)00736-7. [DOI] [Google Scholar]
- 201.Padgett MJ, Allen L. Orbital angular momentum exchange in cylindrical-lens mode converters. J. Opt. B Quantum Semiclassical Opt. 2002;4:S17–S19. doi: 10.1088/1464-4266/4/2/362. [DOI] [Google Scholar]
- 202.Shen YJ, et al. Observation of spectral modulation coupled with broadband transverse-mode-locking in an Yb:CALGO frequency-degenerate cavity. Chin. Opt. Lett. 2019;17:031404. doi: 10.3788/COL201917.031404. [DOI] [Google Scholar]
- 203.Shen YJ, et al. Vortex lattices with transverse-mode-locking states switching in a large-aperture off-axis-pumped solid-state laser. J. Opt. Soc. Am. B. 2018;35:2940–2944. doi: 10.1364/JOSAB.35.002940. [DOI] [Google Scholar]
- 204.Shen YJ, et al. Periodic-trajectory-controlled, coherent-state-phase-switched, and wavelength-tunable SU(2) geometric modes in a frequency-degenerate resonator. Appl. Opt. 2018;57:9543–9549. doi: 10.1364/AO.57.009543. [DOI] [PubMed] [Google Scholar]
- 205.Ngcobo S, et al. A digital laser for on-demand laser modes. Nat. Commun. 2013;4:2289. doi: 10.1038/ncomms3289. [DOI] [PubMed] [Google Scholar]
- 206.Porfirev AP, Khonina SN. Simple method for efficient reconfigurable optical vortex beam splitting. Opt. Express. 2017;25:18722–18735. doi: 10.1364/OE.25.018722. [DOI] [PubMed] [Google Scholar]
- 207.Ma HX, et al. Generation of circular optical vortex array. Ann. Phys. 2017;529:1700285. doi: 10.1002/andp.201700285. [DOI] [Google Scholar]
- 208.Li L, et al. Generation of optical vortex array along arbitrary curvilinear arrangement. Opt. Express. 2018;26:9798–9812. doi: 10.1364/OE.26.009798. [DOI] [PubMed] [Google Scholar]
- 209.Wan ZS, et al. Quadrant-separable multi-singularity vortices manipulation by coherent superposed mode with spatial-energy mismatch. Opt. Express. 2018;26:34940–34955. doi: 10.1364/OE.26.034940. [DOI] [PubMed] [Google Scholar]
- 210.Hou TY, et al. Spatially-distributed orbital angular momentum beam array generation based on greedy algorithms and coherent combining technology. Opt. Express. 2018;26:14945–14958. doi: 10.1364/OE.26.014945. [DOI] [PubMed] [Google Scholar]
- 211.Gbur G. Fractional vortex Hilbert’s hotel. Optica. 2016;3:222–225. doi: 10.1364/OPTICA.3.000222. [DOI] [Google Scholar]
- 212.Wang YYD, Gbur G. Hilbert’s Hotel in polarization singularities. Opt. Lett. 2017;42:5154–5157. doi: 10.1364/OL.42.005154. [DOI] [PubMed] [Google Scholar]
- 213.Ferrando A, García-March MA. Analytical solution for multi-singular vortex Gaussian beams: the mathematical theory of scattering modes. J. Opt. 2016;18:064006. doi: 10.1088/2040-8978/18/6/064006. [DOI] [Google Scholar]
- 214.Brasselet E. Tunable optical vortex arrays from a single nematic topological defect. Phys. Rev. Lett. 2012;108:087801. doi: 10.1103/PhysRevLett.108.087801. [DOI] [PubMed] [Google Scholar]
- 215.Barboza R, et al. Harnessing optical vortex lattices in nematic liquid crystals. Phys. Rev. Lett. 2013;111:093902. doi: 10.1103/PhysRevLett.111.093902. [DOI] [PubMed] [Google Scholar]
- 216.Ashkin A. Acceleration and trapping of particles by radiation pressure. Phys. Rev. Lett. 1970;24:156–159. doi: 10.1103/PhysRevLett.24.156. [DOI] [Google Scholar]
- 217.Padgett M, Bowman R. Tweezers with a twist. Nat. Photonics. 2011;5:343–348. doi: 10.1038/nphoton.2011.81. [DOI] [Google Scholar]
- 218.Chapin SC, Germain V, Dufresne ER. Automated trapping, assembly, and sorting with holographic optical tweezers. Opt. Express. 2006;14:13095–13100. doi: 10.1364/OE.14.013095. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 219.Tao SH, et al. Fractional optical vortex beam induced rotation of particles. Opt. Express. 2005;13:7726–7731. doi: 10.1364/OPEX.13.007726. [DOI] [PubMed] [Google Scholar]
- 220.Gong L, et al. Optical forces of focused femtosecond laser pulses on nonlinear optical Rayleigh particles. Photonics Res. 2018;6:138–143. doi: 10.1364/PRJ.6.000138. [DOI] [Google Scholar]
- 221.Zhang YQ, et al. Nonlinearity-induced multiplexed optical trapping and manipulation with femtosecond vector beams. Nano Lett. 2018;18:5538–5543. doi: 10.1021/acs.nanolett.8b01929. [DOI] [PubMed] [Google Scholar]
- 222.Shen Z, et al. Visualizing orbital angular momentum of plasmonic vortices. Opt. Lett. 2012;37:4627–4629. doi: 10.1364/OL.37.004627. [DOI] [PubMed] [Google Scholar]
- 223.Zhang YQ, et al. A plasmonic spanner for metal particle manipulation. Sci. Rep. 2015;5:15446. doi: 10.1038/srep15446. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 224.Richardson DJ, Fini JM, Nelson LE. Space-division multiplexing in optical fibres. Nat. Photonics. 2013;7:354–362. doi: 10.1038/nphoton.2013.94. [DOI] [Google Scholar]
- 225.Wang J. Advances in communications using optical vortices. Photonics Res. 2016;4:B14–B28. doi: 10.1364/PRJ.4.000B14. [DOI] [Google Scholar]
- 226.Willner AE, et al. Optical communications using orbital angular momentum beams. Adv. Opt. Photonics. 2015;7:66–106. doi: 10.1364/AOP.7.000066. [DOI] [Google Scholar]
- 227.Lavery MPJ, et al. Free-space propagation of high-dimensional structured optical fields in an urban environment. Sci. Adv. 2017;3:e1700552. doi: 10.1126/sciadv.1700552. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 228.Li L, et al. High-capacity free-space optical communications between a ground transmitter and a ground receiver via a UAV using multiplexing of multiple orbital-angular-momentum beams. Sci. Rep. 2017;7:17427. doi: 10.1038/s41598-017-17580-y. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 229.Yan Y, et al. High-capacity millimetre-wave communications with orbital angular momentum multiplexing. Nat. Commun. 2014;5:4876. doi: 10.1038/ncomms5876. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 230.Jia P, et al. Sidelobe-modulated optical vortices for free-space communication. Opt. Lett. 2013;38:588–590. doi: 10.1364/OL.38.000588. [DOI] [PubMed] [Google Scholar]
- 231.Anguita, J. A., Herreros, J. & Cisternas, J. E. Generation and detection of multiple coaxial vortex beams for free-space optical communications. In Proc. Quantum Electronics and Laser Science Conference. (Optical Society of America, San Jose, California, United States, 2012).
- 232.Heng XB, et al. All-fiber stable orbital angular momentum beam generation and propagation. Opt. Express. 2018;26:17429–17436. doi: 10.1364/OE.26.017429. [DOI] [PubMed] [Google Scholar]
- 233.Xie ZW, et al. Integrated (de)multiplexer for orbital angular momentum fiber communication. Photonics Res. 2018;6:743–749. doi: 10.1364/PRJ.6.000743. [DOI] [Google Scholar]
- 234.Lei T, et al. Massive individual orbital angular momentum channels for multiplexing enabled by Dammann gratings. Light Sci. Appl. 2015;4:e257. doi: 10.1038/lsa.2015.30. [DOI] [Google Scholar]
- 235.Ren YX, et al. Orbital angular momentum-based space division multiplexing for high-capacity underwater optical communications. Sci. Rep. 2016;6:33306. doi: 10.1038/srep33306. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 236.D’Ambrosio V, et al. Complete experimental toolbox for alignment-free quantum communication. Nat. Commun. 2012;3:961. doi: 10.1038/ncomms1951. [DOI] [PubMed] [Google Scholar]
- 237.Zhu L, et al. Orbital angular momentum mode groups multiplexing transmission over 2.6-km conventional multi-mode fiber. Opt. Express. 2017;25:25637–25645. doi: 10.1364/OE.25.025637. [DOI] [PubMed] [Google Scholar]
- 238.Mafu M, et al. Higher-dimensional orbital-angular-momentum-based quantum key distribution with mutually unbiased bases. Phys. Rev. A. 2013;88:032305. doi: 10.1103/PhysRevA.88.032305. [DOI] [Google Scholar]
- 239.Sit A, et al. High-dimensional intracity quantum cryptography with structured photons. Optica. 2017;4:1006–1010. doi: 10.1364/OPTICA.4.001006. [DOI] [Google Scholar]
- 240.Nicolas A, et al. A quantum memory for orbital angular momentum photonic qubits. Nat. Photonics. 2014;8:234–238. doi: 10.1038/nphoton.2013.355. [DOI] [Google Scholar]
- 241.Ding DS, et al. Quantum storage of orbital angular momentum entanglement in an atomic ensemble. Phys. Rev. Lett. 2015;114:050502. doi: 10.1103/PhysRevLett.114.050502. [DOI] [PubMed] [Google Scholar]
- 242.Zhou ZQ, et al. Quantum storage of three-dimensional orbital-angular-momentum entanglement in a crystal. Phys. Rev. Lett. 2015;115:070502. doi: 10.1103/PhysRevLett.115.070502. [DOI] [PubMed] [Google Scholar]
- 243.Chen LX, Lei JJ, Romero J. Quantum digital spiral imaging. Light Sci. Appl. 2014;3:e153. doi: 10.1038/lsa.2014.34. [DOI] [Google Scholar]
- 244.Nagali E, et al. Optimal quantum cloning of orbital angular momentum photon qubits through Hong–Ou–Mandel coalescence. Nat. Photonics. 2009;3:720–723. doi: 10.1038/nphoton.2009.214. [DOI] [Google Scholar]
- 245.Ndagano B, et al. Creation and detection of vector vortex modes for classical and quantum communication. J. Lightwave Technol. 2018;36:292–301. doi: 10.1109/JLT.2017.2766760. [DOI] [Google Scholar]
- 246.Wang XL, et al. Quantum teleportation of multiple degrees of freedom of a single photon. Nature. 2015;518:516–519. doi: 10.1038/nature14246. [DOI] [PubMed] [Google Scholar]
- 247.Wang XL, et al. 18-qubit entanglement with six photons’ three degrees of freedom. Phys. Rev. Lett. 2018;120:260502. doi: 10.1103/PhysRevLett.120.260502. [DOI] [PubMed] [Google Scholar]
- 248.Toninelli E, et al. Concepts in quantum state tomography and classical implementation with intense light: a tutorial. Adv. Opt. Photonics. 2019;11:67–134. doi: 10.1364/AOP.11.000067. [DOI] [Google Scholar]
- 249.Sephton B, et al. A versatile quantum walk resonator with bright classical light. PLoS ONE. 2019;14:e0214891. doi: 10.1371/journal.pone.0214891. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 250.Ndagano B, et al. Characterizing quantum channels with non-separable states of classical light. Nat. Phys. 2017;13:397–402. doi: 10.1038/nphys4003. [DOI] [Google Scholar]
- 251.Vieira J, et al. Amplification and generation of ultra-intense twisted laser pulses via stimulated Raman scattering. Nat. Commun. 2016;7:10371. doi: 10.1038/ncomms10371. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 252.Lenzini F, et al. Optical vortex interaction and generation via nonlinear wave mixing. Phys. Rev. A. 2011;84:061801. doi: 10.1103/PhysRevA.84.061801. [DOI] [Google Scholar]
- 253.Jiang W, et al. Computation of topological charges of optical vortices via nondegenerate four-wave mixing. Phys. Rev. A. 2006;74:043811. doi: 10.1103/PhysRevA.74.043811. [DOI] [Google Scholar]
- 254.Li GX, et al. Continuous control of the nonlinearity phase for harmonic generations. Nat. Mater. 2015;14:607–612. doi: 10.1038/nmat4267. [DOI] [PubMed] [Google Scholar]
- 255.Tymchenko M, et al. Gradient nonlinear Pancharatnam-Berry metasurfaces. Phys. Rev. Lett. 2015;115:207403. doi: 10.1103/PhysRevLett.115.207403. [DOI] [PubMed] [Google Scholar]
- 256.Keren-Zur S, et al. Nonlinear beam shaping with plasmonic metasurfaces. ACS Photonics. 2015;3:117–123. doi: 10.1021/acsphotonics.5b00528. [DOI] [Google Scholar]
- 257.Li GX, Zentgraf T, Zhang S. Rotational Doppler effect in nonlinear optics. Nat. Phys. 2016;12:736–740. doi: 10.1038/nphys3699. [DOI] [Google Scholar]
- 258.Musarra G, et al. Rotation-dependent nonlinear absorption of orbital angular momentum beams in ruby. Opt. Lett. 2018;43:3073–3075. doi: 10.1364/OL.43.003073. [DOI] [PubMed] [Google Scholar]
- 259.Qiu CW, Yang YJ. Vortex generation reaches a new plateau. Science. 2017;357:645. doi: 10.1126/science.aan6359. [DOI] [PubMed] [Google Scholar]
- 260.Toda Y, et al. Single orbital angular mode emission from externally feed-backed vertical cavity surface emitting laser. Appl. Phys. Lett. 2017;111:101102. doi: 10.1063/1.4989479. [DOI] [Google Scholar]
- 261.Miao P, et al. Orbital angular momentum microlaser. Science. 2016;353:464–467. doi: 10.1126/science.aaf8533. [DOI] [PubMed] [Google Scholar]
- 262.Wang J. Metasurfaces enabling structured light manipulation: advances and perspectives [Invited] Chin. Opt. Lett. 2018;16:050006. doi: 10.3788/COL201816.050006. [DOI] [Google Scholar]
- 263.Huang LL, et al. Dispersionless phase discontinuities for controlling light propagation. Nano Lett. 2012;12:5750–5755. doi: 10.1021/nl303031j. [DOI] [PubMed] [Google Scholar]
- 264.Sun YZ, et al. Vector beam generation via micrometer-scale photonic integrated circuits and plasmonic Nano-antennae. J. Opt. Soc. Am. B. 2016;33:360–366. doi: 10.1364/JOSAB.33.000360. [DOI] [Google Scholar]
- 265.Chen P, et al. Digitalizing self-assembled chiral superstructures for optical vortex processing. Adv. Mater. 2018;30:1705865. doi: 10.1002/adma.201705865. [DOI] [PubMed] [Google Scholar]
- 266.Chen Y, et al. Mapping twisted light into and out of a photonic chip. Phys. Rev. Lett. 2018;121:233602. doi: 10.1103/PhysRevLett.121.233602. [DOI] [PubMed] [Google Scholar]
- 267.Jin Y, et al. Dynamic modulation of spatially structured polarization fields for real-time control of ultrafast laser-material interactions. Opt. Express. 2013;21:25333–25343. doi: 10.1364/OE.21.025333. [DOI] [PubMed] [Google Scholar]
- 268.Allegre OJ, et al. Complete wavefront and polarization control for ultrashort-pulse laser microprocessing. Opt. Express. 2013;21:21198–21207. doi: 10.1364/OE.21.021198. [DOI] [PubMed] [Google Scholar]
- 269.Toyoda K, et al. Transfer of light helicity to nanostructures. Phys. Rev. Lett. 2013;110:143603. doi: 10.1103/PhysRevLett.110.143603. [DOI] [PubMed] [Google Scholar]
- 270.Syubaev S, et al. Direct laser printing of chiral plasmonic nanojets by vortex beams. Opt. Express. 2017;25:10214–10223. doi: 10.1364/OE.25.010214. [DOI] [PubMed] [Google Scholar]
- 271.Masuda K, et al. Azo-polymer film twisted to form a helical surface relief by illumination with a circularly polarized Gaussian beam. Opt. Express. 2017;25:12499–12507. doi: 10.1364/OE.25.012499. [DOI] [PubMed] [Google Scholar]
- 272.Takahashi F, et al. Picosecond optical vortex pulse illumination forms a monocrystalline silicon needle. Sci. Rep. 2016;6:21738. doi: 10.1038/srep21738. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 273.Torner L, Torres JP, Carrasco S. Digital spiral imaging. Opt. Express. 2005;13:873–881. doi: 10.1364/OPEX.13.000873. [DOI] [PubMed] [Google Scholar]
- 274.Tan PS, et al. High-resolution wide-field standing-wave surface plasmon resonance fluorescence microscopy with optical vortices. Appl. Phys. Lett. 2010;97:241109. doi: 10.1063/1.3525173. [DOI] [Google Scholar]
- 275.Zhang CL, et al. Perfect optical vortex enhanced surface plasmon excitation for plasmonic structured illumination microscopy imaging. Appl. Phys. Lett. 2016;108:201601. doi: 10.1063/1.4948249. [DOI] [Google Scholar]
- 276.Xie XS, et al. Harnessing the point-spread function for high-resolution far-field optical microscopy. Phys. Rev. Lett. 2014;113:263901. doi: 10.1103/PhysRevLett.113.263901. [DOI] [PubMed] [Google Scholar]
- 277.Wei SB, et al. Sub-100nm resolution PSIM by utilizing modified optical vortices with fractional topological charges for precise phase shifting. Opt. Express. 2015;23:30143–30148. doi: 10.1364/OE.23.030143. [DOI] [PubMed] [Google Scholar]
- 278.Kozawa Y, Matsunaga D, Sato S. Superresolution imaging via superoscillation focusing of a radially polarized beam. Optica. 2018;5:86–92. doi: 10.1364/OPTICA.5.000086. [DOI] [Google Scholar]
- 279.Willig KI, et al. STED microscopy reveals that synaptotagmin remains clustered after synaptic vesicle exocytosis. Nature. 2006;440:935–939. doi: 10.1038/nature04592. [DOI] [PubMed] [Google Scholar]
- 280.Wang SB, Chan CT. Lateral optical force on chiral particles near a surface. Nat. Commun. 2014;5:3307. doi: 10.1038/ncomms4307. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 281.Brullot W, et al. Resolving enantiomers using the optical angular momentum of twisted light. Sci. Adv. 2016;2:e1501349. doi: 10.1126/sciadv.1501349. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 282.Zhao Y, et al. Chirality detection of enantiomers using twisted optical metamaterials. Nat. Commun. 2017;8:14180. doi: 10.1038/ncomms14180. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 283.Jeffries GDM, et al. Using polarization-shaped optical vortex traps for single-cell nanosurgery. Nano Lett. 2007;7:415–420. doi: 10.1021/nl0626784. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 284.Stellinga D, et al. An organic vortex laser. ACS Nano. 2018;12:2389–2394. doi: 10.1021/acsnano.7b07703. [DOI] [PubMed] [Google Scholar]
- 285.Lavery MPJ, et al. Detection of a spinning object using light’s orbital angular momentum. Science. 2013;341:537–540. doi: 10.1126/science.1239936. [DOI] [PubMed] [Google Scholar]
- 286.Cvijetic N, et al. Detecting lateral motion using light’s orbital angular momentum. Sci. Rep. 2015;5:15422. doi: 10.1038/srep15422. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 287.Kravets VG, et al. Singular phase nano-optics in plasmonic metamaterials for label-free single-molecule detection. Nat. Mater. 2013;12:304–309. doi: 10.1038/nmat3537. [DOI] [PubMed] [Google Scholar]
- 288.Xie GD, et al. Using a complex optical orbital-angular-momentum spectrum to measure object parameters. Opt. Lett. 2017;42:4482–4485. doi: 10.1364/OL.42.004482. [DOI] [PubMed] [Google Scholar]
- 289.Fu SY, Gao CQ. Influences of atmospheric turbulence effects on the orbital angular momentum spectra of vortex beams. Photonics Res. 2016;4:B1–B4. doi: 10.1364/PRJ.4.0000B1. [DOI] [Google Scholar]
- 290.Li Y, Yu L, Zhang YX. Influence of anisotropic turbulence on the orbital angular momentum modes of Hermite-Gaussian vortex beam in the ocean. Opt. Express. 2017;25:12203–12215. doi: 10.1364/OE.25.012203. [DOI] [PubMed] [Google Scholar]
- 291.Min CJ, et al. Plasmonic nano-slits assisted polarization selective detour phase meta-hologram. Laser Photonics Rev. 2016;10:978–985. doi: 10.1002/lpor.201600101. [DOI] [Google Scholar]
- 292.Xie ZW, et al. On-chip spin-controlled orbital angular momentum directional coupling. J. Phys. D Appl. Phys. 2017;51:014002. doi: 10.1088/1361-6463/aa9947. [DOI] [Google Scholar]
- 293.Zhang C, Ma L. Detecting the orbital angular momentum of electro-magnetic waves using virtual rotational antenna. Sci. Rep. 2017;7:4585. doi: 10.1038/s41598-017-04313-4. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 294.Mei ST, et al. On-chip discrimination of orbital angular momentum of light with plasmonic nanoslits. Nanoscale. 2016;8:2227–2233. doi: 10.1039/C5NR07374J. [DOI] [PubMed] [Google Scholar]
- 295.Foo G, Palacios DM, Swartzlander GA. Optical vortex coronagraph. Opt. Lett. 2005;30:3308–3310. doi: 10.1364/OL.30.003308. [DOI] [PubMed] [Google Scholar]
- 296.Lee JH, et al. Experimental verification of an optical vortex coronagraph. Phys. Rev. Lett. 2006;97:053901. doi: 10.1103/PhysRevLett.97.053901. [DOI] [PubMed] [Google Scholar]
- 297.Swartzlander GA, et al. Astronomical demonstration of an optical vortex coronagraph. Opt. Express. 2008;16:10200–10207. doi: 10.1364/OE.16.010200. [DOI] [PubMed] [Google Scholar]
- 298.Mawet D, et al. Annular groove phase mask coronagraph. Astrophys. J. 2005;633:1191–1200. doi: 10.1086/462409. [DOI] [Google Scholar]
- 299.Absil, O. et al. Three years of harvest with the vector vortex coronagraph in the thermal infrared. In Proc. SPIE 9908, Ground-based and Airborne Instrumentation for Astronomy VI. 99080Q (SPIE, Edinburgh, United Kingdom, 2016).
- 300.Aleksanyan A, Kravets N, Brasselet E. Multiple-star system adaptive vortex coronagraphy using a liquid crystal light valve. Phys. Rev. Lett. 2017;118:203902. doi: 10.1103/PhysRevLett.118.203902. [DOI] [PubMed] [Google Scholar]
- 301.Aleksanyan A, Brasselet E. High-charge and multiple-star vortex coronagraphy from stacked vector vortex phase masks. Opt. Lett. 2018;43:383–386. doi: 10.1364/OL.43.000383. [DOI] [PubMed] [Google Scholar]
- 302.Tamburini F, et al. Twisting of light around rotating black holes. Nat. Phys. 2011;7:195–197. doi: 10.1038/nphys1907. [DOI] [Google Scholar]
- 303.Battersby S. Twisting the light away. New Sci. 2004;182:36–40. [Google Scholar]
- 304.Yin JY, et al. Microwave vortex-beam emitter based on spoof surface plasmon polaritons. Laser Photonics Rev. 2018;12:1600316. doi: 10.1002/lpor.201600316. [DOI] [Google Scholar]
- 305.Marzo A, Caleap M, Drinkwater BW. Acoustic virtual vortices with tunable orbital angular momentum for trapping of Mie particles. Phys. Rev. Lett. 2018;120:044301. doi: 10.1103/PhysRevLett.120.044301. [DOI] [PubMed] [Google Scholar]
- 306.Bialynicki-Birula I, Charzyński S. Trapping and guiding bodies by gravitational waves endowed with angular momentum. Phys. Rev. Lett. 2018;121:171101. doi: 10.1103/PhysRevLett.121.171101. [DOI] [PubMed] [Google Scholar]