Evidence on two- and three-state models of the calcium regulation models of muscle contractions remain in favor of three-state models.
Abstract
In a recent JGP article, Heeley et al. (2019. J. Gen. Physiol. https://doi.org/10.1085/jgp.201812198) reopened the debate about two- versus three-state models of thin filament regulation. The authors review their work, which measures the rate constant of Pi release from myosin.ADP.Pi activated by actin or thin filaments under a variety of conditions. They conclude that their data can be described by a two-state model and raise doubts about the generally accepted three-state model as originally formulated by McKillop and Geeves (1993. Biophys. J. https://doi.org/10.1016/S0006-3495(93)81110-X). However, in the following article, we follow Plato’s dictum that “twice and thrice over, as they say, good it is to repeat and review what is good.” We have therefore reviewed the evidence for the three- and two-state models and present our view that the evidence is overwhelmingly in favor of three structural states of the thin filament, which regulate access of myosin to its binding sites on actin and, hence, muscle contractility.
Introduction
In a recent paper in the Journal of General Physiology, Heeley et al. (2019) argue that investigation of thin filament activation of myosin ATPase either by transient kinetic or by equilibrium binding studies can lead to conflicting models of muscle regulation. They proceed to provide evidence for such inconsistency derived from kinetic studies of Pi release from actin.myosin following ATP hydrolysis. From their analysis, they go on to propose that two thin filament activity states are sufficient to explain regulation of the process, in conflict with the widely accepted McKillop–Geeves three-state model (McKillop and Geeves, 1993).
Background
The argument over whether two or three regulatory states govern muscle contractile activity is reminiscent of similar differences discussed earlier in the Biophysical Journal in 2002 (Chalovich et al., 2002; Geeves and Lehrer, 2002) and evaluated further in 2012 (Geeves, 2012). Thus, the conflict between opposing interpretations of raw and modeled data is not a new one, and apparently differences have not been resolved. Nonetheless, understanding the molecular steps involved in controlling myosin motor activity on muscle thin filaments, i.e., the purpose of such experimentation and modeling, is of significant biomedical importance and deserves revisiting. Here, we outline the basis of the two models before critically evaluating key elements supporting the models.
It is generally accepted that activation of the muscle contractile machinery occurs in steps. Influx of calcium ions into the muscle sarcoplasm triggers the process but is insufficient to fully switch on the interaction of myosin and actin to result in force generation. Evidence that calcium itself is not enough to fully activate the thin filament, and that myosin is needed as a modulator (not just as an enzyme), came from seminal studies on the kinetics of actin-myosin ATPase (Weber and Murray, 1973; Lehrer and Morris, 1982). Conversely, removal of calcium, while triggering the process of relaxation, does not alone result in relaxation; in fact, relaxation lags considerably behind the fall in calcium concentration (Poggesi et al., 2005). This is usually interpreted as representing a slow decay in the number of cycling myosin cross-bridges that continues to maintain the thin filament in the on state, well after calcium has been removed. The on state is prolonged until a critical threshold number of actin-bound cross-bridges is passed, which then allows rapid complete relaxation.
The McKillop–Geeves three-state model
The work of McKillop and Geeves (1993) yielded a formal understanding of the two-step activation/relaxation process described above. By merging the steric-blocking model of regulation proposed by Hanson and Lowy (1964), Moore et al. (1970) and Huxley (1970) with earlier puzzling biochemistry on myosin binding to regulated and unregulated actin, McKillop and Geeves (1993) extended previous work into a more complete and understandable model. In its simplest heuristic formulation, the three-state hypothesis has proven invaluable in explaining data and formulating new experiments. However, conceptualizing the three-state model quantitatively can be difficult, since the states are in dynamic equilibrium and are biased in one direction or another by the effects of troponin (Tn) binding to actin.tropomyosin (Tpm), Ca2+ binding to Tn, myosin binding to actin.Tpm, and the catalysis of ATP hydrolysis by myosin, all compounded in their complexity by interdependent cooperative and allosteric effects (Geeves and Lehrer, 1994; Lehrer and Geeves, 1998; Mijailovich et al., 2012b). Still, while the McKillop and Geeves (1993) work itself is widely cited, it may not be as widely read or even understood implicitly in simplified form (Geeves, 2012; Lehman, 2017). This dissonance between general acceptance and overall assessment leaves the work exposed to challenge. In fact, the recent opinion piece in the journal (Heeley et al., 2019), as mentioned, questions the validity of the three-state model.
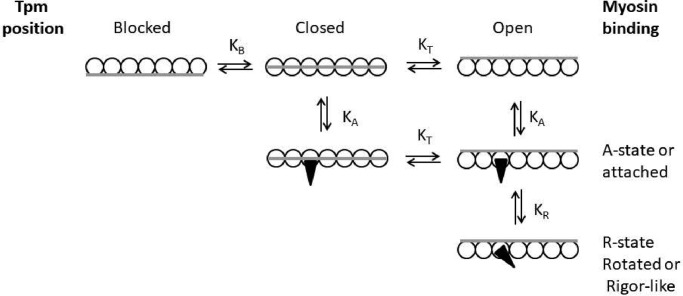
The question, as posed by Heeley et al. (2019), is itself somewhat ironic since the three-state model was originally proposed to account for discrepancies between equilibrium and transient kinetic myosin binding results which, even when conducted in the same laboratory with the same proteins, resulted in different, incompatible estimates of the fraction of the actin filament turned on or off in the presence and absence of calcium (McKillop and Geeves, 1993). To explain this discrepancy, three actin.Tpm structural states that sterically block and unblock myosin binding were proposed, whose occupancy quantitatively depended on calcium and myosin occupation (McKillop and Geeves, 1993; see Fig. 1 and Table 1 for details). The three states were originally termed blocked (B), closed (C), and open (O), but the names have altered slightly as the three states have been gradually become more connected to three structural states observed in x-ray fiber diffraction studies and electron microscope reconstructions (Holmes, 1995; Vibert et al., 1997). The B state is one in which the majority of the myosin binding site on actin is blocked by Tpm, and this predominates in the absence of calcium, although the other states are also present in the equilibrium mix of states. The Calcium-induced, Closed, or C state is one in which calcium removes the interaction of TnI with actin.Tpm and allows Tpm to revert to its most favorable binding site on actin, thereby exposing much but not all of the myosin binding site on actin. Note that the binding site for Tpm is not a fixed, well-defined site but a shallow free energy well that allows frequent thermally driven excursions of Tpm over the surface of actin, away from the shallow minima (Orzechowski et al., 2014; Kiani et al., 2019). In the McKillop and Geeves estimation, Tpm in a calcium-loaded thin filament spends ∼80% of its time in the C state. In the C state, myosin exhibits limited binding to actin, Tpm is in a position to block complete binding, and in some structural interpretations, Tpm would impede myosin cleft closure (Lorenz et al., 1995; Poole et al., 2006). To allow myosin to bind into the well-defined rigor conformation, Tpm needs to move further away from the B position to the myosin-induced (M) or O state. Whether Tpm accesses this site under its own thermal motion or is physically displaced by myosin binding remains an open debate. But in the McKillop–Geeves model, 20% of Tpm will be in the O state at saturating calcium in the absence of any myosin binding.
Figure 1.
Diagrammatic version of the three-state model as originally envisaged by McKillop and Geeves (1993). Tpm on a single strand of seven actin monomers can sit in one of three positions on the actin surface B, C, or O. In the B position, the major binding sites of myosin on actin are blocked by Tpm, and no significant binding of myosin is possible (weak electrostatic interaction may be possible). In the C position, myosin can bind to some of its binding site to form the relatively weakly Attached or A state, but rotation into the rigor-like state (R) is prevented by Tpm. More recent structural interpretations of the transition from A to R state would suggest that the A state is formed by the lower 50 kD domain of myosin binding to actin. The R state requires closure of the cleft between the upper and lower 50 kD domains (linked to switch one opening), allowing the upper 50 kD to access its binding site on actin. In the C state of the thin filament, the position of Tpm would sit between the upper and lower 50 kD domains forming a molecular gag preventing cleft closure. See Table 1 for the occupancy of the different states under different conditions. The term Ki refers to the equilibrium constants for each step of the scheme defined in the left to right or top down direction.
Table 1. Properties and occupancy of the three states in the McKillop and Geeves model as assayed by different methods.
| TF states | Blocked | Closed | Open | |
|---|---|---|---|---|
| Tpm position on actin | Outer domain | Inner/Outer domain | Inner domain | |
| ATPase/activity | Off/low | Off/low | On/high | |
| Myosin binding | Transient kinetic | Off | On | On |
| Equilibrium | Off | Off | On | |
| ActinTpm occupancy | −Tn | 0 | 0.8 | 0.2 |
| +Tn −Ca | 0.7 | 0.25 | 0.05 | |
| +Tn +Ca | 0 | 0.8 | 0.3 | |
| +Myosin (1:1 ratio of myosin:actin) (+/−Tn, +/−Ca) | 0 | 0 | 1.0 |
Tpm position as defined in EM and x-ray fiber diffraction data. Inner and outer refer to the position of Tpm on the inner subdomains (3 and 4) or outer subdomains (1 and 2) of actin. Myosin binding refers to measurements of myosin binding to actin following rapid mixing of myosin S1 with thin filaments (kinetic) or in titration studies where myosin S1 is titrated slowly into a solution of thin filaments (equilibrium). In both cases the binding can be followed using the fluorescence of a pyrene label attached to actin. Actin-Tpm occupancy is the interpretation of the occupancy of the three actin.Tpm.Tn states from the myosin binding studies.
Table 1 sets out the fractional occupation of actin sites in the three states under a variety of conditions. Note, however, that this simple outline neglects the degree of cooperativity between the various players, i.e., calcium with TnC; TnC with TnI; TnI with actin; Tpm with myosin; and actin with Tpm. In addition, the calcium-TnC complex and actin compete with each other for TnI binding, while myosin and TnI compete with each other for actin.Tpm. Thus, each of the players is connected to all of the others directly or indirectly through TnI. Estimates of the cooperativity in the system suggest that TnI and one strongly bound myosin control seven actin sites (the size of a single Tpm). A single TnI binding to actin.Tpm will put seven actin binding sites into the B state, while a single strongly bound myosin anywhere in the vicinity will displace one TnI from actin.Tpm and put seven actin sites into the O or M state. If TnI has already been displaced by calcium binding, then a single myosin binding to an actin.Tpm will put up to fourteen actin sites into the M state.
The Heeley et al. (2019) two-state model
The Heeley et al. (2019) review of their previously published work dealt with measurement of the rate of Pi release from the myosin cross-bridge once rapidly mixed with an excess of actin (either pure actin or Tn-Tpm regulated thin filaments). In these data, Pi release is accelerated by actin, and the degree of acceleration is a function of the presence of calcium and tightly bound myosin heads. This is in agreement with the widely held view that calcium alone is insufficient to fully activate the thin filament. Crucially, however, the data of Heeley et al. (2019) show that strongly bound myosin heads alone are also unable to fully activate the thin filament, and this observation contradicts expectations of the three-state model as well as earlier biochemistry. Such a surprising observation deserves careful evaluation to determine if, indeed, the Pi release data do undermine the three-state model.
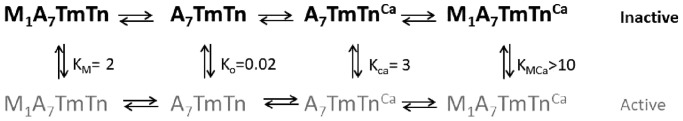
Heeley et al. (2019) go on to propose that the thin filaments regulate Pi release directly but not by controlling access of myosin to its binding site on actin, as proposed in the original steric blocking model and incorporated into the three-state model. This revives arguments from the 1980s and 1990s of Eisenberg and Chalovich (Chalovich and Eisenberg, 1982; Chen et al., 2001), who also favored models in which Pi release was a regulated step in the myosin ATPase pathway, but at the time were formulated without detailed structural data available. Following up on the Eisenberg–Chalovich interpretation and with their own new kinetics, Heeley et al. (2019) present a two-state actin model in which actin notionally is either active, A (able to promote Pi release from myosin), or inactive, I (unable to promote Pi release). Both calcium and myosin can bind to both A and I actin sites and rebalance the A/I equilibrium of actin toward the A form. See Fig. 2 for the two-state model and Table 2 for a comparison of the occupancy of the states predicted in the Heeley and Eisenberg and Chalovich models.
Figure 2.
Two-state model of the thin filament based on Scheme 2 of Heeley et al. (2019). In this model, the thin filament has two activity states, I and A. The linkage between structural transitions of thin filament complex and activity states are not detailed by Heeley et al. (2019). To avoid any assumptions about the structural transitions, the two activity states are shown as black and gray, respectively. The thin filament is predominantly in the I form in the absence of both calcium and myosin. The binding of either calcium (Ca2+) or a single strongly bound M will bias the system toward the A state, but neither is sufficient on its own to switch the system totally to the A form. See Table 2 for the fraction of the system on under different conditions.
Table 2. Properties and occupancy of the two activity states in the Heeley et al. (2019) two-state model.
| States | I | A | |
|---|---|---|---|
| ATPase | Low | High | |
| Occupancy | +Tn −Ca | 0.95 (0.95) | 0.05 (0.05) |
| +Tn +Ca | 0.25 (0.8) | 0.75 (0.2) | |
| +Myosin (ratio of myosin: actin, 1:7) | 0.33 (0) | 0.67 (1.0) |
The occupancy is based on the Keq values given in Scheme 2 of Heeley et al. (2019) where Keq is the equilibrium constant between the A and I states. The values in brackets are the occupancies predicted by the earlier two-state model of Hill et al. (1980).
While the two-state model of Heeley et al. (2019) incorporates many of the features of the earlier two-state models developed by Hill and Eisenberg (Hill et al., 1980) and developed further by Chalovich and Eisenberg (1982), the model as proposed here does not attempt to address well-established complexities of the cooperative nature of the interactions of calcium and myosin with the filament. The cooperativity of the thin filament was a core element of both the Hill two-state model and the McKillop–Geeves three-state model. Similarly the two-state model as presented by Heeley et al. (2019) does not address a structural basis for the regulatory mechanism. It is noteworthy that Heeley et al. (2019) have quite distinct estimates for the on (A) and off (I) states from those of the earlier Hill et al. (1980) model, and the reason for this discrepancy is not addressed (Table 2). In the Heeley et al. (2019) model, the thin filament is 95% in the I state 5% in the A state. Calcium or rigor bridges reduce the fraction in the I form but not to zero.
Discussion
Any analysis of contrasting kinetic models will inevitably concentrate on two major issues: the details of the experimental evidence, and the model proposed. We will focus first on the nature of the Heeley et al. (2019) two-state model since this is relatively straightforward. Evaluation of the experimental data of Heeley et al. (2019) is more nuanced, which may not be fully appreciated by a nonspecialist audience and requires explanation with greater attention to detail.
Two-state versus three-state models
Heeley et al. (2019) suggest that two thin filament activity states are sufficient to explain calcium regulation of the myosin-ATPase activity and thus muscle contraction. However, they do so without acknowledging the wider experimental support for three states or providing a structural basis for their proposed regulatory mechanism. In the following section, we will discuss the structural, physiological, and biochemical evidence for the three-state model. We contend that the experimental data in question can easily be incorporated in a standard three-state model with modestly adjusted equilibria.
The need for a three-state regulatory model becomes logically imperative once the accumulated evidence for three structural states of the thin filament is considered. Fiber-diffraction studies of Lorenz et al. (1995) showed that addition of calcium to intact fibers only partially activates the thin filament to produce a closed-state-like configuration of Tpm on actin that still, in fact, partially obstructs myosin binding. Further myosin binding is then necessary to fully activate the filament by producing the O state structure (Lorenz et al., 1995). EM reconstructions of Vibert et al. (1997) confirmed these results, and later Poole et al. (2006) consolidated results of these approaches to show a strict correspondence between the diffraction studies on fibers and the EM studies on isolated filaments. These structural studies, identifying static configurations presumed to be in the force generation pathway, are not mentioned by Heeley et al. (2019). More recently, Bershitsky et al. (2017) presented muscle fiber diffraction evidence for the O state in contracting muscle preparations—countering the argument that the open structure is an artifact of rigor-type cross-bridges binding actin, as might have been previously contemplated by Heeley et al. (2019).
Three identifiable structural states of Tpm on actin with two partially blocking myosin binding (Vibert et al., 1997; Poole et al., 2006) to actin are not obviously compatible with a two-activity-state model of the thin filament. More recently, significant progress has been made in defining the dynamics of Tpm movement on actin, which are broadly compatible with the Tpm being in an equilibrium mixture of at least two states under all conditions, except when thin filaments are saturated with myosin cross-bridges, as predicted in the three-state model (compare Geeves, 2012; Lehman, 2016). Such a view is supported by results of EM reconstructions of thin filaments preserved in the absence and presence of calcium (Pirani et al., 2005; Risi et al., 2017) and confirmed by assessment of energy landscape plots of Tpm transitions across actin (Orzechowski et al., 2014; Kiani et al., 2019).
Given the lack of identifiable structural support for the two-state model, it is difficult to understand and to formulate a convincing and detailed framework for how myosin binds to actin and then how Pi is released. Conceptualizing how Tn-Tpm then regulates the process is even more difficult without a structural context. These events are not a just simple single step binding phenomenon on actin or on regulated thin filaments. Instead, they involve a complex process of docking at the interface between the two proteins, not the least of which is the requirement for (1) the major cleft on myosin to close to form the full rigor like interface and (2) the docking with actin to be transmitted to the nucleotide pocket to trigger Pi release and the myosin power stroke on actin. These two steps in the actin-myosin cycle may be the same event or represent distinct phenomena. However, in the C, i.e., calcium-induced, position, Tpm appears to act as a molecular gag sitting between the jaws of the upper and lower 50 kD domains of myosin (Lorenz et al., 1995; Poole et al., 2006), incompatible with the closing of the myosin cleft, which means that the calcium-induced C state cannot be the same as either the B or O state.
In addition to the structural data just outlined, substantial evidence from mechanical studies of single muscle fibers also requires three thin filament states to fully explain corresponding data. Again, simple observation shows that both myosin cross-bridges and calcium are required to fully activate the thin filament not only in vitro but also in situ (Gordon et al., 2000). For example, physiological studies with muscle fibers equivalent to the biochemical studies of Weber and Murray (1973) produced similar force versus pCa curves with a comparable pCa50%—i.e., a midpoint usually referred to as the calcium sensitivity. This sensitivity is not just found to be an inherent property of the thin filament. It also depends upon the number of cross-bridges actively cycling on actin; thus, any treatment of the fibers with an agent (e.g., low ATP or high ADP concentrations) that increases the number of cross-bridges attached to actin in the steady-state will increase the calcium sensitivity (i.e., less calcium is required to activate the contraction) and vice versa for agents that reduce the number of cross-bridges (e.g., inhibitors of Pi release during ATP hydrolysis). Complementary calcium dependent kinetics of rigor-like myosin binding to thin filaments shows precisely the same calcium sensitivity (McKillop and Geeves, 1993; Boussouf et al., 2007a,b). Here, the rate of myosin binding to actin varies with calcium concentration, while the pCa50% parallels that expected for calcium affinity to TnC in the filament that can be altered by treatments that affect TnI or TnC behavior or, alternatively, by preloading the filament with small amounts of rigor myosin heads bound to actin.
These studies taken as a whole gave rise to a view of Ca2+ activation of thin filaments as a classic allosteric system (Lehrer and Geeves, 1998) in which myosin and TnI compete for binding to actin.Tpm. In the presence of ATP and low calcium, TnI dominates and the system is off (actinTpm in B state). In the presence of calcium and ATP, myosin can bind and the system is on (actinTpm in O state). Anything that changes the balance between the TnI and myosin competition will alter the calcium sensitivity. Mutations in myosin or Tn provide a way to explore the rebalancing in relative affinities.
An elegant example of this balance between TnI-induced inhibition and myosin-induced activation is a study in Drosophila flight muscle by Kronert et al. (1999) on mutations in muscle proteins: For example, a mutation in TnI (heldup2) disrupts the regulation of contraction and causes the muscle to be hypercontractile. This is consistent with the mutant TnI no longer being able to prevent myosin from binding to actin. A second mutation, D45, this time in myosin, showed a twofold weaker than normal affinity for actin was hypocontractile, but then in combination with heldup2 reverted to wild-type phenotype, i.e., the Drosophila could fly. Thus, the balance between TnI and myosin binding for actin was restored when both modulators had a similar reduction in affinity for actin.
The evidence for such an allosteric relationship between TnI and myosin accordingly is quite robust. Moreover, Mijailovich et al. (2012a) demonstrated that these studies can be modeled using the three-state paradigm. Calcium binding to TnC alters the equilibrium between B and C states with smaller effects on the equilibrium between O and C states. Other allosteric effectors operating via actin.Tpm.Tn or myosin can be readily incorporated into this computational system. Current versions of this modeling with three states can generate sarcomere force-pCa curves and twitch contractions under a variety of conditions (Campbell et al., 2018; Mijailovich et al., 2019a). In contrast, Mijailovich et al. (2012a) were unable to model the calcium dependence of myosin binding to thin filaments using two-state thin filament models without imposing unrealistic calcium dependencies on the model parameters. Heeley et al. (2019) have not presented any data on the calcium dependencies of their own parameters, but based on attempts to by Mijailovich et al. (2012a) to model the calcium dependency of related two-state models, this is unlikely to be successful.
Phosphate release
While the work of Heeley et al. (2019) does raise some important questions requiring serious appraisal, the authors appear to raise the three-state model as a straw man to be taken down without adequately addressing the entirety of the outstanding evidence. Their presentation relies on measurement of the rate of Pi release from M.ADP.Pi when the complex is rapidly mixed with actin or thin filaments. Fig. 3 of Heeley et al. (2019), documenting the cardiac system, indicates that the rate constant of Pi release can be increased from 0.49 s−1 in the absence of actin to 36 s−1 at high thin filament concentration when both rigor cross-bridges and calcium are present (a similar value was seen using pure actin). If just calcium is present, the acceleration is only to 27 s−1 or 24 s−1 for only rigor bridges present. This result is compatible with the view that both calcium and strong binding myosin bridges are required to achieve maximum acceleration of Pi release, a feature common to both two- and three-state models. However, the authors’ evidence that rigor bridges alone cannot fully activate the thin filament needs to be considered carefully, since it contradicts the original three-state regulatory scheme. Two factors complicate a simple interpretation of the effect of strong binding myosin rigor bridges: (1) Regulatory protein motions in each state of the three-state model should be considered as oscillating back and forth across low energy-barriers (Maytum et al., 2008; Orzechowski et al., 2014); and (2) the cooperativity of the system needs to be defined to allow an assessment of how many myosin bridges are required to bind an actin filament in order to fully activate the system. This latter point is important because in a cooperative system in solution, the myosins will tend to cluster at O state actin sites. This constraint is different in muscle fibers where filament geometry limits the number of myosin that can bind to each section of an actin filament.
We bear in mind that the experiments of Heeley et al. (2019) are technically challenging and to date have not been repeated by any other laboratory group. Their approach requires working at very low ionic strength and high actin concentrations (as do most steady-state ATPase assays). The low ionic strength used will affect the stability of electrostatic interactions between either Tpm or TnI and actin, and again between myosin and actin. As we have stated above, this can alter the balance between the cooperative competitive interactions of TnI and myosin with actin. In addition, the rates of individual steps in the ATPase cycle and the stability of the regulatory states of the thin filament can change at the very low salt concentrations required in their assays. The very high actin concentrations also can influence the homogeneity of proteins mixed in a highly viscous solution of actin and therefore the homogeneity of myosin binding to actin. As alluded to by Heeley et al. (2019), it is important to be careful about nonphysiological conditions. The requirement for high actin concentrations means that, in most cases, Heeley et al. (2019) were only able to use actin concentrations up to approximately twice the value of that predicted to produce a half maximal ATPase rate (the effective Km or KA for actin binding in the steady-state). This will lead to uncertainty in the extrapolated values for the maximum value of the Pi release rate constant. Such estimates are normally understood to require three to four times the effective Km for reliable estimates of the maximum rate constant. The lack of error analysis or the statistical significance of the differences in the values presented by Heeley et al. (2019) is also conspicuous.
In essence, evidence related by Heeley et al. (2019) is that Pi release can be activated 30% by calcium over that achieved by rigor cross-bridges (the approximately twofold effect is a little larger for the skeletal system). They assert that this is not predicted by the three-state model and is consistent with direct activation of the Pi release step. However, the twofold change is rather small on the scale of the changes in ATPase or Pi release rates normally noted during actin activation (up to 1,000-fold). We again note that measuring the maximum Pi release rate with high precision is challenging. In fact, thin filaments in the in vitro system examined by Heeley et al. (2019) may not be fully activated by rigor-like cross-bridges under their set of conditions, and the rigor bridge activation may thus be actually underestimated. Even if the Pi information provided is correct, a wholesale rejection of the three-state mode is not required or prudent. The perspective offered in McKillop and Geeves (1993) 25 yr ago was based on the best available evidence at the time. A small effect of calcium on the Pi release could be easily accommodated within the three-state model, but we doubt the two-state model proposed by Heeley et al. (2019) could easily account for the broader dataset discussed here.
Heeley et al. (2019) argue that the McKillop and Geeves data and modeling are flawed because the rigor-like cross-bridges in that study (i.e., using apo-myosin or with ADP, pyrophosphate, or other analogues bound) were fundamentally different in their interaction with thin filaments compared with typical nucleotide association in the steady-state of the ATPase cycle, either because of some intrinsic difference in the nature of the interaction or because of the transient nature of the interaction in the presence of ATP. Heeley et al. (2019) refer to rigor cross-bridge conformations as in a nonphysiological form. While this is hard to prove convincingly, it would require all of the evidence on structural states of the cross-bridge based on crystallography or cryo-EM to be similarly dismissed as nonphysiological.
Finally, we consider a principal problem with the Heeley et al. (2019), two-state model to be the lack of correlation with any obviously defined or predictable structural states, other than nebulously referenced A and I conformations. Understandably, kinetic data alone cannot provide unambiguous structures or conformations. That means that any possible substates in this model due to the effects of calcium and myosin binding remain undefined structurally, making the two-state model appear to be a semantic artifact. In our opinion, lacking obvious structural correlates, the two-state hypothesis has little apparent predictive power. In contrast, defining three structural states of actin.Tpm and in actin.TpmTn, whose equilibrium is affected by calcium, myosin, and other factors (ionic milieu, temperature, pH, etc.), provides a malleable model that has stood the test of time. Unlike the two-state hypothesis, the more pliable three-state model continues to evolve to incorporate ancillary regulatory effects of myosin-binding protein C (Mun et al., 2014), nebulin, and titin (Mijailovich et al., 2019b) as well as adjust to separate modulation of thick filament responsiveness (Lehman, 1978; Irving, 2017).
Conclusion
The three-state model as formulated by McKillop and Geeves in 1993 is not the last word on how muscle contraction is regulated by calcium. Indeed, the model has undergone several revisions since first proposed. These include the following: (1) the size of the cooperative unit differing for the B to C and C to M transitions (Geeves and Lehrer, 1994); (2) the incorporation of a worm-like-chain model of Tpm on the surface of actin (Smith and Geeves, 2003); and (3) the addition of a fourth state, a substate of the O or M state with myosin bound in the absence of calcium, implicated in hypertrophic cardiomyopathy (Lehrer and Geeves, 2014). Each of these has required significant adaptations of the original model. Future modeling will need to include the influence of myosin binding protein C on thin filament activation (Mun et al., 2014; Irving, 2017). The ability of the model to adapt to such new experimental data or ways of thinking is a tribute to the utility of the original formulation of the basic model. Recent work on the roles of thick filament strain and myosin binding protein C in the activation of contraction will require further developments to overall models of how contraction is regulated. As always, new evidence needs to be carefully evaluated to understand if it truly is a paradigm shift in how we understand the mechanism of regulation or just more precise data that require a new tweak to a well-defined system.
Acknowledgments
Henk L. Granzier served as editor.
W. Lehman is supported by National Institutes of Health grant RO1H036153.
The authors declare no competing financial interests.
References
- Bershitsky S.Y., Koubassova N.A., Ferenczi M.A., Kopylova G.V., Narayanan T., and Tsaturyan A.K.. 2017. The Closed State of the Thin Filament Is Not Occupied in Fully Activated Skeletal Muscle. Biophys. J. 112:1455–1461. 10.1016/j.bpj.2017.02.017 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Boussouf S.E., Agianian B., Bullard B., and Geeves M.A.. 2007a The regulation of myosin binding to actin filaments by Lethocerus troponin. J. Mol. Biol. 373:587–598. 10.1016/j.jmb.2007.07.066 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Boussouf S.E., Maytum R., Jaquet K., and Geeves M.A.. 2007b Role of tropomyosin isoforms in the calcium sensitivity of striated muscle thin filaments. J. Muscle Res. Cell Motil. 28:49–58. 10.1007/s10974-007-9103-z [DOI] [PubMed] [Google Scholar]
- Campbell K.S., Janssen P.M.L., and Campbell S.G.. 2018. Force-Dependent Recruitment from the Myosin Off State Contributes to Length-Dependent Activation. Biophys. J. 115:543–553. 10.1016/j.bpj.2018.07.006 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Chalovich J.M., and Eisenberg E.. 1982. Inhibition of actomyosin ATPase activity by troponin-tropomyosin without blocking the binding of myosin to actin. J. Biol. Chem. 257:2432–2437 http://www.ncbi.nlm.nih.gov/pubmed/6460759. [PMC free article] [PubMed] [Google Scholar]
- Chalovich J.M., Yan B., Brenner B., and Chen Y.-D.. 2002. Response to the Letter by Geeves and Lehrer. Biophys. J. 82:1679–1681. 10.1016/S0006-3495(02)75519-7 [DOI] [Google Scholar]
- Chen Y., Yan B., Chalovich J.M., and Brenner B.. 2001. Theoretical kinetic studies of models for binding myosin subfragment-1 to regulated actin: Hill model versus Geeves model. Biophys. J. 80:2338–2349. 10.1016/S0006-3495(01)76204-2 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Geeves M.A. 2012. 4.13 Thin Filament Regulation. In Comprehensive Biophysics. Vol. Vol. 4 Goldmann Y.E., and Ostap E.M., editors. Elsevier; 251–267. [Google Scholar]
- Geeves M.A., and Lehrer S.S.. 1994. Dynamics of the muscle thin filament regulatory switch: the size of the cooperative unit. Biophys. J. 67:273–282. 10.1016/S0006-3495(94)80478-3 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Geeves M.A., and Lehrer S.S.. 2002. Modeling thin filament cooperativity. Biophys. J. 82:1677–1681. 10.1016/S0006-3495(02)75518-5 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Gordon A.M., Homsher E., and Regnier M.. 2000. Regulation of contraction in striated muscle. Physiol. Rev. 80:853–924 http://www.ncbi.nlm.nih.gov/pubmed/10747208. 10.1152/physrev.2000.80.2.853 [DOI] [PubMed] [Google Scholar]
- Hanson J., and Lowy J.. 1964. The structure of actin filaments and the origin of the axial periodicity in the I-substance of vertebrate striated muscle. Proc. R. Soc. Lond. B Biol. Sci. 160:449–460. 10.1098/rspb.1964.0055 [DOI] [PubMed] [Google Scholar]
- Heeley D.H., White H.D., and Taylor E.W.. 2019. Investigation into the mechanism of thin filament regulation by transient kinetics and equilibrium binding: Is there a conflict? J. Gen. Physiol. 151:628–634. 10.1085/jgp.201812198 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Hill T.L., Eisenberg E., and Greene L.. 1980. Theoretical model for the cooperative equilibrium binding of myosin subfragment 1 to the actin-troponin-tropomyosin complex. Proc. Natl. Acad. Sci. USA. 77:3186–3190 http://www.ncbi.nlm.nih.gov/pmc/articles/PMC349579/pdf/pnas00493-0128.pdf. 10.1073/pnas.77.6.3186 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Holmes K.C. 1995. The actomyosin interaction and its control by tropomyosin. Biophys. J. 68(suppl 4):2S–5S, discussion:6S–7S. [PubMed] [Google Scholar]
- Huxley H.E. “ Structural changes in muscle and muscle proteins during contraction,” in Proceedings of the 8th International Congress of Biochemistry, Interlaken, edited byGregory J. G. (American Society of Biological Chemists, Rockville MD, 1970), Vol. 4, p. 23. [Google Scholar]
- Irving M. 2017. Regulation of Contraction by the Thick Filaments in Skeletal Muscle. Biophys. J. 113:2579–2594. 10.1016/j.bpj.2017.09.037 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Kiani F.A., Lehman W., Fischer S., and Rynkiewicz M.J.. 2019. Spontaneous transitions of actin-bound tropomyosin toward blocked and closed states. J. Gen. Physiol. 151:4–8. 10.1085/jgp.201812188 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Kronert W.A., Acebes A., Ferrús A., and Bernstein S.I.. 1999. Specific myosin heavy chain mutations suppress troponin I defects in Drosophila muscles. J. Cell Biol. 144:989–1000. 10.1083/jcb.144.5.989 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Lehman W. 1978. Thick-filament-linked calcium regulation in vertebrate striated muscle. Nature. 274:80–81. 10.1038/274080a0 [DOI] [PubMed] [Google Scholar]
- Lehman W. 2016. Thin Filament Structure and the Steric Blocking Model. In Comprehensive Physiology. John Wiley & Sons, Inc., Hoboken, NJ: 1043–1069. 10.1002/cphy.c150030 [DOI] [PubMed] [Google Scholar]
- Lehman W. 2017. Switching Muscles On and Off in Steps: The McKillop-Geeves Three-State Model of Muscle Regulation. Biophys. J. 112:2459–2466. 10.1016/j.bpj.2017.04.053 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Lehrer S.S., and Geeves M.A.. 1998. The muscle thin filament as a classical cooperative/allosteric regulatory system. J. Mol. Biol. 277:1081–1089. 10.1006/jmbi.1998.1654 [DOI] [PubMed] [Google Scholar]
- Lehrer S.S., and Geeves M.A.. 2014. The myosin-activated thin filament regulatory state, M−-open: a link to hypertrophic cardiomyopathy (HCM). J. Muscle Res. Cell Motil. 35:153–160. 10.1007/s10974-014-9383-z [DOI] [PubMed] [Google Scholar]
- Lehrer S.S., and Morris E.P.. 1982. Dual effects of tropomyosin and troponin-tropomyosin on actomyosin subfragment 1 ATPase. J. Biol. Chem. 257:8073–8080 http://www.ncbi.nlm.nih.gov/pubmed/6123507. [PubMed] [Google Scholar]
- Lorenz M., Poole K.J., Popp D., Rosenbaum G., and Holmes K.C.. 1995. An Atomic Model of the Unregulated Thin Filament Obtained by X-Ray Fiber Diffraction on Oriented Actin-Tropomyosin Gels. J. Mol. Biol . 246:108–119. http://www.sciencedirect.com/science/article/B6WK7-45S98CR-60/2/02c17dd72f2a075e0aafcc47b9dcaa91. [DOI] [PubMed] [Google Scholar]
- Maytum R., Hatch V., Konrad M., Lehman W., and Geeves M.A.. 2008. Ultra short yeast tropomyosins show novel myosin regulation. J. Biol. Chem. 283:1902–1910. 10.1074/jbc.M708593200 [DOI] [PubMed] [Google Scholar]
- McKillop D.F., and Geeves M.A.. 1993. Regulation of the interaction between actin and myosin subfragment 1: evidence for three states of the thin filament. Biophys. J. 65:693–701. 10.1016/S0006-3495(93)81110-X [DOI] [PMC free article] [PubMed] [Google Scholar]
- Mijailovich S.M., Kayser-Herold O., Li X., Griffiths H., and Geeves M.A.. 2012b Cooperative regulation of myosin-S1 binding to actin filaments by a continuous flexible Tm-Tn chain. Eur. Biophys. J. 41:1015–1032. 10.1007/s00249-012-0859-8 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Mijailovich S.M., Li X., Griffiths R.H., and Geeves M.A.. 2012a The Hill model for binding myosin S1 to regulated actin is not equivalent to the McKillop-Geeves model. J. Mol. Biol. 417:112–128. 10.1016/j.jmb.2012.01.011 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Mijailovich S.M., Prodanovic M., Vasovic L., Stojanovic B., Maric M., Prodanovic D., Powers J.D., Davis J., Geeves M.A., and Regnier M.. 2019a Modulation of Calcium Sensitivity and Twitch Contractions in Cardiac Muscle with Troponin-C Mutations: Simulations and Experiments. Biophys. J. 116:116a 10.1016/j.bpj.2018.11.654 [DOI] [Google Scholar]
- Mijailovich S.M., Stojanovic B., Nedic D., Svicevic M., Geeves M.A., Irving T.C., and Granzier H.L.. 2019b Nebulin and titin modulate cross-bridge cycling and length-dependent calcium sensitivity. J. Gen. Physiol. 151:680–704. 10.1085/jgp.201812165 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Moore P.B., Huxley H.E., and DeRosier D.J.. 1970. Three-dimensional reconstruction of F-actin, thin filaments and decorated thin filaments. J. Mol. Biol. 50:279–295. 10.1016/0022-2836(70)90192-0 [DOI] [PubMed] [Google Scholar]
- Mun J.Y., Previs M.J., Yu H.Y., Gulick J., Tobacman L.S., Beck Previs S., Robbins J., Warshaw D.M., and Craig R.. 2014. Myosin-binding protein C displaces tropomyosin to activate cardiac thin filaments and governs their speed by an independent mechanism. Proc. Natl. Acad. Sci. USA. 111:2170–2175. 10.1073/pnas.1316001111 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Orzechowski M., Fischer S., Moore J.R., Lehman W., and Farman G.P.. 2014. Energy landscapes reveal the myopathic effects of tropomyosin mutations. Arch. Biochem. Biophys. 564:89–99. 10.1016/j.abb.2014.09.007 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Pirani A., Xu C., Hatch V., Craig R., Tobacman L.S., and Lehman W.. 2005. Single particle analysis of relaxed and activated muscle thin filaments. J. Mol. Biol. 346:761–772. 10.1016/j.jmb.2004.12.013 [DOI] [PubMed] [Google Scholar]
- Poggesi C., Tesi C., and Stehle R.. 2005. Sarcomeric determinants of striated muscle relaxation kinetics. Pflugers Arch. 449:505–517. 10.1007/s00424-004-1363-5 [DOI] [PubMed] [Google Scholar]
- Poole K.J., Lorenz M., Evans G., Rosenbaum G., Pirani A., Craig R., Tobacman L.S., Lehman W., and Holmes K.C.. 2006. A comparison of muscle thin filament models obtained from electron microscopy reconstructions and low-angle X-ray fibre diagrams from non-overlap muscle. J. Struct. Biol. 155:273–284. 10.1016/j.jsb.2006.02.020 [DOI] [PubMed] [Google Scholar]
- Risi C., Eisner J., Belknap B., Heeley D.H., White H.D., Schröder G.F., and Galkin V.E.. 2017. Ca2+-induced movement of tropomyosin on native cardiac thin filaments revealed by cryoelectron microscopy. Proc. Natl. Acad. Sci. USA. 114:6782–6787. 10.1073/pnas.1700868114 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Smith D.A., and Geeves M.A.. 2003. Cooperative regulation of myosin-actin interactions by a continuous flexible chain II: actin-tropomyosin-troponin and regulation by calcium. Biophys. J. 84:3168–3180. 10.1016/S0006-3495(03)70041-1 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Vibert P., Craig R., and Lehman W.. 1997. Steric-model for activation of muscle thin filaments. J. Mol. Biol. 266:8–14. 10.1006/jmbi.1996.0800 [DOI] [PubMed] [Google Scholar]
- Weber A., and Murray J.M.. 1973. Molecular control mechanisms in muscle contraction. Physiol. Rev. 53:612–673. 10.1152/physrev.1973.53.3.612 [DOI] [PubMed] [Google Scholar]