Skip to main content
PMC home page
Human Brain Mapping logoLink to Human Brain Mapping
. 2009 Jan 26;30(8):2453–2465. doi: 10.1002/hbm.20676

Differential force scaling of fine‐graded power grip force in the sensorimotor network

Birgit Keisker 1,✉, Marie‐Claude Hepp‐Reymond 2, Armin Blickenstorfer 1, Martin Meyer 1, Spyros S Kollias 1
PMCID: PMC6871245  PMID: 19172654

Abstract

Force scaling in the sensorimotor network during generation and control of static or dynamic grip force has been the subject of many investigations in monkeys and human subjects. In human, the relationship between BOLD signal in cortical and subcortical regions and force still remains controversial. With respect to grip force, the modulation of the BOLD signal has been mostly studied for forces often reaching high levels while little attention has been given to the low range for which electrophysiological neuronal correlates have been demonstrated. We thus conducted a whole‐brain fMRI study on the control of fine‐graded force in the low range, using a power grip and three force conditions in a block design. Participants generated on a dynamometer visually guided repetitive force pulses (ca. 0.5 Hz), reaching target forces of 10%, 20%, and 30% of maximum voluntary contraction. Regions of interest analysis disclosed activation in the entire cortical and subcortical sensorimotor network and significant force‐related modulation in several regions, including primary motor (M1) and somatosensory cortex, ventral premotor and inferior parietal areas, and cerebellum. The BOLD signal, however, increased monotonically with force only in contralateral M1 and ipsilateral anterior cerebellum. The remaining regions were activated with force in various nonlinear manners, suggesting that other factors such as visual input, attention, and muscle recruitment also modulate the BOLD signal in this visuomotor task. These findings demonstrate that various regions of the sensorimotor network participate differentially in the production and control of fine‐graded grip forces. Hum Brain Mapp 2009. © 2009 Wiley‐Liss, Inc.

Keywords: motor control, low force range, dynamic force pulses, motor cortex, visual feedback, fMRI

INTRODUCTION

The investigation of force scaling in primary motor cortex (M1) and other cortical and subcortical regions has started many decades ago with the pioneering contribution of Evarts [1968] who showed increased firing rate of M1 neurons during wrist flexion and extension opposing or assisting loads. This first study was followed by many others for wrist and elbow movements, precision grip, and reaching movements [Cheney and Fetz,1980; Conrad et al.,1977; Evarts et al.,1983; Georgopoulos et al.,1992; Hepp‐Reymond et al.,1978,1989; Riehle et al.,1994; Smith et al.,1975; Taira et al.,1996; Thach,1978]. The reported relationships between neuronal firing and force as well as rate of force change were linear, sigmoid, or even logarithmic [see Ashe,1997 for review]. During the control of fine‐graded isometric force in precision grip, corticospinal and even corticomotoneuronal cells in M1 were monotonically increasing, but some were also decreasing their firing rate with force [Maier et al.,1993]. Moreover, similarly responding neurons were also found in the dorsal and ventral premotor areas (PMv, PMd), in primary somatosensory cortex (S1), in the cerebellum, thalamus, and pallidum [Anner‐Baratti et al.,1986; Hepp‐Reymond et al.,1994,1999; Smith and Bourbonnais,1981; Wannier et al.,1991; Werner et al.,1991], indicating that force is generated and controlled by a widespread cortical and subcortical network.

Several neuroimaging studies in humans investigated the brain areas responsible for the generation and control of force and, particular to fMRI methodology, the relationship between BOLD signal changes and force [Boecker et al.,2005; Cramer et al.,2002; Dai et al.,2001; Dettmers et al.,1995,1996; Ehrsson et al.,2001; Kinoshita et al.,2000; Kuhtz‐Buschbeck et al.,2001,2008; Ludman et al.,1996; Muley et al.,2001; Peck et al.,2001; Pope et al.,2005; Schmitz et al.,2005; Spraker et al.,2007; Thickbroom et al.,1998,1999; Vaillancourt et al.,2003,2004,2007; Vaillancourt and Russell,2002; van Duinen et al.,2008; Wexler et al.,1997]. Some studies report monotonic activation increase, whereas others failed to find a relationship between BOLD signal and force. Several factors may account for these discrepancies. One potential reason relates to the question whether force was sustained for several seconds (static force) or whether repetitive force pulses (dynamic force) were exerted. Other important factors are the type of motor tasks or movements studied, e.g., whether or not they are guided by external cues (i.e., visual, auditory, and tactile) and whether force is exerted in simple finger tapping, or isometrically in precision or in power grip, or for lifting weights. Finally, the selected force range is of utmost importance. High force recruits many muscles in the arm and thus the resulting brain responses may not be specific to the grip itself.

With respect to isometric power grip force, the central control has been addressed in various studies (Begliomini et al.,2007; Cramer et al.,2002; Dai et al.,2001; Ehrsson et al.,2000; Floyer‐Lea and Matthews,2004,2005; Kuhtz‐Buschbeck et al.,2008; Liu et al.,2004), and three of them have also looked systematically at the correlates between BOLD signal and force. Dai et al. [2001], for static force ranging up to 80% maximum voluntary contraction (MVC), reported monotonic increase in volume and signal intensity in several primary and secondary motor areas. Cramer et al. [2002] during repetitive squeezing at 1 Hz, showed some relationship between the number of pixels and percent signal change with peak force in the sensorimotor cortex and supplementary motor area (SMA), but with a large interindividual variability. In the sensorimotor cortex only, the correlation coefficient reached the significance level because of the high number of activated pixels at high force (ca. 90% MVC). Kuhtz‐Buschbeck et al. [2008], comparing power and precision grip, tested the relation between the BOLD signal and force pulses within the low force range and found linearity in M1 and the cerebellum. However, their measurements included only two force levels.

So far, the data on force control in power grip suggest that the BOLD signal does not reflect as well dynamic force pulses as static force. They also imply that high force pulses recruit many hand and arm muscles, thus contributing to the larger volumes and signal intensity of BOLD activation. As neuronal correlates for low and fine‐graded forces have been revealed in conscious monkeys [Ashe,1997; Evarts et al.,1983; Hepp‐Reymond et al.,1989], we wondered whether a better modulation of the BOLD signal could occur for force pulses within a low range. In the clinic, simple and precise tools are used to quantify the recovery of function over time after a stroke, one important function being force in hand and fingers, and to understand the processes occurring during rehabilitation. We have thus decided to test force scaling in cortical and subcortical motor regions for force pulses in power grip within a low force range, using fMRI and a visuomotor paradigm. To measure force accurately, a special MR‐compatible device with optical measurement providing a high degree of reproducibility, even in the low force range, was used. The main objective was to find out whether the BOLD signal correlates with force, whether the force scaling is linear, and whether it behaves the same way in all the areas involved in generation and control of force.

MATERIALS AND METHODS

Participants

Fourteen healthy subjects (seven females, seven males, age range: 21–33 years) without any history of neurological or psychiatric disorder were recruited for this study. Hand dominance according to the Edinburgh‐handedness inventory [Oldfield,1971] showed right‐hand dominance. All participants gave their written consent, and the study was approved by the local ethics committee.

Force Measurement Device

The power grip was measured with a custom made MR‐compatible dynamometer developed by the Sensory‐Motor Systems Laboratory of ETH Zurich (http://www.mrsensor.ethz.ch/Fig. 1). It is based on the optical force measurement principle and consists of a plastic handgrip containing optical fibres that transmit laser signals to an interface box, which produces analogue and digital force outputs. The measured signal is a quasi‐linear function of the applied force. Multipoint calibration in the processing unit ensures good linearity and accuracy of the force sensor. The dynamometer was individually calibrated for each subject. It is easy to install into any experimental fMRI environment, easy to use, and can be synchronized with other recording processes.

Figure 1.

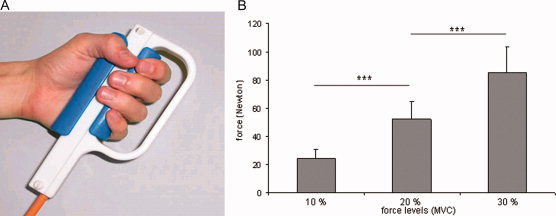
Figure 1

Open in a new tab

(A) MR‐compatible dynamometer. (B) Mean peak forces and standard deviations for the three force levels (14 subjects).

Activation Paradigm

Subjects were holding with the right‐hand the dynamometer shown in Figure 1 with the thumb opposing the four other fingers, i.e., in power grip. They were asked to generate isometrically repetitive visually guided force pulses at a rate of ca. 0.5 Hz, reaching target forces of 10%, 20%, and 30% of their MVC. Visual feedback was displayed on a screen in front of the subject. On a blue background, target and exerted forces were visualized as two grey concentric rectangles in which the colour of the inner square (exerted force) had to match the colour of the outer square (target force). The stronger the force was the darker was the grey. The task was practiced before the scanning procedure to ensure accurate execution. A block design with 21 s periods of rest alternating with 21 s for each force condition was used. The three force conditions were presented in a pseudorandom order (ABCCBAACBBACABC, with A = 10%, B = 20%, C = 30% MVC) and repeated five times. During the control condition, subjects had to fixate a small cross displayed in the middle of the blue screen to prevent eye movements. Before training, the MVC was assessed for each subject and the MR‐compatible handgrip was then accordingly calibrated. During scanning, the subjects hold their right arm in a comfortable slightly flexed position, at an angle of approximately 90° relative to their upper arm with the left arm positioned along the body. The output of the MR‐compatible handgrip was recorded simultaneously with the fMRI data acquisition.

fMRI‐Acquisition and Postprocessing

MRI was performed in a 3.0‐T MR system (Philips Medical Systems, Eindhoven, The Netherlands) equipped with an eight‐channel SENSE™ head coil. For functional imaging, a T2*‐weighted, single‐shot, fast field echo, EPI sequence of the whole brain (TR = 3000 ms, TE = 40 ms, flip angle = 82°, FOV = 220 mm × 220 mm, acquisition matrix = 128 × 128, in plane resolution = 1.7 mm × 1.7 mm, slice thickness = 3 mm, slice gap = 0, slices = 39) with a SENSE factor 2 was used (Pruessmann et al.,1999). Anatomical reference images of the whole brain were acquired at the end of the imaging session using a 3D, T1‐weighted, field echo sequence (TR = 20 ms, TE = 2.3 ms, flip angle = 20°, in plane resolution = 0.9 mm × 0.9 mm, slice thickness = 0.75 mm, 210 slices). These slices were transformed to iso‐voxel size (1 mm × 1 mm × 1 mm), and to Talairach space [Talairach and Tournoux,1988].

Postprocessing and data analysis were performed with the Brain Voyager QX 1.8 software package (Brain Innovation, Maastricht, The Netherlands). To remove unwanted signal components, data preprocessing was done for each subject before the computation of group analyses. Thereby, the standard parameters implemented in Brain Voyager QX 1.8 were adopted to diminish arbitrary selection of preprocessing parameters. Images were 3D motion corrected by means of trilinear interpolation. Spatial smoothing was performed by applying a Gaussian filter of 4 mm FWHM, to allow for the integration of signals in an area of less than a centimeter. Within this range, smoothing merely reduces the noise by simultaneously enhancing the signal. Temporal smoothing included linear trend removal and high pass filter (limited to three cycles). Before group analysis, functional volumes were automatically coregistered to the individual three‐dimensional structural scans and transformed into Talairach space [Talairach and Tournoux,1988].

Data Analysis

For the single subject analysis, the stimulation condition was modeled using a general linear model (GLM) convolved with the standard two‐gamma haemodynamic response functions resulting in t‐contrast maps corrected for multiple comparisons with q(FDR) ≤ 0.01 showing the contrast force (e.g., 10%, 20%, and 30% MVC) versus a resting condition. False discovery rate (FDR) is a recent development in statistical hypothesis testing to control the Type I error (rejection of a true null hypothesis). FDR has a higher power than Bonferroni correction as the threshold varies automatically across subjects with consequent gain in sensitivity. The parameter q has the advantageous feature of being comparable across studies. The correction accounts for cluster size, i.e., the bigger the cluster the more unlikely are nonrandom activations hence a lesser correction is accounted for (see Genovese et al.,2002).

For the group analysis, the significance level was set at qFDR ≤ 0.01. A two‐step analysis was performed on the basis of the linear model. A random effect analysis was performed using the multistudy option of the analysis software to detect the brain regions involved in the visuomotor task. For each force level (10%, 20%, and 30% MVC) group activations were compared to rest. The whole‐brain analysis identified regions responding more strongly to the generation of the three forces than to baseline. The statistical threshold for the whole‐brain analysis was t = 4.5 (qFDR ≤ 0.01 corrected).

After the group analysis, we also performed a post hoc region of interest (ROI) analysis, which enables to test whether BOLD responses obtained from distinct regions of the visuomotor network may vary as a function of force. ROIs were defined based on the whole‐brain activation patterns obtained from the contrast between all three forces summed together (10% +20% +30% MVC) and rest, with a statistical threshold of t = 4.5 (qFDR ≤ 0.01 corrected). Peaks of activation in each region were identified and significant voxels surrounding those peaks were selected and defined as ROIs (5 mm × 5 mm × 5 mm). For each ROI, group statistical analysis was performed by using the GLM option in the analysis software and for each force level and each participant beta values were obtained. These beta values were then subjected to analysis of variances (ANOVAs) with factors region and force (see Results section).

Behavioural Data Analysis

To assess the motor performance, four parameters were computed: peak force per block and force level (measured for each force pulse from the baseline to the maximal force), integrated sum of exerted force (surface under the force traces), number of force pulses per block, and the first derivative of the force (dF/dt measured for each force pulse). The obtained measures were compared and checked for variance.

RESULTS

Behavioural Results

The analysis revealed that for the three parameters, peak force, integrated force, and number of pulses per block, the values of the five blocks were comparable despite the pseudorandom presentation order. The most reproducible force parameter chosen for comparison with the functional data was the mean peak force. The correlation between the first derivative of force dF/dt and the mean peak force assessed by the Pearson's correlation coefficient was also highly significant (r = 0.851, P ≤ 0.01). To assess the intraindividual variance of subjects' force production, the peak force of each pulse per block and force level was measured. These data were subjected to a one‐way ANOVA which showed that the intraindividual variance reached significance more frequently in the lower force range (10% and 20% MVC) than in the 30% MVC range.

To check for significant differences between the three force levels, the mean peak forces per block and force level for all the subjects (15 values for 13 individuals and 14 for one) were subjected to another one‐way ANOVA. The differences between the conditions were highly significant (F 2,206) = 363.73, P ≤ 0.000. These findings indicate that the three mean peak forces did not overlap and that the inter‐ and intraindividual variability had no significant influence.

To investigate whether the frequency of the force pulses may influence the haemodynamic response, we assessed the number of pulses per block and force condition in each subject. In most subjects the variability of the number of pulses per force level was minimal. However, changes in the force pulses frequency occurred between different force levels and, in most subjects (11 of 14), a negative relationship between the mean number of pulses and mean peak force was observed. Two subjects had equal mean frequency values for 20% and 30% MVC, and the last subject had the highest pulse number at the highest force level.

The integrated sum of exerted force behaved in a very similar way as the peak force but was more variable, and for this reason was discarded from further analysis.

fMRI Results

Whole‐brain analysis

The three forces (10%, 20%, and 30% MVC) elicited overlapping BOLD activations in a widespread sensorimotor network, including bilateral frontal and parietal cortical areas and subcortically, the cerebellum and basal ganglia (statistical threshold t = 4.5, qFDR ≤ 0.01 corrected). Significant frontal activations were found contralaterally in M1, bilaterally in the supplementary and cingulate motor areas (SMA and CMA, respectively), and in (PMv), and ipsilaterally in the anterior insula. Parietal activations revealed three significant foci, namely one in the contralateral (S1) and two in the ipsilateral inferior parietal lobule (IPL). Subcortical activations included the ipsilateral pallidum (GP) and the cerebellum (CB), ipsilaterally in its anterior‐medial part [Larsell's lobule III, IV, Larsell and Jansen,1972] and bilaterally in the posterior hemisphere (lobule VI, VII). The coordinates of the centres of gravity, the average t values and the volumes of the activated regions are listed in Table I and the areas are illustrated in Figure 2.

Table I.

Mean t values, coordinates of the centres of gravity (Talairach and Tournoux,1988), and volumes of activated tissue of the regions with significant activation (t = 4.5, FDR 0.01 corrected) for the contrasts 10% + 20% + 30% MVC versus baseline in 14 subjects

Anatomical region Left hemisphere Right hemisphere
T value x y z Volume T value x y z Volume
M1 7.04 −36 −25 54 6545
S1 5.97 −46 −30 48 6424
SMA 5.82 1 −6 57 1792
CMA 5.02 6 7 50 362
PMv 5.02 −51 3 32 405 6.09 50 4 37 1945
PMv 5.35 −43 2 11 679 5.53 53 8 13 2434
Pallidum 5.24 21 3 10 337
Anterior insula 5.12 35 17 10 300
IPL 6.15 49 −36 44 2945
IPL 6.13 35 −45 42 1862
Anterior CB 6.53 17 −50 −18 3703
Posterior CB 6.02 −31 −59 −21 3259 11.9 28 −58 −18 2762
Open in a new tab

M1, primary motor cortex; S1, primary somatosensory cortex; SMA, supplementary motor area; CMA, cingulate motor area; PMv, ventral premotor cortex; IPL, inferior parietal lobe; CB, cerebellum.

Figure 2.

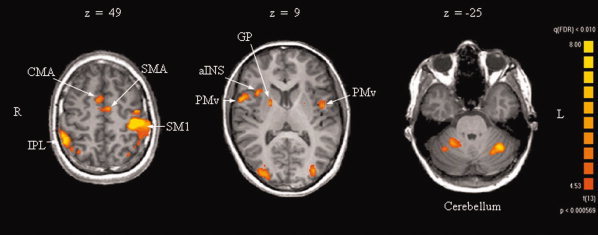
Figure 2

Open in a new tab

Transversal sections showing activation patterns obtained for the three forces versus baseline. M1: primary motor cortex, S1: primary somatosensory cortex, SMA: supplementary motor area, CMA: cingulate motor area, aINS: anterior insula, PMv: ventral premotor cortex, IPL: inferior parietal lobe, GP: pallidum.

ROI analysis (group analysis)

Fifteen ROIs were functionally identified in the two hemispheres (see Methods section). The beta values obtained from the ROI analysis were subjected to a two‐way ANOVA with factors force × ROI (3 × 15) that revealed a main effect of force (F 1,2) = 8.64, P ≤ 0.005, a main effect of ROI (F 1,14) = 10.18, P ≤ 0.000, and interaction of ROI and force (F 2,28) = 4.4, P ≤ 0.000. On the basis of these data, we performed a separate one‐way ANOVA for each of the 15 ROIs. A main effect of force was found in nine ROIs, namely contralateral M1 and S1, bilateral PMv, right IPL (2 foci), right anterior and bilateral posterior CB. Talairach and Tournoux [1988] coordinates of the activation peaks, maximum t values, means and standard errors (in parentheses) of the beta values for these nine ROIs are listed in Table II.

Table II.

Significant (t = 4.5, FDR 0.01 corrected) activation foci for the three forces versus rest obtained in the ROI analysis

Anatomical region Side x y z T value 10 % MVC 20% MVC 30% MVC
M1 L −36 −25 55 20.2 6.0 (0.7) 6.8 (0.6) 8.9 (0.8)
S1 L −45 −22 49 12 4.1 (0.5) 4.2 (0.5) 5.7 (0.6)
PMv L −42 −4 13 9.2 3.7 (0.5) 2.7 (0.4) 3.6 (0.6)
PMv R 57 8 8 8.4 4.9 (0.9) 3.2 (0.7) 5.5 (1.4)
IPL R 45 −40 46 10.4 3.5 (0.4) 2.9 (0.5) 4.2 (0.4)
IPL R 36 −40 40 12.2 3.0 (0.4) 2.4 (0.3) 3.6 (0.4)
Anterior CB R 11 −52 −17 12.9 3.4 (0.5) 4.3 (0.6) 6.1 (0.6)
Posterior CB L −36 −52 −23 9.6 4.3 (0.8) 4.2 (0.7) 5.4 (0.9)
Posterior CB R 30 −67 −17 13.4 5.5 (0.7) 5.6 (0.7) 6.6 (0.6)
Open in a new tab

Talairach and Tournoux (1988) coordinates of the activation peaks, the maximum t values, the mean and standard error (in parentheses) of the beta weights for each ROI and force are depicted. Abbreviations: see Table I.

To determine the relationship between the BOLD signal and force within these distinct regions, the beta values obtained for the three forces were then tested against each other with paired sample t test (Table III).

Table III.

Comparison between force levels

Anatomical region Side 20% vs. 10% 30% vs. 20% 30% vs. 10%
M1 L P ≤ 0.04 P ≤ 0.001 P ≤ 0.0001
S1 L ns P ≤ 0.001 P ≤ 0.0001
PMv L P ≤ 0.05 P ≤ 0.06 ns
PMv R P ≤ 0.05 ns ns
IPL R ns P ≤ 0.001 ns
IPL R ns P ≤ 0.005 P ≤ 0.04
ant CB R P ≤ 0.06 P ≤ 0.001 P ≤ 0.0001
post CB L ns P ≤ 0.06 P ≤ 0.005
post CB R ns P ≤ 0.05 P ≤ 0.004
Open in a new tab

Significant difference of beta weights for each ROI in the paired sample t test. Abbreviations: see Table I.

Linear and Nonlinear Correlation Between BOLD Signal and Force

Figure 3 displays for eight ROIs the mean beta values obtained for each force level (10%, 20%, and 30% MVC) averaged over the 14 subjects. As one can see (Fig. 3A), task‐related activation in M1 increased linearly as a function of increasing grip strength. The beta values obtained for the three forces reached the highest values compared with the other ROIs and increased from the lowest to the highest force in a monotonic fashion. In the anterior CB (Fig. 3B), the BOLD signal increased with force in a similar manner as in M1, although the differences in beta values between 10% and 20% MVC were only close to significance. In contrast, the beta values in S1 (Fig. 3C) and posterior CB (Fig. 3D) did not increase monotonically. Because the beta values of both foci in posterior CB behaved similarly, only one of them is displayed in Figure 3. In these three regions, almost the same beta values were obtained for 10% and 20% and an increase occurred only for 30% MVC. Finally, in left and right PMv (Fig. 3E, F), as well as in the two foci of the right IPL (Fig. 3G, H), similar relationships between peak force and beta values were noticed, namely a high cortical activation during 10%, a significant decrease for 20%, followed by a strong increase for 30% MVC (Table III). Bilateral PMv regions and the two IPL foci had the lowest beta values.

Figure 3.

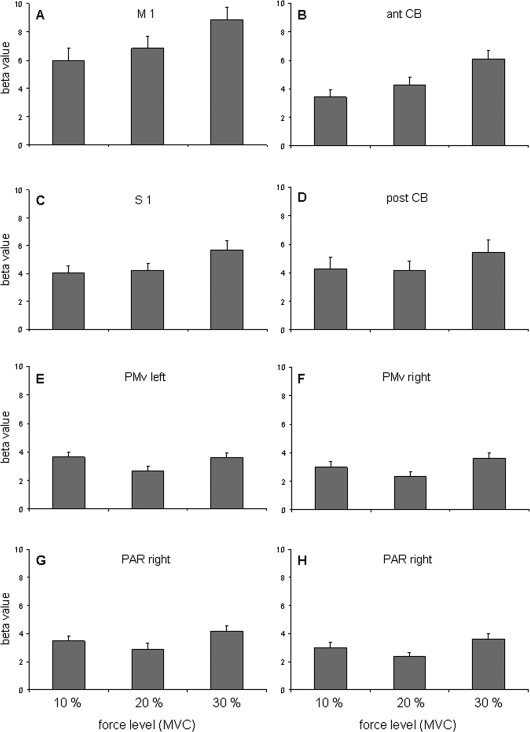
Figure 3

Open in a new tab

Mean and standard error of the beta weights collected for each force level in left primary motor cortex (A), right anterior cerebellum (B), left somatosensory cortex (C), left posterior cerebellum (D), left and right ventral premotor cortex (E, F), and two foci in the right inferior parietal lobule (G, H).

For the eight ROIs shown in Figure 3, linear correlation between the beta values and mean peak force and the first derivate of force dF/dt were assessed by the Pearson's correlation coefficient and considered significant for P ≤ 0.05. As illustrated in Figure 4, the beta values in most ROIs were positively correlated for both, peak force and dF/dt, but the significance level was reached only in M1, S1, and anterior CB, i.e., for the peak force (M1, r = 0.44, P ≤ 0.01; S1, r = 0.31, P ≤ 0.05; anterior CB, r = 0.4, P ≤ 0.05) and for dF/dt (M1 (r = 0.32, P ≤ 0.01; S1, r = 0.34, P ≤ 0.05; in anterior CB, r = 0.45, P ≤ 0.05). To test whether the number of force pulses had an influence on the resulting BOLD signal, we also correlated the beta values with the number of force pulses. In four of eight ROIs, namely M1, left PMv, and the two IPL foci, the beta values correlated negatively with the number of force pulses, in three of them even significantly (M1, r = −0.35, P ≤ 0.01, PMv left, r = −0.32, P ≤ 0.05, IPL right, r = −0.55, P ≤ 0.01).

Figure 4.

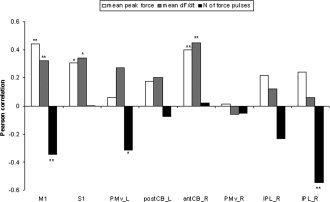
Figure 4

Open in a new tab

Pearson's correlation coefficients between the beta values of eight ROIs and mean peak forces (white columns), mean dF/dt (grey columns) and mean number of force pulses (black columns) for the 14 subjects. **P ≤ 0.01, *P ≤ 0.05. Abbreviations: see legend of Figure 2.

Single Subject ROI Analysis in M1

Single subject ROI analysis was performed to better understand the nature of the linear force scaling in M1 and the potential influence of the number of force pulses. In most subjects (10/14) the increase of the beta values with applied force was linear, with correlation coefficients between 0.88 and 1.0. Nine of these 10 subjects, showed strongly negative relationships between beta values and number of force pulses (correlations coefficients between 0.87 and 0.99). The four remaining subjects shown in Figure 5 did not display any monotonic increase between beta values and the three peak forces, but in two out of these four a strong activation increase occurred for 30% MVC (VP2 and VP3). In these four subjects, the relationship between beta values and number of force pulses showed the same negative tendency as in the other 10 (VP1 r = −0.13, VP2 r = −0.37, VP3 r = −0.68, VP4 r = −0.12).

Figure 5.

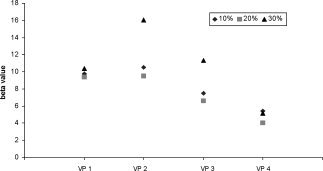
Figure 5

Open in a new tab

Individual beta values in primary motor cortex (M1) for the four subjects who did not show any linear relationship between those values and force (VP 1, 2, 3, and 4).

DISCUSSION

The aim of the present study was to understand how the scaling of a low range, visually guided force is reflected in various regions of the sensorimotor network of healthy humans. We report three major observations for dynamic force in power grip. First, we confirm that power grip activates all motor cortical and subcortical regions. Second, brain activation is modulated by the force amplitude in several, but not all regions, including contralateral M1 and S1, bilateral PMv and inferior parietal areas IPL, and CB. The third and most important finding shows that within these regions only two showed a linear scaling of the BOLD signal with grip force, namely left M1 and right anterior CB. In all other force‐related areas, i.e., PMv, S1, IPL, and posterior CB, the scaling is nonlinear, showing either an activation increase only at the highest force level or even more complex modulations. Brain activation did not correlate positively with the frequency of force pulses in any of the regions where brain activation was modulated by force. Our findings lead to the following conclusions: force is differentially represented in various sensorimotor regions, cortical activation does not necessarily increase as a function of power grip strength, and other factors, such as visual input, instruction, and attention, may modulate brain activation in visuomotor tasks.

Cortical and Subcortical Regions Activated by Power Grip

A large number of cortical and subcortical areas were activated by the visuomotor task. Most regions have already been described in previous brain imaging studies on force control, mainly related to precision grip [Ehrsson et al.,2000,2001; Pope et al.,2005; Spraker et al.,2007; Vaillancourt et al.,2003,2004,2006,2007] and more rarely to power grip [Cramer et al.,2002; Dai et al.,2001; Ehrsson et al.,2000; Kutz‐Buschbeck et al.,2008] and index finger or wrist movement [Dettmers et al.,1995,1996; Ludman et al.,1996; Peck et al.,2001; Thickbroom et al.,1999; van Duinen et al.,2008]. Thus, our data confirm task‐related activation in contralateral M1 and S1, in SMA/CMA, in parietal and premotor regions, mostly bilaterally, as well as in basal ganglia and cerebellum. In these previous investigations, force‐related activation was mainly reported for the control of static force, i.e., for forces, which had to be maintained for several seconds [Dai et al.,2001; Kuhtz‐Buschbeck et al.,2001; Peck et al.,2001; Spraker et al.,2007; Thickbroom et al.,1999; Vaillancourt et al.,2003,2004,2006; van Duinen et al.,2008]. Only a few studies addressed dynamic force generated either with finger flexion or wrist movements [Dettmers et al.,1995,1996; Ludman et al.,1996; Peck et al.,2001; Ramnani et al.,2001; Thickbroom et al.,1999] or with precision and power grip [Cramer et al.,2002; Ehrsson et al.,2000; Kuhtz‐Buschbeck et al.,2008; Pope et al.,2005; Vaillancourt et al.,2004,2006].

From all the regions showing task‐related activation in our study, force increase was associated with significant BOLD signal changes only in nine sites, i.e., contralateral M1 and S1, right IPL (2 foci), bilateral PMv, and cerebellum (3 foci). In contrast, the strong activation in medial motor structures (SMA/CMA) did not correlate with the amplitude of the dynamic force pulses. It is interesting to mention that, in early neurophysiological investigations in monkeys exerting force in precision grip, single‐cell firing rate in SMA/CMA was poorly related to force [Cadoret and Smith,1997; Smith,1979]. The lack of modulation within the low force range in SMA is in accordance with the findings of Cramer et al. [2002] showing force‐related increase in BOLD signal only in some subjects, mainly between medium and hard squeeze. However, these data are at odd with Dai et al. [2001] who reported that for a static handgrip the amplitude of the BOLD signal was directly proportional to the degree of muscle activation in several motor cortical fields including SMA and with those of van Duinen et al. [2008] for isometric contraction of the first dorsal interosseus muscle. Major differences in the control of static force and of dynamic force pulses and in the force range tested may account for this discrepancy. Similarly, we found no positive regression coefficients between the activation in the basal ganglia and the exerted force, which contrasts with a recent investigation by Spraker et al. [2007] showing positive increase in BOLD signal with static force in pallidum and subthalamus over a broad force range.

Linearity Between Power Grip Force and BOLD Signal

Primary motor cortex

In the group analysis, the BOLD signal in contralateral M1 increased linearly as a function of grip strength. This finding is supported by several human brain mapping studies [Cramer et al.,2002; Dai et al.,2001; Dettmers et al.,1995,1996; Ehrsson et al.,2001; Kuhtz‐Buschbeck et al.,2008; Peck et al.,2001; Vaillancourt et al.,2004] reporting significant correlations between force or electromyogram and number of activated pixels and/or average signal intensity. Interestingly, in almost all subjects tested by Cramer et al. [2002] a clear activation increase mainly occurred between the medium and hard squeeze levels, which is in concordance with our observations. Three main factors may account for the stronger BOLD signal with higher force: first the increased firing rate of corticospinal neurons being repeatedly reported in conscious monkeys [Ashe,1997; Cheney and Fetz,1980; Evarts,1968; Hepp‐Reymond et al.,1978; Maier et al.,1993; Smith et al.,1975], second the recruitment of a larger number of M1 neurons with higher forces, resulting in an increase in activation volume, and finally the increased somatosensory feedback because of stronger stimulation of cutaneous receptors [Johnson‐Frey,2004; Witney et al.,2004] and of proprioceptors associated with higher grip force. This stimulation may lead to increased input to the M1 neurons, either directly via the thalamus, or indirectly via S1 and corticocortical connections, and is supported by our finding that power grip force at 30% MVC produced the strongest fMRI signal in S1.

In some previous human imaging studies the relation between BOLD signal increase in M1 and dynamic force did not reach significance and often only the volume of activation but not the intensity of the signal showed an increment [Ehrsson et al.,2001; Ludman et al.,1996; Thickbroom et al.,1999]. This discrepancy can be attributed to differences in the range of force applied and the movements tested. In Thickbroom et al. [1999], the motor task consisted of generating force in finger flexion within a relatively low and narrow force range. Nevertheless, the lack of significant activation increase in M1 with a force increase from 5% to 10% MVC in this investigation is comparable to our data showing less significant BOLD signal increase between 10% and 20% MVC than between 20% and 30% MVC. However, these findings are at odd with single‐cell studies which, for a precision grip task, revealed linear regression coefficients for static and fine‐graded low force [Ashe,1997; Hepp‐Reymond et al.,1978,1999; Maier and Hepp‐Reymond,1995; Wannier et al.,1991]. The differences between neuroimaging and electrophysiological studies suggest that the BOLD signal in M1 does not always simply reflect dynamic force within a low range. It may also be modulated by other factors, such as attention required for the precise force control, leading to activation increase in sensorimotor cortical areas, including M1 [Binkofski et al.,2002; Ehrsson et al.,2001; Indovina and Sanes,2001; Johansen‐Berg and Matthews,2002; Rowe et al.,2002].

Anterior cerebellum

The importance of the cerebellum in controlling grasping is well known in clinical neurology [Holmes,1917], but cerebellar neural correlates related to grip force are rare. In conscious monkey, positive linear regression coefficients have been disclosed for both force and rate of force change in unidentified neurons, whereas the Purkinje cells decreased their firing with force [Smith and Bourbonnais,1981]. In our investigation, similar to M1, the BOLD signal increased linearly with the applied force in the ipsilateral anterior CB, as recently suggested by Kuhtz‐Buschbeck et al. [2008]. According to somatotopical investigations of the cerebellum the localization of our activation clusters corresponds to the reported anterior hand representation [Dimitrova et al.,2006; Grodd et al.,2001; Rijntjes et al.,1999]. The anterior cerebellum is part of the spinocerebellum and has two main sources of afferents. It receives information from proprioceptors (muscle spindles, tendon organs) and skin, via the spinocerebellar tracts [Bushara et al.,2001] and additionally descending information from motor areas, which conveys an efference copy of the ongoing movement via the pontine nuclei [Kelly and Strick,2003; Nowak et al.,2007; Ramnani et al.,2001; Ramnani,2006]. It is thus not surprising that the BOLD signal in the anterior cerebellum behaves in a quite similar way to that in M1. It has been proposed that the cerebellum predominantly processes sensory information from the target muscles to optimise movements [Jueptner and Weiller,1998; Kording and Wolpert,2006; Nowak et al.,2007]. Therefore, the BOLD signal may be not merely related to force generation and control but mainly caused by an additional recruitment in the sensorimotor feedback from muscle spindles and tendon receptors.

Nonlinear Relationship Between BOLD Signal and Power Grip Force

In S1, IPL, PM, and posterior cerebellum, the relationships between brain activation and power grip force were quite complex, either nonmonotonic or showing modulations, which cannot be simply interpreted as force correlates.

Primary somatosensory cortex

The BOLD signal in S1 did not correlate linearly over the whole force range. Similar beta values were obtained for low force pulses of 10% and 20% MVC, and a significant increase was only observed for 30% MVC. Recent neuroimaging investigations suggest that the level of required attention is an important factor mediating cortical responsiveness [Arthurs et al.,2004; Johansen‐Berg and Matthews,2002; Rowe et al.,2002]. Moreover, early somatosensory evoked potentials in S1 were modulated during attended sensory‐motor tracking [Legon and Staines,2006]. Thus, increased attention to the sensory feedback and, connected to this, increased processing of somatosensory signals in S1 during fine force control may have led to the nearly equivalent BOLD signal observed for 10% and 20% MVC. Our findings are in accordance neither with the significant positive correlation coefficients of static force in monkeys' S1 [Wannier et al.,1991] nor with brain imaging data of Dai et al., [2001] showing a linear increase in number of activated pixels and average intensity in S1 with static handgrip force. These observations once more suggest that force is centrally controlled in different manners for the static and dynamic force conditions.

Parietal and premotor cortex

Our main finding was that relationships between the BOLD signal and power grip force were complex and without any trend to linearity in both parietal and premotor areas bilaterally. Previous studies have suggested that parietal and premotor regions are involved in planning and online control of visually guided movements [Ehrsson et al.,2001,2003; Elsinger et al.,2006; Hamzei et al.,2002; Ogawa et al.,2006; Vaillancourt et al.,2003,2007]. Thereby, the transformation of visual input into action is controlled by a neuronal circuit where the PM cortex receives visual information from the extrastriate visual cortex via parietal areas [Hamzei et al.,2002]. The essential role of the parietal cortex for dynamic, goal‐directed sensorimotor integration suggested by Tunik et al. [2007] is supported by recent studies [Elsinger et al.,2006; Vaillancourt et al.,2007] demonstrating increased BOLD signal either with frequent compared to less frequent, intermittent visual feedback, or with external versus internally guided movements. Furthermore, parietal areas play an important role in the control of demanding motor tasks such as fine force control in precision grip [Ehrsson et al.,2001]. In line with these results, the stronger cortical activation in both IPL foci with 10% as compared to 20% MVC grip force, probably reflects the higher requirements in the precise control of low forces. This assumption is reinforced by the more frequent variability found at the individual level at our low force range. The predominant activation in the right posterior parietal cortex can be interpreted as involvement in conscious error evaluation, as suggested in a previous study using a visually guided motor task [Ogawa et al.,2006].

In PMv, the relationship between the BOLD signal and the applied force were almost identical to that in IPL. This can be explained by the fact that PMv receives projections from IPL, particularly from the AIP region, which is related to visuomotor control of the hand, in particular grasp [Hamzei et al.,2002; Shikata et al.,2003; Tanne‐Gariepy et al.,2002]. The stronger cortical activity with 10% compared to 20% MVC found in both ipsilateral and contralateral PMv, as well as in the parietal lobe, is supported by earlier reports using force pulses and maintained grip force, which also describe stronger activation in these regions for small grip forces than for high ones [Ehrsson et al.,2001; Kuhtz‐Buschbeck et al.,2001]. Primate studies have revealed that the neuronal firing rate in PMv can correlate with isometric force in precision grip, however these correlations were clearly context dependent [Hepp‐Reymond et al.,1999]. Our present findings provide further support to the suggestion of these previous studies that coding of force in PMv can be influenced by several factors.

Posterior cerebellum

In posterior CB, two main observations are worth discussing. First, the foci were bilateral and located mainly in lobule VIIA and Crus I, both belonging to the neocerebellum. According to several investigations these regions also have a hand representation, but mainly receive bilateral projections from premotor, prefrontal, and parietal cortices through the pontine nuclei [Dimitrova et al.,2006; Kelly and Strick,2003; Rijntjes et al.,1999]. In other words, the posterior CB seems to be essential for movement coordination [Ramnani et al.,2001] and visuomotor transformation [Miall et al.,2000; Vaillancourt et al.,2003]. Second, the BOLD signal in the posterior cerebellar foci also did not correlate linearly with the production of dynamic force pulses. The slightly higher activation of 10% as compared to 20% MVC in the posterior CB mirrors the cortical parietal and premotor activation and thus may reflect the more demanding sensory and attentional processing in visually guided force production during the 10% MVC condition. This finding is in accordance with studies reporting the greatest activation under attention to action [Allen et al.,1997; Indovina and Sanes,2001] and during complex manipulation [Milner et al.,2007]. Moreover, Vaillancourt et al. [2006] showed increase in activation volume and percent signal change in the lateral posterior CB with high‐frequency visual intermittent feedback during force control in precision grip. These previous observations corroborate our finding that posterior parts of the cerebellum are more strongly involved in the visuomotor processing rather than in simple force generation.

In conclusion, the current study on the generation of repetitive force pulses in power grip provides new insights on the neural organization of visually guided force control. We have shown that cortical activation does not necessarily scale with increased grip strength and that force control in humans is differentially represented in the cortical and subcortical sensorimotor network. Our findings suggest that fine‐graded forces are mainly controlled by M1 and the corresponding anterior cerebellar region. Activation in premotor and parietal cortical areas, as well as posterior cerebellum during the specific task is strongly modulated by visual input and context‐dependent information. These observations may be of potential clinical significance in recovery following an infarct involving M1 in the sense that premotor and parietal cortical areas may poorly contribute to recovery in the control of low forces, whereas the anterior cerebellum with its direct peripheral input may play a primary role.

Supporting information

Additional Supporting Information may be found in the online version of this article.

Supporting Figure 1

Click here for additional data file. (2.6MB, tif)

Table I annexe: Mean peak force, standard deviation, intra‐individual variance of the subjects' force output for each force level, and the significance value in the ANOVA analysis. The variance of the performance was more frequently significant in the 10% and 20% MVC force range than for 30% MVC.

Click here for additional data file. (91KB, doc)

REFERENCES

  1. Allen G,Buxton RB,Wong EC,Courchesne E ( 1997): Attentional activation of the cerebellum independent of motor involvement. Science 275: 1940–1943. [DOI] [PubMed] [Google Scholar]
  2. Anner‐Baratti R,Allum JH,Hepp‐Reymond MC ( 1986): Neural correlates of isometric force in the “motor” thalamus. Exp Brain Res 63: 567–580. [DOI] [PubMed] [Google Scholar]
  3. Arthurs OJ,Johansen‐Berg H,Matthews PM,Boniface SJ ( 2004): Attention differentially modulates the coupling of fMRI BOLD and evoked potential signal amplitudes in the human somatosensory cortex. Exp Brain Res 157: 269–274. [DOI] [PubMed] [Google Scholar]
  4. Ashe J ( 1997): Force and the motor cortex. Behav Brain Res 87: 255–269. [DOI] [PubMed] [Google Scholar]
  5. Begliomini C,Caria A,Grodd W,Castiello U ( 2007): Comparing natural and constrained movements: New insights into the visuomotor control of grasping. PLoS ONE 2: e1108. [DOI] [PMC free article] [PubMed] [Google Scholar]
  6. Binkofski F,Fink GR,Geyer S,Buccino G,Gruber O,Shah NJ,Taylor JG,Seitz RJ,Zilles K,Freund HJ ( 2002): Neural activity in human primary motor cortex areas 4a and 4p is modulated differentially by attention to action. J Neurophysiol 88: 514–519. [DOI] [PubMed] [Google Scholar]
  7. Boecker H,Lee A,Muhlau M,Ceballos‐Baumann A,Ritzl A,Spilker ME,Marquart C,Hermsdorfer J ( 2005): Force level independent representations of predictive grip force‐load force coupling: A PET activation study. Neuroimage 25: 243–252. [DOI] [PubMed] [Google Scholar]
  8. Bushara KO,Wheat JM,Khan A,Mock BJ,Turski PA,Sorenson J,Brooks BR ( 2001): Multiple tactile maps in the human cerebellum. Neuroreport 12: 2483–2486. [DOI] [PubMed] [Google Scholar]
  9. Cadoret G,Smith AM ( 1997): Comparison of the neuronal activity in the SMA and the ventral cingulate cortex during prehension in the monkey. J Neurophysiol 77: 153–166. [DOI] [PubMed] [Google Scholar]
  10. Cheney PD,Fetz EE ( 1980): Functional classes of primate corticomotoneuronal cells and their relation to active force. J Neurophysiol 44: 773–791. [DOI] [PubMed] [Google Scholar]
  11. Conrad B,Wiesendanger M,Matsunami K,Brooks VB ( 1977): Precentral unit activity related to control of arm movements. Exp Brain Res 29: 85–95. [DOI] [PubMed] [Google Scholar]
  12. Cramer SC,Weisskoff RM,Schaechter JD,Nelles G,Foley M,Finklestein SP,Rosen BR ( 2002): Motor cortex activation is related to force of squeezing. Hum Brain Mapp 16: 197–205. [DOI] [PMC free article] [PubMed] [Google Scholar]
  13. Dai TH,Liu JZ,Sahgal V,Brown RW,Yue GH ( 2001): Relationship between muscle output and functional MRI‐measured brain activation. Exp Brain Res 140: 290–300. [DOI] [PubMed] [Google Scholar]
  14. Dettmers C,Fink GR,Lemon RN,Stephan KM,Passingham RE,Silbersweig D,Holmes A,Ridding MC,Brooks DJ,Frackowiak RS ( 1995): Relation between cerebral activity and force in the motor areas of the human brain. J Neurophysiol 74: 802–815. [DOI] [PubMed] [Google Scholar]
  15. Dettmers C,Connelly A,Stephan KM,Turner R,Friston KJ,Frackowiak RS,Gadian DG ( 1996): Quantitative comparison of functional magnetic resonance imaging with positron emission tomography using a force‐related paradigm. Neuroimage 4: 201–209. [DOI] [PubMed] [Google Scholar]
  16. Dimitrova A,de Greiff A,Schoch B,Gerwig M,Frings M,Gizewski ER,Timmann D ( 2006): Activation of cerebellar nuclei comparing finger, foot and tongue movements as revealed by fMRI. Brain Res Bull 71: 233–241. [DOI] [PubMed] [Google Scholar]
  17. Ehrsson HH,Fagergren A,Jonsson T,Westling G,Johansson RS,Forssberg H ( 2000): Cortical activity in precision‐versus power‐grip tasks: An fMRI study. J Neurophysiol 83: 528–536. [DOI] [PubMed] [Google Scholar]
  18. Ehrsson HH,Fagergren E,Forssberg H ( 2001): Differential fronto‐parietal activation depending on force used in a precision grip task: An fMRI study. J Neurophysiol 85: 2613–2623. [DOI] [PubMed] [Google Scholar]
  19. Ehrsson HH,Fagergren A,Johansson RS,Forssberg H ( 2003): Evidence for the involvement of the posterior parietal cortex in coordination of fingertip forces for grasp stability in manipulation. J Neurophysiol 90: 2978–2986. [DOI] [PubMed] [Google Scholar]
  20. Elsinger CL,Harrington DL,Rao SM ( 2006): From preparation to online control: Reappraisal of neural circuitry mediating internally generated and externally guided actions. Neuroimage 31: 1177–1187. [DOI] [PubMed] [Google Scholar]
  21. Evarts EV ( 1968): Relation of pyramidal tract activity to force exerted during voluntary movement. J Neurophysiol 31: 14–27. [DOI] [PubMed] [Google Scholar]
  22. Evarts EV,Fromm C,Kroller J,Jennings VA ( 1983): Motor cortex control of finely graded forces. J Neurophysiol 49: 1199–1215. [DOI] [PubMed] [Google Scholar]
  23. Floyer‐Lea A,Matthews PM ( 2004): Changing brain networks for visuomotor control with increased movement automaticity. J Neurophysiol 92: 2405–2412. [DOI] [PubMed] [Google Scholar]
  24. Floyer‐Lea A,Matthews PM ( 2005): Distinguishable brain activation networks for short‐ and long‐term motor skill learning. J Neurophysiol 94: 512–518. [DOI] [PubMed] [Google Scholar]
  25. Genovese CR,Lazar NA,Nichols T ( 2002): Thresholding of statistical maps in functional neuroimaging using the false discovery rate. Neuroimage 15: 870–878. [DOI] [PubMed] [Google Scholar]
  26. Georgopoulos AP,Ashe J,Smyrnis N,Taira M ( 1992): The motor cortex and the coding of force. Science 256: 1692–1695. [DOI] [PubMed] [Google Scholar]
  27. Grodd W,Hulsmann E,Lotze M,Wildgruber D,Erb M ( 2001): Sensorimotor mapping of the human cerebellum: fMRI evidence of somatotopic organization. Hum Brain Mapp 13: 55–73. [DOI] [PMC free article] [PubMed] [Google Scholar]
  28. Hamzei F,Dettmers C,Rijntjes M,Glauche V,Kiebel S,Weber B,Weiller C ( 2002): Visuomotor control within a distributed parieto‐frontal network. Exp Brain Res 146: 273–281. [DOI] [PubMed] [Google Scholar]
  29. Hepp‐Reymond MC,Wyss UR,Anner R ( 1978): Neuronal coding of static force in the primate motor cortex. J Physiol (Paris) 74: 287–291. [PubMed] [Google Scholar]
  30. Hepp‐Reymond MC,Wannier TM,Maier MA,Rufener EA ( 1989): Sensorimotor cortical control of isometric force in the monkey. Prog Brain Res 80: 451–463; discussion 427–430. [DOI] [PubMed] [Google Scholar]
  31. Hepp‐Reymond MC,Husler EJ,Maier MA,Ql HX ( 1994): Force‐related neuronal activity in two regions of the primate ventral premotor cortex. Can J Physiol Pharmacol 72: 571–579. [DOI] [PubMed] [Google Scholar]
  32. Hepp‐Reymond M,Kirkpatrick‐Tanner M,Gabernet L,Qi HX,Weber B ( 1999): Context‐dependent force coding in motor and premotor cortical areas. Exp Brain Res 128: 123–133. [DOI] [PubMed] [Google Scholar]
  33. Holmes G ( 1917): The symptoms of acute cerebellar injuries due to gunshot injuries. Brain 40: 461–535. [Google Scholar]
  34. Indovina I,Sanes JN ( 2001): Combined visual attention and finger movement effects on human brain representations. Exp Brain Res 140: 265–279. [DOI] [PubMed] [Google Scholar]
  35. Johansen‐Berg H,Matthews PM ( 2002): Attention to movement modulates activity in sensori‐motor areas, including primary motor cortex. Exp Brain Res 142: 13–24. [DOI] [PubMed] [Google Scholar]
  36. Johnson‐Frey SH ( 2004): The neural bases of complex tool use in humans. Trends Cogn Sci 8: 71–78. [DOI] [PubMed] [Google Scholar]
  37. Jueptner M,Weiller C ( 1998): A review of differences between basal ganglia and cerebellar control of movements as revealed by functional imaging studies. Brain 121(part 8): 1437–1449. [DOI] [PubMed] [Google Scholar]
  38. Kelly RM,Strick PL ( 2003): Cerebellar loops with motor cortex and prefrontal cortex of a nonhuman primate. J Neurosci 23: 8432–8444. [DOI] [PMC free article] [PubMed] [Google Scholar]
  39. Kinoshita H,Oku N,Hashikawa K,Nishimura T ( 2000): Functional brain areas used for the lifting of objects using a precision grip: A PET study. Brain Res 857: 119–130. [DOI] [PubMed] [Google Scholar]
  40. Kording KP,Wolpert DM ( 2006): Bayesian decision theory in sensorimotor control. Trends Cogn Sci 10: 319–326. [DOI] [PubMed] [Google Scholar]
  41. Kuhtz‐Buschbeck JP,Ehrsson HH,Forssberg H ( 2001): Human brain activity in the control of fine static precision grip forces: An fMRI study. Eur J Neurosci 14: 382–390. [DOI] [PubMed] [Google Scholar]
  42. Kuhtz‐Buschbeck JP,Gilster R,Wolff S,Ulmer S,Siebner H,Jansen O ( 2008): Brain activity is similar during precision and power gripping with light force: An fMRI study. Neuroimage 40: 1469–1481. [DOI] [PubMed] [Google Scholar]
  43. Larsell O,Jansen J ( 1972): The Comparative Anatomy and Histology of the Cerebellum The Human Cerebellum, Cerebellar Connections, and Cerebellar Cortex. Minneapollis: University of Minnesota Press. [Google Scholar]
  44. Legon W,Staines WR ( 2006): Predictability of the target stimulus for sensory‐guided movement modulates early somatosensory cortical potentials. Clin Neurophysiol 117: 1345–1353. [DOI] [PubMed] [Google Scholar]
  45. Liu JZ,Zhang L,Brown RW,Yue GH ( 2004): Reproducibility of fMRI at 1.5 T in a strictly controlled motor task. Magn Reson Med 52: 751–760. [DOI] [PubMed] [Google Scholar]
  46. Ludman CN,Cooper TG,Ploutz‐Synder LL,Potchen EJ,Meyer RA ( 1996): Force of voluntary exercise does not affect sensorimotor cortex activation as detected by functional MRI at 1.5 T. NMR Biomed 9: 228–232. [DOI] [PubMed] [Google Scholar]
  47. Maier MA,Bennett KM,Hepp‐Reymond MC,Lemon RN ( 1993): Contribution of the monkey corticomotoneuronal system to the control of force in precision grip. J Neurophysiol 69: 772–785. [DOI] [PubMed] [Google Scholar]
  48. Maier MA,Hepp‐Reymond MC ( 1995): EMG activation patterns during force production in precision grip. II. Muscular synergies in the spatial and temporal domain. Exp Brain Res 103: 123–136. [DOI] [PubMed] [Google Scholar]
  49. Miall RC,Imamizu H,Miyauchi S ( 2000): Activation of the cerebellum in co‐ordinated eye and hand tracking movements: An fMRI study. Exp Brain Res 135: 22–33. [DOI] [PubMed] [Google Scholar]
  50. Milner TE,Franklin DW,Imamizu H,Kawato M ( 2007): Central control of grasp: Manipulation of objects with complex and simple dynamics. Neuroimage 36: 388–395. [DOI] [PubMed] [Google Scholar]
  51. Muley SA,Strother SC,Ashe J,Frutiger SA,Anderson JR,Sidtis JJ,Rottenberg DA ( 2001): Effects of changes in experimental design on PET studies of isometric force. Neuroimage 13: 185–195. [DOI] [PubMed] [Google Scholar]
  52. Nowak DA,Topka H,Timmann D,Boecker H,Hermsdorfer J ( 2007): The role of the cerebellum for predictive control of grasping. Cerebellum 6: 7–17. [DOI] [PubMed] [Google Scholar]
  53. Ogawa K,Inui T,Sugio T ( 2006): Separating brain regions involved in internally guided and visual feedback control of moving effectors: An event‐related fMRI study. Neuroimage 32: 1760–1770. [DOI] [PubMed] [Google Scholar]
  54. Oldfield RC ( 1971): The assessment and analysis of handedness: The Edinburgh inventory. Neuropsychologia 9: 97–113. [DOI] [PubMed] [Google Scholar]
  55. Peck KK,Sunderland A,Peters AM,Butterworth S,Clark P,Gowland PA ( 2001): Cerebral activation during a simple force production task: Changes in the time course of the haemodynamic response. Neuroreport 12: 2813–2816. [DOI] [PubMed] [Google Scholar]
  56. Pope P,Wing AM,Praamstra P,Miall RC ( 2005): Force related activations in rhythmic sequence production. Neuroimage 27: 909–918. [DOI] [PubMed] [Google Scholar]
  57. Pruessmann KP,Weiger M,Scheidegger MB,Boesiger P ( 1999): SENSE: Sensitivity encoding for fast MRI. Magn Reson Med 42: 952–962. [PubMed] [Google Scholar]
  58. Ramnani N,Toni I,Passingham RE,Haggard P ( 2001): The cerebellum and parietal cortex play a specific role in coordination: A PET study. Neuroimage 14: 899–911. [DOI] [PubMed] [Google Scholar]
  59. Ramnani N ( 2006): The primate cortico‐cerebellar system: Anatomy and function. Nat Rev Neurosci 7: 511–522. [DOI] [PubMed] [Google Scholar]
  60. Riehle A,MacKay WA,Requin J ( 1994): Are extent and force independent movement parameters? Preparation‐ and movement‐related neuronal activity in the monkey cortex. Exp Brain Res 99: 56–74. [DOI] [PubMed] [Google Scholar]
  61. Rijntjes M,Buechel C,Kiebel S,Weiller C ( 1999): Multiple somatotopic representations in the human cerebellum. Neuroreport 10: 3653–3658. [DOI] [PubMed] [Google Scholar]
  62. Rowe J,Friston K,Frackowiak R,Passingham R ( 2002): Attention to action: Specific modulation of corticocortical interactions in humans. Neuroimage 17: 988–998. [PubMed] [Google Scholar]
  63. Schmitz C,Jenmalm P,Ehrsson HH,Forssberg H ( 2005): Brain activity during predictable and unpredictable weight changes when lifting objects. J Neurophysiol 93: 1498–1509. [DOI] [PubMed] [Google Scholar]
  64. Shikata E,Hamzei F,Glauche V,Koch M,Weiller C,Binkofski F,Buchel C ( 2003): Functional properties and interaction of the anterior and posterior intraparietal areas in humans. Eur J Neurosci 17: 1105–1110. [DOI] [PubMed] [Google Scholar]
  65. Smith AM,Hepp‐Reymond MC,Wyss UR ( 1975): Relation of activity in precentral cortical neurons to force and rate of force change during isometric contractions of finger muscles. Exp Brain Res 23: 315–332. [DOI] [PubMed] [Google Scholar]
  66. Smith AM ( 1979): The activity of supplementary motor area neurons during a maintained precision grip. Brain Res 172: 315–327. [DOI] [PubMed] [Google Scholar]
  67. Smith AM,Bourbonnais D ( 1981): Neuronal activity in cerebellar cortex related to control of prehensile force. J Neurophysiol 45: 286–303. [DOI] [PubMed] [Google Scholar]
  68. Spraker MB,Yu H,Corcos DM,Vaillancourt DE ( 2007): Role of individual basal ganglia nuclei in force amplitude generation. J Neurophysiol 98: 821–834. [DOI] [PMC free article] [PubMed] [Google Scholar]
  69. Taira M,Boline J,Smyrnis N,Georgopoulos AP,Ashe J ( 1996): On the relations between single cell activity in the motor cortex and the direction and magnitude of three‐dimensional static isometric force. Exp Brain Res 109: 367–376. [DOI] [PubMed] [Google Scholar]
  70. Talairach J,Tournoux P ( 1988): Co‐planar Stereotaxic Atlas of the Human Brain. New York: New York Thieme. [Google Scholar]
  71. Tanne‐Gariepy J,Rouiller EM,Boussaoud D ( 2002): Parietal inputs to dorsal versus ventral premotor areas in the macaque monkey: Evidence for largely segregated visuomotor pathways. Exp Brain Res 145: 91–103. [DOI] [PubMed] [Google Scholar]
  72. Thach WT ( 1978): Correlation of neural discharge with pattern and force of muscular activity, joint position, and direction of intended next movement in motor cortex and cerebellum. J Neurophysiol 41: 654–676. [DOI] [PubMed] [Google Scholar]
  73. Thickbroom GW,Phillips BA,Morris I,Byrnes ML,Mastaglia FL ( 1998): Isometric force‐related activity in sensorimotor cortex measured with functional MRI. Exp Brain Res 121: 59–64. [DOI] [PubMed] [Google Scholar]
  74. Thickbroom GW,Phillips BA,Morris I,Byrnes ML,Sacco P,Mastaglia FL ( 1999): Differences in functional magnetic resonance imaging of sensorimotor cortex during static and dynamic finger flexion. Exp Brain Res 126: 431–438. [DOI] [PubMed] [Google Scholar]
  75. Tunik E,Rice HJ,Hamilton A,Grafton ST ( 2007): Beyond grasping: Representation of action in human anterior intraparietal sulcus. Neuroimage 36( Suppl 2): T77–T86. [DOI] [PMC free article] [PubMed] [Google Scholar]
  76. Vaillancourt DE,Russell DM ( 2002): Temporal capacity of short‐term visuomotor memory in continuous force production. Exp Brain Res 145: 275–285. [DOI] [PubMed] [Google Scholar]
  77. Vaillancourt DE,Thulborn KR,Corcos DM ( 2003): Neural basis for the processes that underlie visually guided and internally guided force control in humans. J Neurophysiol 90: 3330–3340. [DOI] [PubMed] [Google Scholar]
  78. Vaillancourt DE,Mayka MA,Thulborn KR,Corcos DM ( 2004): Subthalamic nucleus and internal globus pallidus scale with the rate of change of force production in humans. Neuroimage 23: 175–186. [DOI] [PubMed] [Google Scholar]
  79. Vaillancourt DE,Mayka MA,Corcos DM ( 2006): Intermittent visuomotor processing in the human cerebellum, parietal cortex, and premotor cortex. J Neurophysiol 95: 922–931. [DOI] [PMC free article] [PubMed] [Google Scholar]
  80. Vaillancourt DE,Yu H,Mayka MA,Corcos DM ( 2007): Role of the basal ganglia and frontal cortex in selecting and producing internally guided force pulses. Neuroimage 36: 793–803. [DOI] [PMC free article] [PubMed] [Google Scholar]
  81. Van Duinen H,Renken R,Maurits NM,Zijdewind I ( 2008): Relation between muscle and brain activity during isometric contractions of the first dorsal interosseus muscle. Hum Brain Mapp 29: 281–299. [DOI] [PMC free article] [PubMed] [Google Scholar]
  82. Wannier TM,Maier MA,Hepp‐Reymond MC ( 1991): Contrasting properties of monkey somatosensory and motor cortex neurons activated during the control of force in precision grip. J Neurophysiol 65: 572–589. [DOI] [PubMed] [Google Scholar]
  83. Werner W,Bauswein E,Fromm C ( 1991): Static firing rates of premotor and primary motor cortical neurons associated with torque and joint position. Exp Brain Res 86: 293–302. [DOI] [PubMed] [Google Scholar]
  84. Wexler BE,Fulbright RK,Lacadie CM,Skudlarski P,Kelz MB,Constable RT,Gore JC ( 1997): An fMRI study of the human cortical motor system response to increasing functional demands. Magn Reson Imaging 15: 385–396. [DOI] [PubMed] [Google Scholar]
  85. Witney AG,Wing A,Thonnard JL,Smith AM ( 2004): The cutaneous contribution to adaptive precision grip. Trends Neurosci 27: 637–643. [DOI] [PubMed] [Google Scholar]

Associated Data

This section collects any data citations, data availability statements, or supplementary materials included in this article.

Supplementary Materials

Additional Supporting Information may be found in the online version of this article.

Supporting Figure 1

Click here for additional data file. (2.6MB, tif)

Table I annexe: Mean peak force, standard deviation, intra‐individual variance of the subjects' force output for each force level, and the significance value in the ANOVA analysis. The variance of the performance was more frequently significant in the 10% and 20% MVC force range than for 30% MVC.

Click here for additional data file. (91KB, doc)

Articles from Human Brain Mapping are provided here courtesy of Wiley

RESOURCES