Cerebral blood flow rates in recent great apes are greater than in Australopithecus species that had equal or larger brains

Abstract

Brain metabolic rate (MR) is linked mainly to the cost of synaptic activity, so may be a better correlate of cognitive ability than brain size alone. Among primates, the sizes of arterial foramina in recent and fossil skulls can be used to evaluate brain blood flow rate, which is proportional to brain MR. We use this approach to calculate flow rate in the internal carotid arteries $({\dot{Q}}_{\mathrm{ICA}})$, which supply most of the primate cerebrum. ${\dot{Q}}_{\mathrm{ICA}}$ is up to two times higher in recent gorillas, chimpanzees and orangutans compared with 3-million-year-old australopithecine human relatives, which had equal or larger brains. The scaling relationships between ${\dot{Q}}_{\mathrm{ICA}}$ and brain volume (V_br) show exponents of 1.03 across 44 species of living haplorhine primates and 1.41 across 12 species of fossil hominins. Thus, the evolutionary trajectory for brain perfusion is much steeper among ancestral hominins than would be predicted from living primates. Between 4.4-million-year-old Ardipithecus and Homo sapiens, V_br increased 4.7-fold, but ${\dot{Q}}_{\mathrm{ICA}}$ increased 9.3-fold, indicating an approximate doubling of metabolic intensity of brain tissue. By contrast, ${\dot{Q}}_{\mathrm{ICA}}$ is proportional to V_br among haplorhine primates, suggesting a constant volume-specific brain MR.

1. Introduction

Brain size is the usual measure in discussions of the evolution of cognitive ability among primates, despite recognized shortcomings [1]. Although absolute brain size appears to correlate better with cognitive ability than encephalization quotient, progression index or neocortex ratio [2,3], an even better correlate might be brain metabolic rate (MR), because it represents the energy cost of neurological function. However, brain MR is difficult to measure directly in living primates and impossible in extinct ones.

One solution to the problem has been to measure oxygen consumption rates and glucose uptake rates on living mammals in relation to brain size and then apply the results to brain sizes of living and extinct primates. Because physiological rates rarely relate linearly to volumes or masses of tissues, any comparison requires allometric analysis. For example, brain MR can be analysed in relation to endocranial volume (≈ brain volume, V_br) with an allometric equation of the form, MR = aV_br^b, where a is the elevation (or scaling factor, indicating the height of the curve) and b is the scaling exponent (indicating the shape of the curve on arithmetic axes). If b = 1.0, then MR is directly proportional to brain size. If b is less than 1, then MR increases with brain size, but the metabolic intensity per unit volume of neural tissue decreases. If b is greater than 1, the metabolic intensity of neural tissue increases. The exponent for brain MR measured as oxygen consumption and glucose use across several mammalian species is approximately 0.86, and the exponent for cortical brain blood flow rate in mammals is between 0.81 and 0.87 [4,5]. The similarity of the exponents indicates that blood flow rate is a good proxy for brain MR in mammals in general. The exponents are less than 1.0, which shows that brain MR and blood flow rate increase with brain size but with decreasing metabolic and perfusion intensities of the neural tissue.

Recent studies show that blood flow rate in the internal carotid artery $({\dot{Q}}_{\mathrm{ICA}})$ can be calculated from the size of the carotid foramen through which it passes to the brain [6]. The artery occupies the foramen lumen almost entirely [7–9], therefore defining the outer radius of the artery (r_o), from which inner lumen radius (r_i) can be estimated, assuming that arterial wall thickness (r_o – r_i) is a constant ratio (w) with lumen radius (w = (r_o – r_i)/r_i), according to the law of Laplace. The haemodynamic equation used to calculate ${\dot{Q}}_{\mathrm{ICA}}$ is referred to as the ‘shear stress equation’, and attributed to Poiseuille: $\dot{Q}=(\tau \pi r_i^3)/(4\eta )$, where $\dot{Q}$ is the blood flow rate (cm³ s⁻¹), τ is the wall shear stress (dyn cm⁻²), r_i is the arterial lumen radius (cm) and η is the blood viscosity (dyn s cm⁻²) [10]. The technique was validated in mice, rats and humans, but was initially criticized [11], defended [12] and subsequently accepted [13]. However, the calculations involved three questionable assumptions: flow in the cephalic arteries conforms to Poiseuille flow theory, arterial wall shear stress can be calculated accurately from body mass (although there is no clear functional relationship between them) and the arterial wall thickness-to-lumen radius ratio (w) was a certain constant derived from only two values in the literature.

We have now made significant advancements to the initial methodology by replacing the shear stress equation, and its assumptions, with a new equation derived empirically from a meta-analysis of $\dot{Q}$ versus r_i in 30 studies of seven cephalic arteries of six mammalian genera, arriving at an allometric, so-called ‘empirical equation’, $\dot{Q}$ = 155 r_i^2.49 (R² = 0.94) [14]. The equation is based on stable cephalic flow rates, which vary little between rest, intense physical activity, mental exercise or sleep [14]. The equation also eliminates reliance on the somewhat tenuous estimation of arterial wall shear stress from body mass. We have also improved the calculation with a more extensive re-evaluation of carotid arterial wall thickness ratio (w = 0.30) from 14 imaging studies on humans (electronic supplementary material, text and table S1 for data and references). The present investigation implements these recent methodological advancements and re-evaluates the scaling of ${\dot{Q}}_{\mathrm{ICA}}$ as a function of V_br in extant haplorhine primates and in fossil hominins. The point of our study is to clarify these relationships between Homo sapiens, Australopithecus and modern great apes (Pongo, Pan, Gorilla) to resolve an apparent allometric conundrum within our previous studies: one analysis based on 34 species of extant Haplorhini, including H. sapiens, resulted in the equation ${\dot{Q}}_{\mathrm{ICA}}\hspace{0.17em}=8.82\times {10}^{-3}$ V_br^0.95 [6], while another analysis of 11 species of fossil hominin, also including H. sapiens, produced the equation ${\dot{Q}}_{\mathrm{ICA}}=1.70\times {10}^{-4}$ V_br^1.45 [15]. Humans are on both analyses with the largest brains, but the exponents of these equations are markedly different, and the lines converge. The present study confirms that hominin ancestors had lower ${\dot{Q}}_{\mathrm{ICA}}$ than predicted from V_br with the haplorhine equation. ${\dot{Q}}_{\mathrm{ICA}}$ in modern great apes is about twice that in Australopithecus species, despite similar or smaller V_br.

2. Results

(a). Internal carotid artery blood flow rate in extant great apes and Australopithecus

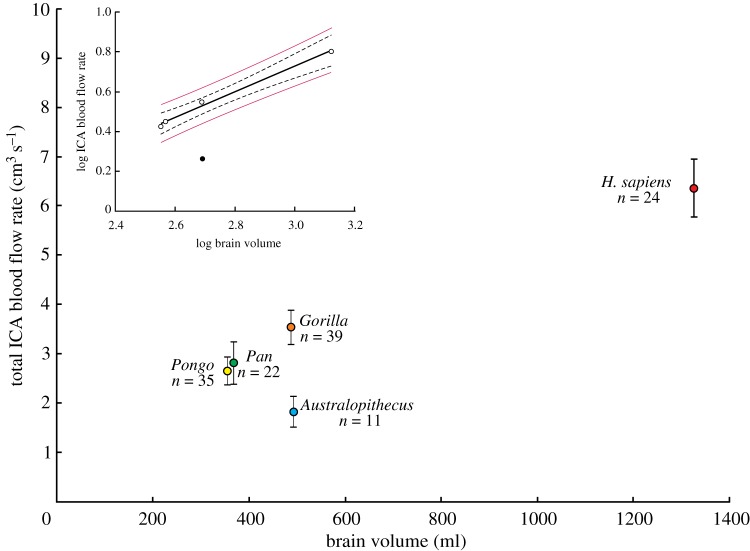
Because two internal carotid arteries (ICAs) enter the brain, all reported values of ${\dot{Q}}_{\mathrm{ICA}}$ are the sum of flows in the right and left ICAs derived from individual foramina or twice the value calculated from one intact foramen in fossil skulls. ${\dot{Q}}_{\mathrm{ICA}}$ in relation to V_br in recent Pongo, Pan, Gorilla and Homo and in fossil Australopithecus reveal significant differences (figure 1; see electronic supplementary material, table S2 for individual species data). ANOVA and Tukey's multiple comparisons test distinguish all five genera from one another, except between Pongo and Pan (table 1). ${\dot{Q}}_{\mathrm{ICA}}$ in Australopithecus was the lowest of all genera and it sits very far below the 95% confidence belt, and below the 95% prediction limits for a linear regression of log₁₀ ${\dot{Q}}_{\mathrm{ICA}}$ on log₁₀ V_br for the living genera (figure 1 inset). The prediction limits are a statistically rigorous way of testing whether a single point is significantly different from a group [16]. Thus, ${\dot{Q}}_{\mathrm{ICA}}$ is significantly higher in all genera of modern great apes compared to Australopithecus despite equal or smaller V_br.

Figure 1.

Total blood flow rate of both ICAs $({\dot{Q}}_{\mathrm{ICA}}),$ calculated from ICA foramen radius data with the new, ‘empirical equation’ and a wall thickness-to-lumen radius ratio of w = 0.30, in relation to brain volume (V_br) in selected hominids. Means, 95% CI and sample sizes are shown. Inset is the regression of log₁₀ ${\dot{Q}}_{\mathrm{ICA}}$ against log₁₀ V_br for four genera of hominids, namely Pongo, Pan, Gorilla and archaic H. sapiens (unfilled circles), showing that Australopithecus (filled circle) falls well below the regression mean's 95% confidence bands (dashed lines) and 95% prediction limits (thin red lines). See electronic supplementary material, table S2 for statistics of individual species. (Online version in colour.)

Table 1.

Results of ANOVA (F_4,126 = 43, p < 0.0001) and Tukey's post hoc test comparing differences in total blood flow rate of both internal carotid arteries $({\dot{Q}}_{\mathrm{ICA}})$ in four genera of hominids. p-values less than or equal to 0.05 indicate a statistically significant difference.

	Pongo	Pan	Homo	Australopithecus
Gorilla	0.0012	0.036	<0.0001	<0.0001
Pongo		0.98 (n.s.)	<0.0001	0.0123
Pan			<0.0001	0.0066
Homo				<0.0001

(b). Internal carotid artery blood flow rate in fossil hominins and extant haplorhine primates

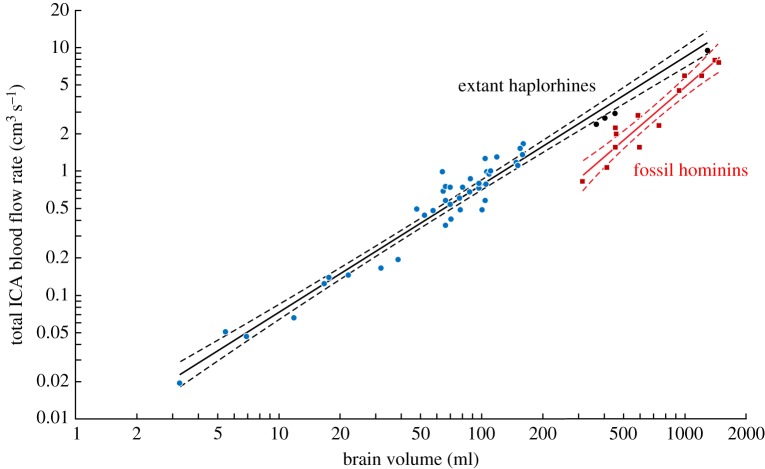
Carotid foramen radius (r_o, cm) and endocranial brain volume (V_br, ml) in fossil hominin skulls, including Ardipithecus ramidus, produce the regression r_o = 0.0048 V_br^{0.57±0.12 CI} (R² = 0.91). New values of ${\dot{Q}}_{\mathrm{ICA}}$ calculated with the empirical equation and w = 0.30 in 44 species of extant haplorhine primates and 12 species of fossil hominins are compared in figure 2 (see electronic supplementary material, table S3 for individual species data). The new regressions are ${\dot{Q}}_{\mathrm{ICA}}=0.0068$ V_br ^{1.03±0.07 CI} (R² = 0.95) for haplorhines and ${\dot{Q}}_{\mathrm{ICA}}=0.00028$ V_br^{1.41±0.30 CI} (R² = 0.91) for hominins. The two exponents are significantly different (F_1,53 = 6.27; p = 0.015), and there is no overlap of data between the two groups (Johnson–Neyman test revealed significant differences in the ${\dot{Q}}_{\mathrm{ICA}}$ data below V_br = 1708 ml, which exceeds all data). The exponent for haplorhines is not significantly different from 1.0, meaning that ${\dot{Q}}_{\mathrm{ICA}}$ is directly proportional to V_br. By contrast, the extremely high exponent for hominins shows that V_br increased 4.7-fold, whereas ${\dot{Q}}_{\mathrm{ICA}}$ increased 9.3-fold, between Ardipithecus and H. sapiens, revealing an approximately twofold increase in volume-specific perfusion rate of the neural tissue across hominin evolution.

Figure 2.

Total blood flow rate of both ICAs $({\dot{Q}}_{\mathrm{ICA}})$ in relation to brain volume (V_br). ${\dot{Q}}_{\mathrm{ICA}}$ is calculated from ICA foramen radius data with a wall thickness-to-lumen radius ratio (w) of 0.30 and the new empirical equation. The regression equation for 44 species of extant haplorhine primates (blue and black circles) is ${\dot{Q}}_{\mathrm{ICA}}=0.0068$ V_br ^{1.03±0.07 CI} (R² = 0.95), and that for 12 species of fossil hominins (red squares) is ${\dot{Q}}_{\mathrm{ICA}}=0.00028$ V_br^{1.41±0.30 CI} (R² = 0.91). Solid lines are the regression means; dashed lines are 95% confidence bands. Black circles are Pongo, Pan, Gorilla and H. sapiens. See electronic supplementary material, table S3 for data derived from [6,13,15]. (Online version in colour.)

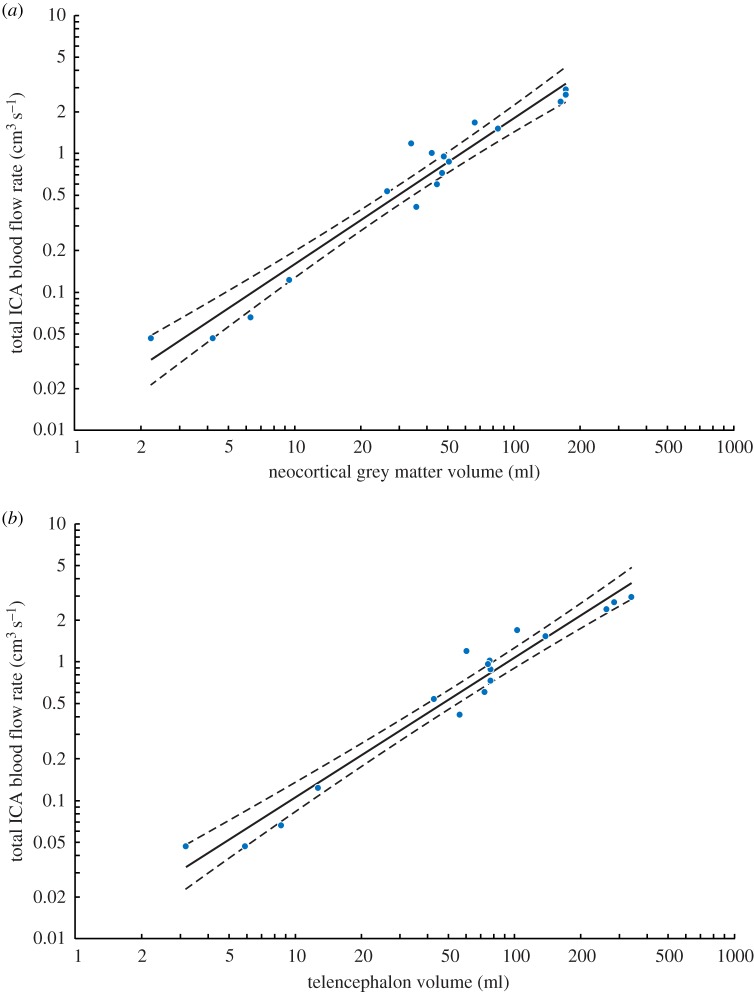
Recent analyses of primate regional brain anatomy, including the volumes of neocortical grey matter (V_grey) and telencephalon (V_tele), are now available [17–19] (electronic supplementary material, table S4). These data include 17 species of extant haplorhine primates for which we have ICA foramen radius [6,13]. Assuming w = 0.30 and using the empirical equation, we find ${\dot{Q}}_{\mathrm{ICA}}=0.0135$ V_grey ^{1.06±0.14 CI} (R² = 0.95) (figure 3a) and ${\dot{Q}}_{\mathrm{ICA}}=0.0103$ V_tele ^{1.01±0.12 CI} (R² = 0.96) (figure 3b). Both exponents are not significantly different from 1.0. We also have ICA foramen sizes for 26 species of haplorhine primates and 15 species of strepsirrhine primates in which total volume of the neocortex (V_neo), including white and grey matter, has been measured [20,21] (electronic supplementary material, table S5). The regressions for these species are ${\dot{Q}}_{\mathrm{ICA}}=0.0062$ V_neo ^{1.12±0.15 CI} (R² = 0.91) for haplorhines and ${\dot{Q}}_{\mathrm{ICA}}=0.0012$ V_neo ^{0.96±0.42 CI} (R² = 0.66) for strepsirrhines (electronic supplementary material, figure S1). The regression for strepsirrhines is not particularly tight, so the exponent is not significantly different from the haplorhines (F_1,37 = 0.692; p = 0.41), but the elevation is significantly lower in strepsirrhines (F_1,38 = 74.18; p < 0.0001). Strepsirrhine primates have reduced ICA and a different pattern of brain perfusion [22], which explains low ${\dot{Q}}_{\mathrm{ICA}}$ found in this and previous studies [6]. Therefore, we consider only haplorhines, in which only the internal carotid and vertebral arteries supply the brain. The scaling of ICA blood flow rate in haplorhine primates in relation to different measures of total or partial brain volumes show exponents not significantly different from 1.0, but significantly higher than 0.86, the exponent apparent in mammals in general [4,5].

Figure 3.

Total blood flow rate of both ICAs $({\dot{Q}}_{\mathrm{ICA}})$ in relation to (a) neocortical grey matter volume (V_grey) and (b) telencephalon volume (V_tele) in 17 species of extant haplorhine primates. ${\dot{Q}}_{\mathrm{ICA}}$ values calculated with the new empirical equation and w = 0.30 from ICA foramen radii in [6,13]. Volume data are from [17], which include data from [18,19]; duplicate or triplicate data were averaged to obtain one point for each species. The equations are, ${\dot{Q}}_{\mathrm{ICA}}=0.0135$ V_grey^{1.06±0.14 CI} (R² = 0.95) and ${\dot{Q}}_{\mathrm{ICA}}=0.0103$ V_tele^{1.01±0.12 CI} (R² = 0.96). Solid line is the regression mean; dashed lines represent the 95% confidence bands. See electronic supplementary material, table S4 for individual data. (Online version in colour.)

3. Discussion

The major point of this paper is a comparison of blood flow rate in the internal carotid arteries $({\dot{Q}}_{\mathrm{ICA}})$ of recent great apes and extinct species of Australopithecus, as assessed with a new, empirical method from the size of the carotid foramina in the skull. It was unexpected to find that ${\dot{Q}}_{\mathrm{ICA}}$ was significantly higher in recent great apes (figure 1), because the ICAs are the major blood supply to the cerebral cortex, and the cognitive ability of Australopithecus is considered superior to that of great apes [23,24]. To understand the basis of this finding, §3(a) considers the sources of brain blood flow and how they relate to brain volume among extant haplorhine primates, including the roles of the ICAs and the vertebral arteries (VAs). Next, §3(b) follows with the implications of the great difference between the scaling of ${\dot{Q}}_{\mathrm{ICA}}$ with brain volume between extant haplorhines and extinct hominins, including the role of the VAs in total brain perfusion. Finally, §3(c) discusses the connection between brain perfusion, brain MR and cognitive ability in great apes and Australopithecus.

(a). Brain perfusion in extant haplorhine primates

The entire brain of haplorhine primates is supplied by two paired arteries, the ICAs and VAs. These arteries communicate with each other in the circle of Willis (cerebral arterial circle) through the paired posterior communicating arteries. In humans, the ICAs supply all of the anterior and middle cerebral arteries, while the VAs supply mainly the arteries of the upper spinal cord, brainstem and cerebellum. Supply to the posterior cerebral arteries is shared. A meta-analysis of 19 studies of normal human cephalic circulation shows that the sum of the flow rates in three paired cerebral arteries is 9.2 cm³ s⁻¹ and the sum of the flow rates in both ICAs is 8.1 cm³ s⁻¹ [25]. Thus, the ICAs account for 88%, and the VAs account for 12%, of total cerebral perfusion.

Although the ICAs supply approximately 75%, and the VAs 25%, of total brain perfusion $({\dot{Q}}_{\mathrm{TOTAL}})$ in humans [25–31], these proportions approach each other in extant haplorhine species with smaller brains until they become approximately equal in brains smaller than 10 ml. (See the electronic supplementary material, including figure S5, for a detailed analysis of the roles of the VAs and ICAs in extant haplorhines.) Allometrically, these relationships manifest as divergent exponents: ${\dot{Q}}_{\mathrm{ICA}}=0.0065$ V_br^1.01, ${\dot{Q}}_{\mathrm{VA}}=0.0083$ V_br^0.84 and ${\dot{Q}}_{\mathrm{TOTAL}}=0.0143$ V_br^0.95. Thus the scaling of ${\dot{Q}}_{\mathrm{ICA}}$ against V_br is isometric (directly proportional), but the scaling of ${\dot{Q}}_{\mathrm{VA}}$ against V_br is hypoallometric (less than proportional). Not only is the ${\dot{Q}}_{\mathrm{ICA}}$ exponent in haplorhines near 1.0 in relation to brain volume (figure 2), it is also near 1.0 in relation to neocortical grey matter volume (figure 3a), telencephalon volume (figure 3b) and neocortex volume (electronic supplementary material, figure S1). The exponents are all statistically significantly higher than 0.86 expected for total brain perfusion in mammals in general [4,5]. The difference between exponents of 1.0 and 0.86 is not trivial. Over the range of V_br of the haplorhines, volume-specific ${\dot{Q}}_{\mathrm{ICA}}$ does not change at all with an exponent of 1.0, but drops to 50% with an exponent of 0.86.

The neocortex appears to be a constant fraction of total brain volume in primates, but the degree of superficial folding (gyrification) of the cortical surface area increases disproportionately [32,33]. Increased cortical surface area has been linked to intelligence [34]. Haplorhine ${\dot{Q}}_{\mathrm{ICA}}$ increases in proportion to neocortical grey matter volume (figure 3a), telencephalon volume (figure 3b) and neocortex volume (electronic supplementary material, figure S1). Most of the volume increase in the cerebral cortex is due to an increase in the communication network (white matter) rather than the cognitive tissue, rich in synapses (grey matter). In haplorhine primates, the volume of grey matter increases with total brain volume to the 0.985 power (nearly linearly proportional), but white matter increases with the 1.241 power [33]. Our analysis shows that ${\dot{Q}}_{\mathrm{ICA}}$ scales with V_br^1.03 (figure 2), corresponding well to the scaling of neocortical grey matter but not white matter. This seems reasonable because grey matter has an approximately 80-fold higher density of synapses [35], fourfold greater volume-specific energy use [36] and two to four times greater volume-specific perfusion rate than white matter [37,38]. Thus, grey matter may have a greater influence on the scaling of ${\dot{Q}}_{\mathrm{ICA}}$ against V_br than white matter. Because cognition is largely associated with synapses in grey matter, it follows that blood flow rate is related to cognition.

Because both body mass and V_br tended to increase during primate evolution, and because ${\dot{Q}}_{\mathrm{ICA}}$ is proportional to V_br, the allometry of ${\dot{Q}}_{\mathrm{ICA}}$ and V_br in living species (figure 2) may roughly reflect their evolutionary history. The Haplorhini split from the Strepsirrhini around 66–69 Ma; within the Haplorhini, the Platyrrhini (New World monkeys) split from the Catarrhini (Old World monkeys and apes) around 46 Ma; within the Catarrhini, the Hominoidea (apes) arose around 32 Ma and the Hylobatidae (lesser apes and gibbons) split from the Hominidae (great apes) around 19 Ma [39]. Thus, the smaller-brained platyrrhines tend to have the oldest lineages, and larger-brained hominids represent the youngest. The progression of increasing perfusion correlates with the evolutionary order of ‘information processing capacity’ among haplorhines as indicated not only from anatomy and physiology (e.g. number of neurons, degree of connectivity, axonal conduction velocity), but also from behavioural measures (e.g. capacity for memory, mental manipulation, social interactions) [24,40]. Unfortunately, we have no data on ICA foramen size from fossil non-hominin haplorhines to test this idea.

(b). Internal carotid artery blood flow rate in extant great apes and Australopithecus

The present study indicates that the extant non-human great apes have a significantly greater absolute cerebral blood flow, and by inference metabolic demand, than ancestral Australopithecus (figure 1). Although Gorilla has V_br values similar to Australopithecus, Gorilla ${\dot{Q}}_{\mathrm{ICA}}$ is approximately twice as high. Pongo and Pan have smaller V_br than Australopithecus, but significantly higher ${\dot{Q}}_{\mathrm{ICA}}$. The difference is also apparent in ${\dot{Q}}_{\mathrm{ICA}}$ scaling of extant haplorhine primates and fossil hominins from the literature (figure 2), where the great apes (black points) are higher than those for hominins. The implication of these disparate results is that the human brain appears to be a proportionately scaled up (nearly isometric exponent of 1.03) version of a recent haplorhine primate brain, something noted previously [41–43], but the allometry of ${\dot{Q}}_{\mathrm{ICA}}$ in relation to V_br among the fossil hominins is very much steeper (hyperallometric exponent of 1.41).

The hominin exponent is similar to 1.45 calculated formerly from the shear stress equation [15]. The major determinate of ${\dot{Q}}_{\mathrm{ICA}}$ is the extremely high allometric exponent of ICA foramen radius, which scales with V_br^0.57. If the skull increased in size while maintaining the same shape (isometric scaling), ICA radius would scale with V_br^0.33. If brain perfusion is assumed to scale with hypoallometry (e.g. V_br ^0.86), then foramen radius would scale with an exponent even less than 0.33. Therefore, the high exponent for hominin ICA foramen radius can mean nothing but an extraordinary increase in brain perfusion by the ICAs.

Although the ICAs appear to be associated with the cerebral cortex and cognition, it is reasonable to ask whether the VAs could have supplied significant blood to the cerebral cortex of Australopithecus to compensate for a hypothetically low ICA component. Assuming that the 500 ml brain of Australopithecus conformed to the pattern set by extant haplorhines, the ICAs would contribute 69%, and the VAs 31%, of total perfusion (see the equations in §3(a) of the discussion and electronic supplementary material, figure S5). So even if ${\dot{Q}}_{\mathrm{VA}}=0.82\hspace{0.17em}\text{c}{\text{m}}^3\hspace{0.17em}{\text{s}}^{-1}$ were added to the mean of ${\dot{Q}}_{\mathrm{ICA}}=1.84\hspace{0.17em}\text{c}{\text{m}}^3\hspace{0.17em}{\text{s}}^{-1}$ for Australopithecus, the total 2.66 cm³ s⁻¹ would still be less than ${\dot{Q}}_{\mathrm{ICA}}=3.54\hspace{0.17em}\text{c}{\text{m}}^3\hspace{0.17em}{\text{s}}^{-1}$ in the gorilla's ICAs alone, without any flow from its VAs (figure 1). It has been estimated from the carotid and transverse foramina of Gorilla that ${\dot{Q}}_{\mathrm{VA}}$ may be higher than ${\dot{Q}}_{\mathrm{ICA}}$ [13]. Therefore, Australopithecus cannot reach the level of total brain perfusion in Gorilla with scaling derived from extant haplorhine primates.

Moreover, it is difficult to reconcile the extremely high exponent for ${\dot{Q}}_{\mathrm{ICA}}$ in hominins (1.41) with any expected scaling of ${\dot{Q}}_{\mathrm{TOTAL}}$. Assuming an isometric exponent for ${\dot{Q}}_{\mathrm{TOTAL}}$ (1.0), the exponent for ${\dot{Q}}_{\mathrm{VA}}$ would need to be very much lower, approximately 0.36. (See the electronic supplementary material, including figure S6, for a model of the roles of the VAs and ICAs in hominins.) Under these assumptions, the 500 ml brain of Australopithecus would receive 47% of the blood from the ICAs and 53% from the VAs. Such a scenario would require the size of the VAs to decrease relative to the size of the brain during the evolution from Australopithecus to H. sapiens. This is possible, but cannot be easily tested, because, unlike the carotid foramen that contains the ICA and little else, the transverse foramen on the cervical vertebra passes not only the VA but also a large venous plexus [12]. If a cervical vertebra from Australopithecus could be analysed with certain assumptions [13], however, it may shed light on this question. For now, we hypothesize that the high scaling of ${\dot{Q}}_{\mathrm{ICA}}$ is more likely to be associated with rapid increase in cortical MR during hominin evolution than a greater role of the VAs in Australopithecus.

(c). Implications of internal carotid artery brain perfusion

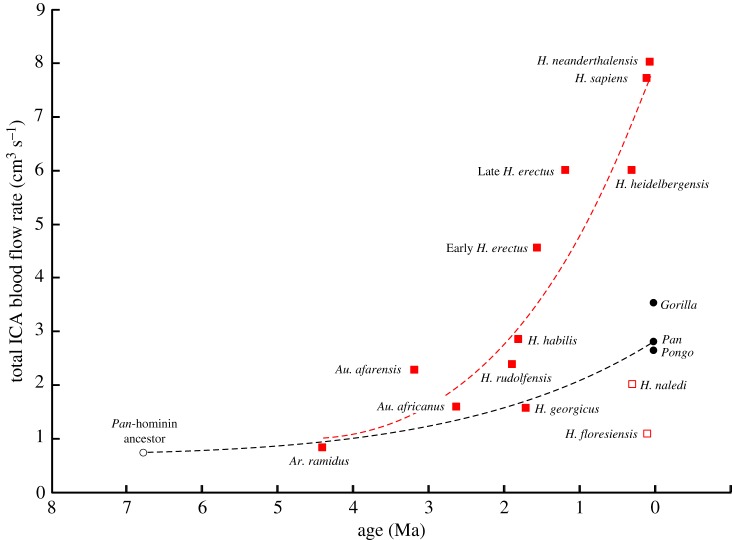
The results cast doubt over the notion that the neurological and cognitive traits of recent great apes adequately represent the abilities of Australopithecus species. The use of modern primates as a proxy for hominin evolution may have prevailed historically due to similar brain sizes, with the great apes between 300 and 500 ml, compared with 315 ml in Ardipithecus [44] and 475–500 ml in Australopithecus (electronic supplementary material, table S2). The fact that ${\dot{Q}}_{\mathrm{ICA}}$ is up to twofold higher in Gorilla than in Australopithecus is surprising, given that the australopithecines have been placed between great apes and humans on the basis of several measures relating to brain and intelligence [23,24]. Apparently, the underlying assumptions that cognitive ability, brain MR and blood flow rate all scale with brain size in parallel, and that the patterns evident in living haplorhine primates apply to hominins, are incorrect. Indeed, this study shows that the scaling of ${\dot{Q}}_{\mathrm{ICA}}$ follows quite different allometries in haplorhines and hominins (figure 2). Chimpanzee and human lineages diverged around 6–7.5 Ma [39,45,46], so it is likely that ${\dot{Q}}_{\mathrm{ICA}}$ was equal or lower than we measure in Ardipithecus from 4.4 Ma [47]. From that point, recent chimpanzees ended up higher than Australopithecus and modern humans even higher (figure 4). The trajectories of the older lineages of gorillas and orangutans were apparently also rising.

Figure 4.

Total blood flow rate of both ICAs $({\dot{Q}}_{\mathrm{ICA}})$ in relation to age of hominins (red squares) and recent great apes (black circles). Two unusual hominin species (open squares) are Homo naledi and Homo floresiensis, which are excluded from the third-order polynomial curve used to visualize hominin evolution. A hypothetical common ancestor of Pan–Homo sapiens lineages is proposed at 6.75 Ma [39,45,46] with ${\dot{Q}}_{\mathrm{ICA}}$ similar to that of A. ramidus. The evolutionary trajectories from this ancestor are both upward, but of different magnitudes. (Online version in colour.)

Because the ICAs supply almost all of the cerebrum in humans, we assume that, in addition to increasing brain size, the rise in volume-specific ${\dot{Q}}_{\mathrm{ICA}}$ in the hominins traces a rapid evolutionary rise in the metabolic intensity of the neural tissue. This would correlate with an increase in cognitive ability from Australopithecus, as the early representatives of hominin evolution, to modern H. sapiens, that exceeded that determined by brain volume alone. Such development is not inevitable, however, as evidenced by similar values of ${\dot{Q}}_{\mathrm{ICA}}$ and V_br in H. naledi and H. floresiensis to those in Australopithecus species, despite approximately 2.5 million years between them [48,49] (figure 4). It is thought that H. naledi and H. floresiensis branched from ancient small-brained Homo ancestors, possibly independently [46,50,51]. These two recent species raise problems in relating cognitive ability to measures of brain perfusion rate as well as brain size [23].

The high cerebral perfusion in recent great apes may relate to living in social family groups [52]. Presumably, a relatively high cognitive capacity is required to interpret family interrelationships and hierarchies and to partake in social behaviours that would render individuals fit for mating and territory defence [53,54]. Such social drivers for cognitive evolution are often attributed to Australopithecus and early Homo, but could also be ascribed to the great apes that likewise exhibit complex family cooperation. This would have contributed to increases in ancestral great ape cerebral metabolic and ICA blood flow rates, in a similar way to the Homo predecessors. It can be argued that the cognitive capacity that favoured the survival of the genus Homo equally facilitated the survival of ancestral great apes. The requirement for cerebral specialisation may have ultimately contributed to modern great apes being more cognitively advanced than Australopithecus.

4. Methods

New measurements were made from 82 skulls from museum collections that represent all six species of non-human great apes (see electronic supplementary material for specimen data). Endocranial volume (V_br; ml) was measured by sealing large foramina in the skull with cotton wool and filling the brain case with rice or plastic beads to the level of the foramen magnum, gently agitating to achieve uniform filling and compaction, and then emptying into a graduated cylinder and recording the compacted volume. Because the carotid artery occupies the foramen lumen almost entirely, the outer radius of the artery is defined by the radius of the foramen (r_o; cm). With recently defined methods [55], the radii of the left and right carotid foramina were measured from photographs, captured orthogonal to the opening, together with a 0.5 mm graduated scale, positioned at the level of the opening. An ellipse was fitted to the inside of the foramen opening with ImageJ (open source, imagej.net), and r_o was taken as the radius of a perfect circle with the measured elliptical area.

The literature was searched for data on lumen radius (r_i, cm) and wall thickness of normally pressurized internal carotid and common carotid arteries. Wall thickness was taken as the difference between outer radius (r_o) and lumen radius (r_i), and was expressed as the ratio w = (r_o – r_i)/r_i. The mean value of w was 0.30, which was used in the calculations (see electronic supplementary material, table S1 for data).

Blood flow rate in the ICA was calculated from carotid foramen radius (r_o) by first calculating lumen radius [r_i = r_o/(1 + w)] and then applying the new empirical equation $({\dot{Q}}_{\mathrm{ICA}}=155r_i^{2.49})$. The results of this approach are slightly better statistically, but are similar to those from the Poiseuille-derived shear stress equation used previously (see electronic supplementary material for detailed comparison of computational approaches and their results).

The new calculations were applied to r_o and V_br data from previous studies of 44 species of extant haplorhine primates [6,13], and 12 species of hominin (two points for early and late Homo erectus), including eight specimens attributed to Australopithecus africanus and three attributed to A. afarensis [43]. A new r_o datum for Ardipithecus ramidus was obtained by measuring three scaled figures of the basal skull and taking the mean V_br from the publications [44,56]. In addition, ${\dot{Q}}_{\mathrm{ICA}}$ was compared allometrically with the volumes of neocortical grey matter, telencephalon and total neocortex from recent publications [17–19].

Data are presented as means with 95% confidence intervals (CIs). Allometric data were log₁₀-transformed prior to fitting ordinary least-squares linear regressions [57,58] and performing ANCOVA to test for significant differences in scaling exponents and elevations [59]. If the exponents differed significantly, differences in the elevation could not be evaluated, so the Johnson–Neyman technique defined the region in which data from individual species were not significantly different [60]. Statistics on mean data also included ANOVA and Tukey's multiple comparisons test.

Data accessibility

All of the data are in the electronic supplementary material section.

Authors' contributions

R.S.S.: conceptualization; R.S.S., V.B., E.P.S., Q.H., T.J.N., P.C.C., B.Z. and C.V.M.: data gathering; R.S.S., Q.H., V.B. and E.P.S.: formal analysis; R.S.S. and V.B.: writing—original draft; R.S.S., E.P.S., Q.H., T.J.N., P.C.C., B.Z. and C.V.M.: writing—review and editing; R.S.S.: project administration.

Competing interests

We declare we have no competing interests.

Funding

This project ‘Design of the cardiovascular system of living and fossil vertebrates' was supported by the Australian Research Council (grant no. DP 170104952).