UVA and Seasonal Patterning of 56 370 Myocardial Infarctions Across Scotland, 2000–2011

Abstract

Background

Myocardial infarction exhibits seasonal patterning, with higher amplitude at increased latitude. Epidemiological evidence suggests that sunlight is protective against cardiovascular disease, independent of ambient temperature, but ultraviolet B–mediated vitamin D production has been discounted as causal. We aimed to determine whether ultraviolet A is associated with the seasonal patterning of myocardial infarction.

Methods and Results

Routine hospitalization data were used to determine monthly incidence of myocardial infarction in Scotland between 2000 and 2011. Small‐area–level aggregated data were obtained on ambient temperature from the Meteorological Office and ultraviolet A and ultraviolet B irradiance from NASA satellites. Autoregressive distributed lag models were run for ultraviolet A and myocardial infarction, including adjustment for ambient temperature and ultraviolet B. Monthly incidence of myocardial infarction displayed winter peaks and summer troughs superimposed on the underlying trend, with a mean amplitude of 0.31 (95% CI: 0.21, 0.41) myocardial infarctions per 100 000 population per month. Ultraviolet A exposure was inversely associated with myocardial infarction independent of ambient temperature (coefficient, −0.05; 95% CI, −0.09, −0.01; P=0.015) and ultraviolet B UVB (coefficient, −0.05; 95% CI, −0.09, −0.02; P=0.004).

Conclusions

Further research is required to explore whether an ultraviolet‐mediated mechanism different to vitamin D, such as nitric oxide–mediated vasodilatation, may play a causal role in the seasonal and geographical patterning of myocardial infarction.

Clinical Perspective

What Is New?

• Seasonal patterning of myocardial infarction (winter peaks and summer troughs) suggests an environmental factor.

• Some evidence exists for an association with solar radiation, but a causal role for ultraviolet B (through vitamin D) has been discredited.

• Our study showed an association between ultraviolet A (which impacts on nitric oxide–mediated vasodilatation) and myocardial infarction that is independent of temperature and ultraviolet B.

What Are the Clinical Implications?

• Winter peaks in myocardial infarction represent preventable morbidity and mortality and a preventable burden on health services.

• Trials should be conducted on whether interventions, such as phototherapy boxes or supplements, could safely and effectively reduce winter peaks and thereby overall incidence.

Introduction

Cardiovascular disease incidence and mortality demonstrate seasonal patterning, with winter peaks in both hemispheres and higher amplitude further from the equator.1, 2, 3 Earlier studies focused on ambient temperature, which may act directly on cardiovascular physiology or biomarkers or be mediated by seasonal patterning of lifestyle.1, 4, 5, 6 However, other environmental exposures, such as solar radiation, may also play a role. A number of studies have demonstrated associations between solar radiation and cardiovascular disease. In a Swedish cohort, patients with malignant melanoma who avoided sun exposure had significantly reduced life expectancy, explained largely by their increased risk of cardiovascular disease.7 Similarly, a Danish case‐control study reported that both melanoma and nonmelanoma skin cancers were associated with reduced risk of myocardial infarction.8 Finally, a Swedish general population cohort study demonstrated an inverse linear relationship between sun‐exposure score and incidence of myocardial infarction, with the effect size associated with low sun exposure comparable with that of smoking.9

Sunlight contains a spectrum of wavelengths, including ultraviolet (UV) radiation. UV levels are strongly correlated with season and therefore with other seasonally patterned exposures, such as ambient temperature. However, superimposed on the annual cycle in UV radiation, there is an 11‐year solar cycle over which the number of sunspots changes with the sun's oscillatory magnetic field. As a result, UV levels vary between years. Hence, associations with UV radiation can be disentangled from associations with other seasonally patterned phenomena, such as ambient temperature. Furthermore, while ultraviolet A (UVA) radiation covers longer wavelengths (321–400 nm) and is not absorbed by the ozone layer, ultraviolet B (UVB) covers shorter wavelengths (280–315 nm) and therefore is mostly absorbed before reaching the earth's surface.10 The actual amount of UVB reaching the earth's surface varies greatly with weather conditions, such as cloud cover.11 Therefore, while levels of UVA and UVB are correlated, there is sufficient variation to determine whether associations with UV radiation are driven by UVA or UVB.

UVA penetrates the skin more deeply than UVB, and they produce different biological and physiological responses.12 To date, studies on cardiovascular disease have focused on the possible role of UVB in promoting production of vitamin D. However, Mendelian randomization studies and clinical trials refute a causal role for vitamin D.13, 14, 15, 16 Therefore, the aim of this study was to determine whether UVA was associated with the seasonal patterning of acute myocardial infarction independent of ambient temperature and UVB.

Methods

The study involved secondary analysis of a data set for which the authors are not the data custodians. Because of the sensitive nature of the data used in this study, requests to access the data set from qualified researchers trained in information governance may be sent to eDRIS (Electronic Data Research and Innovation Service) at NSS.EDRIS@nhs.net. The East of Scotland Research Ethics Service (REC reference 16/ES/0112; IRAS project ID 206758) provided National Health Service ethics approval for eDRIS linked data linkage projects involving secondary use of anonymized data extracts without written consent, and approval for this study was granted by the Public Benefit and Privacy Panel (reference 1617‐0056).

Data Sources, Inclusion Criteria, and Definitions

We used the Scottish Morbidity Record 01 (SMR01) database for the period 1990–2011 inclusive to ascertain emergency admissions to hospital for myocardial infarction, defined as an International Classification of Diseases code 410.X (International Classification of Diseases, Ninth Revision; ICD‐9) or I21.X (International Classification of Diseases, Tenth Revision; ICD‐10) recorded in the principal position. We used retrospective record linkage to exclude admissions preceded by a hospital admission for myocardial infarction in the previous 10 years. Hence, the study cohort comprised incident hospitalized myocardial infarctions between 2000 and 2011 inclusive. Both fatal and nonfatal myocardial infarctions were included. Mid‐year population counts were obtained from the National Records of Scotland website (https://www.nrscotland.gov.uk/statistics-and-data/statistics/statistics-by-theme/population/population-estimates/mid-year-population-estimates) and linearly interpolated and extrapolated to provide population counts for each month over the study years. The data on incident myocardial infarctions and general population were used to derive monthly incidence rate per 100 000 population over the study period, standardized to a common month length using the formula: count×100 000 × 365.25/12×population×days per month.

Mean monthly ambient temperatures for Scotland were obtained from the Meteorological Office (https://www.metoffice.gov.uk/pub/data/weather/uk/climate/datasets/Tmean/date/Scotland.txt), which has developed models that provide spatially detailed estimates of past weather patterns at 5‐km² resolution across the country. The project to develop these models ended in 2011, and we used the latest available data. Global 5‐km UV radiance‐irradiance data were produced by the Japan Aerospace Exploration Agency (JAXA) using measurements from the MODIS (Moderate Resolution Imaging Spectroradiometer) instrument on board NASA's aqua and terra satellites. Downward irradiance values, combining direct and diffuse radiation on a horizontal plane, for UVA and UVB were available from the publicly accessible JAXA website (ftp://apollo.eorc.jaxa.jp/pub/JASMES/Global_05km/) at a daily resolution from 2000 for each latitude and longitude on earth. We obtained data relating to the Great Britain bounding box (48°N to 63°N; −11°W to 4°E). The points were then interpolated, by inverse weighted distance, to raster images. The rasters were projected to the OSGB 1936/British National Grid. Finally, we took the northing and easting centroids of each postcode unit in Scotland (Ordnance Survey Code‐Point ver 2017.4.0) and used these to extract the UVA and UVB values for those grid squares covering residential areas in Scotland. Scotland‐wide monthly means were then calculated resulting in a monthly time series of UVA and UVB irradiance values measured in W/m². In order to be consistent with other epidemiological studies, these values were converted to kJ/m² by applying the formula: ×86 400 (seconds in the day)/1000.

Statistical Analyses

We estimated mean amplitude and peak phase for the data using cosinor models, allowing for variable annual phase and amplitude, with 5000 simulations and a burn‐in of 1000. Parameters of the model were adjusted using the correlogram of the residuals from the fitted model in order to model as much seasonal variation as possible.

Multicollinearity between UVA, UVB, and temperature was investigated using Belsley's method,17 which uses the concept of condition indices of a matrix to assess whether collinearity among a set of regressors is likely to have a deleterious impact on estimated regression coefficients and standard errors. Simulation studies run by Belsley suggest that condition indices in the range 5 to 10 represent a weak dependence among the regressors and indices from 30 to 100 a strong dependency.

In order to explore the short‐ and long‐run associations between solar radiation and myocardial infarction incidence, we used the autoregressive distributed lag (ARDL) modeling methodology of Pesaran et al.18 ARDL uses the econometric concept of cointegration to separate out short‐term associations among variables, characterized by the inclusion of time lags in effect and differences, from long‐term relationships which do not involve lags or differences. Using this technique avoids the problem of “spurious regression” where variables in a time‐series model appear to be highly statistically significant, when, in fact, they are not. The incorrect conclusion arises because of the presence of trend in the data, which is the main driver of the statistical significance. In addition to separating out the short‐ and long‐term relationships among the variables, the ARDL methodology also provides an estimate of how quickly the variables return to their long‐run underlying relationship after any event, or disruption, that may have knocked them off this path. The readjustment is called the error correction mechanism and is estimated along with the other regression coefficients in the model.

To identify an appropriate ARDL, we used an automatic estimation procedure based on the Bayesian information criterion. This procedure evaluates a number of models with different lag lengths for the dependent and independent variables and selects the model with the lowest Bayesian information criterion statistic. We included 2 dummy variables for the implementation of the Scottish smoke‐free legislation, in March 2006, and the introduction of a high‐sensitivity troponin assay for diagnosis of myocardial infarction, in October 2010, to allow for possible structural breaks in the series. We then tested the residuals of the best‐fitting model for any remaining serial correlation using Breusch–Godfrey–Lagrange multiplier tests.

To check model stability over time, we used the recursive residuals, CUSUM and CUSUMSQ graphical tests. The underlying model was then subjected to the Bounds testing procedure of Pesaran et al18 to identify appropriate cointegrating relationships and long‐run measures of association. Myocardial infarction incidence, sunshine hours, UVA, and UVB were logged before modeling, while an inverse hyperbolic sine transformation was applied to ambient temperature to mitigate the presence of zero and negative values. We ran 3 sets of models, including: UVA and ambient temperature; UVB and ambient temperature; and UVA, UVB, and ambient temperature. Initial data cleaning was done in Stata software (14.1 MP, StataCorp 2015; StataCorp LP, College Station, TX) and statistical analyses in MATLAB (The Mathworks, Inc., Natick, MA), EViews 10.1 (IHS Global Inc, London, UK), and R software (version 3.5.1; R Foundation for Statistical Computing, Vienna, Austria).

Results

Over the 12‐year study period, there were 56 370 incident admissions for myocardial infarction across Scotland. This equated to a mean annual incidence of 7.60 myocardial infarctions per 100 000 population. The monthly incidence of myocardial infarction demonstrated a seasonal pattern, with a February peak, superimposed on an underlying trend whereby the incidence fell gently from 2000 to 2009 and then increased from 2010, coinciding with the introduction of high‐sensitivity troponin assays for the diagnosis of myocardial infarction (Figure 1). The mean amplitude over the study period was 0.31 (95% CI, 0.21, 0.41) myocardial infarctions per 100 000 population per month. UVA, UVB, and ambient temperature all demonstrated seasonal patterns with summer peaks (Figure 2A through 2C). Over the study period, the ratio of UVA to UVB ranged from 49 to 748, with a mean of 135. The Pearson correlation coefficient between UVA and UVB was 0.98. However, Belsley's procedure produced a condition index of 8.3, implying weak dependence between the variables. This result suggests that the inclusion of both variables in a regression model would not result in problems with multicollinearity.

Figure 1.

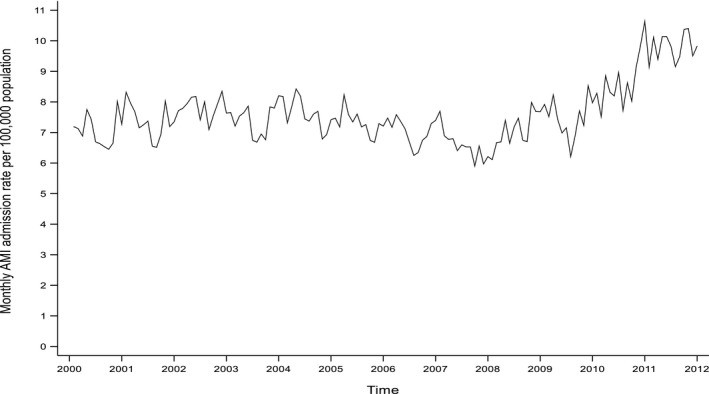
Crude monthly incidence of acute myocardial infarction (AMI) admissions.

Figure 2.

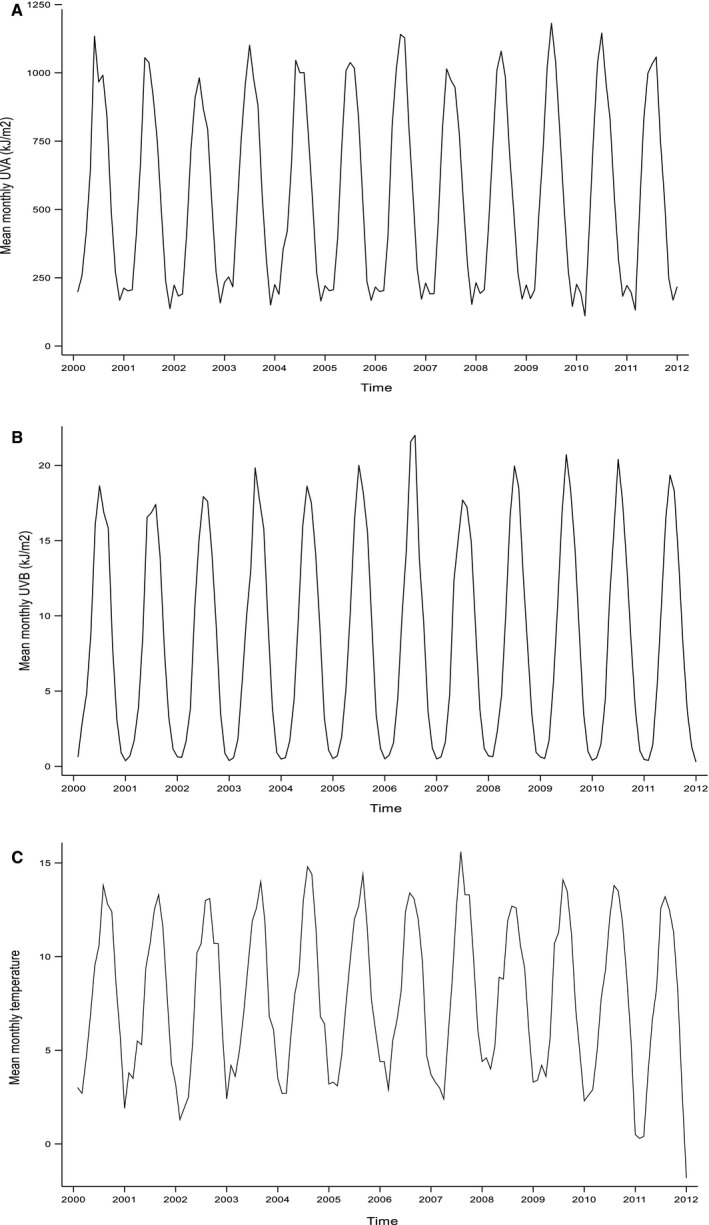
Monthly environmental exposure measurements. A, Ultraviolet A irradiance. (B) Ultraviolet B irradiance. (C) Ambient temperature.

In the ADRL model, UVA exposure was inversely associated with incidence of myocardial infarction in the following month, independent of ambient temperature (coefficient, −0.05; 95% CI, −0.09, −0.01; P=0.015) and remained so when UVB was added to the model (coefficient, −0.05; 95% CI, −0.09, −0.02; P=0.004; Table 1). A coefficient of −0.05 can be interpreted as a 1% higher ambient temperature being associated with a 0.05% lower incidence of myocardial infarction or a 10% higher ambient temperature being associated with a 0.5% lower incidence. In contrast to UVA, following adjustment for ambient temperature, the association between UVB and myocardial infarction just failed to reach statistical significance (P=0.067) and became nonsignificant when UVA was added to the model (P=0.820). All 3 models were a good fit with adjusted R ² values of 74% to 76%, and all models passed the test for white‐noise residuals (Figure 3). On subgroup analysis, the independent association with UVA was observed both aged >60 years (coefficient, −0.06; 95% CI, −0.10, −0.02; P=0.006) and ≤60 years (coefficient, −0.03; 95% CI, −0.06, −0.00; P=0.040). It was present in men (coefficient, −0.06; 95% CI, −0.10, −0.02; P=0.002), but was nonsignificant in women.

Table 1.

Autoregressive Distributed Lag Model of the Associations With Incidence of Acute Myocardial Infarction Admissions of Ultraviolet A and Ultraviolet B

	Ultraviolet A			Ultraviolet B			Ultraviolet A and B
	Coefficienta	95% CI	P Value	Coefficienta	95% CI	P Value	Coefficienta	95% CI	P Value
Fitted ADRL models
Log incidence (1‐mo lag)	0.36	0.22 to 0.49	<0.001	0.36	0.23 to 0.49	<0.001	0.35	0.20 to 0.50	<0.001
Log incidence (2‐mo lag)	0.23	0.07 to 0.39	0.006	0.21	0.06 to 0.36	0.007	···	···	···
Log ambient temperature	0.02	0.0002 to 0.0400	0.048	0.02	−0.01 to 0.02	0.843	0.01	−0.01 to 0.03	0.264
Log UVA	−0.01	−0.04 to 0.02	0.603	···	···	···	0.01	−0.03 to 0.04	0.774
Log UVA (1 mo lag)	−0.05	−0.09 to −0.01	0.015	···	···	···	−0.05	−0.09 to −0.02	0.004
Log UVB	···	···		−0.01	−0.03 to 0.001	0.067	0.002	−0.02 to 0.02	0.820
Introduction of smoke‐free legislation	−0.06	−0.11 to −0.01	0.010	−0.06	−0.12 to −0.01	0.031	−0.04	−0.09 to 0.01	0.107
Introduction of high‐sensitivity troponin assays	0.08	0.02 to 0.13	0.006	0.07	0.02 to 0.12	0.008	0.04	−0.02 to 0.10	0.152
Intercept	0.88	0.52 to 1.23	<0.001	0.82	0.47 to 1.17	<0.001	1.30	1.00 to 1.59	<0.001

Regression diagnostics: UVA: adjusted R ², 0.76; F statistic, 46.56; P<0.001; residual Q statistic, P=0.99 at lag 13. UVB: adjusted R ², 0.75; F statistic, 49.36; P<0.001; residual Q statistic, P=0.99 at lag 13. UVA and UVB: adjusted R ², 0.76; F statistic, 42.78; P<0.001; Residual Q statistic, P=0.50 at lag 13. ARDL indicates autoregressive distributed lag; UVA, ultraviolet A; UVB, ultraviolet B.

^aPercent change in myocardial infarction incidence per unit change in exposure variable (1°C for temperature and 1 kJ/m² for UVA/B).

Figure 3.

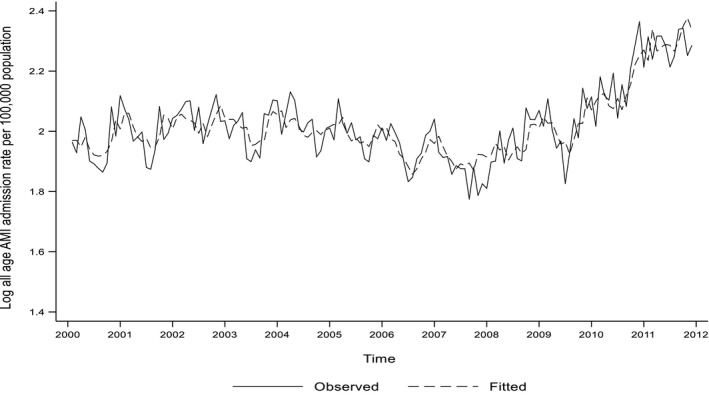
Fitted ARDL model including UVA, UVB, and ambient temperature. AMI indicates acute myocardial infarction; ARDL, autoregressive distributed lag; UVA, ultraviolet A irradiance; UVB, ultraviolet A irradiance.

The long‐run models produced similar results and showed that the associations were quick to adjust to deviations in their long‐run path, with 42% and 43% of the disruption to myocardial infarction incidence corrected within 1 month following changes to UVA and UVB, respectively (Table 2). When both UVA and UVB were included in the same model, the speed of adjustment increased to 63%. Graphs of the CUSUM and CUSUMSQ recursive residuals revealed no instability in the estimated coefficients in any of the models, with all coefficient paths within the 95% CI bounds.

Table 2.

Long‐Run Relationship Between Incidence of Acute Myocardial Infarction Admissions and Ultraviolet A and Ultraviolet B

	Ultraviolet A			Ultraviolet B			Ultraviolet A and B
	Coefficienta	95% CI	P Value	Coefficienta	95% CI	P Value	Coefficienta	95% CI	P Value
Long‐run coefficients
Log ambient temperature	0.04	−0.01 to 0.09	0.087	0.004	−0.03 to 0.04	0.844	0.02	−0.01 to 0.05	0.264
Log UVA	−0.13	−0.23 to −0.03	0.011	···	···	···	−0.08	−0.13 to −0.02	0.008
Log UVB	···	···	···	−0.03	−0.07 to 0.006	0.080	0.003	−0.03 to 0.03	0.821
Intercept	2.09	1.98 to 2.20	<0.001	1.92	1.68 to 2.16	<0.001	1.99	1.81 to 2.18	<0.001
Error correction	−0.42	−0.54 to −0.30	<0.001	−0.43	−0.57 to −0.29	<0.001	−0.65	−0.80 to −0.51	<0.001

UVA indicates ultraviolet A; UVB, ultraviolet B.

^aPercent change in myocardial infarction incidence per unit change in exposure variable (1°C for temperature and 1 kJ/m² for UVA/B).

Discussion

Myocardial infarction and UVA exhibited opposite seasonal patterns with winter and summer peaks, respectively. Year‐on‐year variations in UVA, over and above these seasonal patterns, were associated with myocardial infarction incidence independent of both ambient temperature and UVB. Therefore, we hypothesize that low UVA may be contributing to both the winter peaks and higher overall incidence of myocardial infarction in higher‐latitude countries.

Studies exploring the associations between solar radiation and cardiovascular disease have, so far, focused on vitamin D. Inverse relationships have been demonstrated between 25‐hydroxy vitamin D concentrations and cardiovascular risk factors and disease,19, 20, 21 and because exposure to UVB is the major source of vitamin D, individuals living in higher‐latitude countries commonly experience vitamin D deficiency over winter months.21 However, Mendelian randomization studies do not support a causal link between vitamin D and cardiovascular disease,13 and vitamin D supplementation studies have not been effective at reducing it.14, 15 Therefore, vitamin D may simply be a marker for solar radiation, which is acting through another mechanism. Our finding of an association that was specific to UVA supports this, given that UVA does not promote vitamin D production. A causal role for UVA is biologically plausible. UVA mobilizes nitric oxide in the skin, which then acts on vascular smooth muscle causing vasodilatation,22 resulting in reduced blood pressure, increased heart rate, and reduced peripheral resistance, independent of ambient temperature.23, 24 Hypertension is a major risk factor for cardiovascular disease.25

A strength of this study is the use of unselected, Scotland‐wide data on admissions for myocardial infarction. Cases were ascertained from routine administrative health data. However, these undergo regular quality‐assurance checks to ensure high completeness and accuracy. Given that UV could potentially impact on prognosis as well as occurrence, retrospective linkage was used to ensure that only incident cases were included. Over the period studied, accepted clinical practice was to admit all patients suffering from myocardial infarction; therefore, the data are likely to be complete in relation to nonfatal myocardial infarctions. However, prehospital deaths were not included. Also, we lacked statistical power to undertake subgroup analyses by age and sex. Having data over a 12‐year period (the maximum period with both ambient temperature and UV data) provided sufficient coverage of the solar cycle to distinguish between associations with solar radiation and other seasonally patterned phenomena, as well as sufficient variations in weather to distinguish between associations with UVA and UVB. A further strength of the study is the use of the ARDL cointegration methodology to reveal statistically meaningful long‐run relationships in the data, which traditional time‐series regression models ignore. Our use of cointegration allowed us to conclude that there is a long‐run and statistically significant relationship between acute myocardial infarction incidence and UV radiation that previous research has not been able to detect or quantify.

We calculated mean monthly levels of UVA and UVB for Scotland as a whole. Mainland Scotland measures 441 km (274 miles) from north to south; this equates to a relatively small range of latitudes of 0.2° (from 58.4° to 58.6° North). Our calculation of UV exposure assumed that residents of Scotland were in Scotland at the time of UV measurement. This will be the case for measurement contemporaneous with myocardial infarction. For measurements ≥1 months earlier, a small proportion of participants may have been on holiday or work trips aboard. Similarly, we had no data on individual differences in exposure to UV attributable to the proportion of time spent outdoors, type of clothing, or use of sunblock.

In conclusion, low exposure to UVA radiation was associated with winter peaks in myocardial infarction and higher overall incidence in a higher‐latitude country. A causal relationship is biologically plausible in light of the known effect of UVA exposure on blood pressure, but further research is needed, including measures of individual exposure. If corroborated, consideration should be given to conducting a randomized controlled trial of UV phototherapy boxes, a relatively inexpensive intervention that could be administered at home.

Sources of Funding

Hastie was funded by Health Data Research UK. Provision of the UV data was cofunded by the Natural Environment Research Council, Medical Research Council, and Chief Scientist Office (reference NE/P010911/1).

Disclosures

None.

(J Am Heart Assoc. 2019;8:e012551 DOI: 10.1161/JAHA.119.012551.)