Unshielded magnetocardiography: Repeatability and reproducibility of automatically estimated ventricular repolarization parameters in 204 healthy subjects

Abstract

Background

Magnetocardiographic mapping (MCG) provides quantitative assessment of the magnetic field (MF) induced by cardiac ionic currents, is more sensitive to tangential currents, and measures vortex currents undetectable by ECG, with higher reported sensitivity of MCG ventricular repolarization (VR) parameters for earlier detection of acute myocardial ischemia. Aims of this study were to validate the feasibility of in‐hospital unshielded MCG and to assess repeatability and reproducibility of quantitative VR parameters, considering also possible gender‐ and age‐related variability.

Methods

MCG of 204 healthy subjects [114 males—mean age 43.4 ± 17.3 and 90 females—mean age 40.2 ± 15.7] was retrospectively analyzed, with a patented proprietary software automatically estimating twelve VR parameters derived from the analysis of the dynamics of the T‐wave MF extrema (five parameters) and from the inverse solution with the effective magnetic dipole model giving the effective magnetic vector components (seven parameters). MCG repeatability was calculated as coefficient of variation (CV) ±standard error of the mean (SEM). Reproducibility was assessed as intraclass correlation coefficient (ICC).

Results

The repeatability of all MCG parameters was 16 ± 1.2 (%) (average CV ± SEM). Optimal (ICC > 0.7) reproducibility was found for 11/12 parameters (mean values) and in 8/12 parameters (single values). No significant gender‐related difference was observed; six parameters showed a strong/moderate correlation with age.

Conclusion

Reliable MCG can be performed into an unshielded hospital ambulatory, with repeatability and reproducibility of quantitative assessment of VR adequate for clinical purposes. Wider clinical use is foreseen with the development of multichannel optical magnetometry.

1. INTRODUCTION

Multichannel magnetocardiographic mapping (MCG) is a noninvasive/noncontact and radiation‐free method detecting the cardiac magnetic field (CMF), through high‐sensitivity magnetometers such as the direct current superconducting quantum interference devices (DC‐SQUIDs) (Fenici, Brisinda, & Meloni, 2005; Fenici, Brisinda, Sorbo, & Venuti, 2011; Leder et al., 2001; Sosnytska, 2011; Tavarozzi, Comani, Del Gratta, Di Luzio et al., 2002) or, more recently, optical ones (Lau, Petkovic, & Haueisen, 2016; Morales et al., 2017). Compared to the electrocardiogram (ECG), MCG provides additional information, being CMF less affected by tissue conductivities, and is more sensitive to tangential currents, and capable to detect vortex currents too (Brockmeier et al., 1997; Fenici Brisinda, & Meloni 2005). Although mainly confined in research laboratories, MCG is increasingly used to study cardiac arrhythmias (Fenici, Brisinda, Venuti, & Sorbo, 2013; Fenici, Brisinda, & Meloni, 2005; Kwong, Leithäuser, Park, & Yu, 2013; Mäkijärvi et al., 2010) and ventricular repolarization (VR) abnormalities (VRa) due to different cardiopathy (Kawakami et al., 2017), especially ischemic heart disease (IHD) (Agarwal, Saini, Alyousef, & Umscheid, 2012; Brisinda, Bottelli, Napolitano, Priori, & Fenici, 2007; Fenici, Brisinda, Meloni, Sternickel, & Fenici, 2005; Hailer, Chaikovsky, Auth‐Eisernitz, Schäfer, & Van Leeuwen, 2005; Kandori et al., 2010; Kwon et al., 2010; Leithäuser, Park, Hill, Lam, & Jung, 2013; Li et al., 2015; Lim et al., 2007; Ogata et al., 2009; Park & Hill, 2005; Park, Leithäuser, Hill, & Jung, 2008; Park et al., 2015; Shin et al., 2015; Steinisch et al., 2013; Tolstrup et al., 2006; Wu et al., 2013).

MCG instrumentation is typically classified by the method used to improve the signal‐to‐noise ratio (S/Nr) (Fenici et al., 2011; Mäkijärvi et al., 2010; Tavarozzi, Comani, Del Gratta, Di Luzio et al., 2002). Although electromagnetic shielding provides a better S/Nr, it is unpractical for clinical use, impeding quick ambulatory screening and bedside MCG. Alternatively, instrumentations for unshielded MCG have been also developed (Chen, Thompson, Nolan, Clarke, & Bakharev, 2002; Chen, Thomson, Nolan, & Clarke, 2004; Fenici et al., 2013; Hailer, Van Leeuwen et al., 2005; Leder et al., 2001; Steinberg et al., 2005; Tolstrup et al., 2006); however, most of them feature a limited number of sensors (4–9 channels), implying sequential mapping (Chen et al., 2002, 2004; Leder et al., 2001; Quan et al., 2008; Steinberg et al., 2005), a potential possible source of error especially for dynamic evaluation of transient events (Smith et al., 2002).

Although a direct comparison among different MCG systems and standardization of analytic methods is still missing, some attempts to standardize MCG format and to assess reproducibility and age‐ or gender‐dependent variations of several MCG parameters (e.g., interval durations, waveforms, VR dipole motion, vector parameters) have been reported (Chen et al., 2004; Kandori, Ogata, Miyashita, Watanabe et al., 2008; Lim et al., 2007; Steinberg et al., 2005). Given the availability of a unique multichannel MCG instrumentation fully operational into our unshielded hospital laboratory for interventional electrophysiology since 2002 (Fenici, Brisinda, & Meloni 2005; Fenici, Brisinda, Meloni, Sternickel, & Fenici, 2005; Fenici et al., 2011, 2013), we have retrospectively selected and analyzed MCG data of 204 healthy subjects to assess the repeatability and reproducibility of automatically calculated VR parameters used for diagnosis of IHD (Bakharev, 2011; Kwong et al., 2013; Park & Jung, 2004; Steinberg et al., 2004, 2005; Tolstrup et al., 2006), to set up a normality database obtained with simultaneous mapping (Fenici, Brisinda, Meloni, & Fenici, 2003), from a larger cohort compared to previous studies (Chen et al., 2002, 2004; Hailer, Van Leeuwen et al., 2005; Tolstrup et al., 2006) and to evaluate gender‐ and/or age‐related variability.

2. METHODS

2.1. Subjects

MCG data of 204 healthy subjects selected among those consecutively screened between 2004 and 2016, mainly to assess fitness for sports activity, were retrospectively analyzed. MCG data were accepted only if averaged signals of all 36 channels were free from artifacts and with a stable baseline (thus, no interpolation of even one channel was required).

All subjects were free from cardiac risk factors and from use of any medication and had normal blood pressure and rest ECG. When appropriate, echocardiography and ECG stress test data were also available. Written informed consent to MCG and to retrospective use of de‐identified clinical and MCG data for research purpose had been obtained. The study protocol conforms to the ethical guidelines of the 1975 Declaration of Helsinki and was approved by the local review board.

2.2. Equipment and measurement technique

MCG was performed, into an unshielded laboratory for interventional electrophysiology, with a 36‐channel system featuring DC‐SQUIDs coupled to second‐order axial gradiometers (pickup coil 50–70 mm baseline), arranged in a square grid of 20 × 20 cm (pitch between sensors: 4 cm) (CardioMag Imaging, Inc., Schenectady, NY, USA) (Fenici et al., 2003) (Figure 1). The average intrinsic sensitivity of the system was about 30 fT/√Hz in the frequency range of clinical interest (1–100 Hz). MCG was recorded (bandwidth: DC‐250 Hz, sampling frequency 1 kHz, 24‐bit A/D conversion) in the supine position (after removal of metal objects), with the Dewar as close as possible to (but not touching) the anterior chest wall. MCG session lasted typically 90 seconds. ECG lead II was simultaneously recorded.

Figure 1.

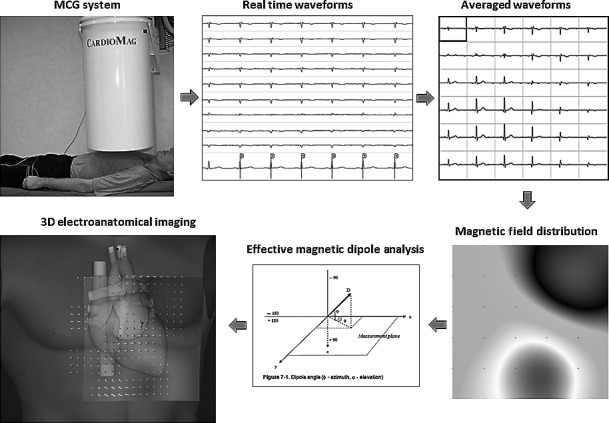
MCG recording setup, signal processing, and multimodal imaging

At least two MCGs were performed for each patient, the second one acquired by the same examiner few minutes after repositioning the subject on the examination table (to test for repeatability), or by different examiners in a different session (to test for reproducibility).

2.3. MCG processing and analysis

MCG parameters of VR were automatically calculated with a patented software tool (Bakharev, 2011), after digital low‐pass filtering, selective time averaging, and automatic computing of CMF distribution (CMFd). In case of residual artifacts at visual control, noisy and/or premature beats were manually removed before averaging. The reference baseline was automatically defined before the P wave, but was also manually selected, if necessary.

VR analysis consisted of:

• Qualitative analysis, based on visual inspection of the CMFd dynamics, aimed to confirm stable dipolar morphology of CMFd during the ST segment and the T wave. If a multipolar pattern was present, in the time interval between 50 milliseconds after the J‐point and the T‐end (Figure 2), with CMF amplitude at least three times stronger than average noise in the TP interval, the VR pattern was considered abnormal and the record discarded.

• Quantitative analysis, based on the solution of the inverse problem with the effective magnetic dipole (EMD) model and automatic calculation of the time‐variant dynamics of: (1) the three‐dimensional (3D) EMD vector (EMDV) components and (2) of the T‐wave magnetic field (MF) extrema:

  1. EMDV parameters: The magnitude and strength of motion of the EMV can be described by seven predefined parameters T‐wave vector parameters calculated both before (Ascending limb, or A) and after (Descending limb, or D) the T‐wave peak:

     • Two azimuth mean: the angles between the projection of 3D average EMDV on XY plane and the x‐axis, being the origin of the axes the vertex (azimuth mean A normal if between −110° and −15 ° and azimuth mean D normal if between −100° and −22°);

     • Two Trajectory Length: EMDV trajectory lengths (Trajectory Length A normal if between 0 and 7.5 cm and Trajectory Length D normal if between 0 and 5 cm);

     • Two Angle Derivative Range: 3D EMDV angular deviations (between T‐start and T‐peak and between T‐peak and T‐end) defined through azimuth and elevation (i.e., the angle between the projection of 3D average EMV on XZ plane and the x‐axis, being the origin of the axes the vertex) (angle derivative range A normal if between 0 and 1.0 radians and angle derivative range D normal if between 0 and 0.7 radians);

     • The azimuth difference (A–D): angular displacement, with its own orientation, on XY plane, of the projection of 3D average EMDV (i.e., azimuth mean A minus azimuth mean D), normal if between −35° and 12°;

  2. T‐wave extrema MF dynamics: five parameters automatically calculated during the first phase of VR, within a frame of 30 ms moving into an interval between the point where MF strength is equal to 1/3 of T‐wave peak MF and the T‐wave peak. Being generated, for each millisecond, an instant magnetic map with a positive and a negative pole (the highest and the lowest intensity of the MF) (Fenici, Brisinda, Meloni, Sternickel, & Fenici, 2005; Park & Jung, 2004; Steinberg et al., 2004, 2005), the following parameters were calculated:

     • Two angle extrema: maximum (angle extrema 1) and minimum (angle extrema 2) α angle between a line through the poles and a horizontal line, the origin set to plus pole (normal if between −110° and 20°);

     • The angle dynamics: α angle rotation in each interval of 30 ms (abnormal if >45°);

     • The distance dynamics: dynamic change of the distance between the poles ± (abnormal if >20 mm);

     • The ratio dynamics: MF strength ratio between the poles ± (abnormal if >0.3).

Figure 2.

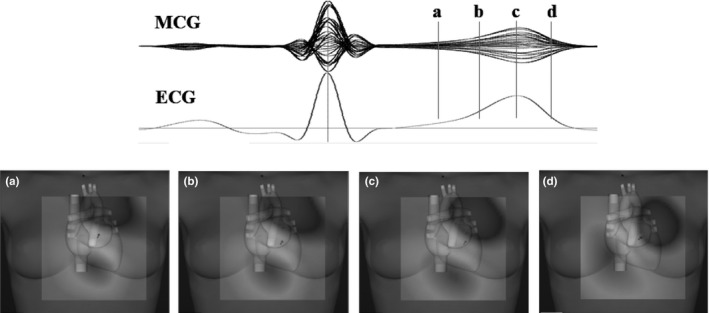
Example of stable dipolar configuration of the magnetic field distribution: during the ST segment (a), beginning (b), peak (c), and end (d) of T wave

2.4. Statistical analysis

Statistical analysis was carried out with SPSS (version 21.0). All MCG parameters were expressed as mean ± standard deviation (SD). A p‐value <.05 was considered statistically significant.

Repeatability (i.e., by definition, the capability of the instrumentation to reproduce results from two subsequent MCG performed by the same operator, under the same conditions and in a short period) was assessed through coefficient of variation (CV) between parameters for each subject. Data were subsequently expressed, for each parameter, as the average CV ± standard error of the mean (SEM). A small CV is an index of good repeatability. Graphically, a Bland–Altman plot for each parameter was created. Conventionally, repeatability must be made within maximum 72 hr; however, in this study we have chosen to analyze recordings taken subsequently within a time interval of few minutes.

Reproducibility (i.e., the degree of correlation between measurements performed at a distance of at least 72 hr by different operators) was tested with the one‐way random‐effects model intraclass correlation coefficient (ICC), accepting ICC >0.6 as index of a good and ICC >0.7 of optimal reproducibility.

To evaluate gender‐related variability of MCG parameters, parametric (t test) or nonparametric tests (Mann–Whitney U test—MW) were used.

To evaluate age‐related variability of MCG parameters, after dividing the whole population in eight age groups, p‐value of Kruskal–Wallis test and Pearson's correlation coefficient (r‐value, greater correlation if equal or close to ±1) were computed. Pearson's correlation coefficient was also calculated between single values and age. To correct for possible effect of obesity, the partial correlation coefficient was computed, using BMI and BSA as control variables.

3. RESULTS

The demographics of 204 investigated healthy volunteers [114 males (M), mean age 43.4 ± 17.3 years; and 90 females (F), mean age 40.2 ± 15.7 years] are summarized in Table 1. A graphic summary of overall results is shown in the Figure 2.

Table 1.

Subject characteristics

	Male (n = 114)	Female (n = 90)	p‐value
Age (years)
Minimum	4	4
Maximum	82	79
Average value	43.4	40.2	.17
Standard deviation	±17.3	±15.7
Weight (kg)
Minimum	17	19
Maximum	110	98
Average value	78.2	58.4	<.05
Standard deviation	±15	±11.7
Height (cm)
Minimum	101	108
Maximum	193	176
Average value	174.6	161.1	<.05
Standard deviation	±11.8	±8.6
BMI (kg/m2)
Minimum	16.67	14.7
Maximum	36.75	40.79
Average value	25.43	22.39	<.05
Standard deviation	±3.72	±3.79
BSA (m2)
Minimum	0.69	0.75
Maximum	2.41	2.06
Average value	1.94	1.61	<.05
Standard deviation	±0.24	±0.19

BMI, body mass index; BSA, body surface area.

3.1. Repeatability

Two MCG recordings of 138 subjects (52% males), subsequently acquired by the same examiner, were analyzed. Average CV ± SEM for each MCG parameter are shown in Table 2. For azimuth difference (A minus D), the absolute value was used. Correspondent Bland–Altman plots are shown in Figure 3.

Table 2.

Repeatability

MCG parameters	Ml AV	M2 AV	Average CV (%)	SEM (%)
Trajectory length range A (cm)	2.46	2.35	15.83	1.18
Angle derivative range A (radians)	0.33	0.32	15.17	1.28
Azimuth mean A (degree)	−57.83	−57.78	4.78	0.47
Trajectory length range D (cm)	1.97	2	18.41	1.11
Angle derivative range D (radians)	0.28	0.28	15.69	1.05
Azimuth mean D (degree)	−50.64	−50.27	4.37	0.41
Azimuth diff AD (degree)	−7.19	−7.51	25.39	2.58
Angle extrema 1 (degree)	−72.54	−71.78	4.4	0.41
Angle extrema 2 (degree)	−62.92	−62.37	3.69	0.45
Angle dynamics (degree)	6.32	5.99	22.53	1.77
Distance dynamics (mm)	8.27	7.78	27.94	2.15
Ratio dynamics	0.16	0.14	33.91	2.51

CV, coefficient of variation; M1 AV, measurement 1 average value; M2 AV, measurement 2 average value; SEM, standard error of the mean.

Figure 3.

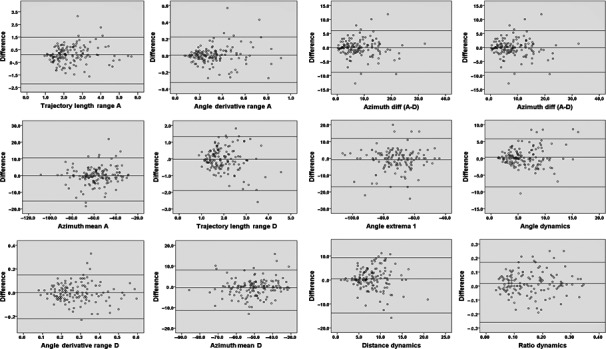
Bland–Altman Plots of VR parameters. The average value of two measures and their difference are plotted, respectively, on the x‐ and on the y‐axis. Central horizontal line corresponds to the average of differences while the line above and the line below represent the average of differences ±1.96 SD: more points fall within the two outside lines is greater repeatability

3.2. Reproducibility

One hundred and thirteen healthy subjects (52% males) had two MCG acquired by different examiners at a distance of at least 72 hr. For each MCG parameter, one‐way random‐effects model ICC between the first and second groups of measurements was calculated, considering the examiners as random effects (as sampled from a larger pool of potential raters). Reproducibility, in terms of average raters absolute ICC, was optimal for 11 of 12 parameters. Single raters absolute ICC, instead, was optimal for 8 and good for 3 of the 12 parameters (Table 3). Only MF extrema distance dynamics showed a low reproducibility which, however, increases considering its log₁₀ (0.35 for single raters absolute ICC and 0.52 for average raters absolute ICC).

Table 3.

Reproducibility

MCG parameters	Single raters ICC	Average raters ICC
Trajectory length range A (cm)	0.71	0.82
Angle derivative range A (radians)	0.78	0.87
Azimuth mean A (degree)	0.93	0.96
Trajectory length range D (cm)	0.55	0.71
Angle derivative range D (radians)	0.77	0.87
Azimuth mean D (degree)	0.90	0.95
Azimuth diff AD (degree)	0.87	0.93
Angle extrema 1 (degree)	0.83	0.9
Angle extrema 2 (degree)	0.83	0.91
Angle dynamics (degree)	0.65	0.79
Distance dynamics (mm)	0.22	0.37
Ratio dynamics	0.61	0.75

ICC, intraclass correlation coefficient.

3.3. Creation of the database of normality

Overall, MCG data of 403 recordings of the whole studied population were used to obtain the database of normal values. To define maximum and minimum values of each parameter, we used all 403 files individually. To define mean values and their SD, single subject's data were obtained from the average of all individual recordings. All parameters (Table 4) were within the previously published normality range (Park & Jung, 2004; Shin et al., 2017; Steinberg et al., 2005; Tolstrup et al., 2006) except ratio dynamics, which was >0.3 in 7.6% of cases and whose highest value was 0.4, for both males and females.

Table 4.

MCG ventricular repolarization parameters values

MCG parameters	Male (n = 114)					Female (n = 90)
	Min	Max	AV	±SD	Min	Max	AV	±SD	p‐value	Test
Trajectory length range A (cm)	0.95	7.01	2.52	1.03	0.93	5.9	2.57	1.10	.94	MWa
Angle derivative range A (radians)	0.07	0.93	0.32	0.15	0.09	1	0.37	0.18	.04	MWa
Azimuth mean A (degree)	−95.47	−25.37	−57.48	15.61	−108.93	−28.65	−61.29	15.65	.09	t test
Trajectory length range D (cm)	0.78	4.81	2.08	0.70	0.81	4.96	2.14	0.82	.86	MWa
Angle derivative range D (radians)	0.07	0.7	0.27	0.13	0.1	0.65	0.32	0.12	.00	MWa
Azimuth mean D (degree)	−82.59	−30.03	−51.39	10.68	−86.65	−26.34	−52.96	11.51	.32	t test
Azimuth diff AD (degree)	−31.58	11.73	−6.26	8.11	−33.05	7.95	−8.33	7.25	.06	t test
Angle extrema 1 (degree)	−108	8	−74.06	14.74	−108	−44	−73.54	13.48	.80	t test
Angle extrema 2 (degree)	−104	73	−63.28	13.13	−101	−41	−64.31	10.40	.54	t test
Angle dynamics (degree)	1	20	6.54	3.37	0.22	27.11	6.30	3.68	.36	MWa
Distance dynamics (mm)	0.88	24.97	8.18	3.82	0.9	20.37	8.07	3.22	.99	MWa
Ratio dynamics	0.01	0.4	0.15	0.09	0.01	0.4	0.17	0.09	.05	MWa

AV, average value; Max, maximum; Min, minimum; SD, standard deviation.

^aMann–Whitney U test.

3.4. Gender‐related differences

To check for gender‐related difference of VR parameters, the Student t test was used when data distribution was normal (five of 12 parameters), while the Mann–Whitney U test was used for non‐normally distributed parameters. Only angle derivative range (A and D) and the ratio dynamics were significantly different (Table 4).

3.5. Evaluation of age‐related variability

To evaluate age‐related variability, the whole study population was arbitrarily divided into 8 age decades. Average values of 8 of the 12 parameters MCG parameters were significantly different among groups (Table 5). Average values of each MCG parameter [azimuth difference (A minus D) calculated using absolute values] of each decade were correlated with age progression (Table 6). An optimal (r‐value >.7) direct correlation for five parameters and a good (r‐value −.5) reverse correlation for a sixth one (angle derivative range D) were found (Figure 4). Instead, the correlation between each MCG parameter and individual age was weak (r‐value <.4), even if corrected for BMI and BSA (Table 7).

Table 5.

Average values of ventricular repolarization parameters of the eight age groups

Age groups	1	2	3	4	5	6	7	8
Age (years)	<10	11–20	21–30	31–40	41–50	51–60	61–70	>70
No of subjects	5	12	42	38	42	35	21	9	p‐value
MCG parameters (average values)
Trajectory length range A (cm)	1.84	1.75	2.21	2.39	2.65	3.34	3.34	2.5	<.001
Angle derivative range A (radians)	0.32	0.25	0.32	0.37	0.34	0.36	0.36	0.38	n.s.
Azimuth mean A (degree)	−82.25	−49.58	−55.88	−64.08	−56.12	−60.3	−60.3	−67.85	<.05
Trajectory length range D (cm)	2.03	1.96	1.97	1.97	2.14	2.54	2.54	2.46	n.s.
Angle derivative range D (radians)	0.47	0.27	0.29	0.32	0.26	0.29	0.29	0.3	n.s.
Azimuth mean D (degree)	−71.08	−46.56	−49.55	−54.2	−49.07	−51.79	−51.79	−62.35	<.01
Azimuth diff AD (degree)	11.18	4.41	7.65	11.01	7.99	8.51	8.51	8.65	n.s.
Angle extrema 1 (degree)	−98.2	−66.6	−69.3	−76.7	−71.5	−77.8	−77.8	−84.2	<.001
Angle extrema 2 (degree)	−90.2	−58.7	−61.3	−65.9	−61.5	−65.3	−65.3	−69.5	<.01
Angle dynamics (degree)	5.999	5.364	5.049	6.988	6.13	7.435	7.435	8.668	<.001
Distance dynamics (mm)	3.54	7.92	6.68	7.91	8.43	10.2	10.2	9.41	<.05
Ratio dynamics	0.196	0.1171	0.1307	0.1461	0.1675	0.1882	0.1882	0.1789	<.05

n.s., not significant.

Table 6.

Pearson's correlation between age groups and average values of ventricular repolarization parameters

MCG parameters (average values)	r‐value
Trajectory length range A (cm)	.82
Angle derivative range A (radians)	.75
Azimuth mean A (degree)	.14
Trajectory length range D (cm)	.83
Angle derivative range D (radians)	−.50
Azimuth mean D (degree)	.11
Azimuth diff AD (degree)	−.01
Angle extrema 1 (degree)	.10
Angle extrema 2 (degree)	.33
Angle dynamics (degree)	.84
Distance dynamics (mm)	.85
Ratio dynamics	.39

Figure 4.

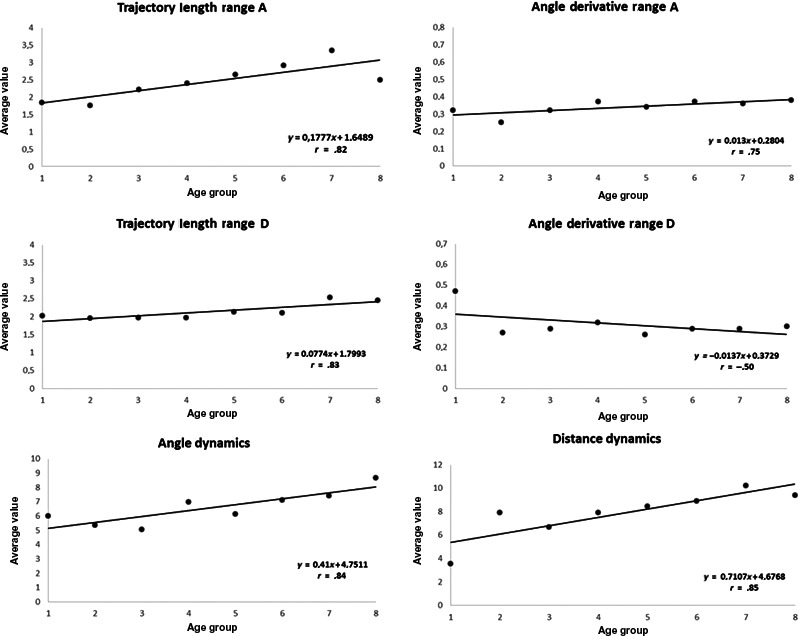
Scatter plot of the Pearson's correlation between age groups (x‐axis) and average values of 6 VR parameters (y‐axis)

Table 7.

Correlation between individual age and ventricular repolarization parameters

Control variable	None	BMI	BSA
MCG parameters	Pearson's r‐value	PCC r‐value	PCC r‐value
Trajectory length range A (cm)	.35	.24	.29
Angle derivative range A (radians)	.15	.21	.21
Azimuth mean A (degree)	−.03	−.03	−.10
Trajectory length range D (cm)	.20	.12	.19
Angle derivative range D (radians)	−.10	−.05	−.01
Azimuth mean D (degree)	−.06	−.05	−.13
Azimuth diff AD (degree)	.01	.03	.05
Angle extrema 1 (degree)	−.08	−.08	−.12
Angle extrema 2 (degree)	.03	.01	−.04
Angle dynamics (degree)	.23	.19	.22
Distance dynamics (mm)	.32	.23	.26
Ratio dynamics	.19	.11	.19

BMI, body mass index; BSA, body surface area; PCC, partial correlation coefficient.

4. DISCUSSION

Mass screening of healthy young people and sports practitioners is not considered cost‐effective even if performed simply with ECG recording (Maron et al., 2014). Body surface potential mapping (Taccardi & Punske, 2004) recently improved with modern multielectrode vests is still an expensive and complex procedure, which requires multimodal integration with CT scan to obtain pre‐interventional electro‐anatomical imaging (Dubois et al., 2015; Zhou, Jin, Yu, Wu, & He, 2016).

MCG is a contactless alternative for faster mass screening (Mäkijärvi et al., 2010; Malmivuo, 1995; Steinhoff et al., 2004; Tavarozzi, Comani, Del Gratta, Romani et al., 2002; Wu et al., 2013) and radiation‐ and risk‐free method for three‐dimensional electro‐anatomical imaging through passive detection of CMF (Agarwal et al., 2012; Brisinda et al., 2007; Brockmeier et al., 1997; Fenici, Brisinda, Meloni, Sternickel, & Fenici, 2005; Hailer, Van Leeuwen et al., 2005; Kandori et al., 2010; Kwon et al., 2010; Kwong et al., 2013; Leithäuser et al., 2013; Li et al., 2015; Lim et al., 2007; Ogata et al., 2009; Park & Hill, 2005; Park et al., 2008, 2015; Shin et al., 2015; Steinisch et al., 2013; Tolstrup et al., 2006; Wu et al., 2013), which is directly related primary electrophysiological source (the impressed current), thus containing information undetectable with ECG (Fenici, Brisinda, & Meloni, 2005; Tavarozzi, Comani, Del Gratta, Di Luzio et al., 2002). VR analysis is considered one of the most useful applications of MCG, mainly to recognize ischemic, inflammatory, or degenerative abnormalities (Tavarozzi, Comani, Del Gratta, Di Luzio et al., 2002). However, although some attempts to assess the reproducibility and comparability of MCG parameters gathered with different instrumentations have been reported (Burghoff, Nenonen, Trahms, & Katila, 2000; Nalbach et al., 2002; Pesola et al., 2000; Smith et al., 2006; Takala et al., 2001), a standardization adequate for clinical application is still missing, especially taking into account the potential source of error due to different S/Nr and/or sequential data acquisition (Bang et al., 2016; Hänninen et al., 2002; Kawakami et al., 2017; Mäkijärvi et al., 2010; Shin et al., 2015) as occurs with most instrumentations for unshielded MCG (Fenici, Brisinda, & Meloni, 2005; Leder et al., 2001) due to their limited number of sensors.

This study aimed at evaluating (and to the best of our knowledge is the first reporting) the normality range of twelve VR parameters automatically calculated from MCG data acquired with an unshielded 36‐channel instrumentation in a hospital environment (Fenici et al., 2003). As the repeatability and reproducibility of conventional time domain parameters (QRS duration, QT interval, etc.) were largely evaluated in the past (Fenici, Brisinda, & Meloni, 2005; Tavarozzi, Comani, Del Gratta, Romani et al., 2002), this study focused only on parameters automatically obtained from the inverse solution and the analysis of the time‐variant CMF dynamics.

Compared to previous studies (Chen et al., 2002, 2004; Steinberg et al., 2004, 2005; Tolstrup et al., 2006), a larger cohort, 204 normal subjects, has been investigated. Moreover, all subjects underwent at least two MCG recordings, carried out under variable conditions (i.e., different hours of the days, implying different intensity of the background electromagnetic noise) to check for repeatability and for reproducibility of data analysis performed with signals acquired in the “real‐life” of an unshielded hospital environment.

Morphological analysis of CMF distribution during VR confirmed a stable dipolar homogeneous pattern during the whole ST interval and the T wave (Figure 2). The range of quantitative T‐wave parameters, automatically calculated after solution of the inverse problem, was consistent with previously applied criteria, except for the ratio dynamics, which exceeded the upper level of 0.3 in 7.6% of investigated cases, without gender‐related difference. As values above 0.4 were not observed, being the studied population certainly normal, we suggest elevating to 0.4 the upper limit of normality for this parameter.

Although significant age‐ and gender‐related differences were previously reported for parameters of normal subjects investigated with a 9‐channel unshielded MCG system (Chen et al., 2004) and with shielded multichannel mapping (Kandori, Ogata, Miyashita, Takaki et al., 2008), no gender‐related differences of the automatically calculated parameters were found in the present study, except for the angle derivative range A and D (p < .05 and .001, respectively).

As concerns the effect of age, after dividing our cohort into eight age groups, differences of distribution by age class resulted significant for 8 of 12 parameters; five parameters (trajectory length range A and D, angle derivative range A, angle dynamics, and distance dynamics) showed high direct correlation (r‐value >.7), while angle derivative range D moderate reverse correlation with decades groups (Figure 2). Instead, the correlations between individual MCG parameters and age, even if corrected for BMI and BSA, were all weak (Table 7).

Repeatability and reproducibility of estimated parameters ranged from optimal to good, thus adequate for clinical application (Bakharev, 2011; Leder et al., 2001; Lim et al., 2007; Steinberg et al., 2005). However, our results were better than previously reported (Steinberg et al., 2005), probably because of the larger cohort analyzed.

4.1. Study limitations

A first limitation of this study is that, to avoid any subjective bias in the interpretation, only the T‐wave parameters automatically calculated by the proprietary software were evaluated. Thus, our results can be applied as normality reference only when using the same or equivalent automatic analysis methods (Steinisch et al., 2013). On the other hand, this does not detract the value of the study because so far, the normality values available in previous investigation were obtained from limited cohorts of local volunteers (Steinberg et al., 2005; Tolstrup et al., 2006) or unpublished. Second, although our sample is relatively large, it was probably insufficient to evaluate gender‐related variability from numerically homogeneous groups. The same could explain the lack of correlation between age and VR parameters when analyzing individual values and not age groups. Thus, to assess gender‐ and age‐related variability, further investigation on larger number of subjects may be required.

Finally, interobserver reproducibility was not evaluated, due to the lack of a systematic collection of data by at least two different operators on the same group of subjects.

5. CONCLUSION

This study demonstrates that unshielded MCG is feasible in hospital environments, with repeatability and reproducibility for VR parameters adequate for clinical application of the method, thus confirming the reliability of previous measurements carried out with smaller systems in noisy emergency departments and other clinical settings (Fenici et al., 2013; Hailer, Van Leeuwen et al., 2005; Kwong et al., 2013; Steinberg et al., 2005; Tolstrup et al., 2006).

Given the theoretical and technical advantages of MCG in detecting ischemic electrophysiological events and their DC components noninvasively (Cohen, Savard, & Rifkin, 1983), with reported predictive accuracy comparable with that of SPECT (Bakharev, 2011; Shin et al., 2017; Steinberg et al., 2005; Steinisch et al., 2013; Tolstrup et al., 2006), once the drawbacks and complexity of cryogenic instrumentations and/or of expensive heavy electromagnetic shielding will be overcome, (Kwong et al., 2013; Shin et al., 2017; Steinisch et al., 2013), its use in emergency departments for quick and early triage of chest pain patients with still normal 12‐lead ECG and high‐sensitivity cardiac troponin assays is foreseen as an attractive radiation‐free option to rule out acute coronary syndrome (ACS) and to reduce indication to stress SPECT and CT coronary angiography (Bakharev, 2011; Shin et al., 2017; Steinberg et al., 2005; Steinisch et al., 2013; Tolstrup et al., 2006). Still, the need of liquid helium and related costs of cryogenic instrumentations may be a limitation. However, recent development of reliable optical magnetometers working at room temperature is foreseen as an important cornerstone for the construction of innovative portable biomagnetic devices, usable in emergency room, at patient's bedside (Morales et al., 2017; Shah, 2013) or even for dynamic monitoring (Lau et al., 2016). Other novel technologies, such as magnetometry with nitrogen‐vacancy defects in diamond are under investigation with advanced testing for biomagnetic measurements (Barry et al., 2016).

Sorbo AR, Lombardi G, La Brocca L, Guida G, Fenici R, Brisinda D. Unshielded magnetocardiography: Repeatability and reproducibility of automatically estimated ventricular repolarization parameters in 204 healthy subjects. Ann Noninvasive Electrocardiol. 2018;23:e12526 10.1111/anec.12526