Theoretical study of phase stability, crystal and electronic structure of MeMgN₂ (Me = Ti, Zr, Hf) compounds

Abstract

Scandium nitride has recently gained interest as a prospective compound for thermoelectric applications due to its high Seebeck coefficient. However, ScN also has a relatively high thermal conductivity, which limits its thermoelectric efficiency and figure of merit (zT). These properties motivate a search for other semiconductor materials that share the electronic structure features of ScN, but which have a lower thermal conductivity. Thus, the focus of our study is to predict the existence and stability of such materials among inherently layered equivalent ternaries that incorporate heavier atoms for enhanced phonon scattering and to calculate their thermoelectric properties. Using density functional theory calculations, the phase stability of TiMgN₂, ZrMgN₂ and HfMgN₂ compounds has been calculated. From the computationally predicted phase diagrams for these materials, we conclude that all three compounds are stable in these stoichiometries. The stable compounds may have one of two competing crystal structures: a monoclinic structure (LiUN₂ prototype) or a trigonal superstructure (NaCrS₂ prototype; R$\overline{3}$mH). The band structure for the two competing structures for each ternary is also calculated and predicts semiconducting behavior for all three compounds in the NaCrS₂ crystal structure with an indirect band gap and semiconducting behavior for ZrMgN₂ and HfMgN₂ in the monoclinic crystal structure with a direct band gap. Seebeck coefficient and power factors are also predicted, showing that all three compounds in both the NaCrS₂ and the LiUN₂ structures have large Seebeck coefficients. The predicted stability of these compounds suggests that they can be synthesized by, e.g., physical vapor deposition.

Introduction

Thermoelectric materials and devices, which directly convert a thermal gradient into an external voltage, are reliable and low-maintenance power-generating materials used for niche applications such as solid-state cooling or electric power supplying units in deep-space exploration. However, the use of thermoelectrics is presently limited [1] by their low efficiency and high cost. For example, the crustal abundance and global production of tellurium is low [2, 3]. This limits widespread use of the benchmark thermoelectric materials (Bi₂Te₃ and PbTe). Thus, there is a need for replacement materials.

The thermoelectric efficiency is directly connected to the dimensionless figure of merit:

$$zT=\left(\frac{S^2\mathit{\sigma }}{\mathit{\kappa }}\right)\times T,$$

where S is the Seebeck coefficient, $\mathit{\sigma }$ is the electrical conductivity, $\mathit{\kappa }$ is the thermal conductivity, and T is the absolute temperature [4]. The product $S^2\mathit{\sigma }$ is known as the power factor. In the limit of $zT\to \infty$, the Carnot engine efficiency (i.e., the maximum efficiency achievable in a heat engine) is obtained. However, designing materials with higher zT values is a difficult challenge, as all three terms are interrelated in a way that typically limits zT to below unity in commonly available materials.

In order to overcome this barrier, Slack proposed the phonon glass–electron crystal (PGEC) approach for thermoelectric material design [5–7]: one should seek a material with a high Seebeck coefficient value and engineer it in such a way that it will behave like a crystal for electrons, but scatter phonons similarly to glass. As a result, added material optimization processes are required to increase the zT of any given material.

As a starting point for this approach of engineering a high $\mathit{zT}$ material, prior works have suggested cubic scandium nitride (ScN) [8]. The Seebeck coefficient of ScN is relatively large (reaching − 180 $\mathrm{\mu }\text{V}/\text{K}$ at 800 $\text{K}$) and because of its low electrical resistivity, large power factors between 2.5 and 3.5 × 10⁻³ ${\text{Wm}}^{-1}{\text{K}}^{-2}$ have been reported [9, 10]. Doping and alloying ScN with heavy elements [11, 12] and/or creating artificial layer interfaces such as metal/semiconductor superlattices [13–16] can alter properties and decrease the thermal conductivity, resulting in an enhanced zT. Furthermore, ScN can also become p-type by Sc-site doping [17, 18]. Although the direction of research is promising, ScN does have a relatively large thermal conductivity [19–22] of approximately 8–12 ${\text{Wm}}^{-1}{\text{K}}^{-1}$. Scandium and nitrogen are both light atoms compared to their heavier counterparts such as lead, bismuth and tellurium which effectively scatter phonons [23], and artificial interfaces seen in superlattices are synthesized at a sub micrometer scale, while thermoelectric power generation requires millimeter-sized bulk samples [24]. Also, scandium does not have phonon isotope scattering as it is an isotopically pure element.

In a recent paper, Alling [25] addressed these issues by proposing an equivalent ternary based on ScN. Scandium (which is a group-3 element) can be replaced with one group-2 and one group-4 element in a 50/50 proportion to cover the same electron valence. The final compound should then have a MeAEN₂ stoichiometry, with Me representing a transition metal from the group-4 elements and AE belonging to the group-2 (alkaline earth) elements, such as magnesium. TiMgN₂ was predicted to be stable using density functional theory (DFT). Band structure calculations predicted stoichiometric TiMgN₂ to have a 1.11 eV band gap using the HSE06 [25, 26] hybrid functional. This methodology has also been used by Tholander et al. [27] to predict zinc-based group-4 transition metal nitride stability and crystal structure. While much research has been done regarding Ti–Si–N [28–30] and Ti–Al–N [31–34] which show superior hardness and/or oxidization resistance compared to TiN, there are much fewer studies reported for Ti–Mg–N [35–39]. TiMgN₂ may crystallize in the B1–L1₁ superstructure [25], which could open a new opportunity for hard coating research by inter-layer dissipation of heat or research for hard coatings with better mechanical properties.

In this paper, we continue the work in investigating ternary structures based on ScN. We also computationally study the phase stability, band structure, Seebeck coefficient and power factor of two more candidate compounds potentially useful in thermoelectric applications, ZrMgN₂ and HfMgN₂. As Ti, Zr and Hf belong to group 4 of the periodic table, all three share similar physical and chemical properties, and it can be assumed that any stable Ti-based ternary may also exist for Zr and Hf.

Computational details

Over 60 different and chemically stoichiometric crystal structures registered in the Inorganic Crystal Structure Database (ICSD) [40] were studied in order to calculate the formation enthalpy of Ti–Mg–N, Zr–Mg–N and Hf–Mg–N and prepare the necessary phase diagrams. Although the binary nitrides are well known, TiMgN₂, ZrMgN₂ and HfMgN₂ are not present in either the Materials Project database [41] or the ICSD. Half of these crystal structures follow the MeMgN₂ stoichiometry, while the remaining crystal structures belong to various Mg-, Ti-, Zr- and Hf-based ternaries. In addition, the opposite sequence, MgMeN₂, was also studied in case some structures would show a different phase when switching the positions of the metal atoms in their respective sublattice.

First-principles calculations were employed using DFT [42, 43] with the projector augmented wave method (PAW) [44] implemented in the Vienna ab initio simulation package (VASP) [45–47] version 5.2. Electronic exchange correlation effects and the electronic band structure were modeled with the generalized gradient approximation (GGA) using Perdew–Burke–Ernzerhof (PBE) functional [48]. It should be noted that the Kohn–Sham gaps of standard GGA calculations are systematically smaller than experimental band gaps, but for the present work this is not an issue since we are mostly concerned with dismissing metallic compositions. To the extent that we identify relevant compounds, they can be further investigated by in-depth theoretical work and/or by laboratory synthesis of the three ternary nitrides. The plane wave energy cutoff was set at 400 eV. The required structure files for the crystal structures were obtained from the ICSD and converted to VASP input files using cif2cell [49]. Phase diagrams were prepared using the software package Pymatgen (Python Materials Genomics) [50], the band structure illustrations by the high-throughput toolkit (httk) [51] and the crystal structures by VESTA [52]. For the phase diagrams, the formation energy per atom was calculated for each ternary compound and related to competing ternary stoichiometries and neighboring binary compounds. The Materials Project database provided the formation enthalpies of all of the binaries (TiN, ZrN, HfN, Mg₃N₂, etc.).

The present work uses the same correction of the N₂ energy as used in the Materials Project, based on work by Wang et al. [53] as standard GGA exchange–correlation functionals in DFT are known to, in general, have systematic errors in the prediction of energy differences between solid and gas phase systems [54]. Hence, to accurately reproduce the formation energy of a system relative to a gas end point, it is common to adjust the gas phase energy.

The calculations used an 11 × 11 × 11 k-point mesh for Brillouin zone sampling and were executed with the Monkhorst–Pack scheme [55]. For band structure calculations, the tetrahedron method was used in order to obtain band gap values with spin polarization included [56].

Finally, the Seebeck coefficient S and power factor $S^2\mathit{\sigma }{\mathit{\tau }}^{-1}$ (being the charge carrier relaxation time) of the predicted semiconductors is calculated at room temperature and 600 K as functions of the chemical potential using Boltzmann transport theory with the constant relaxation time approximation. We use the software BoltzTraP [57] on DFT calculations with a 40 × 40 × 40 k-point mesh for Brillouin zone sampling.

Results

TiMgN₂

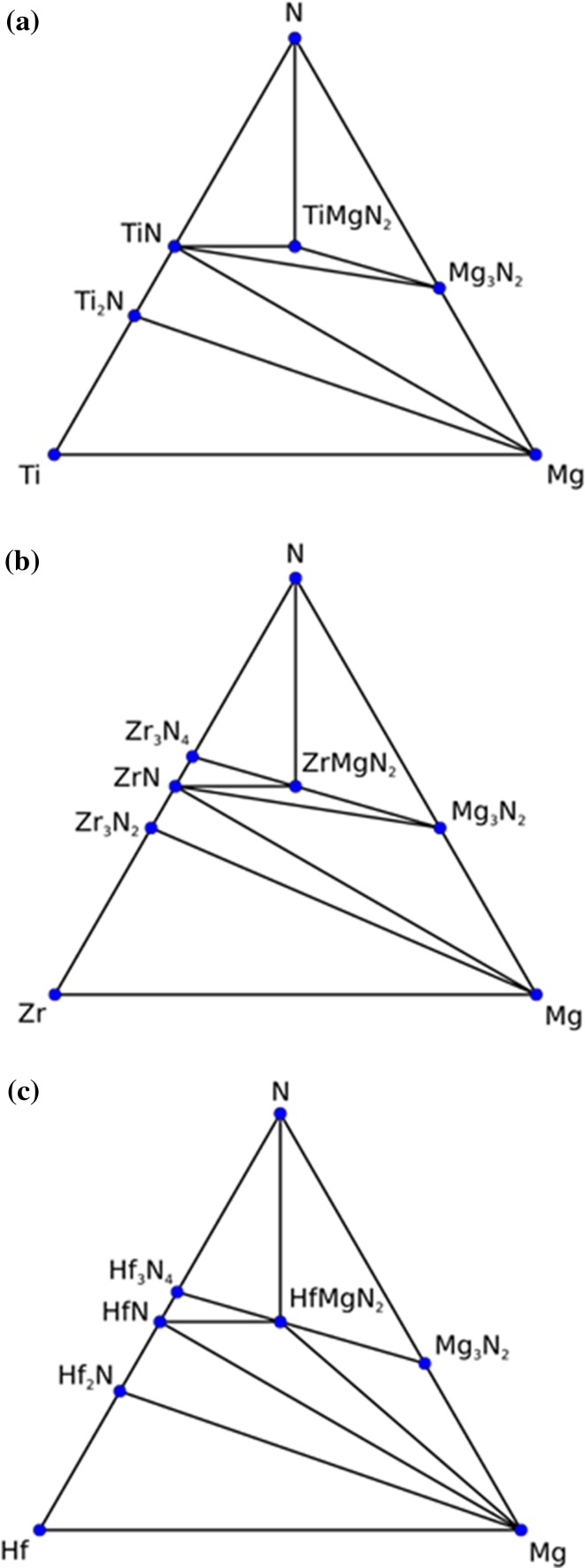
Figure 1a shows the phase diagram for Ti–Mg–N. Although 28 different crystal structures other than those that follow the MeMgN₂ formula (such as Ca₄TiN₄ [58], perovskite CaTiO₃ [59], Ti₂AlN and Ti₄AlN₃ MAX-phases [60]) were tested, only the ordered TiMgN₂ stoichiometry is found to be thermodynamically stable relative to known and investigated phases with the other ordered stoichiometries being either unstable or metastable. Random Ti_1−xMg_xN solid solutions with the rocksalt structure have, however, been found to be thermodynamically stable for a range of compositions [25]. This precise stoichiometry occurred in 29 of the investigated crystal structures. These include the trigonal NaCrS₂ ($\text{R}\overline{3}\text{mH}$) superstructure [61], the tetragonal BaNiS₂ ($\text{P}4/\text{n m m Z}$) superstructure [62], tetragonal LiUN₂ [63], ZnGeN₂ [64] (based on the NaFeO₂-beta structure) and the inverse-MAX BaCeN₂ [65].

Figure 1.

Phase diagram for a Ti–Mg–N, b Zr–Mg–N and c Hf–Mg–N. Only predicted stable structures located on the convex hull are shown. Only the MeMgN₂ stoichiometry is predicted to be stable

In order to differentiate between these structures, Table 1 lists a selected group of examples with their respective formation enthalpies. These results show that crystallization into the NaCrS₂ is the most likely outcome with a − 1.299 eV formation enthalpy and a 0.04 eV difference compared to the LiUN₂ structure which agrees with the findings mentioned in Ref. [25]. It should be noted that the difference between the formation enthalpies of these two crystal structures would most likely mean that NaCrS₂ is the preferred structure, but LiUN₂ is also studied for any comparison needed between TiMgN₂, ZrMgN₂ and HfMgN₂.

Table 1.

Formation enthalpies for TiMgN₂ crystallized in five different structures

TiMgN2
ICSD id	Formation enthalpy (eV/atom)	Nitride example
82537	− 1.299	SrZrN2 (NaCrS2)
98663	− 1.260	LiUN2
74904	− 1.005	BaZrN2 (BaNiS2)
15144	− 1.234	ZnGeN2 (NaFeO2-beta)
74791	− 1.222	BaCeN2, inverse-MAX

The ICSD id is the entry identification number which the structure is based on. The nitride examples represent actual existing ternary compounds and the respective structures which they crystallize in

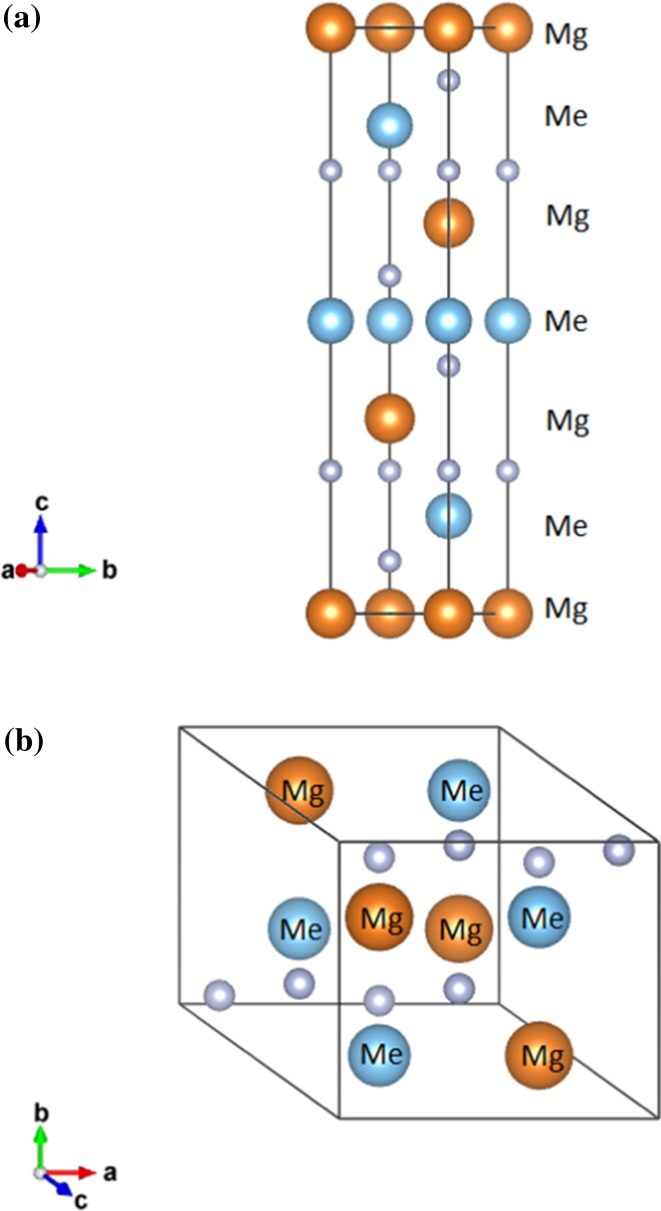
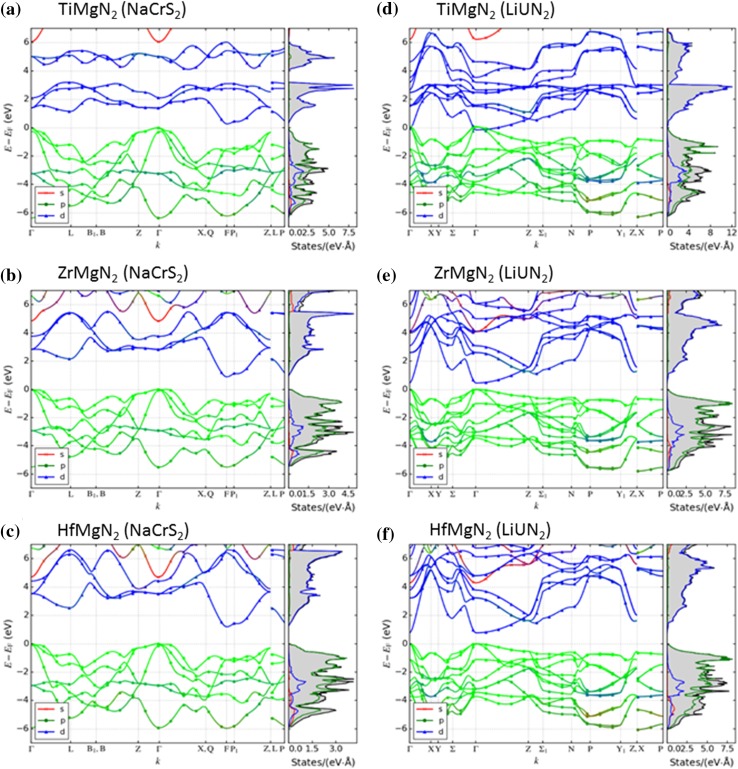
Both crystal structures are shown in Fig. 2. The results suggest that TiMgN₂ will crystallize into the NaCrS₂ superstructure (also viewed as a NaCl-B1 superstructure that includes three alternating layers of Ti and Mg) which could cause phonon scattering at the interface of each layer as mentioned in the introduction. Figure 3a, d shows the band structures for TiMgN₂ in the NaCrS₂ and LiUN₂ structures. According to these results, TiMgN₂ is a semiconductor with a Kohn–Sham PBE band gap of 0.26 eV in the NaCrS₂ structure (Fig. 3a). However, the case for LiUN₂ (Fig. 3d) is different, as band structure calculations show no band gap, i.e., predicting metallic properties. It is possible that TiMgN₂ could crystallize in the LiUN₂ structure as a metastable phase. Table 2 shows the lattice parameters and the band gap energy in both crystal structures. These results show that although the trigonal NaCrS₂ crystal structure remains with only the lattice parameters changing, the LiUN₂ structure relaxes from tetragonal to monoclinic according to the calculated unit cell lattice parameters.

Figure 2.

3D visualization of MeMgN₂ crystallized into: a the NaCrS₂ (trigonal unit cell) and b the LiUN₂ (relaxed monoclinic unit cell) structure

Figure 3.

Band structure of a TiMgN₂, b ZrMgN₂ and c HfMgN₂ for the NaCrS₂ structure (left column) predicting an indirect band gap for all three compounds. Band structure of d TiMgN₂, e ZrMgN₂ and f HfMgN₂ for the LiUN₂ structure (right column) predicting a direct band gap for ZrMgN₂ and HfMgN₂. Note: s, p and d indicate the relative contributions to the total sum, s + p + d, not the absolute projected values

Table 2.

Lattice parameters, unit cell volumes and band gap values for TiMgN₂ crystallized in both NaCrS₂ and LiUN₂

Crystal structure	Compound (MeMgN2)	a (Å)	b (Å)	c (Å)	Volume (Å3)	Band gap (eV)
NaCrS2 (trigonal)	TiMgN2	2.9997 $\mathit{\alpha }={90}^{\circ }$	2.9997 $\mathit{\beta }={90}^{\circ }$	14.8838 $\mathit{\gamma }={120}^{\circ }$	115.9849	Indirect: 0.26
LiUN2 (tetragonal relaxed into monoclinic)	TiMgN2	5.9777 $\mathit{\alpha }={90}^{\circ }$	5.9777 $\mathit{\beta }=$ $55.{1530}^{\circ }$	5.2309 $\mathit{\gamma }={90}^{\circ }$	153.3962	No gap: metallic?

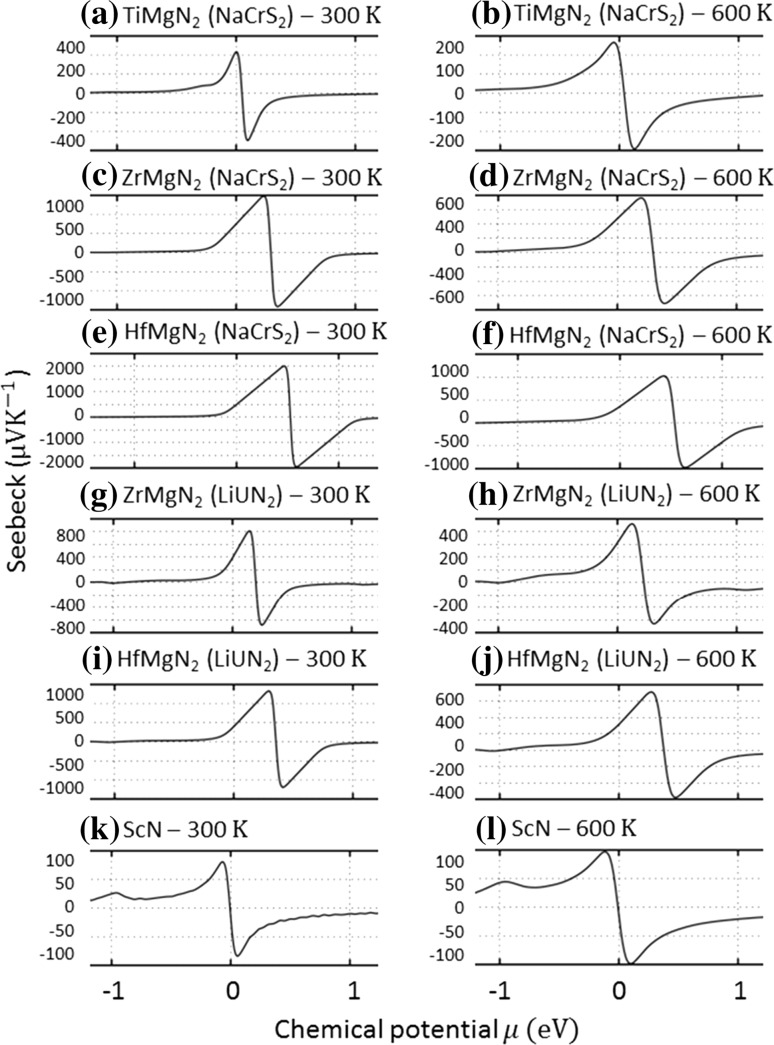
Figure 4a, b shows the Seebeck coefficient of TiMgN₂ versus the chemical potential at room temperature and 600 K, respectively. Only the NaCrS₂ structure was studied as the LiUN₂ structure was predicted with no band gap. These results show relatively high Seebeck coefficient values at the Fermi level. Figure 5a, b shows $\left(S^2\mathit{\sigma }\right)/\mathit{\tau }$ versus the chemical potential at room temperature and 600 K, respectively. Depending on the assumed relaxation time, predicted power factor values could exceed those of ScN (Fig. 5k, l).

Figure 4.

Seebeck coefficient value versus chemical potential of a TiMgN₂, b ZrMgN₂ and c HfMgN₂ for the NaCrS₂ and LiUN₂ structures at room temperature and 600 K. The Seebeck coefficient value of ScN is also added for comparison

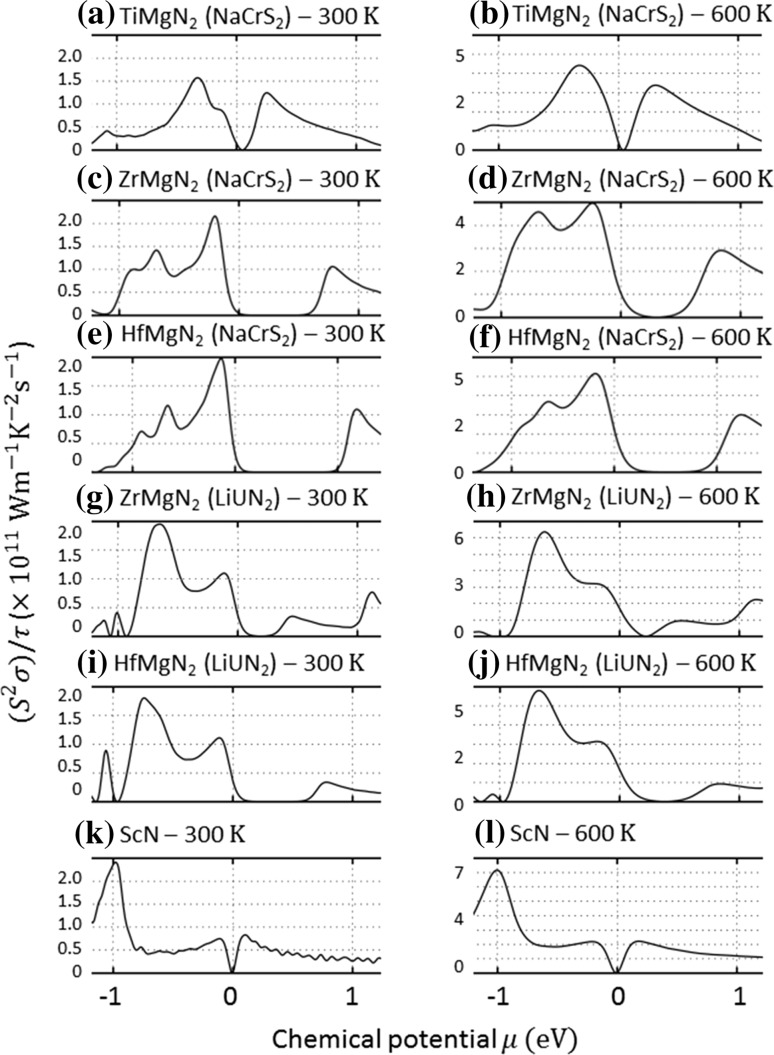
Figure 5.

$\left(S^2\mathit{\sigma }\right)/\mathit{\tau }$ value versus chemical potential of a TiMgN₂, b ZrMgN₂ and c HfMgN₂ for the NaCrS₂ and LiUN₂ structures at room temperature and 600 K. The $\left(S^2\mathit{\sigma }\right)/\mathit{\tau }$ value of ScN is also added for comparison

ZrMgN₂

Figure 1b shows the phase diagram for ZrMgN₂. Also here the only stable ternary has the MeMgN₂ stoichiometry. As for the preferred crystal structure, formation enthalpies for the selected crystal structures are shown in Table 3. In contrast to TiMgN₂, the LiUN₂ structure competes with the NaCrS₂ structure with less than 0.01 eV formation enthalpy difference.

Table 3.

Formation enthalpies for ZrMgN₂ crystallized in five different structures

ZrMgN2
ICSD id	Formation enthalpy (eV/atom)	Nitride example
82537	− 1.307	SrZrN2 (NaCrS2)
98663	− 1.311	LiUN2
74904	− 0.897	BaZrN2 (BaNiS2)
15144	− 1.230	ZnGeN2 (NaFeO2-beta)
74791	− 1.223	BaCeN2, inverse-MAX

The predicted band structures are shown in Fig. 3b, e. In both cases, ZrMgN₂ is a semiconductor regardless of crystal structure. However, for the NaCrS₂ crystal structure we find an indirect Kohn–Sham PBE band gap of 0.89 eV and for the LiUN₂ structure, a direct band gap of 0.46 eV. The respective lattice parameters and band gap energy are shown in Table 4. ZrMgN₂ relaxes in a similar way as TiMgN₂ with the NaCrS₂ structure remaining the same while the tetragonal LiUN₂ structure relaxes into a monoclinic structure according to the calculated unit cell lattice parameters.

Table 4.

Lattice parameters, unit cell volumes and band gap values for ZrMgN₂ crystallized in both NaCrS₂ and LiUN₂

Crystal structure	Compound (MeMgN2)	a (Å)	b (Å)	c (Å)	Volume (Å3)	Band gap (eV)
NaCrS2 (trigonal)	ZrMgN2	3.2077 $\mathit{\alpha }={90}^{\circ }$	3.2077 $\mathit{\beta }={90}^{\circ }$	15.3237 $\mathit{\gamma }={120}^{\circ }$	136.5495	Indirect: 0.89
LiUN2 (tetragonal relaxed into monoclinic)	ZrMgN2	6.3113 $\mathit{\alpha }={90}^{\circ }$	6.3113 $\mathit{\beta }=55.{3470}^{\circ }$	5.5498 $\mathit{\gamma }={90}^{\circ }$	181.8447	Direct: 0.46

Figure 4b, g (room-temperature calculations) and Fig. 4d, h (600 K calculations) shows the Seebeck coefficient of ZrMgN₂ versus the chemical potential in the NaCrS₂ and the LiUN₂ structures. These results show an increase in the Seebeck coefficient values and a slight shift in the chemical potential compared to TiMgN₂ with higher values seen in the NaCrS₂ structure. Figure 5b, g and d, h shows the $\left(S^2\mathit{\sigma }\right)/\mathit{\tau }$ versus chemical potential at room temperature and 600 K, respectively. These results predict power factor values close to the Fermi level which are larger than those of ScN (Fig. 5k, l).

HfMgN₂

Figure 1c shows the phase diagram for Hf–Mg–N. Similar to both TiMgN₂ and ZrHfN₂, the HfMgN₂ stoichiometry is predicted to be stable. Table 5 compares a selected group of crystal structures and shows the NaCrS₂ and LiUN₂ structures with similar formation enthalpies (less than 0.01 eV difference), thus predicting a competition between the two structures.

Table 5.

Formation enthalpies for HfMgN₂ crystallized in five different structures

HfMgN2
ICSD id	Formation enthalpy (eV/atom)	Nitride example
82537	− 1.447	SrZrN2 (NaCrS2)
98663	− 1.453	LiUN2
74904	− 1.034	BaZrN2 (BaNiS2)
15144	− 1.360	ZnGeN2 (NaFeO2-beta)
74791	− 1.362	BaCeN2, inverse-MAX

Figure 3c, f shows the predicted band structures for both NaCrS₂ and LiUN₂. Similar to ZrMgN₂, an indirect band gap of 1.19 eV is predicted for the NaCrS₂ structure, while a 0.77 eV direct band gap is predicted for the LiUN₂ structure. The respective lattice parameters and band gap energies are shown in Table 6. Similar to TiMgN₂ and ZrMgN₂, HfMgN₂ preserves the trigonal NaCrS₂ structure but relaxes from tetragonal LiUN₂ into a monoclinic structure.

Table 6.

Lattice parameters, unit cell volumes and band gap values for HfMgN₂ crystallized in both NaCrS₂ and LiUN₂

Crystal structure	Compound (MeMgN2)	a (Å)	b (Å)	c (Å)	Volume (Å3)	Band gap (eV)
NaCrS2 (trigonal)	HfMgN2	3.1679 $\mathit{\alpha }={90}^{\circ }$	3.1679 $\mathit{\beta }={90}^{\circ }$	15.2463 $\mathit{\gamma }={120}^{\circ }$	132.5114	Indirect: 1.19
LiUN2 (tetragonal relaxed into monoclinic)	HfMgN2	6.2300 $\mathit{\alpha }={90}^{\circ }$	6.2300 $\mathit{\beta }=55.{5036}^{\circ }$	5.5001 $\mathit{\gamma }={90}^{\circ }$	175.9362	Direct: 0.77

Figure 4e, i (room-temperature calculations) and Fig. 4f, j (600 K calculations) shows the Seebeck coefficient of HfMgN₂ versus the chemical potential in the NaCrS₂ and the LiUN₂ structures. These results show an increase in the Seebeck coefficient values and a larger shift in the chemical potential compared to both TiMgN₂ and ZrMgN₂ with higher values seen in the NaCrS₂ structure. Figure 5e, i and f, j shows the $\left(S^2\mathit{\sigma }\right)/\mathit{\tau }$ versus chemical potential at room temperature and 600 K, respectively. These results predict power factor values almost equal to those of ZrMgN₂ and larger than that of ScN close to the Fermi level (Fig. 5k, l).

Discussion

For ZrMgN₂ and HfMgN₂, the formation enthalpies of the NaCrS₂ and the LiUN₂ structure are close, within the accuracy of our approach. This suggests that both of these structures may be possible to synthesize, i.e., with the one higher in energy as a long-lasting metastable state. The shifting between the NaCrS₂ and the LiUN₂ structures could be done by choosing suitable substrates for epitaxial stabilization during the synthesis process. Despite that we cannot with certainty determine which of the structures for ZrMgN₂ and HfMgN₂ are thermodynamically stable, both are semiconductors. This motivates future studies on synthesis for thermoelectrics and other applications. It should be noted that the NaCrS₂ structures show indirect band gaps with larger values and large slopes for the density of states at the Fermi level compared to their direct band gap counterparts in the LiUN₂ structure. Another feature seen in all three compounds is the relation between band gap values and lattice parameters with the transition metal, Me. As the smaller Ti atom is replaced with the larger Zr atom, the lattice parameters, cell volume and band gap value increase, which is expected. However, only the band gap value increases when Zr is replaced with Hf as the f orbital electrons are not effective at screening the increasing charge, resulting in similar atomic size (lanthanide contraction [66]) and similar lattice parameters.

Although the present results are promising, actual attempts to synthesize these prospective compounds would be important. Similar to the synthesis of MAX-phase [67] thin films, it should be possible to synthesize ordered TiMgN₂, ZrMgN₂ and HfMgN₂ outside thermodynamic equilibrium in a magnetron sputtering system. All of the mentioned elements are vacuum compatible, and one could use the deposition parameters needed for stoichiometric TiN, ZrN, HfN and Mg₃N₂ to reach a $\text{Me}/\text{Mg}=1$ ratio and fine-tune the MeMgN₂ stoichiometry. References [36, 38] note the deposition temperature for rocksalt (Ti, Mg)N alloys to be between 200 and 300 °C with oxidization resistance close to 700 °C (suitable for mid-temperature thermoelectric applications). If the layered NaCrS₂ superstructure is preferred, it would be advisable to use either high-temperature direct growth or low-temperature deposition, followed by high-temperature annealing [68] (in ammonia or nitrogen). In this case, GaN or SiC [69] substrates could be considered for their suitable lattice constant and thermal stability.

As for the thermoelectric properties, the calculated Seebeck coefficient values show that in the range of a moderate change in the Fermi level, high room-temperature Seebeck coefficient values can be achieved (Fig. 4), although it seems that HfMgN₂ is either an insulator or would require elemental doping due to the larger shift in the chemical potential.

Note that what we have calculated is the power factor divided by the relaxation time. The results (Fig. 5) can be used as an estimate of the difference in thermoelectric performance at various doping levels between the studied compounds and known materials, e.g., ScN, as shown for comparison in Figs. 4k, l and 5k, l. However, such a comparison is made under the assumptions that the constant relaxation time approximation holds sufficiently well and that the relaxation time for the compounds is similar. For more precise predictions, the relaxation time value needs to be obtained from experimental data, as it can for example for common thermoelectric materials such as Bi₂Te₃ [70, 71].

As ordered TiMgN₂, ZrMgN₂ and HfMgN₂ have not yet been studied experimentally, such data do not exist, and obtaining meaningful numbers for the electrical conductivity is difficult. However, using experimental data from Burmistrova et al. [19] and the classical equation for conductivity ($\mathit{\sigma }=n{\text{e}}^2\mathit{\tau }{m}^{-1}$), the constant relaxation time $\mathit{\tau }$ for ScN (which the ternaries were modeled after) is estimated to be equal to $6.5\times {10}^{-14}\text{s}$.

Conclusions

Theoretical methods were used to study the phase stability and band structure of TiMgN₂, ZrMgN₂ and HfMgN₂. In all three cases, only MeMgN₂ is predicted to be the stable stoichiometry. It is shown that stoichiometric TiMgN₂ crystallizes into the hexagonal NaCrS₂ superstructure with a 0.26 eV indirect Kohn–Sham PBE band gap. ZrMgN₂ and HfMgN₂ were also studied, which shows tendency to crystallize in both the NaCrS₂ superstructure and the LiUN₂ prototype monoclinic structure. Both show semiconducting properties regardless of the crystal structure. ZrMgN₂ shows a 0.89 eV indirect band gap when crystallizing into the NaCrS₂ structure, while as crystallization into the LiUN₂ structure results in a 0.46 eV direct band gap. As for HfMgN₂, the band gap increases as crystallization into NaCrS₂ results in a 1.19 eV indirect band gap and crystallization into LiUN₂ results in a 0.77 eV direct band gap. Lattice parameters and cell volumes increase with the substitution of Ti with Zr, but slightly decrease when Zr is substituted with Hf.

Finally, the Seebeck coefficient and power factor was calculated for all of the semiconducting compounds. The results show that in the range of a moderate change in the Fermi level, high room-temperature Seebeck coefficient values can be achieved.

Thus, the predicted stability and semiconducting properties of these compounds can be further studied both theoretically and experimentally for any prospective thermoelectric properties.